Numerical Determination of the Load-Bearing Capacity of a Perforated Thin-Walled Beam in a Structural System with a Steel Grating
Abstract
1. Introduction
2. Materials and Methods
- Laboratory tests of a 3 m-span structural system with a load transferred to the system through a single steel grating.
- Laboratory tests of a 6 m-span structural system with a load transferred to the system through a single steel grating.
- Laboratory tests of the strength properties of the steel from which the beams and connectors were made.
- Development and validation of a 3 m-span structural system model.
- Development and validation of a 6 m-span structural system model.
- Development of structural system models for spans of 3.0 m, 3.5 m, 4.0 m, 4.5 m, 5.0 m, 5.5 m, and 6.0 m with a load transferred by steel gratings placed over the entire span.
- Conducting simulations to determine load-bearing curves.
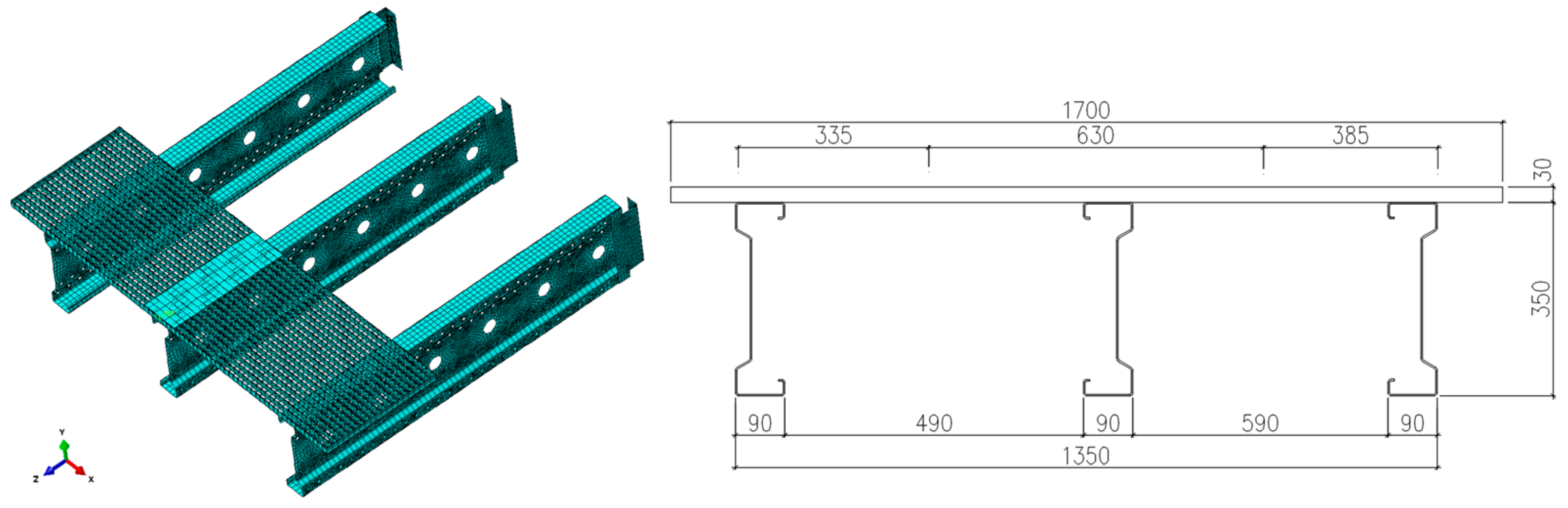
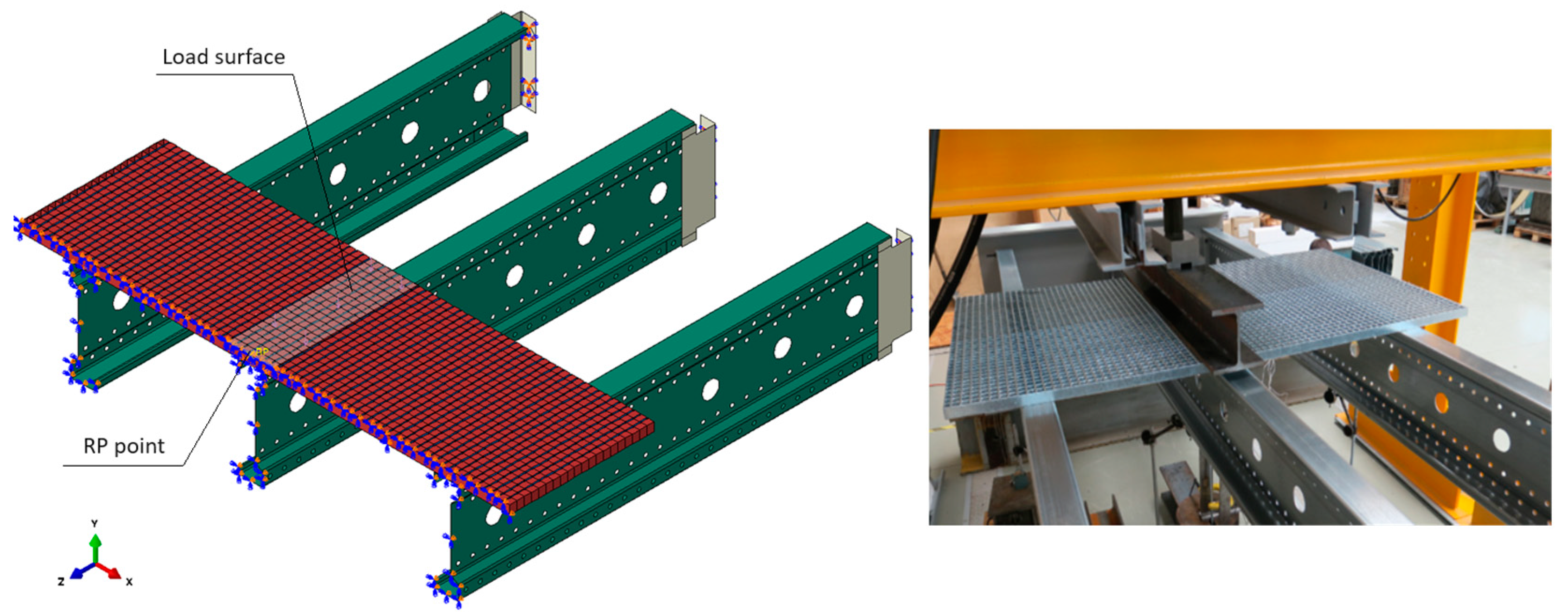
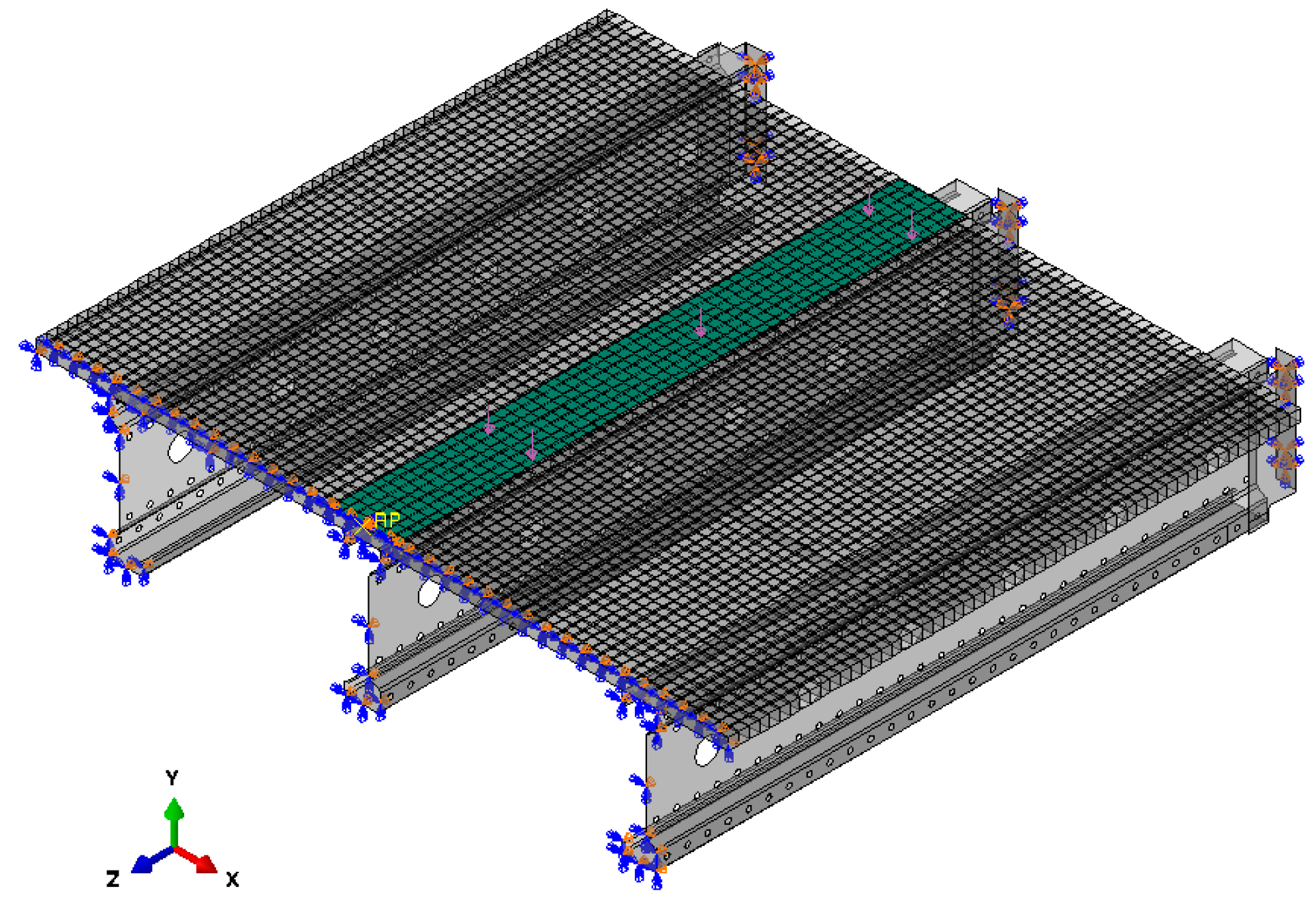
2.1. Numerical Model
- Connectors were fixed at the location of mounting holes on a surface with a diameter of 40 mm.
- Connectors were connected to the beams using a “Tie”-type contact [19], which corresponds to a rigid connection of the connector to the beam.
- Symmetric boundary conditions were applied to the axis of symmetry of the system on the steel grating and beams.
- Normal Behavior—“Hard” Contact.
- Tangential Behavior—Penalty, Friction coefficient = 0.3.
- Young’s modulus E = 205.5 GPa, determined based on laboratory tests.
- Poisson’s ratio ν = 0.3.
- Yield strength RH = 490 MPa, determined based on laboratory tests.
- Young’s modulus E = 200 GPa, determined based on laboratory tests.
- Poisson’s ratio ν = 0.3.
- Yield strength RH = 362 MPa, determined based on laboratory tests.
2.2. Numerical Model Used to Develop Load-Bearing Curves for the Central Beam
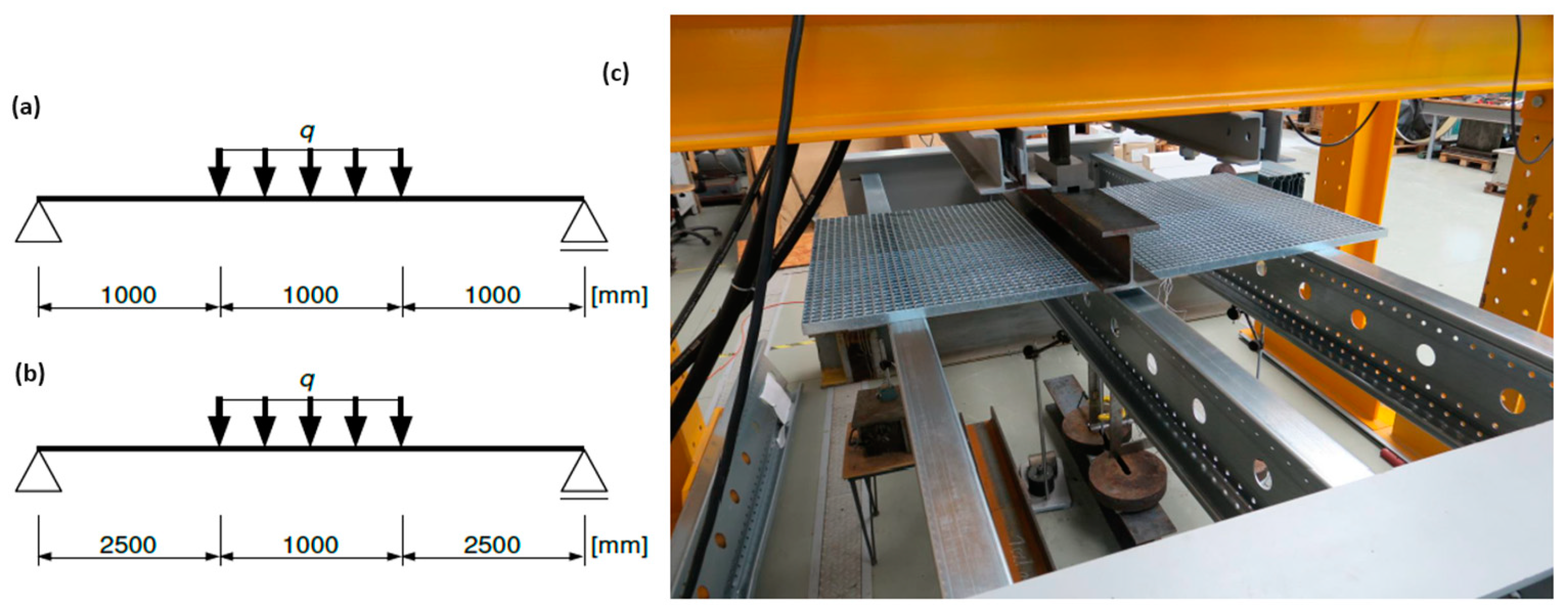
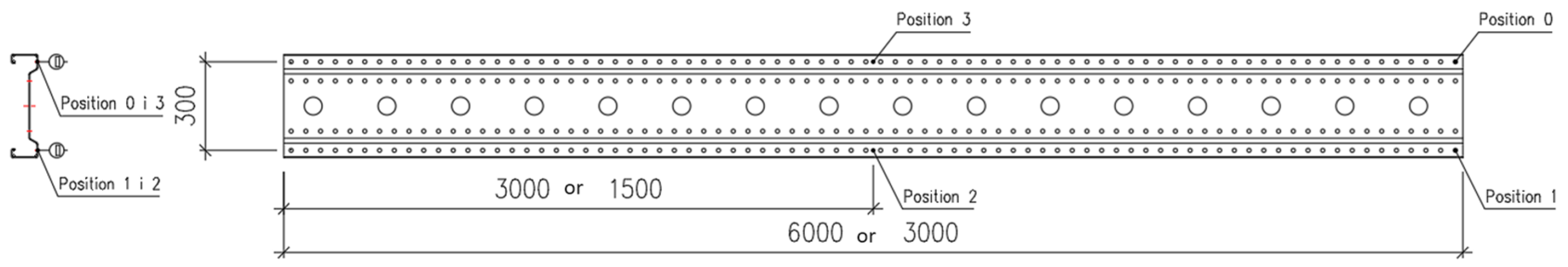
2.3. Laboratory Tests
- Loading to achieve a displacement value of the actuator of the strength testing machine equal to 7.0 mm over 70.0 s for the 3 m beam and 15.0 mm over 150.0 s for the 6 m beams (speed: 0.1 mm/s);
- Unloading to a displacement value of the actuator of the machine equal to 0.0 mm over 70.0 s for the 3 m beam and 150.0 s for the 6 m beams (speed: 0.1 mm/s);
- Elastic phase, in which the displacement value of the actuator was increased from 0.0 mm to 7.0 mm over 70.0 s for the 3 m beam and 15.0 mm over 150.0 s for the 6 m beams (speed: 0.1 mm/s); during this phase, measurements of beam and connector displacements and force were made, and a deflection–force diagram was plotted;
- Destruction phase; after conducting the test of beam operation in the elastic range, the sample was unloaded to a displacement value of the actuator of the strength testing machine equal to 0.0 mm and then loaded until destruction at a speed of 0.1 mm/s. During the test, a deflection–force diagram was plotted.
- Loading to achieve a displacement value of the actuator of the strength testing machine equal to 0.3 mm over 60.0 s (speed: 0.3 mm/min);
- Unloading to a displacement value of the actuator of the machine equal to 0.0 mm over 60.0 s (speed: 0.3 mm/min);
- Main phase, in which the displacement value of the actuator was increased from 0.0 mm to 0.3 mm over 60.0 s (speed: 0.3 mm/min); during this phase, measurements of the elongation of the sample and the increase in force were made, and a deflection–force diagram was plotted.
3. Results and Discussion
3.1. Results of Laboratory Tests
3.2. Results of Numerical Simulations and Model Validation

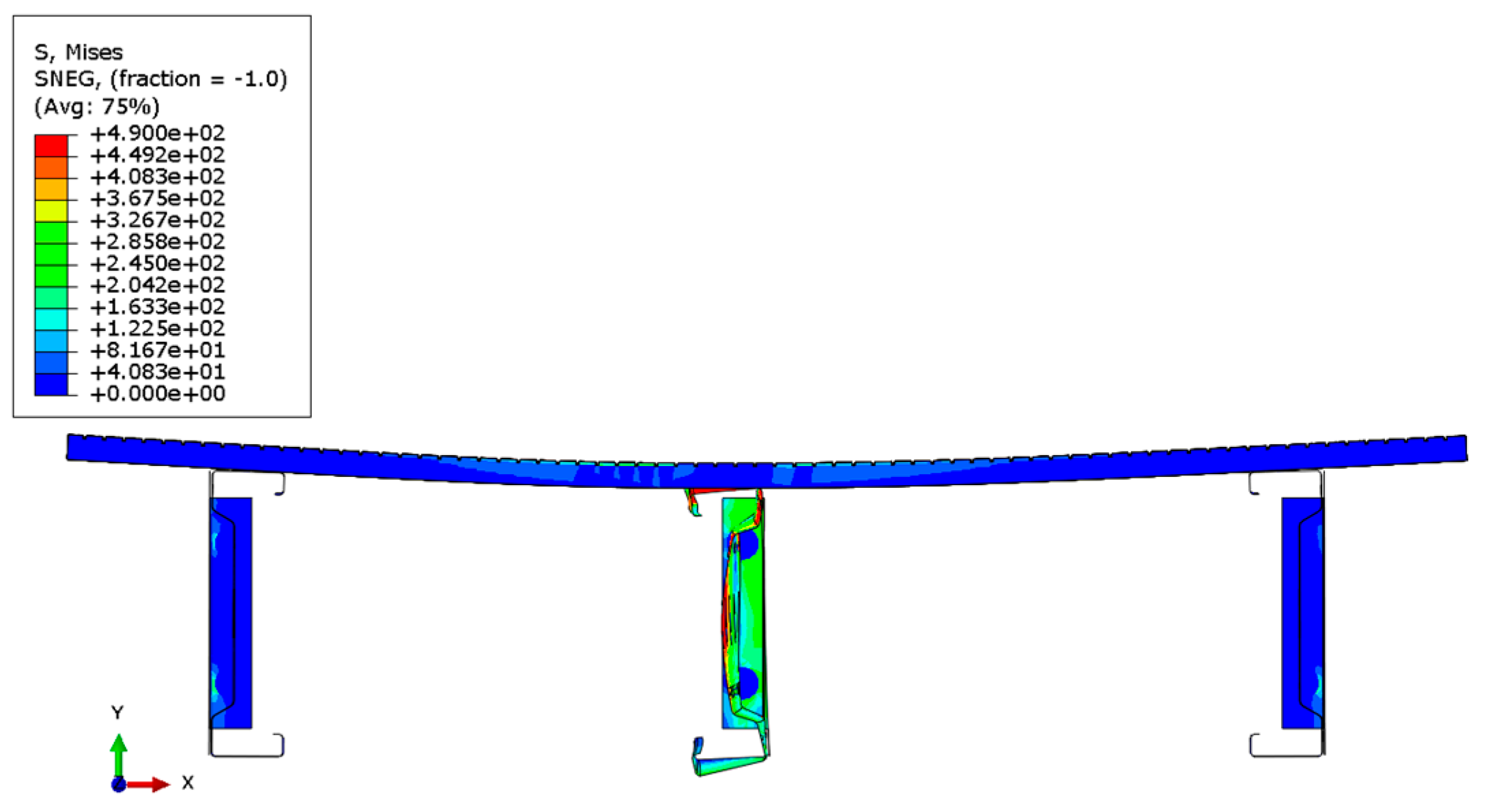
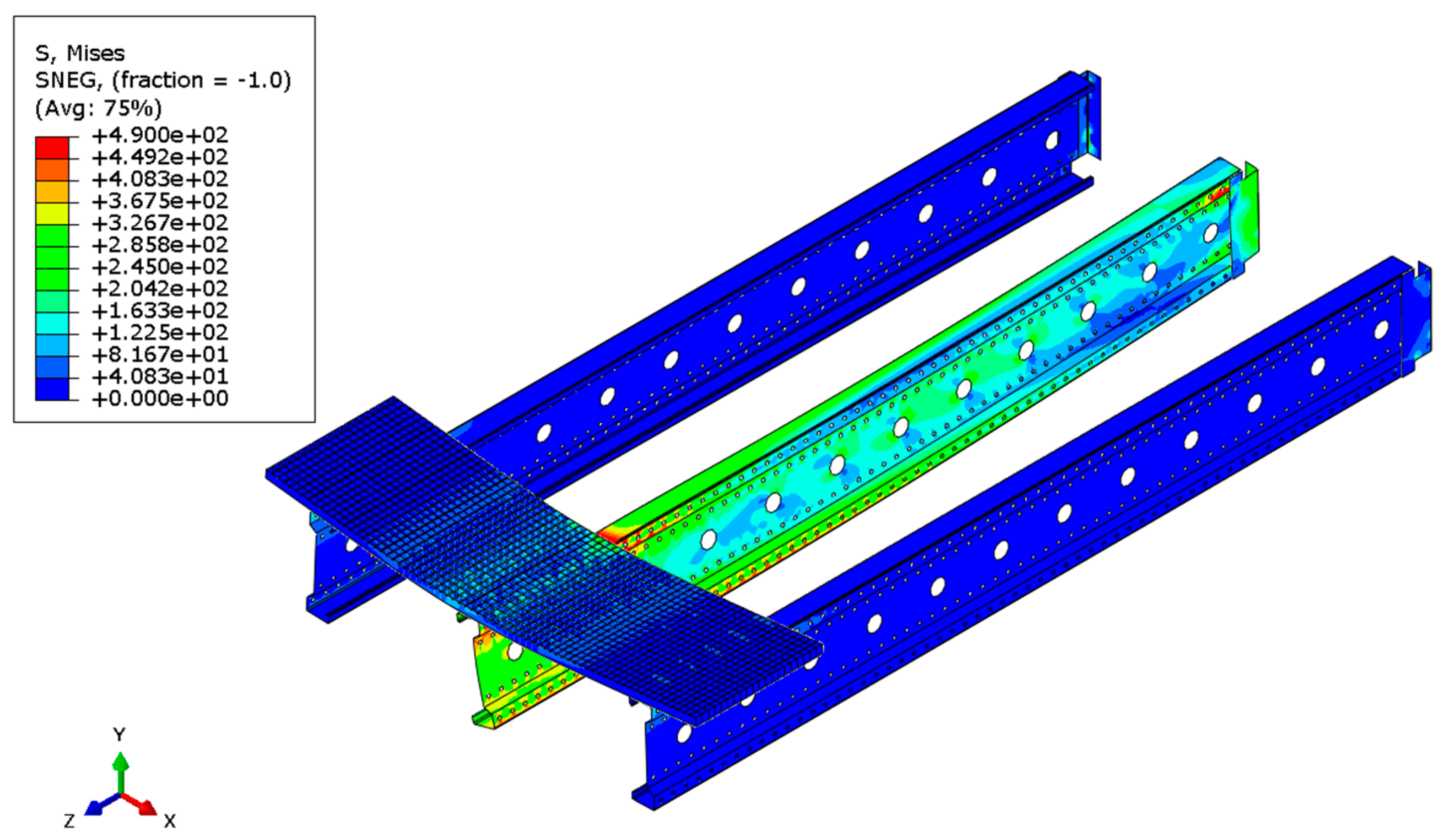
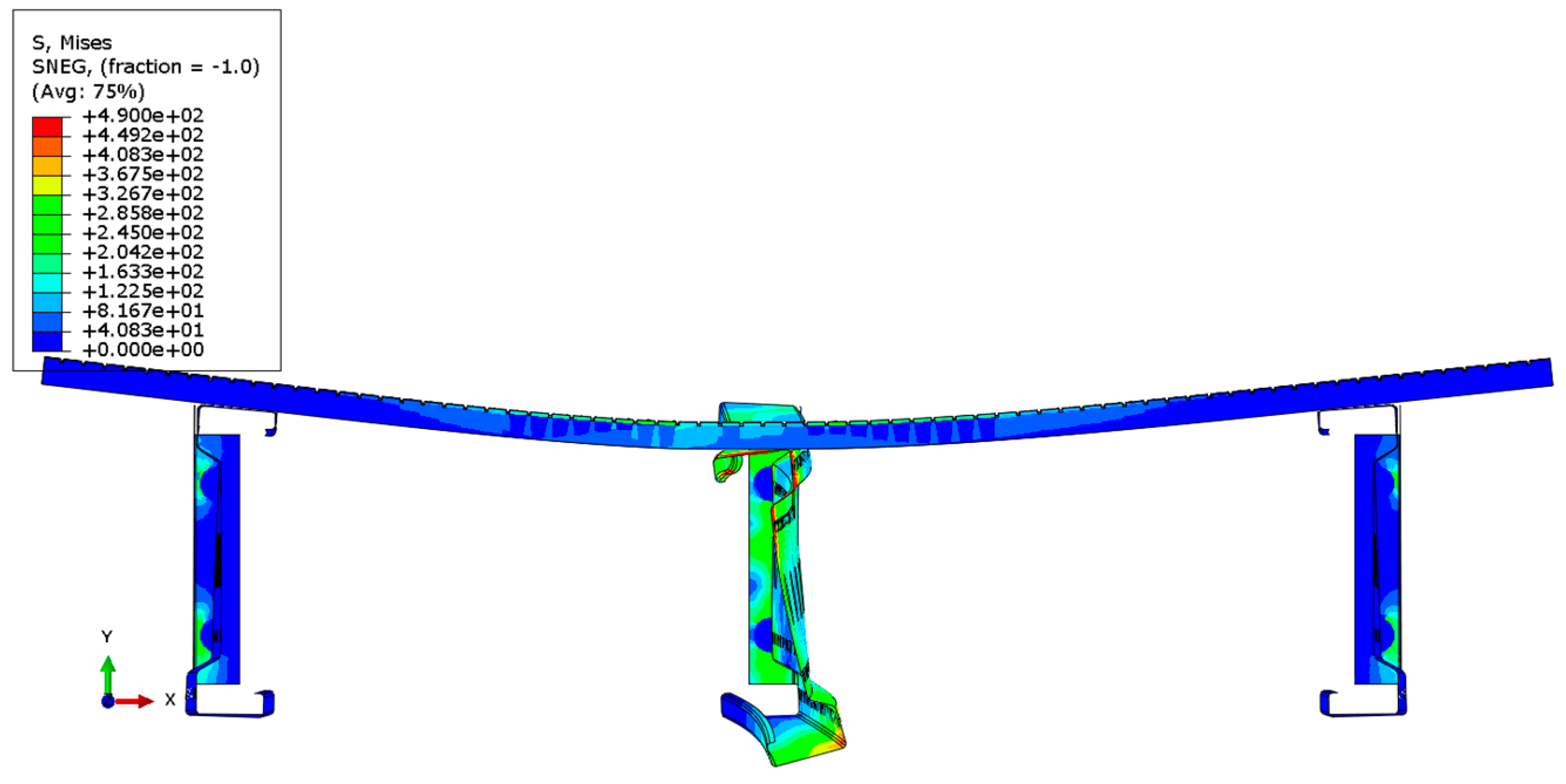


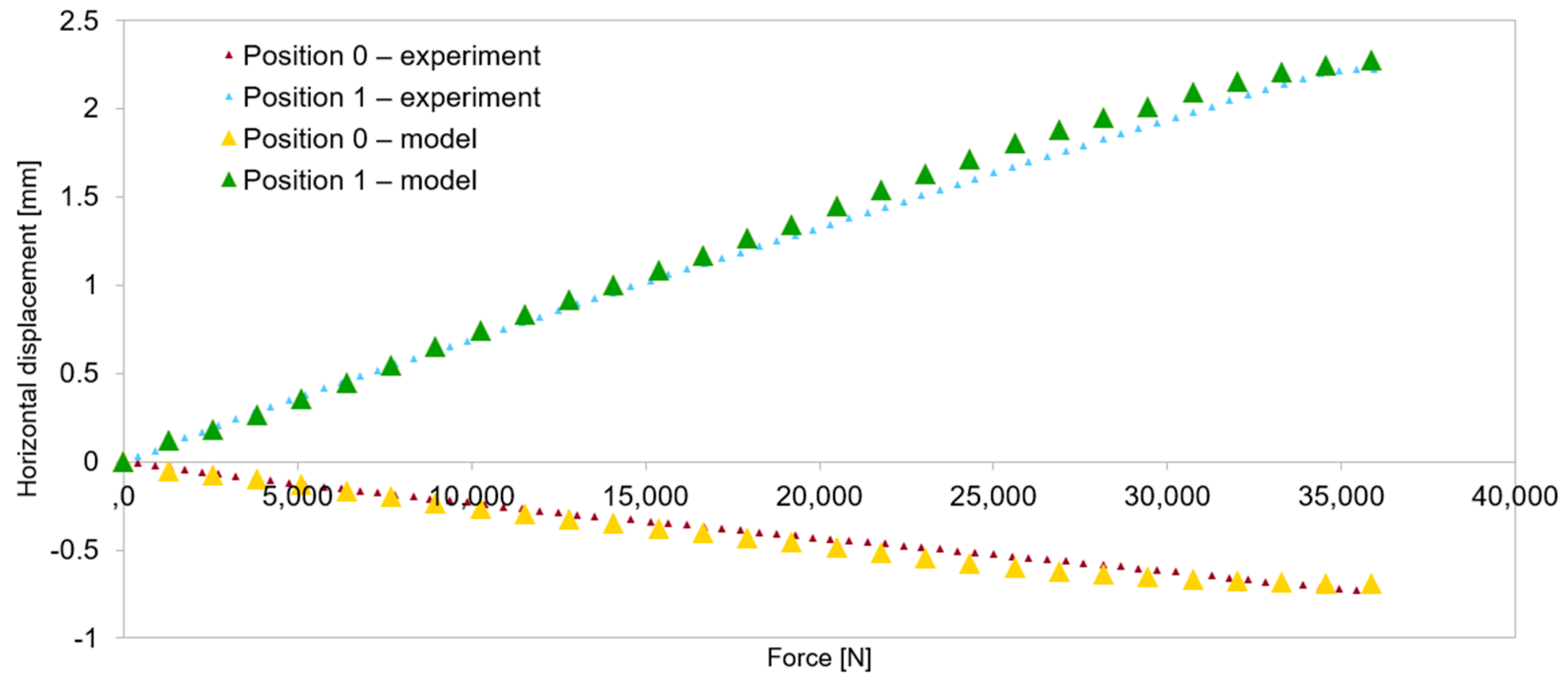
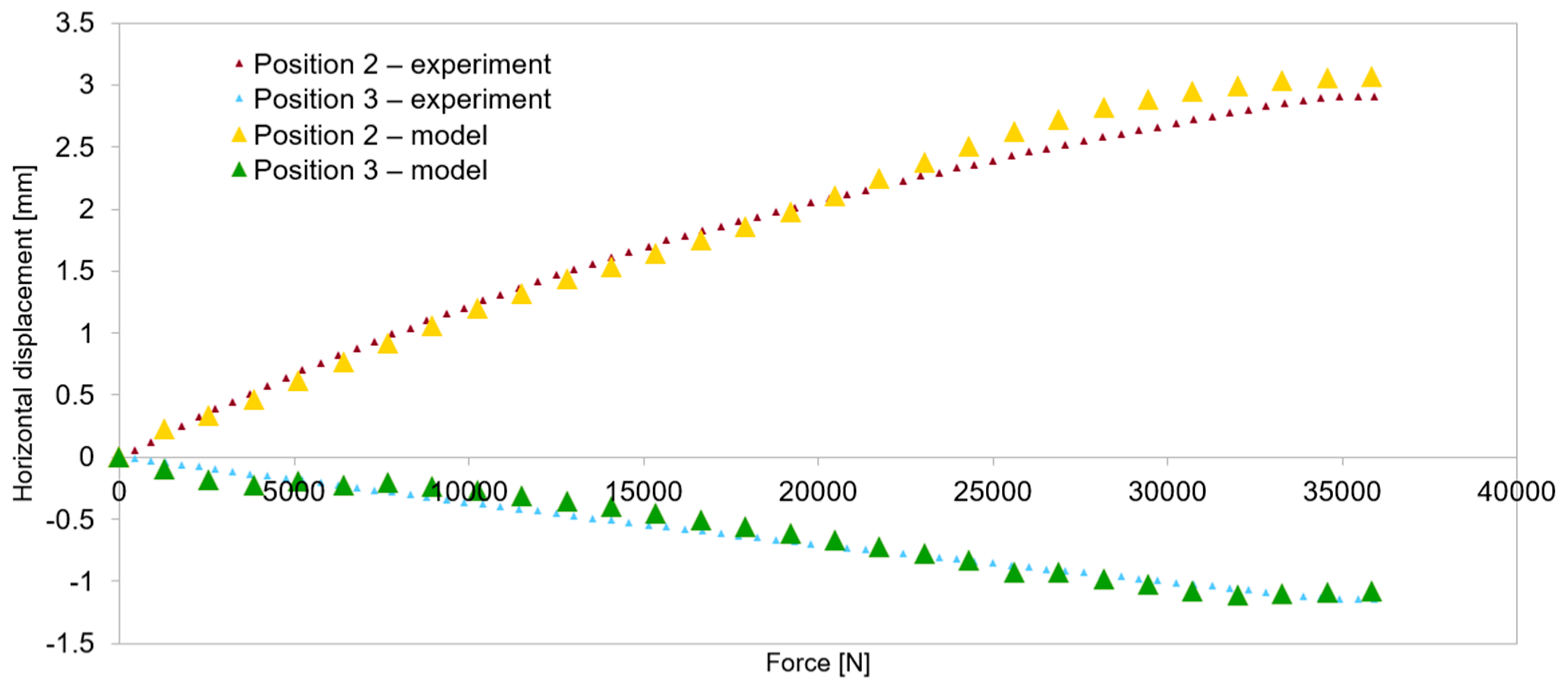
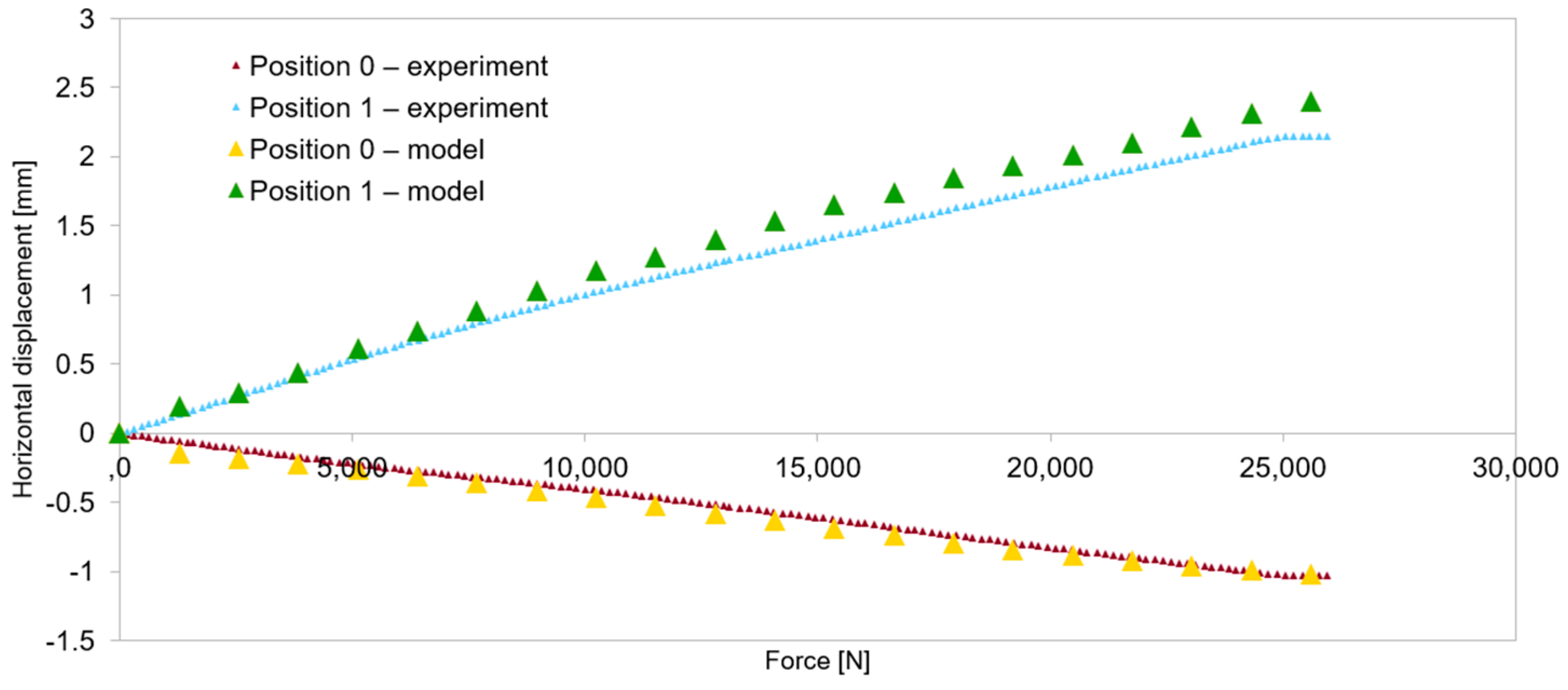

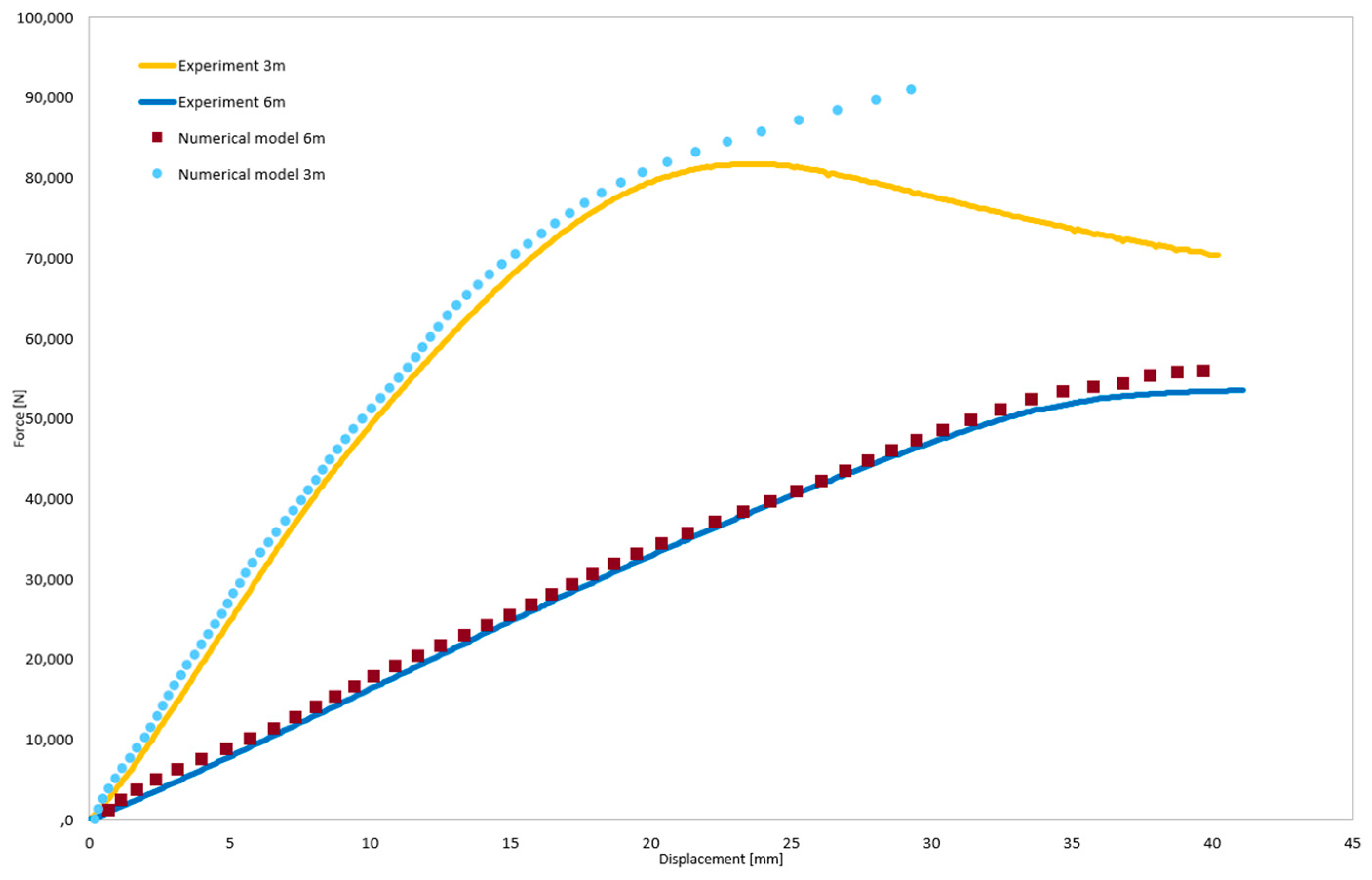
3.3. Results of Targeted Numerical Simulations
- Young’s modulus E = 200 GPa.
- Poisson’s ratio ν = 0.3.
- Yield strength RH = 350 MPa.
- Young’s modulus E = 200 GPa.
- Poisson’s ratio ν = 0.3.
- Yield strength RH = 235 MPa.
4. Conclusions
- The study of 6 m beams showed a pattern of destruction through loss of stability, while 3 m beams were destroyed through yielding.
- Numerical simulations and laboratory tests demonstrated analogous destruction patterns.
- Measurements of horizontal displacements in all tests showed greater displacements in the lower flange of the beam. This is consistent with expectations, as the upper part of the beam is somewhat “braced” by the bridge deck.
- This observation was also taken into account in the numerical model by applying normal and tangential contact with friction between the bridge deck and beams.
- Based on the conducted tests, it is recommended to place additional bracings between the lower flanges of the beams.
- The connector and the support zone of the beam are the weakest elements of the tested system. Each time, the beam’s destruction process started from the yielding of the connector, which was observed in experimental studies and confirmed in the numerical model for all tested lengths.
- The presented study contributes to a better understanding of the operation of such structural systems.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yu, C.; Schafer, B.W. Distortional Buckling Tests on Cold-Formed Steel Members in Bending; Technical Report; Johns Hopkins University: Baltimore, MD, USA, 2005. [Google Scholar]
- Specification for the Design of Light Gage Steel Structural Members. American Iron and Steel Institute (AISI) Specifications, Standards, Manuals and Research Reports (1946–Present); American Iron and Steel Institute: Washington, DC, USA, 1946. [Google Scholar]
- EN 1993-1-3:2006; Eurocode 3: Design of Steel Structures—Part 1–3: General Rules-Supplementary Rules for Cold-Formed Members and Sheeting. ECCS: Noordwijk, The Netherlands, 2007. Available online: https://www.phd.eng.br/wp-content/uploads/2015/12/en.1993.1.3.2006.pdf (accessed on 24 November 2023).
- Landolfo, R. Eurocodes Background and Applications Cold-Formed (CF) Structures. Available online: https://eurocodes.jrc.ec.europa.eu/doc/WS2008/EN1999_8_Landolfo.pdf (accessed on 19 October 2021).
- Yu, W. Cold-Formed Steel Design; Wiley: Hoboken, NJ, USA, 2000; ISBN 0471-348-090. [Google Scholar]
- Blandford, G.E.; Louis, S. Current Research on Cold-Formed Steel Structures; International Specialty Conference on Cold-Formed Steel Structures. 1990. Available online: https://scholarsmine.mst.edu/isccss/10iccfss/10iccfss-session7/3 (accessed on 24 November 2023).
- Lechner, B.; Pircher, M. Analysis of imperfection measurements of structural members. Thin-Walled Struct. 2005, 43, 351–374. [Google Scholar] [CrossRef]
- Amouzegar, H.; Amirzadeh, B.; Zhao, X.; Schafer, B.; Tootkaboni, M. Statistical analysis of the impact of imperfection modes on collapse behavior of cold-formed steel members. In Proceedings of the Structural Stability Research Council Annual Sta, Nashville, TN, USA, 24–27 March 2015. [Google Scholar]
- Farzanian, S.; Louhghalam, A.; Schafer, B.W.; Tootkaboni, M. Geometric imperfection models for CFS structural members, Part I: Comparative review of current models. Thin-Walled Struct. 2019. [Google Scholar] [CrossRef]
- AISI S240-15; North American Standard for Cold Formed Steel Structural Framing. American Iron and Steel Institute: Washington, DC, USA, 2015.
- GB 50017-2017; Steel Structure Design Standard. China Construction Industry Press Beijing: Beijing, China, 2017.
- Gonçalves, R.; Dinis, P.B.; Camotim, D. GBT formulation to analyse the first-order and buckling behaviour of thin-walled members with arbitrary cross-sections. Thin-Walled Struct. 2009, 47, 583–600. [Google Scholar] [CrossRef]
- Gonçalves, R.; Camotim, D. Generalised beam theory-based finite elements for elastoplastic thin-walled metal members. Thin-Walled Struct. 2011, 49, 1237–1245. [Google Scholar] [CrossRef]
- Abambres, M.; Camotim, D.; Silvestre, N. Modal decomposition of thin-walled member collapse mechanisms. Thin-Walled Struct. 2014, 74, 269–291. [Google Scholar] [CrossRef]
- Duan, L.; Zhao, J.; Liu, S. A B-splines based nonlinear GBT formulation for elastoplastic analysis of prismatic thin-walled members. Eng. Struct. 2016, 110, 325–346. [Google Scholar] [CrossRef]
- Hancock, G.J.; Pham, C.H. Buckling analysis of thin-walled sections under localised loading using the semi-analytical finite strip method. Thin-Walled Struct. 2015, 86, 35–46. [Google Scholar] [CrossRef]
- Ádány, S.; Joó, A.L.; Schafer, B.W. Buckling modes identification of thin-walled members using cFSM base functions. Thin-Walled Struct. 2010, 48, 806–817. [Google Scholar] [CrossRef]
- Duan, L.; Zhao, J.; Zou, J. Generalized beam theory-based advanced beam finite elements for linear buckling analyses of perforated thin-walled members. Comput. Struct. 2022, 259, 106683. [Google Scholar] [CrossRef]
- Abaqus Documentation 6.14 (2014); Dassault Systemes Simulia Corp.: Providence, RI, USA, 2014.

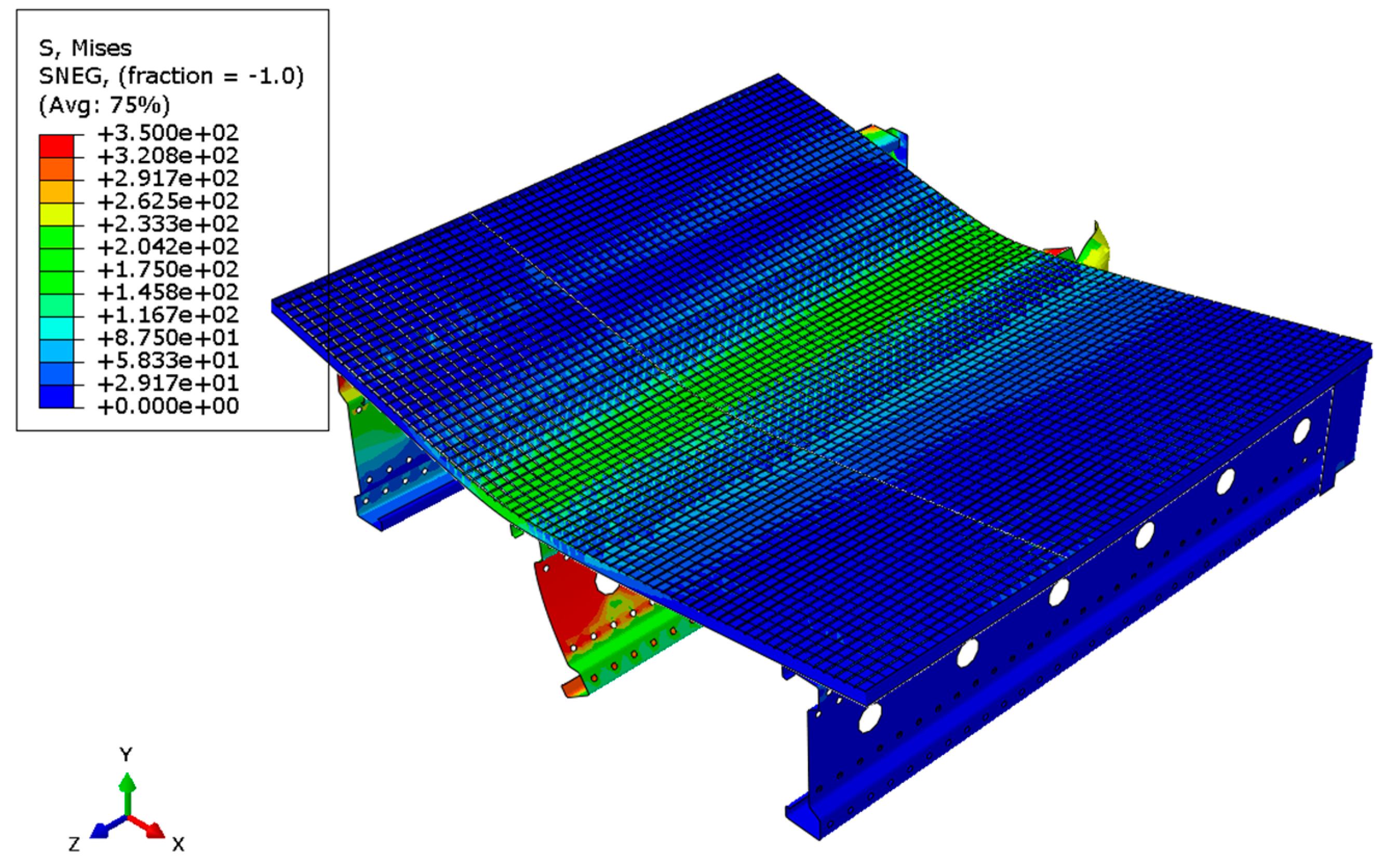
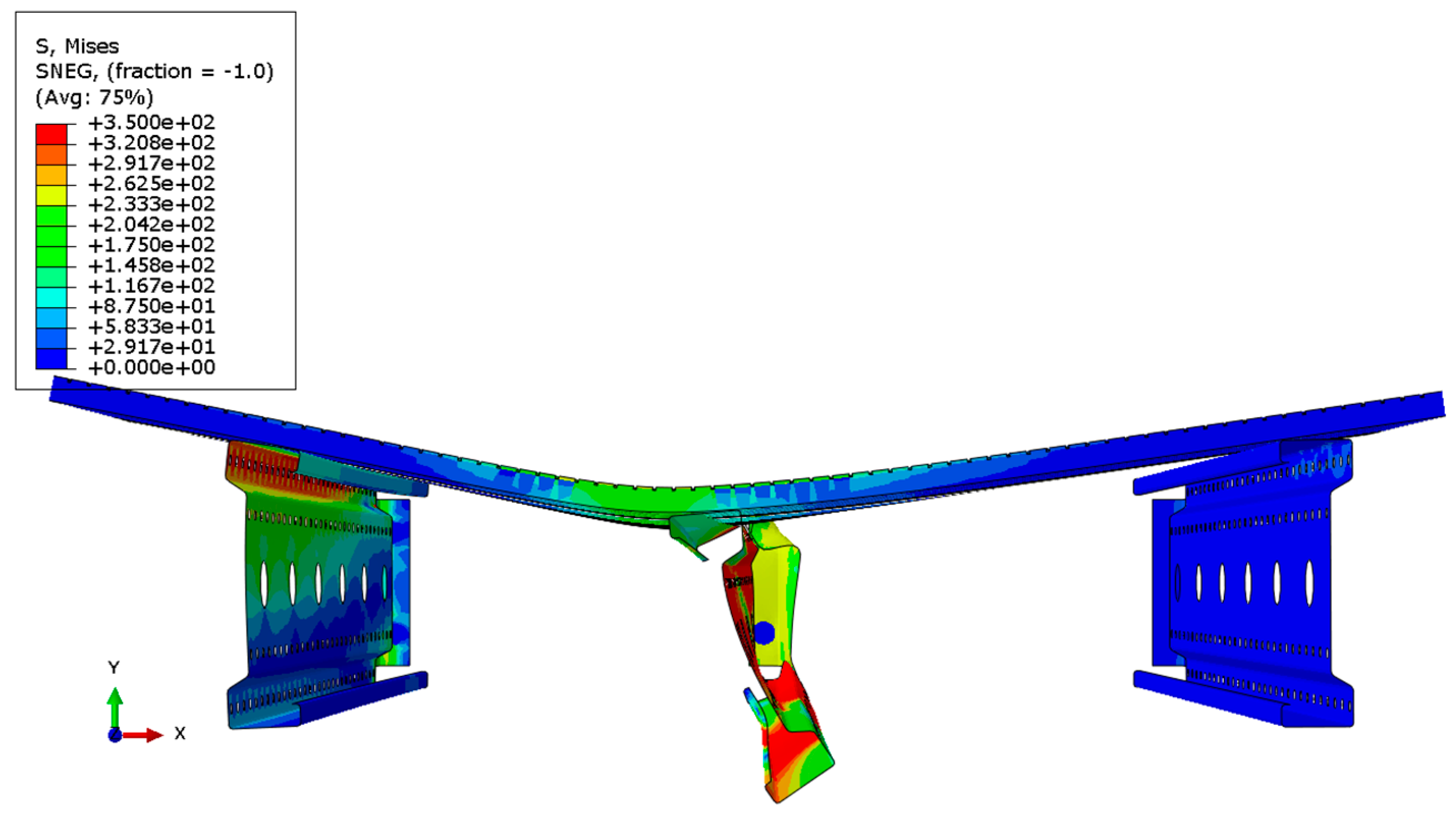
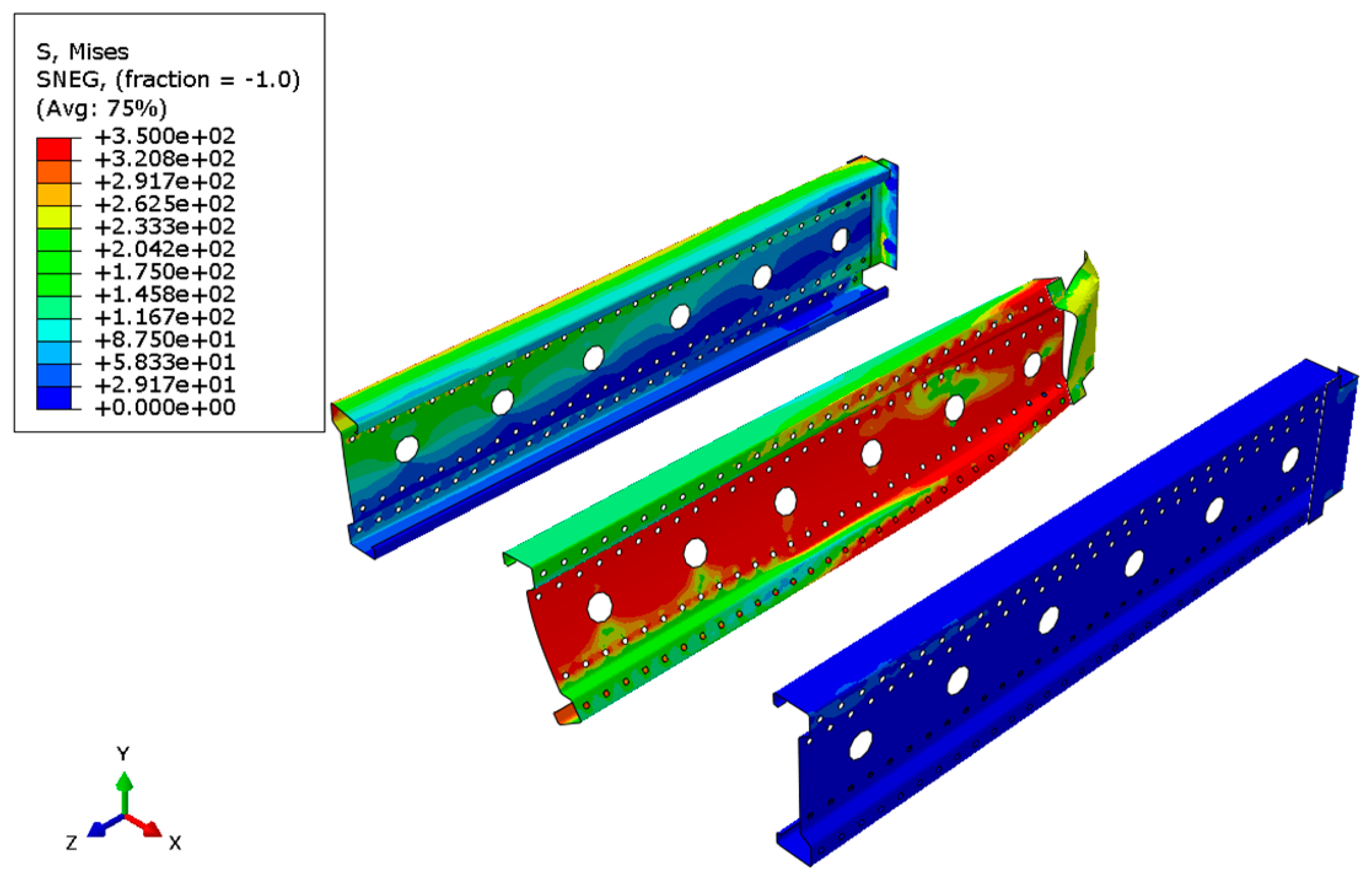
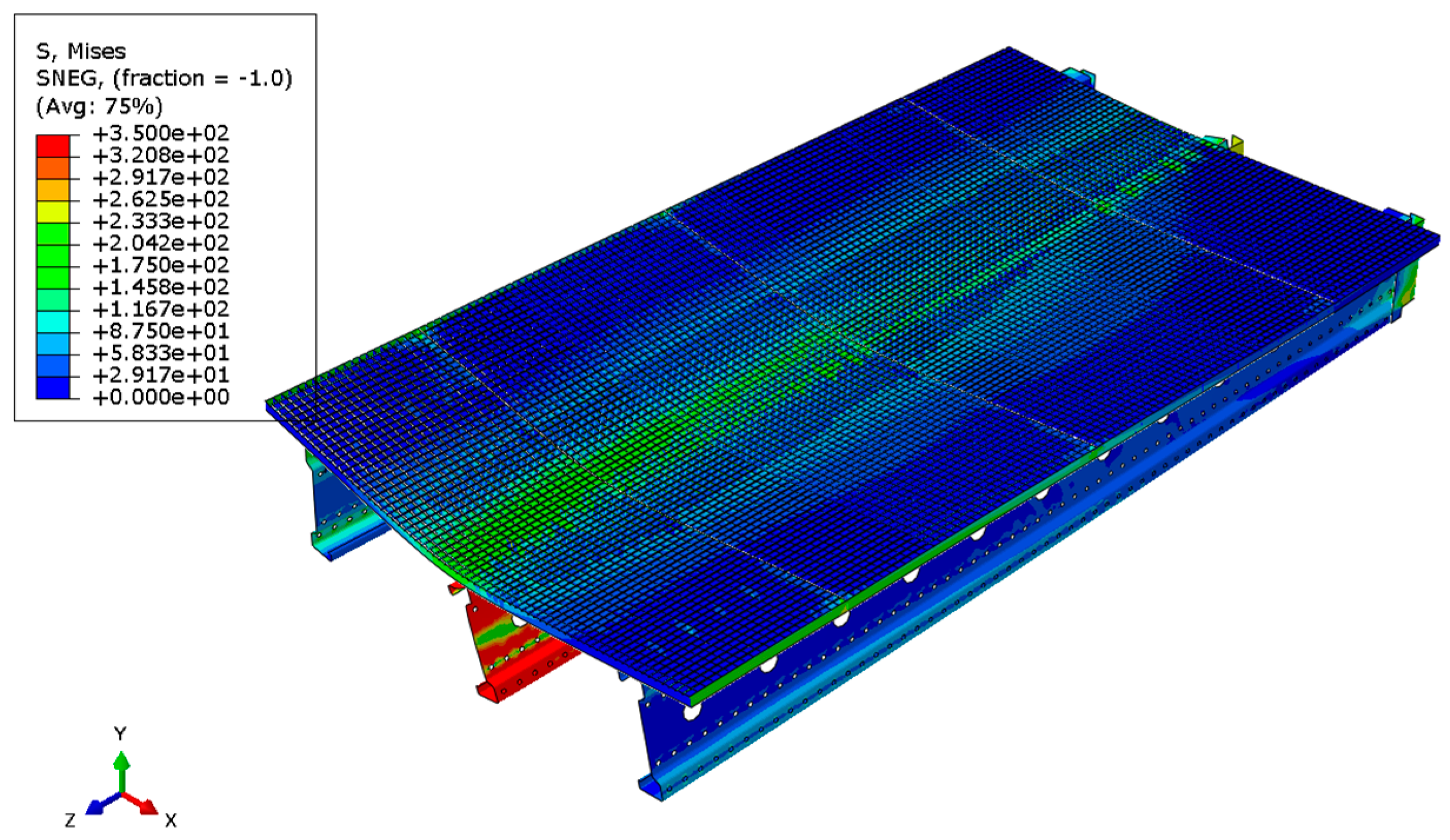
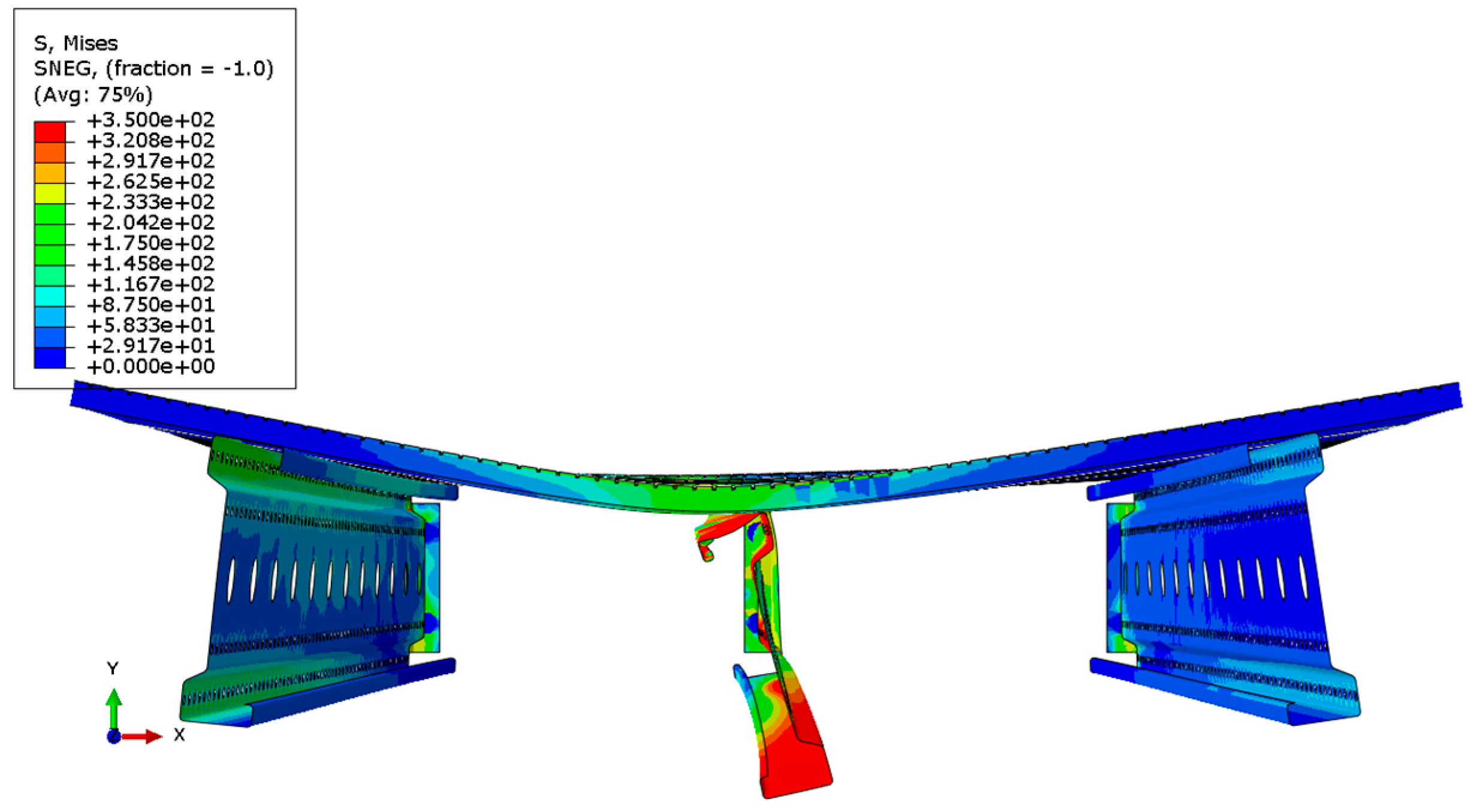

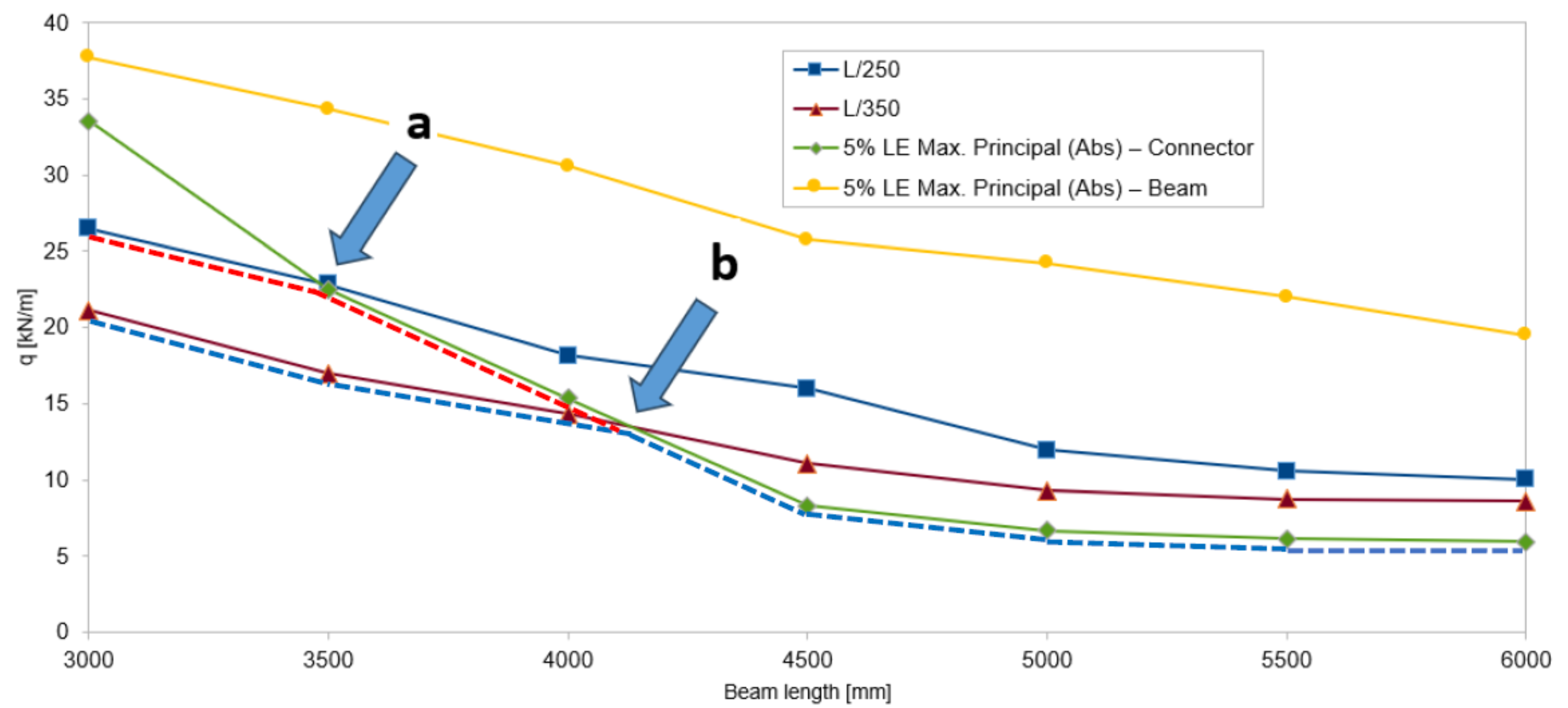
| Load Taken over by the Right Beam [%] | Load Taken over by the Central Beam [%] | Load Taken over by the Left Beam [%] | Length of the Beam [m] |
|---|---|---|---|
| 1.284 | 89.514 | 9.203 | 3.0 |
| 1.903 | 89.582 | 8.515 | 3.5 |
| 3.481 | 86.969 | 9.55 | 4.0 |
| 4.368 | 85.925 | 9.706 | 4.5 |
| 3.682 | 87.064 | 9.254 | 5.0 |
| 3.593 | 87.799 | 8.608 | 5.5 |
| 3.501 | 87.825 | 8.674 | 6.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Denisiewicz, A.; Socha, T.; Kula, K.; Macek, W.; Błażejewski, W.; Lesiuk, G. Numerical Determination of the Load-Bearing Capacity of a Perforated Thin-Walled Beam in a Structural System with a Steel Grating. Appl. Sci. 2024, 14, 1505. https://doi.org/10.3390/app14041505
Denisiewicz A, Socha T, Kula K, Macek W, Błażejewski W, Lesiuk G. Numerical Determination of the Load-Bearing Capacity of a Perforated Thin-Walled Beam in a Structural System with a Steel Grating. Applied Sciences. 2024; 14(4):1505. https://doi.org/10.3390/app14041505
Chicago/Turabian StyleDenisiewicz, Arkadiusz, Tomasz Socha, Krzysztof Kula, Wojciech Macek, Wojciech Błażejewski, and Grzegorz Lesiuk. 2024. "Numerical Determination of the Load-Bearing Capacity of a Perforated Thin-Walled Beam in a Structural System with a Steel Grating" Applied Sciences 14, no. 4: 1505. https://doi.org/10.3390/app14041505
APA StyleDenisiewicz, A., Socha, T., Kula, K., Macek, W., Błażejewski, W., & Lesiuk, G. (2024). Numerical Determination of the Load-Bearing Capacity of a Perforated Thin-Walled Beam in a Structural System with a Steel Grating. Applied Sciences, 14(4), 1505. https://doi.org/10.3390/app14041505








