A Precise Modeling Method for PMSM Servo System of Spacecraft
Abstract
1. Introduction
- (1)
- From the perspective of multi-disciplinary modeling, a PMSM servo system model for spacecraft was established for the first time, which includes three nonlinear factors: elasticity, backlash, and friction. This model comprehensively characterizes the influence mechanism of nonlinear factors on the PMSM servo system.
- (2)
- The backlash features were integrated into the elasticity to form an elastic dead zone model. Besides, considering the effects of impact vibration and environmental temperature on the LuGre friction model, some improvements were made to it.
- (3)
- By analyzing the difference between simulation and experimental data, a high-precision PMSM servo system model was formed, which can provide the theoretical basis for control method research and system performance optimization.
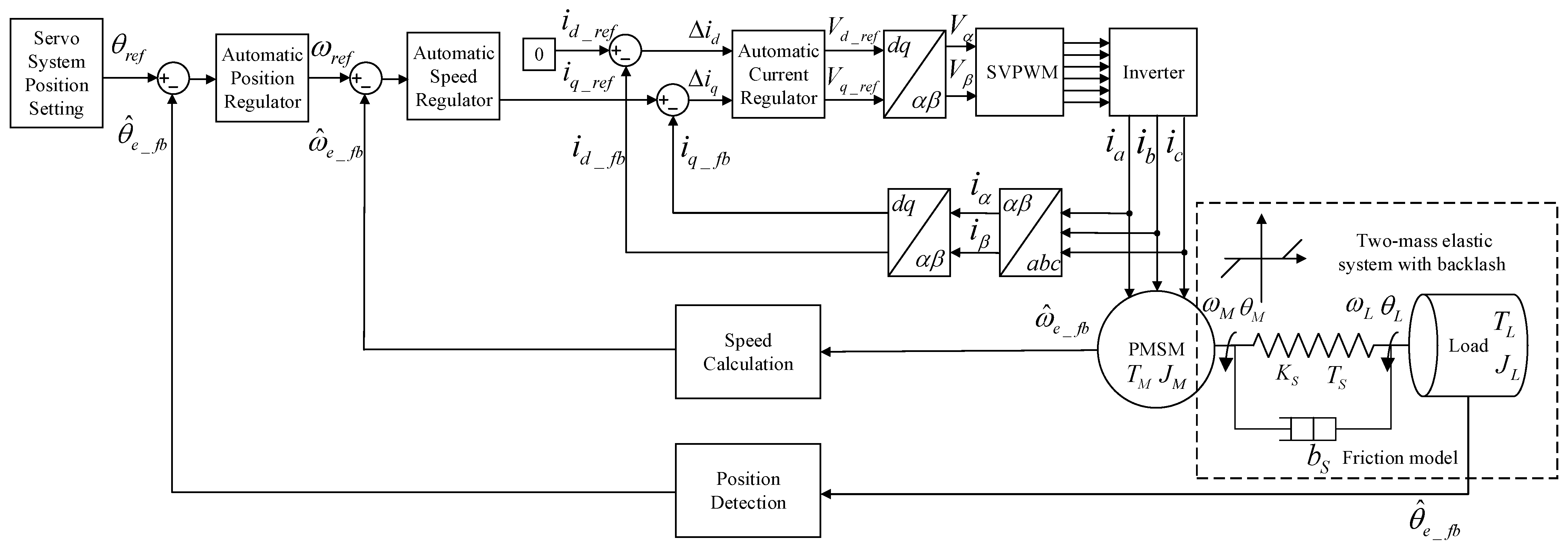
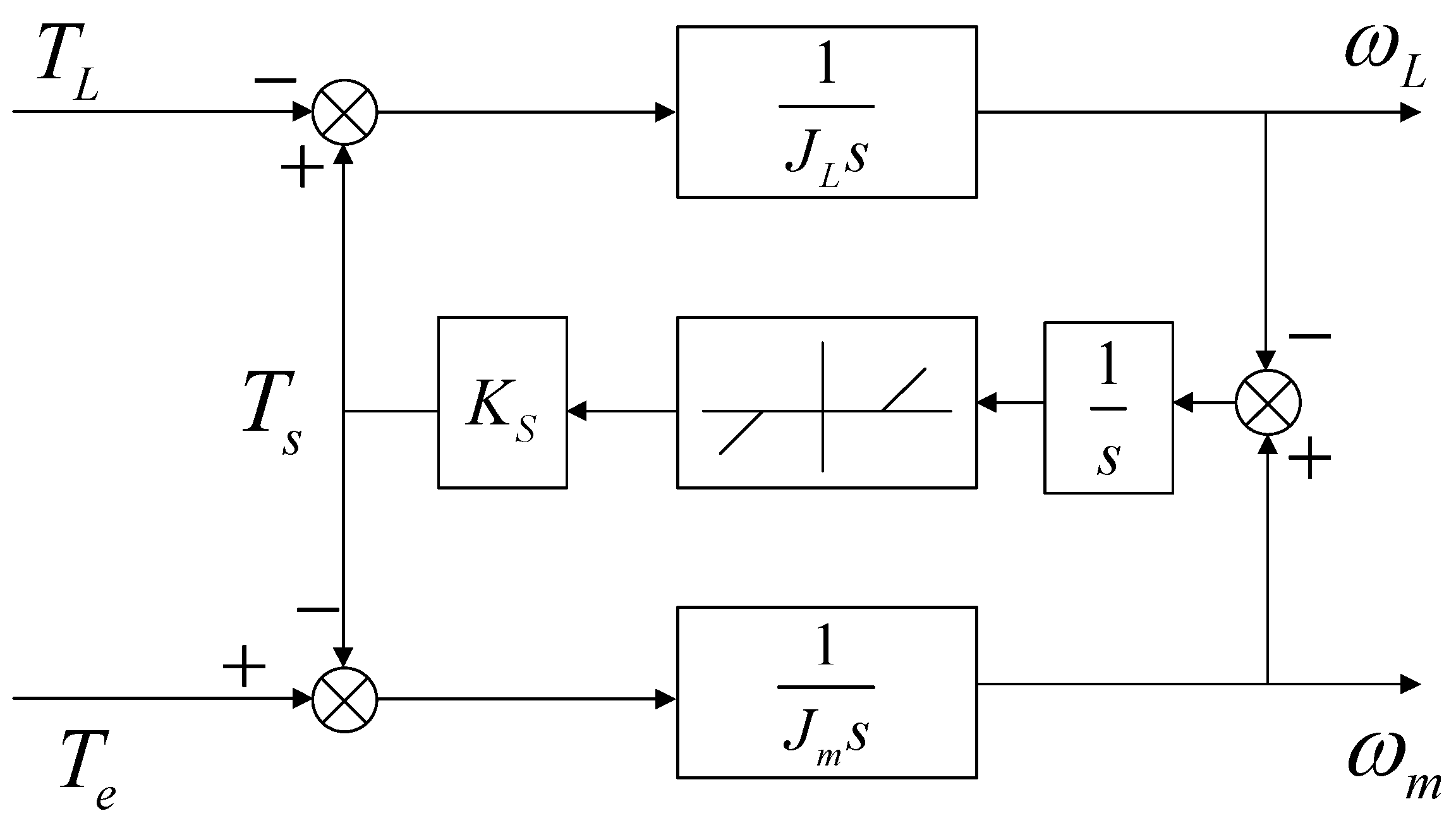
2. PMSM Servo System
3. Nonlinear Precise Modeling
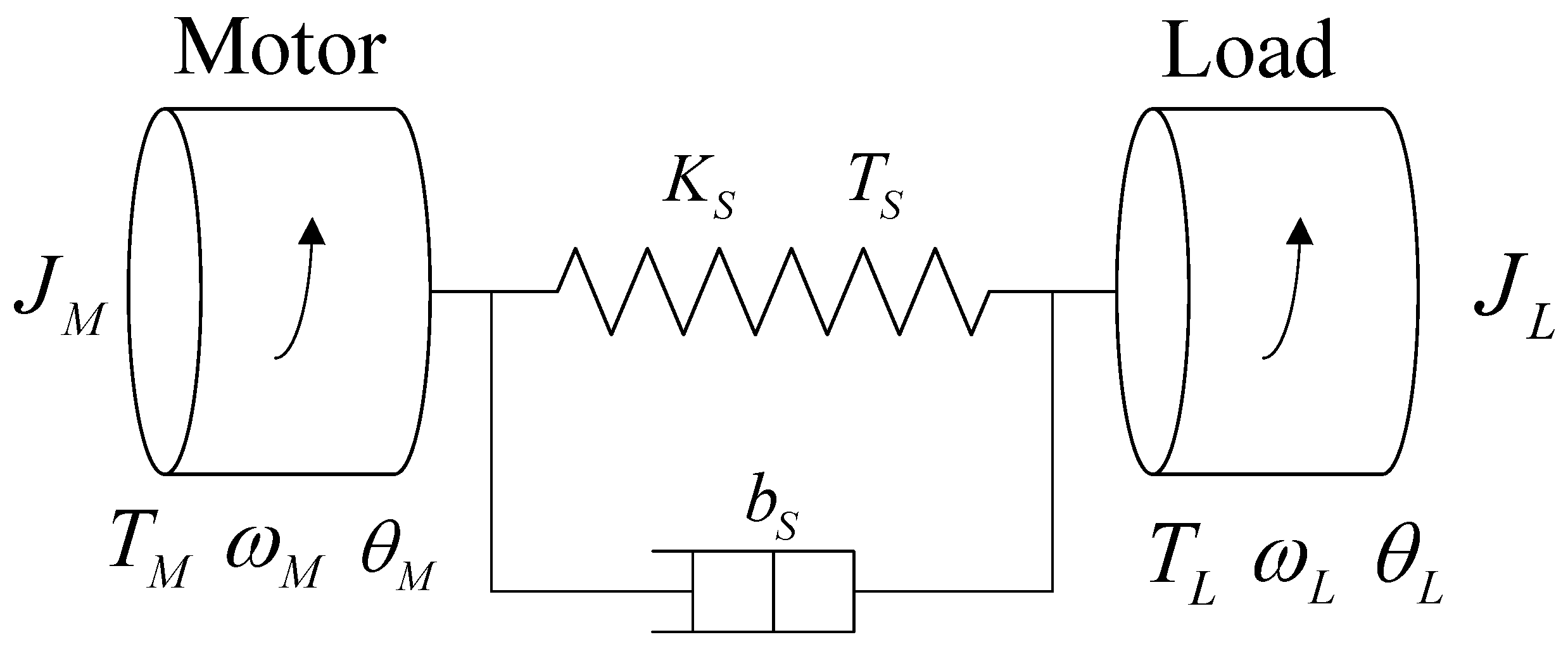
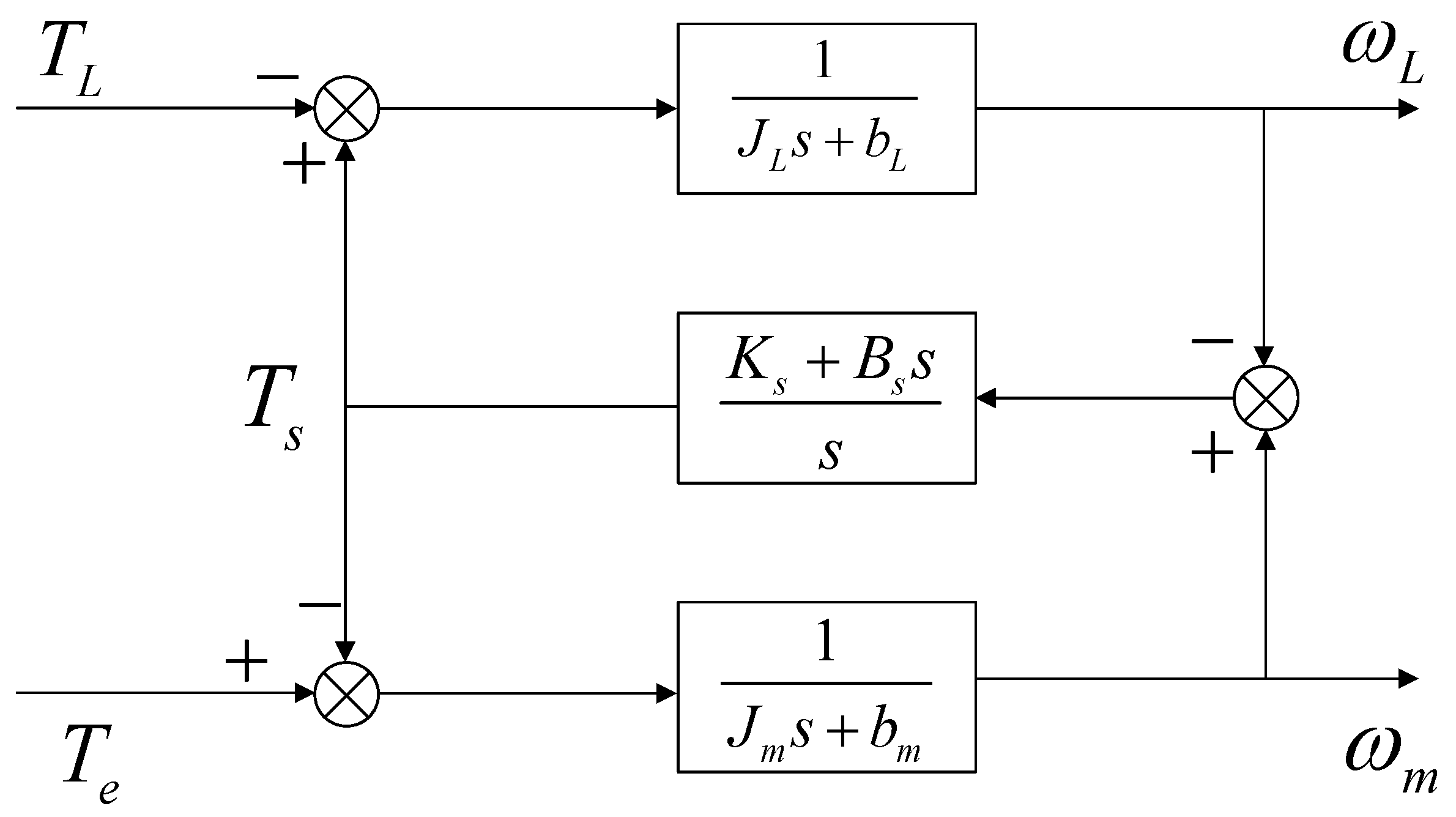
3.1. Modeling of Elastic Characteristics
3.2. Backlash Characteristic Modeling
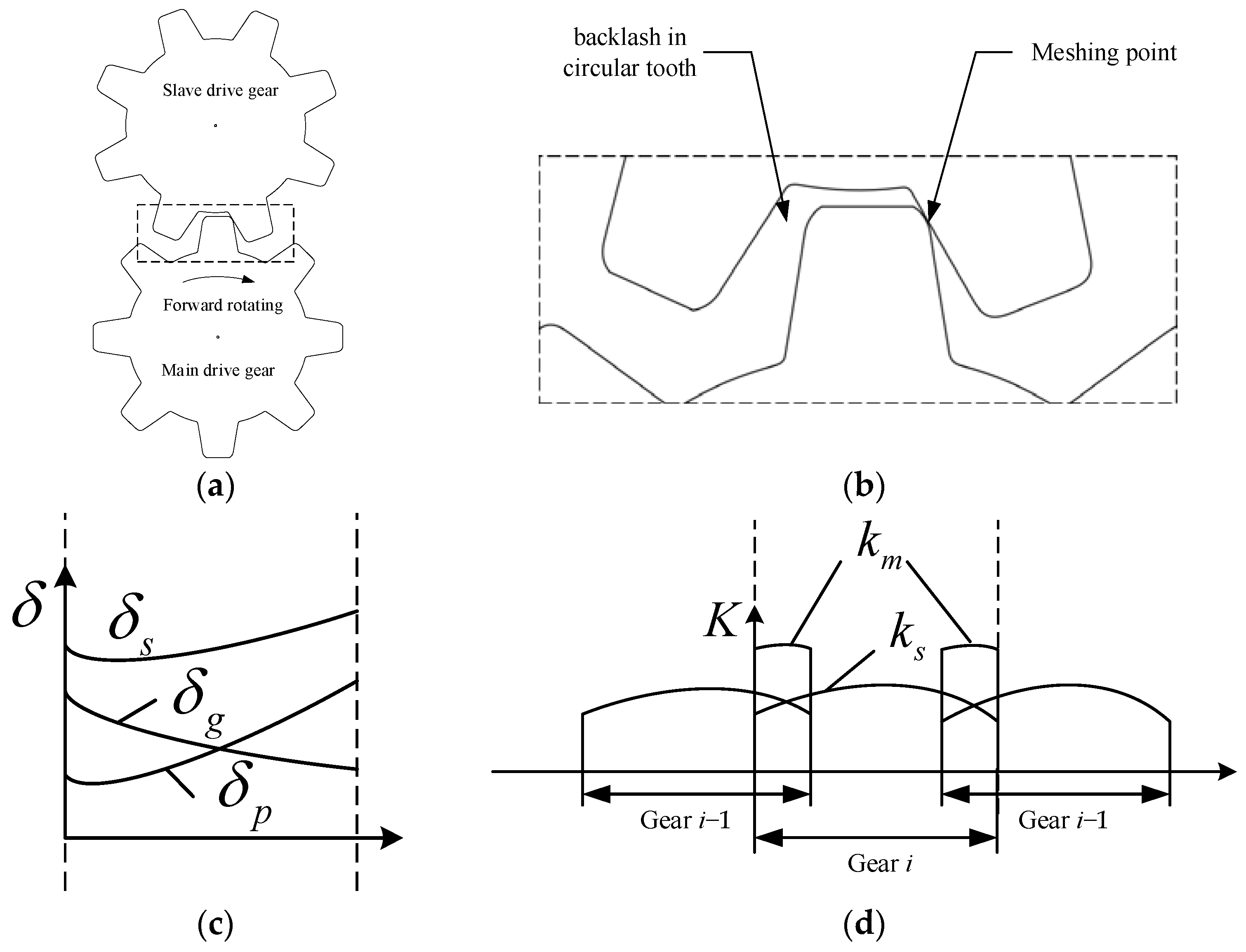
3.3. Friction Characteristics Modeling
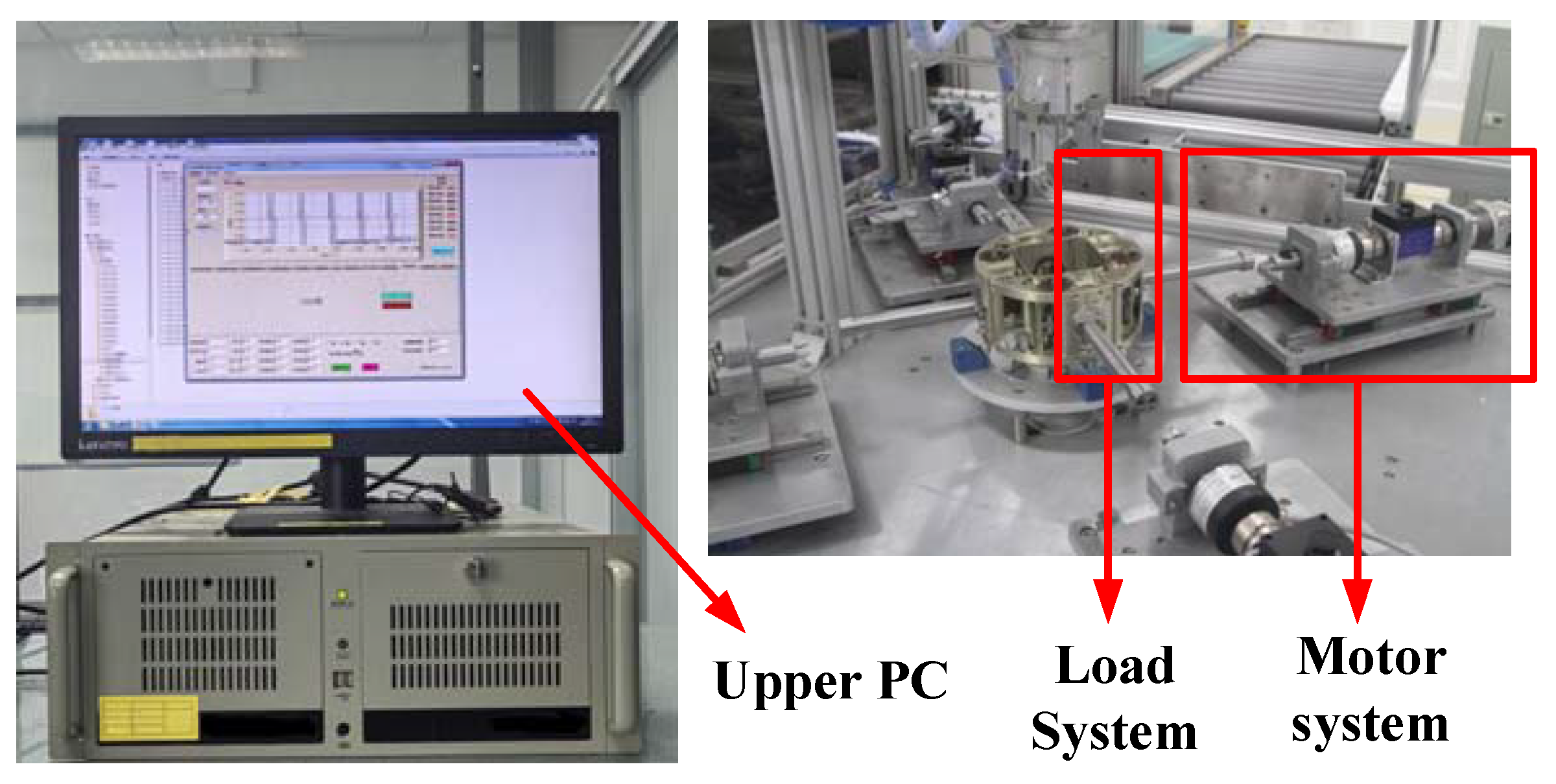
4. Experimental Testing and Validation
4.1. Nonlinear Identification
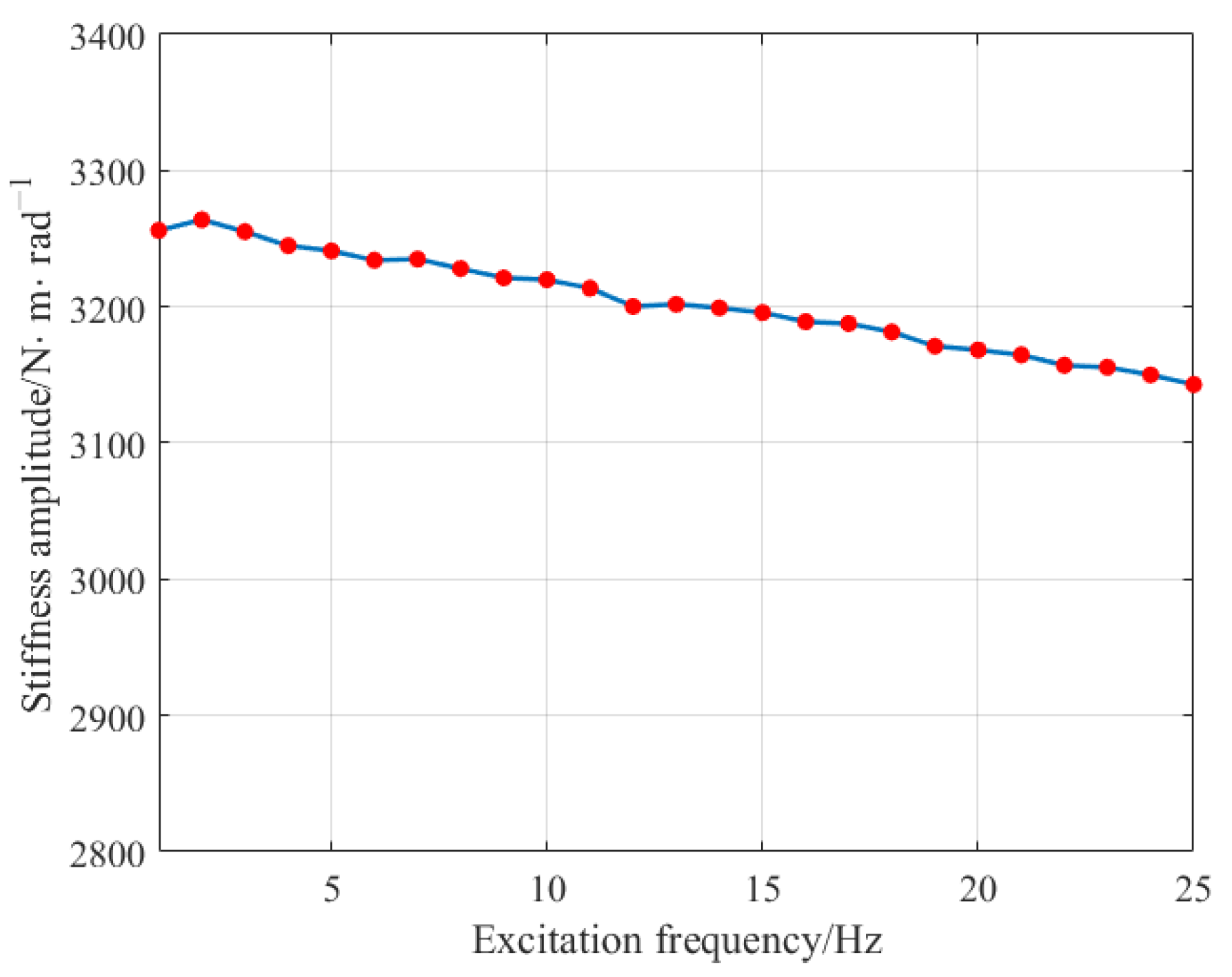
4.1.1. Elastic Model Parameter Identification
4.1.2. Dead-Zone Backlash Parameter Identification
4.1.3. LuGre Friction Parameter Identification
4.2. Servo System Verification
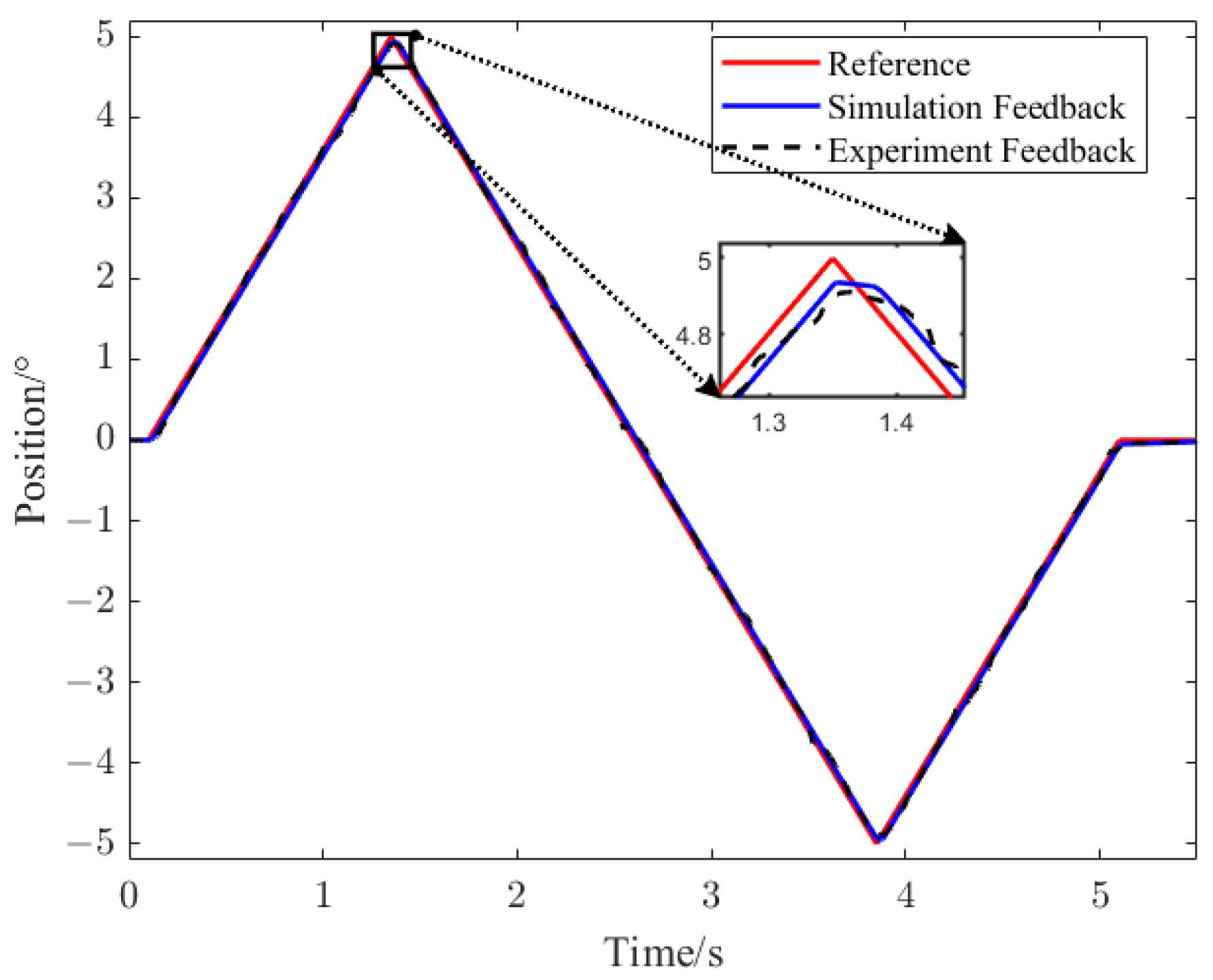
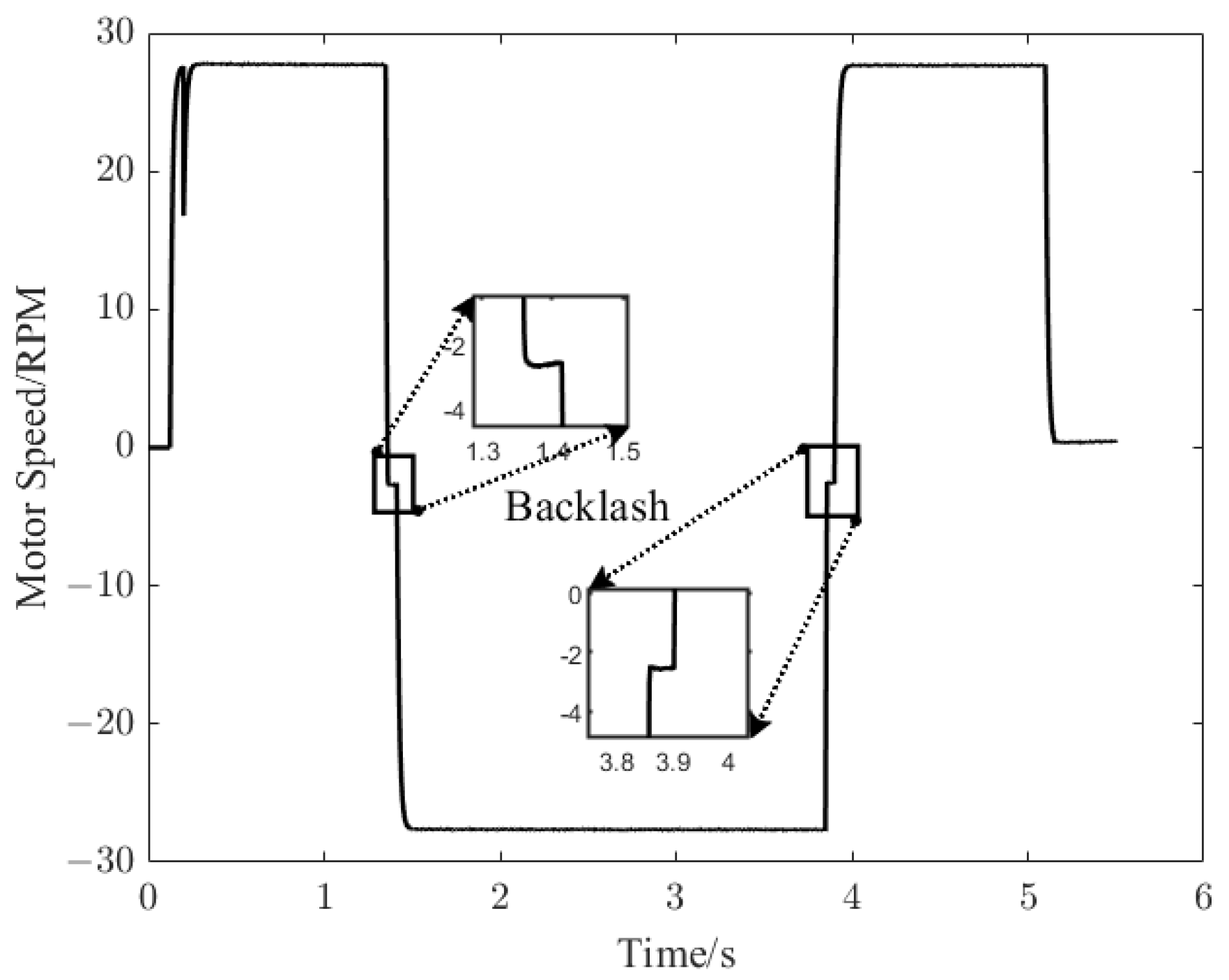
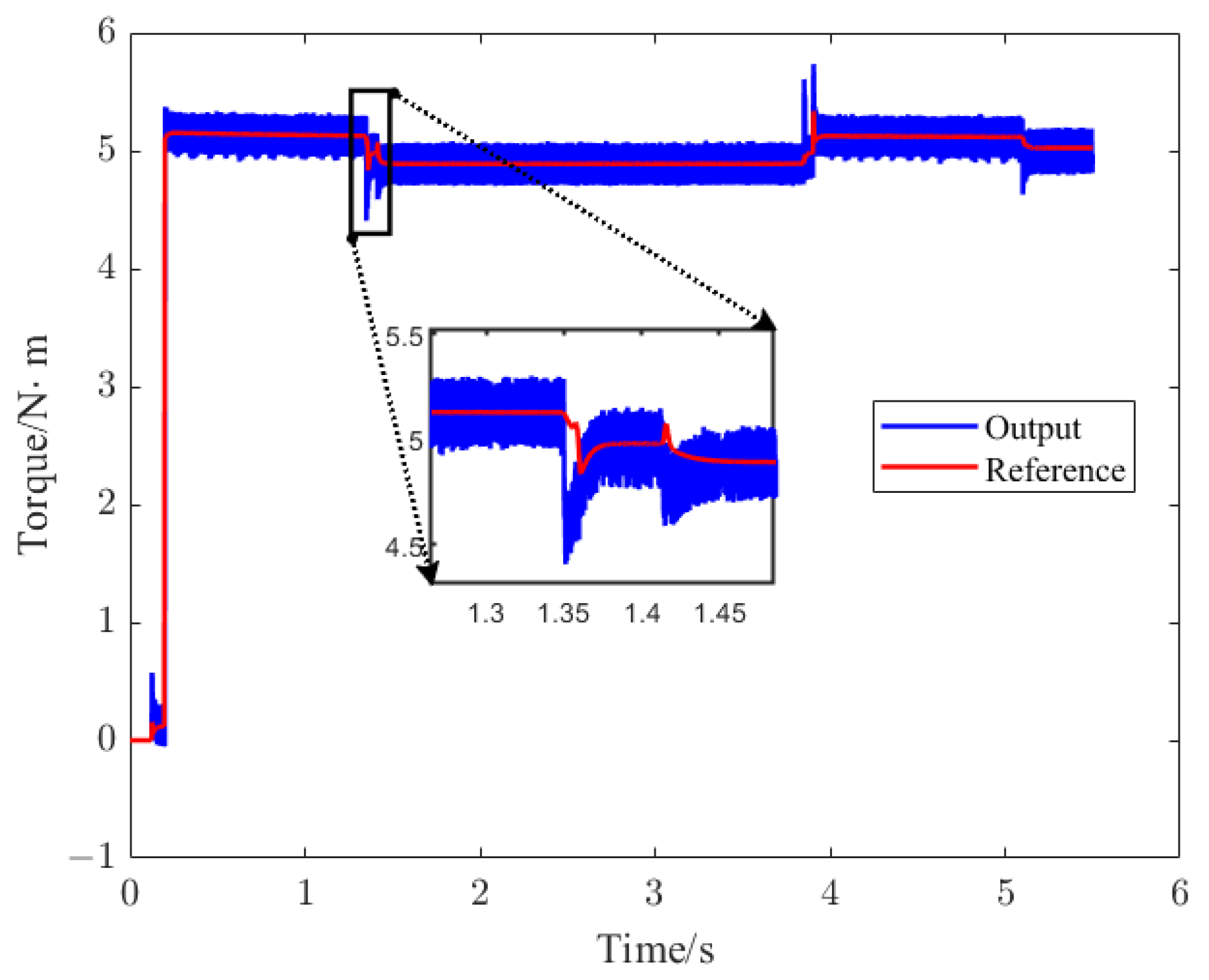
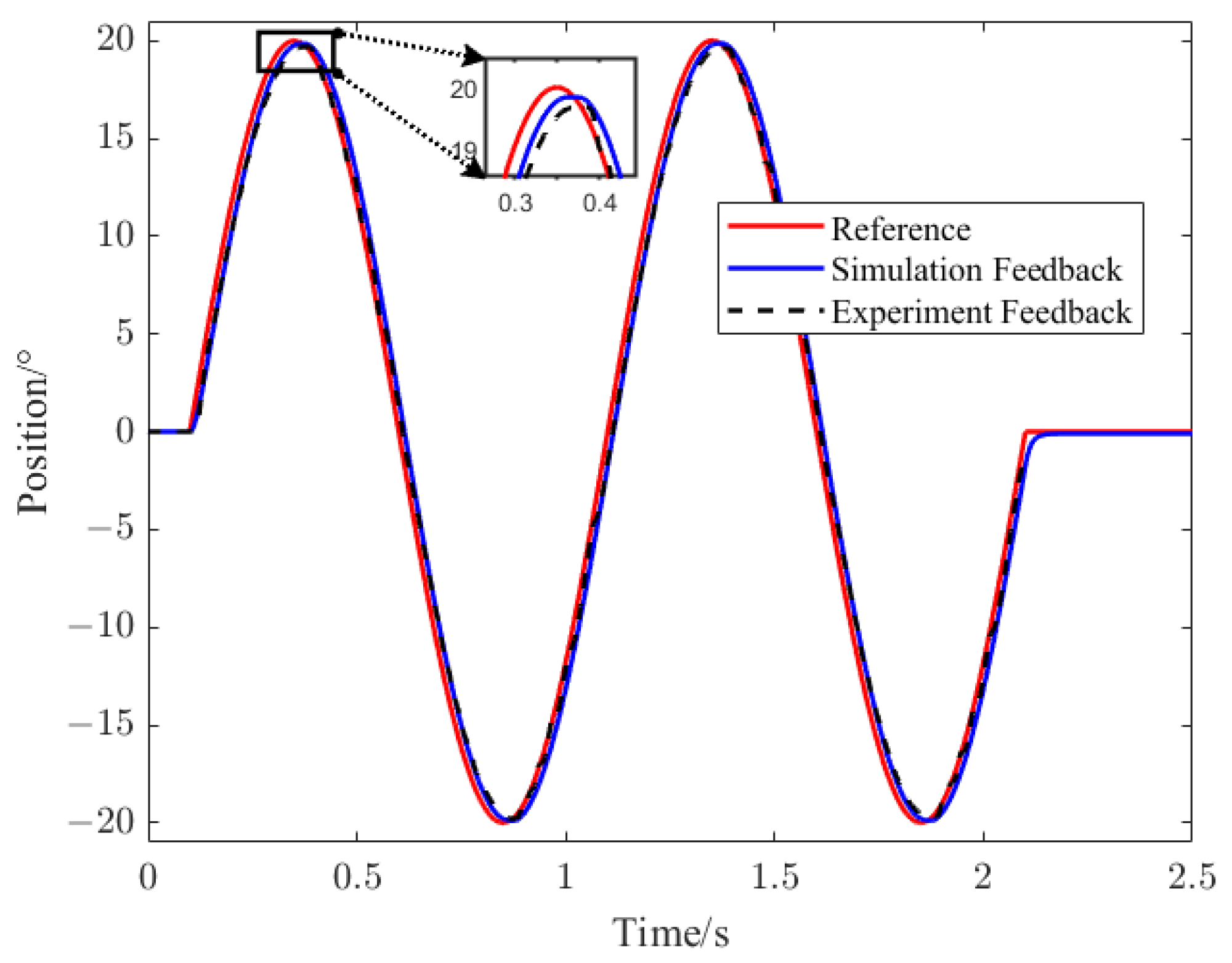
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, C.; Yang, M.; Luan, T.; Xu, D. Review on Identification of External Mechanical Parameters of Two-Mass Elastic Servo System. Proc. CSEE 2016, 36, 804–817. [Google Scholar]
- Padron, J.; Yokokura, Y.; Ohishi, K.; Miyazaki, T.; Kawai, Y. Evaluating the Equivalence between Nonlinear Friction and Backlash in Two-Inertia Systems. In Proceedings of the 2022 IEEE 17th International Conference on Advanced Motion Control (AMC), Padova, Italy, 18–20 February 2022; pp. 335–340. [Google Scholar]
- Nowopolski, K.; Wicher, B.; Zawirski, K.; Tułodziecki, M. Control of Electromechanical Object with Backlash and Elasticity within Interconnection between Motor and Load. In Proceedings of the 2015 Selected Problems of Electrical Engineering and Electronics (WZEE), Kielce, Poland, 17–19 September 2015; pp. 1–6. [Google Scholar]
- Jiao, Z.; Chen, X.; Liu, X.; Li, X.; Qi, P.; Shang, Y.; Qiao, W. An Experimental Study on Outer Frame Position Control of Hydraulic Flight Motion Simulator with Model Compensation. IEEE/ASME Trans. Mechatron. 2022, 27, 3419–3428. [Google Scholar] [CrossRef]
- Santos Ballesteros, H.M.; das Neves Calvo, R.; Filho, A.A. Dynamic Stiffness Enhancement of a Flight Control Actuator Using Control Techniques. In Proceedings of the 2017 IEEE International Conference on Mechatronics (ICM), Churchill, Australia, 13–15 February 2017; pp. 260–265. [Google Scholar]
- Chuthai, T.; Cole, M.O.T.; Wongratanaphisan, T.; Puangmali, P. Adaptive Kinematic Mapping Based on Chebyshev Interpolation: Application to Flexure-Jointed Micromanipulator Control. IEEE/ASME Trans. Mechatron. 2020, 25, 118–129. [Google Scholar] [CrossRef]
- Li, K.; Wang, H.; Liang, X.; Miao, Y. Visual Servoing of Flexible-Link Manipulators by Considering Vibration Suppression Without Deformation Measurements. IEEE Trans. Cybern. 2022, 52, 12454–12463. [Google Scholar] [CrossRef] [PubMed]
- Gao, H.; He, W.; Zhou, C.; Sun, C. Neural Network Control of a Two-Link Flexible Robotic Manipulator Using Assumed Mode Method. IEEE Trans. Ind. Inform. 2019, 15, 755–765. [Google Scholar] [CrossRef]
- Masoud, A.E.; Maas, J. Limit Cycle Analysis for Drive Systems with Backlash Nonlinearity Using an Eigenvalue Method. In Proceedings of the 2019 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Hong Kong, China, 8–12 July 2019; pp. 1409–1414. [Google Scholar]
- Yang, M.; Wang, C.; Xu, D.; Zheng, W.; Lang, X. Shaft Torque Limiting Control Using Shaft Torque Compensator for Two-Inertia Elastic System with Backlash. IEEE/ASME Trans. Mechatron. 2016, 21, 2902–2911. [Google Scholar] [CrossRef]
- Shi, Z.; Zuo, Z. Backstepping Control for Gear Transmission Servo Systems with Backlash Nonlinearity. IEEE Trans. Autom. Sci. Eng. 2015, 12, 752–757. [Google Scholar] [CrossRef]
- Wang, C.; Yang, M.; Zheng, W.; Hu, K.; Xu, D. Analysis and Suppression of Limit Cycle Oscillation for Transmission System with Backlash Nonlinearity. IEEE Trans. Ind. Electron. 2017, 64, 9261–9270. [Google Scholar] [CrossRef]
- Wicher, B.; Brock, S. Tuning Optimization of Extended State Observer for Two Mass System with Elastic Joint and Backlash. In Proceedings of the 2018 18th International Conference on Mechatronics—Mechatronika (ME), Brno, Czech Republic, 5–7 December 2018; pp. 1–6. [Google Scholar]
- Phuong, T.H.; Belov, M.P.; Thuy, D.V. Adaptive Model Predictive Control for Nonlinear Elastic Electrical Transmission Servo Drives. In Proceedings of the 2019 IEEE Conference of Russian Young Researchers in Electrical and Electronic Engineering (EIConRus), Saint Petersburg and Moscow, Russia, 28–31 January 2019; pp. 704–708. [Google Scholar]
- Peng, J.; Wang, C.; Zheng, W.; Pan, J.; Liu, F.; Yang, J. Compound Mechanism Analysis of Backlash and Friction Nonlinearity in Full-Closed-Loop Servo Drive System. In Proceedings of the 2021 IEEE 7th International Conference on Control Science and Systems Engineering (ICCSSE), Qingdao, China, 30 July–1 August 2021; pp. 130–135. [Google Scholar]
- Liu, D.; Liu, H.; He, Y.; Zhang, B.; Liu, Y.; Li, Z.; Luo, C. Research on Flexible Joint Friction Identification of Space Lab Manipulator. In Proceedings of the 2018 IEEE International Conference on Mechatronics and Automation (ICMA), Piscataway, NJ, USA, 5–8 August 2018; pp. 614–619. [Google Scholar]
- Wang, S.; Na, J. Parameter Estimation and Adaptive Control for Servo Mechanisms with Friction Compensation. IEEE Trans. Ind. Inform. 2020, 16, 6816–6825. [Google Scholar] [CrossRef]
- Thenozhi, S.; Sánchez, A.C.; Rodríguez-Reséndiz, J. A Contraction Theory-Based Tracking Control Design with Friction Identification and Compensation. IEEE Trans. Ind. Electron. 2022, 69, 6111–6120. [Google Scholar] [CrossRef]
- Zhu, M.; Liu, A.; Gao, S.; Gao, Q. Linearization Design of Servo System and Parameter Identification Based on LuGre Model. In Proceedings of the 2021 IEEE 16th Conference on Industrial Electronics and Applications (ICIEA), Chengdu, China, 1–4 August 2021; pp. 1944–1947. [Google Scholar]
- Park, S.-H.; Park, J.-C.; Lee, H.-Y.; Kwon, S.-O.; Lim, M.-S. Design of High-Bandwidth Motor System Considering Electrical and Mechanical Time Constants. IEEE Trans. Ind. Appl. 2020, 56, 4738–4747. [Google Scholar] [CrossRef]
- Yao, J.; Deng, W.; Jiao, Z. Adaptive Control of Hydraulic Actuators with LuGre Model-Based Friction Compensation. IEEE Trans. Ind. Electron. 2015, 62, 6469–6477. [Google Scholar] [CrossRef]
- Wang, C.; Peng, J.; Pan, J. A Novel Friction Compensation Method Based on Stribeck Model with Fuzzy Filter for PMSM Servo Systems. IEEE Trans. Ind. Electron. 2023, 70, 12124–12133. [Google Scholar] [CrossRef]
- Sun, L.; Li, X.; Chen, L.; Shi, H.; Jiang, Z. Dual-Motor Coordination for High-Quality Servo with Transmission Backlash. IEEE Trans. Ind. Electron. 2023, 70, 1182–1196. [Google Scholar] [CrossRef]
- Lukichev, D.V.; Demidova, G.L.; Brock, S. Fuzzy Adaptive PID Control for Two-Mass Servo-Drive System with Elasticity and Friction. In Proceedings of the 2015 IEEE 2nd International Conference on Cybernetics (CYBCONF), Gdynia, Poland, 24–26 June 2015; pp. 443–448. [Google Scholar]

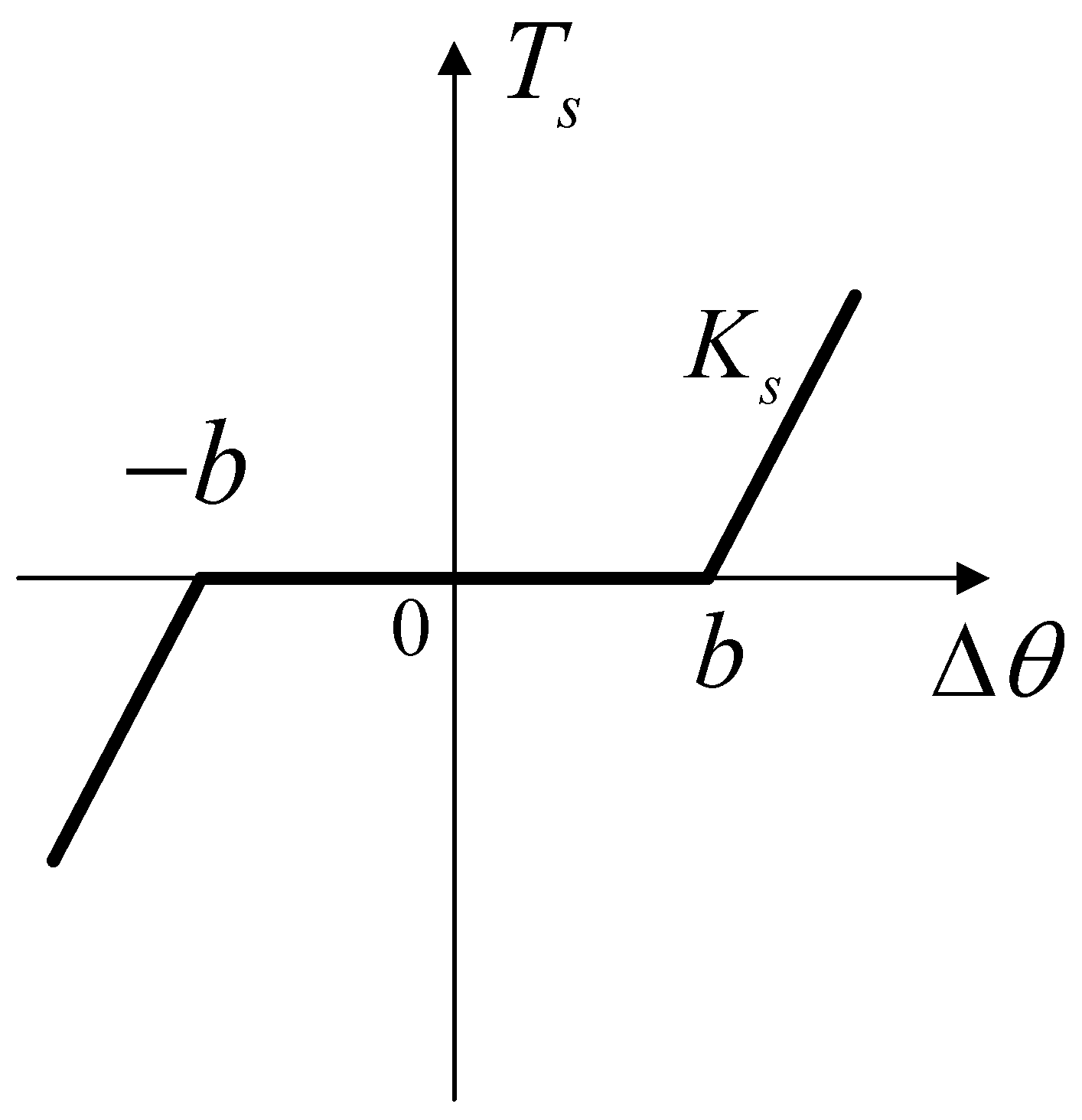
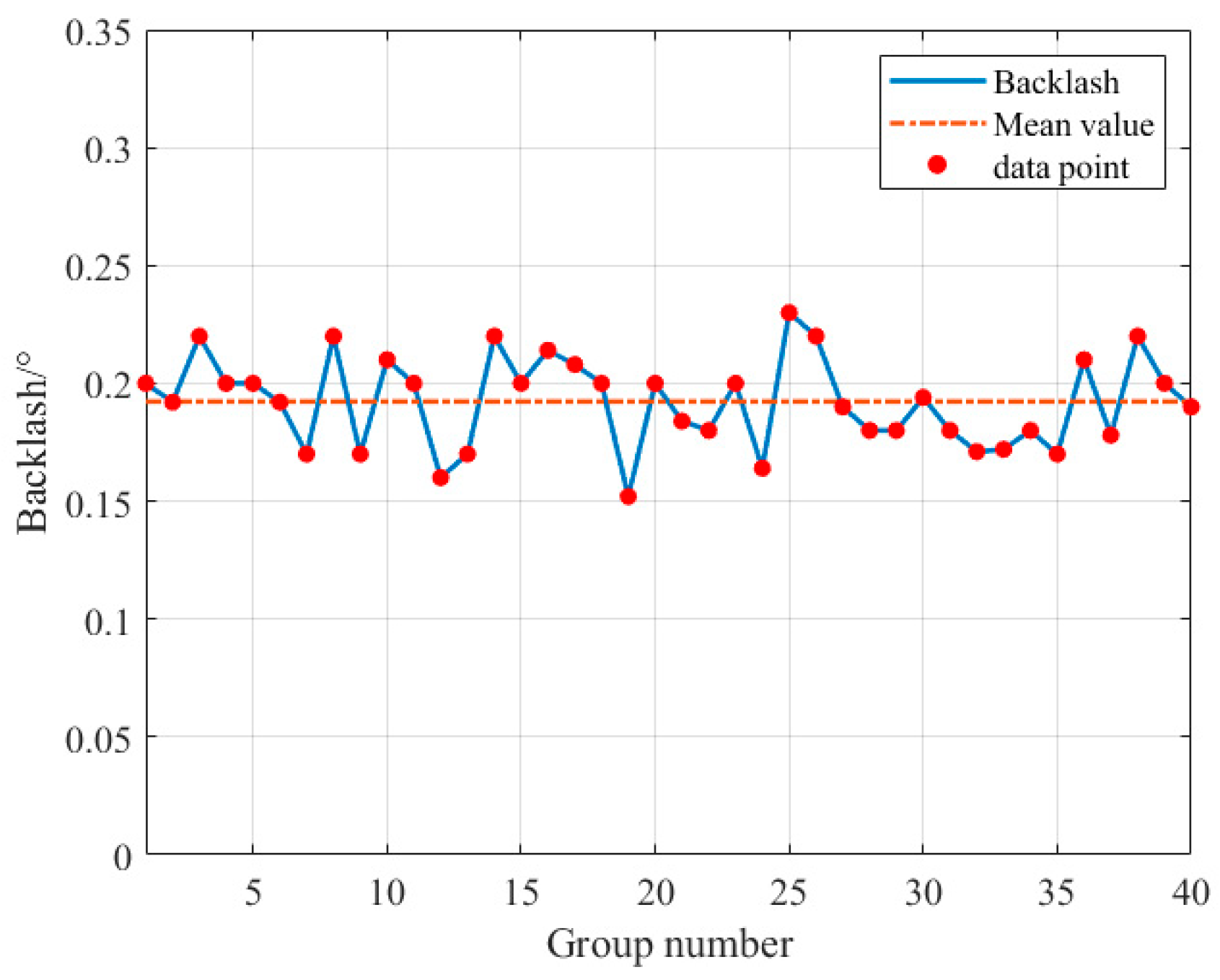
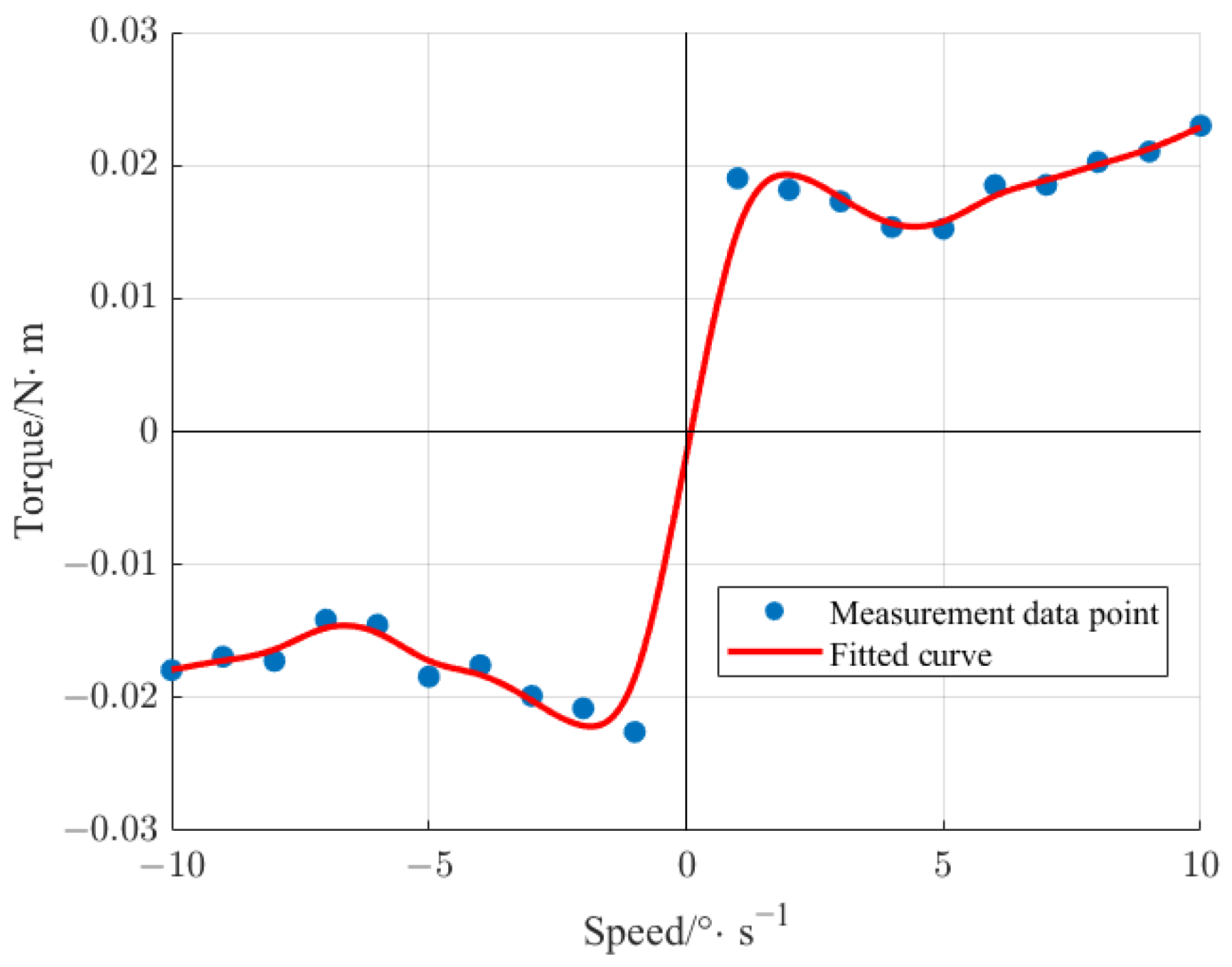
| Static parameters | ||||
| 0.024 | 0.011 | 3.73 |
| Dynamic parameters | ||
| 1.4 | 0.051 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, H.; Song, Z.; Xiao, X.; Mo, Y.; Zhou, W.; Wu, P. A Precise Modeling Method for PMSM Servo System of Spacecraft. Appl. Sci. 2024, 14, 1161. https://doi.org/10.3390/app14031161
Xu H, Song Z, Xiao X, Mo Y, Zhou W, Wu P. A Precise Modeling Method for PMSM Servo System of Spacecraft. Applied Sciences. 2024; 14(3):1161. https://doi.org/10.3390/app14031161
Chicago/Turabian StyleXu, Hang, Zhe Song, Xi Xiao, Yu Mo, Weihong Zhou, and Pengfei Wu. 2024. "A Precise Modeling Method for PMSM Servo System of Spacecraft" Applied Sciences 14, no. 3: 1161. https://doi.org/10.3390/app14031161
APA StyleXu, H., Song, Z., Xiao, X., Mo, Y., Zhou, W., & Wu, P. (2024). A Precise Modeling Method for PMSM Servo System of Spacecraft. Applied Sciences, 14(3), 1161. https://doi.org/10.3390/app14031161






