Modeling of Phosphate Sorption Process on the Surface of Rockfos® Material Using Langmuir Isotherms
Abstract
1. Introduction
2. Materials and Methods
2.1. Material
2.2. Method
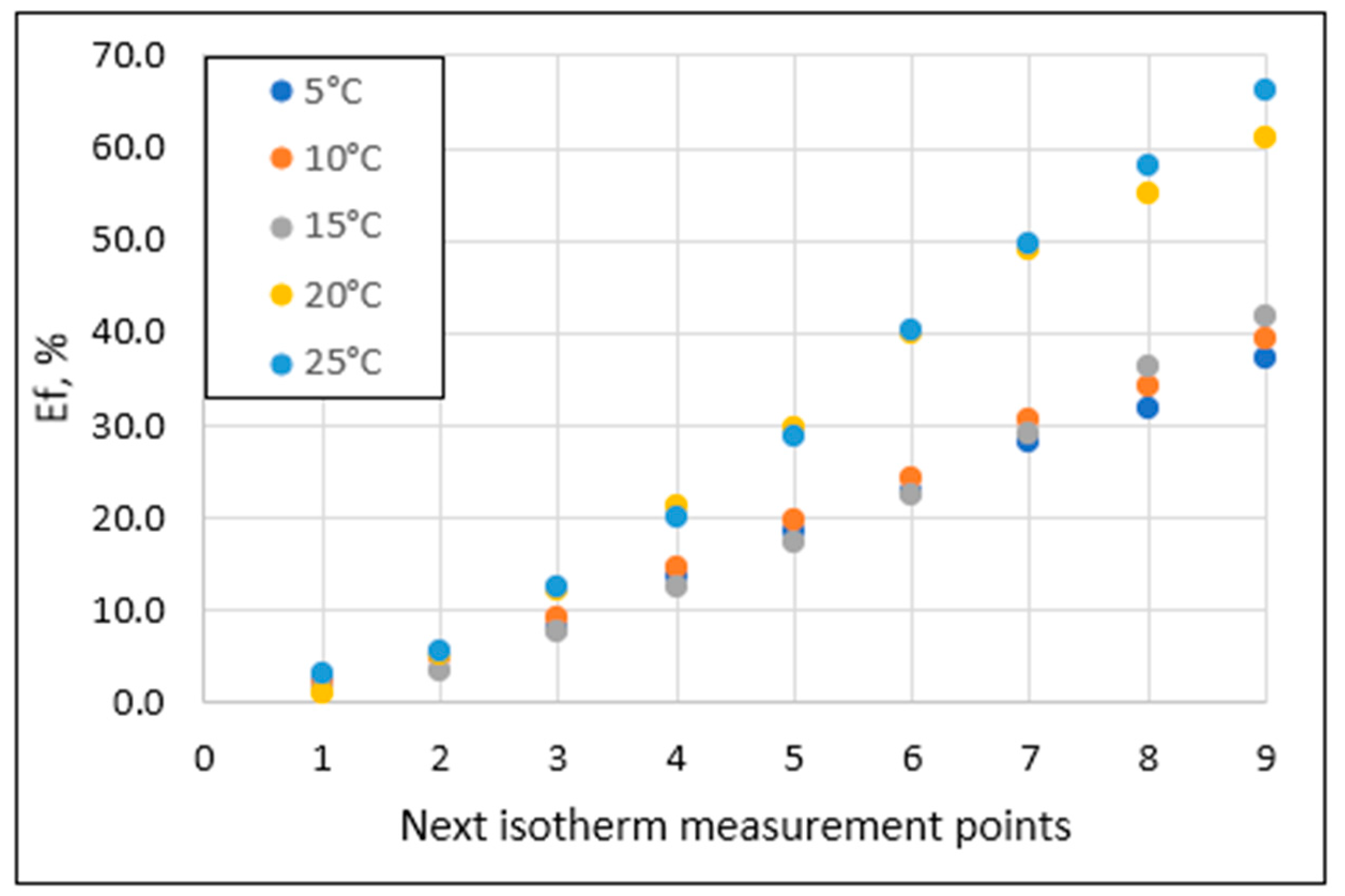
2.3. Evaluation of Phosphate Retention Efficiency
2.4. Models
2.5. Statistical Analysis
2.6. Thermodynamic Analysis
3. Results and Discussion
4. Conclusions
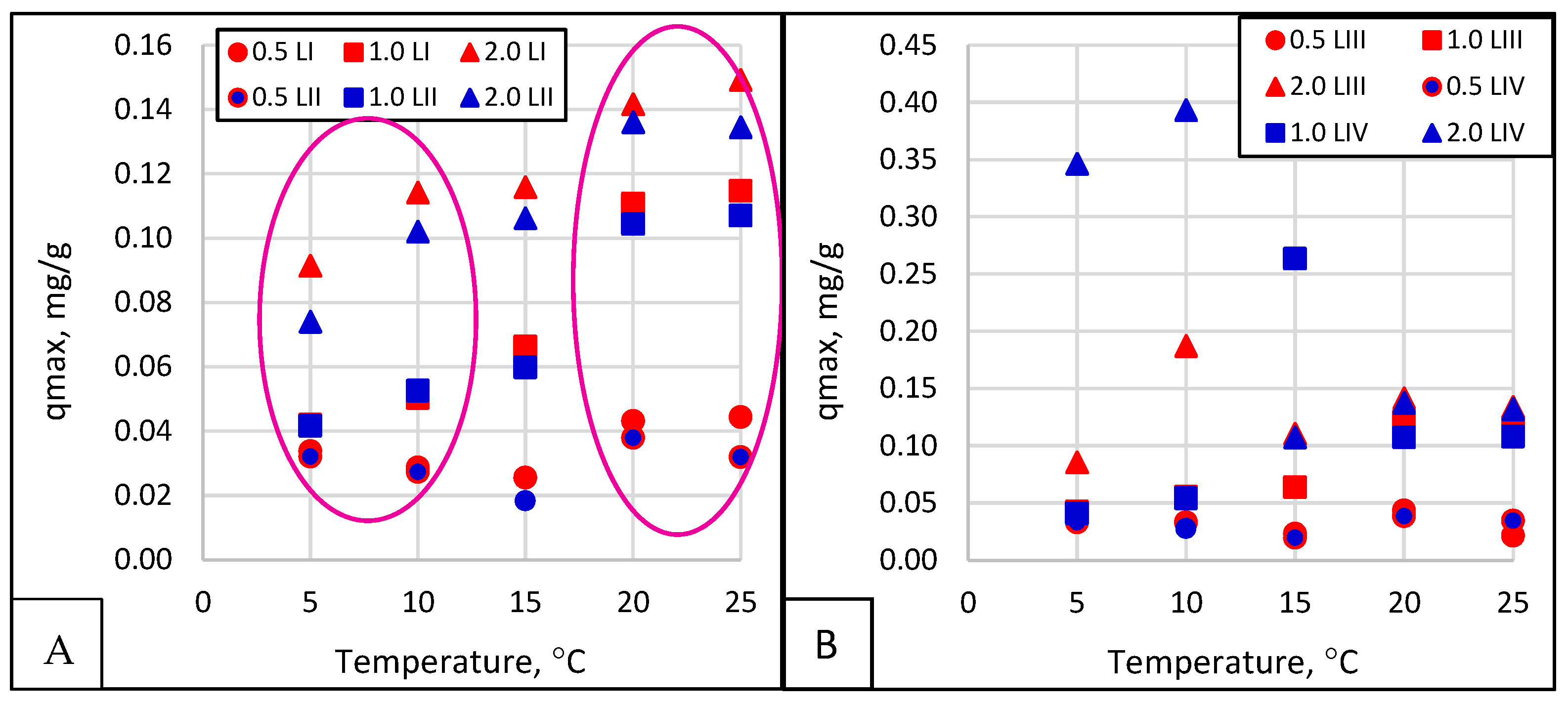
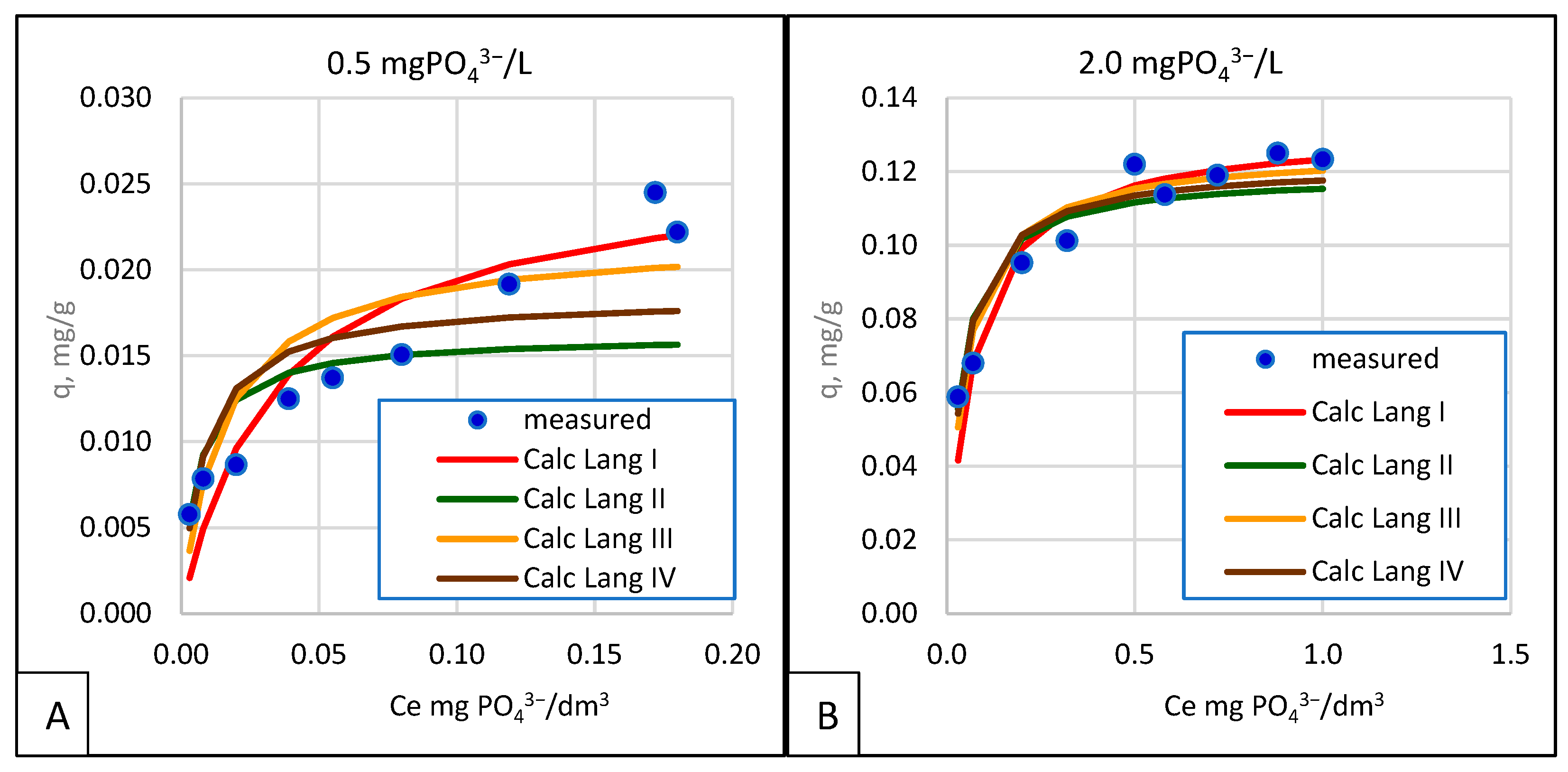
- The Langmuir isotherm, especially in the linearized form proposed by Langmuir, gave results that were the closest to those obtained from measurements. Linearization according to the Lineweaver–Burke suggestion ranked second. Only in some series did the calculations made according to the Scatchard and Eadie–Hofstee linearization show a good fit and a small error, but these results are too scanty to recommend use.
- In assessing the degree of fit between the trend line and the survey results, it is insufficient to rely on the R2 coefficient of determination since even a few outliers from the main set cause a sharp drop in the R2 value. Additional error analyses, such as ESRQ, HYBRD, ARE, Fisher’s test, and Chi-square test (χ2), are instrumental in better illustrating the degree of fit, since they are performed on the model in its general form. At the same time, R2; refers to the linearized function.
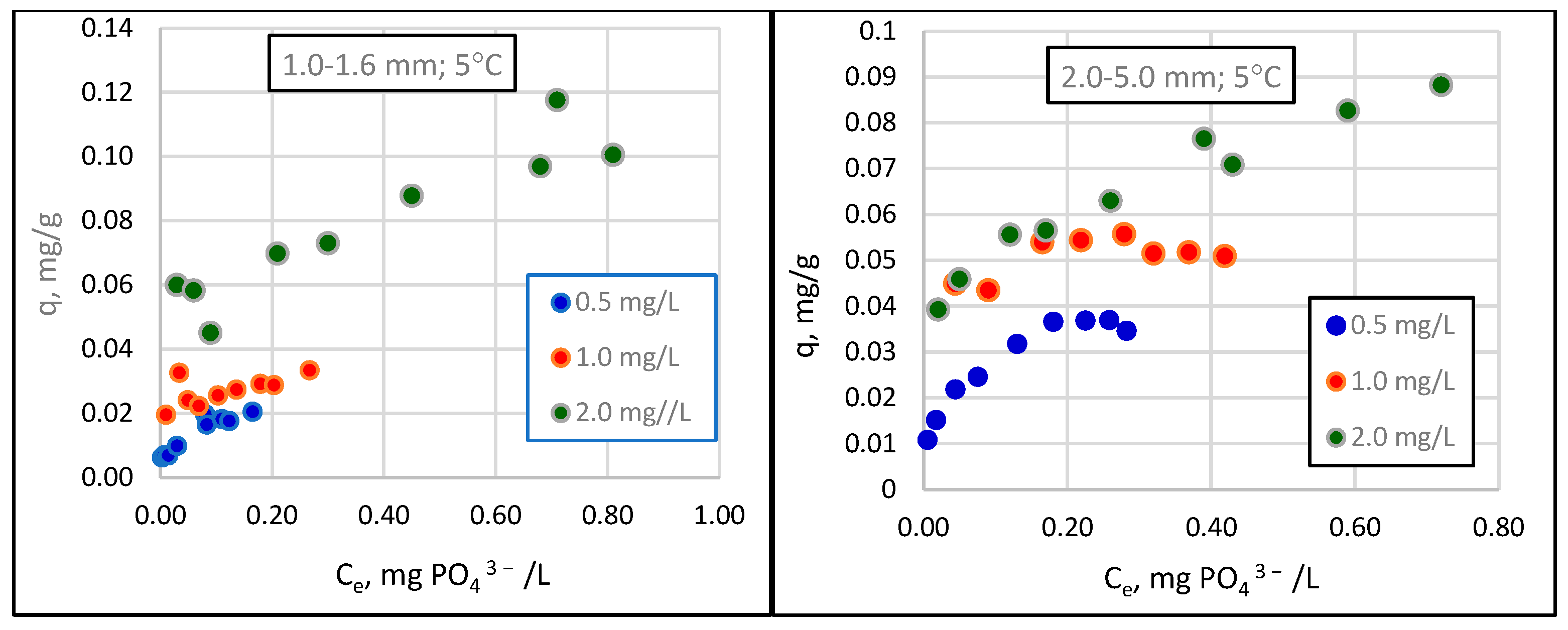
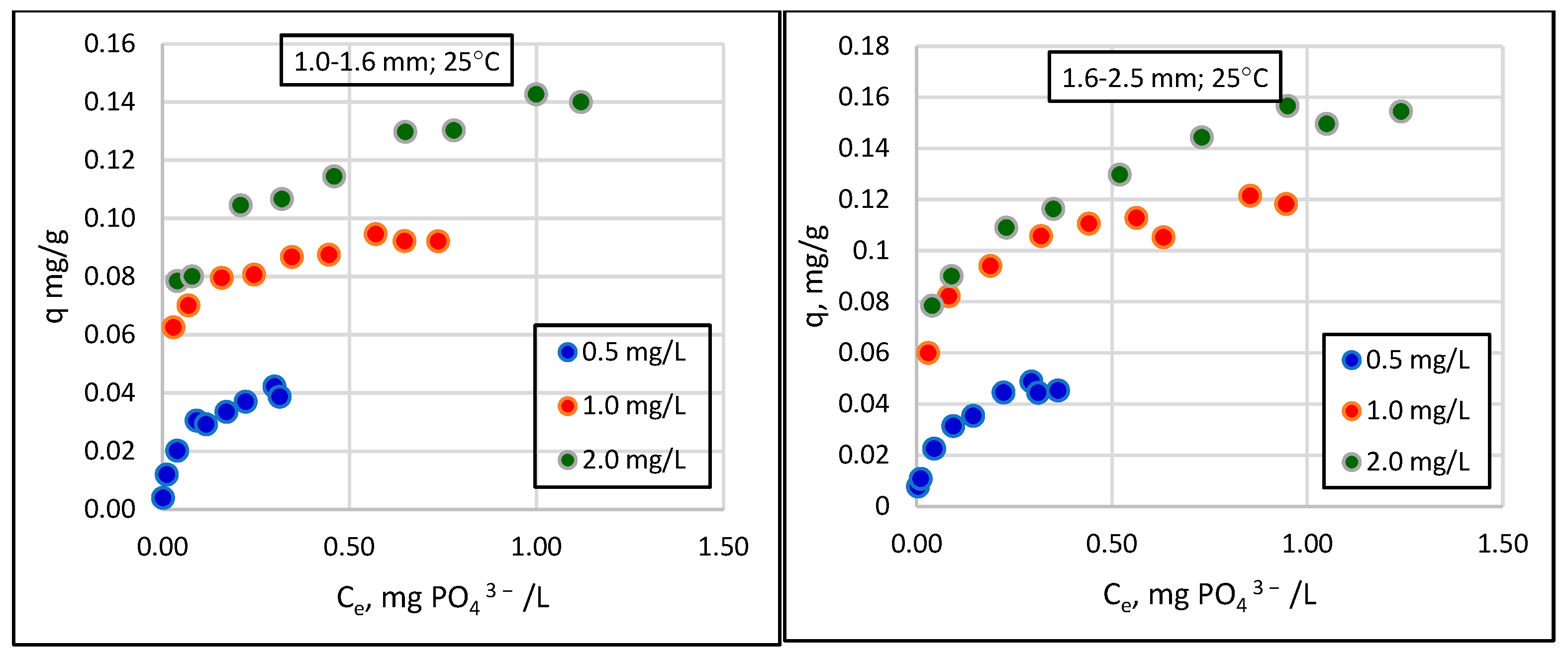
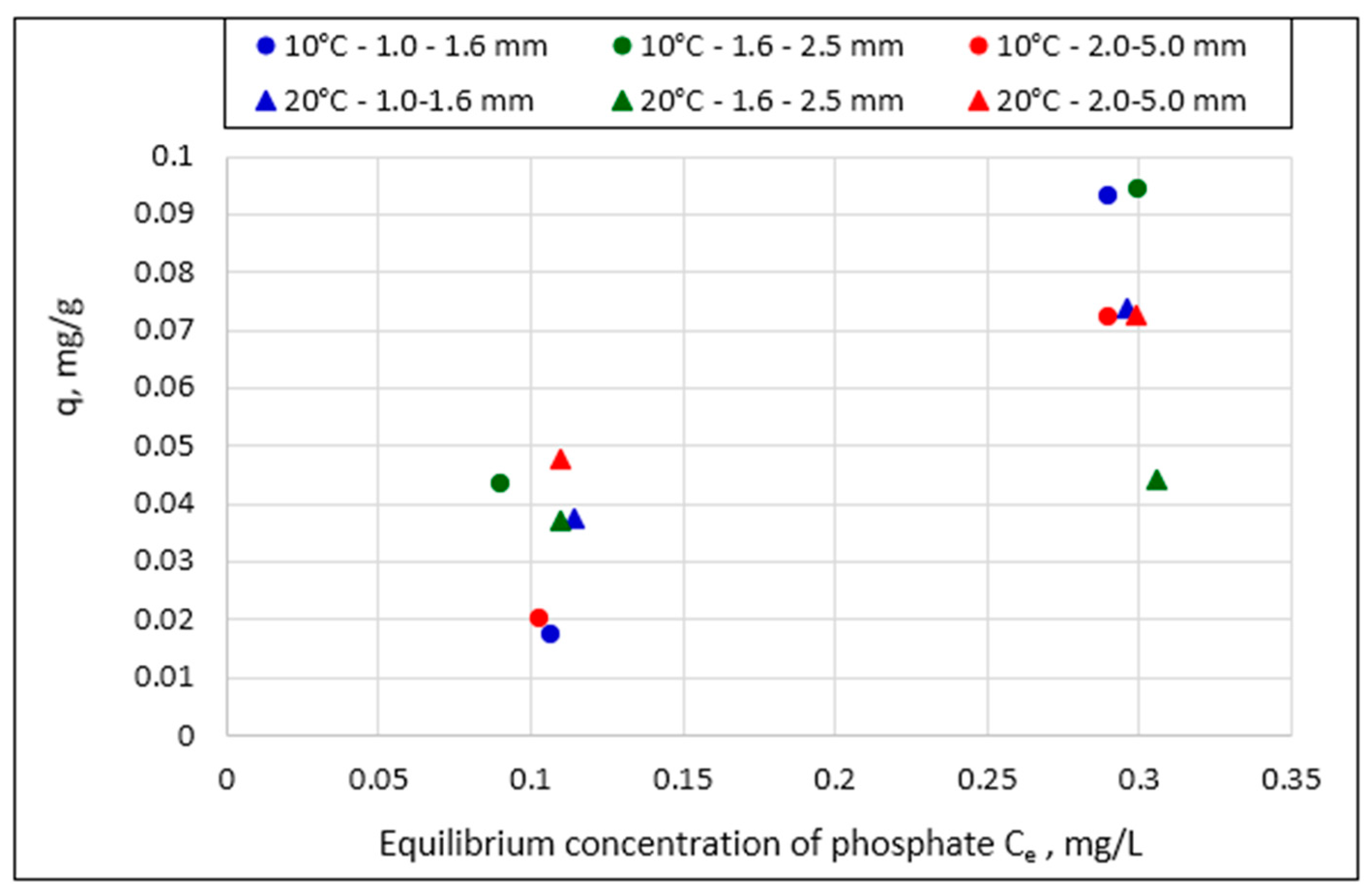
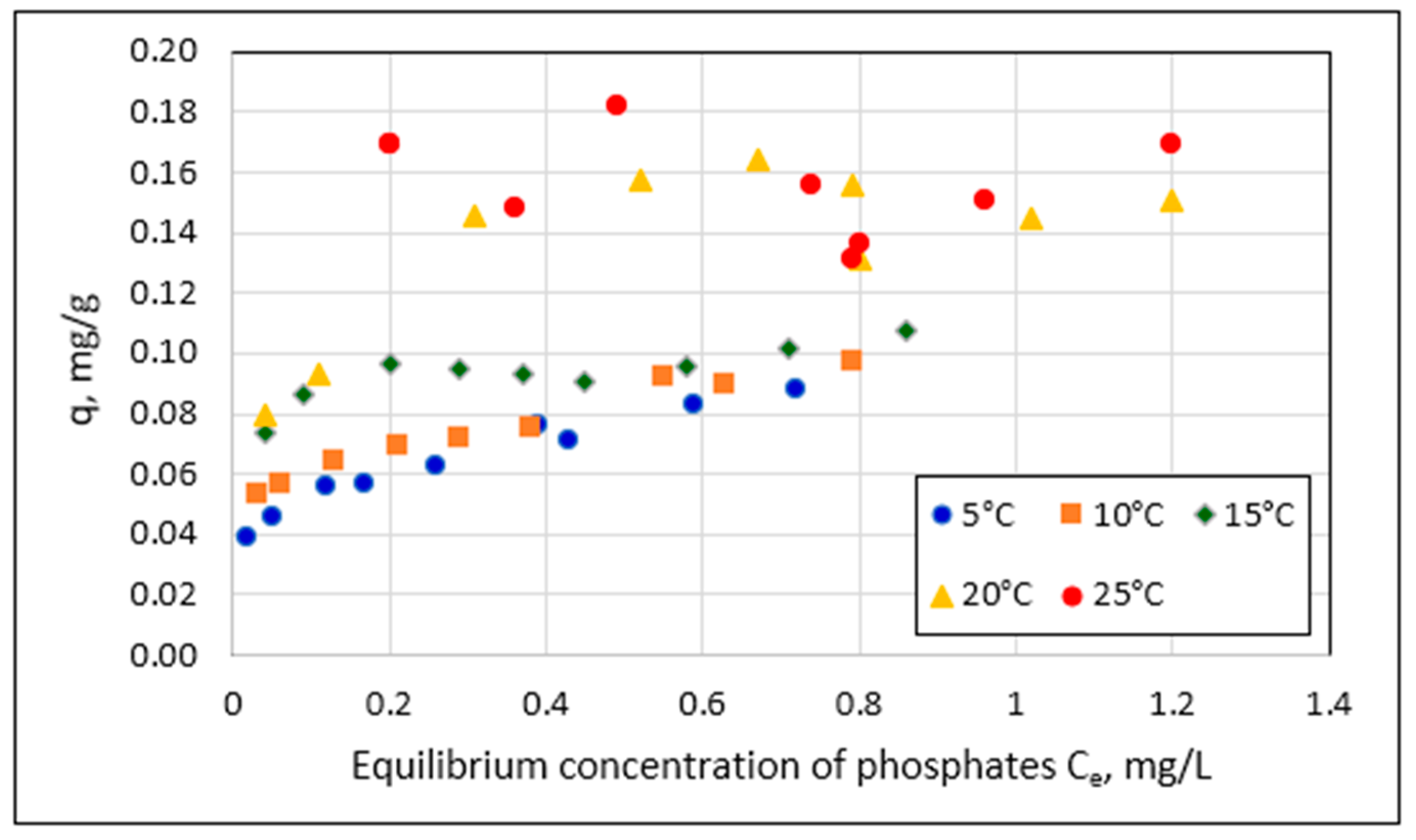
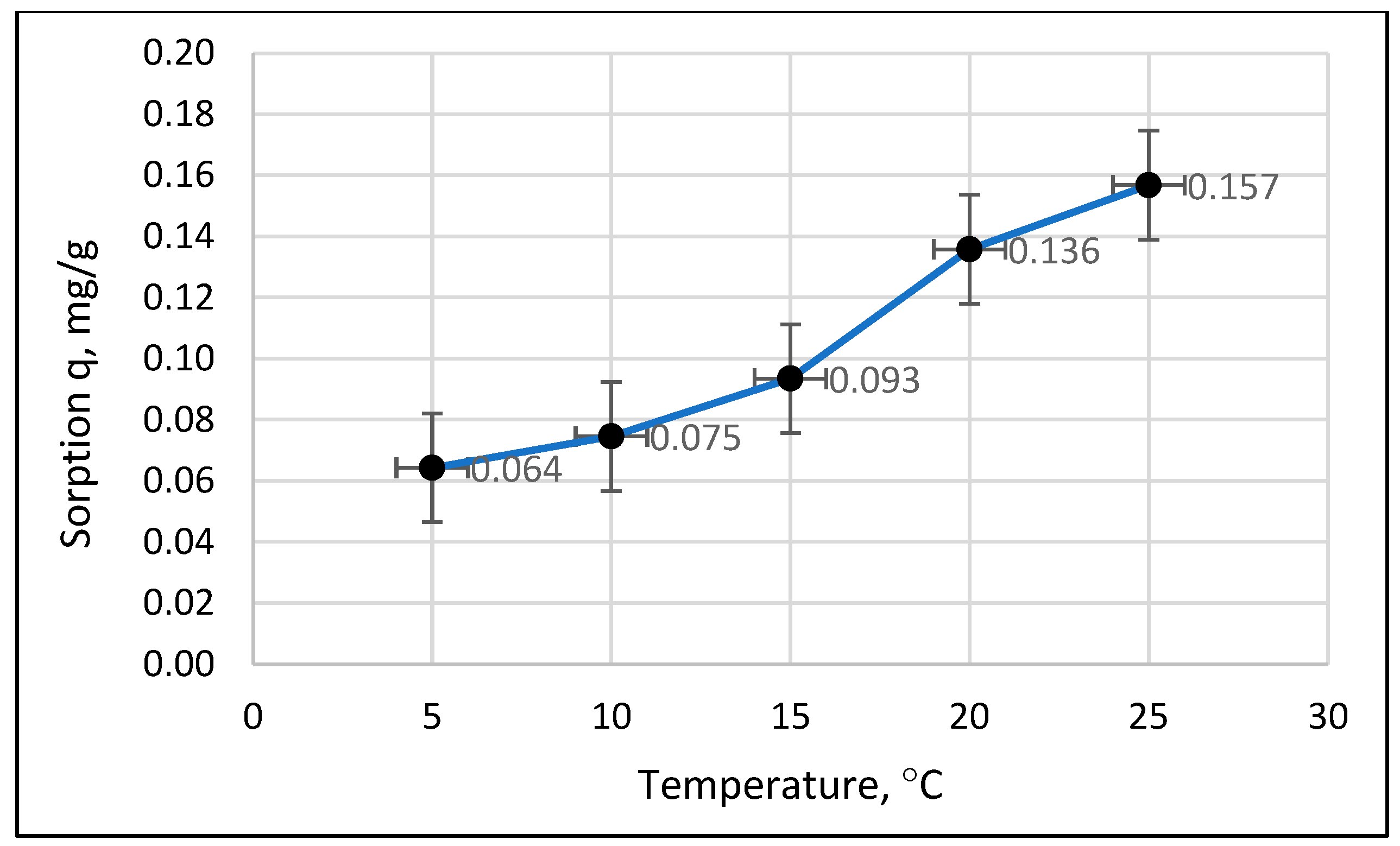
- The relationship between the amount of phosphate sorption and the granularity of the material analyzed was not demonstrated. The mass of adsorbed phosphates and the efficiency of their removal from the solution increased with the increase in the initial concentration of phosphates in the solution. The effect of temperature on phosphate removal efficiency was statistically significant.
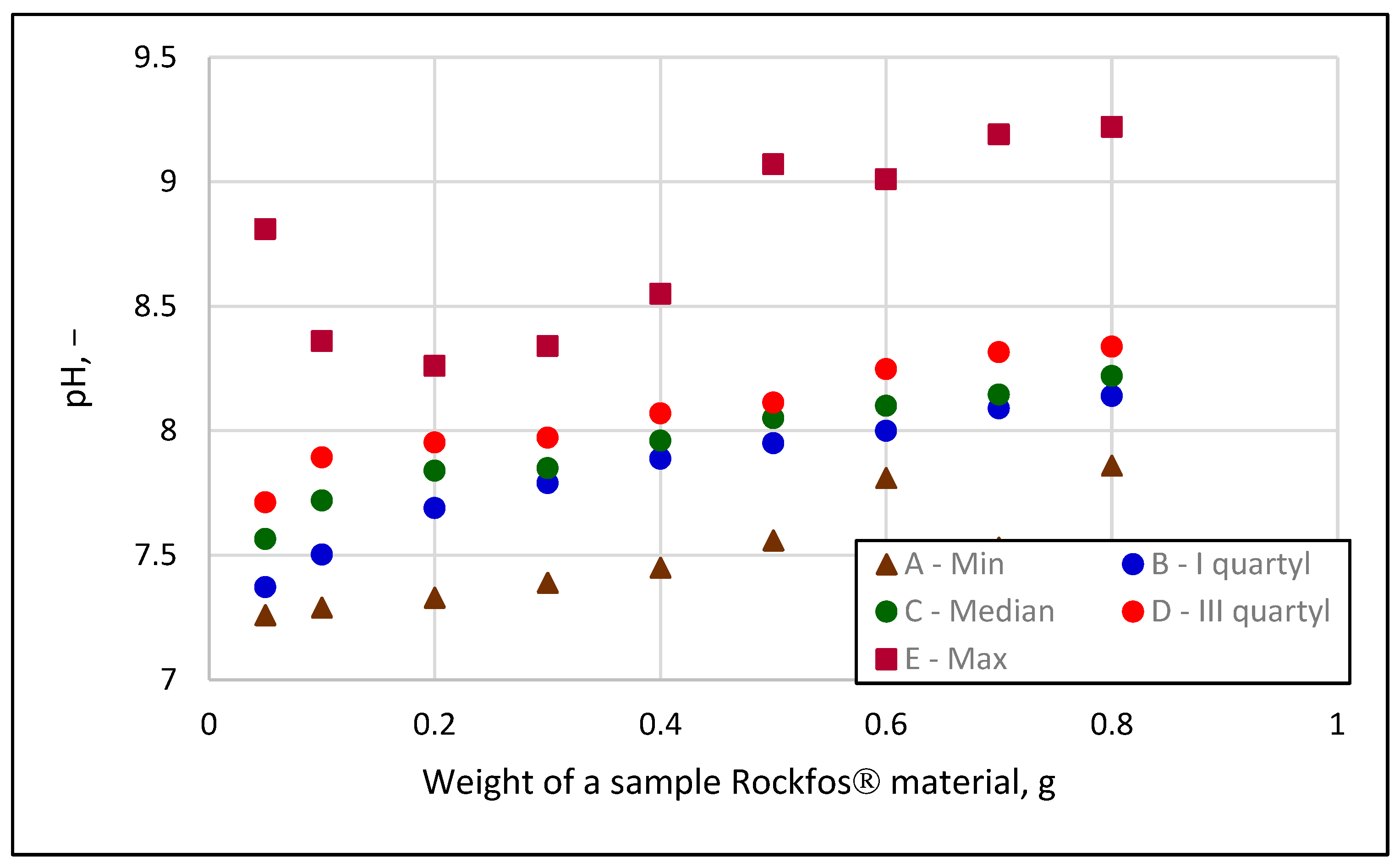
- The near-zero values of the separation coefficient RL prove that the sorption of phosphates on Rockfos® material is irreversible (chemisorption). That is, phosphates react with the active parts of the material. This conclusion was confirmed by qualitative changes in the solution, including increasing pH, hardness, and calcium concentrations with increasing sorbent weight. The pH can even exceed 10 at large weightings, and at that point, phosphate removal relies more on precipitation than adsorption.
- The tested material can be used in practice in wastewater treatment, and maximum sorption capacities of 0.6–0.8 mgPO43−/g of sorbent in the winter and 0.8–1.0 mgPO43−/g in the summer can be assumed for designing the mass and adequate time of filter beds.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feng, W.; Wang, T.; Zhu, Y.; Sun, F.; Giesy, J.P.; Wu, F. Chemical composition, sources, and ecological effect of organic phosphorus in water ecosystems: A review. Carbon Res. 2023, 2, 12. [Google Scholar] [CrossRef]
- Zahed, M.A.; Salehi, S.; Tabari, Y.; Farraji, H.; Ataei-Kachooei, S.; Zinatizadeh, A.A.; Kamali, N.; Mahjouri, M. Phosphorus removal and recovery: State of the science and challenges. Environ. Sci. Pollut. Res. 2022, 29, 58561–58589. [Google Scholar] [CrossRef] [PubMed]
- Feng, W.; Yang, F.; Zhang, C.; Liu, J.; Song, F.; Chen, H.; Zhu, Y.; Liu, S.; Giesy, J.P. Composition characterization and biotransformation of dissolved, particulate and algae organic phosphorus in eutrophic lakes. Environ. Pollut. 2020, 265 Pt B, 114838. [Google Scholar] [CrossRef]
- Online Legal Acts System Home Page. Available online: https://isap.sejm.gov.pl/isap.nsf/download.xsp/WDU20190001311/O/D20191311.pdf (accessed on 24 August 2024). (In Polish)
- Przywara, L. Warunki i Możliwości Usuwania Fosforanów i Fosforu Ogólnego ze Ścieków Przemysłowych. Ph.D. Thesis, Politechnika Krakowska, Kraków, Poland, 2006. [Google Scholar]
- Cordell, D.; White, S. Life’s bottleneck: Sustaining the world’s phosphorus for a food secure future. Annu. Rev. Environ. Resour. 2014, 39, 161–188. [Google Scholar] [CrossRef]
- Adelagun, R.O.A. Technological options for phosphate removal and recovery from aqua system: A review. Chem. Sci. Rev. Lett. 2016, 5, 19–34. [Google Scholar]
- Kasprzyk, M.; Gajewska, M. Phosphorus removal by application of natural and semi-natural materials for possible recovery according to assumptions of circular economy and closed circuit of P. Sci. Total Environ. 2019, 650, 249–256. [Google Scholar] [CrossRef]
- Chu, W.; Shi, Y.; Zhang, L. Recovery of phosphorus in wastewater in the form of polyphosphates: A review. Processes 2022, 10, 144. [Google Scholar] [CrossRef]
- Jusino-Maldonado, M.; Rianço-Silva, R.; Mondal, J.A.; Pasek, M.; Laneuville, M.; Cleaves, H.J. A global network model of abiotic phosphorus cycling on Earth through time. Sci. Rep. 2022, 12, 9348. [Google Scholar] [CrossRef]
- Zawadzka, B.; Siwiec, T.; Marzec, M.; Jóźwiakowski, K.; Listosz, A. Meandering Flow Filter for Phosphorus Removal as a Component of Small Wastewater Treatment Plants—A Case Study. Water 2023, 15, 2703. [Google Scholar] [CrossRef]
- Cucarella, V.; Zaleski, T.; Mazurek, R. Phosphorus sorption capacity of different types of opoka. Ann. Wars. Univ. Life Sci.–SGGW. Land Reclam. 2007, 38, 11–18. [Google Scholar] [CrossRef]
- Vohla, C.; Kõiv, M.; Bavor, H.J.; Chazarenc, F.; Mander, Ü. Filter materials for phosphorus removal from wastewater in treatment wetlands—A review. Ecol. Eng. 2011, 37, 70–89. [Google Scholar] [CrossRef]
- Strawn, D.G. Sorption mechanisms of chemicals in soils. Soil Syst. 2021, 5, 13. [Google Scholar] [CrossRef]
- Bonilla-Petriciolet, A.; Mendoza-Castillo, D.I.; Reynel-Ávila, H.E. Adsorption Processes for Water Treatment and Purification; Springer: Berlin, Germany, 2017; Volume 256. [Google Scholar]
- Osman, A.I.; El-Monaem, E.M.A.; Elgarahy, A.M.; Aniagor, C.O.; Hosny, M.; Farghali, M.; Rashad, E.; Ejimofor, M.I.; López-Maldonado, E.A.; Ihara, I.; et al. Methods to prepare biosorbents and magnetic sorbents for water treatment: A review. Environ. Chem. Lett. 2023, 21, 2337–2398. [Google Scholar] [CrossRef]
- Tran, H.N. Adsorption Technology for Water and Wastewater Treatments. Water 2023, 15, 2857. [Google Scholar] [CrossRef]
- Agarwala, R.; Mulky, L. Adsorption of dyes from wastewater: A comprehensive review. ChemBioEng Rev. 2023, 10, 326–335. [Google Scholar] [CrossRef]
- Sukmana, H.; Bellahsen, N.; Pantoja, F.; Hodur, C. Adsorption and coagulation in wastewater treatment–Review. Prog. Agric. Eng. Sci. 2021, 17, 49–68. [Google Scholar] [CrossRef]
- Sales, F.R.P.; Serra, R.B.G.; de Figueirêdo, G.J.A.; da Hora, P.H.A.; de Sousa, A.C. Wastewater treatment using adsorption process in column for agricultural purposes. Rev. Ambiente Água 2019, 14, e2178. [Google Scholar] [CrossRef]
- Yousef, R.; Qiblawey, H.; El-Naas, M.H. Adsorption as a process for produced water treatment: A review. Processes 2020, 8, 1657. [Google Scholar] [CrossRef]
- Ho, Y.-S.; Chiu, W.-T.; Hsu, C.-S.; Huang, C.-T. Sorption of lead ions from aqueous solution using tree fern as a sorbent. Hydrometallurgy 2004, 73, 55–61. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J.; Zhao, R.; Li, Y.; Li, C.; Zhang, C. Adsorption of Pb (II) on activated carbon prepared from Polygonum orientale Linn.: Kinetics, isotherms, pH, and ionic strength studies. Bioresour. Technol. 2010, 101, 5808–5814. [Google Scholar] [CrossRef]
- Reczek, L.; Michel, M.M.; Siwiec, T.; Zukowska, E. Sorption of Pb (II) ions onto some filter materials used in water treatment technology. Przem. Chem. 2014, 93, 1978–1982. [Google Scholar]
- Soliman, A.M.; Elwy, H.M.; Thiemann, T.; Majedi, Y.; Labata, F.T.; Al-Rawashdeh, N.A. Removal of Pb (II) ions from aqueous solutions by sulphuric acid-treated palm tree leaves. J. Taiwan Inst. Chem. Eng. 2016, 58, 264–273. [Google Scholar] [CrossRef]
- Gupta, S.S.; Bhattacharyya, K.G. Adsorption of Ni (II) on clays. J. Colloid Interface Sci. 2006, 295, 21–32. [Google Scholar] [CrossRef] [PubMed]
- Ghodbane, I.; Nouri, L.; Hamdaoui, O.; Chiha, M. Kinetic and equilibrium study for the sorption of cadmium (II) ions from aqueous phase by eucalyptus bark. J. Hazard. Mater. 2008, 152, 148–158. [Google Scholar] [CrossRef] [PubMed]
- Kalam, S.; Abu-Khamsin, S.A.; Kamal, M.S.; Patil, S. Surfactant adsorption isotherms: A review. ACS Omega 2021, 6, 32342–32348. [Google Scholar] [CrossRef]
- Kasprzyk, M.; Węgler, J.; Gajewska, M. Analysis of efficiency of phosphates sorption by different granulation of selected reactive material. In E3S Web of Conferences; EDP Sciences: Les Ulis, France, 2018. [Google Scholar]
- Jamali, S.; Mofarahi, M.; Rodrigues, A.E. Investigation of a novel combination of adsorbents for hydrogen purification using Cu-BTC and conventional adsorbents in pressure swing adsorption. Adsorption 2018, 24, 481–498. [Google Scholar] [CrossRef]
- Elboughdiri, N.; Ferkous, H.; Rouibah, K.; Boublia, A.; Delimi, A.; Yadav, K.K.; Erto, A.; Ghernaout, D.; Salih, A.A.M.; Benaissa, M.; et al. Comprehensive investigation of Cu2+ adsorption from wastewater using olive-waste-derived adsorbents: Experimental and molecular insights. Int. J. Mol. Sci. 2024, 25, 1028. [Google Scholar] [CrossRef]
- Khamseh, A.A.G.; Ghorbanian, S.A.; Amini, Y.; Shadman, M.M. Investigation of kinetic, isotherm and adsorption efficacy of thorium by orange peel immobilized on calcium alginate. Sci. Rep. 2023, 13, 8393. [Google Scholar] [CrossRef]
- Okano, K.; Uemoto, M.; Kagami, J.; Miura, K.; Aketo, T.; Toda, M.; Honda, K.; Ohtake, H. Novel technique for phosphorus recovery from aqueous solutions using amorphous calcium silicate hydrates (A-CSHs). Water Res. 2013, 47, 2251–2259. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, X.; Zhao, J. Phosphate recovery from wastewater using calcium silicate hydrate (CSH): Sonochemical synthesis and properties. Environ. Sci. Water Res. Technol. 2019, 5, 131–139. [Google Scholar] [CrossRef]
- Bus, A.; Karczmarczyk, A. BazaAgro. Available online: https://agro.icm.edu.pl/agro/element/bwmeta1.element.agro-c4b86c9a-65d4-4c84-9aa2-0f1f14492abd/c/7.pdf (accessed on 24 August 2024). (In Polish).
- Kietlińska, A.; Renman, G. An evaluation of reactive filter media for treating landfill leachate. Chemosphere 2005, 61, 933–940. [Google Scholar] [CrossRef] [PubMed]
- Hylander, L.D.; Kietlińska, A.; Renman, G.; Simán, G. Phosphorus retention in filter materials for wastewater treatment and its subsequent suitability for plant production. Bioresour. Technol. 2006, 97, 914–921. [Google Scholar] [CrossRef]
- Available online: http://www.ceramika-kufel.pl/rockfos/ (accessed on 24 August 2024).
- Jóźwiakowski, K.; Bugajski, P.; Kurek, K.; Cáceres, R.; Siwiec, T.; Jucherski, A.; Czekała, W.; Kozłowski, K. Technological reliability of pollutant removal in different seasons in one-stage constructed wetland system with horizontal flow operating in the moderate climate. Sep. Purif. Technol. 2020, 238, 116439. [Google Scholar] [CrossRef]
- Karczmarczyk, A.; Renman, G. Phosphorus accumulation pattern in a subsurface constructed wetland treating residential wastewater. Water 2011, 3, 146–156. [Google Scholar] [CrossRef]
- Jóźwiakowski, K.; Bugajski, P.; Kurek, K.; Carvalho, M.d.F.N.d.; Almeida, M.A.A.; Siwiec, T.; Borowski, G.; Czekała, W.; Dach, J.; Gajewska, M. The efficiency and technological reliability of biogenic compounds removal during long-term operation of a one-stage subsurface horizontal flow constructed wetland. Sep. Purif. Technol. 2018, 202, 216–226. [Google Scholar] [CrossRef]
- Jóźwiakowski, K.; Gajewska, M.; Pytka, A.; Marzec, M.; Gizińska-Górna, M.; Jucherski, A.; Walczowski, A.; Nastawny, M.; Kamińska, A.; Baran, S. Influence of the particle size of carbonate-siliceous rock on the efficiency of phosphorous removal from domestic wastewater. Ecol. Eng. 2017, 98, 290–296. [Google Scholar] [CrossRef]
- Jucherski, A.; Walczowski, A.; Bugajski, P.; Jóźwiakowski, K.; Rodziewicz, J.; Janczukowicz, W.; Wu, S.; Kasprzyk, M.; Gajewska, M.; Mielcarek, A. Long-term operating conditions for different sorption materials to capture phosphate from domestic wastewater. Sustain. Mater. Technol. 2022, 31, e00385. [Google Scholar] [CrossRef]
- Pytka-Woszczyło, A.; Różańska-Boczula, M.; Gizińska-Górna, M.; Marzec, M.; Listosz, A.; Jóźwiakowski, K. Efficiency of filters filled with rockfos for phosphorus removal from domestic sewage. Adv. Sci. Technol. Res. J. 2022, 16, 176–188. [Google Scholar] [CrossRef]
- Cucarella, V.; Renman, G. Phosphorus sorption capacity of filter materials used for on-site wastewater treatment determined in batch experiments–a comparative study. J. Environ. Qual. 2009, 38, 381–392. [Google Scholar] [CrossRef]
- Li, X.; Liang, H.; Mo, Y.; Wei, Y. Preparation and phosphorus removal performance of Zr–La–Fe ternary composite adsorbent embedded with sodium alginate. Processes 2022, 10, 1761. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B.H. Insights into the modeling of adsorption isotherm systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Kasprzyk, M. Treatment Wetland effluent quality improvement by usage sorbents of various origin. In E3S Web of Conferences; EDP Sciences: Les Ulis, France, 2019. [Google Scholar]
- Tran, H.N.; You, S.-J.; Chao, H.-P. Thermodynamic parameters of cadmium adsorption onto orange peel calculated from various methods: A comparison study. J. Environ. Chem. Eng. 2016, 4, 2671–2682. [Google Scholar] [CrossRef]
- PN-EN ISO 6878; Water Quality—Determination of Phosphorus—Spectrometric Method with Ammonium Molybdate. Polish Committee for Standardization: Warszawa, Poland, 2006.
- PN-EN ISO 10523; Water Quality—Determination of pH. Polish Committee for Standardization: Warszawa, Poland, 2012.
- Ayawei, N.; Ebelegi, A.; Donbebe, W. Modelling and Interpretation of Adsorption Isotherms. Hindawi J. Chem. 2017, 2017, 3039817. [Google Scholar] [CrossRef]
- Saoudi Hassani, E.M.; Azzouni, D.; Alanazi, M.M.; Mehdaoui, I.; Mahmoud, R.; Kabra, A.; Taleb, A.; Taleb, M.; Rais, Z. Innovative plant-derived biomaterials for sustainable and effective removal of Cationic and Anionic dyes: Kinetic and thermodynamic study. Processes 2024, 12, 922. [Google Scholar] [CrossRef]
- Chutkowski, M.; Petrus, R.; Warchol, J.; Koszelnik, P. Sorption equilibrium in processes of metal ion removal from environment. Statistical verification of mathematical models. Przem. Chem. 2008, 87, 436–438. [Google Scholar]
- Müller, M. Generalized Linear Models. In Handbook of Computational Statistics: Concepts and Methods; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Karaca, S.; Gurses, A.; Ejder, M.; Acikyildiz, M. Kinetic modeling of liquid-phase adsorption of phosphate on dolomite. J. Colloid Interface Sci. 2004, 277, 257–263. [Google Scholar] [CrossRef] [PubMed]
- Ayoub, G.M.; Kalinian, H. Removal of low-concentration phosphorus using a fluidized raw dolomite bed. Water Environ. Res. 2006, 78, 353–361. [Google Scholar] [CrossRef]
- Kpannieu, D.E.; Ruby, C.; Coulibaly, L.; Abdelmoula, M.; Mallet, M. Removal of phosphate by ivory coast shale in a homogeneous reactor and under hydrodynamic conditions: Influence of soluble species. Clays Clay Miner. 2018, 66, 500–514. [Google Scholar] [CrossRef]
- Cyrus, J.S.; Reddy, G.B. Sorption and Desorption of Nitrogen and Phosphorus by Zeolite and Shale. In Proceedings of the 2007 National Conference on Environmental Science and Technology; Nzewi, E., Reddy, G., Luster-Teasley, S., Kabadi, V., Chang, S.-Y., Uzochukwu, G., Eds.; Springer Science+Buisness Media, LLC: New York, NY, USA, 2009; pp. 353–364. [Google Scholar]
- Cyrus, J.S.; Reddy, G.B. Sorption and desorption of phosphorus by shale: Batch and column studies. Water Sci. Technol. 2010, 61, 599–606. [Google Scholar] [CrossRef]
- Brogowski, Z.; Renman, G. Characterization of opoka as a basis for its use in wastewater treatment. Pol. J. Environ. Stud. 2004, 13, 15–20. [Google Scholar]
- Lomovskiy, I.; Kiryanov, A.; Skripkina, T. The Effect of Reverse Sorption on an Extraction Kinetics Melanin Case. Processes 2023, 11, 3192. [Google Scholar] [CrossRef]
- Cao, S.; Yin, W.; Yang, B.; Zhu, Z.; Sun, H.; Sheng, Q.; Chen, K. Insights into the influence of temperature on the adsorption behavior of sodium oleate and its response to flotation of quartz. Int. J. Min. Sci. Technol. 2022, 32, 399–409. [Google Scholar] [CrossRef]
- Marecka, A. Influence of Temperature on Sorption of Carbon Dioxide in Coals. Inżynieria Miner. 2014, 15, 143–148. [Google Scholar]
- Zhang, L.; Aziz, N.; Ren, T.X.; Wang, Z. Influence of temperature on coal sorption characteristics and the theory of coal surface free energy. Procedia Eng. 2011, 26, 1430–1439. [Google Scholar] [CrossRef]
- Chen, D.; Zheng, W.; Wang, T.; Liu, F.; Cheng, T.; Chen, H.; Miao, T. Influence of temperature on the adsorption and diffusion of heavy oil in quartz nanopore: A molecular dynamics study. Energies 2022, 15, 5870. [Google Scholar] [CrossRef]
- Kasprzyk, M.; Czerwionka, K.; Gajewska, M. Waste materials assessment for phosphorus adsorption toward sustainable application in circular economy. Resour. Conserv. Recycl. 2021, 168, 105335. [Google Scholar] [CrossRef]
- Rayshouni, H.; Wazne, M. Effects of Calcium on the Removal of Ammonium from Aged Landfill Leachate by Struvite Precipitation. Water 2022, 14, 1933. [Google Scholar] [CrossRef]
- Soares, A.; Czajkowska, J.; Colprim, J.; Gali, A.; Johansson, S.; Masic, A.; March, A.; McMeod, A.; Nenov, V. Nutrients Recovery from Wastewater Streams; IWA Publishing: London, UK, 2017; Volume 2017. [Google Scholar]
- Westholm, L.J. The use of blast furnace slag for removal of phosphorus from wastewater in Sweden—A review. Water 2010, 2, 826–837. [Google Scholar] [CrossRef]
- Ehiomogue, P.; Ahuchaogu, I.I.; Ahaneku, I.E. REVIEW OF ADSORPTION ISOTHERMS MODELS. Acta Tech. Corviniensis-Bull. Eng. 2021, 14.4. [Google Scholar]


| Lp. | General Form | Linear Form | References |
|---|---|---|---|
| 1 | (3) | [47,49] | |
| 2 | Linearization according to Langmuir | (4) | [47,49] |
| 3 | Linearization according to Lineweaver–Burke | (5) | [47,49] |
| 4 | Linearization according to Scatchard | (6) | [47,49] |
| 5 | Linearization according to Eadie–Hofstee | (7) | [47,49] |
| T, °C | KL [dm3 /g]. | qmax [mg/g]. | RL - | R2 - | KL [dm3 /g]. | qmax [mg/g]. | RL - | R2 - | KL [dm3 /g]. | qmax [mg/g]. | RL - | R2 - | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| C0 | 0.5 mg/dm2 | 1.0 mg/dm3 | 2.0 mg/dm3 | ||||||||||
| LI—Langmuir isotherm in Langmuir linearization [49] | |||||||||||||
| 5 °C | 1.0 ÷ 1.60 | 45.74 | 0.0221 | 0.04 | 0.967 | 54.92 | 0.0330 | 0.02 | 0.975 | 9.67 | 0.1157 | 0.05 | 0.959 |
| 1.6 ÷ 2.5 | 81.50 | 0.0392 | 0.02 | 0.987 | 20.88 | 0.0399 | 0.05 | 0.931 | 6.23 | 0.0664 | 0.07 | 0.926 | |
| 2.0 ÷ 5.0 | 35.01 | 0.0400 | 0.05 | 0.987 | 185.17 | 0.0530 | 0.01 | 0.995 | 12.99 | 0.0921 | 0.04 | 0.978 | |
| 10 °C | 1.0 ÷ 1.60 | 70.17 | 0.0208 | 0.03 | 0.987 | 67.98 | 0.0313 | 0.01 | 0.955 | 17.91 | 0.1304 | 0.03 | 0.980 |
| 1.6 ÷ 2.5 | 83.40 | 0.0340 | 0.02 | 0.971 | 13.64 | 0.0617 | 0.07 | 0.904 | 23.52 | 0.1107 | 0.02 | 0.981 | |
| 2.0 ÷ 5.0 | 37.85 | 0.0314 | 0.05 | 0.957 | 60.14 | 0.0578 | 0.02 | 0.995 | 13.38 | 0.1016 | 0.04 | 0.982 | |
| 15 °C | 1.0 ÷ 1.60 | 28.95 | 0.0262 | 0.06 | 0.939 | 19.73 | 0.0722 | 0.05 | 0.964 | 15.48 | 0.1312 | 0.03 | 0.996 |
| 1.6 ÷ 2.5 | 30.54 | 0.0246 | 0.06 | 0.953 | 66.17 | 0.0551 | 0.01 | 0.993 | 17.33 | 0.1099 | 0.03 | 0.988 | |
| 2.0 ÷ 5.0 | 88.00 | 0.0258 | 0.02 | 0.979 | 42.42 | 0.0718 | 0.02 | 0.995 | 28.26 | 0.1066 | 0.02 | 0.991 | |
| 20 °C | 1.0 ÷ 1.60 | 45.60 | 0.0436 | 0.04 | 0.956 | 111.32 | 0.0687 | 0.01 | 0.976 | 16.93 | 0.1179 | 0.03 | 0.995 |
| 1.6 ÷ 2.5 | 24.51 | 0.0506 | 0.08 | 0.981 | 37.04 | 0.1309 | 0.03 | 0.952 | 11.87 | 0.1535 | 0.04 | 0.995 | |
| 2.0 ÷ 5.0 | 27.65 | 0.0353 | 0.07 | 0.959 | 6.65 | 0.1327 | 0.13 | 0.947 | 32.30 | 0.1535 | 0.02 | 0.982 | |
| 25 °C | 1.0 ÷ 1.60 | 24.66 | 0.0443 | 0.08 | 0.984 | 32.87 | 0.0961 | 0.03 | 0.998 | 11.39 | 0.1491 | 0.04 | 0.992 |
| 1.6 ÷ 2.5 | 21.44 | 0.0520 | 0.09 | 0.985 | 19.79 | 0.1234 | 0.05 | 0.994 | 10.26 | 0.1650 | 0.05 | 0.993 | |
| 2.0 ÷ 5.0 | 21.23 | 0.0369 | 0.09 | 0.917 | 20.38 | 0.1246 | 0.05 | 0.970 | 13.25 | 0.1335 | 0.04 | 0.963 | |
| LII—Langmuir isotherm in Lineweaver–Burke linearization [49] | |||||||||||||
| 5 °C | 1.0 ÷ 1.60 | 192.08 | 0.0150 | 0.01 | 0.662 | 218.69 | 0.0289 | 0.00 | 0.496 | 48.43 | 0.0850 | 0.01 | 0.360 |
| 1.6 ÷ 2.5 | 63.98 | 0.0491 | 0.03 | 0.908 | −26.63 | 0.0427 | −0.04 | 0.577 | −5.99 | 0.0646 | −0.09 | 0.697 | |
| 2.0 ÷ 5.0 | 94.53 | 0.0321 | 0.02 | 0.889 | 130.72 | 0.0533 | 0.01 | 0.779 | 51.95 | 0.0726 | 0.01 | 0.802 | |
| 10 °C | 1.0 ÷ 1.60 | 184.56 | 0.0170 | 0.01 | 0.571 | 230.73 | 0.0286 | 0.00 | 0.346 | 25.33 | 0.1237 | 0.02 | 0.967 |
| 1.6 ÷ 2.5 | 108.50 | 0.0412 | 0.02 | 0.726 | −32.41 | 0.0746 | −0.03 | 0.387 | 70.94 | 0.0994 | 0.01 | 0.096 | |
| 2.0 ÷ 5.0 | 348.48 | 0.0238 | 0.01 | 0.268 | 134.38 | 0.0546 | 0.01 | 0.795 | 50.19 | 0.0832 | 0.01 | 0.735 | |
| 15 °C | 1.0 ÷ 1.60 | 166.47 | 0.0162 | 0.01 | 0.815 | 92.58 | 0.0601 | 0.01 | 0.739 | 29.04 | 0.1193 | 0.02 | 0.903 |
| 1.6 ÷ 2.5 | 143.34 | 0.0159 | 0.01 | 0.688 | 228.17 | 0.0518 | 0.00 | 0.588 | 46.92 | 0.0988 | 0.01 | 0.888 | |
| 2.0 ÷ 5.0 | 177.66 | 0.0229 | 0.01 | 0.622 | 92.05 | 0.0676 | 0.01 | 0.679 | 69.61 | 0.1005 | 0.01 | 0.854 | |
| 20 °C | 1.0 ÷ 1.60 | 27.88 | 0.0475 | 0.07 | 0.987 | 31.93 | 0.0835 | 0.03 | 0.967 | 29.93 | 0.1079 | 0.02 | 0.961 |
| 1.6 ÷ 2.5 | 46.03 | 0.0410 | 0.04 | 0.824 | 15.21 | 0.1427 | 0.06 | 0.998 | 17.06 | 0.1459 | 0.03 | 0.996 | |
| 2.0 ÷ 5.0 | 141.66 | 0.0253 | 0.01 | 0.632 | 36.33 | 0.0869 | 0.03 | 0.622 | 24.65 | 0.1543 | 0.02 | 0.856 | |
| 25 °C | 1.0 ÷ 1.60 | 63.58 | 0.0337 | 0.03 | 0.984 | 67.71 | 0.0905 | 0.01 | 0.998 | 32.38 | 0.1287 | 0.02 | 0.992 |
| 1.6 ÷ 2.5 | 57.85 | 0.0381 | 0.03 | 0.922 | 34.64 | 0.1159 | 0.03 | 0.968 | 26.99 | 0.1428 | 0.02 | 0.957 | |
| 2.0 ÷ 5.0 | 109.60 | 0.0239 | 0.02 | 0.113 | 50.61 | 0.1145 | 0.02 | 0.476 | 11.51 | 0.1321 | 0.04 | 0.517 | |
| LIII—Langmuir isotherm in Scatchard linearization [49] | |||||||||||||
| 5 °C | 1.0 ÷ 1.60 | 77.54 | 0.0205 | 0.03 | 0.523 | 64.63 | 0.0350 | 0.02 | 0.243 | 14.77 | 0.1139 | 0.03 | 0.317 |
| 1.6 ÷ 2.5 | 30.22 | 0.0595 | 0.06 | 0.904 | −19.26 | 0.0375 | −0.05 | 0.303 | −5.47 | 0.0610 | −0.10 | 0.960 | |
| 2.0 ÷ 5.0 | 56.58 | 0.0371 | 0.03 | 0.730 | 90.73 | 0.0552 | 0.01 | 0.707 | 28.93 | 0.0817 | 0.02 | 0.641 | |
| 10 °C | 1.0 ÷ 1.60 | 94.57 | 0.0205 | 0.02 | 0.527 | 28.65 | 0.0449 | 0.03 | 0.070 | 20.28 | 0.1301 | 0.02 | 0.823 |
| 1.6 ÷ 2.5 | 47.07 | 0.0481 | 0.04 | 0.313 | −18.55 | 0.0653 | 0.06 | 0.537 | 2.24 | 0.3180 | 0.18 | 0.005 | |
| 2.0 ÷ 5.0 | 69.49 | 0.0304 | 0.03 | 0.234 | 92.05 | 0.0565 | 0.01 | 0.717 | 22.34 | 0.1133 | 0.02 | 0.607 | |
| 15 °C | 1.0 ÷ 1.60 | 67.28 | 0.0218 | 0.03 | 0.543 | 46.94 | 0.0661 | 0.02 | 0.552 | 22.49 | 0.1256 | 0.02 | 0.841 |
| 1.6 ÷ 2.5 | 60.89 | 0.0212 | 0.03 | 0.479 | 115.99 | 0.0542 | 0.01 | 0.517 | 35.11 | 0.1029 | 0.01 | 0.755 | |
| 2.0 ÷ 5.0 | 91.18 | 0.0265 | 0.02 | 0.562 | 61.06 | 0.0707 | 0.02 | 0.637 | 52.63 | 0.1030 | 0.01 | 0.767 | |
| 20 °C | 1.0 ÷ 1.60 | 38.43 | 0.0455 | 0.05 | 0.879 | 22.34 | 0.0919 | 0.04 | 0.505 | 24.35 | 0.1128 | 0.02 | 0.868 |
| 1.6 ÷ 2.5 | 32.21 | 0.0525 | 0.06 | 0.750 | 12.91 | 0.1504 | 0.07 | 0.826 | 16.47 | 0.1472 | 0.03 | 0.957 | |
| 2.0 ÷ 5.0 | 53.62 | 0.0319 | 0.04 | 0.464 | 11.89 | 0.1214 | 0.08 | 0.434 | 17.58 | 0.1642 | 0.03 | 0.740 | |
| 25 °C | 1.0 ÷ 1.60 | 42.46 | 0.0395 | 0.04 | 0.851 | 54.57 | 0.0926 | 0.02 | 0.846 | 21.42 | 0.1383 | 0.02 | 0.713 |
| 1.6 ÷ 2.5 | 33.71 | 0.0475 | 0.06 | 0.769 | 30.17 | 0.1184 | 0.03 | 0.917 | 18.40 | 0.1534 | 0.03 | 0.757 | |
| 2.0 ÷ 5.0 | −10.64 | −0.023 | −0.22 | 0.032 | 17.86 | 0.1330 | 0.05 | 0.352 | −6.92 | 0.1102 | −0.08 | 0.899 | |
| LIV—Langmuir isotherm in Eadie–Hofstee linearization [49] | |||||||||||||
| 5 °C | 1.0 ÷ 1.60 | 149.25 | 0.0172 | 0.01 | 0.533 | 263.16 | 0.0290 | 0.00 | 0.317 | 46.51 | 0.899 | 0.01 | 0.243 |
| 1.6 ÷ 2.5 | 100.00 | 0.0474 | 0.02 | 0.303 | -21.32 | 0.0400 | -0.05 | 0.904 | -5.70 | 0.0647 | -0.10 | 0.960 | |
| 2.0 ÷ 5.0 | 77.52 | 0.0345 | 0.03 | 0.730 | 128.21 | 0.0536 | 0.01 | 0.707 | 45.05 | 0.0754 | 0.01 | 0.641 | |
| 10 °C | 1.0 ÷ 1.60 | 178.57 | 0.0179 | 0.01 | 0.527 | 416.67 | 0.0284 | 0.00 | 0.700 | 24.63 | 0.1255 | 0.02 | 0.823 |
| 1.6 ÷ 2.5 | 149.25 | 0.0406 | 0.01 | 0.313 | 34.60 | 0.0786 | 0.03 | 0.537 | 476.19 | 0.969 | 0.00 | 0.005 | |
| 2.0 ÷ 5.0 | 294.12 | 0.0246 | 0.01 | 0.234 | 128.21 | 0.0549 | 0.01 | 0.717 | 45.05 | 0.0855 | 0.01 | 0.607 | |
| 15 °C | 1.0 ÷ 1.60 | 123.46 | 0.0184 | 0.01 | 0.543 | 84.75 | 0.0612 | 0.01 | 0.552 | 26.74 | 0.1220 | 0.02 | 0.841 |
| 1.6 ÷ 2.5 | 126.58 | 0.0169 | 0.02 | 0.479 | 222.22 | 0.0520 | 0.00 | 0.517 | 46.51 | 0.0994 | 0.01 | 0.755 | |
| 2.0 ÷ 5.0 | 161.29 | 0.0239 | 0.01 | 0.562 | 96.15 | 0.677 | 0.01 | 0.637 | 68.49 | 0.1007 | 0.01 | 0.767 | |
| 20 °C | 1.0 ÷ 1.60 | 43.67 | 0.0439 | 0.04 | 0.879 | 44.25 | 0.0803 | 0.02 | 0.505 | 28.09 | 0.1100 | 0.02 | 0.868 |
| 1.6 ÷ 2.5 | 46.95 | 0.0443 | 0.04 | 0.750 | 15.63 | 0.1434 | 0.06 | 0.826 | 17.21 | 0.1459 | 0.03 | 0.957 | |
| 2.0 ÷ 5.0 | 116.28 | 0.0270 | 0.02 | 0.464 | 27.40 | 0.0984 | 0.04 | 0.434 | 23.87 | 0.1567 | 0.02 | 0.736 | |
| 25 °C | 1.0 ÷ 1.60 | 50.00 | 0.0377 | 0.04 | 0.851 | 64.52 | 0.0911 | 0.02 | 0.846 | 30.03 | 0.1314 | 0.02 | 0.713 |
| 1.6 ÷ 2.5 | 43.86 | 0.0440 | 0.04 | 0.769 | 32.89 | 0.1170 | 0.03 | 0.917 | 24.33 | 0.1466 | 0.02 | 0.757 | |
| 2.0 ÷ 5.0 | 333.33 | 0.0218 | 0.01 | 0.032 | 50.76 | 0.1160 | 0.02 | 0.352 | −7.7101 | 0.1176 | -0.07 | 0.899 | |
| T, °C | ESRQ | HYBRYD | ARE | Fisher | χ2 | ||
|---|---|---|---|---|---|---|---|
| 5 °C | 1.0 ÷ 1.6 | 0.5 | L.I. 3.57 · 10−5 | L.I. 5.96 · 10−2 | L.I. 18.3 | L.I. 7.04 | L.I. 4.17 · 10−3 |
| 1.0 | L.II. 1.11 · 10−4 | L.II. 5.46 · 10−2 | L.II. 9.42 | L.IV. 1.34 | L.II. 3.82 · 10−3 | ||
| 2.0 | L.I. 2.04 · 10−3 | L.I. 4.49 · 10−1 | L.I. 17.7 | L.I. 1.89 | L.I. 3.14 · 10−2 | ||
| 1.6 ÷ 2.5 | 0.5 | L.IV. 5.06 · 10−4 | L.IV. 1.53 · 10−3 | L.II. 13.2 | L.IV. 1.81 | L.IV. 1.07 · 10−2 | |
| 1.0 | L.IV. 2.81 · 10−3 | L.II. 4.65 · 10−1 | L.II. 18.4 | L.IV. 2.29 | L.II. 3.25 · 10−2 | ||
| 2.0 | L.II. 1.93 · 10−2 | L.II. 1.23 | L.II. 16.5 | L.II. 3.79 | L.II. 8.61 · 10−2 | ||
| 2.0–5.0 | 0.5 | L.I. 5.98 · 10−5 | L.I. 5.09 · 10−1 | L.I. 10.6 | L.I. 11.7 | L.I. 3.56 · 10−3 | |
| 1.0 | L.IV. 6.60 · 10−5 | L.II. 1.97 · 10−2 | L.II. 4.26 | L.IV. 3.38 | L.II. 1.38 · 10−3 | ||
| 2.0 | L.III. 5.28 · 10−4 | L.III. 1.28 · 10−1 | L.II. 4.26 | L.IV. 3.38 | L.II. 1.38 · 10−3 | ||
| 10 °C | 1.0 ÷ 1.6 | 0.5 | L.I. 3.20 · 10−5 | L.I. 4.62 · 10−2 | L.I. 12.2 | L.III. 4.55 | L.I. 3.23 · 10−3 |
| 1.0 | L.IV. 1.94 · 10−4 | L.II. 9.35 · 10−2 | L.II. 12.8 | L.IV. 1.05 | L.II. 6.55 · 10−3 | ||
| 2.0 | L.III. 9.15 · 10−4 | L.IV. 1.22 · 10−1 | L.II. 7.29 | L.III. 6.36 | L.IV. 8.54 · 10−3 | ||
| 1.6 ÷ 2.5 | 0.5 | L.IV. 2.29 · 10−4 | L.IV. 8.84 · 10−2 | L.II. 11.5 | L.IV. 1.58 | L.IV. 6.19 · 10−3 | |
| 1.0 | L.II. 3.80 · 10−3 | L.II. 5.27 · 10−1 | L.II. 20.0 | L.II. 1.19 | L.II. 3.69 · 10−2 | ||
| 2.0 | L.II. 2.16 · 10−3 | L.II. 3.90 · 10−1 | L.I. 12.5 | L.II. 9.59 · 10−1 | L.II. 2.73 · 10−2 | ||
| 2.0–5.0 | 0.5 | L.IV. 1.16 · 10−4 | L.II. 6.94 · 10−2 | L.II. 13.7 | L.IV. 1.23 | L.II. 4.86 · 10−3 | |
| 1.0 | L.IV. 6.63 · 10−5 | L.IV. 1.81 · 10−2 | L.II. 4.29 | L.IV. 3.47 | L.IV. 1.27 · 10−3 | ||
| 2.0 | L.IV. 6.23 · 10−4 | L.IV. 1.12 · 10−1 | L.II. 10.1 | L.IV. 2.75 | L.IV. 7.85 · 10−3 | ||
| 15 °C | 1.0 ÷ 1.6 | 0.5 | L.I. 4.96 · 10−5 | L.I. 7.38 · 10−2 | L.II. 19.1 | L.I. 6.00 | L.I. 5.17 · 10−3 |
| 1.0 | L.IV. 3.13 · 10−4 | L.IV. 6.87 · 10−2 | L.II. 6.97 | L.IV. 2.12 | L.IV. 4.81 · 10−3 | ||
| 2.0 | L.III. 3.76 · 10−4 | L.III. 6.36 · 10−2 | L.I. 5.95 | L.III. 11.3 | L.III. 4.45 · 10−3 | ||
| 1.6 ÷ 2.5 | 0.5 | L.I. 3.45 · 10−5 | L.I. 6.46 · 10−2 | L.II. 18.9 | L.I. 9.13 | L.I. 4.52 · 10−3 | |
| 1.0 | L.IV. 6.12 · 10−5 | L.II. 1.75 · 10−2 | L.II. 3.85 | L.IV. 1.89 | L.II. 1.22 · 10−3 | ||
| 2.0 | L.IV. 3.66 · 10−4 | L.II. 5.59 · 10−2 | L.II. 5.91 | L.IV. 4.10 | L.II. 3.91 · 10−3 | ||
| 2.0–5.0 | 0.5 | L.I. 5.76 · 10−5 | L.I. 5.52 · 10−2 | L.II. 11.1 | L.I. 3.71 | L.I. 3.86 · 10−3 | |
| 1.0 | L.IV. 1.13 · 10−4 | L.II. 2.87 · 10−2 | L.II. 4.77 | L.II. 3.23 | L.II. 2.01 · 10−3 | ||
| 2.0 | L.IV. 1.59 · 10−4 | L.II. 2.29 · 10−2 | L.II. 3.13 | L.IV. 3.96 | L.IV. 1.60 · 10−3 | ||
| 20 °C | 1.0 ÷ 1.6 | 0.5 | L.III. 9.77 · 10−5 | L.III. 4.35 · 10−2 | L.I. 9.70 | L.III. 16.4 | L.III. 3.05 · 10−3 |
| 1.0 | L.IV. 9.48 · 10−4 | L.IV. 1.81 · 10−1 | L.II. 11.1 | L.IV. 2.84 | L.IV. 1.27 · 10−2 | ||
| 2.0 | L.III. 3.92 · 10−4 | L.III. 7.16 · 10−2 | L.II. 6.20 | L.III. 10.3 | L.III. 5.01 · 10−3 | ||
| 1.6 ÷ 2.5 | 0.5 | L.I. 9.93 · 10−5 | L.I. 6.02 · 10−2 | L.I. 11.3 | L.I. 17.1 | L.I. 4.21 · 10−3 | |
| 1.0 | L.IV. 1.93 · 10−3 | L.II. 2.16 · 10−1 | L.II. 9.62 | L.IV. 6.54 | L.II. 1.52 · 10−2 | ||
| 2.0 | L.III. 3.02 · 10−4 | L.II. 3.50 · 10−2 | L.II. 3.71 | L.III. 26.6 | L.II. 2.45 · 10−3 | ||
| 2.0–5.0 | 0.5 | L.III. 1.42 · 10−4 | L.IV. 1.00 · 10−1 | L.II. 15.6 | L.III. 2.37 | L.IV. 7.02 · 10−3 | |
| 1.0 | L.I. 1.63 · 10−3 | L.I. 4.29 · 10−1 | L.I. 20.0 | L.I. 4.56 | L.I. 3.00 · 10−2 | ||
| 2.0 | L.IV. 1.32 · 10−3 | L.IV. 1.52 · 10−1 | L.II. 8.16 | L.IV. 4.64 | L.II. 1.07 · 10−2 | ||
| 25 °C | 1.0 ÷ 1.6 | 0.5 | L.I. 4.01 · 10−5 | L.I. 3.07 · 10−2 | L.I. 11.3 | L.I. 2.92 | L.I. 2.15 · 10−3 |
| 1.0 | L.III. 1.14 · 10−4 | L.IV. 2.05 · 10−2 | L.II. 3.82 | L.III. 7.21 | L.IV. 1.44 · 10−3 | ||
| 2.0 | L.III. 8.27 · 10−4 | L.III. 1.17 · 10−1 | L.II. 8.84 | L.III. 4.84 | L.III. 8.20 · 10−3 | ||
| 1.6 ÷ 2.5 | 0.5 | L.I. 6.92 · 10−5 | L.I. 4.81 · 10−2 | L.I. 11.7 | L.I. 24.1 | L.I. 3.37 · 10−3 | |
| 1.0 | L.III. 1.94 · 10−4 | L.III. 2.75 · 10−2 | L.II. 3.96 | L.III. 13.8 | L.III. 1.92 · 10−3 | ||
| 2.0 | L.III. 9.98 · 10−4 | L.III. 1.29 · 10−1 | L.II. 8.95 | L.III. 5.77 | L.III. 9.02 · 10−3 | ||
| 2.0–5.0 | 0.5 | L.II. 6.52 · 10−4 | L.I. 3.32 · 10−1 | L.I. 23.3 | L.II. 8.87 | L.I. 2.33 · 10−2 | |
| 1.0 | L.IV. 1.32 · 10−3 | L.II. 1.73 · 10−1 | L.II. 9.06 | L.IV. 1.52 | L.II. 1.21 · 10−2 | ||
| 2.0 | L.IV. 3.41 · 10−2 | L.IV. 2.67 | L.IV. 25.9 | L.IV. 1.42 | L.IV. 1.87 · 10−1 |
| Granulation, mm | Concentration C0, mg/L | ΔS, J/mol | ΔH, J/(mol · K) | ΔG, J/(mol · K) |
|---|---|---|---|---|
| 1.0–1.6 | 0.5 | 77.321 | −29,099.00 | −7254.18 ÷ −6073.08 |
| 1.0–1.6 | 1.0 | 108.980 | −38,475.53 | −7953.77 ÷ −5947.07 |
| 1.0–1.6 | 2.0 | 1.238 | −7015.85 | −6720.82 ÷ −6419.69 |
| 1.6–2.5 | 0.5 | 52.998 | −21,633.86 | −6346.95 ÷ −5601.56 |
| 1.6–2.5 | 1.0 | 132.284 | −32,214.26 | −7603.71 ÷ −5256.99 |
| 1.6–2.5 | 2.0 | 87.970 | −32,214.26 | −8151.18 ÷ −6229.76 |
| 2.0–5.0 | 0.5 | 0.853 | −6992.24 | −6146.72 ÷ −6673.73 |
| 2.0–5.0 | 1.0 | 92.028 | −32,647.42 | −6835.24 ÷ −5440.45 |
| 2.0–5.0 | 2.0 | 28.528 | −15,138.13 | −7258.97 ÷ −6816.61 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zawadzka, B.; Siwiec, T.; Reczek, L.; Marzec, M.; Jóźwiakowski, K. Modeling of Phosphate Sorption Process on the Surface of Rockfos® Material Using Langmuir Isotherms. Appl. Sci. 2024, 14, 10996. https://doi.org/10.3390/app142310996
Zawadzka B, Siwiec T, Reczek L, Marzec M, Jóźwiakowski K. Modeling of Phosphate Sorption Process on the Surface of Rockfos® Material Using Langmuir Isotherms. Applied Sciences. 2024; 14(23):10996. https://doi.org/10.3390/app142310996
Chicago/Turabian StyleZawadzka, Beata, Tadeusz Siwiec, Lidia Reczek, Michał Marzec, and Krzysztof Jóźwiakowski. 2024. "Modeling of Phosphate Sorption Process on the Surface of Rockfos® Material Using Langmuir Isotherms" Applied Sciences 14, no. 23: 10996. https://doi.org/10.3390/app142310996
APA StyleZawadzka, B., Siwiec, T., Reczek, L., Marzec, M., & Jóźwiakowski, K. (2024). Modeling of Phosphate Sorption Process on the Surface of Rockfos® Material Using Langmuir Isotherms. Applied Sciences, 14(23), 10996. https://doi.org/10.3390/app142310996








