Modeling Shearing and Bending Behavior of Kiwifruit Branches Using the Discrete Element Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Description of Kiwifruit Branch Samples
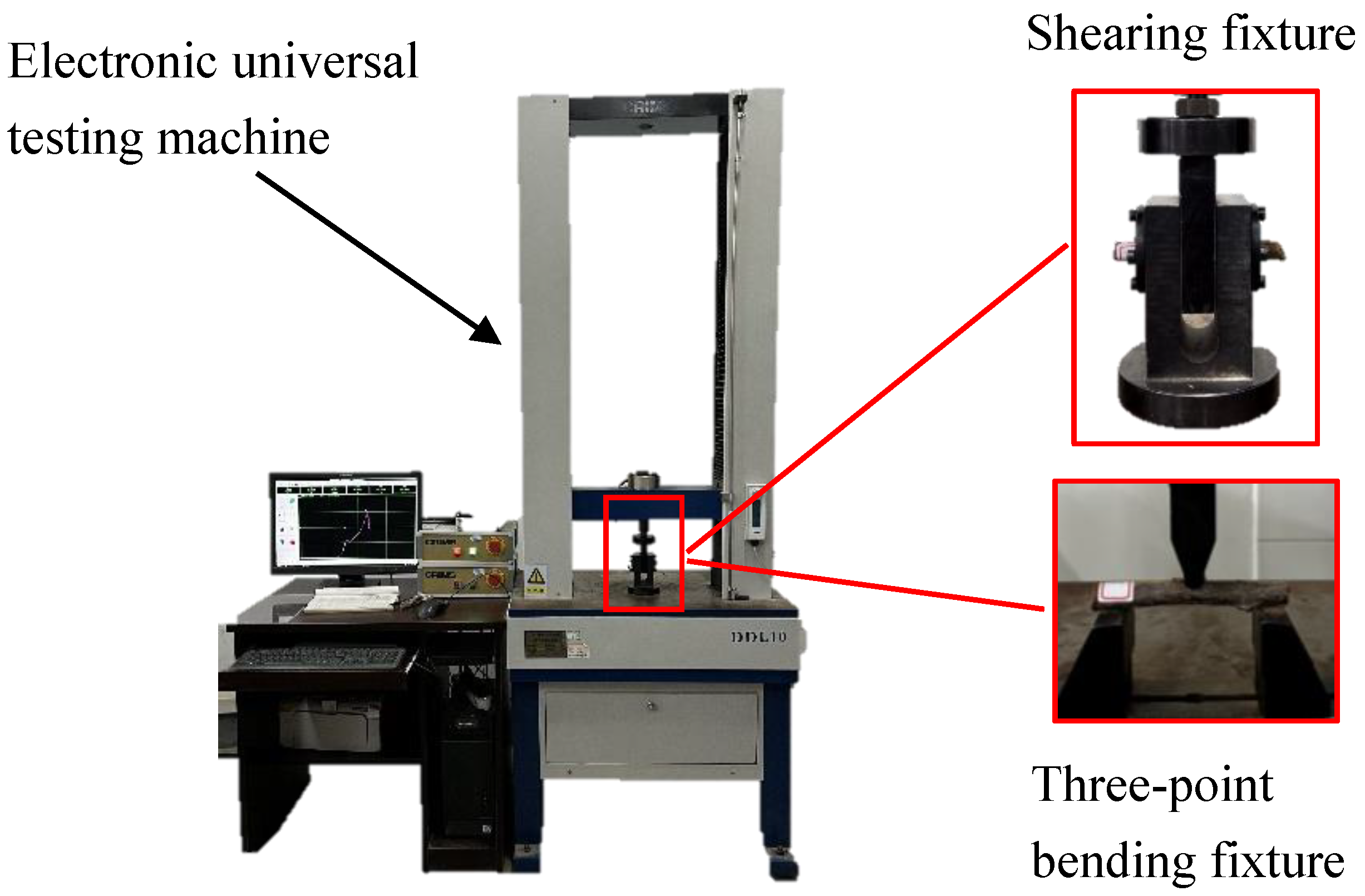
2.2. Mechanical Property Test of Kiwifruit Branch
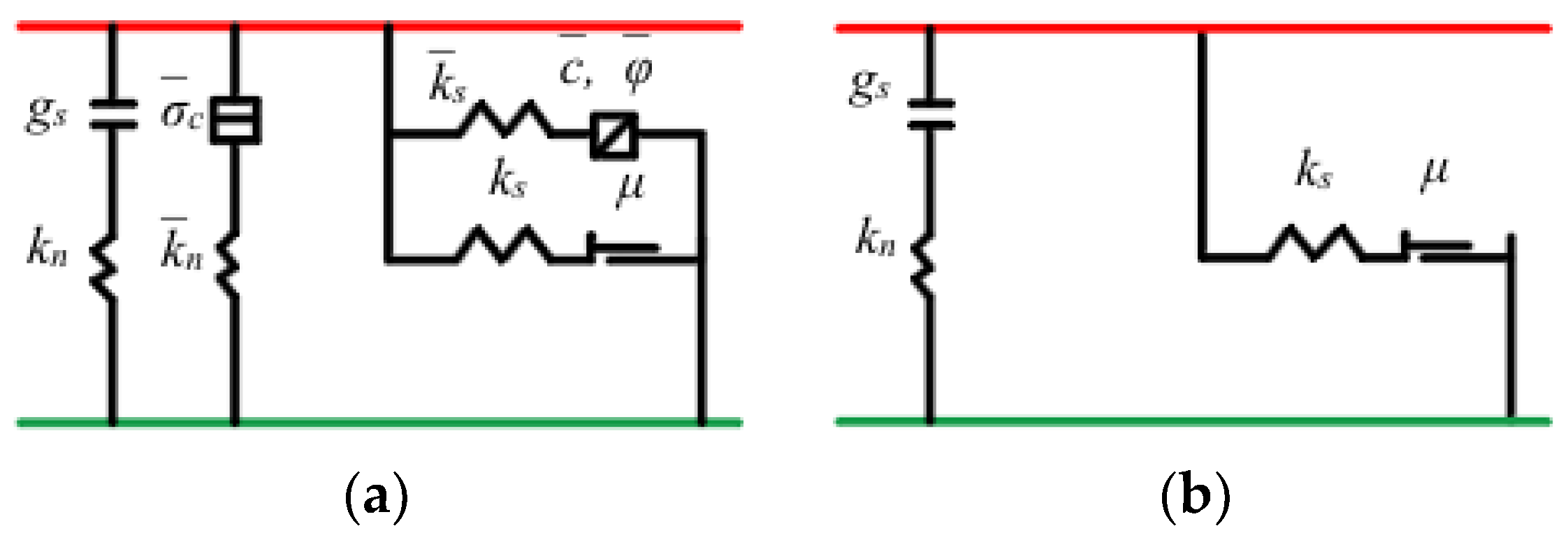
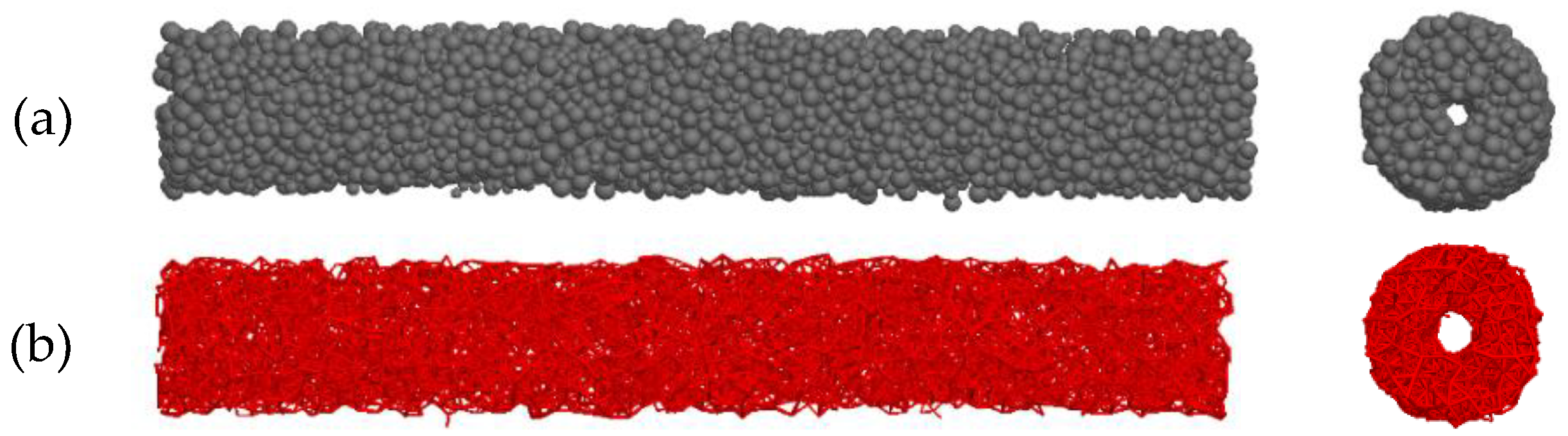
2.3. Discrete Element Modeling of Kiwifruit Branch
2.4. Calibration for DEM Model Parameters
3. Results and Discussion
3.1. Experimental Result
3.2. Screening Out Significant Parameters
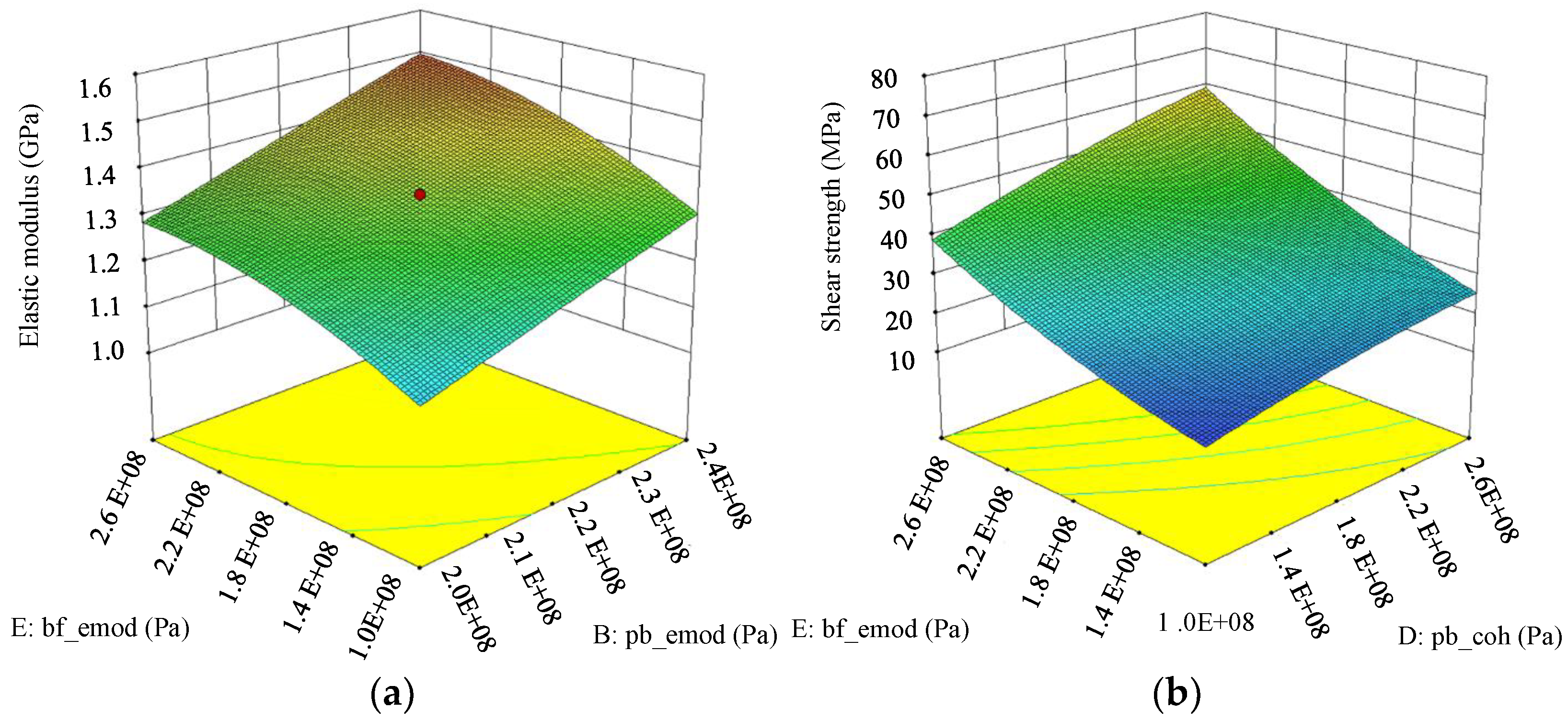
3.3. Determination of the Optimal Ranges of Significant Parameters
3.4. Determination of Optimal Values for the DEM Parameters
R2 = 0.98
R2 = 0.97
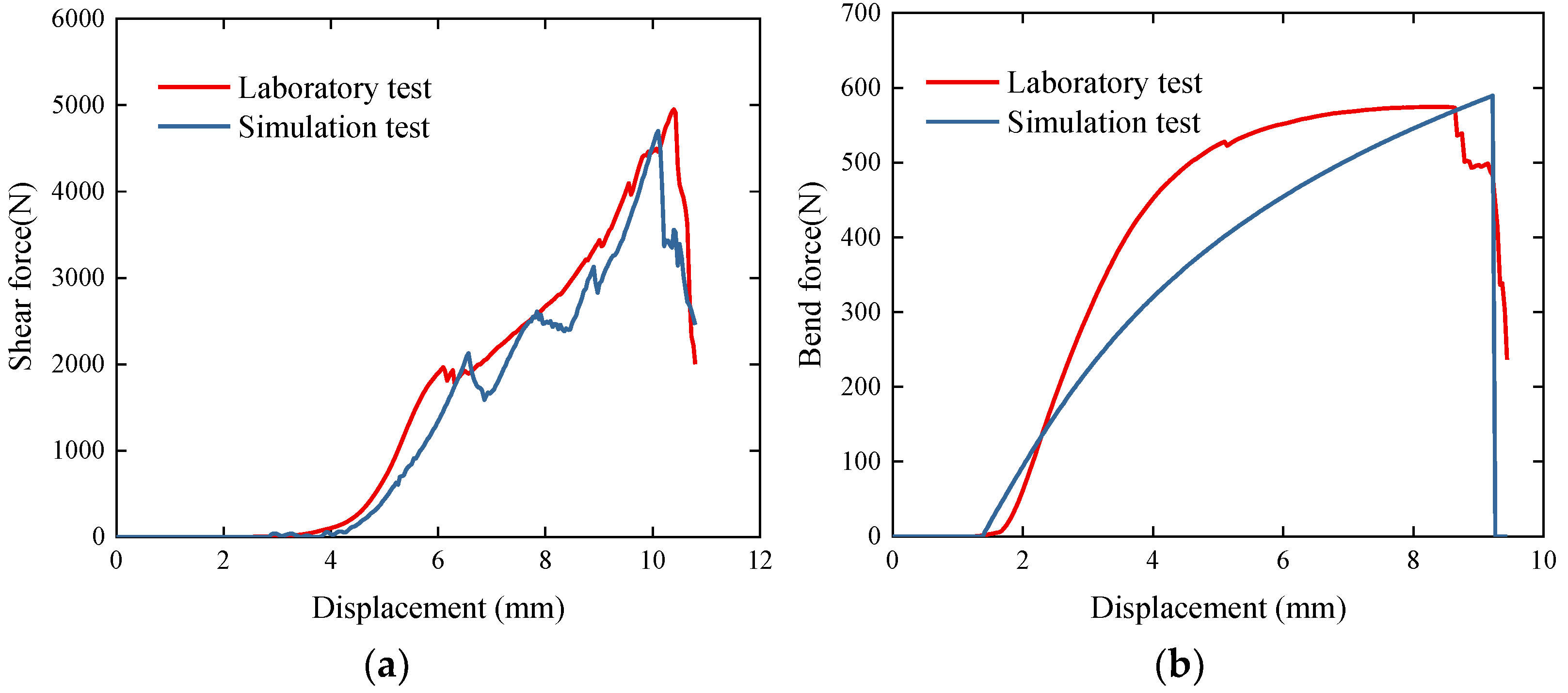
3.5. Parameter Verification and Simulation of Shearing and Bending Process
4. Conclusions
- (1)
- The shear modulus and elastic modulus of the kiwifruit branch samples were determined to be 31.38 MPa and 1.21 GPa, respectively, through shearing and three-point bending tests.
- (2)
- Four DEM parameters were identified as having a significant impact on the mechanical response of the kiwifruit branch model: effective modulus of bond, cohesion of bond, effective modulus between ball and wall, and normal-to-shear stiffness ratio.
- (3)
- Through response surface methodology, the functions relating DEM parameters to the mechanical response of the kiwifruit branch model were established. The optimum parameter combination of the effective modulus of bond, cohesion of bond, effective modulus between ball and wall, and normal-to-shear stiffness ratio was determined to be 2.2 × 109 Pa, 2.56 × 108 Pa, 1.27 × 108 Pa, and 1.6.
- (4)
- The verification tests conducted with the optimal parameter combination revealed a relative error of 4.5% between the simulation and experimental results. The displacement–force curves demonstrated a high degree of congruence, affirming the reliability of the developed model.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sun, X.Y.; Wei, X.F.; Zhang, J.X.; Ge, Q.; Liang, Y.Y.; Ju, Y.L.; Zhang, A.; Ma, T.T.; Fang, Y.L. Biomass estimation and physicochemical characterization of winter vine prunings in the Chinese and global grape and wine industries. Waste Manag. 2020, 104, 119–129. [Google Scholar] [CrossRef] [PubMed]
- Jing, W.T.; Zhao, J.H.; Zhuang, T.F.; Liu, Z.J.; Yang, X.J.; Liu, L.J. Review on theory and equipment of mechanical vibration picking of forest fruits. Trans. Chin. Soc. Agric. Mach. 2023, 54 (Suppl. S1), 144–160. [Google Scholar]
- Yuan, Y.W.; Bai, S.H.; Niu, K.; Zhou, L.M.; Zhao, B.; Wei, L.G.; Xiong, S.; Liu, L.J. Research progress on mechanized harvesting technology and equipment for forest fruit. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2022, 38, 53–63. [Google Scholar]
- Cai, Z.Y.; Mao, B.Q.; Ao, C.L.; Liu, B.Q. Public preferences and willingness to pay for environmental benefits of straw return: Empirical evidence from Northeast China. J. Environ. Manag. 2024, 371, 123078. [Google Scholar] [CrossRef] [PubMed]
- Xie, X.; Rahman, M.M.; Pang, R.L.; Qiao, C.K.; Guo, L.L.; Xie, H.Z.; Pang, R.L.; Tian, F.J. Effect of forchlorfenuron (CPPU) on fruits quality and residue analysis in kiwifruits. J. Food Compos. Anal. 2024, 133, 106383. [Google Scholar] [CrossRef]
- Yan, Y.C.; Hao, S.H.; Gao, Y.L.; Xin, D.; Niu, Z.J. Design of kiwifruit orchard disease and pest detection system based on aerial and ground multi-source information. Trans. Chin. Soc. Agric. Mach. 2023, 54, 291–300. [Google Scholar]
- Chen, Z.G.; Wang, D.F.; Li, L.Q.; Shan, R.X. Experiment on tensile and shearing characteristics of rind of corn stalk. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2012, 28, 59–65. [Google Scholar]
- Shi, N.; Guo, K.Q.; Fan, Y.J.; Liu, B.X.; Yuan, X.L. Peeling and shearing mechanical performance test of cotton stalks in extrusion state. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2017, 33, 51–58. [Google Scholar]
- Yang, H.W.; Zhang, L.Y.; Tang, Z.Q.; Yu, F.H.; Xu, T.Y. Effects of mechanical and physicochemical properties on the lodging resistance of hybrid japonica rice. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2024, 40, 44–52. [Google Scholar]
- Kang, F.; Tong, S.Y.; Zhang, H.S.; Li, W.B.; Chen, Z.J.; Zheng, Y.J. Analysis and experiments of reciprocating cutting parameters for apple tree branches. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2020, 36, 9–16. [Google Scholar]
- Bu, L.X.; Chen, C.K.; Hu, G.R.; Zhou, J.G.; Sugirbay, A.; Chen, J. Investigating the dynamic behavior of an apple branch-stemfruit model using experimental and simulation analysis. Comput. Electron. Agric. 2021, 186, 106224. [Google Scholar] [CrossRef]
- Fu, S.K. Design and Experiment of Organic Fertilizer Application Machine for Kiwi Orchard. Master’s Thesis, Northwest A&F University, Yangling, China, 2021. [Google Scholar]
- Gao, X.J.; Xie, G.F.; Xu, Y. Application of a staggered symmetrical spiral groove wheel on a quantitative feeding device and investigation of particle motion characteristics based on DEM. Powder Technol. 2022, 407, 117650. [Google Scholar] [CrossRef]
- Gong, H.; Chen, Y.; Wu, S.L.; Tang, Z.Y.; Liu, C.; Wang, Z.Q.; Fu, D.B.; Zhou, Y.H.; Qi, L. Simulation of canola seedling emergence dynamics under different soil compaction levels using the discrete element method (DEM). Soil Tillage Res. 2022, 223, 105461. [Google Scholar] [CrossRef]
- László, P.; Bence, S.; Kornél, T. Measuring and modelling of soil displacement from a horizontal penetrometer and a sweep using an IMU sensor fusion and DEM. Soil Tillage Res. 2024, 244, 106207. [Google Scholar] [CrossRef]
- Mak, J.; Chen, Y.; Sadek, M.A. Determining parameters of a discrete element model for soil–tool interaction. Soil Tillage Res. 2012, 118, 117–122. [Google Scholar] [CrossRef]
- Itasca. User’s Manual for PFC3D Version PFC5.0; Itasca Consulting Group, Inc.: Minneapolis, MN, USA, 2017. [Google Scholar]
- Li, M.; Xu, S.; Yang, Y.W.; Guo, L.; Tong, J. A 3D simulation model of corn stubble cutting using finite element method. Soil Tillage Res. 2017, 166, 43–51. [Google Scholar] [CrossRef]
- Shi, L.R.; Zhao, W.Y.; Sun, W. Parameter calibration of soil particles contact model of farmland soil in northwest arid region based on discrete element method. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2017, 33, 181–187. [Google Scholar]
- Zhao, H.B.; Huang, Y.X.; Liu, Z.D.; Liu, W.Z.; Zheng, Z.Q. Applications of discrete element method in the research of agricultural machinery: A review. Agriculture 2021, 11, 425. [Google Scholar] [CrossRef]
- Shi, Y.Y.; Jiang, Y.; Wang, X.C.; Thuy, N.T.D.; Yu, H.M. A mechanical model of single wheat straw with failure characteristics based on discrete element method. Biosyst. Eng. 2023, 230, 1–15. [Google Scholar] [CrossRef]
- Liu, Y.G.; Zhao, J.G.; Yin, B.Z.; Ma, Z.K.; Hao, J.J.; Yang, X.; Feng, X.J.; Ma, Y.J. Discrete element modelling of the yam root-soil complex and its verification. Biosyst. Eng. 2022, 220, 55–72. [Google Scholar] [CrossRef]
- Xie, K.T.; Zhang, Z.G.; Wang, F.A.; Jiang, S.F.; Wang, C.L. Mathematical model construction and verification of soil and Panax Notoginseng root adhesion. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2022, 38, 131–141. [Google Scholar]
- Zhang, Y.Q.; Cui, Q.L.; Li, H.B. Study on biomechanical properties of oat straw. Farm Prod. Process. 2021, 539, 24–27. [Google Scholar]
- Gong, H.; Chen, Y.; Zheng, W.H.; Zeng, Z.W.; Li, S.; Qi, L. Measurements and DEM modelling of soybean seed expansion. Comput. Electron. Agric. 2023, 208, 107786. [Google Scholar] [CrossRef]
- Wang, H.; Li, X.; Huang, W.H.; Vanapalli, S.K. Experimental investigations of the shear strength and deformation behavior of a frozen unsaturated silt. Cold Reg. Sci. Technol. 2024, 12, 104332. [Google Scholar] [CrossRef]
- Wang, M.; Cao, P. Calibrating the micromechanical parameters of the PFC2D 3D models using the improved simulated annealing algorithm. Math. Probl. Eng. Theory Methods Appl. 2017, 2017, 6401835. [Google Scholar] [CrossRef]
- Zheng, Z.Q.; Zhao, H.B.; Liu, P.; He, J. Maize straw cutting process modelling and parameter calibration based on discrete element Method (DEM). INMATEH-Agric. Eng. 2021, 63, 461–468. [Google Scholar] [CrossRef]
- Vahid, S.; Chen, Y. Simulation of tensile behavior of plant fibers using the Discrete Element Method (DEM). Compos. Part A Appl. Sci. Manuf. 2018, 114, 196–203. [Google Scholar] [CrossRef]
- Su, Y.; Xu, Y.; Cui, T.; Gao, X.J.; Xia, G.Y.; Li, Y.B.; Qiao, M.M. Determination and interpretation of bonded particle model parameters for simulation of maize kernels. Biosyst. Eng. 2021, 210, 193–205. [Google Scholar] [CrossRef]
- Islam, M.S.; Larpruenrudee, P.; Rahman, M.M.; Li, G.L.; Husain, S.; Munir, A.; Zhao, M.; Sauret, E.; Gu, Y.T. Pharmaceutical aerosol transport in airways: A combined machine learning (ML) and discrete element model (DEM) approach. Powder Technol. 2024, 448, 120271. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, J.; Du, D.D.; Long, S.F.; Wang, Y.W.; Han, C.J.; Xu, Y. Optimization and validation of root-cutting device for Chinese cabbage harvester based on discrete element method. Comput. Electron. Agric. 2023, 214, 108314. [Google Scholar] [CrossRef]
- Zhang, Z.G.; Zeng, C.; Xing, Z.Y.; Xu, P.; Guo, Q.F.; Shi, R.M.; Wang, Y.Z. Discrete element modelling and parameter calibration of safflower biomechanical properties. Int. J. Agric. Biol. Eng. 2024, 17, 37–46. [Google Scholar]
- Zhang, X.R.; Hu, X.H.; Liu, J.X.; Yang, Y.M.; Li, Y. Calibration and verification of bonding parameters of banana straw simulation model based on discrete element method. Trans. Chin. Soc. Agric. Mach. 2023, 54, 121–130. [Google Scholar]
- Schramm, M.; Tekeste, M.Z.; Plouffe, C.; Harby, D. Estimating bond damping and bond Young’s modulus for a flexible wheat straw discrete element method model. Biosyst. Eng. 2019, 186, 349–355. [Google Scholar] [CrossRef]
- Zhang, F.W.; Song, X.F.; Zhang, X.K.; Zhang, F.Y.; Wei, W.C.; Dai, F. Simulation and experiment on mechanical characteristics of kneading and crushing process of corn straw. Trans. Chin. Soc. Agric. Eng. 2019, 35, 58–65. [Google Scholar]
- Tian, Y.Y.; Zeng, Z.W.; Gong, H.; Zhou, Y.H.; Qi, L.; Zhen, W.B. Simulation of tensile behavior of tobacco leaf using the discrete element method (DEM). Comput. Electron. Agric. 2023, 205, 107570. [Google Scholar] [CrossRef]
- Sun, K.; Yu, J.Q.; Zhao, J.W.; Liang, L.S.; Wang, Y.; Yu, Y.J. A DEM-based general modeling method and experimental verification for wheat plants in the mature period. Comput. Electron. Agric. 2023, 214, 108283. [Google Scholar] [CrossRef]




| Model Parameters | Values |
|---|---|
| Density/(kg/m3) | 577 |
| Effective modulus of ball, emod/Pa | To be calibrated |
| Effective modulus of bond, pb_emod/Pa | To be calibrated |
| Effective modulus between ball and wall, bf_emod/Pa | To be calibrated |
| Friction coefficient between straw and wall, f | 0.35 |
| Ball local damping coefficient, β | 0.7 |
| Bond gap, G/mm | 0.6 |
| Friction angle, pb_fa/(°) | 50 |
| Tensile strength of bond, pb_ten/Pa | To be calibrated |
| Cohesion of bond, pb_coh/Pa | To be calibrated |
| Normal-to-shear stiffness ratio, kratio | To be calibrated |
| Factors | Levels | ||
|---|---|---|---|
| −1 | 1 | ||
| A | Effective modulus of ball, emod/Pa | 5 × 107 | 1 × 108 |
| B | Effective modulus of bond, pb_emod/Pa | 2 × 109 | 3 × 109 |
| C | Tensile strength of bond, pb_ten/Pa | 1 × 108 | 5 × 108 |
| D | Cohesion of bond, pb_coh/Pa | 1 × 108 | 5 × 108 |
| E | Effective modulus between ball and wall, bf_emod/Pa | 1 × 108 | 5 × 108 |
| F | Normal-to-shear stiffness ratio, kratio | 1 | 1.5 |
| Mechanical Properties | Max | Min | Average | Standard Deviation |
|---|---|---|---|---|
| External diameter (mm) | 13.5 | 8.1 | 10.5 | 1.4 |
| Inner diameter (mm) | 4.5 | 1.6 | 3.0 | 0.7 |
| Shear strength (MPa) | 20.37 | 49.78 | 31.38 | 6.72 |
| Elastic modulus (GPa) | 1.79 | 0.72 | 1.21 | 0.34 |
| No. | A | B | C | D | E | F | Shear Strength (MPa) | Elastic Modulus (GPa) |
|---|---|---|---|---|---|---|---|---|
| 1 | 1 | −1 | −1 | 1 | 1 | 1 | 58.20 | 1.21 |
| 2 | 1 | −1 | 1 | −1 | −1 | 1 | 14.63 | 1.03 |
| 3 | −1 | 1 | −1 | 1 | 1 | −1 | 63.46 | 2.00 |
| 4 | 1 | −1 | 1 | −1 | 1 | −1 | 39.67 | 1.42 |
| 5 | −1 | 1 | −1 | 1 | −1 | 1 | 16.42 | 1.44 |
| 6 | 1 | −1 | 1 | 1 | −1 | −1 | 30.55 | 1.21 |
| 7 | −1 | −1 | 1 | 1 | 1 | 1 | 66.15 | 1.03 |
| 8 | −1 | 1 | 1 | −1 | −1 | −1 | 27.11 | 1.53 |
| 9 | 1 | 1 | 1 | 1 | 1 | 1 | 57.34 | 1.57 |
| 10 | −1 | −1 | −1 | −1 | −1 | −1 | 14.03 | 1.17 |
| 11 | −1 | 1 | −1 | −1 | 1 | 1 | 38.78 | 1.73 |
| 12 | 1 | 1 | −1 | −1 | −1 | −1 | 12.17 | 1.55 |
| Source | Sum of Squares | F-Value | p-Value |
|---|---|---|---|
| Response | Shear strength (MPa) | ||
| Model | 4374.424 | 14.358 | 0.005 |
| A-emod | 55.108 | 1.0853 | 0.345 |
| B-pb_emod | 1.024 | 0.020 | 0.892 |
| C-ten | 113.906 | 2.243 | 0.194 |
| D-coh | 599.463 | 11.806 | 0.019 |
| E-bf_emod | 2437.502 | 48.003 | 0.001 |
| F-kratio | 57.961 | 1.1419 | 0.334 |
| Response | Elastic modulus (GPa) | ||
| Model | 0.872 | 11.153 | 0.009 |
| A-emod | 0.001 | 0.085 | 0.783 |
| B-pbemod | 0.482 | 36.962 | 0.002 |
| C-ten | 0.016 | 1.197 | 0.324 |
| D-coh | 0.000 | 0.001 | 0.979 |
| E-bfemod | 0.154 | 11.796 | 0.019 |
| F-kratio | 0.129 | 9.883 | 0.026 |
| No. | Test Factors | Shear Strength (MPa) | Elastic Modulus (GPa) | Relative Error (%) | |||
|---|---|---|---|---|---|---|---|
| B | D | E | F | ||||
| 1 | 2 × 109 | 1 × 108 | 1 × 108 | 1 | 13.49 | 1.17 | 30.1 |
| 2 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.37 | 1.35 | 7.2 |
| 3 | 2.4 × 109 | 2.6 × 108 | 2.6 × 108 | 1.2 | 50.71 | 1.41 | 38.9 |
| 4 | 2.6 × 109 | 3.4 × 108 | 3.4 × 108 | 1.3 | 75.48 | 1.57 | 85.1 |
| 5 | 2.8 × 109 | 4.2 × 108 | 4.2 × 108 | 1.4 | 107.81 | 1.72 | 97.4 |
| 6 | 3 × 109 | 5 × 108 | 5 × 108 | 1.5 | 29.57 | 1.76 | 144.6 |
| No. | Test Factors | Shear Strength (MPa) | Elastic Modulus (GPa) | |||
|---|---|---|---|---|---|---|
| B | D | E | F | |||
| 1 | 2 × 109 | 1 × 108 | 2.6 × 108 | 1.0 | 34.98 | 1.24 |
| 2 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.3 | 31.98 | 1.28 |
| 3 | 2 × 109 | 2.6 × 108 | 2.6 × 108 | 1.2 | 58.48 | 1.17 |
| 4 | 2.2 × 109 | 0.45 × 108 | 1.8 × 108 | 1.1 | 19.07 | 1.11 |
| 5 | 2.2 × 109 | 1.8 × 108 | 0.45 × 108 | 1.1 | 14.42 | 1.04 |
| 6 | 1.86 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.62 | 1.17 |
| 7 | 2.2 × 109 | 1.8 × 108 | 3.15 × 108 | 1.1 | 72.02 | 1.43 |
| 8 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.37 | 1.35 |
| 9 | 2.4 × 109 | 2.6 × 108 | 2.6 × 108 | 1.0 | 48.90 | 1.53 |
| 10 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.37 | 1.35 |
| 11 | 2 × 109 | 1 × 108 | 1 × 108 | 1.0 | 13.45 | 1.14 |
| 12 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.37 | 1.35 |
| 13 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.37 | 1.35 |
| 14 | 2.54 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 39.39 | 1.50 |
| 15 | 2.4 × 109 | 1 × 108 | 2.6 × 108 | 1.2 | 35.86 | 1.34 |
| 16 | 2 × 109 | 2.6 × 108 | 1 × 108 | 1.2 | 23.50 | 1.03 |
| 17 | 2.4 × 109 | 2.6 × 108 | 1 × 108 | 1.0 | 23.64 | 1.31 |
| 18 | 2.4 × 109 | 1 × 108 | 1 × 108 | 1.2 | 16.78 | 1.26 |
| 19 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 0.9 | 31.00 | 1.43 |
| 20 | 2.2 × 109 | 1.8 × 108 | 1.8 × 108 | 1.1 | 32.37 | 1.35 |
| 21 | 2.2 × 109 | 3.15 × 108 | 1.8 × 108 | 1.1 | 44.78 | 1.23 |
| Source | y1: Elastic Modulus (GPa) | y2: Shear Strength (MPa) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Sum of Squares | df | Mean Square | F-Value | p-Value | Sum of Squares | df | Mean Square | F-Value | p-Value | |
| Model | 0.374 | 14 | 0.027 | 21.8 | 0.00 | 3850.913 | 14 | 275.065 | 12.6 | 0.00 |
| B | 0.053 | 1 | 0.053 | 43.2 | 0.00 | 22.909 | 1 | 22.909 | 1.1 | 0.34 |
| D | 0.008 | 1 | 0.008 | 6.8 | 0.04 | 330.352 | 1 | 330.352 | 15.2 | 0.01 |
| E | 0.105 | 1 | 0.105 | 85.5 | 0.00 | 2862.918 | 1 | 2862.918 | 131.6 | 0.00 |
| F | 0.010 | 1 | 0.010 | 8.1 | 0.03 | 0.482 | 1 | 0.482 | 0.0 | 0.89 |
| BD | 0.000 | 1 | 0.000 | 0.4 | 0.56 | 6.631 | 1 | 6.631 | 0.3 | 0.60 |
| BE | 0.001 | 1 | 0.001 | 0.4 | 0.53 | 18.503 | 1 | 18.503 | 0.9 | 0.39 |
| BF | 0.003 | 1 | 0.003 | 2.5 | 0.17 | 3.061 | 1 | 3.061 | 0.1 | 0.72 |
| DE | 0.004 | 1 | 0.004 | 3.1 | 0.13 | 48.154 | 1 | 48.154 | 2.2 | 0.19 |
| DF | 0.000 | 1 | 0.000 | 0.4 | 0.55 | 23.536 | 1 | 23.536 | 1.1 | 0.34 |
| EF | 0.001 | 1 | 0.001 | 0.8 | 0.40 | 6.588 | 1 | 6.588 | 0.3 | 0.60 |
| B2 | 0.000 | 1 | 0.000 | 0.0 | 0.88 | 0.322 | 1 | 0.322 | 0.0 | 0.91 |
| D2 | 0.054 | 1 | 0.054 | 44.4 | 0.00 | 25.041 | 1 | 25.041 | 1.2 | 0.32 |
| E2 | 0.021 | 1 | 0.021 | 17.2 | 0.01 | 108.858 | 1 | 108.858 | 5.0 | 0.07 |
| F2 | 0.000 | 1 | 0.000 | 0.3 | 0.61 | 31.330 | 1 | 31.330 | 1.4 | 0.28 |
| Lack of Fit | 0.007 | 2 | 0.004 | 130.528 | 2 | 65.264 | ||||
| Pure Error | 0 | 4 | 0 | 0 | 4 | 0 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Zheng, Z.; Tan, R.; Liu, W.; Zhang, Z. Modeling Shearing and Bending Behavior of Kiwifruit Branches Using the Discrete Element Method. Appl. Sci. 2024, 14, 10920. https://doi.org/10.3390/app142310920
Zhao H, Zheng Z, Tan R, Liu W, Zhang Z. Modeling Shearing and Bending Behavior of Kiwifruit Branches Using the Discrete Element Method. Applied Sciences. 2024; 14(23):10920. https://doi.org/10.3390/app142310920
Chicago/Turabian StyleZhao, Hongbo, Zhiqi Zheng, Ruihong Tan, Wenzheng Liu, and Zhiqiang Zhang. 2024. "Modeling Shearing and Bending Behavior of Kiwifruit Branches Using the Discrete Element Method" Applied Sciences 14, no. 23: 10920. https://doi.org/10.3390/app142310920
APA StyleZhao, H., Zheng, Z., Tan, R., Liu, W., & Zhang, Z. (2024). Modeling Shearing and Bending Behavior of Kiwifruit Branches Using the Discrete Element Method. Applied Sciences, 14(23), 10920. https://doi.org/10.3390/app142310920





