Optimal Configuration of Soft Open Point and Energy Storage Based on Snowflake-Shaped Grid Characteristics and Sensitivity Analysis
Abstract
1. Introduction
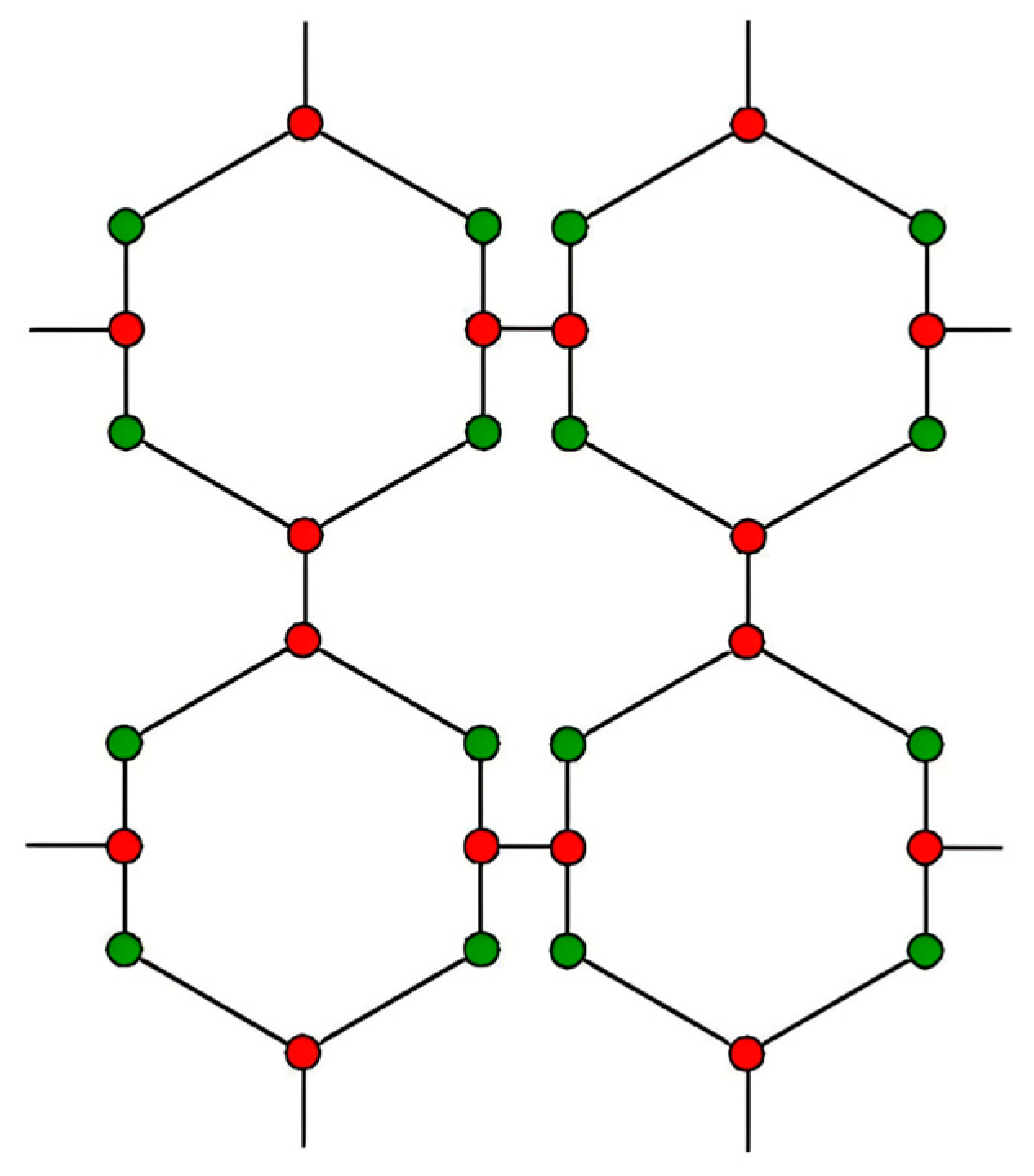
2. SOP Siting Based on the Characteristics of a Snowflake-Shaped Grid Structure
3. Energy Storage Siting Based on Sensitivity Analysis
4. SOP and Energy Storage Optimization Configuration Model
4.1. Objective Function
4.2. Constraint Condition
4.2.1. System Power Flow Constraints
4.2.2. System Operation Constraints
4.2.3. SOP Operation Constraints
4.2.4. Energy Storage Operation Constraints
5. Solution Algorithm and Process
6. Example Analysis
6.1. Example Introduction
6.2. Analysis of SOP and Energy Storage Siting Results
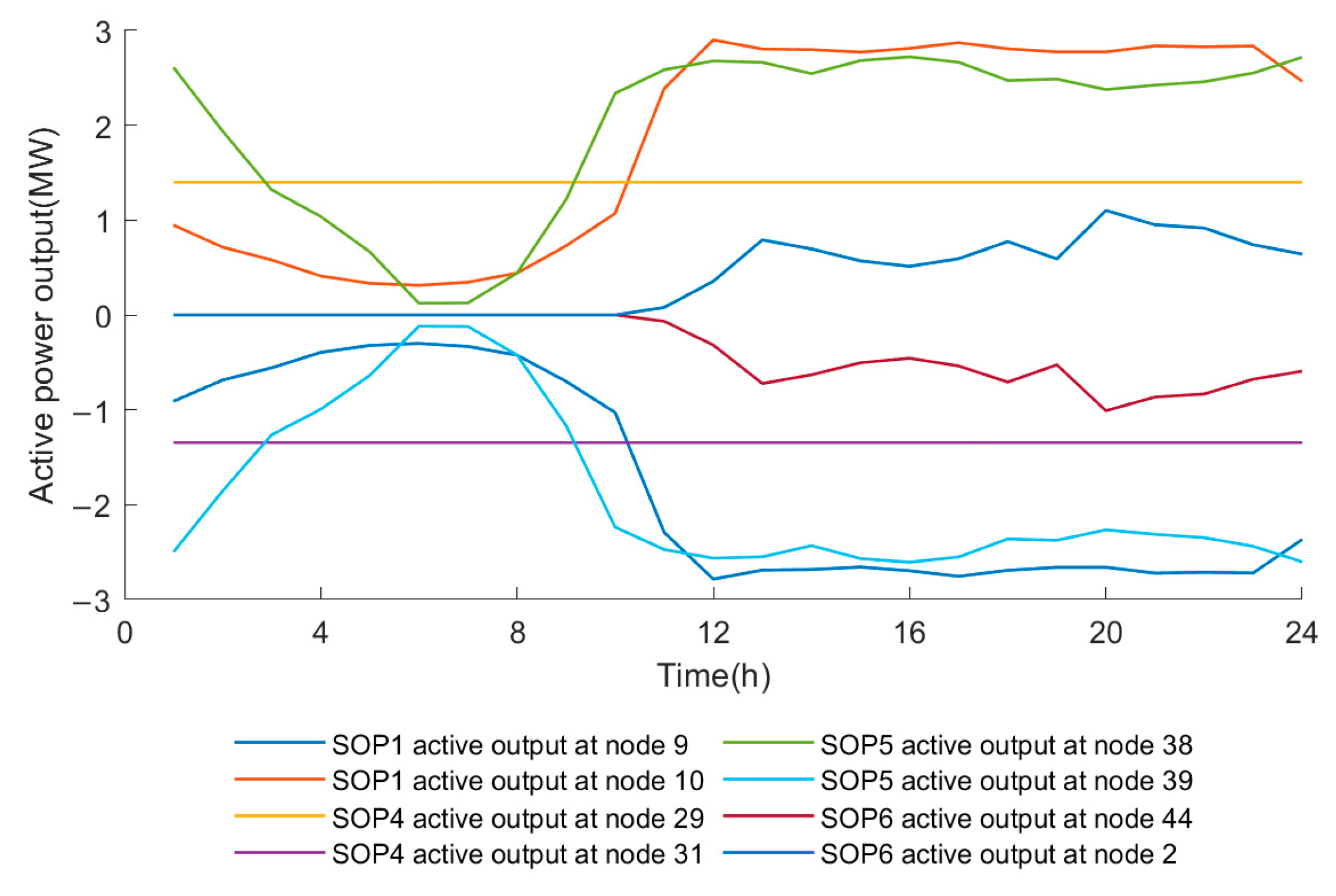
6.3. Analysis of SOP and Energy Storage Optimization Configuration Results
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Correction Statement
Abbreviations
| Symbols | |
| the voltage change | |
| the active power change | |
| the reactive power change | |
| voltage | |
| phase angle | |
| the voltage variation of node j | |
| the active power change of node i | |
| the reactive power change of node i | |
| the sensitivity of the active power change of node i to the voltage of node j | |
| the sensitivity of the reactive power change of node i to the voltage of node j | |
| the sensitivity of power changes at node i to the voltage at node j | |
| the sensitivity weight coefficients for active voltage | |
| the sensitivity weight coefficients for reactive voltage | |
| the ideal point | |
| the actual value of the ith evaluated object | |
| the Euclidean distance of the ith evaluated object | |
| the weight coefficient of the jth evaluation indicator | |
| the calculated value of the jth evaluation indicator for the ith evaluated object | |
| the ideal point for the jth evaluation metric | |
| the power factor angle of load growth | |
| the active power growth coefficient | |
| the reactive power growth coefficient | |
| the annual comprehensive cost of SOP and energy storage | |
| the load balancing degree | |
| the normalized values of | |
| the normalized values of | |
| the weight of in the objective function | |
| the weight of in the objective function | |
| the maximum values obtained when is solved separately | |
| the maximum values obtained when is solved separately | |
| the minimum values obtained when solving separately | |
| the minimum values obtained when solving separately | |
| the investment cost of SOP | |
| the cost of SOP operation and maintenance | |
| the investment cost of energy storage | |
| the cost of energy storage operation and maintenance | |
| the cost of power supply loss in the distribution network | |
| the SOP investment discount rate | |
| the service life of SOP | |
| the number of SOP installations to be selected | |
| the unit capacity investment cost of the kth SOP | |
| the capacity of the kth SOP | |
| the coefficient of operation and maintenance costs | |
| the discount rate for energy storage investment | |
| the service life of energy storage | |
| the number of energy storage installations to be selected | |
| the investment cost per unit capacity of the kth energy storage | |
| the capacity of the kth energy storage | |
| the operating and maintenance cost of the kth energy storage unit’s rated power | |
| the rated power of the kth energy storage; c is the electricity price | |
| the total number of time periods | |
| the collection of all branches in the distribution system | |
| the resistance of the branch ij | |
| the current amplitude of branch ij during the tth time period | |
| the total number of nodes in the distribution system | |
| the active power loss of SOP at node i during the tth time period | |
| the total number of power nodes in the distribution system | |
| the active power output of the ith power node during the tth time period | |
| the average active power output of all power nodes in the distribution system over one day | |
| the reactance of branch ij | |
| the active power flowing from node i to node j on the branch | |
| the reactive power flowing from node i to node j on the branch | |
| the sum of active power injected into node i | |
| the active power injected by the energy storage at node i | |
| the active power injected by the SOP at node i | |
| the active power consumed by the load at node i | |
| the sum of the reactive power injected at node i | |
| the reactive power injected by the energy storage at node i | |
| the reactive power injected by the SOP at node i | |
| the reactive power consumed by the load at node i | |
| the minimum allowable node voltage values of the system | |
| the maximum allowable node voltage values of the system | |
| the maximum allowable branch current value of the system | |
| the loss of SOP connected to node i | |
| the loss coefficient of SOP connected to node i | |
| the capacity of SOP connected to node i | |
| the charging power of energy storage at time t | |
| the discharging power of energy storage at time t | |
| the charging states of energy storage at time t | |
| the discharging states of energy storage at time t | |
| the maximum charging and discharging power of energy storage | |
| the state of charge of energy storage at time t | |
| the charging efficiency of energy storage | |
| the discharging efficiency of energy storage | |
| the minimum values of the energy storage state of charge | |
| the maximum values of the energy storage state of charge | |
References
- Zhuo, Z.Y.; Zhang, N. Key Technologies and Developing Challenges of Power System with High Proportion of Renewable Energy. Autom. Electr. Power Syst. 2021, 45, 171–191. [Google Scholar]
- Zhang, Z.G.; Kang, C.Q. Challenges and Prospects for Constructing the New-type Power System Towards a Carbon Neutrality Future. Proc. CSEE 2022, 42, 2806–2819. [Google Scholar]
- Wang, C.S.; Wang, S.Y. Economy and Reliability Analysis of Different Connection Modes in MV Distribution Systems. Autom. Electr. Power Syst. 2002, 26, 34–39. [Google Scholar]
- Ruan, Q.T.; Xie, W. Research and Practice on the Upgrading for Diamond Distribution Network. Electr. Power 2020, 53, 1–7. [Google Scholar]
- Wang, Z.; Duan, J.L. Exploration on Snow-shaped Grid Structure of Urban Medium Voltage Distribution Network. Proc. CSU-EPSA 2024, 36, 51–60. [Google Scholar]
- Xiong, Z.Y.; Chen, T.H. Optimal Allocation of Soft Open Point in Active Distribution Network Based on Improved Sensitivity Analysis. Autom. Electr. Power Syst. 2021, 45, 129–137. [Google Scholar]
- Ge, S.Y.; Zhou, C.X. Soft Open Point Planning for Active Distribution Network Considering Influence of Access Mode Between Feeder Loops and Reliability. Autom. Electr. Power Syst. 2021, 45, 120–128. [Google Scholar]
- Wang, C.S.; Song, G.Y. Optimal Configuration of Soft Open Point for Active Distribution Network Considering the Characteristics of Distributed Generation. Proc. CSEE 2017, 37, 1889–1897. [Google Scholar]
- Wang, H.Z.; Zhao, Q.Y. Improvement method of photovoltaic consumption capacity of AC/DC hybrid distribution network considering the time-of-use electricity price and SOP collaboration. Distrib. Util. 2023, 40, 1–9. [Google Scholar]
- Guo, Y.; Wu, Q. MPC-based Coordinated Voltage Regulation for Distribution Networks with Distributed Generation and Energy Storage System. IEEE Trans. Sustain. Energy 2019, 10, 1731–1739. [Google Scholar] [CrossRef]
- Chen, B.; Chen, C. Multi-time Step Service Restoration for Advanced Distribution Systems and Microgrids. IEEE Trans. Smart Grid 2018, 9, 6793–6805. [Google Scholar] [CrossRef]
- Liu, X.; Aichhorn, A. Coordinated Control of Distributed Energy Storage System with Tap Changer Transformers for Voltage Rise Mitigation under High Photovoltaic Penetration. IEEE Trans. Smart Grid 2012, 3, 897–906. [Google Scholar] [CrossRef]
- Bai, L.; Jiang, T. Distributed Energy Storage Planning in Soft Open Point Based Active Distribution Networks Incorporating Network Reconfiguration and DG Reactive Power Capability. Appl. Energ. 2018, 210, 1082–1091. [Google Scholar] [CrossRef]
- Sun, C.B.; Li, J.R. Two-stage Optimization Method of Soft Open Point and Energy Storage System in Distribution Network Based on Interval Optimization. High Volt. Eng. 2021, 47, 45–54. [Google Scholar]
- Li, J.; Liu, T.Q. Multi-criterion Integrated-sensitivity Voltage Stability Evaluation Index Based on Ideal Point Method. Electr. Power Autom. Equip. 2014, 34, 108–112. [Google Scholar]
- Gao, B.; Morison, G.K. Towards the Development of A Systematic Approach for Voltage Stability Assessment of Largescale Power Systems. IEEE Trans. Power Syst. 1996, 11, 1314–1324. [Google Scholar] [CrossRef]
- Song, G.Y. Operation and Planning Method of Soft Open Point for Multiple Scenarios in Active Distribution Network. Ph.D. Thesis, Tianjin University, Tianjin, China, 2017. [Google Scholar]
- Li, P.; Ji, H. Coordinated Control Method of Voltage and Reactive Power for Active Distribution Networks Based on Soft Open Point. IEEE Trans. Sustain. Energy 2017, 4, 1430–1442. [Google Scholar] [CrossRef]



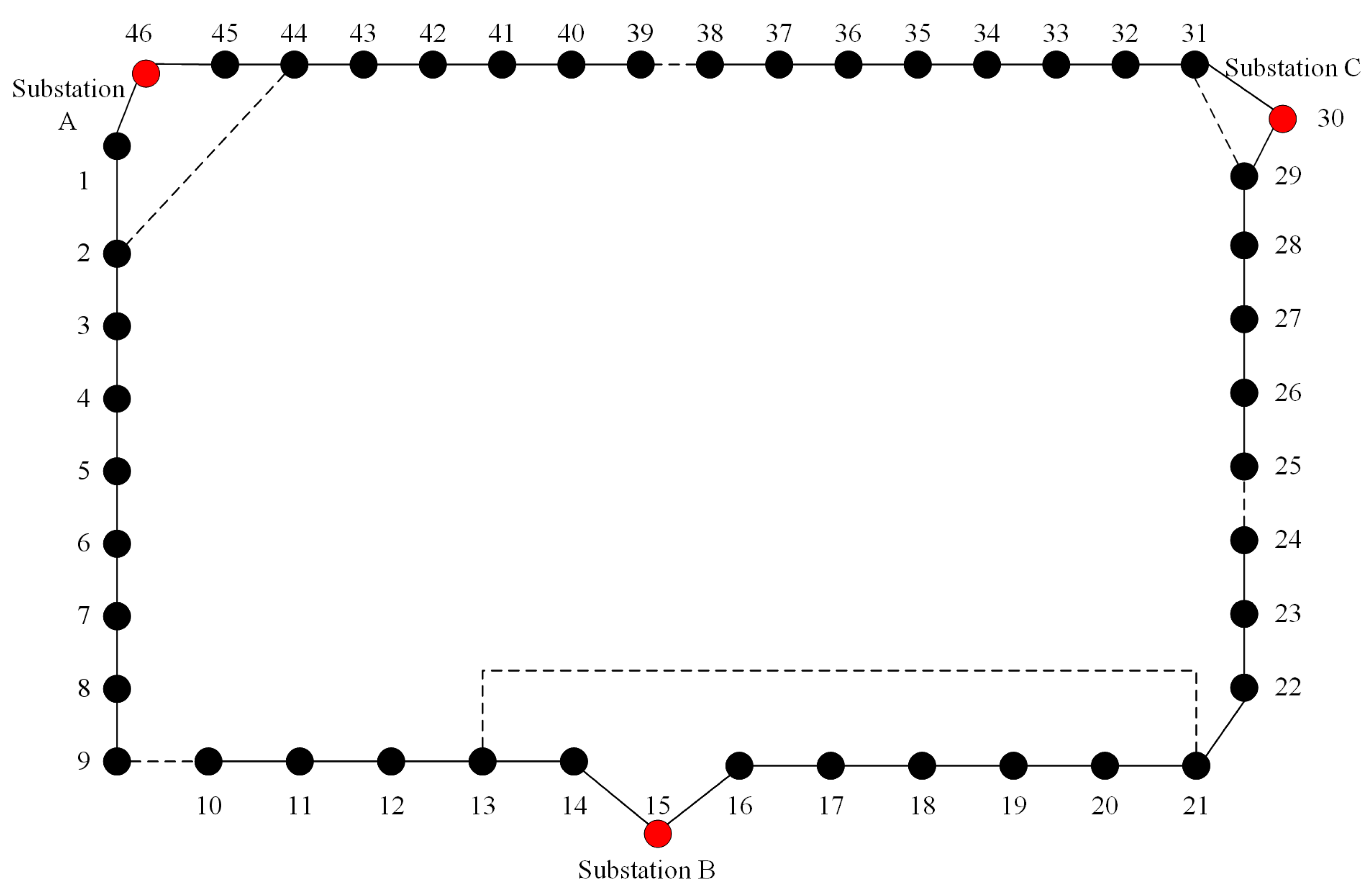




| Head Node | End Node | Resistance/Ω | Reactance/Ω |
|---|---|---|---|
| 1 | 2 | 0.1633 | 0.1975 |
| 2 | 3 | 0.0294 | 0.0356 |
| 3 | 4 | 0.0189 | 0.0228 |
| 4 | 5 | 0.0132 | 0.0160 |
| 5 | 6 | 0.0244 | 0.0295 |
| 6 | 7 | 0.0170 | 0.0206 |
| 7 | 8 | 0.0212 | 0.0256 |
| 8 | 9 | 0.0256 | 0.0309 |
| 48 | 14 | 0.0679 | 0.1000 |
| 14 | 13 | 0.0980 | 0.1186 |
| 13 | 12 | 0.0253 | 0.0306 |
| 12 | 11 | 0.0300 | 0.0363 |
| 11 | 10 | 0.0186 | 0.0225 |
| 15 | 16 | 0.0679 | 0.1000 |
| 16 | 17 | 0.0566 | 0.0684 |
| 17 | 18 | 0.0211 | 0.0255 |
| 18 | 19 | 0.0072 | 0.0087 |
| 19 | 20 | 0.0083 | 0.0100 |
| 20 | 21 | 0.0124 | 0.0150 |
| 21 | 22 | 0.0253 | 0.0306 |
| 22 | 23 | 0.0300 | 0.0363 |
| 23 | 24 | 0.0186 | 0.0225 |
| 30 | 29 | 0.0699 | 0.1029 |
| 29 | 28 | 0.0083 | 0.0100 |
| 28 | 27 | 0.0232 | 0.0281 |
| 27 | 26 | 0.0202 | 0.0244 |
| 26 | 25 | 0.0142 | 0.0171 |
| 49 | 31 | 0.0439 | 0.0646 |
| 31 | 32 | 0.0566 | 0.0684 |
| 32 | 33 | 0.0189 | 0.0228 |
| 33 | 34 | 0.0132 | 0.0160 |
| 34 | 35 | 0.0244 | 0.0295 |
| 35 | 36 | 0.0166 | 0.0201 |
| 36 | 37 | 0.0212 | 0.0256 |
| 37 | 38 | 0.0260 | 0.0315 |
| 47 | 45 | 0.0637 | 0.0938 |
| 45 | 44 | 0.1616 | 0.1954 |
| 44 | 43 | 0.0535 | 0.0648 |
| 43 | 42 | 0.0142 | 0.0171 |
| 42 | 41 | 0.0202 | 0.0244 |
| 41 | 40 | 0.0277 | 0.0336 |
| 40 | 39 | 0.0083 | 0.0100 |
| 46 | 1 | 0.0511 | 0.0752 |
| Access Plan | Scheme 1 | Scheme 2 | Scheme 3 |
|---|---|---|---|
| Node number for SOP access | 9–10 29–31 38–39 44–2 | 9–10 29–31 38–39 44–2 | 9–10 13–21 24–25 29–31 38–39 44–2 |
| SOP access capacity/kVA | 2600 100 3000 2600 | 2900 1400 3000 2300 | 3000 1200 700 2300 3000 2300 |
| Node number for energy storage access | 39 40 | 40 | 38 40 |
| Energy storage access capacity/kVA | 2.52 1650 | 1670.02 | 770.09 1696.35 |
| Annual investment cost of equipment/CNY 10,000 | 108.1 | 121.59 | 162.49 |
| Annual operation and maintenance cost of equipment/CNY 10,000 | 8.32 | 9.62 | 12.52 |
| Annual power loss cost of distribution network/CNY 10,000 | 80.24 | 113.63 | 203.23 |
| Annual comprehensive cost/CNY 10,000 | 196.65 | 244.84 | 378.24 |
| Load balancing | 199.04 | 97.67 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Zhang, Z.; Luo, F.; Qiu, X.; Zhang, X.; Duan, J. Optimal Configuration of Soft Open Point and Energy Storage Based on Snowflake-Shaped Grid Characteristics and Sensitivity Analysis. Appl. Sci. 2024, 14, 10503. https://doi.org/10.3390/app142210503
Wang Z, Zhang Z, Luo F, Qiu X, Zhang X, Duan J. Optimal Configuration of Soft Open Point and Energy Storage Based on Snowflake-Shaped Grid Characteristics and Sensitivity Analysis. Applied Sciences. 2024; 14(22):10503. https://doi.org/10.3390/app142210503
Chicago/Turabian StyleWang, Zhe, Zhang Zhang, Fengzhang Luo, Xiaoyu Qiu, Xuefei Zhang, and Jiali Duan. 2024. "Optimal Configuration of Soft Open Point and Energy Storage Based on Snowflake-Shaped Grid Characteristics and Sensitivity Analysis" Applied Sciences 14, no. 22: 10503. https://doi.org/10.3390/app142210503
APA StyleWang, Z., Zhang, Z., Luo, F., Qiu, X., Zhang, X., & Duan, J. (2024). Optimal Configuration of Soft Open Point and Energy Storage Based on Snowflake-Shaped Grid Characteristics and Sensitivity Analysis. Applied Sciences, 14(22), 10503. https://doi.org/10.3390/app142210503







