Conservative Analysis of Transient Heat Transfer in Thermal Protection Systems with Interval Parameters
Abstract
1. Introduction
2. Transient Heat Transfer Equations with Interval Parameters
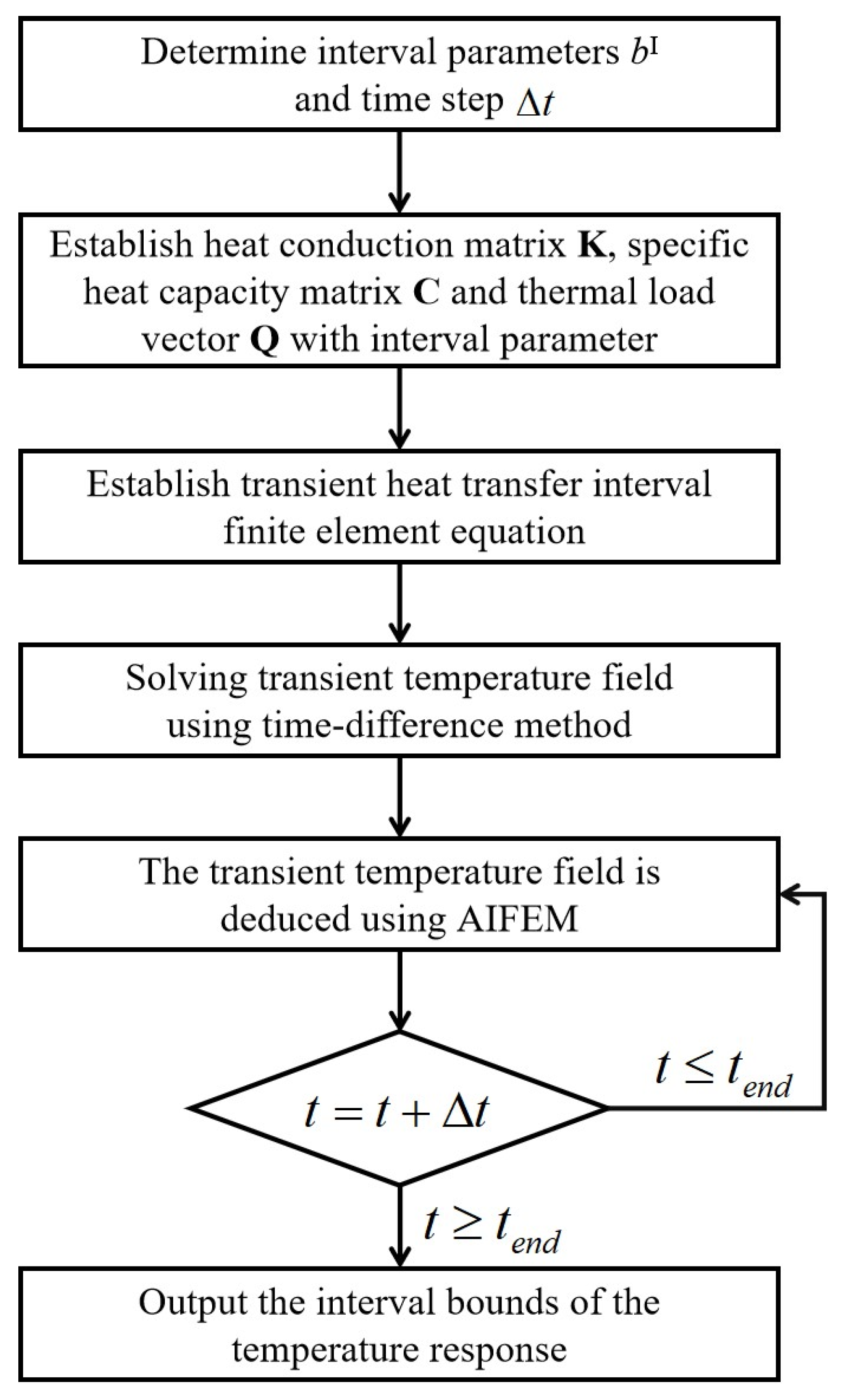
3. Affine Interval Finite Element Method
4. Numerical Examples
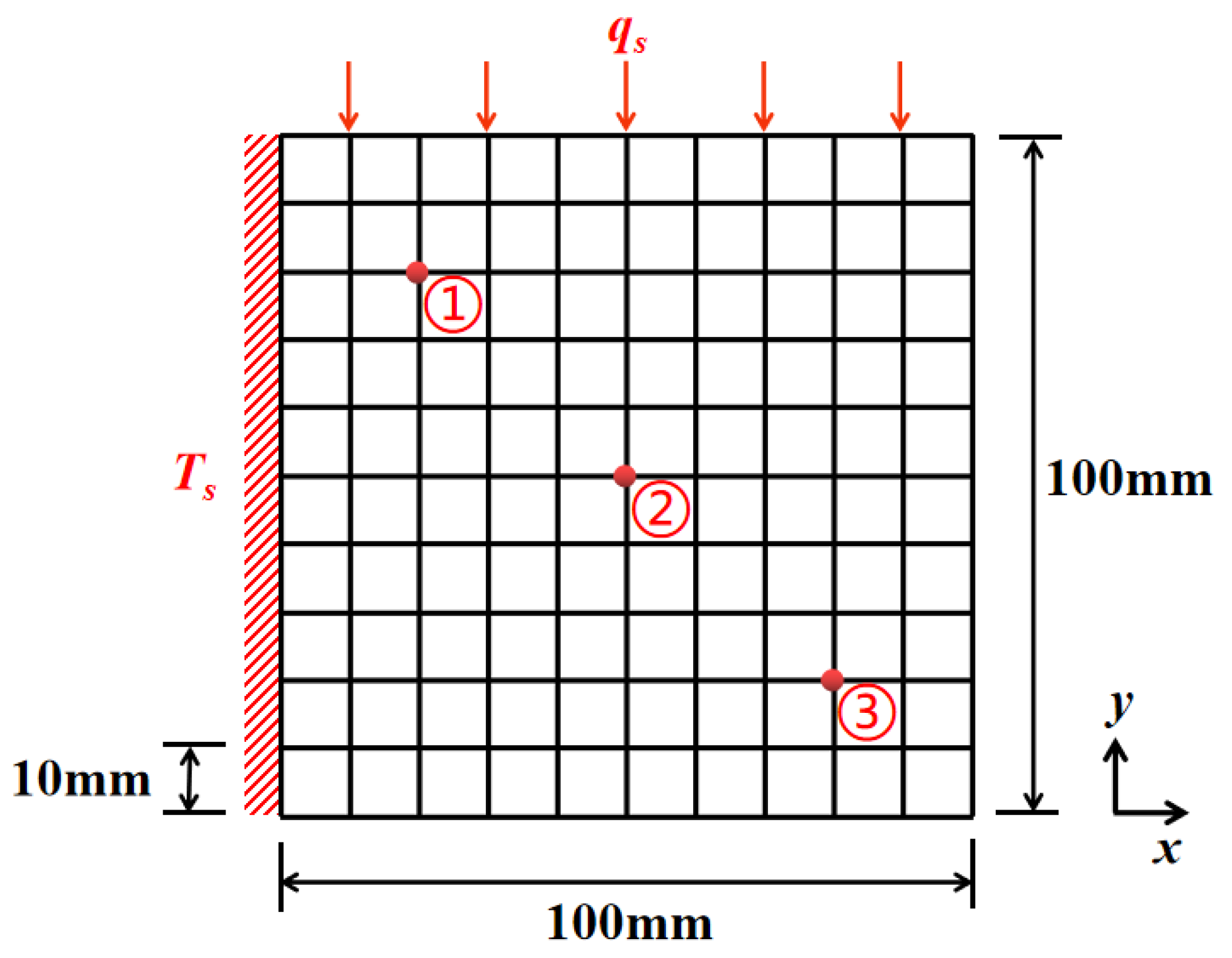
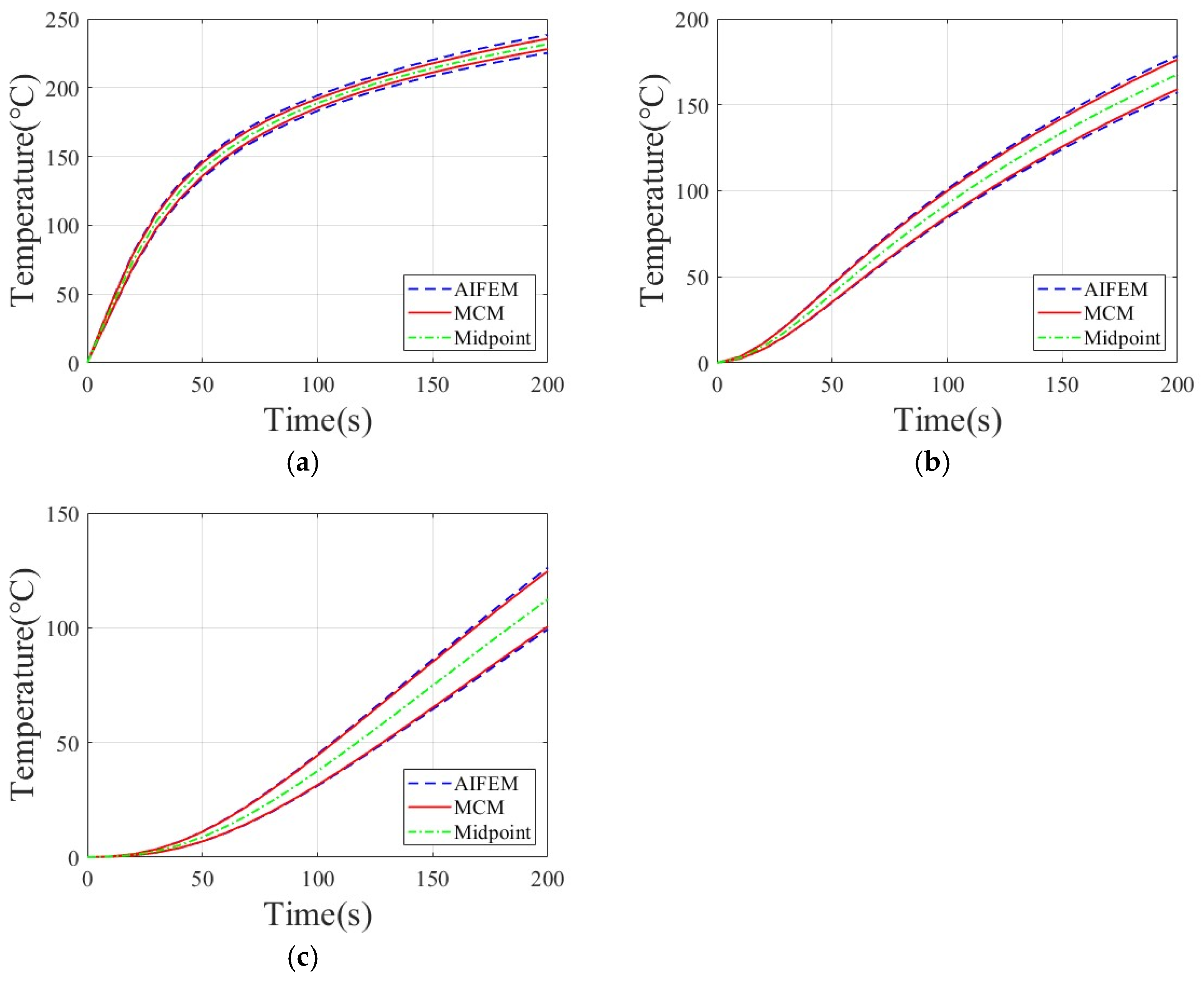
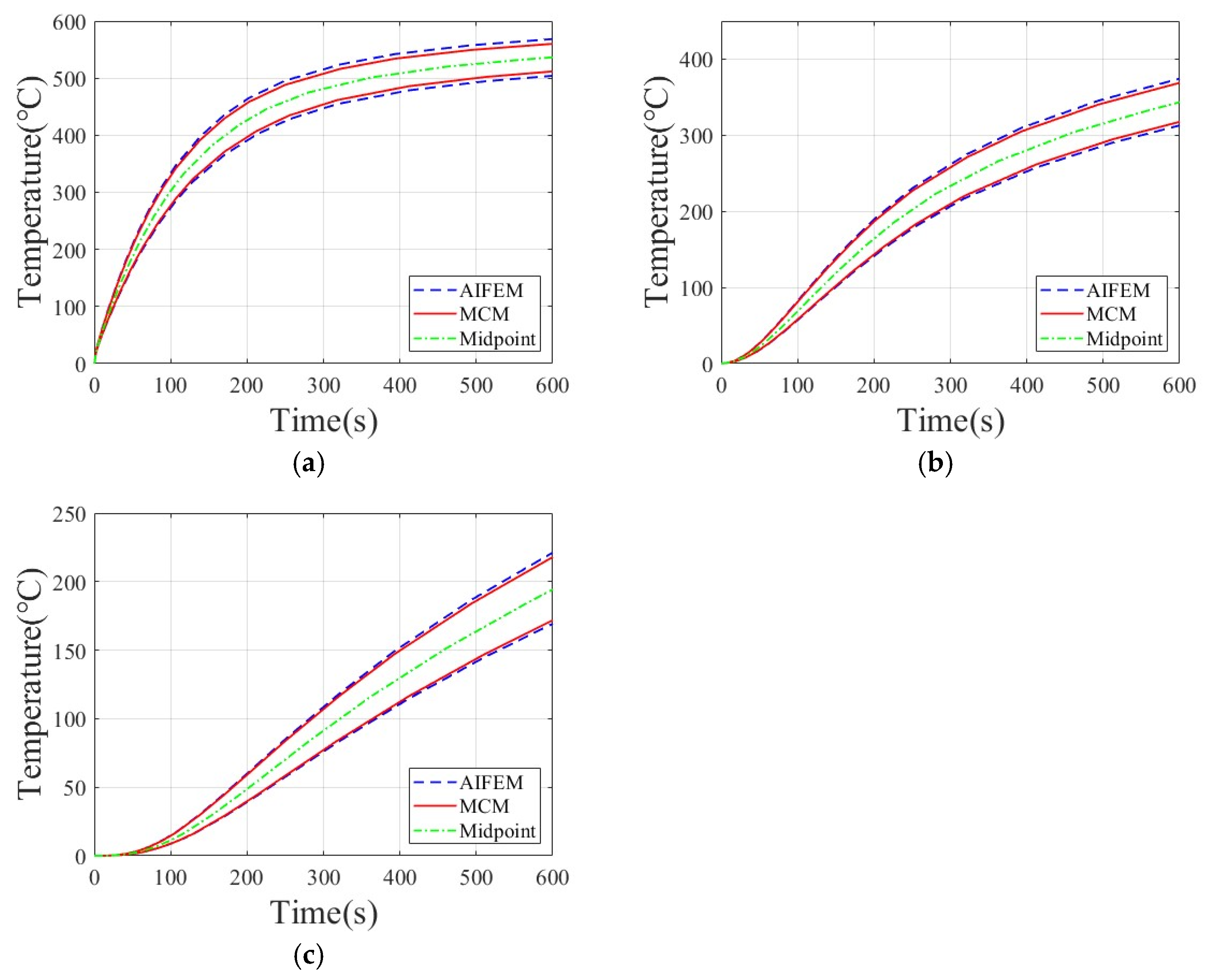
4.1. Plate Model
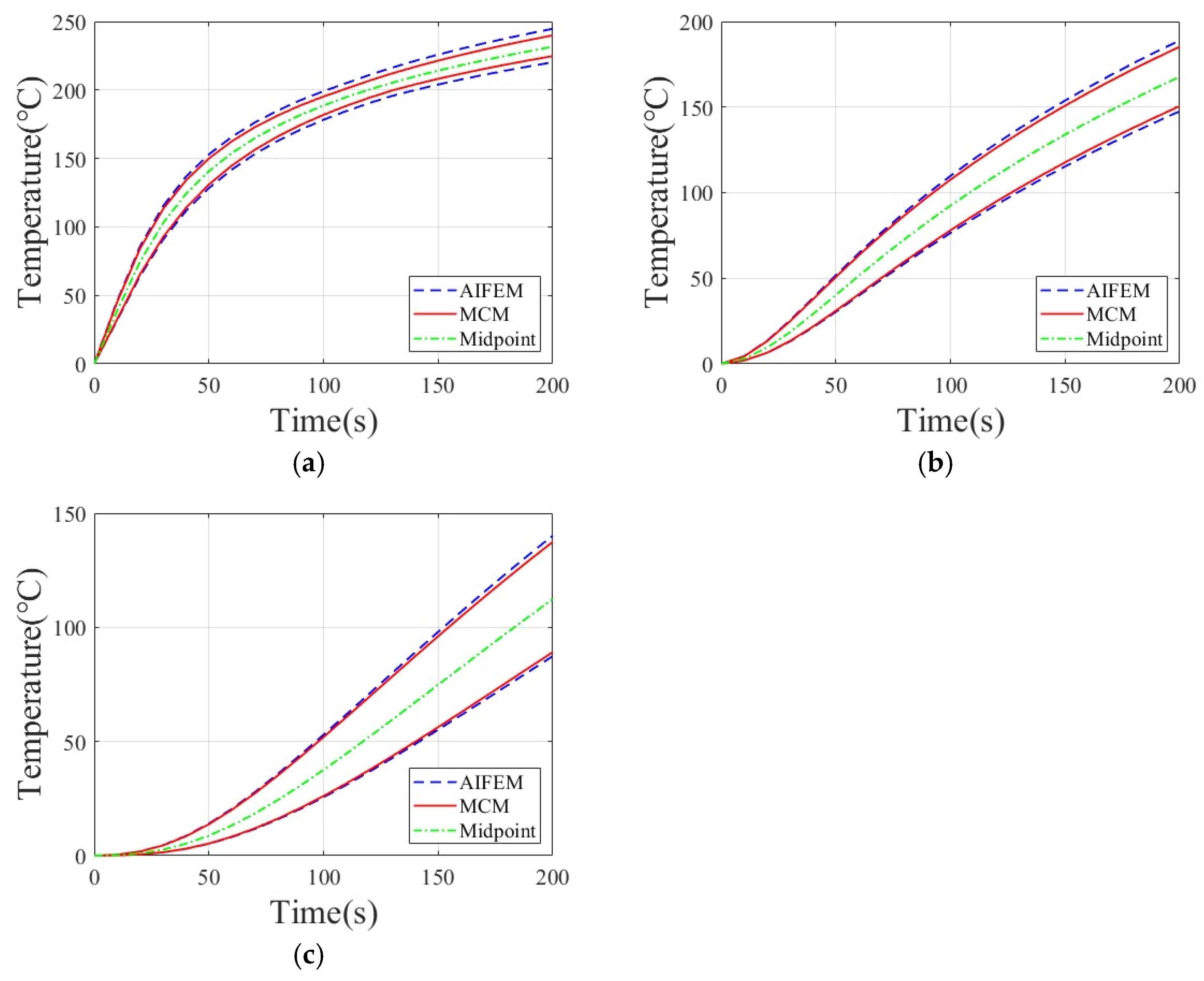
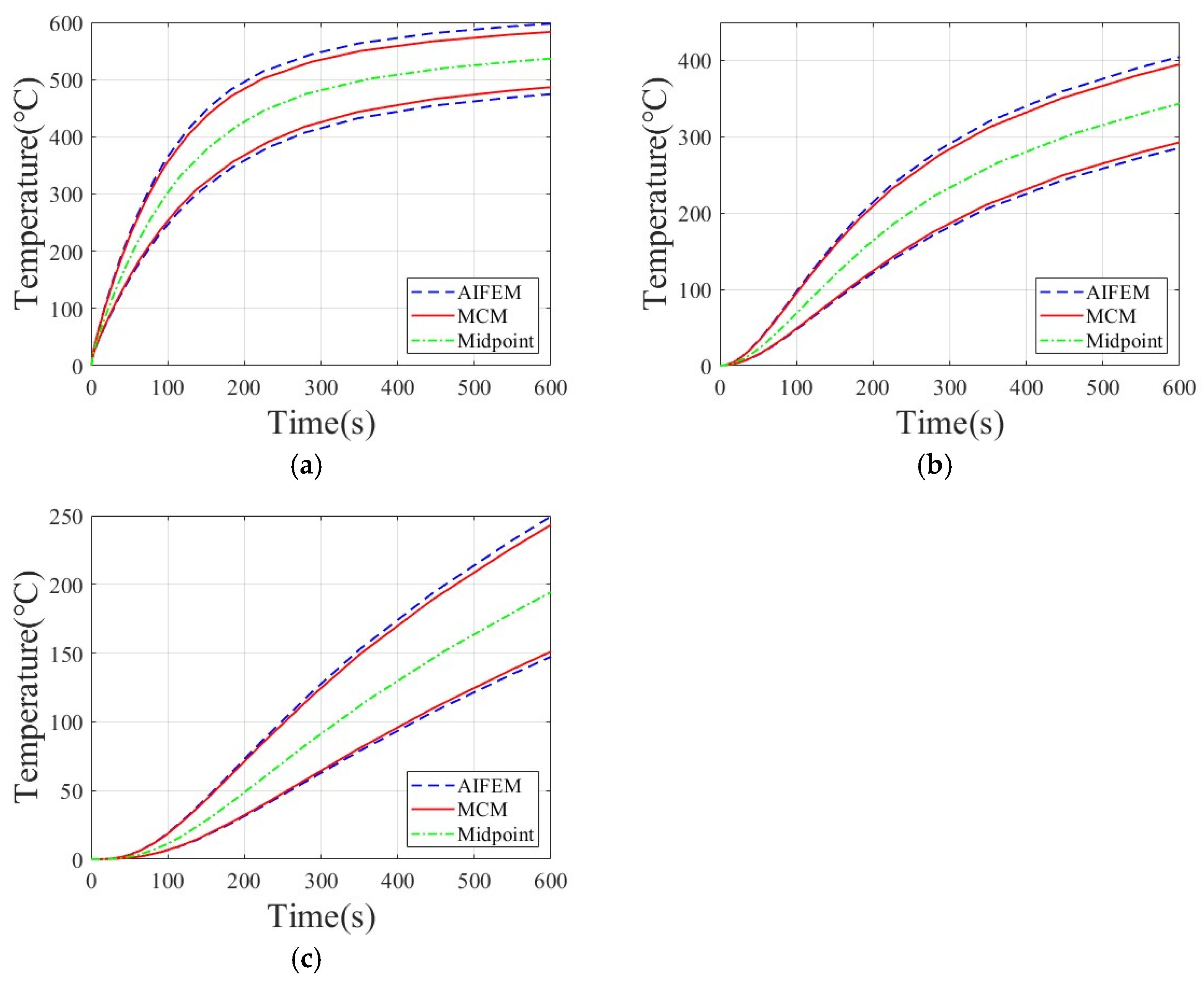
4.2. TPS Model
4.3. Comparison of Computing Efficiency between AIFEM and MCM
5. Conclusions
- (1)
- The uncertainty of the TPS in the transient heat transfer analysis had a significant impact on its temperature response, so it is necessary to analyze the uncertainty of TPSs.
- (2)
- The temperature response bounds obtained by the AIFEM were wider than those obtained by the MCM, and the AIFEM can generally obtain a more conservative temperature response. The conservative AIFEM is a safe approach in uncertain TPS designs under transient heat transfer conditions.
- (3)
- The temperature response bounds provided by the MCM offered a real solution, but their calculation was very time-consuming. The AIFEM effectively improved the calculation efficiency. From the perspective of calculation efficiency, the AIFEM is a reasonable method to predict the temperature response of uncertain TPSs under transient heat transfer.
- (4)
- Given the uncertainty of TPS design under transient heat transfer conditions, the AIFEM can be used as an efficient and safe method. Next, the authors will try to study the conservatism of uncertain TPS thermal stress based on the AIFEM, and when conservative analysis is needed in other fields, try to use this method for research.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Natali, M.; Kenny, J.M.; Torre, L. Science and technology of polymeric ablative materials for thermal protection systems and propulsion devices: A review. Prog. Mater. Sci. 2016, 84, 192–275. [Google Scholar] [CrossRef]
- Uyanna, O.; Najafi, H. Thermal protection systems for space vehicles: A review on technology development, current challenges and future prospects. Acta Astronaut. 2020, 176, 341–356. [Google Scholar] [CrossRef]
- Le, V.T.; San, H.N.; Goo, N.S. Advanced sandwich structures for thermal protection systems in hypersonic vehicles: A review. Compos. Part B-Eng. 2021, 226, 109301. [Google Scholar] [CrossRef]
- Biamino, S.; Antonini, A.; Eisenmenger-Sittner, C.; Fuso, L.; Pavese, M.; Fino, P.; Bauer, E.; Badini, C. Multilayer SiC for thermal protection system of space vehicles with decreased thermal conductivity through the thickness. J. Eur. Ceram. Soc. 2010, 30, 1833–1840. [Google Scholar] [CrossRef]
- Palmer, G.; Kontinos, D.; Sherman, B. Surface Heating Effects of X-33 Vehicle Thermal-Protection-System Panel Bowing. J. Spacecr. Rocket. 1999, 36, 836–841. [Google Scholar] [CrossRef]
- Wei, K.; Cheng, X.; Mo, F.; Wen, W.; Fang, D. Design and analysis of integrated thermal protection system based on lightweight C/SiC pyramidal lattice core sandwich panel. Mater. Des. 2016, 111, 435–444. [Google Scholar] [CrossRef]
- Wang, X.; Wei, K.; Tao, Y.; Yang, X.; Zhou, H.; He, R.; Fang, D. Thermal protection system integrating graded insulation materials and multilayer ceramic matrix composite cellular sandwich panels. Compos. Struct. 2019, 209, 523–534. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, L.; He, R.; Ma, Y.; Zhang, K.; Bai, X.; Xu, B.; Chen, Y. Integrated thermal protection system based on C/SiC composite corrugated core sandwich plane structure. Aerosp. Sci. Technol. 2019, 91, 607–616. [Google Scholar] [CrossRef]
- Ding, C.; Liu, X.; Xie, F.; Du, C.; Wang, Y. Heat transfer and pyrolysis gas flow characteristics of light-weight ablative thermal protection system in the blunt body. Int. J. Therm. Sci. 2023, 186, 108122. [Google Scholar] [CrossRef]
- Rivier, M.; Lachaud, J.; Congedo, P.M. Ablative thermal protection system under uncertainties including pyrolysis gas composition. Aerosp. Sci. Technol. 2019, 84, 1059–1069. [Google Scholar] [CrossRef]
- Brune, A.J.; West, T.K.; Hosder, S. Uncertainty quantification of planetary entry technologies. Prog. Aerosp. Sci. 2019, 111, 100574. [Google Scholar] [CrossRef]
- Moens, D.; Vandepitte, D. Recent advances in non-probabilistic approaches for non-deterministic dynamic finite element analysis. Arch. Comput. Method E. 2006, 13, 389–464. [Google Scholar] [CrossRef]
- Xia, B.; Yu, D. Interval analysis of acoustic field with uncertain-but-bounded parameters. Comput. Struct. 2012, 112, 235–244. [Google Scholar] [CrossRef]
- Moens, D.; Hanss, M. Non-probabilistic finite element analysis for parametric uncertainty treatment in applied mechanics: Recent advances. Finite Elem. Anal. Des. 2011, 47, 4–16. [Google Scholar] [CrossRef]
- Jiang, C.; Fu, C.; Ni, B.; Han, X. Interval arithmetic operations for uncertainty analysis with correlated interval variables. Acta Mech. Sin. 2016, 32, 743–752. [Google Scholar] [CrossRef]
- Qiu, Z.; Elishakoff, I. Antioptimization of structures with large uncertain-but-non-random parameters via interval analysis. Comput. Method Appl. Mech. Eng. 1998, 152, 361–372. [Google Scholar] [CrossRef]
- Deng, Z.; Guo, Z.; Zhang, X. Non-probabilistic set-theoretic models for transient heat conduction of thermal protection systems with uncertain parameters. Appl. Therm. Eng. 2016, 95, 10–17. [Google Scholar] [CrossRef]
- Sofi, A.; Romeo, E.; Barrera, O.; Cocks, A. An interval finite element method for the analysis of structures with spatially varying uncertainties. Adv. Eng. Softw. 2019, 128, 1–19. [Google Scholar] [CrossRef]
- Xiang, Y.; Shi, Z. Interval Analysis of Interior Acoustic Field with Element-By-Element-Based Interval Finite-Element Method. J. Eng. Mech.-ASCE 2021, 147, 04021085. [Google Scholar] [CrossRef]
- Degrauwe, D.; Lombaert, G.; Roeck, G.D. Improving interval analysis in finite element calculations by means of affine arithmetic. Comput. Struct. 2010, 88, 247–254. [Google Scholar] [CrossRef]
- He, F.; Shi, Z.; Zhang, Z.; Qian, D.; Feng, X. Antioptimization of mass-in-mass acoustic metamaterials based on interval analysis. Mech. Syst. Signal Process. 2023, 205, 110855. [Google Scholar] [CrossRef]

| Uncertainty Level (%) | Bound | c (J/(kg·°C)) | λ (W/(m·°C)) |
|---|---|---|---|
| 5 | UB | 210 | 10.5 |
| LB | 190 | 9.5 | |
| 10 | UB | 220 | 11 |
| LB | 180 | 9 |
| Uncertain Level (%) | Time (s) | Bound | AIFEM (°C) | MCM (°C) | Error (%) |
|---|---|---|---|---|---|
| 5 | 100 | UB | 101.71 | 99.90 | 1.81 |
| LB | 83.52 | 85.14 | 1.90 | ||
| 200 | UB | 179.61 | 176.42 | 1.81 | |
| LB | 156.16 | 159.11 | 1.85 | ||
| 10 | 100 | UB | 110.26 | 107.61 | 2.46 |
| LB | 76.08 | 78.02 | 2.49 | ||
| 200 | UB | 190.43 | 185.26 | 2.79 | |
| LB | 146.33 | 150.59 | 2.83 |
| Uncertainty Level (%) | Bound | c (J/(kg·°C)) | λ (W/(m·°C)) | qs (W/m2) | Ta (°C) |
|---|---|---|---|---|---|
| 5 | UB | 641.55 | 7.14 | 1.05 × 105 | −76 |
| LB | 580.45 | 6.46 | 0.95 × 105 | −84 | |
| 10 | UB | 672.10 | 7.48 | 1.1 × 105 | −72 |
| LB | 549.90 | 6.12 | 0.9 × 105 | −88 |
| Uncertain Level (%) | Time (s) | Bound | AIFEM (°C) | MCM (°C) | Error (%) |
|---|---|---|---|---|---|
| 5 | 300 | UB | 263.38 | 257.72 | 2.20 |
| LB | 205.23 | 209.73 | 2.15 | ||
| 600 | UB | 378.41 | 368.87 | 2.59 | |
| LB | 308.91 | 317.68 | 2.76 | ||
| 10 | 300 | UB | 292.03 | 283.80 | 2.90 |
| LB | 181.18 | 186.48 | 2.84 | ||
| 600 | UB | 406.84 | 394.97 | 3.01 | |
| LB | 283.73 | 292.75 | 3.08 |
| Method | Plate Model | TPS Model |
|---|---|---|
| AIFEM | 0.021 s | 1.208 s |
| MCM | 120.4 s | 13,246.9 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, X.; Shi, Z.; Yang, Z. Conservative Analysis of Transient Heat Transfer in Thermal Protection Systems with Interval Parameters. Appl. Sci. 2024, 14, 9446. https://doi.org/10.3390/app14209446
Feng X, Shi Z, Yang Z. Conservative Analysis of Transient Heat Transfer in Thermal Protection Systems with Interval Parameters. Applied Sciences. 2024; 14(20):9446. https://doi.org/10.3390/app14209446
Chicago/Turabian StyleFeng, Xuelei, Zhiyu Shi, and Zheng Yang. 2024. "Conservative Analysis of Transient Heat Transfer in Thermal Protection Systems with Interval Parameters" Applied Sciences 14, no. 20: 9446. https://doi.org/10.3390/app14209446
APA StyleFeng, X., Shi, Z., & Yang, Z. (2024). Conservative Analysis of Transient Heat Transfer in Thermal Protection Systems with Interval Parameters. Applied Sciences, 14(20), 9446. https://doi.org/10.3390/app14209446





