Stalling in Queuing Systems with Heterogeneous Channels
Abstract
1. Introduction
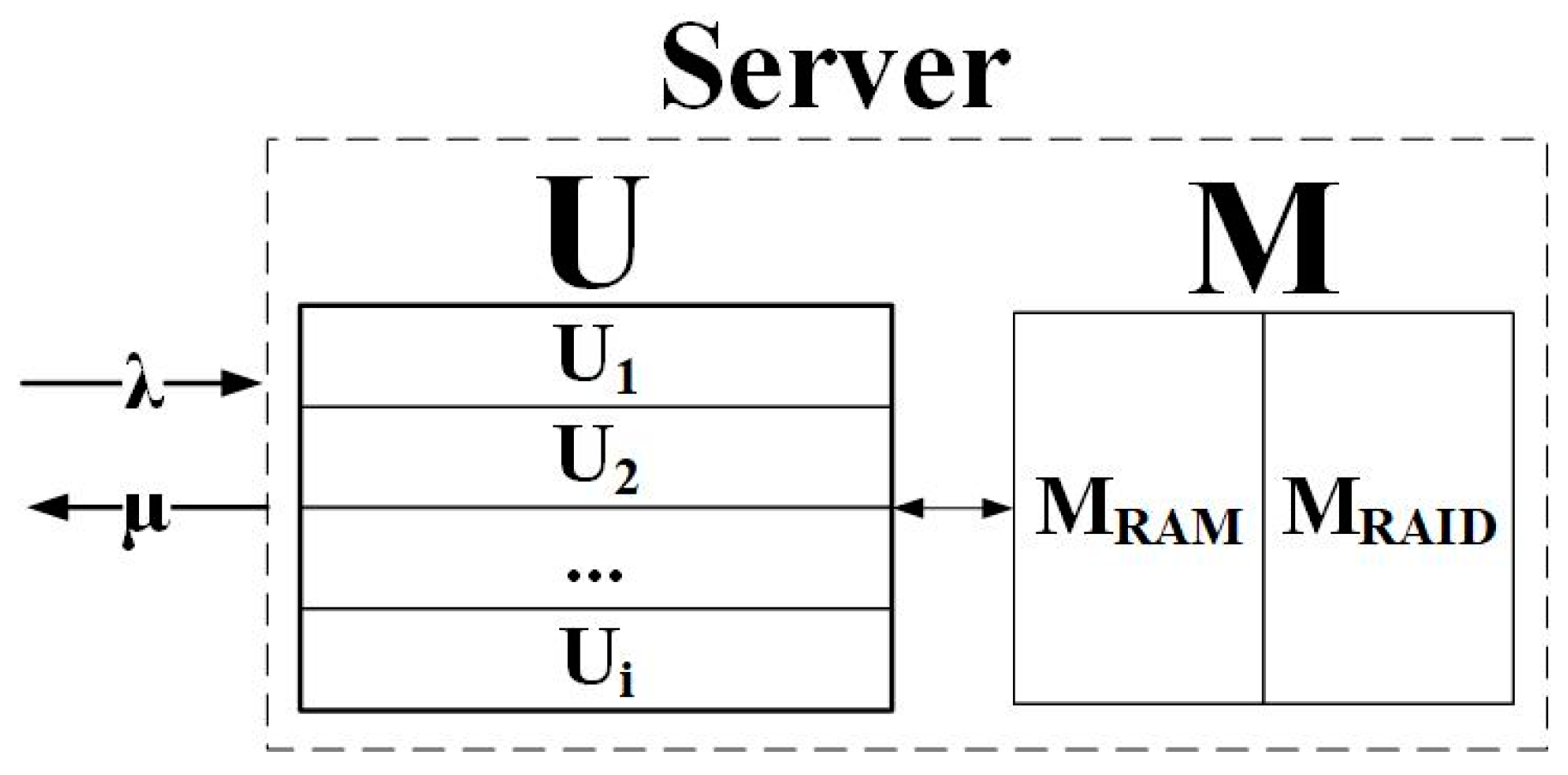
2. QS with Heterogeneous Channels and Stalling Buffer
2.1. State Graph of QS
2.2. Calculation of Steady-State Probabilities
- Coefficient of utilization of fast servers with switched-out slow channels ;
- Rate of capacity of fast and slow servers
- Stalling buffer length K;
- Waiting queue length M.
3. Queuing Characteristics and Optimization
3.1. Queueing Characteristics
3.2. Optimization of Stalling Buffer
- (i)
- (ii)
4. Applications
4.1. Modelling of Heterogeneous Server Cluster with Stalling Buffer
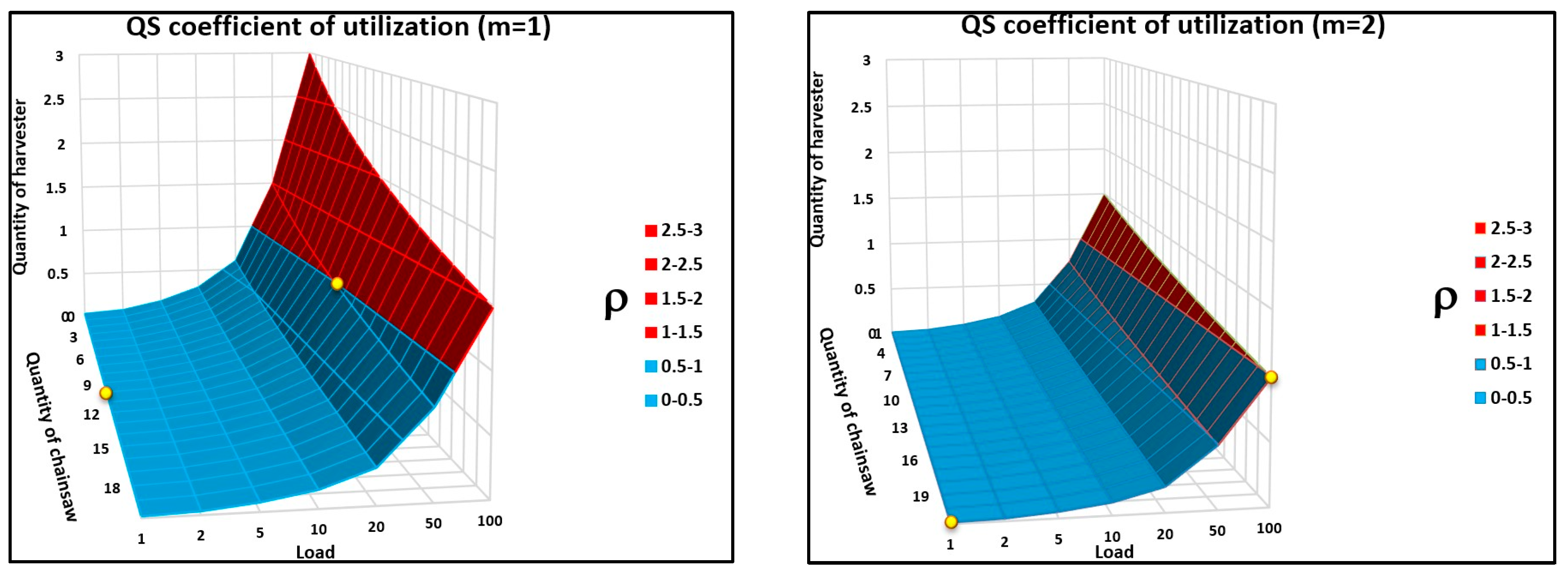
4.2. Modelling of Harvesters and Chainsaws Work Productivity
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Kleinrock, L. Queueing Systems, Volume 1: Theory; Wiley: New York, NY, USA, 2009. [Google Scholar]
- Larsen, R.L. Control of Multiple Exponential Servers with Application to Computer Systems. Ph.D. Thesis, University of Maryland at College Park, College Park, MD, USA, 1981. [Google Scholar]
- Rubinovitch, M. The slow server problem: A queue with stalling. J. Appl. Probab. 1985, 22, 879–892. [Google Scholar] [CrossRef]
- De Vericourt, F.; Zhou, Y.P. On the incomplete results for the heterogeneous server problem. Queueing Syst. 2006, 52, 189–191. [Google Scholar] [CrossRef]
- Goswami, V.; Samanta, S.K. Discrete-time bulk-service queue with two heterogeneous servers. Comput. Ind. Eng. 2009, 56, 1348–1356. [Google Scholar] [CrossRef]
- Rykov, V.V.E.; Efrosinin, D.V. On the slow server problem. Autom. Remote Control 2009, 70, 2013–2023. [Google Scholar] [CrossRef]
- Efrosinin, D.; Rykov, V. Heuristic solution for the optimal thresholds in a controllable multi-server heterogeneous queueing system without preemption. In Proceedings of the International Conference on Distributed Computer and Communication Networks, Moscow, Russia, 19–22 October 2015; pp. 238–252. [Google Scholar]
- Shi, C.; Li, Y.; Zhang, J.; Sun, Y.; Philip, S.Y. A survey of heterogeneous information network analysis. IEEE Trans. Knowl. Data Eng. 2017, 29, 17–37. [Google Scholar] [CrossRef]
- Kaklauskas, L.; Sakalauskas, L.; Denisovas, V. Stalling for solving slow server problem. RAIRO Oper. Res. 2019, 53, 1097–1107. [Google Scholar] [CrossRef]
- Kalyanaraman, R.; Hellen, A. M/M/2 Heterogeneous Server Queue with Variant Breakdown and with Discouraged Arrivals. Math. Stat. Eng. Appl. 2022, 71, 1447–1458. [Google Scholar]
- Kalyanaraman, R.; Hellen, A. A Two Heterogeneous Server Queue with Variant Breakdown and with Discouraged Arrivals, Balking. Neuro Quantol. 2022, 20, 4142. [Google Scholar]
- Elsayed, E.A. Multichannel queueing systems with ordered entry and finite source. Comput. Oper. Res. 1983, 10, 213–222. [Google Scholar] [CrossRef]
- Rana, P.S. A discrete time queueing problem with S heterogeneous groups of channels. Microelectron. Reliab. 1985, 25, 455–459. [Google Scholar]
- Rana, P.S. A queueing problem with random memory arrivals and heterogeneous servers. Microelectron. Reliab. 1985, 25, 645–650. [Google Scholar]
- Rosberg, Z.; Makowski, A.M. Optimal routing to parallel heterogeneous servers-small arrival rates. IEEE Trans. Autom. Control 1990, 35, 789–796. [Google Scholar] [CrossRef]
- El-Taha, M.; Stidham, S., Jr. Deterministic analysis of queueing systems with heterogeneous servers. Theor. Comput. Sci. 1992, 106, 243–264. [Google Scholar] [CrossRef]
- Chirkova, J.; Mazalov, V.; Morozov, E. Equilibrium in a queueing system with retrials. Mathematics 2022, 10, 428. [Google Scholar] [CrossRef]
- Cabral, F.B. The slow server problem for uninformed customers. Queueing Syst. 2005, 50, 353–370. [Google Scholar] [CrossRef]
- Rykov, V.V. Monotone control of queueing systems with heterogeneous servers. Queueing Syst. 2001, 37, 391–403. [Google Scholar] [CrossRef]
- Efrosinin, D.; Sztrik, J. Performance analysis of a two-server heterogeneous retrial queue with threshold policy. Qual. Technol. Quant. Manag. 2011, 8, 211–236. [Google Scholar] [CrossRef]
- Efrosinin, D.; Sztrik, J. Optimal control of a two-server heterogeneous queueing system with breakdowns and constant retrials. In Proceedings of the International Conference on Information Technologies and Mathematical Modelling, Katun, Russia, 12–16 September 2016; pp. 57–72. [Google Scholar]
- Pankratova, E.; Moiseeva, S.; Farkhadov, M. Infinite-Server Resource Queueing Systems with Different Types of Markov-Modulated Poisson Process and Renewal Arrivals. Mathematics 2022, 10, 2962. [Google Scholar] [CrossRef]
- Dudin, A.; Dudina, O.; Dudin, S.; Gaidamaka, Y. Self-service system with rating dependent arrivals. Mathematics 2022, 10, 297. [Google Scholar] [CrossRef]
- Efrosinin, D.; Stepanova, N. Estimation of the optimal threshold policy in a queue with heterogeneous servers using a heuristic solution and artificial neural networks. Mathematics 2021, 9, 1267. [Google Scholar] [CrossRef]
- Efrosinin, D.; Stepanova, N.; Sztrik, J. Algorithmic analysis of finite-source multi-server heterogeneous queueing systems. Mathematics 2021, 9, 2624. [Google Scholar] [CrossRef]
- Hetherington, T.H.; Rogers, T.G.; Hsu, L.; O’Connor, M.; Aamodt, T.M. Characterizing and evaluating a key-value store application on heterogeneous CPU-GPU systems. In Proceedings of the IEEE International Symposium on Performance Analysis of Systems and Software (ISPASS), New Brunswick, NJ, USA, 1–3 April 2012; pp. 88–98. [Google Scholar]
- Ciznicki, M.; Kierzynka, M.; Kopta, P.; Kurowski, K.; Gepner, P. Benchmarking JPEG 2000 implementations on modern CPU and GPU architectures. J. Comput. Sci. 2014, 5, 90–98. [Google Scholar] [CrossRef]
- Kadjo, D.; Ayoub, R.; Kishinevsky, M.; Gratz, P.V. A control-theoretic approach for energy efficient CPU-GPU subsystem in mobile platforms. In Proceedings of the 52nd Annual Design Automation Conference, San Francisco, CA, USA, 8–12 June 2015; p. 62. [Google Scholar]
- Choi, W.; Duraisamy, K.; Kim, R.G.; Doppa, J.R.; Pande, P.P.; Marculescu, D.; Marculescu, R. On-Chip Communication Network for Efficient Training of Deep Convolutional Networks on Heterogeneous Manycore Systems. IEEE Trans. Comput. 2018, 67, 672–686. [Google Scholar] [CrossRef]
- Almusaddar, G.; Naghibijouybari, H. Exploiting Parallel Memory Write Requests for Covert Channel Attacks in Integrated CPU-GPU Systems. arXiv 2023, arXiv:2307.16123. [Google Scholar]
- Jin, X.; Min, G. Analytical queue length distributions of GPS systems with long range dependent service capacity. Simul. Model. Pract. Theory 2009, 17, 1500–1510. [Google Scholar] [CrossRef]
- Lu, J.; Ma, M. Cross-layer QoS support framework and holistic opportunistic scheduling for QoS in single carrier WiMAX system. J. Netw. Comput. Appl. 2011, 34, 765–773. [Google Scholar] [CrossRef]
- Okorogu, V.N.; Okafor, C.S. Improving Traffic Management in a Data Switched Network Using an Adaptive Discrete Time Markov Modulated Poisson Process. Eur. J. Sci. Innov. Technol. 2023, 3, 542–562. [Google Scholar]
- Hruby, T.; Bos, H.; Tanenbaum, A.S. When Slower Is Faster: On Heterogeneous Multicores for Reliable Systems. In Proceedings of the USENIX Annual Technical Conference, San Jose, CA, USA, 26–28 June 2013; pp. 255–266. [Google Scholar]
- Wang, J.; Zhang, Q.; Rong, H.; Xu, G.H.; Kim, M. Leveraging Hardware Probes and Optimizations for Accelerating Fuzz Testing of Heterogeneous Applications. In Proceedings of the 31st ACM Joint European Software Engineering Conference and Symposium on the Foundations of Software Engineering, San Jose, CA, USA, 3–9 November 2023; pp. 1101–1113. [Google Scholar]
- Hewlet Packard Enterprice. HPE ProLiant DL380 Gen9 Server. 2019. Available online: https://h20195.www2.hpe.com/v2/getpdf.aspx/c04346247.pdf (accessed on 15 November 2022).
- Hewlet Packard Enterprice Support Center. HPE ProLiant DL180 G6 Server—Overview. 2019. Available online: https://support.hpe.com/hpsc/doc/public/display?docId=emr_na-c01709693 (accessed on 15 November 2022).
- Kovalenko, S.M.; Kazancceva, L.V.; Supronenko, D.V. Integrated Indicator of the Performance of Servers. The Online Scientific and Methodological Journal “Herald of MSTU MIREA”. 2014. Available online: https://rtj.mirea.ru/upload/medialibrary/3df/10-kovalenko_kazantsev.pdf (accessed on 15 November 2022).
- Mizaras, S.; Kombaris, N. Economic assessment of multifunctional forests in conservation land areas of Varniai regional park. Agric. Sci. 2012, 19, 98–105. [Google Scholar]



| Characteristics | Denotation | Formula |
|---|---|---|
| The occupancy probability of all channels and stalling buffer | ||
| Downtime probability | ||
| Occupancy probability of only all slow channels | ||
| Stuck probability | ||
| Number of stuck queries | ||
| Probability of queries in slow channels | ||
| Number of queries in slow channels | ||
| Probability of queries in fast channels | ||
| Number of queries in fast channels | ||
| Probability of stalling | 1 − | |
| Number of queries in stalling buffer | , | K − |
| Probability of queue | ||
| Number of queries in waiting line | ||
| Probability of queries loss | ||
| Average number of queries in QS | m+ |
| Parameters | Denotation | Formula | Value |
|---|---|---|---|
| Basic characteristics of clusters with stalling | |||
| Intensity of interarrival | Λ | - | 0.58 (Gbps) |
| Capacity of fast server | µ1 | - | 0.146 (Gbps) |
| Capacity of slow server | µ2 | - | 0.045 (Gbps) |
| Number of fast servers | m | - | 5 |
| Number of slow servers | n | - | 2 |
| Coefficient of QS utilization | 0.71 | ||
| Coefficient of utilization of fast server | Q | 0.751 | |
| Rate of capacity of fast and slow servers | R | 6.959 | |
| Calculated characteristics of clusters with stalling | |||
| Characteristics | Denotation | Value | |
| Occupancy probability of fast channel in QS without slow channel and stalling | 0.464 | ||
| The occupancy probability of the fast channels and stalling buffer and free slow channels | 0.981 | ||
| Downtime probability | 0.0185 | ||
| Stuck probability | 0 | ||
| Number of stuck queries | 0 | ||
| Occupancy probability of all slow channel with free fast channel with stalling buffer | 0 | ||
| Probability of queries in slow channels | 0 | ||
| Number of queries in slow channels | 0 | ||
| Probability of queries in fast channels | 0.9814 | ||
| Number of queries in fast channels | 3.7561 | ||
| Probability of stalling | 0.3486 | ||
| Number of queries in stalling buffer | 1.4014 | ||
| Probability of queue | 0 | ||
| Number of queries in waiting line | 0 | ||
| Probability of loss queries | 0 | ||
| Average number of queries | 5.1575 | ||
| Volume of Tree Trunk (m3) | Harvester | Chainsaw | ||
|---|---|---|---|---|
| Service (Lumbering) Time (h/m3) | Work Intensity (µ1, m3/h) | Service (Lumbering) time (h/m3) | Work Intensity (µ2, m3/h) | |
| 0.1 | 0.111 | 9 | 0.872 | 1.2 |
| 0.2 | 0.047 | 21.2 | 0.71 | 1.4 |
| 0.3 | 0.035 | 28.4 | 0.641 | 1.6 |
| 0.4 | 0.03 | 33.4 | 0.599 | 1.7 |
| 0.5 | 0.027 | 37.4 | 0.571 | 1.8 |
| 0.6 | 0.025 | 40.6 | 0.549 | 1.8 |
| 0.7 | 0.023 | 43.3 | 0.532 | 1.9 |
| 0.8 | 0.022 | 45.6 | 0.518 | 1.9 |
| 0.9 | 0.021 | 47.7 | 0.507 | 2 |
| 1 | 0.02 | 49.6 | 0.497 | 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sakalauskas, L.; Kaklauskas, L.; Macaitiene, R. Stalling in Queuing Systems with Heterogeneous Channels. Appl. Sci. 2024, 14, 773. https://doi.org/10.3390/app14020773
Sakalauskas L, Kaklauskas L, Macaitiene R. Stalling in Queuing Systems with Heterogeneous Channels. Applied Sciences. 2024; 14(2):773. https://doi.org/10.3390/app14020773
Chicago/Turabian StyleSakalauskas, Leonidas, Liudvikas Kaklauskas, and Renata Macaitiene. 2024. "Stalling in Queuing Systems with Heterogeneous Channels" Applied Sciences 14, no. 2: 773. https://doi.org/10.3390/app14020773
APA StyleSakalauskas, L., Kaklauskas, L., & Macaitiene, R. (2024). Stalling in Queuing Systems with Heterogeneous Channels. Applied Sciences, 14(2), 773. https://doi.org/10.3390/app14020773





