Performance Estimate of a Spin-Stabilized Drag Sail for Spacecraft Deorbiting
Abstract
1. Introduction
2. Mathematical Model
2.1. Aerodynamic Effects
2.2. Earth’s Oblateness
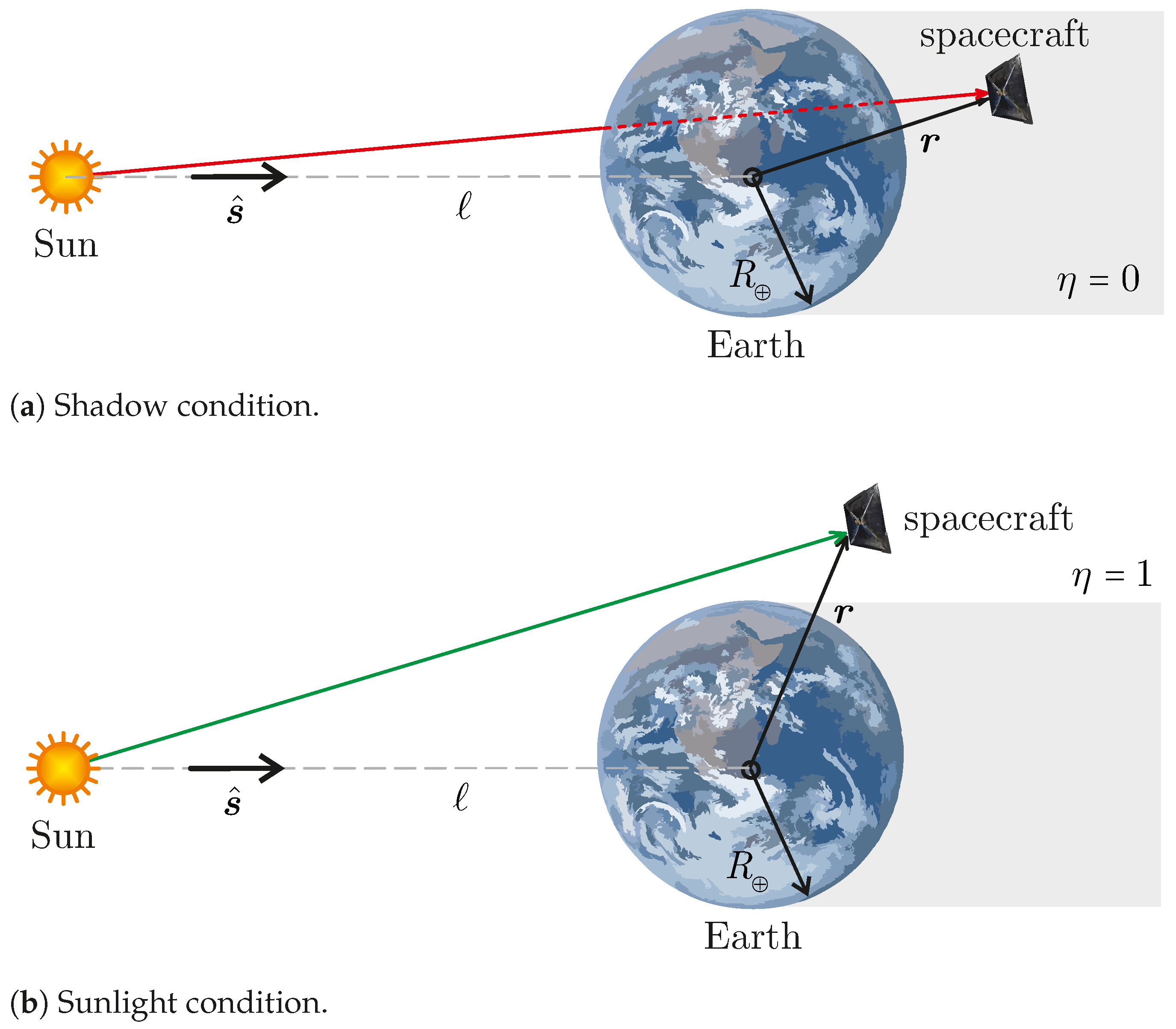
2.3. Solar Radiation Pressure
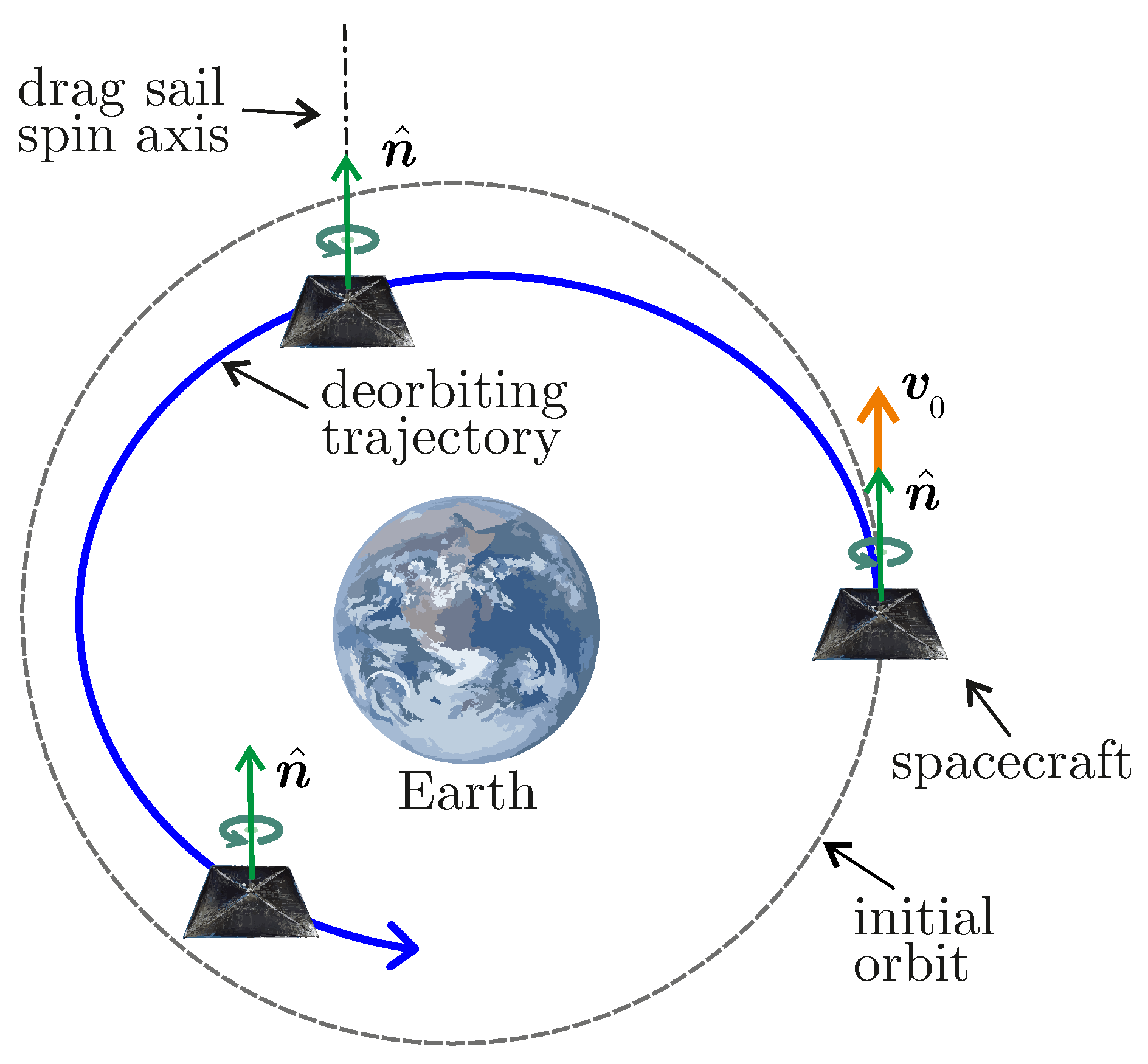
3. Spinning Drag Sail Dynamics
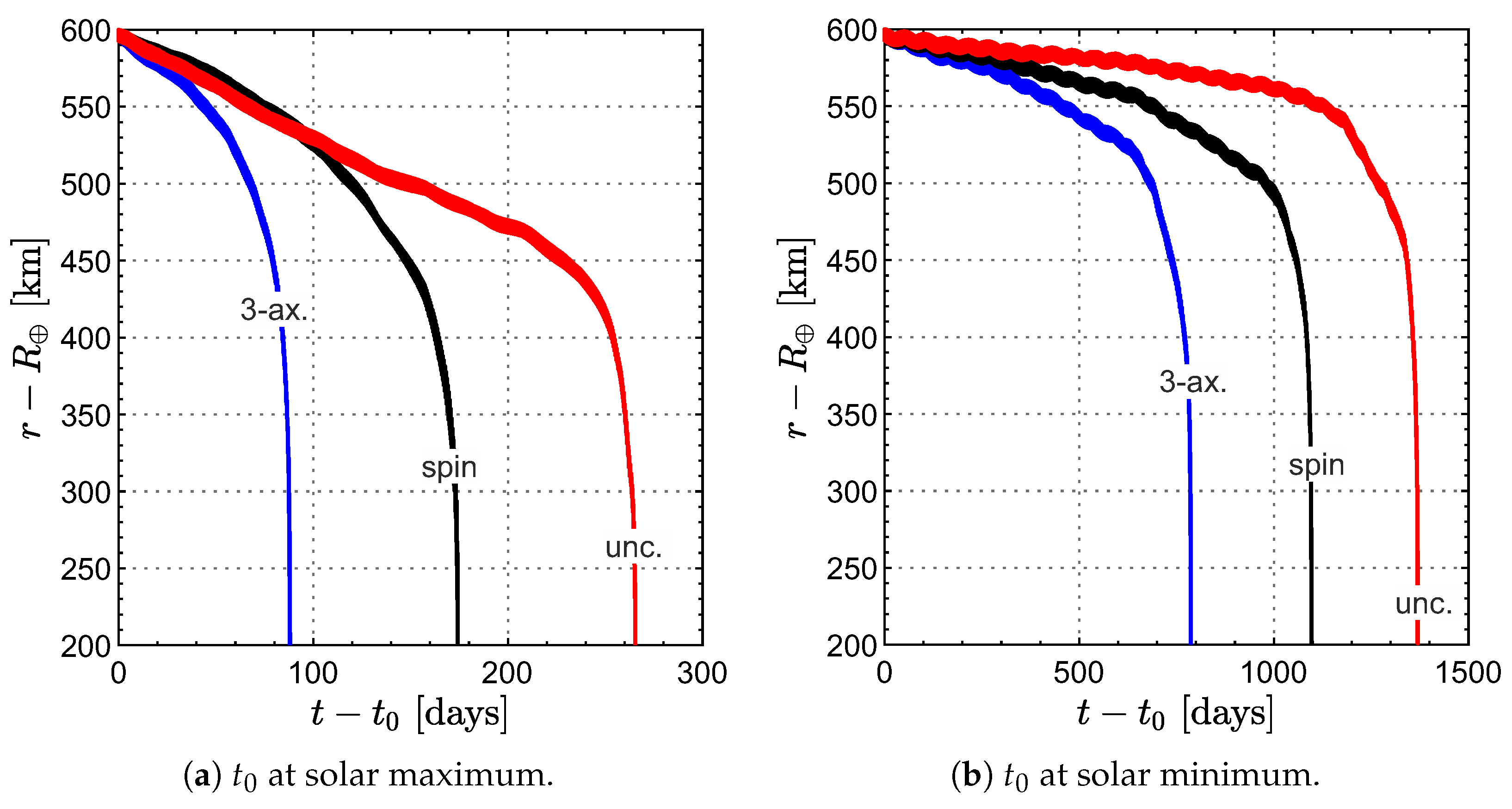
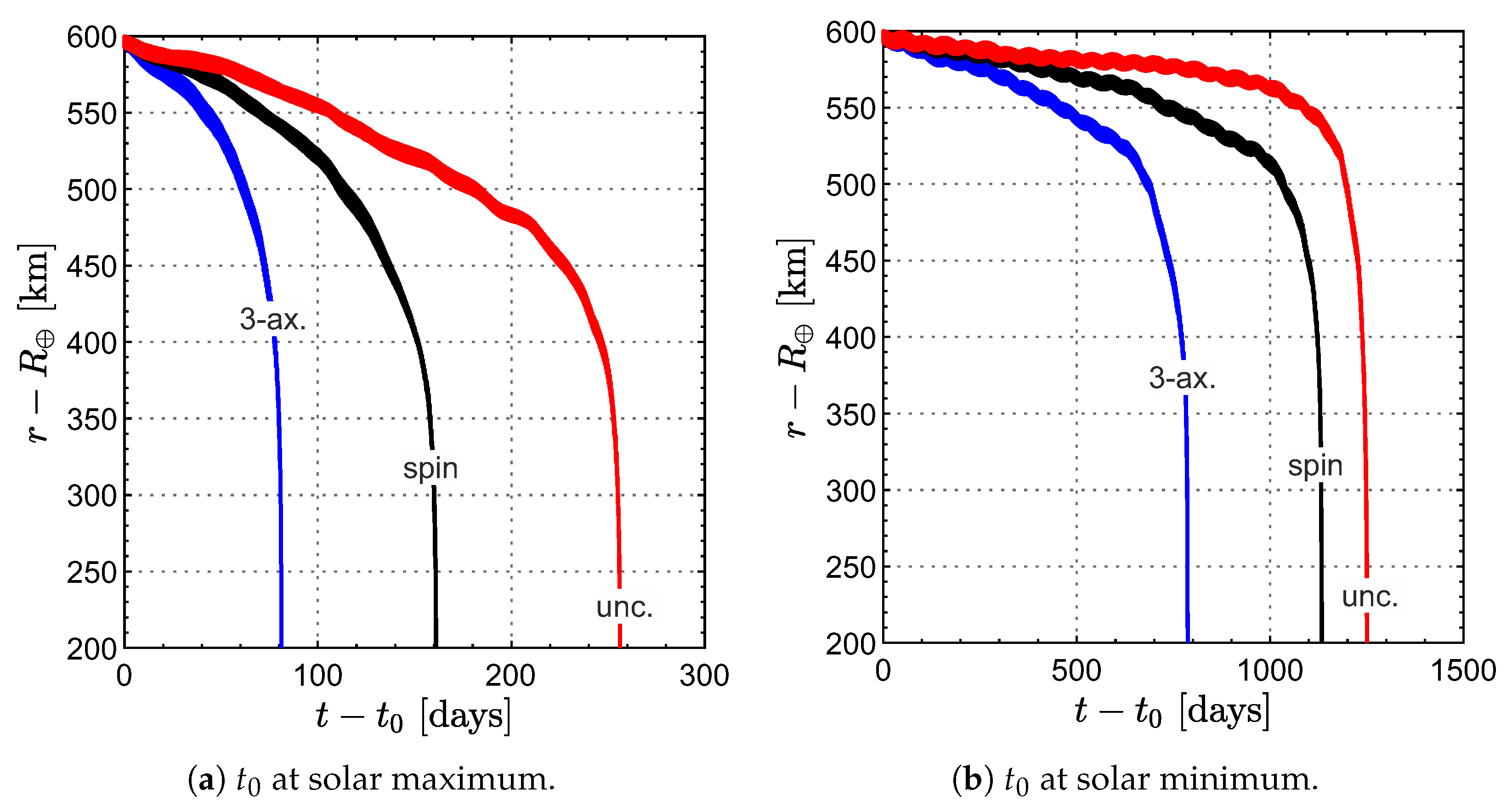
4. Performance Estimate of Spinning Drag Sail
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| ECI | Earth-centered inertial |
| LEO | low Earth orbit |
| MEOE | modified equinoctial orbital element |
| RTN | radial-tangential-normal |
| SRP | solar radiation pressure |
| SSO | Sun-synchronous orbit |
| Nomenclature | |
| state dynamical matrix, see Equation (4) | |
| A | drag sail area [m] |
| a | osculating orbit semimajor axis [km] |
| perturbative acceleration vector [km/s] | |
| auxiliary vector, see Equation (5) | |
| drag coefficient, see Equation (11) | |
| lift coefficient, see Equation (12) | |
| reflection coefficient | |
| set of atmospheric density values, see Equation (22) | |
| e | osculating orbit eccentricity |
| G | Earth’s center of mass |
| Earth’s second zonal harmonics | |
| i | osculating orbit inclination [deg] |
| ECI frame unit vectors | |
| RTN frame unit vectors | |
| lift unit vector, see Equation (10) | |
| Sun–Earth distance [au] | |
| m | spacecraft total mass [kg] |
| N | number of density evaluations |
| unit vector normal to the sail | |
| normal unit vector associated with SRP perturbation, see Equation (15) | |
| O | spacecraft center of mass |
| solar radiation pressure at 1 au from the Sun [Pa] | |
| modified equinoctial orbital elements of the osculating orbit | |
| rotational matrix from ECI frame to RTN frame | |
| Earth’s equatorial radius [km] | |
| Earth–spacecraft vector [km] | |
| r | Earth–spacecraft distance [km] |
| set of time instants, see Equation (21) | |
| Sun–spacecraft unit vector | |
| Earth-centered inertial frame | |
| radial-tangential-normal frame | |
| T | osculating orbit period [hours] |
| t | time instant [days] |
| u | osculating orbit argument of latitude [deg] |
| ratio of particle thermal velocity to orbital velocity | |
| spacecraft orbital velocity vector [km/s] | |
| state vector | |
| eclipse parameter | |
| Earth’s gravitational parameter [km/s] | |
| spacecraft true anomaly on the osculating orbit [deg] | |
| atmospheric density, [kg/m] | |
| normal momentum accommodation coefficient | |
| tangential momentum accommodation coefficient | |
| osculating orbit right ascension of the ascending node [deg] | |
| osculating orbit argument of pericenter [deg] | |
| Subscripts | |
| initial value | |
| D | drag perturbation |
| ECI | relative to the ECI frame |
| Earth’s oblateness perturbation | |
| L | lift perturbation |
| RTN | relative to the RTN frame |
| SRP | solar radiation pressure perturbation |
| Superscripts | |
| derivative with respect to time | |
| ∧ | unit vector |
| ∼ | generic time instant |
| T | transpose matrix |
References
- Sweeting, M.N.; Foquet, M. Earth observation using low cost micro/minisatellites. Acta Astronaut. 1996, 39, 823–826. [Google Scholar] [CrossRef]
- Wekerle, T.; Filho, J.B.P.; da Costa, L.E.V.L.; Trabasso, L.G. Status and trends of smallsats and their launch vehicles—An up-to-date review. J. Aerosp. Technol. Manag. 2017, 9, 269–286. [Google Scholar] [CrossRef]
- Niccolai, L.; Bassetto, M.; Quarta, A.A.; Mengali, G. A review of Smart Dust architecture, dynamics, and mission applications. Prog. Aerosp. Sci. 2019, 106, 1–14. [Google Scholar] [CrossRef]
- Twiggs, R.J. Space system developments at Stanford University: From launch experience of microsatellites to the proposed future use of picosatellites. Proc. SPIE 2000, 4136, 79–86. [Google Scholar] [CrossRef]
- Kestilä, A.; Tikka, T.; Peitso, P.; Rantanen, J.; Näsilä, A.; Nordling, K.; Saari, H.; Vainio, R.; Janhunen, P.; Praks, J.; et al. Aalto-1 nanosatellite-technical description and mission objectives. Geosci. Instrum. Methods Data Syst. 2013, 2, 121–130. [Google Scholar] [CrossRef]
- Umansky-Castro, J.S.; Mesquita, J.M.B.; Kumar, A.; Anderson, M.; Tung, T.Y.; Wen, J.J.; Adams, V.H.; Peck, M.A.; Filo, A.; Carabellese, D.; et al. Design of the Alpha CubeSat: Technology demonstration of a chipsat-equipped retroreflective light sail. In Proceedings of the AIAA SciTech Forum, Virtual Event, 11–15 & 19–21 January 2021. [Google Scholar] [CrossRef]
- Dalbins, J.; Allaje, K.; Ehrpais, H.; Iakubivskyi, I.; Ilbis, E.; Janhunen, P.; Kivastik, J.; Merisalu, M.; Noorma, M.; Pajusalu, M.; et al. Interplanetary student nanospacecraft: Development of the LEOdemonstrator ESTCube-2. Aerospace 2023, 10, 503. [Google Scholar] [CrossRef]
- Murtaza, A.; Pirzada, S.J.H.; Xu, T.; Jianwei, L. Orbital debris threat for space sustainability and way forward (review article). IEEE Access 2020, 8, 6100–61019. [Google Scholar] [CrossRef]
- Ren, S.; Yang, X.; Wang, R.; Liu, S.; Sun, X. The interaction between the LEO satellite constellation and the space debris environment. Appl. Sci. 2021, 11, 9490. [Google Scholar] [CrossRef]
- ESA’s Annual Space Debris Environment Report; Techreport; ESA: Wan Chai, Hong Kong, 2022.
- Kessler, D.J.; Cour-Palais, B.G. Collision frequency of artificial satellites: The creation of a debris belt. J. Geophys. Res. 1978, 83, 2637–2646. [Google Scholar] [CrossRef]
- Kessler, D.J. Collisional cascading: The limits of population growth in low earth orbit. Adv. Space Res. 1991, 11, 63–66. [Google Scholar] [CrossRef]
- Liou, J.C.; Johnson, N.L. Risks in space from orbiting debris. Science 2006, 311, 340–341. [Google Scholar] [CrossRef]
- Kessler, D.J.; Johnson, N.L.; Liou, J.C.; Matney, M. The Kessler Syndrome: Implications to future space operations. Adv. Astronaut. Sci. 2010, 137, 47–61. [Google Scholar]
- Schäfer, F.; Lambert, M.; Christiansen, E.; Kibe, S.; Stokes, H.; Reimerdes, H.G.; Meshcheryakov, S.A.; Angrilli, F.; Zengyao, H. The Inter-Agency Space Debris Coordination Committee (IADC) protection manual. In Proceedings of the 4th European Conference on Space Debris, Darmstadt, Germany, 18–20 April 2005. [Google Scholar]
- Yakovlev, M. The “IADC space debris mitigation guidelines” and support document. In Proceedings of the 4th European Conference on Space Debris, Darmstadt, Germany, 18–20 April 2005. [Google Scholar]
- Liou, J.C.; Johnson, N.L. Instability of the present LEO satellite populations. Adv. Space Res. 2008, 41, 1046–1053. [Google Scholar] [CrossRef]
- Liu, M.; Wang, H.; Yi, H.; Xue, Y.; Wen, D.; Wang, F.; Shen, Y.; Pan, Y. Space debris detection and positioning technology based on multiple star trackers. Appl. Sci. 2022, 12, 3593. [Google Scholar] [CrossRef]
- Shan, M.; Guo, J.; Gill, E. Review and comparison of active space debris capturing and removal methods. Prog. Aerosp. Sci. 2016, 80, 18–32. [Google Scholar] [CrossRef]
- Mark, C.P.; Kamath, S. Review of active space debris removal methods. Space Policy 2019, 47, 194–206. [Google Scholar] [CrossRef]
- Hubert Dekuskem, M.; Christidi-Loumpasefski, O.O.; Yalçin, B.C.; Li, X.; Olivares-Mendez, M.; Martinez, C. Hybrid-compliant system for soft capture of uncooperative space debris. Appl. Sci. 2023, 13, 7968. [Google Scholar] [CrossRef]
- Tang, C.; Deng, Y.; Bai, Z.; Wei, C.; Zhao, Y. Dynamics analysis of space netted pocket system capturing non-cooperative target. Appl. Sci. 2023, 13, 10377. [Google Scholar] [CrossRef]
- Sanchez-Arriaga, G.; Sanmartin, J.R.; Lorenzini, E.C. Comparison of technologies for deorbiting spacecraft from low-Earth-orbit at end of mission. Acta Astronaut. 2017, 138, 536–542. [Google Scholar] [CrossRef]
- Colombo, C.; Rossi, A.; Dalla Vedova, F.; Francesconi, A.; Bombardelli, C.; Trisolini, M.; Gonzalo, J.L.; Di Lizia, P.; Giacomuzzo, C.; Khan, S.B.; et al. Effects of passive de-orbiting through drag and solar sails and electrodynamic tethers on the space debris environment. In Proceedings of the 69th International Astronautical Congress (IAC), Bremen, Germany, 1–5 October 2018. [Google Scholar]
- Zhong, R.; Zhu, Z.H. Dynamics of nanosatellite deorbit by bare electrodynamic tether in Low Earth Orbit. J. Spacecr. Rocket. 2013, 50, 691–700. [Google Scholar] [CrossRef]
- Janhunen, P. Electrostatic plasma brake for deorbiting a satellite. J. Propuls. Power 2010, 26, 370–372. [Google Scholar] [CrossRef]
- Orsini, L.; Niccolai, L.; Mengali, G.; Quarta, A.A. Plasma brake model for preliminary mission analysis. Acta Astronaut. 2017, 144, 297–304. [Google Scholar] [CrossRef]
- Iakubivskyi, I.; Janhunen, P.; Praks, J.; Allik, V.; Bussov, K.; Clayhills, B.; Dalbins, J.; Eenmäe, T.; Ehrpais, H.; Envall, J.; et al. Coulomb drag propulsion experiments of ESTCube-2 and FORESAIL-1. Acta Astronaut. 2020, 177, 771–783. [Google Scholar] [CrossRef]
- Palmroth, M.; Praks, J.; Vainio, R.; Janhunen, P.; Kilpua, E.K.J.; Afanasiev, A.; Ala-Lahti, M.; Alho, A.; Asikainen, T.; Asvestari, E.; et al. FORESAIL-1 CubeSat mission to measure radiation belt losses and demonstrate deorbiting. J. Geophys. Res. Space Phys. 2019, 124, 5783–5799. [Google Scholar] [CrossRef]
- Fernandez, J.M.; Visagie, L.; Schenk, M.; Stohlman, O.R.; Aglietti, G.S.; Lappas, V.J.; Erb, S. Design and development of a gossamer sail system for deorbiting in low Earth orbit. Acta Astronaut. 2014, 103, 204–225. [Google Scholar] [CrossRef]
- Visagie, L.; Lappas, V.J.; Erb, S. Drag sails for space debris mitigation. Acta Astronaut. 2015, 109, 65–75. [Google Scholar] [CrossRef]
- Serfontein, Z.; Kingston, J.; Hobbs, S.; Holbrough, I.E.; Beck, J.C. Drag augmentation systems for space debris mitigation. Acta Astronaut. 2021, 188, 278–288. [Google Scholar] [CrossRef]
- Spencer, D.A.; Johnson, L.; Long, A.C. Solar sailing technology challenges. Aerosp. Sci. Technol. 2019, 93, 105276. [Google Scholar] [CrossRef]
- Quarta, A.A.; Mengali, G. Solar sail orbit raising with electro-optically controlled diffractive film. Appl. Sci. 2023, 13, 7078. [Google Scholar] [CrossRef]
- Colombo, C.; Rossi, A.; Francesconi, A.; Dalla Vedova, F.; Gonzalo, J.L.; Braun, V.; Bastida Virgili, B.; Krag, H. Long-term simulations to assess the effects of drag and solar sails on the space debris environment. In Proceedings of the 70th International Astronautical Congress (IAC), Washington, DC, USA, 21–25 October 2019. [Google Scholar]
- Kerr, E.; Macdonald, M. Limits of drag augmentation at spacecraft end-of-mission and a mitigation strategy. Astrodynamics 2021, 5, 109–120. [Google Scholar] [CrossRef]
- Johnson, L.; Whorton, M.; Heaton, A.; Pinson, R.; Laue, G.; Adams, C. NanoSail-D: A solar sail demonstration mission. Acta Astronaut. 2011, 68, 571–575. [Google Scholar] [CrossRef]
- Svitek, T.; Friedman, L.; Nye, W.; Biddy, C.; Nehrenz, M. Voyage continues-LightSail-1 mission by the planetary society. In Proceedings of the 61st International Astronautical Congress, Prague, Czech Republic, 27 September–1 October 2010. [Google Scholar]
- Ridenoure, R.W.; Spencer, D.A.; Stetson, D.A.; Betts, B.; Munakata, R.; Wong, S.D.; Diaz, A.; Plante, B.A.; Foley, J.D.; Ballardo, J.M. Status of the dual CubeSat LightSail program. In Proceedings of the AIAA SPACE Conference and Exposition, Pasadena, CA, USA, 31 August–2 September 2015. [Google Scholar] [CrossRef][Green Version]
- Nutter, A.; Bauda, C.; Culeux, J.; Straubel, M.; Zander, M.E.; Hillebrandt, M. Objectives, design and initial test results of the upcoming GAMA-Beta solar sail in-orbit demonstration. In Proceedings of the 6th International Symposium on Space Sailing (ISSS), New York, NY, USA, 5–9 June 2023. [Google Scholar]
- Kuwahara, T.; Yoshida, K.; Sakamoto, Y.; Tomioka, Y.; Fukuda, K.; Fukuyama, M.; Tanabe, T.; Shibuya, Y. Qualification results of a sail deployment mechanism for active prevention and reduction of space debries. In Proceedings of the 63rd International Astronautical Congress (IAC), Naples, Italy, 30 September–5 October 2012. [Google Scholar]
- Underwood, C.; Viquerat, A.; Schenk, M.; Taylor, B.; Massimiani, C.; Duke, R.; Stewart, B.; Fellowes, S.; Bridges, C.; Aglietti, G.S.; et al. InflateSail de-orbit flight demonstration results and follow-on drag-sail applications. Acta Astronaut. 2019, 162, 344–358. [Google Scholar] [CrossRef]
- Bonin, G.; Hiemstra, J.; Sears, T.; Zee, R.E. The CanX-7 drag sail demonstration mission: Enabling environmental stewardship for nano- and microsatellites. In Proceedings of the 27th Annual AIAA/USU Conference on Small Satellites, Logan, UT, USA, 4 August 2013. [Google Scholar]
- Nock, K.T.; Gates, K.L.; Aaron, K.M.; McRonald, A.D. Gossamer orbit lowering device (GOLD) for safe and efficient de-orbit. In Proceedings of the AIAA/ASS Astrodynamics Specialist Conference, Toronto, ON, Canada, 2–5 August 2010. [Google Scholar] [CrossRef]
- Black, A.; Spencer, D.A. DragSail systems for satellite deorbit and targeted reentry. J. Space Saf. Eng. 2020, 7, 397–403. [Google Scholar] [CrossRef]
- Miguel, N.; Colombo, C. Deorbiting spacecraft with passively stabilised attitude using a simplified quasi-rhombic-pyramid sail. Adv. Space Res. 2021, 67, 2561–2576. [Google Scholar] [CrossRef]
- Zhang, J.R.; Zhang, R.N.; Yang, K.Y. Attitude stability analysis and configuration design of pyramid drag sail for deorbit missions. J. Aerosp. Eng. 2022, 35, 04022084. [Google Scholar] [CrossRef]
- Zhang, R.N.; Yang, R.Y.; Zhang, J.R.; Bi, S.F. Dynamics analysis and optimal strategy of pyramid deorbit sail. In Proceedings of the 73rd International Astronautical Congress (IAC), Paris, France, 18–22 September 2022. [Google Scholar]
- MacNeal, R.H. The Heliogyro—An Interplanetary Flying Machine; Techreport CR 84469; NASA: Washington, DC, USA, 1967.
- Burton, R.; Coverstone, V.; Hargens-Rysanek, J.; Ertmer, K.; Botter, T.; Benavides, G.; Woo, B.; Carroll, D.; Gierow, P.; Farmer, G.; et al. UltraSail-Ultra-lightweight solar sail concept. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference, Tucson, AZ, USA, 10–13 July 2005. [Google Scholar]
- Hargens-Rysanek, J. The Dynamics and Control of the UltraSail System. Ph.D. Thesis, University of Illinois, Champaign, IL, USA, 2006. [Google Scholar]
- Hargens-Rysanek, J.; Coverstone, V.L.; Burton, R.L. Orbital precession via cyclic pitch for the UltraSail system. In Proceedings of the 17th AAS/AIAA Space Flight Mechanics Meeting, Sedona, AZ, USA, 28 January–1 February 2007. [Google Scholar]
- Sawada, H.; Mori, O.; Okuizumi, N.; Shirasawa, Y.; Miyazaki, Y.; Natori, M.; Matunaga, S.; Furuya, H.; Sakamoto, H. Mission report on the solar power sail deployment demonstration of IKAROS. In Proceedings of the 52nd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Denver, CO, USA, 4–7 April 2011. [Google Scholar] [CrossRef]
- Tsuda, Y.; Mori, O.; Funase, R.; Sawada, H.; Yamamoto, T.; Saiki, T.; Endo, T.; Yonekura, K.; Hoshino, H.; Kawaguchi, J. Achievement of IKAROS-Japanese deep space solar sail demonstration mission. Acta Astronaut. 2013, 82, 183–188. [Google Scholar] [CrossRef]
- Sakamoto, H.; Shirasawa, Y.; Haraguchi, D.; Sawada, H.; Mori, O. A spin-up control scheme for contingency deployment of the sailcraft IKAROS. In Proceedings of the 52nd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Denver, CO, USA, 4–7 April 2011. [Google Scholar] [CrossRef]
- Walker, M.J.H.; Ireland, B.; Owens, J. A set of modified equinoctial orbit elements. Celest. Mech. 1985, 36, 409–419. [Google Scholar] [CrossRef]
- Lücking, C.; Colombo, C.; McInnes, C.R. A passive satellite deorbiting strategy for medium Earth orbit using solar radiation pressure and the J2 effect. Acta Astronaut. 2012, 77, 197–206. [Google Scholar] [CrossRef]
- Lücking, C.; Colombo, C.; McInnes, C.R. Solar radiation pressure-augmented deorbiting: Passive end-of-life disposal from high-altitude orbits. J. Spacecr. Rocket. 2013, 50, 1256–1267. [Google Scholar] [CrossRef]
- Carzana, L.; Visser, P.; Heiligers, J. Locally optimal control laws for Earth-bound solar sailing with atmospheric drag. Aerosp. Sci. Technol. 2022, 127, 107666. [Google Scholar] [CrossRef]
- Storch, J.A. Aerodynamic Disturbances on Spacecraft in Free-Molecular Flow; Technical Report ADA410696; The Aerospace Corporation: El Segundo, CA, USA, 2002. [Google Scholar]
- Mengali, G.; Quarta, A.A. Near-optimal solar-sail orbit-raising from low Earth orbit. J. Spacecr. Rocket. 2005, 42, 954–958. [Google Scholar] [CrossRef]
- Stolbunov, V.; Ceriotti, M.; Colombo, C.; McInnes, C.R. Optimal law for inclination change in an atmosphere through solar sailing. J. Guid. Control. Dyn. 2013, 36, 1310–1323. [Google Scholar] [CrossRef]
- Dachwald, B. Minimum transfer times for nonperfectly reflecting solar sailcraft. J. Spacecr. Rocket. 2004, 41, 693–695. [Google Scholar] [CrossRef]
- Stelzl, D.; Seefeldt, P.; Killian, M.; Hofmann, L.; Puttich, G.; Lopez-Corralez, X.; Garcia Mora, C.; Pimpi, J.; Schuhbaur, M.; Stolz, O.; et al. The ADEO space sail products. In Proceedings of the 6th International Symposium on Space Sailing (ISSS), New York, NY, USA, 5–9 June 2023. [Google Scholar]
- Colombo, C.; Rossi, A.; Dalla Vedova, F.; Brun, V.; Bastida Virgili, B.; Krag, H. Drag and solar sail deorbiting: Re-entry time versus cumulative collision probability. In Proceedings of the 68th International Astronautical Congress (IAC), Adelaide, Australia, 25–29 September 2017. [Google Scholar]
- Picone, J.M.; Hedin, A.E.; Drob, D.P.; Aikin, A.C. NRLMSISE-00 empirical model of the atmosphere: Statistical comparisons and scientific issues. J. Geophys. Res. Space Phys. 2002, 107, 1468. [Google Scholar] [CrossRef]
- Vallado, D.A. Fundamentals of Astrodynamics and Applications; Chapter 3.5; McGraw-Hill: New York, NY, USA, 1997; pp. 181–184. [Google Scholar]
- Mahdi, M.C. Attitude Stabilization for CubeSat: Concepts and Technology; Chapter 2; Cambridge Scholars Publishing: Newcastle upon Tyne, UK, 2018; pp. 37–50. [Google Scholar]
- Wertz, J.R. (Ed.) Spacecraft Attitude Determination and Control; Chapter Appendix D; Kluwer Academic Publishers: Alphen aan den Rijn, The Netherlands, 1978; pp. 758–759. [Google Scholar]
- Wie, B.; Barba, P.M. Quaternion feedback for spacecraft large angle maneuvers. J. Guid. Control. Dyn. 1985, 8, 360–365. [Google Scholar] [CrossRef]
- Markley, F.L.; Crassidis, J.L. Fundamentals of Spacecraft Attitude Determination and Control; Chapter 2.9.3; Springer: Berlin/Heidelberg, Germany, 2014; pp. 45–48. [Google Scholar] [CrossRef]
- Mansell, J.R.; Bellardo, J.M.; Betts, B.; Plante, B.; Spencer, D.A. LightSail 2 solar sail control and orbit evolution. Aerospace 2023, 10, 579. [Google Scholar] [CrossRef]


| Parameter | Value | Unit |
|---|---|---|
| Total spacecraft mass m | 15 | |
| Spacecraft body mass | ||
| Drag sail mass | ||
| Spacecraft body dimensions | ||
| Drag sail area A | 5 | |
| Moments of inertia |
| Orbit | Sail Configuration | Decay Time [Years] | Time Saving [%] |
|---|---|---|---|
| dawn-dusk SSO | three-axis | 0.241 | 66.8 |
| spinning | 0.477 | 34.4 | |
| uncontrolled | 0.727 | ||
| noon-midnight SSO | three-axis | 0.222 | 68.3 |
| spinning | 0.441 | 37.1 | |
| uncontrolled | 0.702 | ||
| medium inclination | three-axis | 0.231 | 67.8 |
| spinning | 0.777 | −8.5 | |
| uncontrolled | 0.717 | ||
| equatorial | three-axis | 0.209 | 73.0 |
| spinning | 0.407 | 47.5 | |
| uncontrolled | 0.775 |
| Orbit | Sail Configuration | Decay Time [Years] | Time Saving [%] |
|---|---|---|---|
| dawn-dusk SSO | three-axis | 2.154 | 42.6 |
| spinning | 3.103 | 17.2 | |
| uncontrolled | 3.750 | ||
| noon-midnight SSO | three-axis | 2.071 | 39.5 |
| spinning | 2.984 | 12.8 | |
| uncontrolled | 3.424 | ||
| medium inclination | three-axis | 2.187 | 36.4 |
| spinning | 3.434 | 0.1 | |
| uncontrolled | 3.437 | ||
| equatorial | three-axis | 2.079 | 38.8 |
| spinning | 3.093 | 8.9 | |
| uncontrolled | 3.396 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niccolai, L.; Mengali, G. Performance Estimate of a Spin-Stabilized Drag Sail for Spacecraft Deorbiting. Appl. Sci. 2024, 14, 612. https://doi.org/10.3390/app14020612
Niccolai L, Mengali G. Performance Estimate of a Spin-Stabilized Drag Sail for Spacecraft Deorbiting. Applied Sciences. 2024; 14(2):612. https://doi.org/10.3390/app14020612
Chicago/Turabian StyleNiccolai, Lorenzo, and Giovanni Mengali. 2024. "Performance Estimate of a Spin-Stabilized Drag Sail for Spacecraft Deorbiting" Applied Sciences 14, no. 2: 612. https://doi.org/10.3390/app14020612
APA StyleNiccolai, L., & Mengali, G. (2024). Performance Estimate of a Spin-Stabilized Drag Sail for Spacecraft Deorbiting. Applied Sciences, 14(2), 612. https://doi.org/10.3390/app14020612






