Numerical Analysis of Stabilization of a Horse’s Third Metacarpal Bone Fracture for Prediction of the Possibility of Bone Union
Abstract
1. Introduction
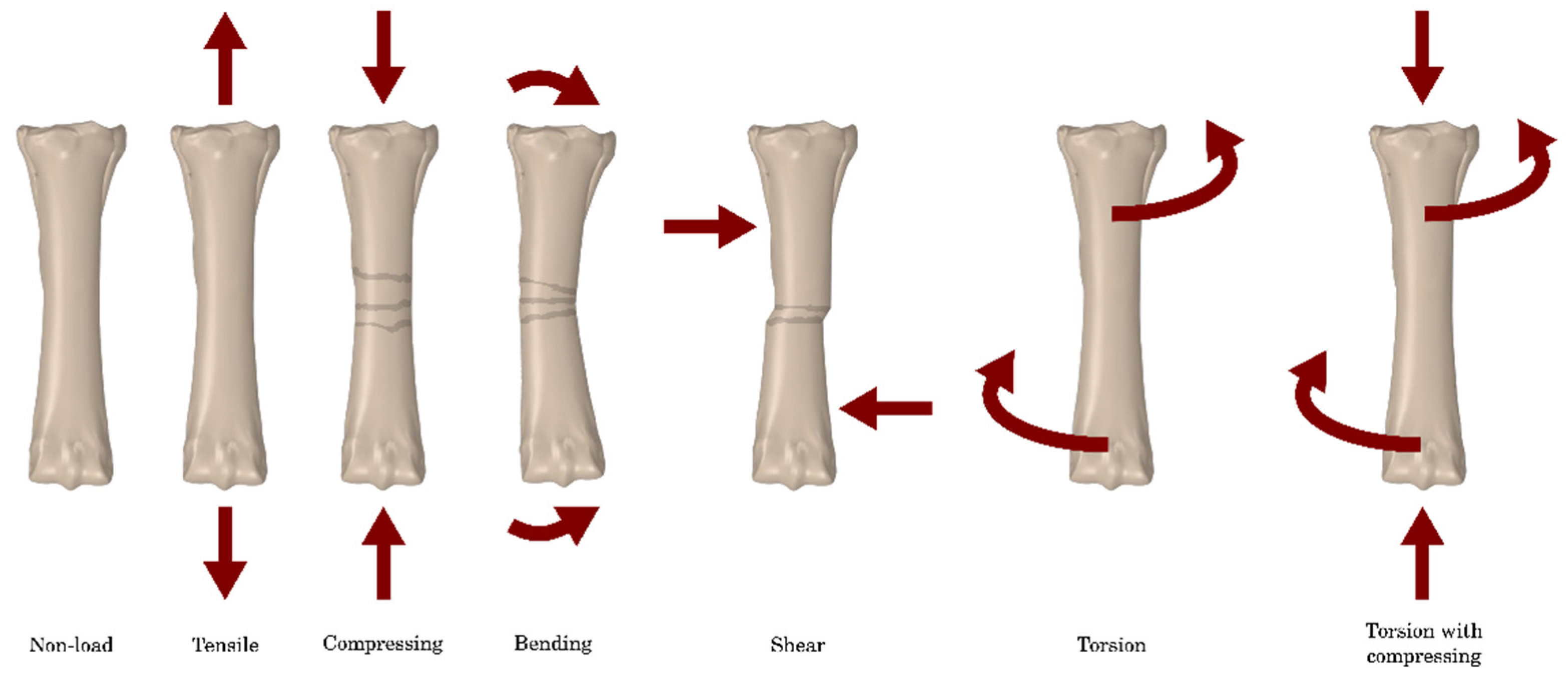
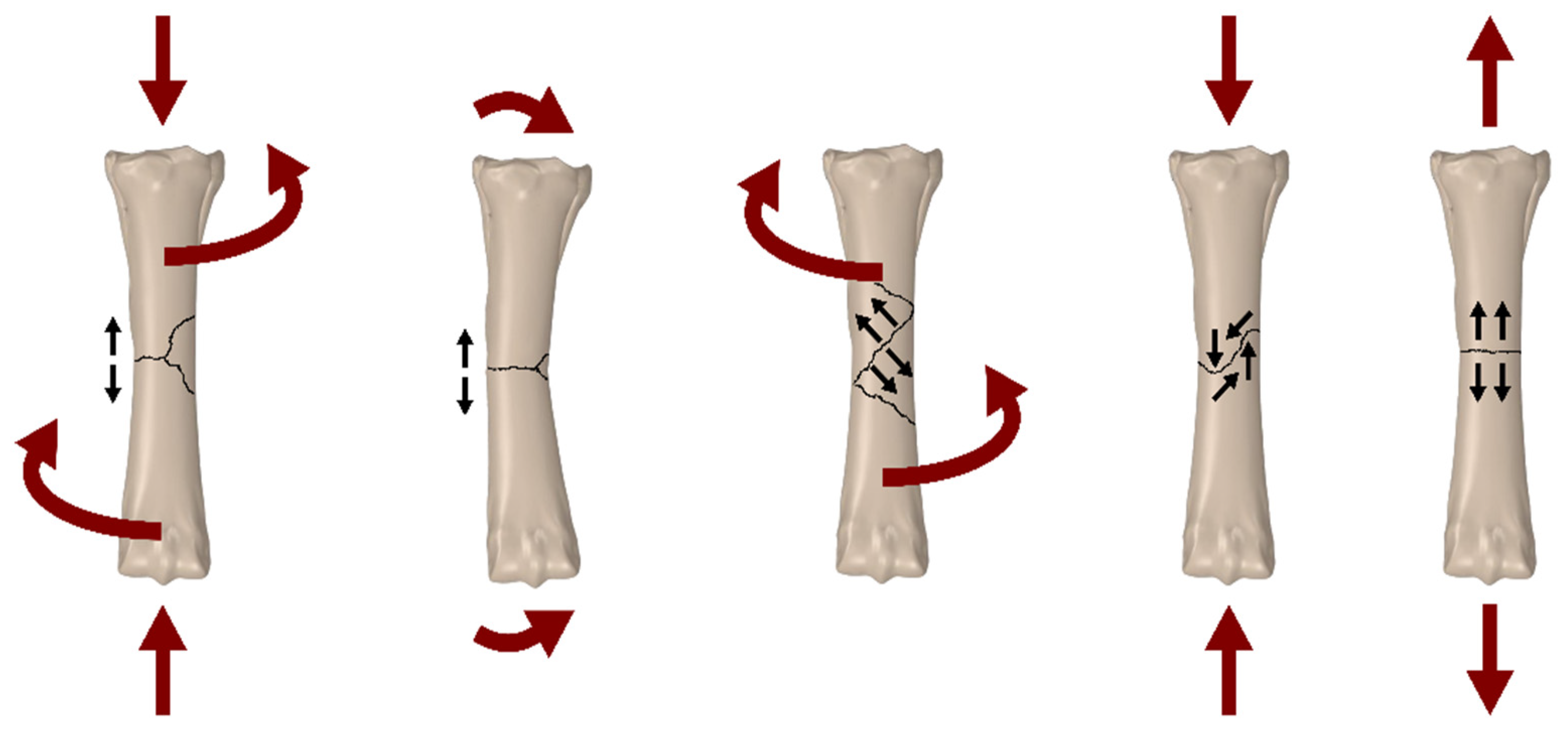
2. Description of Problem
3. Materials and Methods
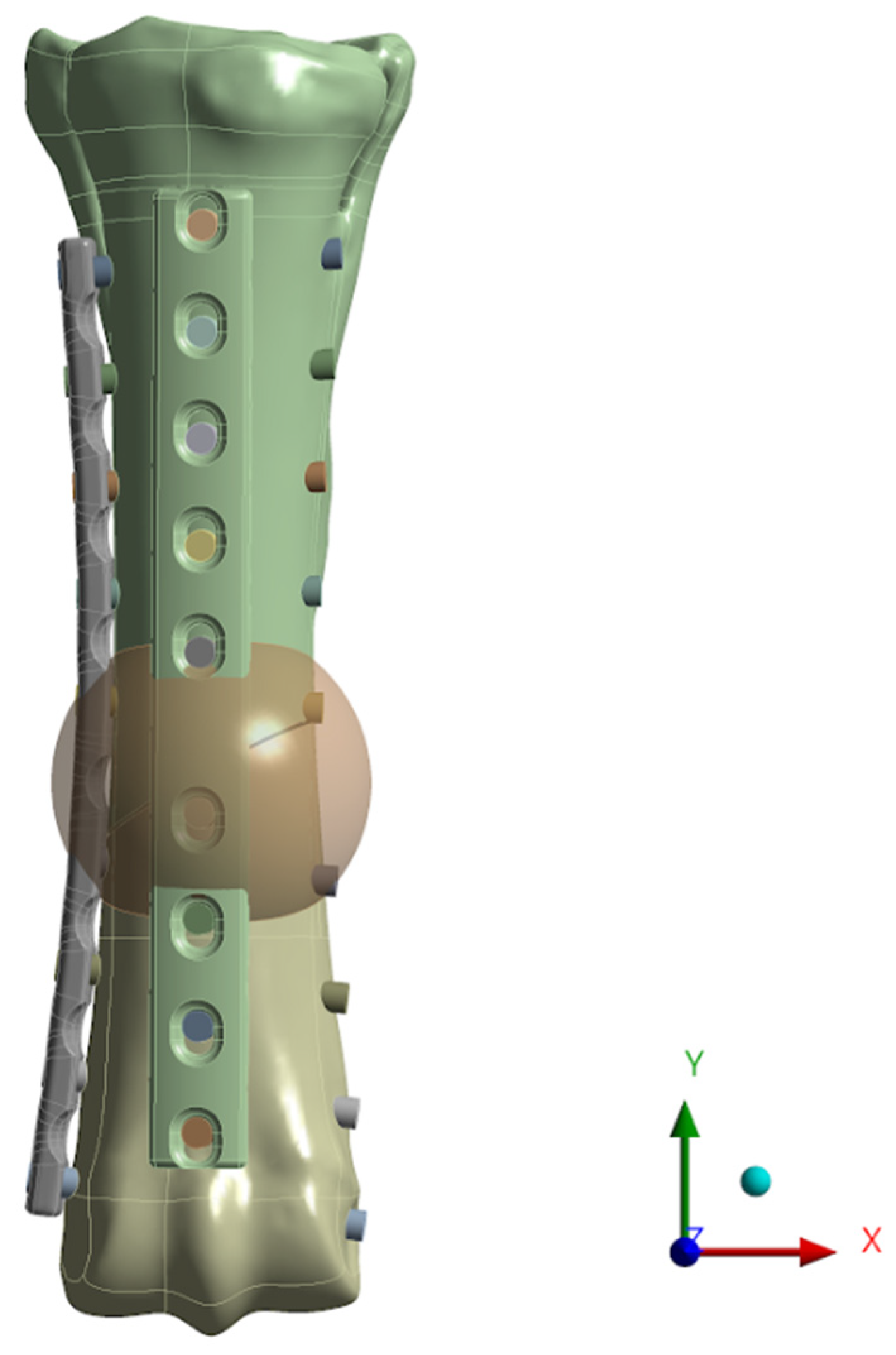
3.1. Preparation of a Numerical Model
3.2. Material Models to Describe the Behaviour of Materials
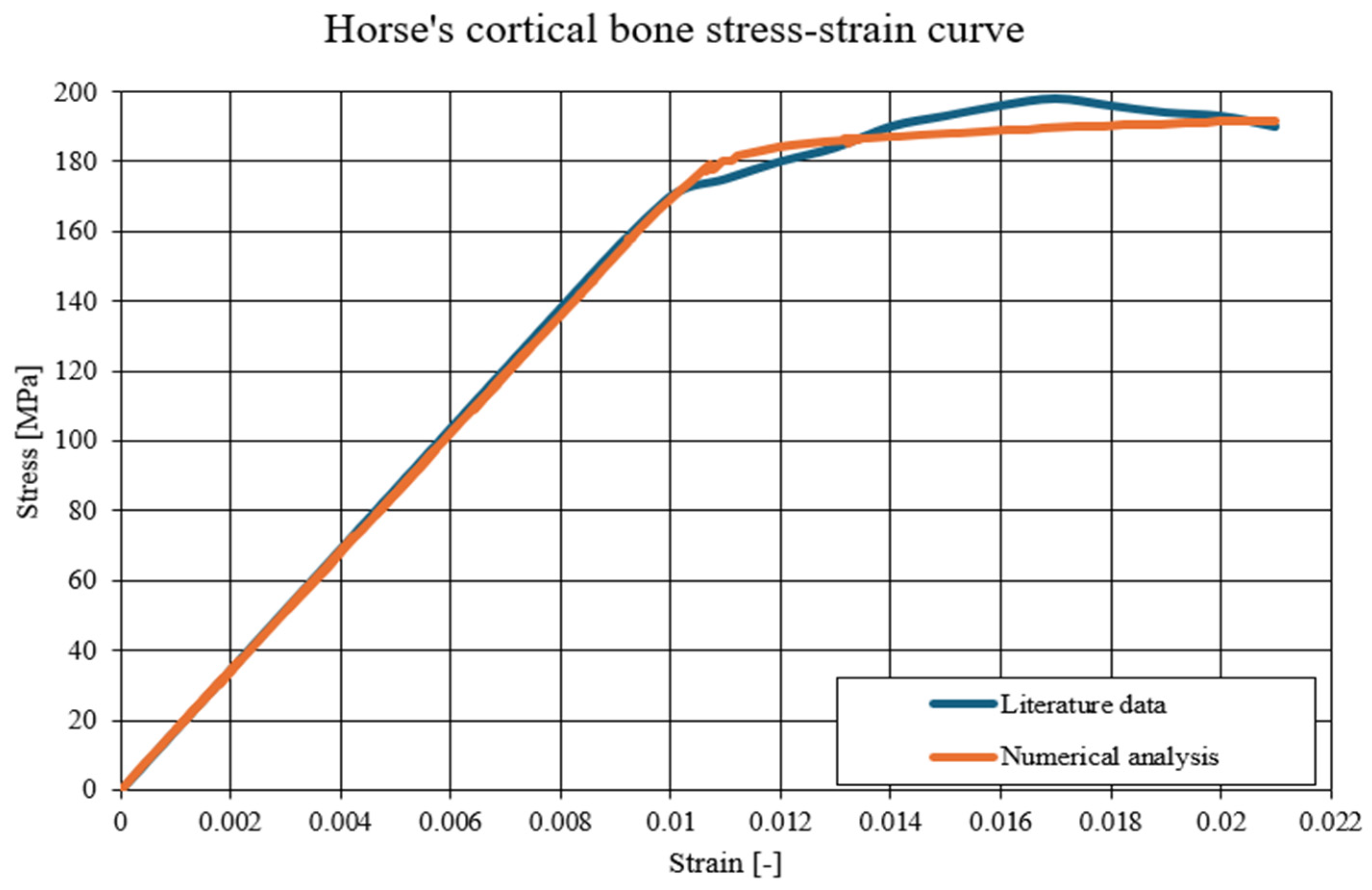
| Linear Simulations | ||||
|---|---|---|---|---|
| Material | E [GPa] | v [-] | ρ [kg/m3] | |
| 316L Steel | 200.0 | 0.29 | 7900 | |
| Cortical Bone | 17.0 | 0.30 | 1800 | |
| Trabecular Bone | 0.5 | 0.30 | 700 | |
| Non-linear simulations | ||||
| Material | A [MPa] | B [MPa] | n [-] | c [-] |
| 316L Steel | 490 | 600 | 0.21 | 0.015 |
| Cortical Bone | 150 | 58 | 0.21 | - |
| D1 [-] | D2 [-] | D3 [-] | D4 [-] | |
| 316L Steel | 0.05 | 3.44 | 2.12 | 0.002 |
| εlimit [-] | ||||
| Cortical Bone | 0.021 | |||
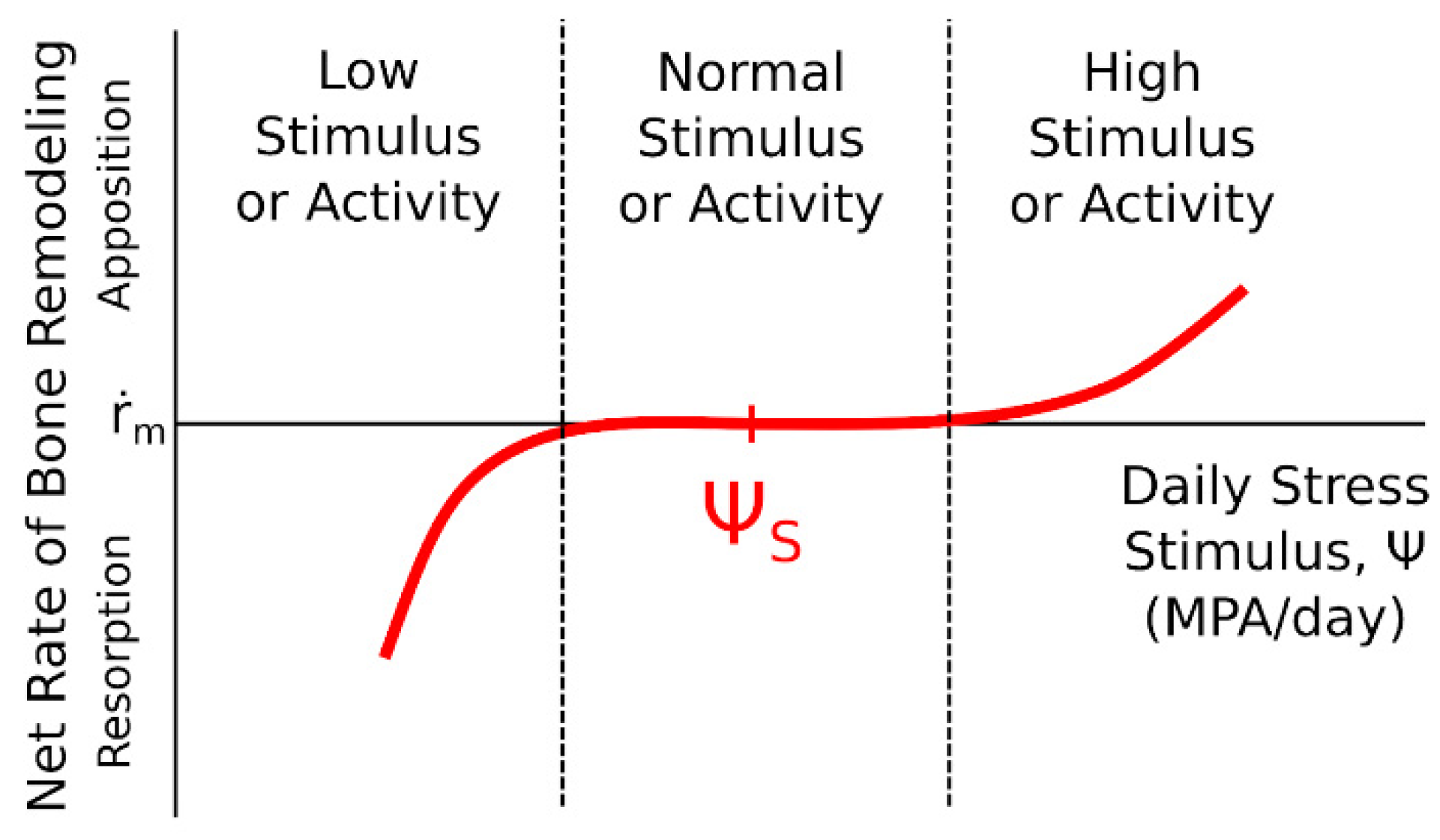
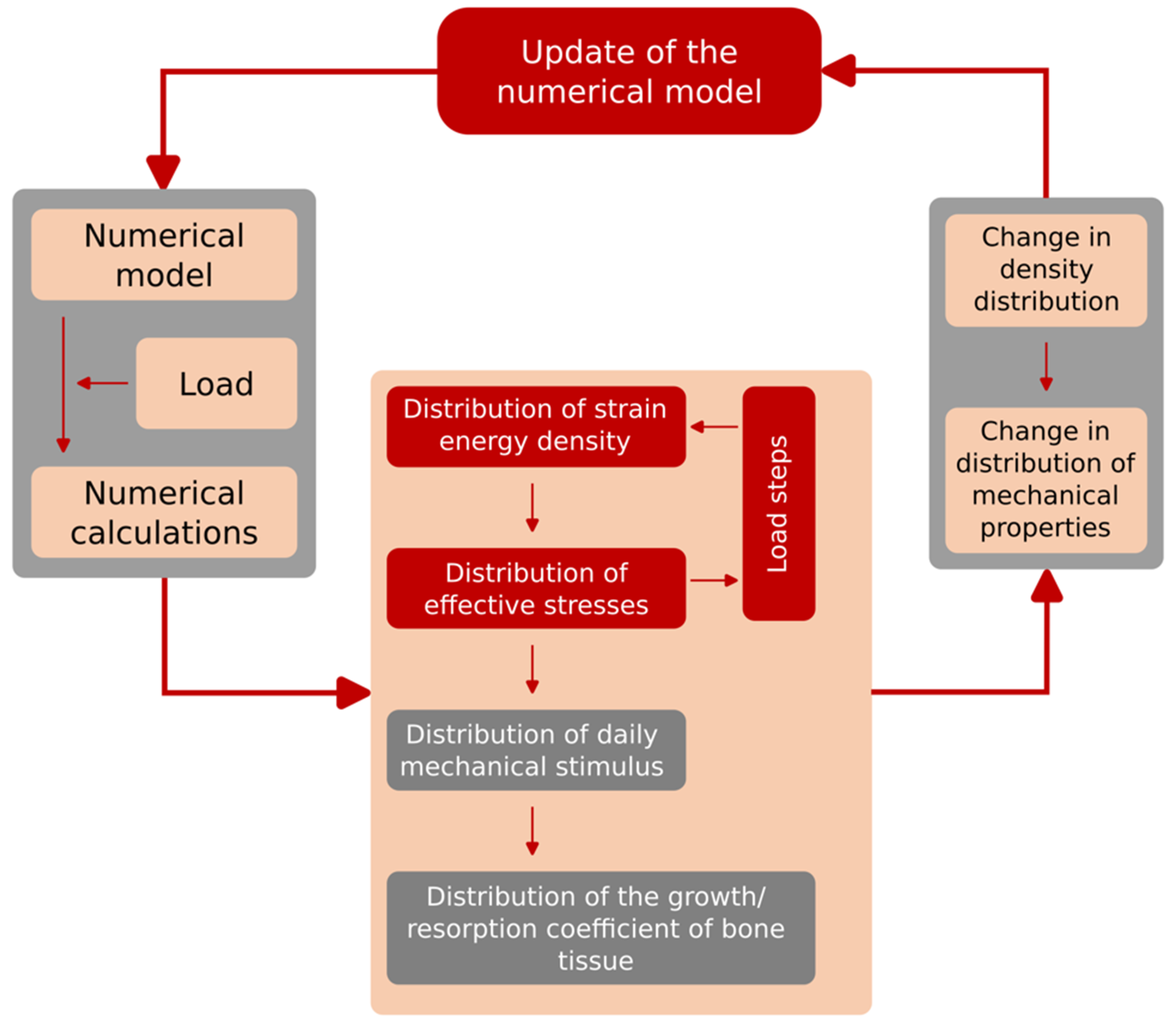
3.3. Preparation of an Algorithm to Simulate the Bone Union Process
4. Results
4.1. Results of Static Simulations
4.2. Results of Dynamic Simulations
4.3. Results of Bone Remodelling Process Simulations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Auer, J.A.; Stick, J.A.; Kümmerle, J.M.; Prange, T. Equine Surgery, 5th ed.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 1–1882. [Google Scholar] [CrossRef]
- Markel, M.D. Fracture Biomechanics; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2019; pp. 12–23. [Google Scholar] [CrossRef]
- Nixon, A.J. Equine Fracture Repair, 2nd ed.; Wiley-Blackwell: Hoboken, NJ, USA, 2019; ISBN 978-1-119-10875-7. [Google Scholar]
- McClure, S.R.; Watkins, J.P.; Glickman, N.W.; Hawkins, J.F.; Glickman, L.T. Complete Fractures of the Third Metacarpal or Metatarsal Bone in Horses: 25 Cases (1980–1996). J. Am. Vet. Med. Assoc. 1998, 213, 847–850. [Google Scholar] [CrossRef]
- Nixon, A.J.; Fortier, L.A. Fractures of the Small Metacarpal and Metatarsal (Splint) Bones. In Equine Fracture Repair; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2019; pp. 465–479. [Google Scholar] [CrossRef]
- Lescun, T.B.; McClure, S.R.; Ward, M.P.; Downs, C.; Wilson, D.A.; Adams, S.B.; Hawkins, J.F.; Reinertson, E.L. Evaluation of Transfixation Casting for Treatment of Third Metacarpal, Third Metatarsal, and Phalangeal Fractures in Horses: 37 Cases (1994–2004). J. Am. Vet. Med. Assoc. 2007, 230, 1340–1349. [Google Scholar] [CrossRef] [PubMed]
- Brianza, S.; Brighenti, V.; Lansdowne, J.L.; Schwieger, K.; Bouré, L. Finite Element Analysis of a Novel Pin-Sleeve System for External Fixation of Distal Limb Fractures in Horses. Vet. J. 2011, 190, 260–267. [Google Scholar] [CrossRef]
- Galuppo, L.D.; Stover, S.M.; Aldridge, A.; Hewes, C.; Taylor, K.T. An In Vitro Biomechanical Investigation of an MP35N Intramedullary Interlocking Nail System for Repair of Third Metacarpal Fractures in Adult Horses. Vet. Surg. 2002, 31, 211–225. [Google Scholar] [CrossRef] [PubMed]
- Berg, E.L.; Causey, A. The Life-Changing Power of the Horse: Equine-Assisted Activities and Therapies in the U.S. Anim. Front. 2014, 4, 72–75. [Google Scholar] [CrossRef]
- Borioni, N.; Marinaro, P.; Celestini, S.; Del Sole, F.; Magro, R.; Zoppi, D.; Mattei, F.; Dall’ Armi, V.; Mazzarella, F.; Cesario, A.; et al. Effect of Equestrian Therapy and Onotherapy in Physical and Psycho-Social Performances of Adults with Intellectual Disability: A Preliminary Study of Evaluation Tools Based on the ICF Classification. Disabil. Rehabil. 2012, 34, 279–287. [Google Scholar] [CrossRef]
- Diab, S.S.; Stover, S.M.; Carvallo, F.; Nyaoke, A.C.; Moore, J.; Hill, A.; Arthur, R.; Uzal, F.A. Diagnostic Approach to Catastrophic Musculoskeletal Injuries in Racehorses. J. Vet. Diagn. Investig. 2017, 29, 405–413. [Google Scholar] [CrossRef] [PubMed]
- Wright, I.M. Fractures of the Distal Condyles of the Third Metacarpal and Third Metatarsal Bones; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2022; pp. 445–484. [Google Scholar] [CrossRef]
- Choi, J.; Seo, H.J.; Shin, J.; Byun, J.H.; Jung, S.N. The Effect of Steroid and Mannitol Combination Treatment on Postoperative Rehabilitation of Multiple Metacarpal Bone Fractures. Medicina 2023, 59, 783. [Google Scholar] [CrossRef]
- Stewart, S.; Richardson, D.; Boston, R.; Schaer, T.P. Risk Factors Associated with Survival to Hospital Discharge of 54 Horses with Fractures of the Radius. Vet. Surg. 2015, 44, 1036–1041. [Google Scholar] [CrossRef]
- Bischofberger, A.S.; Fürst, A.; Auer, J.; Lischer, C. Surgical Management of Complete Diaphyseal Third Metacarpal and Metatarsal Bone Fractures: Clinical Outcome in 10 Mature Horses and 11 Foals. Equine Vet. J. 2009, 41, 465–473. [Google Scholar] [CrossRef]
- Kawcak, C.E. Bone Healing. In Fractures in the Horse; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2022; pp. 97–115. ISBN 9781119431749. [Google Scholar]
- Ercin, E.; Hurmeydan, O.M.; Karahan, M. Bone Anatomy and the Biologic Healing Process of a Fracture. In Bio-Orthopaedics: A New Approach; Springer: Berlin/Heidelberg, Germany, 2017; pp. 437–447. [Google Scholar] [CrossRef]
- Allen, M.; Burr, D. Chapter 4. Bone Modeling and Remodeling. In Basic and Applied Bone Biology; Academic Press: Cambridge, MA, USA, 2014; pp. 75–90. ISBN 9780124160156. [Google Scholar]
- Chamay, A.; Tschantz, P. Mechanical Influences in Bone Remodeling. Experimental Research on Wolff’s Law. J. Biomech. 1972, 5, 173–180. [Google Scholar] [CrossRef]
- Hadjiargyrou, M.; O’Keefe, R.J. The Convergence of Fracture Repair and Stem Cells: Interplay of Genes, Aging, Environmental Factors and Disease. J. Bone Miner. Res. 2014, 29, 2307–2322. [Google Scholar] [CrossRef]
- Firth, E.C.; Goodship, A.E.; Delahunt, J.; Smith, T. Osteoinductive Response in the Dorsal Aspect of the Carpus of Young Thoroughbreds in Training Occurs within Months. Equine Vet. J. Suppl. 1999, 30, 552–554. [Google Scholar] [CrossRef]
- Oryan, A.; Monazzah, S.; Bigham-Sadegh, A. Bone Injury and Fracture Healing Biology. Biomed. Environ. Sci. 2015, 28, 57–71. [Google Scholar] [CrossRef]
- Ortved, K.F.; Richardson, D.W. Complications of Equine Orthopedic Surgery. In Complications in Equine Surgery; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2021; pp. 629–666. ISBN 9781119190332. [Google Scholar]
- Lescun, T.B. Complications of Splint Bone Fractures. In Complications in Equine Surgery; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2021; pp. 718–729. ISBN 9781119190332. [Google Scholar]
- Levine, D.G.; Richardson, D.W. Clinical Use of the Locking Compression Plate (LCP) in Horses: A Retrospective Study of 31 Cases (2004–2006). Equine Vet. J. 2007, 39, 401–406. [Google Scholar] [CrossRef] [PubMed]
- Pilliner, S.; Elmhurst, S.; Davies, Z. The Horse in Motion; Willey-Blackweell: Hoboken, NJ, USA, 2002; pp. 197–202. ISBN 063205137X. [Google Scholar]
- Clayton, H.M.; Hobbs, S.J. Reference to Collected Trot, Passage and Piaffe in Dressage Horses. Animals 2019, 9, 763–782. [Google Scholar] [CrossRef] [PubMed]
- Merritt, J.S.; Pandy, M.G.; Brown, N.A.T.; Burvill, C.R.; Kawcak, C.E.; McIlwraith, C.W.; Davies, H.M.S. Mechanical Loading of the Distal End of the Third Metacarpal Bone in Horses during Walking and Trotting. J. Am. Vet. Med. Assoc. 2010, 71, 508–514. [Google Scholar] [CrossRef]
- Voutat, C.; Nohava, J.; Wandel, J.; Zysset, P. The Dynamic Friction Coefficient of the Wet Bone-Implant Interface: Influence of Load, Speed, Material and Surface Finish. Biotribology 2019, 17, 64–74. [Google Scholar] [CrossRef]
- Beaupré, G.S.; Orr, T.E.; Carter, D.R. An Approach for Time-Dependent Bone Modeling and Remodeling—Theoretical Development. J. Orthop. Res. 1990, 8, 651–661. [Google Scholar] [CrossRef]
- Toma, M.; Singh-Gryzbon, S.; Frankini, E.; Wei, Z.; Yoganathan, A.P. Clinical Impact of Computational Heart Valve Models. Materials 2022, 15, 3302. [Google Scholar] [CrossRef]
- Klekiel, T.; Arkusz, K.; Sławiński, G.; Malesa, P.; Będziński, R. Numerical Analyses of Fracture Mechanism of the Pelvic Ring during Side-Impact Load. Materials 2022, 15, 5734. [Google Scholar] [CrossRef]
- Sybilski, K.; Fernandes, F.A.O.; Ptak, M.; Alves de Sousa, R.J. Injury Biomechanics Evaluation of a Driver with Disabilities during a Road Accident—A Numerical Approach. Materials 2022, 15, 7956. [Google Scholar] [CrossRef] [PubMed]
- Barbosa, A.; Fernandes, F.A.O.; de Sousa, R.J.A.; Ptak, M.; Wilhelm, J. Computational Modeling of Skull Bone Structures and Simulation of Skull Fractures Using the YEAHM Head Model. Biology 2020, 9, 267. [Google Scholar] [CrossRef] [PubMed]
- Kuc, A.E.; Sybilski, K.; Kotuła, J.; Piątkowski, G.; Kowala, B.; Lis, J.; Saternus, S.; Sarul, M. The Hydrostatic Pressure Distribution in the Periodontal Ligament and the Risk of Root Resorption—A Finite Element Method (FEM) Study on the Nonlinear Innovative Model. Materials 2024, 17, 1661. [Google Scholar] [CrossRef]
- ABAQUS. Version 6.6. Analysis User’s Manual Documentation; Washington University in St. Louis: St. Louis, MO, USA, 2009. [Google Scholar]
- Nowicki, A.; Osypko, K.; Kurzawa, A.; Roszak, M.; Krawiec, K.; Pyka, D. Mechanical and Material Analysis of 3D-Printed Temporary Materials for Implant Reconstructions—A Pilot Study. Biomedicines 2024, 12, 870. [Google Scholar] [CrossRef]
- Zhang, D.-N.; Shangguan, Q.-Q.; Xie, C.-J.; Liu, F. A Modified Johnson–Cook Model of Dynamic Tensile Behaviors for 7075-T6 Aluminum Alloy. J. Alloys Compd. 2015, 619, 186–194. [Google Scholar] [CrossRef]
- Murugesan, M.; Jung, D.W. Johnson Cook Material and Failure Model Parameters Estimation of AISI-1045 Medium Carbon Steel for Metal Forming Applications. Materials 2019, 12, 609. [Google Scholar] [CrossRef] [PubMed]
- Zochowski, P.; Bajkowski, M.; Grygoruk, R.; Magier, M.; Burian, W.; Pyka, D.; Bocian, M.; Jamroziak, K. Comparison of Numerical Simulation Techniques of Ballistic Ceramics under Projectile Impact Conditions. Materials 2022, 15, 18. [Google Scholar] [CrossRef]
- Atkins, A.; Dean, M.N.; Habegger, M.L.; Motta, P.J.; Ofer, L.; Repp, F.; Shipov, A.; Weiner, S.; Currey, J.D.; Shahar, R. Remodeling in Bone without Osteocytes: Billfish Challenge Bone Structure-Function Paradigms. Proc. Natl. Acad. Sci. USA 2014, 111, 16047–16052. [Google Scholar] [CrossRef]
- Bigley, R.F.; Gibeling, J.C.; Stover, S.M.; Hazelwood, S.J.; Fyhrie, D.P.; Martin, R.B. Volume Effects on Yield Strength of Equine Cortical Bone. J. Mech. Behav. Biomed. Mater. 2008, 1, 295–302. [Google Scholar] [CrossRef][Green Version]
- Lescun, T.B.; Adams, S.B.; Main, R.P.; Nauman, E.A.; Breur, G.J. Finite Element Analysis of Six Transcortical Pin Parameters and Their Effect on Bone-Pin Interface Stresses in the Equine Third Metacarpal Bone. Vet. Comp. Orthop. Traumatol. 2020, 33, 121–129. [Google Scholar] [CrossRef] [PubMed]
- Elkaseer, A.; Abdelaziz, A.; Saber, M.; Nassef, A. FEM-Based Study of Precision Hard Turning of Stainless Steel 316L. Materials 2019, 12, 2522. [Google Scholar] [CrossRef] [PubMed]
- Harbin, Z.; Sohutskay, D.; Vanderlaan, E.; Fontaine, M.; Mendenhall, C.; Fisher, C.; Voytik-Harbin, S.; Tepole, A.B. Computational Mechanobiology Model Evaluating Healing of Postoperative Cavities Following Breast-Conserving Surgery. Comput. Biol. Med. 2023, 165, 107342. [Google Scholar] [CrossRef] [PubMed]
- Gharahi, H.; Garimella, H.T.; Chen, Z.J.; Gupta, R.K.; Przekwas, A. Mathematical Model of Mechanobiology of Acute and Repeated Synaptic Injury and Systemic Biomarker Kinetics. Front. Cell. Neurosci. 2023, 17, 1007062. [Google Scholar] [CrossRef]
- Mao, W.; Huai, Y.; Wang, X.; Hu, L.; Qian, A.; Chen, Z. Chapter 2—Methods and Models of Bone Cell Mechanobiology. In Bone Cell Biomechanics, Mechanobiology and Bone Diseases; Qian, A., Hu, L., Mechanobiology and Bone Diseases, L.B.T.-B.C.B., Eds.; Academic Press: Cambridge, MA, USA, 2024; pp. 31–52. ISBN 978-0-323-96123-3. [Google Scholar]
- Martin, R.B. Toward a Unifying Theory of Bone Remodeling. Bone 2000, 26, 1–6. [Google Scholar] [CrossRef]
- Hernandez, C.J.; Beaupré, G.S.; Carter, D.R. A Model of Mechanobiologic and Metabolic Influences on Bone Adaptation. J. Rehabil. Res. Dev. 2000, 37, 235–244. [Google Scholar]
- Słowiński, J. Analysis of the Stress State in the Design of an Individual Bone Implant. Doctoral Thesis, Wrocław University of Science and Technology Publishing House, Wrocław, Poland, 2011. [Google Scholar]
- Carol, M.; Granella, S.; Souza, F. De External Skeletal Fixation in Large Animals: A Review. Appl. Vet. Res. 2024, 3, 2024004. [Google Scholar]
- Brabon, A.; Hughes, K.J.; Jensen, K.; Xie, G.; Labens, R. Influence of Screw Configuration on Reduction and Stabilization of Simulated Complete Lateral Condylar Fracture in Equine Limbs. Vet. Surg. 2024, 53, 447–459. [Google Scholar] [CrossRef]
- Fürst, A.E.; Jackson, M.A. Comminuted Fractures of the Proximal Third of the Fourth Metatarsal Bone: Treatment Strategies. Equine Vet. Educ. 2023, 35, 628–631. [Google Scholar] [CrossRef]

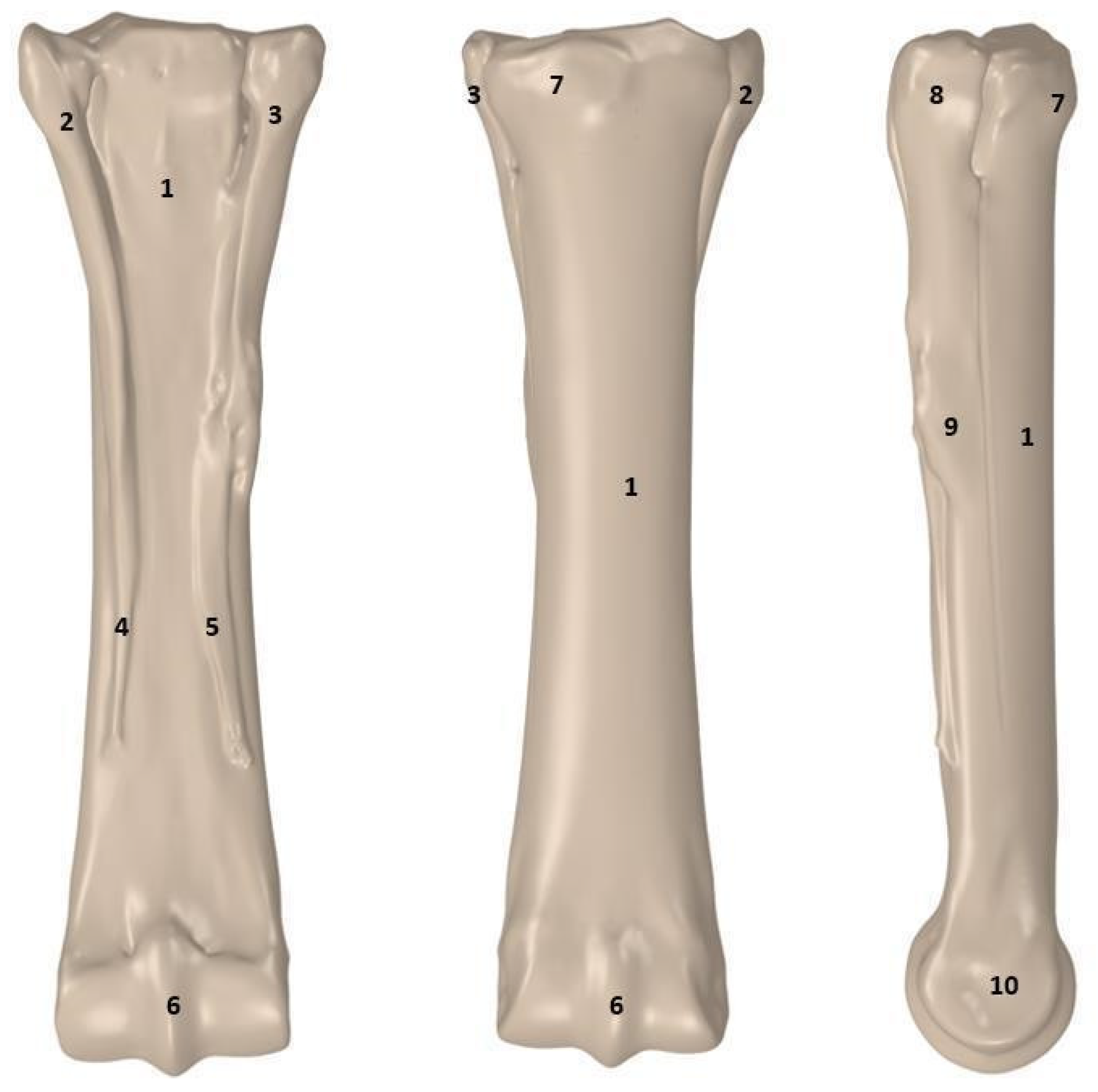
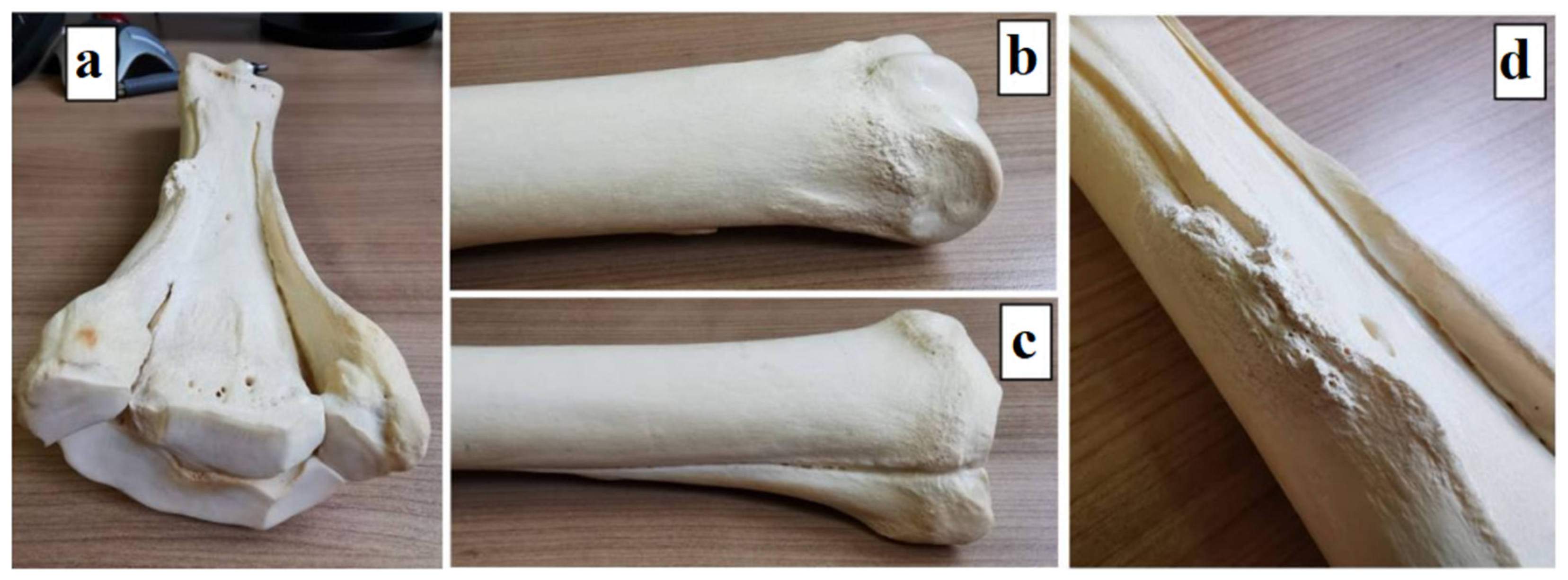

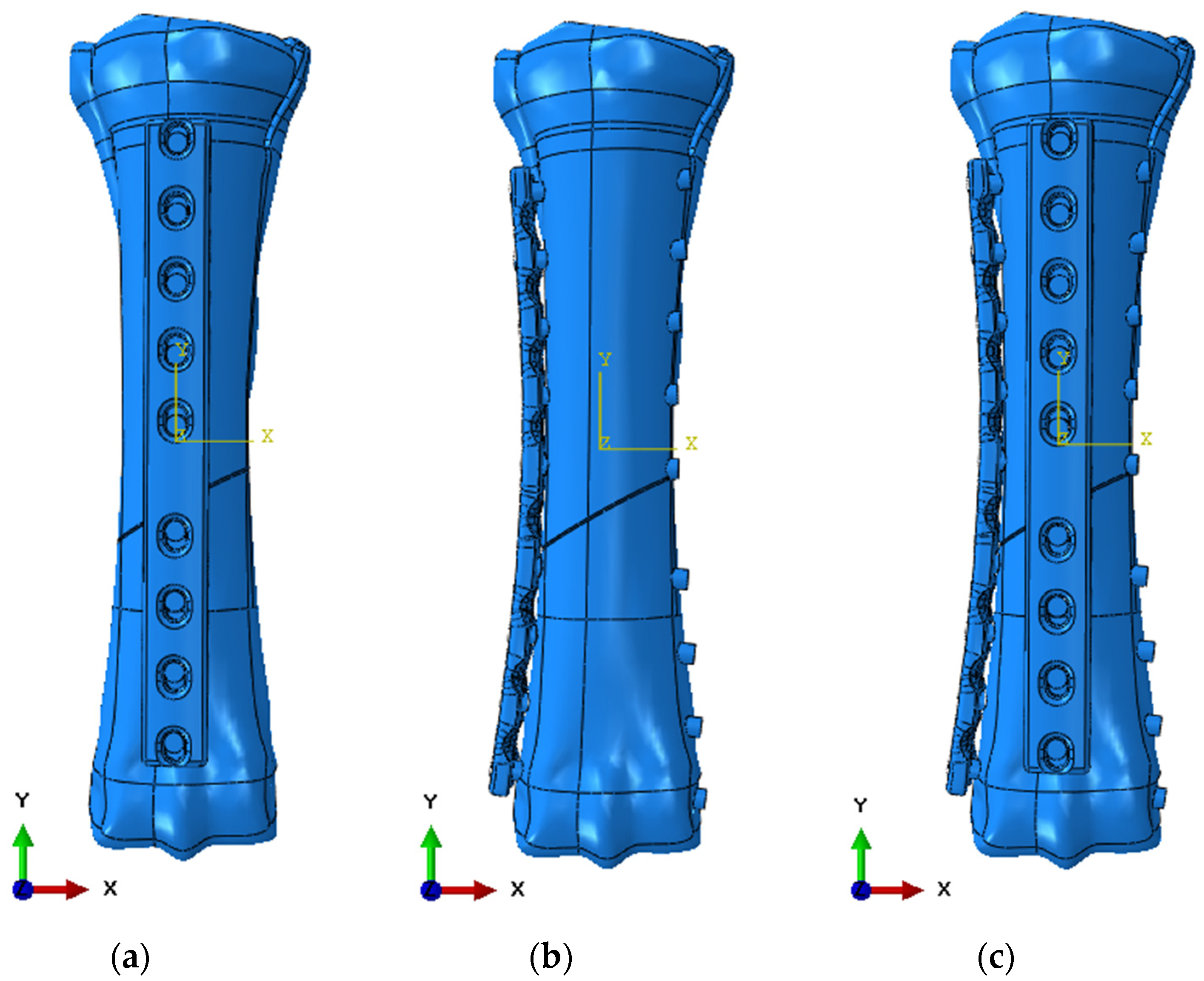

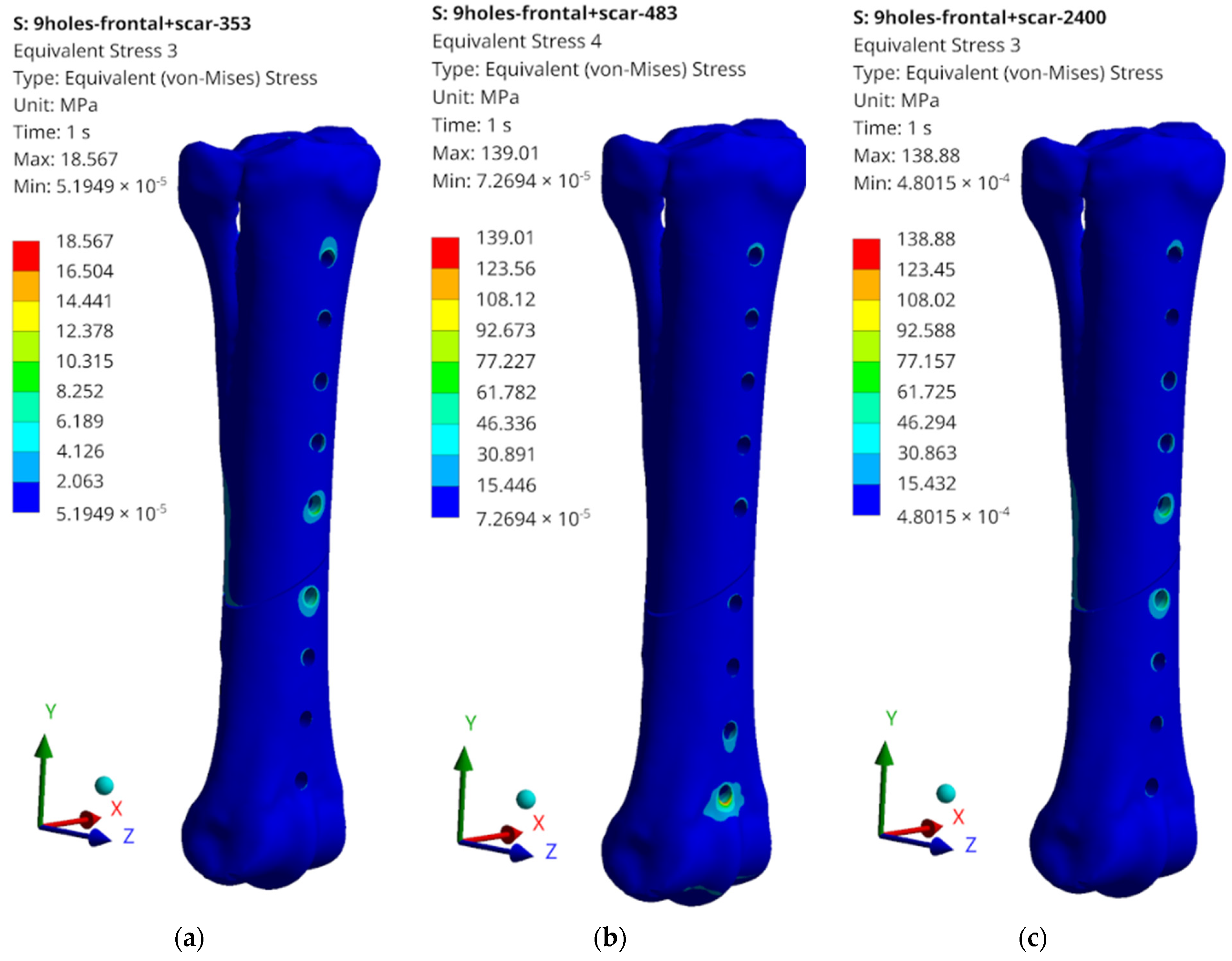
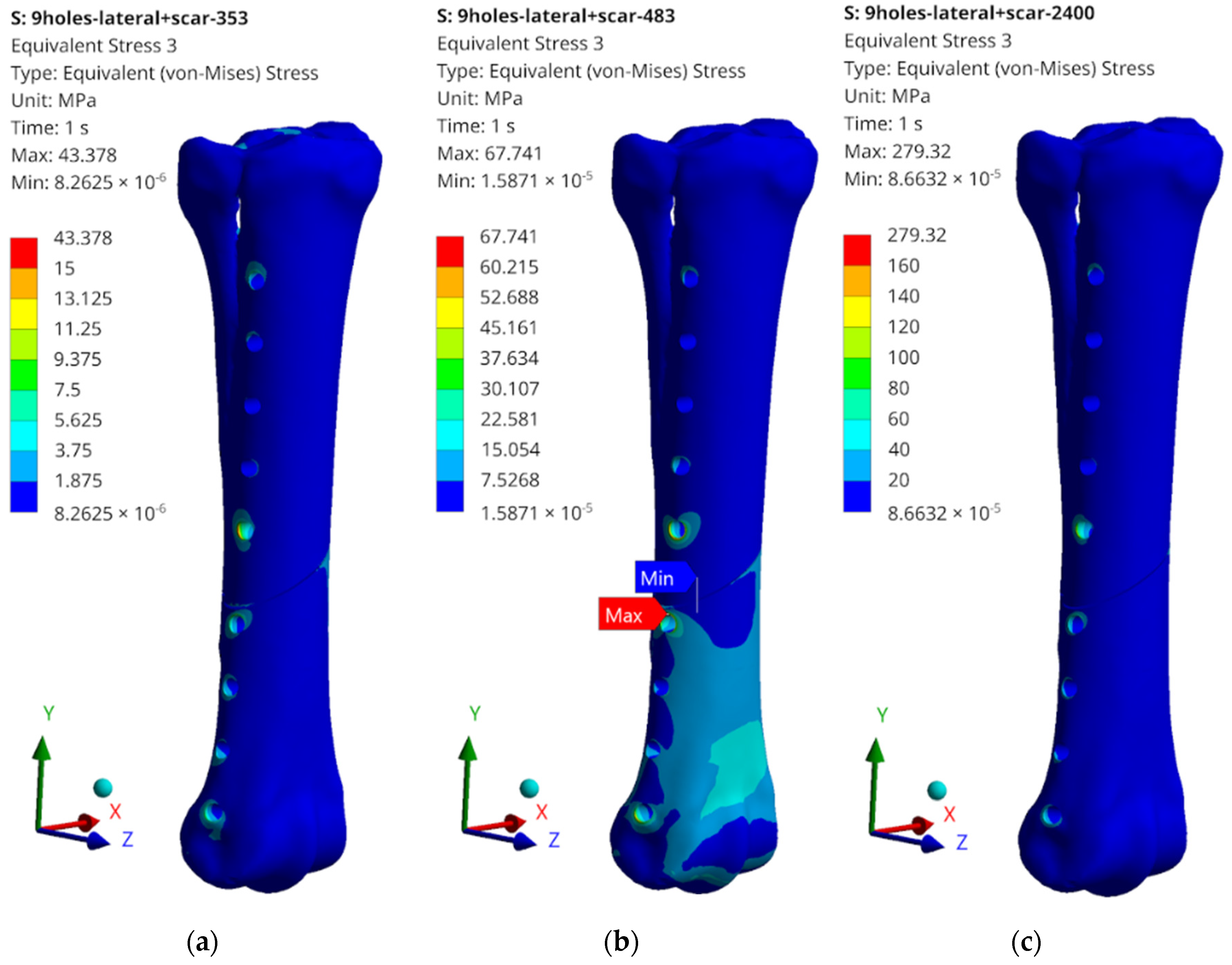


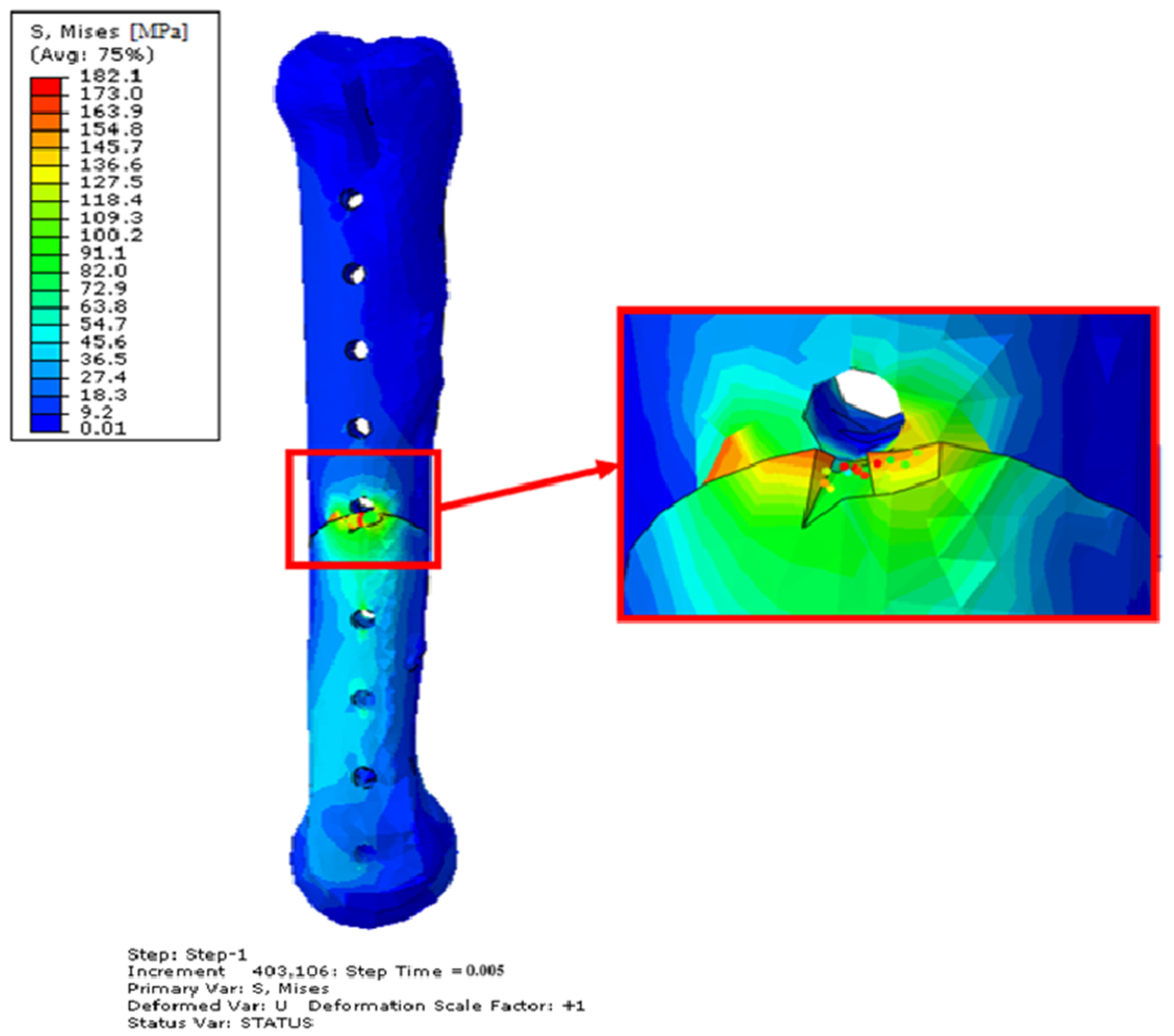

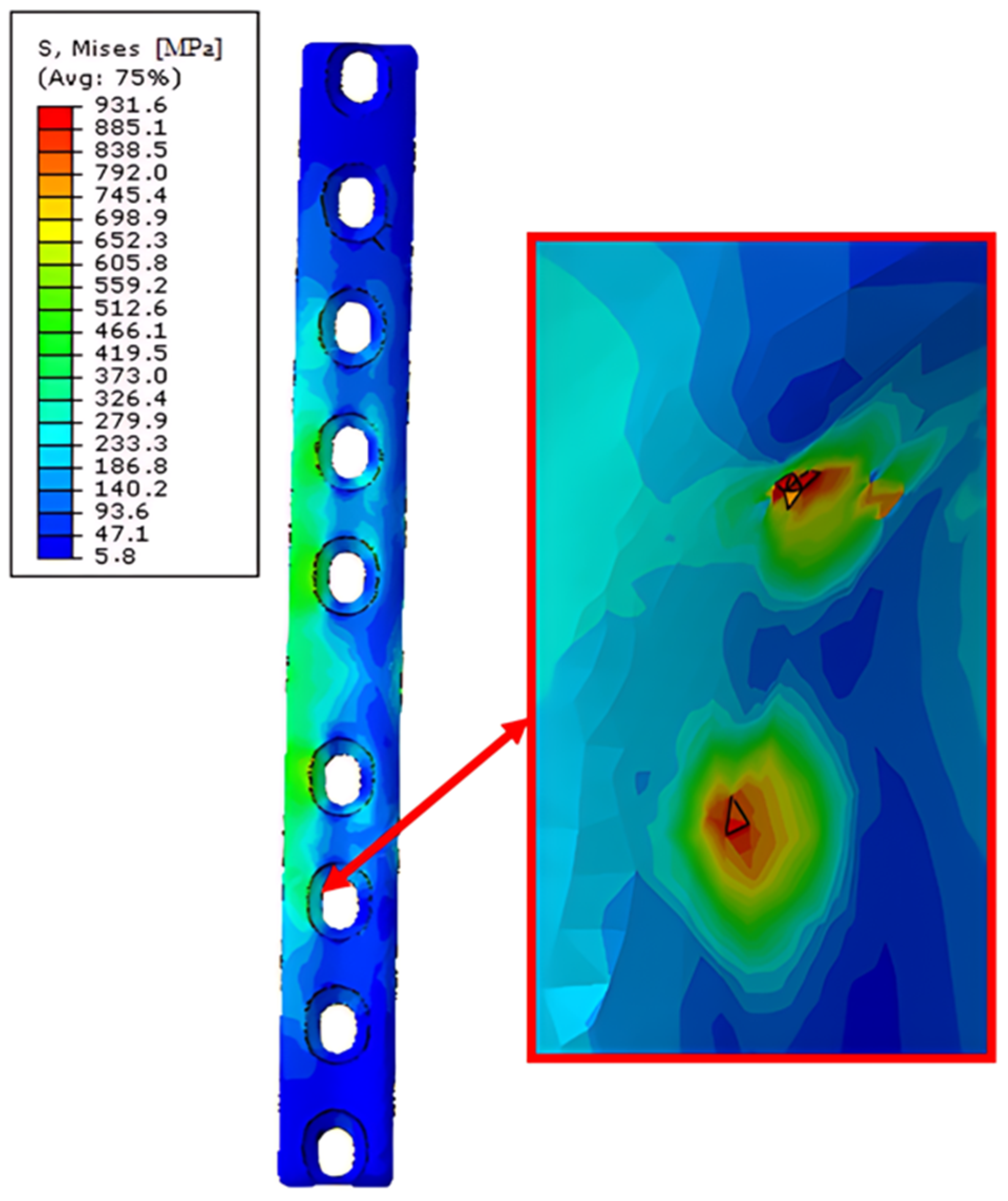

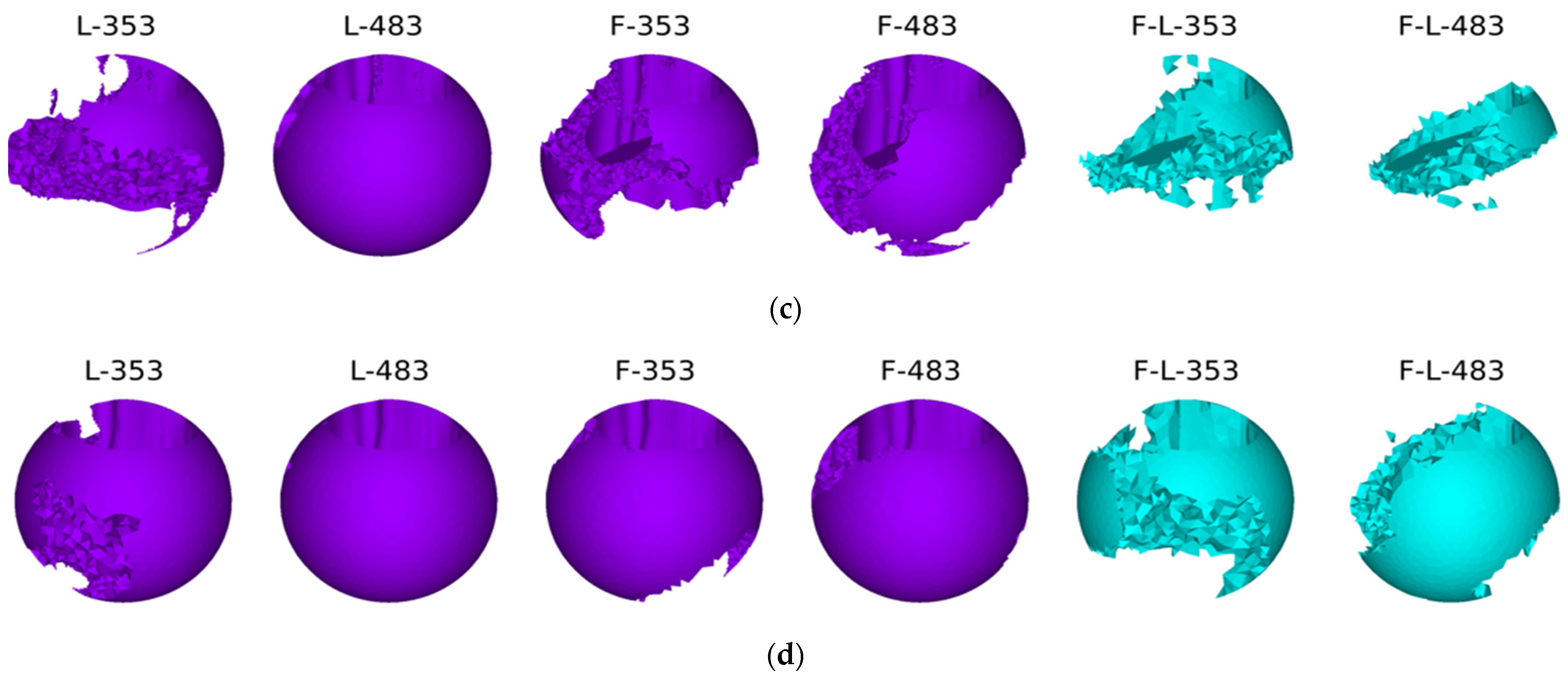
| Type of Stabilization | Maximum H–M–H Stress [MPa] | ||
|---|---|---|---|
| Lateral Stabilizer | Frontal Stabilizer | Bone | |
| Frontal-353 N | - | 161.0 | 18.6 |
| Lateral-353 N | 351.1 | - | 43.4 |
| Anterolateral-353 N | 241.7 | 80.6 | 16.2 |
| Frontal-483 N | - | 403.2 | 139.0 |
| Lateral-483 N | 518.3 | - | 67.7 |
| Anterolateral-483 N | 118.0 | 290.6 | 117.4 |
| Frontal-2400 N | - | 1202.7 | 138.9 |
| Lateral-2400 N | 2436.2 | - | 279.3 |
| Anterolateral-2400 N | 1734.7 | 578.3 | 115.5 |
| Type of Stabilization | Maximum H–M–H Stress [MPa] | ||
|---|---|---|---|
| Lateral Stabilizer | Frontal Stabilizer | Bone | |
| Frontal | - | 675.2 | 163.8 |
| Lateral | 931.6 | - | 189.0—destruction |
| Anterolateral | 1032.0 | 362.9 | 189.0—destruction |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Słowiński, J.; Roszak, M.; Krawiec, K.; Henklewski, R.; Jamroziak, K. Numerical Analysis of Stabilization of a Horse’s Third Metacarpal Bone Fracture for Prediction of the Possibility of Bone Union. Appl. Sci. 2024, 14, 7976. https://doi.org/10.3390/app14177976
Słowiński J, Roszak M, Krawiec K, Henklewski R, Jamroziak K. Numerical Analysis of Stabilization of a Horse’s Third Metacarpal Bone Fracture for Prediction of the Possibility of Bone Union. Applied Sciences. 2024; 14(17):7976. https://doi.org/10.3390/app14177976
Chicago/Turabian StyleSłowiński, Jakub, Maciej Roszak, Karina Krawiec, Radomir Henklewski, and Krzysztof Jamroziak. 2024. "Numerical Analysis of Stabilization of a Horse’s Third Metacarpal Bone Fracture for Prediction of the Possibility of Bone Union" Applied Sciences 14, no. 17: 7976. https://doi.org/10.3390/app14177976
APA StyleSłowiński, J., Roszak, M., Krawiec, K., Henklewski, R., & Jamroziak, K. (2024). Numerical Analysis of Stabilization of a Horse’s Third Metacarpal Bone Fracture for Prediction of the Possibility of Bone Union. Applied Sciences, 14(17), 7976. https://doi.org/10.3390/app14177976







