A Study on the Amplification Effect and Optimum Control of the Intermediate Column–Lever Negative Stiffness Viscous Damper
Abstract
1. Introduction
2. Composition of the CLNVD and Solution of Its Amplification Coefficient
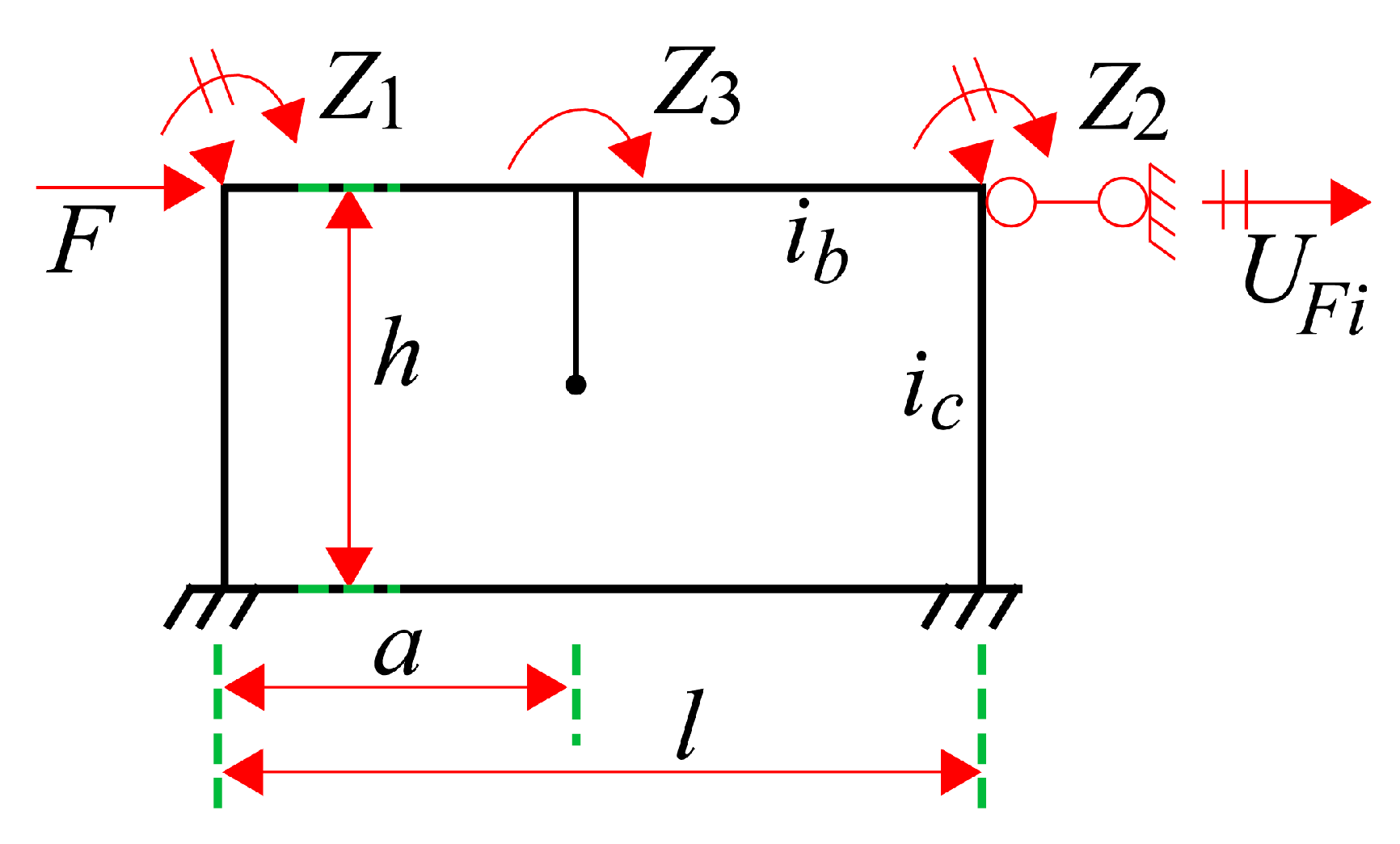
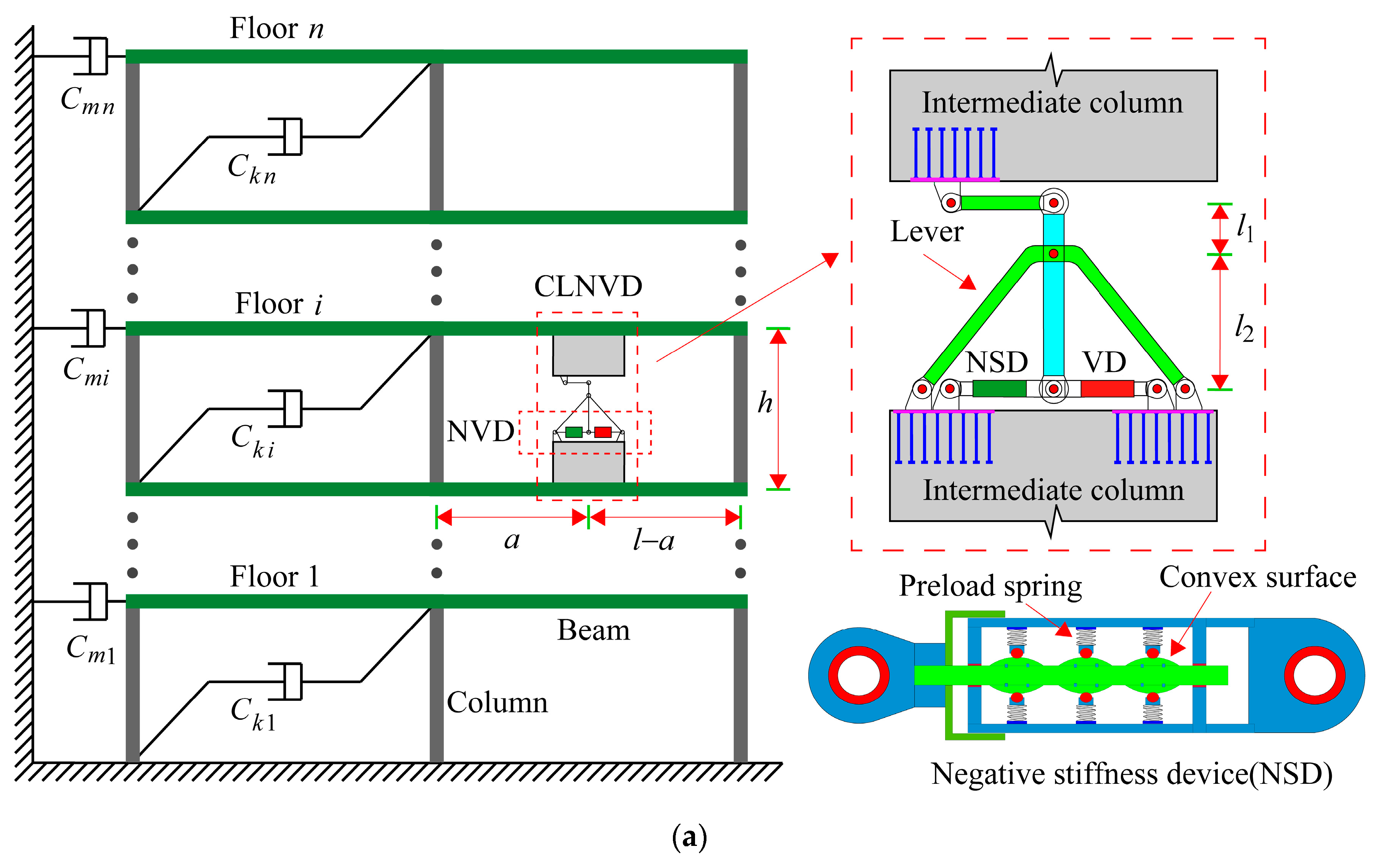
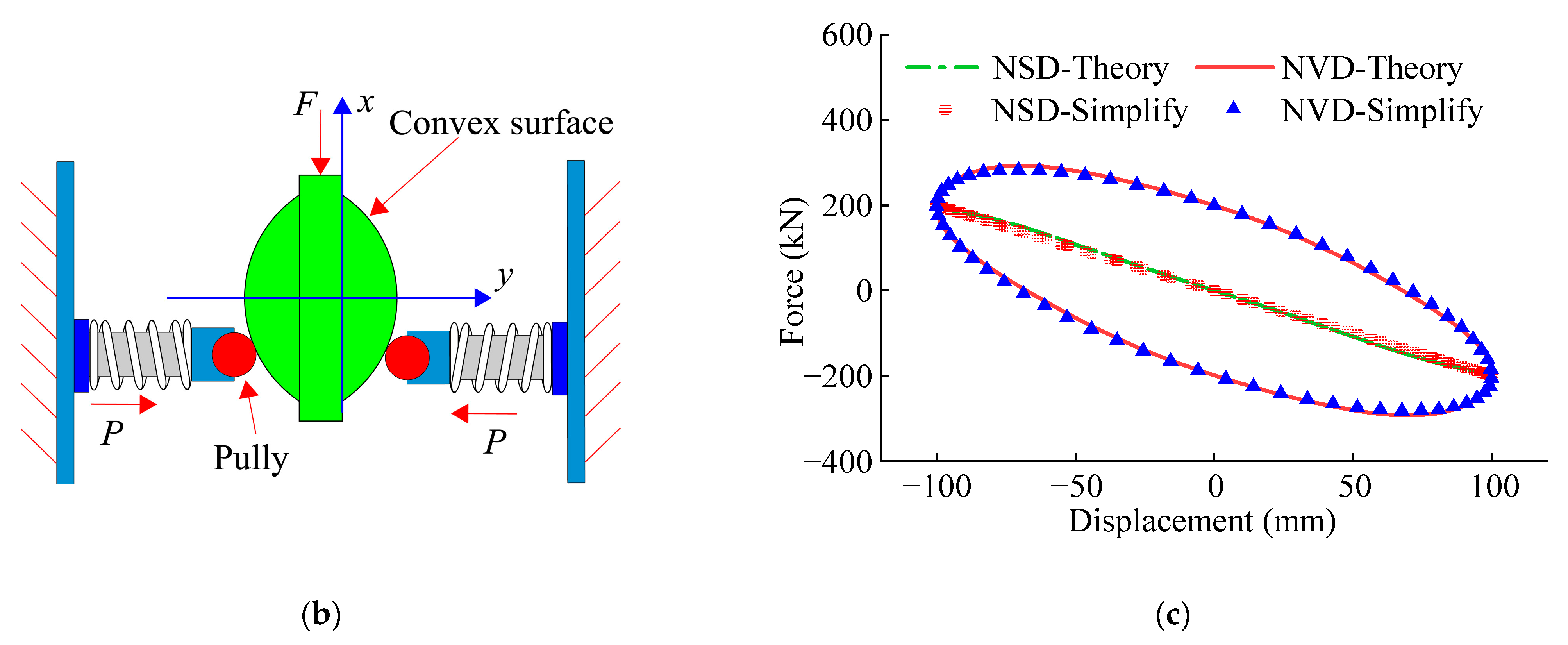
2.1. Composition and Features of the CLNVD
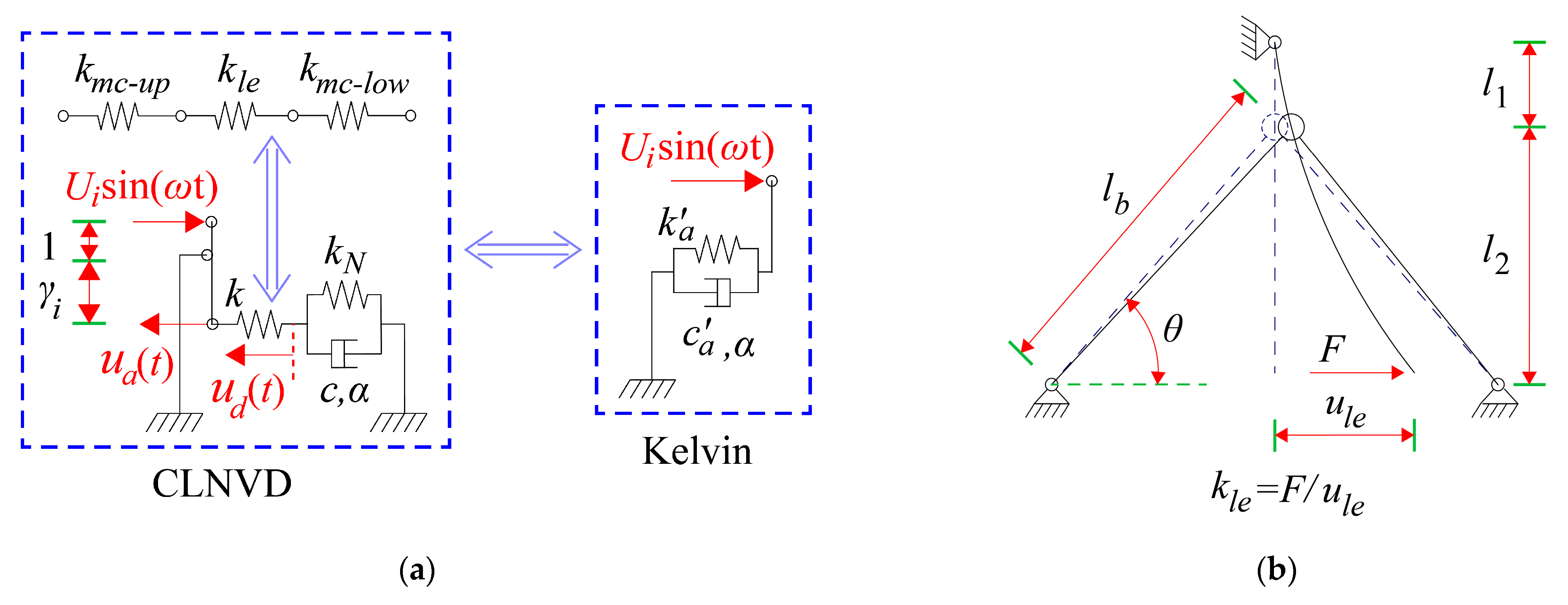
2.2. The CLNVD’s Displacement Amplification Coefficient and Energy Dissipation Coefficient
2.2.1. The CLNVD’s Geometric Amplification Coefficient
2.2.2. The CLNVD’s Effective Displacement Factor
3. Parameter Analysis and Optimization Design
- (1)
- The damper displacement is assumed to be equal to the inter-story displacement .
- (2)
- is substituted into Equation (17) for calculation, and Equations (20) and (21) are utilized to calculate and .
- (3)
- The error is calculated. When , is made to be equal to , and steps from 2 to 3 are repeated. When , the iteration ends.
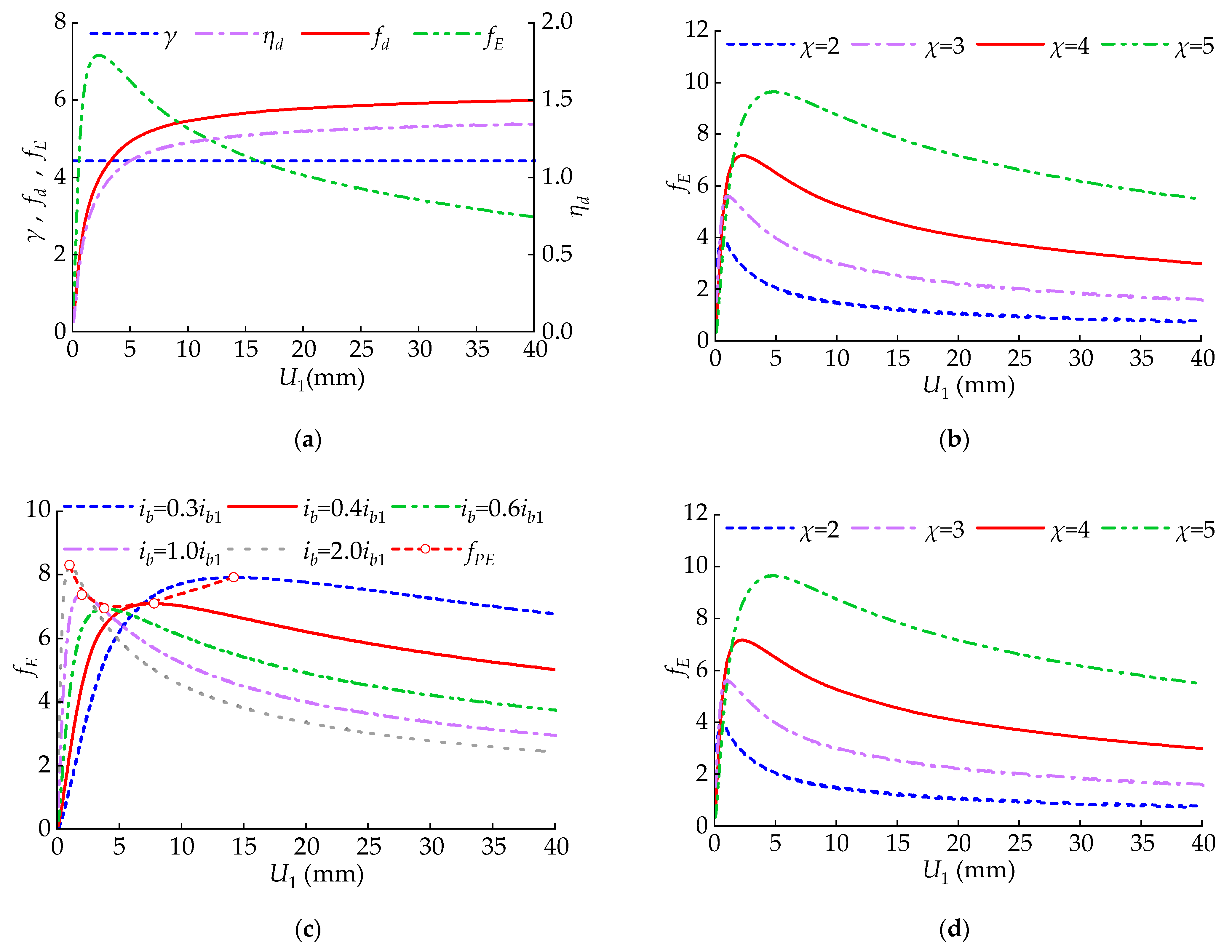
3.1. Parameter Analysis
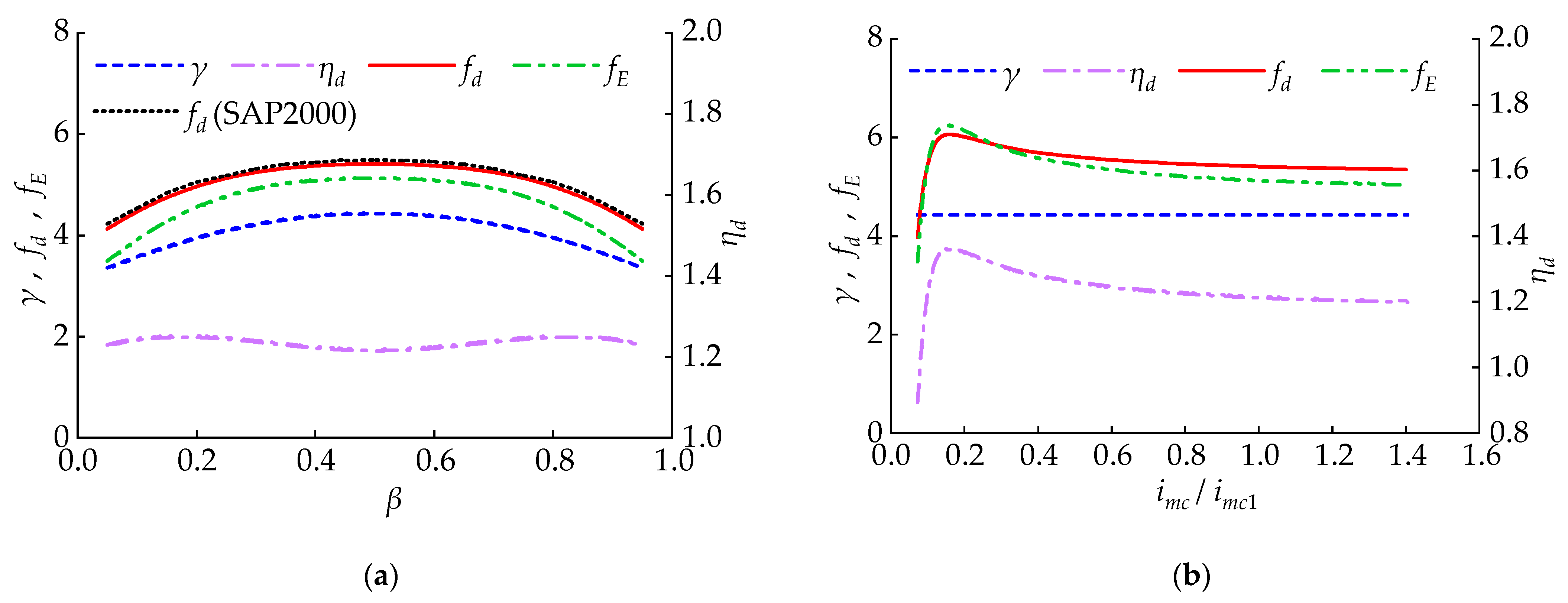
3.1.1. Impact of the Intermediate Column’s Position and Its Bending Line Stiffness
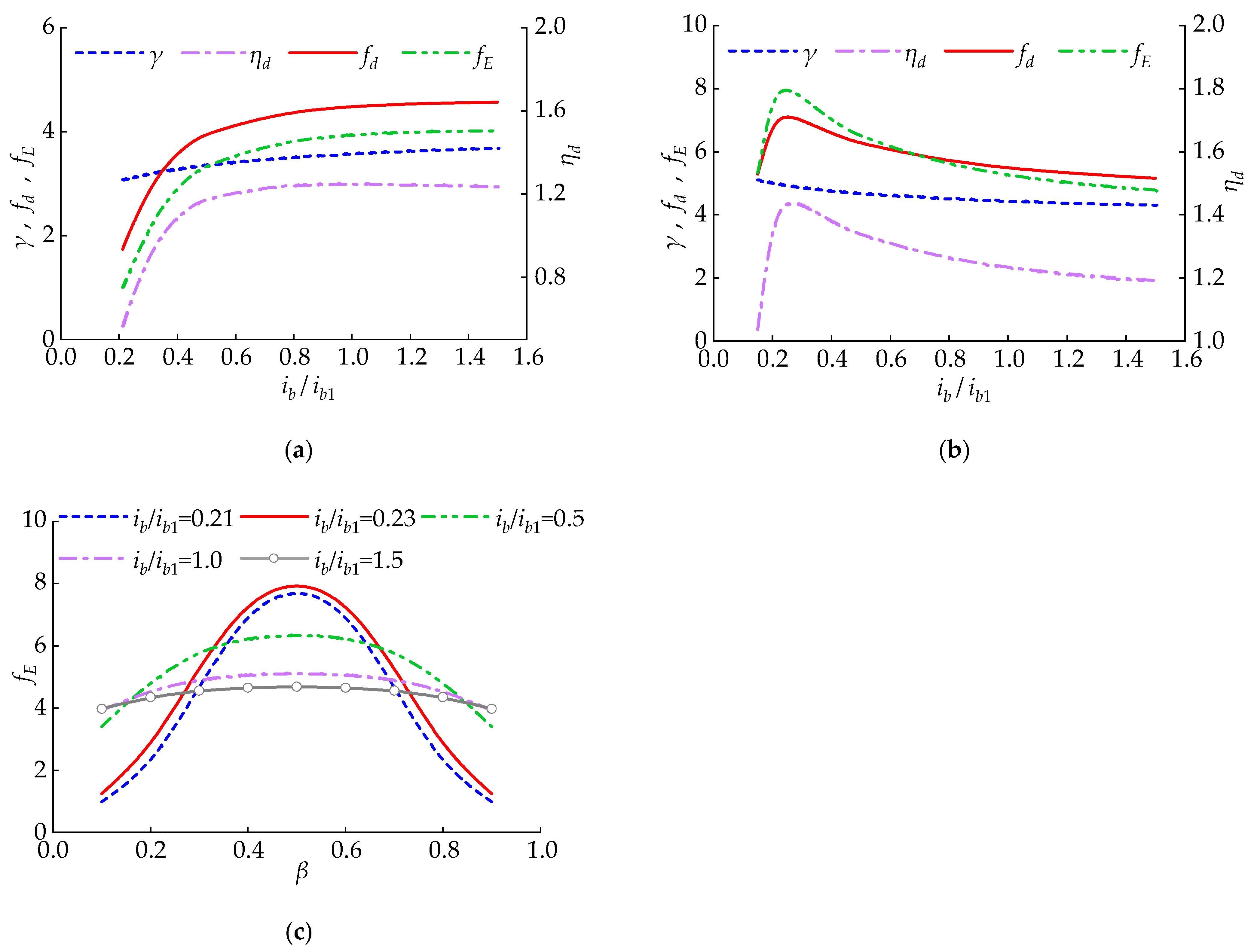
3.1.2. Impact of Beam’s Bending Line Stiffness
3.1.3. Impact of Lever’s Bending Line Stiffness and Leverage Amplification Coefficient
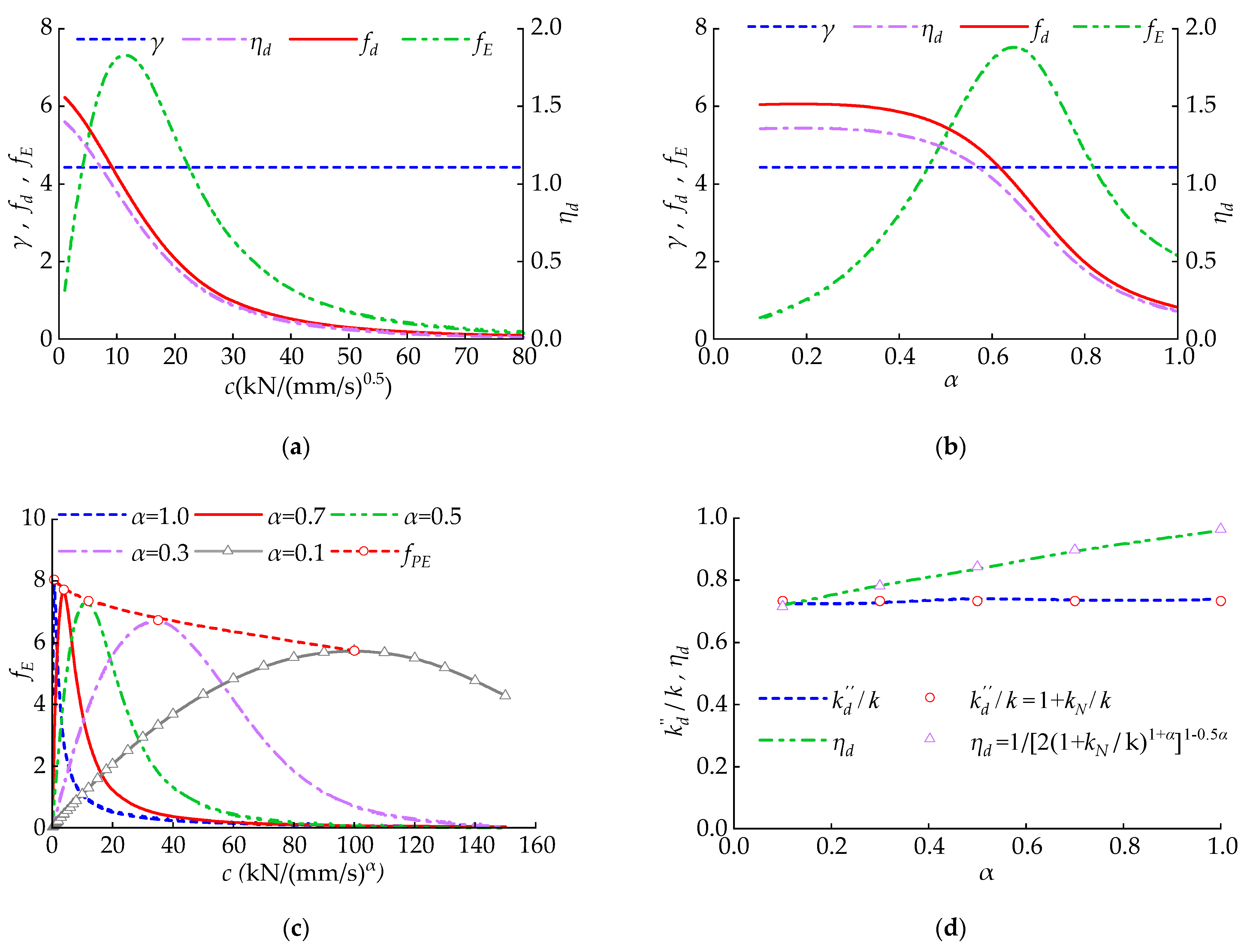
3.1.4. Impact of the Damping Coefficient and Damping Exponent
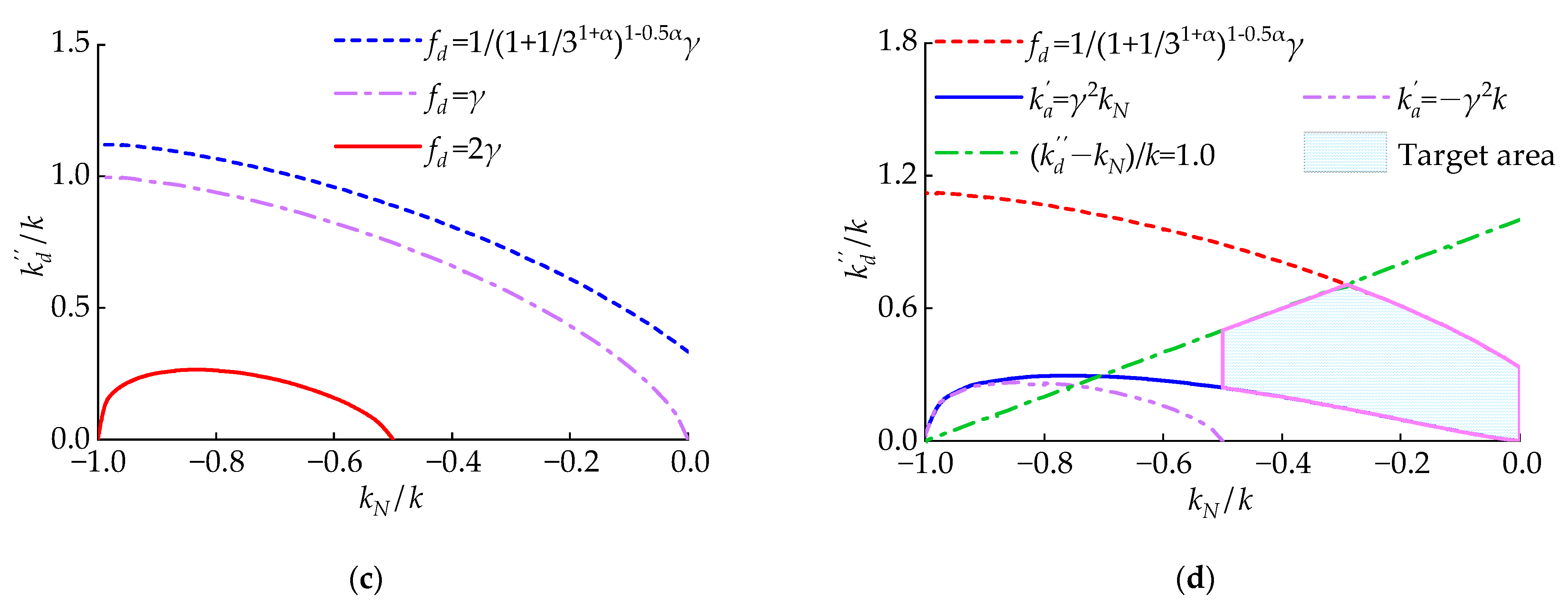
3.1.5. Impact of NSD’s Negative Stiffness
3.1.6. Impact of Inter-Story Displacement
3.2. CLNVD’s Optimum Design
3.2.1. Optimum Strategies for the CLNVD
- (1)
- The position of the intermediate column is selected, as well as the beam section and lever amplification factor ; the line stiffness ratio of beam to column is calculated. According to Equations (9) and (10), the geometric amplification coefficient is calculated. is judged to estimate whether it is higher in comparison with the pre-set target of . If it is not higher, the following methods are applied: to increase , or to decrease .
- (2)
- CLNVD’s parameters (such as intermediate column section, lever section, negative stiffness, damping coefficient, and damping index) are selected so that the values of and are within the target area (See Figure 11d). The inter-story displacement target value and the iterative method in Section 3.1 can be used to calculate ; the is calculated through Equation (18).
- (3)
- and are substituted into Equation (3) to calculate the displacement amplification coefficient , and Equation (4) is utilized to calculate the energy dissipation coefficient .
- (4)
- is judged if the predetermined goals are met. If not, the following methods are applied for optimization: to enhance the stiffness and damping coefficient ; to enhance the stiffness and the lever’s coefficient ; to increase the lever’s coefficient and reduce the damping coefficient ; or to reduce the NSD’s negative stiffness . It is necessary to verify again if and are within the region proposed in Section 3.1.5, and Step (3) and Step (4) should be repeated. If cannot meet the set target when is on the upper limit of the target region in Figure 11d, we should increase the beam section and return to Step (3).
3.2.2. Optimum Design for Structures with the CLNVD
- (1)
- The target values are set for , , maximum inter-story displacement angle, and additional damping ratio.
- (2)
- The stiffness ratio damping of the structure, the seismic response of structures without CLNVD, and the seismic response of structures with additional expected damping ratios are calculated. The floors with the CLNVD layout based on the seismic response of the structure without the CLNVD are selected, and the number of CLNVDs based on the building area (a ratio of building area to number of dampers between 100 and 200 is more reasonable) is selected. The number of CLNVDs for each floor based on the total number of CLNVDs and the layout floor is set. The parameters of CLNVD are set, and CLNVD is optimized according to Section 3.2.1.
- (3)
- The time history analysis is conducted under the circumstance of frequent or fortification earthquakes, and it is judged whether , , damping ratio, and displacement angle satisfy the goals. If not, we should increase the quantity of the CLNVDs or return to Step (2) for optimum strategies.
- (4)
- The time history analysis is conducted under the circumstance of rare earthquakes, and it is judged if the displacement angle satisfies the goals. If the goals are not satisfied, we should raise the quantity of the CLNVDs and return to Step (3).
4. Engineering Example Analysis
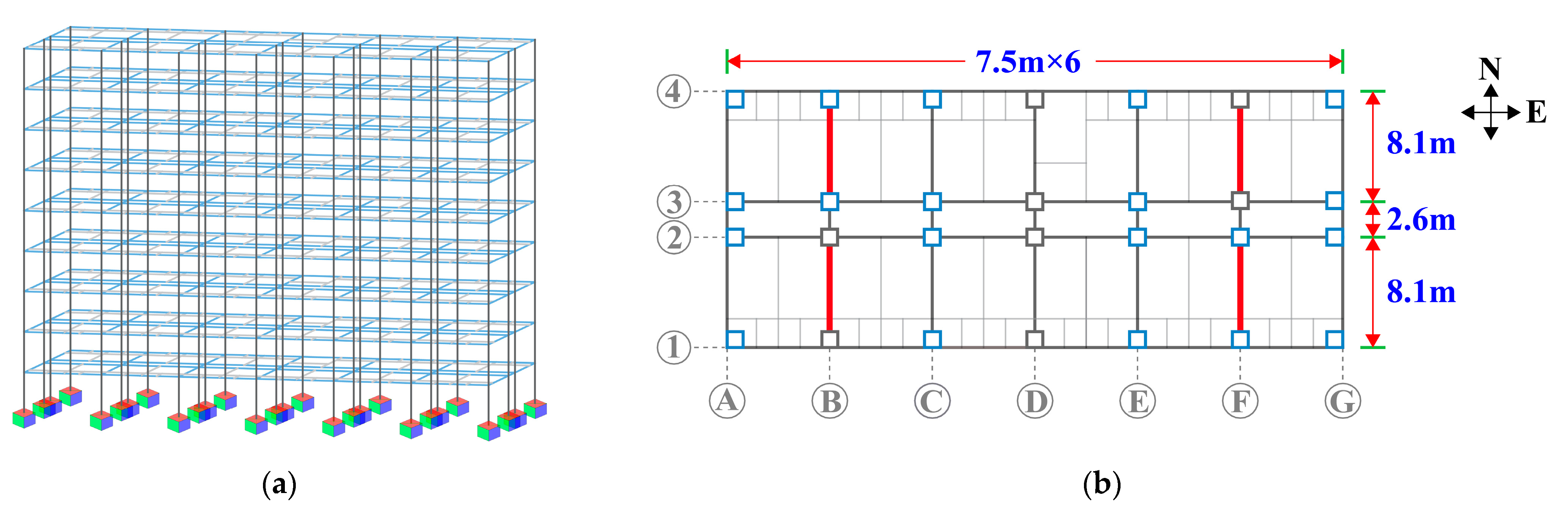
4.1. Project Overview
4.2. Scheme Design
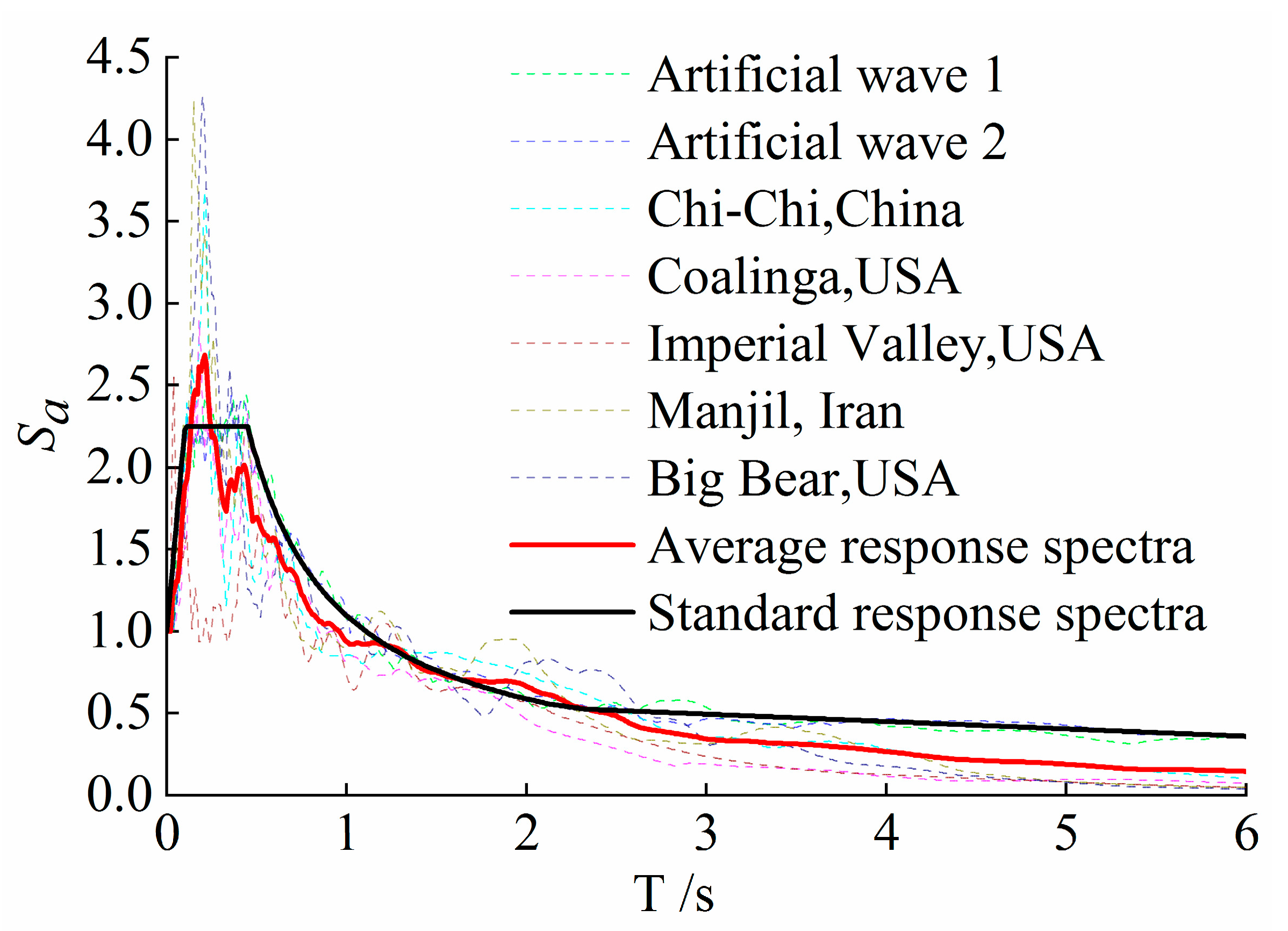
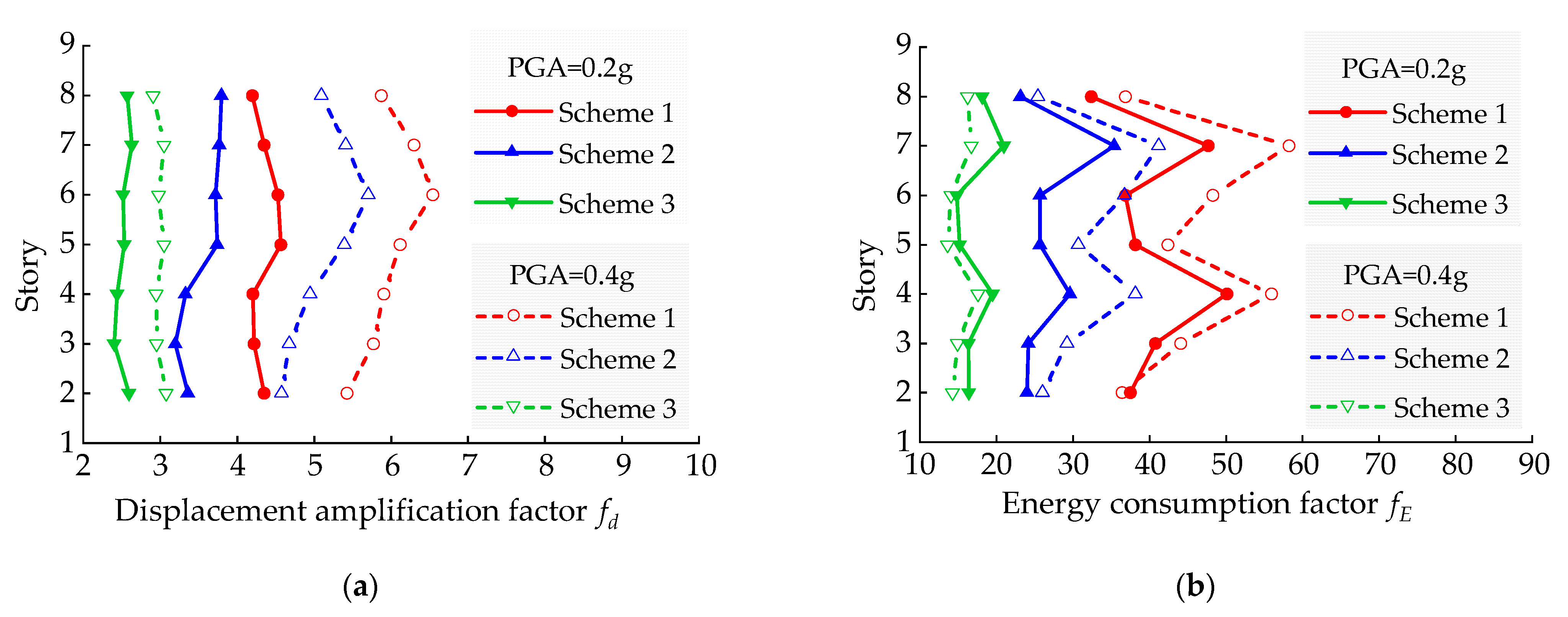
4.3. Vibration-Reduction Effect
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Cao, S.; Ozbulut, O.; Shi, F.; Deng, J.D. Experimental and numerical investigations on hysteretic response of a multi-level SMA/lead rubber bearing seismic isolation system. Smart Mater. Struct. 2022, 31, 035024. [Google Scholar] [CrossRef]
- Xu, H.; He, W.F.; Zhang, L.L.; Liu, W.G. Shaking table test of a novel Three-dimensional seismic isolation system with inclined rubber bearings. Eng. Struct. 2023, 293, 116609. [Google Scholar] [CrossRef]
- Frappa, G.; Pauletta, M. Seismic retrofitting of a reinforced concrete building with strongly different stiffness in the main directions. In Proceedings of the 14th FIB International PhD Symposium in Civil Engineering, Rome, Italy, 5–7 September 2022. [Google Scholar]
- Mazzon, L.; Frappa, G.; Pauletta, M. Effectiveness of tuned mass damper in reducing damage caused by strong earthquake in a medium-rise building. Appl. Sci. 2023, 13, 6815. [Google Scholar] [CrossRef]
- Gioiella, L.; Tubaldi, E.; Gara, F.; Dezi, L.; Dall’Asta, A. Modal properties and seismic behaviour of buildings equipped with external dissipative pinned rocking braced frames. Eng. Struct. 2018, 172, 807–819. [Google Scholar] [CrossRef]
- Zhou, Q.; Pan, W.; Lan, X. Analysis of Amplification Effect and Optimal Control of the Toggle-Style Negative Stiffness Viscous Damper. Buildings 2024, 14, 1625. [Google Scholar] [CrossRef]
- Ou, J.P.; Li, H. Design approaches for active, semi-active and passive control systems based on analysis of characteristics of active control force. Earthq. Eng. Eng. Vib. 2009, 8, 493–506. [Google Scholar] [CrossRef]
- Bhowmik, K.; Debnath, N. Semi-active Vibration Control of Soft-Storey Building with Magnetorheological Damper under Seismic Excitation. J. Vib. Eng. Technol. 2024, 12, 6943–6961. [Google Scholar] [CrossRef]
- Jamkhaneh, M.E.; Ebrahimi, A.H.; Amiri, M.S. Experimental and numerical investigation of steel moment resisting frame with U-shaped metallic yielding damper. Int. J. Steel Struct. 2019, 19, 806–818. [Google Scholar] [CrossRef]
- Rayegani, A.; Soureshjani, O.K.; Alaee, S.A.M.; Mualla, I.H.; Nemati, F. Seismic Performance of Buildings Equipped with Four-Joint Rotational Friction Dampers in Mainshock–Aftershock Sequences. J. Struct. Eng. 2024, 150, 04023235. [Google Scholar] [CrossRef]
- Naeem, A.; Kim, J. Seismic retrofit of structures using rotational friction dampers with restoring force. Adv. Struct. Eng. 2020, 23, 16. [Google Scholar] [CrossRef]
- Pollini, N.; Lavan, O.; Amir, O. Minimum–cost optimization of nonlinear fluid viscous dampers and their supporting members for seismic retrofitting. Earthq. Eng. Struct. Dyn. 2017, 46, 1941–1961. [Google Scholar] [CrossRef]
- Chen, Y.T.; Chai, Y.H. Effects of brace stiffness on performance of structures with supplemental Maxwell model–based brace–damper systems. Earthq. Eng. Struct. Dyn. 2011, 40, 75–92. [Google Scholar] [CrossRef]
- Li, S.Y.; Chen, Y.T.; Chai, Y.H. Effects of Brace Stiffness and Nonlinearity of Viscous Dampers on Seismic Performance of Structures. Int. J. Struct. Stab. Dyn. 2021, 21, 2150188. [Google Scholar] [CrossRef]
- Lan, X.; Pan, W.; Kuang, H.W. Correction additional damping of an energy–dissipation structure based on a story drifts utilization ratio method. J. Vib. Shock. 2017, 36, 64–71. (In Chinese) [Google Scholar]
- Çerçevik, A.E.; Avşar, Ö.; Dilsiz, A. Optimal placement of viscous wall dampers in RC moment resisting frames using metaheuristic search methods. Eng. Struct. 2021, 249, 113108. [Google Scholar] [CrossRef]
- Lan, X.; Zhang, L.F.; Sun, B.F.; Pan, W. Study on the Damping Efficiency of a Structure with Additional Viscous Dampers Based on the Shaking Table Test. Buildings 2024, 14, 1506. [Google Scholar] [CrossRef]
- Zhou, Q.; Pan, W.; Lan, X. Study on Vibration Reduction Effect of the Building Structure Equipped with Intermediate Column–Lever Viscous Damper. Buildings 2024, 14, 1881. [Google Scholar] [CrossRef]
- Sonmez, M.; Aydin, E.; Karabork, T. Using an artificial bee colony algorithm for the optimal placement of viscous dampers in planar building frames. Struct. Multidiscip. Optim. 2013, 48, 395–409. [Google Scholar] [CrossRef]
- Cetin, H.; Aydin, E.; Ozturk, B. Optimal design and distribution of viscous dampers for shear building structures under seismic excitations. Front. Built Environ. 2019, 5, 90. [Google Scholar] [CrossRef]
- Lan, X.; Wei, G.L.; Zhang, X.X. Study on the Influence and Optimization Design of Viscous Damper Parameters on the Damping Efficiency of Frame Shear Wall Structure. Buildings 2024, 14, 497. [Google Scholar] [CrossRef]
- Bishop, J.A.; Striz, A.G. On using genetic algorithms for optimum damper placement in space trusses. Struct. Multidiscip. Optim. 2004, 28, 2–3. [Google Scholar] [CrossRef]
- Sarcheshmehpour, M.; Estekanchi, H.E.; Ghannad, M.A. Optimum placement of supplementary viscous dampers for seismic rehabilitation of steel frames considering soil–structure interaction. Struct. Des. Tall Spec. Build. 2020, 29, e1682. [Google Scholar] [CrossRef]
- Patro, S.R.; Panda, S.; Ramana, G.V.; Banerjee, A. Optimal multiple tuned mass dampers for monopile supported offshore wind turbines using Genetic Algorithm. Ocean. Eng. 2024, 298, 11735. [Google Scholar] [CrossRef]
- Ribakov, Y.; Gluck, J.; Gluck, N. Practical design of MDOF structures with supplemental viscous dampers using mechanical levers. In Proceedings of the 8th ASCE Specialty Conference on Probabilistic Mechanics and Structural Reliability, Notre Dame, Indiana, 24–26 July 2000; The American Society of Civil Engineers: Reston, VA, USA, 2000. [Google Scholar]
- Ribakov, Y.; Reinhorn, A.M. Design of Amplified Structural Damping Using Optimal Considerations. J. Struct. Eng. 2003, 129, 1422–1427. [Google Scholar] [CrossRef]
- Aly, S.A.; Zasso, A.; Resta, F. Proposed Configurations for the Use of Smart Dampers with Bracings in Tall Buildings. Smart Mater. Res. 2012, 2012, 251543. [Google Scholar]
- Yang, Q.R.; Kong, L.X.; Guo, Y. Vibration reduction effect analysis of building structure equipped with displacement amplification oil damper. Earthq. Eng. Eng. Dyn. 2017, 37, 45–48. [Google Scholar]
- He, W.; Xu, H.; Xu, Z.; Liu, W. Experimental investigation and earthquake response analysis of a multilayer viscous damping wall with amplified deformation. Eng. Struct. 2022, 251, 113427. [Google Scholar] [CrossRef]
- Shang, F.; Liu, W.G.; Zhang, Q.; Xu, H.; Guo, Y. Shaking table test and seismic behaviour evaluation of viscous damping wall with amplification mechanism. Eng. Struct. 2023, 284, 115974. [Google Scholar] [CrossRef]
- Yang, L.Y.; Ye, M.; Huang, Y.H.; Dong, J.K. Study on Mechanical Properties of Displacement-Amplified Mild Steel Bar Joint Damper. Iran. J. Sci. Technol. Trans. Civ. Eng. 2023, 7, 01268. [Google Scholar] [CrossRef]
- Constantinou, M.C.; Tsopelas, P.; Hammel, W.; Sigaher, A.N. New configurations of fluid viscous dampers for improved performance. In Proceedings of the Passive Structural Control Symposium; Tokyo Institute of Technology: Yokohama, Japan, 2000. [Google Scholar]
- Constantinou, M.C.; Tsopelas, P.; Hammel, W.; Sigaher, A.N. Toggle–brace–damper seismic energy dissipation systems. J. Struct. Eng. 2001, 127, 105–112. [Google Scholar] [CrossRef]
- Taylor, D.P. Toggle brace dampers: A new concept for structural control. In Proceedings of the Structures Congress, Philadelphia, PA, USA, 8–10 May 2000; The American Society of Civil Engineers: Reston, VA, USA, 2000. [Google Scholar]
- Hwang, J.S.; Huang, Y.N.; Hung, Y.H. Analytical and experimental study of toggle–brace–damper systems. J. Struct. Eng. 2005, 131, 1035–1043. [Google Scholar] [CrossRef]
- Huang, H.C. Efficiency of the motion amplification device with viscous dampers and its application in high–rise buildings. Earthq. Eng. Eng. Vib. 2009, 8, 521–536. [Google Scholar] [CrossRef]
- Sigaher, A.N.; Constantinou, M.C. Scissor-jack-damper energy dissipation system. Earthq. Spectra. 2003, 19, 133–158. [Google Scholar] [CrossRef]
- Polat, E.; Constantinou, M.C. Open–space damping system description, theory, and verification. J. Struct. Eng. 2017, 143, 04016201. [Google Scholar] [CrossRef]
- Lan, B.J.; He, H.X.; Wu, S.; Cheng, S.T.; Chen, Y.F. Multi–performance objectives optimum design method and vibration control application of generalized toggle–brace–damper system. Earthq. Eng. Struct. Dyn. 2022, 51, 1410–1435. [Google Scholar] [CrossRef]
- Kang, J.D.; Tagawa, H. Seismic performance of steel structures with seesaw energy dissipation system using fluid viscous dampers. Eng. Struct. 2013, 56, 431–442. [Google Scholar] [CrossRef]
- Kang, J.D.; Tagawa, H. Experimental evaluation of dynamic characteristics of seesaw energy dissipation system for vibration control of structures. Earthq. Eng. Struct. Dyn. 2014, 43, 1889–1895. [Google Scholar] [CrossRef]
- Javanbakht, M.; Cheng, S.; Ghrib, F. Impact of support stiffness on the performance of negative stiffness dampers for vibration control of stay cables. Struct. Control Health Monit. 2020, 27, e2610. [Google Scholar] [CrossRef]
- Nagarajaiah, S.; Reinhorn, A.M.; Constantinou, M.C. True Adaptive Negative Stiffness: A New Structural Modification Approach for Seismic Protection. In Proceedings of the 5th World Conference on Structural Control and Monitoring, Tokyo, Japan, 12–14 July 2010. [Google Scholar]
- Pasala, D.T.R.; Sarlis, A.A.; Nagarajaiah, S.; Reinhorn, A.M.; Constantinou, M.C. Adaptive negative stiffness: New structural modification approach for seismic protection. J. Struct. Eng. 2013, 139, 1112–1123. [Google Scholar] [CrossRef]
- Pasala, D.T.R.; Sarlis, A.A.; Reinhorn, A.M.; Nagarajaiah, S.; Constantinou, M.C. Simulated bilinear-elastic behavior in a SDOF elastic structure using negative stiffness device: Experimental and analytical study. J. Struct. Eng. 2014, 140, 04013049. [Google Scholar] [CrossRef]
- Chen, L.; Sun, L.M.; Nagarajaiah, S. Cable with discrete negative stiffness device and viscous damper: Passive realization and general characteristics. Smart Struct. Syst. 2015, 15, 627–643. [Google Scholar] [CrossRef]
- Kwon, I.Y.; Yang, H.T.; Hansma, P.K.; Randall, C.J. Implementable Bio-Inspired Passive Negative Spring Actuator for Full-Scale Structural Control under Seismic Excitation. J. Struct. Eng. 2016, 142, 04015079. [Google Scholar] [CrossRef]
- Shi, X.; Zhu, S.Y.; Nagarajaiah, S. Performance comparison between passive negative stiffness damper and active control in cable vibration mitigation. J. Bridge Eng. 2017, 22, 04017054. [Google Scholar] [CrossRef]
- Sun, T.W.; Peng, L.Y.; Li, X.; Guan, Y.X. Developing and Applying a Double Triangular Damping Device with Equivalent Negative Stiffness for Base-Isolated Buildings. Buildings 2023, 13, 3008. [Google Scholar] [CrossRef]
- Zhou, P.; Liu, M.; Li, H. Optimized negative stiffness damper with flexible support for stay cables. Struct. Control Health Monit. 2021, 28, e2717. [Google Scholar] [CrossRef]
- Wang, M.; Sun, F.; Yang, J.Q.; Nagarajaiah, S. Seismic protection of SDOF systems with a negative stiffness amplifying damper. Eng. Struct. 2019, 190, 128–141. [Google Scholar] [CrossRef]
- Sun, T.; Li, H.N.; Lai, Z.L.; Nagarajaiah, S. Study on structural vibration control using curve-based negative stiffness device. J. Vib. Eng. 2017, 30, 449–456. [Google Scholar]
- Kasai, K.; Suzuki, A.; Oohara, K. Equivalent linearization of a passive control system having viscous dampers dependent on fractional power of velocity. J. Struct. Constr. Eng. (Trans. AIJ) 2003, 574, 77–84. (In Japanese) [Google Scholar] [CrossRef][Green Version]
- JGJ 297-2013; Chinese Standard, Technical Specification for Seismic Energy Dissipation of Buildings. Chinese Architecture and Building Press: Beijing, China, 2013.
- GB 50011-2016; Chinese Standard, Code for Seismic Design of Buildings. Chinese Architecture and Building Press: Beijing, China, 2016.












| 600 × 600 | 400 × 800 | 1600 × 250 | 0.5 | 4 | 5 | −2 |
| Story | Concrete Material | Column Section | Main Beam Section | Secondary Beam Section |
|---|---|---|---|---|
| (mm × mm) | (mm × mm) | (mm × mm) | ||
| 1 | C40 | 700 × 700 | 300 × 600 | 200 × 400 |
| 2 | C40 | 600 × 600 | 300 × 600 | 200 × 400 |
| 3–4 | C40 | 550 × 550 | 300 × 600 | 200 × 400 |
| 5–9 | C35 | 500 × 500 | 300 × 600 | 200 × 400 |
| Mode | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| Period (s) | 1.60 | 1.58 | 1.38 | 0.56 | 0.54 | 0.48 |
| Direction | X (E-W) | Y (N-S) | Rotation | X (E-W) | Y (N-S) | Rotation |
| Effective Mass (%) | 77 | 76 | 76 | 11 | 12 | 12 |
| Scheme | Story | ||||||
|---|---|---|---|---|---|---|---|
| Scheme 1 | 5–8 | 0.5 | 400 × 800 | 1700 × 350 | 4 | 8 | −2 |
| 2–4 | 0.5 | 400 × 800 | 1700 × 350 | 4 | 12 | −2 | |
| Scheme 2 | 5–8 | 0.2 | 400 × 800 | 1700 × 350 | 4 | 8 | −2 |
| 2–4 | 0.2 | 400 × 800 | 1700 × 350 | 4 | 12 | −2 | |
| Scheme 3 | 5–8 | 0.2 | 400 × 800 | 1700 × 350 | 4 | 8 | 0 |
| 2–4 | 0.2 | 400 × 800 | 1700 × 350 | 4 | 12 | 0 |
| Earthquake Wave | Scheme 1 | Scheme 2 | Scheme 3 |
|---|---|---|---|
| Manjil | 19.1% | 13.5% | 7.9% |
| Coalinga | 20.6% | 13.8% | 8.7% |
| Imperial Valley | 21.3% | 15.8% | 9.2% |
| Chi-Chi | 23.3% | 15.5% | 8.8% |
| Big Bear | 19.6% | 13.8% | 8.5% |
| Artificial wave 1 | 19.7% | 13.8% | 8.0% |
| Artificial wave 2 | 20.1% | 13.5% | 8.4% |
| Average of multiple waves | 20.5% | 14.2% | 8.5% |
| Energy Composition | Scheme 1 | Scheme 2 | Scheme 3 |
|---|---|---|---|
| Internal model damping/Input | 21.3% | 27.1% | 36.5% |
| Damper/Input | 77.9% | 70.8% | 57.4% |
| Dissipated inelastic/Input | 0.8% | 2.1% | 6.1% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Q.; Pan, W.; Lan, X.; Li, Z. A Study on the Amplification Effect and Optimum Control of the Intermediate Column–Lever Negative Stiffness Viscous Damper. Appl. Sci. 2024, 14, 7627. https://doi.org/10.3390/app14177627
Zhou Q, Pan W, Lan X, Li Z. A Study on the Amplification Effect and Optimum Control of the Intermediate Column–Lever Negative Stiffness Viscous Damper. Applied Sciences. 2024; 14(17):7627. https://doi.org/10.3390/app14177627
Chicago/Turabian StyleZhou, Qiang, Wen Pan, Xiang Lan, and Zuwei Li. 2024. "A Study on the Amplification Effect and Optimum Control of the Intermediate Column–Lever Negative Stiffness Viscous Damper" Applied Sciences 14, no. 17: 7627. https://doi.org/10.3390/app14177627
APA StyleZhou, Q., Pan, W., Lan, X., & Li, Z. (2024). A Study on the Amplification Effect and Optimum Control of the Intermediate Column–Lever Negative Stiffness Viscous Damper. Applied Sciences, 14(17), 7627. https://doi.org/10.3390/app14177627






