Suboptimal Analysis of the Differential System of the Conceptual Trailer Air Brake Valve

Featured Application

The results are applicable to the configuration of design parameters of pneumatic brake valves. The proposed conceptual solution for the valve’s differential part has implementation potential. The analyses meet the trend of increasing the speed of pneumatic braking systems. Pneumatic systems, despite being slower than hydraulic systems, pose less environmental risk during use or failure.

Abstract

Motivation: To increase the efficiency of the brake valve by adding a corrective member. Background: The speed of response and smooth transition between modes of operation in the braking system are the primary research questions. Objective and research question: Will the optimal selection of the input parameters of the differentiating part of a conceptual brake valve ensure the speed of operation and enable a smooth transition from the accelerating mode to the tracking mode? Methods: The mathematical model of the differentiating part of the brake valve uses the lumped method, and the solution was obtained by numerical means. Results: Within the assumed range of variation of spring stiffness and control piston bore throughput, the distribution maps of action times and piston lift were determined, and the optimal configuration of the analyzed input parameters was obtained by a genetic algorithm. Future research: future activities will focus on the development of a system of smooth variation of the throughput of the connecting chamber of the differential part of the valve. Conclusions: The determined maps of functional parameter distributions are the basis for the selection of components of the braking system; optimization indicates the directions of modification of the valve in order to obtain an acceptable performance system.

1. Introduction

Vehicle and trailer air braking systems must meet the requirements set forth in UNECE Regulation No. 13 [1]. The provisions outlined there define the magnitudes of the control parameters that are the basis for approval and subsequent inspections during use. Although air braking systems are slower in operation relative to liquid systems [2], they are still popular on the market. The enthalpy of the working medium in the form of compressed air, also known as thermodynamic potential, is responsible for the operation of this system. The advantage of pneumatic systems over liquid ones is their environmental friendliness. Air, which is widely available, requires only compression and further appropriate distribution to controls and actuators. Any kind of connection or malfunction (except for the compressor, which is lubricated with oil) does not pose an ecological hazard. Agricultural tractors, semi-trailers, or trains are equipped with air brake control systems for trailers, semi-trailers, or working machines in various system configurations. The increase in the dimensions of convoys traveling on the road, including road or rail trains, creates the need to correct the speed of operation of the individual members of the convoys [3]. In addition, military operations and the need to transport combat equipment also intensify the scope of use of air braking systems. An important characteristic of any braking system, including air brakes, is a predictable response to the driver’s intentions. Hence the need for additional valves on the tractor–trailer valve–actuator line [4]. Forcing from the side of the vehicle towing the trailer should result in an intended response from the brake valves and operating actuators [5].

Depending on the target operating parameters and purpose, different types of trailer valves are used in air braking systems [6,7]. The system can function without a trailer valve, but its response to forcing, due to the volume of the system, will be prolonged and may be insufficient at the initial stage to realize full braking. Some of the most commonly used valves in trailer air braking systems are relay valves [8,9,10]. In operation, in addition to the control system, they use additional air reservoirs located close to the valve. A momentary override to the additional reservoir increases braking efficiency. This significantly reduces the response time of the brake actuators to driver forcing [11]. Such valves can perform a specific function without having the ability to smoothly transition between operating modes [12]. The lack of smooth transition between operating modes, which can be critical under varying forcing conditions, resulted in the proposal to use differential valves, which were discontinued in [13]. Although it has been a long time since the presentation of this paper, the differential valve still remains only in the area of scientific research. There are a number of studies of its use in brake systems with long control lines [14,15] demonstrating its applicability. Often, a valve of this type, due to cost reduction, is practically realized by modifying the design already existing on the market. As shown in W [16], the differential valve gives the driver the possibility to operate in a tracking mode or accelerating mode and smoothly transitions between modes, depending on the driver’s forcing. There are a number of post-medium solutions, such as various types of acceleration members mounted additionally in the valves [17], pneumatic-only combination valves [18], or electro-pneumatic valves [2]. It is the electro-pneumatic systems that are seen as the target for increasing the efficiency of trailer and work machinery braking systems, as confirmed by studies [19,20,21]. The increasing automation of vehicle driving requires the use of complex systems that work with already existing vehicle systems (ABS, BAS). In this case, as well as in other utility applications, systems that use a PWM signal to regulate operating pressure are proving their worth [11]. Electronically controlled air braking systems also have applications in autonomous vehicles, where performance and reliability criteria are tightened relative to conventional vehicles [22].

Computational analyses involving pneumatic braking systems can be divided into two groups: analytical–empirical modeling and modeling using CFD. In the first group, mainly empirical modeling creates its own mathematical descriptions of the components of the braking system [23], the solution of which is sought by numerical methods using Matlab–Simulink or AMESim [18]. In this group, there is a noticeable trend that exploits the discretization of the system into small, repeated alternating components, referred to as the lumped method [13]. This method is successfully used in the calculations of systems with relay valves [9] or differential valves [15]. In the analytical and empirical methods, a quantitative evaluation consisting of the determination of pressure waveforms in the components of the braking system is set as the goal. On this basis, the speed of response of the system or the course of the braking force is evaluated. In the second group of computational methods, those using CFD, it has become common to use dedicated software for 2D and 3D analysis, such as, for example, KIVA, Ansys Fluent, and others [24,25,26,27]. Due to the complexity of the flow process, the mathematical description here is based on Reynolds average Navier–Stokes methods (RANS) [28,29]. In addition to quantitative evaluation, CDF software allows for qualitative flow analysis. It is used as an alternative to experimental studies, which are expensive to set up and conduct and whose results are successfully used in the empirical modeling of entire systems [30]. A significant number of studies are directed at optimizing the design of pneumatic brake system components as well as process control using information from various sensors [31,32]. An analysis of the literature revealed a research gap related to the use of certain types of brake valve performance-correcting members. Based on this, it was deemed necessary to analyze a differential member that will be able to increase the efficiency of the brake valve.

The purpose of the analysis presented in the study was to evaluate the effect of spring stiffness and throughput of the piston bore of the differential part of the valve on its functional characteristics. It was decided to use the following methods and means for such a goal:

• Propose the scope of modification of the existing brake valve necessary to realize the design of the conceptual differential valve;
• Develop a mathematical model of the differentiating part of the valve and indicate how to solve it;
• Carry out multivariate calculations to collect a group of results;
• Based on the group of results, conduct a suboptimal functional analysis to determine the variant that meets the conditions set.

The rest of the paper is as follows: Section 2 describes the object of analysis, presents the mathematical model, the conditions necessary to initiate the calculations, and the solution method. Section 3 presents the results and discussion, dividing them into the determination of functional features and suboptimal functional analysis. A further section, Section 4, proposes future research. Conclusions are included in Section 5.

2. Materials and Methods

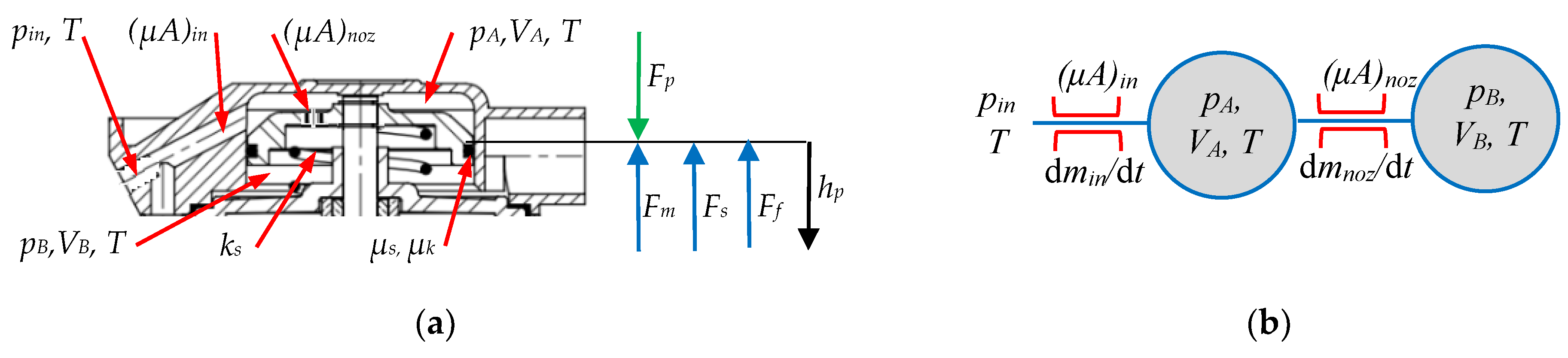
2.1. Object of Analysis

The realization of a conceptual brake valve with a differential part was decided by modifying a commercial solution of the Visteon trailer relay valve 44100110 (Figure 1a). In the original version, this valve can work with both single- and dual-line tractor braking systems. In the case of a single-line system, the connection M is connected to the power and control port (black line), while the connection Zm is left unconnected and sealed. In a two-line system, connector M connects to the power port (red line of the tractor connection), and Zm connects to the control port (yellow line of the tractor connection). In both systems, connection V connects to the trailer’s compressed air reservoir, and connection Z connects to the brake force regulator or directly to the actuator. A detailed description of the valve’s operation can be found in [12].

The extent of the modification of the Visteon trailer relay valve 44100110 is shown in Figure 1b,c. In the first step, the interlock a was removed from the upper body and the channel between the bodies b was plugged. In addition, the f and g connections M and Zm were blanked off. Necessary for the realization of the differential function was the drilling of a hole c in the piston and the permanent immobilization of the piston on the pusher d. The next step involved replacing the one-way seal e with a seal that works both ways. It was decided to use the vent W as a functional channel. Schematically, the conceptual valve is shown in Figure 1d.

When the control pressure p_in (blue line in Figure 1b) feeding W and channel a builds up with a small gradient, the hole in the piston does not allow the pressure difference above and below the piston to be large enough to overcome the force coming from the spring. Then, the brake actuators, by connection Z, are supplied with a pressure p_in in tracking mode. In the case of a large gradient p_in, the piston is displaced, changing the supply to the actuators from connection W to V. The V connection is supplied from an additional reservoir close to the valve, so this mode is referred to as acceleration mode. Depending on the stiffness of the spring under the piston controlling the valve modes and the diameter of the hole in the piston, the timing of the appearance of the accelerating mode and its disappearance can be controlled. While accelerating action can be achieved with various valve configurations, such as a relay valve, guaranteeing a smooth transition to the tracking function is difficult. The basic problem of differential valve operation is the selection of the stiffness of the spring under the control piston and the diameter of the piston bore, which formed the basis of the analyses in this study.

2.2. Mathematical Model

The high complexity of the design of the entire valve (Figure 1) was the reason that only the differential part was analyzed in the following section. A number of simplifications had to be adopted in the development of the mathematical model. The most important of these include the following:

• The mechanical system is perfectly rigid;
• The pneumatic system is perfectly tight;
• The position of the piston depends on the balance of forces acting in the system;
• The air pressure is distributed uniformly in the volumes under analysis;
• The state of the air in the volumes has a constant temperature and depends on time and the inlet–outlet mass balance;
• The flow was considered isentropic in an adiabatic sheath;
• The spring generates a force due to its stiffness and deflection and preload;
• Friction depends on the movement of the piston and has different components;
• Flow-limiting elements open and close gradually.

Functional, mechanic, and pneumatic schemes were created based on the adopted simplifications (Figure 2a,b).

The position of the piston controlling the action of the differential system (Figure 2a) is the result of forces of various origins, whose equilibrium state is written in the following form:

$$F_p-F_s-F_f-F_m=0,$$

(1)

where F_p—pressure force; F_s—spring force; F_f—friction force; and F_m—mass inertia drag force.

The force from the air pressure F_p depends on the pressure difference over p_A (chamber A) and under the piston p_B (chamber B). Due to the presence of a control p_in, it was necessary to take into account the differences in surface areas under and over the piston.

$$F_p={\displaystyle \frac{\pi D^2}{4}}{p}_A-{\displaystyle \frac{\pi \left(D^2-d^2\right)}{4}}{p}_B,$$

(2)

where D—piston diameter and d—piston rod diameter.

The air pressure over the piston p_A was the result of the flow into chamber A from the inlet side, as well as the flow into and out of chamber B through the hole made in the piston. The lumped method [13,33] was used to calculate the pressures in the chambers. Differential equations describing the pressure changes in chambers A and B were constructed from the diagram (Figure 2b) and written as follows:

$${\displaystyle \frac{\mathrm{d}{p}_A}{\mathrm{d}t}}={\displaystyle \frac{\kappa RT}{V_A}}\left({\displaystyle \frac{\mathrm{d}{m}_{in}}{\mathrm{d}t}}-{\displaystyle \frac{\mathrm{d}{m}_{noz}}{\mathrm{d}t}}-{\displaystyle \frac{p_A}{RT}}{\displaystyle \frac{\mathrm{d}{V}_A}{\mathrm{d}t}}\right),$$

(3)

$${\displaystyle \frac{\mathrm{d}{p}_B}{\mathrm{d}t}}={\displaystyle \frac{\kappa RT}{V_B}}\left({\displaystyle \frac{\mathrm{d}{m}_{out}}{\mathrm{d}t}}-{\displaystyle \frac{p_B}{RT}}{\displaystyle \frac{\mathrm{d}{V}_B}{\mathrm{d}t}}\right),$$

(4)

where κ—adiabatic exponent; R—air constant; T—air temperature; and V_A and V_B—volume A and B chambers, respectively.

The air mass fluxes flowing through the inlet local resistance (µA)_in in and the piston bore local resistance (µA)_noz are described by differential equations in the following forms:

$${\displaystyle \frac{\mathrm{d}{m}_{in}}{\mathrm{d}t}}={\left(\mu A\right)}_{in}{v}_{\max }{\rho }_{in}{\phi }_{\max }\left(\sigma \right)f{\left(\sigma \right)}_{in},$$

(5)

$${\displaystyle \frac{\mathrm{d}{m}_{noz}}{\mathrm{d}t}}={\left(\mu A\right)}_{noz}{v}_{\max }{\rho }_{noz}{\phi }_{\max }\left(\sigma \right)f{\left(\sigma \right)}_{noz},$$

(6)

where v_max—velocity of sound in a stationary gas $v_{\max }=\sqrt{\kappa RT}$; ρ—air density ${\rho }_{in}=p_{in}/\left(RT\right)$ and ${\rho }_{noz}=p_A/\left(RT\right)$; φ_max(σ)—maximum value of the theoretical flow function Saint-Venant and Wantzel φ_max(σ) = 0.785; f(σ)—flow function (Miatluk-Avtushko [13] $f\left(\sigma \right)=1.13{\displaystyle \frac{1-\sigma }{1.13-\sigma }}$), σ—pressure ratio $\sigma =p_{i+1}/p_i$.

The force from the spring F_s located in chamber B was calculated from the sum of the preload force and the variable force due to piston displacement and spring stiffness:

$$F_s=F_0+k{h}_p,$$

(7)

where F₀—preload force; k—spring stiffness; h_p—piston displacement.

The force resulting from the friction F_s of the piston seal against the valve housing is divided into two types, static F_fs and kinetic F_fk, depending on the movement of the piston or lack thereof.

$$F_f=\{\begin{array}{lll}{F}_{fs}={\mu }_s{F}_N& \mathrm{if}\hfill & h_p=0,\hspace{1em}{h}_p=h_{\max }\hfill \\ F_{fk}={\mu }_k{F}_N\mathrm{sgn}\left({\dot{h}}_p\right)& \mathrm{if}\hfill & h_p=\mathrm{var}\hfill \end{array},$$

(8)

where μ_s—static friction coefficients; μ_k—kinetic friction coefficients; and F_n—contact force.

The mass inertia drag force F_m was calculated from the following:

$$F_m=m{\displaystyle \frac{{\mathrm{d}}^2{h}_p}{\mathrm{d}{t}^2}},$$

(9)

where m—piston and piston rod mass

Based on Equations (1)–(9), a system of differential equations was formed as follows:

$$\{\begin{array}{l}{\displaystyle \frac{\mathrm{d}{h}_p}{\mathrm{d}t}}=v\\ {\displaystyle \frac{\mathrm{d}v}{\mathrm{d}t}}={\displaystyle \frac{F_p-F_s-F_f}{m}}\\ {\displaystyle \frac{\mathrm{d}{p}_A}{\mathrm{d}t}}={\displaystyle \frac{\kappa RT}{V_A}}\left({\displaystyle \frac{\mathrm{d}{m}_{in}}{\mathrm{d}t}}-{\displaystyle \frac{\mathrm{d}{m}_{noz}}{\mathrm{d}t}}-{\displaystyle \frac{p_A}{RT}}\hspace{1em}{\displaystyle \frac{\mathrm{d}{V}_A}{\mathrm{d}t}}\right)\\ {\displaystyle \frac{\mathrm{d}{p}_B}{\mathrm{d}t}}={\displaystyle \frac{\kappa RT}{V_B}}\left({\displaystyle \frac{\mathrm{d}{m}_{out}}{\mathrm{d}t}}-{\displaystyle \frac{p_B}{RT}}\hspace{1em}{\displaystyle \frac{\mathrm{d}{V}_B}{\mathrm{d}t}}\right)\\ {\displaystyle \frac{\mathrm{d}{m}_{in}}{\mathrm{d}t}}={\left(\mu A\right)}_{in}{v}_{\max }{\rho }_{in}{\phi }_{\max }\left(\sigma \right)f{\left(\sigma \right)}_{in}\\ {\displaystyle \frac{\mathrm{d}{m}_{noz}}{\mathrm{d}t}}={\left(\mu A\right)}_{noz}{v}_{\max }{\rho }_{noz}{\phi }_{\max }\left(\sigma \right)f{\left(\sigma \right)}_{noz}\end{array}.$$

(10)

Among the range of optimization methods, such as grey wolf [34], whale [35], Harris hawks [36], body shape [37], particle swarm [38], game theory [39], and many others, the genetic algorithm (GA) was chosen [40]. The GA algorithm was designed to simulate biological processes, so biological terminology is used in its description. Although genetic algorithms may differ in details, they almost always have common components, which include the follow [41]:

• Fitness function necessary for optimization;
• Population of all chromosomes;
• Selection from the population of chromosomes that will reproduce;
• Crossing over chromosomes to produce the next generation of chromosomes;
• Random mutation in the new generation of chromosomes.

The most important component of the algorithm is the fitness function, and from its notation the optimal solution is obtained. A chromosome is considered a numerical value that presents the solution to the problem. For a problem with N-pairs of dimensions, each chromosome is encoded as follows:

$$\mathrm{chromosome}=\left[p_1,p_2,\dots ,p_{Npar}\right],$$

(11)

where p—particular value of the i-th parameter.

Each chromosome is evaluated with a fitness function in terms of problem solving. With a non-negative fitness function, the probability of selecting chromosome A₁₁ for further reproduction can be calculated as follows:

$$P\left(A_{11}\right)=\left|{\displaystyle \frac{f\left(A_{11}\right)}{{\displaystyle \sum _{i=1}^{N_{pop}}f\left(A_{11}\right)}}}\right|,$$

(12)

The selection of the chromosome to be exchanged can repeat from the position of the operator, and the crossover and recombination resemble the phenomena found in biology. Disposing of parental chromosomes:

$$\left[1101011100 1000\right]\hspace{1em}\mathrm{and}\hspace{1em}\left[0101110101 0010\right],$$

(13)

They can be crossed, for example, after the fourth bit, obtaining the following descendants:

$$\left[0101011100 1000\right]\hspace{1em}\mathrm{and}\hspace{1em}\left[1101110101 0010\right].$$

(14)

Furthermore, the second generation is tested by the fitness function and the process repeats itself, where evaluation and selection take place. Mutation prevents the optimization process from getting stuck in a local minimum. The algorithm runs until a solution is found that meets the termination criterion, which, in many cases, is a compromise between the stated requirements.

The use of the Matlab environment in GA optimization is common in a variety of issues, not only biological or medical, but also technical. It has been successfully used in the design of intelligent engineering systems [42], improving the efficiency of power grids [43] or the parameters of a solar cells [44].

2.3. Initial and Boundary Conditions

In order to initiate calculations enabling further analysis of the results, it was necessary to adopt initial and boundary conditions. With a real object at their disposal and a virtual model, a series of measurements and estimates of these values were made, the results of which are included in

Table 1. Initial conditions, boundary conditions, and variables adopted in the analyses.

Parameter	Unit	Value
initial conditions
diameter of the piston	m	65.5 × 10−3
diameter of the piston rod	m	10 × 10−3
max piston displacement	m	7.3 × 10−3
piston and piston rod mass	kg	46.8 × 10−3
spring preload	N	70
seal pressure force on the wall	N	5
static friction coefficient	-	0.8
kinetic friction coefficient	-	0.76
inlet throughput	m2	5 × 10−6
adiabatic exponent	-	1.4
air constant	J/(kg·K)	287.5
air temperature	K	293.15
boundary conditions at t = 0 s
volume of the upper chamber	m3	2.75 × 10−5
volume of the lower chamber	m3	2.28 × 10−5
piston displacement	m	0
pressure in volume	Pa	1 × 105
variable parameter during analysis
bore throughput in the piston	m2	(0.05…0.525) × 10−6
spring stiffness	N/m	(0.50…1.45) × 105

.

To initiate the calculations, it was necessary to assume the pressure waveform at the inlet to the trailer brake valve p_in under analysis. For this purpose, the waveforms presented in [45] were used, where a constructurally similar valve in a braking system with a 20 m control and supply line was modeled. In Figure 3a, the individual waveforms denote the following: F—forcing force on the tractor’s brake valve; p_tank—pressure in the tractor’s supply reservoir (without refilling with the compressor); p_{line_out}—pressure at the end of the 20 m line connecting the tractor’s valve to the trailer’s brake valve; p_cA and p_cB—pressures in the valve chambers; and p_act—pressure in the brake actuator. Having the waveforms contained in Figure 3a, a waveform p_{line_out} was extracted from them, which represented the end of the 20 m supply line connecting the tractor valve and the trailer valve. In the simulations presented in [45], the brake valve in both the differentiating and transmitting parts operated in a stepwise manner. What was missing was an accurate representation of the movement of the actuators and the resulting changes in throughput. As shown in (Figure 3a), the pressure in the p_act actuator reached 0.75 p_max in close to 0.6 s, which is consistent with the requirements included in the UNECE regulation No. 13 [1]. For the purpose of the realization of the calculations, this waveform was ‘smoothed’ in the part of the power supply change from the supply line to the additional trailer tank (Figure 3b). This resulted in a waveform p_correct, which was implemented as p_in in the calculations that were carried out, according to (Figure 2).

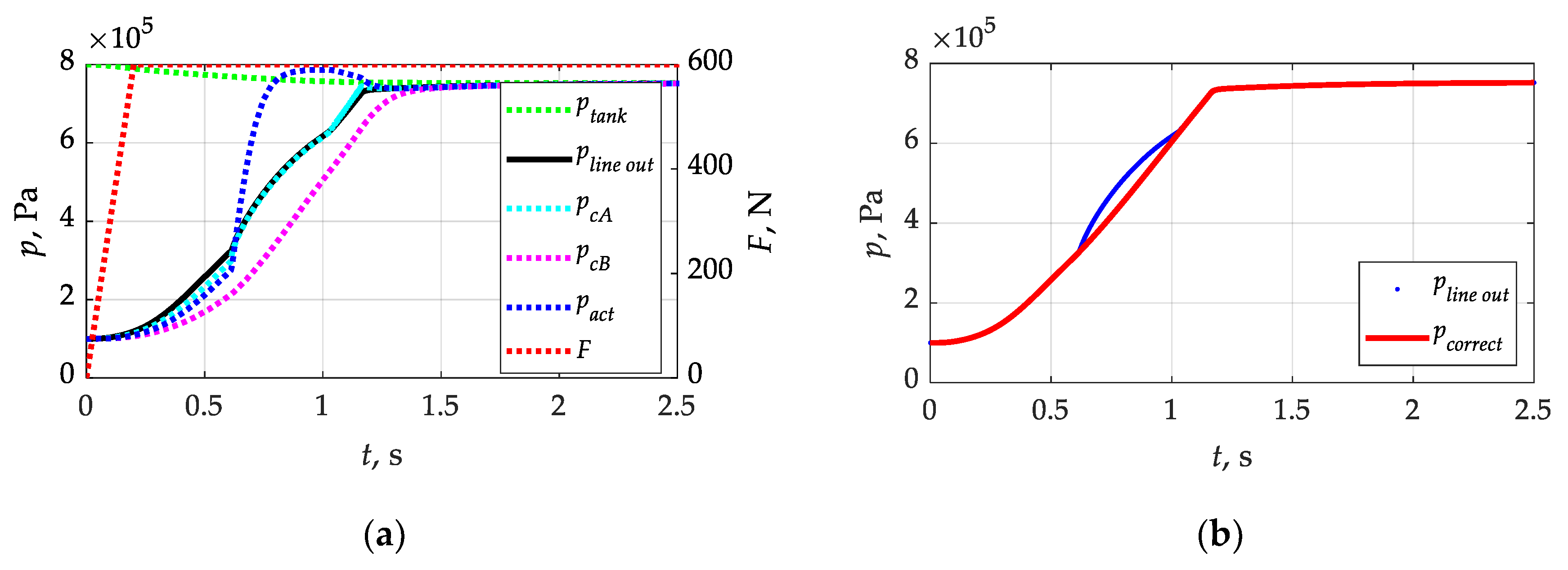
2.4. Method to Find a Solution

The system of differential Equation (10) was solved numerically using the implicit trapezoidal method with variable-step backward differentiation. Adjustment of the value of the input pressure waveform to the variable step of the numerical solution was achieved by interpolation using the cubic method with shape-preserving variation in the spring stiffness and piston bore capacity, which was achieved by combining code elements written in Matlab and a block functional diagram built in Simulink.

3. Results

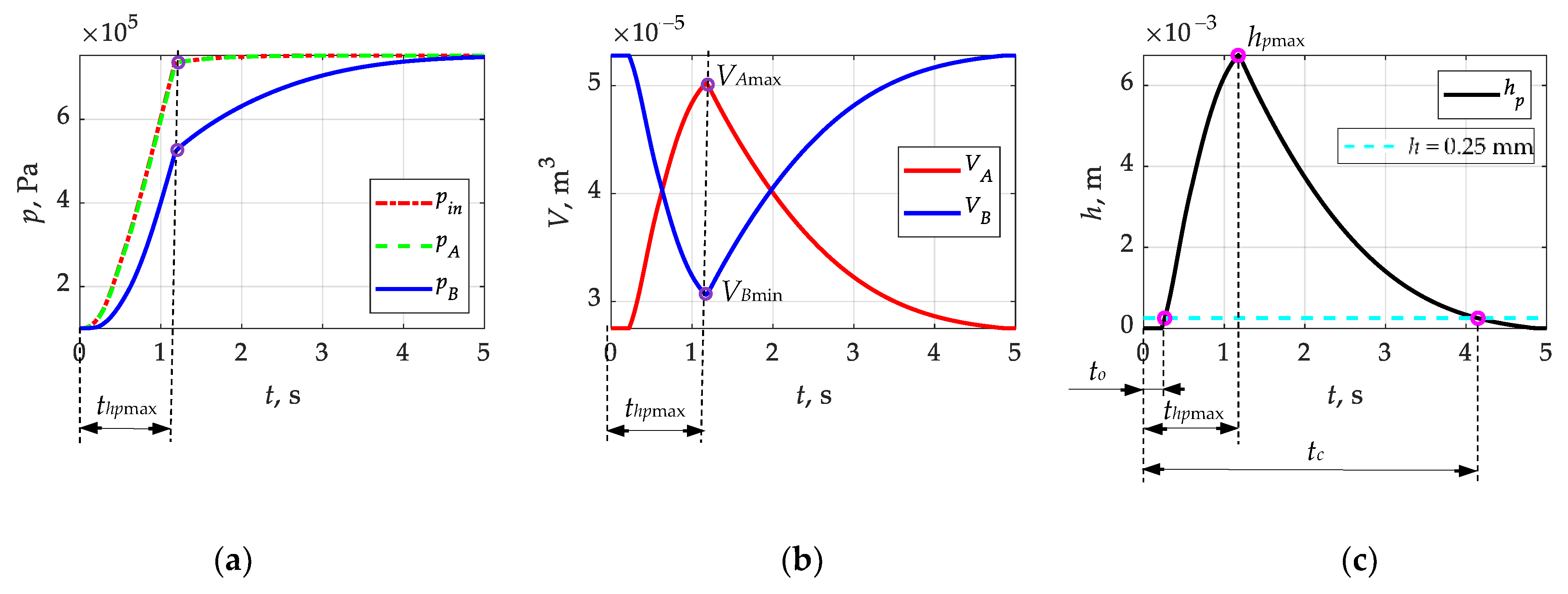
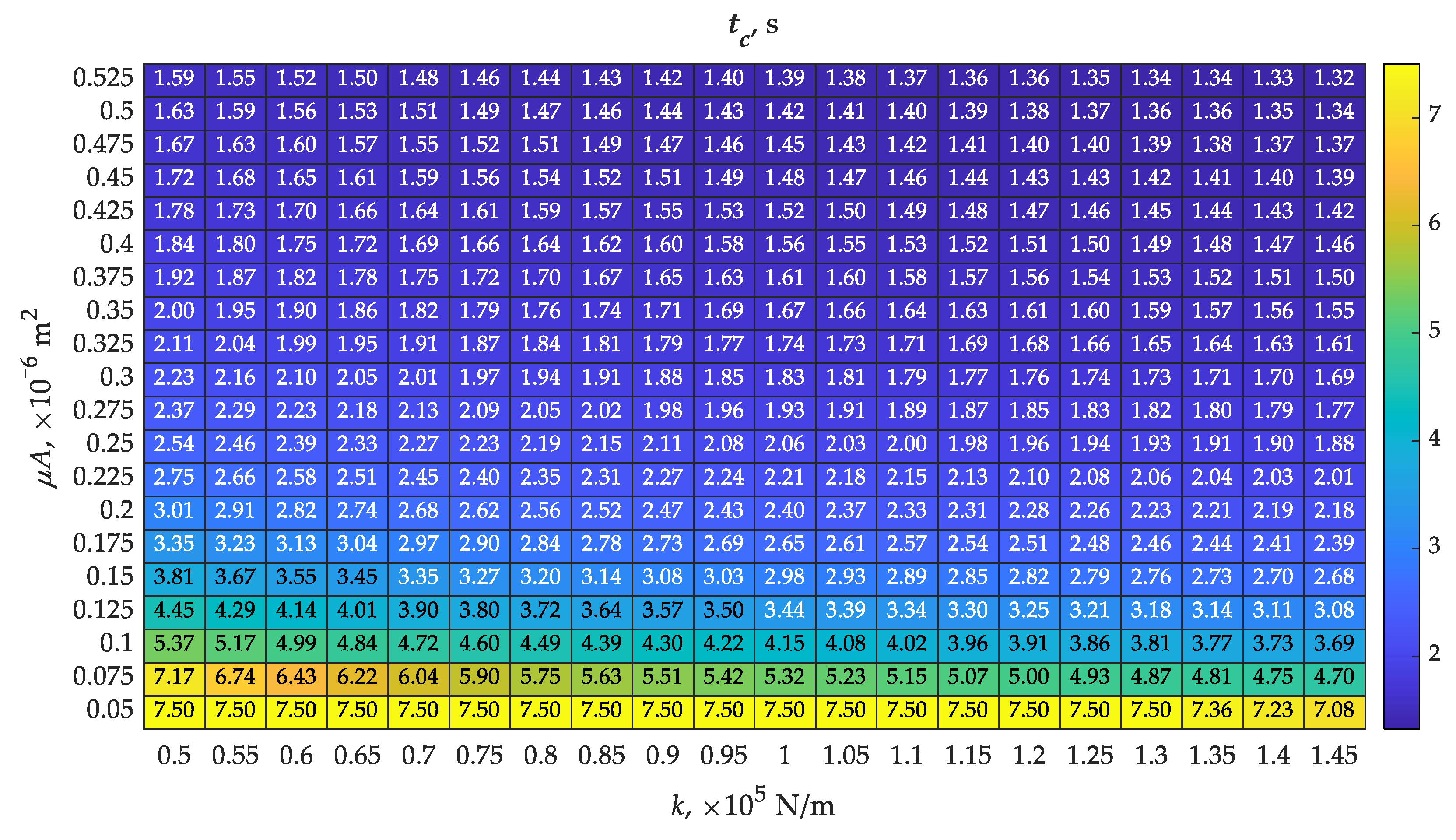
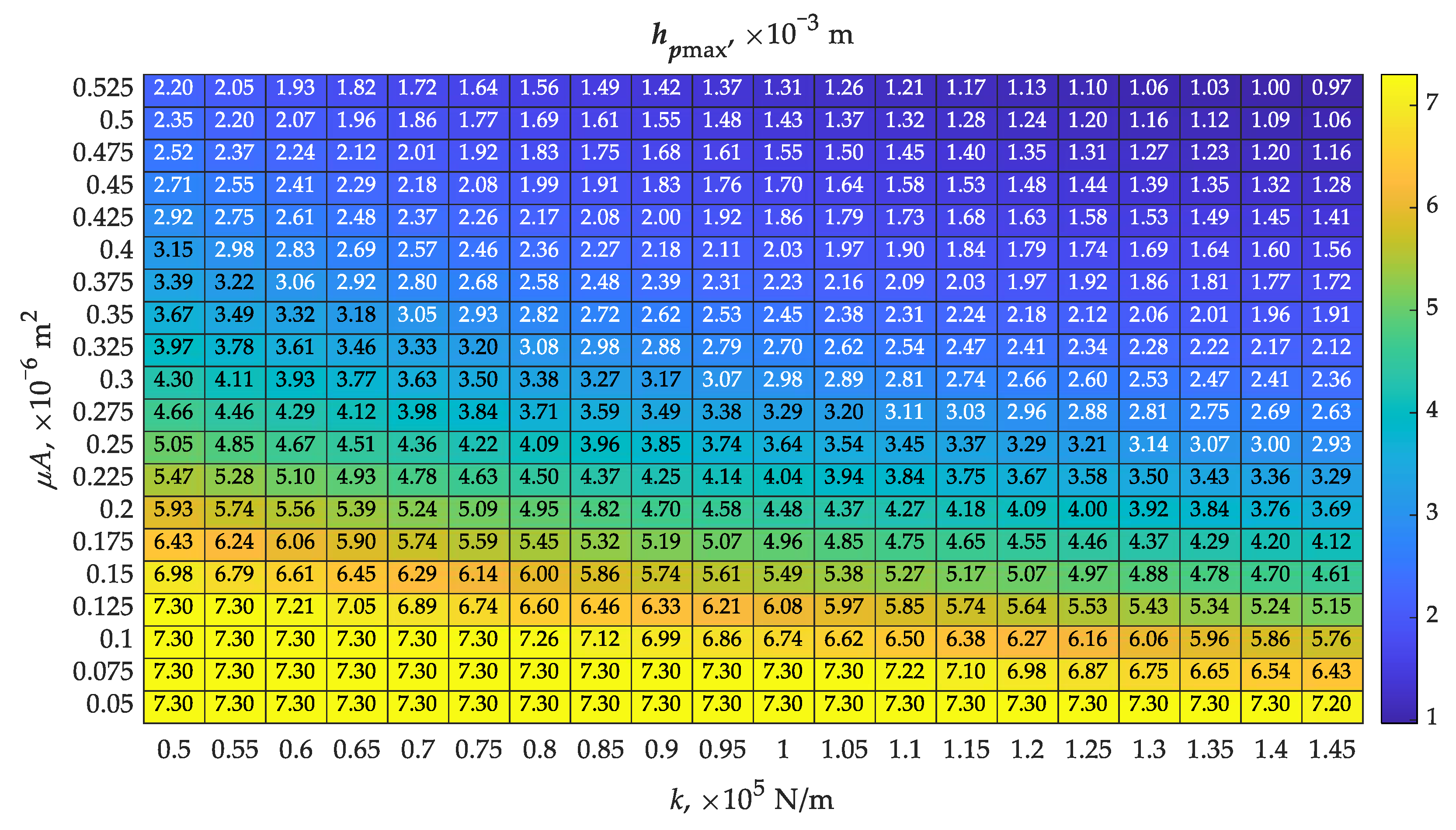
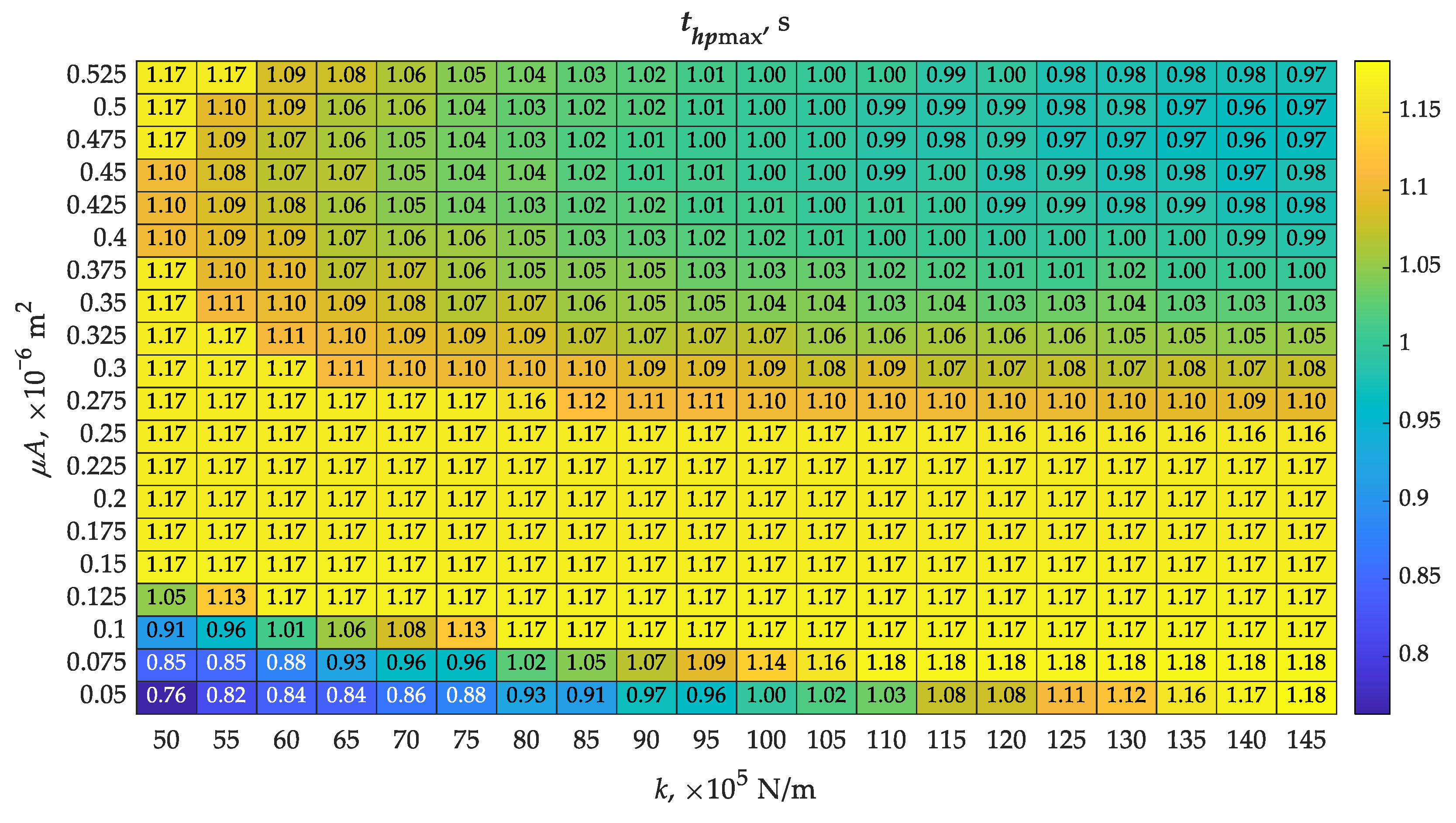
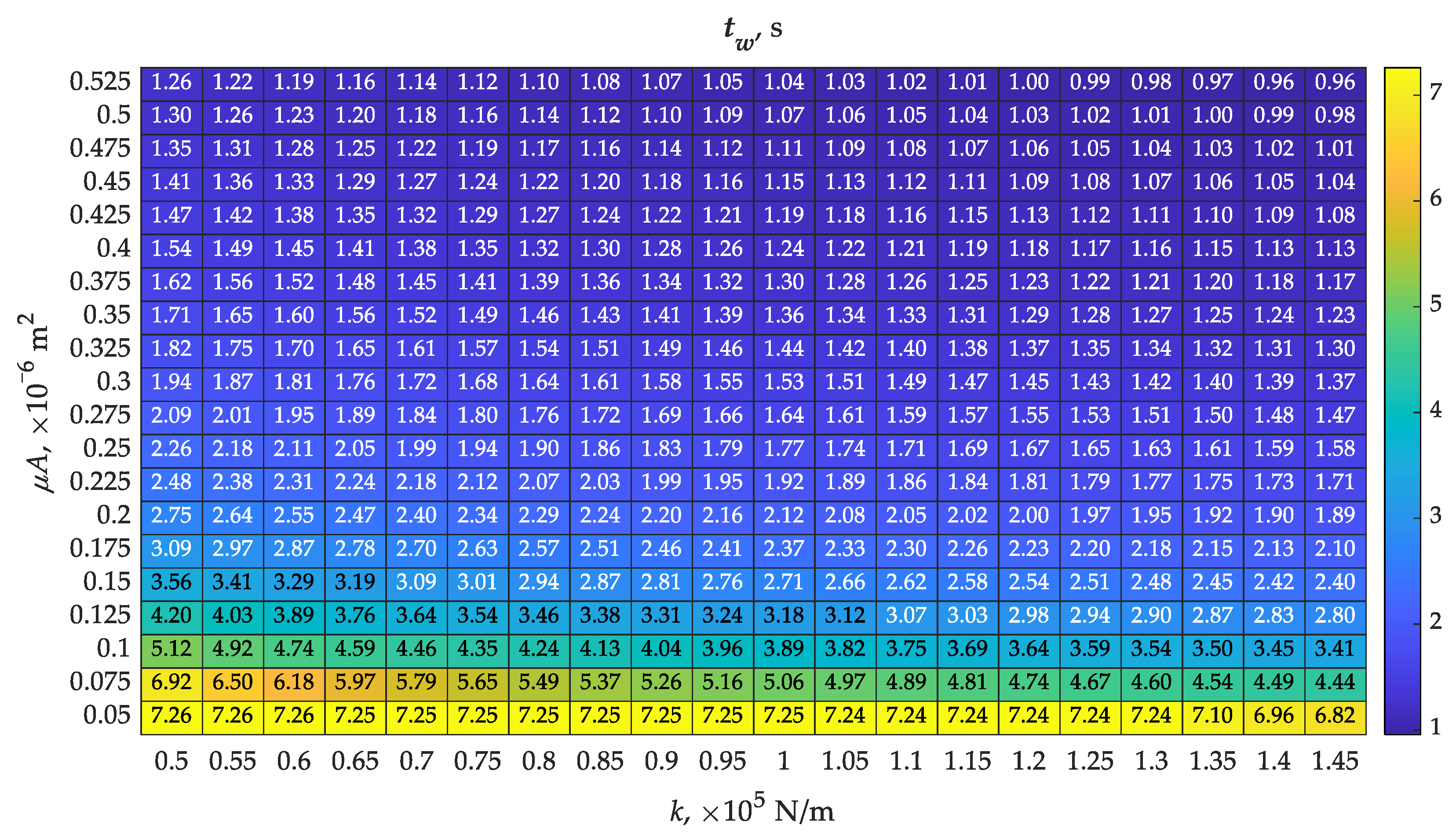
3.1. Determination of Characteristic Operating Times and Maximum Displacement of the Valve Piston

Implemented in the Matlab–Simulink environment, the calculation procedures based on Equation (10) and Table 1 enabled the determination of pressure waveforms in the valve chambers, chamber volume changes, and piston displacement. Example waveforms are shown in Figure 4. The correlation of the pressure waveforms (Figure 4a) with the change in chamber volume (Figure 4b) and the displacement of the valve piston (Figure 4c) can be seen, which confirms the correctness of the adopted mathematical description and calculation methodology. At the point where the piston reaches its maximum displacement (h_pmax), chamber A increases its volume to its maximum value (V_Amax), the opposite of chamber B (V_Bmin). At the moment of actuation of the differential part of the valve p_in, due to the proximity of chamber A and the relatively high value of the inlet capacity (μA)_in, the course p_A differs little from the course p_in (Figure 4a). The initial increase in pressure in chamber B until the piston reaches maximum displacement h_pmax is due to air compression, since this chamber is perfectly sealed and has no outlet. The reason why the pressure p_A is not able to equalize with p_B at the point where the piston reaches displacement h_pmax is due to the presence of a spring in chamber B. After the piston reaches h_pmax, the pressure in chamber B increases with a smaller gradient than until it reaches h_pmax. The reason for this fact is the throughput of the opening in the piston (μA)_noz and the associated possibility of air flow between chambers A and B.

From the waveforms shown in Figure 4, one can see the dominant effect of spring stiffness and piston bore throughput on the performance of the differential part of the brake valve. Increasing the spring stiffness will delay the opening of the valve t_o but shorten its closing t_c (Figure 4c). In this case, t_o and t_c were determined when the piston exceeded 0.25 mm in the opening and closing processes. It may also prevent the maximum piston displacement h_pmax from being achieved, according to Table 1. The differential valve should accelerate the braking process at the initial stage but also allow a smooth transition to tracking action. The accelerating action will be realized only if the pressure in chamber A builds up with a greater gradient than in chamber B and is also able to overcome the force coming from the spring. The result will be a displacement of the piston. With a smaller input pressure gradient p_in, the throughput (μA)_noz, combined with the stiffness of the spring k, should only provide tracking action for the valve. This is confirmed by the results presented in [45]. This confirmed the validity of the objective adopted in this study. The evaluation of the influence of the spring stiffness and the throughput of the piston bore opening of the valve’s differential part on its functional characteristics is important and can determine the direction of design development for this type of device.

Guaranteeing a short response time (t_o = 0.24 s) of the valve in the differential member to the forcing was possible with a spring with the smallest stiffness of 0.5 × 10⁵ N/m and the smallest throughput value of 0.05 × 10⁻⁶ m² among those analyzed (Figure 5). The air supply to chamber A encountered the smallest force from the spring among the analyzed cases, resulting in the fastest movement of the piston in the initial phase until h_pmax was reached. The smallest value of throughput of the piston opening delayed the flow of air between chambers. This case had the opposite effect on the values of closing time, obtaining the highest value of t_c = 7.50 s (Figure 6). The spring was responsible for this state of affairs, which was unable to move the piston back to its initial state, with the additional flow resistance resulting from the small throughput value of the piston bore. The extreme opposite case to that described above was found with a stiffness of 1.45 × 10⁵ N/m and a throughput of 0.525 × 10⁻⁶ m². The opening time increased by more than 54% to 0.37 s (Figure 5), while the closing time decreased by more than 82% to 1.32 s (Figure 6). Based on this, it was concluded that the choice of spring stiffness and throughput of the opening in the piston of the differential part of the valve must be a compromise. The opening times were arranged almost symmetrically on the map (Figure 5), indicating a relationship close to proportionality. The situation was different for closing times (Figure 6), where only throughputs of less than 0.15 × 10⁻⁶ m² resulted in a significant increase in this time. The differences in opening times t_o in the cases studied were just over 54%, while closing times t_c were over 82%. A lack of symmetry is evident, the reason for which, in addition to the parameters in question, could also be the difference in inlet throughput (μA)_in and (μA)_noz. This difference affected the intensification of the air filling and compression processes in chambers A and B.

The piston did not achieve its maximum displacement in all analyzed cases (Figure 7). The part of the map with the lowest values of spring stiffness and piston bore throughput showed the achievement of the possible maximum piston displacement of 7.3 mm (Table 1), while the opposite part of the map showed only 0.97 mm. There is some correlation between the map of piston lift h_pmax and the map of opening times t_o (Figure 5), indicating that a shorter opening time t_o favors the piston to achieve greater displacement, approaching h_pmax. However, this is not binding, as flow is possible between chambers A and B as the piston moves, especially at higher values of piston bore throughput. Therefore, the piston may not have reached its maximum displacement. This is confirmed by a map showing the times to reach maximum piston displacement (Figure 8), where several results showed t_hpmax = 1.17 s. Juxtaposing this with Figure 7, one can see the different values of maximum displacements that accompany this.

An important functional parameter of the differential part of the valve was the duration of operation in acceleration mode. This mode meant assisting the braking system with an additional reservoir located close to the trailer brake valve. The acceleration action time was determined from the relationship t_w = t_c − t_o and is shown in Figure 9. The map t_w was similar in distribution to the h_pmax map (Figure 7). As can be seen in Figure 9, the acceleration action in the longest time dimension was possible at low throughputs, especially at 0.05 × 10⁻⁶ m². At all adopted spring stiffness, the time exceeded 6.8 s. Obtaining the shortest acceleration action time t_w was possible at a throughput of 0.525 × 10⁻⁶ m² and a spring stiffness of 1.45 × 10⁵ N/m.

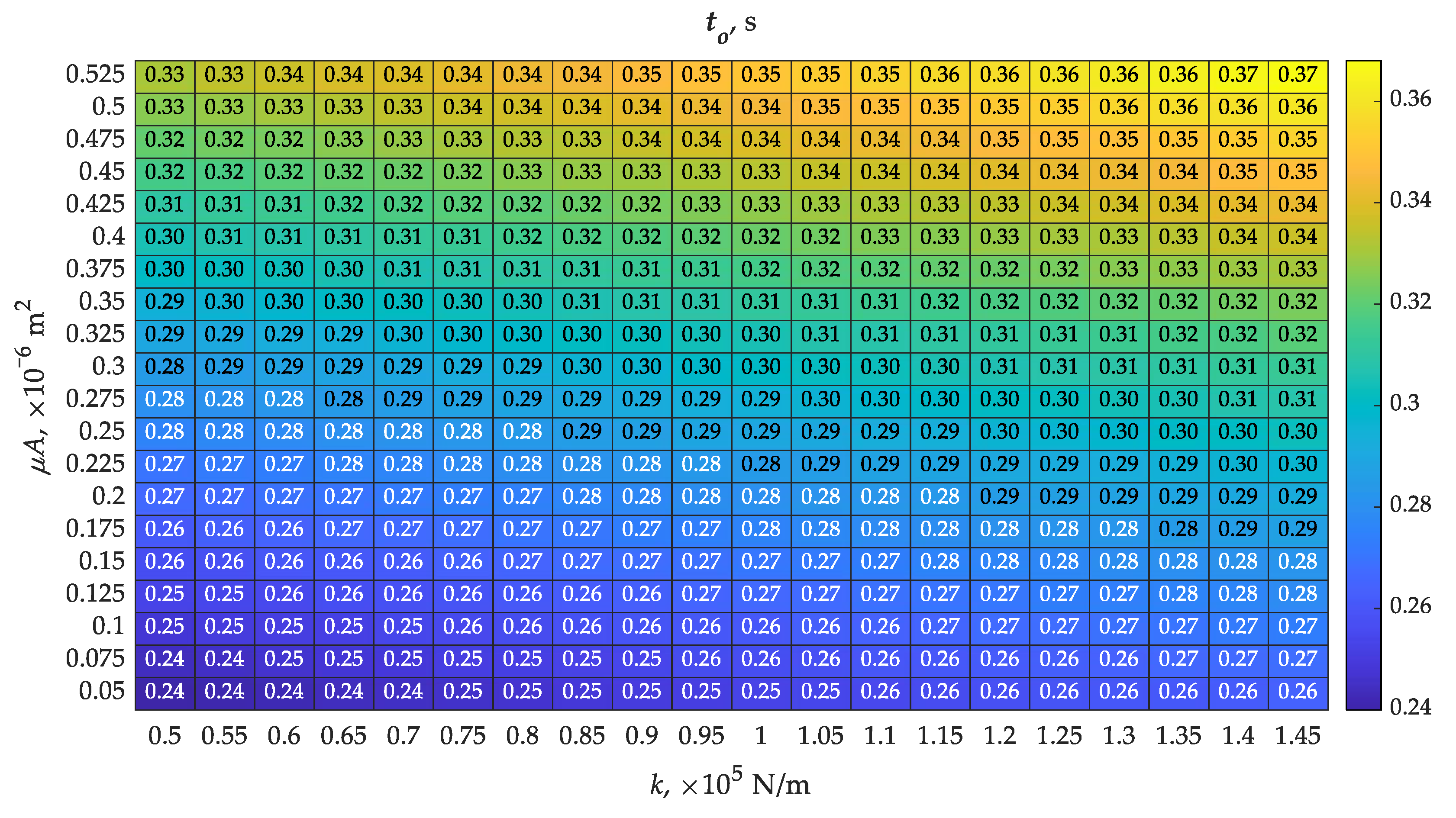
3.2. Suboptimal Functional Analysis

The selection of spring stiffness and bore throughput in the piston controlling the differential action of the trailer brake valve is a multi-faceted issue. As the maps of the individual functional parameters determined in the calculations show (Figure 5, Figure 6, Figure 7, Figure 8 and Figure 9), each of the adopted variants will be a compromise with greater or lesser side effects. The operation of the differential valve requires a different response to the forcing. Under rapid braking, this valve should switch from tracking mode to accelerating mode in the shortest possible time. Under slow braking, it should retain the feature of only tracking the driver’s intentions. Both variants of such braking are described in [45]. The question of the duration of the differential mode, beyond which the valve will switch to tracking mode, remains open. Increasing the stiffness of the spring will cause some delay in the displacement of the control piston at the beginning of the accelerating mode but will reduce the total time of its operation. The capacity of the piston bore will determine the process of abandoning the accelerating mode.

The maps obtained in the computational course and the need to determine the required parameters of the spring stiffness and the capacity of the hole in the valve control piston provided the rationale for using optimization based on the obtained maps.

Having the result maps (Figure 5, Figure 6, Figure 7, Figure 8 and Figure 9), the opening t_o and closing t_c times were taken as inputs for optimization. On this basis, the fitness function necessary for the initialization of GA optimization was assumed in the following form:

$$f_{fit}{\left(x,t_o,t_c\right)}_{\min }=x-t_{oi,j}\pm t_{ci,j}$$

(15)

where i and j are consecutive values from the matrix of calculation results, which, in this case, are the input data.

Equation (15) takes into account the return of maximization to the level of minimization. Depending on the sign through the last term, plus or minus, it allows the determination of two optimal solutions:

• Possibly short opening time and short closing time (opt_1);
• Possibly short opening time and long closing time (opt_2).

The two adopted cases (opt_1 and opt_2) represent the extreme responses of the analyzed system to the input forcing. At the initial stage, the results from (Figure 5) and (Figure 6) were coded, assigning numbers 1–20. This created two 20 × 20 matrices, which were necessary for the minimization of the function f_fit. In determining the size of the matrix, lower and upper constraints were also defined based on its size. The solution was sought without considering linear equations. After determining the optimal location of the solution, taking into account rounding to the nearest indices, the results were decoded, indicating the spring stiffness k and throughput (μA)_noz. In addition, other parameter values, such as h_pmax from Figure 7, t_hpmax from Figure 8, and t_w from Figure 9, were determined for the optimal location. The results are shown in

Table 2. Optimization results and values read from maps.

Parameter	to, s	tc, s	hmax, ×10−3 m	thmax, s	tw, s
range	0.24…0.37	1.32…7.50	0.97…7.30	0.73…1.18	0.96…7.26
opt_1	k = 1.45×105 N/m; (μA)noz = 0.525 × 10−6 m2
	0.37	1.32	0.99	0.97	0.96
opt_2	k = 1.30×105 N/m; (μA)noz = 0.05 × 10−6 m2
	0.26	7.50	7.30	1.12	7.24

.

The first solution (opt_1) indicated the following parameters as optimal values: spring stiffness k = 1.45 × 10⁵ N/m and piston bore throughput (μA)_noz = 0.525 × 10⁻⁶ m² (Table 2). The corresponding opening time t_o obtained the largest value of 0.37 s, as the calculation was determined by the short closing time t_c of 1.32 s. This resulted in a very short acceleration time of t_w = 0.96 s. The effect of the set optimization condition was achieved, but with h_max = 0.97 × 10⁻³ m, it is difficult to expect the efficiency of the valve operation. The situation is completely different in the case of the second solution (opt_2: spring stiffness k = 1.30 × 10⁵ N/m and piston bore throughput (μA)_noz = 0.05 × 10⁻⁶ m²). In this case, t_o reached a minimum value of 0.26 s and t_c a maximum value of 7.5 s. As a result, the valve operates in acceleration mode t_w = 7.24 s, which is almost a 30-fold increase over opt_1. The efficiency of the acceleration operation will increase significantly due to the piston reaching its maximum displacement h_max, which is 7.3 × 10⁻³ m. This guarantees the full opening of the valve. An additional optimization was still performed on three parameters, completing Equation (15) with h_max. Also in this variant, the results were consistent with those presented in Table 2. Although in [45], the differential valve was modeled in a slightly different way from the one presented in this study, the trend of changes is similar. This indicates the correctness of the adopted model and the proper control of the optimization parameters.

Summarizing the suboptimal analysis of the functional parameters of the differential part of the brake valve, it should be noted that the GA algorithm is able to accurately identify the optimal solutions in terms of the specified objective. However, only by evaluating and confronting the remaining functional quantities does the final selection of the optimal variant become possible.

4. Future Research

The calculations and analyses carried out show the effect of spring stiffness and control piston bore throughput on the performance of the differential part of the brake valve. This will result in a change in the functional characteristics of the entire braking system. The obtained maps of the distribution of indicators important in the operation of the differential part will be helpful in configuring the braking system. Further work in this area will focus on developing a system that smoothly changes the capacity of the opening connecting the two chambers below and above the control piston. Changing the spring stiffness seems too complicated at this stage. One of the options adopted is a by-pass channel connecting the two chambers of the differentiating part, in which it is planned to install an electro-valve with appropriate control. This measure is considered necessary due to the possible varying degree of load on the trailer during its operation. The variable throughput between the chambers of the differential part could ultimately relieve the operation of the brake force regulator.

5. Conclusions

Suboptimal analysis of the differential system of the pneumatic valve made it possible to evaluate its performance. Spring stiffness and piston bore throughput were used as input variables. The mathematical model, adopted on the basis of the necessary simplifications and lumped method, allowed a number of groups of results (maps) to be obtained numerically. These results served as input data for further processing aimed at selecting the most optimal solution. On this basis, it should be concluded that the work has been achieved. The main conclusions of the analysis are:

• The smallest spring stiffness analyzed of 0.5 × 10⁵ N/m showed an opening time of 0.24 (0.33) s and a valve closing time of 7.08 (7.5) s depending on the throughput of the piston bore;
• The highest stiffness (1.45 × 10⁵ N/m) resulted in a slight increase in opening time to 0.26 (0.37) s and a marked decrease in closing time to 1.32 (7.08) s, depending on the throughput of the piston bore;
• The smallest piston bore throughput of 0.05 × 10⁻⁶ m² resulted in an opening time of 0.24 (0.36) s and a closing time of 7.08 (7.5) s depending on the spring stiffness;
• The highest piston bore throughput of 0.525 × 10⁻⁶ m² showed a similar opening time of 0.24 (0.33) s with a significant reduction in closing time to 1.32 (1.59) s;
• Not all analyzed configurations were able to achieve a maximum piston displacement (valve opening) of 7.3 mm, which may affect the effectiveness of the valve acceleration mode;
• Different times of accelerating action calculated as the difference of closing and opening times did not guarantee constant efficiency due to different displacements of the piston;
• The optimization carried out using the genetic algorithm yielded results corresponding to its stated purpose but required complementary analysis to select the optimal variant.

The results of calculations, analysis, and optimization show that the differential brake valve, although developed in the second half of the 20th century, is still a functional challenge. However, the mathematical model of the differential part of the valve presented in the paper and the proposal to use genetic optimization show the way to increase the efficiency of this type of design. The purpose of the differential valve is to accelerate the system’s operation during the first phase of sudden braking and to be able to switch to tracking operation. The suboptimal analysis included in this study indicated that this is possible.

The widespread use of electronics in actuators and their appropriate control, especially in the throughput area of the inter-chamber opening, may result in the emergence of a design of this type of valve with adaptive capabilities to trailer loading conditions.