Experimental and Theoretical Investigation of Thermal Parameters Influencing the Freezing Process of Ice Cream
Abstract
1. Introduction
2. Materials and Methods
2.1. Calculation of Thermal Properties
2.1.1. Initial Freezing Temperature
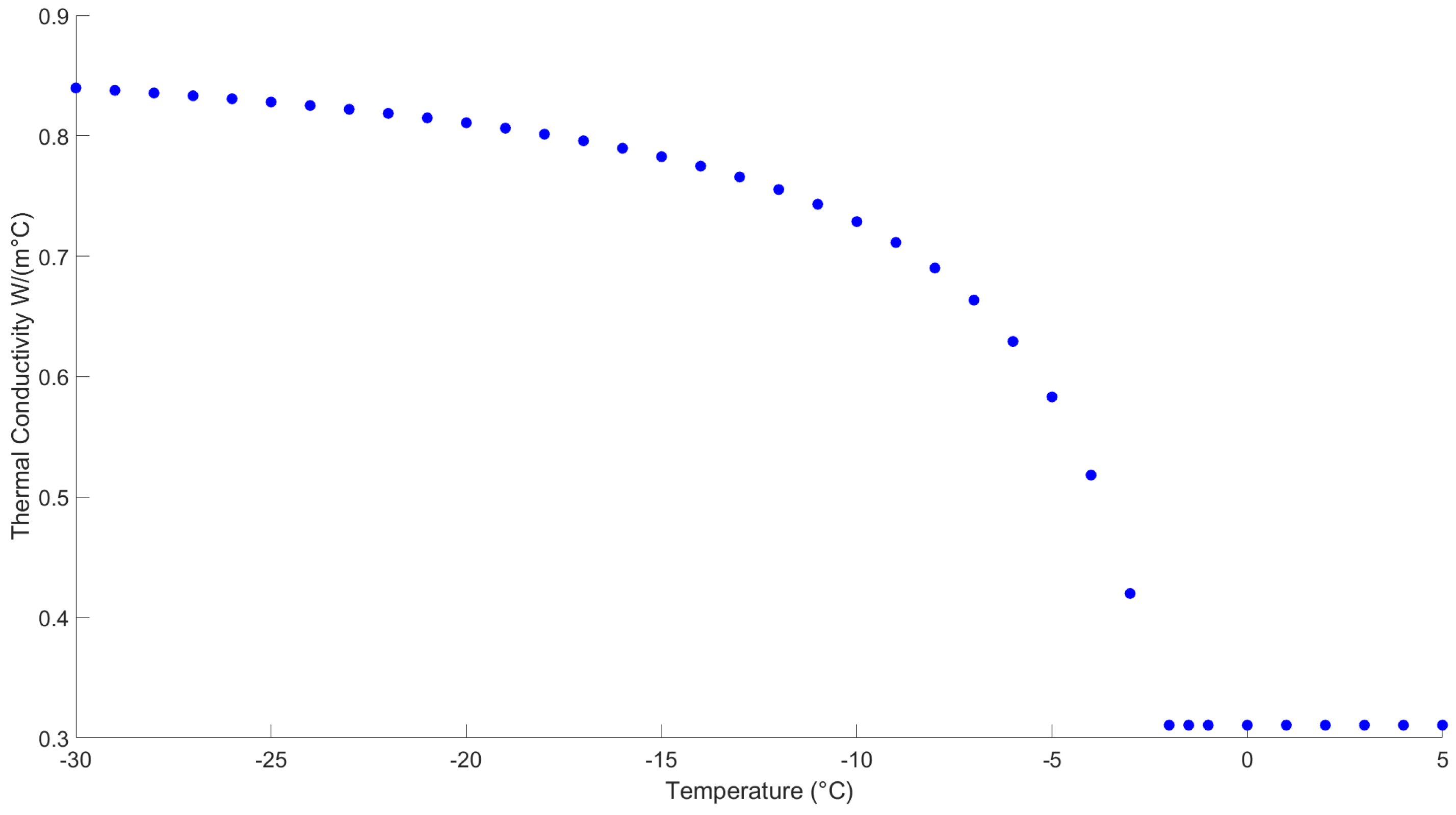
2.1.2. Thermal Conductivity
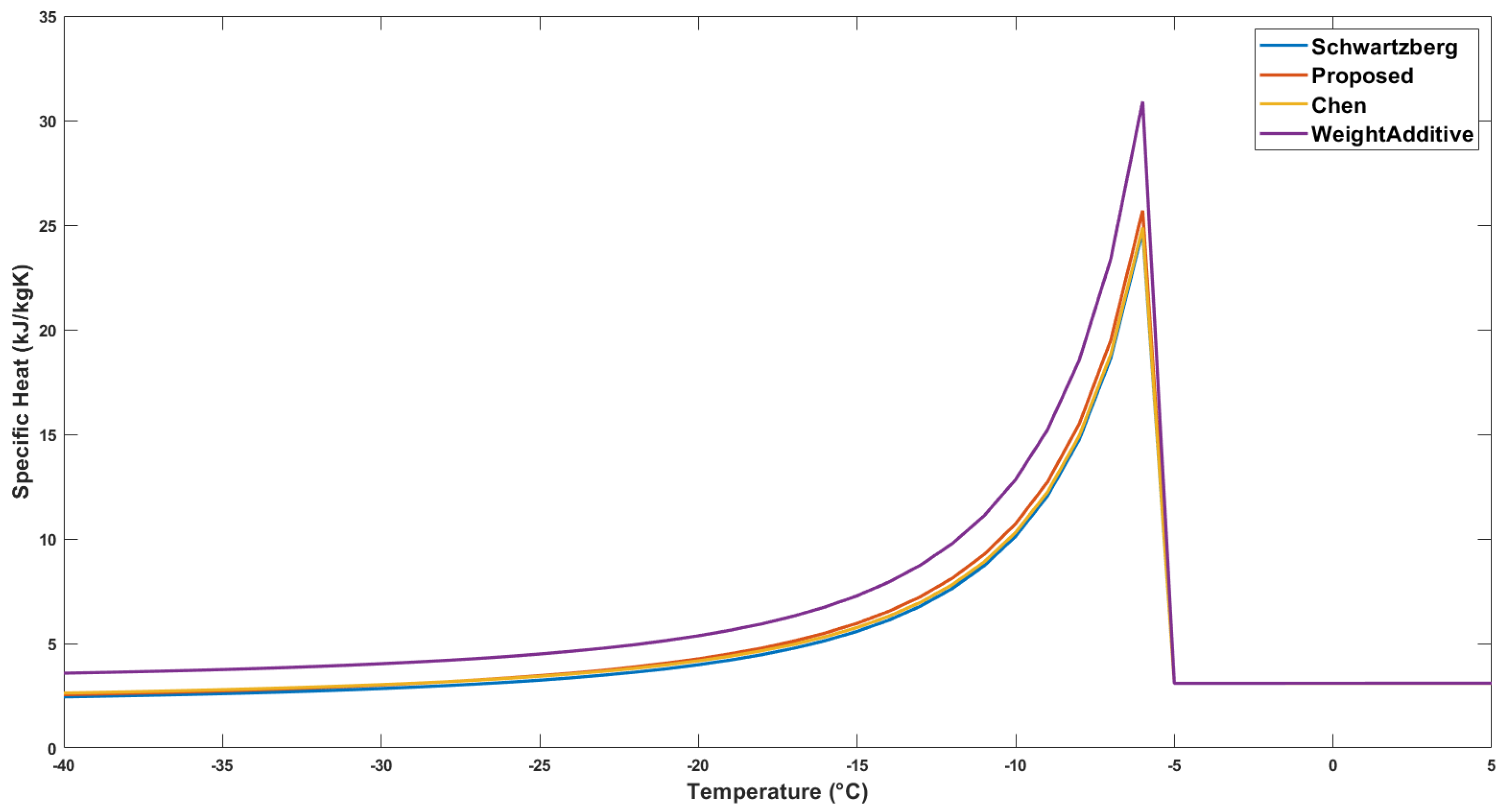
2.1.3. Specific Heat
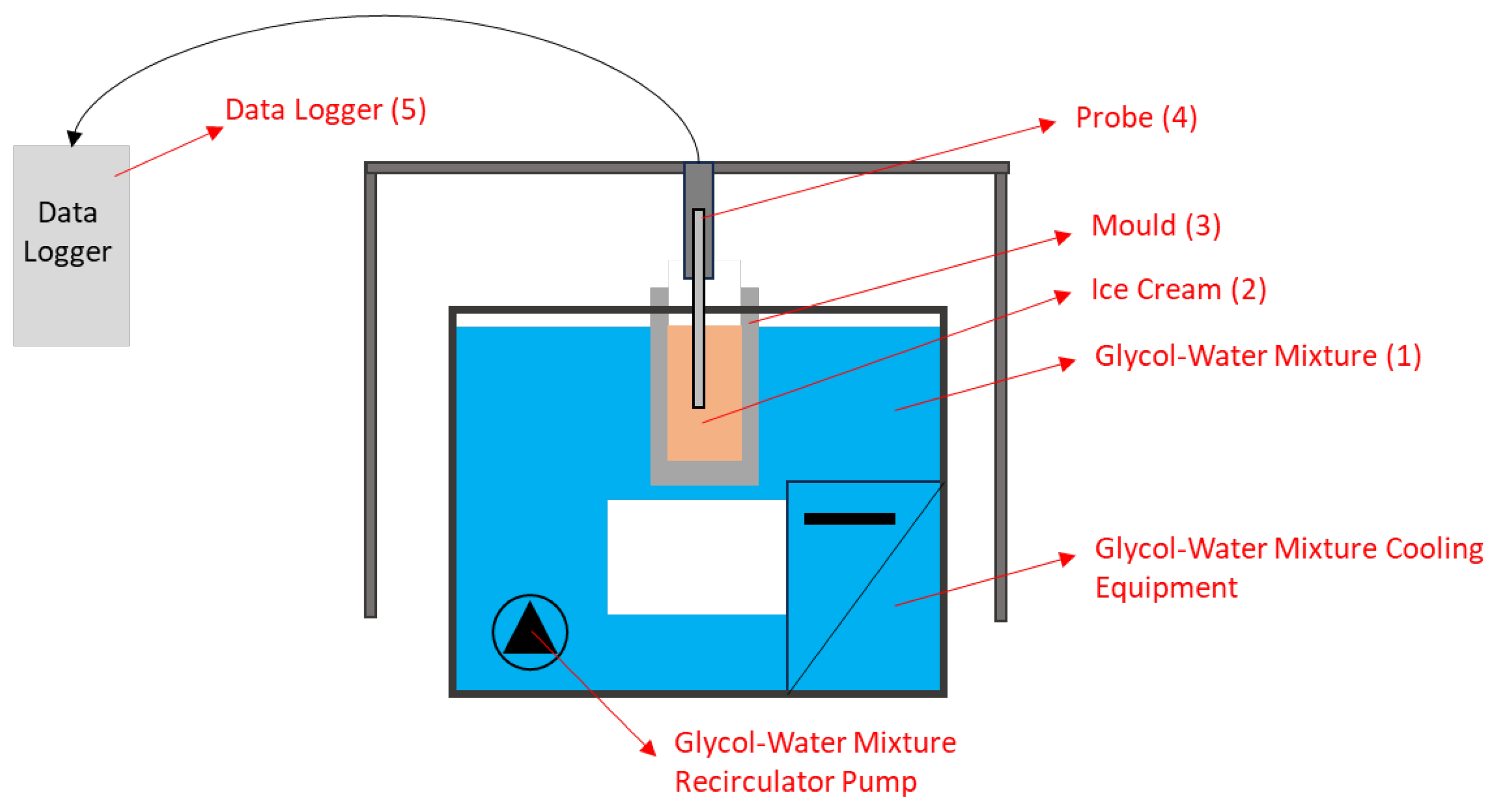
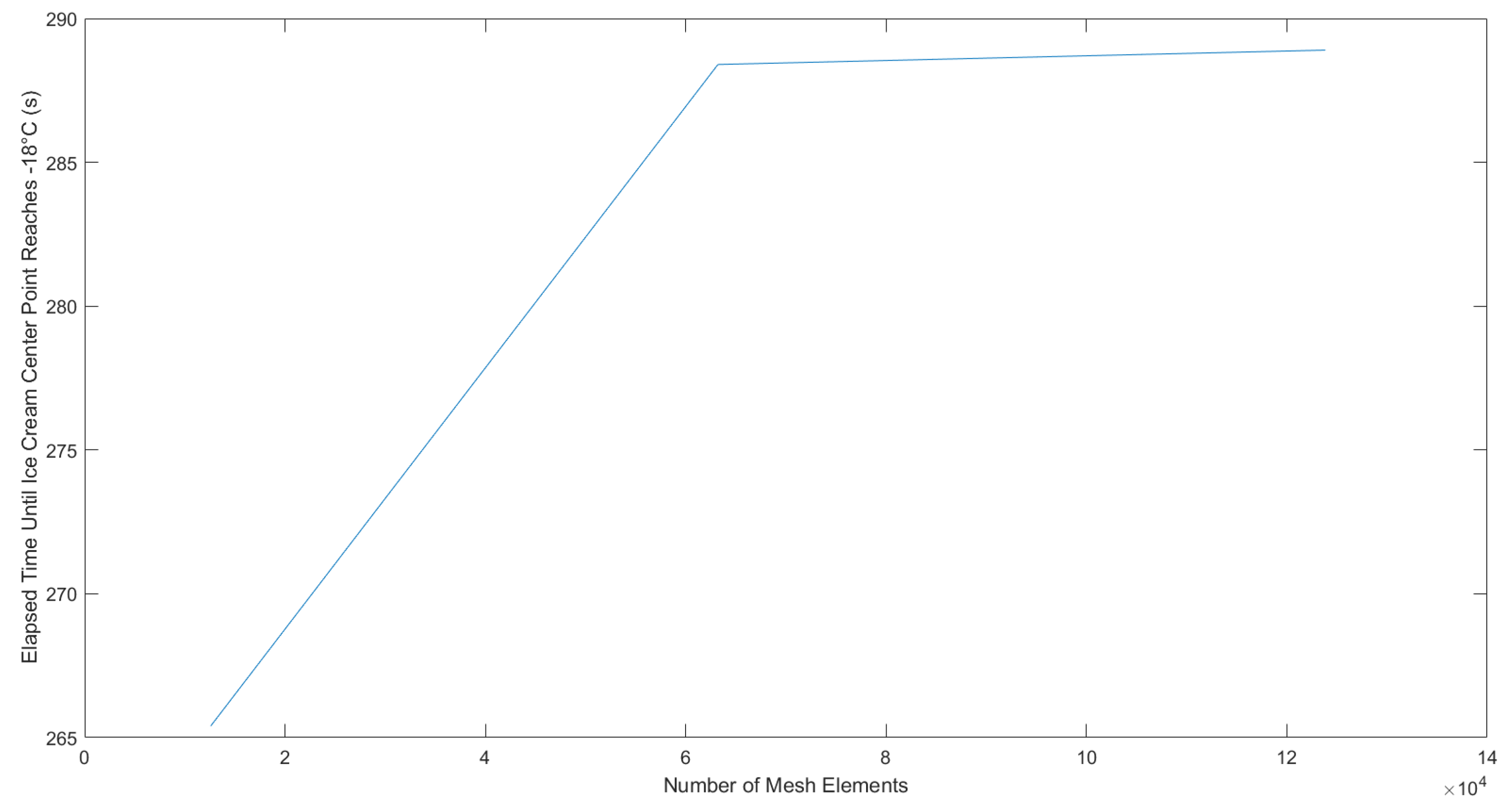
2.2. Numerical and Experimental Studies
3. Results
3.1. Calculation of Experimental Ice Cream Sample Thermal Properties
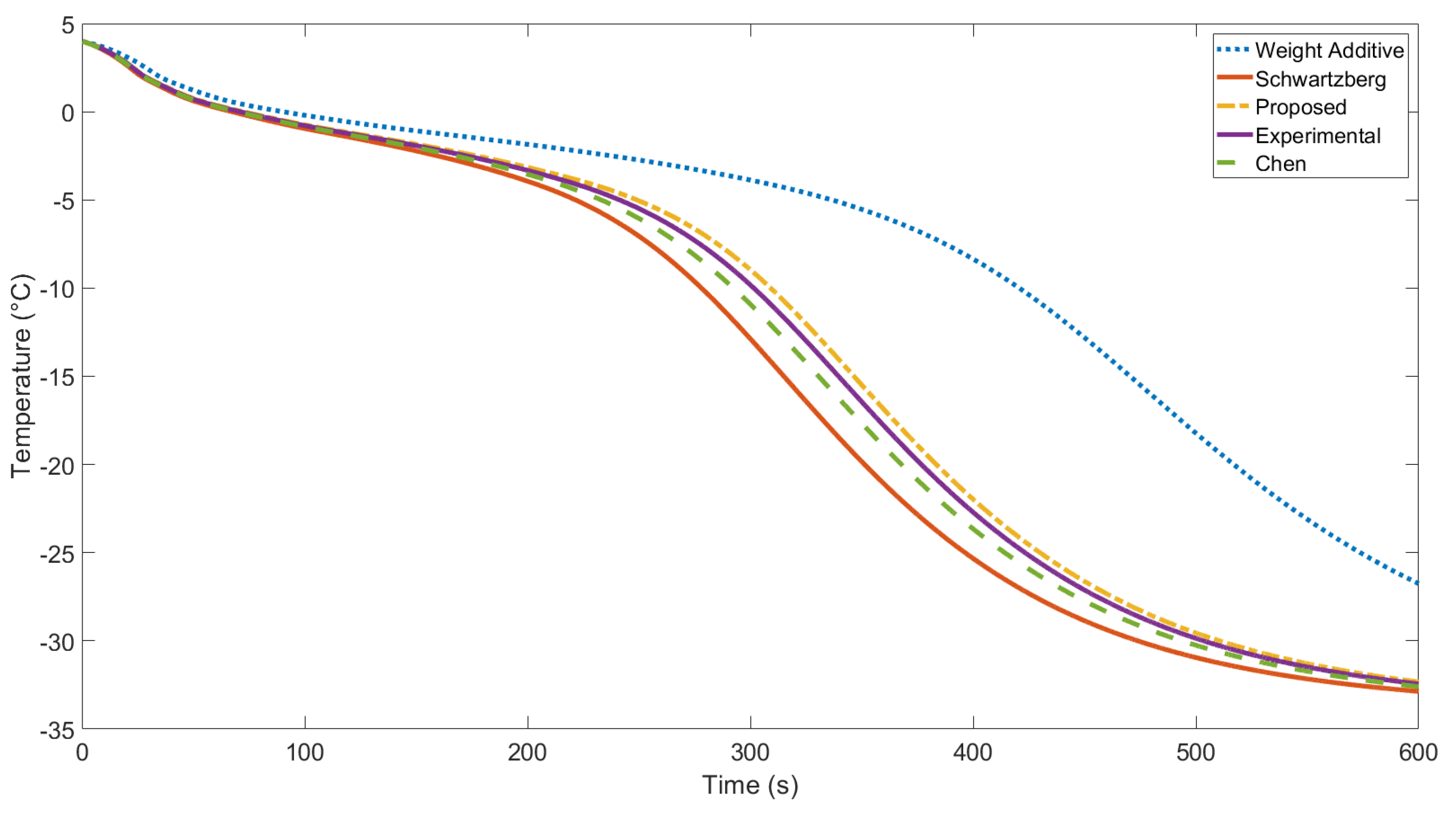
3.2. Experimental and Numerical Model Results
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lind, I. The measurement and prediction of thermal properties of food during freezing and thawing —A review with particular reference to meat and dough. J. Food Eng. 1991, 13, 285–319. [Google Scholar] [CrossRef]
- Sanz, P.; Alonso, M.D.; Mascheroni, R. Thermophysical Properties of Meat Products: General Bibliography and Experimental Values. Trans. ASAE 1987, 30, 283–289. [Google Scholar] [CrossRef][Green Version]
- Simion, A.; Dobrovici, P.E.; Lacramioara, R.; Gavrilă, L. Modeling of the thermo-physical properties of grape juice I. Thermal conductivity and thermal diffusivity. Annals. Food Sci. Technol. 2009, 10, 363–368. [Google Scholar]
- Telis-Romero, J.; Telis, V.R.N.; Gabas, A.L.; Yamashita, F. Thermophysical properties of Brazilian orange juice as affected by temperature and water content. J. Food Eng. 1998, 38, 27–40. [Google Scholar] [CrossRef]
- Magerramov, M. Heat capacity of natural fruit juices and of their concentrates at temperatures from 10 to 120 °C. J. Eng. Phys. Thermophys. 2007, 80, 1055–1063. [Google Scholar] [CrossRef]
- Magerramov, M.; Abdulagatov, A.; Abdulagatov, I.; Azizov, N. Thermal conductivity of peach, raspberry, cherry and plum juices as a function of temperature and concentration. J. Food Process. Eng. 2006, 29, 304–326. [Google Scholar] [CrossRef]
- Cogné, C.; Andrieu, J.; Laurent, P.; Besson, A.; Nocquet, J. Experimental data and modelling of thermal properties of ice creams. J. Food Eng. 2003, 58, 331–341. [Google Scholar] [CrossRef]
- Sudhir, K.S.; Ashis, K.D. Thermal Properties of Frozen Peas, Clams and Ice Cream. Can. Inst. Food Sci. Technol. J. 1984, 17, 242–246. [Google Scholar] [CrossRef]
- Heldman, D.R.; Hedrick, T.I. Refrigeration requirements for ice cream freezing. J. Dairy Sci. 1970, 53, 1194–1200. [Google Scholar] [CrossRef]
- Bongers, P.M.M. A heat transfer model of a scraped surface heat exchanger for ice cream. In 16th European Symposium on Computer Aided Process Engineering and 9th International Symposium on Process Systems Engineering; Marquardt, W., Pantelides, C., Eds.; Computer Aided Chemical Engineering; Elsevier: Amsterdam, The Netherlands, 2006; Volume 21, pp. 539–544. [Google Scholar] [CrossRef]
- Boccardi, G.; Celata, G.P.; Lazzarini, R.; Saraceno, L.; Trinchieri, R. Development of a heat transfer correlation for a Scraped-Surface Heat Exchanger. Appl. Therm. Eng. 2010, 30, 1101–1106. [Google Scholar] [CrossRef]
- Imran, A.; Rana, M.A.; Siddiqui, A.M.; Shoaib, M. Flow of second grade fluid in a scraped surface heat exchanger. J. Food Process. Eng. 2017, 40, e12393. [Google Scholar] [CrossRef]
- Masuda, H.; Sawano, M.; Ishihara, K.; Shimoyamada, M. Effect of agitation speed on freezing process of ice cream using a batch freezer. J. Food Process. Eng. 2020, 43, e13369. [Google Scholar] [CrossRef]
- Cogné, C.; Laurent, P.; Andrieu, J.; Ferrand, J. Experimental data and modelling of ice cream freezing. Chem. Eng. Res. Des. 2003, 81, 1129–1135. [Google Scholar] [CrossRef]
- Schwartzberg, H.G. Effective heat capacities for the freezing and thawing of food. J. Food Sci. 1976, 41, 152–156. [Google Scholar] [CrossRef]
- Chen, C.S. Thermodynamic analysis of the freezing and thawing of foods: Enthalpy and apparent specific heat. J. Food Sci. 1985, 50, 1158–1162. [Google Scholar] [CrossRef]
- Leighton, A. On the calculation of the freezing point of ice cream mixes and of the quantities of ice separated during the freezing process. J. Dairy Sci. 1927, 10, 300–308. [Google Scholar] [CrossRef]
- Chang, H.D.; Tao, L.C. Correlations of enthalpies of food systems. J. Food Sci. 1981, 46, 1493–1497. [Google Scholar] [CrossRef]
- Marshall, R.T.; Goff, H.D.; Hartel, R.W. Ice Cream; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Miles, C.A.; van Beek, G.; Veerkamp, C.H. Calculation of thermophysical properties of foods. In Physical Properties of Foods; Jowitt, R., Ed.; Applied Science Publishers: London, UK, 1983. [Google Scholar]
- Fricke, B.; Becker, B. Evaluation of Thermophysical Property Models for Foods. HVAC&R Res. 2001, 7, 311–330. [Google Scholar] [CrossRef]
- American Society of Heating, Refrigerating, and Air-Conditioning Engineers. ASHRAE Handbook: Refrigeration; American Society of Heating, Refrigerating, and Air-Conditioning Engineers: Peachtree Corners, Georgia, 2006. [Google Scholar]
- Choi, Y.; Okos, M.R. Effects of the temperature and composition on the thermal properties of foods. In Food Engineering and Process Applications; IIR: Charlotte, NC, USA, 1986; pp. 93–101. [Google Scholar]
- Pyenson, H.; Dahle, C.D. Bound Water and its Relation to Some Dairy Products: I. The Bound Water Content of Some Milk Products and Other Products Used in the Dairy Industry. J. Dairy Sci. 1938, 21, 169–185. [Google Scholar] [CrossRef]


| Sucrose Amount Dissolved in 100 g Water (g) | Freezing Point Depression (°C) |
|---|---|
| 3 | 0.18 |
| 6 | 0.35 |
| 9 | 0.53 |
| 12 | 0.72 |
| 15 | 0.90 |
| 18 | 1.10 |
| 21 | 1.29 |
| 24 | 1.47 |
| 27 | 1.67 |
| 30 | 1.86 |
| 33 | 2.03 |
| 36 | 2.21 |
| 39 | 2.40 |
| 42 | 2.60 |
| 45 | 2.78 |
| 48 | 2.99 |
| 51 | 3.20 |
| Food Item | Water Xwo | Protein Xp | Fat Xf | Carbohydrate Xc | Ash Xa |
|---|---|---|---|---|---|
| Chocolate ice cream | 55.7 | 3.8 | 11 | 29.4 | 1 |
| Correlation | Specific Heat Above Freezing kJ/(kg K) | Specific Heat Below Freezing kJ/(kg K) |
|---|---|---|
| ASHRAE | 3.11 | 2.75 |
| Chen | 3.09 | 2.79 |
| Schwartzberg | 3.10 | 2.60 |
| Weight Additive | 3.10 | 3.75 |
| Proposed | 3.10 | 2.74 |
| Glycol–Water Mixture Temperature (°C) | Initial Ice Cream Temperature (°C) | Aeration Amount (%) | Elapsed Time Until Ice Cream Center Point Reaches −18 °C (s) |
|---|---|---|---|
| −40 | −1 | 30 | 229.6 |
| −40 | −1 | 50 | 238.4 |
| −40 | −1 | 80 | 311 |
| −40 | 1 | 30 | 242.4 |
| −40 | 1 | 50 | 251.6 |
| −40 | 1 | 80 | 328.2 |
| −40 | 4 | 30 | 260.2 |
| −40 | 4 | 50 | 269.8 |
| −40 | 4 | 80 | 351.6 |
| −37 | −1 | 30 | 254 |
| −37 | −1 | 50 | 263.6 |
| −37 | −1 | 80 | 344 |
| −37 | 1 | 30 | 268.4 |
| −37 | 1 | 50 | 278.6 |
| −37 | 1 | 80 | 363.2 |
| −37 | 4 | 30 | 288.4 |
| −37 | 4 | 50 | 299.2 |
| −37 | 4 | 80 | 389.6 |
| −34 | −1 | 30 | 284.8 |
| −34 | −1 | 50 | 303.2 |
| −34 | −1 | 80 | 385.6 |
| −34 | 1 | 30 | 301.2 |
| −34 | 1 | 50 | 312.6 |
| −34 | 1 | 80 | 407.3 |
| −34 | 4 | 30 | 324.4 |
| −34 | 4 | 50 | 336.4 |
| −34 | 4 | 80 | 437.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Atici, Ö.A.; Özkan, D.B. Experimental and Theoretical Investigation of Thermal Parameters Influencing the Freezing Process of Ice Cream. Appl. Sci. 2024, 14, 6194. https://doi.org/10.3390/app14146194
Atici ÖA, Özkan DB. Experimental and Theoretical Investigation of Thermal Parameters Influencing the Freezing Process of Ice Cream. Applied Sciences. 2024; 14(14):6194. https://doi.org/10.3390/app14146194
Chicago/Turabian StyleAtici, Ömer Alp, and Derya Burcu Özkan. 2024. "Experimental and Theoretical Investigation of Thermal Parameters Influencing the Freezing Process of Ice Cream" Applied Sciences 14, no. 14: 6194. https://doi.org/10.3390/app14146194
APA StyleAtici, Ö. A., & Özkan, D. B. (2024). Experimental and Theoretical Investigation of Thermal Parameters Influencing the Freezing Process of Ice Cream. Applied Sciences, 14(14), 6194. https://doi.org/10.3390/app14146194






