Abstract
A parametric investigation of several unbraced steel frames with regular and irregular geometry subjected to elevated temperatures is carried out in this study to determine the most accurate procedure and buckling lengths to be considered during the structural design under a fire situation. In such conditions, the stiffness and strength of steel decrease considerably due to high temperatures, and uncertainty remains in the application of the fire design rules of Eurocode 3 Part 1-2 (EN 1993-1-2) for unbraced frames as no information is given regarding the treatment of the deformed geometry (the so-called second-order effects). More precisely, it is unclear in the norm whether the verification based on the buckling length concept could be used or if a second-order analysis to calculate the internal forces is sufficient to ensure the stability of the frame in case of fire. Based on the linear buckling analysis of the steel frames accounting for the temperature development during a fire, recommendations for the appropriate buckling lengths to be used are given. Finally, it is demonstrated that using the recommended buckling lengths together with the design rules of EN 1993-1-2 leads to results in favor of safety when compared to the results obtained with the finite element method. On the other hand, it is concluded that using second-order internal forces and the real length of the columns as the buckling length, as suggested for room temperature design, yielded results outside safety when compared with the finite element method, and this analysis and verification procedure should not be used for the case of fire.
1. Introduction
Despite the importance of fire safety, there are still some gaps in national and international standards, particularly with regard to fire design. Most often, steel structures need to be protected from fire to ensure compliance with regulatory requirements []. Fire design is a fundamental part of determining the necessary protection or to demonstrate that certain parts of the structure do not need passive protection to ensure structural safety required by regulations. On the other hand, the comprehensive analysis of steel structures requires an extensive examination of both vertical and horizontal external loads, along with their resultant effects on frame deformation [,]. Increased displacement within the structure induces changes in the stresses affecting its structural elements, thereby directly impacting structural stability.
Under normal temperature conditions, the holistic assessment of stresses and displacements within a structure relies primarily on several key factors: (i) deformability and stiffness characteristics; (ii) overall stability and the stability of individual elements; (iii) cross-sectional behavior; (iv) joint behavior; and (v) imperfections and deformability of supports. In terms of second-order effects, a clarification of the different methods of structural analysis applicable for the ultimate limit state design in the future revision of the Eurocode 3 is provided in [].
In the case of fire, the reduction in strength and stiffness of steel presents an additional difficulty for each of the mentioned points, and different studies on the behavior of frames in the case of fire have been reported in the past [,,,,,,]. Indeed, resulting from material degradation at elevated temperatures, the elements suffer large deformations, and there is also the effect of thermal expansion that increases the axial forces in beams and columns [,,]. These effects would only be negligible if the structures were heated uniformly in all members, which is unrealistic [].
Therefore, in the case of fire, the calculation of the internal forces must account with such effects through the concept of buckling lengths associated with the global instability mode of the frame []. However, following that this is a simplified approach to account for the second-order effects at elevated temperatures, it is necessary to check if the safety verification produces results in the favor of safety by comparison with more refined analysis, as in the present study.
To calculate the buckling lengths in the case of fire, both EN 1993-1-2 [] and ABNT NBR 14323 [] standards recommend that normal temperature procedures are used. In addition, the EN 1993-1-2 defines that, for braced frames, the values to adopt are for columns belonging to intermediate stories and for columns on the upper stories. No guidance is provided, however, for columns belonging to unbraced frames. In this context, Gomes et al. [] proposed equations for calculating buckling lengths of columns belonging to braced frames, while Couto et al. [] validated the recommended values suggested by EN1993-1-2 for the buckling lengths of columns in braced frames. In their work, it was recommended buckling lengths for columns of unbraced frames as well however, this study was limited to columns heated on four sides and beams on three sides, with generalized fire occurring in each story at a time, and only frames with regular geometry were considered. Although this work was later extended to natural fires [], confirming the validity of the suggested recommendations, there is still the need to cover other fire design situations.
A new method for evaluating the stability of unbraced steel frames under fire conditions was proposed in []. This method identifies significant fire scenarios that cause instability at the story level, using temperature and locality functions to quantify the severity of these scenarios. The method allows to identify the critical columns. In one study [], a new method for computing the lateral stiffness of an unbraced semi-rigid steel story frame with three-segmented members was proposed. Their method can be applied towards many modeling problems where non-linear or piece-wise temperature gradients occur longitudinally in members. The story stability of unbraced steel frames subjected to non-uniform elevated temperature distribution has been studied in [].
A review of the progressive collapse mechanisms of steel frames in the case of fire is provided in [], and it has been identified that the load ratio, fire scenario, bracing layout, and fire protection are key factors affecting the collapse mechanism of steel structure. Li et al. [] have investigated experimentally and numerically the collapse modes of single span frames in the case of fire and identified four main collapse modes, including column lateral collapse, column buckling collapse, and overall collapse modes (inward and outward). The authors also identified that the heating conditions and rotational stiffness of the column base had a great influence on these collapse modes. In addition, the collapse modes related to columns seem to be influenced by temperature gradients, fire protection levels, and load ratios. Venkatachari and Kodur [] investigated the system-level response of braced frames under fire exposure scenarios and later, the same authors, studied the effect of transient creep on fire-induced instability in steel-framed structures []. In particular, it was demonstrated that the response of steel frames depends significantly on the location and type of fire exposure, as well as with the number of compartments under fire exposure. Notably, the stability of the structure was identified to be critically affected when the fire occurs in the lower levels of the building. However, the creep effects tend to be higher when the upper stories are subjected to fire, mainly because of the relatively slender members used in the top stories of the building []. Gernay et al. have developed fire fragility curves for steel frames [,]. Risco et al. [] have investigated the post-earthquake fire response of steel buildings. A review on the behavior of steel frames made of high strength steels under fire exposure is provided in [].
This study extends the previous investigation, carried out in [], by assessing the evolution of the critical load of several steel frames with irregular geometry in fire and for 12 different fire cases corresponding to different heating schemes mostly not covered in the former study. In addition, it was considered a generalized fire in a single isolated story or/and two stories simultaneously. These different assumptions allow to analyze the most suitable buckling lengths for the verification of structures in fire in a wider number of situations than those covered in [,]. Moreover, the present study investigated the fire resistance of steel frames using the simplified method, with the formulations proposed by EN1993-1-2 [], and then compared those results with the ones yielded by advanced calculation methods, i.e., the finite element method (FEM). The aim of such comparison was to validate the proposed buckling lengths and to show that the recommendations of EN1993-1-1 [] to use a buckling length equal to the system length when second-order analyses are used to calculate the internal forces leads to results outside of safety in the verification procedure and should not be used in case of fire.
2. Materials and Methods
This study analyzed 9 steel frames compromising regular and irregular geometries that were firstly designed at room temperature. At this step, several types of geometry were investigated for the irregular ones, with horizontal and vertical spans of different heights between stories and with cantilevered stories. It is assumed that frames have pinned or fixed supports with rigid connections between beams and columns that remained rigid during the evolution of the temperature. A more appropriate characterization of the rigidity of the connections during a fire is out of the-scope of the present study. Then, the calculation of the buckling length of the columns in the case of fire comprised 12 different fire cases. These buckling lengths were then used to calculate the fire resistance using the simplified design methods of the EN1993-1-2 []. Finally, the results obtained with the EN1993-1-2 were compared with those yielded by advanced design methods, i.e., the finite element method (FEM). In the FEM calculations, the members were divided into ten beam finite elements, and no heat transfer between compartments was considered; that is, only the compartment elements that are under fire conditions were subject to the action of temperature while the others remained at normal temperature. To allow the direct comparison with the simplified design methods, the variation in temperature over the element was not considered, thus it was constant along the cross-section and the member. Finally, the thermal expansion was only accounted for in the calculation of the fire resistance of the frames.
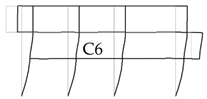
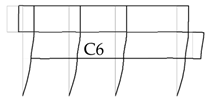
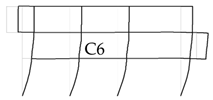
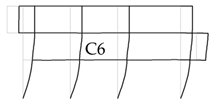
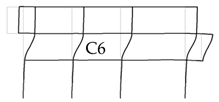
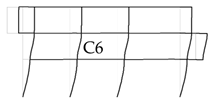
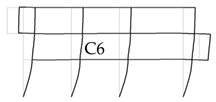
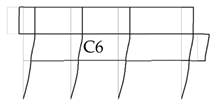
It can be seen in Figure 1 a summary of all case studies on this work. It is noteworthy that two analyses were conducted in determining the buckling factor for each scenario: (1) considering the fire at each story individually; (2) considering the fire on two stories simultaneously; (3) considering frames with pinned and fixed supports; and finally, (4) considering braced and unbraced frames. Frame geometries are detailed in Figure 2.
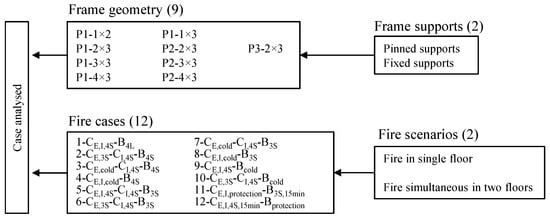
Figure 1.
Cases considered in this study.
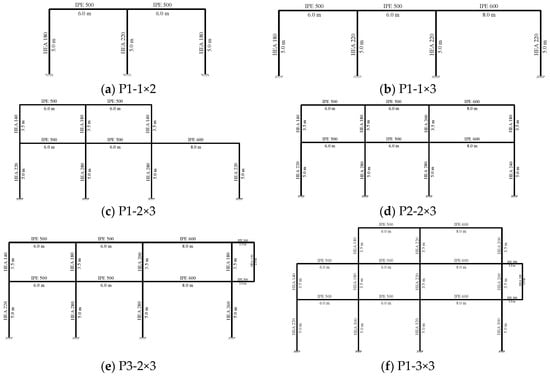
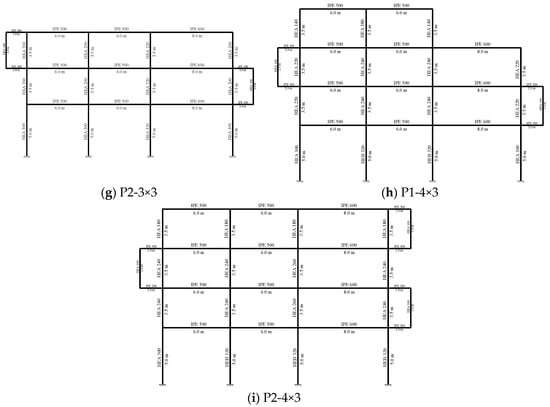
Figure 2.
Geometries, dimensions (in meters), and cross-sections of the studied frames.
2.1. Methodology Used in the Design of the Structure
Structural analysis of the frames to calculate the internal forces was carried out using the SAP 2000 [] program. Second-order effects (P-Δ) were included in the analysis and the design, which was performed in the elastic range. In addition, global frame imperfections were modeled by the use of equivalent horizontal forces, as suggested in EN 1993-1-1 [].
The design of the beams and columns of the frames was performed in accordance with EN 1993-1-1. The columns are of type HEA and HEB and beams of type IPE. All the members were designed in S355 steel and were calculated with the buckling coefficient in all members. It should be emphasized that the values of the actions and combinations considered are in accordance with the relevant Eurocodes [,]. Figure 2 shows the final sections obtained.
For the design in case of a fire, and because fire is classified as an accidental action, in the EN 1990 [], accordingly the design effect of actions for the fire situation, , must be obtained using the accidental load combination given by:
Table 1 shows the load combinations used in this study at elevated temperatures, where “Combination 1” was used in the buckling analysis and “Combinations 1 and 2” were used in the calculation of the fire resistance, where, is the characteristic value of the variable action, is the characteristic value of the permanent action, is the characteristic value of the wind action, and are the combination factors affecting the leading action as defined in EN1991-1-2 and represent the design value of the indirect thermal action due to the fire. In the load combinations of Table 1, the frequent value of the leading variable action was used.

Table 1.
Load combinations.
2.2. Methodology Used to Determine the Critical Column of the Frame at Normal Temperature (20 °C) and in Fire
At normal temperature, the critical column is the one that belongs to the critical story and has the highest ratio between axial compression force and the Euler critical load . Thus, the parameter can be defined as:
where l is the member length, E is the Young modulus at room temperature, and I is the section moment of inertia. Similarly, in the case of fire, the critical column corresponds to the one with the highest parameter , where the subscript “” denotes the fire situation and can be calculated as:
the is the reduction factor for the Young modulus at elevated temperature according to EN1993-1-2.
2.3. Methodology Used to Determine Buckling Lengths in Fire
For the critical columns in fire, as explained in Section 2.2, the following methodology was followed. First, a thermal analysis using the software Elefir-EN v1.4.5 [] was carried out considering uniform temperature in the sections. Then, the elastic critical amplifier in fire () was calculated for the same assumptions using linear buckling analysis in CAST3M 2015 []. The temperatures were varied between 20 °C and 1100 °C. Finally, the buckling length in fire, , can be computed as follows:
where is the critical buckling load of the column, is the axial force in the column under fire conditions, is the column length, and is the associated buckling coefficient.
2.4. Determination of Fire Cases
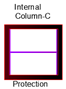
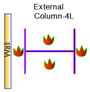
To cover a wide range of fire situations, two fire scenarios and twelve heating scenarios were investigated. Accordingly, it was considered that the frames were subjected to a generalized fire in one story, and additionally, a generalized fire acting simultaneously in two stories was also considered. Although the event of a fire might affect only one story, there are several situations reported where fire was acting on two stories simultaneously. For each fire scenario, twelve combinations, or fire cases, regarding the heating scheme of the sections were defined and are presented in Table 2. In this table, “C” denotes a column, “B” denotes a beam, “E” and “I” refer to external or internal, respectively, and “4S”, “3S”, “cold” indicate the heating of the section as 4-sides, 3-sides, or cold.

Table 2.
Fire cases.
Cases 1, 5, and 9 simulate a building in which the steel structure is internal and the enclosure (outer walls) is external. Cases 2, 6, and 10 relate to buildings in which the steel structure is partially internal and the shuttering is embedded therein. Alternatively, cases 3, 4, 7, and 8 simulate buildings in which the steel structure is external to the shuttering, with the latter serving as protection for these columns.
Regarding the exposure of the beams to the fire, on cases 1 to 4, it is considered that the beams are heated on four sides, i.e., the story does not protect the upper flange of the steel beam; cases 5 to 8 have fire-exposed beams on three sides, i.e., the story protects the upper flange of the steel beam; in cases 9 and 10, the beams are protected by the story and by the ceiling, i.e., they are at normal temperature (see Table 2).
For the columns in cases 1 to 3, 5 to 7, 9 and 10, it is considered that the internal columns are heated on all four sides, while cases 4 and 8 have the internal columns protected. Finally, case 11 simulates one building where the steel structure is protected for a certain period of time and a failure in the beam protection occurs at 15 min, i.e., after 15 min the beams heated on three sides and the columns remain protected; conversely, in case 12, the protection failure occurs in the column, with columns becoming heated on four sides and beams remaining protected (see Table 2).
2.5. Methodology Used for Determination of Fire Resistance
The fire resistance of the frames was calculated for the ISO 834 [] fire curve with simplified and advanced design methods. The first corresponds to the application of design rules of the EN1993-1-2 [], for which the ELEFIR-EN [] software was used. The advanced design methods consist in the calculation using the finite element method; in this work, the software SAFIR v2019.a.6 [] was used for that purpose.
In the simple design methods, the temperature was considered constant in the section, while for the advanced design method, the temperature on the cross-sections was calculated in SAFIR considering a discretization mesh with 108 finite elements in the cross-section (see Figure 3).

Figure 3.
Discretization mesh with 108 finite elements in the cross-section.
3. Results and Discussion
3.1. Determination of the Buckling Coefficient
This section presents the results for the buckling coefficient for the unbraced frame P2-3×3 for all fire cases described in Table 2 and for all frames exposed to the fire case 6.
3.1.1. Critical Column of the Frame at 20 °C
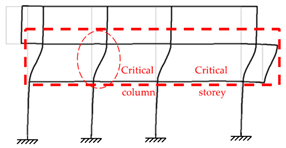
Table 3 highlights the critical stories of the frames for the instant t = 0 (room temperature), corresponding to the ground story for the pinned supports and the first story for the fixed supports. In this table it is also identified the critical column, as explained in Section 2.2.

Table 3.
Critical mode of instability of frames with fixed and pinned support at time t = 0 min.
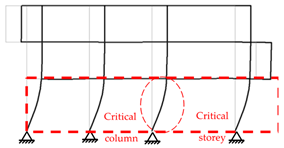
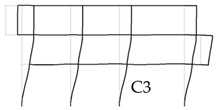
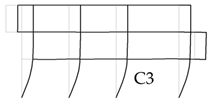
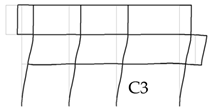
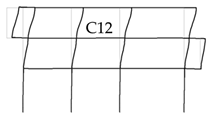
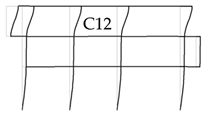
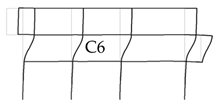
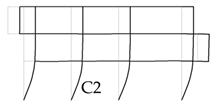
Furthermore, in Table 4 the critical columns of the unbraced frame are calculated. At the story 0 (ground story), it is observed that the column with the highest η is the column C3. In story 1, it is column C6, and at story 2, it is the column C10. Thus, it can be concluded that the critical column of frame P2-3×3, with pinned support, is column C3. A similar calculation is performed for the fixed support cases, where it was determined that column C6 was the critical one.

Table 4.
Critical column of the unbraced frame P-3×3 for normal temperature.
3.1.2. Critical Column in Fire
As defined previously in Section 2.2, the critical column in a fire situation corresponds to the column with the highest ratio between axial compression force () and the Euler critical load as a function of temperature (). For all the cases considered in this study, it is highlighted in Table 5 the critical columns in fire situations. Table 6 summarizes the findings.

Table 5.
Critical mode of instability of frame P2-3×3 with fixed and pinned support at the instant the frame loses instability.

Table 6.
Critical Column of Frames in Fire.
During the fire, as the temperature increases, the Euler critical load decreases and, therefore, the parameter increases. Due to this variation of , the critical column may change between normal temperature and fire. For example, for the frame P2-3×3, the critical column at normal temperature is column C6 of story 1 and column C3 of story 0, respectively, for fixed and pinned supports, as shown in Table 3. However, for a generalized fire on story 2, after a certain temperature threshold, the critical column of the frame becomes column C12 of story 2, irrespectively of the supporting conditions, as shown in Table 5.
3.1.3. Critical Load Parameter in Fire
Figure 4 presents the evolution of the critical load parameter () relative to temperature with different support conditions (columns with fixed or pinned supports at the base) for the various fire cases.
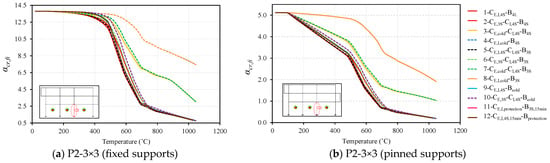

Figure 4.
Variation of the critical load parameter with changes in the temperature of unbraced frames P2-3×3.
As was the case in Couto et al. [], during the fire, the decreases because of the stiffness reduction that occurs in the members with increasing temperature. In addition, the evolution of the critical load parameter as a function of the temperature depends on whether the most critical columns, i.e., those with the highest at normal temperature, are affected by fire. In general, for a certain fire scenario, if the critical column in fire and normal temperature are the same, the reduces for lower temperature values, i.e., from the starting of the fire, as shown in Figure 4b,c,g–i. Otherwise, the critical load parameter remains unchanged, as illustrated in Figure 4a,d–f,j, until a certain temperature, and only after such temperature is reached does the story in fire begin to control the stability of the frame.
Finally, it was found that the phenomena mentioned in the above paragraphs occur in a similar manner for fires isolated in one story or occurring, simultaneously, in two adjacent stories.
Cases 1-(CE,I,4S-B4S), 2-(CE,3S-CI,4S-B4S), 5-(CE,I,4S-B3S), 6-(CE,3S-CI,4S-B3S), 9-(CE,I,4S-Bcold), 10-(CE,3S-CI,4S-Bcold), 11-(CE,I,protection-B3L,15min) and 12-(CE,I,3L,15min-Bprotection) showed similar behavior, i.e., whether columns and beams are heated in three or four sides did not significantly influence the critical load parameter. This phenomenon is justified by the fact that there are no significant temperature differences between columns and beams heated on 3 (3S) or 4 (4S) sides (see Figure 5).
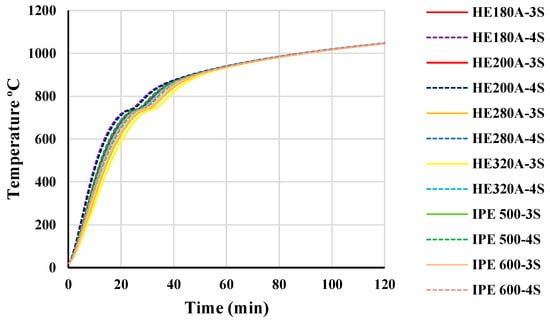
Figure 5.
Comparison between sections heated on 3 or 4 sides.
It was observed that cases 3-(CE, cold-CI,4S-B4S) and 7-(CE,cold-CI,4S-B3S) showed better behavior, i.e., for the same temperature the critical load parameter of these two cases was higher than in the other mentioned in the previous paragraph, since the story in fire presents greater stiffness for these cases. Only the internal columns and beams were heated, while the external columns were at normal temperature. This phenomenon is more evident for cases where the heated story is not the critical story at normal temperature. Finally, it was also observed that frames with pinned supports are more sensitive to instability (see Figure 4).
Meanwhile, cases 4-(CE,I,cold-B4S) and 8-(CE,I,cold-B3S) had better performance than all other cases, since only the beams are heated, and thus there was not much reduction of stiffness in the frames. For the cases where the frames have pinned supports and for which the beams in fire do not belong to the critical story at normal temperature, it was found that fire in this story had little influence (and in some cases no effect at all) on the instability of the frames (see Figure 4d–f).
3.1.4. Buckling Lengths
The buckling length of a column depends on whether the instability of the frame is governed (or not) by the story for which the column belongs. In case of a fire, such a principle has a particular influence as the critical column may change from one story to another depending on the temperature evolution, as it is detailed in the next sections for the P2-3×3 frame with fixed and pinned supports.
Frame P2-3×3 with Fixed Supports for All Fire Cases
For the P2-3×3 frame with fixed supports, the critical column at normal temperature (20 °C) is located in the story 1 (see Table 3). However, analyzing Figure 6, is observed that, when the temperature on story 0 reaches 485 °C, the instability of the frame becomes governed by a column in this story, making it the critical one. In conclusion, from 20 °C up to 485 °C, the critical column that governs the instability of the unbraced frame with fixed supports is in story 1. However, due to high temperatures in story 0 there is a loss of rigidity in that story. Consequently, when temperature reaches 485 °C, this story governs the overall stability of the frame. This makes the critical load parameter vary significantly only from that temperature onwards. The critical load parameter () for this temperature is then used to determine values for Euler critical load (Equation (6)), and the corresponding buckling lengths (Equation (7)) and buckling factor (Equation (8)) at elevated temperatures for the several fire cases. It is worth mentioning that the same phenomenon is observed for a generalized fire on story 2, i.e., when the temperature in this story reaches approximately 545 °C (see Figure 4e), it becomes the critical one.
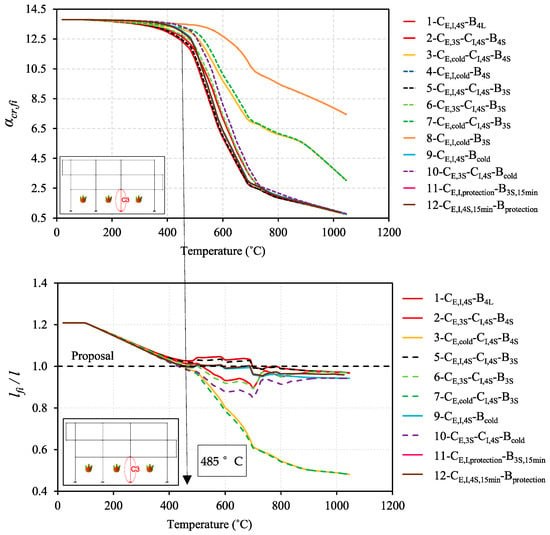
Figure 6.
Generalized fire in the story 0 of frame P2-3×3 with fixed supports for a standard fire.
Furthermore, in Figure 6, it is shown that the buckling length at normal temperature (20 °C) is while for a generalized fire in the story 0 the value of the buckling length corresponding to a temperature of 485 °C is approximately . When the fire is in story 1 or simultaneously in stories 0 and 1 and also simultaneously in stories 1 and 2, the critical column of the unbraced frame with fixed supports at 20 °C corresponds to the same as for the fire situation and thus governs the instability of the story both at 20 °C and at high temperatures. In these cases, it can be seen that the critical load parameter varies sharply since the beginning of the fire (Figure 4c,g,i) but the buckling lengths remain more or less constant during the fire, as shown in Figure 7. For both cases, whether the fire is developing on the critical story at normal temperature or not, it is concluded that it is conservative to use the buckling length determined at normal temperature when verifying the fire resistance of a column belonging to a steel frame as established by EN 1993-1-2 [] and ABNT NBR 14323: 2013 [].
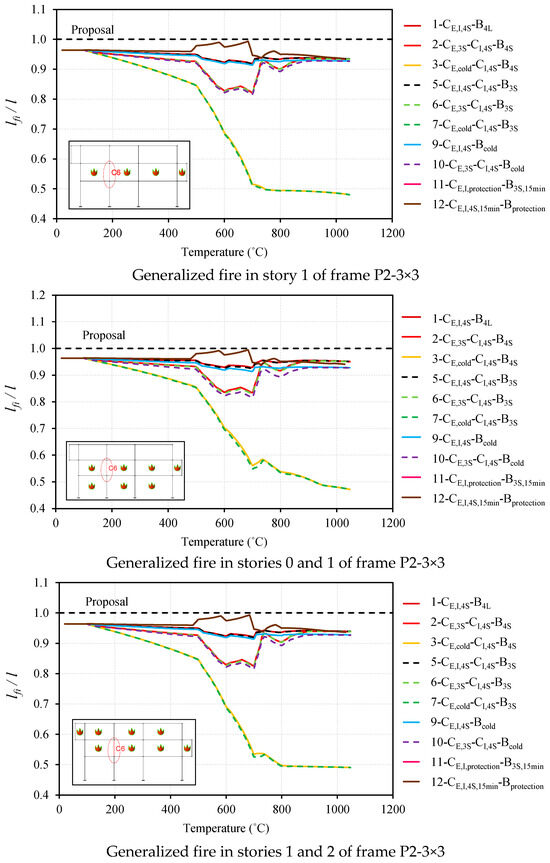
Figure 7.
Variation of the buckling factor with the temperature of the most critical column in frame P2-3×3 with fixed supports for a standard fire.
Frame P2-3×3 with Pinned Supports for All Fire Cases
For the frame P2-3×3 with pinned supports, the critical column at normal temperature (20 °C) is located in the story 0 (see Table 3). In this case, Figure 8 shows that, when the temperature on story 1 reaches 570 °C, the critical column of the frame now belongs to this story, and it governs the instability of the frame. In conclusion, from 20 °C up to 570 °C, the critical column that controls the instability of the pinned frame is in story 0 and due to high temperatures that develop in story 1, the critical column of this story gradually loses rigidity, and when the temperature reaches 570 °C in the story 1 it becomes the critical one. The same phenomenon is observed for story 2 when the temperature reaches approximately 670 °C (see Figure 4f).

Figure 8.
Generalized fire in story 1 of frame P2-3×3 with pinned supports for a standard fire.
As observed in Figure 8, the buckling length at normal temperature (20 °C) is and for a generalized fire in the story 1 the value of the buckling length corresponding to a temperature of 570 °C was approximately . Thus, it is safe to use the buckling lengths calculated at normal temperature in the case of fire for frames with pinned supports. Such observation is also valid when the fire is in story 0 (Figure 4b) or simultaneously in stories-0 and 1 (Figure 4h), since the critical column of the pinned frame at 20 °C is the same as in fire. This fact is responsible for the instability of the story both at 20 °C and at high temperatures, with the buckling coefficient being calculated at normal temperature (20 °C), and buckling length remains more or less constant during the fire, as shown in Figure 9.
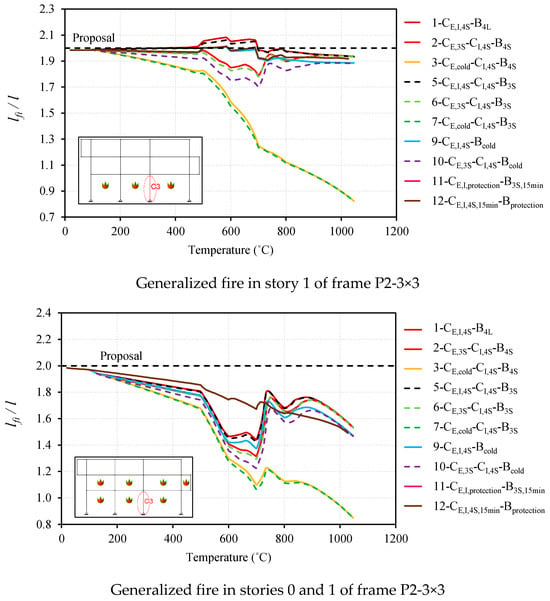
Figure 9.
Variation of the buckling factor with the temperature of the most critical column in frame P2-3×3 with pinned supports for a standard fire.
For cases 1-(CE,I,4S-B4L), 2-(CE,3S-CI,4S-B4S), 5-(CE,I,4S-B3S), 6-(CE,3S-CI,4S-B3S), 9-(CE,I,4S-Bcold), 10-(CE,3S-CI,4S-Bcold), 11-(CE,I,protection-B3S,15min) and 12-(CE,I,3S,15min-Bprotection), the highest buckling length value was considered. Thus, for generalized fire in a story at a time or, simultaneously, in two adjacent stories in fire, the buckling coefficient of was considered for all columns. However, for columns belonging to story 0 of the frame with pinned supports, the coefficient to be used is , (see Figure 10 and Figure 11).

Figure 10.
Buckling lengths of columns in unbraced frames with pinned supports.
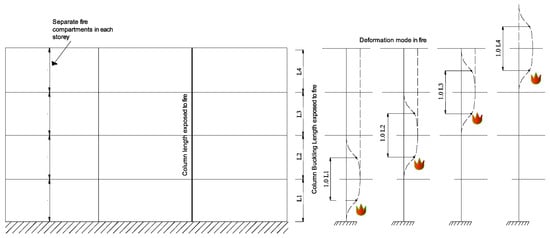
Figure 11.
Buckling lengths of columns in unbraced frames with fixed supports.
Cases 3-(CE,cold-CI,4S-B4S) and 7-(CE,cold-CI,4S-B3S), where the external columns are at normal temperature (20 °C) and internal columns and beams are heated on 3 or 4 sides, presented lower buckling lengths. For these cases, a buckling factor of was considered for all columns except those belonging to story 0 of the frame with pinned supports, for which the value to be used is .
Finally, it was found that for cases 4 and 8, where the columns are at normal temperature (20 °C) and internal beams are heated, due to loss of stiffness provided by the heated beams, the buckling lengths were higher. One must pay attention to these cases, especially if the utilization factors of the columns are high; such an event may lead to the collapse of the columns even if they are protected and/or not heated.
Buckling Lengths for All Different Frames for Fire Case-6
In this section, all steel frames with fixed and pinned supports, as shown in Figure 2, were analyzed for the case of fire 6-(CE,3S-CI,4S-B3S), considering that the fire was located in a single story and also the situation where two stories are simultaneously exposed to fire.
It was observed in Figure 12 and Figure 13 that, for different geometries of steel frames (i.e., regular and irregular), the differences between the values of the buckling factors have not shown to be significant.
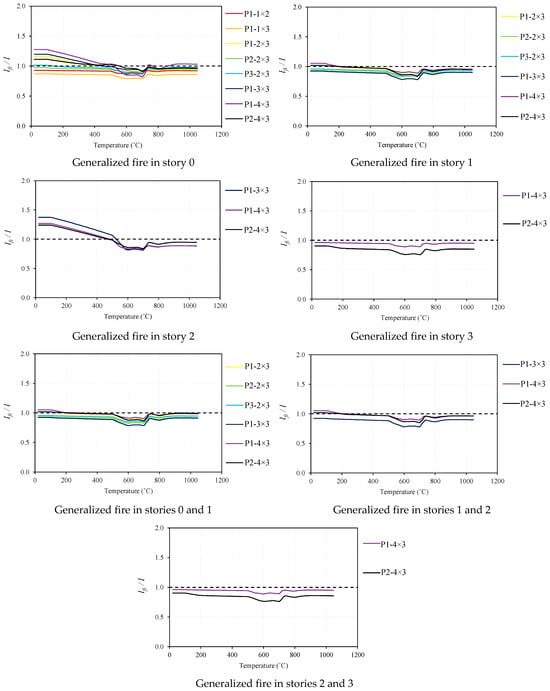
Figure 12.
Variation of the buckling factor with the temperature of the most critical column in all frames for scenario 6 with fixed supports.
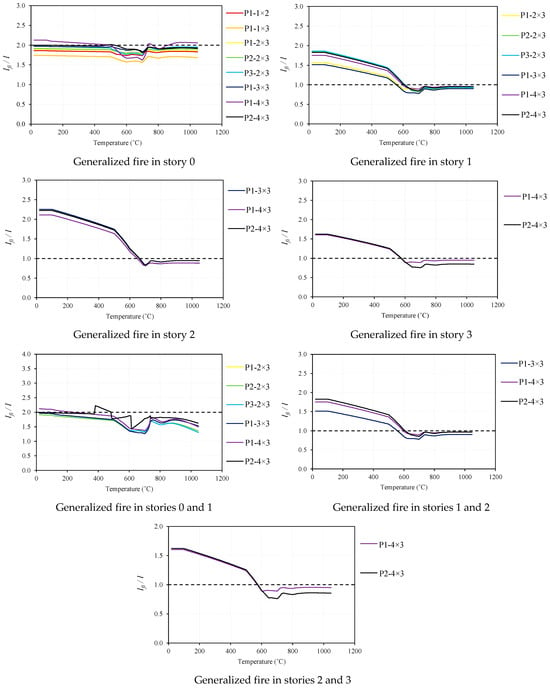
Figure 13.
Variation of the buckling factor with the temperature of the most critical column in all frames for scenario 6 with pinned supports.
We concluded that it is possible to propose approximate buckling lengths to verify the safety of regular and irregular unbraced steel frames fire was located in one story at a time or in two stories simultaneously. As a good approximation for the use of buckling length for an unbraced frame, the buckling length of a continuous column of a lower story will be for frames with fixed supports and for frames with pinned supports; in the remaining stories, the buckling length should be for frames with pinned and fixed supports, where l is the length of the column at the relevant story (see Figure 11 and Figure 14).
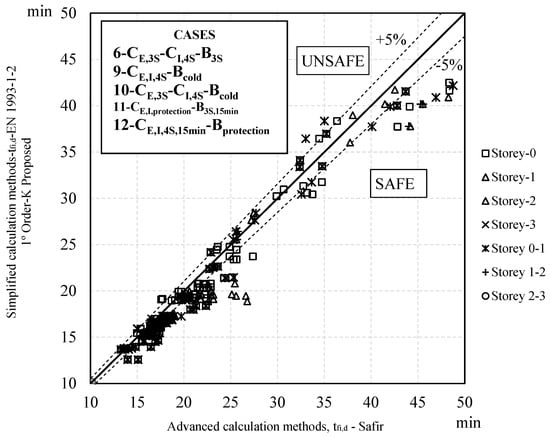
Figure 14.
Fire resistance time results for cases 6, 9, 10, 11, and 12, with fire in 1 story and 2 stories simultaneously at a time (1st simplified design method) vs. advanced design method).
3.2. Fire Resistance Analysis
This section reports the fire resistance analyses conducted in the steel frames studied for the various fire cases described in Section 2.4. The analyses used the simplified design method and the formulations proposed by EN1993-1-2 []. The results will be later compared with those yielded by finite element method (FEM) analyses with the software SAFIR []. The abscissa axis represents the fire resistance times obtained with SAFIR [], i.e., using an advanced design method based in the FEM. The ordinate axis shows the fire resistance time results calculated using the simplified method, considering first-order internal forces and using the buckling coefficients proposed in Section 3.1.4. The simplified design method results are deemed safe when lower fire resistance is obtained when compared to the resistance predicted by SAFIR and unsafe otherwise.
Fire Resistance Analysis for Various Fire Cases
The analyses that follow consider the results plotted in Figure 14 for all regular and irregular frames studied and for all fire cases (see Section 2.4). Considering fires in a single story or, simultaneously, in two adjacent stories, it can be observed that when first-order stresses and buckling coefficients (kfi), as proposed in this study, are used in the simple design methods, then satisfactory results are obtained on all stories for frames with fixed and pinned supports. This conclusion comes from the comparison between the results obtained from simple methods with the results obtained from a more advanced design method. In most cases, the differences between the simplified and advanced methods were of ±5%. However, considering second-order internal forces and buckling coefficients of kfi = 1.0), as can be seen in Figure 15, the simple design methods presented unsafe results for frames with pinned supports and fire in the lower story. In other cases, results are within the safety margin.
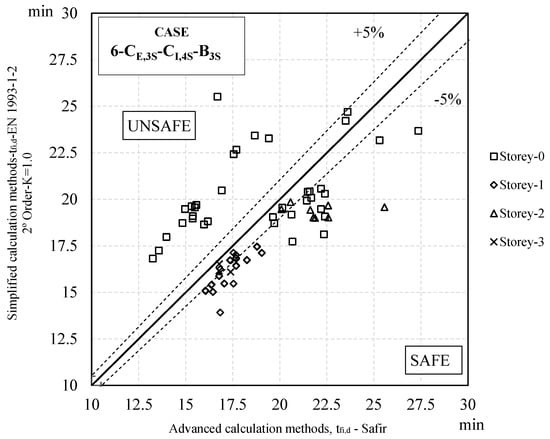
Figure 15.
Fire resistance time results for case 6 with fire in one story at a time (second simplified design method vs. advanced design method).
Regarding cases 3-(CE,cold-CI,4S-B4S) and 7-(CE,cold-CI,4S-B3S) (see Figure 16), it was found that these were too conservative for the cases in question. In other words, fire resistance times calculated by the simplified design method using a buckling factor of for all columns (except those belonging to story 0 of the pinned frame, for which was used) were well below the values calculated with the advanced FEM design. Moreover, it is observed in Figure 17 that, when the buckling coefficient used tends to for all columns (except those belonging to story 0 of the pinned frame, for which was used), the results were quite satisfactory.

Figure 16.
Fire resistance time results for cases 3 and 7 with fire in one story and two stories simultaneously at a time (second simplified design method (K = 1.0 and 2.0) vs. advanced design method).

Figure 17.
Fire resistance time results for cases 3 and 7 with fire in one story and two stories simultaneously at a time (simplified design method (K = 0.5 and 1.0) vs. advanced design method).
In all fire cases wherein the columns were heated, those were responsible for the collapse of the structure. However, in fire cases 4 and 8, in which the external and internal columns are at room temperature and only the beams are heated on 3 or 4 sides, the collapse of the structure was governed by the beams. According to Figure 18, it was found that in most cases the results were satisfactory and well within safety margins.
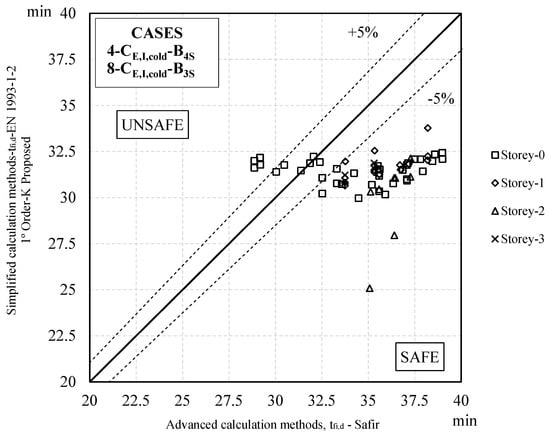
Figure 18.
Fire resistance time results for cases 4 and 8 with fire in one story at a time (simplified design method vs. advanced design method).
4. Conclusions
Steel frames exposed to fire lose strength and stiffness. Therefore, designers must adequately assess the behavior of steel frame elements in fire, trying to be as realistic as possible.
For the studied cases, it can be concluded that:
- Through a linear buckling analysis that considers the effect of temperature on the reduction in steel stiffness, it was found that the critical load of a steel frame in fire decreases as a function of the increase in temperature. All steel frames studied in this research presented lateral displacement of their joints at some point during the fire. For that reason, the most consistent manner to classify steel structures in fire is into braced and unbraced structures. In this study, braced frames were considered by simply restraining the lateral displacements, and in real situations, such restraint should be provided by the bracing system. The influence of the fire and its impact on the bracing system might have a significant influence on the behavior of the frames in case of fire. Further studies on this particular matter should be carried out but are outside the scope of the present paper. The instability of the steel frame during fire is not always controlled by the critical column at normal temperature;
- With this study, we concluded that it is possible to propose approximate buckling lengths to verify the safety of regular and irregular unbraced steel frames. For cases 1, 2, 5, 6, 9, 10, 11, and 12 (defined in Section 2.4), fire was located in one story at a time or in two stories, simultaneously. For the columns in the ground story, it is recommended a buckling length of and for frames with fixed or pinned supports, respectively; for the remaining columns of the remaining stories, a buckling length of is suggested.
- It was observed that, for different geometries of steel frames (i.e., regular and irregular), as well as for various fire cases, the differences between the values of the buckling factors have not shown to be significant.
- Considering the second-order stresses calculated at room temperature and taking into account the respective geometrical imperfections for pinned frames, it was concluded that adopting a buckling coefficient of 1.0 yielded results outside safety parameters. Such conclusion is possible when compared with the advanced design method. Therefore, such buckling coefficients used in case of fire are not recommended.
- Finally, it follows that a global first-order analysis of steel frames in fire without including the effects of imperfections in the verification of an equivalent column in relation to buckling phenomena and considering buckling lengths corresponding to the global mode of instability of the structure in fire is the best methodology for a simplified analysis of steel structures in fire.
Author Contributions
Conceptualization, T.S. and C.C.; methodology, C.C.; software, T.S. and C.C.; validation, C.C., P.V.R. and N.L.; investigation, T.S.; data curation, C.C.; writing—original draft preparation, T.S.; writing—review and editing, C.C., N.L. and P.V.R.; visualization, T.S.; supervision, C.C., P.V.R. and N.L. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by national funds through FCT—Fundação para a Ciência e a Tecnologia, I.P., under the Scientific Employment Stimulus-Institutional Call–awarded to the second author (CEECINST/00026/2018).
Data Availability Statement
The data will be made available upon reasonable request to the corresponding author.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Franssen, J.-M.; Vila Real, P. Fire Design of Steel Structures; ECCS; Ernst & Sohn: Berlin, Germany, 2010; ISBN 9789291470990. [Google Scholar]
- CEN EN 1993-1-1; Eurocode 3: Design of Steel Structures—Part 1-1: General Rules and Rules for Buildings. CEN: Brussels, Belgium, 2005.
- Simões da Silva, L.; Simões, R.; Gervásio, H. Design of Steel Structures Eurocode 3: Design of Steel Structures; Part 1-1: General Rules and Rules for Buildings; Ernst: Berlin, Germany, 2010; ISBN 9783433029732. [Google Scholar]
- Bureau, A.; Snijder, H.H.; Knobloch, M.; Kuhlmann, U.; Gardner, L.; Taras, A.; da Silva, L.S. Revision of EN 1993-1-1—Design Rules for Structural Analysis, Cross-sectional Resistance and Member Buckling. Steel Constr. 2022, 15, 202–212. [Google Scholar] [CrossRef]
- Couto, C.; Vila Real, P.; Lopes, N.; Rodrigues, J.P. Buckling Analysis of Braced and Unbraced Steel Frames Exposed to Fire. Eng. Struct. 2013, 49, 541–559. [Google Scholar] [CrossRef]
- Talebi, E.; Md Tahir, M.; Zahmatkesh, F.; Kueh, A.B.H. Comparative Study on the Behaviour of Buckling Restrained Braced Frames at Fire. J. Constr. Steel Res. 2014, 102, 1–12. [Google Scholar] [CrossRef]
- Silva, T.; Carić, M.; Couto, C.; Vila Real, P.; Lopes, N.; Skejic, D. Buckling Analysis of Steel Frames Exposed to Natural Fire Scenarios. Structures 2017, 10, 76–88. [Google Scholar] [CrossRef]
- Rackauskaite, E.; El-Rimawi, J. A Study on the Effect of Compartment Fires on the Behaviour of Multi-Storey Steel Framed Structures. Fire Technol. 2015, 51, 867–886. [Google Scholar] [CrossRef]
- Xu, L.; Ma, T.; Zhuang, Y. Storey-Based Stability of Unbraced Structural Steel Frames Subjected to Variable Fire Loading. J. Constr. Steel Res. 2018, 147, 145–153. [Google Scholar] [CrossRef]
- Skowrofiski, W. Plastic Load Capacity and Stability of Frames in Fire. Science 1997, 19, 764–771. [Google Scholar]
- Toh, B.W.S.; Tan, K.H.; Fung, T.C. Strength and Stability of Steel Frames in Fire: Rankine Approach. Manager 2001, 127, 461–469. [Google Scholar] [CrossRef]
- Li, G.Q.; He, J.L.; Jiang, S.C. Fire-Resistant Experiment and Theoretical Calculation of a Steel Beam. China Civ. Eng. J. 2000, 32, 23–26. [Google Scholar]
- Liu, T.C.; Fahad, M.; Davies, J. Experimental Investigation of Behaviour of Axially Restrained Steel Beams in Fire. J. Constr. Steel Res. 2002, 58, 1211–1230. [Google Scholar] [CrossRef]
- Shepherd, P.G.; Burgess, I.W. On the Buckling of Axially Restrained Steel Columns in Fire. Eng. Struct. 2011, 33, 2832–2838. [Google Scholar] [CrossRef]
- CEN EN 1993-1-2; Eurocode 3: Design of Steel Structures—Part 1-2: General Rules–Structural Fire Design. CEN: Brussels, Belgium, 2005.
- ABNT NBR 14323; Projeto de Estruturas de Aço e de Estruturas Mistas de Aço e Concreto de Edifícios em Situação de Incêndio. Associação Brasileira de Normas Técnicas: São Paulo, SP, Brazil, 2013.
- Gomes, F.; Providência e Costa, P.; Rodrigues, J.; Neves, I. Buckling Length of a Steel Column for Fire Design. Eng. Struct. 2007, 29, 2497–2502. [Google Scholar] [CrossRef][Green Version]
- Ma, T.; Xu, L. Storey-Based Stability of Unbraced Steel Frames under Piece-Linear Temperature Distributions. Eng. Struct. 2019, 194, 147–160. [Google Scholar] [CrossRef]
- Xu, L.; Zhuang, Y. Storey Stability of Unbraced Steel Frames Subjected to Non-Uniform Elevated Temperature Distribution. Eng. Struct. 2014, 62–63, 164–173. [Google Scholar] [CrossRef]
- Cao, Y.; Jiang, J.; Lu, Y.; Chen, W.; Ye, J. Progressive Collapse of Steel Structures Exposed to Fire: A Critical Review. J. Constr. Steel Res. 2023, 207, 107985. [Google Scholar] [CrossRef]
- Li, G.-Q.; Ji, W.; Feng, C.-Y.; Wang, Y.; Lou, G.-B. Experimental and Numerical Study on Collapse Modes of Single Span Steel Portal Frames under Fire. Eng. Struct. 2021, 245, 112968. [Google Scholar] [CrossRef]
- Venkatachari, S.; Kodur, V.K.R. System Level Response of Braced Frame Structures under Fire Exposure Scenarios. J. Constr. Steel Res. 2020, 170, 106073. [Google Scholar] [CrossRef]
- Venkatachari, S.; Kodur, V.K.R. Effect of Transient Creep on Fire Induced Instability in Steel Framed Structures. J. Constr. Steel Res. 2021, 181, 106618. [Google Scholar] [CrossRef]
- Gernay, T.; Elhami Khorasani, N.; Garlock, M. Fire Fragility Curves for Steel Buildings in a Community Context: A Methodology. Eng. Struct. 2016, 113, 259–276. [Google Scholar] [CrossRef]
- Gernay, T.; Khorasani, N.E.; Garlock, M. Fire Fragility Functions for Steel Frame Buildings: Sensitivity Analysis and Reliability Framework. Fire Technol. 2019, 55, 1175–1210. [Google Scholar] [CrossRef]
- Risco, G.V.; Zania, V.; Giuliani, L. Numerical Assessment of Post-Earthquake Fire Response of Steel Buildings. Saf. Sci. 2023, 157, 105921. [Google Scholar] [CrossRef]
- Pang, S.; Kodur, V.; Wang, W. Fire Resistance of Steel Structures, Incorporating HSS—Review and Recommendations. J. Constr. Steel Res. 2024, 217, 108677. [Google Scholar] [CrossRef]
- SAP2000 V14.0.0: Integrated Software for Structural Analysis and Design; CSI: Berkeley, CA, USA, 1995.
- CEN EN 1990; Eurocode—Basis of Structural Design. CEN: Brussels, Belgium, 2002.
- EN 1991-1-1; Eurocode 1: Actions on Structures—Part 1-1: General Actions—Densities, Self-Weight, Imposed Loads for Buildings. CEN: Brussels, Belgium, 2002.
- Vila Real, P.; Franssen, J.-M. Elefir-EN V1.4.5. Software for Fire Design of Steel Structural Members According the Eurocode 3. 2011. Available online: http://elefiren.web.ua.pt (accessed on 26 June 2015).
- CEA CAST 3M Is a Research FEM Environment; Its Development Is Sponsored by the French Atomic Energy Commission. 2015. Available online: http://www-cast3m.cea.fr/ (accessed on 26 June 2015).
- ISO 834-1:1999; Fire-resistance tests—Elements of building construction—Part 1: General requirements. International Organization for Standardization: Geneva, Switzerland, 1999.
- Franssen, J.-M.; Gernay, T. Modeling Structures in Fire with SAFIR: Theoretical Background and Capabilities. J. Struct. Fire Eng. 2017, 8, 300–323. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).