Modelling Wear Phenomena Specific to Mixer Blades in Concrete Production Plants
Abstract
1. Introduction
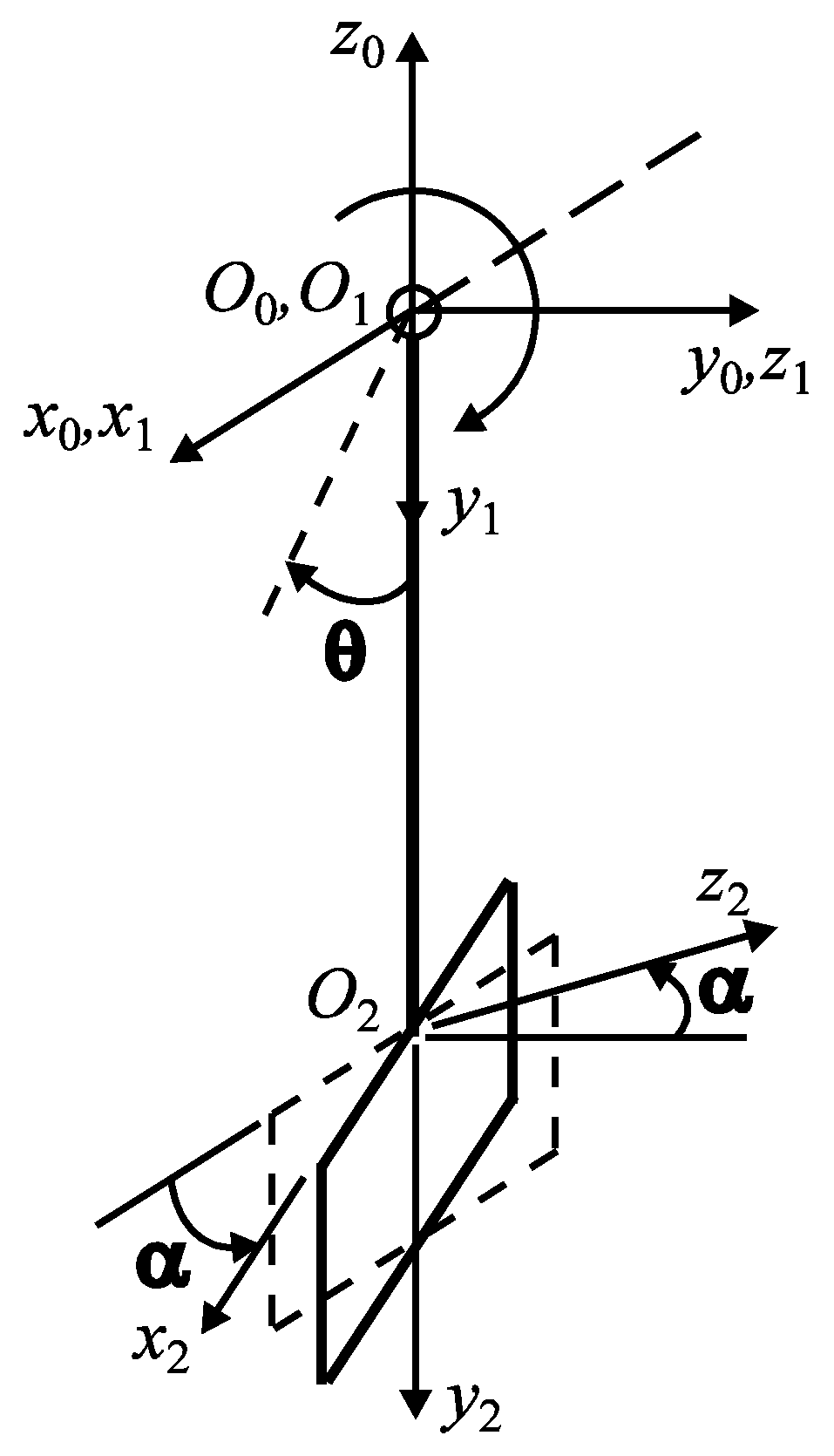
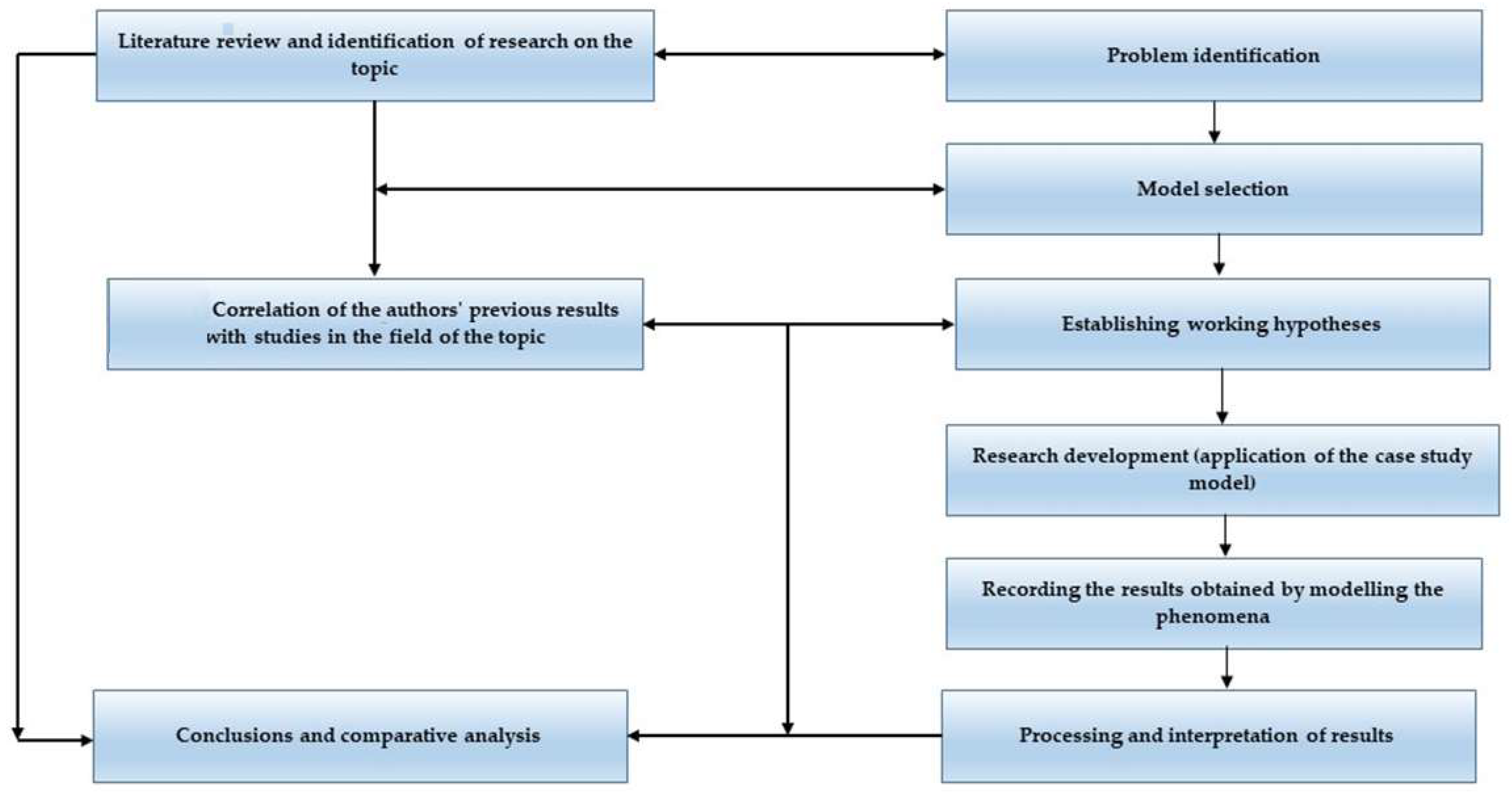
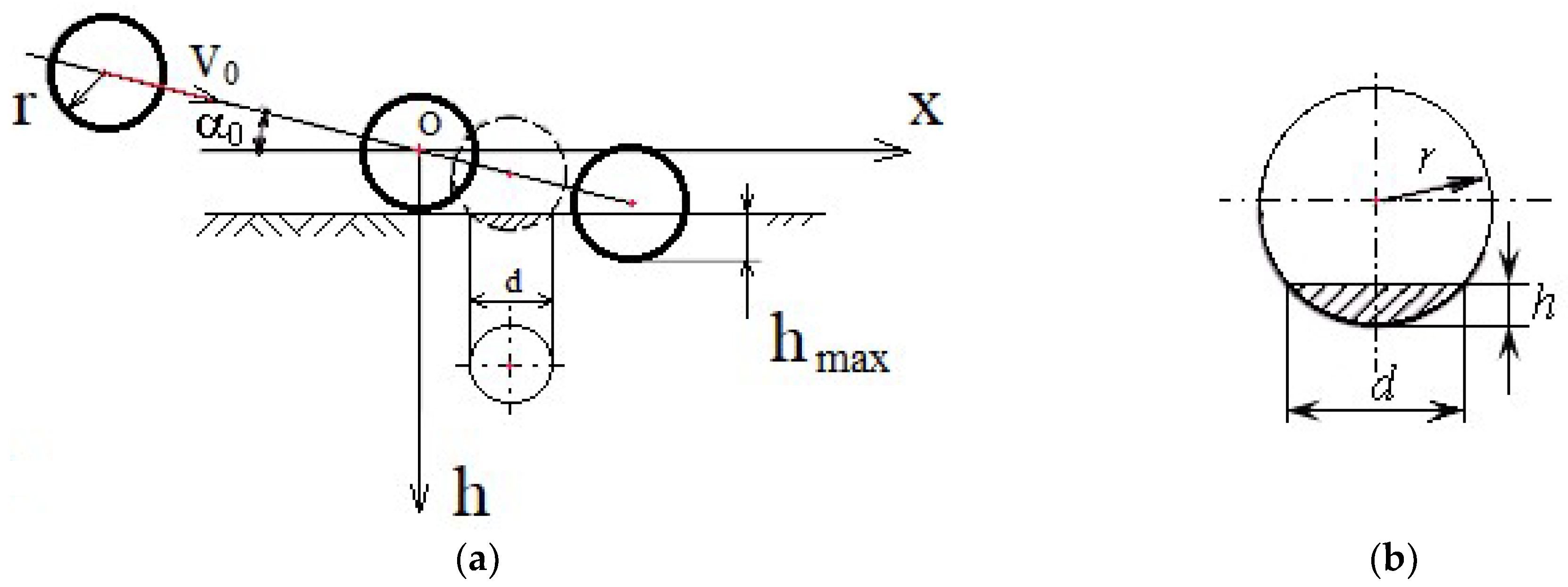
2. Materials and Methods
- -
- The material from which the mixing blades are made is cast iron alloyed with chrome;
- -
- The angle of incidence of the abrasive particles (mineral aggregate) is given by the value of the angle of attack of the mixing blades, with the values: 30°, 45°, and 60°;
- -
- -
- The specific speed of the aggregate particle has the value of 2.5 m/s, in correspondence with the real mixer (the double-shaft mixer studied, located in the concrete station of the company Strabenbau Logistic SRL, Blejoi, Prahova, Romania, was a twin-shaft type, with a capacity of 2 m3, produced by CM Srl., Conselice, Italy/SICOMA, Ponte Valleceppi, Italy) [26], for which the speed of the experimental stand [8] was adopted, with the study being carried out under accelerated conditions.
- The tangential component of the particle velocity cancels with increasing surface deformation ()—case H1;
- The tangential component of the particle velocity cancels as the surface deformation does ()—case H2;
- The tangential component of the particle velocity does not cancel in the process of surface deformation ()—case H3.
- -
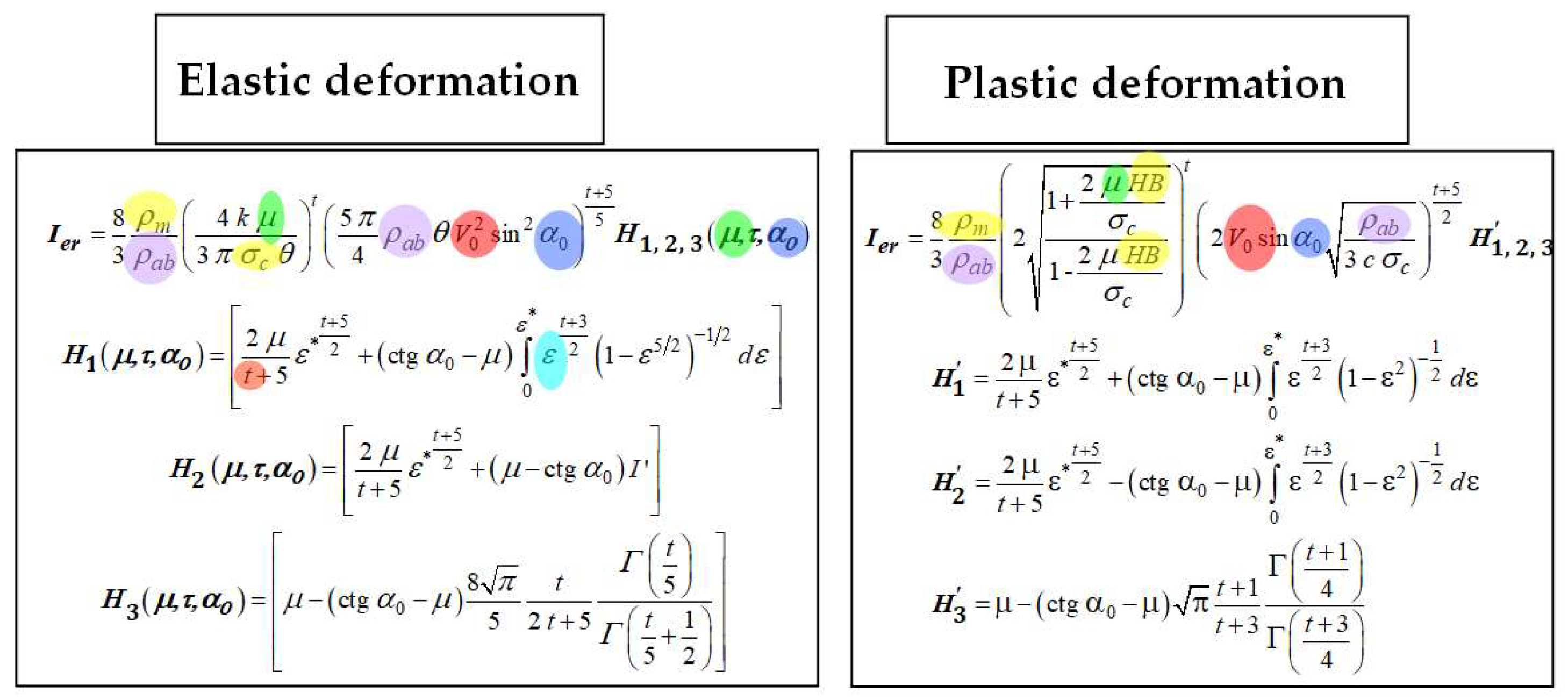
- for elastic deformation:
- -
- for plastic deformation:
- —Variation as a function of the angle of incidence, for the velocity v0 = 2.5 m/s, for three considered values of the friction coefficient (µ: 0.25, 0.3, and 0.35);
- —Variation as a function of impact velocity, for µ = 0.25, for three values of the angle of incidence (α0: 30°, 45°, and 60°);
- —Variation as a function of the friction coefficient, for the velocity v0 = 2.5 m/s, for three values of the angle of incidence (α0: 30°, 45°, and 60°).
3. Results and Discussion
- (a)
- Depending on the angle of impact, three values of the coefficient of friction (Figure 5):
- ⮚
- Elastic deformation:
- The maximum value of the erosion intensity occurs at an angle of approx. 30–35°, and its value decreases as the friction coefficient increases;
- Between the angle values of 50 and 65°, the wear intensity becomes zero, and the values depend on the friction coefficient (the higher the friction coefficient, the lower the angle value at which the intensity is zero);
- Between angle 50° and 90° a maximum wear intensity value of 70–75° is repeated, but much lower than the wear intensity at 30°;
- ⮚
- Plastic deformation:
- The maximum value of erosion intensity occurs at an angle of approximately 15°;
- Between the angle values of 55 and 65°, the wear intensity becomes zero;
- Between the angle values of 55° and 90°, a maximum wear intensity value of 70° is repeated, but much lower than the wear intensity at 30°;
- (b)
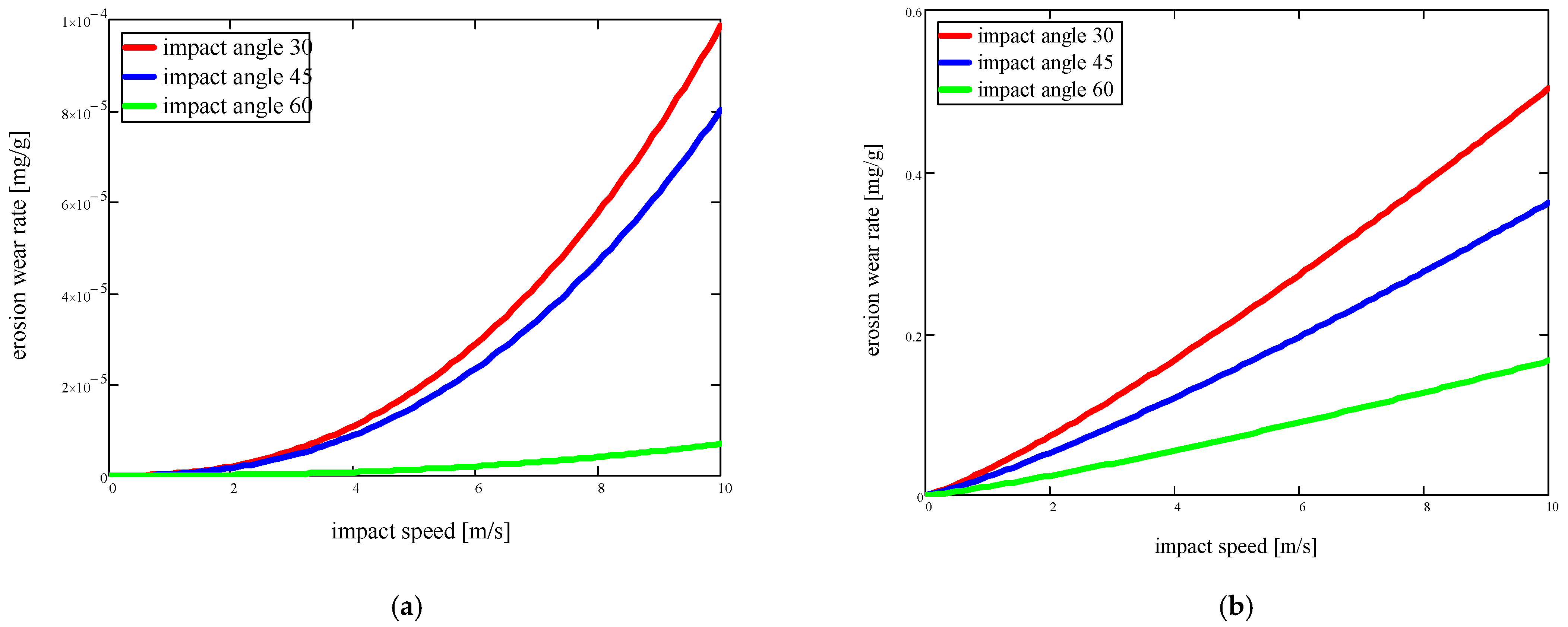
- Depending on the impact speed, three impact angle values (Figure 6):
- ⮚
- Elastic deformation:
- The intensity of wear is almost constant at 60°;
- For lower impact-angle values (30° and 45°), the wear intensity increases exponentially with the change in speed, the slope being differentiated and influenced by the size of the angle, and the maximum value being at 45°;
- ⮚
- Plastic deformation:
- The wear intensity increases linearly with increasing speed; the slope is differentiated by the angle size, and the larger the angle value, the smaller the influence of speed on the wear intensity;
- (c)
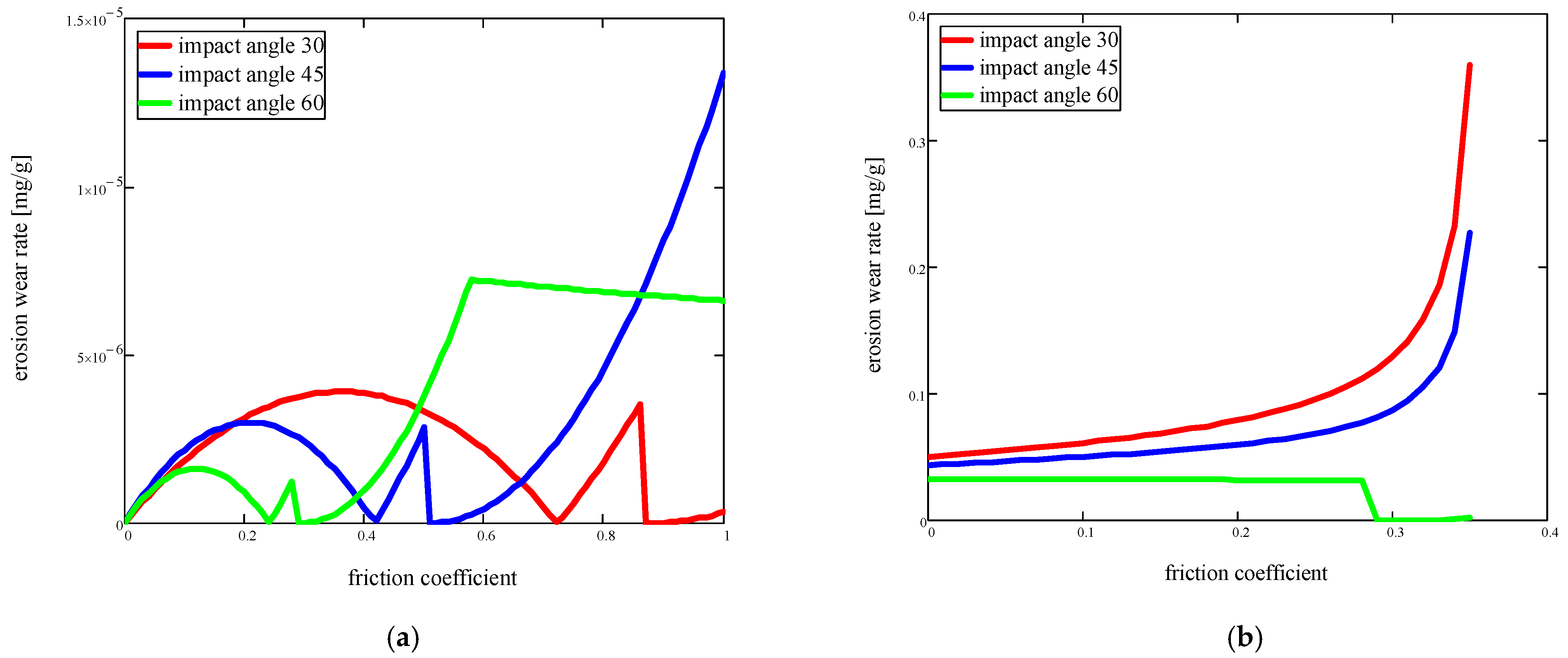
- Depending on the friction coefficient, three values of the impact angle (Figure 7):
- ⮚
- Elastic deformation:
- For a 30° angle: the wear intensity has a parabolic variation according to the value of the friction coefficient, for two areas, 0–0.7 and 0.7–0.9, with the maximum value for wear intensity in the middle of the intervals and the minimum value for a friction coefficient of 0.7 and 0.85; above 0.85, the variation becomes exponential;
- For a 45° angle: the wear intensity has a parabolic variation according to the value of the friction coefficient, for two ranges, 0–0.4 and 0.4–0.5, with the maximum value for wear intensity at the halfway point of the ranges and the minimum value for a friction coefficient of 0.4 and 0.5; above 0.5, the variation becomes exponential.
- For a 60° angle: the wear intensity has a parabolic variation depending on the value of the friction coefficient, for two ranges, 0–0.25 and 0.25–0.35, with the maximum value at the halfway point of the range and the minimum value of the wear intensity for a friction coefficient of 0.2 and 0.3; above 0.3, the variation becomes exponential up to the value friction coefficient 0.55; above this, the variation begins linearly decreasing depending on the value of the friction coefficient.
- ⮚
- Plastic deformation:
- The wear intensity increases linearly with the increasing friction coefficient up to the value of 0.3; above this value, the increase becomes exponential for 30° and 45° and decreases for 60°; the slope is differentiated by the angle size, and the smaller the angle value, the greater the influence of the friction coefficient;
- The maximum values of wear intensity are recorded for the 0.35 friction coefficient value; the smaller the angle, the higher the value for the erosion intensity.
4. Conclusions
- The wear intensity decreases with the increase in the angle of attack of the mixing blade; the lowest values of wear intensity are noted at the 60°angle of attack;
- The wear intensity increases linearly with increasing speed; the slope is differentiated by the size of the angle, and the larger the angle value, the smaller the influence of speed on the wear intensity;
- The wear intensity increases with an increasing coefficient of friction; the slope is differentiated by the size of the angle, and the smaller the angle value, the greater the influence of the friction coefficient.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Anatoly Fedorovich, T.; Drozdov, A. Automatic Control of the Concrete Mixture Homogeneity in Cycling Mixers. IOP Conf. Ser. Mater. Sci. Eng. 2018, 317, 012043. [Google Scholar] [CrossRef]
- Zhao, W.; Yang, J.; Zhao, W.; Yang, C. Experimental Study on the Influence of Mixing Time on Concrete Performance under Different Mixing Modes. Sci. Eng. Compos. Mater. 2021, 28, 638–651. [Google Scholar] [CrossRef]
- Stel’makh, S.A.; Shcherban’, E.M.; Shuiskii, A.I.; Prokopov, A.Y.; Madatyan, S.M.; Parinov, I.A.; Cherpakov, A.V. Effects of the Geometric Parameters of Mixer on the Mixing Process of Foam Concrete Mixture and Its Energy Efficiency. Appl. Sci. 2020, 10, 8055. [Google Scholar] [CrossRef]
- Cazacliu, B. In-Mixer Measurements for Describing Mixture Evolution during Concrete Mixing. Chem. Eng. Res. Des. 2008, 86, 1423–1433. [Google Scholar] [CrossRef]
- Ferraris, C.F. Concrete Mixing Methods and Concrete Mixers: State of the Art. J. Res. Natl. Inst. Stand. Technol. 2001, 106, 391. [Google Scholar] [CrossRef] [PubMed]
- Valigi, M.C.; Logozzo, S.; Landi, L.; Braccesi, C.; Galletti, L. Twin-Shaft Mixers’ Mechanical Behavior Numerical Simulations of the Mix and Phases. Machines 2019, 7, 39. [Google Scholar] [CrossRef]
- Labiapari, W.S.; Gonçalves, R.J.; De Alcântara, C.M.; Pagani, V.; Di Cunto, J.C.; De Mello, J.D.B. Understanding Abrasion-Corrosion to Improve Concrete Mixer Drum Performance: A Laboratory and Field Approach. Wear 2021, 477, 203830. [Google Scholar] [CrossRef]
- Niță, A.; Laudacescu, E.; Petrescu, M.G.; Dumitru, T.; Burlacu, A.; Bădoiu, D.G.; Tănase, M. Experimental Research Regarding the Effect of Mineral Aggregates on the Wear of Mixing Blades of Concrete Mixers. Materials 2023, 16, 5047. [Google Scholar] [CrossRef] [PubMed]
- Niță, A.; Petrescu, M.G.; Dumitru, T.; Burlacu, A.; Tănase, M.; Laudacescu, E.; Ramadan, I. Experimental Research on the Wear Behavior of Materials Used in the Manufacture of Components for Cement Concrete Mixers. Materials 2023, 16, 2326. [Google Scholar] [CrossRef]
- Fasano, J.; Janz, E.E.; Myers, K. Design Mixers to Minimize Effects of Erosion and Corrosion Erosion. Int. J. Chem. Eng. 2012, 2012, 171838. [Google Scholar] [CrossRef][Green Version]
- Sapate, S.G.; RamaRao#, A.V. Effect of Erodent Particle Hardness on Velocity Exponent in Erosion of Steels and Cast Irons. Mater. Manuf. Process. 2003, 18, 783–802. [Google Scholar] [CrossRef]
- Stack, M.M.; Stott, F.H.; Wood, G.C. The Significance of Velocity Exponents in Identifying Erosion-Corrosion Mechanisms. J. Phys. IV Fr. 1993, 3, C9-687–C9-694. [Google Scholar] [CrossRef][Green Version]
- Khalid, Y.A.; Sapuan, S.M. Wear Analysis of Centrifugal Slurry Pump Impellers. Ind. Lubr. Tribol. 2007, 59, 18–28. [Google Scholar] [CrossRef]
- Niță, A. Contributions to Increase the Wear Resistance of Mixers in Concrete Production Plants; Scientific Research Report No. 3; Petroleum Gas University of Ploiesti: Ploiesti, Romania, 2023. [Google Scholar]
- Niță, A. Contributions to the Study of Specific Wear Phenomena of Mixers in Concrete Production Plants; Scientific Research Report No. 2; Petroleum Gas University of Ploiesti: Ploiesti, Romania, 2023. [Google Scholar]
- Zhang, H.; Feng, P.; Ying, W. Abrasive Wear and Optimal Installation Angle of Concrete Double-Horizontal Shaft Mixer Stirring Blades. SN Appl. Sci. 2020, 2, 1067. [Google Scholar] [CrossRef]
- Arabnejad, H.; Mansouri, A.; Shirazi, S.A.; McLaury, B.S. Development of Mechanistic Erosion Equation for Solid Particles. Wear 2015, 332–333, 1044–1050. [Google Scholar] [CrossRef]
- Mansouri, A.; Arabnejad, H.; Shirazi, S.A.; McLaury, B.S. A Combined CFD/Experimental Methodology for Erosion Prediction. Wear 2015, 332–333, 1090–1097. [Google Scholar] [CrossRef]
- Ashrafizadeh, H.; Ashrafizadeh, F. A Numerical 3D Simulation for Prediction of Wear Caused by Solid Particle Impact. Wear 2012, 276–277, 75–84. [Google Scholar] [CrossRef]
- Hadavi, V.; Moreno, C.E.; Papini, M. Numerical and Experimental Analysis of Particle Fracture during Solid Particle Erosion, Part II: Effect of Incident Angle, Velocity and Abrasive Size. Wear 2016, 356–357, 146–157. [Google Scholar] [CrossRef]
- Zhu, H.; Lin, Y.; Zeng, D.; Zhou, Y.; Xie, J.; Wu, Y. Numerical Analysis of Flow Erosion on Drill Pipe in Gas Drilling. Eng. Fail. Anal. 2012, 22, 83–91. [Google Scholar] [CrossRef]
- Zhu, X.; Liu, S.; Tong, H.; Huang, X.; Li, J. Experimental and Numerical Study of Drill Pipe Erosion Wear in Gas Drilling. Eng. Fail. Anal. 2012, 26, 370–380. [Google Scholar] [CrossRef]
- Peng, Z.; Zhang, X.; Zhang, Y.; Liu, L.; Xu, G.; Wang, G.; Zhao, M. Wear Resistance Enhancement of Inconel 718 via High-Speed Ultrasonic Vibration Cutting and Associated Surface Integrity Evaluation under High-Pressure Coolant Supply. Wear 2023, 530–531, 205027. [Google Scholar] [CrossRef]
- Peng, Z.; Zhang, X.; Liu, L.; Xu, G.; Wang, G.; Zhao, M. Effect of High-Speed Ultrasonic Vibration Cutting on the Microstructure, Surface Integrity, and Wear Behavior of Titanium Alloy. J. Mater. Res. Technol. 2023, 24, 3870–3888. [Google Scholar] [CrossRef]
- Meng, H.C.; Ludema, K.C. Wear Models and Predictive Equations: Their Form and Content. Wear 1995, 181–183, 443–457. [Google Scholar] [CrossRef]
- Hassan, S.; Khan, S.A.; Naveed, R.; Saleem, M.Q.; Mufti, N.A.; Farooq, M.U. Investigation on Tool Wear Mechanisms and Machining Tribology of Hardened DC53 Steel through Modified CBN Tooling Geometry in Hard Turning. Int. J. Adv. Manuf. Technol. 2023, 127, 547–564. [Google Scholar] [CrossRef]
- Farooq, M.U.; Anwar, S.; Bhatti, H.A.; Kumar, M.S.; Ali, M.A.; Ammarullah, M.I. Electric Discharge Machining of Ti6Al4V ELI in Biomedical Industry: Parametric Analysis of Surface Functionalization and Tribological Characterization. Materials 2023, 16, 4458. [Google Scholar] [CrossRef]
- Niţă, A.; Laudacescu, E.; Ramadan, I.N.; Petrescu, M.G. An Example for Determining the Physical Parameters Used in DEM Modelling for the Interaction Process between Aggregates and Working Equipment. IOP Conf. Ser. Mater. Sci. Eng. 2022, 1262, 012028. [Google Scholar] [CrossRef]
- Parsi, M.; Najmi, K.; Najafifard, F.; Hassani, S.; McLaury, B.S.; Shirazi, S.A. A Comprehensive Review of Solid Particle Erosion Modeling for Oil and Gas Wells and Pipelines Applications. J. Nat. Gas Sci. Eng. 2014, 21, 850–873. [Google Scholar] [CrossRef]
- Tudor, A.; Vlase, M. Uzarea Materialelor (Materials Wear); Editure Bren: Bucharest, Romania, 2010. [Google Scholar]
- Vlase, M.; Tudor, A. Pumping and Transport of Concrete through Pipes; Editure Bren: Bucharest, Romania, 2009. [Google Scholar]

| Symbol | Meaning of Terms |
|---|---|
| the particle–target-surface friction coefficient | |
| angle of incidence | |
| particle velocity | |
| particle radius (spherical particle) | |
| HB | the hardness of the target surface |
| the material density of the target surface | |
| the density of the particle material | |
| the elasticity parameter of the surface material | |
| the tensile strength of the target surface material | |
| the flow resistance of the surface material | |
| e0 | the relative deformation at break through stretching of the surface material |
| k | a constant depending on the assumed breaking assumption |
| n | the number of cycles at which the wear particle occurs—ne—elastic and np—plastic |
| the volume of material deformed by the abrasive particle | |
| the volume of material deformed by a particle | |
| a | the diameter of the contact surface |
| the mass of the used material | |
| the mass of the particles that caused the wear | |
| V | the volume of used material |
| the number of abrasive particles hitting the surface | |
| h | deformation—the distance from the original surface to the deformation trace |
| note | |
| t | friction fatigue parameter [30] |
| Elastic Deformation | Plastic Deformation |
|---|---|
| Em—modulus of elasticity of the surface material—1.85 × 1011 N/m2; vp—Poisson coefficient—0.27 | HB—target surface hardness—490 × 106 N/m2 |
| —angle of incidence—0° ÷ 90°; with three specific values: 30°, 45° and 60° —particle velocity—0.1 ÷ 10 m/s; with a specific velocity of 2.5 m/s —coefficient of friction between particle and material—0.25 ÷ 0.35 —material density of the target surface (cast iron)—7600 kg/m3; —density of particle material (crushed mineral aggregate)—2700 kg/m3; σc—flow resistance of pallet material—350 × 106 N/m2; k—constant depending on the assumed breaking assumption—3; t—friction fatigue parameter—1. | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petrescu, M.G.; Popovici, A.-I.; Niță, A.; Isbășoiu, D.; Dumitru, T.; Tănase, M. Modelling Wear Phenomena Specific to Mixer Blades in Concrete Production Plants. Appl. Sci. 2024, 14, 3988. https://doi.org/10.3390/app14103988
Petrescu MG, Popovici A-I, Niță A, Isbășoiu D, Dumitru T, Tănase M. Modelling Wear Phenomena Specific to Mixer Blades in Concrete Production Plants. Applied Sciences. 2024; 14(10):3988. https://doi.org/10.3390/app14103988
Chicago/Turabian StylePetrescu, Marius Gabriel, Aristia-Ioana Popovici, Adrian Niță, Dan Isbășoiu, Teodor Dumitru, and Maria Tănase. 2024. "Modelling Wear Phenomena Specific to Mixer Blades in Concrete Production Plants" Applied Sciences 14, no. 10: 3988. https://doi.org/10.3390/app14103988
APA StylePetrescu, M. G., Popovici, A.-I., Niță, A., Isbășoiu, D., Dumitru, T., & Tănase, M. (2024). Modelling Wear Phenomena Specific to Mixer Blades in Concrete Production Plants. Applied Sciences, 14(10), 3988. https://doi.org/10.3390/app14103988





