A Time-Scale Varying Finite Difference Method for Analyzing the Influence of Rainfall and Water Level on the Stability of a Bank Slope
Abstract
1. Introduction
2. Method
2.1. Shear Strength Theory of Unsaturated Soil
2.2. Principle of TSFDM
2.3. Strength Reduction Method
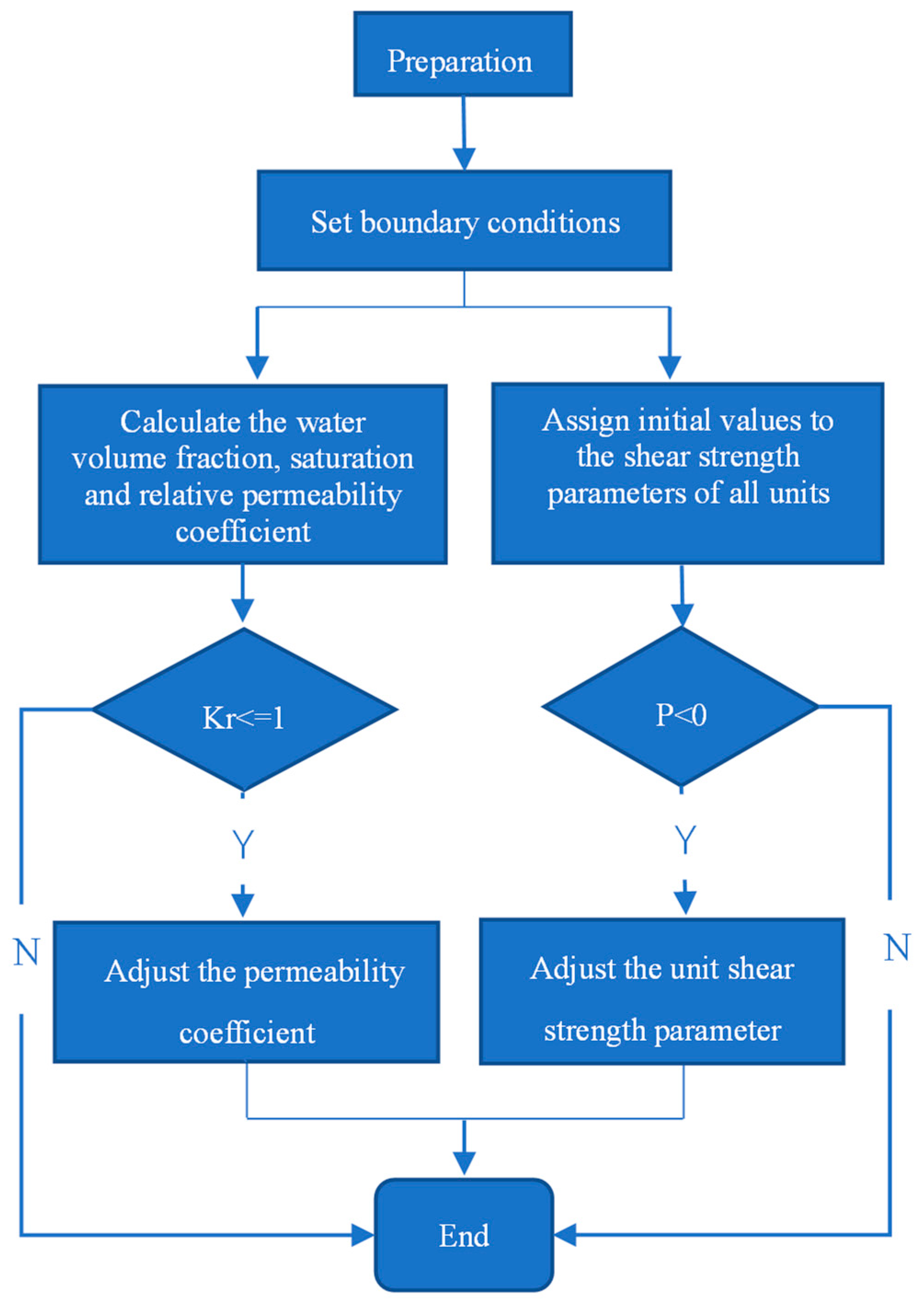
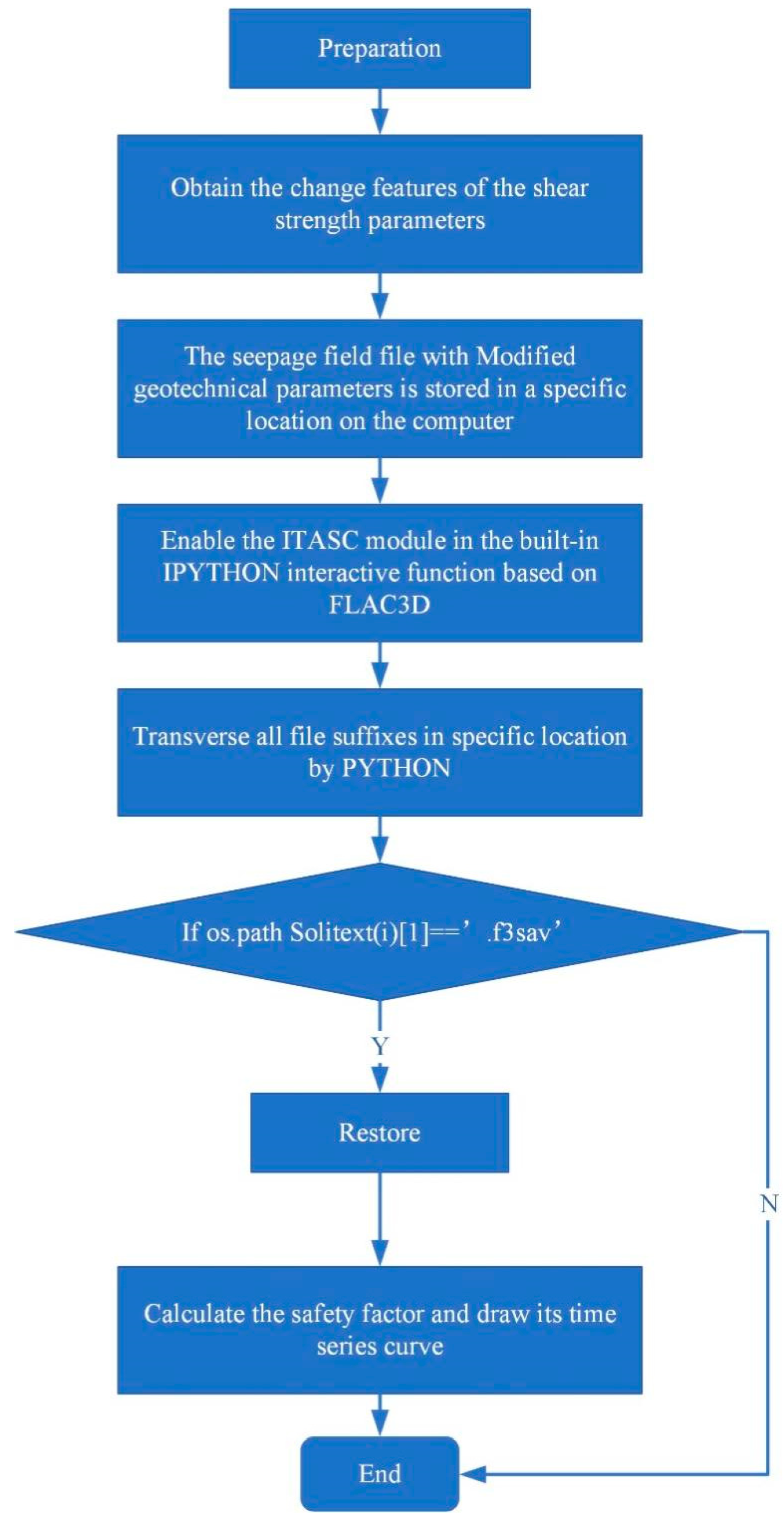
2.4. Improvement of FISH Function
2.5. Development of the FISH Program
2.6. Establish a Finite Difference Model
3. Results
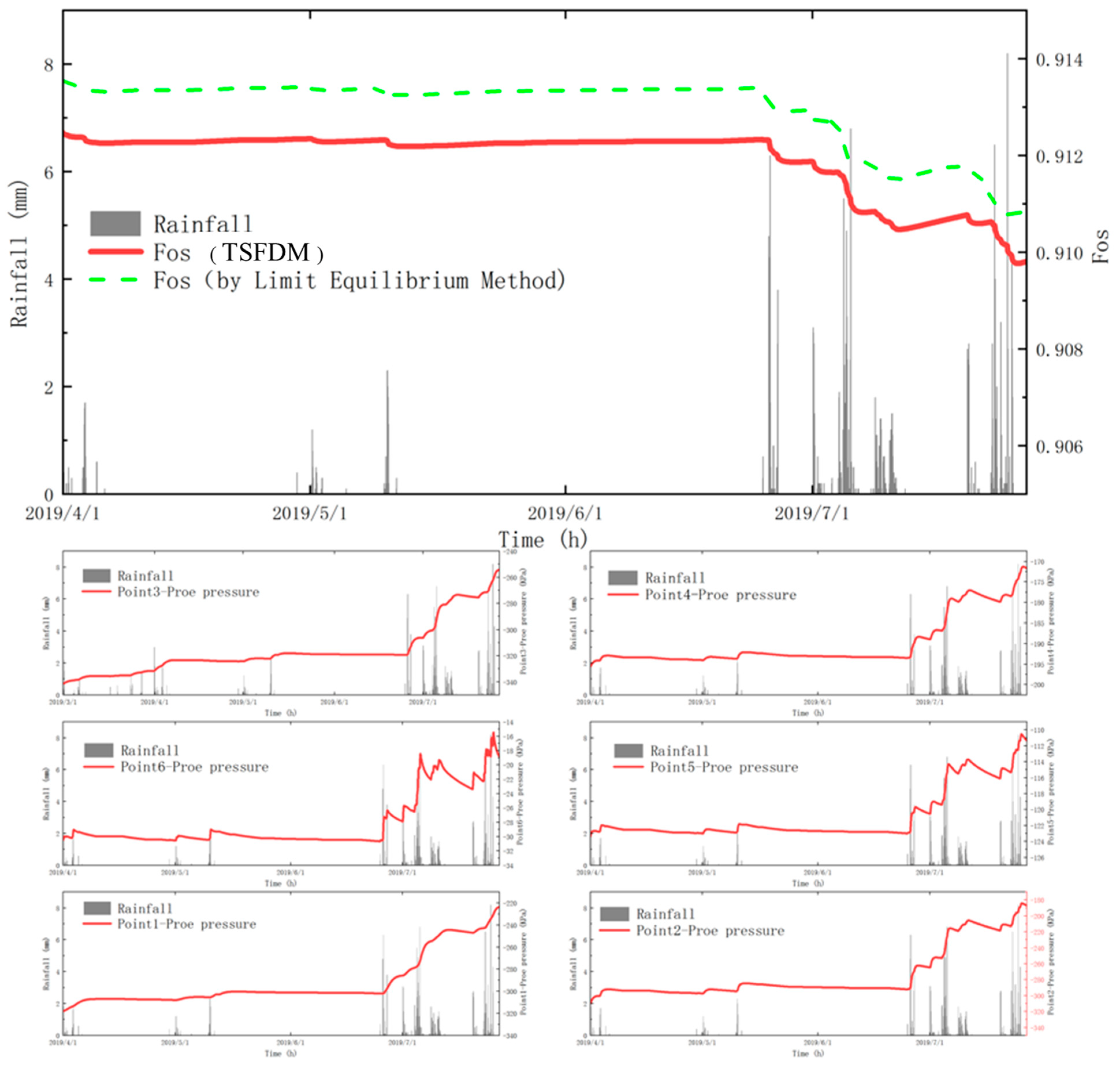
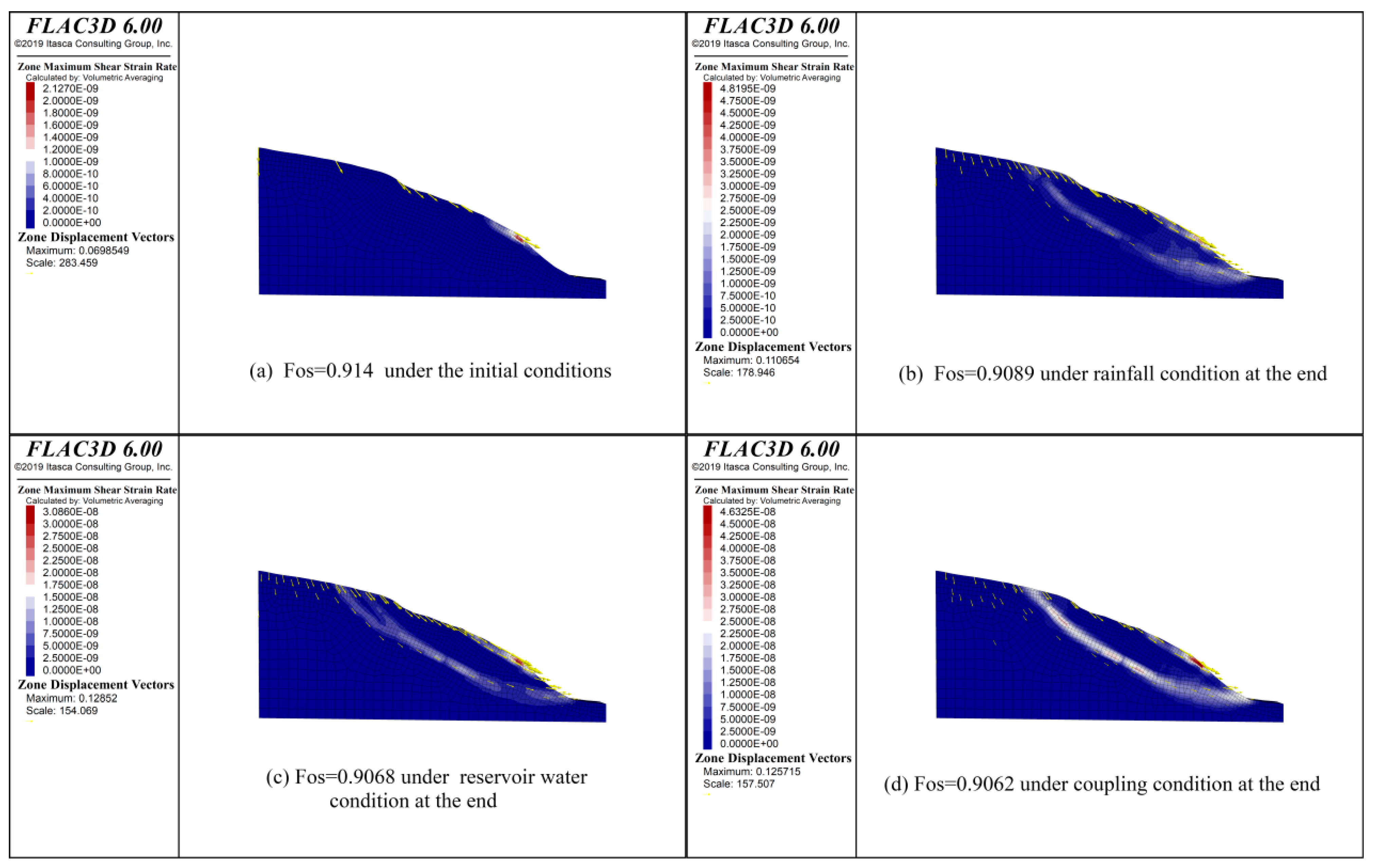
3.1. Temporal Changes of the Seepage in the Saturated and Unsaturated Slopes
3.2. Impacts of Real Rainfall and Pond Water Level Changes on Slope Stability
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, G.Q.; Huang, R.Q.; Shi, Y.C.; Xu, Q. Stability Analysis of Slope Based on Dynamic and Whole Strength Reduction Methods. Chin. J. Rock Mech. Eng. 2014, 33, 243–256. [Google Scholar]
- Cao, Z.Y. Construction mechanics and time-varying mechanics in civil engineering. China Civ. Eng. J. 2001, 34, 41–46. [Google Scholar]
- Zhang, D.; Jian, W.B.; Ye, Q.; Lin, W. A time-varying analytic model of tailings slope and its application. Rock Soil Mech. 2014, 35, 835–840. [Google Scholar]
- Jiang, Z.M.; Xiong, X.H.; Zeng, L. Unsaturated seepage analysis of slope under rainfall condition based on FLAC3D. Rock Soil Mech. 2014, 35, 855–861. [Google Scholar]
- Chu, W.J.; Xu, W.Y.; Yang, S.Q.; Zhou, W.Y. Secondary development of a viscoelasto-plastic rheological constitutive model of rock based on FLAC3D. Rock Soil Mech. 2006, 27, 2005–2010. [Google Scholar]
- He, Z.; Dai, B. Secondary Development of a Nonlinear Creep Model Based on Fractional Derivative in FLAC3D. In Proceedings of the 2018 11th International Conference on Intelligent Computation Technology and Automation (ICICTA), Changsha, China, 22–23 September 2018. [Google Scholar]
- Wang, C.B.; Ding, W.Q.; Qiao, Y.F. Development and application of hardening soil constitutive model in FLAC(3D). Chin. J. Rock Mech. Eng. 2014, 33, 199–208. [Google Scholar]
- Fredlund, D.G.; Rahardjo, H. Soil Mechanics for Unsaturated Soils; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1993. [Google Scholar]
- Ou, X.P.; Bai, K.; Zhu, Y.S.; Yuan, C.; Wang, J.; Liu, D.T.; Liu, H.J. The Strength Reduction Method for Stability Analysis of Slope Based on FLAC-3D. J. Wuhan Univ. Technol. 2009, 31, 59–61. [Google Scholar]
- Song, Y.S.; Chae, B.G.; Lee, J. A method for evaluating the stability of an unsaturated slope in natural terrain during rainfall. Eng. Geol. 2016, 210, 84–92. [Google Scholar] [CrossRef]
- Genuchten, M.V. A Closed-form Equation for Predicting Hydraulic Conductivity for Unsaturated Soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Chen, X.; Fu, J.J.; Zhao, H.B.; Huang, T.P.; Shi, K. Analysis of Slope Reliability by Finite-Difference Strength-Reduction Method Considering the Thought of Monte Carlo Method. J. Yangtze River Sci. Res. Inst. 2011, 28, 36–40. [Google Scholar]
- Jiang, X.L.; Yang, H.; Cao, P. FLAC(3D) analysis of interaction between goaf and opencut mining slope with 3D geological model using SURPAC. Rock Soil Mech. 2011, 32, 1234–1240. [Google Scholar]
- Liu, C.H.; Chen, C.X.; Feng, X.T.; Xiao, G.F. Effect of groundwater on stability of slopes at reservoir bank. Rock Soil Mech. 2005, 26, 419–422. [Google Scholar]
- Liu, J.X.; Liu, Y.T.; Hu, Q.J. Stability of embankment slope subjected to rainfall infiltration considering both runoff-underground seepage and fluid-solid coupling. Rock Soil Mech. 2010, 31, 903–910. [Google Scholar]
- Koner, R.; Chakravarty, D. Numerical analysis of rainfall effects in external overburden dump. Int. J. Min. Sci. Technol. 2016, 26, 825–831. [Google Scholar] [CrossRef]
- Mousavi, S.M. Landslide Susceptibility in Cemented Volcanic Soils, Ask Region, Iran. Indian Geotech. J. 2016, 47, 115–130. [Google Scholar] [CrossRef]
- Nguyen, T.S.; Borgesson, L.; Chijimatsu, M.; Rutqvist, J.; Fujita, T.; Hernelind, J.; Kobayashi, A.; Ohnishi, Y.; Tanaka, M.; Jing, L. Hydro-mechanical response of a fractured granitic rock mass to excavation of a test pit—The Kamaishi Mine experiment in Japan. Int. J. Rock Mech. Min. Sci. 2001, 38, 79–94. [Google Scholar] [CrossRef]
- Wang, J.X.; Li, Z.; Chen, W. Lower bound analysis of soil slope stability using finite elements subjected to pore water pressure. Rock Soil Mech. 2005, 16, 1258–1262+1268. [Google Scholar]
- Wang, L.F.; Li, L.G.; Yang, X. Instability initiation mechanism of gravel soil slope in Three Gorges Reservoir: Case study of Hongyanzi landslide in Wushan county. Chin. J. Geot. Eng. 2018, 40, 209–214. [Google Scholar]
- Wang, M.H.; Yan, E.C. Study on influence of reservoir water impounding on reservoir landslide. Rock Soil Mech. 2007, 28, 2722–2725. [Google Scholar]
- Zhang, W.; Goh, A.T. Reliability assessment on ultimate and serviceability limit states and determination of critical factor of safety for underground rock caverns. Tunn. Undergr. Space Technol. 2012, 32, 221–230. [Google Scholar] [CrossRef]





| Strata | Lithology | Status | Elastic Modulus (MPa) | Poisson’s Ratio | Unit Weight (KN/m3) | Cohesion (KPa) | Internal Friction Angle (°) |
|---|---|---|---|---|---|---|---|
| I | GS | Natural | 261.6 | 0.4 | 20.5 | 15 | 32 |
| Saturated | 241.6 | 0.41 | 21 | 10 | 30 | ||
| II | SWCS | Natural | 2644.9 | 0.38 | 22.4 | 93.6 | 33.3 |
| Saturated | 975.8 | 0.40 | 23.5 | 77.4 | 28.9 | ||
| III | WCS | Saturated | 3786.1 | 0.36 | 27 | 100 | 30 |
| Natural | 5561 | 0.35 | 26.5 | 120.0 | 35.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, G.; Lu, G.; Xia, C.; Bai, D.; Liu, T. A Time-Scale Varying Finite Difference Method for Analyzing the Influence of Rainfall and Water Level on the Stability of a Bank Slope. Appl. Sci. 2023, 13, 5268. https://doi.org/10.3390/app13095268
Zhang G, Lu G, Xia C, Bai D, Liu T. A Time-Scale Varying Finite Difference Method for Analyzing the Influence of Rainfall and Water Level on the Stability of a Bank Slope. Applied Sciences. 2023; 13(9):5268. https://doi.org/10.3390/app13095268
Chicago/Turabian StyleZhang, Guorong, Guangyin Lu, Chengzhi Xia, Dongxin Bai, and Taoying Liu. 2023. "A Time-Scale Varying Finite Difference Method for Analyzing the Influence of Rainfall and Water Level on the Stability of a Bank Slope" Applied Sciences 13, no. 9: 5268. https://doi.org/10.3390/app13095268
APA StyleZhang, G., Lu, G., Xia, C., Bai, D., & Liu, T. (2023). A Time-Scale Varying Finite Difference Method for Analyzing the Influence of Rainfall and Water Level on the Stability of a Bank Slope. Applied Sciences, 13(9), 5268. https://doi.org/10.3390/app13095268





