Model Updating for a Continuous Concrete Girder Bridge Using Data from Construction Monitoring
Abstract
1. Introduction
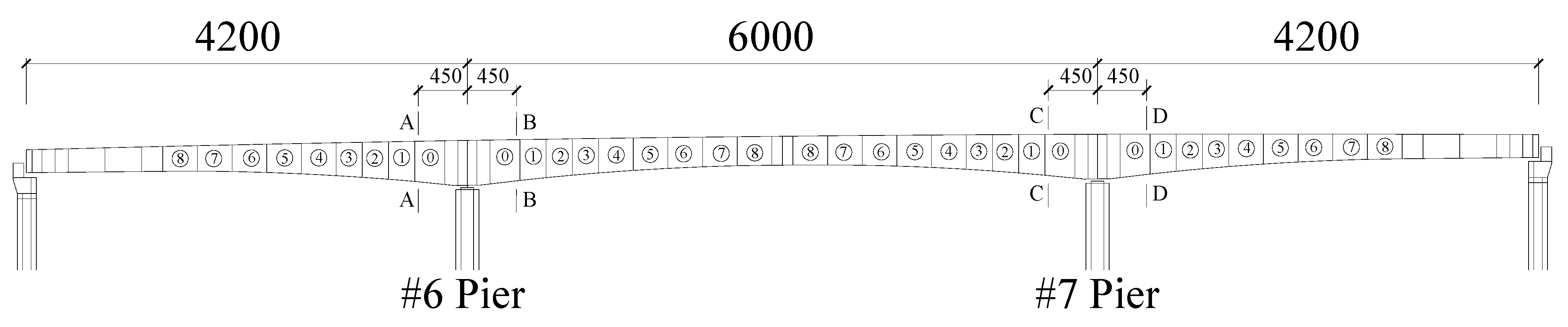
2. Engineering Background: Huangsha Harbor Bridge
3. Stress Measurements and Data Updating Based on Grey Theory
3.1. On-Site Stress Measurements for Huangsha Harbor Bridge under Construction
3.2. Compensation for the Deviation in the Measured Stress Due to the Uneven Temperature
3.3. Data Updating
3.3.1. Theoretical Background of Grey Theory
3.3.2. Data Updating Based on GM (1,1) Models
4. Numerical Simulation Based on MIDAS FE Platform
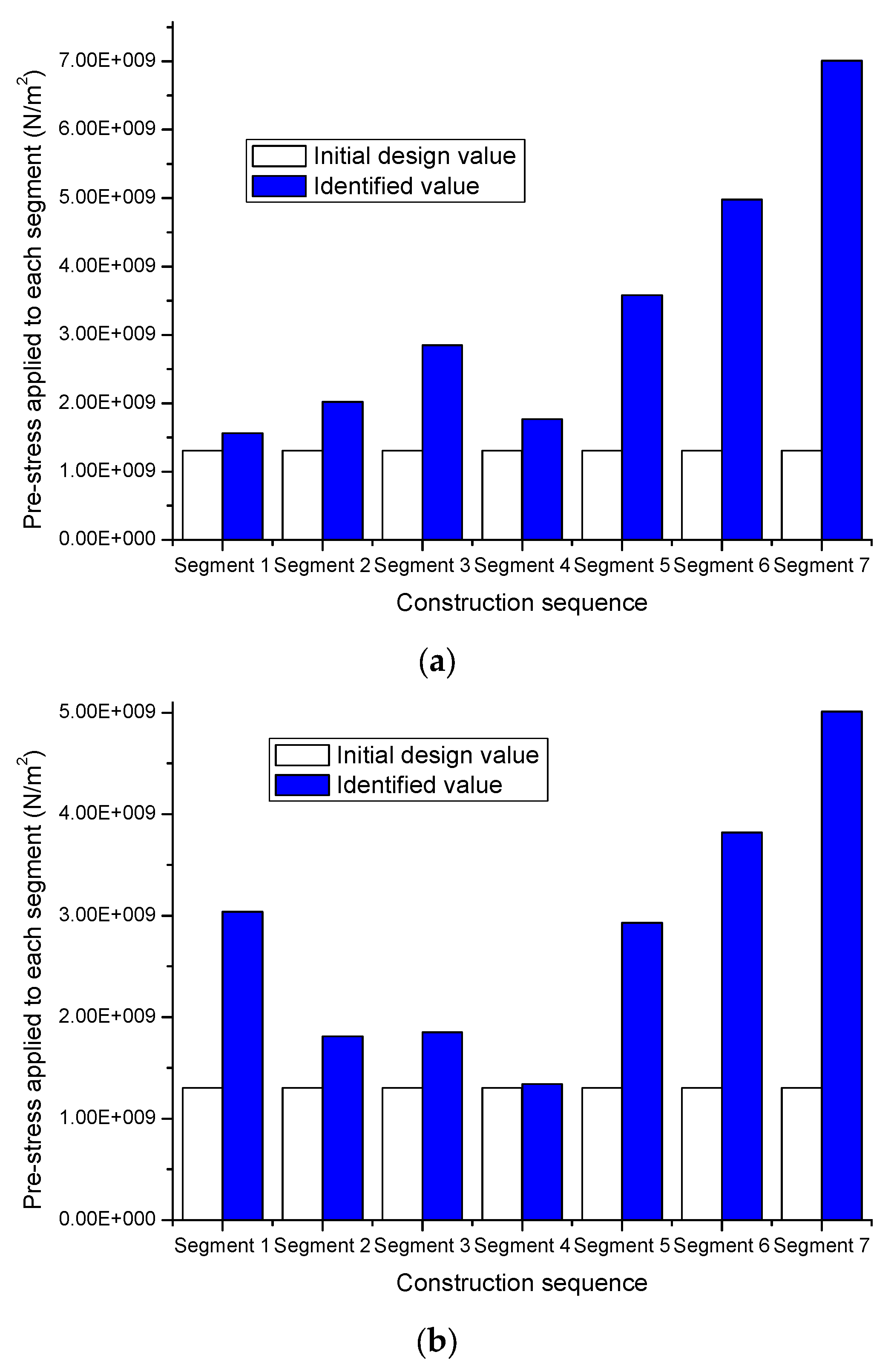
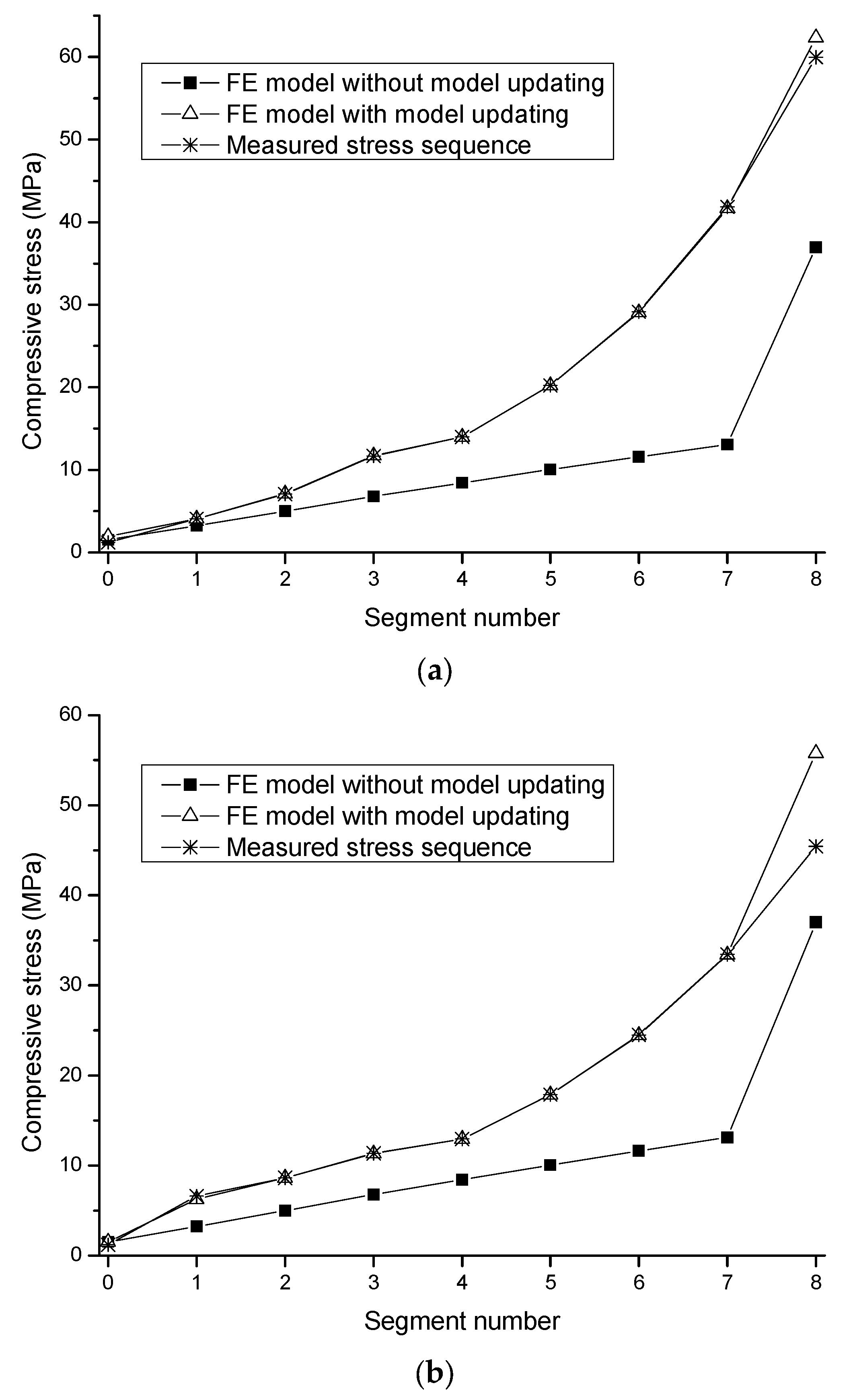
5. Model Updating Using Data from Construction Monitoring
6. Comparisons between Static Responses of the Numerical Models and Full-Scale Measurement Data
7. Conclusions
- (1)
- The abundant data measured on bridges in construction stages can enhance the reference information for the more effective FE model updating of the structures. In addition, with the proposed model updating method utilizing data from the construction monitoring, the uncertain parameters with the initial FE model of the structure can be progressively and easily identified using many single-variable linear regression models, which are superior to one complicated multi-variable mathematical or numerical model employed by most traditional model updating approaches to simultaneously identify many uncertain parameters, as the latter generally leads to non-unique solutions rendered by normal optimization algorithms.
- (2)
- Based on the engineering case of Huangsha Harbor Bridge, it is demonstrated that the proposed model updating approach is effective in practical use, and it is superior to the traditional model updating method using structural responses of the built structure. It is calculated that the RMS error of static structural responses is 32.74% for the model of Huangsha Harbor Bridge updated using the new method and 41.07% and 38.48% for the initial model without model updating and the model updated using the traditional method, respectively. The improved accuracy of the model updated using the new method can be considered as an advantage of the proposed method compared to the analogue, i.e., the traditional model updating approach based on orthogonal experimental design and support vector regression (SVR). Since Huangsha Harbor Bridge’s FE model is complicated, the sensitivity-analysis-based model updating approach is inapplicable due to its high computational costs, and the surrogate-model-based model updating approach of high computing efficiency is attempted for the traditional model updating approach. Sampling based on orthogonal experimental design and surrogate model establishment based on SVR are undertaken for the traditional model updating to take place. This model updating approach is indicated as the alternative method as a basis for the comparison.
- (3)
- The deviation in the stress measured on the continuous concrete girder bridge due to the uneven temperature can be compensated for using the approach adopted by the present research. In addition, stresses measured in concrete using vibrating wire stress transducers might be inaccurate when the vibrating wire stress transducers are used over a certain period. The stress arrays measured using the technique on continuous concrete girder bridges under construction using the symmetric cantilever casting method can be updated based on the GM (1,1) models.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Baseline FE Model Updated Using Static Structural Responses of the Built Structure
Appendix A.1. Sampling on the Initial FE Model Based on Seven-Factor Orthogonal Experimental Design
| Run | Pre-Stresses Applied to Segment (N/m2) | ||||||
|---|---|---|---|---|---|---|---|
| Segment 1 | Segment 2 | Segment 3 | Segment 4 | Segment 5 | Segment 6 | Segment 7 | |
| 1 | 1.30 × 109 | 1.30 × 109 | 1.30 × 109 | 1.30 × 109 | 1.30 × 109 | 1.30 × 109 | 1.30 × 109 |
| 2 | 1.30 × 109 | 1.30 × 109 | 1.30 × 109 | 1.96 × 109 | 1.96 × 109 | 1.96 × 109 | 1.96 × 109 |
| 3 | 1.30 × 109 | 1.96 × 109 | 1.96 × 109 | 1.30 × 109 | 1.30 × 109 | 1.96 × 109 | 1.96 × 109 |
| 4 | 1.30 × 109 | 1.96 × 109 | 1.96 × 109 | 1.96 × 109 | 1.96 × 109 | 1.30 × 109 | 1.30 × 109 |
| 5 | 1.96 × 109 | 1.30 × 109 | 1.96 × 109 | 1.30 × 109 | 1.96 × 109 | 1.30 × 109 | 1.96 × 109 |
| 6 | 1.96 × 109 | 1.30 × 109 | 1.96 × 109 | 1.96 × 109 | 1.30 × 109 | 1.96 × 109 | 1.30 × 109 |
| 7 | 1.96 × 109 | 1.96 × 109 | 1.30 × 109 | 1.30 × 109 | 1.96 × 109 | 1.96 × 109 | 1.30 × 109 |
| 8 | 1.96 × 109 | 1.96 × 109 | 1.30 × 109 | 1.96 × 109 | 1.30 × 109 | 1.30 × 109 | 1.96 × 109 |
Appendix A.2. SVR Method
Appendix A.3. FE Model Updating Based on SVR Models
References
- Mufti, A. Structural health monitoring of innovative bridge decks. Struct. Infrastruct. Eng. 2005, 1, 119–133. [Google Scholar] [CrossRef]
- Friswell, M.I.; Mottershead, J.E. Finite Element Model Updating in Structural Dynamics; Kluwer Academic: Boston, MA, USA, 1995. [Google Scholar]
- Zhang, Q.W.; Chang, T.Y.P.; Chang, C.C. Finite element model updating for the Kap Shui Mun cable-stayed bridge. J. Bridge Eng. 2001, 6, 285–293. [Google Scholar] [CrossRef]
- Brownjohn, J.M.W.; Xia, P.Q. Dynamic assessment of curved cable-stayed bridge by model updating. J. Struct. Eng. 2000, 126, 252–260. [Google Scholar] [CrossRef]
- Jaishi, B.; Ren, J.X. Structural finite element model updating using ambient vibration test results. J. Struct. Eng. 2005, 131, 617–628. [Google Scholar] [CrossRef]
- Deng, L.; Cai, C.S. Bridge model updating using response surface method and genetic algorithm. J Bridge Eng. 2010, 15, 553–564. [Google Scholar] [CrossRef]
- Aloisio, A.; Alaggio, R.; Fragiacomo, M. Dynamic identification and model updating of full-scale concrete box girders based on the experimental torsional response. Constr. Build. Mater. 2020, 264, 120146. [Google Scholar] [CrossRef]
- Ren, W.X.; Fang, S.E.; Deng, M.Y. Response surface based finite element model updating using structural static responses. J. Eng. Mech. 2011, 137, 248–257. [Google Scholar] [CrossRef]
- Cheng, X.X.; Wu, G.; Zhang, L.; Ma, F.B. A new damage detection method for special-shaped steel arch bridges based on Fractal Theory and the model updating technique. Int. J. Struct. Stab. Dyn. 2021, 21, 2150030. [Google Scholar] [CrossRef]
- De Domenico, D.; Messina, D.; Recupero, A. Quality control and safety assessment of prestressed concrete bridge decks through combined field tests and numerical simulation. Structures 2022, 39, 1135–1157. [Google Scholar] [CrossRef]
- Xia, Y.; Ni, Y.-Q.; Zhang, P.; Liao, W.-Y.; Ko, J.-M. Stress Development of a Supertall Structure during Construction: Field Monitoring and Numerical Analysis. Comput. Aided Civ. Infrastruct. Eng. 2011, 26, 542–559. [Google Scholar] [CrossRef]
- Teng, J.; Lu, W.; Cui, Y.; Zhang, R. Temperature and Displacement Monitoring to Steel Roof Construction of Shenzhen Bay Stadium. Int. J. Struct. Stab. Dyn. 2016, 16, 1640020. [Google Scholar] [CrossRef]
- Baas, E.J.; Riggio, M.; Barbosa, A.R. A methodological approach for structural health monitoring of mass-timber buildings under construction. Constr. Build. Mater. 2021, 268, 121153. [Google Scholar] [CrossRef]
- Park, H.S.; Shin, Y.; Choi, S.W.; Kim, Y. An Integrative Structural Health Monitoring System for the Local/Global Responses of a Large-Scale Irregular Building under Construction. Sensors 2013, 13, 9085–9103. [Google Scholar] [CrossRef] [PubMed]
- Wenner, M.; Kading, M.; Marx, S. Monitoring of bridges under construction. Bautechnik 2018, 95, 44–52. [Google Scholar] [CrossRef]
- Abdal, S.; Mansour, W.; Agwa, I.; Nasr, M.; Abadel, A.; Özkılıç, Y.O.; Akeed, M.H. Application of Ultra-High-Performance Concrete in Bridge Engineering: Current Status, Limitations, Challenges, and Future Prospects. Buildings 2023, 13, 185. [Google Scholar] [CrossRef]
- JTG D60-2015; General Specifications for Design of Highway Bridges and Culverts. CCCC Highway Consultants Co., Ltd.: Beijing, China, 2015. (In Chinese)
- Xiang, M.; Lu, W.; Zhu, H.; Zhou, D. Process control technology during cast-in-place cantilever construction in long span prestressed concrete continuous beam bridge. J. Highw. Transp. Res. Dev. 2004, 21, 71–73. (In Chinese) [Google Scholar]
- Cheng, X.; Han, X.; Miao, C.; Bi, L. Stress prediction and control in cantilever construction of continuous beam bridge based on Grey Theory. World Bridges 2009, 4, 46–49. (In Chinese) [Google Scholar]
- Dai, L.; Cui, M.-D.; Cheng, X.-X. Structural-Health-Monitoring-Oriented Finite Element Model for a Specially Shaped Steel Arch Bridge and Its Application. Math. Comput. Appl. 2023, 28, 33. [Google Scholar] [CrossRef]
- Cheng, X.X.; Dong, J.; Han, X.L.; Fei, Q.G. Structural health monitoring-oriented finite-element model for a large transmission tower. Int. J. Civ. Eng. 2018, 16, 79–92. [Google Scholar] [CrossRef]
- Waldo, W.G.; Katnani, A.D.; Sachdev, H.S. Resist optimization for SOR X-ray lithography using an orthogonal experimental design. In Electron-Beam, X-ray, and Ion-Beam Submicrometer Lithographies for Manufacturing; SPIE: Bellingham, WA, USA, 1992. [Google Scholar] [CrossRef]
- Wang, G.; Ma, X.; Hu, T.; Zhang, D. Experimental and analytical study on factors influencing biomimetic undulating fin propulsion performance based on orthogonal experimental design. Adv. Robot. 2013, 27, 597–609. [Google Scholar] [CrossRef]
- Shao, S.; Li, Z.; Zhang, J.; Gao, K.; Liu, Y.; Jiao, W. Preparation of Ce-MnOX/γ-Al2O3 by high gravity-assisted impregnation method for efficient catalytic ozonation. Chem. Eng. Sci. 2022, 248, 117246. [Google Scholar] [CrossRef]
- Teng, J.; Zhu, Y.-H.; Zhou, F.; Li, H.; Ou, J.-P. Finite element model updating for large span spatial steel structure considering uncertainties. J. Cent. South Univ. Technol. 2010, 17, 857–862. [Google Scholar] [CrossRef]



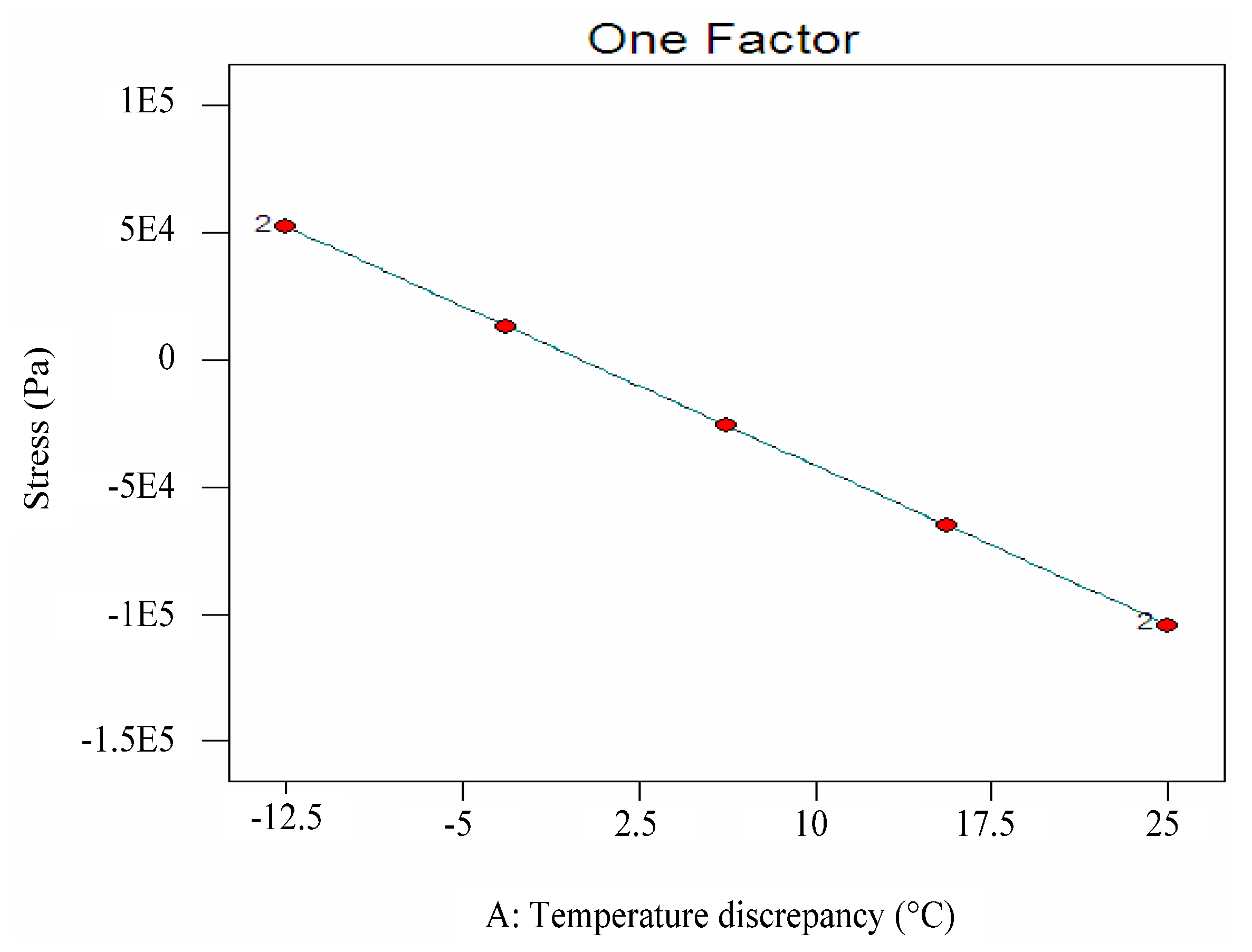
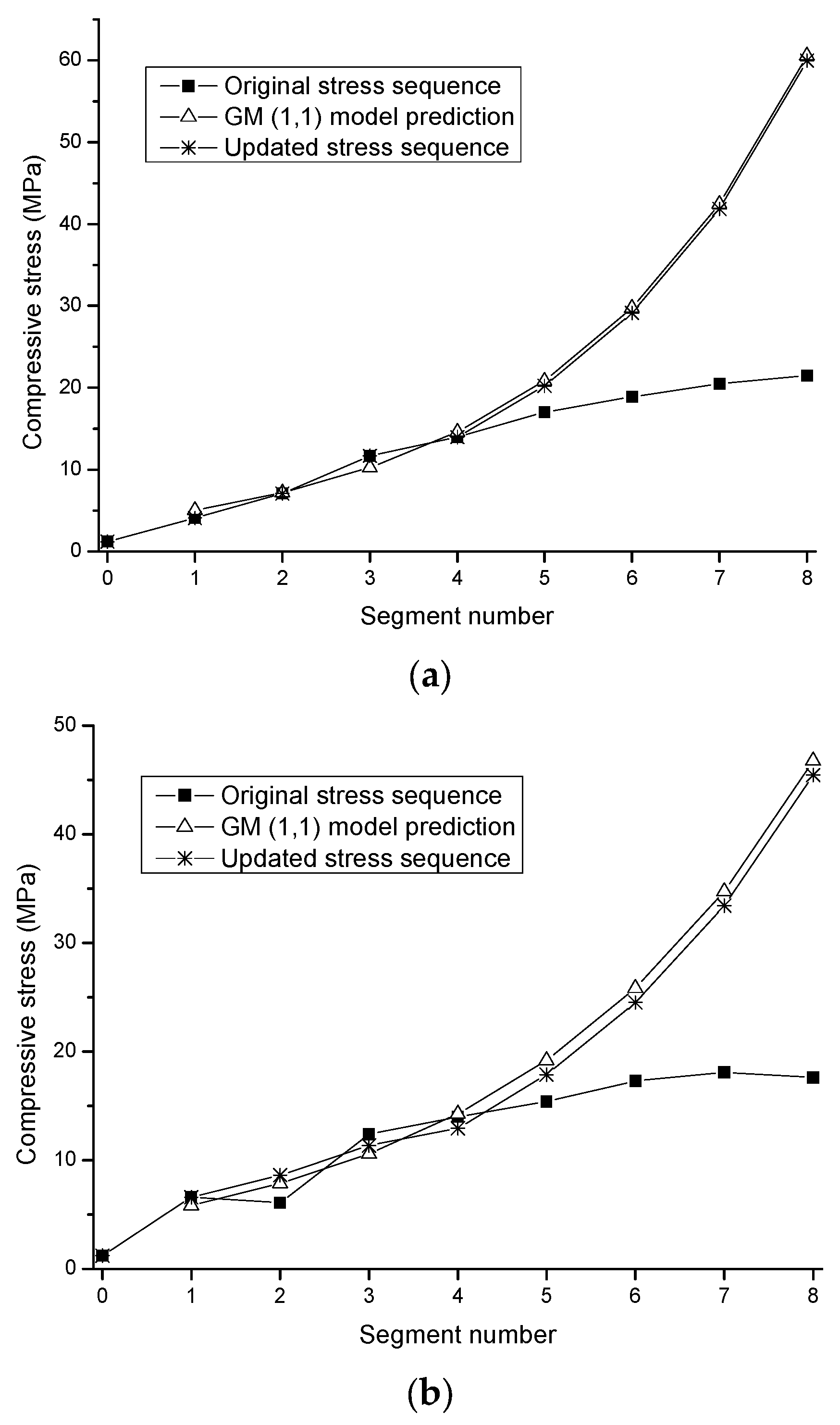

| Run | Temperature Discrepancy (°C) | Stress at Upper Measurement Point (Pa) |
|---|---|---|
| 1 | −3.125 | 13,066.9387 |
| 2 | 6.25 | −26,092.13 |
| 3 | 25 | −104,368.52 |
| 4 | 15.625 | −65,251.1987 |
| 5 | 25 | −104,368.52 |
| 6 | −12.5 | 52,184.26 |
| 7 | −12.5 | 52,184.26 |
| Factor | Sum of Squares | DF | Mean Square | F Value | Prob > F | R2 | Adequate Precision |
|---|---|---|---|---|---|---|---|
| Regression model (factor) | 2.758 × 1010 | 1 | 2.758 × 1010 | 1.780 × 108 | <0.0001 | 1.0000 | 23,532.462 |
| Residual | 774.60 | 5 | 154.92 | ||||
| Lack of fit | 774.60 | 3 | 258.20 | ||||
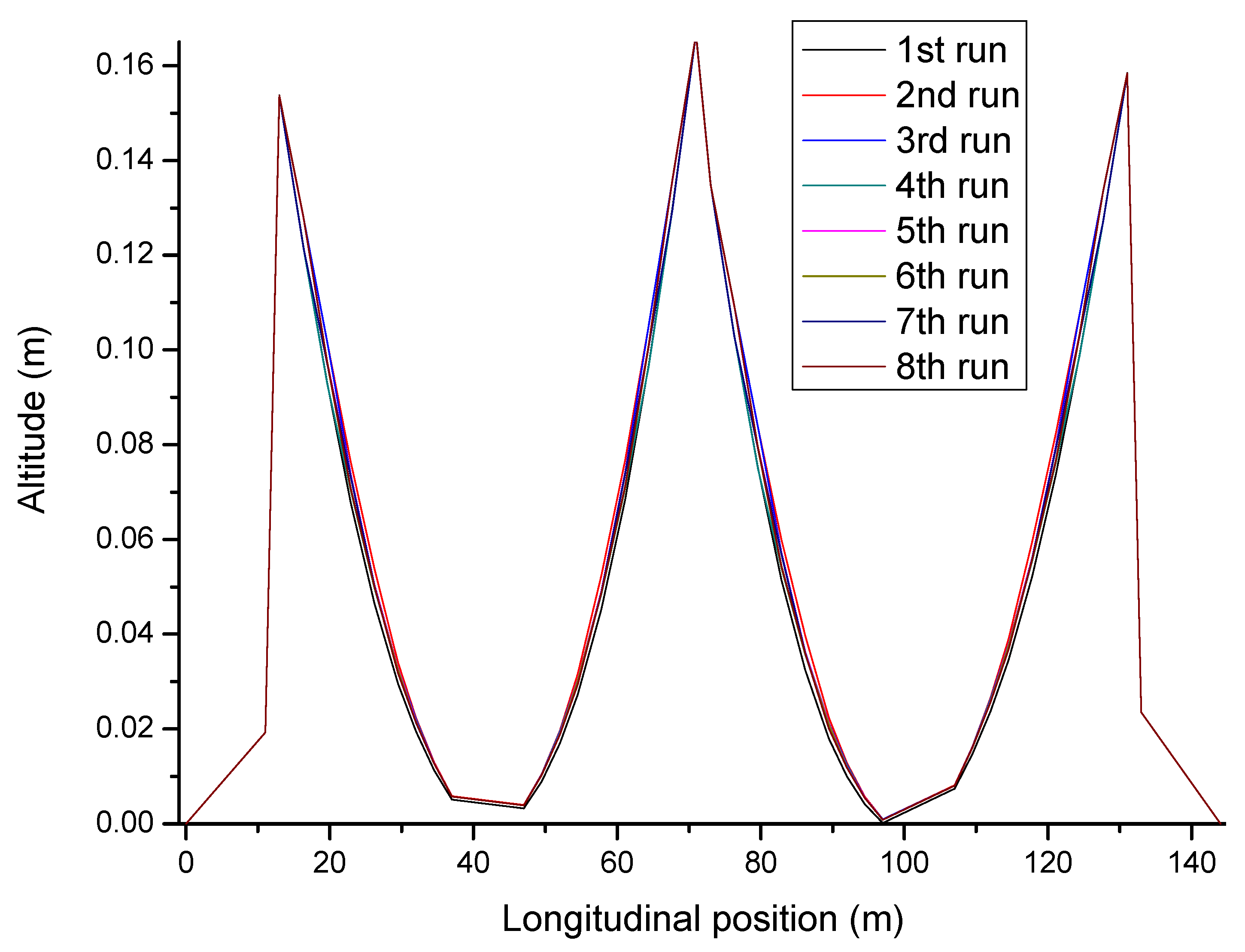
| Along-Bridge Position (m) | Full-Scale Altitude (m) | Initial Model without Model Updating | Model Updated Using the Proposed Method | Model Updated Using the Traditional Method | |||
|---|---|---|---|---|---|---|---|
| Altitude (m) | Relative Error (%) | Altitude (m) | Relative Error (%) | Altitude (m) | Relative Error (%) | ||
| 0 | −0.00261 | 0 | 100 | 0 | 100 | 0 | 100 |
| 11 | 0.01918 | 0.01926 | 0.42 | 0.01938 | 1.04 | 0.01926 | 0.42 |
| 13 | 0.15796 | 0.15375 | 2.67 | 0.15171 | 3.96 | 0.153715 | 2.69 |
| 16.3 | 0.1679 | 0.12186 | 27.42 | 0.1656 | 1.37 | 0.124063 | 26.11 |
| 19.6 | 0.14583 | 0.09311 | 36.15 | 0.14545 | 0.26 | 0.09616 | 34.06 |
| 22.9 | 0.11277 | 0.06771 | 39.96 | 0.11298 | 0.19 | 0.070769 | 37.24 |
| 26.2 | 0.07171 | 0.04654 | 35.10 | 0.07775 | 8.42 | 0.049133 | 31.48 |
| 29.5 | 0.05565 | 0.02958 | 46.85 | 0.05095 | 8.45 | 0.031481 | 43.43 |
| 32 | 0.03987 | 0.01945 | 51.22 | 0.03375 | 15.35 | 0.020846 | 47.72 |
| 34.5 | 0.01509 | 0.01133 | 24.92 | 0.01972 | 30.68 | 0.012221 | 19.01 |
| 37 | 0.00732 | 0.00506 | 30.87 | 0.00898 | 22.68 | 0.005481 | 25.12 |
| 47 | 0.00522 | 0.00328 | 37.16 | 0.00606 | 16.09 | 0.003676 | 29.58 |
| 49.5 | 0.01044 | 0.00903 | 13.51 | 0.01568 | 50.19 | 0.009899 | 5.18 |
| 52 | 0.02467 | 0.01695 | 31.29 | 0.02889 | 17.11 | 0.018314 | 25.76 |
| 54.5 | 0.0429 | 0.02728 | 36.41 | 0.04565 | 6.41 | 0.029139 | 32.08 |
| 57.8 | 0.07183 | 0.04523 | 37.03 | 0.07252 | 0.96 | 0.04778 | 33.48 |
| 61.1 | 0.10877 | 0.06838 | 37.13 | 0.10877 | 0.00 | 0.071389 | 34.37 |
| 64.4 | 0.14771 | 0.09686 | 34.43 | 0.14324 | 3.03 | 0.099851 | 32.40 |
| 67.7 | 0.16664 | 0.12983 | 22.09 | 0.16644 | 0.12 | 0.131949 | 20.82 |
| 71 | 0.15758 | 0.16697 | 5.96 | 0.15657 | 0.64 | 0.166815 | 5.86 |
| 73 | 0.13736 | 0.13504 | 1.69 | 0.13298 | 3.19 | 0.134976 | 1.74 |
| 76.3 | 0.1363 | 0.10321 | 24.28 | 0.13342 | 2.11 | 0.105379 | 22.69 |
| 79.6 | 0.11823 | 0.0753 | 36.31 | 0.11163 | 5.58 | 0.07832 | 33.76 |
| 82.9 | 0.08417 | 0.05144 | 38.89 | 0.08289 | 1.52 | 0.05448 | 35.27 |
| 86.2 | 0.05511 | 0.0324 | 41.21 | 0.05307 | 3.70 | 0.034968 | 36.55 |
| 89.5 | 0.03805 | 0.01799 | 52.72 | 0.03127 | 17.82 | 0.01987 | 47.78 |
| 92 | 0.01127 | 0.01 | 11.27 | 0.01874 | 66.28 | 0.011376 | 0.94 |
| 94.5 | 0.0055 | 0.00414 | 24.73 | 0.00971 | 76.55 | 0.005016 | 8.80 |
| 97 | 0.00872 | 2.18 × 10 −4 | 97.50 | 0.00276 | 68.35 | 0.000625 | 92.83 |
| 107 | 0.01862 | 0.00743 | 60.10 | 0.01012 | 45.65 | 0.007842 | 57.88 |
| 109.5 | 0.02284 | 0.01474 | 35.46 | 0.02054 | 10.07 | 0.015623 | 31.60 |
| 112 | 0.03907 | 0.02374 | 39.24 | 0.03279 | 16.07 | 0.025126 | 35.69 |
| 114.5 | 0.04429 | 0.03457 | 21.95 | 0.04826 | 8.96 | 0.036474 | 17.65 |
| 117.8 | 0.07723 | 0.05218 | 32.44 | 0.07339 | 4.97 | 0.054785 | 29.06 |
| 121.4 | 0.10344 | 0.07364 | 28.81 | 0.10578 | 2.26 | 0.076721 | 25.83 |
| 124.4 | 0.13011 | 0.09897 | 23.93 | 0.13611 | 4.61 | 0.102044 | 21.57 |
| 127.7 | 0.15504 | 0.12731 | 17.89 | 0.15844 | 2.19 | 0.129533 | 16.45 |
| 131 | 0.15498 | 0.15848 | 2.26 | 0.15742 | 1.57 | 0.158447 | 2.24 |
| 133 | 0.02476 | 0.02351 | 5.05 | 0.02352 | 5.01 | 0.023505 | 5.07 |
| 144 | −0.00145 | 0 | 100 | 0 | 100 | 0 | 100 |
| Expectation | 0.0723 | 0.0544 | 0.0718 | 0.0559 | |||
| Deviation | 0.0583 | 0.0506 | 0.0578 | 0.0508 | |||
| Kurtosis | 1.5761 | 2.3619 | 1.5707 | 2.2658 | |||
| Skewness | 0.3274 | 0.7932 | 0.3417 | 0.7420 | |||
| RMS error | 41.07 | 32.74 | 38.48 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, X.-X. Model Updating for a Continuous Concrete Girder Bridge Using Data from Construction Monitoring. Appl. Sci. 2023, 13, 3422. https://doi.org/10.3390/app13063422
Cheng X-X. Model Updating for a Continuous Concrete Girder Bridge Using Data from Construction Monitoring. Applied Sciences. 2023; 13(6):3422. https://doi.org/10.3390/app13063422
Chicago/Turabian StyleCheng, Xiao-Xiang. 2023. "Model Updating for a Continuous Concrete Girder Bridge Using Data from Construction Monitoring" Applied Sciences 13, no. 6: 3422. https://doi.org/10.3390/app13063422
APA StyleCheng, X.-X. (2023). Model Updating for a Continuous Concrete Girder Bridge Using Data from Construction Monitoring. Applied Sciences, 13(6), 3422. https://doi.org/10.3390/app13063422





