Nonlinear Dynamic Mechanical Characteristics of Air Springs Based on a Fluid–Solid Coupling Simulation Method
Abstract
:1. Introduction
2. Materials and Methods
2.1. Fluid-Structure Coupling Construction Method
2.1.1. Fluid Cavity Model
2.1.2. Fluid Model
2.1.3. Adiabatic Process
2.2. Fluid Model Characterization
2.2.1. Virtual Work Principle
2.2.2. Fluid Action of the Chamber
3. Results of Air Spring Compression Experiment
3.1. Model Parameters for Air Spring
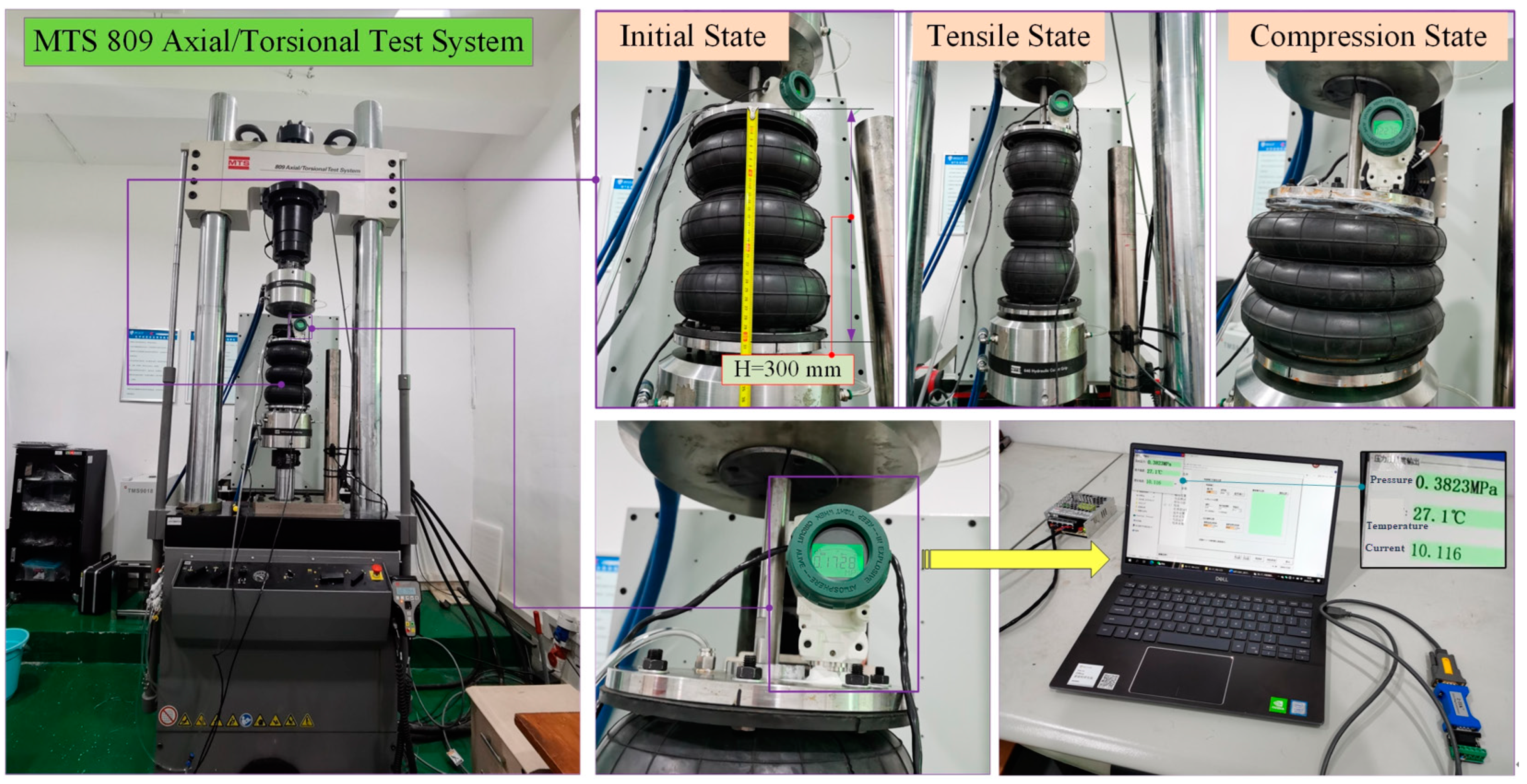
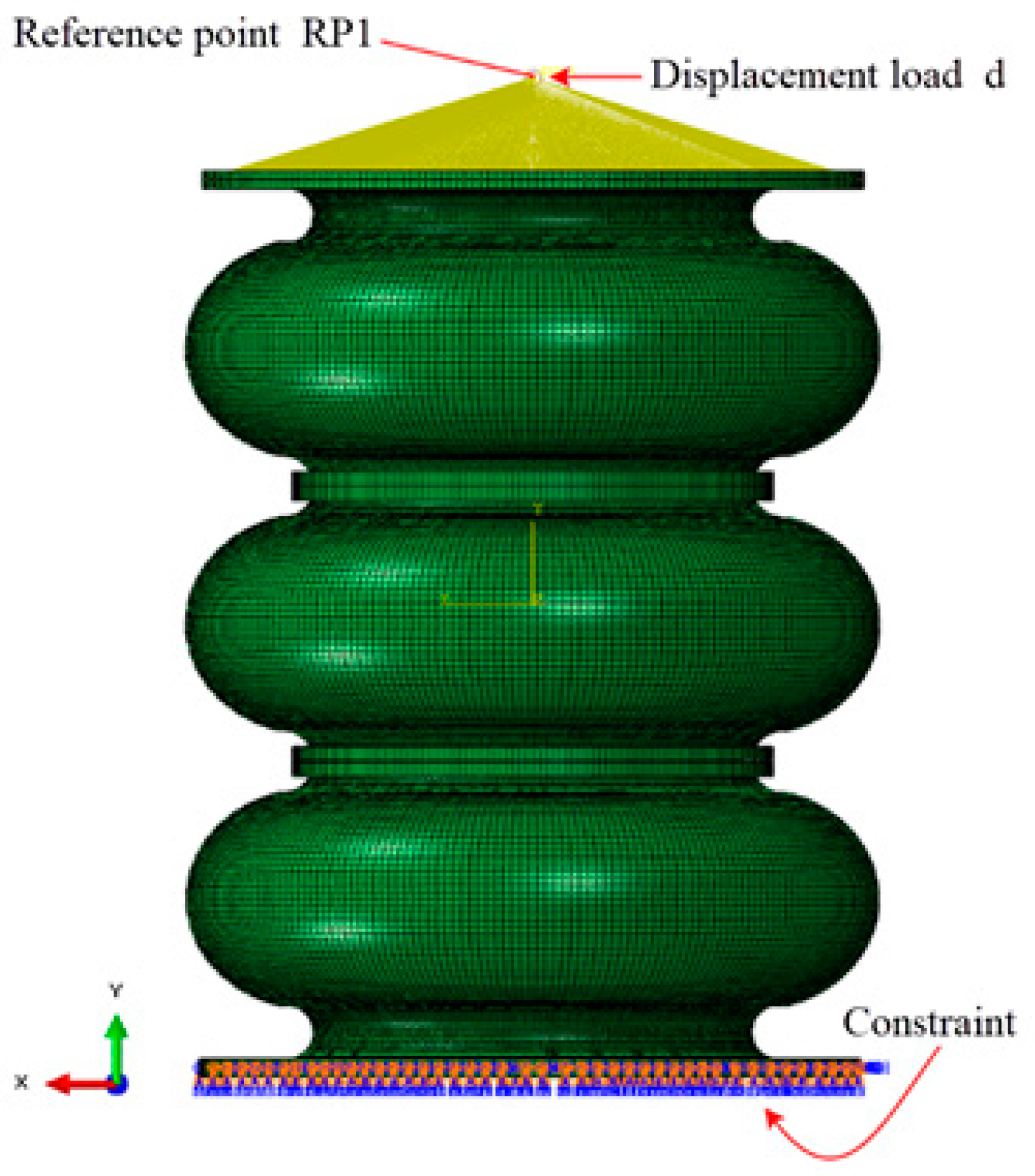
3.2. Testing Method
3.3. Experimental Verification
4. Results and Discussion
4.1. Axial Dynamic Mechanical Characteristics of the Air Spring
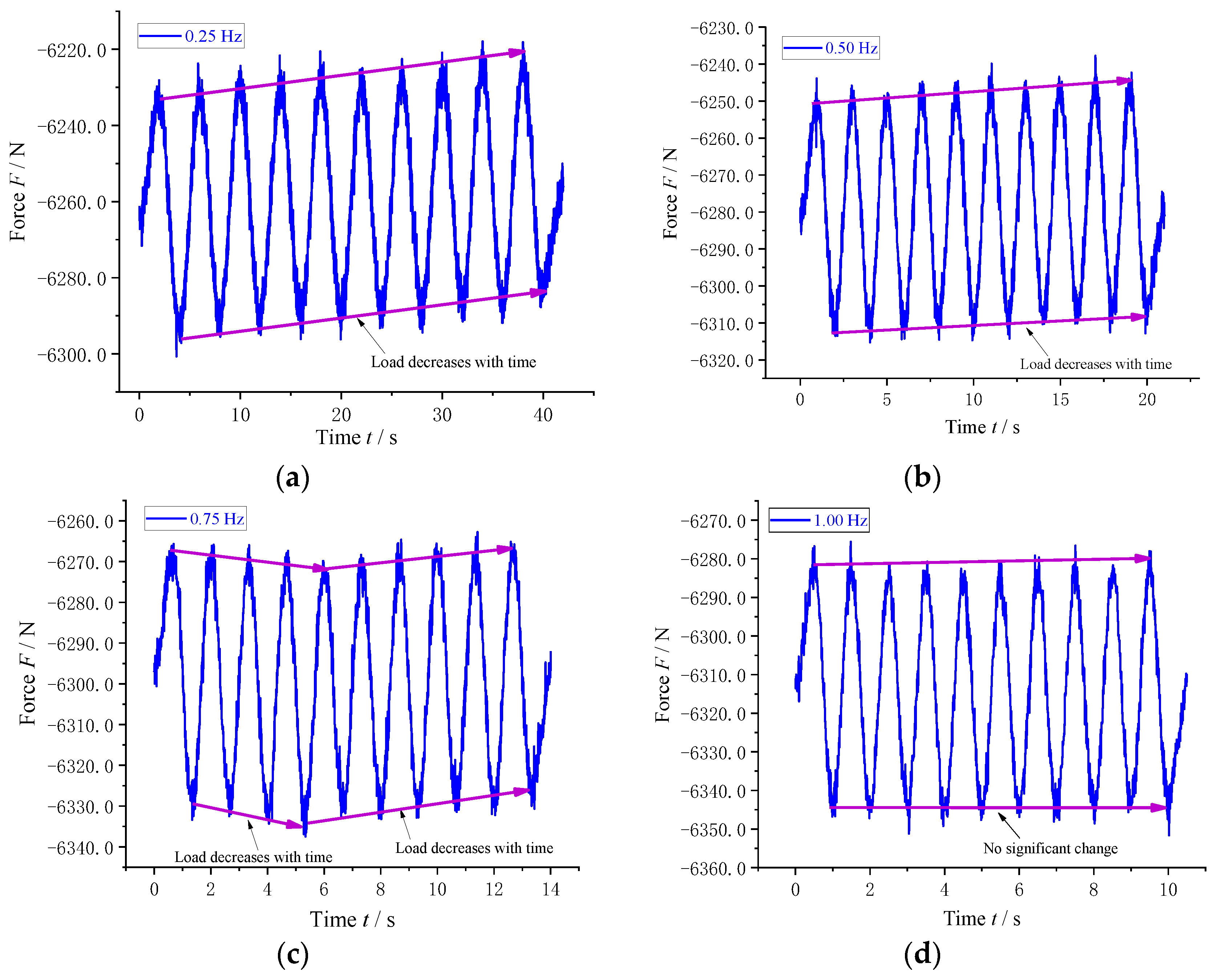
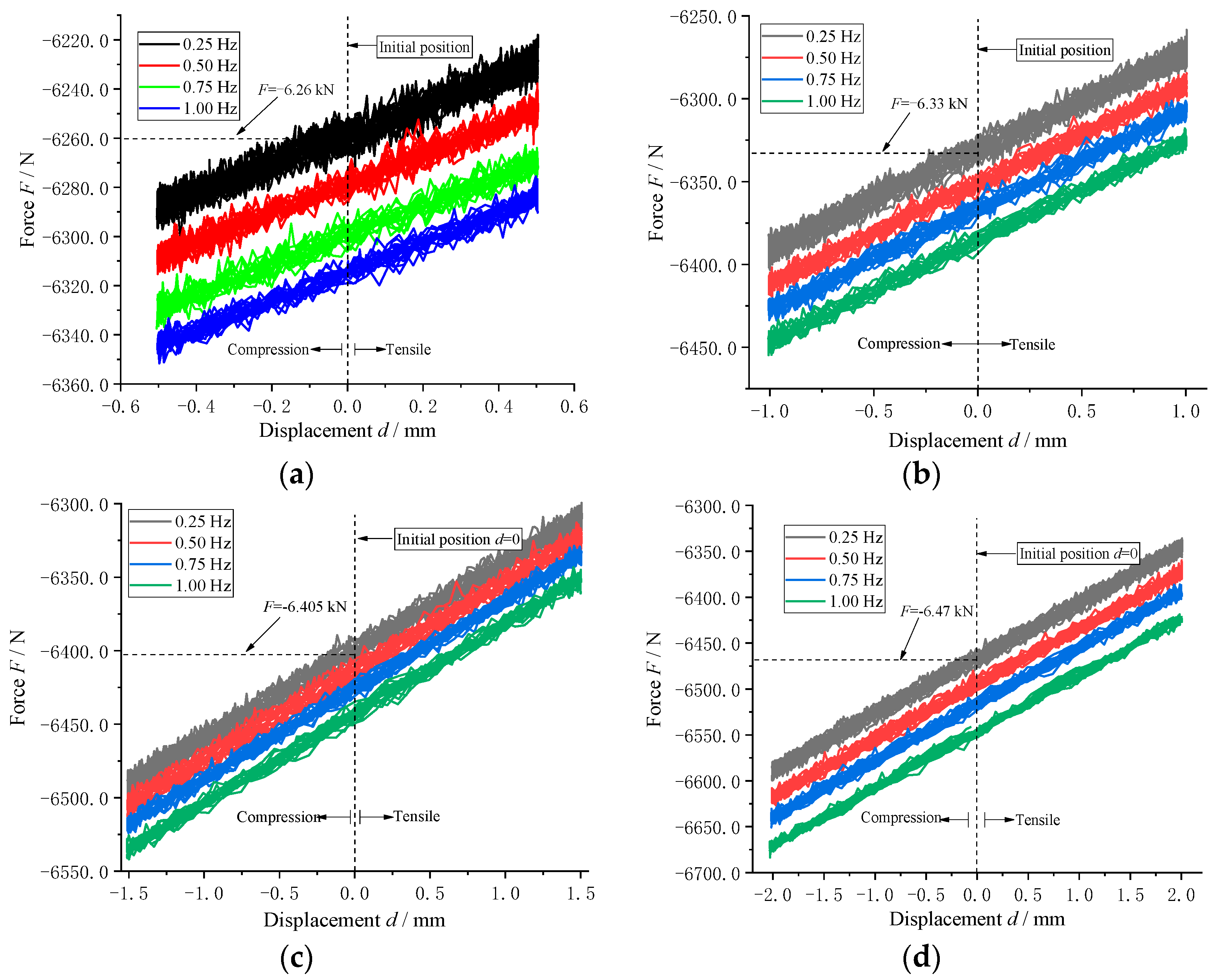
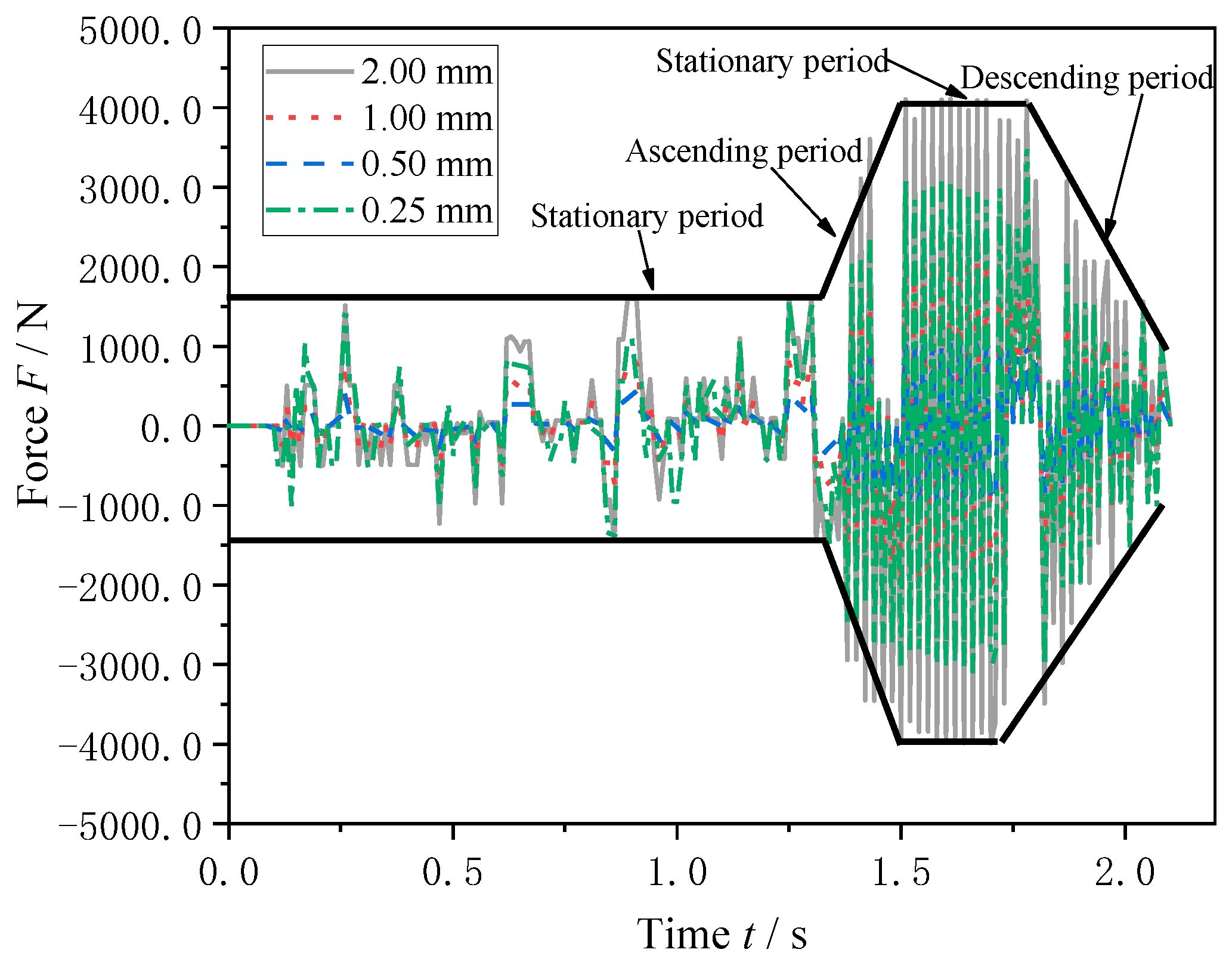
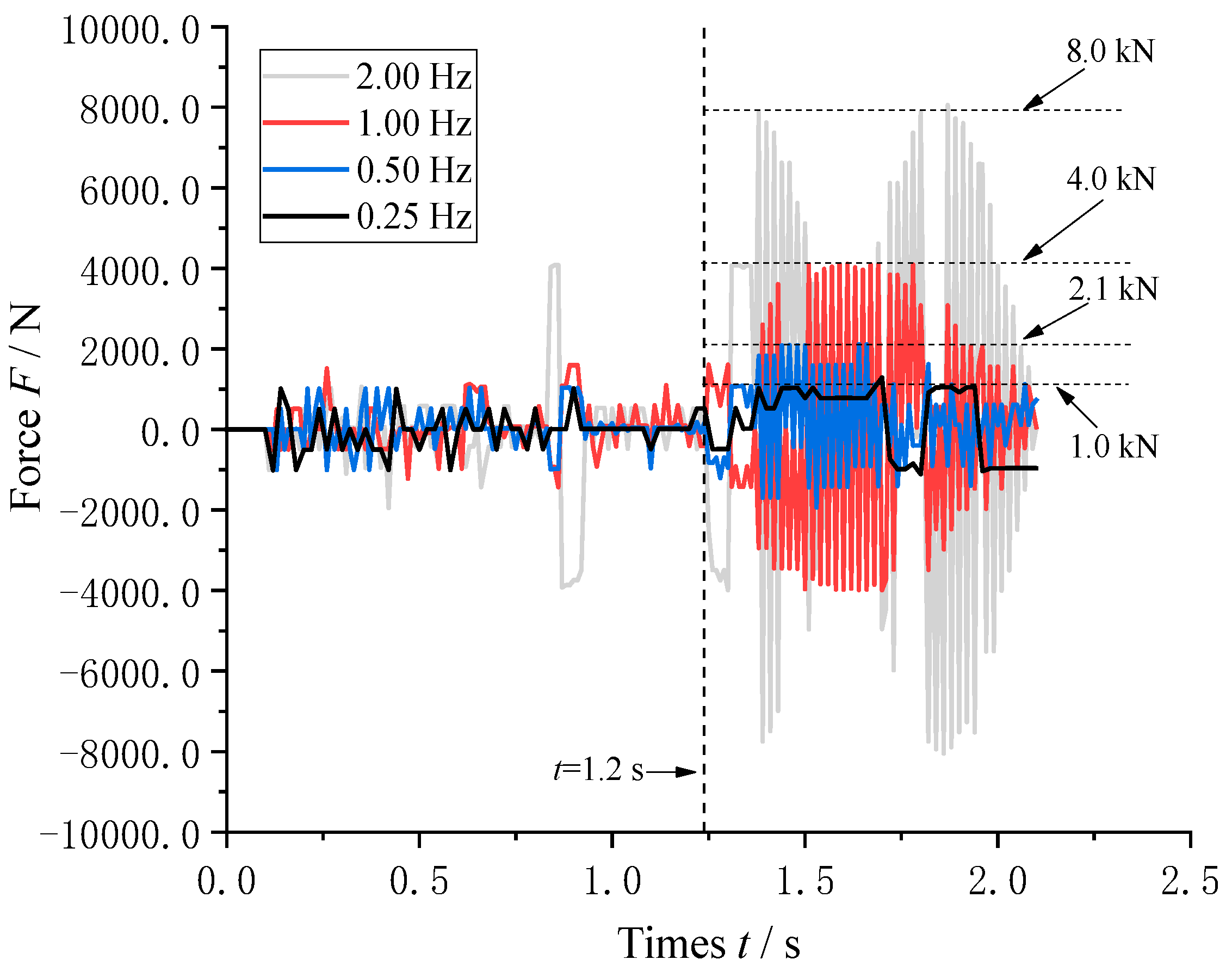
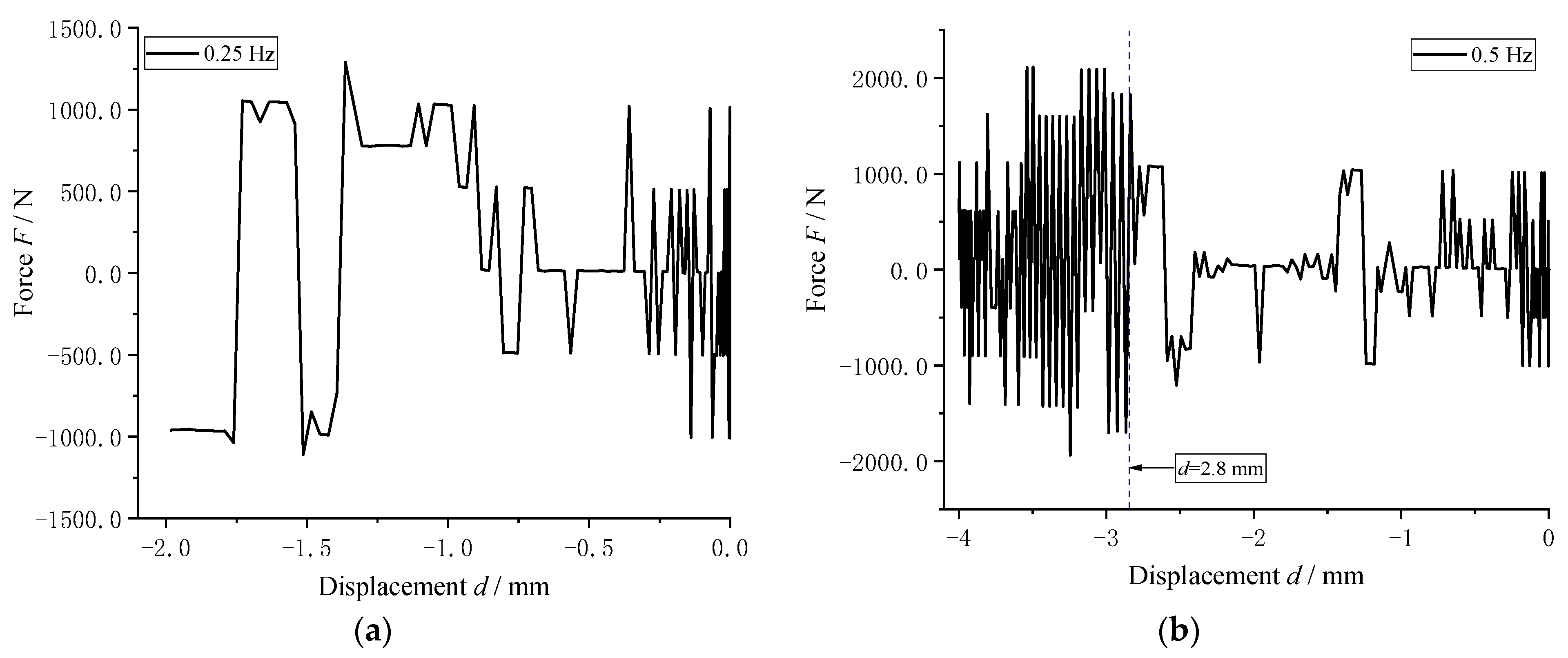
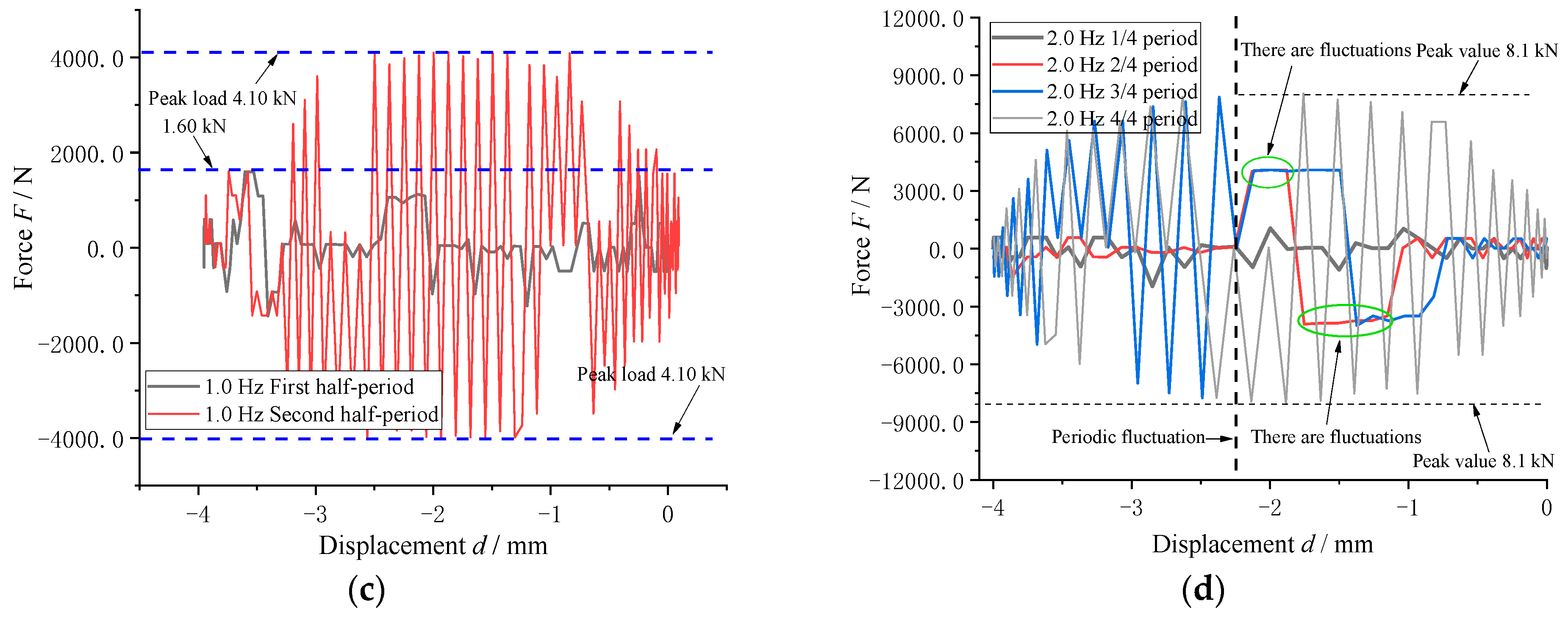
4.1.1. Influence of Frequency Excitation on Axial Load Characteristics of Air Spring
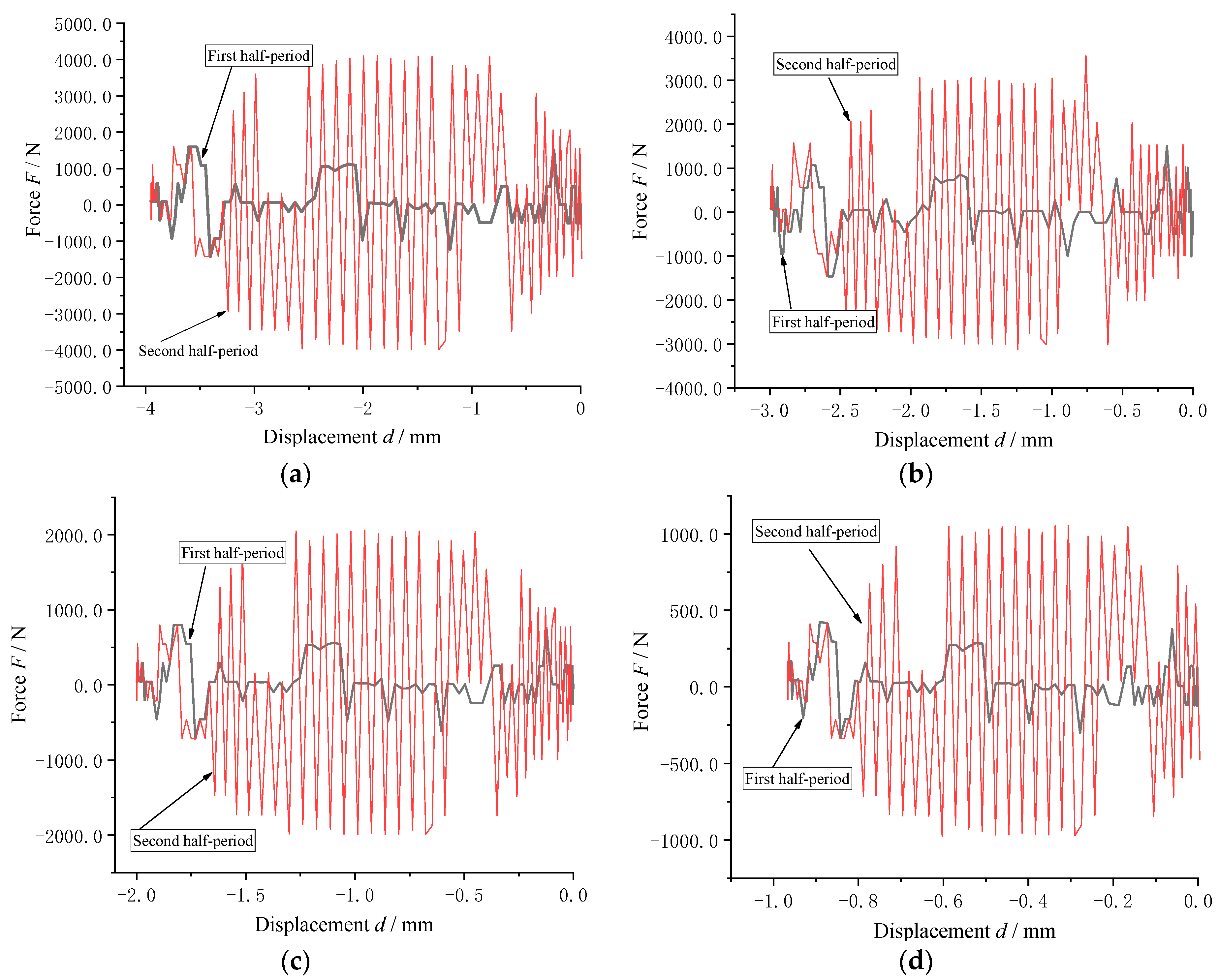
4.1.2. Effect of Amplitude Excitation on Axial Load Characteristics of Air Spring
4.1.3. Effect of Different Amplitudes on Axial Load Characteristics of Air Spring
4.2. Radial Dynamic Mechanical Characteristics of the Air Spring
4.2.1. Influence of Amplitude Excitation on Radial Load Characteristics
4.2.2. Influence of Excitation Frequency on Radial Load Characteristics
5. Conclusions
- (1)
- The construction of the air spring experimental platform, based on the proposed experimental principle, was successful and the validity of the established numerical analysis model was demonstrated through experimentation. This serves as a solid foundation for future studies of dynamic load simulation analyses.
- (2)
- The results from the low-frequency and low-amplitude excitation experiments of the air spring in the axial direction, conducted on the established experimental platform, demonstrate the influence of low frequency and low amplitude on the axial load. The impact of low frequency and low amplitude was found to be more pronounced in axial compression.
- (3)
- The results of the radial dynamic simulation analysis of the air spring demonstrate that there is a significant increase in radial load with increasing frequency, highlighting the substantial impact of low-frequency excitation on the axial load of the air spring.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Karpenkoa, M.; Skačkauskas, P.; Prentkovskis, O. Methodology for the Composite Tire Numerical Simula-tion Based on the Frequency Response Analysis. Eksploat. Niezawodn.-Maint. Reliab. 2023, 25, 1–11. [Google Scholar] [CrossRef]
- Skrickij, V.; Savitski, D.; Ivanov, V.; Skačkauskas, P. Investigation of Cavitation Process in Monotube Shock Absorber. Int. J Automot. Technol. 2018, 19, 801–810. [Google Scholar] [CrossRef]
- Wu, M.Y.; Li, X.B.; Yin, H.; Lu, J.C.; Wei, Y.T. Identification of Key Nonlinear Parameters and Research on Dynamic Characteristics of Air Spring Dynamic Stiffness Model. J. Mech. Eng. 2022, 58, 88–96. [Google Scholar]
- Quaglia, G.; Guala, A. Evaluation and validation of an air spring analytical model. Int. J. Fluid Power 2003, 4, 43–54. [Google Scholar] [CrossRef]
- Quaglia, G.; Sorli, M. Air suspension dimensionless analysis and design procedure. Veh. Syst. Dyn. 2001, 35, 443–475. [Google Scholar] [CrossRef]
- Chen, J.J.; Guo, K.H.; Yin, Z.H.; Wang, Q.Y.; Zhang, L. Research on Unified Model of Vertical Stiffness for Convoluted Air Spring. J. Mech. Eng. 2022, 58, 180–187. [Google Scholar]
- Tang, C.Y.; Zhang, Y.M.; Li, Y.G.; Zhao, G.Y.; Li, X. Analysis of Stiffness Characteristics and Influencing Factors Based on Single Chamber Cross-section Air Spring. J. Mech. Eng. 2014, 50, 137–144. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, J.; Wu, W.; Huang, C. Semi-active control of air suspension with auxiliary chamber subject to parameter uncertainties and time-delay. Int. J. Robust Nonlinear Control 2020, 30, 7130–7149. [Google Scholar] [CrossRef]
- Gokul, P.S.; Malar, M.K. Analytical approach for the design of convoluted air suspension and experimental validation. Acta Mech. Sin. 2019, 35, 1093–1103. [Google Scholar]
- Evans, J.R. Rail vehicle dynamic simulations using vampier. Veh. Syst. Dyn. 1999, 31, 119–140. [Google Scholar]
- Chen, Y.; Hou, Y.; Peterson, A.; Ahmadian, M. Failure mode and effects analysis of dual levelling valve airspring suspensions on truck dynamics. Veh. Syst. Dyn. 2019, 57, 617–635. [Google Scholar] [CrossRef]
- Ryaboy, V.M. Static and dynamic stability of pneumatic vibration isolators and systems of isolators. J. Sound Vib. 2014, 333, 31–51. [Google Scholar] [CrossRef]
- Li, Y.; He, L.; Shuai, C.-G.; Wang, C.-Y. Improved hybrid isolator with maglev actuator integrated in air spring for active-passive isolation of ship machinery vibration. J. Sound Vib. 2017, 407, 226–239. [Google Scholar] [CrossRef]
- Wu, X.W.; Chi, M.R.; Zhu, M.H.; Ceng, J.; Yang, F. Influences of air spring models on dynamics performance of railway vehicle. J. Traffic Transp. Eng. 2013, 13, 54–59. [Google Scholar]
- Mazzola, L.; Berg, M. Secondary Suspension of Railway Vehicle-Air Spring Modelling: Performance and Critical Issues. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2014, 228, 225–241. [Google Scholar] [CrossRef]
- Qi, Z.; Wang, X.L.; Mo, R.L. Air Spring Modeling and Vehicle Dynamics Analysis Based on Fractional Calculus Theory. J. China Railw. Soc. 2021, 43, 67–76. [Google Scholar]
- Facchinetti, A.; Mazzola, L.; Alfi, S.; Bruni, S. Mathematical modeling of the secondary air spring suspension in railway vehicles and its effect on safety and ride comfort. Veh. Syst. Dyn. 2010, 48, 429–449. [Google Scholar] [CrossRef]
- Zeng, X.; Zhang, L.; Yu, Y.; Shi, M.; Zhou, J. The stiffness and damping characteristics of a dual-chamber air spring device applied to motion suppression of marine structures. Appl. Sci. 2016, 6, 74. [Google Scholar] [CrossRef]
- Li, X.; He, Y.; Liu, W.; Wei, Y. Research on the vertical stiffness of a rolling lobe air spring. Proc. Inst. Mech. Eng. Part F J. Rail Transit 2016, 230, 1172–1183. [Google Scholar] [CrossRef]
- Bhattacharyya, S.; Ghosh, A.; Basu, B. Nonlinear modeling and validation of air spring effects in a sealed tuned liquid column damper for structural control. J. Sound Vib. 2017, 410, 269–286. [Google Scholar] [CrossRef]
- Zargar, B.; Fahim, A.; Jnifene, A. Development, validation, and parameter sensitivity analyses of a nonlinear mathematical model of air springs. J. Vib. Control 2011, 08, 1777–1787. [Google Scholar] [CrossRef]
- Chen, J.J.; Yin, Z.H.; He, J.H.; Shangguan, W. Study on Modelling and Dynamic Characteristic of Air Spring with Throttling Damping Orifice and Auxiliary Chamber. J. Mech. Eng. 2017, 53, 166–174. [Google Scholar] [CrossRef]
- Lee, J.H.; Kim, K.J. Modeling of nonlinear complex stiffness of dual-chamber pneumatic spring for precision vibration isolations. J. Sound Vib. 2007, 301, 909–926. [Google Scholar] [CrossRef]
- de Melo, F.; Pereira, A.; Morais, A. The Simulation of an Automotive Air Spring Suspension Using a Pseudo-Dynamic Procedure. Appl. Sci. 2018, 8, 1049. [Google Scholar] [CrossRef]
- Chang, F.; Lu, Z.H. Dynamic model of an air spring and integration into a vehicle dynamics model. Proceedings of the institution of mechanical engineers. Part D-J. Automoble Eng. 2008, 222, 1713–1825. [Google Scholar]
- Zhu, H.; Yang, J.; Zhang, Y.; Feng, X.; Ma, Z. Nonlinear dynamic model of air spring with a damper for vehicle ride comfort. Nonlinear Dyn. 2017, 89, 1545–1568. [Google Scholar] [CrossRef]
- Zhu, H.; Yang, J.; Zhang, Y.; Feng, X. A novel air spring dynamic model with pneumatic thermodynamics, effective friction and viscoelastic damping. J. Sound Vib. 2017, 408, 87–104. [Google Scholar] [CrossRef]
- Ren, J.; Zhong, J.L. The accurate prediction method of tension modulus for nylon cord/rubber composite material. Appl. Mech. Mater. 2014, 575, 115–120. [Google Scholar] [CrossRef]
- Levin, V.; Zingerman, K.; Vershinin, A.; Yakovlev, M. Numerical analysis of effective mechanical properties of rubber-cord composites under finite strains. Compos. Struct. 2015, 13, 25–26. [Google Scholar] [CrossRef]
- Yuan, C.Y.; Zhou, K.K.; Wu, L.Q. Structural Analysis Method of automotive air-spring rubber air-bag. J. Mech. Eng. 2009, 45, 221–225. [Google Scholar] [CrossRef]
- Liu, Z.B.; Xiao, S.N.; Luo, S.H. Simulation of Airbag Ejection Acceleration Process on a Train Collision Testbed. J. Southwest Jiaotong Univ. 2017, 52, 127–132. [Google Scholar]
- Li, Z.Z.; Zhu, T.; Xiao, S.N.; Zhang, J.K.; Wang, X.R.; Ding, H.X. Simulation method for train curve derailment collision and the effect of curve radius on collision response. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2023, 237, 1130–1139. [Google Scholar] [CrossRef]
- Zhu, T.; Ding, H.; Wang, C.; Liu, Y.; Xiao, S.; Yang, G.; Yang, B. Parameters calibration of the GISSMO failure model for SUS301L-MT. Chin. J. Mech. Eng. 2023, 36, 20. [Google Scholar] [CrossRef]
- Li, Y.; Xiao, S.; Yang, B.; Zhu, T.; Yang, G.; Xiao, S. Study on the influence factors of impact ejection performance for flexible airbag. Adv. Mech. Eng. 2018, 10, 1687814018807333. [Google Scholar] [CrossRef]
- Li, Y.R.; Yang, B.; Xie, J.K. Study of Influence Factors on Ejection Impact Performance of Air Spring. J. Mech. Eng. 2020, 56, 144–153. [Google Scholar]
- Li, Y.R.; Xiao, S.N.; Xie, J.K.; Zhu, T.; Yang, B.; Yang, G.W.; Xiao, S.D. Influence of Cord Parameters on the Load Characteristics of Airbags. Shock Vib. 2021, 2021, 8670882. [Google Scholar] [CrossRef]
- Dijk, R.; Keulen, F.; Sterk, J.C. Simulation of closed thin-walled structures partially filled with fluid. Int. J. Solids Struct. 2000, 37, 6063–6083. [Google Scholar] [CrossRef]
- Berry, D. Formulation and Experimental Verification of Pneumatic and Hydraulic Finite Elements; Indiana Purdue University: West Lafayette, IN, USA, 1994. [Google Scholar]
- Hiremath, S.S.; Singaperumal, M. Investigations on actuator dynamics through theoretical and finite element approach. Math. Probl. Eng. 2010, 2010, 191898. [Google Scholar] [CrossRef]
- Liu, Q.F.; Zhang, Z.G.; Wang, Y.; Zheng, H.X.; Xie, J.L. Finite Element Calculation of the Nonlinear Lateral Characteristic of Air Spring. J. China Railw. Soc. 2015, 37, 29–33. [Google Scholar]
- Mooney, M. A theory of large elastic deformation. J. Appl. Phys. 2004, 11, 582–592. [Google Scholar] [CrossRef]

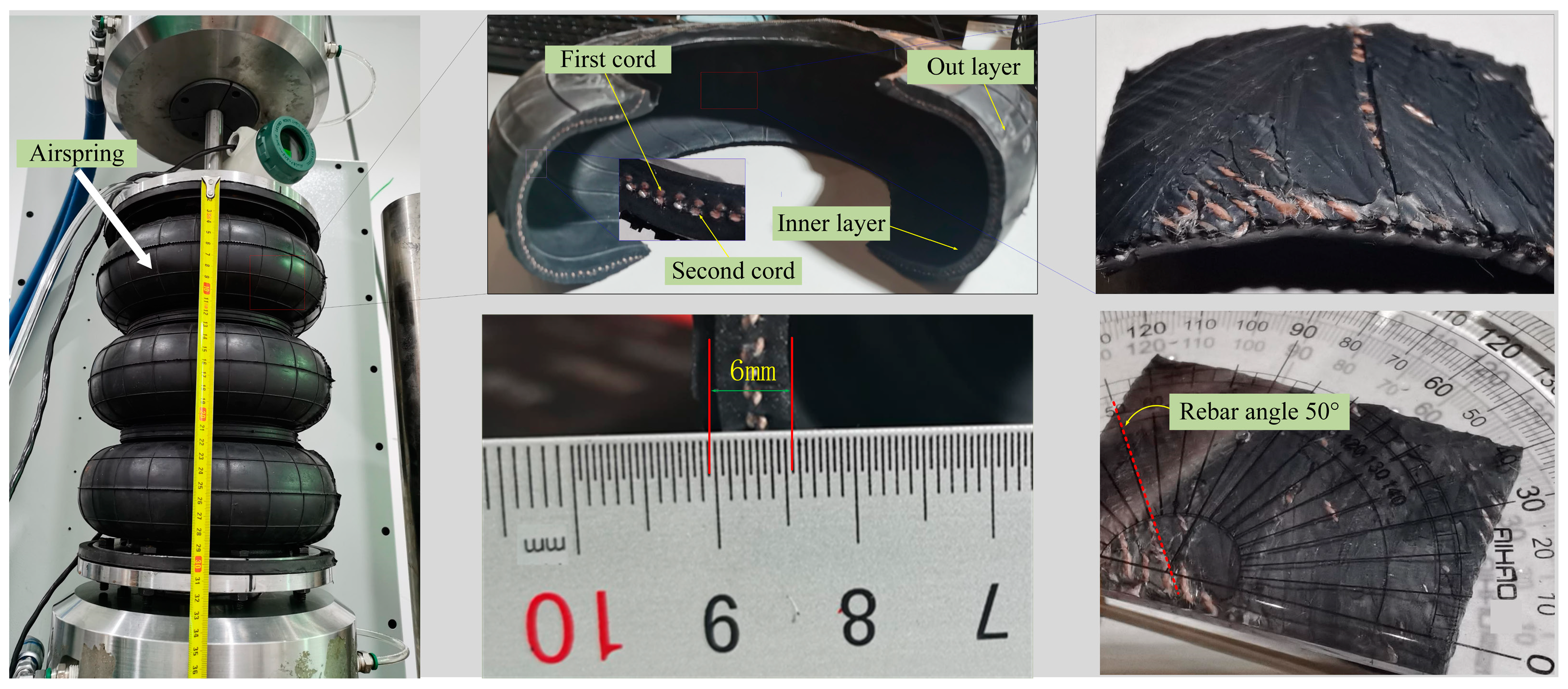




| Rubber Parameters | Cord Parameters | ||||||
|---|---|---|---|---|---|---|---|
| C10 | C01 | D | (t/mm3) | E/MPa | (t/mm3) | Rebar Angle/° | |
| 7.2 | 3.2 | 0 | 1.19 × 10−9 | 2500 | 0.4 | 1.19 × 10−9 | 50 |
| Cord | Rebar Angle θ/° | Spacing d1/mm | Cord Diameter φ/mm | Position of Rebar d2/mm |
|---|---|---|---|---|
| First cord Second cord | 50 −50 | 1.5 1.5 | 0.5 0.5 | 1 −1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Xiao, S.; Xie, J.; Zhu, T.; Zhang, J. Nonlinear Dynamic Mechanical Characteristics of Air Springs Based on a Fluid–Solid Coupling Simulation Method. Appl. Sci. 2023, 13, 12677. https://doi.org/10.3390/app132312677
Li Y, Xiao S, Xie J, Zhu T, Zhang J. Nonlinear Dynamic Mechanical Characteristics of Air Springs Based on a Fluid–Solid Coupling Simulation Method. Applied Sciences. 2023; 13(23):12677. https://doi.org/10.3390/app132312677
Chicago/Turabian StyleLi, Yuru, Shoune Xiao, Junke Xie, Tao Zhu, and Jingke Zhang. 2023. "Nonlinear Dynamic Mechanical Characteristics of Air Springs Based on a Fluid–Solid Coupling Simulation Method" Applied Sciences 13, no. 23: 12677. https://doi.org/10.3390/app132312677
APA StyleLi, Y., Xiao, S., Xie, J., Zhu, T., & Zhang, J. (2023). Nonlinear Dynamic Mechanical Characteristics of Air Springs Based on a Fluid–Solid Coupling Simulation Method. Applied Sciences, 13(23), 12677. https://doi.org/10.3390/app132312677






