Unveiling the Strain Rate Sensitivity of G18NiCrMo3-6 CAST Steel in Tension/Compression Asymmetry
Abstract
:1. Introduction
2. Materials and Methods
2.1. Studied Material
2.2. Mechanical Testing
2.3. Determination of SRS
3. Results
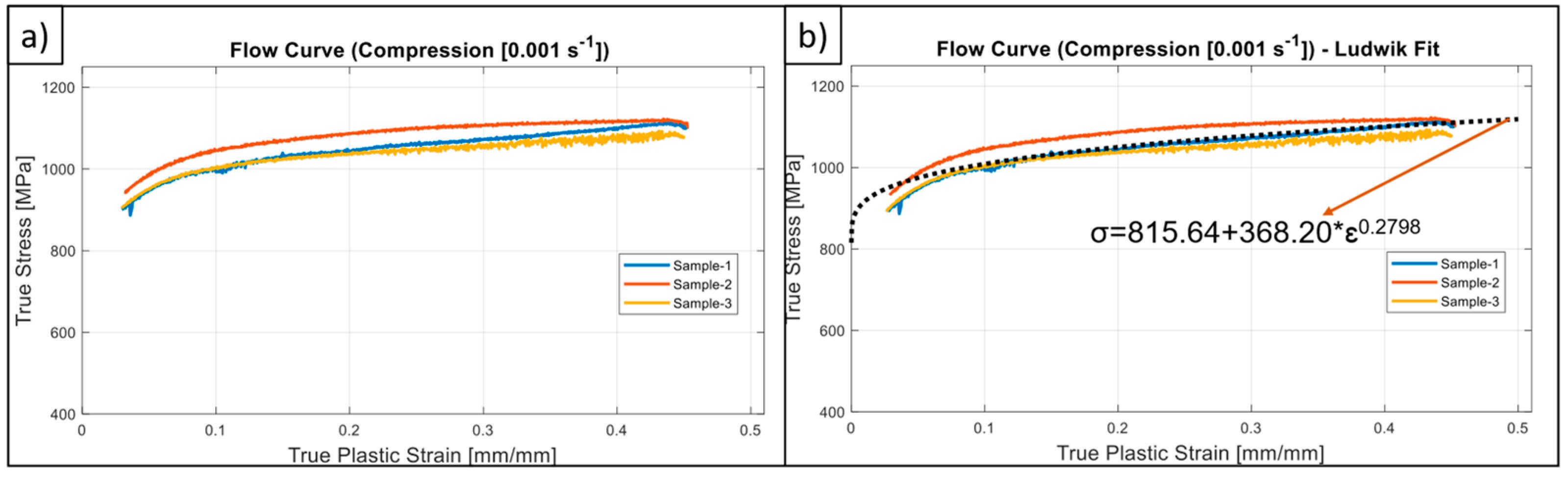
3.1. Basic Formulation
3.2. Time Integration Procedure
- Initialization: Uptake the material parameters, state variables, and the deformation gradient (F) at beginning of the time increment.
- Computation of strain tensor: Compute the Hencky strain tensor through the use of F (our code was constructed as finite-strain basis in such a way that free body translations were eliminated by means of Hencky strain measure [30], however Kroner decomposition [31] was discarded for the present study). Hencky strain measure is one of the most appropriate ways to deal with moderate deformations [32].
- Check the plasticity criterion: Compute the trial stress (Equation (21)) and check for the yield locus definition (Equation (23)):
- Update state variables:If ≤ 0 → step is elastic (∆λ = 0) thus, conserve the state variables.If > 0 → step is plastic, solve for ∆λ (Equation (20)). Update , , , .
- Finalization: Deliver the state variables and tangent modulus to Abaqus solver for the convergence check. For the present study, perturbation-based numerical tangent matrix formulation is used. (Equation (24)):
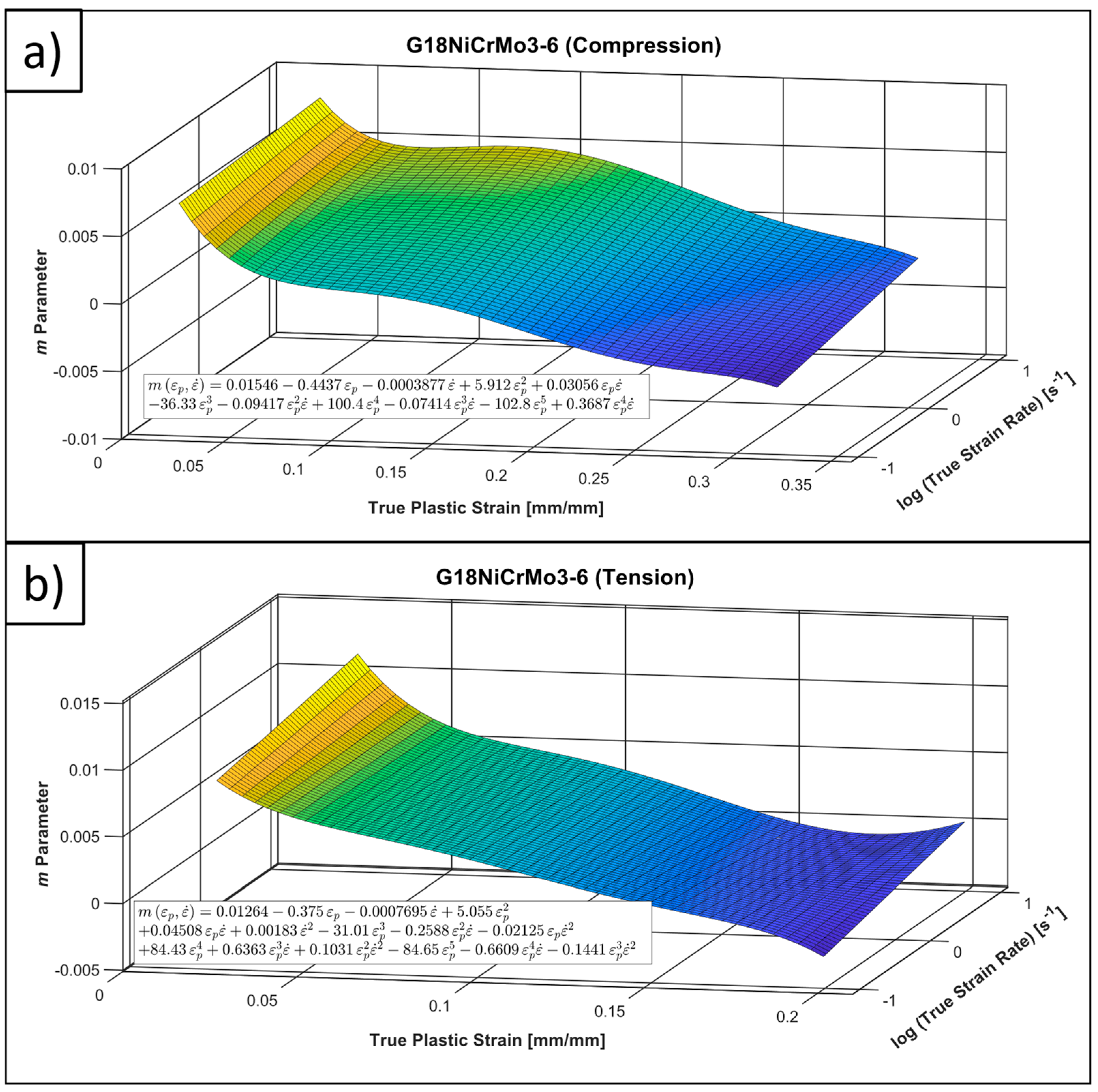
3.3. Imposing the Asymmetry and Rate Dependence
4. Results and Discussion
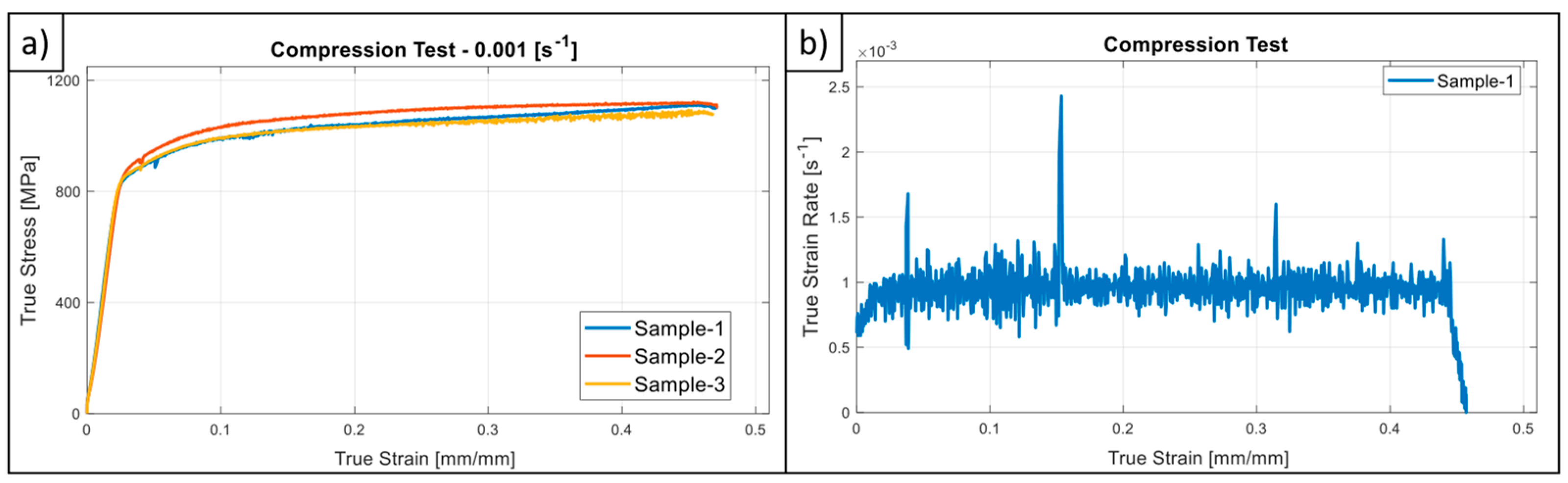
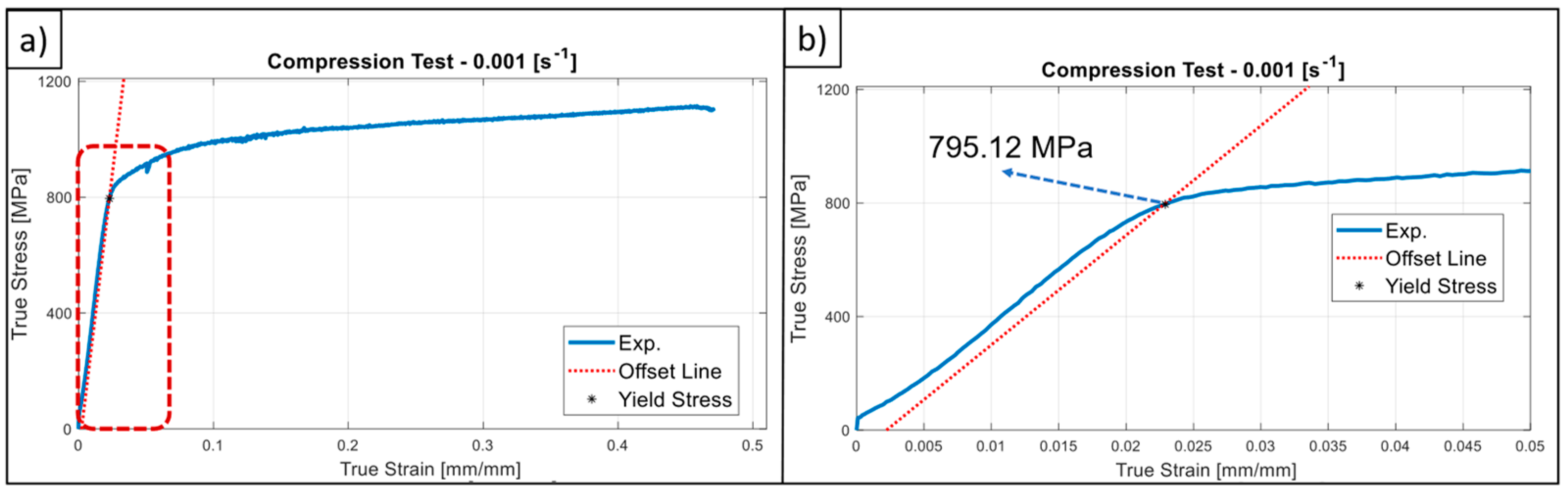
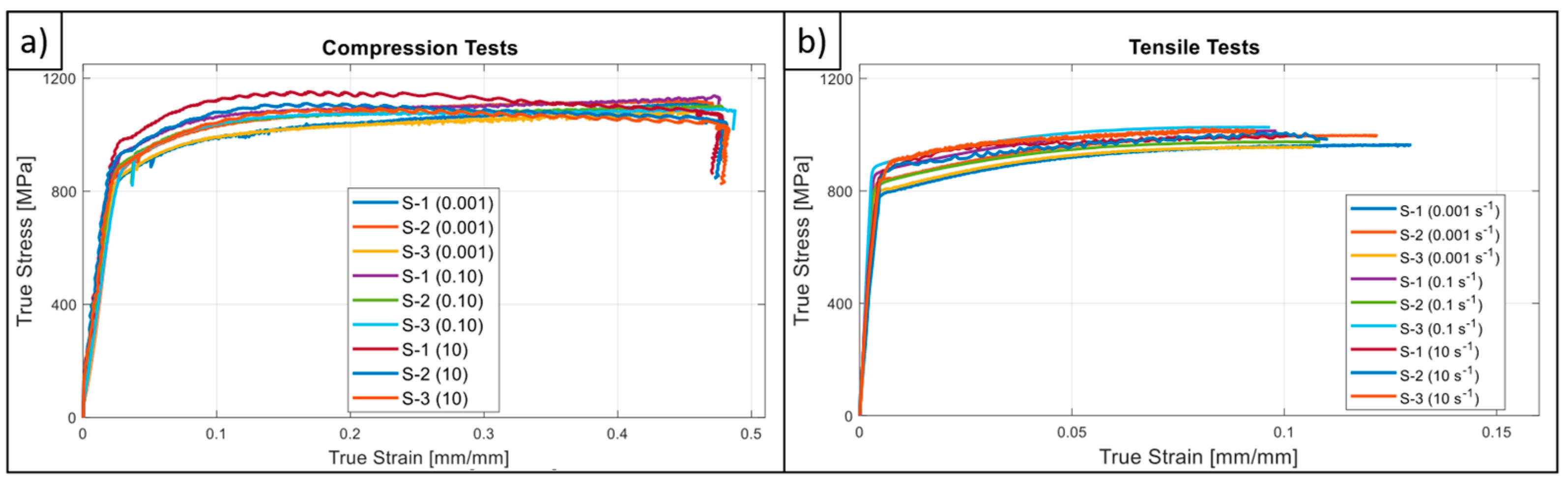
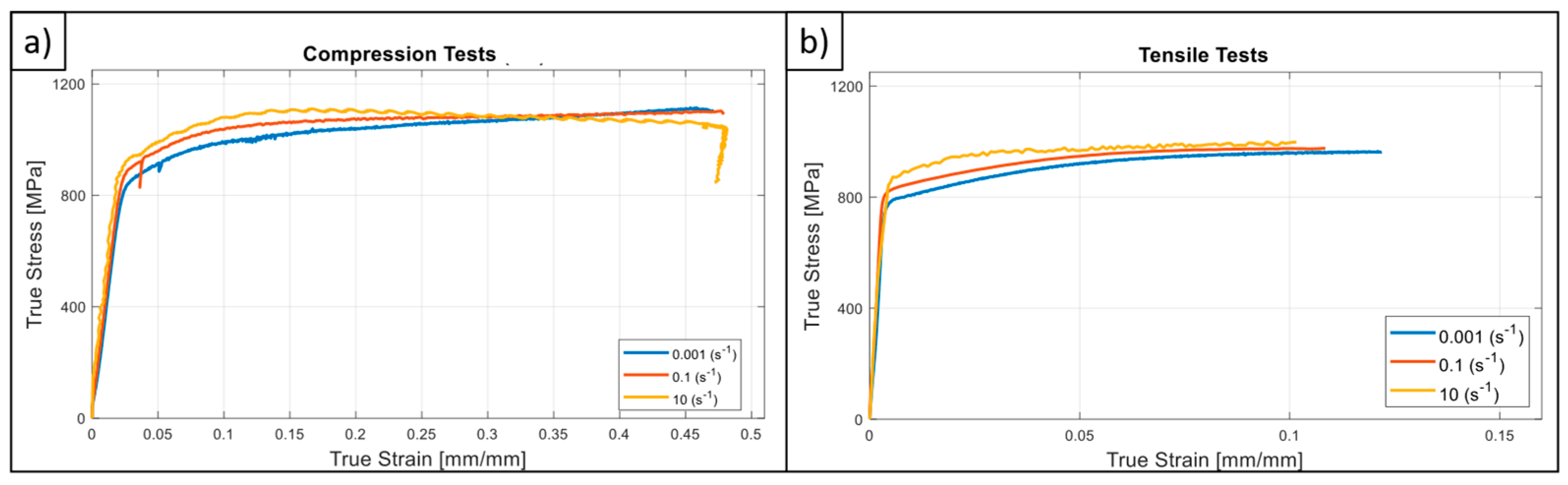
4.1. Mechanical Testing
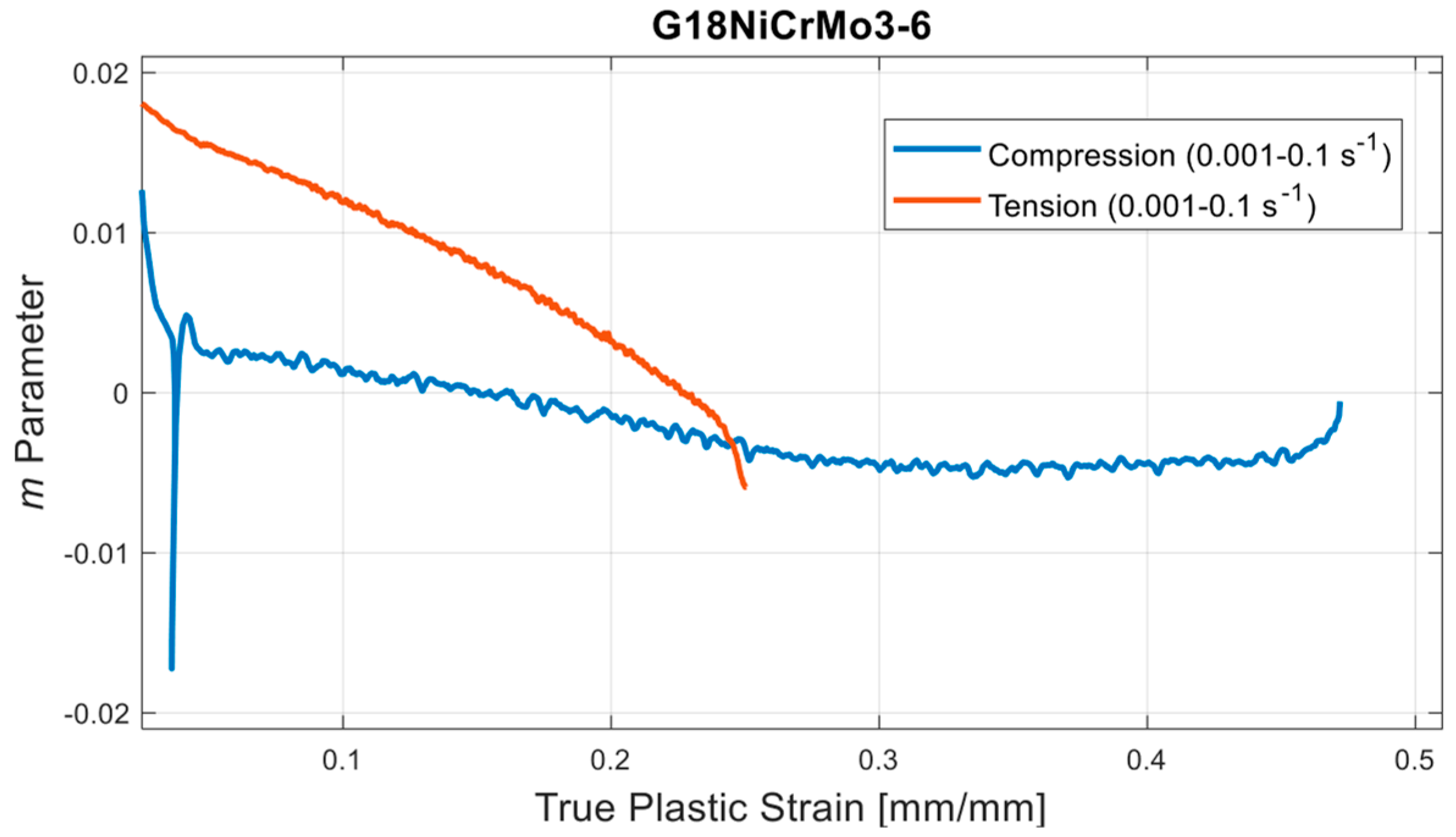
4.2. Determination of SRS
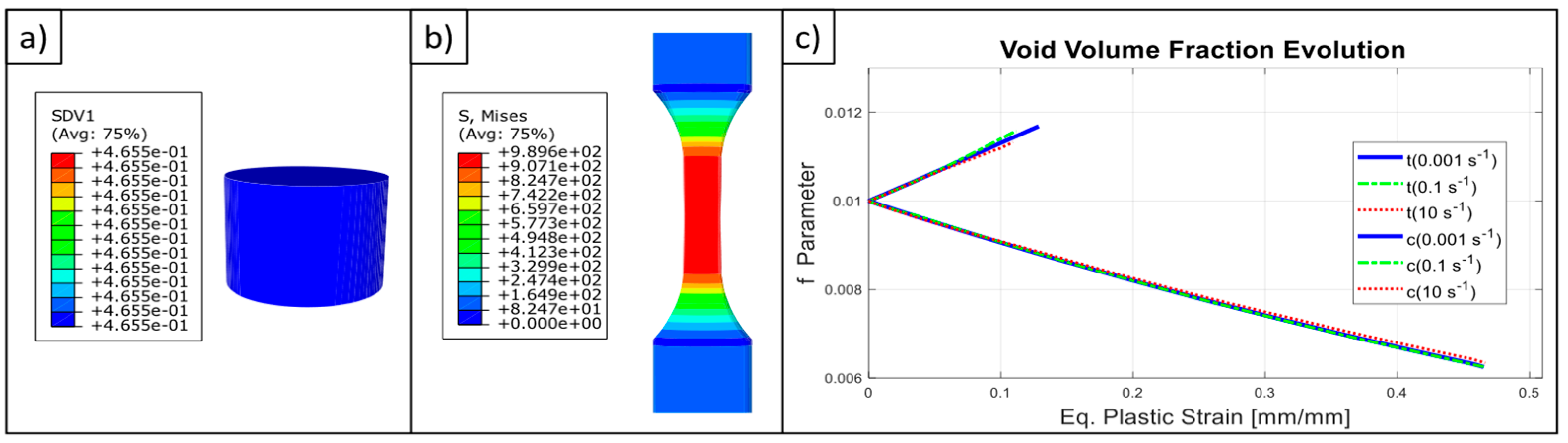
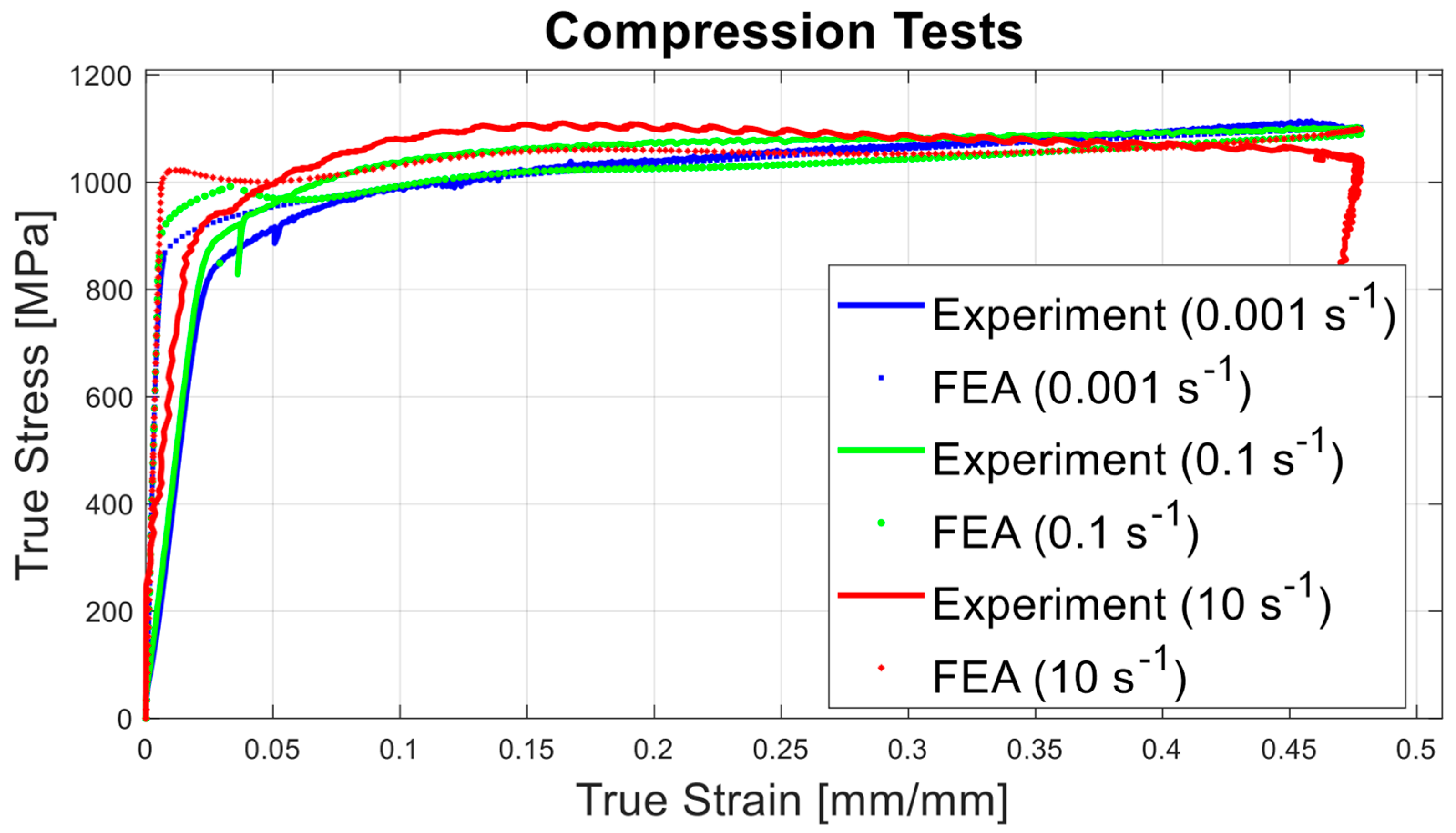
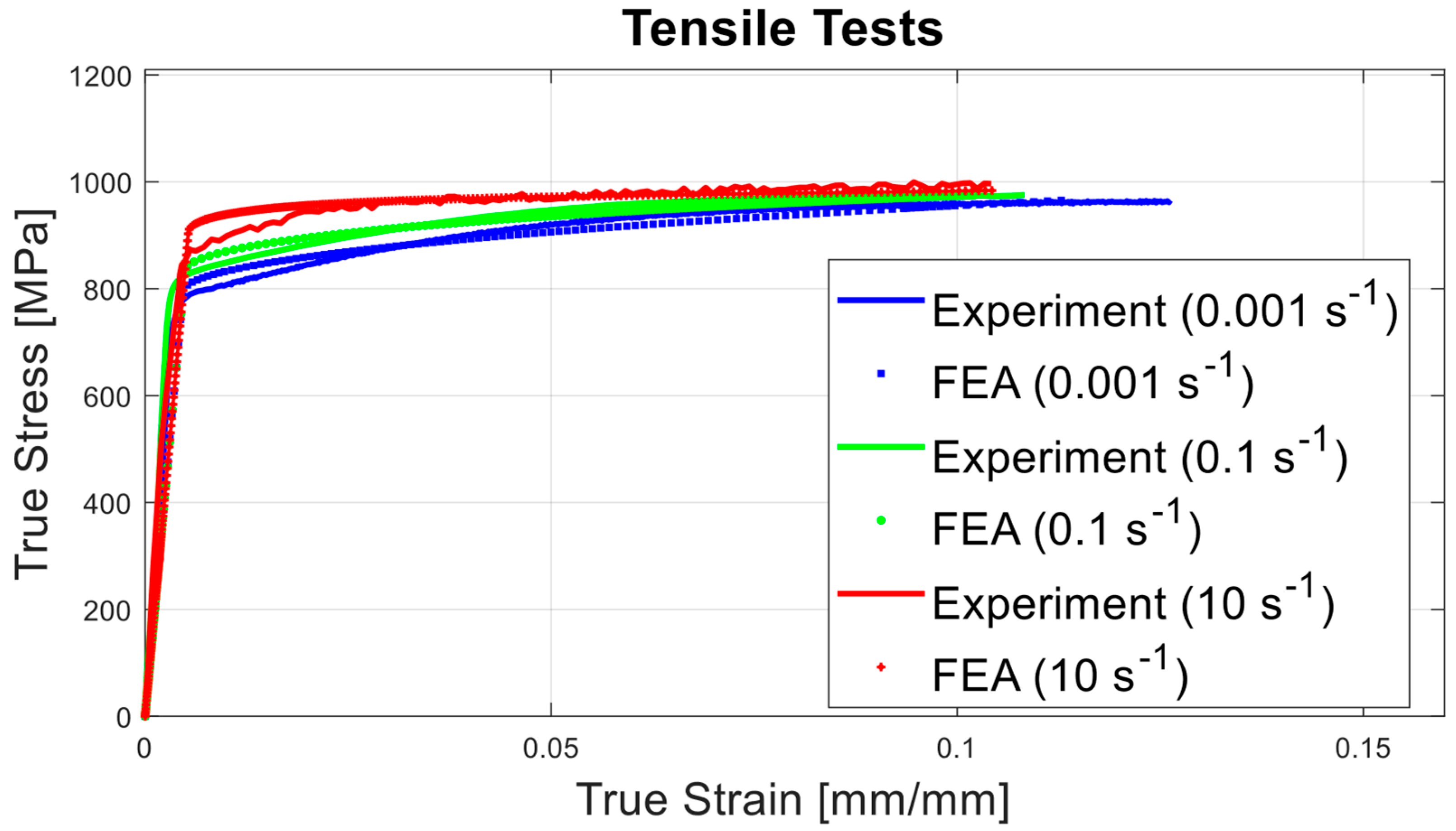
4.3. FEA Models
5. Conclusions
- The G18NiCrMo3-6 material possess an asymmetric yielding character, in other words, it yields stress in compression at a rate 4% bigger than the tensile one.
- Indeed, the strain hardening and SRS character is also quite asymmetric for the studied material. This fact was quantified with the help of power-law-based flow stress formulation and the determination of the m parameter. From a general point-of-view, SRS is more dominant in the tensile direction; however, it has a decreasing tendency with increasing strain as in the compressive stress states. In the view of the authors’, the shared material data within this study provide an important resource for the upcoming research activities on G18NiCrMo3-6 about which the existing literature data are extremely limited.
- Unlike the tensile test in a medium-strain rate regime, strain softening phenomena were observed in compression tests which were interpreted as the effect of adiabatic heating. Owing to this finding on the compression side, the material exhibits a rate sensitivity character up to a certain strain value but then the strain softening effect contributes to the plastic response. This fact can be handled through defining SRS parameters as a function of strain as in the proposed constitutive model. On the contrary, a conventional Johnson–Cook type formalism cannot catch up with these phenomena where the effect of rate contribution is formulated through a constant multiplier term.
- The created constitutive model (UMAT file) runs without any problem which was formulated on a finite strain basis and uses implicit time integration scheme. This UMAT file could easily serve in inspecting the effect of material parameters (like the initial void volume fraction, etc.) on the macro-mechanical performance of any design which is made up of G18NiCrMo3-6. However, it is also noteworthy that in the proposed model, the void growth is just linked to the volumetric strains, meaning that any void coalescence effect is not included in the formulation. Indeed, this fact may also give rise to precision loss especially in a low-stress triaxiality regime.
- In our view, the present material model and the verified parameter set significantly enhance the technical knowledge level related to the G18NiCrMo3-6 material and would serve as a solid basis for the upcoming research studies. Our efforts will focus on creating proper coupling between the estimated void volume fraction and any appropriate damage rule to improve the proposed model which would account for both damage, void coalescence, and localization phenomena. Furthermore, plasticity-induced heating would also be studied to estimate the strain softening behavior via proper thermo-coupled plasticity formulations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| FEA | Finite element analysis |
| SRS | Strain rate sensitivity |
| UMAT | User subroutine to define a material’s mechanical behavior |
| UTS | Ultimate tensile strength |
References
- Drucker, D.C.; Prager, W. Soil mechanics and plastic analysis or limit design. Q. Appl. Math. 1952, 10, 157–165. [Google Scholar] [CrossRef]
- Gurson, A.L. Continuum Theory of Ductile Rupture by Void Nucleation and Growth: Part I—Yield Criteria and Flow Rules for Porous Ductile Media. J. Eng. Mater. Technol. 1977, 99, 2–15. [Google Scholar] [CrossRef]
- Trillat, M.; Pastor, J. Limit analysis and Gurson’s model. Eur. J. Mech. A/Solids 2005, 24, 800–819. [Google Scholar] [CrossRef]
- Coffin, L.F.J. The Flow and Fracture of a Brittle Material. J. Appl. Mech. 2021, 17, 233–248. [Google Scholar] [CrossRef]
- Wiese, J.W.; Dantzig, J.A. Modeling stress development during the solidification of gray iron castings. Metall. Trans. A 1990, 21, 489–497. [Google Scholar] [CrossRef]
- Hjelm, H.E. Yield Surface for Grey Cast Iron Under Biaxial Stress. J. Eng. Mater. Technol. 1994, 116, 148–154. [Google Scholar] [CrossRef]
- Altenbach, H.; Stoychev, G.; Tushtev, K. On elastoplastic deformation of grey cast iron. Int. J. Plast. 2001, 17, 719–736. [Google Scholar] [CrossRef]
- Hosseini, E.; Holdsworth, S.R.; Flueeler, U. A temperature-dependent asymmetric constitutive model for cast irons under cyclic loading conditions. J. Strain Anal. Eng. Des. 2018, 53, 106–114. [Google Scholar] [CrossRef]
- Smith, M. ABAQUS/Standard User’s Manual, Version 6.9; Dassault Systèmes Simulia Corp: Dearborn, MI, USA, 2009.
- Josefson, B.L.; Hjelm, H.E. Modelling Elastoplastic Deformations in Grey Cast Iron. In Low Cycle Fatigue and Elasto-Plastic Behaviour of Materials—3; Springer: Dordrecht, The Netherlands, 1992; pp. 465–472. [Google Scholar] [CrossRef]
- Metzger, M.; Seifert, T. Computational assessment of the microstructure-dependent plasticity of lamellar gray cast iron—Part II: Initial yield surfaces and directions. Int. J. Solids Struct. 2015, 66, 194–206. [Google Scholar] [CrossRef]
- Andriollo, T.; Thorborg, J.; Tiedje, N.S.; Hattel, J. Modeling of damage in ductile cast iron—The effect of including plasticity in the graphite nodules. IOP Conf. Ser. Mater. Sci. Eng. 2015, 84, 012027. [Google Scholar] [CrossRef]
- Pina, J.; Shafqat, S.; Kouznetsova, V.; Hoefnagels, J.; Geers, M. Microstructural study of the mechanical response of compacted graphite iron: An experimental and numerical approach. Mater. Sci. Eng. A 2016, 658, 439–449. [Google Scholar] [CrossRef]
- Fernandino, D.O.; Cisilino, A.P.; Boeri, R.E. Determination of effective elastic properties of ferritic ductile cast iron by computational homogenization, micrographs, and microindentation tests. Mech. Mater. 2015, 83, 110–121. [Google Scholar] [CrossRef]
- Brauer, S.A.; Whittington, W.R.; Johnson, K.L.; Li, B.; Rhee, H.; Allison, P.G.; Crane, C.K.; Horstemeyer, M.F. Strain Rate and Stress-State Dependence of Gray Cast Iron. J. Eng. Mater. Technol. 2017, 139, 021013. [Google Scholar] [CrossRef]
- Cocks, A. Inelastic deformation of porous materials. J. Mech. Phys. Solids 1989, 37, 693–715. [Google Scholar] [CrossRef]
- Yalçinkaya, T.; Erdoğan, C.; Tandoğan, I.T.; Cocks, A. Formulation and Implementation of a New Porous Plasticity Model. Procedia Struct. Integr. 2019, 21, 46–51. [Google Scholar] [CrossRef]
- Erdoğan, C. Numerical Implementation and Analysis of a Porousplasticity Model for Ductile Damage Prediction. Ph.D. Thesis, Middle East Technical University, Ankara, Turkey, January 2021. [Google Scholar]
- Gul, A.; Aslan, O.; Kayali, E.S.; Bayraktar, E. Assessing Cast Aluminum Alloys with Computed Tomography Defect Metrics: A Gurson Porous Plasticity Approach. Metals 2023, 13, 752. [Google Scholar] [CrossRef]
- Maier, V.; Durst, K.; Mueller, J.; Backes, B.; Höppel, H.W.; Göken, M. Nanoindentation strain-rate jump tests for determining the local strain-rate sensitivity in nanocrystalline Ni and ultrafine-grained Al. J. Mater. Res. 2011, 26, 1421–1430. [Google Scholar] [CrossRef]
- Ghosh, A. On the measurement of strain-rate sensitivity for deformation mechanism in conventional and ultra-fine grain alloys. Mater. Sci. Eng. A 2007, 463, 36–40. [Google Scholar] [CrossRef]
- Acharya, S.; Gupta, R.; Ghosh, J.; Bysakh, S.; Ghosh, K.; Mondal, D.; Mukhopadhyay, A. High strain rate dynamic compressive behavior of Al6061-T6 alloys. Mater. Charact. 2017, 127, 185–197. [Google Scholar] [CrossRef]
- Kumaresan, G.; Kalaichelvan, K. Multi-dome forming test for determining the strain rate sensitivity index of a superplastic 7075Al alloy sheet. J. Alloys Compd. 2014, 583, 226–230. [Google Scholar] [CrossRef]
- Nemes, J.A.; Eftis, J.; Randles, P.W. Viscoplastic Constitutive Modeling of High Strain-Rate Deformation, Material Damage, and Spall Fracture. J. Appl. Mech. 1990, 57, 282–291. [Google Scholar] [CrossRef]
- Hao, S.; Brocks, W. The Gurson-Tvergaard-Needleman-model for rate and temperature-dependent materials with isotropic and kinematic hardening. Comput. Mech. 1997, 20, 34–40. [Google Scholar] [CrossRef]
- Fan, H.; Li, Y.; Jin, X.; Chen, B. Effect of tempering temperature on microstructure and mechanical properties of G18NiMoCr3-6. Jinshu Rechuli/Heat Treat. Met. 2017, 42, 163–165. [Google Scholar] [CrossRef]
- Salemi, A.; Abdollah-zadeh, A. The effect of tempering temperature on the mechanical properties and fracture morphology of a NiCrMoV steel. Mater. Charact. 2008, 59, 484–487. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Fried, E.; Anand, L. The Mechanics and Thermodynamics of Continua; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar] [CrossRef]
- Panicker, S.S.; Panda, S.K. Formability Analysis of AA5754 Alloy at Warm Condition: Appraisal of Strain Rate Sensitive Index. Mater. Today Proc. 2015, 2, 1996–2004. [Google Scholar] [CrossRef]
- Hencky, H. The Elastic Behavior of Vulcanized Rubber. J. Appl. Mech. 2021, 1, 45–48. [Google Scholar] [CrossRef]
- Kröner, E. Allgemeine Kontinuumstheorie der Versetzungen und Eigenspannungen. Arch. Ration. Mech. Anal. 1959, 4, 273–334. [Google Scholar] [CrossRef]
- Anand, L.; On, H. Hencky’s Approximate Strain-Energy Function for Moderate Deformations. J. Appl. Mech. 1979, 46, 78–82. [Google Scholar] [CrossRef]
- Ludwik, P. Elemente der Technologischen Mechanik; Springer: Berlin/Heidelberg, Germany, 1909. [Google Scholar] [CrossRef]
- Sorini, C.; Chattopadhyay, A.; Goldberg, R.K. Micromechanical modeling of the effects of adiabatic heating on the high strain rate deformation of polymer matrix composites. Compos. Struct. 2019, 215, 377–384. [Google Scholar] [CrossRef]
- Soares, G.; Patnamsetty, M.; Peura, P.; Hokka, M. Effects of Adiabatic Heating and Strain Rate on the Dynamic Response of a CoCrFeMnNi High-Entropy Alloy. J. Dyn. Behav. Mater. 2019, 5, 320–330. [Google Scholar] [CrossRef]
- Nasraoui, M.; Forquin, P.; Siad, L.; Rusinek, A. Influence of strain rate, temperature, and adiabatic heating on the mechanical behavior of poly-methyl-methacrylate: Experimental and modeling analyses. Mater. Des. 2012, 37, 500–509. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. A Constitutive Model and Data for Metals Subjected to Large Strains, High Strain Rates, and High Temperatures. In Proceedings of the 7th International Symposium on Ballistics, The Hague, The Netherlands, 19–21 April 1983; Volume 21, pp. 541–547. [Google Scholar]
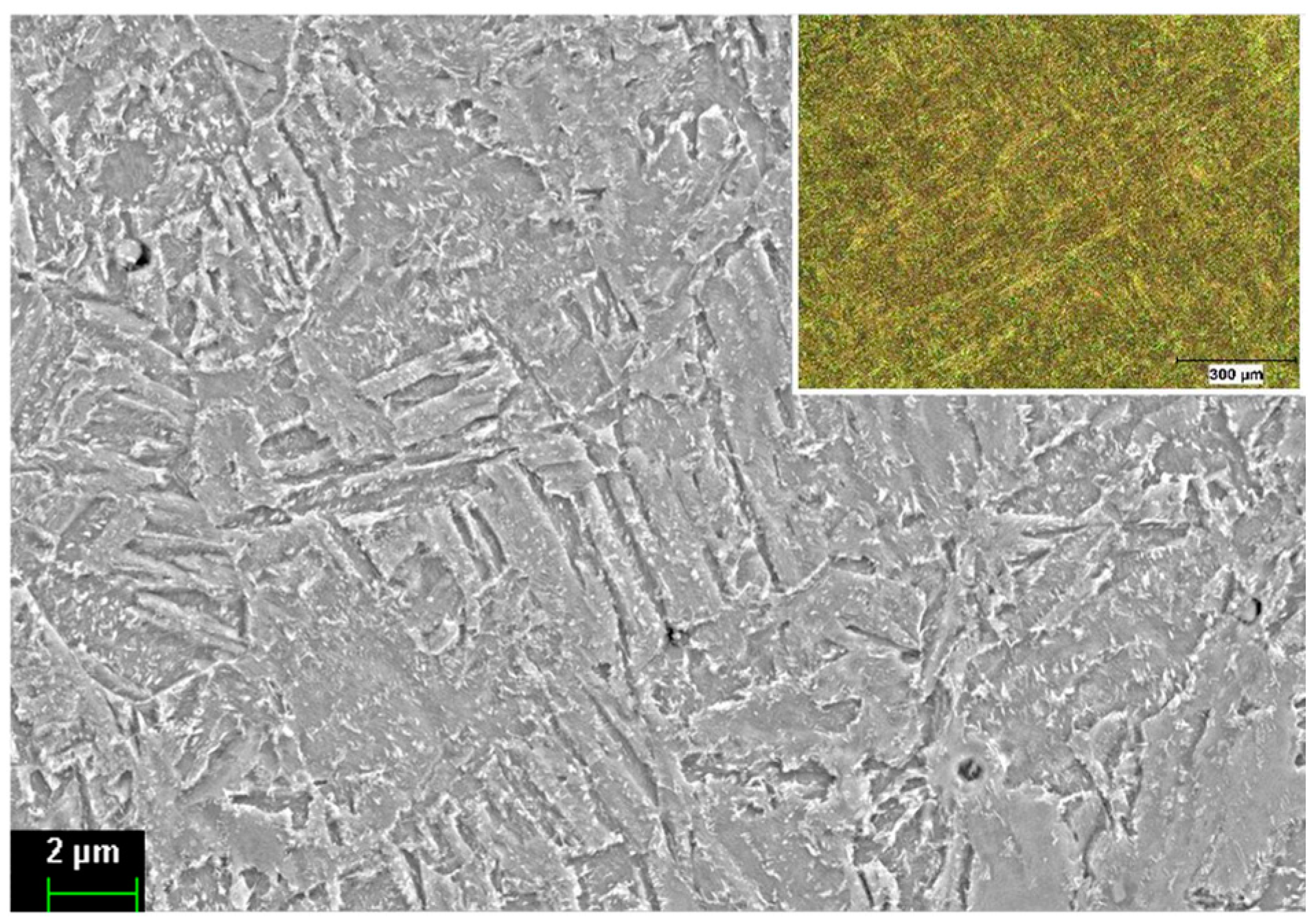
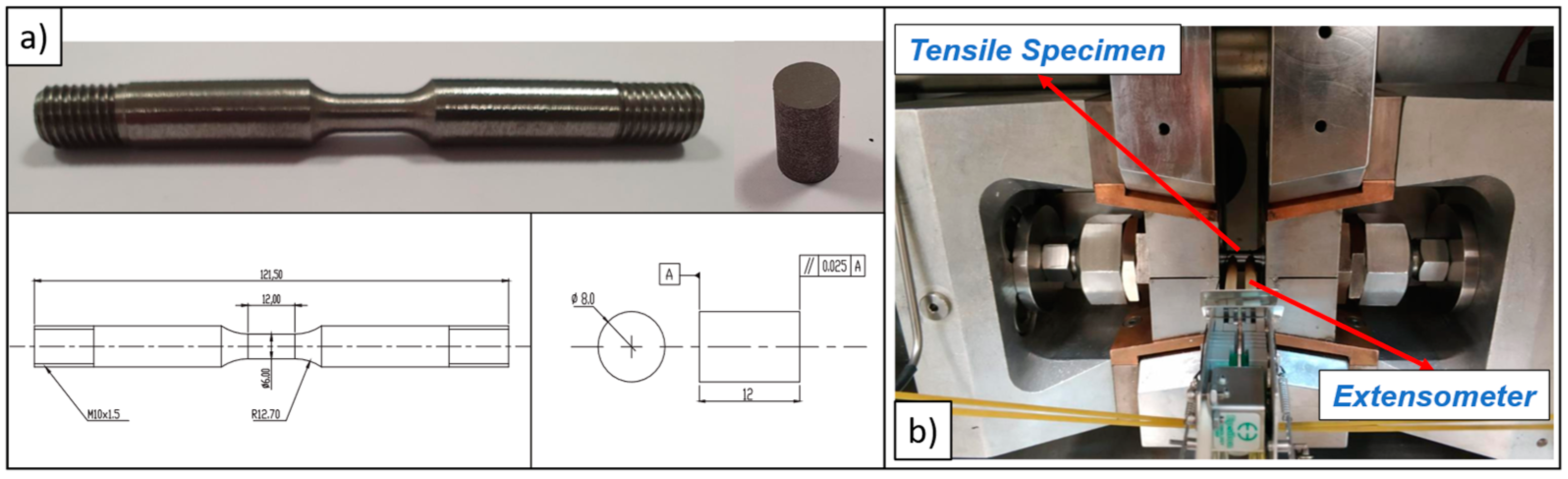

| C | Si | Mn | Ni | Cr | Mo | Cu | Fe |
|---|---|---|---|---|---|---|---|
| 0.20 | 0.50 | 0.90 | 0.80 | 0.50 | 0.45 | 0.15 | Balance |
| B | n | ||||
|---|---|---|---|---|---|
| Compression | 815.64 (±1.93%) | 862.79 (±1.89%) | 882.18 (±2.89%) | 368.20 | 0.2798 |
| Tension | 808.65 (±2.70%) | 858.73 (±2.84%) | 876.80 (±1.36%) | 551.30 | 0.5075 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Çetin, B.; Bayraktar, E.; Aslan, O. Unveiling the Strain Rate Sensitivity of G18NiCrMo3-6 CAST Steel in Tension/Compression Asymmetry. Appl. Sci. 2023, 13, 11891. https://doi.org/10.3390/app132111891
Çetin B, Bayraktar E, Aslan O. Unveiling the Strain Rate Sensitivity of G18NiCrMo3-6 CAST Steel in Tension/Compression Asymmetry. Applied Sciences. 2023; 13(21):11891. https://doi.org/10.3390/app132111891
Chicago/Turabian StyleÇetin, Barış, Emin Bayraktar, and Ozgur Aslan. 2023. "Unveiling the Strain Rate Sensitivity of G18NiCrMo3-6 CAST Steel in Tension/Compression Asymmetry" Applied Sciences 13, no. 21: 11891. https://doi.org/10.3390/app132111891
APA StyleÇetin, B., Bayraktar, E., & Aslan, O. (2023). Unveiling the Strain Rate Sensitivity of G18NiCrMo3-6 CAST Steel in Tension/Compression Asymmetry. Applied Sciences, 13(21), 11891. https://doi.org/10.3390/app132111891








