A Fully Automatic DEXP Method for Gravity Data and Its Application on a Potash Salt Deposit
Abstract
1. Introduction
2. Methodology
2.1. Theory of the Automatic DEXP Mehod
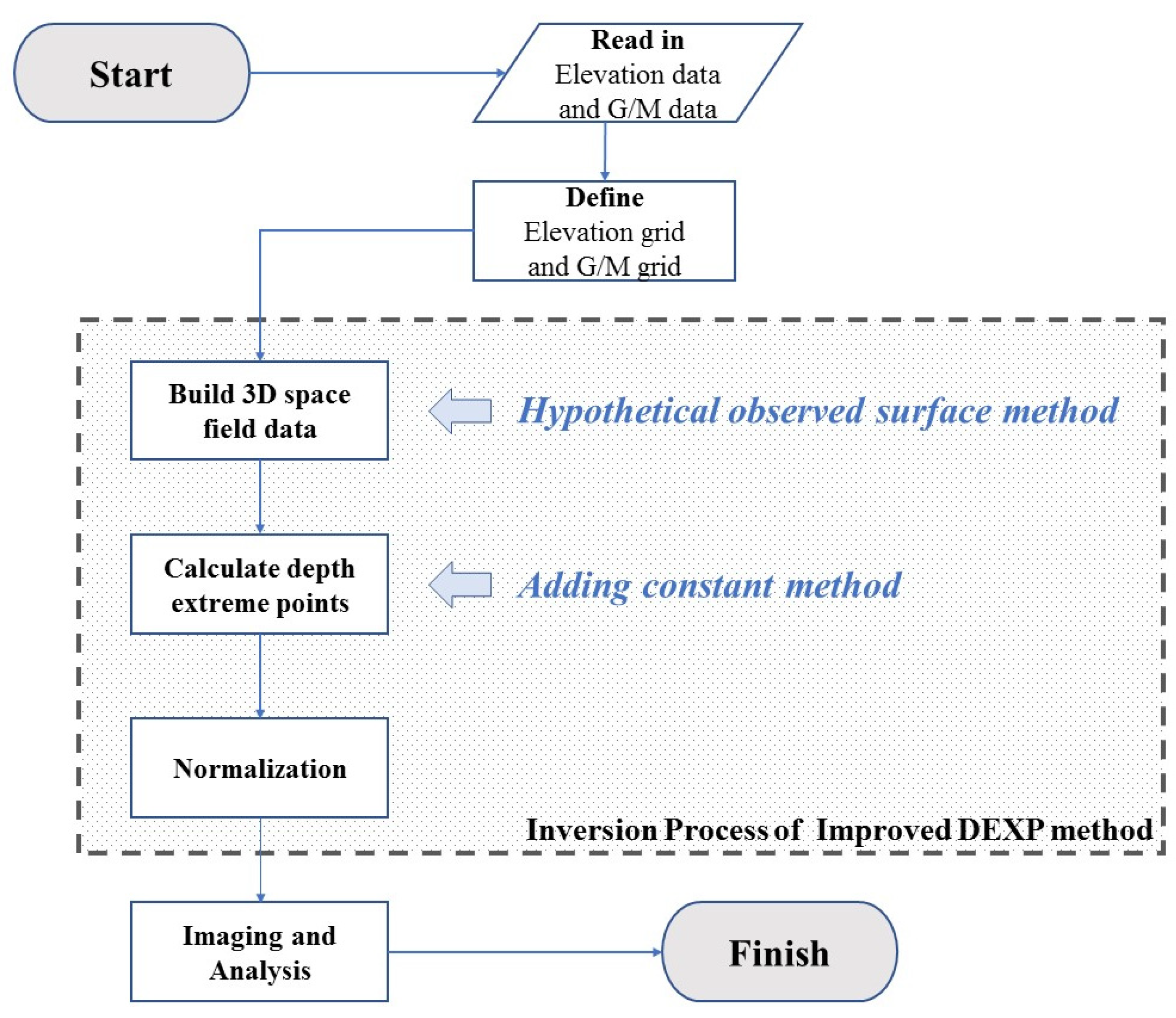
2.2. Theory of the Improved DEXP Method
2.2.1. Basic Equation
2.2.2. The Adding Constant Method
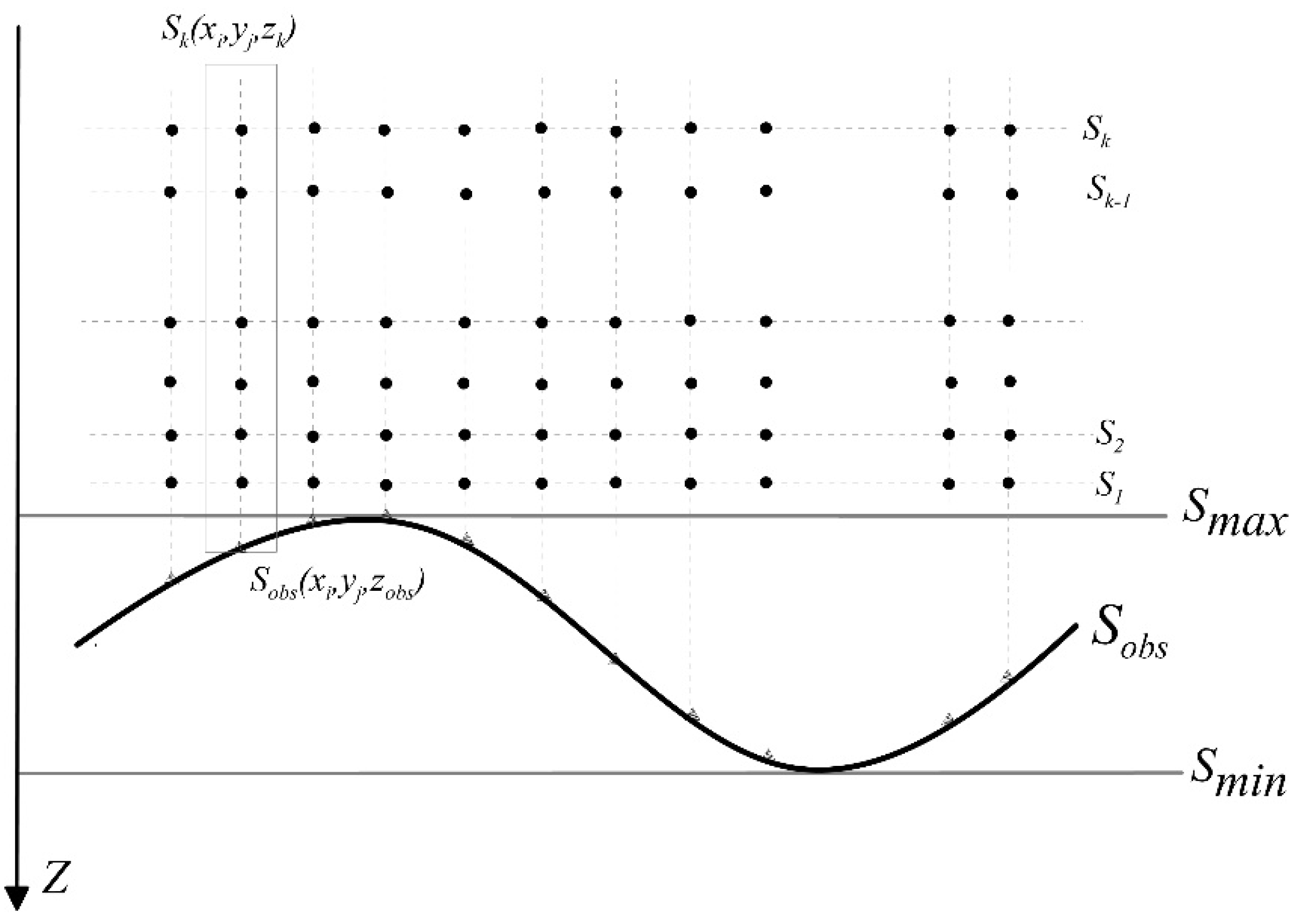
2.2.3. Hypothetical Observed Surface Method
3. Application of Synthetic Data
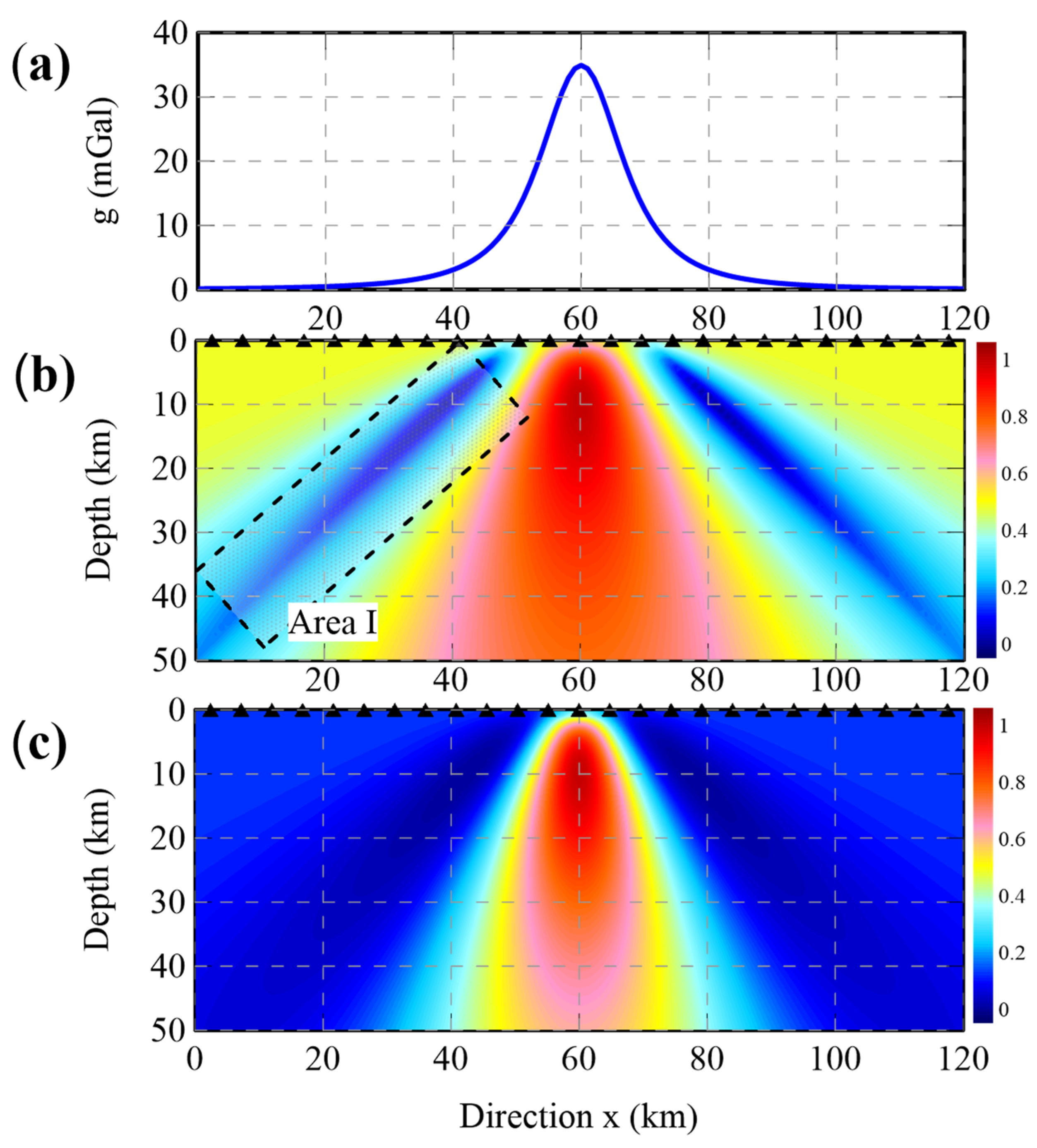
3.1. Imaging Results for Flat Terrain
3.2. Imaging Result for Undulating Terrain
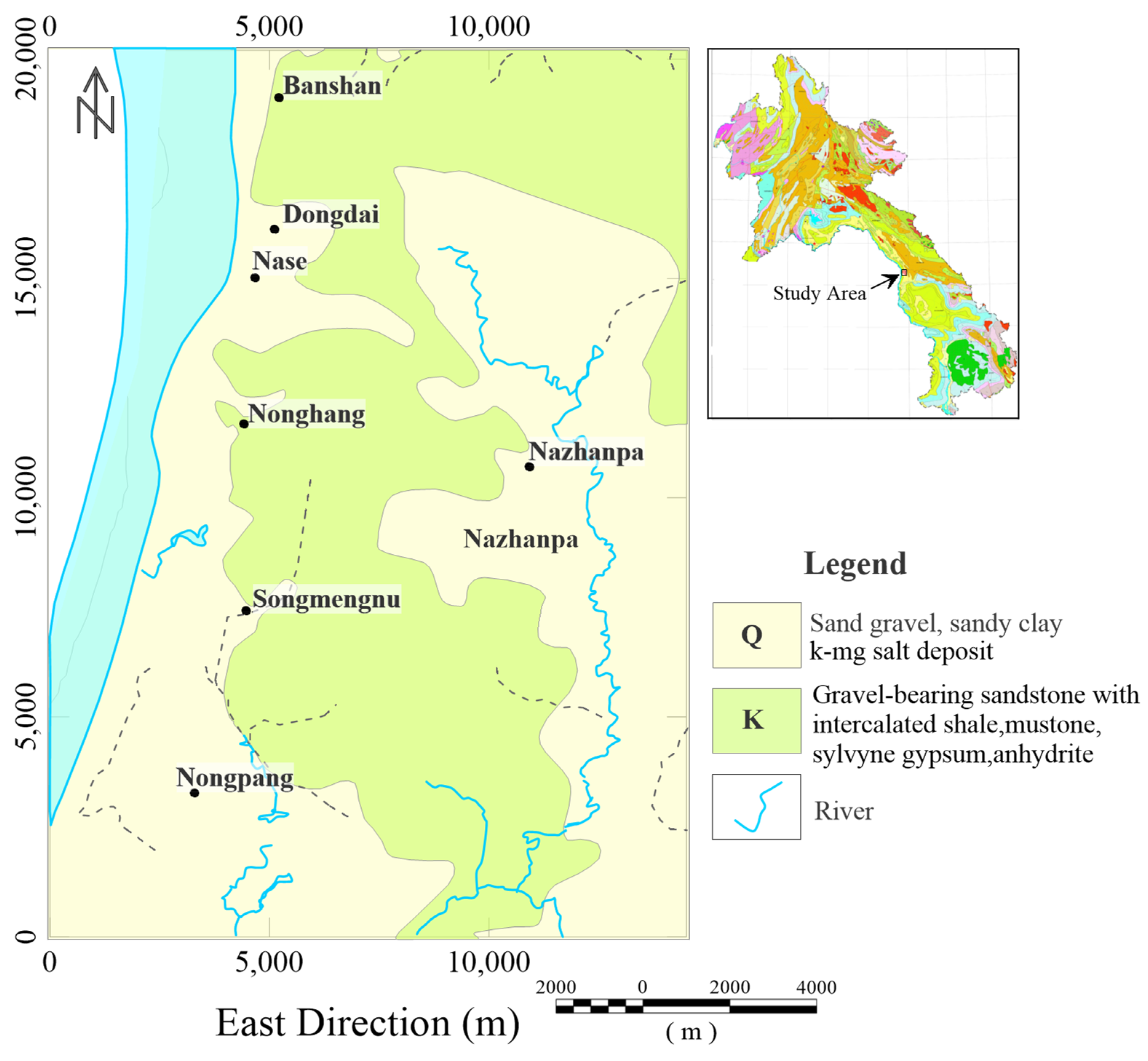
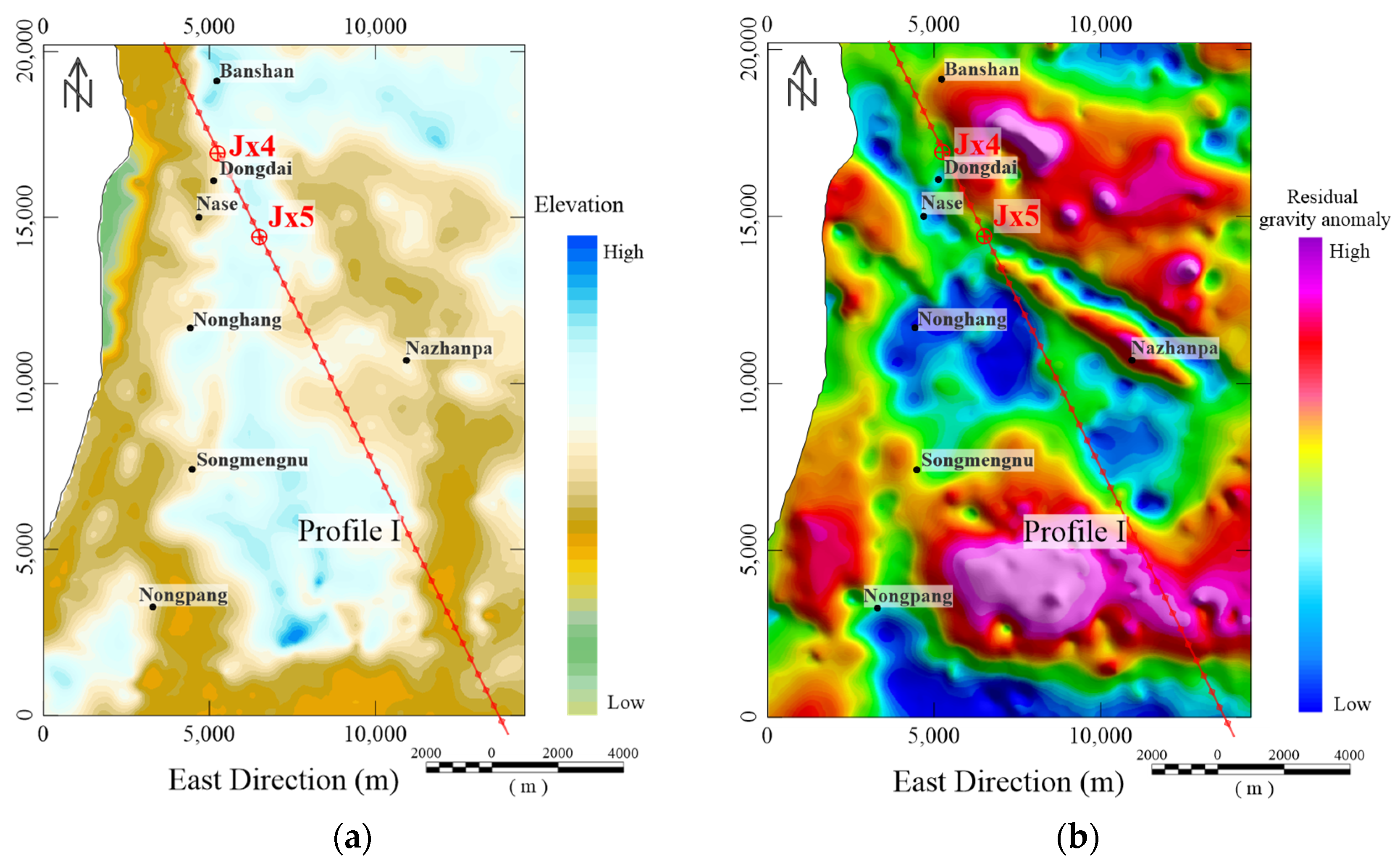
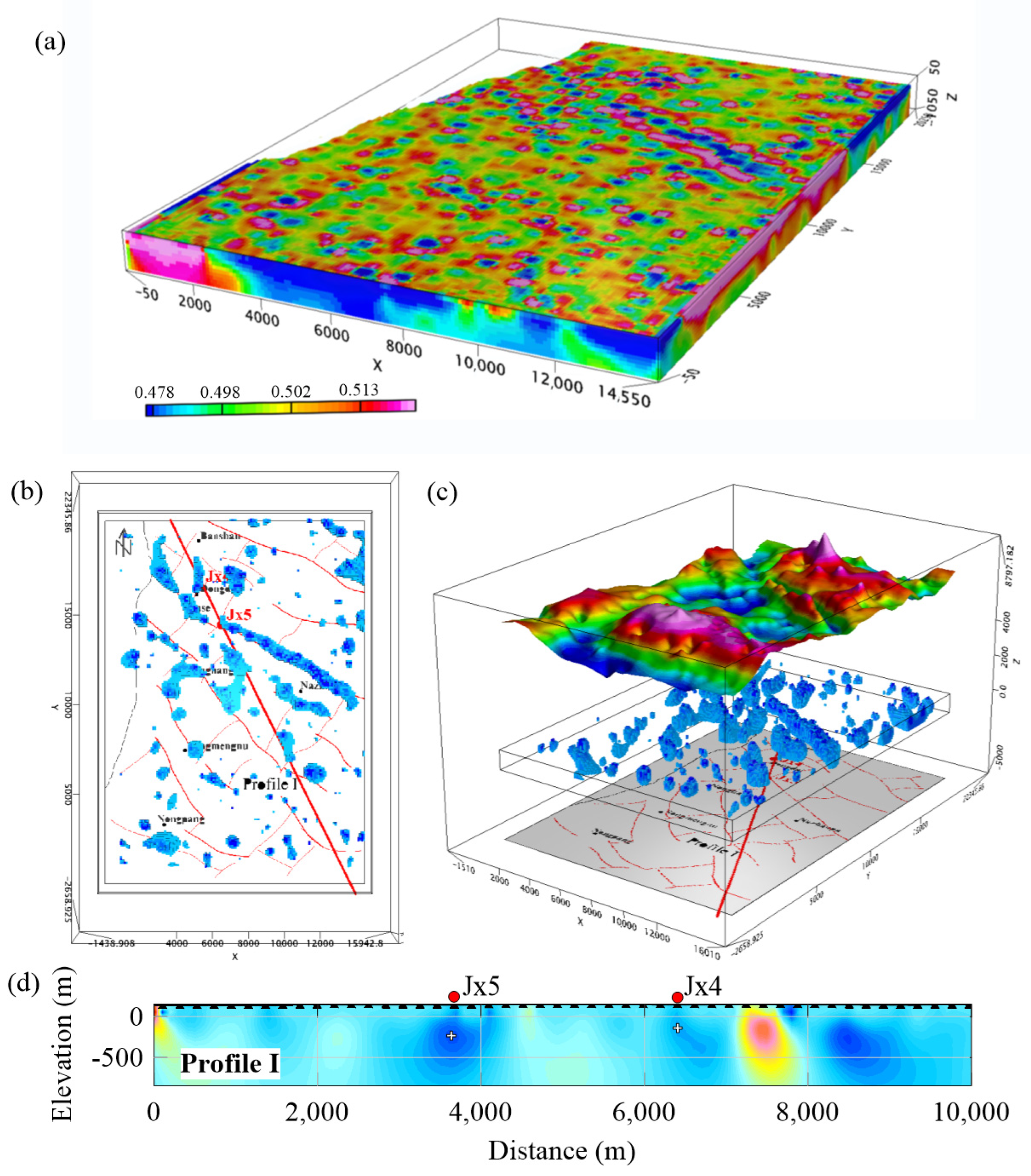
4. Application on Real Gravity Data
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Patella, D. Introduction to ground surface self-potential tomography. Geophys. Prospect. 1997, 45, 653–681. [Google Scholar] [CrossRef]
- Mauriello, P.; Patella, D. Gravity probability tomography: A new tool for buried mass distribution imaging. Geophys. Prospect. 2001, 49, 1–12. [Google Scholar] [CrossRef]
- Cui, X. Research on Probability Tomography of Geophysical Field. Master’s Thesis, Chinese University Geoscience, Beijing, China, 2009. [Google Scholar]
- Guo, L.H.; Meng, X.H.; Shi, L. 3-D correlation imaging for gravity and gravity gradiometry data. Chin. J. Geophys. 2009, 52, 1098–1106. [Google Scholar] [CrossRef]
- Guo, L.H.; Meng, X.H.; Shi, L. 3-D correlation imaging for magnetic anomaly ΔT data. Chin. J. Geophys. 2010, 53, 435–441. [Google Scholar]
- Meng, X.H.; Liu, G.F.; Chen, Z.X.; Guo, L.-H. 3-D gravity and magnetic inversion for physical properties based on residual anomaly correlation. Chin. J. Geophys. 2012, 55, 304–309. [Google Scholar] [CrossRef]
- Ma, G.Q.; Du, X.J.; Li, L.L. Improved Potential field Correlation Imaging method. Earth Sci. 2013, 38, 1121–1127. [Google Scholar]
- Zhdanov, M.S. Geophysical Inverse Theory and Regularization Problems; Elsevier Press: Amsterdam, The Netherlands, 2002. [Google Scholar]
- Fedi, M. DEXP: A fast method to determine the depth and the structural index of potential fields sources. Geophysics 2007, 72, I1–I11. [Google Scholar] [CrossRef]
- Zhao, G.X.; Wu, Y.T.; Sun, Y.; Zhang, B.B.; Zhou, X.; Wang, F.-J. Automatic DEXP method derived from Euler’s Homogeneity equation. Appl. Geophys. 2023, 1–8. [Google Scholar] [CrossRef]
- Florio, G.; Fedi, M.; Rapolla, A. Interpretation of regional aeromagnetic data by the scaling function method: The case of Southern Apennines Italy. Geophys. Prospect. 2009, 57, 479–489. [Google Scholar] [CrossRef]
- Moreau, F.; Gibert, D.; Holschneider, M.; Saracco, G. Wavelet analysis of potential fields. Inverse Probl. 1997, 13, 165–178. [Google Scholar] [CrossRef]
- Hornby, P.; Boschetti, F.; Horowitz, F.G. Analysis of potential field data in the wavelet domain. Geophys. J. Int. 1999, 137, 175–196. [Google Scholar] [CrossRef]
- Fedi, M.; Cella, F.; Quarta, T.; Villani, A. 2D Continuous Wavelet Transform of potential fields due to extended source distributions. Appl. Comput. Harmon. Anal. 2010, 283, 320–337. [Google Scholar] [CrossRef]
- Sailhac, P.; Gibert, D. Identification of sources of potential fields with the continuous wavelet transform: Two-dimensional wavelets and multipolar approximations. J. Geophys. Res. Solid Earth 2003, 108, 19455–19475. [Google Scholar] [CrossRef]
- Vallée, M.A.; Keating, P.; Smith, R.S.; St-Hilaire, C. Estimating depth and model type using the continuous wavelet transform of magnetic data. Geophysics 2004, 69, 191–199. [Google Scholar] [CrossRef]
- Antoine, J.P.; Murenzi, R.; Vandergheynst, P.; Ali, S.T. Two-Dimensional Wavelets and Their Relatives; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Chen, Y.D. Inversion of gravity anomaly with continuous complex potential wavelet transform. Geophys. Geochem. Explor. 2003, 5, 354–361. [Google Scholar]
- Chen, Y.D. The identification of gravity anomaly with continuous complex potential wavelet transform. Geophys. Geochem. Explor. 2006, 2, 141–147. [Google Scholar]
- Fedi, M.; Pilkington, M. Understanding imaging methods for potential field data. Geophysics 2012, 77, G13–G24. [Google Scholar] [CrossRef]
- Abbas, M.A.; Fedi, M.; Florio, G. Improving the local wavenumber method by automatic DEXP transformation. J. Appl. Geophys. 2014, 111, 250–255. [Google Scholar] [CrossRef]
- Fedi, M.; Abbas, M.A. A fast interpretation of self-potential data using the depth from extreme points method. Geophysics 2013, 78, E107–E116. [Google Scholar] [CrossRef]
- Fedi, M.; Florio, G. Determination of the maximum-depth to potential field sources by a maximum structural index method. J. Appl. Geophys. 2013, 88, 154–160. [Google Scholar] [CrossRef]
- Cella, F.; Fedi, M.; Florio, G. Toward a full multiscale approach to interpret potential fields. Geophys. Prospect. 2009, 57, 543–557. [Google Scholar] [CrossRef]
- Abbas, M.A.; Fedi, M. Automatic DEXP imaging of potential fields independent of the structural index. Geophys. J. Int. 2014, 199, 1625–1632. [Google Scholar] [CrossRef]
- Henderson, R.G.; Cordell, L. Reduction of unevenly spaced potential field data to a horizontal plane by means of finite harmonic series. Geophysics 1971, 36, 856–866. [Google Scholar] [CrossRef]
- Naidu, P.S.; Mathew, M.P. Fast reduction of potential field measured over an uneven surface to a plane surface. IEEE Trans. Geosci. Remote Sens. 1994, 32, 508–512. [Google Scholar] [CrossRef][Green Version]
- Wang, Z.M.; Zhang, X.F. Theory of 3D potential field upward continuation observed with random undulated terrain. J. Jilin Univ. Earth Sci. Ed. 1980, 1, 94–98. [Google Scholar]
- Huang, Y.H. Boundary Element Method in Infinite Domain and Terrain Corrections in Gravity-Magnetic Interpretation. J. Fuzhou Univ. Nat. Sci. Ed. 1989, 17, 15–21. [Google Scholar]
- Kuang, Y.P.; Zhang, X.L. The boundary element method for three-dimensional magnetic field data conversion of curved-surface to horizontal-plane. Comput. Technol. Geophys. Geochem. 2008, 30, 216–220. [Google Scholar]
- Emilia, D.A. Equivalent source used as analytic base for processing total magnetic field. Geophysics 1973, 38, 339–348. [Google Scholar] [CrossRef]
- Battacharyya, B.K.; Chen, K.C. Reduction of magnetic gravity data on an arbitrary surface acquired in a region of high topographic relief. Geophysics 1977, 42, 1141–1430. [Google Scholar] [CrossRef]
- Ji, X.L.; Wang, W.Y. Experimental research using the frequency-domain dipole layer method for the processing and transformation of potential field on curved surface. Prog. Geophys. 2014, 9, 2669–2678. [Google Scholar]
- Cordell, L.; Grauch, V. Mapping basement magnetization zones from aeromagnetic data in San Juan bansin, New Mexico. In The Utility of Regional Gravity and Magnetic Anomaly Maps; Society of Exploration Geophysics: Houston, TX, USA, 1985; pp. 181–197. [Google Scholar]
- Guspi, F. Frequency-domain reduction of potential field measurements to a horizontal plane. Geoexploration 1987, 24, 87–98. [Google Scholar] [CrossRef]
- Liu, J.L.; Wang, W.Y. Reduction of potential field data to a horizontal plane by a successive approximation procedure. Chin. J. Geophys. 2007, 50, 1551–1557. [Google Scholar]
- Guan, Z.N.; Zhang, P.Q.; Yao, C.L. A new rapid surface to plane method of 3-D potential field data in large amount. Annu. J. Chin. Geophys. Soc. 1999, 1. [Google Scholar]
- Wang, Y.G.; Zhang, F.X.; Wang, Z.W. Taylor series iteration for downward continuation of potential fields. Oil Geophys. Prospect. 2011, 46, 657–662. [Google Scholar]
- Xiao, F. Study on gravity data processing method and apply it to sylvite mine exploration. Ph.D. Thesis, Jilin University, Changchun, China, 2005. [Google Scholar]
- Xu, M.L.; Yang, C.B.; Wu, Y.G.; Chen, J.-Y. Edge detection in the potential field using the correlation coefficients of multidirectional standard deviations. Appl. Geophys. 2015, 12, 23–34. [Google Scholar] [CrossRef]
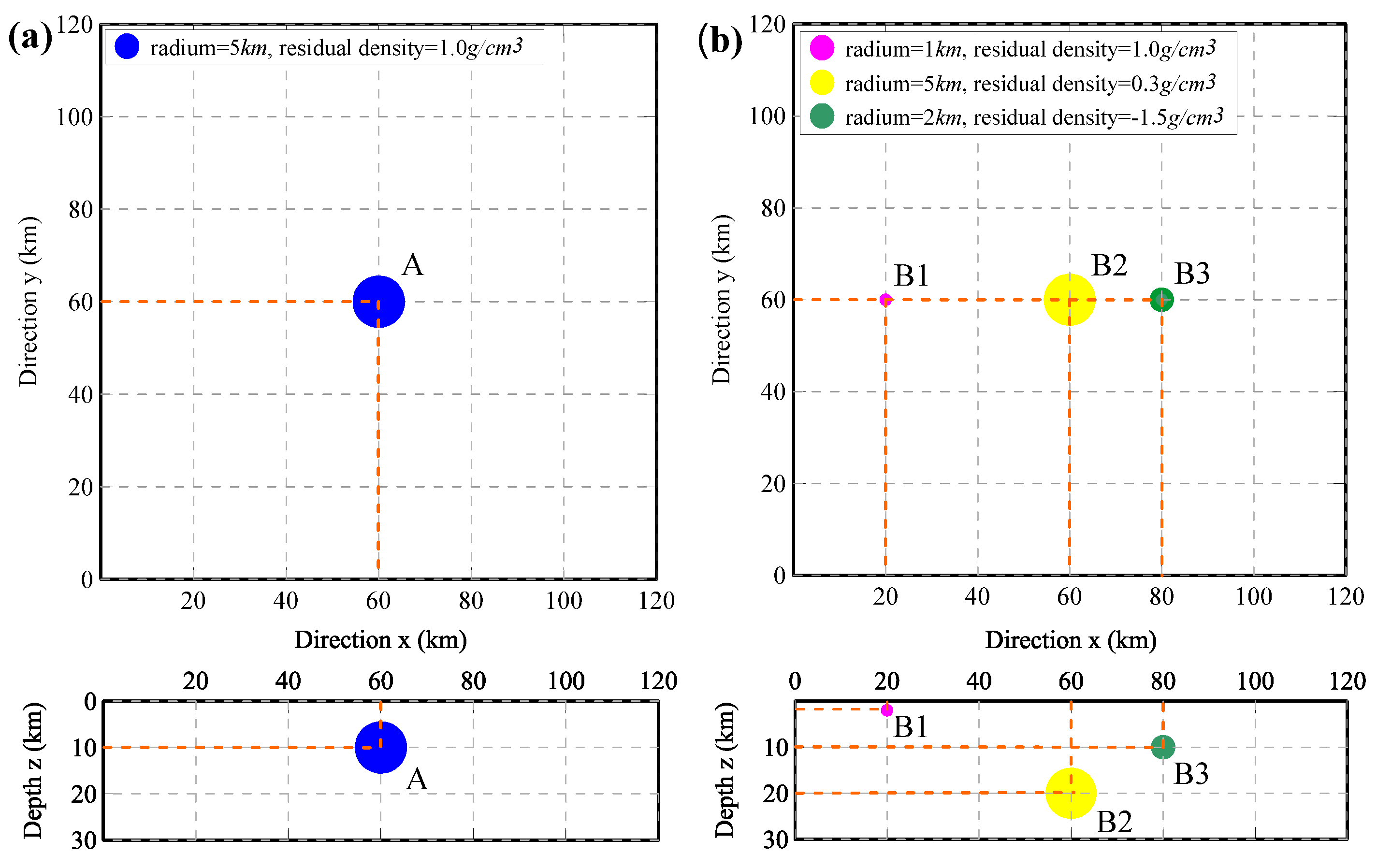

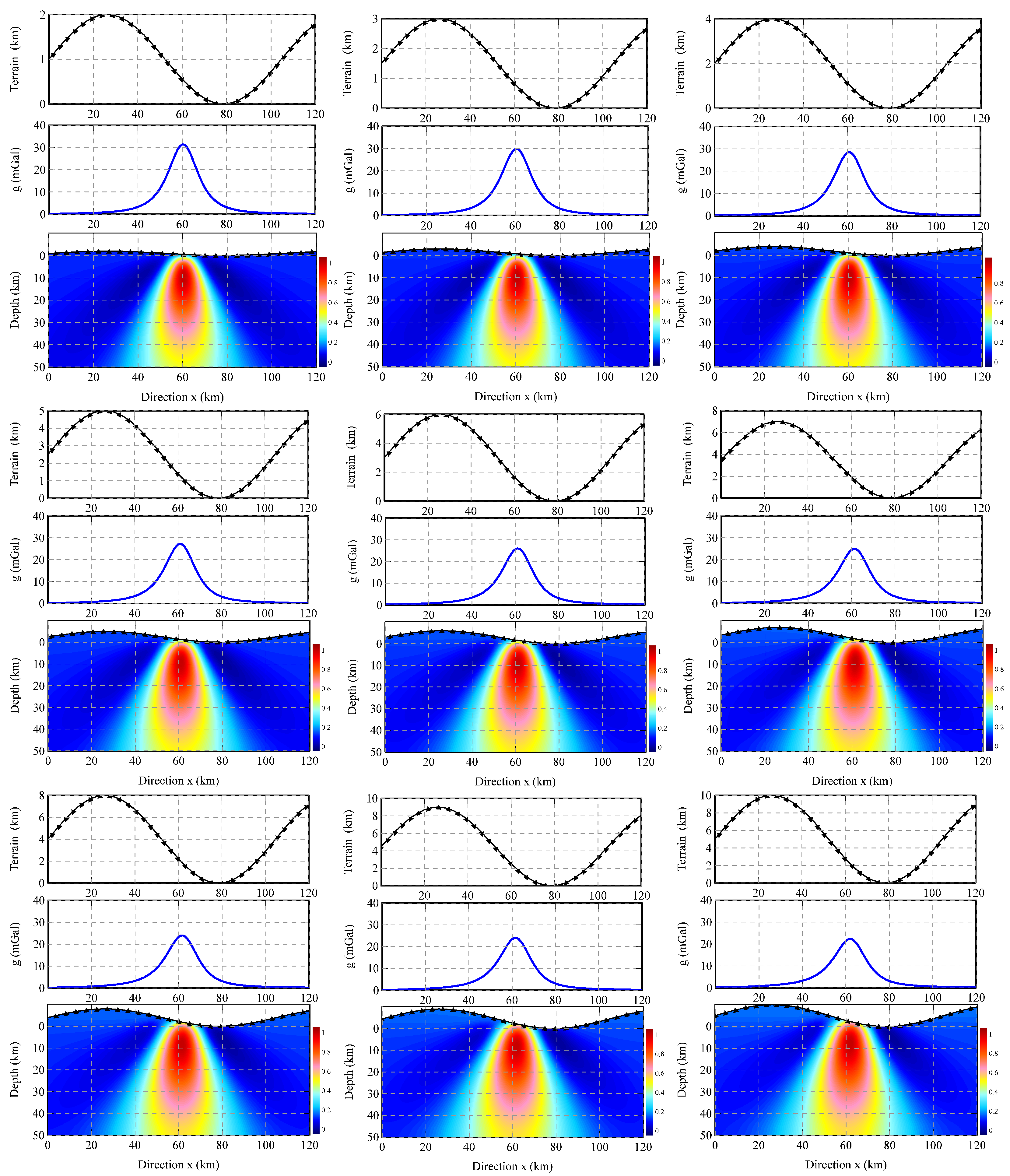
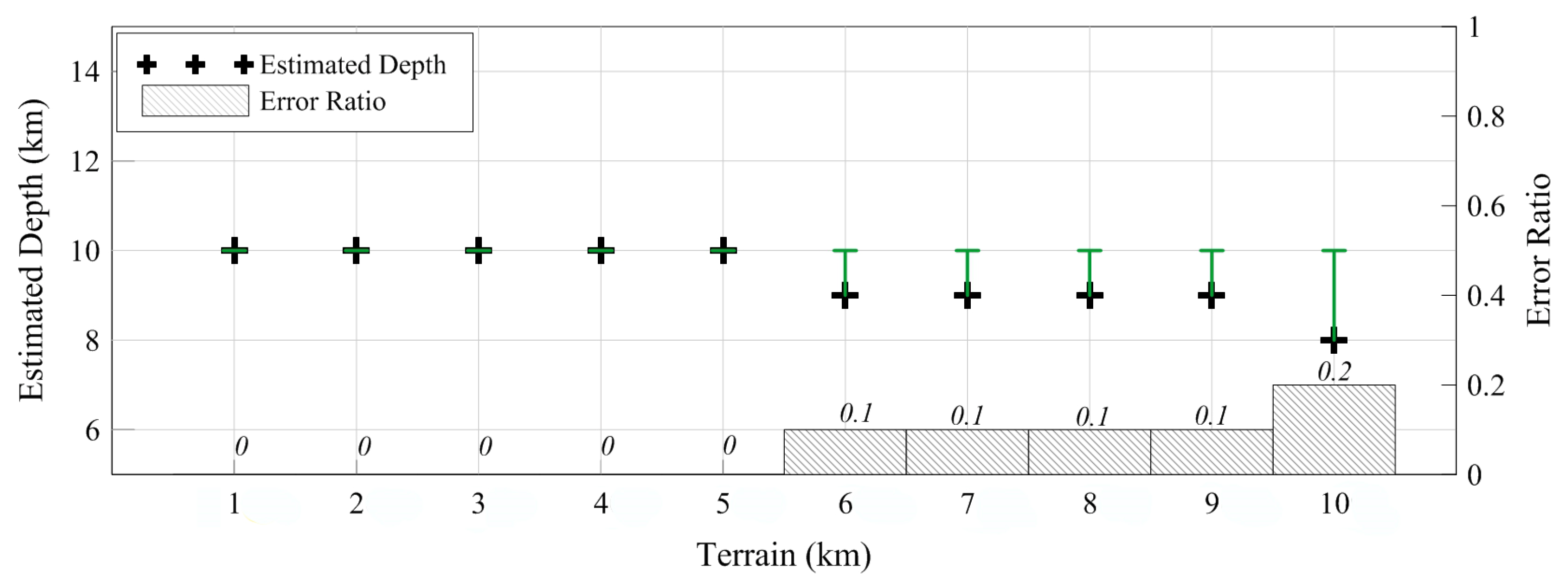
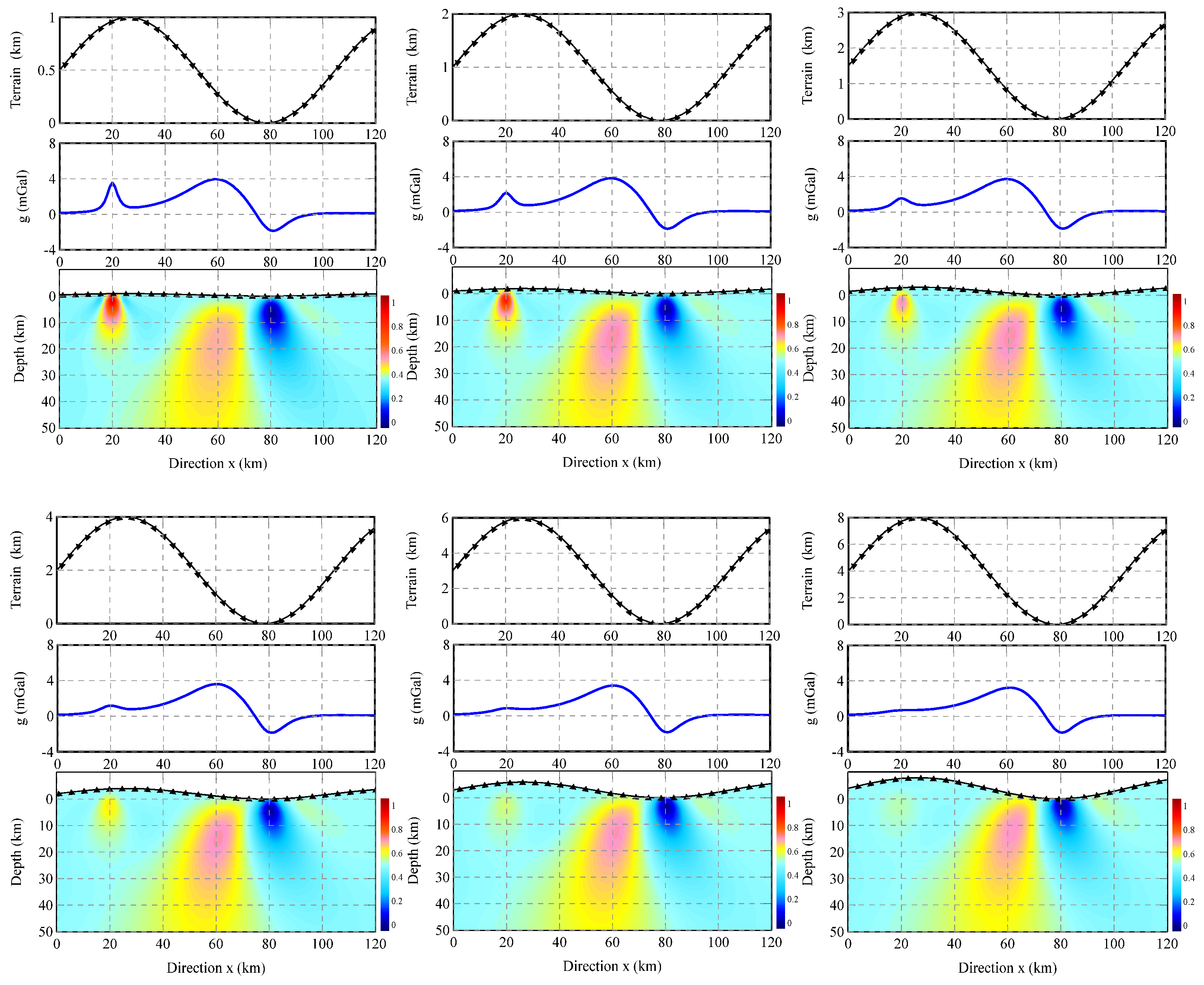


| Model | Location | Depth | Radium | Residual Density |
|---|---|---|---|---|
| A | x = 60 km, y = 60 km | 10 km | 5 km | 1.0 g/cm3 |
| B1 | x = 20 km, y = 60 km | 2 km | 1 km | 1.0 g/cm3 |
| B2 | x = 60 km, y = 60 km | 20 km | 5 km | 0.3 g/cm3 |
| B3 | x = 80 km, y = 60 km | 10 km | 2 km | −1.5 g/cm3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, M.; Yang, Y.; Wu, Y. A Fully Automatic DEXP Method for Gravity Data and Its Application on a Potash Salt Deposit. Appl. Sci. 2023, 13, 10821. https://doi.org/10.3390/app131910821
Xu M, Yang Y, Wu Y. A Fully Automatic DEXP Method for Gravity Data and Its Application on a Potash Salt Deposit. Applied Sciences. 2023; 13(19):10821. https://doi.org/10.3390/app131910821
Chicago/Turabian StyleXu, Menglong, Yabin Yang, and Yangang Wu. 2023. "A Fully Automatic DEXP Method for Gravity Data and Its Application on a Potash Salt Deposit" Applied Sciences 13, no. 19: 10821. https://doi.org/10.3390/app131910821
APA StyleXu, M., Yang, Y., & Wu, Y. (2023). A Fully Automatic DEXP Method for Gravity Data and Its Application on a Potash Salt Deposit. Applied Sciences, 13(19), 10821. https://doi.org/10.3390/app131910821





