Safety Evaluation of Crossing Tunnel Engineering: A Case Study

Abstract

With the growing demand for transportation, there has been a significant increase in road-related projects, leading to potential risks in the safety of existing structures. This paper presents a study on the influence of new tunnel construction on the stability of an adjacent existing tunnel and provides valuable insights for the safety technology evaluation of similar tunnel crossing projects. In order to evaluate the influence of tunnel excavation on adjacent tunnels, the excavation process of a new tunnel near the original tunnel is simulated using the finite element software Midas GTS. This analysis includes the evaluation of static and dynamic responses. The results indicate that the horizontal and vertical displacements caused by the excavation of the railway tunnel are minimal. Furthermore, during the blasting excavation of the railway tunnel, the vibration velocity experienced by the highway tunnel remains below the safe allowable limit. These findings demonstrate that in this project, the influence of blasting excavation for the railway tunnel on the highway tunnel is both safe and manageable.

1. Introduction

With the rapid development of China’s economy in recent years, there has been an increasing demand for transportation. In order to meet the transportation demands, a considerable number of new road projects are being implemented, resulting in a proliferation of existing roads intersecting or running parallel to one another, and leading to the rise of road-related projects [1]. The construction of road-related projects poses significant risks for existing road infrastructure, especially for road tunnels which require excavation and explosion [2,3]. During the construction process of such projects, blasting vibrations induced by new tunnel excavation may cause fissures, cracks or even collapse in medium and harder rocks [4,5,6,7], and cause ground collapse in strongly weathered rock mass [8,9,10], thereby compromising road safety. Consequently, it is of immense significance to conduct technical assessments on the safety of road-related projects [11].

On-site observation and monitoring is the most widely employed approach for evaluating the safety of such projects. By documenting deformation and failure characteristics before and during construction, the safety of the construction process can be effectively appraised [12,13,14,15]. The physical model test method shrinks the tunnel in equal proportion, and studies the stability of the project by reducing the true stress state of the surrounding rock [16,17,18]. By changing the inclination angle of the ground surface of a geotechnical model with a scale factor of 1/20, Lei [19] studied the change laws and distribution forms of structural stress and the failure mechanism of tunnel under different unsymmetrical pressures. However, both the on-site observation and physical models have drawbacks as are they costly and time-consuming [20]. The development of computer technology has led to the widespread adoption of numerical simulation in the field of safety assessment [21,22,23,24]. In the evaluation of tunnel support design, Kadir Yertutanol [25] employed a finite element numerical simulation analysis to predict the vertical displacements of a tunnel during its construction. Liang [1] investigated the effect of blast-induced vibration from a new railway tunnel on an existing adjacent railway tunnel. Asker [26] studied the interaction mechanism between a tunnel, protection technique, and soil. Various soft computing techniques involving data mining and machine learning have also found their application in the tunnelling-related problems [8]. Hybrid neural network approach is used to predict ground settlements induced by building shield tunneling [27]. In addition, artificial neural networks (ANNs), radial basis functions (RBFs), decision trees (DTs), random forest (RF) method, support vector machines (SVMs) are also wildly applied in the prediction of engineering response of tunnels and underground excavations [28,29,30,31,32,33]. To improve the accuracy of the safety determination, fuzzy analytical hierarchy process (FAHP) is introduced in the field of tunnel safety assessment, which has greater convenience and consumes less time [34]. However, the above studies evaluate the safety of tunnel construction from a single aspect, and fail to comprehensively evaluate the risks of tunnel construction from an engineering reality perspective.

This article takes the project of the Jijiapo highway tunnel overpass on the newly built Yangmuling railway tunnel as the engineering background. By combining laboratory experiments, on-site monitoring data, and numerical simulation methods, the blasting design and corresponding parameters are adjusted to mitigate the maximum vibration velocity resulting from the blasts and ensure construction safety. A numerical calculation model was established using the finite element numerical software Midas GTS. The influence of the new railway tunnel construction on the existing highway tunnel was analyzed from both static and dynamic responses. This study provides references and guidance for the safety evaluation of similar overpass road projects, which involve crossing highways and railways.

2. Geological Conditions

2.1. Geological Conditions of the Existing Highway Tunnel

The entrance of the existing Jijiapo tunnel is situated near Tao Jia Xi in San Dou Ping town, Yichang. The length of the tunnel is 3527 m and the maximum depth at which the tunnel is buried is approximately 390 m. Both the left and right tunnel have the same gradient of 1.6%; the design speed for driving through the tunnel is set at 80 km/h. The seismic intensity is represented by a peak ground motion acceleration of g = 0.05 g, and a characteristic period T = 0.35 s is taken into account for strengthening measures. Within the section spanning from ZK15 + 289 to ZK15 + 767, the horizontal distance between the highway tunnel and the railway tunnel is less than 40 m. The geological composition in this section primarily consists of thin to medium-thick dolomite; these rock mass exhibits a complete structure, with localized fissure development. The main classification of the Jijiapo tunnel rock mass falls into grade III and IV; the physical and mechanical properties of the surrounding rock are listed in

Table 1. Physical and mechanical parameters of the surrounding rock of tunnel class III and IV.

Surrounding Rock Grade	Density (g/cm3)	Deformation Modulus (GPa)	Poisson Ratio	Elastic Resistance Coefficient (MPa/m)	Friction Angle (°)	Cohesive (MPa)	Calculating Friction Angle (°)
III	2.75	18	0.28	800	45	0.8	65
IV	2.50	5	0.35	400	35	0.4	55

.

2.2. Geological Conditions of the Newly Excavated Railway Tunnel

The newly excavated railway Yangmuling tunnel is a single line tunnel, located in Yiling District, Yichang City, Hubei Province, between Muyang Village and Taojiaxi. The total length of the tunnel is 3640.3 m and the buried depth of the tunnel is about 310~340 m, which means it is a deep-buried tunnel. The surrounding rock of the tunnel is mainly dolomite limestone, with no obvious fissure development and weak karst development. The surrounding rock of this section is divided into III and IV grades, and its parameters and geometric dimensions are shown in

Table 2. Parameters and geometric dimensions of the newly excavated railway tunnel.

Unit Weight (kN/m3)	Friction Angle (°)	Rock Consolidating Coefficient	Excavate Equivalent Diameter (m)	Excavation Height
25	35	2.5	6.5	9.63

.

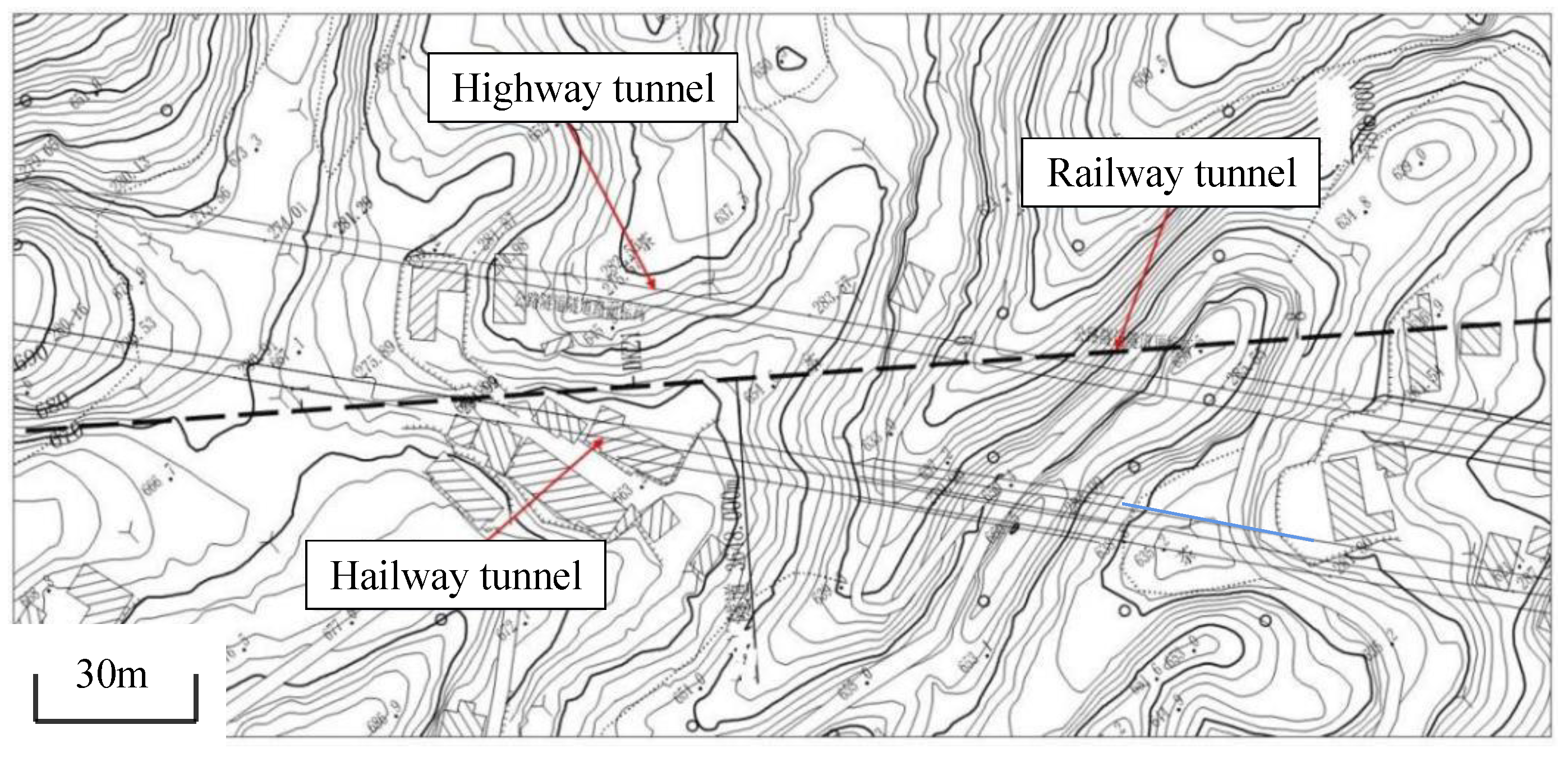
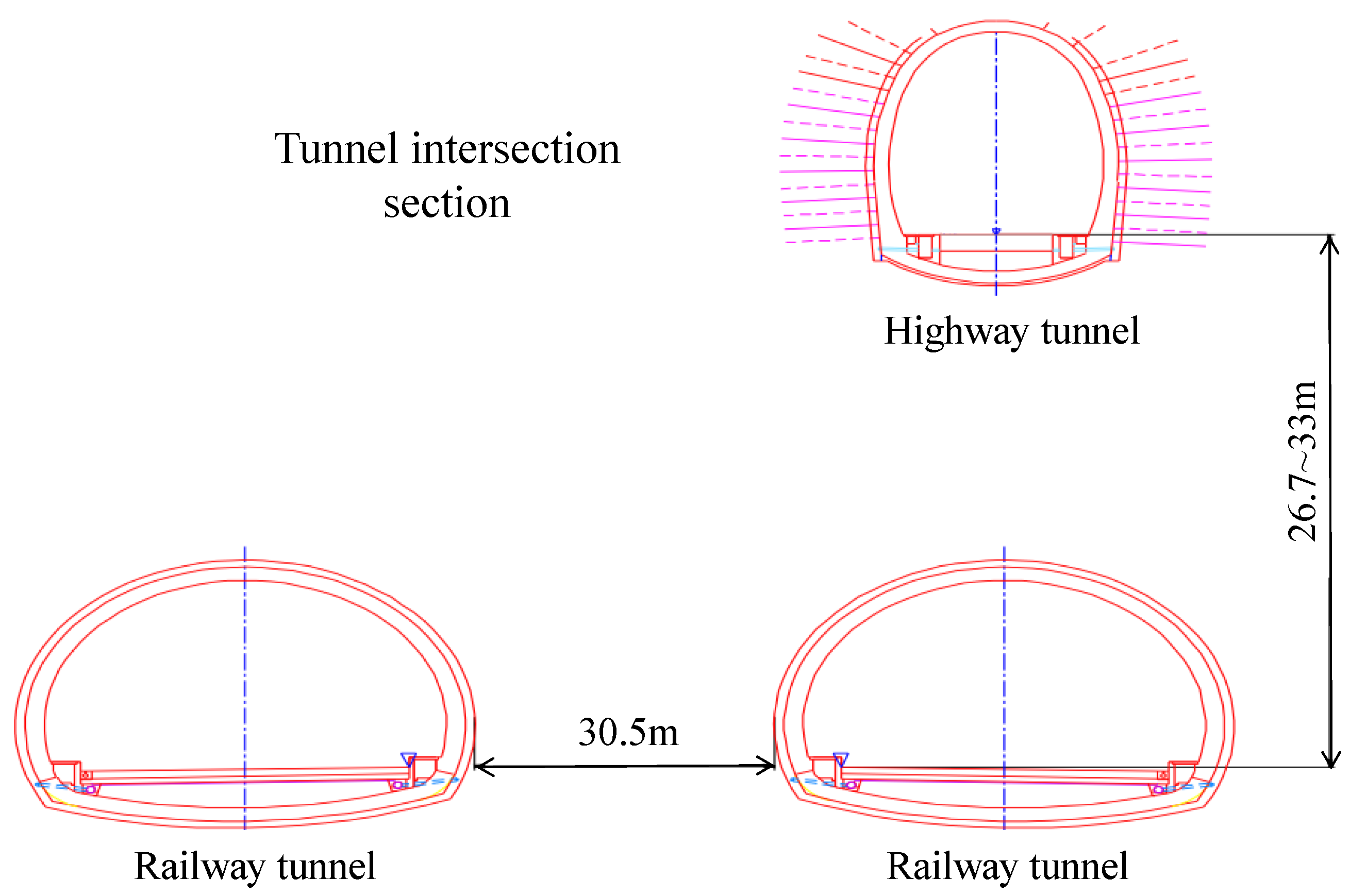
2.3. Intersection Situation of Tunnels

The newly excavated railway tunnel is positioned above the highway tunnel in DK 20 + 880 ~ DK 21 + 080, with a plane projection of 12°. The new railway tunnel intersects the left hole of the highway tunnel in DK 20 + 880~900, and intersects the right hole of the highway tunnel in DK 21 + 050~080. The railway tunnel DK 20 + 880 corresponds to the highway tunnel ZK 15 + 607, and the vertical net distance is 23 m. The railway tunnel DK 21 + 080 corresponds to the highway tunnel YK 15 + 449, and the vertical clear distance 16.7 m. The intersection situation of the tunnels is shown in Figure 1 and Figure 2.

3. Analysis of Highway Tunnel Safety

3.1. Judgment of Vertical Alignment Intersection Closeness in Tunnels

The newly excavated railway tunnel is positioned above the highway tunnel and utilizes the drilling and blasting method for construction. Due to the close proximity of the two tunnels, there is a potential impact on the traffic safety of the expressway tunnel during the construction of the railway tunnel. Thus, it is essential to assess this potential impact due to the proximity between the two tunnels. When a new tunnel is built near the existing tunnel, the disturbance to the surrounding soil will change the stress and strain of the surrounding soil, consequently affecting the stress and strain state of the existing tunnel. In order to evaluate the proximity of tunnel engineering, domestic and foreign scholars put forward several views on theoretical and practical engineering analogy. Based on the Approach Tunnel Construction Design and Guide released by Japan, this paper judges the proximity of the railway tunnel through the existing expressway tunnel of the tunnel, stratum lithology, tunnel structure section, tunnel construction method, etc.

In this project, the vertical distance of the new tunnel outline is D = 9.63 m, and the minimum vertical clear distance between the railway tunnel and the existing highway tunnel is DV = 16.7 m, which is greater than 1.5D (14.4 m) and less than 3D (28.9 m). According to the division of influence degree, the location of the existing highway tunnel falls into the category of requiring attention. This implies that the new construction has some impact on the existing tunnel structure, albeit with a relatively weak influence.

The maximum height of the loose area of the new railway tunnel can be calculated according to Platts theory (Formula (1)). By comparing the vertical distance with the existing highway tunnel vault, one can check whether loosening of the surrounding rock scope caused by the excavation of the new railway tunnel invades the scope of the existing highway tunnel vault.

$${\mathrm{h}}_0=\frac{\frac{S_W}{2}+{\mathrm{W}}_{\mathrm{W}}\times \tan (45-\frac{{\phi }_0}{2})}{\tan ({\phi }_s)}$$

(1)

In the above equation, Sw is the tunnel excavation span (m); W_W is the tunnel excavation height (m); ${\phi }_0$ is the friction angle of the surrounding rock at the side wall (°); and ${\phi }_s$ is the friction angle of the surrounding rock at the vault (°).

Based on the calculation results, the influence height of the loosening zone during the normal excavation of the railway tunnel is determined to be 11.8 m, which is less than the vertical clearance distance between the two tunnels (16.7 m). However, it is important to note that the influence height of the loosening zone is very close to the net distance between the two tunnels. Therefore, it is recommended to reinforce the rock and soil above the highway tunnel to minimize the extent and degree of rock and soil loosening. Failure to do so may result in the expansion of the loosening zone during tunnel excavation, posing a threat to the safety of road traffic.

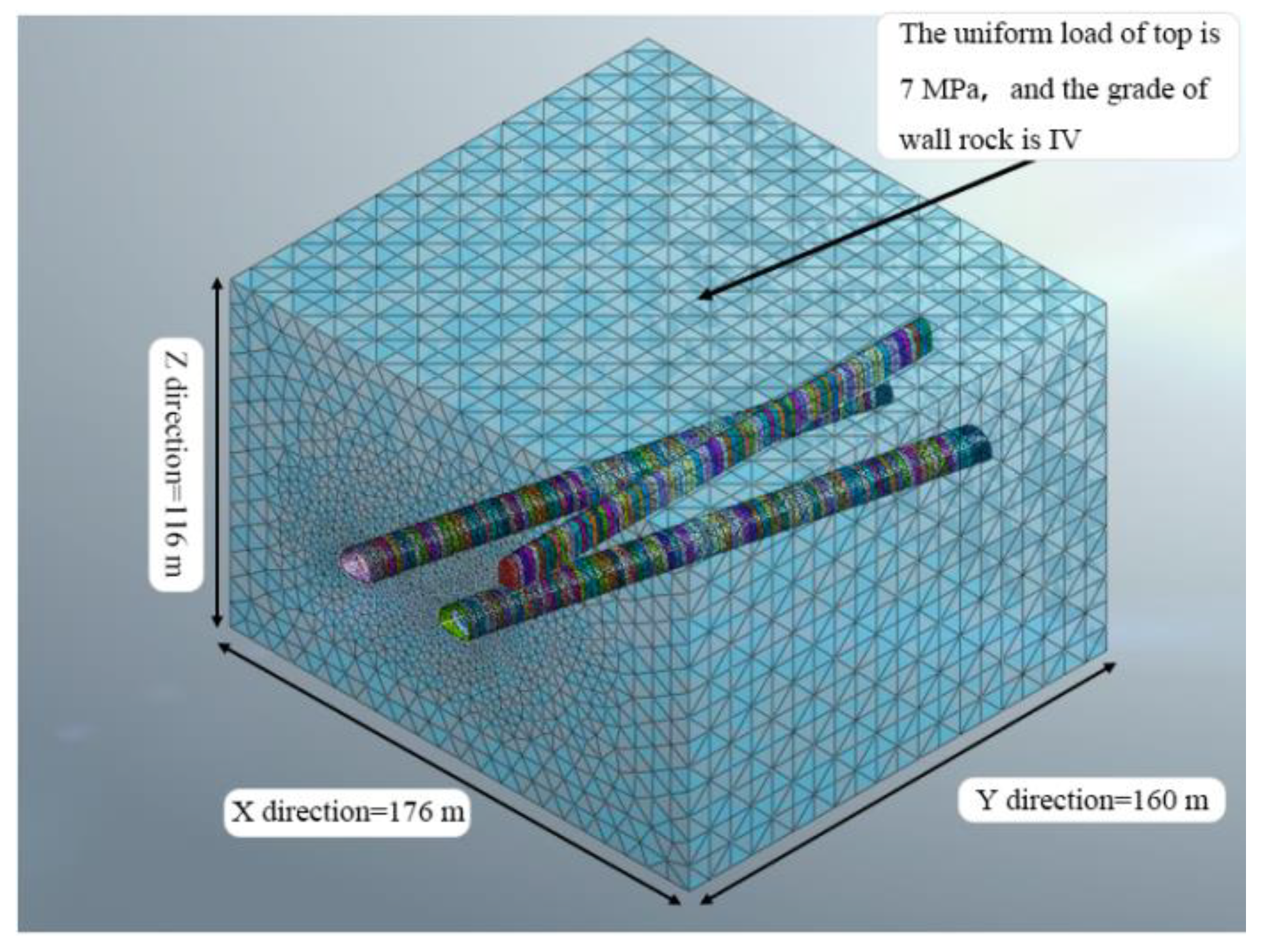
3.2. Establishment of Numerical Calculation Model

From the analysis above, it is evident that the construction of the new railway tunnel has a certain impact on the existing highway tunnel. To ensure safe construction of the railway tunnel, numerical simulation is used to evaluate the construction safety of the tunnel. In this paper, the impact of railway tunnel construction on the existing highway tunnel is considered in two steps. The first step does not consider tunnel blasting, and analyzes the disturbance of railway tunnel construction on the existing highway tunnel. The second step is to consider the impact of tunnel blasting on the existing highway tunnel. The establishment process of the numerical calculation model is carried out in three steps:

(1)

Geometric model and calculation assumptions: The geometric model of the tunnels is defined, and necessary assumptions are made to facilitate the numerical calculations.

(2)

Calculation parameters and boundary condition settings: The required calculation parameters and boundary conditions are determined and defined for the numerical simulation.

(3)

Construction process simulation and implementation: The construction process of the tunnels is simulated using the numerical model, and the calculations are implemented to analyze the behavior and response of the tunnels during construction.

By following these steps, the numerical simulation aims to provide insights into the safety of the construction process and evaluate the potential impact on the existing highway tunnel caused by the construction of the railway tunnel.

3.2.1. Geometric Model and Calculation Assumptions

In this paper, the numerical simulation model is established using the finite element numerical analysis software Midas GTS. This software is currently widely utilized in various geotechnical fields, including the design and evaluation of tunnel excavations and support systems, slope stability analysis, and foundation pit excavations. It enables the simulation of three-dimensional strata, tunnels, slopes, and foundation pit excavations, allowing for a comprehensive analysis of the entire construction process.

Figure 3 illustrates a three-dimensional geometric model diagram generated via the software. The X-axis represents the horizontal direction, the Y-axis corresponds to the tunnel axis direction, and the Z-axis represents the vertical upward direction. The single-lane highway tunnel being excavated has a span of 11.8 m and dimensions of 176 m in the X direction, 100 m in the Z direction, and 160 m in the Y direction. This model is specifically employed to simulate the intersection between the highway tunnel and the railway tunnel. The numerical model comprises 19,071 nodes and 101,888 elements, providing an accurate representation of the system under consideration. These elements and nodes form the basis for conducting the numerical analysis and evaluating the behavior and response of the tunnels during the construction process.

3.2.2. Calculate Parameters and Boundary Conditions Setting

Based on the design documents of the Jijiapo tunnel, the surrounding rock between section ZK15 + 507 and ZK15 + 707 (YK15 + 507 to YK15 + 607) is classified as grade IV, and the tunnel is supported using an S4-2 lining system. In the numerical model, an elastic model is used to represent the behavior of the tunnel lining, while the Mohr–Coulomb constitutive model is utilized to simulate the stratum. The initial support of the highway tunnel is simulated using plate elements, and the secondary lining is represented by solid elements. The supporting structure of the railway tunnel is modeled using plate elements. The physical and mechanical parameters for the formation and support structures are crucial for accurately representing the behavior and response of the tunnel system, and they are provided in

Table 3. Physical and mechanical parameters of the formation structure.

Supporting Materials	Unit Weight (kN/m3)	Deformation Modulus (GPa)	Poisson Ratio
C20 Concrete (initial support)	24	25.5	0.2
C25 Concrete (secondary lining of highway tunnel)	24	28	0.2
C35 Reinforced concrete (secondary lining of railway tunnel)	25	31.5	0.2

and

Table 4. Physical and mechanical parameters of the support structure.

Supporting Materials	Unit Weight (kN/m3)	Deformation Modulus (GPa)	Poisson Ratio
C20 Concrete (initial support)	24	25.5	0.2
C25 Concrete (secondary lining of highway tunnel)	24	28	0.2
C35 Reinforced concrete (secondary lining of railway tunnel)	25	31.5	0.2

, respectively.

3.2.3. Construction Process Simulation and Realization

The simulation of the construction process is conducted in four distinct steps:

(1)

Initial balance, activate stratum, apply displacement boundary condition and gravity, tick displacement zero, and carry on initial balance;

(2)

Simulate the process of highway tunnel construction, simulate the cycle of excavation-initial support-second lining until the completion of highway tunnel construction;

(3)

Simulate the influence of railway tunnel excavation on highway tunnel, and simulate the cycle of excavation-initial support-second lining until the railway construction is completed;

(4)

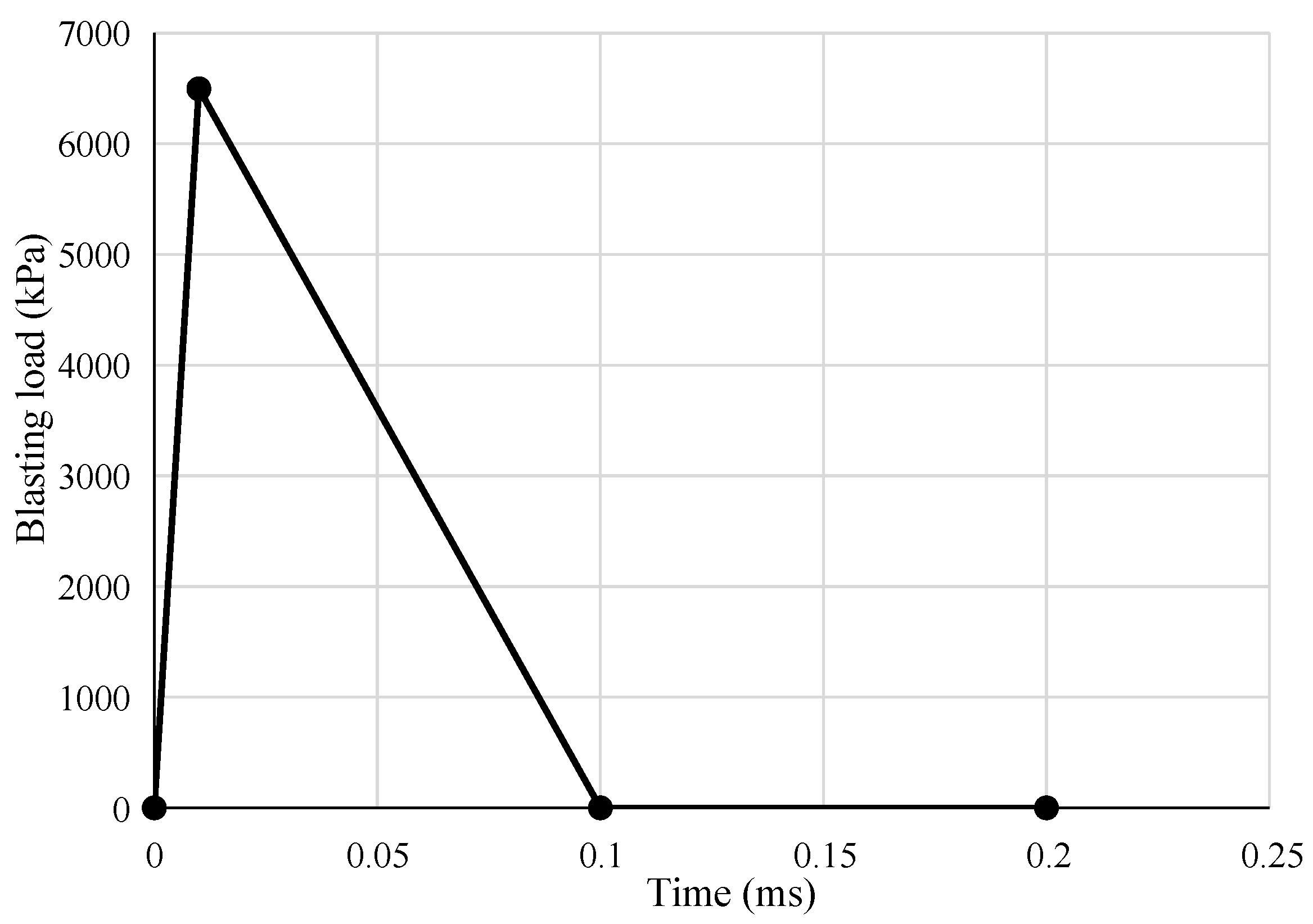
Simulate the influence of blasting on highway tunnel, (3) conduct dynamic analysis based on the stress field obtained with static calculation; when construction reaches the crossing point of railway tunnel and highway tunnel, apply the equivalent blasting load at the crossing point. When the boundary conditions around the model are changed to viscous non-reflection boundary, use nonlinear time history analysis.

By following these four steps, the numerical simulation captures the various stages of construction and assesses the impact of excavation, support installation, and blasting on the behavior and safety of the highway tunnel.

3.3. Static Response Analysis

3.3.1. Analysis of Highway Tunnel Excavation

Before the excavation of the highway tunnel and railway tunnel, an initial in situ stress equilibrium is established to determine the initial stress field. Figure 4 shows the initial in situ stress field, considering the absence of a 300 m high mountain body model. Instead, its influence is represented by an equivalent load of 7 MPa applied to the top of the numerical model. The vertical ground stress gradually increases from approximately 7 MPa to 10 MPa in a top-down manner.

After completion of the highway tunnel construction, the vertical and horizontal displacements of the tunnel are evaluated. Figure 5 illustrates the displacement distribution. The calculation results indicate that, with the completion of the highway tunnel and in the absence of railway tunnel excavation, the bottom of the tunnel’s arch experiences an uplift of 2.421 cm, while the top of the arch undergoes a settlement of 3.317 cm. The vertical displacement at the side wall is estimated to be around 3–4 mm. Moreover, the horizontal displacement of the left side wall is approximately 2.681 cm, while the right side wall experiences a displacement of −3.150 cm. The horizontal displacements of the vault and the bottom are relatively small, ranging from 0.6 mm to 0.8 mm.

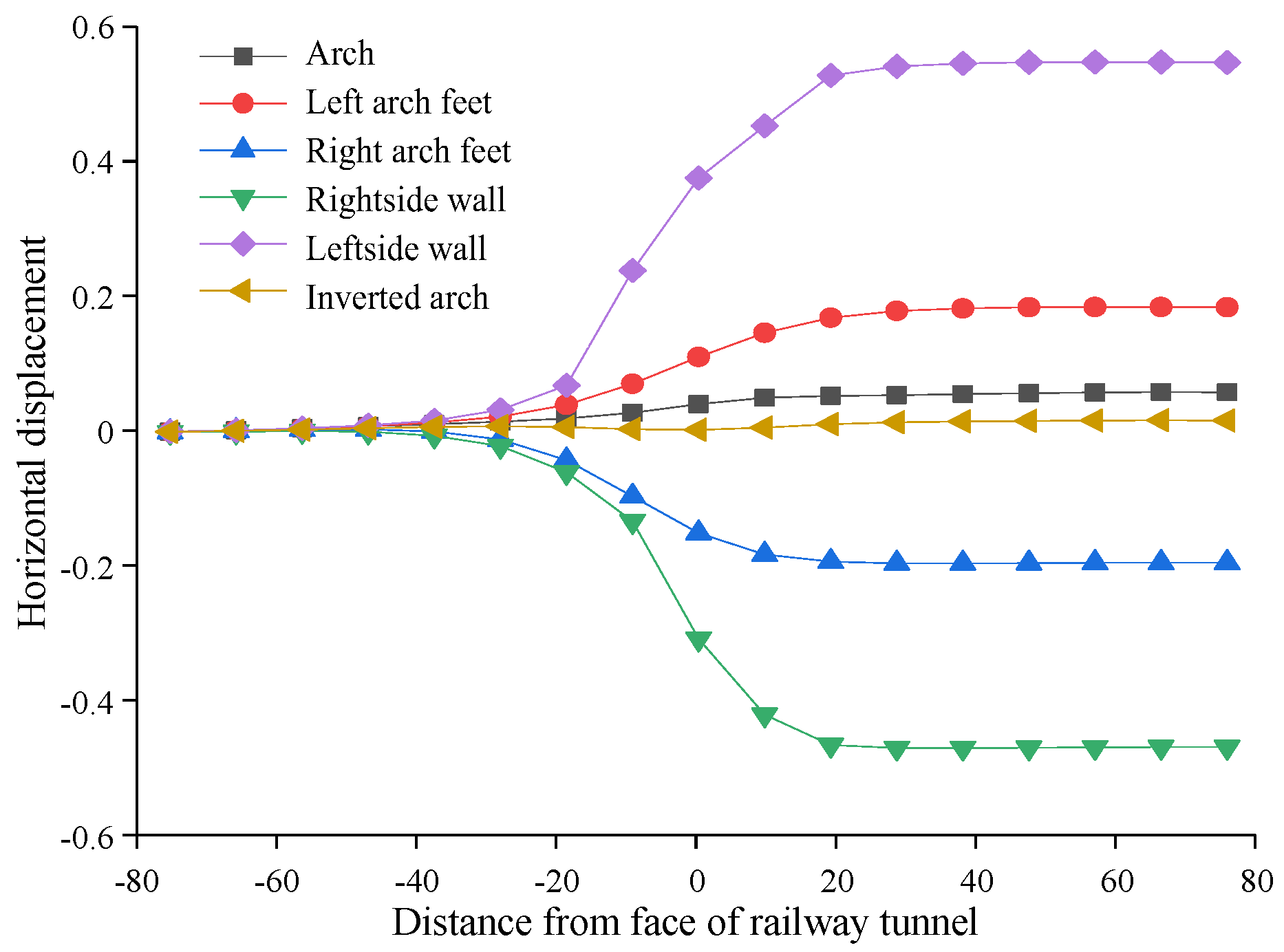
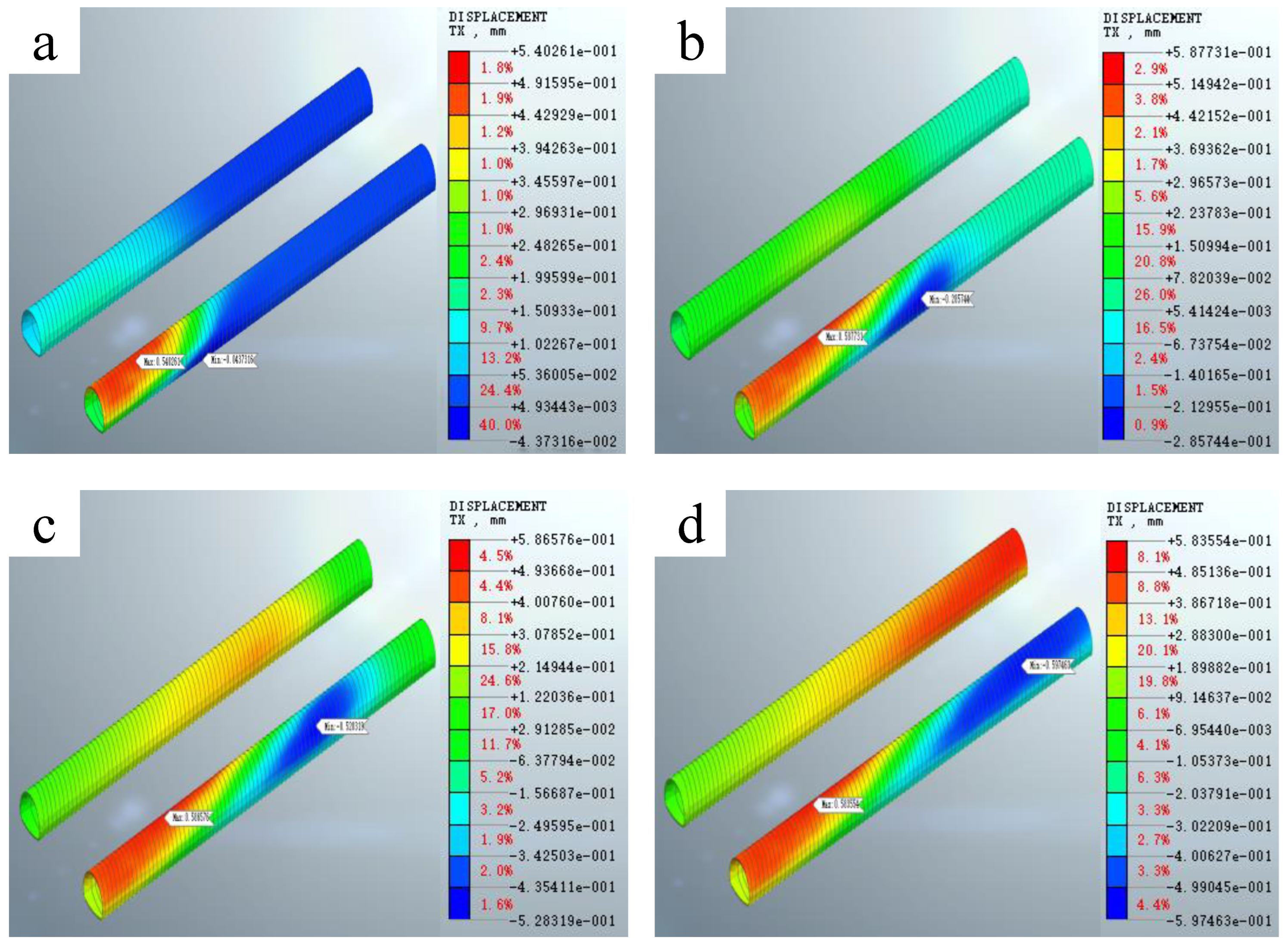
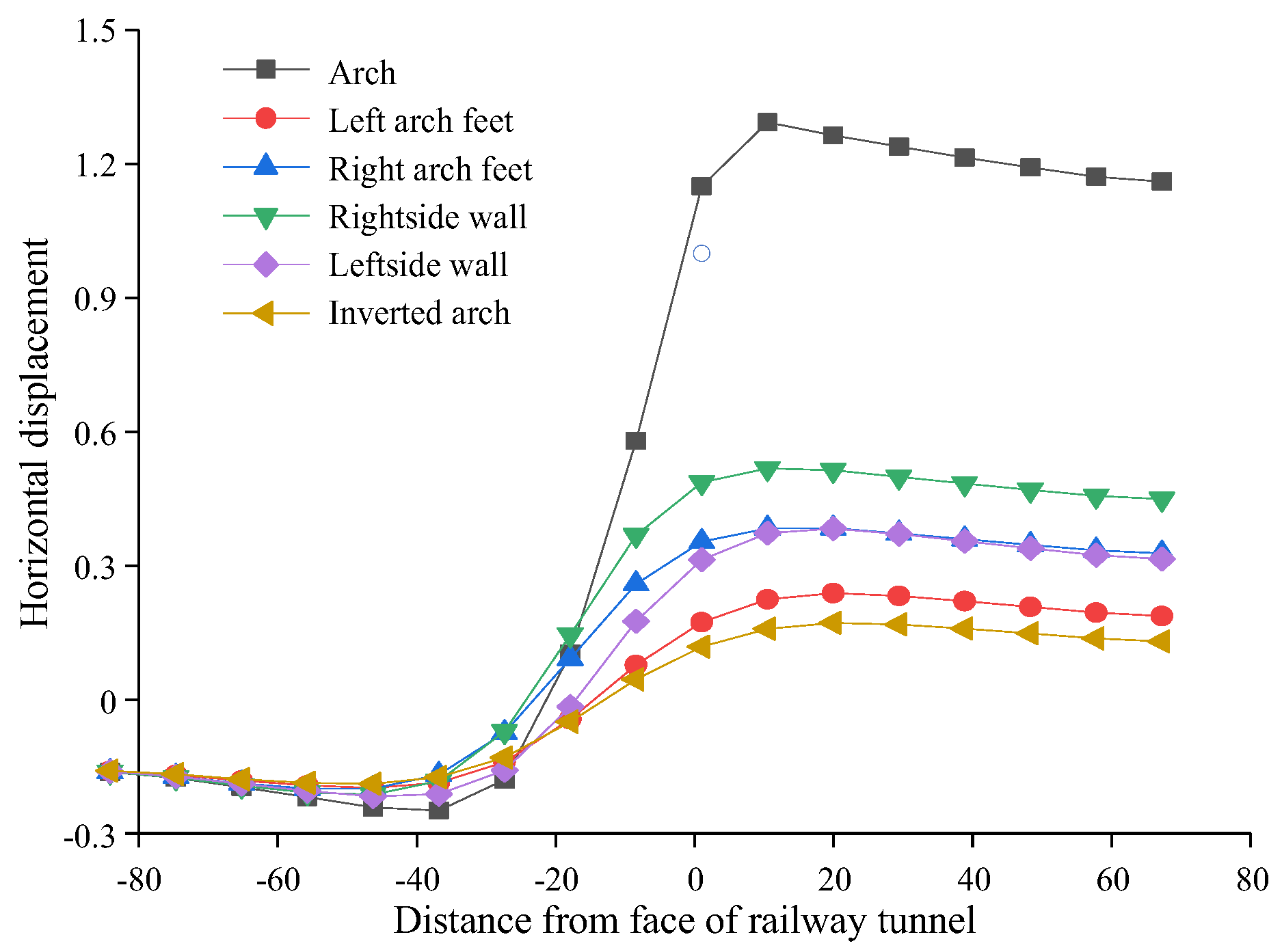
3.3.2. Horizontal Displacement Analysis of Highway Tunnel

For clearly observing the impact of railway tunnel construction on road tunnels, a displacement zeroing process was conducted before the excavation of the railway tunnel. Section A, which is the closest section of the highway tunnel to the railway tunnel, was analyzed. Figure 6 displays the horizontal displacement curve of a representative point in the second lining of the road tunnel at section A. In the figure, positive values indicate displacement to the right, while negative values indicate displacement to the left. The results show that the horizontal displacement at the crown and inverted arch of the road tunnel in section A is minimal. The displacement at the left and right abutments is slightly larger, and the displacement at the left and right walls is the largest. When section A is located between −80 m and −20 m from the face of the railway tunnel excavation, the horizontal displacement of the road tunnel remains nearly constant as the distance increases. Between −20 m and 20 m, the horizontal displacement of the road tunnel increases rapidly with distance, reaching a maximum value of 0.59 mm. Beyond 20 m to 80 m, the horizontal displacement of the road tunnel converges and no longer increases significantly with distance.

Figure 7 presents a contour map of the horizontal displacement of the second lining of the road tunnel during the excavation of the railway tunnel at distances of 40 m, 80 m, 120 m, and 160 m, respectively. The calculation results indicate that the maximum horizontal displacement values for the right tunnel of the road tunnel are 0.540 mm and −0.59 mm during the excavation of the railway tunnel. Overall, the horizontal displacement caused by the railway tunnel excavation near the intersection of the road and railway tunnels is relatively small.

3.3.3. Vertical Displacement Analysis of Highway Tunnel

Figure 8 illustrates the vertical displacement curve of a representative point in the second lining of the highway tunnel at section A. Positive values indicate upward displacement of the tunnel, while negative values indicate downward displacement. It can be seen from the figure that the maximum vertical displacement occurs at the crown of the highway tunnel in section A, while the minimum displacement occurs at the springing. Apart from the significant displacement at the crown, the vertical displacement at the foot of the arch and the side walls is relatively small. When section A is located between -80 m and −20 m from the face of the railway tunnel excavation, the vertical displacement of the highway tunnel does not increase significantly with distance. However, between −20 m and 20 m, the vertical displacement of the highway tunnel increases rapidly with distance, reaching a maximum value of 1.42 mm. Beyond 20 m to 80 m, the vertical displacement of the highway tunnel converges and no longer increases significantly with distance. Figure 9 presents a contour map of the vertical displacement of the second lining of the highway tunnel during the excavation of the railway tunnel at distances of 40 m, 80 m, 120 m, and 160 m, respectively. As the railway tunnel is excavated above the highway tunnel, the vertical displacement is upward.

At 40 m excavation, the maximum vertical displacement of the right tunnel lining of the highway tunnel is 0.944 mm, while it is 0.176 mm in the left tunnel lining. At 80 m excavation, the maximum vertical displacement of the right tunnel lining is 1.421 mm, and the maximum displacement of the left tunnel lining is 0.233 mm. At 120 m excavation, the maximum vertical displacement of the right tunnel lining is 1.393 mm, and the maximum displacement of the left tunnel lining is 0.249 mm. Finally, at 160 m excavation, the maximum vertical displacement of the right tunnel lining is 1.315 mm, and the maximum displacement of the left tunnel lining is 0.249 mm. The calculation results demonstrate that near the intersection of the highway and railway tunnels, the vertical displacement of the highway tunnel caused by the construction of the railway tunnel is relatively small, with a maximum value of 1.42 mm.

3.4. Dynamic Response Analysis

3.4.1. Parameter Selection

In dynamic analysis, several factors have an impact on the calculation results, including elastic modulus, damping parameters, spatial dimensions, blasting vibration intensity, and loading time. Accurate values of these parameters are essential to ensure that the calculated results closely reflect the real situation. Here are some considerations regarding two of these factors:

(1) Elastic modulus: The elastic modulus significantly affects the calculation results. A higher elastic modulus of the rock mass leads to smaller vibration velocities at protected particles. Some researchers propose the concept of dynamic elastic modulus, suggesting that materials may exhibit an increased elastic modulus under the influence of blasting vibrations. However, many researchers do not consider dynamic elastic modulus in dynamic analysis. For safety reasons, this study adopts the static elastic modulus instead of the dynamic one. (2) Damping parameters: Damping parameters in the model are related to the rate of vibration velocity attenuation and have a significant impact on the calculation results. To ensure consistency between numerical calculations and actual measurements, damping parameters often require dynamic adjustment based on on-site monitoring data. There are two main approaches to input damping parameters in numerical calculations. One is to directly input the coefficients of Rayleigh damping, including the mass damping coefficient α and the stiffness damping coefficient β. The other approach involves conducting an eigenvalue analysis on the model to determine the inherent frequency of the structure and calculate the damping parameters based on the inherent frequency and damping ratio. Since there is no available on-site monitoring data on vibration velocity in this case, directly inputting damping parameters α and β lacks a basis for value determination. Therefore, this study adopts the approach of inputting damping ratio and inherent period of the structure to generate damping parameters. The inherent period can be obtained through an eigenvalue analysis. Previous literature suggests that the damping ratio in this model can be set within the range of 0.02–0.05. For safety considerations, a damping ratio of 0.02 is chosen for this calculation. It is worth noting that the other factors mentioned, such as spatial dimensions, blasting vibration intensity, and loading time, should also be carefully considered and accurately valued to ensure the reliability of the dynamic analysis results.

(3) Blasting load intensity: According to the different effects of blasting on rock mass, the blasting action range can be divided into near field, middle field, and far field. The near field is the area where rocks are crushed by blasting, while the middle field often produces fracture damage to the rock mass. In the far field, the rock mass only undergoes elastic vibration due to blasting. According to the requirements of tunnel construction, the surrounding rock of the tunnel should not be damaged during blasting, and the surrounding rock is in an elastic vibration state when establishing the vibration model of the tunnel excavation blasting, with the following simplified assumptions: ➀ the blasting vibration load acts on the tunnel perimeter wall in the form of uniformly distributed pressure load in the normal direction; and ➁ the tunnel perimeter wall is in the far field of blasting action, and the blasting vibration load on the tunnel perimeter wall will not cause surrounding rock damage. Blasting load has a significant impact on the calculation results, with larger blasting peak loads applied to the tunnel wall resulting in greater vibration velocities at protected particles. For concentrated charge or single-hole cylindrical charge, wave theory can be used to accurately calculate the initial peak stress of blasting on the borehole wall and thus calculate the blasting vibration. However, as tunnel excavation blasting generally uses multi-hole charges distributed in a certain space for initiation, the stress produced by blasting is the result of the superposition of multiple stress waves, and currently there is no theoretical method to accurately calculate the stress produced by multi-hole charge initiation at a particular location. Existing data show that the peak blasting load applied to the tunnel wall can be approximately calculated using Formula (2).

$${\mathrm{P}}_{\max }=\frac{139.97}{Z}+\frac{844.81}{Z^2}+\frac{2154}{Z^3}$$

(2)

In the formula, P_max is the peak of blasting stress (kPa), $\mathrm{Z}=\mathrm{R}/\sqrt[3]{\mathrm{Q}}$ is the proportional distance, R is the distance from the hole to the loading surface (m), and Q is the charge of the hole (kg).

The calculated peak blasting load is 6.497 MPa, and the approximate form of the blasting load is a triangular load. The loading time of the blasting load is taken as 10 ms, and the unloading time is taken as 90 ms. The model calculation time is taken as 200 ms, and the blasting load function is shown in Figure 10. As the peak vibration caused by tunnel excavation blasting often occurs during the slot blasting, this calculation only simulates the blast pressure generated by the slot blasting and ignores the effects of auxiliary holes and surrounding holes. When the railway tunnel excavation reaches the closest point to the highway tunnel, the dynamic load is applied to the excavation face of the railway tunnel, perpendicular to the excavation face.

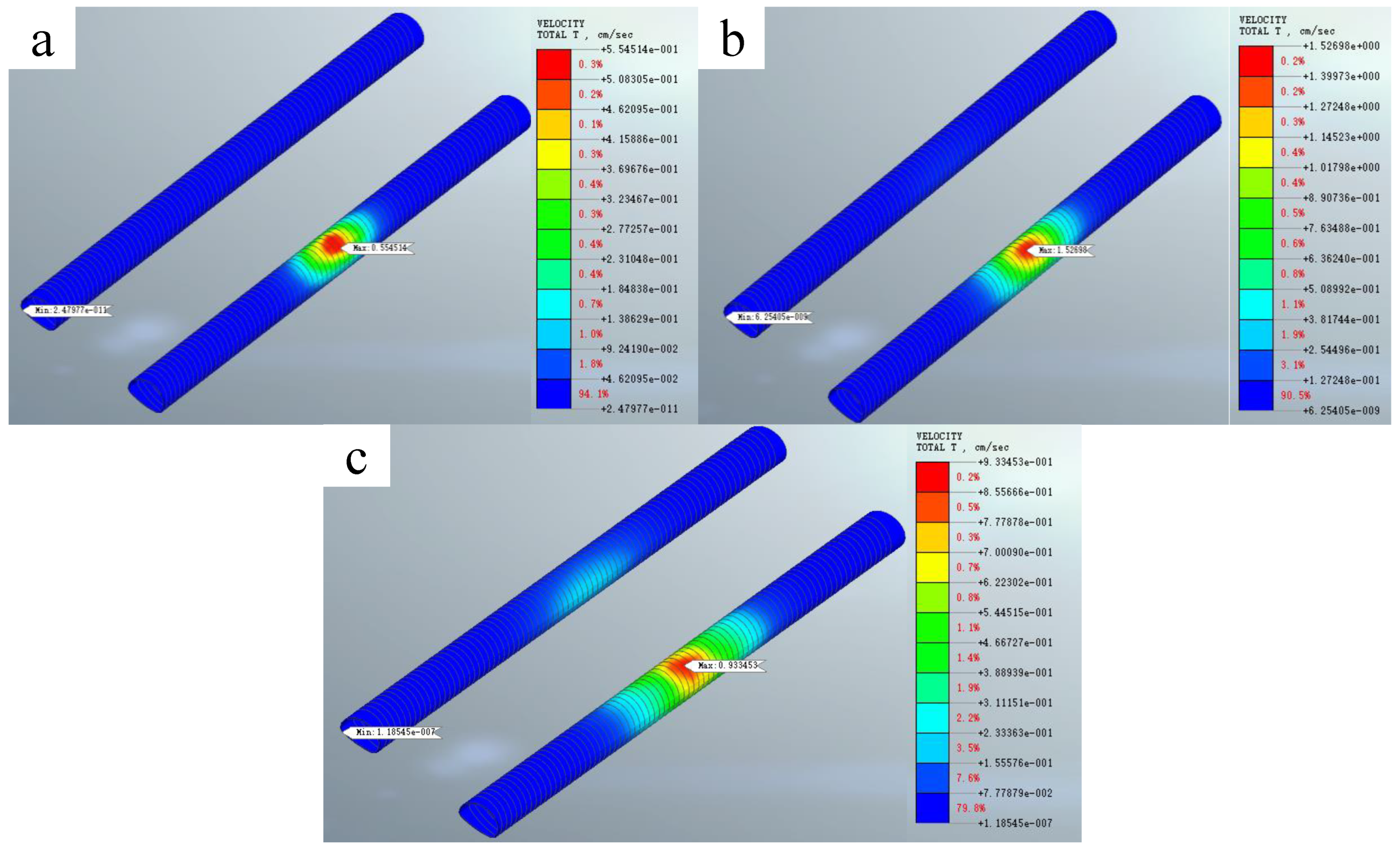
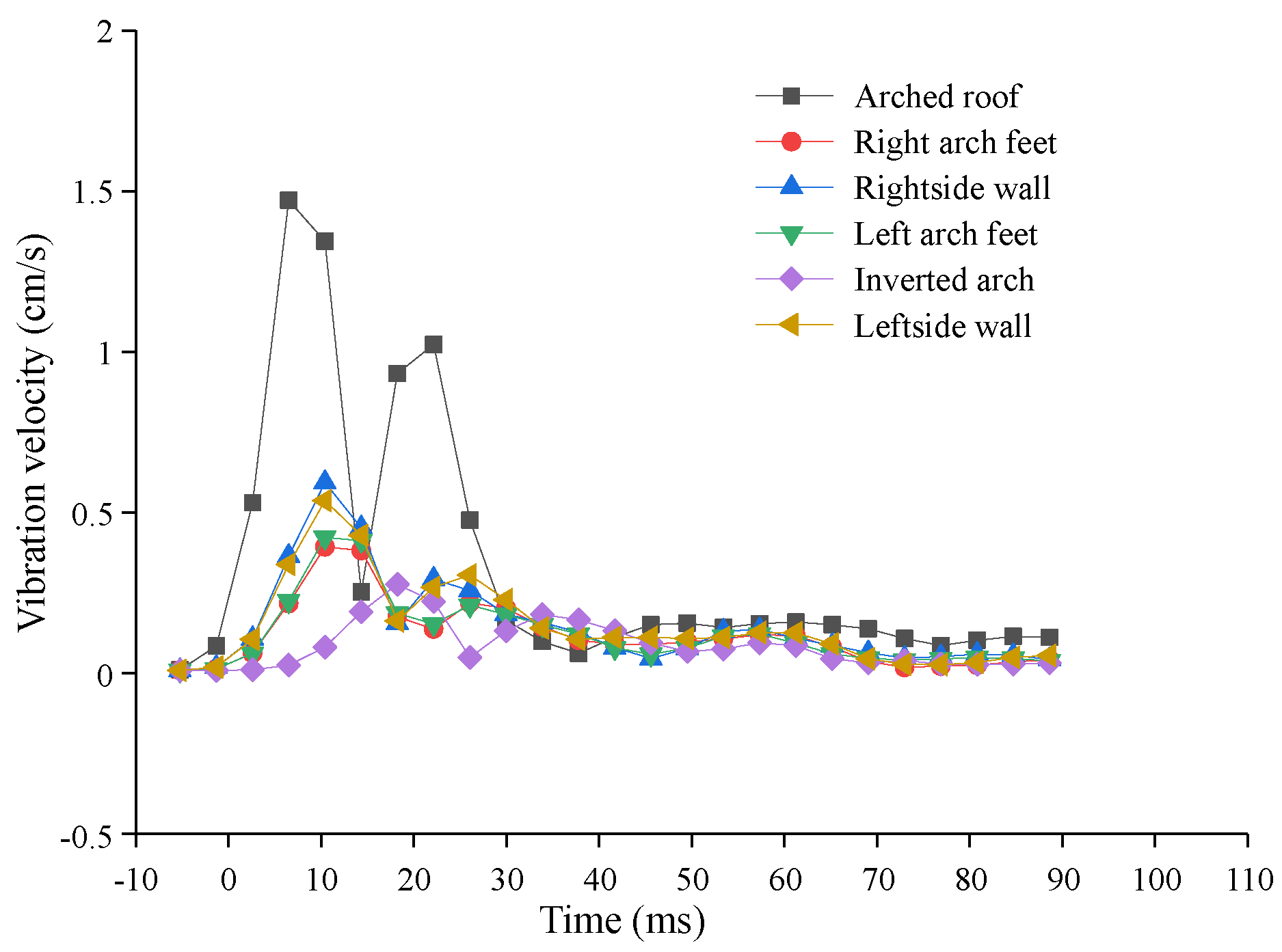
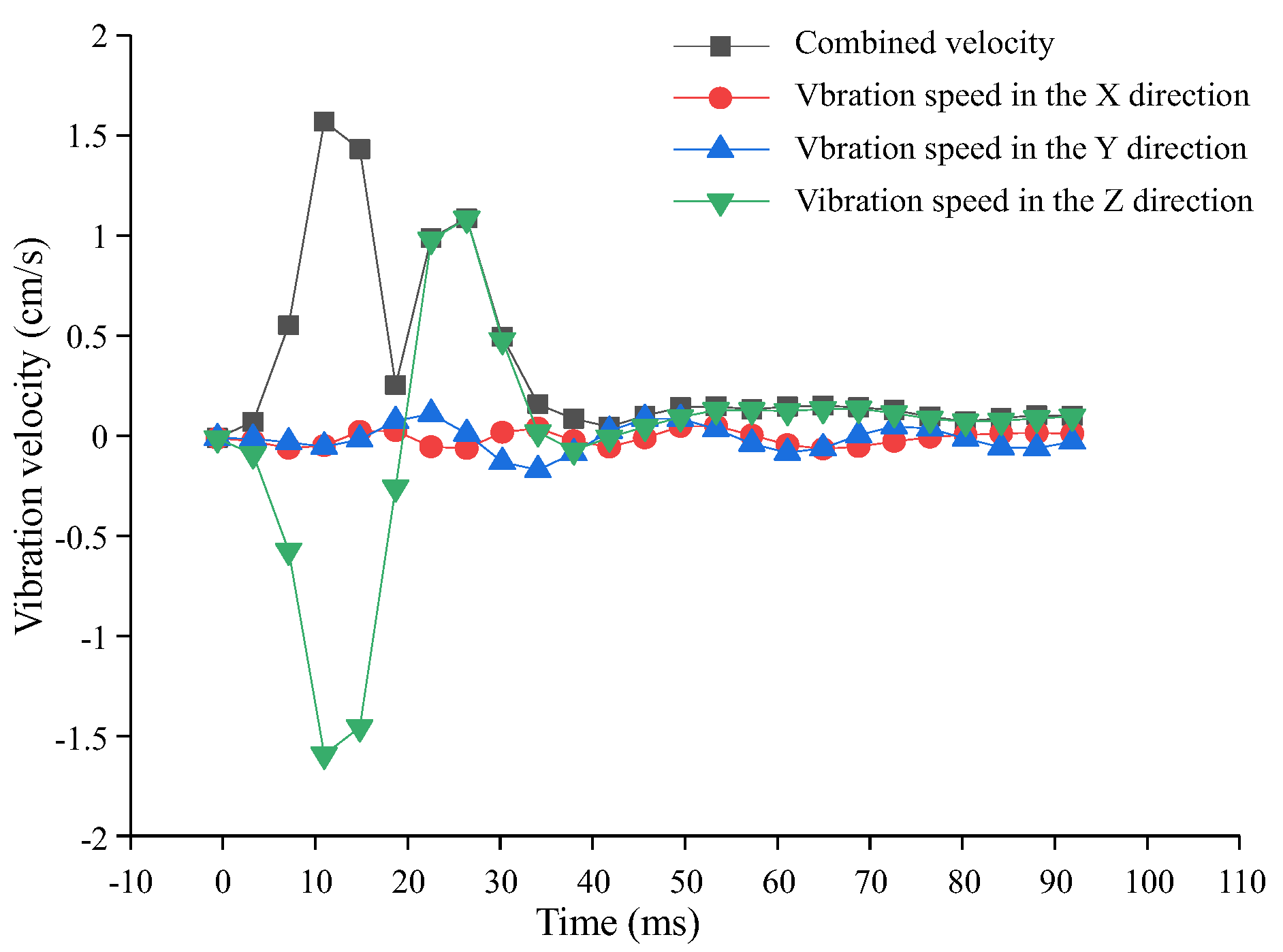
3.4.2. Vibration Speed Analysis

Figure 11 shows the cloud speed of the highway tunnel at 10 ms, 16 ms and 20 ms. The peak vibration velocity occurs at the right tunnel vault of the highway tunnel; the right tunnel is 0.555 cm/s at 10 ms and 0.934 cm/s at 20 ms, and the second lining of the highway tunnel reaches 1.527 cm/s.

Figure 12 shows the vibration time-history curve of a selected point located in the second lining of the right tunnel of the highway tunnel at the intersection of the railway and highway tunnels, and Figure 13 shows the vibration time-history curves of the arch apex mass point in different directions. From Figure 12 and Figure 13, it can be seen that during the railway tunnel blasting construction process, the vibration velocity at the mass point of the highway tunnel arch apex is the highest, and it rapidly increases from 0 to 1.527 cm/s within 0–16 ms, then rapidly decreases, and has a small rebound at 24 ms, after which the vibration velocity slowly decreases and reaching 0.02 cm/s. The peak vibration velocity at the mass point on the left and right walls of the highway tunnel is 0.606 cm/s, and the trend of vibration velocity with time is consistent with the arch apex vibration velocity curve. The vibration at the arch apex mainly vibrates along the Z direction, and the vibration velocity in the X and Y directions is very small.

According to the “Technical Regulations for Monitoring and Measurement of Highway Tunnels” (DB42/T 900-2013) and the “Blasting Safety Regulations” (GB 6722-2014), the permissible safe vibration velocity for the existing highway tunnel, subject to the disturbance caused by the new railway tunnel construction, should be less than 10 cm/s. Considering the operation of the highway tunnel for a duration of 12 years and the resulting degradation of the lining, the allowable safe vibration velocity is reduced to less than 2 cm/s. Throughout the dynamic analysis process, it is evident that the vibration velocity of the existing highway tunnel remains below the allowable safe vibration velocity of 2 cm/s. Consequently, these findings indicate that the impact of railway tunnel blasting excavation on the existing highway tunnel is within manageable limits.

4. Conclusions

This study used the finite element software Midas GTS to establish a numerical model of tunnel excavation and conducted a safety evaluation for the project involving the crossing of the Jijiapo highway tunnel and the Yangmuling railway tunnel. The following conclusions can be drawn:

(1)

Analysis of the vertical alignment intersection closeness in tunnels shows that the new railway tunnel has an effect on the existing highway tunnel structure, but the effect is weak, and it usually does not cause harm;

(2)

The horizontal displacement is the smallest at the invert and vault of the highway tunnel, slightly larger at the left and right arch feet, and the largest at the left and right side walls. The horizontal displacement of the highway tunnel initially remains relatively constant with distance from the front face of the railway tunnel, then increases rapidly, and finally converges without further increase. The maximum horizontal displacement caused by the railway tunnel construction is 0.540 mm and −0.59 mm;

(3)

The vertical displacement is the largest at the arch top and the smallest at the invert of the highway tunnel. Similar to the horizontal displacement, the vertical displacement of the highway tunnel also shows an increasing trend with the distance from the face of the railway tunnel. The maximum vertical displacement caused by railway tunnel construction is 1.42 mm;

(4)

The vibration velocity is highest at the vault of the highway tunnel and increases initially before decreasing with time, reaching a peak value of 1.527 cm/s at 16 ms. Throughout the entire process of blasting construction, the vibration velocity of the highway tunnel remains below 2 cm/s. The results demonstrate that the excavation process for the railway tunnel in this study is safe and manageable for the adjacent highway tunnel.