Stability and Periodic Motions for a System Coupled with an Encapsulated Nonsmooth Dynamic Vibration Absorber
Abstract
1. Introduction
- The fast–slow analysis method is introduced to study the stability of the equilibrium point of the nonsmooth dynamic vibration absorber model. The existence and stability of the admissible equilibrium points of an encapsulated DVA model with piecewise smooth properties are discussed in different parameter regions. The asymptotical stable and unstable regions of an admissible equilibrium point for a nonsmooth DVA model under different parameter conditions are obtained, which provides theoretical guidance for the design of a nonsmooth DVA structure.
- Few studies have focused on the bifurcation and number of periodic solutions of a three-degrees-of-freedom nonsmooth DVA model. In this paper, the expression of a Melnikov vector function suitable for studying the bifurcation of periodic orbits of six-dimensional piecewise smooth systems is given, and it is applied to study the existence and number of periodic solutions of an encapsulated DVA model.
- The relationship figure is introduced to provide guidance for estimating the number of periodic orbits. The existence and number of periodic solutions under different parameter conditions are given, and the geometric description is given.
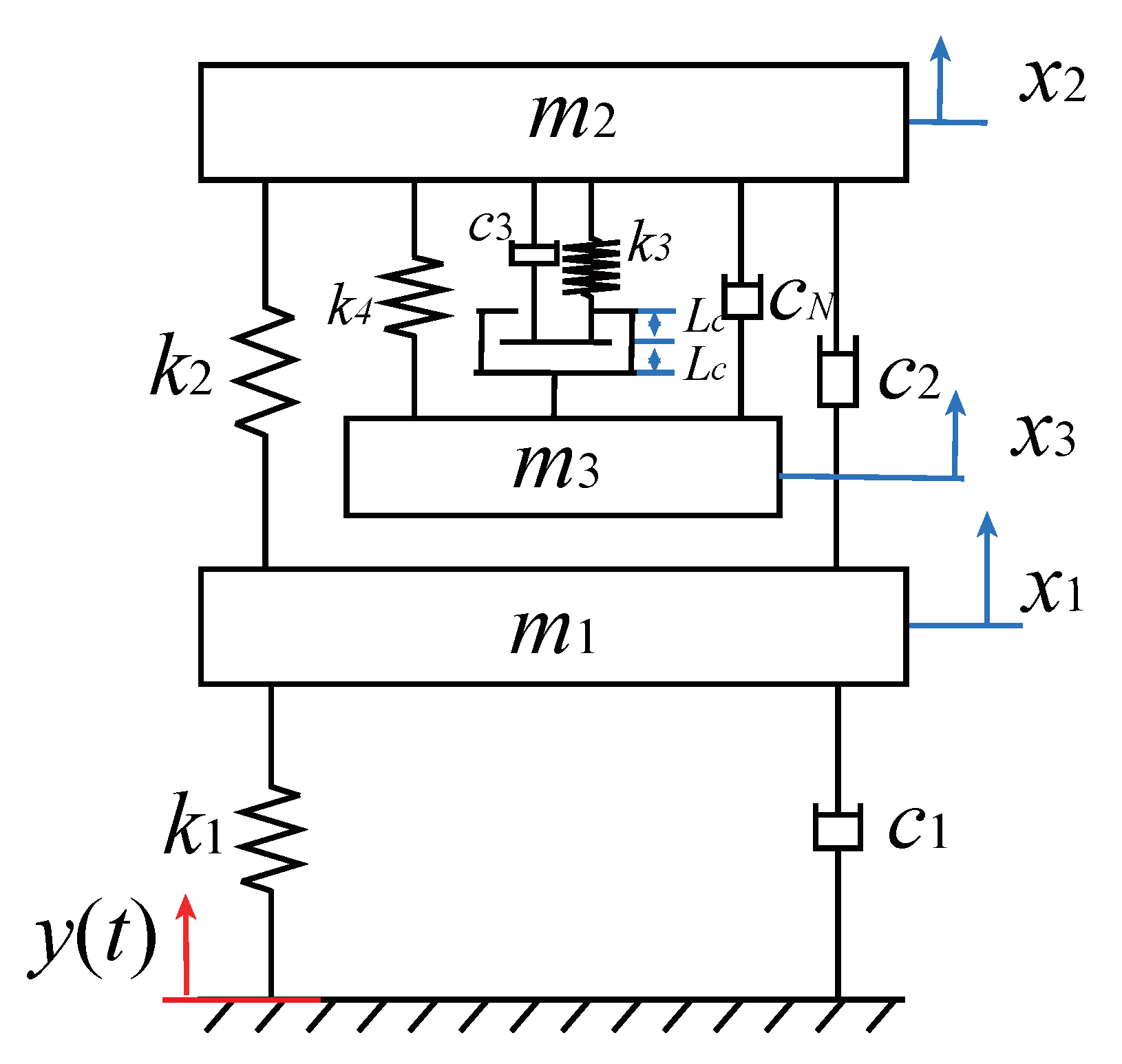
2. System Description
3. Stability of Equilibrium Points
3.1. Equilibrium Points
- is always an admissible equilibrium point;
- is always a virtual equilibrium point, because ;
- is always a virtual equilibrium point, because .
- is always an admissible equilibrium point;
- is always a boundary equilibrium point, because ;
- is always a boundary equilibrium point, because .
- is always an admissible equilibrium point;
- is always an admissible equilibrium point, because ;
- is always a boundary equilibrium point, because .
3.2. Stability
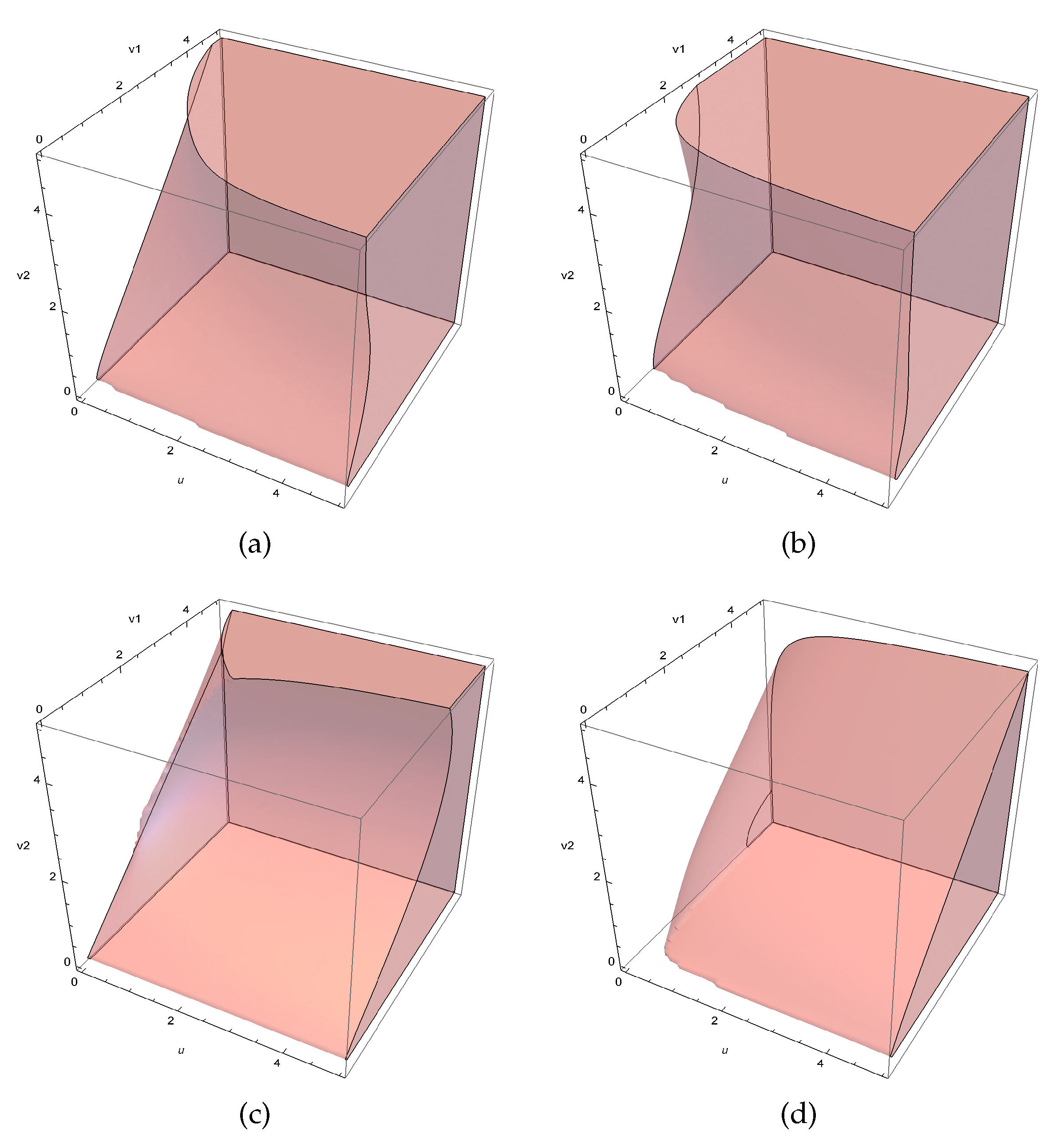
4. Bifurcation of Periodic Orbits
4.1. Melnikov Function
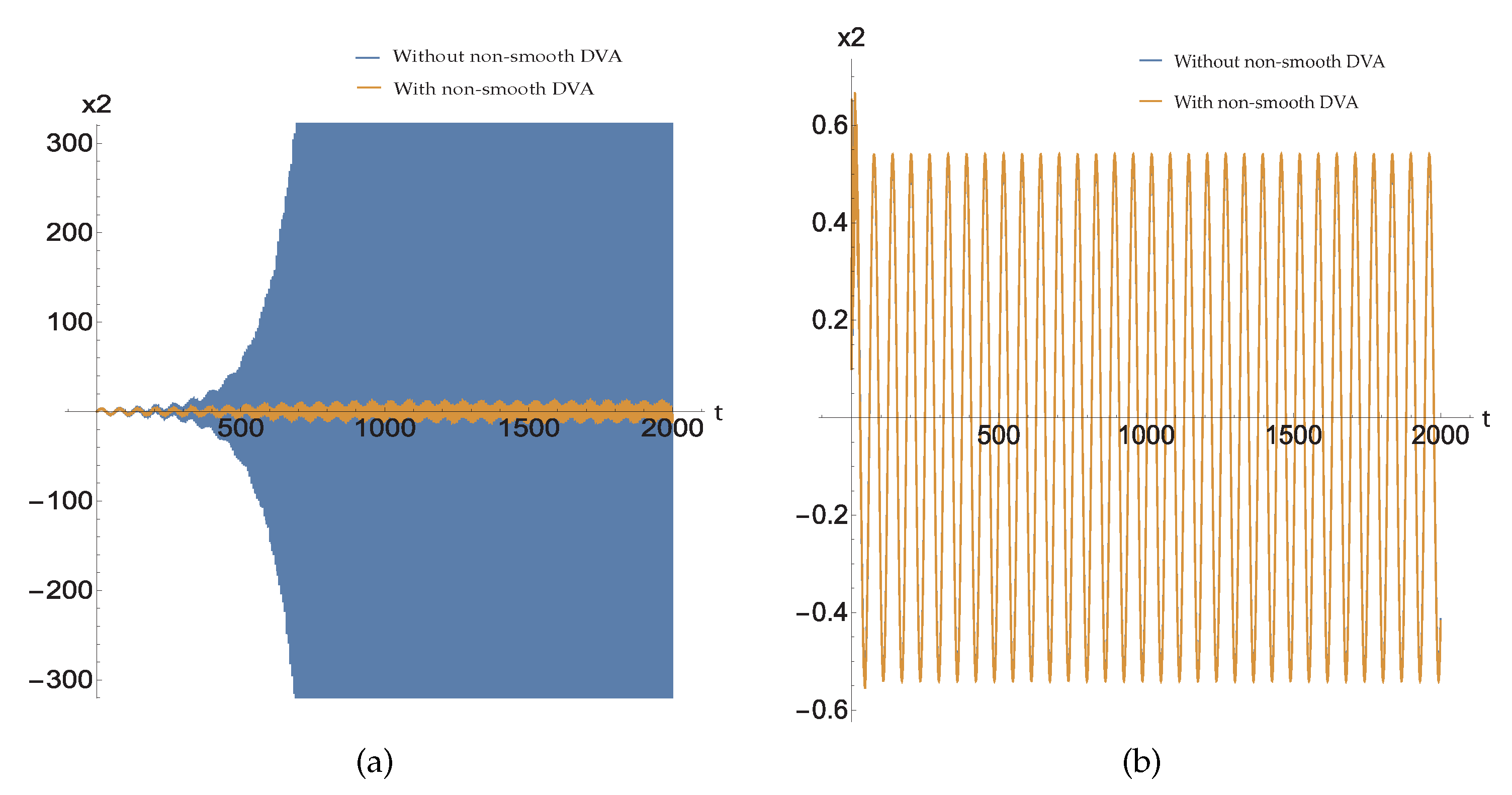
4.2. Numerical Simulation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elias, S.; Matsagar, V. Research developments in vibration control of structures using passive tuned mass dampers. Annu. Rev. Control 2017, 44, 129–156. [Google Scholar] [CrossRef]
- Braga, M.T.; Alves, M.T.S.; Cavalini, A.A.; Steffen, V. Influence of temperature on the passive control of a rotating machine using wires of shape memory alloy in the suspension. Smart Mater. Struct. 2020, 29, 035040. [Google Scholar] [CrossRef]
- Ormondroyd, J.; Den Hartog, J.P. The theory of the dynamic vibration absorber. J. Appl Mech. 1928, 50, 9–22. [Google Scholar]
- Frahm, H. Device for Damping Vibrations of Bodies. U.S. Patent 0,989,958, 30 October 1909. [Google Scholar]
- Roberson, R.E. Synthesis of a nonlinear dynamic vibration absorber. J. Franklin Inst. 1952, 254, 205–220. [Google Scholar] [CrossRef]
- Masri, F. Theory of the dynamic vibration neutralizer with motion limiting stops. J. Appl. Mech. 1972, 39, 563–568. [Google Scholar] [CrossRef]
- Natsiavas, S. Dynamics of multiple-degree-of-freedom oscillators with colliding components. J. Sound Vib. 1993, 165, 439–453. [Google Scholar] [CrossRef]
- Pun, D.; Liu, Y.B. On the design of the piecewise linear vibration absorber. Nonlinear Dyn. 2000, 22, 393–413. [Google Scholar] [CrossRef]
- Georgiades, F.; Vakakis, A.F.; Mcfarland, D.M.; Bergman, L. Shock isolation through passive energy pumping caused by nonsmooth nonlinearities. Int. J. Bifurc. Chaos 2005, 15, 1989–2001. [Google Scholar] [CrossRef]
- Gendelman, O.V. Targeted energy transfer in systems with non-polynomial nonlinearity. J. Sound Vib. 2008, 315, 732–745. [Google Scholar] [CrossRef]
- Lamarque, C.H.; Gendelman, O.V.; Savadkoohi, A.T.; Etcheverria, E. Targeted energy transfer in mechanical systems by means of non-smooth nonlinear energy sink. Acta Mech. 2011, 221, 175–200. [Google Scholar] [CrossRef]
- Weiss, M.; Vaurigaud, B.; Savadkoohi, A.T.; Lamarque, C.H. Control of vertical oscillations of a cable by a piecewise linear absorber. J. Sound Vib. 2018, 435, 281–300. [Google Scholar] [CrossRef]
- Yao, H.L.; Cao, Y.B.; Zhang, S.J.; Wen, B.C. A novel energy sink with piecewise linear stiffness. Nonlinear Dyn. 2018, 94, 2265–2275. [Google Scholar] [CrossRef]
- Chen, J.E.; Sun, M.; Hu, W.H.; Zhang, J.H.; Wei, Z.C. Performance of non-smooth nonlinear energy sink with descending stiffness. Nonlinear Dyn. 2020, 100, 255–267. [Google Scholar] [CrossRef]
- Li, X.L.; Liu, K.F.; Xiong, L.Y.; Tang, L.H. Development and validation of a piecewise linear nonlinear energy sink for vibration suppression and energy harvesting. J. Sound Vib. 2021, 503, 116104. [Google Scholar] [CrossRef]
- Geng, X.F.; Ding, H. Theoretical and experimental study of an enhanced nonlinear energy sink. Nonlinear Dyn. 2021, 104, 3269–3291. [Google Scholar] [CrossRef]
- Geng, X.F.; Ding, H. Two-modal resonance control with an encapsulated nonlinear energy sink. J. Sound Vib. 2022, 520, 116667. [Google Scholar] [CrossRef]
- Fang, B.; Theurich, T.; Krack, M.; Bergman, L.A.; Vakakis, A.F. Vibration suppression and modal energy transfers in a linear beam with attached vibro-impact nonlinear energy sinks. Commun. Nonlinear Sci. Numer. Simul. 2020, 91, 105415. [Google Scholar] [CrossRef]
- Han, X.J.; Bi, Q.S.; Zhang, C.; Yu, Y. Study of mixed-mode oscillations in a parametrically excited van der Pol system. Nonlinear Dyn. 2014, 77, 1285–1296. [Google Scholar] [CrossRef]
- Amer, T.S.; Starosta, R.; Almahalawy, A.; Elameer, A.S. The stability analysis of a vibrating auto-parametric dynamical system near resonance. Appl. Sci. 2022, 12, 1737. [Google Scholar] [CrossRef]
- He, J.H.; Amer, T.S.; Abolila, A.F.; Galal, A.A. Stability of three degrees-of-freedom auto-parametric system. Alex. Eng. J. 2022, 61, 8393–8415. [Google Scholar] [CrossRef]
- Abohamer, M.K.; Awrejcewicz, J.; Amer, T.S. Modeling and analysis of a piezoelectric transducer embedded in a nonlinear damped dynamical system. Nonlinear Dyn. 2023, 111, 8217–8234. [Google Scholar] [CrossRef]
- Amer, T.S.; Abdelhfeez, S.A.; Elbaz, R.F. Modeling and analyzing the motion of a 2DOF dynamical tuned absorber system close to resonance. Arch. Appl. Mech. 2023, 93, 785–812. [Google Scholar] [CrossRef]
- Lelkes, J.; Kalmar-Nagy, T. Analysis of a piecewise linear aeroelastic system with and without tuned vibration absorber. Nonlinear Dyn. 2021, 103, 2997–3018. [Google Scholar] [CrossRef]
- Wang, Y.L.; Li, X.H.; Shen, Y.J. Vibration reduction mechanism of Van der Pol oscillator under low-frequency forced excitation by means of nonlinear energy sink. Int. J. Non-Linear. Mech. 2023, 152, 104389. [Google Scholar] [CrossRef]
- Lee, Y.S.; Nucera, F.; Vakakis, A.F.; McFarland, D.M.; Bergman, L.A. Periodic orbits, damped transitions and targeted energy transfers in oscillators with vibro-impact attachments. Physica D. 2009, 238, 1868–1896. [Google Scholar] [CrossRef]
- Starosvetsky, Y.; Gendelman, O.V. Vibration absorption in systems with a nonlinear energy sink: Nonlinear damping. J. Sound Vib. 2009, 324, 916–939. [Google Scholar] [CrossRef]
- Savadkoohi, A.T.; Lamarque, C.H. Dynamics of coupled DAHL type and nonsmooth systems at different scales of time. Int. J. Bifurc. Chaos 2013, 23, 1350114. [Google Scholar] [CrossRef]
- Al-Shudeifat, M.A.; Saeed, A.S. Periodic motion and frequency energy plots of dynamical systems coupled with piecewise nonlinear energy sink. J. Comput. Nonlinear Dyn. 2022, 17, 041005. [Google Scholar] [CrossRef]
- Geng, X.F.; Ding, H.; Mao, X.Y.; Chen, L.Q. A ground-limited nonlinear energy sink. Acta Mech. Sin. 2022, 38, 521558. [Google Scholar] [CrossRef]
- Hong, J.; Jiang, L.M.; Wang, Y.F.; Su, Z.M.; Ma, Y.H. Nonlinear dynamics of an elastic stop system and its application in a rotor system. Appl. Sci. 2022, 12, 5103. [Google Scholar] [CrossRef]
- Li, J.; Zhang, L.N.; Wang, D. Unique normal form of a class of 3 dimensional vector fields with symmetries. J. Differ. Equ. 2014, 257, 2341–2359. [Google Scholar] [CrossRef]
- di Bernardo, M.; Budd, C.J.; Champneys, A.R.; Kowalczyk, P. Piecewise-Smooth Dynamical Systems: Theory and Applications; Springer: London, UK, 2008. [Google Scholar]
- Li, J.; Guo, Z.Y.; Zhu, S.T.; Gao, T. Bifurcation of periodic orbits and its application for high-dimensional piecewise smooth near integrable systems with two switching manifolds. Commun. Nonlinear Sci. Numer. Simul. 2023, 116, 106840. [Google Scholar] [CrossRef]

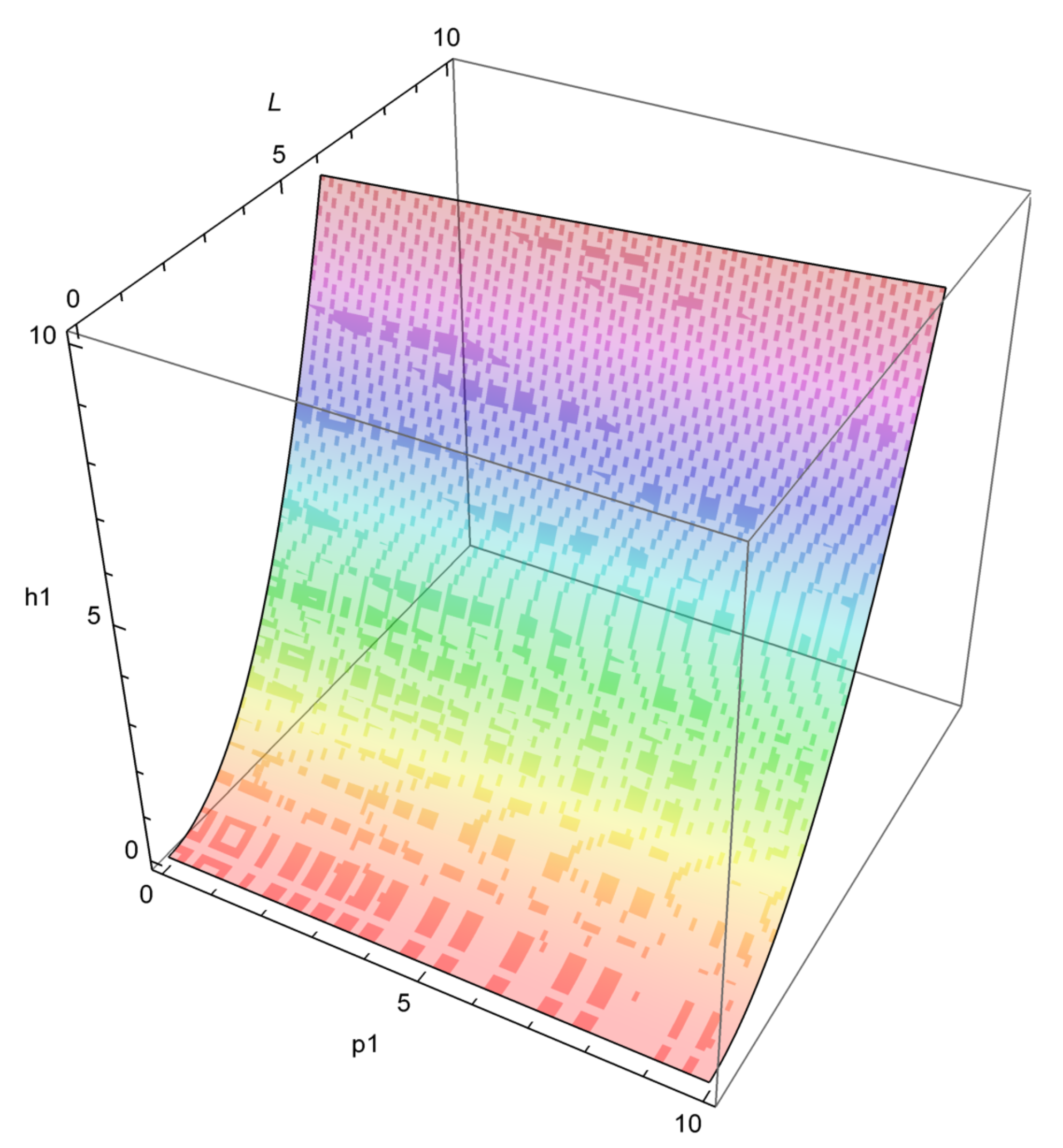
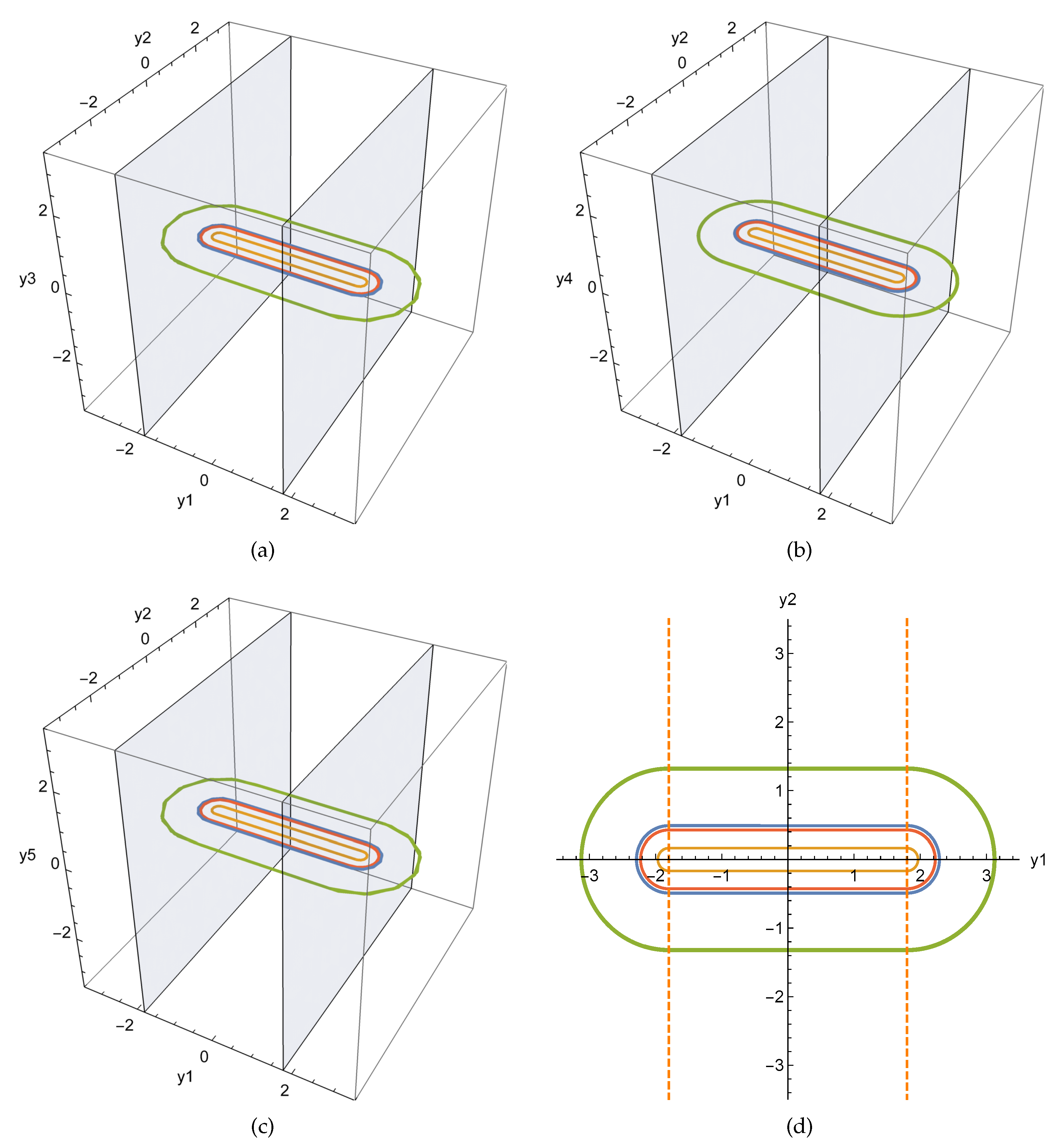
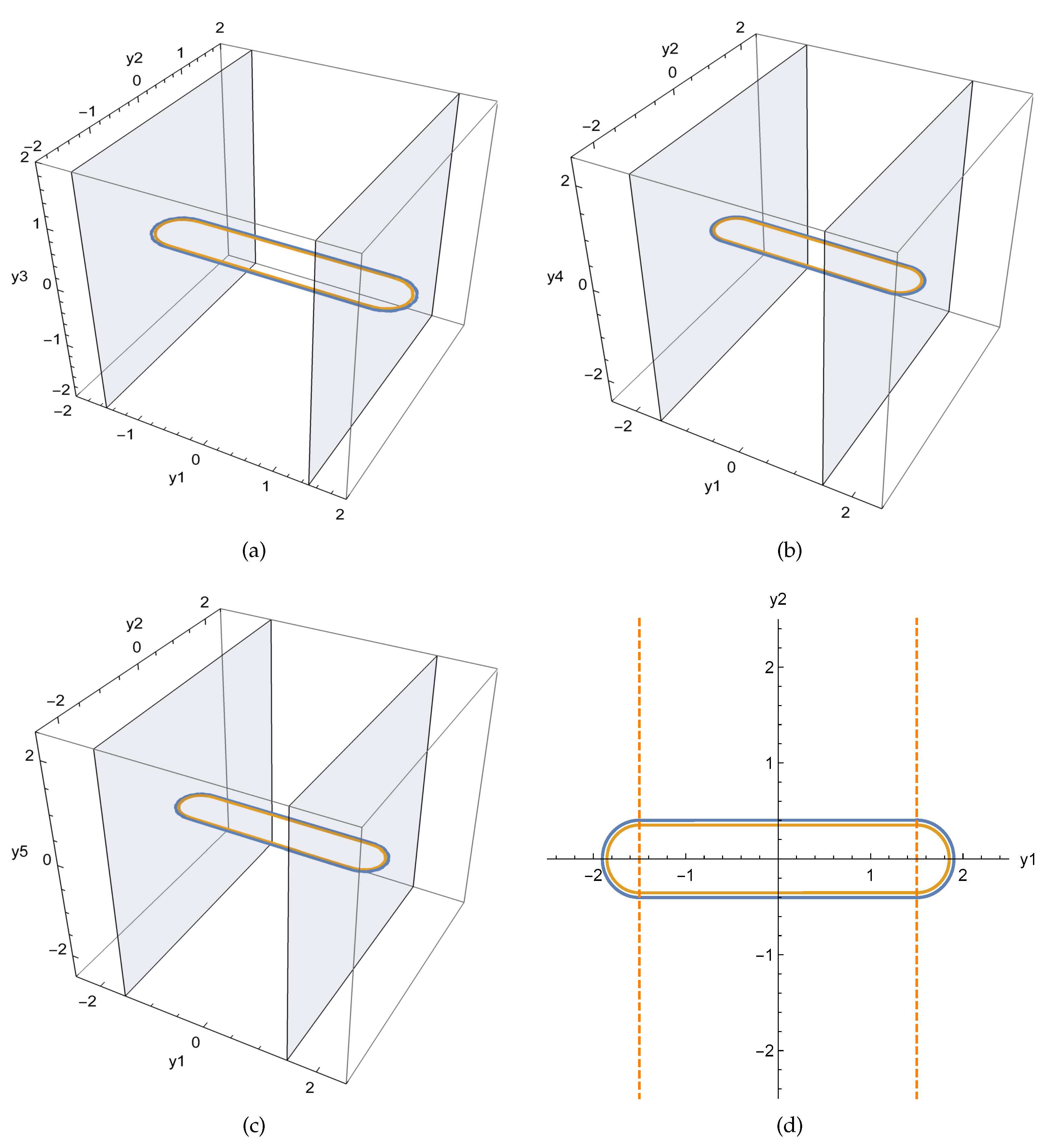
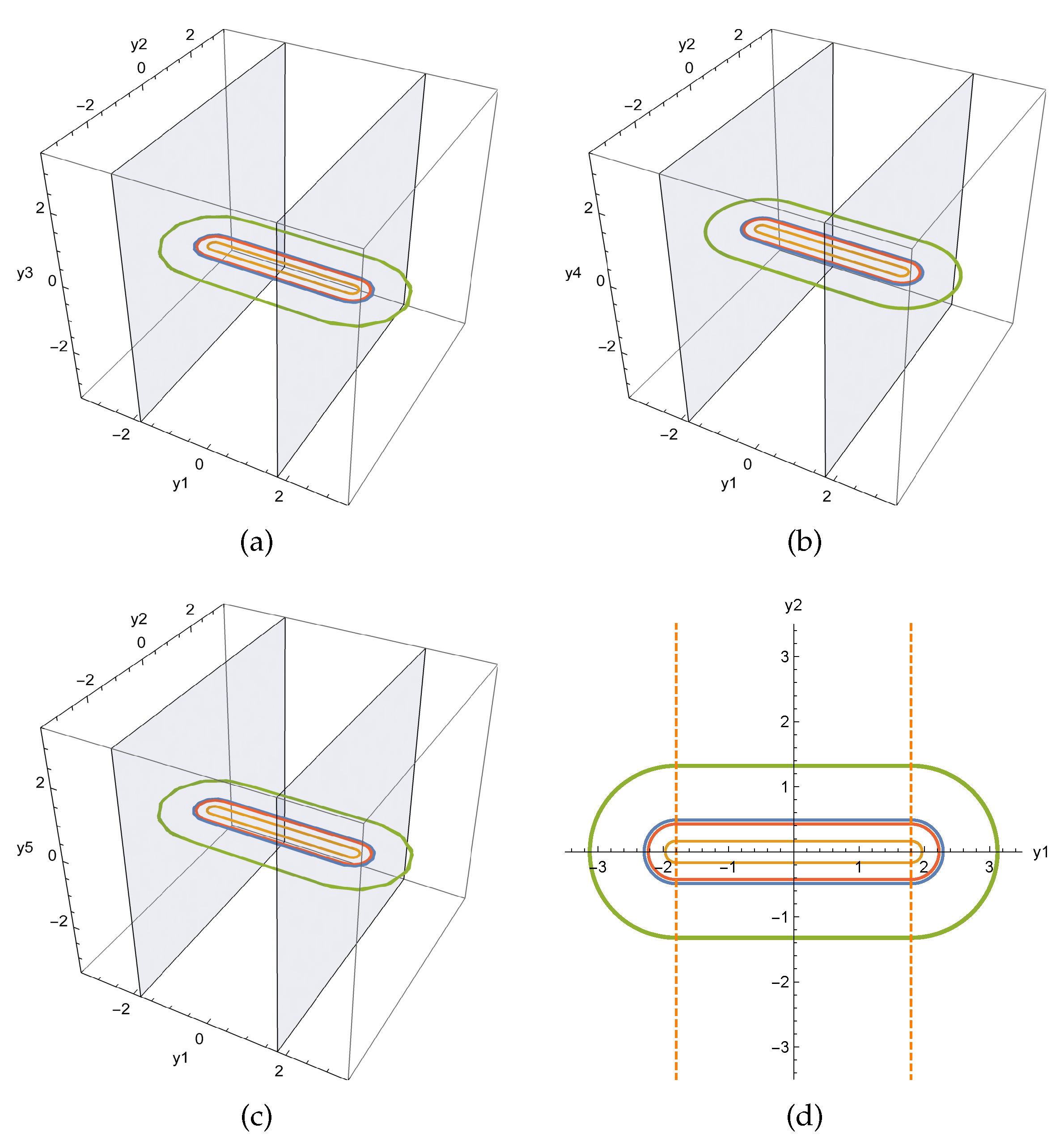

| L | The Number of Periodic Solutions | |
|---|---|---|
| 2 | 50 | 8 |
| 1 | 2 | 4 |
| 1.5 | 18 | 3 |
| 2.5 | 32 | 3 |
| 3 | 72 | 8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Z.; Li, J.; Zhu, S.; Zhang, Y. Stability and Periodic Motions for a System Coupled with an Encapsulated Nonsmooth Dynamic Vibration Absorber. Appl. Sci. 2023, 13, 9006. https://doi.org/10.3390/app13159006
Guo Z, Li J, Zhu S, Zhang Y. Stability and Periodic Motions for a System Coupled with an Encapsulated Nonsmooth Dynamic Vibration Absorber. Applied Sciences. 2023; 13(15):9006. https://doi.org/10.3390/app13159006
Chicago/Turabian StyleGuo, Ziyu, Jing Li, Shaotao Zhu, and Yufeng Zhang. 2023. "Stability and Periodic Motions for a System Coupled with an Encapsulated Nonsmooth Dynamic Vibration Absorber" Applied Sciences 13, no. 15: 9006. https://doi.org/10.3390/app13159006
APA StyleGuo, Z., Li, J., Zhu, S., & Zhang, Y. (2023). Stability and Periodic Motions for a System Coupled with an Encapsulated Nonsmooth Dynamic Vibration Absorber. Applied Sciences, 13(15), 9006. https://doi.org/10.3390/app13159006





