Research on Active Precontrol Strategy for Shape and Performance of Helicopter Spiral Bevel Gears
Abstract
1. Introduction
2. Design of Perfect Surface
3. Analysis of Dynamic Performance
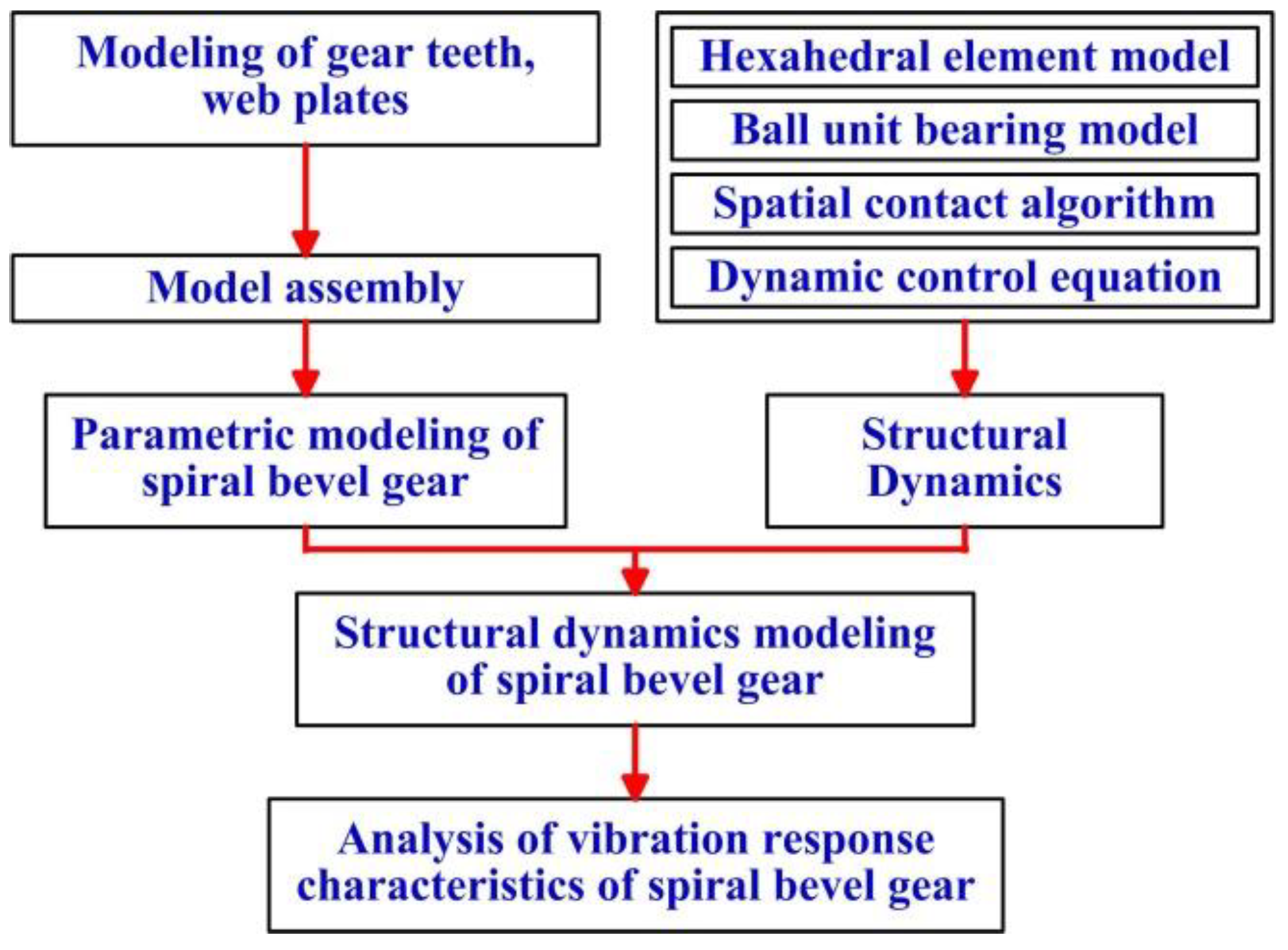
3.1. Dynamic Analysis Based on Lumped Mass Method
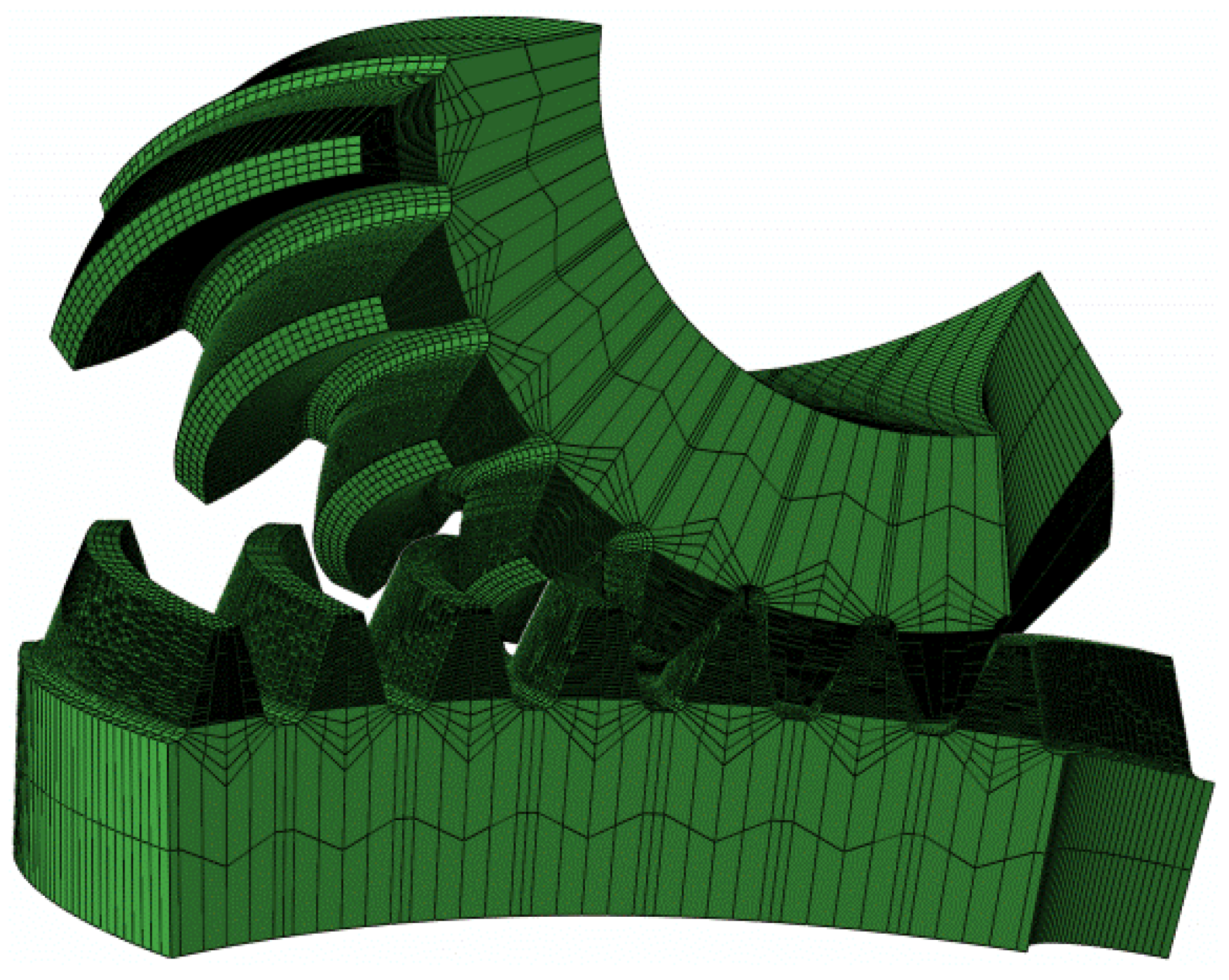
3.2. Structural Dynamics
- (1)
- With the help of the basic theory of vector structural mechanics, a simplified calculation model of a three-dimensional (3D) hexahedron element is constructed, and the calculation expression of the hexahedron element is derived by combining the solution method of reverse motion.
- (2)
- Aimed at the characteristics of high-speed ball bearings in helicopter transmission systems, a ball-bearing unit model is proposed, which simplifies the bearing balls into ball units, whose position information is determined by the centre of the circle.
- (3)
- Drawing on the spatial contact algorithm and combining the contact behaviour characteristics of key components such as high-speed SBG transmissions and bearings, a contact calculation model is constructed. The primary-secondary algorithm is used for a global search, and internal and external algorithms are used for a local search. The spatial contact algorithm equation suitable for vector finite elements is derived.
- (4)
- Drawing inspiration from the meshing damping model in the theory of gear system dynamics, the relative speed of gear and pinion is used as the calculation basis for the meshing damping force to define damping and propose a variable damping model. Based on the variable damping model, the dynamic control equation is derived.
- (5)
- According to the machining principle of the SBG transmissions, parameterised tooth surfaces and transition surfaces are generated based on tooth surface equations with machining parameters as variables, and high-precision finite element 3D models of gear and pinion are constructed.
- (6)
- Based on the relative position and contact relationship between various components, the node coordinates are transformed from the local coordinate system to the assembly coordinate system, completing the assembly of the SBG transmission.
- (7)
- The parameterised model of SBG transmission is combined with vector dynamics to establish a model of vector dynamics of the high-speed SBG transmission system for helicopters.
3.3. Optimization Model Based on Dynamic Performance
4. Numerical Example
5. Conclusions
- (1)
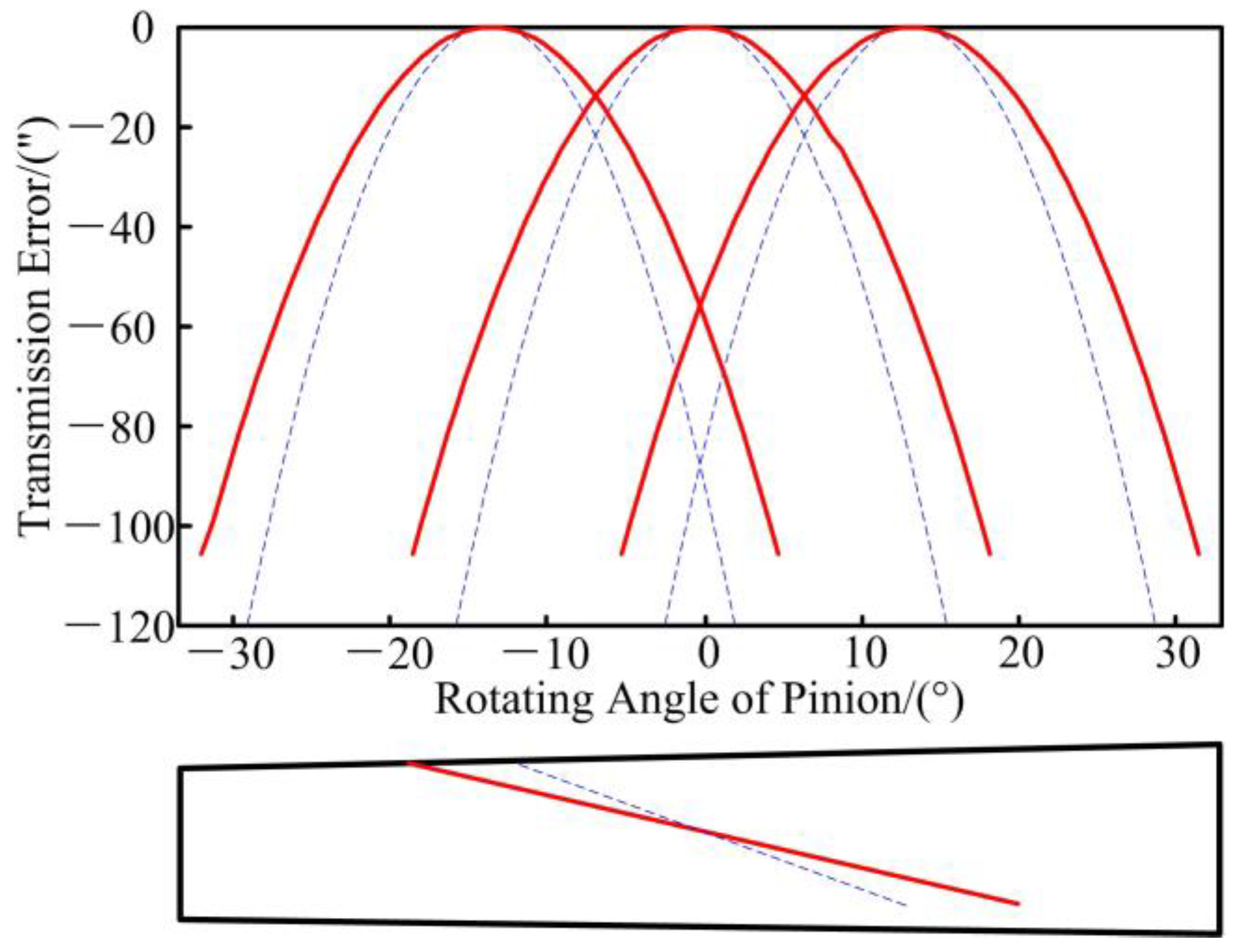
- The MS, LTE and MI are the main internal excitation sources of gears vibration. Combined with TCA, LTCA and MI theory, the MS, LTE and MI of gears are obtained. Based on lumped mass method, the dynamic model is built, and the gear dynamic performance is analysed by solving the differential equation of motion using a numerical method. An optimization model aimed at reducing the RMA of the SBG transmission is established with ease-off, and the redesigned SBG transmission with good dynamic performance is obtained through optimization.
- (2)
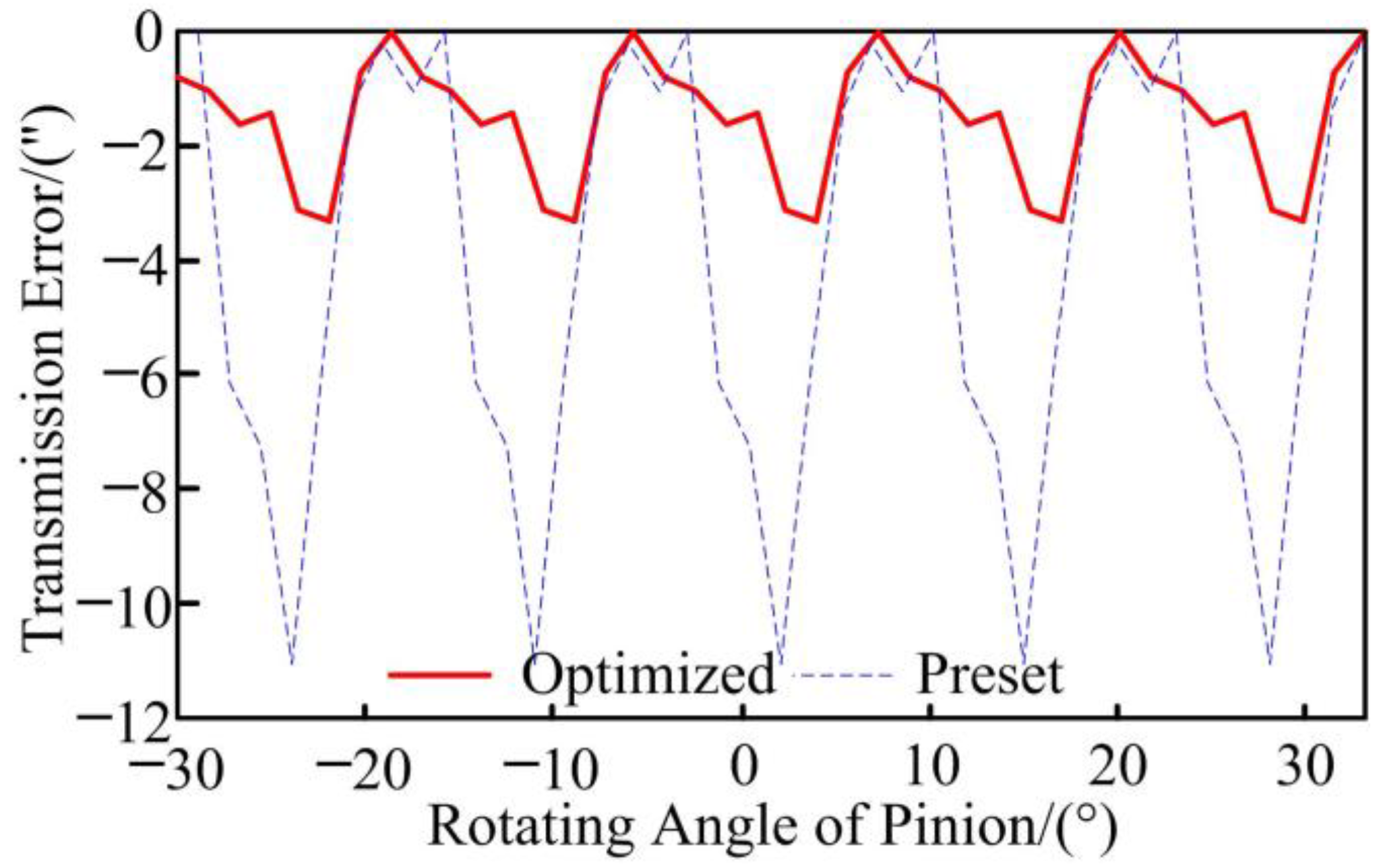
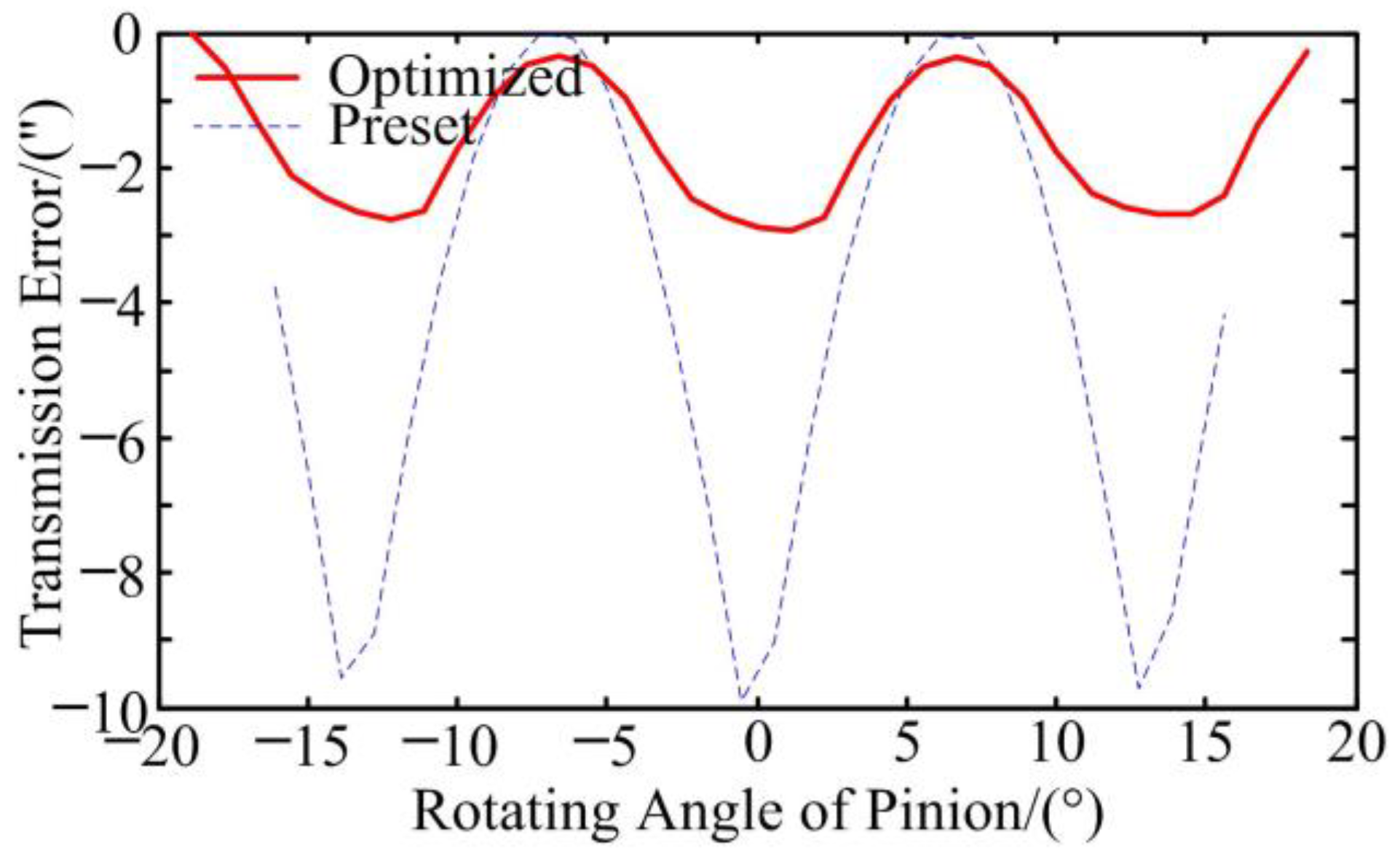
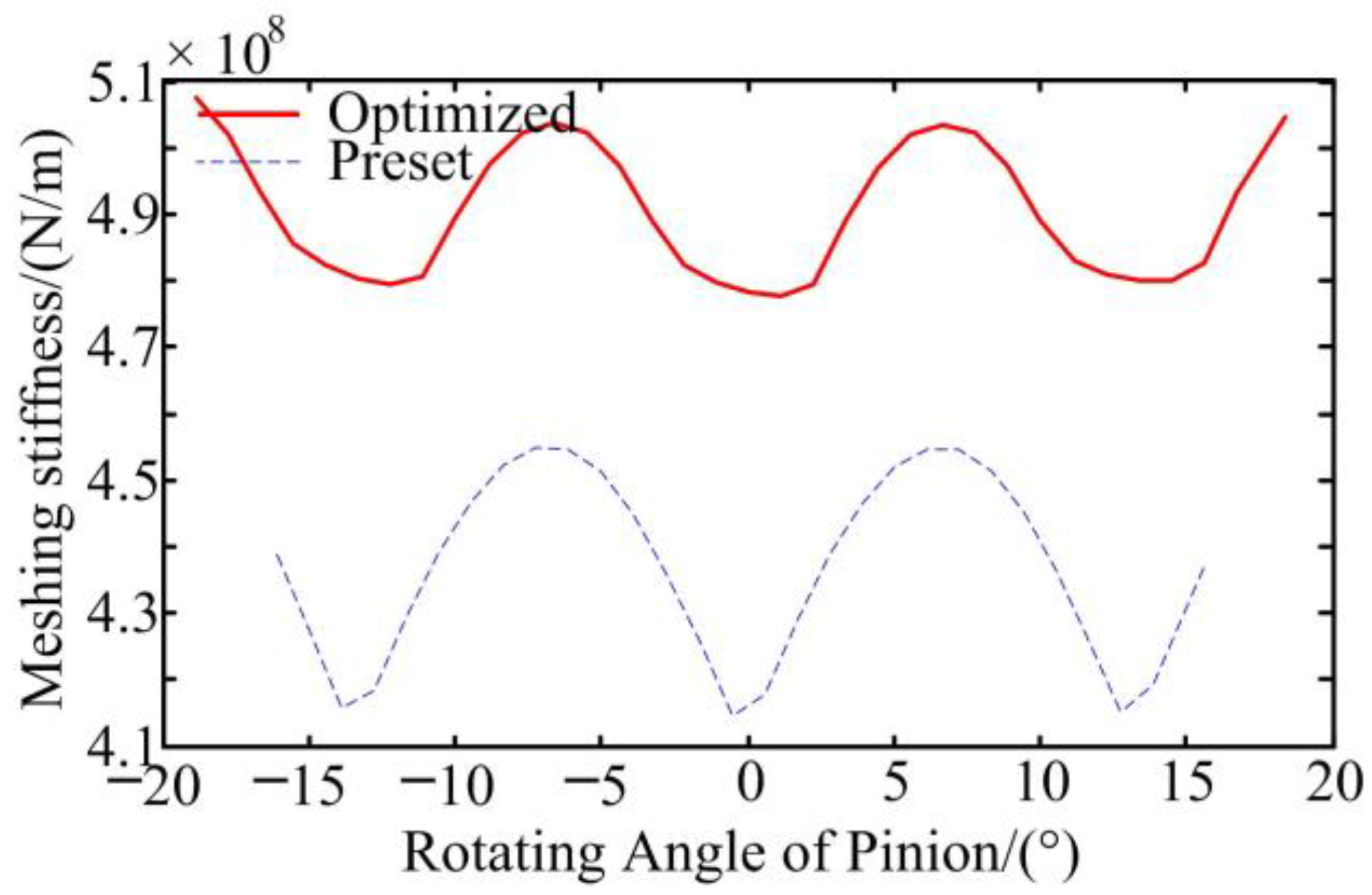
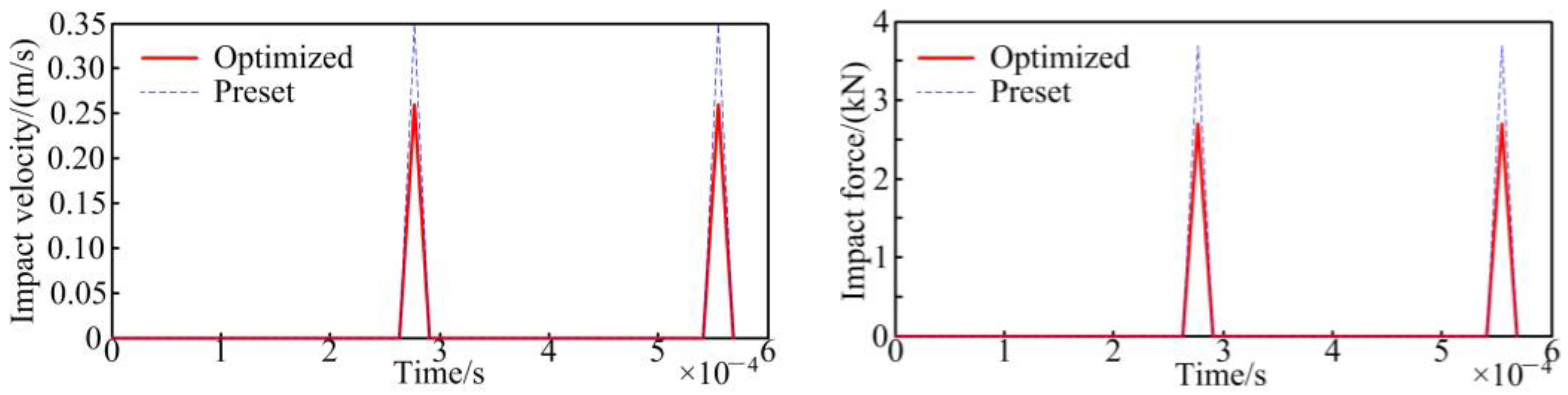
- The LTCA results show that the MS and LTE amplitude of the optimized gear is significantly lower than those of the preset gear, and their accuracy has also been verified using the FEA method. The impact force and impact velocity of the optimized gear are 27.02% and 25.81% lower than the preset gear. Therefore, the meshing quality of the optimized gears is obviously improved.
- (3)
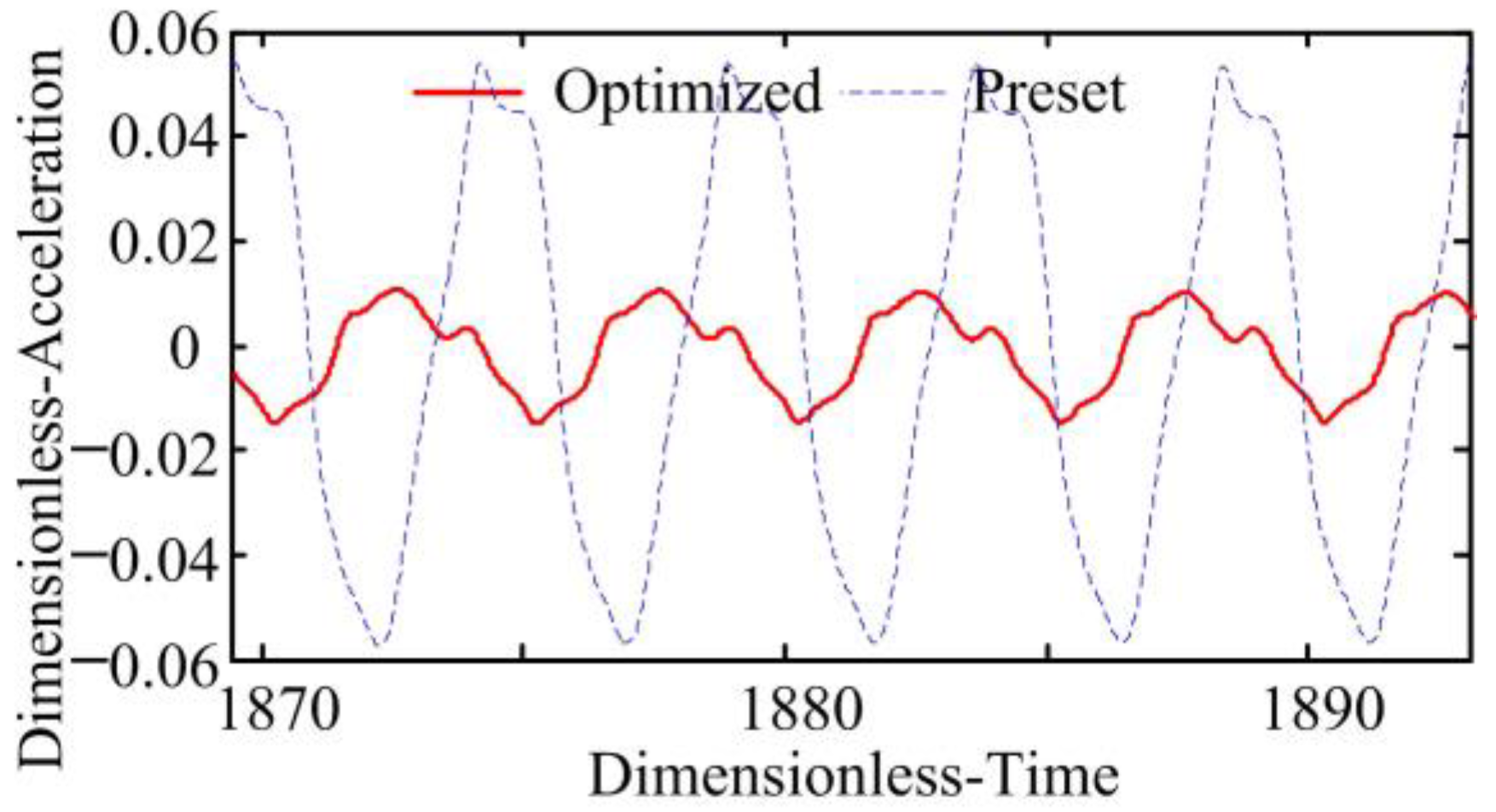
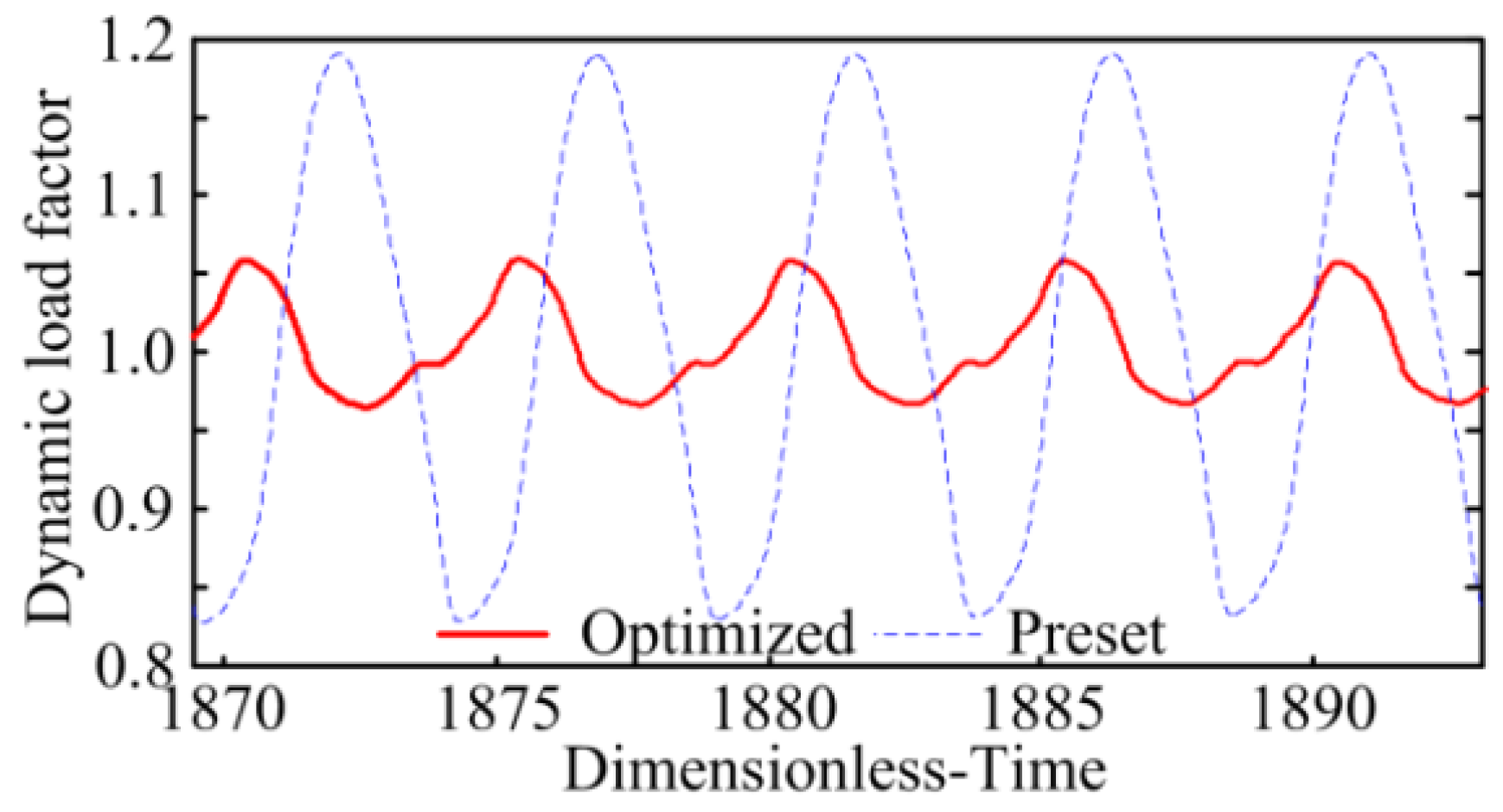
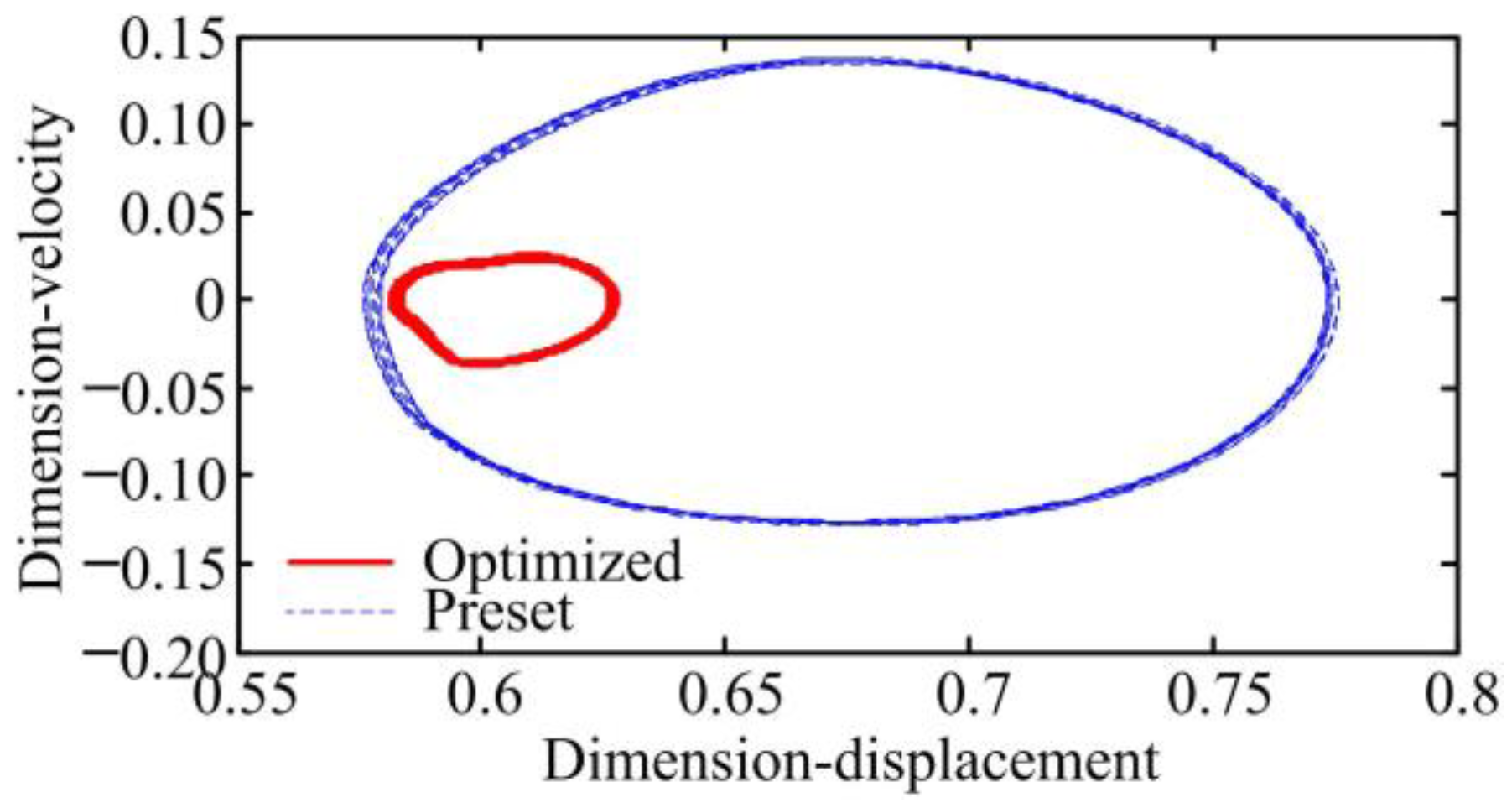
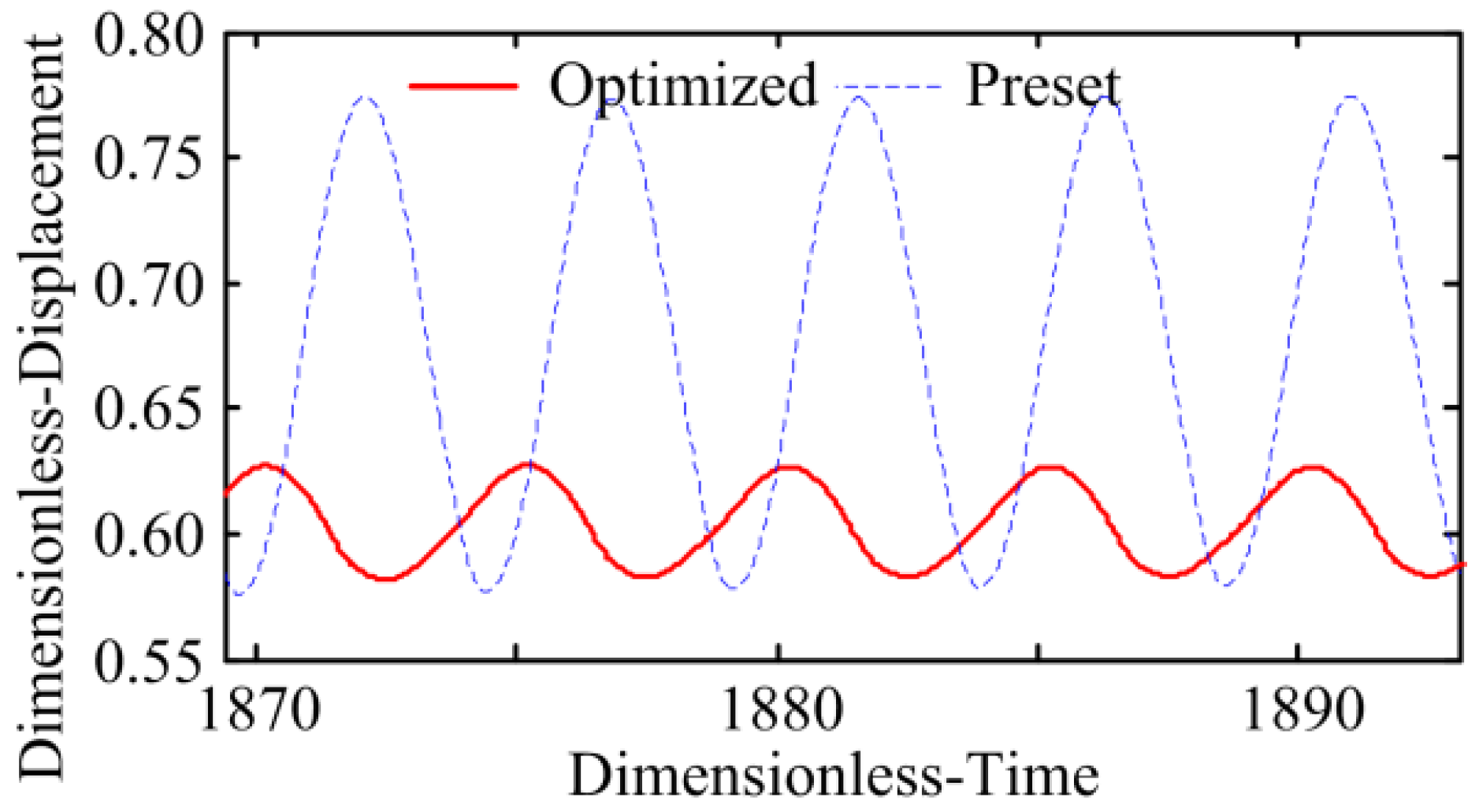
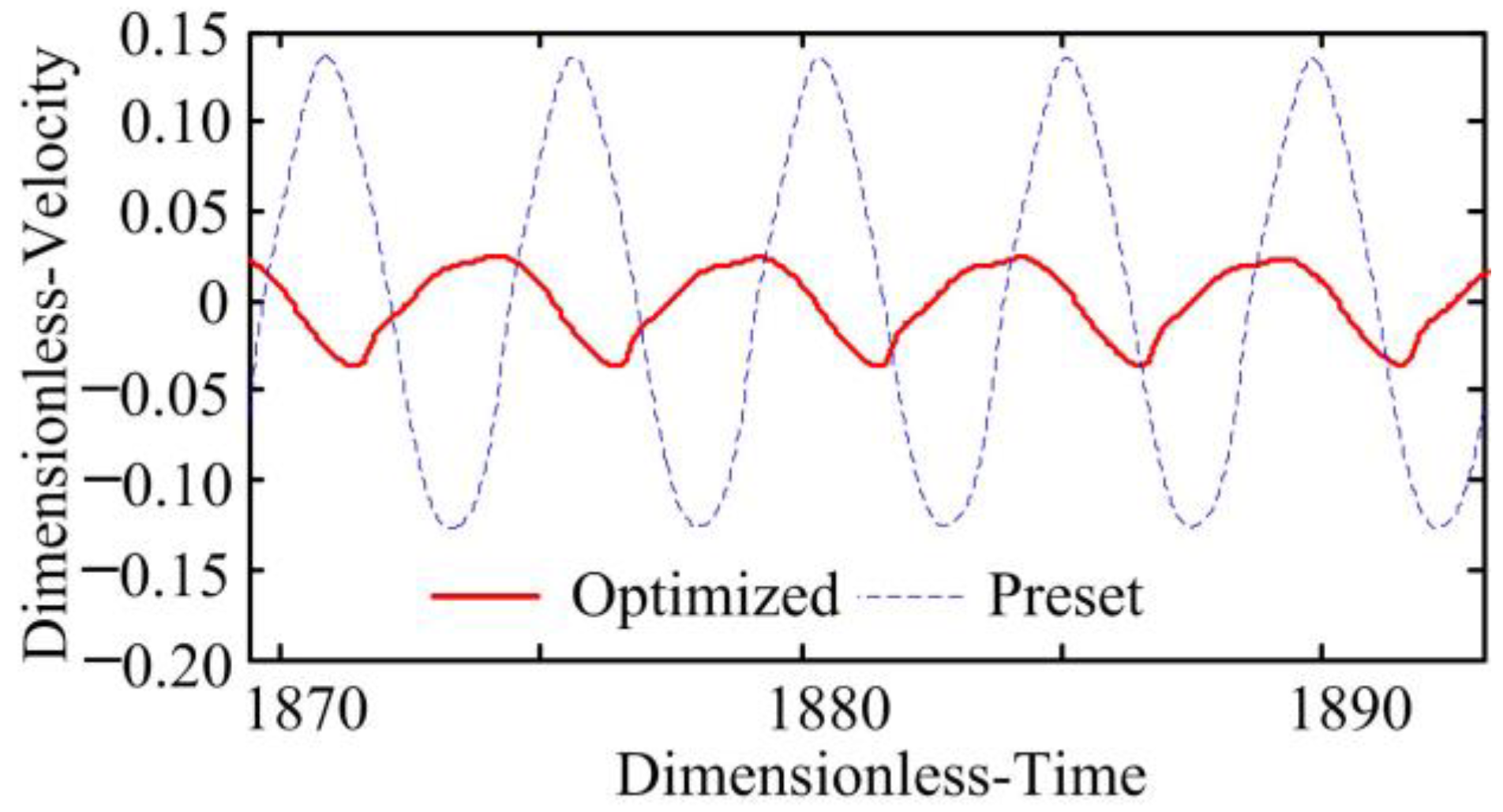
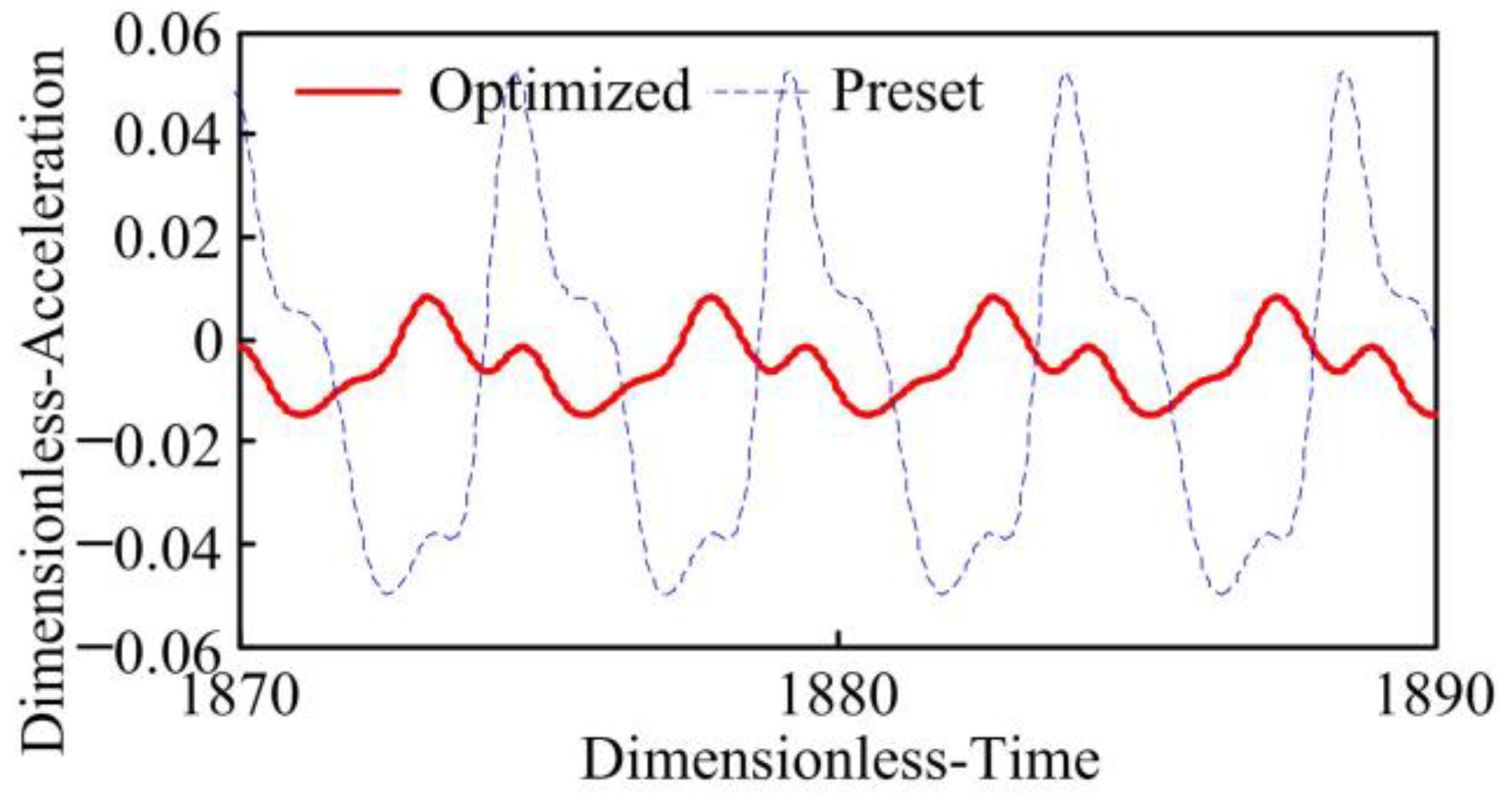
- The results of dynamic analysis based on the lumped mass method show that the dynamic load factor of the optimized gears is much lower than that of the preset gear. The RMA value of the optimized gear is 76.91% lower than that of the preset gear, and they are all validated by the structural dynamics method. The normal vibration velocity and displacement of the optimized gears are obviously lower than the preset gears. Therefore, the running vibration of the gears is cut down after optimization, and their dynamic quality is improved.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tang, J.Y.; Hu, Z.H.; Wu, L.J. Effect of static transmission error on dynamic responses of spiral bevel gear. J. Cent. South Univ. 2013, 22, 640–647. [Google Scholar] [CrossRef]
- Wang, X.; Fang, Z.D.; Mu, Y.M. Optimization design of loaded transmission error for HGT hypoid gear drives. J. Vib. Shock 2017, 36, 34–40. (In Chinese) [Google Scholar]
- Simon, V. Design of face-hobbed spiral bevel gears with reduced maximum tooth contact pressure and transmission errors. Chin. J. Aeronaut. 2013, 26, 21–37. [Google Scholar] [CrossRef]
- Simon, V. Optimal machine-tool settings for the manufacture of face-hobbed spiral bevel gears. ASME J. Mech. Des. 2014, 136, V005T11A001. [Google Scholar] [CrossRef]
- Simon, V. Manufacture of optimized face-hobbed spiral bevel gears on computer numerical control hypoid generator. J. Manuf. Sci. Eng. Trans. Asme 2014, 136, 031008. [Google Scholar] [CrossRef]
- Simon, V. Optimization of face-hobbed hypoid gears. Mech. Mach. Theory 2014, 77, 164–181. [Google Scholar] [CrossRef]
- Simon, V. Design and manufacture of spiral bevel gears with reduced transmission errors. ASME J. Mech. Des. 2015, 131, 041007–041017. [Google Scholar] [CrossRef]
- Simon, V. Optimal machine tool settings for face-hobbed hypoid gears manufactured on CNC hypoid generator. Int. J. Adv. Manuf. Technol. 2017, 88, 1579–1594. [Google Scholar] [CrossRef]
- Astoul, J.; Mermoz, E.; Sartor, M. New methodology to reduce the transmission error of the spiral bevel gears. CIRP Ann. Manuf. Technol. 2014, 63, 165–168. [Google Scholar] [CrossRef]
- Artoni, A.; Bracci, A.; Gabiccini, M. Optimization of the loaded contact pattern in hypoid gears by automatic topography modification. ASME J. Mech. Des. 2009, 31, 011008. [Google Scholar] [CrossRef]
- Artoni, A.; Kolivad, M.; Kahraman, A. An ease-off based optimization of the loaded transmission error of hypoid gears. ASME J. Mech. Des. 2011, 132, 011010. [Google Scholar] [CrossRef]
- Kolivand, M.; Kahraman, A. A load distribution model for hypoid gears using ease-off topography and shell theory. Mech. Mach. Theory 2009, 44, 1848–1865. [Google Scholar] [CrossRef]
- Kolivand, M.; Kahraman, A. An ease-off based method for loaded tooth contact analysis of hypoid gears having local and global surface deviations. ASME J. Mech. Des. 2010, 132, 071004. [Google Scholar] [CrossRef]
- Jiang, J.K.; Fang, Z.D.; Liu, Z. Design of multi-objective tooth optimization for hypoid gear with ease-off topological modification. J. Xi’an Jiaotong Univ. 2013, 53, 44–53. (In Chinese) [Google Scholar]
- Jiang, J.K.; Liu, Z.; Fang, Z.D. Optimization design of vibration reduction for hypoid gears with ease-off flank modification. J. South China Univ. Technol. Nat. Sci. Ed. 2020, 48, 138–145+152. (In Chinese) [Google Scholar]
- Cao, X.M.; Zhou, Y.W.; Deng, X.Z. Relationship between transmission error, contact ratio and noise of spiral bevel gear. J. Mech. Transm. 2003, 27, 43–45. (In Chinese) [Google Scholar]
- Kong, X.N.; Ding, H. Adaptive data-driven modeling, prediction and optimal control for loaded transmission error of helicopter zerol spiral bevel gear transmission system. Mech. Mach. Theory 2021, 165, 104417. [Google Scholar] [CrossRef]
- Mu, Y.M.; He, X.M.; Fang, Z.D. Wave tooth surface design of high contact ratio spiral bevel gear with minimum loaded transmission error. J. Aerosp. Power 2021, 36, 2080–2089. [Google Scholar]
- Yang, J.J.; Shi, Z.H. Dynamic analysis of spiral bevel and hypoid gears with high-order transmission errors. J. Sound Vib. 2018, 417, 149–164. [Google Scholar] [CrossRef]
- Litvin, F.L.; Cutman, Y. A method of local synthesis of gears grounded on the connection between the principal and geodetic curvatures of surfaces. ASME J. Mech. Des. 1981, 103, 114–125. [Google Scholar] [CrossRef]
- Litvin, F.L.; Zhang, Y. Local Synthesis and Tooth Contact Analysis of Face-Milled Spiral Bevel Gears; NASA Lewis Research Center: Chicago, IL, USA, 1991. [Google Scholar]
- Tian, X.B.; Fang, Z.D. Overall optimization on machine-tool settings of spiral bevel gears. J. Aerosp. Power 2000, 15, 75–77. (In Chinese) [Google Scholar]
- Shih, Y.P. A novel ease-off flank modification methodology for spiral bevel and hypoid gears. Mech. Mach. Theory 2010, 45, 1108–1124. [Google Scholar] [CrossRef]
- Shih, Y.P.; Chen, S.D. A flank correction methodology for a five-axis CNC gear profile grinding machine. Mech. Mach. Theory 2012, 47, 31–45. [Google Scholar] [CrossRef]
- Su, J.Z.; He, Z.X. High-precision modification of tooth surface for spiral bevel gears. J. South China Univ. Technol. Nat. Sci. Ed. 2014, 42, 91–96. (In Chinese) [Google Scholar]
- Artoni, A.; Gabiccini, M. Multi-objective ease-off optimization of hypoid gears for their efficiency, noise, and durability performances. ASME J. Mech. Des. 2010, 133, 267–277. [Google Scholar]
- Artoni, A.; Gabiccini, M. Ease-off based compensation of tooth surface deviations for spiral bevel and hypoid gears: Only the pinion needs corrections. Mech. Mach. Theory 2012, 61, 1108–1124. [Google Scholar] [CrossRef]
- Wei, B.Y.; Li, J.Q. Calculation of gear mesh stiffness and loaded tooth contact analysis based on ease-off surface topology. Adv. Mech. Eng. 2022, 14, 16878132221133979. [Google Scholar] [CrossRef]
- Chen, P.; Wang, S.M.; Li, F. A direct preset method for solving ease-off tooth surface of spiral bevel gear. Mech. Mach. Theory 2022, 179, 105123. [Google Scholar] [CrossRef]
- Peng, X.L.; Zhang, L. Manufacturing process for a face gear drive with local bearing contact and controllable unloaded meshing performance based on ease-off surface modification. J. Mech. Des. 2016, 138, 043302. [Google Scholar] [CrossRef]
- Wang, Q.; Zhou, C. An ease-off based approach to designing a high-order transmission motion for face-milled spiral bevel gears. Int. J. Veh. Des. 2019, 81, 241–264. [Google Scholar] [CrossRef]
- Mu, Y.M.; He, X.M.; Fang, Z.D. Design and dynamic performance analysis of high-contact-ratio spiral bevel gear based on ease-off technology. Int. J. Precis. Eng. Manuf. 2021, 22, 1963–1973. [Google Scholar] [CrossRef]
- Mu, Y.M.; He, X.M.; Fang, Z.D. An innovative ease-off flank modification method based on the dynamic performance for high-speed spiral bevel gear with high-contact-ratio. Mech. Mach. Theory 2021, 162, 104345. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, J.K. Design and analysis for hypoid gears with ease-off flank modification. Appl. Sci. 2022, 12, 822. [Google Scholar] [CrossRef]
- Li, G.; Zhu, W.D. An active ease-off topography modification approach for hypoid pinions based on a modified error sensitivity analysis method. J. Mech. Des. 2019, 141, 093302141. [Google Scholar] [CrossRef]
- Mu, Y.M.; He, X.M.; Fang, Z.D. Multiobjective ease-off optimization of high-speed spiral bevel gear for loaded meshing performance. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 382. [Google Scholar] [CrossRef]
- Ting, E.C.; Shih, C.; Wang, Y.K. Fundamentals of a vector form intrinsic finite element: Part II. Plane solid elements. J. Mech. 2004, 20, 123–132. [Google Scholar] [CrossRef]
- Hou, X.Y.; Fang, Z.D.; Zhang, X.J. Static contact analysis of spiral bevel gear based on modified VFIFE (vector form intrinsic finite element) method. Appl. Math. Model. 2018, 60, 192–207. [Google Scholar] [CrossRef]
- Su, J.Z.; Fang, Z.D.; He, Z.X. Study on dynamic of spiral bevel gears based on meshing characteristics. Mech. Sci. Technol. Aerosp. Eng. 2016, 35, 50–55. [Google Scholar]
- Mu, Y.M.; Fang, Z.D.; Li, W.L. Impact analysis and vibration reduction design of spiral bevel gears. Proc. Inst. Mech. Eng. Part K J. Multi Body Dyn. 2019, 233, 668–676. [Google Scholar] [CrossRef]
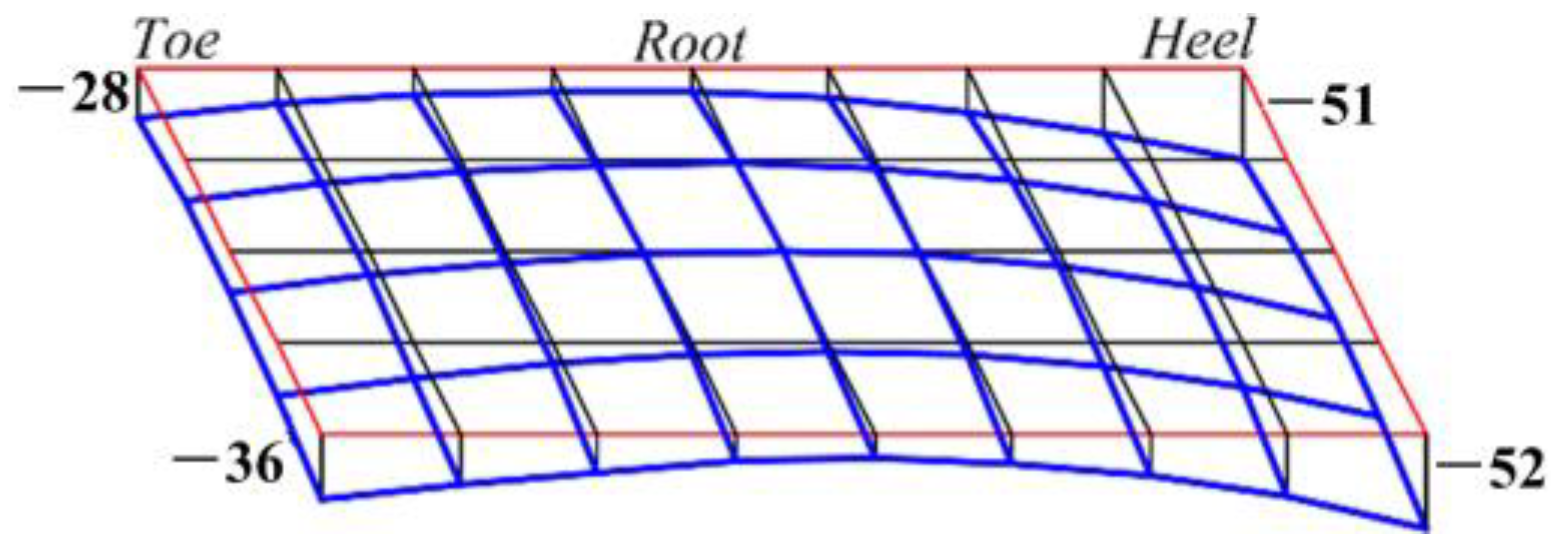

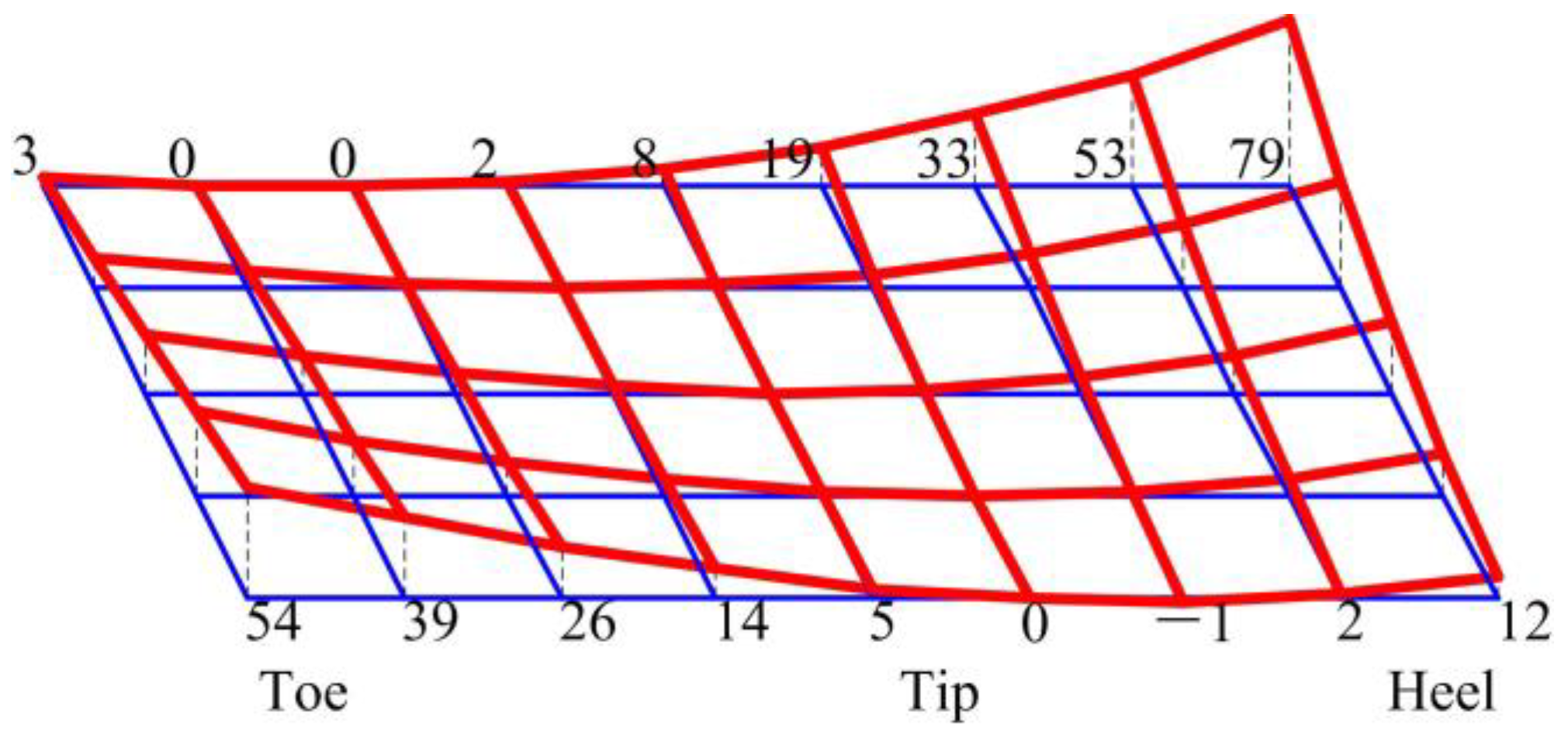


| Parameter | Pinion | Gear |
|---|---|---|
| Number of teeth | 27 | 74 |
| Module/mm | 3.85 | |
| Pressure angle/(°) | 20 | |
| Mean spiral angle/(°) | 30 | |
| Hand of spiral | LH | RH |
| Shaft angle/(°) | 87 | |
| Face width/mm | 40 | |
| Parameter | Gear |
|---|---|
| Radial setting/mm | 119.687 |
| Initial cradle angle setting/(°) | −43.568 |
| Roll ratio | 1.084 |
| Vertical offset/mm | 0 |
| Increment of machine centre to back/mm | 0 |
| Sliding base feed setting/mm | −1.741 |
| Machine root angle/(°) | 66.005 |
| Parameter | Preset | Optimized |
|---|---|---|
| Radial setting/mm | 115.269 | 113.106 |
| Initial cradle angle setting/(°) | 43.535 | 43.111 |
| Roll ratio | 2.884 | 2.834 |
| Vertical offset/mm | −3.584 | −5.580 |
| Increment of machine centre to back/mm | −2.063 | −3.226 |
| Sliding base feed setting/mm | 0.070 | 0.446 |
| Machine root angle/(°) | 18.897 | 18.897 |
| Second-order coefficients | 0.059 | 0.039 |
| Third-order coefficients | 0.003 | 0.019 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mu, Y.; Hou, X.; He, X. Research on Active Precontrol Strategy for Shape and Performance of Helicopter Spiral Bevel Gears. Appl. Sci. 2023, 13, 8527. https://doi.org/10.3390/app13148527
Mu Y, Hou X, He X. Research on Active Precontrol Strategy for Shape and Performance of Helicopter Spiral Bevel Gears. Applied Sciences. 2023; 13(14):8527. https://doi.org/10.3390/app13148527
Chicago/Turabian StyleMu, Yanming, Xiangying Hou, and Xueming He. 2023. "Research on Active Precontrol Strategy for Shape and Performance of Helicopter Spiral Bevel Gears" Applied Sciences 13, no. 14: 8527. https://doi.org/10.3390/app13148527
APA StyleMu, Y., Hou, X., & He, X. (2023). Research on Active Precontrol Strategy for Shape and Performance of Helicopter Spiral Bevel Gears. Applied Sciences, 13(14), 8527. https://doi.org/10.3390/app13148527





