P System with Fractional Reduction
Abstract
1. Introduction
2. Fundamentals of Membrane Computing
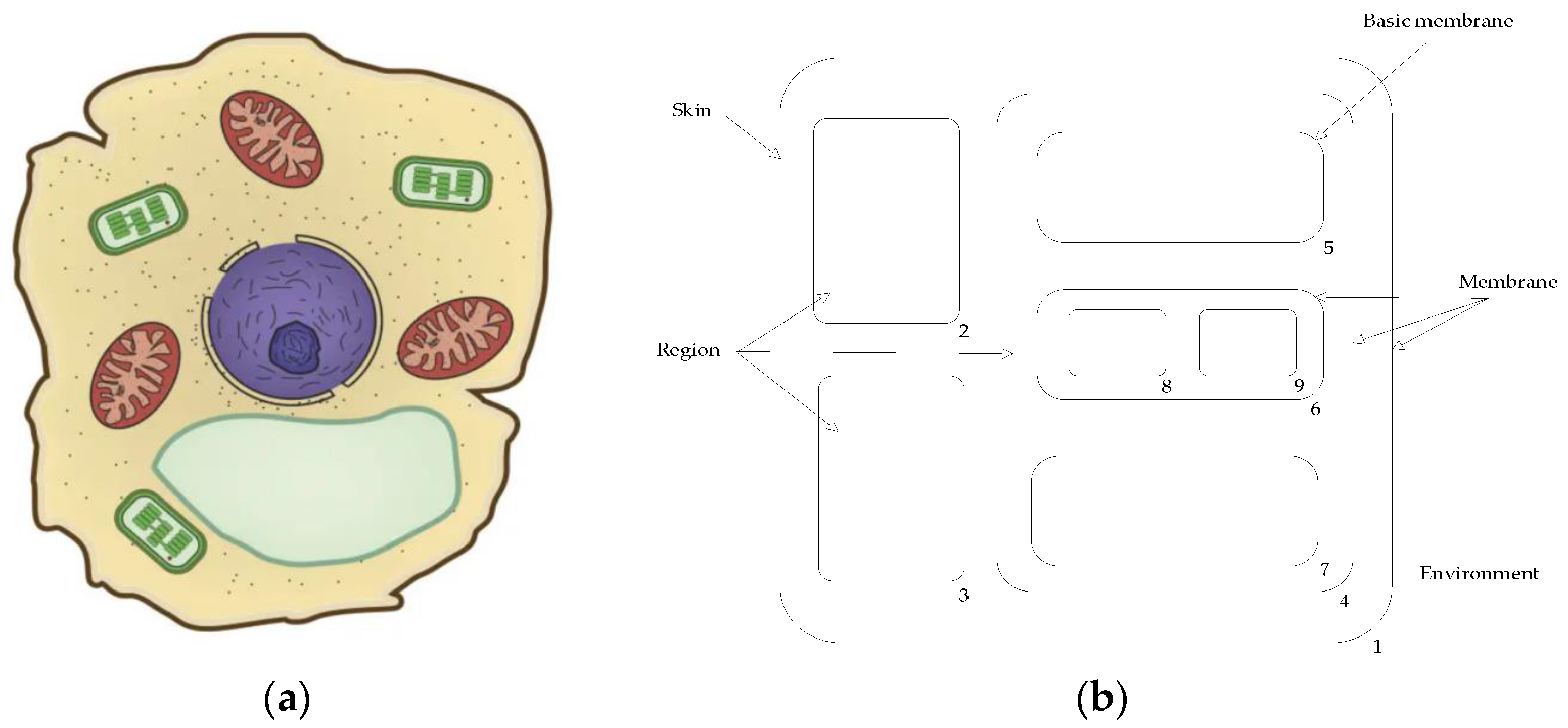
2.1. Biological Basis of Membrane Computation
- (1)
- The skin separates the cell from its external environment, creating an enclosed area inside the cell that contains the internal space of the cell.
- (2)
- The inner membrane divides the cell into regions with a hierarchical structure, within each of which local biochemical reactions take place.
- (3)
- The cell membrane acts as a communication channel between regions.
2.2. Definition of the Cell-Like P System
- (1)
- Non-determinism
- (2)
- Maximum parallelism
3. Principles and Algorithms of Fraction Simplification
3.1. The More Phase Derogation Algorithm
3.1.1. Principle
3.1.2. Improvement of the More Phase Derogation Algorithm
3.1.3. Algorithms
- (i)
- Input ;
- (ii)
- Calculate , where
- (iii)
- Calculate , where
- (iv)
- Output .
| Algorithm 1: Algorithm for fraction simplification using more phase derogation. |
| Input: Output: p1, q1, p1/q1 is the simplest form of x/y fractions. procedure Input: Output: p1, q1, p1/q1 is the simplest form of x/y fractions. procedure
|
3.2. Division Algorithm
3.2.1. Principle
3.2.2. Algorithm
- (i)
- Input ;
- (ii)
- Calculate , where
- (iii)
- Output .
| Algorithm 2: Algorithm for fractional reduction using the division algorithm. |
| Input: Output: k, l, k/l is the simplest form of x/y fractions. procedure
|
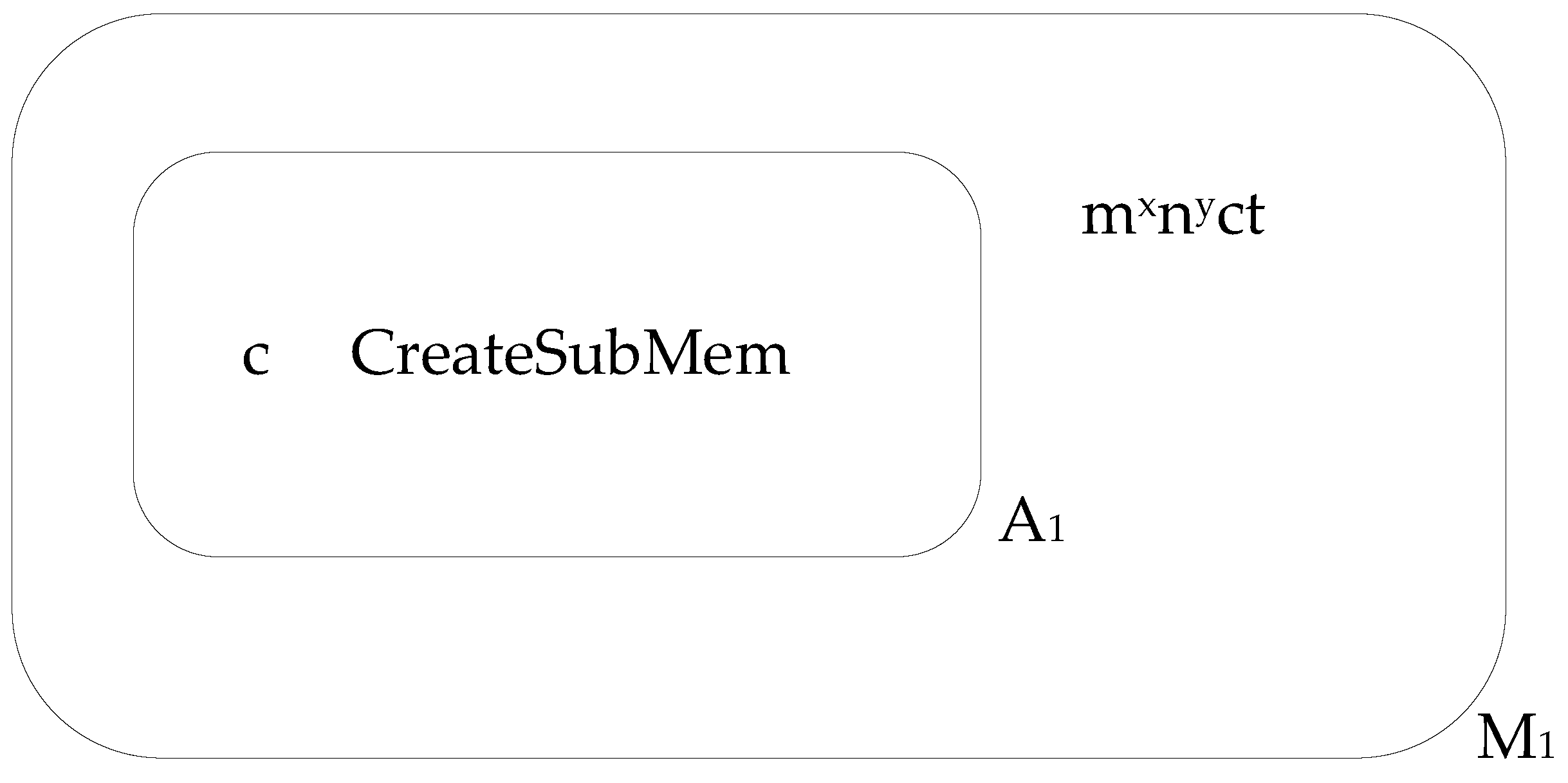
4. Fractional Simplification P System
4.1. P System for Fractional Simplification of More Phase Derogation Algorithm
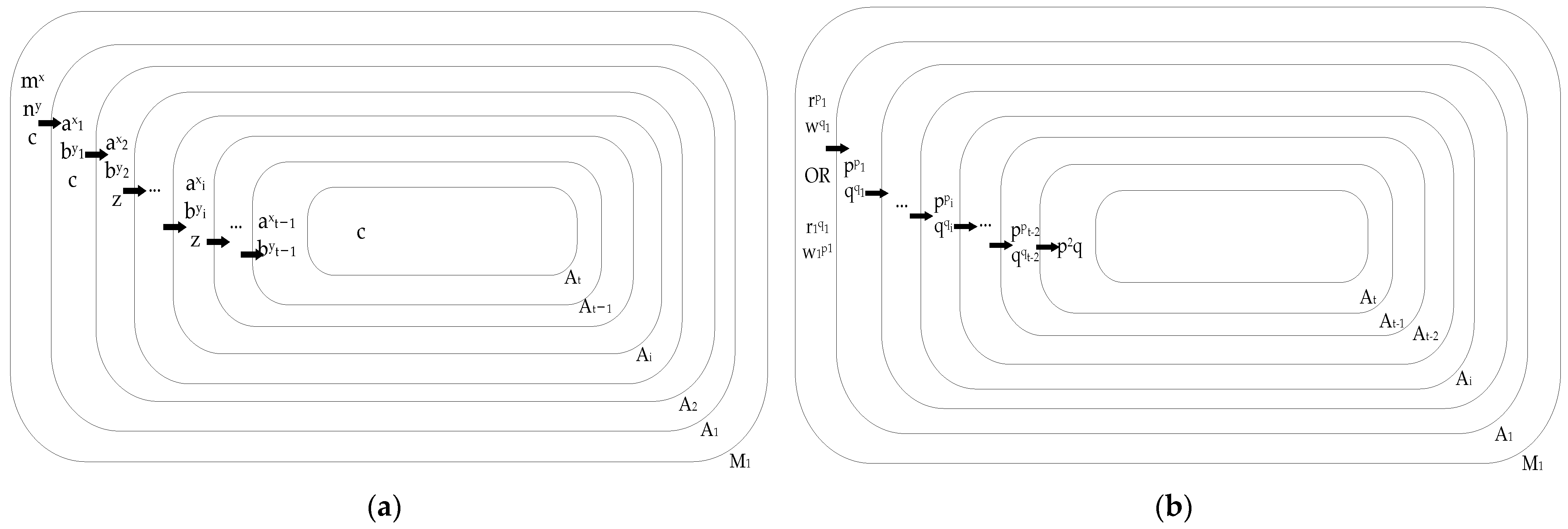
4.1.1. Definition of the P System for More Phase Derogation Algorithm
- (i)
- In the initial state, a partial rule is executed in membrane to determine the magnitude of the numerator x and denominator y, passing the larger and smaller values to object a and object b in membrane , respectively. For e.g., if , then the rule is executed to generate a multiset in membrane , i.e., in the corresponding sequence.
- (ii)
- In membrane , the multiset is consumed by partial execution of the rule, a judgment is made as to whether is produced while a new membrane is produced, and the multiset is produced by passing some material into the submembrane. This step is repeated until no new membranes are generated when the stopping condition for more phase loss is met in membrane , and the computation of the sequence , , is completed.
- (iii)
- In general, within the membrane (2 ≤ i < t − 1), the multisets and generated by substances transported by the membrane are then transformed into new multisets into the submembrane according to the corresponding rules, respectively, until the end when is equal to .
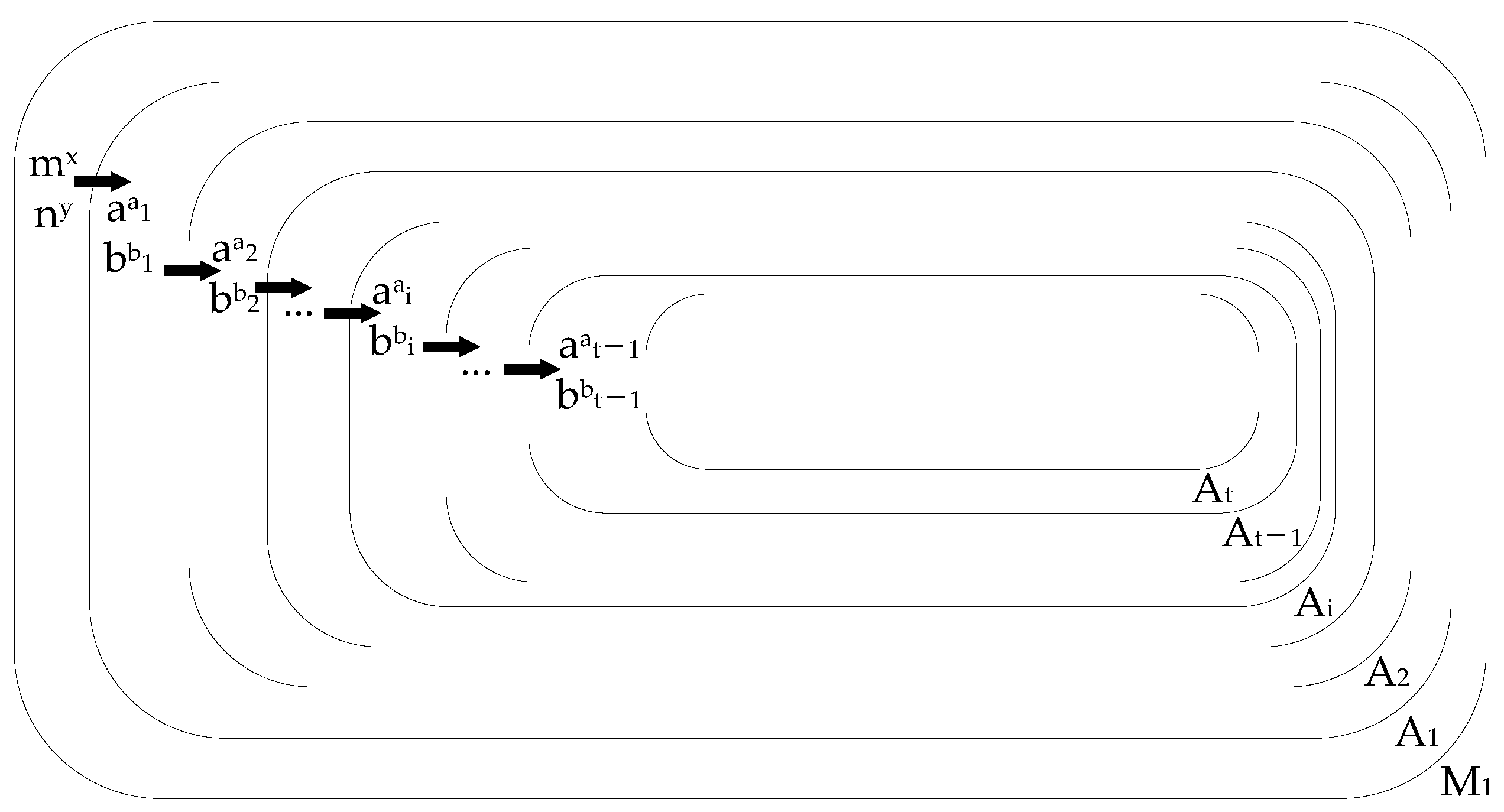
4.1.2. Membrane Rules for the More Phase Derogation Algorithm
- (i)
- When x > y, the membrane rules are executed in the following order: . Execution of rule , which corresponds to converting object n to g, also leaves x − y m in the membrane System. Executing under the condition that both g and m are present backs up the difference of x − y in the membrane as a multiset , while generating a multiset to pass into the submembrane .
- (ii)
- When x < y, the membrane rules are executed in the following order: . Executing rule , there is no remaining m in the membrane, so executing backs up the excess n in the membrane as multiset , while generating multiset to pass into submembrane .
- (i)
- Execution of rule : In , objects a and b are consumed to generate the multiset , indicating that the numerator and denominator values are consumed in both copies, leaving copies of object a. Matter x and y are used to back up the subtractor b and to compare with the difference , respectively. Rule is executed under the condition of the existence of object c. The multiset is generated by executing , where l is used to backup the difference , and u is used to compare the size with the subtraction y.
- (ii)
- Execute rule under the condition that there is an object u: c generates object k and f, k is used to control the loop, and f is used to check the size of and . If > , the rules are executed in the first order. If < , the rules are executed in the second order. When = , the dynamic generation of submembranes has ended, and will be discussed in the subsequent calculation of sequence , , which will not be explained in detail here.
- (iii)
- Execute rule and y and u evolve into v. Compare the size of and by observing which object remains within the environment. If there is still y in the membrane after evolving into v, then = , = , and in the child membrane ; otherwise, = , = , and (i.e., there is no substance z in the child membrane). So when y is still present within the environment, rules and are executed with object v as catalyst.
- (iv)
- Before executing , we must first execute to consume and a Num in the membrane to generate a child membrane, and then we can subsequently pass the object into the child membrane. Each class A membrane is initialized with a , and Num is passed from the parent membrane to the submembrane; to ensure sufficient Num, the number of initialized Num is . Execute and : object k generates a new c and sends it to the submembrane; if , then y and f evolves to i and generates a new object z and sends it to the submembrane; then, execute after consuming a Num; the remaining Num is passed into the submembrane for the submembrane to dynamically generate membranes.
- (v)
- If object i exists, it is shown that both a and b values of the submembrane need to be changed, for which rules and are executed: passes the subtractive backup x to the a of the submembrane (i.e., = ) and passes the differential backup l to the b of the submembrane (i.e., = ). If u is left in the environment, rules and are executed with object v as the catalyst. When h is generated in the environment, it is proved that = in the submembrane . Therefore, and are executed to pass the subtractive backup to x to the submembrane and the differential backup l to in the submembrane, respectively. The above steps are executed several times until the dynamic generation ends when = 2.
- (i)
- The order of the execution of the rules in membrane is {}. To avoid confusion, the following explanation is given: in the membrane rule, means that the membrane contains 2 objects p, and p1 means that the System contains an object named “p1”.
- (a)
- Since the final step of the method of finding the maximum convention by the more phase derogation algorithm is that the subtraction is equal to the difference, and this equal value is the sought maximum convention, in the absence of objects y,u,a, and b, which is the case when proving that the subtractors are equal to the difference after executing rule , the object f evolves to object (i.e., ) by executing rule , which means that the subtracted number in membrane is two times the subtracted number.
- (b)
- Then execute rules and : p and q evolve into and , respectively, for saving and in this membrane, while generating objects r and w into the parent membrane for backup. Execute rule : when there is z in the membrane, evolve z to object o and release it to the parent membrane.
- (ii)
- Execution order of the rule in membrane : or (
- (a)
- Under the condition that an object o exists, prove the existence of z in the submembrane, which requires changing . Execute rules : the multiset evolves into the multiset and the multiset evolves into the multiset (at this time ). Under the condition that there is no object o, execute rules and : the multiset evolves into the multiset , and the multiset evolves into the object (at this time ).
- (b)
- Then execute and to pass and in this membrane to the parent membrane, and if there is z in this membrane, execute to generate o to pass to the parent membrane.
- (c)
- The above steps are repeatedly executed until membrane finally generates the results and . Execute rules and to output the multiset , where and are the values after simplification of the larger and smaller values in the numerator denominator, respectively.
- (d)
- Finally, the comparison is performed in membrane : if the numerator ≥ denominator, the result / is directly derived (i.e., the quantity ratio of objects r and w), and if the numerator < denominator, and are replaced in membrane as and , respectively, and the final result is / (i.e., the quantity ratio of objects and ).
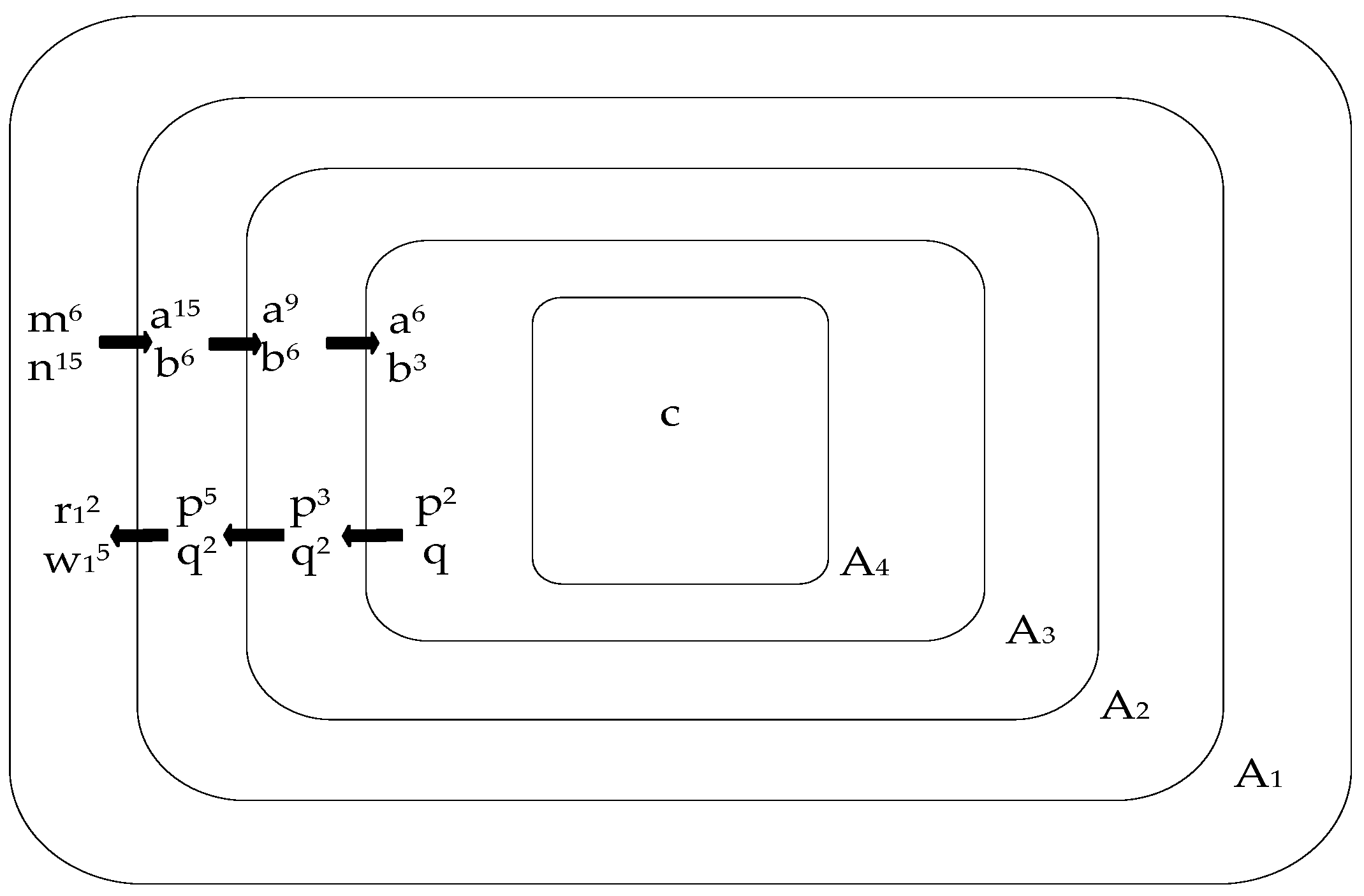
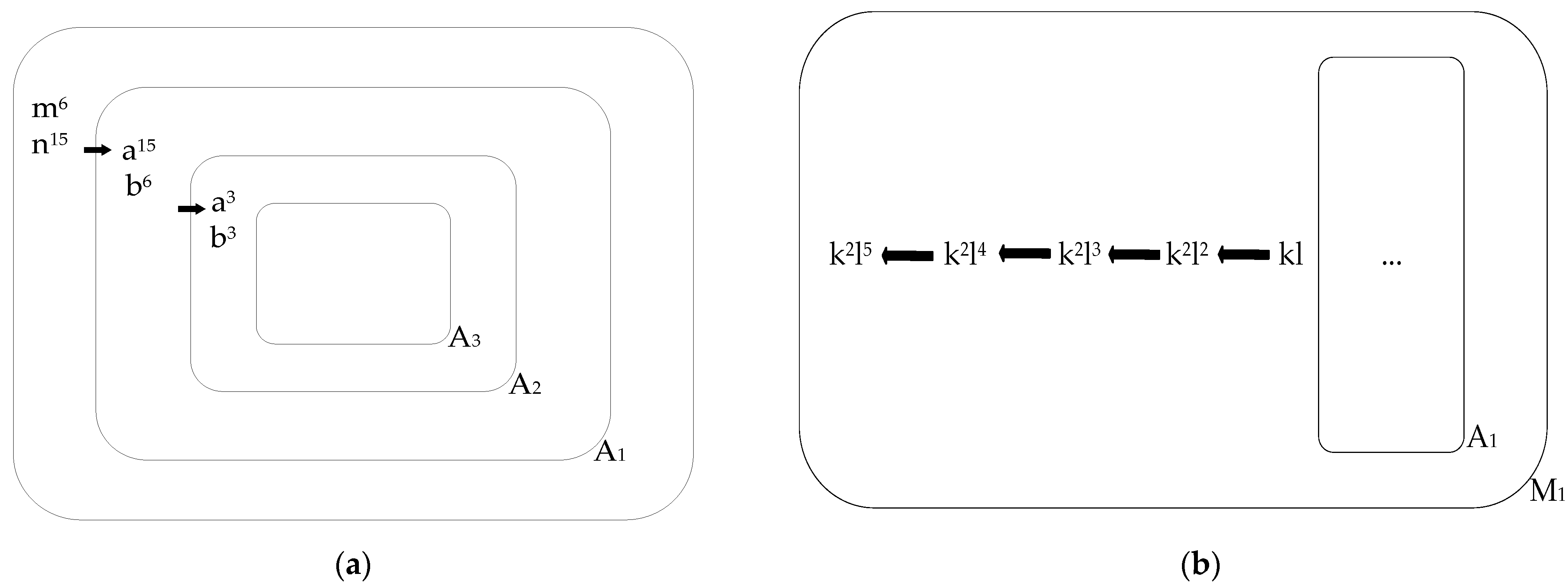
4.1.3. Example of Fraction Simplification by the More Phase Derogation Method
- (i)
- The rules available in membrane are executed in the following order: {, ,}{,}.
- (a)
- Execute the rule 6 times in the membrane until is completely consumed, generating the multiset and remains; execute the rule under the condition that the object c exists, generating .
- (b)
- At this point, only the rule can be executed: evolve c to the multisets k and f under the condition that the object u exists; execute the rule six times to generate the multiset , at which point, the multiset in the membrane is . Execute the rule , as described in Section 4.1.2, consuming the CreateSubMem and a Num in this membrane to generate the membrane .
- (c)
- Execute the rules {,, } within the same time slice. Execute the rule to generate a new object c from k into the control loop in membrane ; execute the rule so that the multiset u and f is consumed to generate object h, and execute to pass the remaining Num into the submembrane for subsequent continuation of submembrane generation.
- (d)
- The next time slice executes {, } at the same time. Execute the rule 6 times to evolve into the multiset and send into the membrane , execute at the same time to evolve into the multiset and send it into the membrane .
- (ii)
- At this time, there are multiple sets in membrane . Execute the rules in the following order: {, ,}{,}
- (a)
- After executing the membrane rule in the order of , the multiset within the membrane is , and a new submembrane is generated.
- (b)
- As described in Section 4.1.2, the next time slice simultaneously executes the rule {,,}: execute the rule , object k evolves into object c to be sent to membrane ; while executing the rule , object y and object f evolves into object i and sends z to membrane , and executes the rule to pass the remaining Num to membrane .
- (c)
- Execute the rules {,} under the condition that object i exists. The rule is executed 6 times, and the multiset evolves into the multiset into the membrane , while the rule is executed 3 times, and the evolves into the multiset into the membrane .
- (i)
- At this time, the multiset in the membrane is . The P System executes the rules in the following order: {,,}.
- (a)
- Execute the rule , f generate the multiset ; at this point, the multiset in the membrane is ;
- (b)
- The next time slice simultaneously executes the rules {,,}: execute to evolve into the multiset , and generate the multiset into the membrane ; execute the rule to evolve into the multiset , generate the object w into the membrane , and execute the rule to generate the multiset o of object z to output into .
- (ii)
- At this time, the multiset in membrane is and the order of execution of the rules is {,}{,}.
- (a)
- Under the condition that the object o exists, execute the rules {,}: w generates the multiset p, generates the multiset , at which point, the multiset in is ;
- (b)
- Next, execute the rule {,} to generate the multiset within the membrane , and also generate the multiset to pass into the membrane .
- (iii)
- At this time, the multiset in membrane is , and the order of execution of the rules is {,}{,}.
- (a)
- Execute the rule {,}, the multiset generates the multiset , and the multiset generates the multiset . At this time, the multiset in the membrane is .
- (b)
- Then execute {,} to pass out r and w, respectively. In the membrane, obtains , corresponding with the numerator and denominator, and then converts it to . After that there is no rule to execute, so the whole System stops. So the final simplification of 6/15 results in 2/5.
4.2. P System for Fraction Simplification in the Division Algorithm
4.2.1. Definition of the P System for Rolling Division
4.2.2. Membrane Rule of Division Algorithm
- (i)
- When the rules are executed in the following order: {,}.
- (a)
- Execution of rule within membrane : consume the multiset , generating , which means that the values of the denominator and numerator are consumed in copies at the same time, leaving copies of the object , while executing to generate a new submembrane ; execution of rule : object c evolves into y under the condition that object x exists; this step is used to control the loop, while execution passes the remaining Num into the submembrane ;
- (b)
- Then execute , the object y evolves into object d, where plays a catalytic role; under the condition that d exists, execute rule ; the object x evolves into object b and returns to . At this time, the existing multiset in the membrane is , and the System satisfies the execution conditions of and continues to generate x.
- (c)
- Execute , object d evolves into object c; at this time, there are x generated in the membrane , so the execution conditions of are satisfied again. Because there is only one CreateSubMem object in the membrane, and in a membrane can only be executed once, but the rule may be cyclically executed n times, until stops the cycle of execution, or proves that the remainder is 0; then, the System has found the maximum convention x and stops the cycle of execution.
- (ii)
- When in the membrane , the rules are executed in the following order: .
- (a)
- Execution rule : the multiset is consumed to generate the multiset . This means that the values of the denominator and numerator are consumed in both copies, leaving copies of object b. At this time, the number of x is the remainder of divided by .
- (b)
- Under the condition that object x exists, rule is executed to evolve object c into y. This step is used to control the loop; rule is executed, and object y evolves into objects e and g under the action of catalyst b.
- (c)
- Execute rules : consume CreateSubMem with a Num to generate a new submembrane under the condition that object b exists, while g evolves into object h for synchronization control.
- (d)
- Then execute the rules : under the condition that the object h exists, convert the multiset to multiset directly into the submembrane and the multiset to multiset into the submembrane; at this time, and , while the object h evolves into c into the membrane to continue the execution. In membrane , we continue to judge the size relationship between and and execute the corresponding rules.
- (iii)
- When , the rules are executed in the following order: .
- (a)
- Rule , is executed as above. At this time, there is no excess a or b in the membrane, proving that the two numbers have no remainder, which also means that they have been integregated; therefore, the execution of rule : the object y evolves into z and e. Under the condition that there is z, the execution of rule converts the multiset into the multiset directly into the parent membrane, i.e., is the maximum common divisor.
- (b)
- In membranes after receiving the of the submembrane, rule is executed to output the maximum common divisor, and finally output it from membrane to membrane . Calculate k and l according to the rules in membrane .
- (i)
- The rules involved in simplifying the molecule x and the order of execution are as follows: .
- (a)
- Execute rule : The multiset evolves into . At this point, object i retains the number of conventions , and there are objects a remaining in the System. Because is the largest convention of the numerator denominator, object a must be an integer multiple of .
- (b)
- In the condition that object i exists, execute rule : object c evolves into object y. This step is used to control the loop; execute : object y evolves into object d under the action of catalyst a. This step proves that there is no end to integer division in the System, at this time;
- (c)
- Execute rule under the condition that object d exists: object i evolves into object w, and then the value of the convention number is passed to w; at this point, execution conditions are met, and rule is executed while continuing to execute : object d evolves into objects k and c, i.e., the convention number is subtracted once for one more k, which is equivalent to a counter.
- (d)
- The above steps can be executed several times. Until there is no object a in the System after executing again, it indicates that the molecules have been completely divided. Execution rule and : object y evolves into object k. The last number of k is the value of the numerator after the approximate simplification, and also, the number of times the subtraction is used.
- (ii)
- The rules involved in simplifying the denominator and the order of execution are as follows: . The execution rules are similar to the steps for dividing the numerator by the greatest common divisor, and the final number of l is the value of the denominator after simplification. Due to the maximum parallelism of the membrane calculation, the number of steps required to implement the division function is the number of steps in the larger value simplification.
4.2.3. Example of Fraction Simplification by the Division Algorithm
- (i)
- Execute rules in the following order: . In membrane execute rule 6 times to generate object , while executing rule , under the condition of having b directly consume CreateSubMem and a Num to generate submembrane for backup. The existing object in Membrane is . Execute rule to evolve object c to y under the condition that object x exists, while executing to pass the remaining Num value into submembrane .
- (ii)
- At this time a < b, the rules are executed in the order of ; execute the rule three times to generate the multiset ; execute the rule , and the object c evolves into the object y; thus, at this time the multiset in the membrane is ; this case can only execute the rule , converting the multiset b and y to the multiset b,e,g; then execute the rule , converting the object to ; under the condition that exists at the same time, execute the rules , generating passed to membrane .
- (iii)
- At this time, the multiset in membrane is , i.e.,, according to ; execute the rule three times, consume all the a and b to generate , while executing to generate submembrane ; execute the rule , the multiset c evolves into the multiset y, and at this time, the multiset in the membrane is , while executing the rule to pass the remaining Num values to the submembrane . In this environment, one can only execute the rule to evolve y into the multiset z and e; execute under the condition that the multiset z exists, and rule transforms into and passes it to membrane ; rule continues in membrane and passes the multiset to membrane , where the number of objects x is the maximum convention . This concludes the computation of the maximum convention .
- (i)
- Execute rule in membrane , backing up the maximum convention as for simultaneous simplification of the numerator and denominator; execute rule three times, consuming the multiset to generate , and execute rule three times simultaneously, consuming the multiset to generate ;
- (ii)
- Execute rule to evolve object c into y under the condition that object i exists, and the execution of the rule involving the evolution of multiset to multiset under the condition that object h exists;
- (iii)
- Simultaneous execution of the rules ,, evolving y to d in the presence of catalyst a and e to h in the presence of catalyst b.
- (iv)
- Simultaneously execute the rules ,, under the condition that d exists, the object evolves into , and evolves into . Then simultaneously execute the rules , , evolve d into and h into , respectively; at this time, k and l start to accumulate, and the multiset in the membrane is .
- (v)
- After the numerator is repeated once in the order and the denominator is repeated once in the order , the multiset inside the membrane is . Since a has been completely consumed, the rule is stopped and the denominator continues to be executed. After repeating the execution three times in sequence, the multiset within the membrane is now , at which point, only rule can be executed and object e evolves to l. At this time, the multiset in the membrane is .
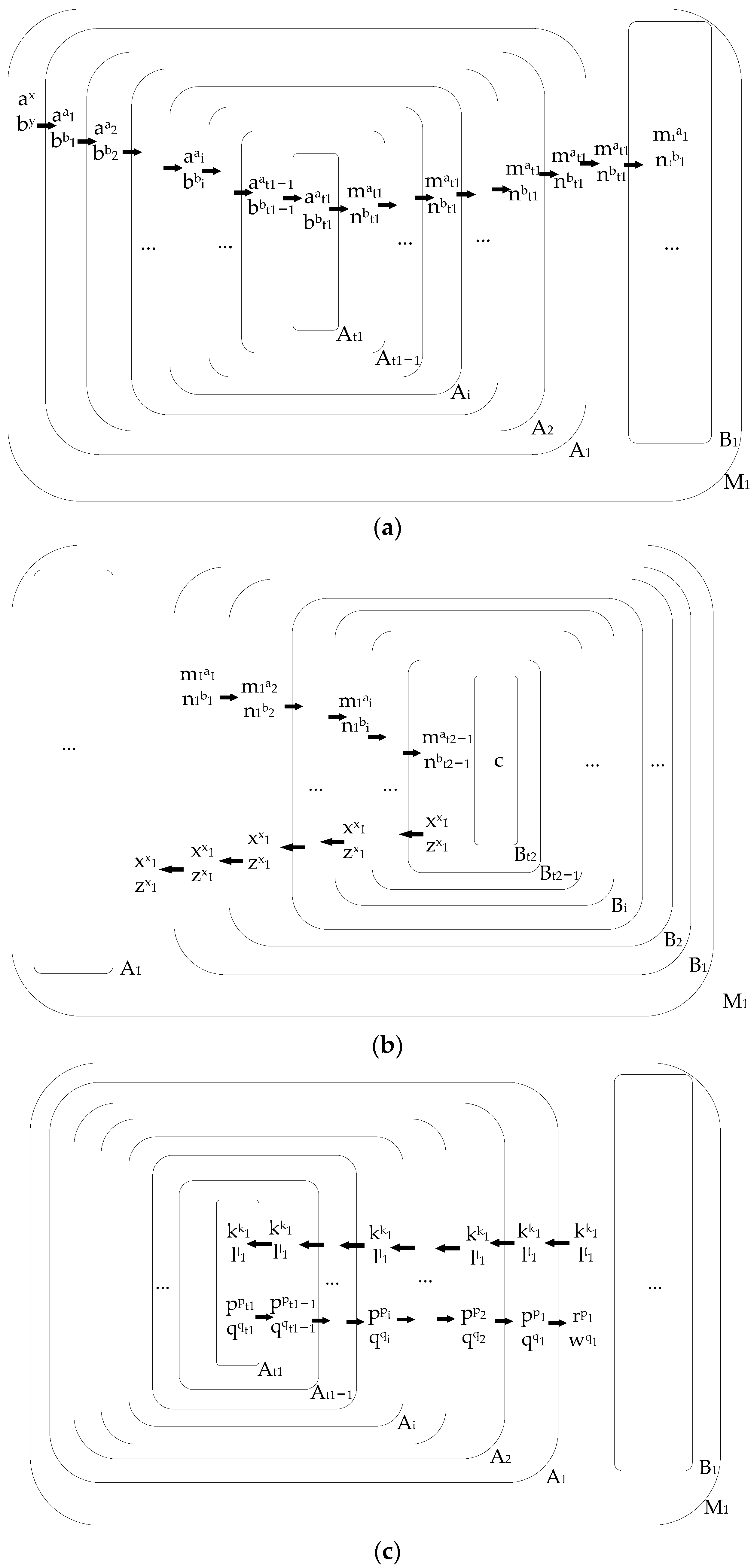
4.3. P System Combined with the More Phase Derogation and the Tossing and Division Algorithm
4.3.1. Definition of a Combinatorial P System
4.3.2. Membrane Rules for Combining the Two Methods
- (i)
- When x > y, the rules within membrane are executed in the following order: .
- (a)
- Execution rule : objects a and b are consumed and the multiset generates the multiset , with remaining. This means that the numerator and denominator values are consumed in y copies at the same time, leaving of object a;
- (b)
- Execute the rule under the condition that object g exists, passing the remaining a to object a in the submembrane;
- (c)
- Execute to convert the multiset into and transfer it to the submembrane; at this time, the number of a in membrane and membrane is equivalent to performing a cumulative addition to obtain The multiplicity set, is used to generate membranes dynamically.
- (ii)
- When x < y, the rules in membrane are executed in the following order: .
- (a)
- Execution rule : objects a and b are consumed and the multiset generates the multiset , with remaining. This means that x copies of the numerator and denominator are consumed simultaneously, leaving y − x objects, b;
- (b)
- Execute rule in the presence of object g, passing the remaining b to object a in the submembrane;
- (c)
- Execute to convert the multiset into and transfer it to the submembrane; at this time, the object a in membrane and membrane is also equivalent to performing an addition, obtaining . The multiset is used for the dynamic generation of membranes.
- (i)
- In class A membranes, the rules are executed in the following order: . Taking membrane as an example, the multiset generated by executing rule is the value used for temporary storage and is used to compare with ξ to determine whether to convert; converts c to ; execute to converts the remaining multiset to multiset for backup (): passes the difference to the submembrane and does not participate in other comparisons; object u compares size with y and decides how to pass and to submembrane ; and object i compares value with d and determines whether to end the more phase derogation algorithm and start using the division algorithm. Execute to compare the difference between and ξ, at this point.
- (ii)
- When (i.e., after executing object i remains in the membrane after the execution), the execution of converts i and g to f and continues using the more phase derogation algorithm, the order of execution in this case is or .
- (a)
- Execute rule to convert y and u into object to determine the magnitude of the subtraction and the difference. When there is in the membrane, is executed to dynamically generate Class A submembrane. After the object is consumed, it indicates that the submembrane has been generated. At the same time, is executed to transfer c into the submembrane .
- (b)
- As the in the membrane is consumed, is then executed to pass the remaining multiset,, into the submembrane for continued dynamic generation of the membrane. At this point, if the object y is still remaining within the membrane, it indicates that the minus number is larger and that both the larger and smaller values in the submembrane need to be replaced. Execution of generates within this membrane and also generates z to be passed into the inner membrane , i.e., .
- (c)
- Object serves as the condition for executing , passing the subtractive backup of the membrane to the subtractive of the submembrane, and executing to transfer the differential backup to the subtractive b of the submembrane, i.e., = , = .
- (d)
- If there is still object u in membrane , it proves that only the subtracted number a needs to be replaced in the submembrane , and the value of b is equal to that of the membrane , i.e., = . = , and (indicating that there is no object z inside the submembrane and no need to execute the rule); at this time, there are still objects u and e in the membrane, and executing generates h; under the catalysis of h, execute to transfer the backup subtracted number to the subtracted number in the submembrane, and execute to transfer the difference backup to the subtracted number in the submembrane.
- (iii)
- When , the order of rule execution in this membrane, at this time, is . When the object g exists and both i and f do not exist, execute to convert g to o. The presence of o indicates that the P System is to be converted from the more phase derogation algorithm to the division algorithm; stop generating new membranes and execute and transfer the backup subtractor to m and n in the parent membrane; execute and transfer the backup difference to m in the parent membrane. Execute , pass the backup difference to m in the parent membrane; at which point, the number of m in the parent membrane is .
- (i)
- Firstly, the membrane sends , to membrane and then executes to gradually transfer and to the submembrane into the submembrane. When and reaches the innermost membrane, the order of execution in the innermost class A substratum is .
- (a)
- Simultaneous execution of rules and translate the quantities of into the initial values and ;
- (b)
- Under the condition that multiset of exists within membrane , simultaneous execution of conversion to is passed directly to the parent membrane , with deciding whether or not to execute based on the presence or absence of z in each layer, and. if the layer has z, convert z into and output it to the parent membrane.
- (ii)
- The order of execution of all class A membranes is or .
- (a)
- If is received from the parent membrane, it means that the minus number changes to the minus number during the more phase derogation algorithm, so it is necessary to convert the multiset to and then the multiset to the object . At this point, , i.e., the execution . If no is passed in the substratum, it means that the decrement is not replaced. Execute directly, converting objects w to p,q and r to p, i.e., .
- (b)
- Any class A membrane with performs the same process as the innermost submembrane, and finally membrane performs to output the multiset to in the membrane, which is then assigned according to the size of the numerator and denominator: when the numerator > denominator, the fraction simplification result is ; when the numerator < denominator, the objects r and w are swapped in membrane and transformed into and , respectively, and the final fraction simplification result is .
4.3.3. Examples of Fractional Simplification by Combining the Two Methods
- (i)
- At this time, the multiset in membrane is and the order of execution of the rules is: .
- (a)
- Execute the rule and the multiset evolves to ; while executing the rule , the object evolves to ;
- (b)
- Execute the rule and the multiset evolved to ; then the rule is executed to compare the numerical values of 25 and ξ, and generate the multiset ;
- (c)
- Because of the remaining , the conditional execution rule is met and the multiset evolves to ;
- (d)
- Under the condition that the object f exists, execute the rule , and the multiset evolves to generate the multiset ;
- (e)
- If object exists, the System executes the rule to generates a new submembrane , while executing to evolve u and e into object h;
- (f)
- Simultaneous execution of rules and is passed directly into the newly created submembrane . At which point, the remaining multiset in membrane is
- (ii)
- At this point, the initialized multiset in membrane is , and the rules are executed in the order .
- (a)
- Execute the rule and the multiset evolves to multiset ; while executing the rule c evolves to multiset k,e,j;
- (b)
- Execute the rule , and the multiset is evolved to ; then execute the rule to evolve to multiset ; then the multiset in the membrane is .
- (c)
- Execute the rule in the absence of object i and object f. The multiset evolves into ; by executing rule in the presence of o, the multisets and evolve into the multisets and , respectively, which are transferred to the parent membrane .
- (i)
- The multiset in membrane is , and the rules are executed in the order .
- (a)
- Implementation rules , multiset evolves to ; then execute the rule that evolves object c to y, subject to the existence of object x;
- (b)
- Implementation rules , multiset evolves to ; execute the rule under the condition that object d exists, so that evolves into the multiplicity set ; then execute the rule , and the object d evolves to object c;
- (ii)
- At this time, the multiset in membrane is , and the rules are executed in the order .
- (a)
- Execute the rule that evolves to the multiset ; execute the rule to evolve c into y under the condition that object x exists; then execute the rule r6 to evolve the multiset to ;
- (b)
- Execution rules , consume CreateSubMem and a Num to generate a submembrane , while the object g evolves to h;
- (c)
- Under the condition that the multiset h exists, execute the rule that transfers the multiset to the membrane ;
- (d)
- Execute the rule , and the multiset h evolves into c and feeds into the membrane .
- (iii)
- The initialized multiset in membrane B2 is . The rules are executed in the same order . After the rules are executed, the remaining multiset in membrane is e. The multiset obtained from membrane is .
- (iv)
- At this point, the initialized multiset in is . And the rules are executed in the order .
- (a)
- Implementation rules , multiset evolves to , and then, execute the rule to evolve c into the object y;
- (b)
- Under the condition that there are no objects and , execute rules that evolves y to z; at this point, the condition is met to execute , the multiset evolves to and is fed into membrane ;
- (i)
- At this point, the multiset in membrane is and the condition is met to execute rule to pass the multiset into membrane , respectively.
- (ii)
- When membrane receives a multiset from the parent membrane, the rules are executed in the order .
- (a)
- First executing rule in membrane converts the multisets and into and , respectively;
- (b)
- Then executing rule converts the multiset into a multiset to pass directly into membrane ;
- (iii)
- The order of execution in membrane at this time, is: .
- (a)
- In the absence of object , rule is executed at the same time, and the multiset evolves into ;
- (b)
- Execute rule to convert the multiset to and feed it into the membrane .
- (iv)
- When the membrane receives the multiset , the object is generated when the rule is executed because the numerator is smaller than the denominator, so the corresponding rule is executed to evolve the multiset into the multiset . At this time, the multiset in the membrane is , and there is no rule to execute in the System, so the whole System is stopped. The number of objects and represent the values of denominator and numerator, respectively, so the final reduction result of 15/40 is 3/8.
5. Experimental Results
5.1. Simulation Experiments
5.1.1. UPSimulator: P System Simulation Software
5.1.2. Simulation Examples
5.2. Parameter Experiments
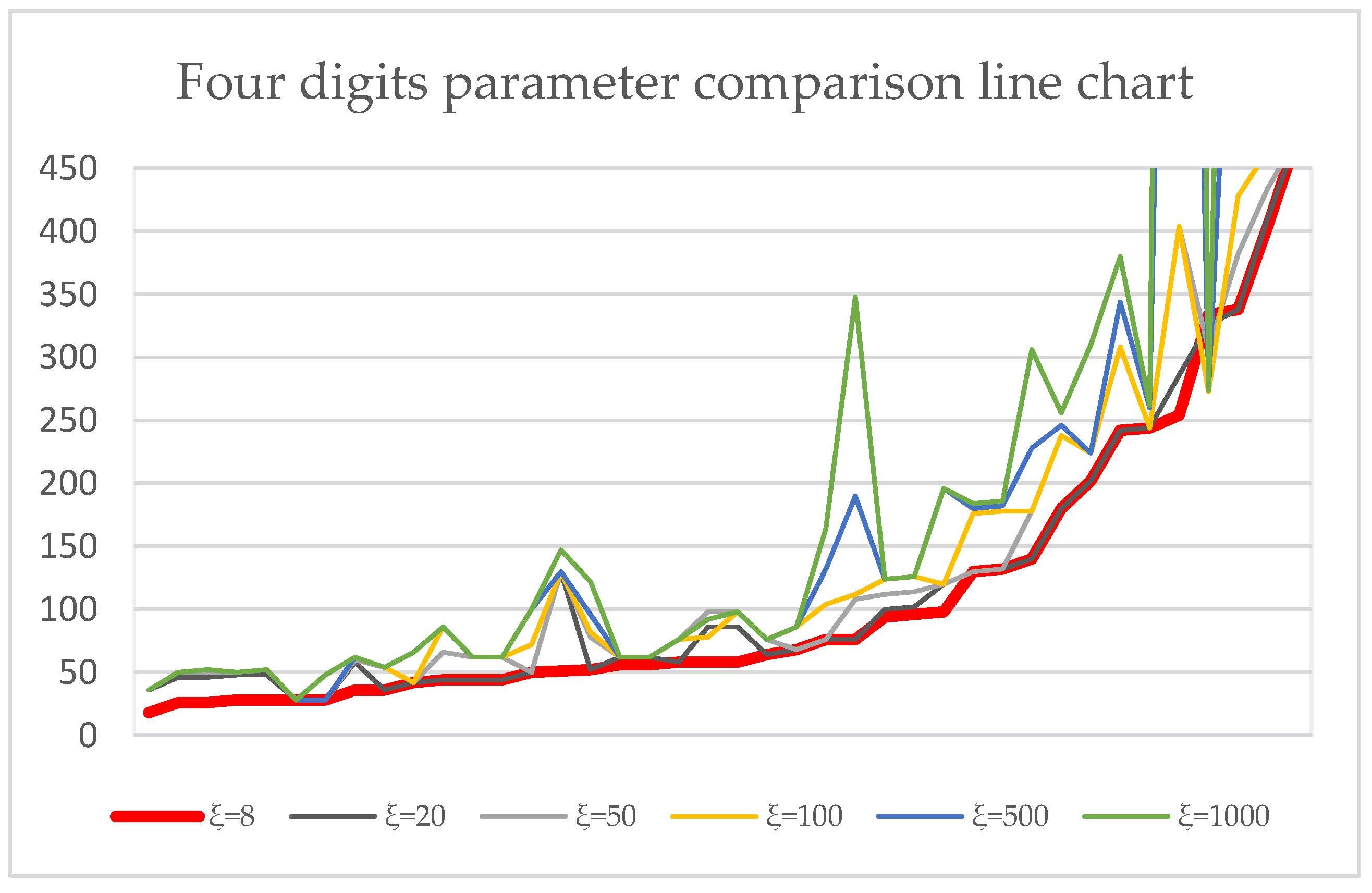
5.2.1. Four-Digit Experimental Result
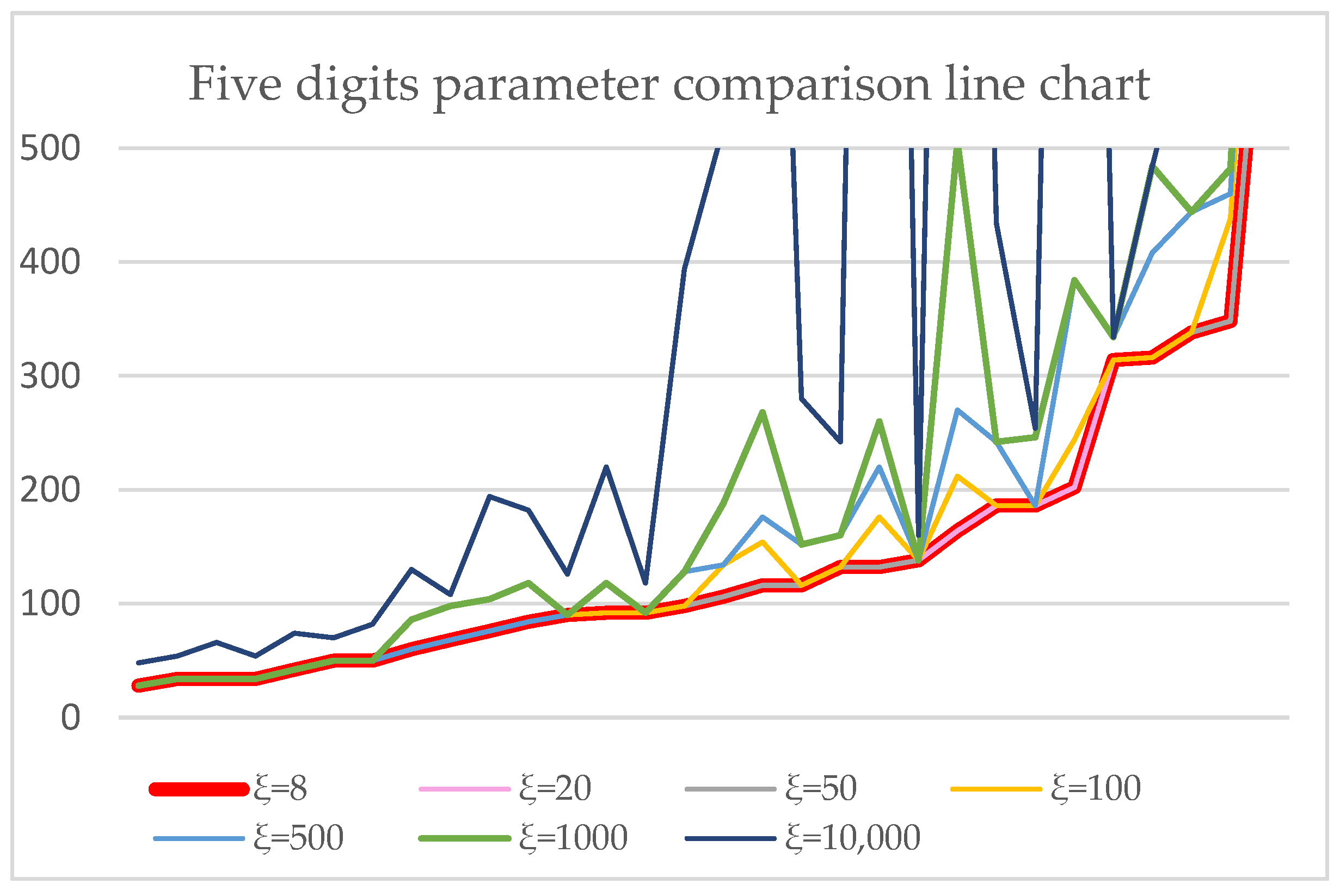
5.2.2. Five-Digit Experimental Result
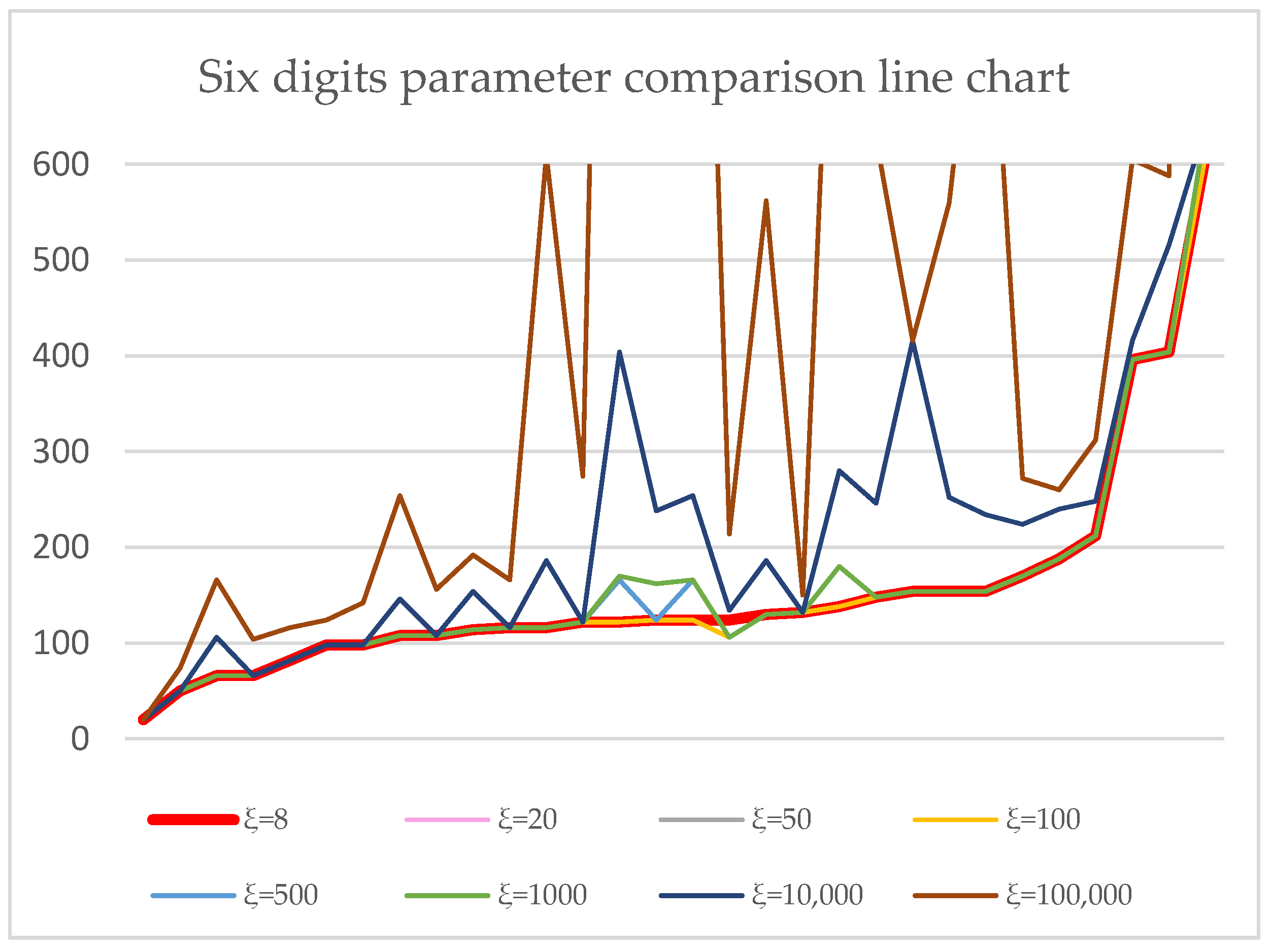
5.2.3. Six-Digit Experimental Result
5.2.4. Summary of Parameter Experiments
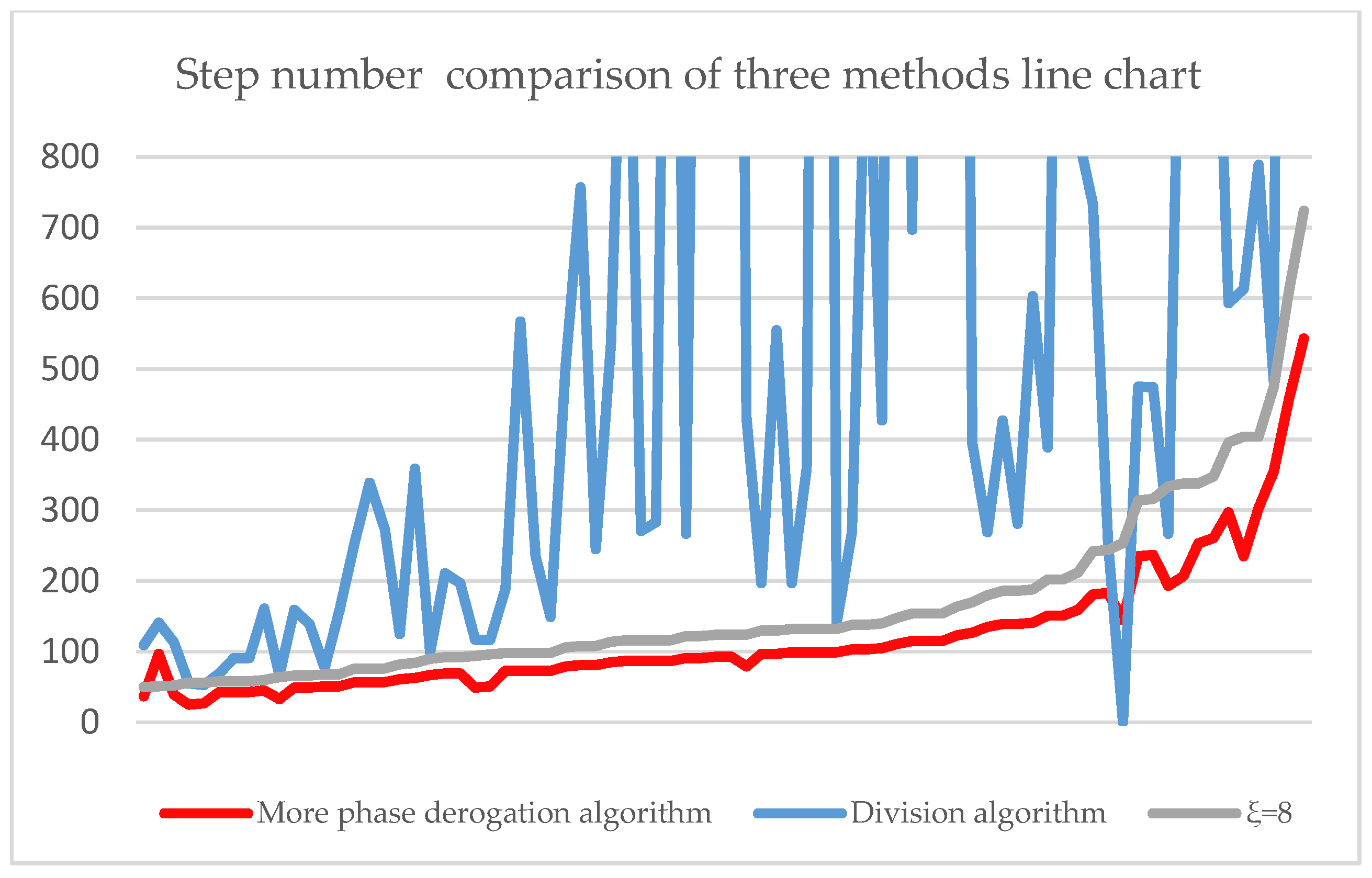
5.3. Comparative Experiment of Three Methods
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Time Slice | Rules for Implementation | Results of the Implementation |
|---|---|---|
| Initial Status | None | M1: a15, b40, c, t; A1: c, d20, CreateSubMem; B1: c, CreateSubMem; |
| 1 | M1: r1; A1: r2 | M1: b25, c, t, g15; A1: d20, e, j, k, CreateSubMem; B1: c, CreateSubMem; |
| 2 | M1: r3, r4; | M1: c, t, b225; A1: a40, b15, d20, e, Num40, j, k, CreateSubMem; B1: a40, b15, c, Num40, CreateSubMem; |
| 3 | A1: r3, r1; B1: r14, r13; | M1: c, t, b225; A1: d20, e, Num40, u25, x15, y15, i25, j, k, CreateSubMem, a125; B1: c, Num40, λ55, CreateSubMem; |
| 4 | A1: r4; | M1: c, t, b225; A1: e, Num40, u25, g20, x15, y15, i5, j, k, CreateSubMem, a125; B1: c, Num40, λ55, CreateSubMem; |
| 5 | A1: r5; | M1: c, t, b225; A1: e, Num40, u25, f5, g15, x15, y15, j, k, CreateSubMem, a125; B1: c, Num40, λ55, CreateSubMem; |
| 6 | A1: r6; | M1: c, t, b225; A1: e, Num40, u10, f5, g15, x15, j, lan15, k, CreateSubMem, a125; B1: c, Num40, λ55, CreateSubMem; |
| 7 | A1: r13, r7, r10; | M1: c, t, b225; A1: Num39, u9, f5, g15, x15, h, j, λ15, a125; A2: c, d20, CreateSubMem; B1: c, Num40, λ55, CreateSubMem; |
| 8 | A1: r8, r14, r15; A2: r2; | M1: c, t, b225; A1: u9, f5, g15, h, j, λ15; A2: a25, b15, d20, Num39, e, j, k, CreateSubMem; B1: c, Num40, λ55, CreateSubMem; |
| 9 | A2: r1, r3; | M1: c, t, b225; A1: u9, f5, g15, h, j, λ15; A2: d20, Num39, e, u10, x15, y15, i10, j, k, CreateSubMem, a110; B1: c, Num40, λ55, CreateSubMem; |
| 10 | A2: r4; | M1: c, t, b225; A1: u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, g10, x15, y15, j, k, CreateSubMem, a110; B1: c, Num40, λ55, CreateSubMem; |
| 11 | A2: r16; | M1: c, t, b225; A1: u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, x15, y15, j, k, CreateSubMem, o10, a110; B1: c, Num40, λ55, CreateSubMem; |
| 12 | A2: r17, r18; | M1: c, t, b225; A1: u9, f5, g15, h, j, λ15, m25, n15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: c, Num40, λ55, CreateSubMem; |
| 13 | A1: r30, r31; | M1: c, t, m25, n15, b225; A1: u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: c, Num40, λ55, CreateSubMem; |
| 14 | M1: r6, r5; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: c, n115, m125, λ55, Num40, CreateSubMem; |
| 15 | B1: r1, r15; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: c, m110, x15, Num39, λ55; B2: CreateSubMem; |
| 16 | B1: r16, r2; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1:m110, x15, λ55, y; B2: Num39, CreateSubMem; |
| 17 | B1: r3; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: d, m110, x15, λ55; B2: Num39, CreateSubMem; |
| 18 | B1: r5, r4; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: n115, c, m110, λ55; B2: Num39, CreateSubMem; |
| 19 | B1: r1; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: n15, c, x10, λ55; B2: Num39, CreateSubMem; |
| 20 | B1: r2; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: n15, x10, y, λ55; B2: Num39, CreateSubMem; |
| 21 | B1: r6; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: n15, x10, e, g, λ55; B2: Num39, CreateSubMem; |
| 22 | B1: r7; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: n15, x10, e, h, λ55; B2: Num39, CreateSubMem; |
| 23 | B1: r8, r9, r10; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: n110, c, m15, Num39, CreateSubMem; |
| 24 | B2: r1, r15; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: n15, c, x5, Num38; B3: CreateSubMem; |
| 25 | B2: r2, r16; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: n15, x5, y; B3: Num38, CreateSubMem; |
| 26 | B2: r6; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: n15, x5, e, g; B3: Num38, CreateSubMem; |
| 27 | B2: r7; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: n15, x5, e, h; B3: Num38, CreateSubMem; |
| 28 | B2: r8, r9, r10; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: e; B3: n15, m15, c, Num38, CreateSubMem; |
| 29 | B3: r1, r15; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: e; B3: c, Num37, x5; B4: CreateSubMem; |
| 30 | B3: r16, r2; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: e; B3: y, x5; B4: Num37, CreateSubMem; |
| 31 | B3: r11; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: e; B3: z, x5; B4: Num37, CreateSubMem; |
| 32 | B3: r12; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55; B2: e, x5, z5; B3: z; B4: Num37, CreateSubMem; |
| 33 | B2: r12; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55, x5, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 34 | B1: r12; | M1: c, t, b115, b225, a125, x5, z5; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 35 | M1: r7, r13; | M1: c, t, f5, i5, b110, b225, a120; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 36 | M1: r8, r14; | M1: e, f5, i5, b110, b225, a120; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 37 | M1: r9, r15; | M1: d, f5, h, i5, b110, b225, a120; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 38 | M1: r10, r11, r16, r17; | M1: c, t, z5, x5, k, l, b110, b225, a120; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 39 | M1: r7, r13, r20, r21 | M1: c, t, f5, i5, k1, l1, b15, b225, a115; A1: n115, m125, u9, f5, g15, k1, l1, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: k1, l1, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 40 | A1: r19, r20; M1: r8, r14; | M1: e, f5, i5, k1, l1, b15, b225, a115; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: d10, Num39, e, u10, k1, l1, y15, j, k, CreateSubMem, o10; B1: k1, l1, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 41 | A2: r21, r22; M1: r9, r15; | M1: d, f5, i5, k1, l1, b15, b225, a115; A1: n115, m125, u9, f5, g15, h, j, λ15; A2: q, p, d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: k1, l1, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 42 | A2: r24, r23; M1: r10, r11, r16, r17; | M1: c, t, k1, l1, x5, z5, k, l, b15, b225, a115; A1: r, n115, m125, u9, f5, g15, w, h, j, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: k1, l1, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 43 | M1: r7, r13, r20, r21; A1: r28, r29; | M1: c, t, k12, l12, f5, i5, b225, a110; A1: n115, m125, l1, k1, f5, g15, h, j, p2, q, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: k12, l12, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 44 | M1: r8, r14; A1: r19, r20, r23, r24; | M1: r2, k12, e, l12, f5, w, i5, y, b225, a110; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, l1, k1, y15, j, k, CreateSubMem, o10; B1: k12, l12, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 45 | M1: r9, r18, r22 r23; A2: r21, r22; | M1: d, l12, k12, f5, i5, λ2, l, r1, b225, a110; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: q, d10, Num39, e, u10, y15, j, k, CreateSubMem, o10, p; B1: k12, l12, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 46 | M1: r10, r11, r21 r24; A2: r23, r24; | M1: c, l13, k12, f5, x5, w12, k, r1, b225, a110; A1: n115, m125, l1, f5, g15, h, j, r, u9, w, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: k12, l13, e, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 47 | M1: r7, r20; A1: r20, r28, r29; | M1: c, l13, k13, f5, i5, w12, r1, b225, a15; A1: n115, m125, f5, k1, g15, h, j, p2, q, u9, λ15; A2: d10, Num39, e, u10, l1, y15, j, k, CreateSubMem, o10; B1: e, l13, k13, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 48 | M1: r8; A1: r19, r23, r24; A2: r22; | M1: r2, l13, k13, f5, w, i5, y, w12, r1, b225, a15; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: q, k1, d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k13, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 49 | M1: r9, r22, r23; A2: r21, r24; | M1: d, l13, k13, f5, i5, w12, lan2, r12, b225, a15; A1: n115, m125, f5, g15, h, j, u9, w, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k13, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 50 | M1: r10, r11, r24; A1: r28; A2: r23; | M1: c, l13, k13, f5, x5, w14, k, r12, b225, a15; A1: n115, m125, f5, g15, h, j, p, q, r, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10, p; B1: e, l13, k13, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 51 | M1: r7, r20; A1: r23, r24, r29; | M1: r, c, l13, k14, f5, w, i5, w14, r12, b225; A1: n115, m125, f5, g15, k1, h, j, p, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10, p; B1: e, l13, k14, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 52 | M1: r8, r22, r23; A1: r19, r23; | M1: r, l13, k14, f5, i5, y, w14, λ, r13, b225; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, y15, k1, j, k, CreateSubMem, o10; B1: e, l13, k14, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 53 | M1: r12, r22, r24; A2: r21; | M1: l13, k14, f5, i5, w15, λ, k, r13, b225; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10, p; B1: e, l13, k14, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 54 | M1: r20, r24; A2: r23; | M1: l13, k15, f5, i5, w16, r13, b225; A1: n115, m125, f5, k1, g15, h, j, r, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 55 | A1: r19, r29; | M1: l13, k15, f5, i5, w16, r13, b225; A1: n115, m125, f5, g15, h, j, p, u9, λ15; A2: d10, Num39, e, u10, k1, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 56 | A1: r23; A2: r21; | M1: r, l13, k15, f5, i5, w16, r13, b225; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10, p; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 57 | M1: r22; A2: r23; | M1: l13, k15, f5, i5, w16, r13, b225, λ; A1: n115, m125, f5, g15, h, j, r, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 58 | M1: r24; A1: r29; | M1: l13, k15, f5, i5, w17, r13, b225; A1: n115, m125, f5, g15, h, j, p, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 59 | A1: r23; | M1: r, l13, k15, f5, i5, w17, r13, b225; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 60 | M1: r22 | M1: l13, k15, f5, i5, w17, r13, b225, λ; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 61 | M1: r24 | M1: l13, k15, f5, i5, w18, r13, b225; A1: n115, m125, f5, g15, h, j, u9, λ15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, λ55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 62 | No enforceable rules |
References
- de Castro, N.L. Fundamentals of natural computing: An overview. Phys. Life Rev. 2007, 4, 1–36. [Google Scholar] [CrossRef]
- Păun, G.; Mario, J.P.-J. Membrane computing: Brief introduction, recent results and applications. Biosystems 2006, 85, 11–22. [Google Scholar] [CrossRef] [PubMed]
- Păun, G. A quick introduction to membrane computing. J. Log. Algebraic Program. 2010, 79, 291–294. [Google Scholar] [CrossRef]
- Pérez Hurtado de Mendoza, I.; Orellana Martín, D.; Martínez del Amor, M.Á.; Valencia Cabrera, L. A Membrane Computing Framework for Social Navigation in Robotics. Comput. Electr. Eng. 2021, 95, 107408. [Google Scholar] [CrossRef]
- Mohan, B.S.; Mahmood, A.A.; Mohammed, M.Q.; Zaki, N.D. Replicating the MAP Kinase Cascade in Membrane Computing; IOP Publishing: Bristol, UK, 2021; Volume 1963, p. 012156. [Google Scholar]
- Frisco, P.; Gheorghe, M.; Pérez-Jiménez, M.J. Applications of Membrane Computing in Systems and Synthetic Biology; Springer: Berlin/Heidelberg, Germany, 2014; ISBN 3-319-03191-0. [Google Scholar]
- Ciobanu, G.; Păun, G.; Pérez-Jiménez, M.J. Applications of Membrane Computing; Springer: Berlin/Heidelberg, Germany, 2006; Volume 17. [Google Scholar]
- Atanasiu, A.; Carlos, M. Arithmetic with membranes. In Proceedings of the Workshop on Mutiset Processing, Dubrovnik, Croatia, 29 June 2000; pp. 1–17. [Google Scholar]
- Ciobanu, G. A Programming perspective of the membrane systems. Int. J. Comput. Commun. Control 2006, 1, 13–24. [Google Scholar] [CrossRef]
- Guo, P.; Jing, C. Arithmetic operation in membrane system. In Proceedings of the 2008 International Conference on BioMedical Engineering and Informatics, Sanya, China, 27–30 May 2008; Volume 1, pp. 231–234. [Google Scholar]
- Guo, P.; Zhang, H. Arithmetic operation in single membrane. In Proceedings of the 2008 International Conference on Computer Science and Software Engineering, Wuhan, China, 12–14 December 2008; Volume 3, pp. 532–535. [Google Scholar]
- Guo, P.; Luo, M. Signed numbers arithmetic operation in multi-membrane. In Proceedings of the 2009 First International Conference on Information Science and Engineering, Nanjing, China, 26–28 December 2009; pp. 393–396. [Google Scholar]
- Guo, P.; Liu, S.J. Arithmetic expression evaluation in membrane computing with priority. In Advanced Materials Research; Trans Tech Publications Ltd.: Stafa-Zurich, Switzerland, 2011; Volume 225, pp. 1115–1119. [Google Scholar]
- Guo, P.; Chen, H.; Zheng, H. Arithmetic expression evaluations with membranes. Chin. J. Electron. 2014, 23, 55–60. Available online: https://cje.ejournal.org.cn/article/id/8163 (accessed on 16 July 2022).
- Guo, P.; Chen, H. Arithmetic expression evaluation by P systems. Appl. Math 2013, 7, 549–553. [Google Scholar] [CrossRef]
- Guo, P.; Zheng, H.; Chen, H.; Chen, J. Fraction arithmetic operations performed by P systems. Chin. J. Electron. 2013, 22, 690–694. [Google Scholar]
- Rich, A.D.; Stoutemyer, D.R. Representation. Simplification and Display of Fractional Powers of Rational Numbers in Computer Algebra. arXiv 2013, arXiv:1302.2169. [Google Scholar]
- Guo, P.; Zhang, H.; Chen, H.; Liu, R. Fraction reduction in membrane systems. Sci. World J. 2014, 2014, 858527. [Google Scholar] [CrossRef] [PubMed]
- Păun, G. From cells to computers: Computing with membranes (P systems). Biosystems 2001, 59, 139–158. [Google Scholar] [CrossRef] [PubMed]
- Martın-Vide, C.; Gheorghe, P.; Alfonso, R.-P. On P systems with membrane creation. Comput. Sci. J. Mold. 2001, 9, 26. [Google Scholar]
- Backhouse, R.; Joao, F.F. On Euclid’s algorithm and elementary number theory. Sci. Comput. Program. 2011, 76, 160–180. [Google Scholar] [CrossRef]
- Rogers, H. The Euclidean Algorithm as a Means of Simplifying Fractions. Arith. Teach. 1970, 17, 657–662. [Google Scholar] [CrossRef]
- Guo, P.; Quan, C.; Ye, L. UPSimulator: A general P system simulator. Knowl.-Based Syst. 2019, 170, 20–25. [Google Scholar] [CrossRef]
- Raghavan, S.; Chandrasekaran, K. Tools and simulators for membrane computing—A literature review. In Bio-Inspired Computing—Theories and Applications; Gong, M., Pan, L., Song, T., Zhang, G., Eds.; BIC-TA 2016; Communications in Computer and Information Science; Springer: Singapore, 2016; Volume 681, pp. 249–277. [Google Scholar] [CrossRef]
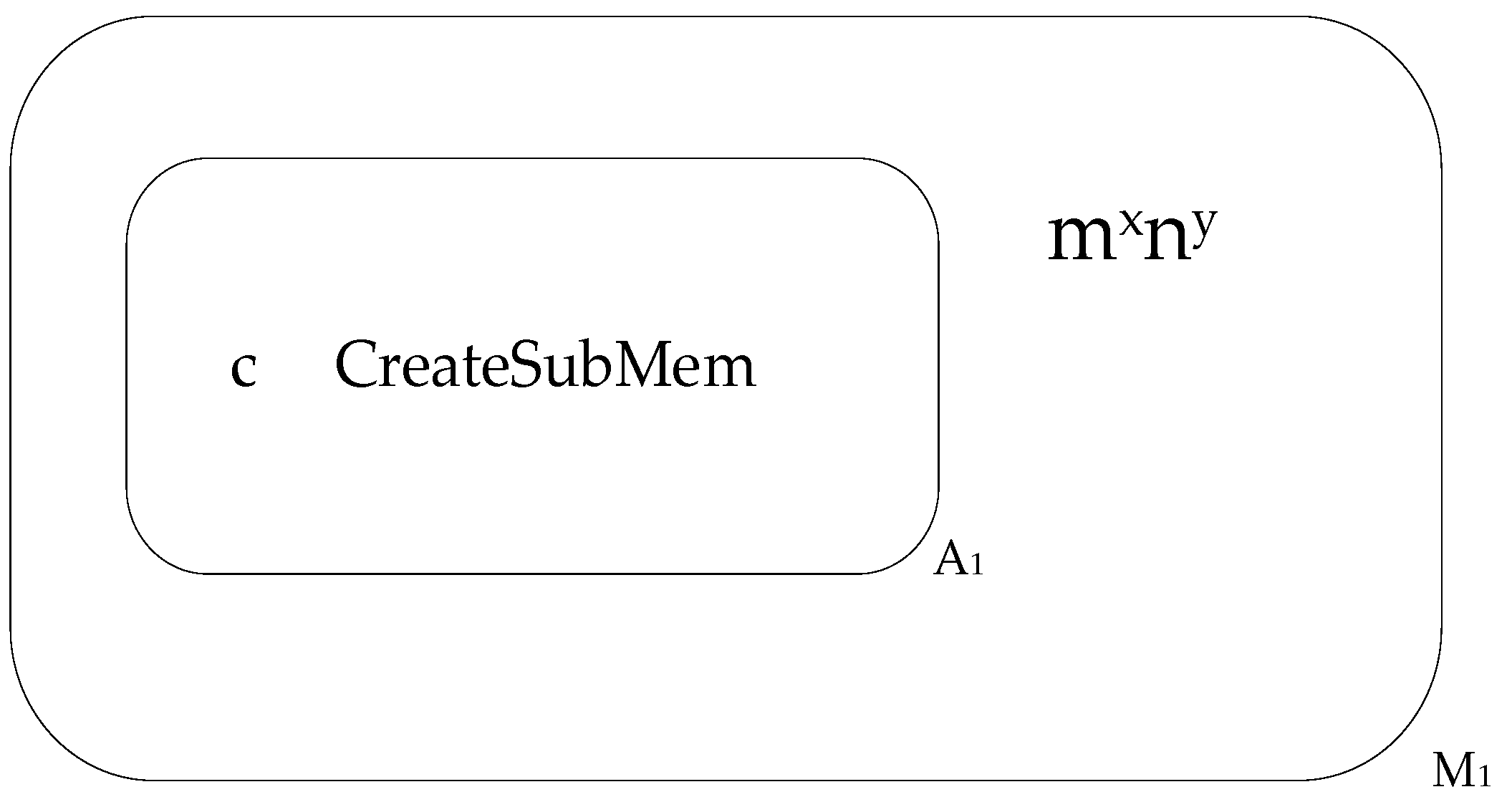

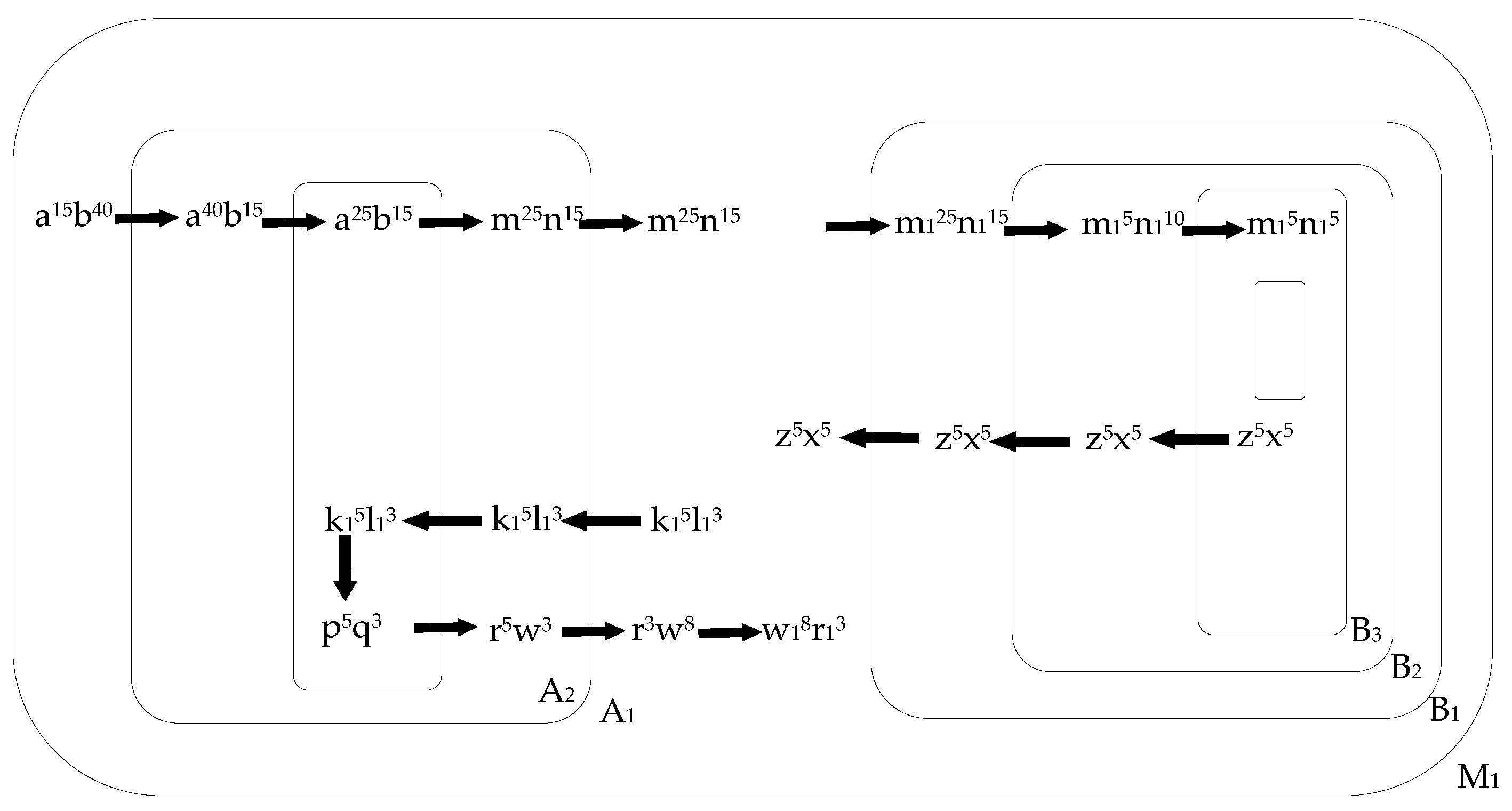



| Time Slice | Rules for Implementation | Results of the Implementation |
|---|---|---|
| Initial Status | None | M1: m6, n15; A1: c, CreateSubMem; |
| 1 | r1 | M1: g6, n9; |
| 2 | r3, r4 | M1: b9; A1: a15, b6, c, Num6, CreateSubMem; |
| 3 | r1, r2 | A1: c, Num6, u9, x6, y6, CreateSubMem, l9; |
| 4 | r3, r4 | A1: Num6, u3, f, v6, x6, k, CreateSubMem, l9; |
| 5 | r5, r7, r12 | A1: Num5, u2, v6, x6, h, l9; A2: c, CreateSubMem; |
| 6 | r8, r10, r13 | A1: u2, v6, h; A2: a9, b6, c, Num5, CreateSubMem; |
| 7 | r1, r2 | A2: c, Num5, u3, x6, y6, CreateSubMem, l3; |
| 8 | r3, r4 | A2: Num5, f, v3, x6, y3, k, CreateSubMem, l3; |
| 9 | r5, r7, r12 | A2: Num4, v3, x6, y2, i, l3; A3: c, z, CreateSubMem; |
| 10 | r8, r10, r13, r17 | A2: v3, y2, i, o; A3: a6, b3, c, Num4, CreateSubMem; |
| 11 | r1, r2 | A3: c, Num4, u3, x3, y3, CreateSubMem, l3; |
| 12 | r3, r4 | A3: Num4, f, v3, x3, k, CreateSubMem, l3; |
| 13 | r5, r12, r14 | A3: q, Num3, v3, x3, l3, p2; A4: c, CreateSubMem; |
| 14 | r13, r15, r16 | A3: p12, v3, x3, l3, q1; A4: c, Num3, CreateSubMem; |
| 15 | r18, r19 | A3: p12, v3, x3, l3, q1; |
| 16 | r15, r16 | A2: p13, v3, y2, i, o, q12; |
| 17 | r20, r21 | A1: q2, u2, v6, h, p5; A2: p13, v3, y2, i, o, q12; |
| 18 | r15, r16 | M1: b9, r5, w2; A1: p15, u2, v6, h, q12; |
| 19 | r20, r21 | M1: b9, w15, r12; |
| 20 | No enforceable rules |
| Time Slice | Rules for Implementation | Results of the Implementation |
|---|---|---|
| Initial Status | None | M1: m6, n15, c, t; A1: c, CreateSubMem; |
| 1 | r1 | M1: g6, n9, c, t; |
| 2 | r3, r4 | M1: a6, b15, c, t; A1: a15, b6, c, Num15, CreateSubMem; |
| 3 | r1, r7 | A1: a9, c, Num14, x6; A2: CreateSubMem; |
| 4 | r2, r14 | A1: a9, x6, y; A2: Num14, CreateSubMem; |
| 5 | r3 | A1: a9, x6, d; |
| 6 | r4, r5 | A1: a9, b6, c; |
| 7 | r1 | A1: a3, x6, c; |
| 8 | r2 | A1: a3, x6, y; |
| 9 | r3 | A1: a3, x6, d; |
| 10 | r4 | A1: a3, b6, c; |
| 11 | r1 | A1: b3, x3, c; |
| 12 | r2 | A1: b3, x3, y; |
| 13 | r6 | A1: b3, x3, e, g; |
| 14 | r8 | A1: b3, x3, e, h; |
| 15 | r9, r10 | A1: e; A2: a3, b3, c, Num14, CreateSubMem; |
| 16 | r1, r7 | A2: c, Num13, x3; A3: CreateSubMem; |
| 17 | r2, r14 | A2: x3, y; A3: Num13, CreateSubMem; |
| 18 | r12 | A2: e, x3, z; |
| 19 | r13 | A1: e, x3, z3; A2: e, z; |
| 20 | r13 | M1: a6, b15, c, t, x3, z3; A1: e, z3; A2: e, z; |
| 21 | r5 | M1: a6, b15, c, t, v3, w3, z3 |
| 22 | r6, r12 | M1: a3, b12, c, t, f3, i3, z3; |
| 23 | r7, r13 | M1: a3, b12, e, f3, i3, y, z3; |
| 24 | r8, r14 | M1: a3, b12, d, f3, h, i3, z3; |
| 25 | r9, r10,r15,r16 | M1: a3, b12, c, t, v3, w3, z3, k, l; |
| 26 | r6, r12 | M1: b9, c, t, f3, i3, z3, k, l; |
| 27 | r7, r13 | M1: b9, e, f3, i3, y, z3, k, l; |
| 28 | r11, r14 | M1: b9, f3, h, i3, z3, k2, l; |
| 29 | r15, r16 | M1: b9, t, v3, i3, z3, k2, l2; |
| 30 | r12 | M1: b6, t, f3, i3, z3, k2, l2; |
| 31 | r13 | M1: b6, e, f3, i3, z3, k2, l2; |
| 32 | r14 | M1: b6, f3, h, i3, z3, k2, l2; |
| 33 | r15, r16 | M1: b6, t, v3, i3, z3, k2, l3; |
| 34 | r12 | M1: b3, t, f3, i3, z3, k2, l3; |
| 35 | r13 | M1: b3, e, f3, i3, z3, k2, l3; |
| 36 | r14 | M1: b3, f3, h, i3, z3, k2, l3; |
| 37 | r15, r16 | M1: b3, t, v3, i3, z3, k2, l4; |
| 38 | r12 | M1:t, f3, i3, z3, k2, l4; |
| 39 | r13 | M1: e, f3, i3, z3, k2, l4; |
| 40 | r17 | M1: f3, i3, z3, k2, l5; |
| 41 | No enforceable rules |
| Time Slice | Rules for Implementation | Results of the Implementation |
|---|---|---|
| Initial Status | None | M1: a15, b40, c, t; A1: c, d20, CreateSubMem; B1: c, CreateSubMem; |
| 2 | M1: r3, r4; | M1: c, t, b225; A1: a40, b15, d20, e, Num40, j, k, CreateSubMem; B1: a40, b15, c, Num40, CreateSubMem; |
| 7 | A1: r13, r7, r10; | M1: c, t, b225; A1: Num39, u9, f5, g15, x15, h, j, 15, a125; A2: c, d20, CreateSubMem; B1: c, Num40, 55, CreateSubMem; |
| 13 | A1: r30, r31; | M1: c, t, m25, n15, b225; A1: u9, f5, g15, h, j, 15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: c, Num40, 55, CreateSubMem; |
| 14 | M1: r6, r5; | M1: c, t, b115, b225, a125; A1: n115, m125, u9, f5, g15, h, j, 15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: c, n115, m125, 55, Num40, CreateSubMem; |
| 34 | B1: r12; | M1: c, t, b115, b225, a125, x5, z5; A1: n115, m125, u9, f5, g15, h, j, 15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, 55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 54 | M1: r20, r24; A2: r23; | M1: l13, k15, f5, i5, w16, r13, b225; A1: n115, m125, f5, k1, g15, h, j, r, u9, 15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, 55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 61 | M1: r24 | M1: l13, k15, f5, i5, w18, r13, b225; A1: n115, m125, f5, g15, h, j, u9, 15; A2: d10, Num39, e, u10, y15, j, k, CreateSubMem, o10; B1: e, l13, k15, 55, z5; B2: e, z5; B3: z; B4: Num37, CreateSubMem; |
| 62 | No enforceable rules |
| Serial Number | Maximum Number of Digits | Value Range | Number of Groups |
|---|---|---|---|
| 1 | 4 | 0–9999 | 40 |
| 2 | 5 | 100–99,999 | 30 |
| 3 | 6 | 1000–999,999 | 30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nan, H.; Kong, Y.; Zhan, J.; Zhou, M.; Bai, L. P System with Fractional Reduction. Appl. Sci. 2023, 13, 8514. https://doi.org/10.3390/app13148514
Nan H, Kong Y, Zhan J, Zhou M, Bai L. P System with Fractional Reduction. Applied Sciences. 2023; 13(14):8514. https://doi.org/10.3390/app13148514
Chicago/Turabian StyleNan, Hai, Yumeng Kong, Jie Zhan, Mingqiang Zhou, and Ling Bai. 2023. "P System with Fractional Reduction" Applied Sciences 13, no. 14: 8514. https://doi.org/10.3390/app13148514
APA StyleNan, H., Kong, Y., Zhan, J., Zhou, M., & Bai, L. (2023). P System with Fractional Reduction. Applied Sciences, 13(14), 8514. https://doi.org/10.3390/app13148514






