Numerical Identification of Material Model Parameters of UHPFRC Slab under Blast Loading
Abstract
1. Introduction and Review
2. Tested Specimens
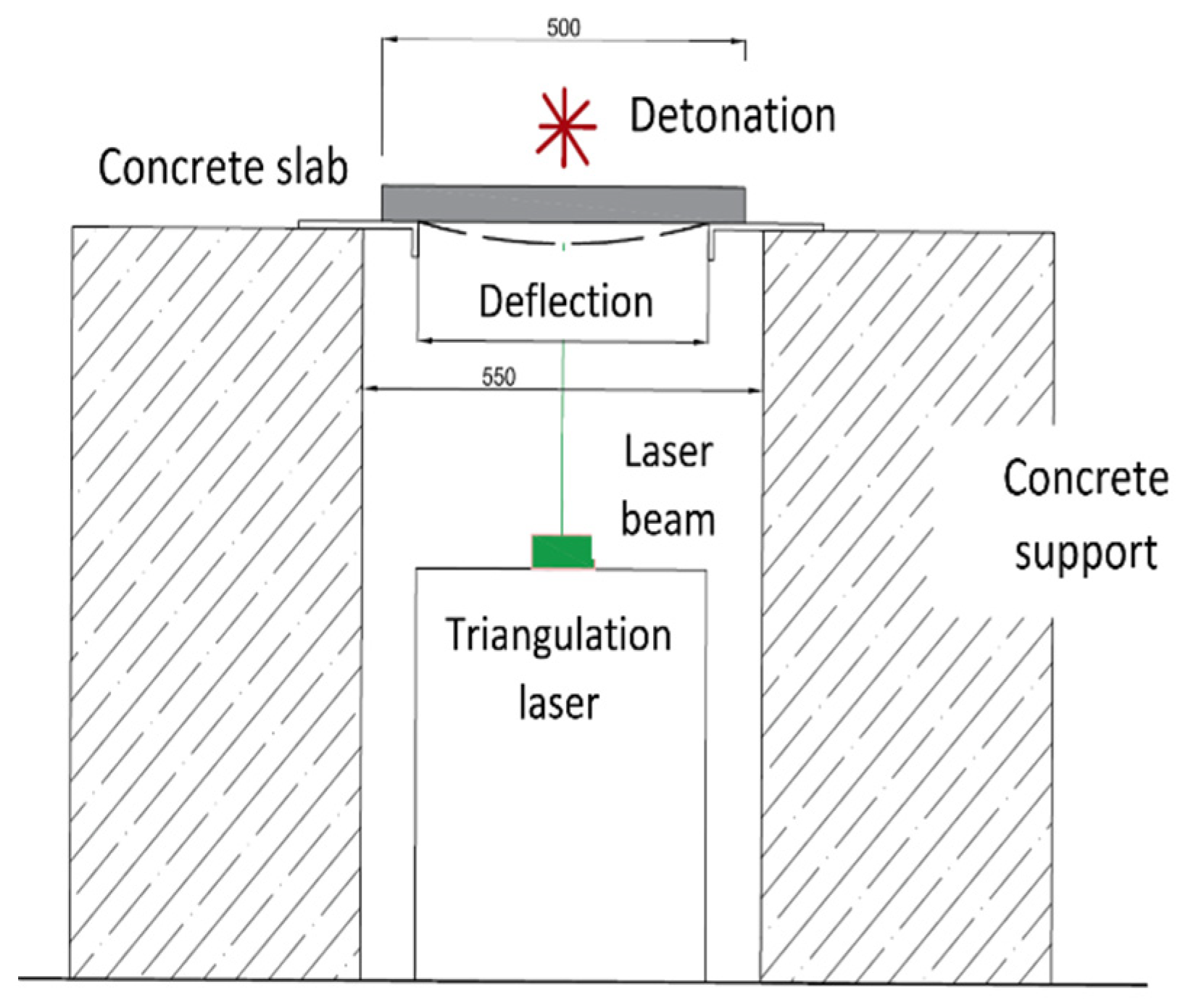
3. Experimental Measurement
4. Material Model of Simulation
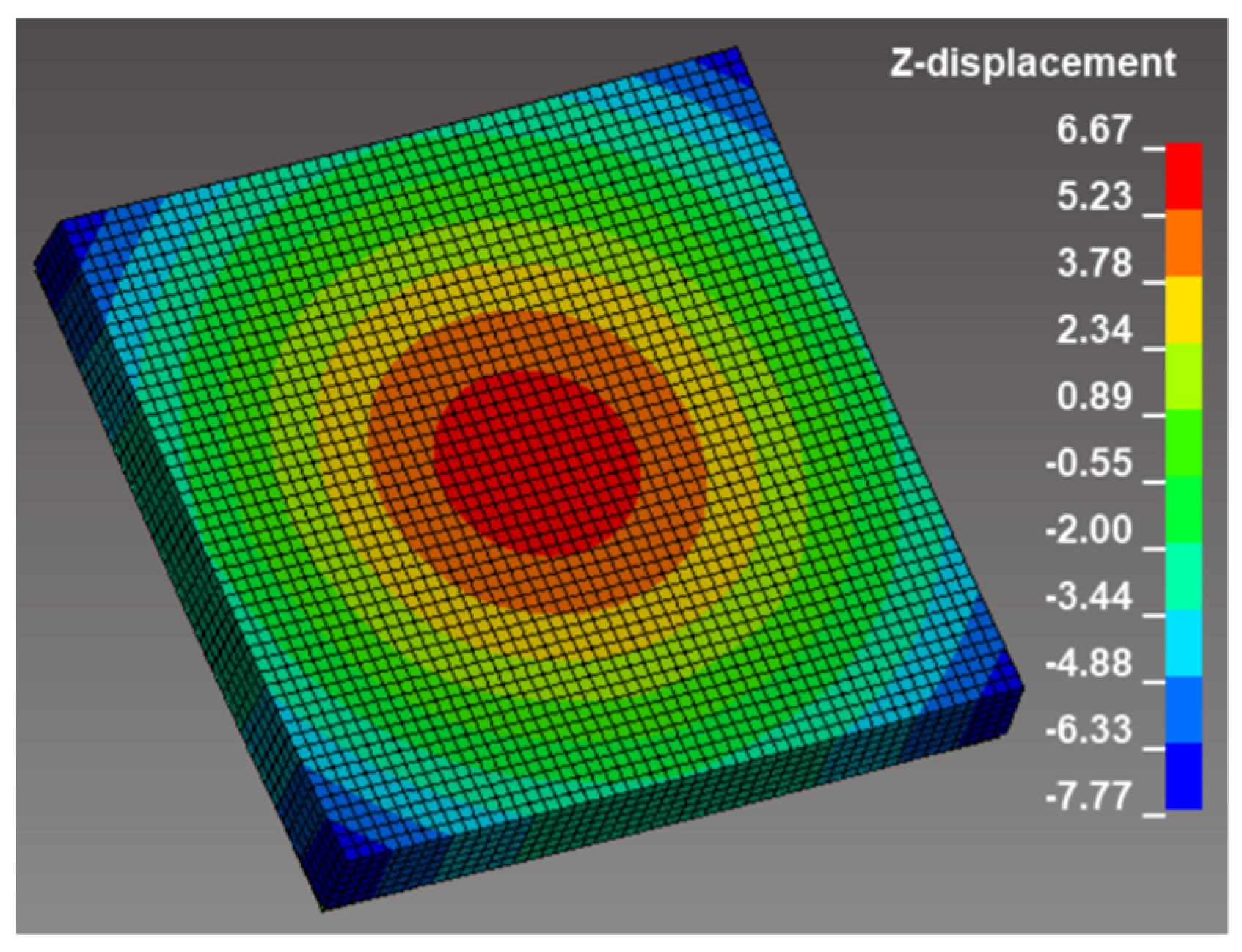
5. Computational Model
6. Inverse Identification
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Coughlin, A.M.; Musselman, E.S.; Schokker, A.J.; Linzell, D.G. Behavior of portable fiber reinforced concrete vehicle barriers subject to blasts from contact charges. Int. J. Impact Eng. 2010, 37, 521–529. [Google Scholar] [CrossRef]
- Drdlová, I.M.; Buchar, J.; Krátký, I.J.; Rídký, I.R. Blast resistance characteristics of concrete with different types of fibre reinforcement. Struct. Concr. 2015, 16, 508–517. [Google Scholar] [CrossRef]
- Ramadoss, P.; Nagaman, K. Impact, characteristics of high-performance steel fiber reinforced concrete under repeated dynamic loading. Int. J. Civ. Eng. 2014, 12, 513–520. [Google Scholar]
- Yazici, H.; Deniz, E.; Baradan, B. The effect of autoclave pressure, temperature and duration time on mechanical properties of reactive powder concrete. Construct. Build. Mater. 2013, 42, 53–63. [Google Scholar] [CrossRef]
- Verma, M.; Prem, P.R.; Rajasankar, J.; Bharatkumar, B.H. On low-energy impact response of ultra-high-performance concrete (UHPC) panels. Mater. Des. 2016, 92, 853–865. [Google Scholar] [CrossRef]
- Wu, Z.; Khayat, K.H.; Shi, C. How do fiber shape and matrix composition affect fiber pullout behavior and flexural properties of UHPC? Cem. Concr. Compos. 2018, 90, 193–201. [Google Scholar] [CrossRef]
- Liu, J.; Wu, C.; Su, Y.; Li, J.; Shao, R.; Chen, G.; Liu, Z. Experimental and numerical studies of ultra-high performance concrete targets against high-velocity projectile impacts. Eng. Struct. 2018, 173, 166–179. [Google Scholar] [CrossRef]
- Yang, S.; Kong, X.; Wu, H.; Fang, Q.; Xiang, H. Constitutive modelling of UHPCC material under impact and blast loadings. Int. J. Impact Eng. 2021, 153, 103860. [Google Scholar] [CrossRef]
- Castedo, R.; Segarra, P.; Alañon, A.; Lopez, L.; Santos, A.; Sanchidrian, J. Air blast resistance of full-scale slabs with different compositions: Numerical modeling and field validation. Int. J. Impact Eng. 2015, 86, 145–156. [Google Scholar] [CrossRef]
- Li, J.; Hao, H. Numerical study of concrete spall damage to blast loads. Int. J. Impact Eng. 2014, 68, 41–55. [Google Scholar] [CrossRef]
- Maazoun, A.; Matthys, S.; Atoui, O.; Belkassem, B.; Lecompte, D. Finite element modelling of RC slabs retrofitted with CFRP strips under blast loading. Eng. Struct. 2021, 252, 113597. [Google Scholar] [CrossRef]
- Morales-Alonso, G.; Cendón, D.A.; Galvez, F.; Erice, B.; Sánchez-Gálvez, V. Blast Response Analysis of Reinforced Concrete Slabs: Experimental Procedure and Numerical Simulation. J. Appl. Mech. 2011, 78, 051010. [Google Scholar] [CrossRef]
- Maazoun, A.; Matthys, S.; Belkassem, B.; Lecompte, D.; Vantomme, J. Blast response of retrofitted reinforced concrete hollow core slabs under a close distance explosion. Eng. Struct. 2019, 191, 447–459. [Google Scholar] [CrossRef]
- Thai, D.-K.; Kim, S.-E. Numerical investigation of the damage of RC members subjected to blast loading. Eng. Fail. Anal. 2018, 92, 350–367. [Google Scholar] [CrossRef]
- Thai, D.-K.; Nguyen, D.-L.; Pham, T.-H.; Doan, Q.H. Prediction of residual strength of FRC columns under blast loading using the FEM method and regression approach. Constr. Build. Mater. 2021, 276, 122253. [Google Scholar] [CrossRef]
- Jayasinghe, L.; Thambiratnam, D.; Perera, N.; Jayasooriya, J. Blast response of reinforced concrete pile using fully coupled computer simulation techniques. Comput. Struct. 2014, 135, 40–49. [Google Scholar] [CrossRef]
- Sadiq, M.; Yun, Z.X.; Rong, P. Simulation analysis of impact tests of steel plate reinforced concrete and reinforced concrete slabs against aircraft impact and its validation with experimental results. Nucl. Eng. Des. 2014, 273, 653–667. [Google Scholar] [CrossRef]
- Thai, D.-K.; Nguyen, D.-L.; Kim, S.-E. Numerical investigation on local damage of proposed RC panels under impact loading. Nucl. Eng. Des. 2019, 341, 377–389. [Google Scholar] [CrossRef]
- Orbovic, N.; Sagals, G.; Blahoianu, A. Influence of transverse reinforcement on perforation resistance of reinforced concrete slabs under hard missile impact. Nucl. Eng. Des. 2015, 295, 716–729. [Google Scholar] [CrossRef]
- Domizio, M.; Ambrosini, D.; Curadelli, O. Nonlinear dynamic numerical analysis of a RC frame subjected to seismic loading. Eng. Struct. 2017, 138, 410–424. [Google Scholar] [CrossRef]
- Jong, K.; Jaehyeon, L.; Jung, J.W.; Dongyeop, H. Testing the anti-explosion protection of HPFRCC for ready-mixed concrete system based on fiber selection and resistance to live explosives. Case Stud. Constr. Mater. 2022, 17. [Google Scholar] [CrossRef]
- Almustafa, M.K.; Nehdi, M.L. Machine learning prediction of structural response of steel fiber-reinforced concrete beams subjected to far-field blast loading. Cem. Concr. Compos. 2022, 126, 104378. [Google Scholar] [CrossRef]
- Zhou, X.; Kuznetsov, V.; Hao, H.; Waschl, J. Numerical prediction of concrete slab response to blast loading. Int. J. Impact Eng. 2008, 35, 1186–1200. [Google Scholar] [CrossRef]
- Mao, L.; Barnett, S.; Tyas, A.; Warren, J.; Schleyer, G.; Zaini, S. Response of small scale ultra high performance fibre reinforced concrete slabs to blast loading. Constr. Build. Mater. 2015, 93, 822–830. [Google Scholar] [CrossRef]
- Mao, L.; Barnett, S.; Begg, D.; Schleyer, G.; Wight, G. Numerical simulation of ultra high performance fibre reinforced concrete panel subjected to blast loading. Int. J. Impact Eng. 2014, 64, 91–100. [Google Scholar] [CrossRef]
- Astarlioglu, S.; Krauthammer, T. Response of normal-strength and ultra-high-performance fiber-reinforced concrete columns to idealized blast loads. Eng. Struct. 2014, 61, 1–12. [Google Scholar] [CrossRef]
- Xu, Z.; Li, J.; Qian, H.; Wu, C. Blast resistance of hybrid steel and polypropylene fibre reinforced ultra-high performance concrete after exposure to elevated temperatures. Compos. Struct. 2022, 294. [Google Scholar] [CrossRef]
- Das, N.; Nanthagopalan, P. State-of-the-art review on ultra high performance concrete—Ballistic and blast perspective. Cem. Concr. Compos. 2022, 127, 104383. [Google Scholar] [CrossRef]
- Su, Q.; Wu, H.; Fang, Q. Calibration of KCC model for UHPC under impact and blast loadings. Cem. Concr. Compos. 2022, 127. [Google Scholar] [CrossRef]
- Luccioni, B.; Isla, F.; Codina, R.; Ambrosini, D.; Zerbino, R.; Giaccio, G.; Torrijos, M. Experimental and numerical analysis of blast response of High Strength Fiber Reinforced Concrete slabs. Eng. Struct. 2018, 175, 113–122. [Google Scholar] [CrossRef]
- Zhang, D.; Yao, S.; Lu, F.; Chen, X.; Lin, G.; Wang, W.; Lin, Y. Experimental study on scaling of RC beams under close-in blast loading. Eng. Fail. Anal. 2013, 33, 497–504. [Google Scholar] [CrossRef]
- Wu, J.; Zhou, Y.; Zhang, R.; Liu, C.; Zhang, Z. Numerical simulation of reinforced concrete slab subjected to blast loading and the structural damage assessment. Eng. Fail. Anal. 2020, 118, 104926. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, D.; Lu, F.; Wang, S.-C.; Tang, F. Experimental study on scaling the explosion resistance of a one-way square reinforced concrete slab under a close-in blast loading. Int. J. Impact Eng. 2012, 49, 158–164. [Google Scholar] [CrossRef]
- Shishegaran, A.; Khalili, M.R.; Karami, B.; Rabczuk, T.; Shishegaran, A. Computational predictions for estimating the maximum deflection of reinforced concrete panels subjected to the blast load. Int. J. Impact Eng. 2020, 139, 103527. [Google Scholar] [CrossRef]
- Tu, H.; Fung, T.C.; Tan, K.H.; Riedel, W. An analytical model to predict spalling and breaching of concrete plates under contact detonation. Int. J. Impact Eng. 2022, 160, 104075. [Google Scholar] [CrossRef]
- Wu, J.; Liu, Z.; Yu, J.; Xu, S. Experimental and numerical investigation of normal reinforced concrete panel strengthened with polyurea under near-field explosion. J. Build. Eng. 2022, 46, 103763. [Google Scholar] [CrossRef]
- Lu, Y.; Xu, K. Modelling of dynamic behaviour of concrete materials under blast loading. Int. J. Solids Struct. 2004, 41, 131–143. [Google Scholar] [CrossRef]
- Johnson, J.; Xu, M.; Jacques, E. Predicting the self-centering behavior of hybrid FRP-steel reinforced concrete beams under blast loading. Eng. Struct. 2021, 247, 113117. [Google Scholar] [CrossRef]
- Grisaro, H.Y. Effect of combined blast and fragmentation load on the dynamic response of reinforced concrete structures. Eng. Struct. 2021, 248, 113244. [Google Scholar] [CrossRef]
- Gomathi, K.A.; Rajagopal, A.; Prakash, S.S. Predicting the failure mechanism of RC slabs under combined blast and impact loading. Theor. Appl. Fract. Mech. 2022, 119. [Google Scholar] [CrossRef]
- Lee, M.; Kwak, H.-G. Numerical simulations of blast responses for SFRC slabs using an orthotropic model. Eng. Struct. 2021, 238, 112150. [Google Scholar] [CrossRef]
- Luccioni, B.M.; Luege, M. Concrete pavement slab under blast loads. Int. J. Impact Eng. 2006, 32, 1248–1266. [Google Scholar] [CrossRef]
- Ning, J.; Yang, S.; Ma, T.; Xu, X. Fragment behavior of concrete slab subjected to blast loading. Eng. Fail. Anal. 2022, 138. [Google Scholar] [CrossRef]
- Anas, S.; Alam, M.; Umair, M. Experimental and numerical investigations on performance of reinforced concrete slabs under explosive-induced air-blast loading: A state-of-the-art review. Structures 2021, 31, 428–461. [Google Scholar] [CrossRef]
- Zhao, C.; Ye, X.; He, K.; Gautam, A. Numerical study and theoretical analysis on blast resistance of fabricated concrete slab. J. Build. Eng. 2020, 32, 101760. [Google Scholar] [CrossRef]
- Kumar, V.; Kartik, K.; Iqbal, M. Experimental and numerical investigation of reinforced concrete slabs under blast loading. Eng. Struct. 2020, 206, 110125. [Google Scholar] [CrossRef]
- European Standard EN 12390-1; Testing Hardened Concrete—Part 1: Shape, Dimensions and Other Requirements for Specimens and Moulds. CEN: Brussels, Belgium, 2021.
- European Standard EN 12390-2; Testing Hardened Concrete—Part 2: Making and Curing Specimens for Strength Tests. CEN: Brussels, Belgium, 2019.
- European Standard EN 12390-3; Testing Hardened Concrete—Part 3: Compressive Strength of Test Specimens. CEN: Brussels, Belgium, 2019.
- Zezulová, E.; Hasilová, K.; Dvořák, P.; Dubec, B.; Komárková, T.; Štoller, J. Experimental Campaign to Verify the Suitability of Ultrasound Testing Method for Steel Fiber Reinforced Concrete Fortification Structures. Appl. Sci. 2021, 11, 8759. [Google Scholar] [CrossRef]
- European Standard EN 12390-5; Testing Hardened Concrete—Part 5: Flexural Strength of Test Specimens. CEN: Brussels, Belgium, 2019.
- Dubec, B.; Manas, P.; Stoller, J.; Stonis, P. Experimental and Numerical Assessment of Fibre Reinforced Concrete Slab under Blast Load. In Proceedings of the 2019 International Conference on Military Technologies (ICMT), Brno, Czech Republic, 30–31 May 2019. [Google Scholar] [CrossRef]
- Dubec, B.; Manas, P.; Stoller, J. Analytical approach in assessment of deflection of thick concrete plates. In Proceedings of the 2021 International Conference on Military Technologies (ICMT), Brno, Czech Republic, 8–11 June 2021; pp. 1–4. [Google Scholar] [CrossRef]
- Livermore Software Technology Corporation. LS-Dyna Keyword User’s Manual, Volume II, Material Models, 10/18/16 (r:7991); Livermore Software Technology Corporation: Livemore, CA, USA, 2015. [Google Scholar]
- Livermore Software Technology Corporation. LS-Dyna, Theory Manual; Livemore Software Technology Corporation: Livemore, CA, USA, 2015. [Google Scholar]
- Livermore Software Technology Corporation. LS-Dyna, Keyword User’s Manual, Volume I, 10/18/16 (r:7991); Livermore Software Technology Corporation: Livemore, CA, USA, 2014. [Google Scholar]
- Schwer, L. Modeling Rebar: The forgotten sister in reinforced concrete modeling. In Proceedings of the 13th-International-LS-Dyna-Conference, Wien, Austria, 8–10 June 2014. [Google Scholar]
- Kral, P.; Husek, M. Concrete in Uniaxial and Triaxial Compression—Experimental and Numerical Analysis in Interaction with Material Parameter Optimization. Conference paper. In Proceedings of the Juniorstav 2017, Brno, Czech Republic, 26 January 2017; pp. 1–6, ISBN 987-80-214-5462-0. [Google Scholar]
- Hokes, F.; Kral, P.; Krnavek, O.; Husek, M. Improved Sensitivity Analysis in the Inverse Identification of the Parameters of a Nonlinear Material Model. Procedia Eng. 2017, 172, 347–354. [Google Scholar] [CrossRef]
- Atoui, O.; Kechagiadakis, G.; Moumen, A.; Maazoun, A.; Belkassem, B.; Pyl, L.; Lecompte, D. An Explosive Driven Shock Tube-Based Laboratory Scale Test for Combined Blast and Fragment Impact Loading. Appl. Sci. 2022, 12, 6854. [Google Scholar] [CrossRef]
- Trevino, T. Applications of Arbitrary Lagrangian Eulerian (ALE) Analysis Approach to Underwater and Air Explosion Problems; Naval Postgraduate School: Monterey, CA, USA, 2000. [Google Scholar]
- Zukas, J.A.; Walter, W. Explosive Effects and Applications (Shock Wave and High Pressure Phenomena); Springer: London, UK, 2002; ISBN 978-0387955582. [Google Scholar]
- Trajkovski, J.; Kunc, R.; Perenda, J.; Prebil, I. Minimum mesh design criteria for blast wave development and structural response—MMALE method. Lat. Am. J. Solids Struct. 2014, 11, 1999–2017. [Google Scholar] [CrossRef]
- Koli, S.; Chellapandi, P.; Rao, L.B.; Sawant, A. Study on JWL equation of state for the numerical simulation of near-field and far-field effects in underwater explosion scenario. Eng. Sci. Technol. Int. J. 2020, 23, 758–768. [Google Scholar] [CrossRef]
- Dubec, B. Concrete Material Model Parameters for Simulation of Transient Dynamics. Dissertation Thesis, University of Defence, Brno, Czech Republic, 2022. [Google Scholar]
- OptiSLang. Methods for Multi-Disciplinary Optimization and Robustness Analysis; Dynardo: Weimar, Germany, 2014. [Google Scholar]
- Most, T.; Will, J. Sensitivity analysis using the Metamodel of Optimal Prognosis. In Proceedings of the Weimar Optimization and Stochastic Days 8.0, Weimar, Germany, 24–25 November 2011. [Google Scholar]
- Evans, J.D. Straightforward Statistics for the Behavioral Sciences; Thomson Brooks/Cole Publishing Co.: Pacific Grove, CA, USA, 1996. [Google Scholar]
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences, 2nd ed.; Lawrence Erlbaum Associates: Hillsdale, NJ, USA, 1988; ISBN 978-0-8058-0283-2. [Google Scholar]
- Kala, F.H.A.J. Selecting the objective function during the inverse identification of the parameters of a material model of concrete. Frat. Ed. Integrita Strutt. 2017, 39, 7–16. [Google Scholar]

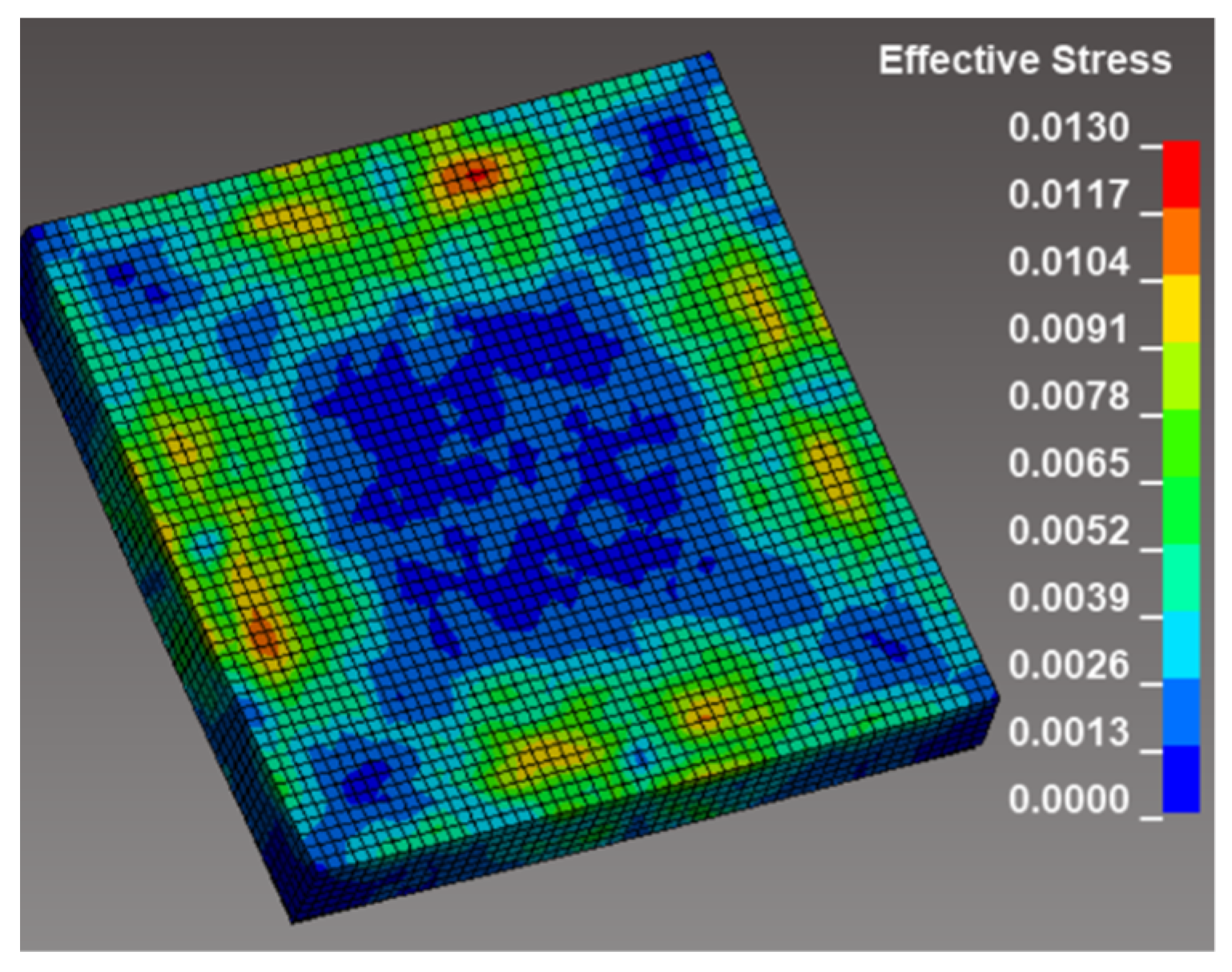
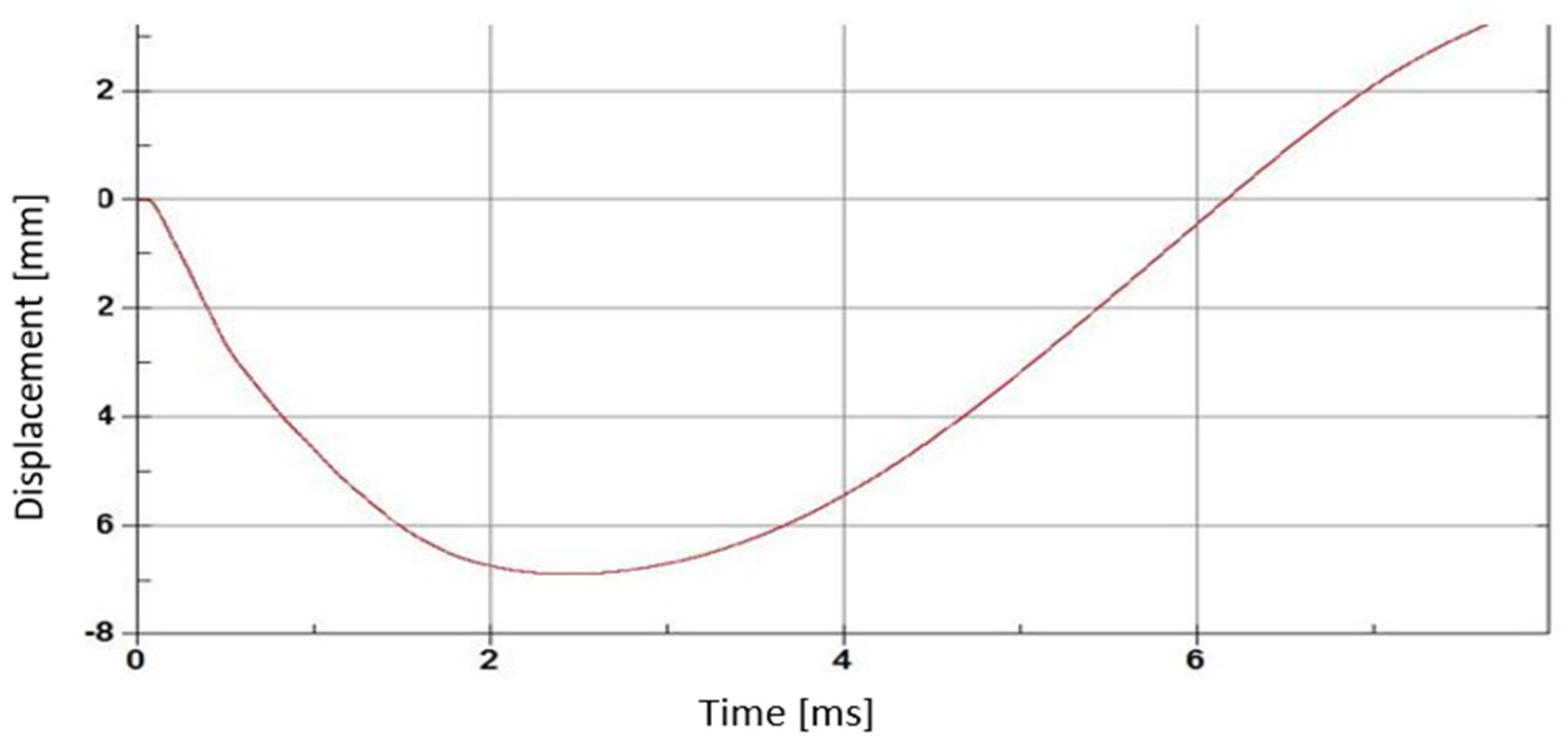

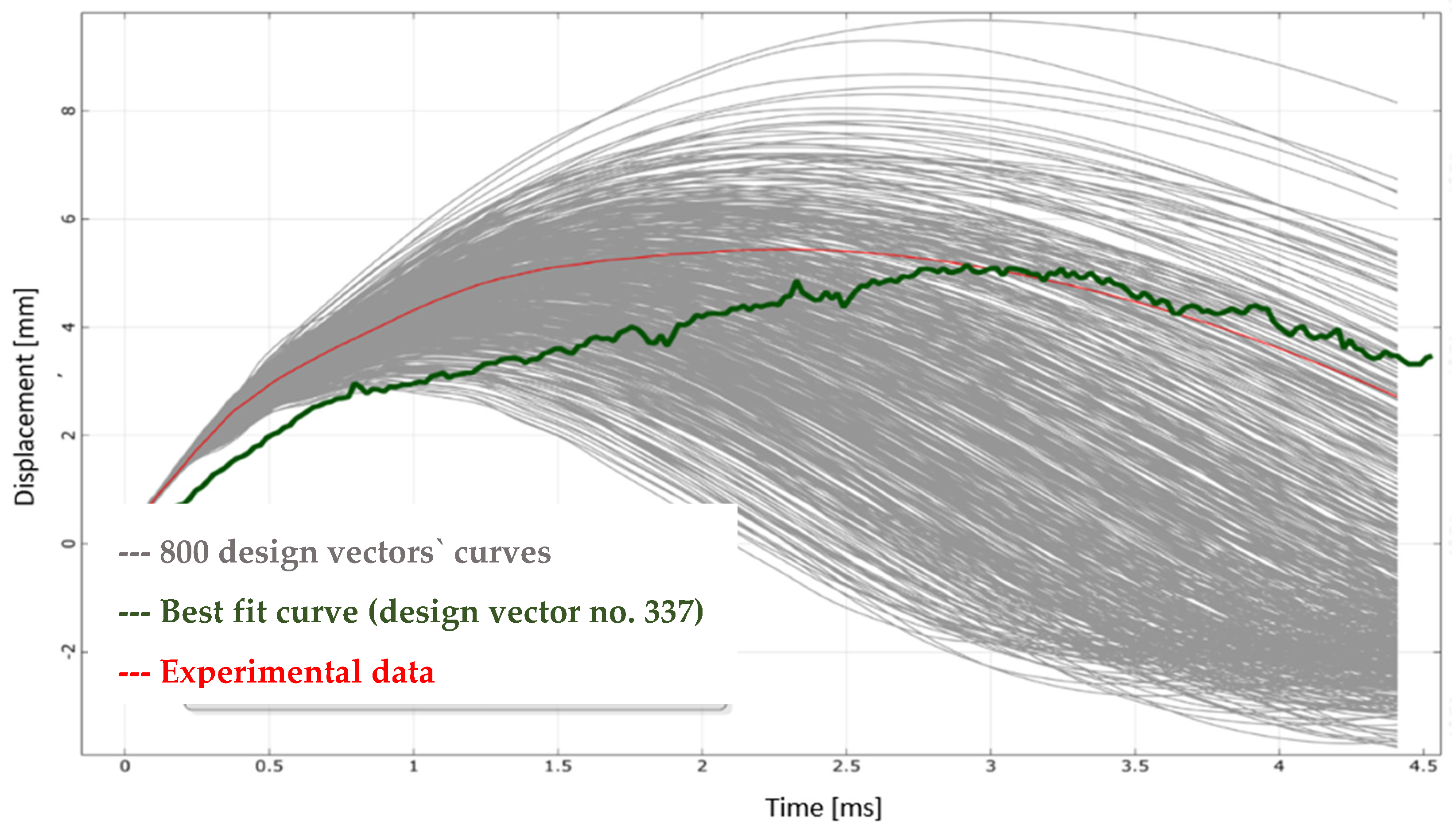
| Dimensions | ||||
|---|---|---|---|---|
| Spec. | Length | Width | Height | Weight |
| No. | (mm) | (mm) | (mm) | (kg) |
| P1 | 149.0 | 150.0 | 150.0 | 9.193 |
| P2 | 148.0 | 150.0 | 150.0 | 8.930 |
| P3 | 146.0 | 150.0 | 150.0 | 9.014 |
| P4.1 | 148.0 | 150.0 | 150.0 | 8.818 |
| P4.2 | 130.8 | 150.0 | 150.0 | 7.805 |
| P4.3 | 150.0 | 150.0 | 150.0 | 9.042 |
| Spec. | Density | Age | Force | Cubic Strength |
|---|---|---|---|---|
| No. | (kg/m3) | (days) | (kN) | (MPa) |
| P1 | 2742 | 1 | 520 | 23.3 |
| P2 | 2682 | 3 | 2300 | 103.6 |
| P3 | 2744 | 7 | 2580 | 117.8 |
| P4.1 | 2648 | 28 | 3020 | 136.0 |
| P4.2 | 2652 | 28 | 3020 | 153.9 |
| P4.3 | 2661 | 28 | 3560 | 157.2 |
| Dimensions | Tensile | |||||||
|---|---|---|---|---|---|---|---|---|
| Spec. | Height | Width | Length | Weight | Density | Age | Force | Strength |
| No. | (mm) | (mm) | (mm) | (kg) | (kg/m3) | (days) | (kN) | (MPa) |
| T1.1 | 101.2 | 100.2 | 400 | 10.50 | 2590 | 28 | 25.84 | 7.64 |
| T1.2 | 101.0 | 100.4 | 400 | 10.49 | 2587 | 28 | 27.82 | 8.20 |
| T1.3 | 101.0 | 100.4 | 400 | 10.49 | 2587 | 28 | 26.53 | 7.82 |
| T2.1 | 100.7 | 100.4 | 400 | 10.58 | 2617 | 28 | 29.42 | 8.70 |
| T2.2 | 100.9 | 99.5 | 400 | 10.24 | 2548 | 28 | 29.44 | 8.84 |
| T2.3 | 101.6 | 100.2 | 400 | 10.41 | 2553 | 28 | 30.82 | 9.05 |
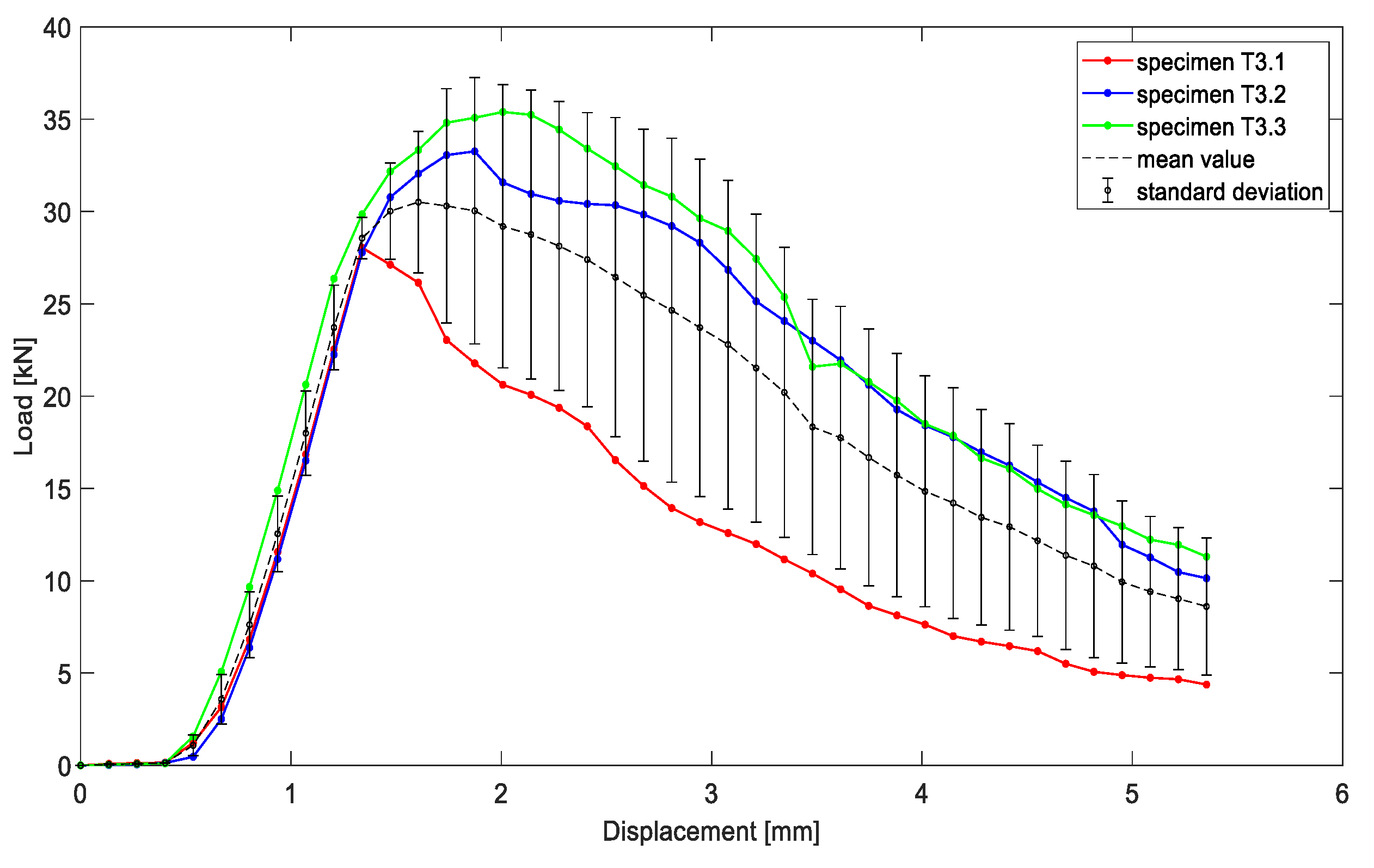
| T3.1 | 100.0 | 99.9 | 400 | 10.39 | 2598 | 28 | 28.92 | 8.68 |
| T3.2 | 100.0 | 100.5 | 400 | 10.49 | 2610 | 28 | 33.36 | 9.92 |
| T3.3 | 98.1 | 100.1 | 400 | 10.39 | 2643 | 28 | 35.54 | 10.84 |
| Test Number | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| TNT mass (g) | 75 | 75 | 200 | 200 | 225 | 225 |
| Distance (mm) | 50 | 50 | 300 | 200 | 250 | 200 |
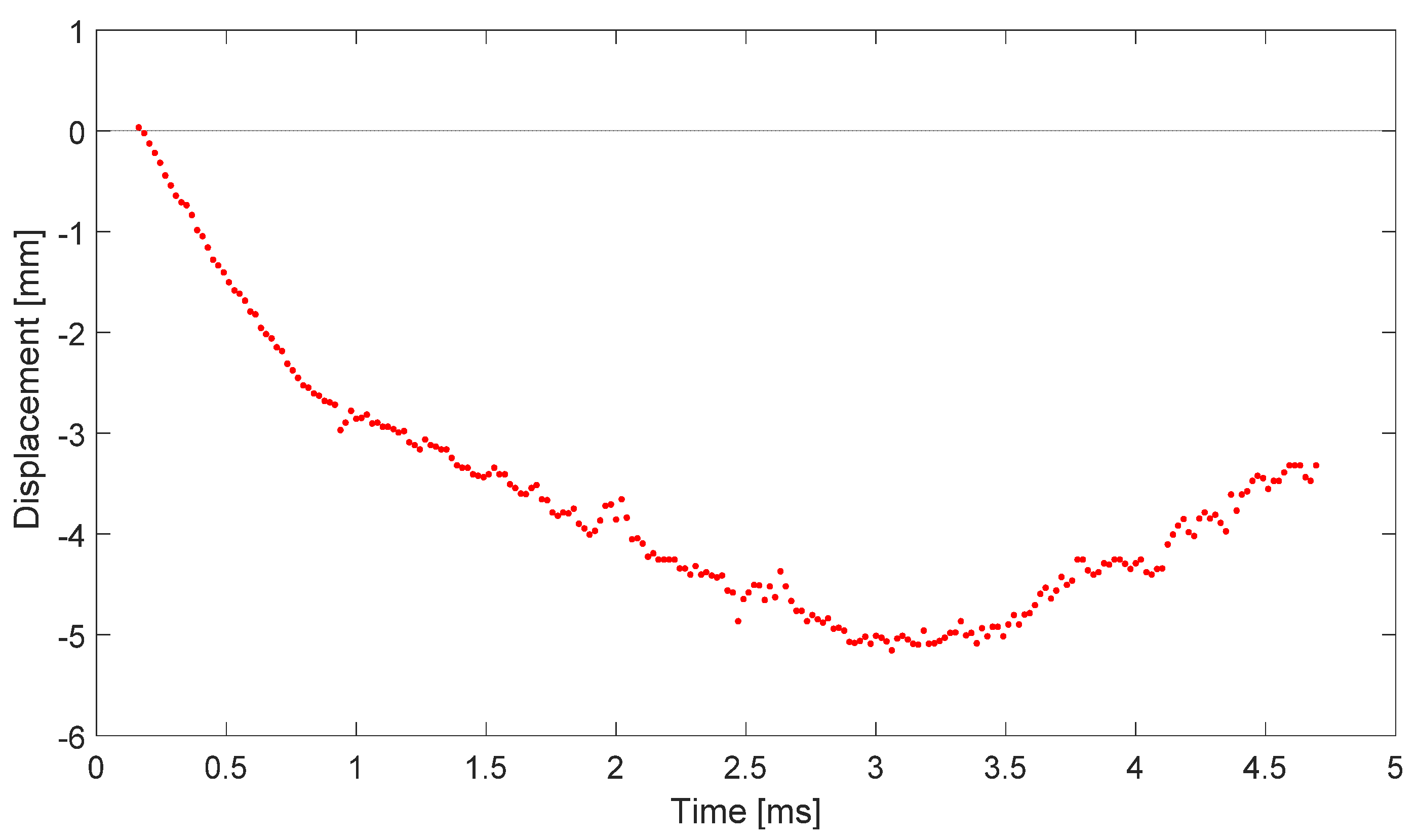
| Displacement (mm) | 2.25 | 2.20 | 3.20 | 4.66 | 4.50 | 5.10 |
| Source | This Paper | [10] | [23] | [24] | [24] | [30] | [30] | [32] | [33] |
|---|---|---|---|---|---|---|---|---|---|
| Chapter, figure, table | Table 4 | Chapter 2.2 | Chapter 5, Figure 6 | Chapter 2, Table 2 | Chapter 2, Table 2 | Chapter 2.1, Table 1 and Table 7 | Chapter 2, Table 1, Table 2 and Table 7 | Figure 2, Table 3 and Table 5 | Table 1 and Table 2 |
| Specimen/ test name | 6 | 2-1 | 6-1 | 2 L30-80 | 2 L60-40 | I | B | ||
| Dimensions [mm] | 500 × 500 × 60 | 1250 × 1250 × 50 | 1300 × 1000 × 100 | 660 × 660 × 25 | 660 × 660 × 25 | 550 × 550 × 50 | 550 × 550 × 50 | 1000 × 1000 × 40 | 750 × 750 × 30 |
| Material | UHPFRC | RC (bars) | SFRC | UHPFRC | UHPFRC | HSFRC | HSFRC | RC (bars) | RC (bars) |
| Fibre dosage [%] | 2.0 | 2.0 | 6.0 (hybrid) | 1.0 | 0.5 | ||||
| Standoff distance [mm] | 200.0 | 500.0 | 100.0 | 500.0 | 500.0 | 242.5 | 242.5 | 400.0 | 300.0 |
| Explosive | TNT | TNT | CompB | PE4 | PE4 | TNT | TNT | TNT | TNT |
| Explosive mass [g] | 225 | 640 | 500 | 200 | 200 | 244 | 244 | 200 | 190 |
| Displac. or deflection [mm] | 5.1 | 19.0 | 9.0 | 2.2 (at 1/4 span) | 1.6 (at 1/4 span) | 2.0 | 5.0 | 10.0 | 26.0 |
| Linear Polynomial EOS | Null Material | |||
|---|---|---|---|---|
| C4 (-) | C5 (-) | E0 (J/m3) | V0 (-) | ρ (kg/m3) |
| 0.4 | 0.4 | 253.4 × 103 | 1 | 1.225 |
| Jones–Wilkins–Lee Equation of State | High Explosive Burn Material | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| A (GPa) | B (GPa) | R1 (-) | R2 (-) | ω (-) | E0 (J/m3) | V0 (-) | ρ (kg/m3) | D (m/s) | Pcj (GPa) |
| 3.712 | 3.231 | 4.15 | 0.95 | 0.3 | 7 × 109 | 1 | 1590 | 6930 | 21 |
| Parameter’s Name (Unit) | Aggregate Size (mm) | Fracture Energy (kN/mm) | Poisson Ratio (-) | Tangent Modulus (GPa) | Compressive Strength (GPa) | Tensile Strength (GPa) |
|---|---|---|---|---|---|---|
| Parameter’s value | 5.0 | 0.001 | 0.2 | 35 | 0.06 | 0.006 |
| Parameter’s Name (Unit) | Aggregate Size (mm) | Fracture Energy (kN/mm) | Poisson Ratio (-) | Tangent Modulus (GPa) | Compressive Strength (GPa) | Tensile Strength (GPa) |
|---|---|---|---|---|---|---|
| Range | 0.25–10 | 0.001–0.02 | 0.12–0.3 | 15–50 | 0.03–0.12 | 0.004–0.012 |
| Vector Number | Aggregate Size (mm) | Fracture Energy (kN/mm) | Poisson Ratio (-) | Tangent Modulus (GPa) | Compressive Strength (GPa) | Tensile Strength (GPa) | O (mm) |
|---|---|---|---|---|---|---|---|
| 337 | 9.6283 | 0.0016 | 0.1590 | 44.8925 | 0.0659 | 0.0070 | 0.840 |
| 141 | 9.6770 | 0.0014 | 0.1347 | 42.1475 | 0.0709 | 0.0083 | 1.023 |
| 246 | 7.8367 | 0.0022 | 0.2128 | 47.3675 | 0.0500 | 0.0060 | 1.144 |
| Correlation Coefficient | (size,O) | ,O) | ,O) | ,O) | ,O) | ,O) |
|---|---|---|---|---|---|---|
| Value | −0.05 | 0.6 | 0.07 | 0.39 | 0.06 | 0.54 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dubec, B.; Maňas, P.; Štoller, J.; Zezulová, E.; Dvořák, P.; Hejmal, Z. Numerical Identification of Material Model Parameters of UHPFRC Slab under Blast Loading. Appl. Sci. 2023, 13, 70. https://doi.org/10.3390/app13010070
Dubec B, Maňas P, Štoller J, Zezulová E, Dvořák P, Hejmal Z. Numerical Identification of Material Model Parameters of UHPFRC Slab under Blast Loading. Applied Sciences. 2023; 13(1):70. https://doi.org/10.3390/app13010070
Chicago/Turabian StyleDubec, Branislav, Pavel Maňas, Jiří Štoller, Eva Zezulová, Petr Dvořák, and Zdeněk Hejmal. 2023. "Numerical Identification of Material Model Parameters of UHPFRC Slab under Blast Loading" Applied Sciences 13, no. 1: 70. https://doi.org/10.3390/app13010070
APA StyleDubec, B., Maňas, P., Štoller, J., Zezulová, E., Dvořák, P., & Hejmal, Z. (2023). Numerical Identification of Material Model Parameters of UHPFRC Slab under Blast Loading. Applied Sciences, 13(1), 70. https://doi.org/10.3390/app13010070







