The Effect of the Yield Strength Coefficient and Natural Vibration Period on the Damage Potential Ranking of Ground Motions
Abstract
1. Introduction
2. Selection of Ground Motion Records
3. Selected Damage Potential IM and Statistical Parameters
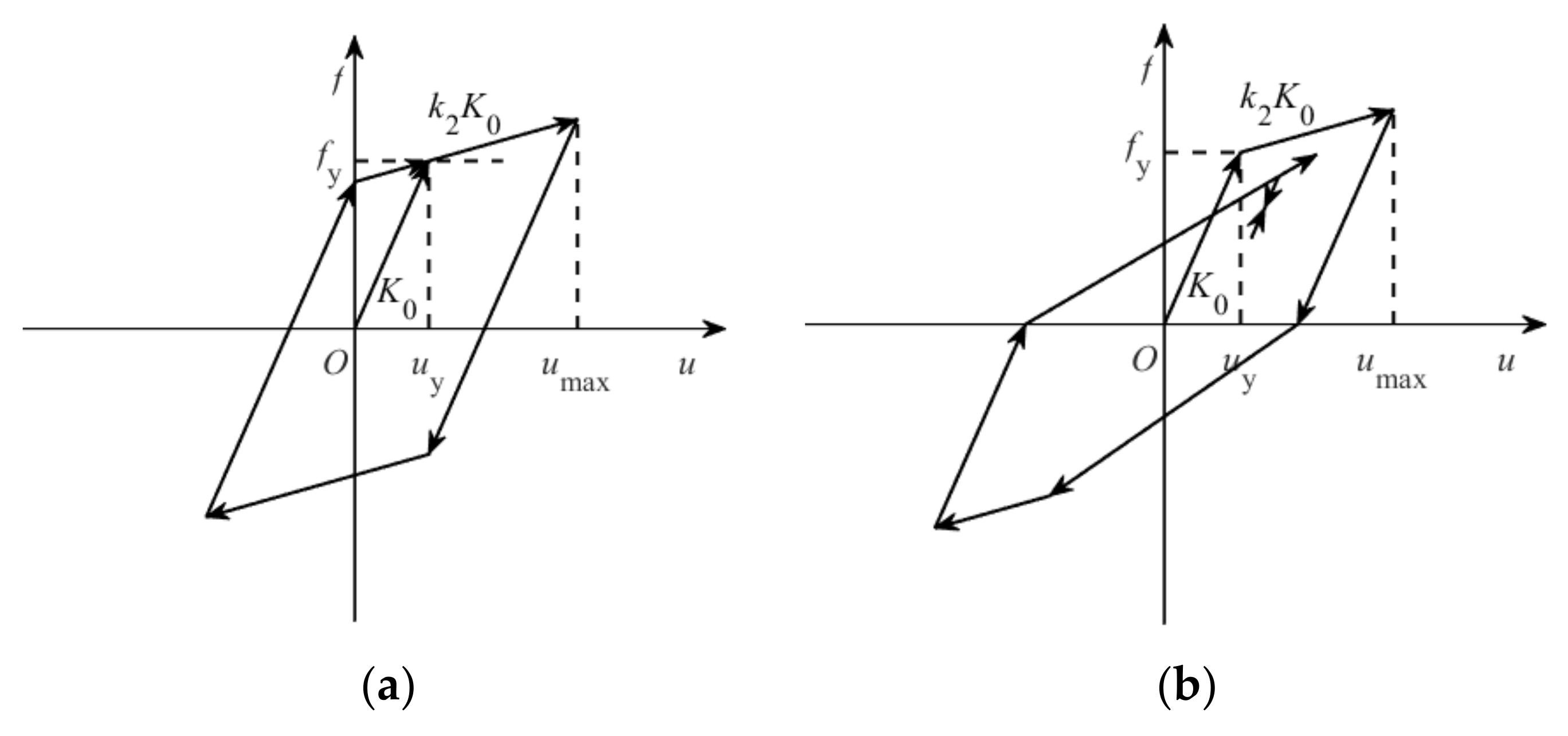
3.1. Damage Potential IM
3.2. Statistical Parameters
- (1)
- Spearman correlation coefficient
- (2)
- Standard deviation and coefficient of variation [31]
4. Effects of Cy and T on Sd Spectrum
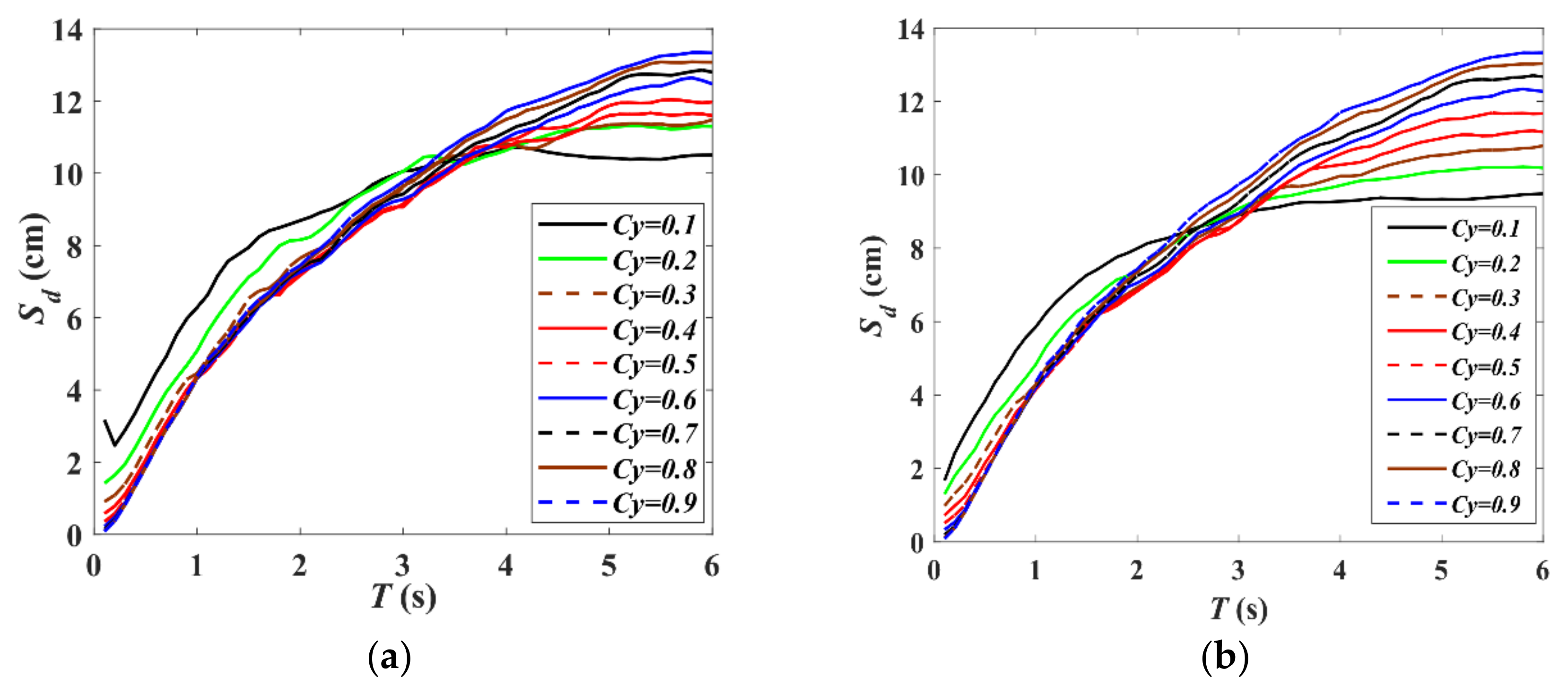
4.1. Analysis of the Sd mean spectrum
- (1)
- The variation law of the Sd mean spectrum under different Cy conditions is the same with the same T based on the two hysteretic models. The larger the T is, the larger the displacement response mean spectrum is.
- (2)
- Although the variation law of the Sd mean spectrum increases with the increase in T, the change speed of the Sd mean spectrum is obviously different under different Cy conditions. The smaller the Cy is, the greater the nonlinear degree of the structure is, and the larger the Sd mean spectrum is in the short- and medium-period ranges, but the smaller the Sd mean spectrum is in the long-period range.
- (3)
- When Cy is equal to 0.1, the change law of the Sd mean spectrum is quite different from that under other Cy conditions. The main reason is that when Cy is equal to 0.1, the yield strength of the structure is only 10% of that of full elasticity, and the character of the SDOF system is too flexible. In addition, it should be analyzed separately.
4.2. Discreteness Analysis of Sd Spectrum
5. Correlation Analysis of Cy and T on the Sd Ranking
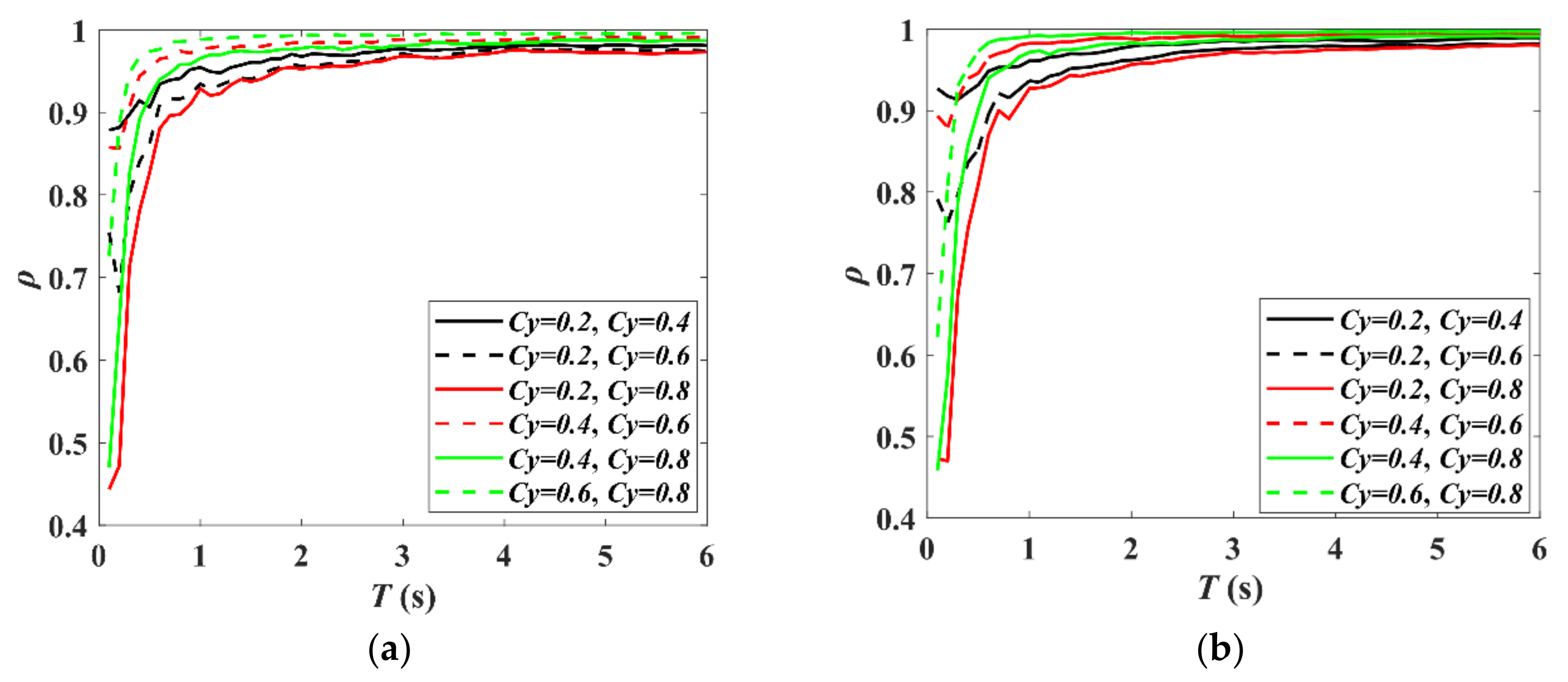
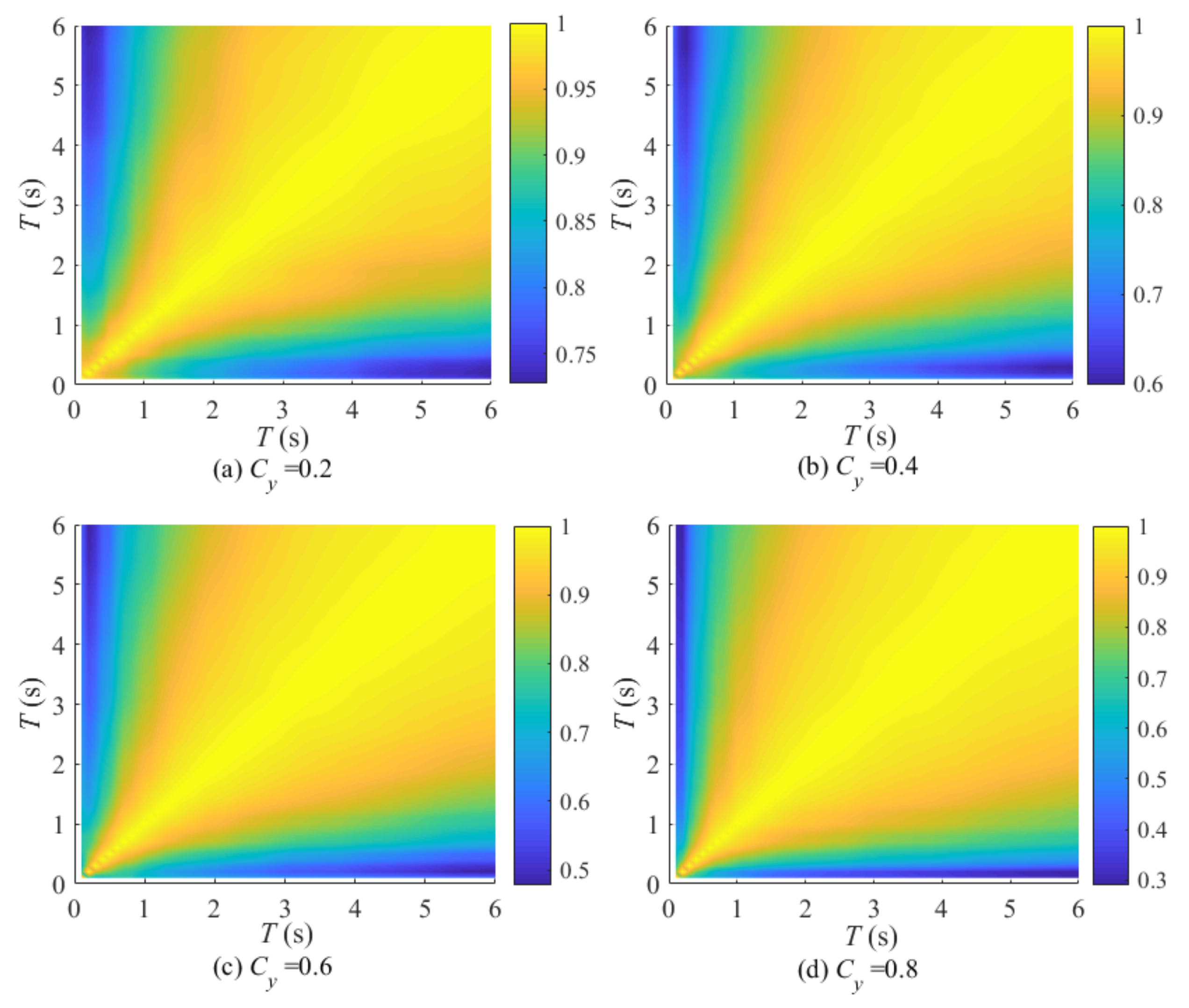
5.1. Analysis of Sd Ranking under Different T and Same Cy Condition
- (1)
- In the short-period range (T < 0.5 s), the correlation coefficients of Sd rankings under different Cy condition is less than 0.8. Especially when the change of Cy is large, the correlation is poor. In the medium- and long-period ranges (T ≥ 0.5 s), the correlation coefficient of the Sd ranking under different Cy conditions is higher, and the correlation is very good. When the Cy changes, the Sd values will change, but the variation of the Sd rankings is small. It can be concluded that the damage potential rankings in the short-period range are more sensitive to the change of Cy than that in the medium- and long-period.
- (2)
- When the change of Cy is smaller, the larger the correlation coefficient of the corresponding Sd rankings is, the smaller the variation of Sd ranking is. On the contrary, when the difference between the Sd rankings under different Cy conditions is larger, the smaller the correlation coefficient of the corresponding Sd rankings is.
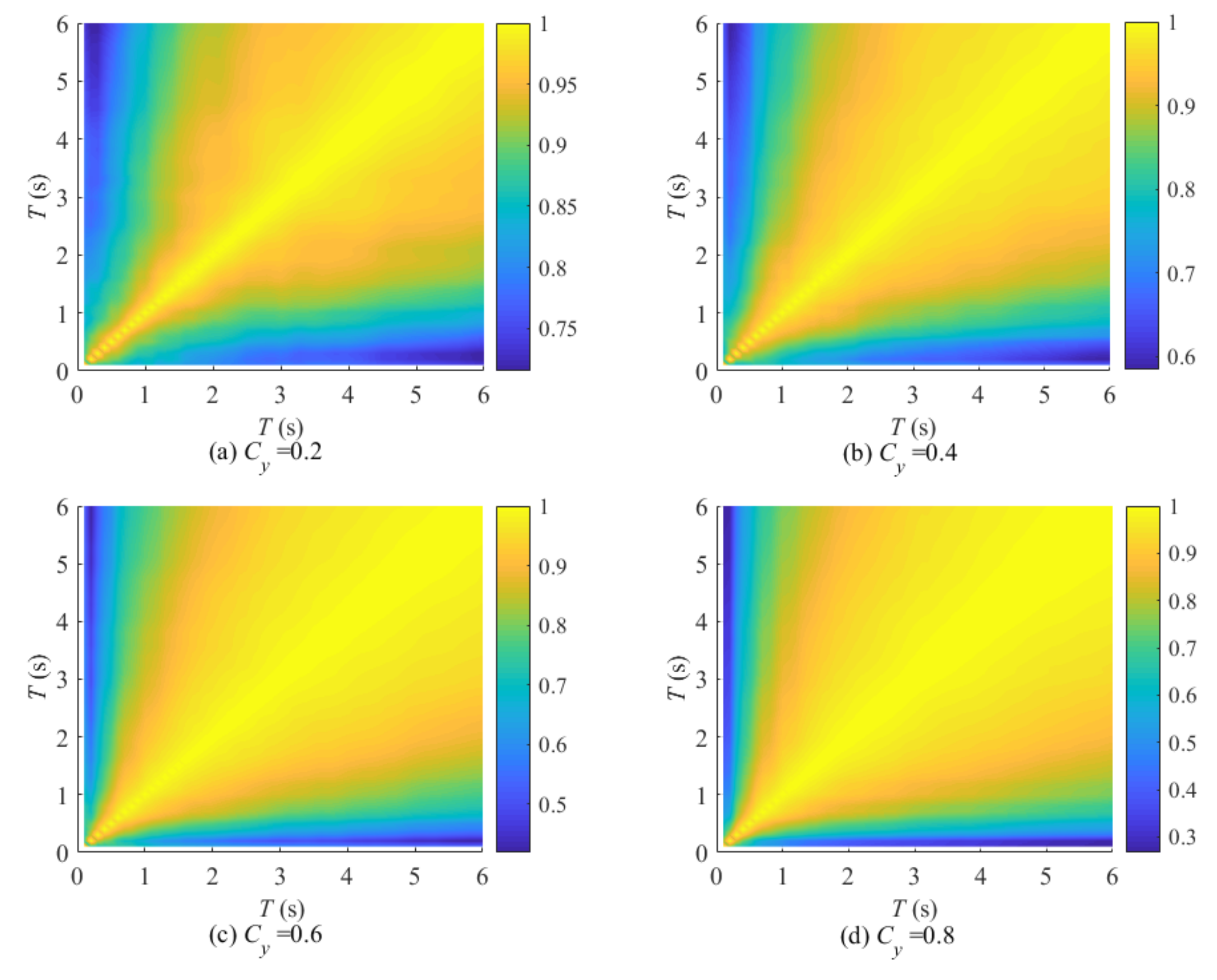
5.2. Correlation Analysis of the Sd Ranking under Different T and Same Cy Condition
- (1)
- The variation law of the Sd ranking at different T is the same. When the ΔT of the two periods is small, the ρ of the corresponding Sd rankings is larger, and the variation of the damage potential ranking is small. On the contrary, when the ΔT of the two periods is larger, the ρ of the corresponding Sd ranking is smaller.
- (2)
- The Sd rankings are more sensitive to the change of T in the short-period compared with the medium- and long-period. More periods need to be selected for research in short-period.
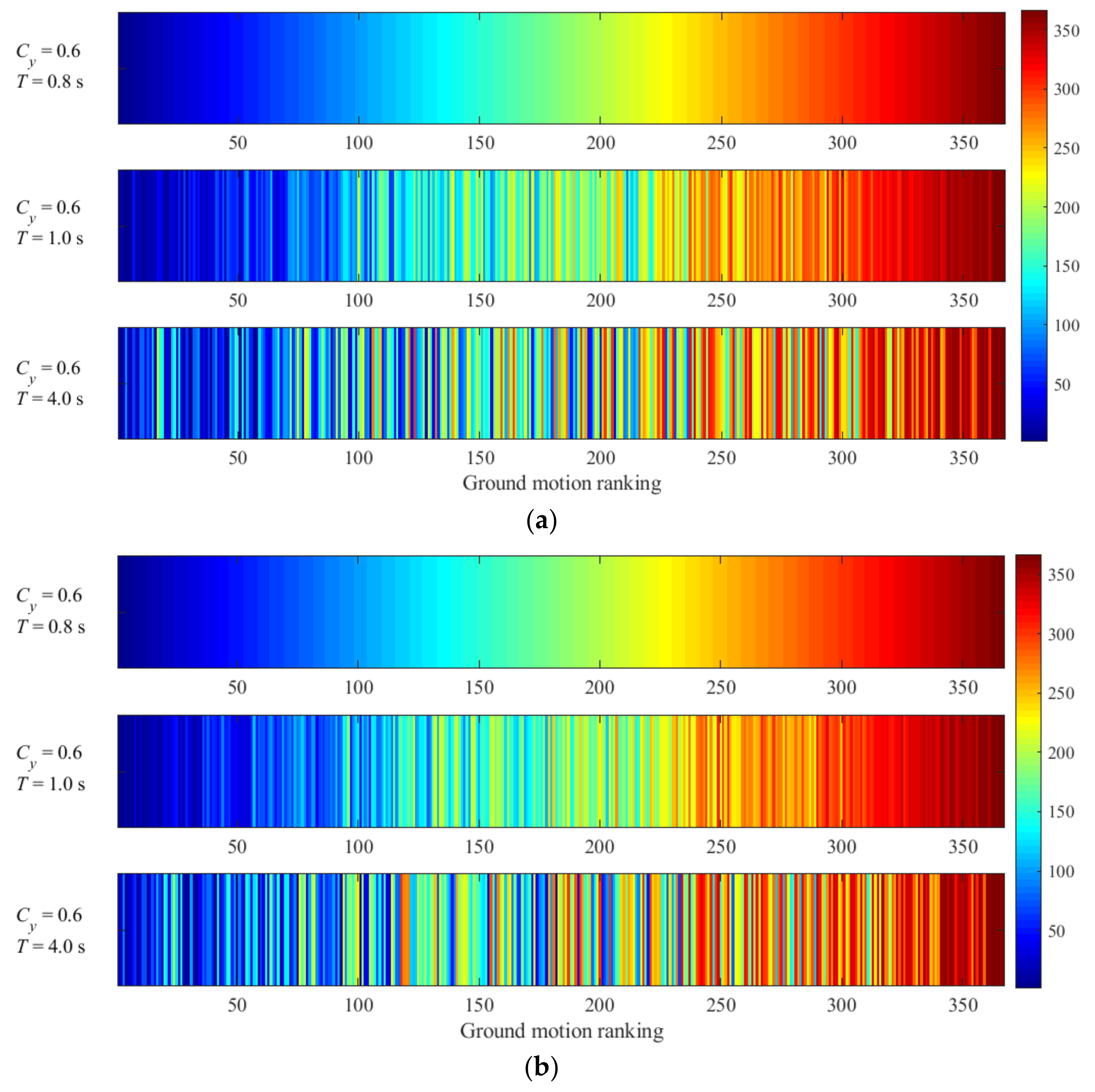
5.3. Comparative Analysis of the Damage Potential Ranking under Specific Cy and T Condition
- (1)
- According to the results of Figure 8 and Table 2, when the T is equal to 0.8 s and 1.0 s, respectively, with a constant Cy value of 0.6, the corresponding ∆T is smaller, and the corresponding damage potential rankings of ground motions have a strong correlation and a smaller discreteness. Whereas the corresponding damage potential rankings results are also quite different when the T is equal to 4.0 s. The coefficient of variation () is increased by 70% and 150%, respectively, based on the bilinear model and modified Clough model compared with the former condition. The corresponding correlation becomes weaker and the discreteness increases.
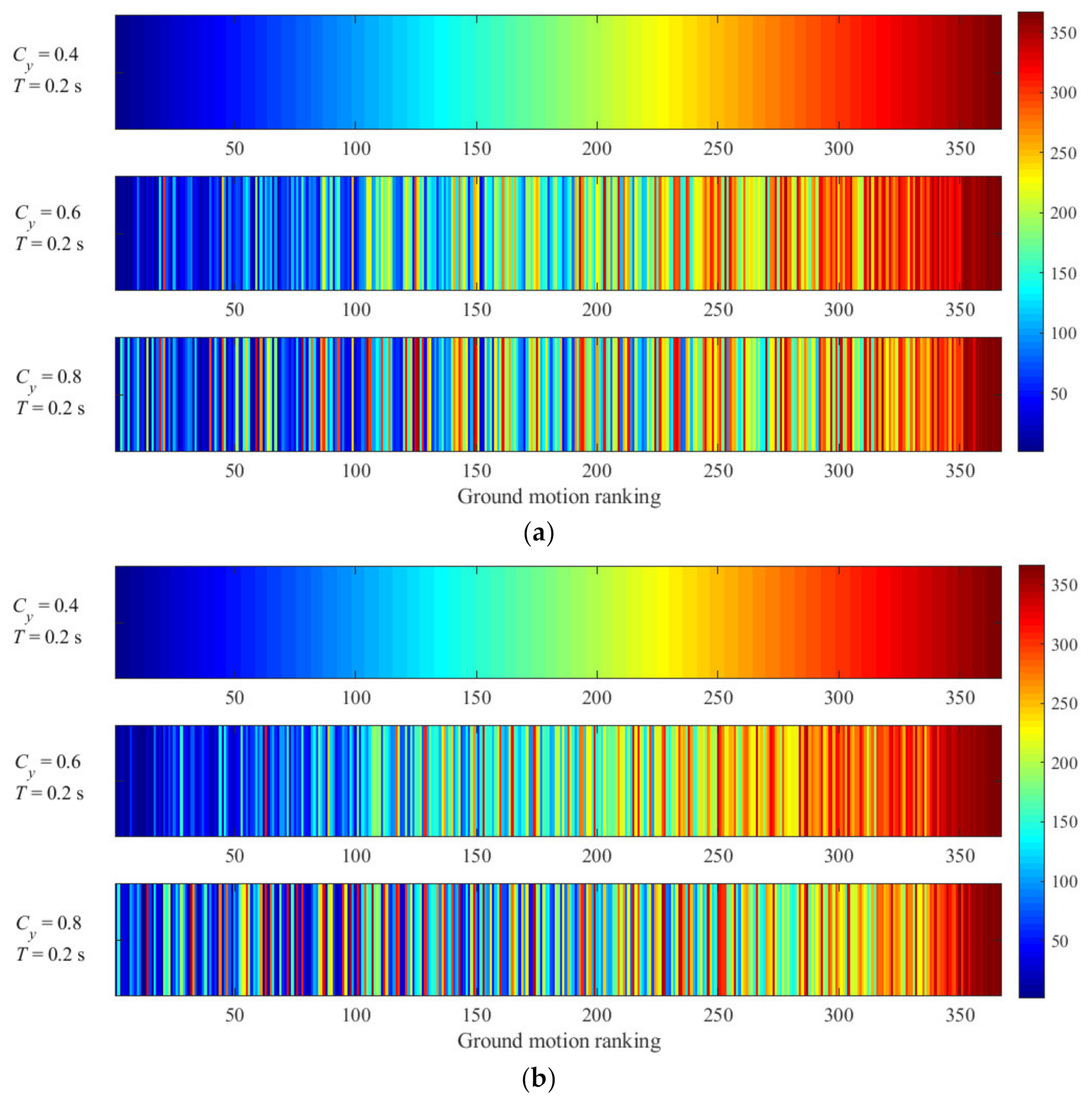
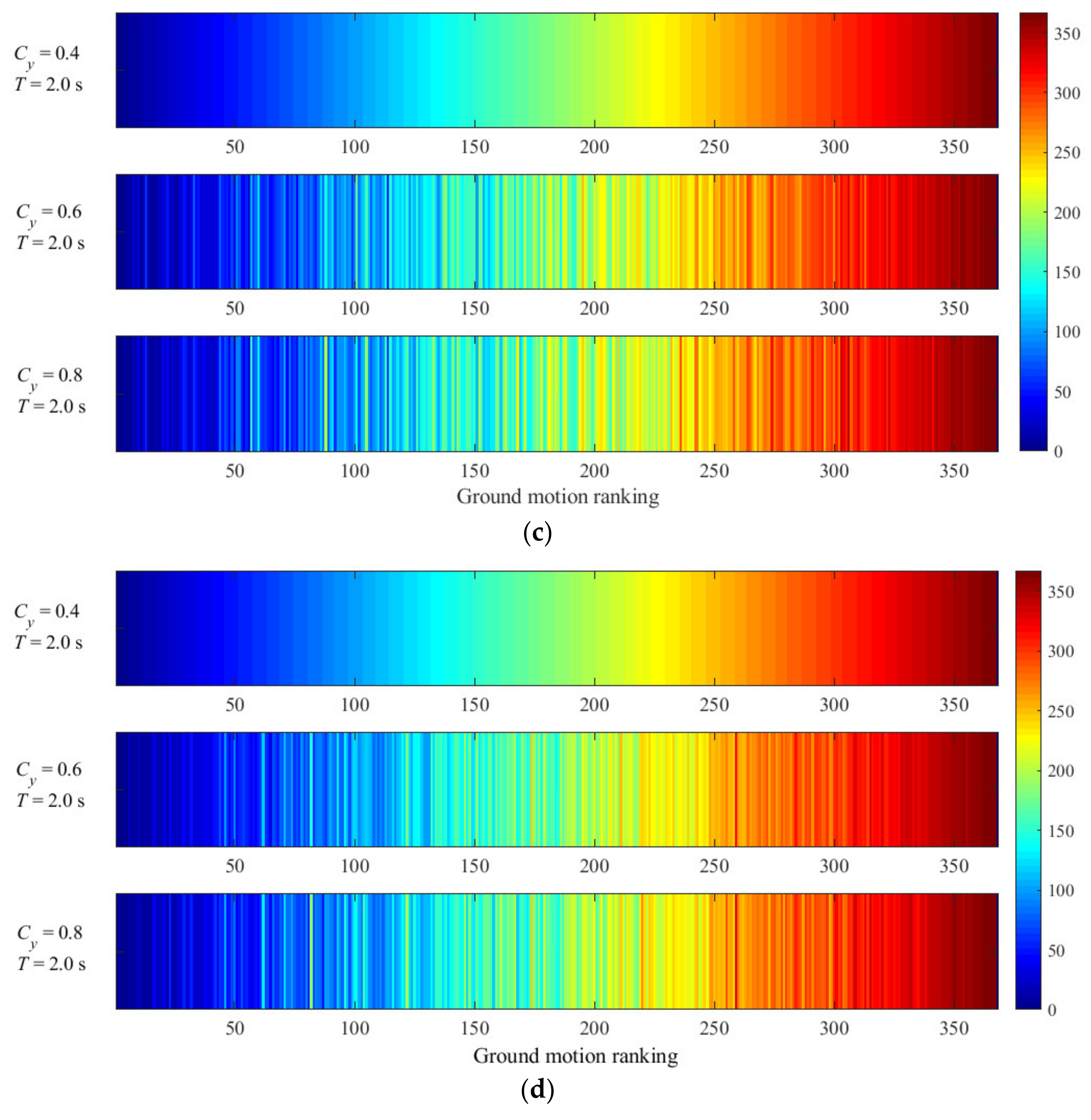
- (2)
- From the results of Figure 9a,b and Table 2, it can be seen that when T is determined (T = 0.2 s, short-period range), there is much difference in Sd ranking under different Cy conditions, and the corresponding coefficient of variation () of the damage potential of ground motions is very large and the correlation coefficient is small. However, it can be seen from Figure 9c,d that when T is determined (T is equal to 2.0 s, medium- and long-period range), the corresponding Sd rankings under different Cy conditions change little. It can be also concluded that the damage potential ranking in the short-period range is more sensitive to the change of Cy than that in the medium- and long-period range.
6. Conclusions
- (1)
- When Cy is constant, the average value of Sd increases with the increase in T. When T is constant, the smaller the Cy in the short- and medium-period ranges, the larger the average value of Sd. On the contrary, the smaller the Cy, the smaller the average value of Sd in the long-period range.
- (2)
- The correlation coefficient of the Sd ranking under different Cy conditions is less than 0.8 in the short-period range. Especially when the difference of Cy is large, the correlation of the corresponding Sd rankings is poor, which indicates that Cy has a great effect on the Sd rankings. In the medium- and long-period range, the correlation coefficient of Sd values corresponding to different Cy is higher, and the correlation is very good, indicating that Cy has almost no effect on the Sd ranking.
- (3)
- In the medium- and long-period range, with the change of T, and when the change of T is small, the Sd value changes obviously, but the change of Sd ranking is very small. When T changes greatly, the Sd value and Sd ranking change greatly.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Infantino, M.; Smerzini, C.; Lin, J. Spatial correlation of broadband ground motions from physics-based numerical simulations. Earthq. Eng. Struct. Dyn. 2021, 50, 2575–2594. [Google Scholar] [CrossRef]
- Wang, W.; Li, D.Q.; Liu, Y.; Du, W. Influence of ground motion duration on the seismic performance of earth slopes based on numerical analysis. Soil Dyn. Earthq. Eng. 2021, 143, 106595. [Google Scholar] [CrossRef]
- Aljawhari, K.; Gentile, R.; Freddi, F.; Galasso, C. Effects of ground-motion sequences on fragility and vulnerability of case-study reinforced concrete frames. Bull. Earthq. Eng. 2021, 19, 6329–6359. [Google Scholar] [CrossRef]
- Bhagat, S.; Wijeyewickrema, A.C.; Subedi, N. Influence of near-fault ground motions with fling-step and forward-directivity characteristics on seismic response of base-isolated buildings. J. Earthq. Eng. 2021, 25, 455–474. [Google Scholar] [CrossRef]
- Cheng, Y.; Fu, L.Y. Nonlinear seismic inversion by physics-informed Caianiello convolutional neural networks for overpressure prediction of source rocks in the offshore Xihu depression, East China. J. Pet. Sci. Eng. 2022, 215, 110654. [Google Scholar] [CrossRef]
- Alam, Z.; Sun, L.; Zhang, C.; Samali, B. Influence of seismic orientation on the statistical distribution of nonlinear seismic response of the stiffness-eccentric structure. Structures 2022, 39, 387–404. [Google Scholar] [CrossRef]
- Huang, S.; Lyu, Y.; Sha, H.; Xiu, L. Seismic performance assessment of unsaturated soil slope in different groundwater levels. Landslides 2021, 18, 2813–2833. [Google Scholar] [CrossRef]
- Kramer, S.L. Geotechnical Earthquake Engineering; Prentice Hall: Englewood Cliffs, NJ, USA, 1996. [Google Scholar]
- Hu, J.J.; Lai, Q.H.; Liu, B.L.; Xie, L.L. Ranking of ground motions destructive capacity for low- and middle-rise RC frame structures based on a comprehensive intensity measure. Adv. Struct. Eng. 2022, 25, 1745–1758. [Google Scholar] [CrossRef]
- Cabanas, L.; Benito, B.; Herraiz, M. An approach to the measurement of the potential structural damage of earthquake ground motions. Earthq. Eng. Struct. Dyn. 1997, 26, 79–92. [Google Scholar] [CrossRef]
- Zhai, C.H.; Xie, L.L. A new approach of selecting real input ground motions for seismic design: The most unfavorable real seismic design ground motions. Earthq. Eng. Struct. Dyn. 2007, 36, 1009–1027. [Google Scholar] [CrossRef]
- Kostinakis, K.; Fontara, I.K.; Athanatopoulou, A.M. Scalar Structure-Specific Ground Motion Intensity Measures for Assessing the Seismic Performance of Structures: A Review. J. Earthq. Eng. 2018, 22, 630–665. [Google Scholar] [CrossRef]
- Zhai, C.H.; Wen, W.P.; Li, S. The damage investigation of inelastic SDOF structure under the mainshock–aftershock sequence-type ground motions. Soil Dyn. Earthq. Eng. 2014, 59, 30–41. [Google Scholar] [CrossRef]
- Zhai, C.H.; Ji, D.F.; Wen, W.P. Constant ductility energy factors for the near-fault pulse-like ground motions. J. Earthq. Eng. 2017, 21, 343–358. [Google Scholar] [CrossRef]
- Li, C.H.; Kunnath, S.; Zhai, C.H. Influence of Early-Arriving Pulse-Like Ground Motions on Ductility Demands of Single-Degree-of-Freedom Systems. J. Earthq. Eng. 2020, 24, 1337–1360. [Google Scholar] [CrossRef]
- Zhai, C.H.; Ji, D.F.; Wen, W.P.; Weidong, L.; Xie, L.; Gong, M. The inelastic input energy spectra for main shock–aftershock sequences. Earthq. Spectra 2016, 32, 2149–2166. [Google Scholar] [CrossRef]
- Rupakhety, R.; Sigbjörnsson, R. Ground-Motion Prediction Equations (GMPEs) for inelastic displacement and ductility demands of constant-strength SDOF systems. Bull. Earthq. Eng. 2009, 7, 661–679. [Google Scholar] [CrossRef]
- Miranda, E. Inelastic Displacement ratios for structures on firm sites. J. Struct. Eng. 2000, 126, 1150–1159. [Google Scholar] [CrossRef]
- Yaghmaei, S.S.; Makaremi, S. Development of duration- dependent damage-based inelastic response spectra. Earthq. Eng. Struct. Dyn. 2017, 46, 771–789. [Google Scholar] [CrossRef]
- Longjun, X.; Yabin, C. Easy detection for the high-pass filter cut-off frequency of digital ground motion record based on STA/LTA method: A case study in the 2008 Wenchuan mainshock. J. Seismol. 2021, 25, 1281–1300. [Google Scholar] [CrossRef]
- Vaiana, N.; Rosati, L. Classification and unified phenomenological modeling of complex uniaxial rate-independent hysteretic responses. Mech. Syst. Signal Process. 2023, 182, 109539. [Google Scholar] [CrossRef]
- Palanci, M.; Senel, S.M. Correlation of earthquake intensity measures and spectral displacement demands in building type structures. Soil Dyn. Earthq. Eng. 2019, 121, 306–326. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, L.; Zhu, X.; Liu, H. A multi-objective ground motion selection approach matching the acceleration and displacement response spectra. Sustainability 2018, 10, 4659. [Google Scholar] [CrossRef]
- Zhai, C.H.; Wen, W.P.; Chen, Z.Q.; Li, S.; Xie, L.-L. Damage spectra for the mainshock–aftershock sequence-type ground motions. Soil Dyn. Earthq. Eng. 2013, 45, 1–12. [Google Scholar] [CrossRef]
- Hu, J.J.; Lai, Q.H.; Li, S.; Xie, L.L. Procedure for ranking ground motion records based on the destructive capacity parameter. KSCE J. Civ. Eng. 2020, 25, 197–207. [Google Scholar] [CrossRef]
- Hu, J.J.; Lai, Q.H.; Liu, X.; Xie, L.L. Effects of structural and seismic factors on the constant-strength ductility spectra based on NGA-West2 database. Shock Vib. 2020, 2020, 8820582. [Google Scholar] [CrossRef]
- Eurocode 8: Design of Structures for Earthquake Resistance-Part 1: General Rules, Seismic Actions and Rules for Buildings; Technical Committee CEN/TC: Brussels, Belgium, 2005.
- GB 50011−2010; Code for Seismic Design of Buildings. Architecture and Building Press: Beijing, China, 2010. (In Chinese)
- American Society of Civil Engineers. Minimum Design Loads for Buildings and Other Structures; Amer Society of Civil Engineers: Reston, VA, USA, 2010. [Google Scholar]
- Lai, Q.H.; Hu, J.J.; Xu, L.; Xie, L.; Lin, S. Method for Ranking Pulse-like Ground Motions According to Damage Potential for Reinforced Concrete Frame Structures. Buildings 2022, 12, 754. [Google Scholar] [CrossRef]
- Wang, D.H. Multivariate Statistical Analysis and SPSS Application; East China University of Science and Technology Press: Sanghai, China, 2010. (In Chinese) [Google Scholar]


| Type of Sites | Vs30 (m/s) | Number |
|---|---|---|
| AB | >750 | 58 |
| C | 360–760 | 113 |
| D | 180–360 | 115 |
| E | <180 | 81 |
| Ground Motion Ranking | Bilinear Model | Modified Clough Model | |||
|---|---|---|---|---|---|
| Ranking 1 | Ranking 2 | ||||
| Cy = 0.6, T = 0.8 s | Cy = 0.6, T = 1.0 s | 0.958 | 0.168 | 0.965 | 0.152 |
| Cy = 0.6, T = 4.0 s | 0.791 | 0.290 | 0.782 | 0.381 | |
| Cy = 0.4, T = 0.2 s | Cy = 0.6, T = 0.2 s | 0.856 | 0.310 | 0.879 | 0.284 |
| Cy = 0.8, T = 0.2 s | 0.647 | 0.485 | 0.572 | 0.533 | |
| Cy = 0.4, T = 2.0 s | Cy = 0.6, T = 2.0 s | 0.984 | 0.102 | 0.988 | 0.089 |
| Cy = 0.8, T = 2.0 s | 0.978 | 0.122 | 0.983 | 0.105 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lai, Q.; Hu, J.; Xie, L. The Effect of the Yield Strength Coefficient and Natural Vibration Period on the Damage Potential Ranking of Ground Motions. Appl. Sci. 2023, 13, 392. https://doi.org/10.3390/app13010392
Lai Q, Hu J, Xie L. The Effect of the Yield Strength Coefficient and Natural Vibration Period on the Damage Potential Ranking of Ground Motions. Applied Sciences. 2023; 13(1):392. https://doi.org/10.3390/app13010392
Chicago/Turabian StyleLai, Qinghui, Jinjun Hu, and Lili Xie. 2023. "The Effect of the Yield Strength Coefficient and Natural Vibration Period on the Damage Potential Ranking of Ground Motions" Applied Sciences 13, no. 1: 392. https://doi.org/10.3390/app13010392
APA StyleLai, Q., Hu, J., & Xie, L. (2023). The Effect of the Yield Strength Coefficient and Natural Vibration Period on the Damage Potential Ranking of Ground Motions. Applied Sciences, 13(1), 392. https://doi.org/10.3390/app13010392






