Influence of Initial Conditions on Wind Characteristics at a Bridge Middle Span in a U-Shaped Valley by CFD and AHP
Abstract
:1. Introduction
2. Numerical Calculation Model
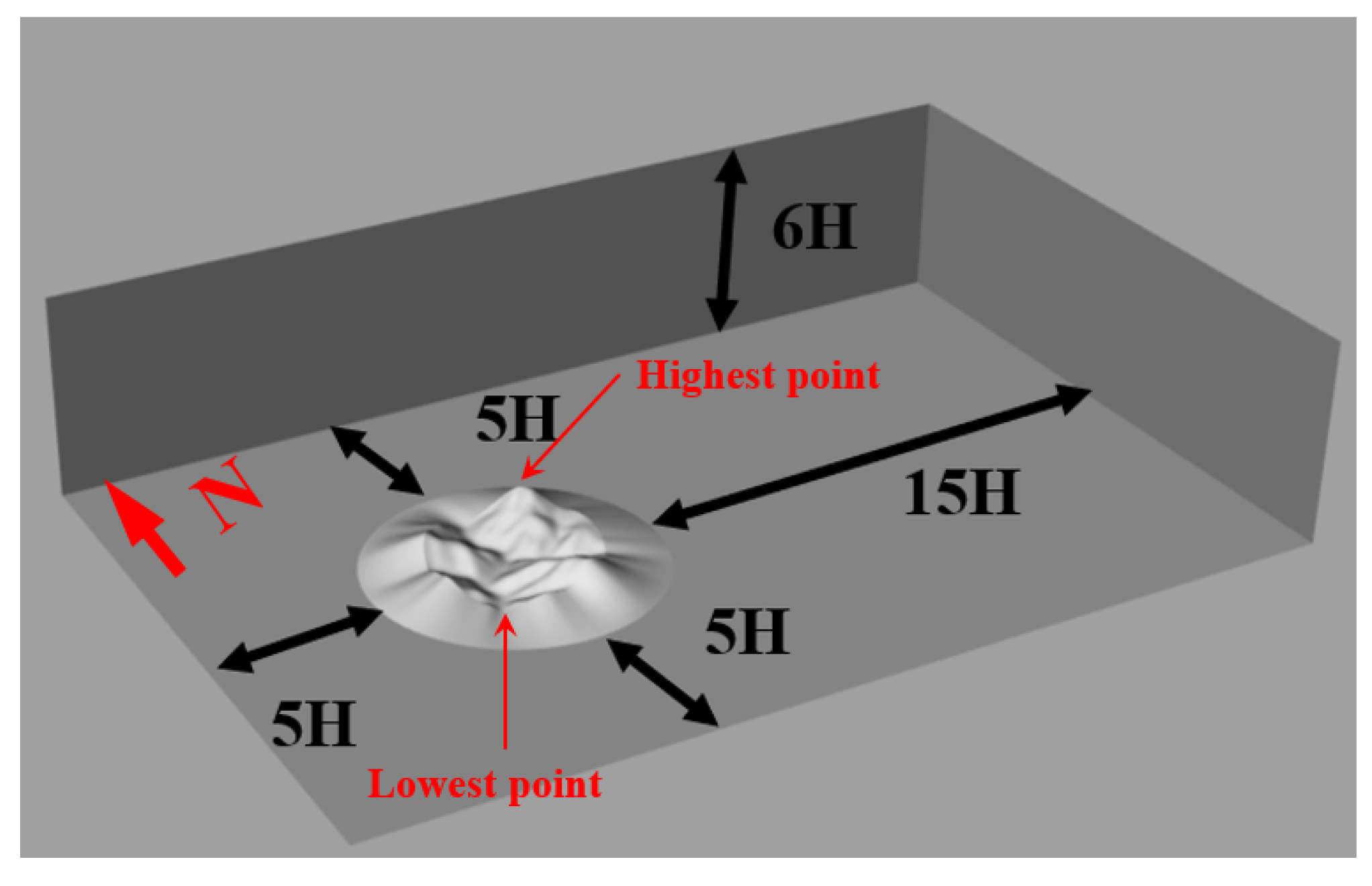
2.1. Terrain and Calculation Domain
2.2. FLUENT Setting
2.3. Mesh Division and Independence Validation
3. Analytic Hierarchy Process and Initial Conditions
3.1. Analytic Hierarchy Process
3.2. Initial Conditions
4. Results and Discussion
4.1. Influence of Initial Conditions on Wind Parameter
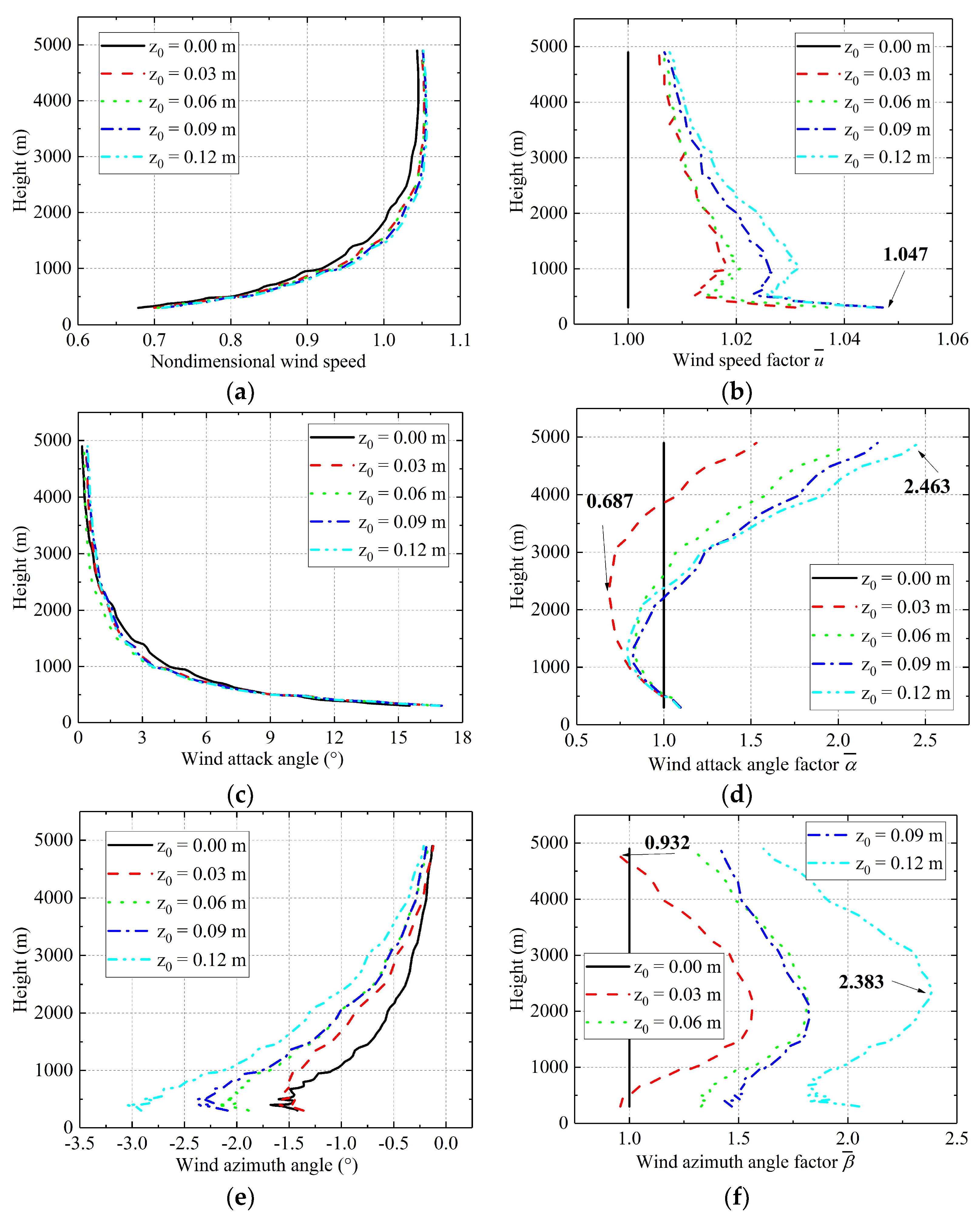
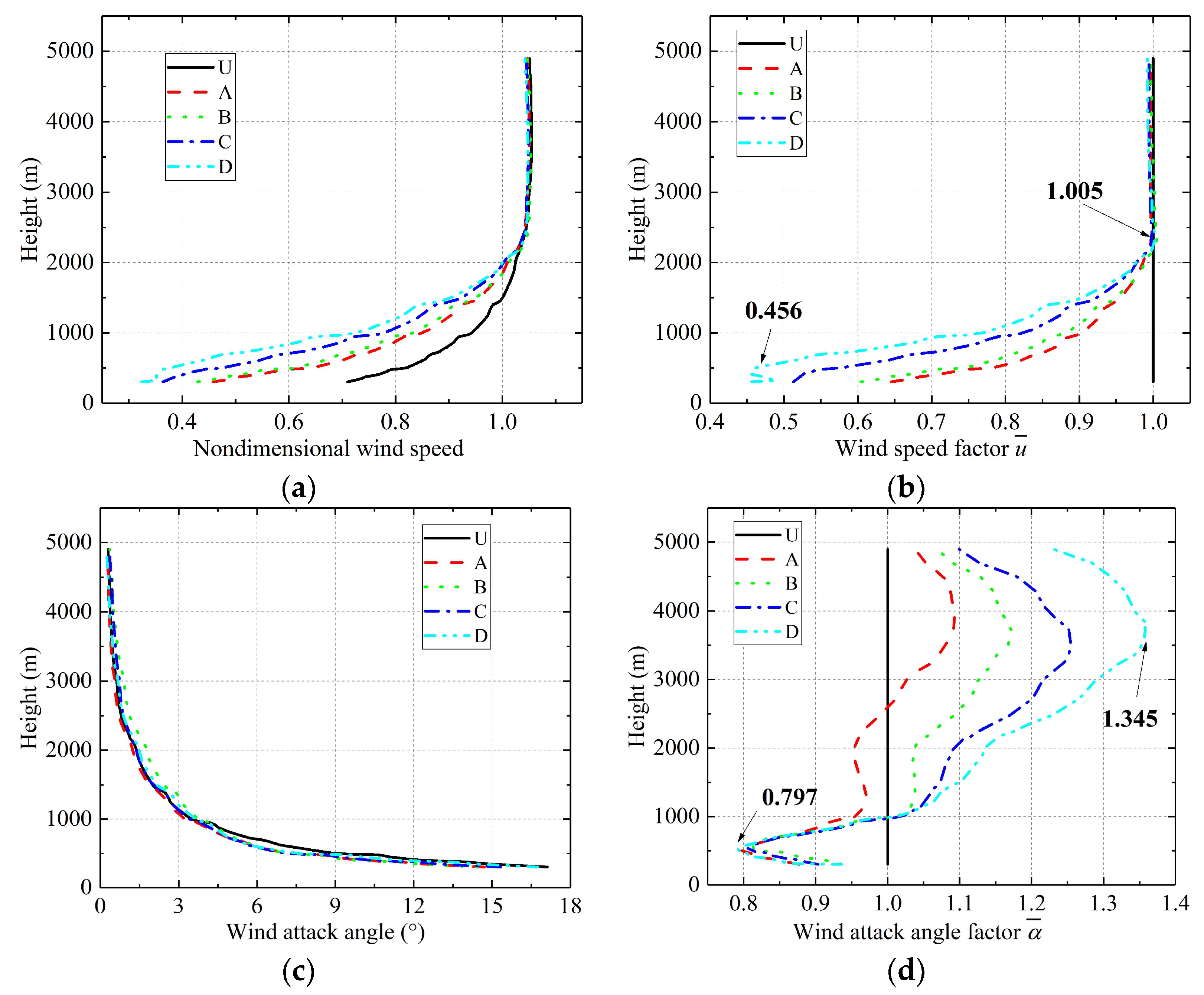
4.1.1. Surface Roughness
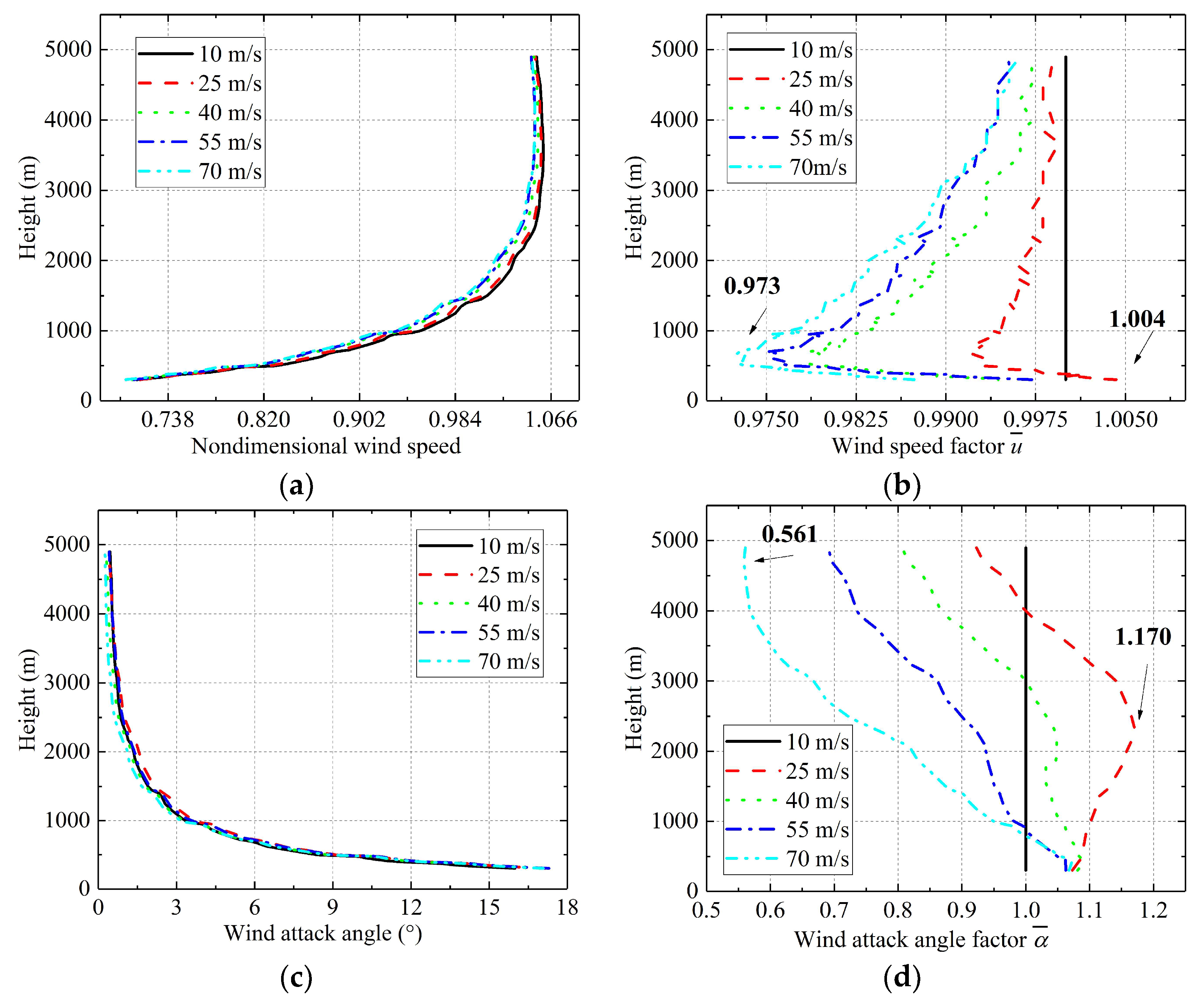
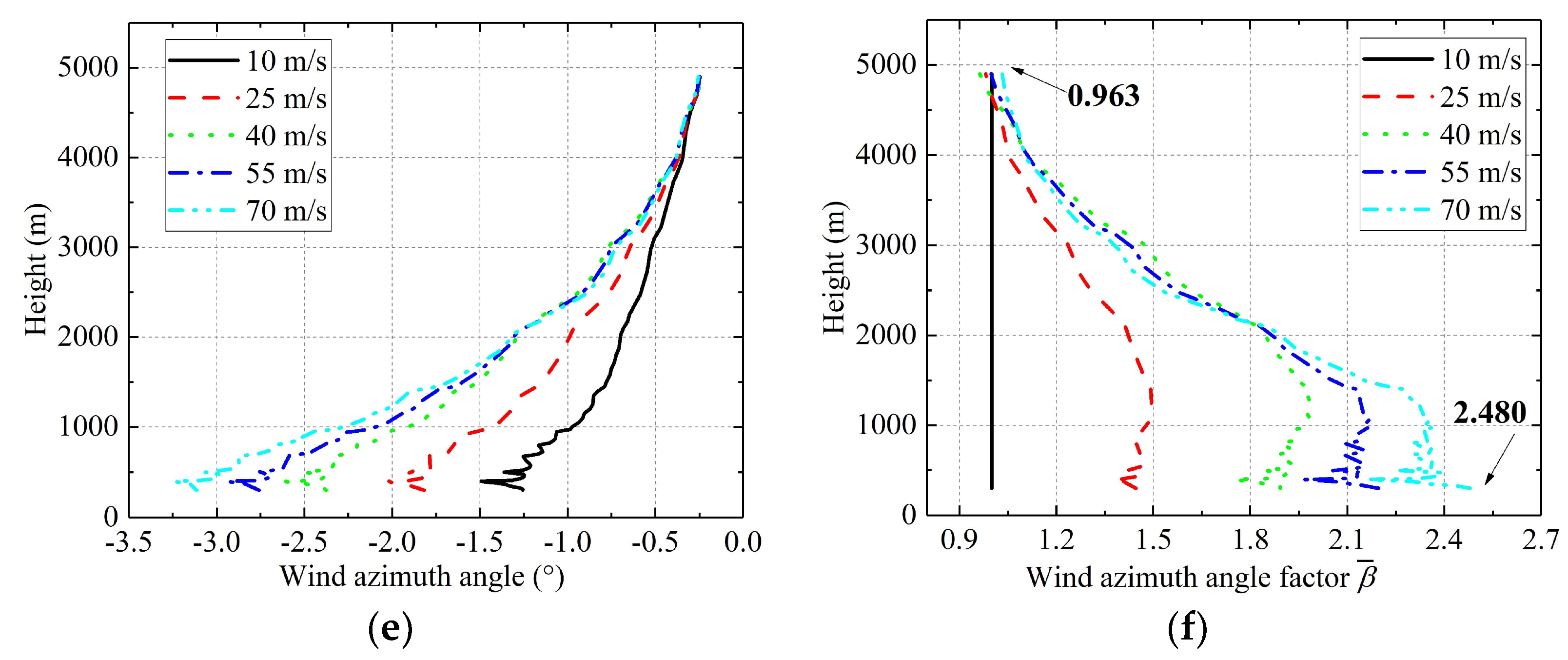
4.1.2. Inlet Wind Speed
4.1.3. Wind Speed Profile
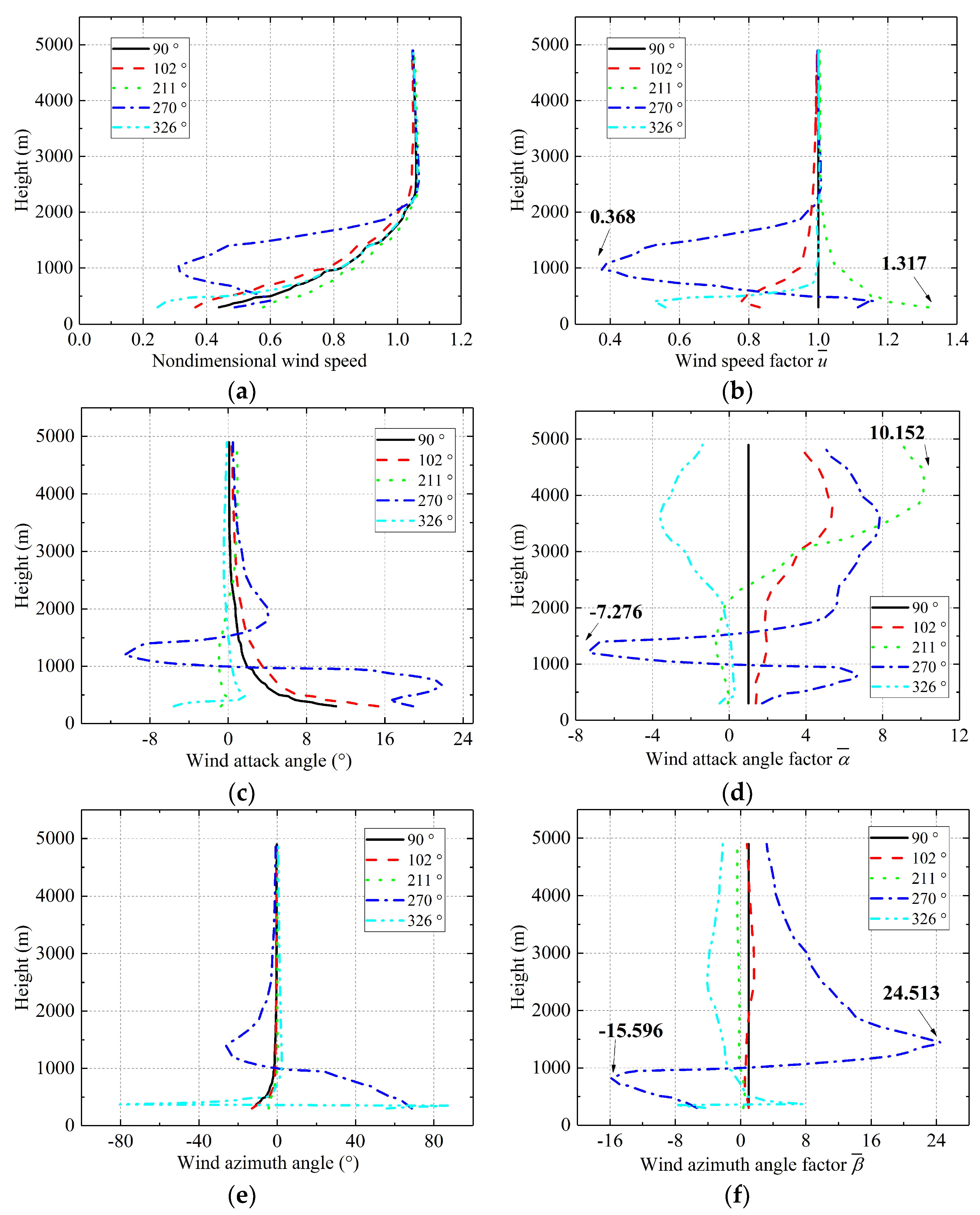
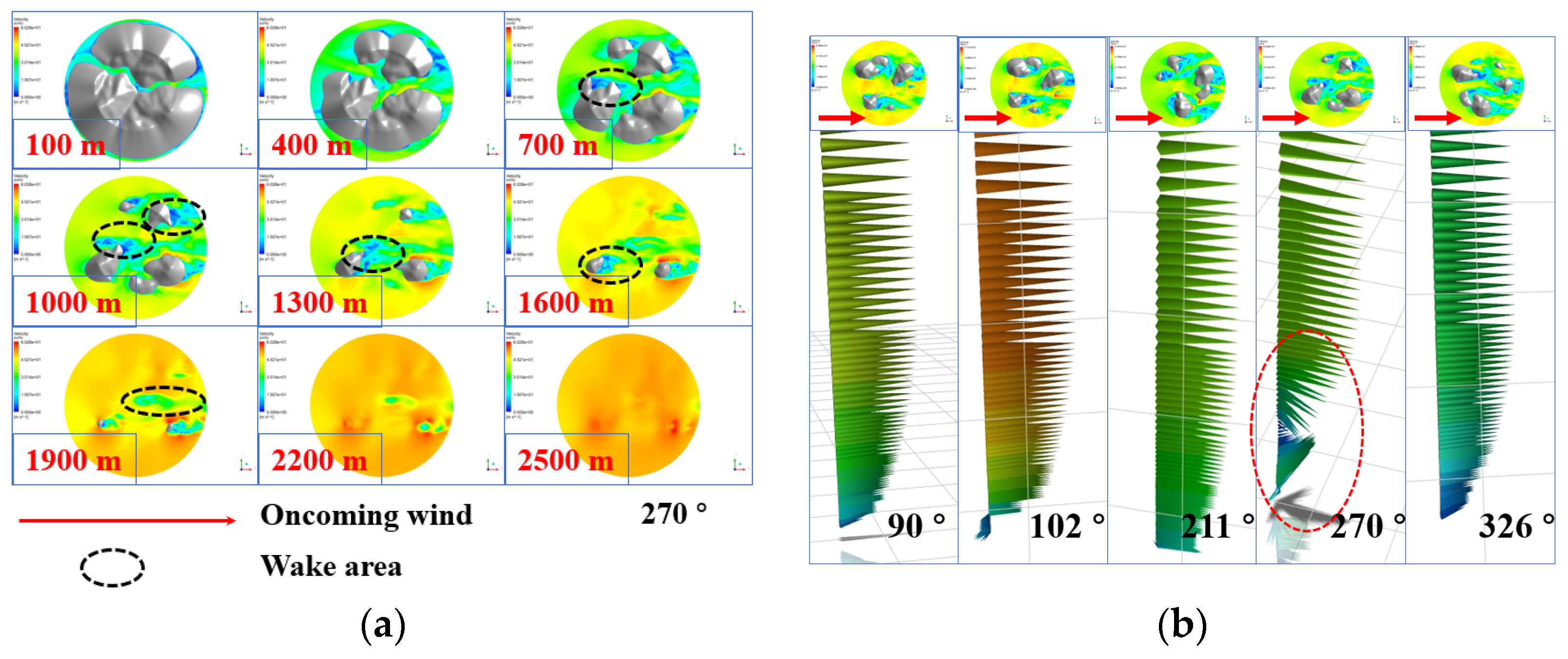
4.1.4. Oncoming Wind Direction
4.2. Impact Assessment
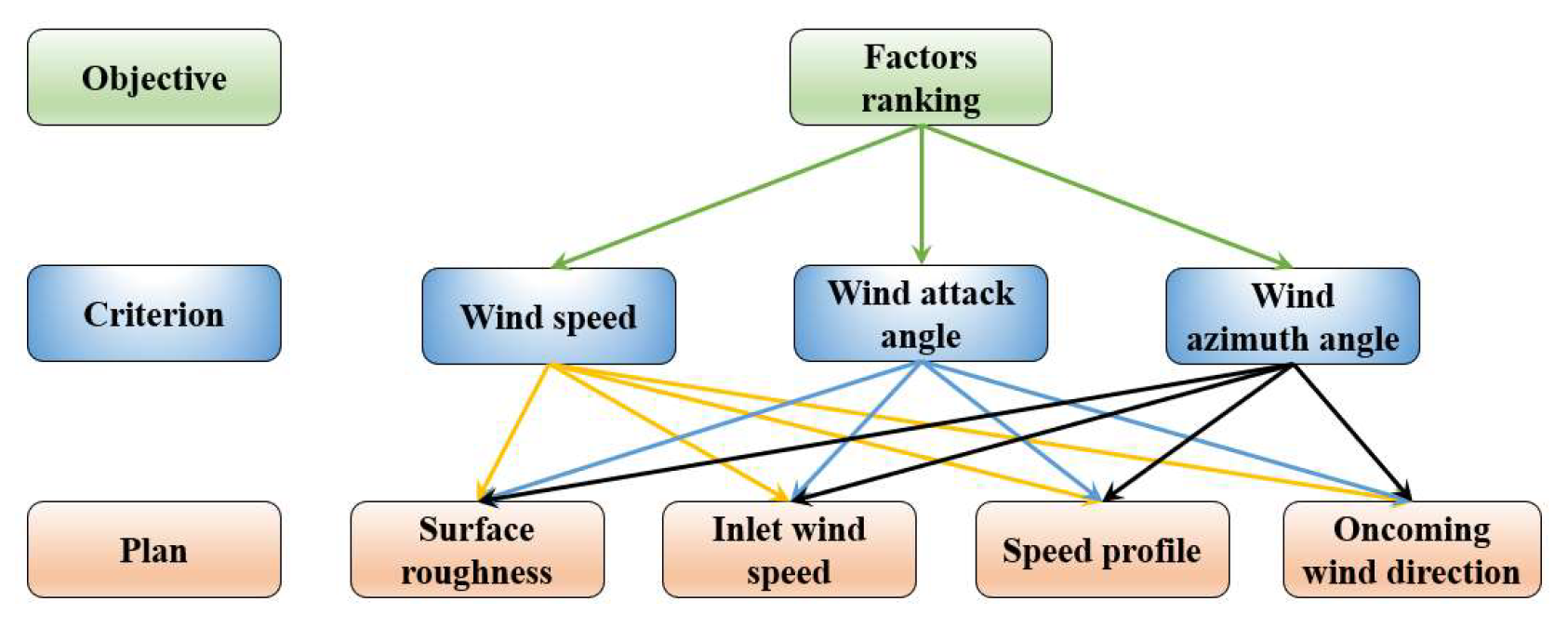
4.2.1. Hierarchical Structure Establishment
4.2.2. Establishment of Quantification Model
4.2.3. Impact Assessment Analysis
- (1)
- For wind speed: oncoming wind direction = wind speed profile > surface roughness > inlet wind speed.
- (2)
- For wind attack angle: oncoming wind direction > surface roughness > inlet wind speed > wind speed profile.
- (3)
- For wind azimuth angle: oncoming wind direction > wind speed profile > surface roughness = inlet wind speed.
5. Conclusions
- (1)
- The numerical results showed that the spatial distribution of wind parameters at the mid-span of a bridge in a U-shaped valley is complex and is significantly affected by the initial conditions.
- (2)
- In order to connect CFD and AHP, the wind parameter factor and factor sensitivity coefficient were proposed. A new quantification model was established to describe the functional relationship between the factor sensitivity coefficient and the scale value, and the logarithmic function reliability was verified. An evaluation system for the influence of initial conditions on wind parameters was formed.
- (3)
- The evaluation results showed that the influence of oncoming wind direction and wind speed profile on wind speed was equivalent, followed by surface roughness and inlet wind speed. The sensitivity of the wind attack angle to the oncoming wind direction was much higher than that of surface roughness, inlet wind speed, and wind speed profile. The oncoming wind direction had the greatest influence on the wind azimuth angle, which was the sum of wind speed profile, surface roughness, and inlet wind speed.
- (4)
- As for the wind-resistant of bridges in the U-shaped valley, oncoming wind direction was the first initial condition and needs the comprehensive consideration. The second was the wind speed profile. The surface roughness and inlet wind speed were the least important factors.
- (5)
- This paper did not fully consider the coupling effect of various initial conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, J.; Li, J.W.; Wang, F.; Hong, G.; Xing, S. Research on wind field characteristics measured by Lidar in a U-shaped valley at a bridge site. Appl. Sci. 2021, 11, 9645. [Google Scholar] [CrossRef]
- Wang, F.; Chen, X.M.; He, R.; Liu, Y.; Hao, J.M.; Li, J.W. Wind characteristics in mountainous valleys obtained through field measurement. Appl. Sci. 2021, 11, 7717. [Google Scholar] [CrossRef]
- Song, J.-L.; Li, J.-W.; Flay, R.G.J. Field measurements and wind tunnel investigation of wind characteristics at a bridge site in a Y-shaped valley. J. Wind Eng. Ind. Aerodyn. 2020, 202, 104199. [Google Scholar] [CrossRef]
- Blocken, B.; van der Hout, A.; Dekker, J.; Weiler, O. CFD simulation of wind flow over natural complex terrain: Case study with validation by field measurements for Ria de Ferrol, Galicia, Spain. J. Wind Eng. Ind. Aerodyn. 2015, 147, 43–57. [Google Scholar] [CrossRef]
- Pirooz, A.A.S.; Flay, R.G.J. Comparison of speed-up over hills derived from wind-tunnel experiments, wind-loading standards, and numerical modelling. Bound.-Layer Meteorol. 2018, 168, 213–246. [Google Scholar] [CrossRef]
- Chung, J.; Bienkiewicz, B. Numerical simulation of flow past 2D hill and valley. Wind Struct. Int. J. 2004, 7, 1–13. [Google Scholar] [CrossRef]
- Braun, A.L.; Awruch, A.M. Finite element simulation of the wind action over bridge sectional models: Application to the Guama river bridge (Para State, Brazil). Finite Elem. Anal. Des. 2008, 44, 105–122. [Google Scholar] [CrossRef]
- Li, L.; Zhang, L.-J.; Zhang, N.; Hu, F.; Jiang, Y.; Xuan, C.-Y.; Jiang, W.-M. Study on the micro-scale simulation of wind field over complex terrain by RAMS/FLUENT modeling system. Wind Struct. 2010, 13, 519–528. [Google Scholar] [CrossRef]
- Yan, Q.; Peng, Y.; Li, J. Scheme and application of phase delay spectrum towards spatial stochastic wind fields. Wind Struct. 2013, 16, 433–455. [Google Scholar] [CrossRef]
- Wang, T.; Cao, S.; Ge, Y. Effects of inflow turbulence and slope on turbulent boundary layers over two-dimensional hills. Wind Struct. 2014, 19, 219–232. [Google Scholar] [CrossRef]
- Ren, H.; Laima, S.; Chen, W.-L.; Zhang, B.; Guo, A.; Li, H. Numerical simulation and prediction of spatial wind field under complex terrain. J. Wind Eng. Ind. Aerodyn. 2018, 180, 49–65. [Google Scholar] [CrossRef]
- Liu, Z.; He, C.; Liu, Z.; Lu, H. Dimension-reduction simulation of stochastic wind velocity fields by two continuous approaches. Wind Struct. 2019, 29, 389–403. [Google Scholar] [CrossRef]
- Lu, X.; Chen, Q.; Qian, C.; Chen, C. Terrain numerical simulation based on RANS/LES hybrid turbulence model. In Proceedings of the 10th International Conference on Environmental Science and Technology, Xiamen, China, 7–9 June 2019; pp. 1–8. [Google Scholar]
- Li, J.; Shen, Z.; Xing, S.; Gao, G. Buffeting response of a free-standing bridge pylon in a trumpet-shaped mountain pass. Wind Struct. 2020, 30, 85–97. [Google Scholar] [CrossRef]
- Wang, L.; Chen, X.; Chen, H. Research on wind barrier of canyon bridge-tunnel junction based on wind characteristics. Adv. Struct. Eng. 2021, 24, 870–883. [Google Scholar] [CrossRef]
- Huang, W.; Zhang, X. Wind field simulation over complex terrain under different inflow wind directions. Wind Struct. 2019, 28, 239–253. [Google Scholar] [CrossRef]
- Song, J.-L.; Li, J.-W.; Xu, R.-Z.; Flay, R.G. Field measurements and CFD simulations of wind characteristics at the Yellow River bridge site in a converging-channel terrain. Eng. Appl. Comput. Fluid Mech. 2022, 16, 58–72. [Google Scholar] [CrossRef]
- Bakioglu, G.; Atahan, A.O. Evaluating the Influencing Factors on Adoption of Self-driving Vehicles by Using Interval-Valued Pythagorean Fuzzy AHP. In Proceedings of the INFUS 2020: Intelligent and Fuzzy Techniques: Smart and Innovative Solutions, Istanbul, Turkey, 21–23 July 2020; Kahraman, C., Onar, S.C., Oztaysi, B., Sari, I.U., Cebi, S., Tolga, A.C., Eds.; Advances in Intelligent Systems and Computing. Springer: Cham, Switzerland, 2021; pp. 503–511. [Google Scholar]
- Chen, Z.-Y.; Dai, Z.-H. Application of group decision-making AHP of confidence index and cloud model for rock slope stability evaluation. Comput. Geosci. 2021, 155, 104836. [Google Scholar] [CrossRef]
- Ma, S.; Xu, X.; Chui, J.; Ni, J.; Ma, H.; Tian, H. A New Model to Evaluate Groundwater Vulnerability that Uses a Hybrid AHP-GIS Approach. J. Geol. Soc. India 2021, 97, 94–103. [Google Scholar] [CrossRef]
- Merhi, M.I. Evaluating the critical success factors of data intelligence implementation in the public sector using analytical hierarchy process. Technol. Forecast. Soc. Chang. 2021, 173, 121180. [Google Scholar] [CrossRef]
- Radhika, E.G.; Sadasivam, G.S. Budget optimized dynamic virtual machine provisioning in hybrid cloud using fuzzy analytic hierarchy process. Expert Syst. Appl. 2021, 183, 115398. [Google Scholar] [CrossRef]
- Zadehmohamad, M.; Bazaz, J.B.; Riahipour, R.; Farhangi, V. Physical modeling of the long-term behavior of integral abutment bridge backfill reinforced with tire-rubber. Int. J. Geo-Eng. 2021, 12, 36. [Google Scholar] [CrossRef]
- Blocken, B. Computational Fluid Dynamics for urban physics: Importance, scales, possibilities, limitations and ten tips and tricks towards accurate and reliable simulations. Build. Environ. 2015, 91, 219–245. [Google Scholar] [CrossRef] [Green Version]
- Huang, G.; Cheng, X.; Peng, L.; Li, M. Aerodynamic shape of transition curve for truncated mountainous terrain model in wind field simulation. J. Wind Eng. Ind. Aerodyn. 2018, 178, 80–90. [Google Scholar] [CrossRef]
- Bitsuamlak, G.T.; Stathopoulos, T.; Bédard, C. Numerical evaluation of wind flow over complex terrain: Review. J. Aerosp. Eng. 2004, 17, 135–145. [Google Scholar] [CrossRef]
- Hu, P.; Han, Y.; Xu, G.; Li, Y.; Xue, F. Numerical simulation of wind fields at the bridge site in mountain-gorge terrain considering an updated curved boundary transition section. J. Aerosp. Eng. 2018, 31, 04018008. [Google Scholar] [CrossRef]
- Hu, P.; Han, Y.; Xu, G.; Cai, C.S.; Cheng, W. Effects of Inhomogeneous Wind Fields on the Aerostatic Stability of a Long-Span Cable-Stayed Bridge Located in a Mountain-Gorge Terrain. J. Aerosp. Eng. 2020, 33, 04020006. [Google Scholar] [CrossRef]
- AANSYS. Ansys Fluent User Guide 2019R2; ANSYS. Inc.: Canonsburg, PA, USA, 2019. [Google Scholar]
- Pattanapol, W.; Wakes, S.J.; Hilton, M.J.; Dickinson, K.J.M. Modeling of Surface Roughness for Flow Over a Complex Vegetated Surface. In Proceedings of the Conference of the World-Academy-of-Science-Engineering-and-Technology, Bangkok, Thailand, 14–16 December 2007; pp. 273–281. [Google Scholar]
- Blocken, B.; Carmeliet, J.; Stathopoulos, T. CFD evaluation of the wind speed conditions in passages between buildings-effect of wall function roughness modifications on the athmospheric boundary layer. J. Wind Eng. Ind. Aerodyn. 2007, 95, 941–962. [Google Scholar] [CrossRef]
- Blocken, B.; Stathopoulos, T.; Carmeliet, J. CFD simulation of the atmospheric boundary layer: Wall function problems. Atmos. Environ. 2007, 41, 238–252. [Google Scholar] [CrossRef]
- Wieringa, J. Updating the Davenport roughness classification. J. Wind Eng. Ind. Aerodyn. 1992, 41, 357–368. [Google Scholar] [CrossRef]
- JTG/T3360-01-2018; Wind-Resistant Design Specification for Hignway Bridges. China Communications Press Co., Ltd.: Beijing, China, 2018.
- Zhang, J.; Zhang, M.; Li, Y.; Jiang, F.; Wu, L.; Guo, D. Comparison of wind characteristics in different directions of deep-cut gorges based on field measurements. J. Wind Eng. Ind. Aerodyn. 2021, 212, 104595. [Google Scholar] [CrossRef]





| Grid Precision | Coarse1 | Coarse2 | Middle1 | Middle2 | Fine1 | Fine2 |
|---|---|---|---|---|---|---|
| Grid quality | 2,909,100 | 5,380,410 | 8,050,575 | 11,494,320 | 13,202,820 | 16,131,445 |
| Maximum of the nondimensional velocity ratio | 1.20 | 1.06 | 1.06 | 1.00 | 1.02 | 1.01 |
| Scale Value | Description |
|---|---|
| 1 | Two factors are of equal importance. |
| 3 | When comparing two factors, one is slightly more important than the other. |
| 5 | When comparing two factors, one is obviously more important than the other. |
| 7 | When comparing two factors, one is strongly more important than the other. |
| 9 | When comparing two factors, one is greatly more important than the other. |
| 2,4,6,8 | Median of two adjacent judgments above. |
| Inverse | when comparing i to j, when comparing j to i. |
| n | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| RI | 0 | 0 | 0.52 | 0.89 | 1.12 | 1.26 | 1.36 | 1.41 | 1.46 | 1.49 | 1.52 | 1.54 | 1.56 | 1.58 |
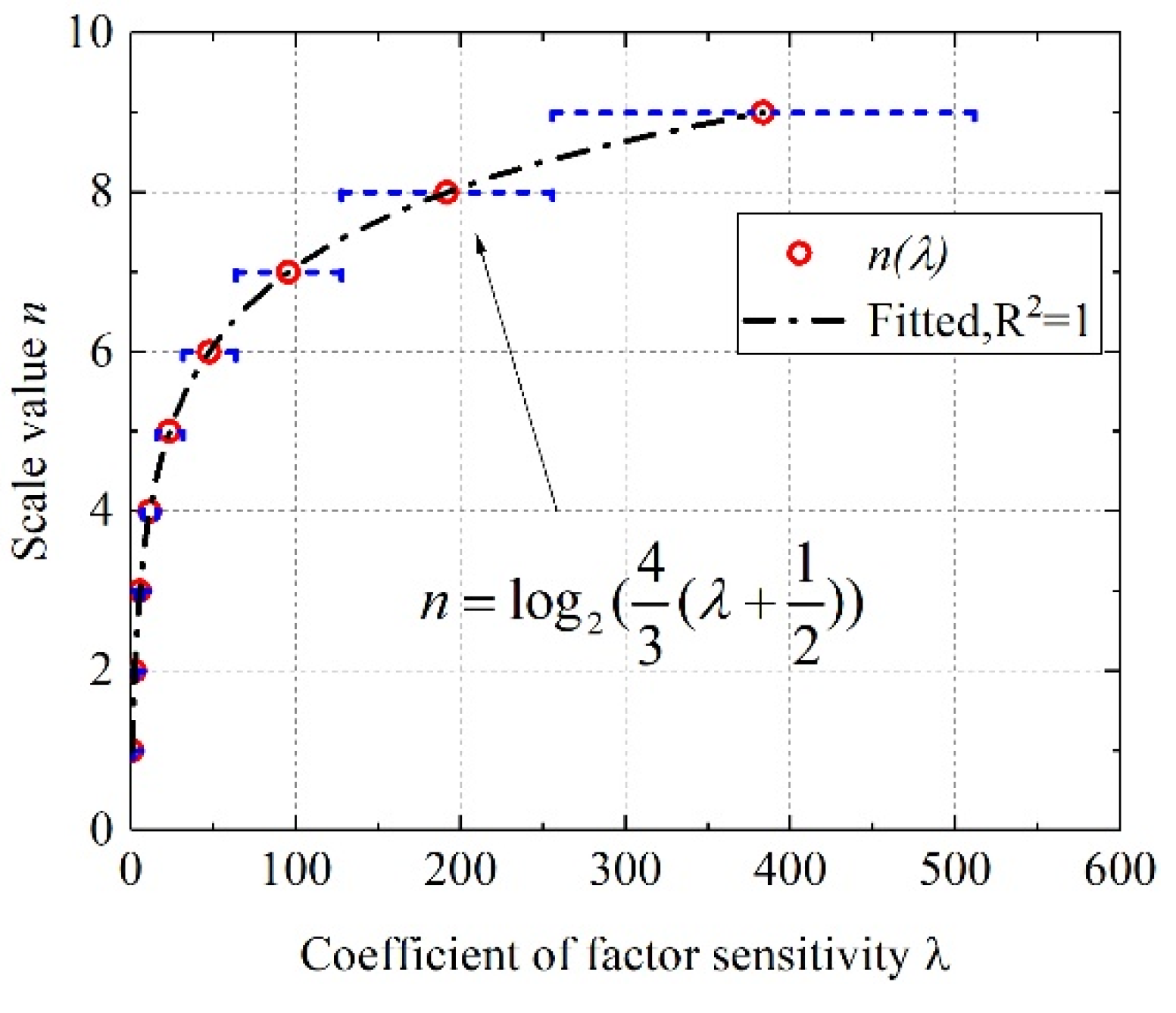
| Factor Sensitivity Coefficient | 0.5~1.5 | 1.5~3.5 | 3.5~7.5 | 7.5~15.5 | 15.5~31.5 | 31.5~63.5 | 63.5~127.5 | 127.5~255.5 | 255.5~ |
|---|---|---|---|---|---|---|---|---|---|
| Scale value | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
| (a) Judgment matrix of the initial conditions effect on the wind speed | ||||||||
| Wind speed | Surface roughness | Inlet wind speed | Wind speed profile | Oncoming wind direction | ||||
| Surface roughness | 1 | 2 | 2/5 | 2/5 | 1.00 | 1.05 | 0.05 | 1.52 |
| Inlet wind speed | 1/2 | 1 | 1/5 | 1/5 | 0.97 | 1.00 | 0.03 | 1.00 |
| Wind speed profile | 5/2 | 5 | 1 | 1 | 0.46 | 1.01 | 0.55 | 17.74 |
| Oncoming wind direction | 5/2 | 5 | 1 | 1 | 0.37 | 1.32 | 0.95 | 30.61 |
| (b) Judgment matrix of the initial conditions effect on the wind attack angle | ||||||||
| Wind attack angle | Surface roughness | Inlet wind speed | Wind speed profile | Oncoming wind direction | ||||
| Surface roughness | 1 | 2 | 2 | 1/3 | 0.69 | 2.46 | 1.78 | 3.24 |
| Inlet wind speed | 1/2 | 1 | 1 | 1/6 | 0.56 | 1.17 | 0.61 | 1.11 |
| Wind speed profile | 1/2 | 1 | 1 | 1/6 | 0.80 | 1.35 | 0.55 | 1.00 |
| Oncoming wind direction | 3 | 6 | 6 | 1 | −7.28 | 10.15 | 17.43 | 31.80 |
| (c) Judgment matrix of the initial conditions effect on the wind azimuth angle | ||||||||
| Wind azimuth angle | Surface roughness | Inlet wind speed | Wind speed profile | Oncoming wind direction | ||||
| Surface roughness | 1 | 1 | 1/3 | 1/5 | 0.93 | 2.38 | 1.45 | 1.00 |
| Inlet wind speed | 1 | 1 | 1/3 | 1/5 | 0.96 | 2.48 | 1.52 | 1.05 |
| Wind speed profile | 3 | 3 | 1 | 3/5 | 0.38 | 6.51 | 6.12 | 4.22 |
| Oncoming wind direction | 5 | 5 | 5/3 | 1 | −15.60 | 24.51 | 40.11 | 27.64 |
| Weight | ||||
|---|---|---|---|---|
| Surface Roughness | Inlet Wind Speed | Wind Speed Profile | Oncoming Wind Direction | |
| Wind speed | 0.132 | 0.094 | 0.387 | 0.387 |
| Wind attack angle | 0.203 | 0.107 | 0.083 | 0.608 |
| Wind azimuth angle | 0.1 | 0.1 | 0.3 | 0.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Wang, J.; Zhao, X.; Wang, F.; Li, Y. Influence of Initial Conditions on Wind Characteristics at a Bridge Middle Span in a U-Shaped Valley by CFD and AHP. Appl. Sci. 2022, 12, 4693. https://doi.org/10.3390/app12094693
Li J, Wang J, Zhao X, Wang F, Li Y. Influence of Initial Conditions on Wind Characteristics at a Bridge Middle Span in a U-Shaped Valley by CFD and AHP. Applied Sciences. 2022; 12(9):4693. https://doi.org/10.3390/app12094693
Chicago/Turabian StyleLi, Jiawu, Jun Wang, Xue Zhao, Feng Wang, and Yu Li. 2022. "Influence of Initial Conditions on Wind Characteristics at a Bridge Middle Span in a U-Shaped Valley by CFD and AHP" Applied Sciences 12, no. 9: 4693. https://doi.org/10.3390/app12094693
APA StyleLi, J., Wang, J., Zhao, X., Wang, F., & Li, Y. (2022). Influence of Initial Conditions on Wind Characteristics at a Bridge Middle Span in a U-Shaped Valley by CFD and AHP. Applied Sciences, 12(9), 4693. https://doi.org/10.3390/app12094693






