Random Vibration Fatigue Analysis Using a Nonlinear Cumulative Damage Model
Abstract
:Featured Application
Abstract
1. Introduction
2. Theorical Background
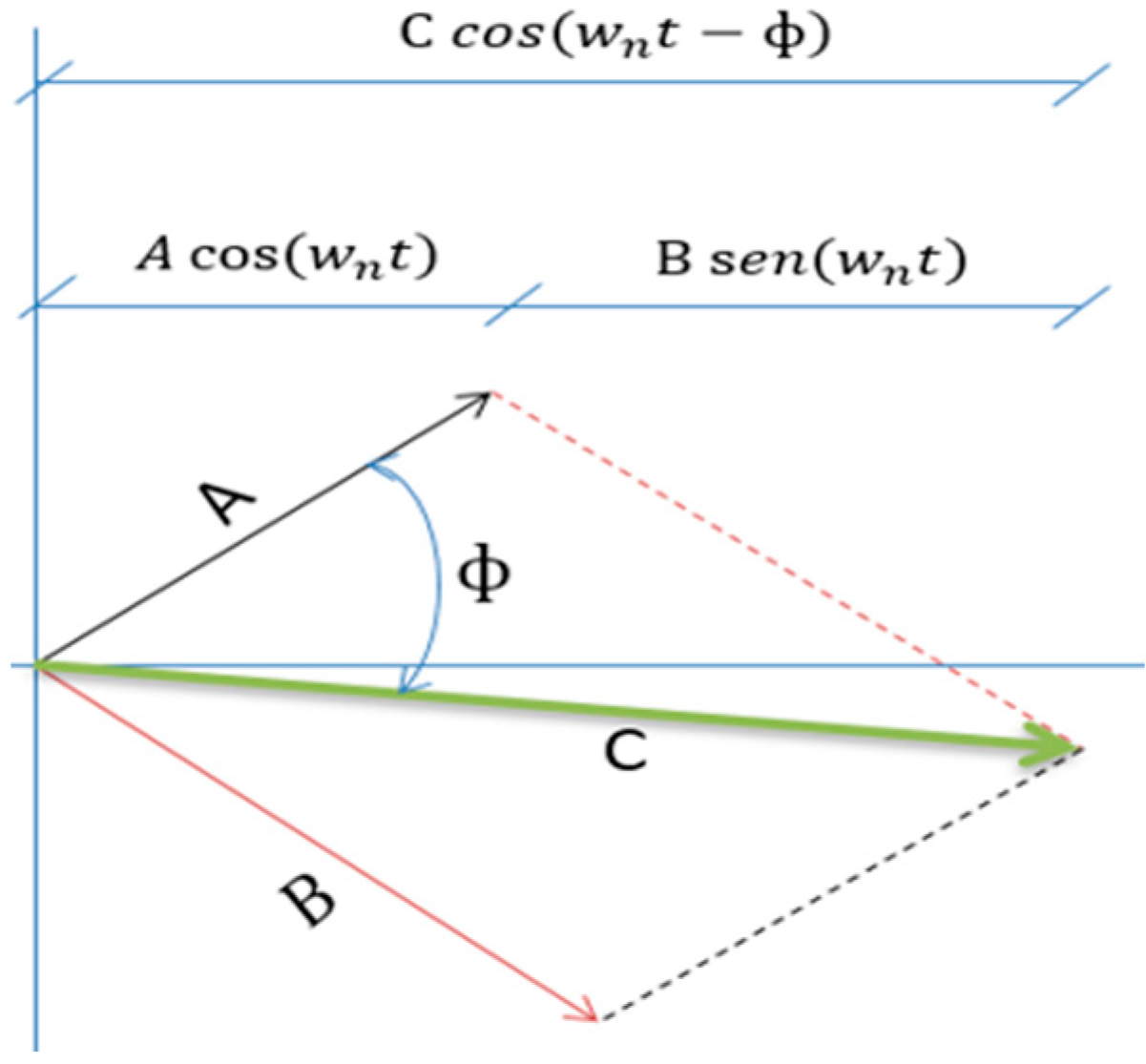
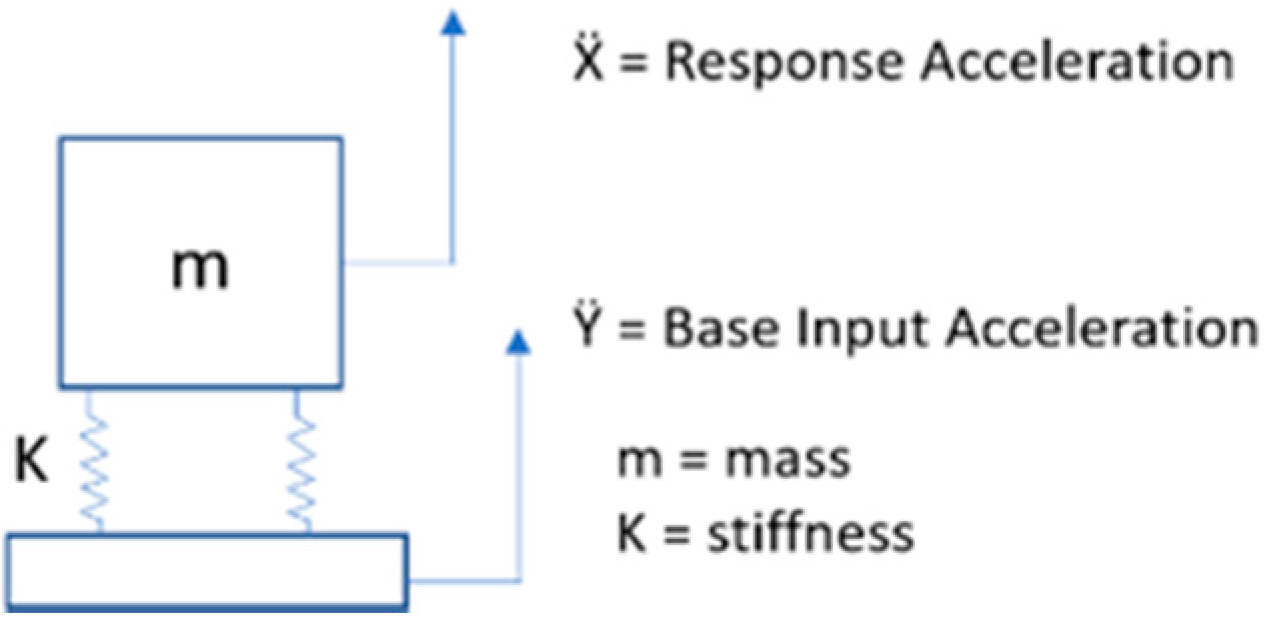
2.1. Random Vibration
2.2. Nonlinear Fatigue Damage Accumulation
3. Modified Nonlinear Fatigue Damage Accumulation Model Considering the Vibration Load Effect
3.1. Calculating the Bending Stress
3.2. Vibration Cycle Counting
3.3. Total Cycle Determination
4. Fatigue Damage Accumulation Procedure
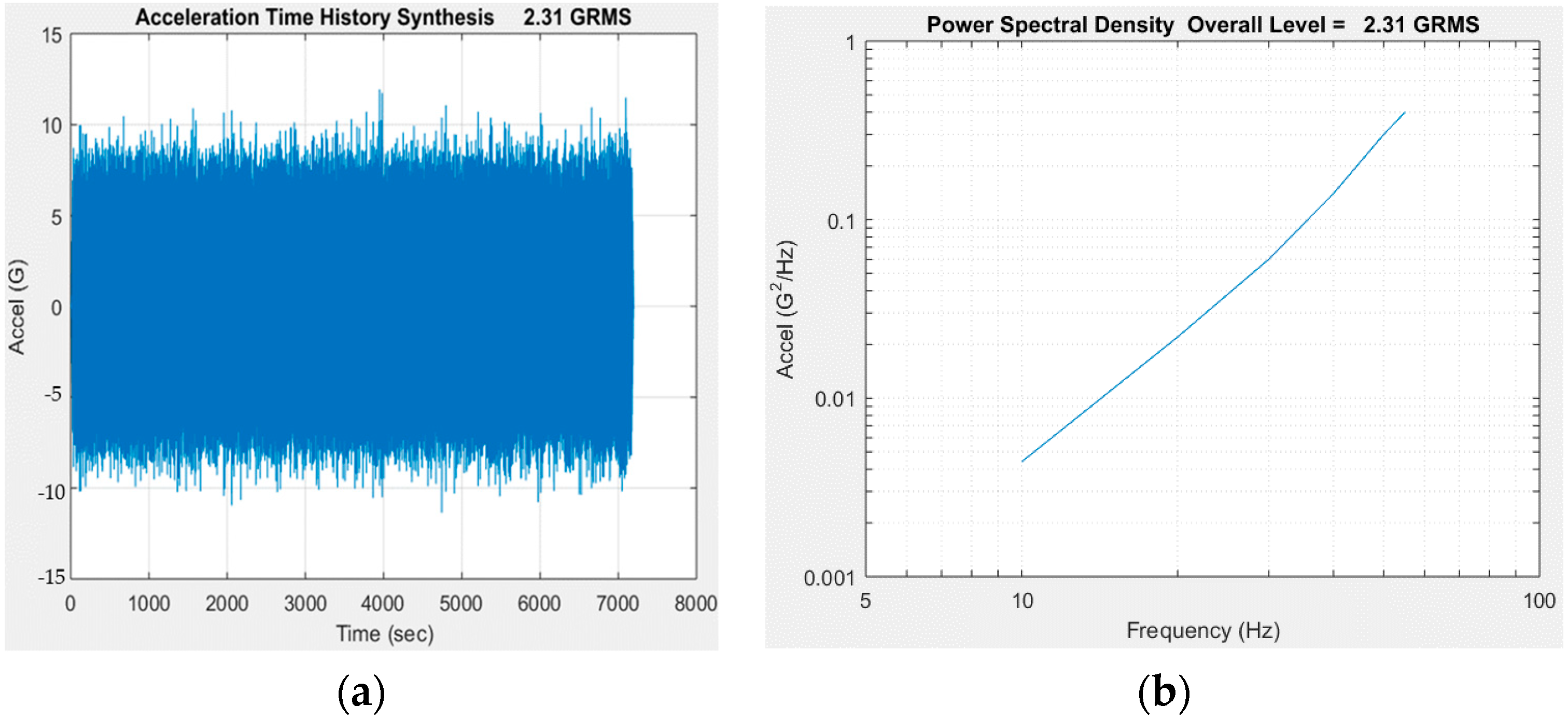
4.1. Study Case
4.2. Bending Stress
4.3. Vibration Cycles Counting
4.4. Total Cycles Determination
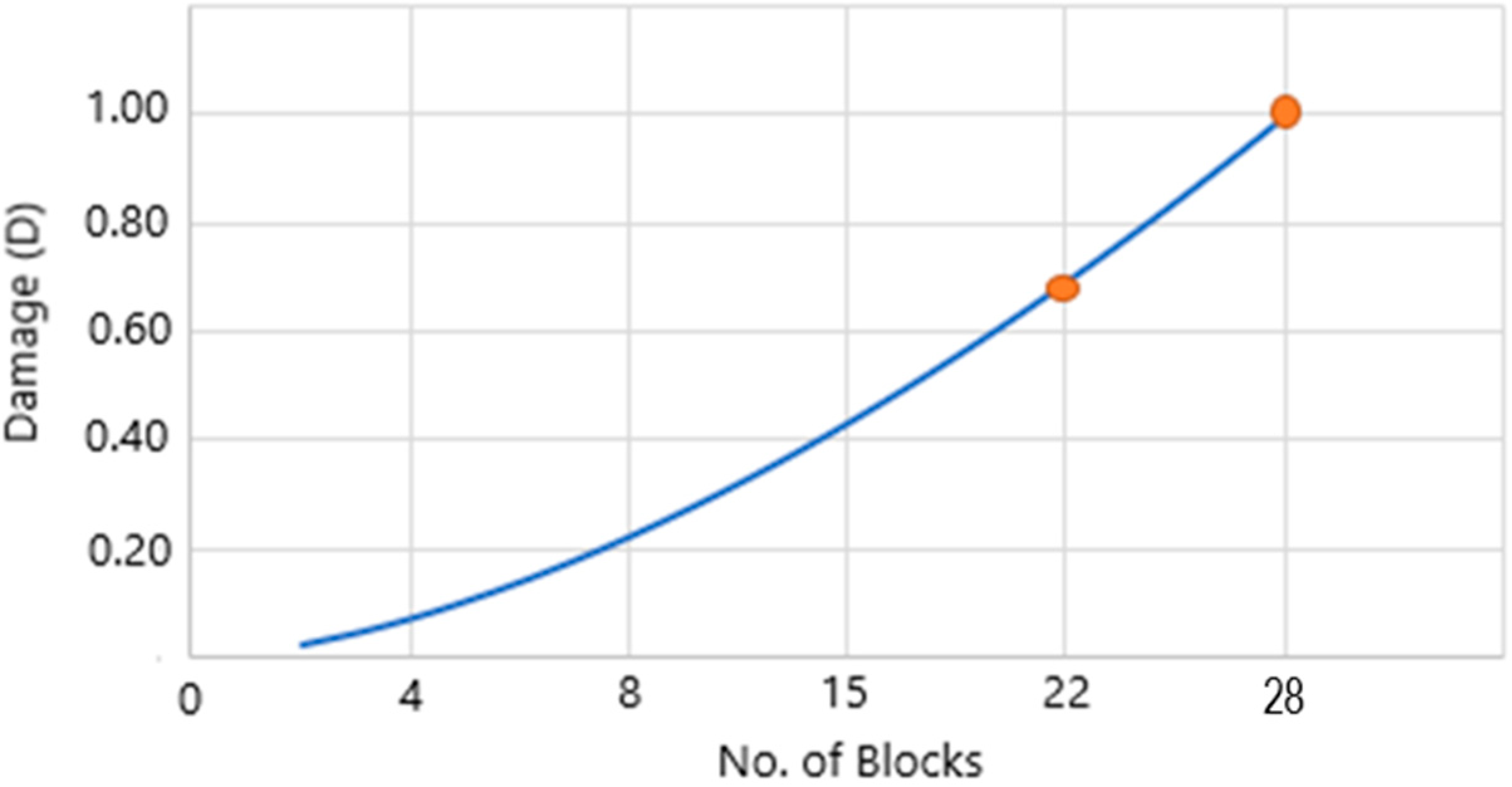
4.5. Fatigue Damage Accumulation
5. Conclusions
- (1)
- Since the inputs for the nonlinear model are the response acceleration’s values, and because, by using the dynamic factor , the vibration bending stresses’ values can be determined, then the proposed model can be applied in any mechanical component analysis submitted to random vibration forces where values are known.
- (2)
- Since the dynamic factor allows us to obtain stress units from acceleration units, then the vibration bending stress values can be used in the proposed model Equation (14) to calculate the fatigue damage accumulation induced by the random vibration.
- (3)
- The constant exponent parameter value of 0.4 in the Manson–Halford model has been replaced by a vibration bending stress relation that considers the effect sequence and interaction loads induced by the applied PSD.
- (4)
- As a result of the mechanical component case study, the model proposed to predict the fatigue damage accumulation shows good agreement with the reported experimental data. The model predicts 28 cycles of load to reach the failure (D = 1), and the experiment data collected from the vibration shaker showing, after 28 cycles of load, the deformation on the component’s material can be considered as a failure.
- (5)
- The efficiency of the model proposed offers the advantage that even though random vibration (PSD) provides a complex loading history, based on the corresponding PSD, it is possible to obtain the fatigue damage accumulation through general analysis.
- (6)
- Since the proposed method is based on the bending stress induced by random vibration PSD function and its response acceleration, then, knowing that the fatigue damage accumulation can also be analyzed from a strain and crack growth propagation point of view, it seems that the proposed model could also be used in those cases, but more research must be undertaken.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Piña-Monarrez, M.R. Weibull analysis for normal/accelerated and fatigue random vibration test. Qual. Reliab. Eng. Int. 2019, 35, 2408–2428. [Google Scholar] [CrossRef]
- Harris, C.M.; Piersol, A.G. Shock and Vibration Handbook, 5th ed.; McGraw Hill: New York, NY, USA, 2002; Volume 15. [Google Scholar] [CrossRef]
- Knitter-Piątkowska, A.; Dobrzycki, A. Application of wavelet transform to damage identification in the steel structure elements. Appl. Sci. 2020, 10, 8198. [Google Scholar] [CrossRef]
- Kavitha, M.; Mahmoud, Z.H.; Kishore, K.H.; Petrov, A.M.; Lekomtsev, A.; Iliushin, P.; Zekiy, A.O.; Salmani, M. Application of steinberg model for vibration lifetime evaluation of Sn-Ag-Cu-based solder joints in power semiconductors. IEEE Trans. Compon. Packag. Manuf. Technol. 2021, 11, 444–450. [Google Scholar] [CrossRef]
- Angeli, A.; Cornelis, B.; Troncossi, M. Synthesis of Sine-on-Random vibration profiles for accelerated life tests based on fatigue damage spectrum equivalence. Mech. Syst. Signal Process. 2018, 103, 340–351. [Google Scholar] [CrossRef]
- Lee, Y.-L.; Barkey, M.E.; Kang, H.T. Metal Fatigue Analysis Handbook: Practical Problem-Solving Techniques for Computer-Aided Engineering; Elsevier Butterworth-Heinemann: Burlington, MA, USA, 2012. [Google Scholar]
- Quigley, J.P.; Lee, Y.L.; Wang, L. Review and Assessment of Frequency-Based Fatigue Damage Models. SAE Int. J. Mater. Manuf. 2016, 9. [Google Scholar] [CrossRef]
- Santecchia, E.; Hamouda, A.M.S.; Musharavati, F.; Zalnezhad, E.; Cabibbo, M.; El Mehtedi, M.; Spigarelli, S. A Review on Fatigue Life Prediction Methods for Metals. Adv. Mater. Sci. Eng. 2016, 2016, 9573524. [Google Scholar] [CrossRef] [Green Version]
- Böhm, E.; Kurek, M.; Łagoda, T.; Łagoda, K. The use of a power law function for fatigue life estimation for block loads. Solid State Phenom. 2016, 250, 1–9. [Google Scholar] [CrossRef]
- Lee, Y.-L.; Pan, J.; Hathaway, R.B.; Barkey, M.E. Fatigue Testing and Analysis: Theory and Practice; Elsevier Butterworth-Heinemann: Burlington, MA, USA, 2005. [Google Scholar]
- Castillo, E.; Fernández-Canteli, A. A Unified Statistical Methodology for Modeling Fatigue Damage; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Molina, A.; Piña-Monarrez, M.R.; Barraza-Contreras, J.M. Weibull S-N fatigue strength curve analysis for A572 Gr. 50 steel, based on the true stress-true strain approach. Appl. Sci. 2020, 10, 5725. [Google Scholar] [CrossRef]
- Osgood, C.C. Analysis of random responses for calculation of fatigue damage. Shock Vib. Bull. 1969, 40, 1–8. [Google Scholar] [CrossRef]
- Wirsching, P.H. Fatigue reliability in welded joints of offshore structures. Int. J. Fatigue. 1980, 2, 77–83. [Google Scholar] [CrossRef]
- Rege, K.; Pavlou, D.G. A one-parameter nonlinear fatigue damage accumulation model. Int. J. Fatigue 2017, 98, 234–246. [Google Scholar] [CrossRef]
- da Silva, L.C.M.; Milani, G. A FE-Based Macro-Element for the Assessment of Masonry Structures: Linear Static, Vibration, and Non-Linear Cyclic Analyses. Appl. Sci. 2022, 12, 1248. [Google Scholar] [CrossRef]
- Manson, S.S.; Halford, G.R. Practical implementation of the double linear damage rule and damage curve approach for treating cumulative fatigue damage. Int. J. Fract. 1981, 17, 169–192. [Google Scholar] [CrossRef] [Green Version]
- Edson, L. The GMW3172 Users Guide; The Electrical Validation Engineers Handbook Series; Electrical Component Testing; 2008; Available online: https://ab-div-bdi-bl-blm.web.cern.ch/ab-div-bdi-bl-blm/RAMS/Handbook_testing.pdf (accessed on 28 January 2022).
- Lalanne, C. Fatigue Damage, 3rd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2014; Volume 4. [Google Scholar]
- Richart, F.E.; Newmark, N.M. A hypothesis for the determination of cumulative damage in fatigue. Proc. Am. Soc. Test. Mater. 1948, 48, 767–800. [Google Scholar]
- Marco, S.M.; Starkey, W.L. A concept of fatigue damage. Trans. ASME 1954, 76, 627–632. [Google Scholar]
- Lv, Z.; Huang, H.Z.; Zhu, S.P.; Gao, H.; Zuo, F. A modified nonlinear fatigue damage accumulation model. Int. J. Damage Mech. 2014, 24, 168–181. [Google Scholar] [CrossRef]
- Gao, H.; Huang, H.-Z.; Zhu, S.-P.; Li, Y.-F.; Yuan, R. A Modified Nonlinear Damage Accumulation Model for Fatigue Life Prediction Considering Load Interaction Effects. Sci. World J. 2014, 2014, 164378. [Google Scholar] [CrossRef] [Green Version]
- Yuan, R.; Li, H.; Huang, H.Z.; Zhu, S.P.; Li, Y.F. A new non-linear continuum damage mechanics model for fatigue life prediction under variable loading. Mechanika 2013, 19, 506–511. [Google Scholar] [CrossRef] [Green Version]
- Steinberg, D.S. Vibration Analysis for Electronic Equipment, 3rd ed.; John Wiley & Sons: New York, NY, USA, 2000. [Google Scholar]
- Manson, S.S.; Halford, G.R. Re-examination of cumulative fatigue damage analysis-an engineering perspective. Eng. Fract. Mech. 1986, 25, 539–571. [Google Scholar] [CrossRef] [Green Version]
- Zalnezhad, E.; Sarhan, A.A.D.M.; Hamdi, M. Surface hardness prediction of CrN thin film coating on AL7075-T6 alloy using fuzzy logic system. Int. J. Precis. Eng. Manuf. 2013, 14, 467–473. [Google Scholar] [CrossRef] [Green Version]
- Weibull, W. A Statistical Theory of the Strength of Materials; Generalstabens Litografiska Anstalts Förlag: Stockholm, Sweden, 1939. [Google Scholar]
- Irvine, T. A Fatigue Damage Spectrum Method for Comparing Power Spectral Density Base Input Specifications. Vibrationdata, 2014. Available online: https://vibrationdata.wordpress.com/(accessed on 28 January 2022).
- Auersch, L. Different Types of Continuous Track Irregularities as Sources of Train-Induced Ground Vibration and the Importance of the Random Variation of the Track Support. Appl. Sci. 2022, 12, 1463. [Google Scholar] [CrossRef]
- Designation: E1049-85; Standard Practices for Cycle Counting in Fatigue Analysis. ASTM International: West Conshohocken, PN, USA, 2017.
- Lalanne, C. Random Vibration, 3rd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2014; Volume 3. [Google Scholar]




| Rainflow Cycles | ||
|---|---|---|
| Path | Cycles | Stress Span |
| A–B | 0.5 | 2 |
| B–C | 0.5 | 3 |
| C–D | 0.5 | 5 |
| E–F | 1 | 2 |
| Frequency (Hz) | Accel. (G) | Accel. (G2/Hz) |
|---|---|---|
| 10 | 0.210 | 0.004 |
| 20 | 0.666 | 0.022 |
| 30 | 1.383 | 0.063 |
| 40 | 2.359 | 0.139 |
| 50 | 3.900 | 0.300 |
| 55 | 4.664 | 0.400 |
| Frequency (Hz) | Accel. Base Input (G) | Accel. Response (G) |
|---|---|---|
| 10 | 0.21 | 0.69 |
| 20 | 0.67 | 3.06 |
| 30 | 1.38 | 5.58 |
| 40 | 2.36 | 9.09 |
| 50 | 3.90 | 13.68 |
| 55 | 4.66 | 12.31 |
| Frequency (Hz) | Accel. Response (G) | Dynamic Factor Equation (22) | Bending Stress Equation (24) |
|---|---|---|---|
| 10 | 0.69 | 22.22 | 15.33 |
| 20 | 3.06 | 67.97 | |
| 30 | 5.58 | 123.95 | |
| 40 | 9.09 | 201.91 | |
| 50 | 13.68 | 303.87 | |
| 55 | 12.31 | 273.44 |
| Frequency (Hz) | |
|---|---|
| 10 | 70,292 |
| 20 | 140,210 |
| 30 | 92,650 |
| 40 | 10,869 |
| 50 | 3010 |
| 55 | 816 |
| Frequency (Hz) | Bending Stress Equation (24) | Total Cycle Equation (29) |
|---|---|---|
| 10 | 15.33 | 2.24 × 1020 |
| 20 | 67.97 | 5.60 × 1012 |
| 30 | 123.95 | 4.81 × 109 |
| 40 | 201.91 | 1.55 × 107 |
| 50 | 303.87 | 1.27 × 105 |
| 55 | 273.44 | 4.40 × 105 |
| 10 Hz | 20 Hz | 30 Hz | 40 Hz | 50 Hz | 55 Hz | |
|---|---|---|---|---|---|---|
| Block No. | D1 | D1+2 | D1+2+3 | D1+2+3+4 | D1+2+3+4+5 | D1+2+3+4+5+6 |
| 1 (2 h) | 3.14 × 10−16 | 3.14 × 10−16 | 3.16 × 10−16 | 3.44 × 10−16 | 2.36 × 10−2 | 2.41 × 10−2 |
| 2 (2 h) | 2.41 × 10−2 | 2.41 × 10−2 | 2.42 × 10−2 | 2.46 × 10−2 | 4.83 × 10−2 | 4.90 × 10−2 |
| 3 (2 h) | 4.90 × 10−2 | 4.90 × 10−2 | 4.93 × 10−2 | 5.01 × 10−2 | 7.37 × 10−2 | 7.48 × 10−2 |
| 4 (2 h) | 7.48 × 10−2 | 7.48 × 10−2 | 7.53 × 10−2 | 7.64 × 10−2 | 1.00 × 10−1 | 1.01 × 10−1 |
| 5 (2 h) | 1.01 × 10−1 | 1.01 × 10−1 | 1.02 × 10−1 | 1.04 × 10−1 | 1.27 × 10−1 | 1.29 × 10−1 |
| 6 (2 h) | 1.29 × 10−1 | 1.29 × 10−1 | 1.30 × 10−1 | 1.32 × 10−1 | 1.55 × 10−1 | 1.57 × 10−1 |
| 7 (2 h) | 1.57 × 10−1 | 1.57 × 10−1 | 1.58 × 10−1 | 1.60 × 10−1 | 1.84 × 10−1 | 1.86 × 10−1 |
| 8 (2 h) | 1.86 × 10−1 | 1.86 × 10−1 | 1.87 × 10−1 | 1.90 × 10−1 | 2.14 × 10−1 | 2.16 × 10−1 |
| 9 (2 h) | 2.16 × 10−1 | 2.16 × 10−1 | 2.17 × 10−1 | 2.20 × 10−1 | 2.44 × 10−1 | 2.47 × 10−1 |
| 10 (2 h) | 2.47 × 10−1 | 2.47 × 10−1 | 2.48 × 10−1 | 2.52 × 10−1 | 2.75 × 10−1 | 2.78 × 10−1 |
| 11 (2 h) | 2.78 × 10−1 | 2.78 × 10−1 | 2.80 × 10−1 | 2.84 × 10−1 | 3.08 × 10−1 | 3.11 × 10−1 |
| 12 (2 h) | 3.11 × 10−1 | 3.11 × 10−1 | 3.13 × 10−1 | 3.17 × 10−1 | 3.41 × 10−1 | 3.44 × 10−1 |
| 13 (2 h) | 3.44 × 10−1 | 3.44 × 10−1 | 3.46 × 10−1 | 3.51 × 10−1 | 3.75 × 10−1 | 3.78 × 10−1 |
| 14 (2 h) | 3.78 × 10−1 | 3.78 × 10−1 | 3.81 × 10−1 | 3.86 × 10−1 | 4.10 × 10−1 | 4.13 × 10−1 |
| 15 (2 h) | 4.13 × 10−1 | 4.13 × 10−1 | 4.16 × 10−1 | 4.22 × 10−1 | 4.45 × 10−1 | 4.49 × 10−1 |
| 16 (2 h) | 4.49 × 10−1 | 4.49 × 10−1 | 4.52 × 10−1 | 4.59 × 10−1 | 4.82 × 10−1 | 4.87 × 10−1 |
| 17 (2 h) | 4.87 × 10−1 | 4.87 × 10−1 | 4.90 × 10−1 | 4.96 × 10−1 | 5.20 × 10−1 | 5.25 × 10−1 |
| 18 (2 h) | 5.25 × 10−1 | 5.25 × 10−1 | 5.28 × 10−1 | 5.35 × 10−1 | 5.59 × 10−1 | 5.25 × 10−1 |
| 19 (2 h) | 5.25 × 10−1 | 5.25 × 10−1 | 5.67 × 10−1 | 5.75 × 10−1 | 5.99 × 10−1 | 6.04 × 10−1 |
| 20 (2 h) | 6.04 × 10−1 | 6.04 × 10−1 | 6.07 × 10−1 | 6.16 × 10−1 | 6.39 × 10−1 | 6.45 × 10−1 |
| 21 (2 h) | 6.45 × 10−1 | 6.45 × 10−1 | 6.49 × 10−1 | 6.58 × 10−1 | 6.81 × 10−1 | 6.87 × 10−1 |
| 22 (2 h) | 6.87 × 10−1 | 6.87 × 10−1 | 6.91 × 10−1 | 7.01 × 10−1 | 7.24 × 10−1 | 7.30 × 10−1 |
| 23 (2 h) | 7.30 × 10−1 | 7.30 × 10−1 | 7.35 × 10−1 | 7.45 × 10−1 | 7.68 × 10−1 | 7.74 × 10−1 |
| 24 (2 h) | 7.74 × 10−1 | 7.74 × 10−1 | 7.79 × 10−1 | 7.90 × 10−1 | 8.13 × 10−1 | 8.20 × 10−1 |
| 25 (2 h) | 8.20 × 10−1 | 8.20 × 10−1 | 8.25 × 10−1 | 8.36 × 10−1 | 8.60 × 10−1 | 8.66 × 10−1 |
| 26 (2 h) | 8.66 × 10−1 | 8.66 × 10−1 | 8.72 × 10−1 | 8.83 × 10−1 | 9.07 × 10−1 | 9.14 × 10−1 |
| 27 (2 h) | 9.14 × 10−1 | 9.14 × 10−1 | 9.20 × 10−1 | 9.32 × 10−1 | 9.56 × 10−1 | 9.63 × 10−1 |
| 28 (2 h) | 9.63 × 10−1 | 9.63 × 10−1 | 9.69 × 10−1 | 9.82 × 10−1 | 1.01 × 100 | 1.01 × 100 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barraza-Contreras, J.M.; Piña-Monarrez, M.R.; Molina, A.; Torres-Villaseñor, R.C. Random Vibration Fatigue Analysis Using a Nonlinear Cumulative Damage Model. Appl. Sci. 2022, 12, 4310. https://doi.org/10.3390/app12094310
Barraza-Contreras JM, Piña-Monarrez MR, Molina A, Torres-Villaseñor RC. Random Vibration Fatigue Analysis Using a Nonlinear Cumulative Damage Model. Applied Sciences. 2022; 12(9):4310. https://doi.org/10.3390/app12094310
Chicago/Turabian StyleBarraza-Contreras, Jesús M., Manuel R. Piña-Monarrez, Alejandro Molina, and Roberto C. Torres-Villaseñor. 2022. "Random Vibration Fatigue Analysis Using a Nonlinear Cumulative Damage Model" Applied Sciences 12, no. 9: 4310. https://doi.org/10.3390/app12094310
APA StyleBarraza-Contreras, J. M., Piña-Monarrez, M. R., Molina, A., & Torres-Villaseñor, R. C. (2022). Random Vibration Fatigue Analysis Using a Nonlinear Cumulative Damage Model. Applied Sciences, 12(9), 4310. https://doi.org/10.3390/app12094310







