L2-Norm Based a Posteriori Error Estimates of Compressible and Nearly-Incompressible Elastic Finite Element Solutions
Abstract
:1. Introduction
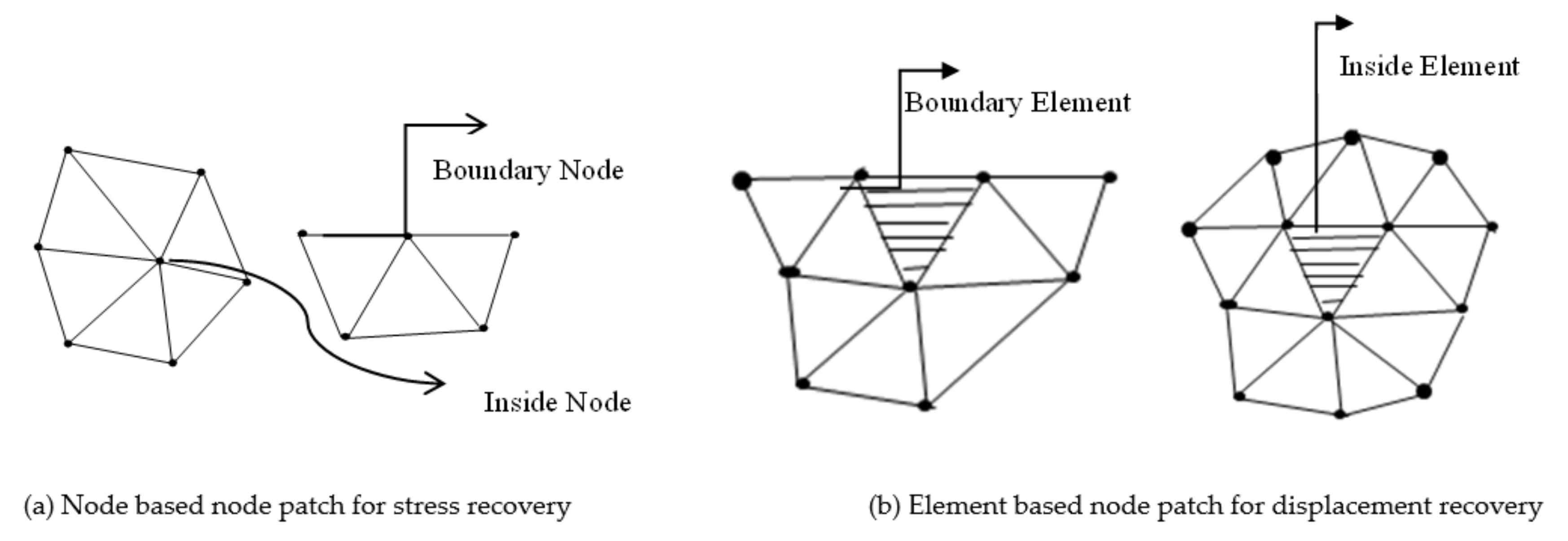
2. Least Squares Interpolation-Based Zienkiewicz–Zhu (ZZ) Stress Recovery Technique
3. Least Squares Interpolation-Based Displacement Recovery Technique
4. L2-Norm-Based Error Quantification and Mesh Improvement
5. Benchmark Example Applications
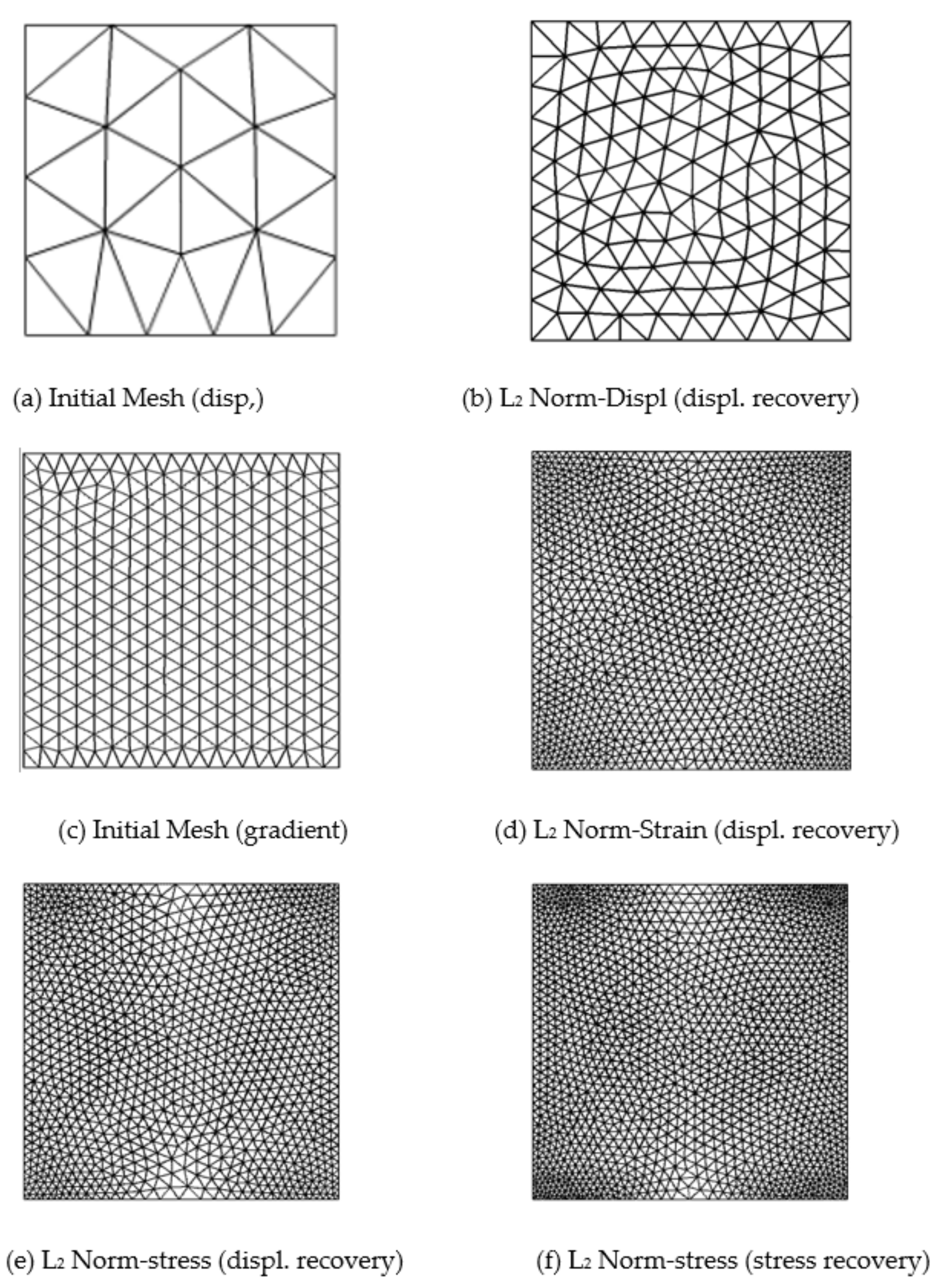
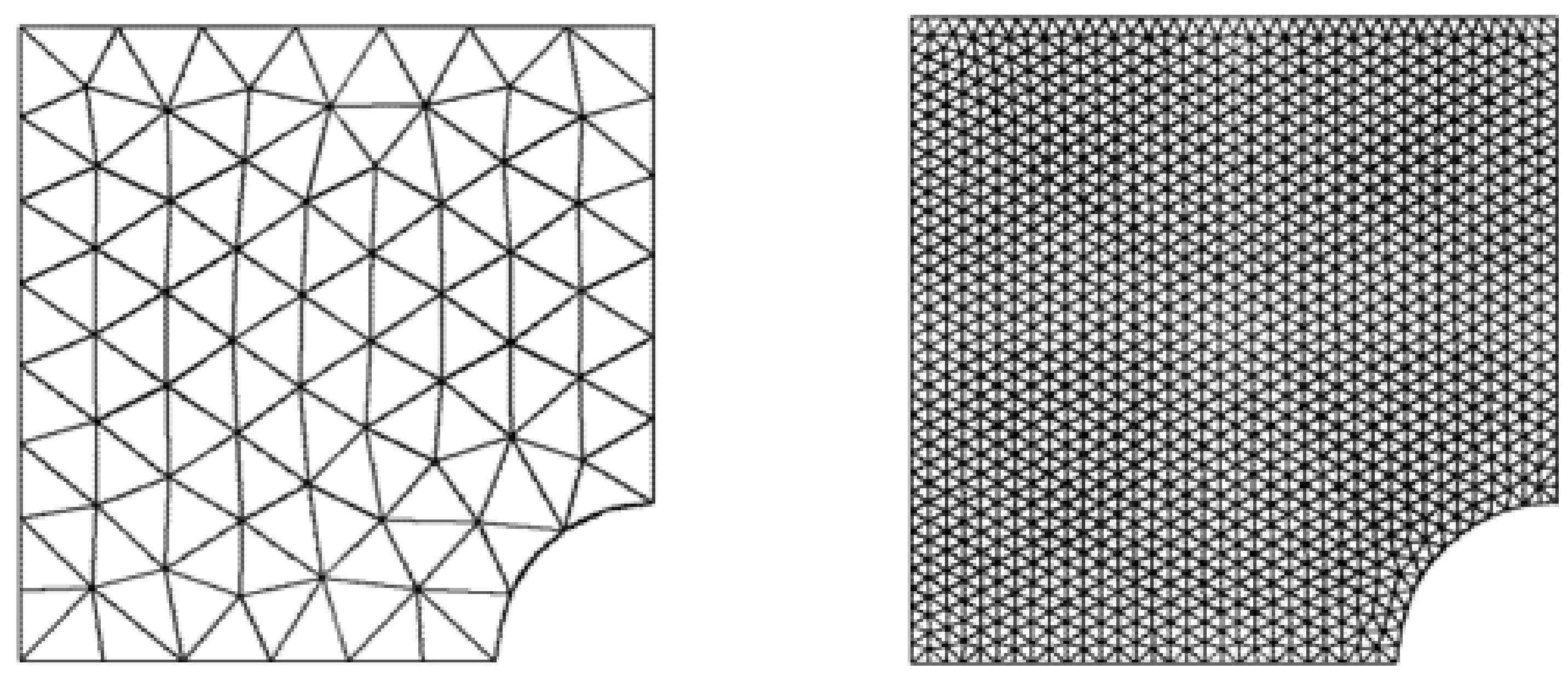
5.1. Application to Compressible Elastic Plate
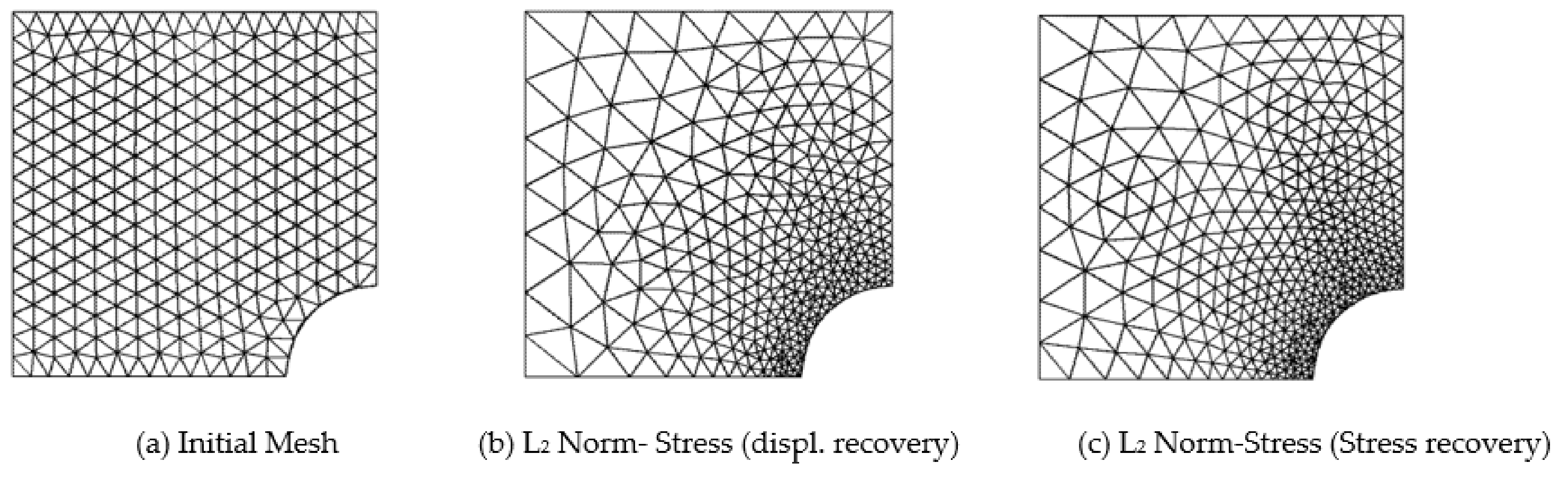
5.2. Application to Compressible Elastic Plate with Rigid Circular Inclusion
5.3. Application to Incompressible Elastic Plate
6. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Taus, M.; Rodin, G.J.; Hughes, T.R.; Scott, M.A. Isogeometric boundary element methods and patch tests for linear elastic problems: Formulation, numerical integration, and applications. Comput. Methods Appl. Mech. Eng. 2019, 357, 112591. [Google Scholar] [CrossRef]
- Cen, S.; Wu, C.J.; Li, Z.; Shang, Y.; Li, C. Some advances in high-performance finite element methods. Eng. Comput. 2019, 36, 2811–2834. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C. Achievement and Some Unsolved Problems of Finite Element Method. Int. J. Numer. Meth. Eng. 2000, 47, 9–28. [Google Scholar] [CrossRef]
- Gratsch, T.; Bathe, K. A posteriori error estimation technique in practical finite element analysis. Comput. Struct. 2005, 83, 75–90. [Google Scholar] [CrossRef] [Green Version]
- Zienkiewicz, O.C.; Zhu, J.Z. Simple error estimator and adaptive procedure for practical engineering analysis. Int. J. Numer. Meth. Eng. 1987, 24, 337–357. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Zhu, J.Z. The Super-convergent patch recovery and a posteriori error estimates, Part I, The Error Recovery technique. Int. J. Numer. Meth. Eng. 1992, 33, 1331–1364. [Google Scholar] [CrossRef]
- Wiberg, N.E.; Abdulwahab, F.; Ziukas, S. Enhanced superconvergent patch recovery incorporating equilibrium and boundary conditions. Int. J. Numer. Meth. Eng. 1994, 37, 3417–3440. [Google Scholar] [CrossRef]
- Boroomand, B.; Zienkiewicz, O.C. Recovery by equilibrium in patches (REP). Int. J. Numer. Meth. Eng. 1997, 40, 137–164. [Google Scholar] [CrossRef]
- Gu, H.; Zong, Z.; Hung, K.C. A modified super-convergent patch recovery method and its application to large deformation problems. Finite Elem. Anal. Des. 2004, 40, 665–687. [Google Scholar] [CrossRef]
- Rodenas, J.J.; Tur, M.; Fuenmayor, F.J.; Vercher, A. Improvement of the super-convergent patch recovery technique by the use of constraint equations: The SPR-C technique. Int. J. Numer. Meth. Eng. 2007, 70, 705–727. [Google Scholar] [CrossRef]
- Kim, K.; Lee, H. A posteriori Error Estimator for non-conforming finite element methods of the linear elasticity problem. J. Comput. Appl. Math. 2010, 235, 186–202. [Google Scholar] [CrossRef] [Green Version]
- Mohite, P.M.; Upadhyay, C.S. Adaptive finite element based shape optimization in laminated composite plates. Comput. Struct. 2015, 153, 19–35. [Google Scholar] [CrossRef]
- Nadal, E.; Dıez, P.; Ródenas, J.J.; Tur, M.; Fuenmayor, F.J. A recovery-explicit error estimator in energy norm for linear elasticity. Comput. Methods Appl. Mech. Eng. 2015, 287, 172–190. [Google Scholar] [CrossRef] [Green Version]
- Rajendran, S.; Liew, K.M. Optimal stress sampling points of plane triangular elements for patch recovery of nodal stresses. Int. J. Numer. Meth. Eng. 2003, 58, 579–607. [Google Scholar] [CrossRef]
- Duflot, M.; Bordas, S. A posteriori error estimation for extended finite elements by an extended global recovery. Int. J. Numer. Meth. Eng. 2008, 76, 1123–1138. [Google Scholar] [CrossRef]
- Cai, D.; Cai, Z. A hybrid a posteriori error estimator for conforming finite element approximations. Comput. Methods Appl. Mech. Eng. 2018, 339, 320–340. [Google Scholar] [CrossRef]
- Zhang, R.; Li, L.; Zhao, L.; Tang, G. An adaptive remeshing procedure for discontinuous finite element limit analysis. Int. J. Numer. Meth. Eng. 2018. 116, 287–307. [CrossRef]
- Dong, Y.; Yuan, S.; Xing, Q. Adaptive finite element analysis with local mesh refinement based on a posteriori error estimate of element energy projection technique. Eng. Comput. 2019, 36, 2010–2033. [Google Scholar] [CrossRef]
- Sharma, R.; Zhang, J.; Langelaar, M.; van Keulen, F.; Aragón, A.M. An improved stress recovery technique for low-order 3D finite elements. Int. J. Numer. Meth. Eng. 2018, 114, 88–103. [Google Scholar] [CrossRef] [Green Version]
- Ahmed, M. Techniques for Mesh Independent Displacement Recovery in Elastic Finite Element Solutions. Trans. Famena 2021, XLV-2, 41–58. [Google Scholar] [CrossRef]
- Ahmed, M.; Singh, D.; AlQadhi, S.; Thanh, N.V. Moving Least Squares Interpolation Based A-Posteriori Error Technique in Finite Element Elastic Analysis. Comput. Modeling Eng. Sci. 2021, 129, 167–189. [Google Scholar] [CrossRef]
- Hauret, P.; Kuhl, E.; Ortiz, M. Diamond elements: A finite element/discrete-mechanics approximation scheme with guaranteed optimal convergence in incompressible elasticity. Int. J. Numer. Meth. Eng. 2007, 72, 253–294. [Google Scholar] [CrossRef]
- Lehrenfeld, C.; Reusken, A. L2-error analysis of an isoparametric unfitted finite element method for elliptic interface problems. J. Numer. Math. 2019, 27, 85–99. [Google Scholar] [CrossRef] [Green Version]
- Ahmed, M.; Singh, D.; AlQadhi, S.; Alrefae, M.A. Improvement of the Zienkiewicz–Zhu Error Recovery Technique Using a Patch Configuration. Appl. Sci. 2021, 11, 8120. [Google Scholar] [CrossRef]
- Nakshatrala, K.B.; Masud, A.; Hjelmstad, K.D. On finite element formulations for nearly incompressible linear elasticity. Comput Mech. 2008, 41, 547–561. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Lui, Y.C.; Huang, G.C. Error Estimates and convergence rate for various incompressible elements. Int. J. Numer. Meth. Eng. 1989, 28, 2192–2202. [Google Scholar] [CrossRef]



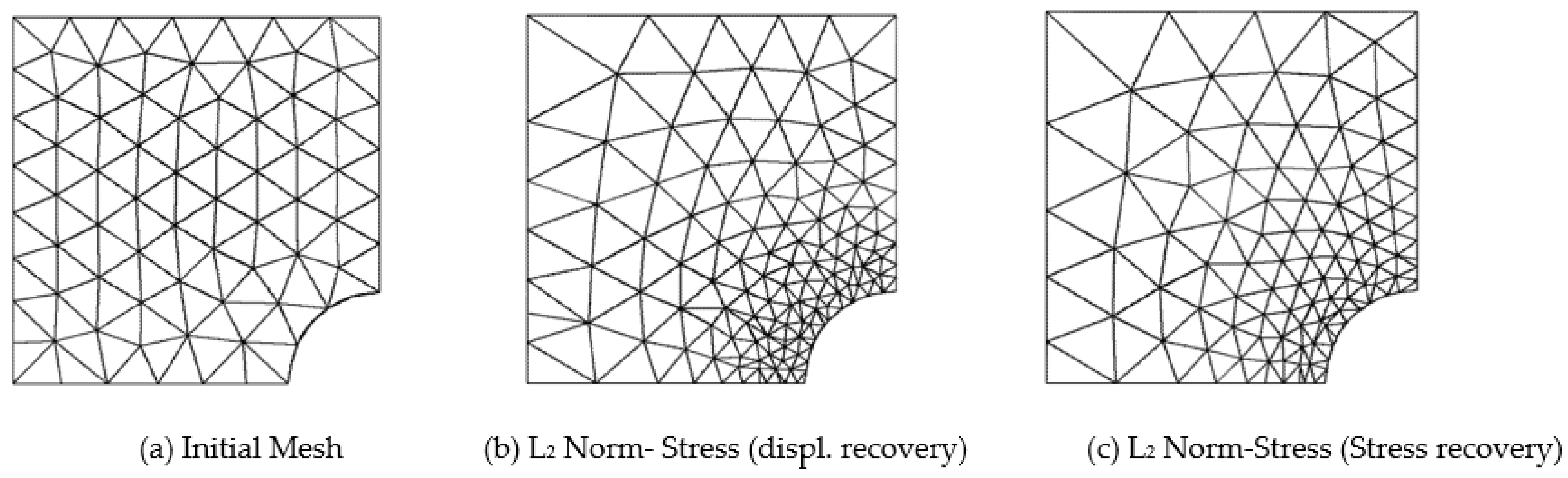

| Linear Triangle (Regular) | Quadratic Triangle (Regular) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Displ. Recovery | Displ. Recovery | ||||||||||||
| Mesh Size (1/h) | FEM Error (×10−3) | Error (Disp.) (×10−3) | θ | FEM Error (×10−3) | Error (Strain) (×10−3) | θ | Mesh Size (1/h) | FEM Error (×10−3) | Error (Disp.) (×10−3) | θ | FEM Error (×10−3) | Error (Strain) (×10−3) | θ |
| ¼ | 5.37 | 3.78 | 0.7095 | 58.33 | 36.51 | 0.9290 | ¼ | 0.25 | 1.03 | 4.2421 | 8.30 | 7.62 | 1.0737 |
| 1/16 | 0.38 | 0.18 | 0.6795 | 15.17 | 27.91 | 0.9894 | 1/12 | 0.01 | 0.02 | 2.4449 | 0.94 | 0.33 | 1.0102 |
| 1/32 | 0.10 | 0.04 | 0.6756 | 7.60 | 0.72 | 0.9970 | 1/24 | 0.001 | 0.001 | 1.5850 | 0.24 | 0.04 | 1.0026 |
| Conv. | 1.9394 | 2.1539 | 0.9799 | 1.8898 | 3.0473 | 3.7215 | 1.9869 | 2.9010 | |||||
| Linear Triangle (Regular) | Quadratic Triangle (Regular) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Mesh Size (1/h) | FEM Error (×10−3) | Displ. Recovery | Stress Recovery | Mesh Size (1/h) | FEM Error (×10−3) | Displ. Recovery | Stress Recovery | ||||
| Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | ||||
| ¼ | 185.99 | 116.42 | 0.9308 | 286.18 | 1.4265 | ¼ | 25.91 | 24.38 | 1.2607 | 9.75 | 0.9503 |
| 1/16 | 48.702 | 8.70 | 0.9899 | 45.10 | 1.2450 | 1/12 | 3.01 | 1.05 | 1.0389 | 0.59 | 0.9528 |
| 1/32 | 24.414 | 2.23 | 0.9971 | 16.54 | 1.1451 | 1/24 | 0.76 | 0.14 | 1.0104 | 0.11 | 0.9572 |
| Conv. | 0.9765 | 1.9012 | 1.3710 | 1.9714 | 2.8999 | 2.5018 | |||||
| Elements | Degree of Freedom (DOF) | Linear Triangle (Irregular) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Displacement Recovery | Stress Recovery | ||||||||||||
| FEM Error (×10−3) | Error (Disp.) (×10−3) | θ | FEM Error (×10−3) | Error (Strain) (×10−3) | θ | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | ||
| 28 | 46 | 87.89 | 64.08 | 0.9867 | 60.50 | 53.411 | 1.03566 | 187.90 | 149.34 | 0.9482 | 187.90 | 311.55 | 1.5597 |
| 580 | 652 | 18.54 | 3.11 | 0.9795 | 11.60 | 2.068 | 0.99084 | 35.70 | 5.85 | 0.9891 | 35.70 | 32.93 | 1.2904 |
| 2512 | 2658 | 6.29 | 0.79 | 0.9912 | 5.42 | 0.465 | 0.99638 | 15.73 | 1.27 | 0.9963 | 15.73 | 9.14 | 1.1282 |
| Elements | DOF | Quadratic Triangle (Irregular) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Displacement Recovery | Stress Recovery | ||||||||||||
| FEM Error (×10−3) | Error (Disp.) (×10−3) | θ | FEM Error (×10−3) | Error (Strain) (×10−3) | θ | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | ||
| 28 | 146 | 0.12 | 0.38 | 3.2678 | 6.47 | 10.73 | 1.8175 | 19.74 | 35.27 | 1.9138 | 19.74 | 14.48 | 0.9435 |
| 340 | 1470 | 0.08 | 0.02 | 2.1982 | 0.57 | 0.20 | 1.0451 | 1.62 | 0.75 | 1.0876 | 1.62 | 0.59 | 0.9454 |
| 1349 | 5608 | 0.02 | 0.015 | 1.4625 | 0.14 | 0.02 | 1.0096 | 0.41 | 0.10 | 1.0203 | 0.41 | 0.13 | 0.9542 |
| Linear Triangle (Regular) | Quadratic Triangle (Regular) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Stress Recovery (ZZ) | Displ. Recovery | Stress Recovery (ZZ) | Displ. Recovery | ||||||||
| Mesh Size (1/h) | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | Mesh Size (1/h) | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ |
| ¼ | 93.75 | 144.2486 | 1.4313 | 58.48 | 0.9300 | ¼ | 13.17 | 4.93 | 0.95024 | 12.26 | 1.2556 |
| 1/16 | 24.44 | 22.6413 | 1.2449 | 4.46 | 0.9896 | 1/12 | 1.52 | 0.30 | 0.95295 | 0.53 | 1.0405 |
| 1/32 | 12.25 | 8.29942 | 1.1451 | 1.16 | 0.9970 | 1/24 | 0.38 | 0.06 | 0.95729 | 0.07 | 1.0127 |
| Conv. | 0.9786 | 1.3731 | 1.8860 | 1.9785 | 2.5062 | 2.8845 | |||||
| Recovery Type/Initial Meshing | Displacement Error Recovery | Stress Error Recovery | ||||||
|---|---|---|---|---|---|---|---|---|
| FEM Error (%) | Error (%) | Adaptive Mesh | FEM Error (%) | Error (%) | Adaptive Mesh | |||
| N | DOF | N | DOF | |||||
| L2-Norm-Displacement (N = 28, DOF = 46) | 15.88 | 16.13 | 239 | 282 | - | - | - | - |
| L2-Norm-Strain (N = 580, DOF = 652) | 7.78 | 7.74 | 2520 | 2678 | - | - | - | - |
| L2-Norm-Stress (N = 580, DOF = 652) | 7.45 | 7.43 | 2154 | 2304 | 7.45 | 9.75 | 3090 | 3296 |
| Recovery Type/Initial Meshing | Displacement Error Recovery | Stress Error Recovery | ||||||
|---|---|---|---|---|---|---|---|---|
| FEM Error (%) | Error (%) | Adaptive Mesh | FEM Error (%) | Error (%) | Adaptive Mesh | |||
| N | DOF | N | DOF | |||||
| L2-Norm-Displacement (N = 28, DOF = 146) | 0.71 | 2.25 | 94 | 430 | - | - | - | - |
| L2-Norm-Strain (N = 28, DOF = 146) | 4.34 | 8.24 | 750 | 3162 | - | - | - | - |
| L2-Norm-Stress (N = 28, DOF = 146) | 4.12 | 8.28 | 477 | 2036 | 4.12 | 3.91 | 203 | 904 |
| Elements | DOF | Linear Triangle | Elements | DOF | Quadratic Triangle | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Displ. Recovery | Stress Recovery | Displ. Recovery | Stress Recovery | ||||||||||
| FEM Error (×10−3) | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | ||||
| 124 | 156 | 515.85 | 428.93 | 0.8696 | 449.68 | 0.9669 | 124 | 558 | 140.79 | 247.01 | 1.7706 | 106.92 | 0.9693 |
| 548 | 618 | 287.44 | 171.89 | 0.9319 | 290.91 | 1.2210 | 548 | 2330 | 54.02 | 71.53 | 1.2195 | 41.27 | 0.6783 |
| 2380 | 2522 | 147.08 | 58.97 | 0.9416 | 140.13 | 1.2185 | 2380 | 9802 | 44.59 | 45.94 | 0.4897 | 41.63 | 0.3341 |
| Recovery Type/Element Type | Displacement Error Recovery | Stress Error Recovery | ||||||
|---|---|---|---|---|---|---|---|---|
| FEM Error | Error | Adaptive Mesh | FEM Error | Error | Adaptive Mesh | |||
| N | DOF | N | DOF | |||||
| L2-Norm-Stress (initial linear triangular mesh: N = 548, DOF = 618, 3% target error) | 7.03 | 6.56 | 667 | 742 | 7.03 | 8.49 | 846 | 934 |
| L2-Norm-Stress (initial quadratic triangular mesh: N = 124, DOF = 558, 1% target error) | 3.44 | 6.07 | 231 | 1012 | 3.44 | 3.34 | 183 | 812 |
| Mesh Size (1/h) | Quadratic Triangle (Regular) | Elements | DOF | Quadratic Triangle (Irregular) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Displ. Recovery | Stress Recovery | Displ. Recovery | Stress Recovery | |||||||||
| FEM Error (×10−3) | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | FEM Error (×10−3) | Error (Stress) (×10−3) | θ | Error (Stress) (×10−3) | θ | |||
| ¼ | 46.78 | 23.13 | 0.9132 | 24.66 | 0.8735 | 28 | 146 | 39.72 | 27.44 | 0.8196 | 32.23 | 0.4999 |
| 1/16 | 16.13 | 3.11 | 0.9693 | 4.82 | 0.9472 | 340 | 1470 | 6.98 | 3.01 | 0.8554 | 3.20 | 0.8517 |
| 1/32 | 8.06 | 0.95 | 0.9746 | 1.69 | 0.9667 | 1349 | 5608 | 2.83 | 1.24 | 0.8699 | 1.36 | 0.8461 |
| Conv. | 0.9818 | 1.7805 | 1.4952 | |||||||||
| Recovery Type/Element Type | Displacement Error Recovery | Stress Error Recovery | ||||||
|---|---|---|---|---|---|---|---|---|
| FEM Error | Error | Adaptive Mesh | FEM Error | Error | Adaptive Mesh | |||
| N | DOF | N | DOF | |||||
| L2-Norm-Stress (initial quadratic triangular mesh: N = 1349, DOF = 5608, 1% target error) | 4.11 | 4.63 | 9666 | 39166 | 4.11 | 3.48 | 8392 | 33970 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmed, M.; Singh, D.; AlQadhi, S.; Kahla, N.B. L2-Norm Based a Posteriori Error Estimates of Compressible and Nearly-Incompressible Elastic Finite Element Solutions. Appl. Sci. 2022, 12, 3999. https://doi.org/10.3390/app12083999
Ahmed M, Singh D, AlQadhi S, Kahla NB. L2-Norm Based a Posteriori Error Estimates of Compressible and Nearly-Incompressible Elastic Finite Element Solutions. Applied Sciences. 2022; 12(8):3999. https://doi.org/10.3390/app12083999
Chicago/Turabian StyleAhmed, Mohd., Devinder Singh, Saeed AlQadhi, and Nabil Ben Kahla. 2022. "L2-Norm Based a Posteriori Error Estimates of Compressible and Nearly-Incompressible Elastic Finite Element Solutions" Applied Sciences 12, no. 8: 3999. https://doi.org/10.3390/app12083999
APA StyleAhmed, M., Singh, D., AlQadhi, S., & Kahla, N. B. (2022). L2-Norm Based a Posteriori Error Estimates of Compressible and Nearly-Incompressible Elastic Finite Element Solutions. Applied Sciences, 12(8), 3999. https://doi.org/10.3390/app12083999






