A Mathematical Study of COVID-19 Spread by Vaccination Status in Virginia
Abstract
:1. Introduction
2. Materials and Methods
2.1. n-Stage Vaccination Model
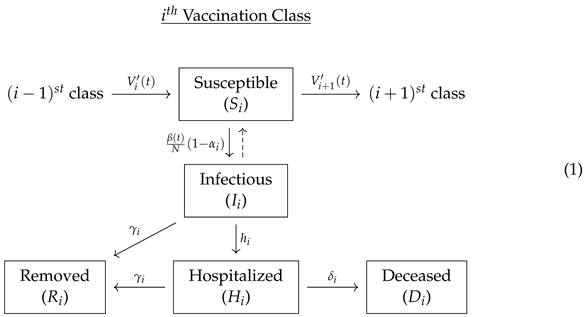
2.2. Governing System of Differential Equations
2.3. Basic and Effective Reproduction Number
2.4. Data Fitting Techniques
2.5. Parameter Sensitivity Analysis
3. Results
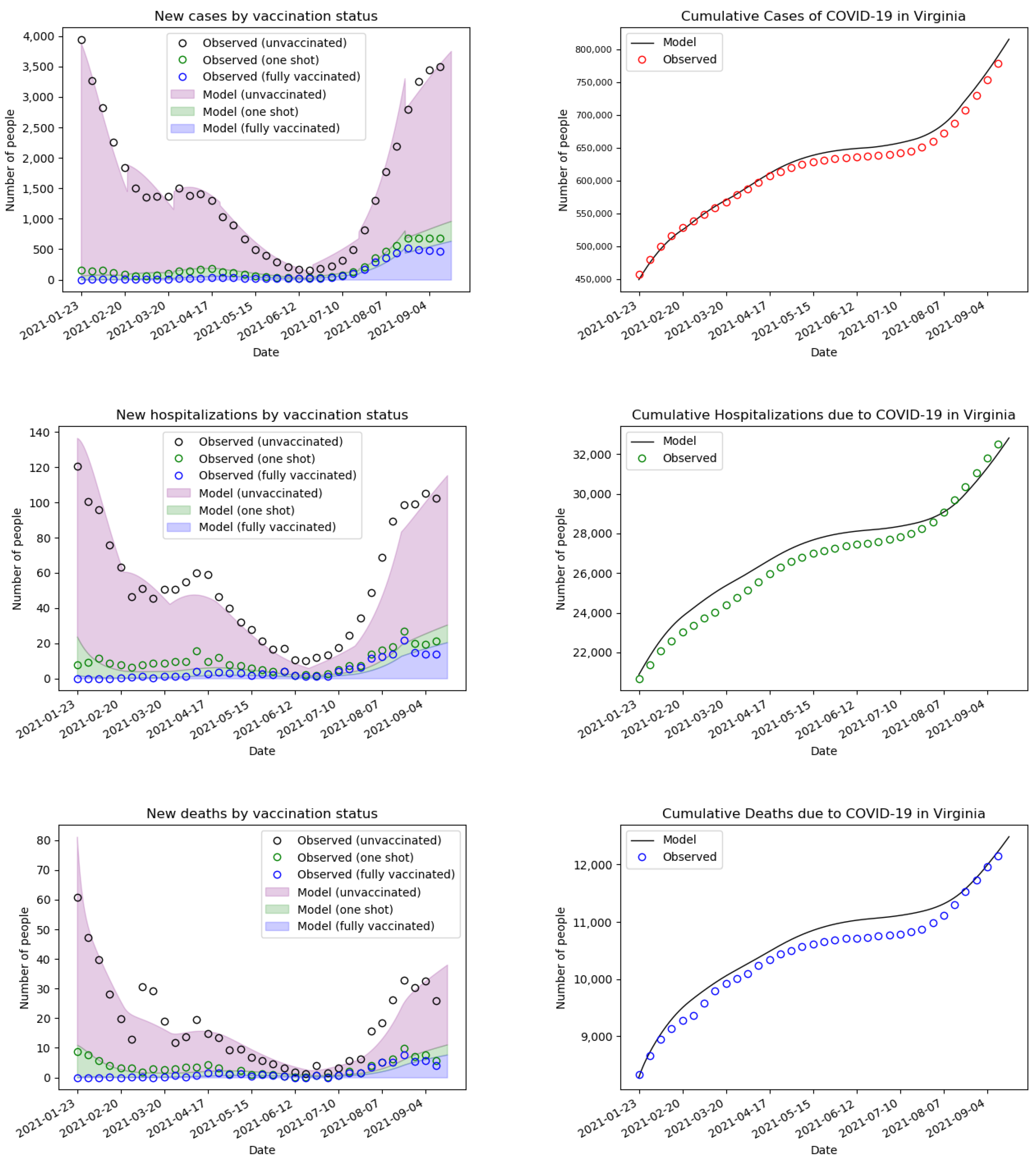
3.1. Fitting Two-Stage Vaccination Model to Virginia Data
3.2. Efficacy of Vaccination
3.3. Transmissibility of Delta Variant
3.4. Effect of Waning Immunity and Delta Variant on Vaccine Efficacy
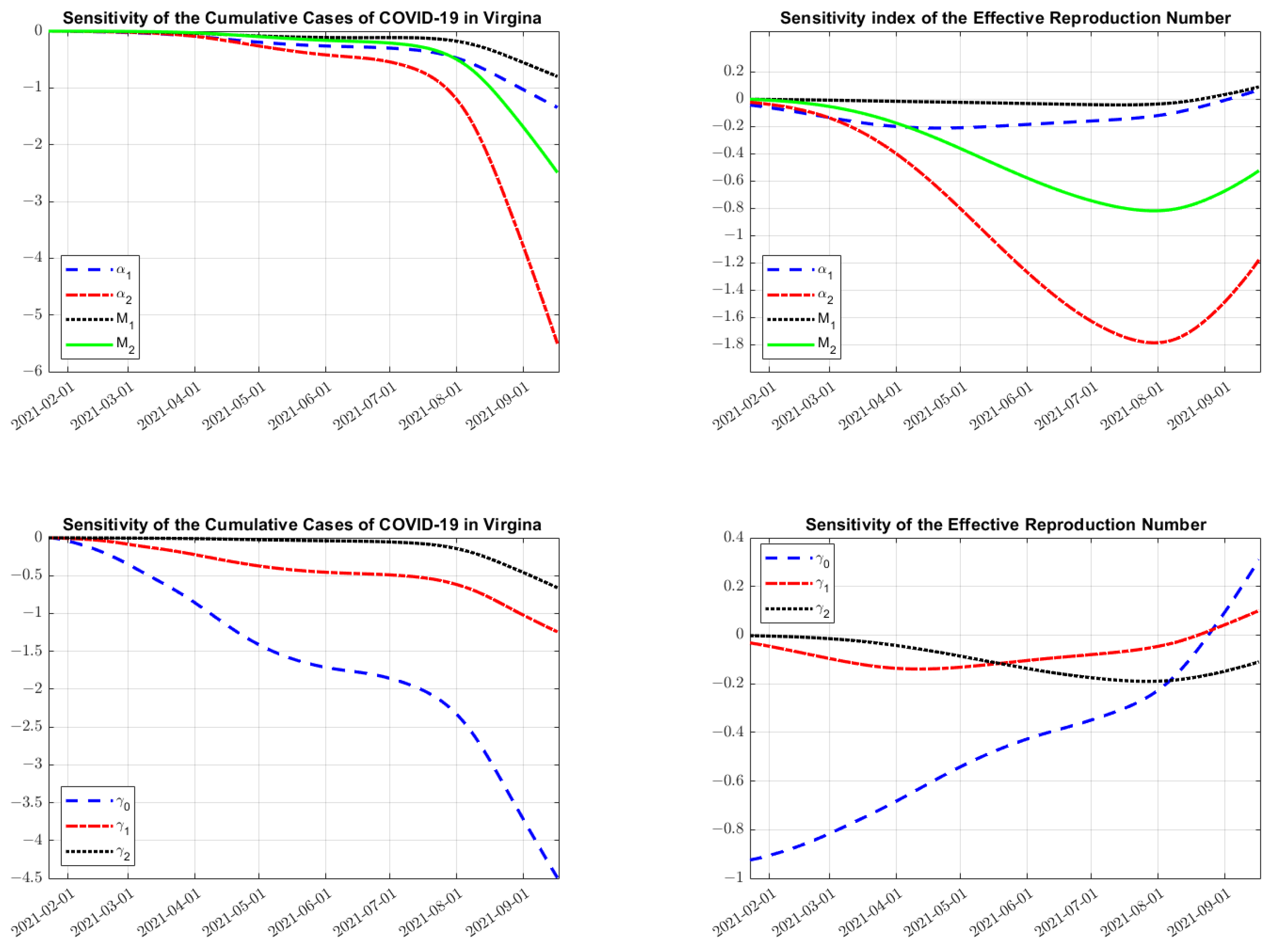
3.5. Parameter Sensitivity Analysis
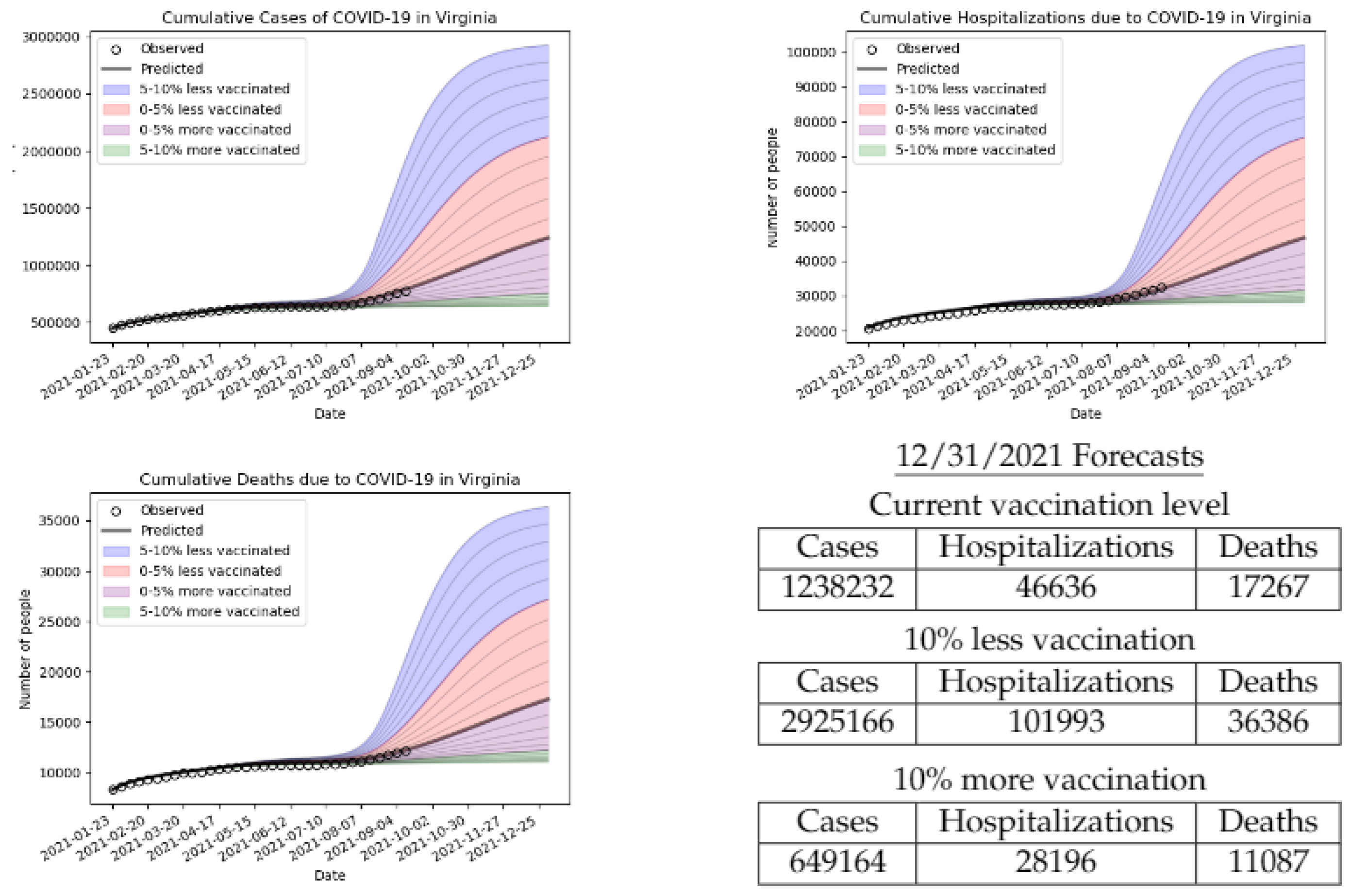
3.6. Modeling with Different Vaccine Coverage Levels
3.7. Modeling with Booster Shots
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fung, M.; Babik, J.M. COVID-19 in Immunocompromised Hosts: What We Know So Far. Clin. Infect. Dis. 2020, 72, 340–350. [Google Scholar] [CrossRef] [PubMed]
- Centers for Disease Control and Prevention. COVID Data Tracker—Variant Proportions. 2021. Available online: https://covid.cdc.gov/covid-data-tracker/#variant-proportions (accessed on 31 January 2022).
- Kermack, W.O.; McKendrick, A.G. A Contribution to the Mathematical Theory of Epidemics. Proc. Roy. Soc. Lond. A 1927, 115, 700–721. [Google Scholar]
- Kucharski, A.J.; Russell, T.W.; Diamond, C.; Liu, Y.; Edmunds, J.; Funk, S.; Eggo, R.M. Early dynamics of transmission and control of COVID-19: A mathematical modelling study. Lancet Infect. Dis. 2020, 20, P553–P558. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.; Diao, M.Y.; Yu, W.; Pei, L.; Lin, Z.; Chen, D. Estimation of the reproductive number of novel coronavirus (COVID-19) and the probable outbreak size on the Diamond Princess cruise ship: A data-driven analysis. Int. J. Infect. Dis. 2020, 93, 201–204. [Google Scholar] [CrossRef] [PubMed]
- Zhuang, Z.; Zhao, S.; Lin, Q.; Cao, P.; Lou, Y.; Yang, L.; Yang, S.; He, D.; Xiao, L. Preliminary estimates of the reproduction number of the coronavirus disease (COVID-19) outbreak in Republic of Korea and Italy by 5 March 2020. Int. J. Infect. Dis. 2020, 95, 308–310. [Google Scholar] [CrossRef] [PubMed]
- Arcede, J.P.; Caga-anan, R.L.; Mentuda, C.Q.; Mammeri, Y. Accounting for Symptomatic and Asymptomatic in a SEIR-type model of COVID-19. Math. Model. Nat. Phenom. 2020, 15, 34. [Google Scholar] [CrossRef]
- Radulescu, A.; Williams, C.; Cavanagh, K. Management strategies in a SEIR-type model of COVID 19 community spread. Sci. Rep. 2020, 10, 21256. [Google Scholar] [CrossRef]
- Das, K.D.; Khatua, A.; Kar, T.K.; Jana, S. The effectiveness of contact tracing in mitigating COVID-19 outbreak: A model-based analysis in the context of India. Appl. Math. Comput. 2021, 404, 126207. [Google Scholar]
- Grimm, V.; Mengel, F.; Schmidt, M. Extensions of the SEIR model for the analysis of tailored social distancing and tracing approaches to cope with COVID-19. Sci. Rep. 2021, 11, 4214. [Google Scholar] [CrossRef]
- Barbarossa, M.; Fuhrmann, J.; Meinke, J.H.; Krieg, S.; Vinod Varma, H.; Castelletti, N.; Lippert, T. Modeling the spread of COVID-19 in Germany: Early assessment and possible scenarios. PLoS ONE 2020, 15, e0238559. [Google Scholar] [CrossRef]
- Bouchnita, A.; Jebrane, A. A hybrid multi-scale model of COVID-19 transmission dynamics to assess the potential of non-pharmaceutical interventions. Chaos Solitons Fractals 2020, 138, 109941. [Google Scholar] [CrossRef] [PubMed]
- Chang, S.L.; Harding, N.; Zachreson, C.; Cliff, O.M.; Prokopenko, M. Modelling transmission and control of the COVID-19 pandemic in Australia. Nat. Commun. 2020, 11, 5710. [Google Scholar] [CrossRef] [PubMed]
- Eikenberry, S.E.; Mancuso, M.; Iboi, E.; Phan, T.; Eikenberry, K.; Kuang, Y.; Kostelich, E.; Gumel, A.B. To mask or not to mask: Modeling the potential for face mask use by the general public to curtail the COVID-19 pandemic. Infect. Dis. Model 2020, 5, 293–308. [Google Scholar] [CrossRef] [PubMed]
- Yavuz, M.; Ozlem Cosar, F.; Gunay, F.; Ozdemir, F. A New Mathematical Modeling of the COVID-19 Pandemic Including the Vaccination Campaign. Open J. Model. Simul. 2021, 9, 299–321. [Google Scholar] [CrossRef]
- Ghostine, R.; Gharamti, M.; Hassrouny, S.; Hoteit, I. An Extended SEIR Model with Vaccination for Forecasting the COVID-19 Pandemic in Saudi Arabia Using an Ensemble Kalman Filter. Mathematics 2021, 9, 636. [Google Scholar] [CrossRef]
- Choi, Y.; Kim, J.S.; Kim, J.E.; Choi, H.; Lee, C.H. Vaccination Prioritization Strategies for COVID-19 in Korea: A Mathematical Modeling Approach. Int. J. Environ. Res. Public Health 2021, 18, 4240. [Google Scholar] [CrossRef]
- Bubar, K.M.; Reinholt, K.; Kissler, S.M.; Lipsitch, M.; Cobey, S.; Grad, Y.H.; Larremore, D.B. Model-informed COVID-19 vaccine prioritization strategies by age and serostatus. Science 2021, 371, 916–921. [Google Scholar] [CrossRef]
- Mahase, E. COVID-19: Pfizer vaccine efficacy was 52% after first dose and 95% after second dose, paper shows. BMJ 2020, 371, m4826. [Google Scholar] [CrossRef]
- Bergwerk, M.; Gonen, T.; Lustig, Y.; Amit, S.; Lipsitch, M.; Cohen, C.; Mandelboim, M.; Levin, E.; Rubin, C.; Indenbaum, V.; et al. COVID-19 Breakthrough Infections in Vaccinated Health Care Workers. N. Engl. J. Med. 2021, 385, 1474–1484. [Google Scholar] [CrossRef]
- Chagla, Z. The BNT162b2 (BioNTech/Pfizer) vaccine had 95% efficacy against COVID-19 ≥7 days after the 2nd dose. Ann. Intern. Med. 2021, 174, JC15. [Google Scholar] [CrossRef]
- Centers for Disease Control and Prevention. CDC Covid Data Tracker—Rates of COVID-19 Cases and Deaths by Vaccination Status. 2021. Available online: https://covid.cdc.gov/covid-data-tracker/#rates-by-vaccine-status (accessed on 31 January 2022).
- Scobie, H.M.; Johnson, A.G.; Suthar, A.B.; Severson, R.; Alden, N.B.; Balter, S.; Bertolino, D.; Blythe, D.; Brady, S.; Cadwell, B.; et al. Monitoring Incidence of COVID-19 Cases, Hospitalizations, and Deaths, by Vaccination Status—13 U.S. Jurisdictions, April 4–July 17, 2021. Morb. Mortal. Wkly. Rep. 2021, 70, 1284–1290. [Google Scholar] [CrossRef] [PubMed]
- Roghani, A. The Influence of Covid-19 Vaccine on Daily Cases, Hospitalization, and Death Rate in Tennessee: A Case Study in the United States. JMIRx Med. 2021, 2, e29324. [Google Scholar] [CrossRef] [PubMed]
- Virginia Department of Health. VDH-COVID-19-PublicUseDataset-Cases-by-Vaccination-Status. 2021. Available online: https://data.virginia.gov/dataset/VDH-COVID-19-PublicUseDataset-Cases-by-Vaccination/vsrk-d6hx (accessed on 12 October 2021).
- Ritchie, H.; Mathieu, E.; Rodés-Guirao, L.; Appel, C.; Giattino, C.; Ortiz-Ospina, E.; Hasell, J.; Macdonald, B.; Beltekian, D.; Roser, M. oronavirus Pandemic (COVID-19). Our World in Data. 2020. Available online: https://ourworldindata.org/coronavirus (accessed on 31 January 2022).
- Liu, Z.; Magal, P.; Seydi, O.; Webb, G. A COVID-19 epidemic model with latency period. Infect. Dis. Model. 2020, 5, 323–337. [Google Scholar] [CrossRef]
- Jin, Y.H.; Cai, L.; Cheng, Z.S.; Cheng, H.; Deng, T.; Fan, Y.P.; Fang, C.; Huang, D.; Huang, L.Q.; Huang, Q.; et al. A rapid advice guideline for the diagnosis and treatment of 2019 novel coronavirus (2019-nCoV) infected pneumonia. Mil. Med. Res. 2020, 7, 4. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goldberg, Y.; Mandel, M.; Bar-On, Y.M.; Bodenheimer, O.; Freedman, L.; Haas, E.J.; Milo, R.; Alroy-Preis, S.; Ash, N.; Huppert, A. Waning immunity of the BNT162b2 vaccine: A nationwide study from Israel. medRxiv 2021. [Google Scholar] [CrossRef]
- Ward, H.; Cooke, G.; Atchison, C.; Whitaker, M.; Elliott, J.; Moshe, M.; Brown, J.C.; Flower, B.; Daunt, A.; Ainslie, K.; et al. Declining prevalence of antibody positivity to SARS-CoV-2: A community study of 365,000 adults. medRxiv 2020. [Google Scholar] [CrossRef]
- Tartof, S.Y.; Slezak, J.M.; Fischer, H.; Hong, V.; Ackerson, B.K.; Ranasinghe, O.N.; Frankland, T.B.; Ogun, O.A.; Zamparo, J.M.; Gray, S.; et al. Six-Month Effectiveness of BNT162B2 mRNA COVID-19 Vaccine in a Large US Integrated Health System: A Retrospective Cohort Study. Available online: http://xxx.lanl.gov/abs/https://ssrn.com/abstract=3909743 (accessed on 31 January 2022).
- Pathak, E.B.; Garcia, R.B.; Menard, J.M.; Salemi, J.L. Out-of-Hospital COVID-19 Deaths: Consequences for Quality of Medical Care and Accuracy of Cause of Death Coding. Am. J. Public Health 2021, 111, S101–S106. [Google Scholar] [CrossRef]
- Richards, F.J. A Flexible Growth Function for Empirical Use. J. Exp. Bot. 1959, 10, 290–301. [Google Scholar] [CrossRef]
- MacDonald, G. The analysis of equilibrium in malaria. Trop. Dis. Bull. 1952, 49, 871–889. [Google Scholar]
- Van den Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef]
- Heffernan, J.M.; Smith, R.J.; Wahl, L.M. Perspectives on the basic reproduction ratio. J. R. Soc. Interface 2005, 2, 281–293. [Google Scholar] [CrossRef] [PubMed]
- Zi, Z. Sensitivity analysis approaches applied to systems biology models. IET Syst. Biol. 2011, 5, 336–346. [Google Scholar] [CrossRef] [PubMed]
- Farinholt, T.; Doddapaneni, H.; Qin, X.; Menon, V.; Meng, Q.; Metcalf, G.; Chao, H.; Gingras, M.C.; Avadhanula, V.; Farinholt, P.; et al. Transmission event of SARS-CoV-2 delta variant reveals multiple vaccine breakthrough infections. BMC Med. 2021, 19, 255. [Google Scholar] [CrossRef]
- Bar-On, Y.M.; Goldberg, Y.; Mandel, M.; Bodenheimer, O.; Freedman, L.; Kalkstein, N.; Mizrahi, B.; Alroy-Preis, S.; Ash, N.; Milo, R.; et al. Protection of BNT162b2 Vaccine Booster against Covid-19 in Israel. N. Engl. J. Med. 2021, 385, 1393–1400. [Google Scholar] [CrossRef] [PubMed]
- Pfizer and BioNTech Announce Phase 3 Trial Data Showing High Efficacy of a Booster Dose of Their COVID-19 Vaccine. Pfizer News. 2021. Available online: http://xxx.lanl.gov/abs/https://www.pfizer.com/news/press-release/press-release-detail/pfizer-and-biontech-announce-phase-3-trial-data-showing (accessed on 31 January 2022).


| Parameter | Units | Interpretation |
|---|---|---|
| N | people | Population size (total) |
| people | Population size (ith class) | |
| people | Vaccination uptake curve (ith class) | |
| days | Vaccination uptake rate (ith class) | |
| people | Maximum vaccination total (ith class) | |
| none | Maximum vaccination proportion (ith class) | |
| days | Vaccination time shift (ith class) | |
| none | Vaccination shape parameter (ith class) | |
| days | Baseline transmission rate for unvaccinated | |
| none | Reduction from baseline transmission rate (ith class) | |
| days | Removal rate (ith class) | |
| days | Hospitalization rate (ith class) | |
| days | Death rate (ith class) | |
| none | Proportion infected to hospitalized (ith class) | |
| none | Proportion hospitalized to deceased (ith class) |
| Initial Conditions | Parameters | ||||
|---|---|---|---|---|---|
| Parameter | Value | Source | Parameter | Value | Source |
| N | 8,535,519 | [25] | fitted | ||
| 8,136,306 | assumed (9) | fitted | |||
| 289,839 | assumed (9) | fitted | |||
| 109,375 | assumed (9) | fitted | |||
| 7,695,938 | assumed (10) | fitted | |||
| 19,557 | fitted | fitted | |||
| 411,709 | fitted | fitted | |||
| 823 | fitted | fitted | |||
| 8279 | assumed | fitted | |||
| 281,970 | assumed (10) | fitted | |||
| 7642 | fitted | fitted | |||
| 227 | fitted | fitted | |||
| 0 | assumed | fitted | |||
| 0 | assumed | fitted | |||
| 108,839 | assumed (10) | fitted | |||
| 536 | fitted | fitted | |||
| 0 | fitted | fitted | |||
| 0 | assumed | fitted | |||
| 0 | assumed | fitted | |||
| Interval | Dates | % Part Vax | % Full Vax | ||
|---|---|---|---|---|---|
| 1 | 1/23–2/21 | 4.4% | 0.5% | 0.153 | 0.786 |
| 2 | 2/22–3/23 | 14.0% | 6.1% | 0.200 | 0.978 |
| 3 | 3/24–4/22 | 26.7% | 14.7% | 0.251 | 1.106 |
| 4 | 4/23–5/21 | 44.3% | 29.1% | 0.241 | 0.906 |
| 5 | 5/22–6/20 | 53.2% | 42.7% | 0.238 | 0.762 |
| 6 | 6/21–7/20 | 58.2% | 50.3% | 0.464 | 1.312 |
| 7 | 7/21–8/19 | 60.7% | 53.7% | 0.539 | 1.404 |
| 8 | 8/20–9/18 | 64.2% | 56.1% | 0.453 | 1.119 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Johnston, M.D.; Pell, B.; Nelson, P. A Mathematical Study of COVID-19 Spread by Vaccination Status in Virginia. Appl. Sci. 2022, 12, 1723. https://doi.org/10.3390/app12031723
Johnston MD, Pell B, Nelson P. A Mathematical Study of COVID-19 Spread by Vaccination Status in Virginia. Applied Sciences. 2022; 12(3):1723. https://doi.org/10.3390/app12031723
Chicago/Turabian StyleJohnston, Matthew D., Bruce Pell, and Patrick Nelson. 2022. "A Mathematical Study of COVID-19 Spread by Vaccination Status in Virginia" Applied Sciences 12, no. 3: 1723. https://doi.org/10.3390/app12031723
APA StyleJohnston, M. D., Pell, B., & Nelson, P. (2022). A Mathematical Study of COVID-19 Spread by Vaccination Status in Virginia. Applied Sciences, 12(3), 1723. https://doi.org/10.3390/app12031723







