An Adapted Two-Steps Approach to Simulate Nonlinear Vibrations of Solid Undergoing Large Deformation in Contact with Rigid Plane—Application to a Grooved Cylinder
Abstract
:1. Introduction
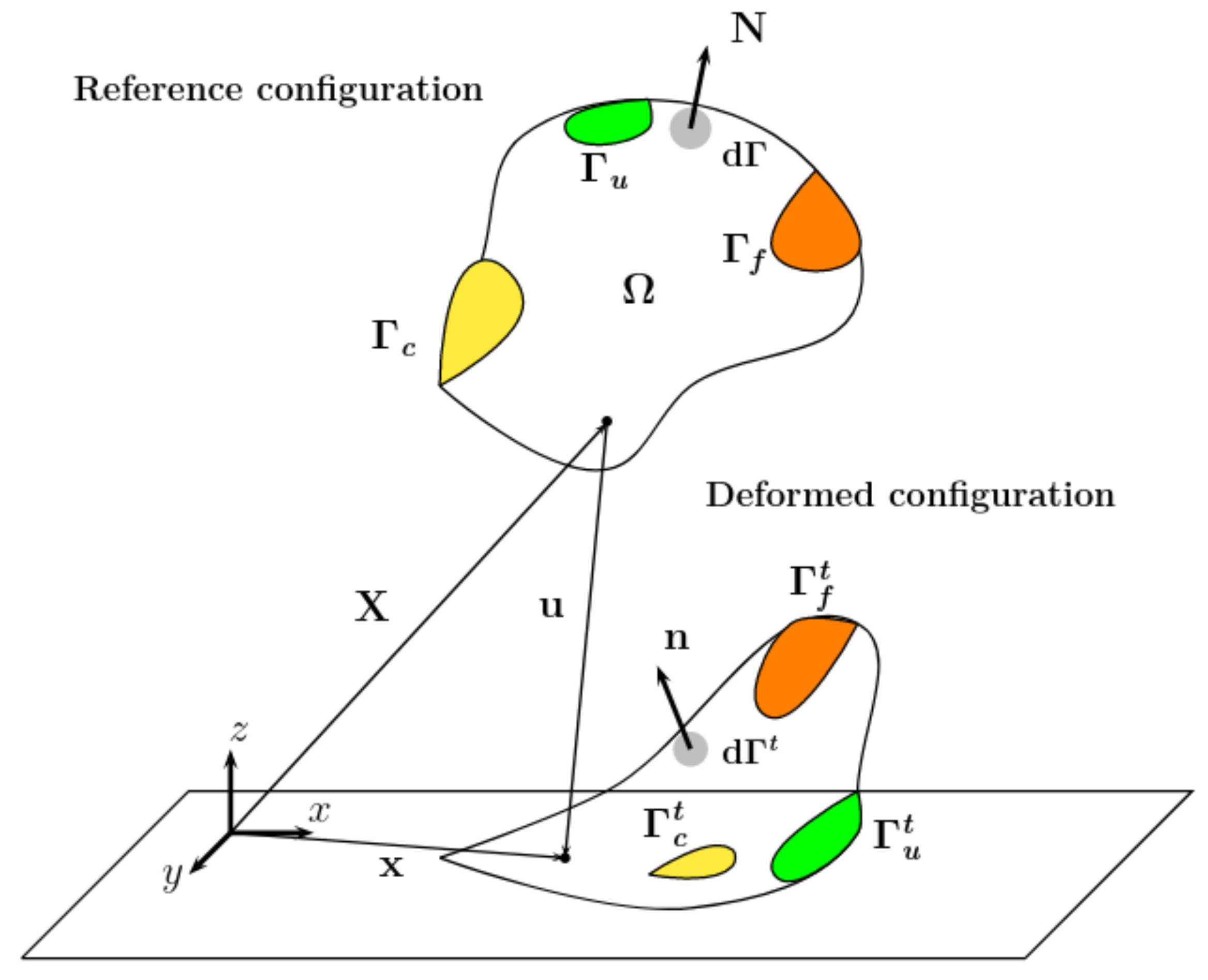
2. Problem Setting
2.1. Notations
2.2. Contact Mechanics
2.2.1. Signorini Conditions
2.2.2. Coulomb Friction Law
2.3. Equations of Motion
2.4. Dynamic Response
2.4.1. Main Hypothesis
2.4.2. Linearization
3. Numerical Results
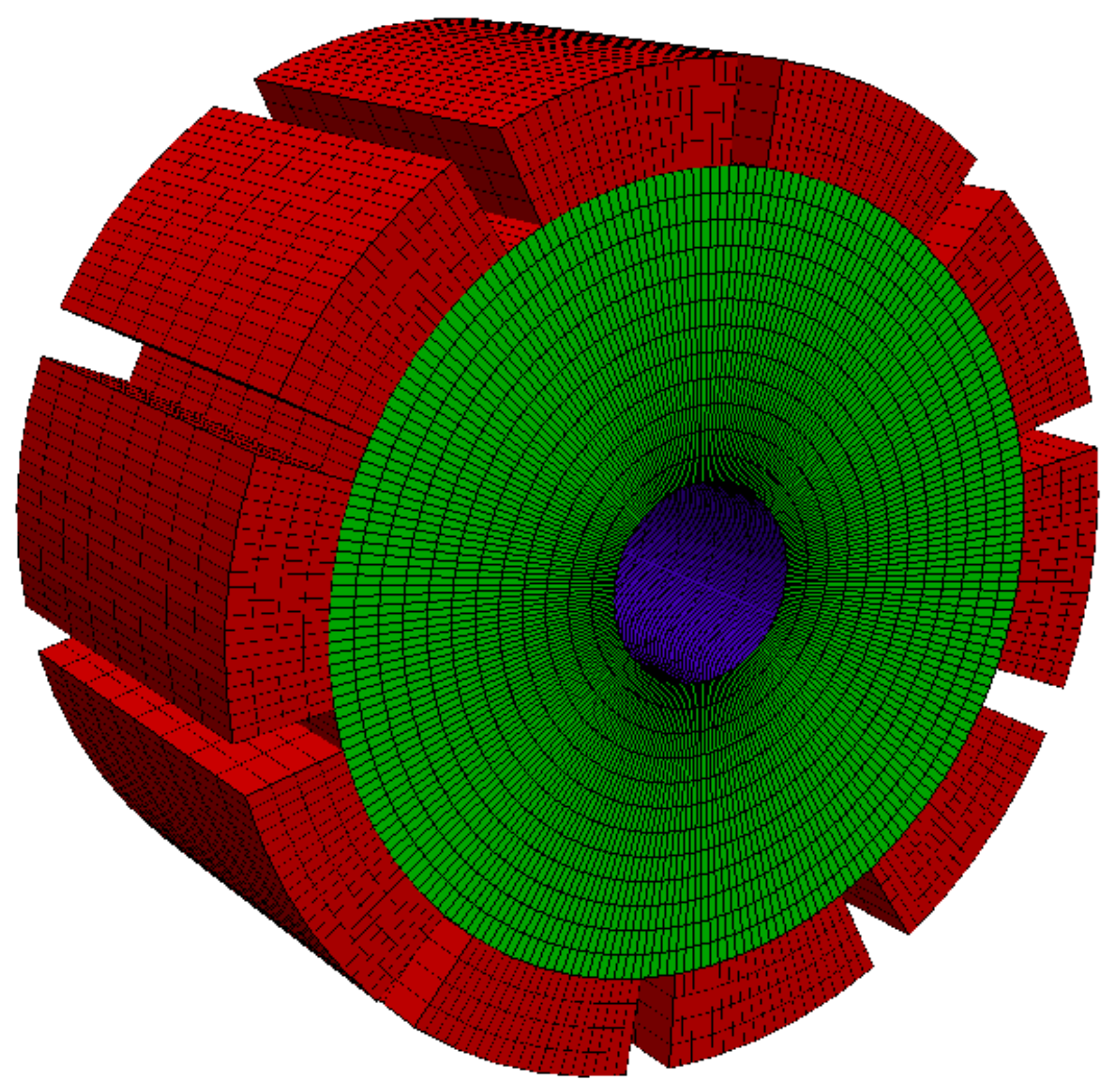
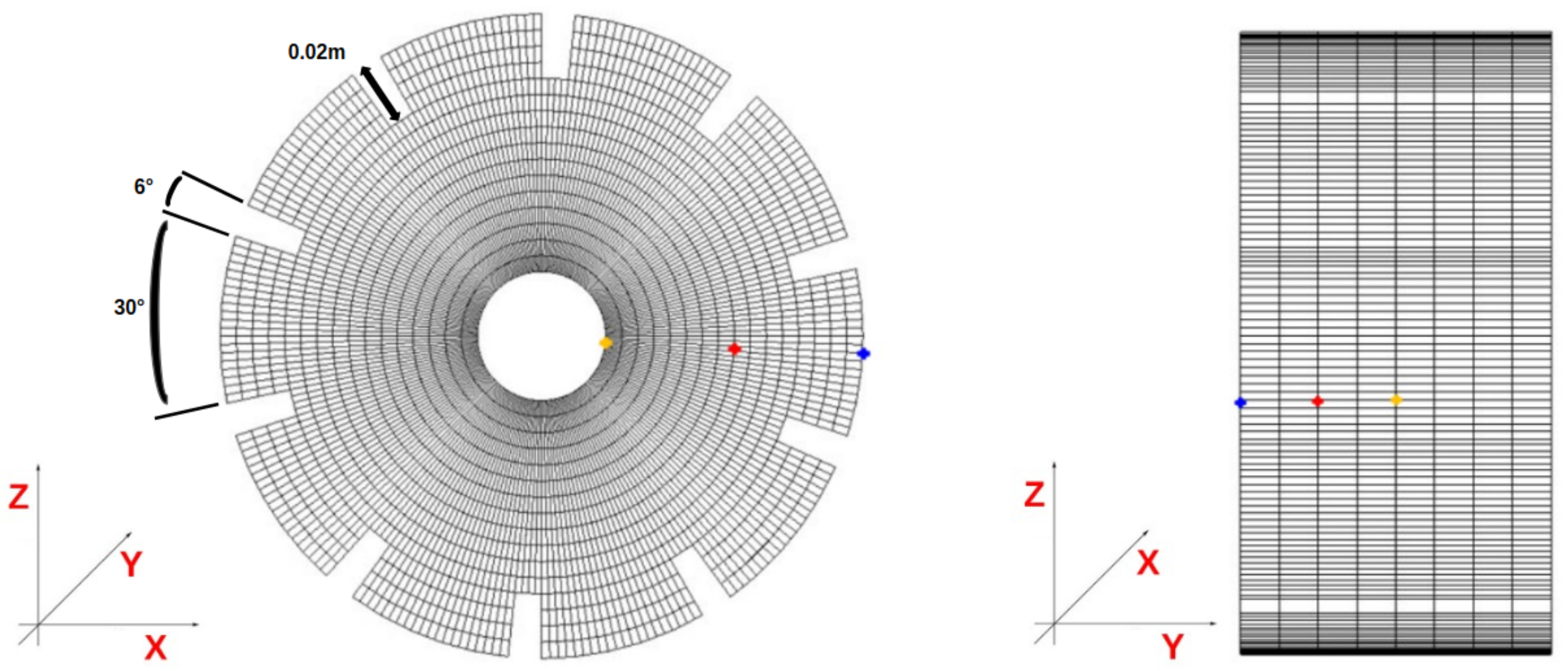
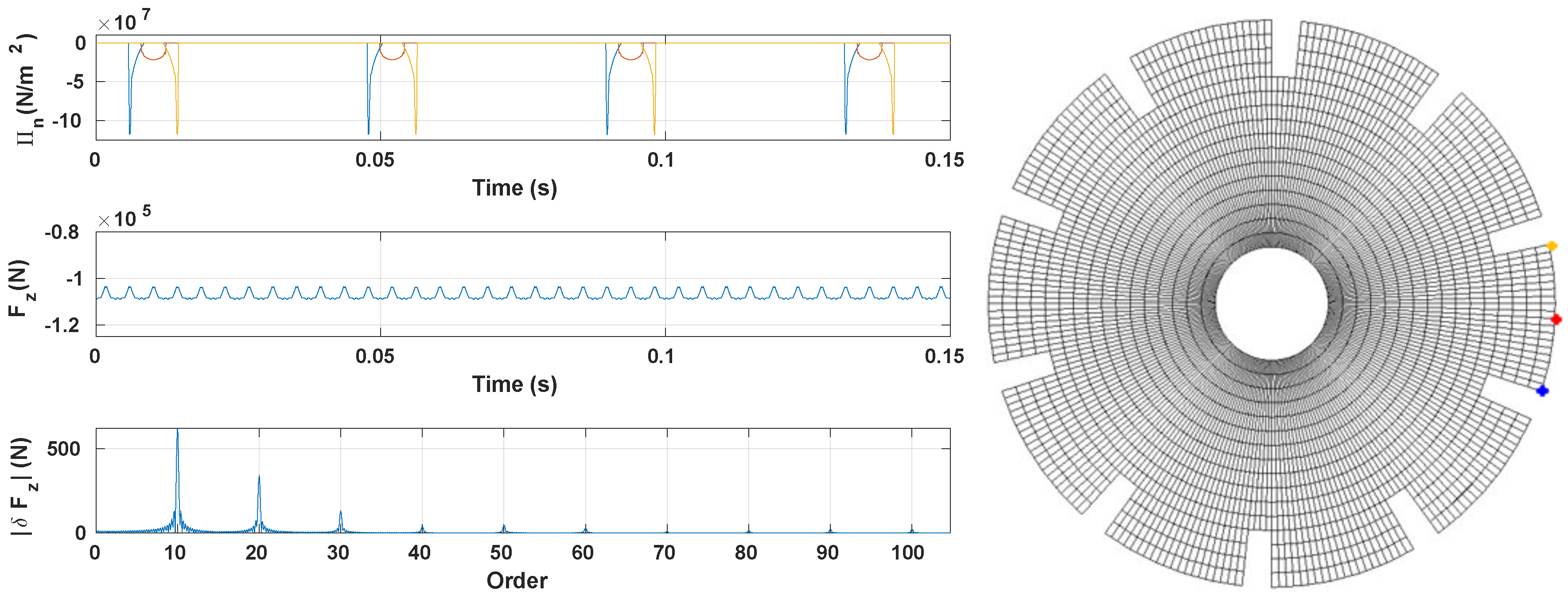
3.1. Prototype
3.2. Quasi-Static Rolling
3.2.1. Preample-Frictionless Contact
3.2.2. Extension by Considering the Impact of the Friction Coefficient
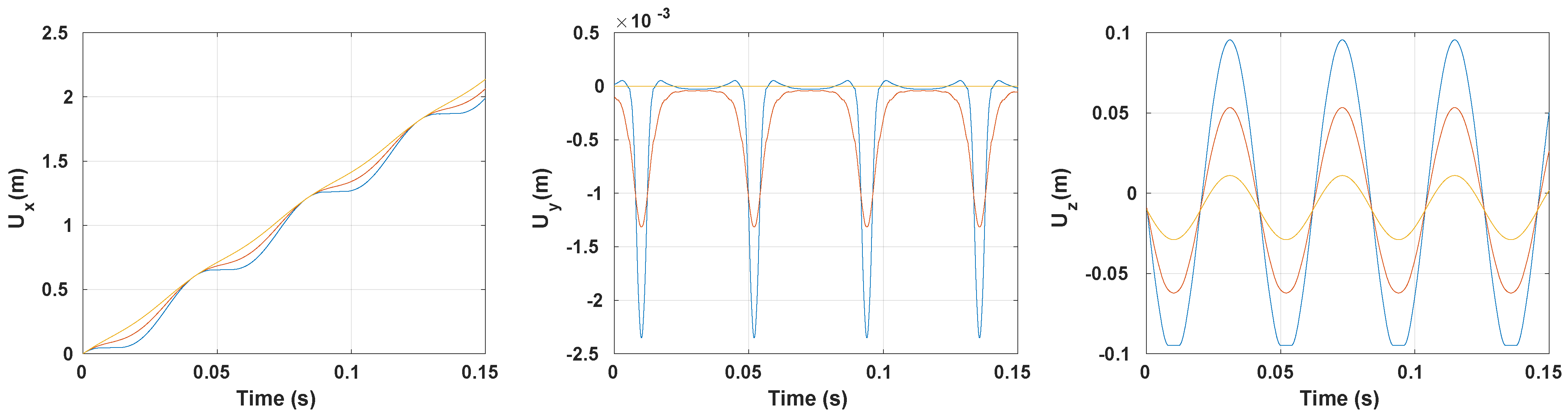
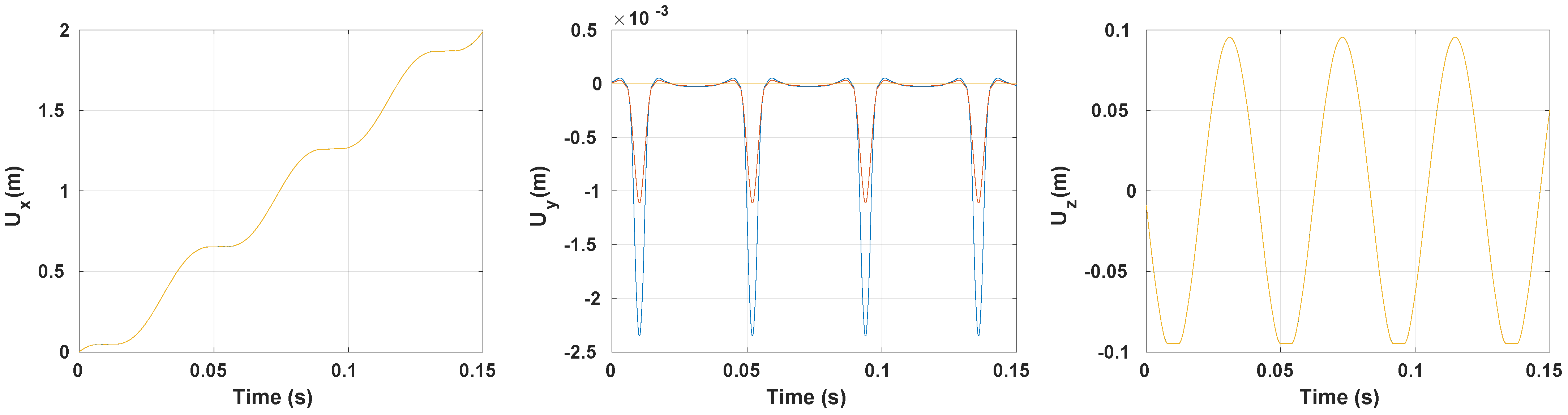
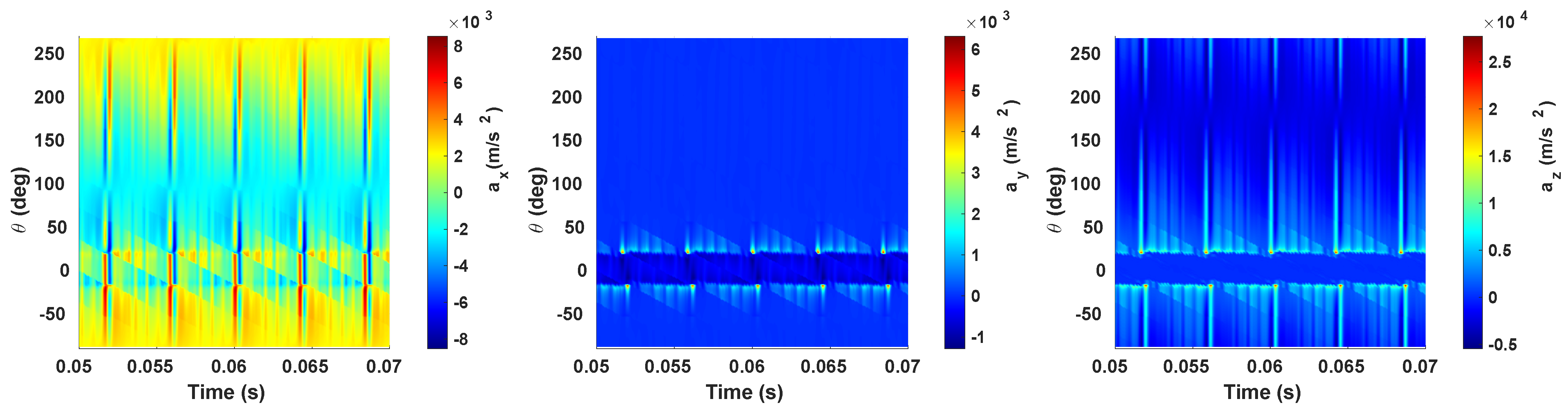
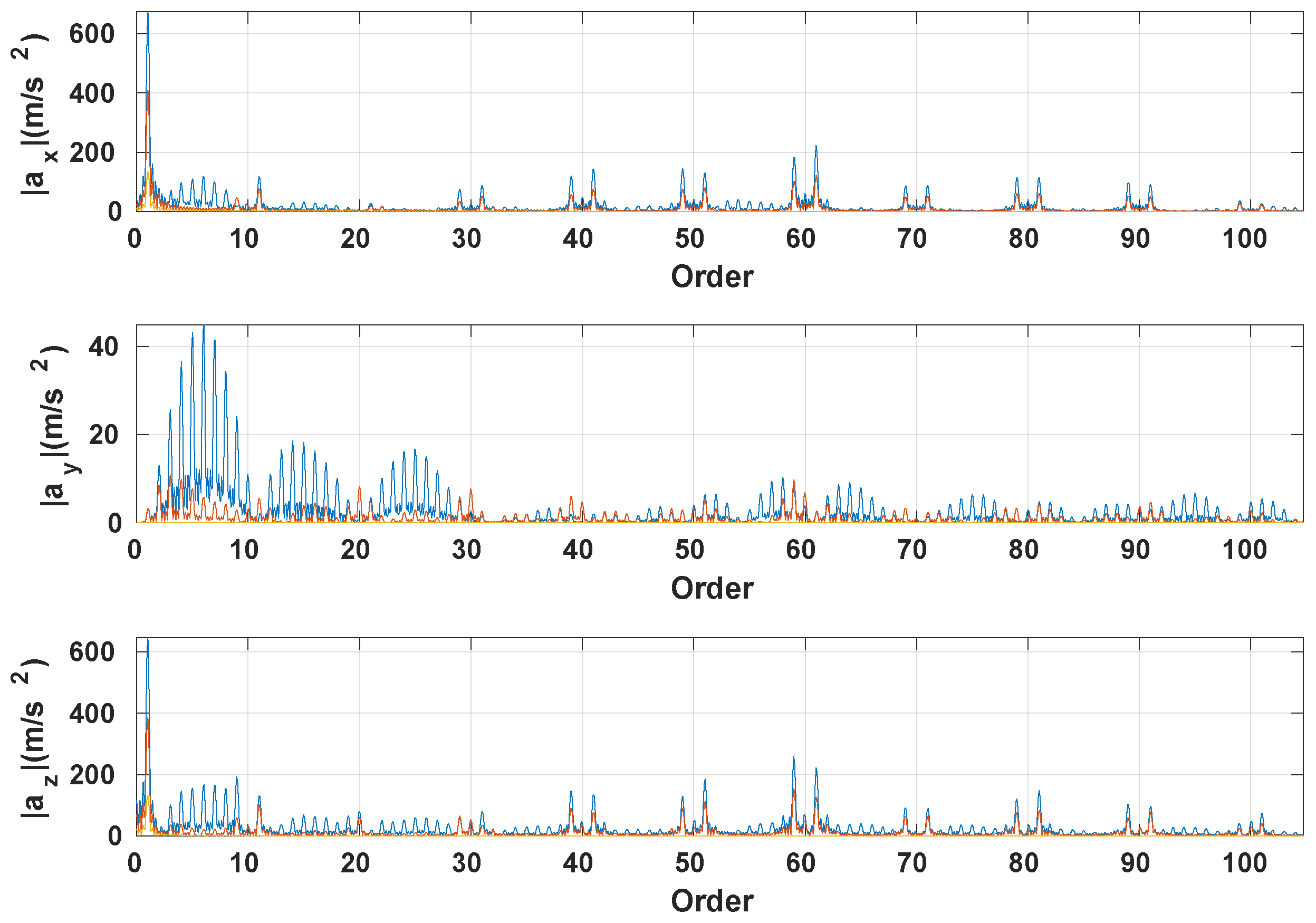
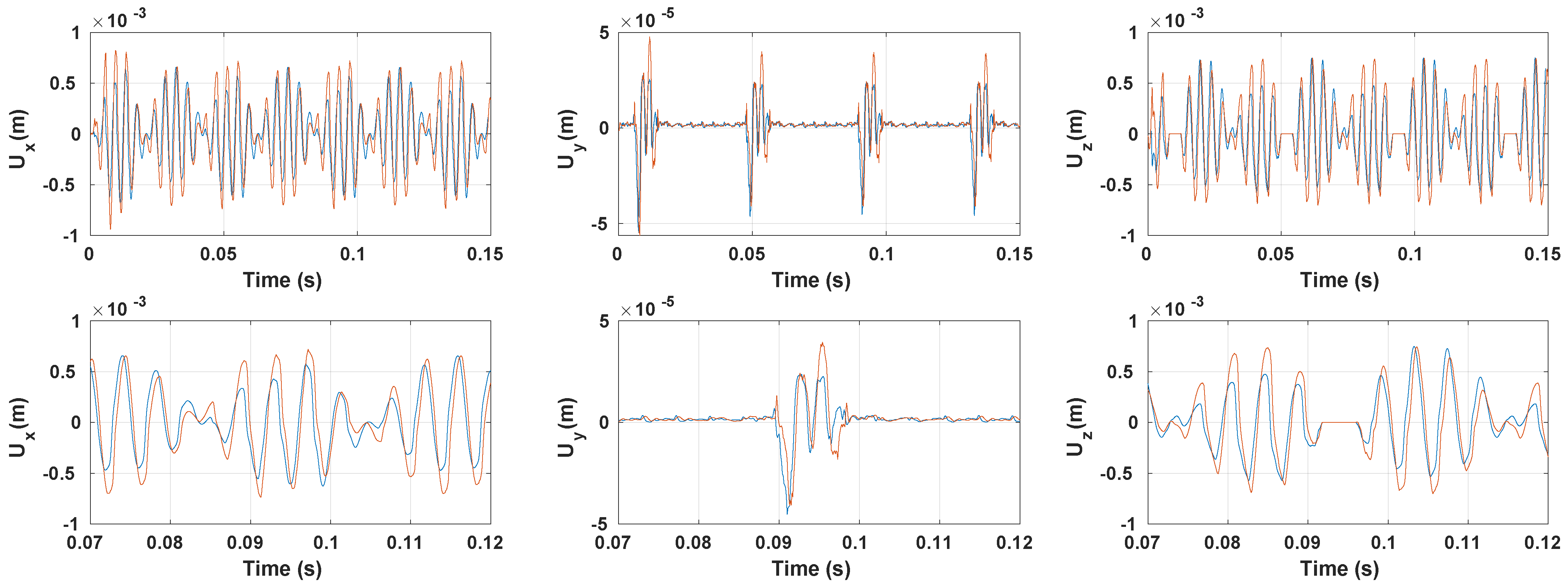
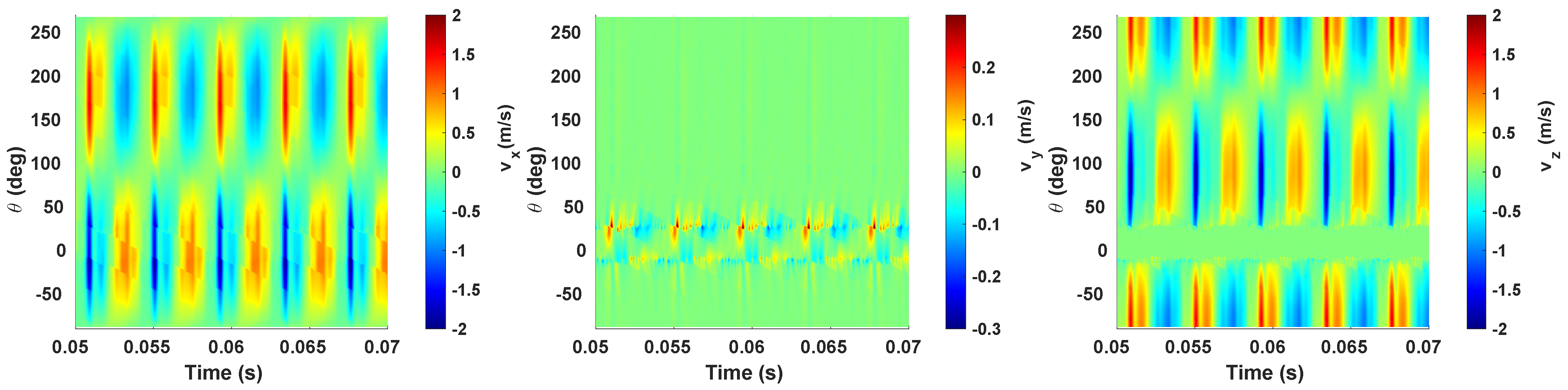
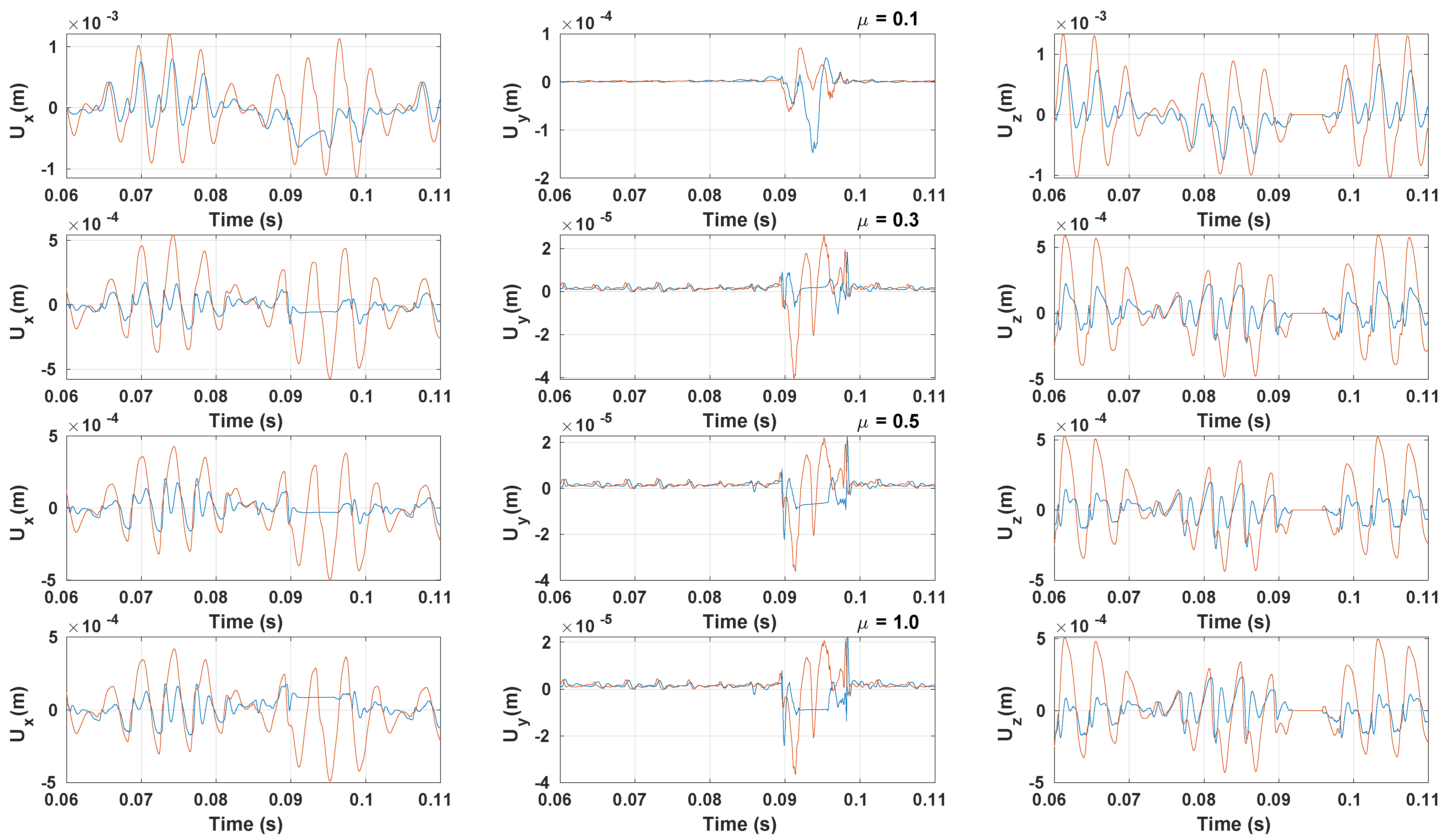
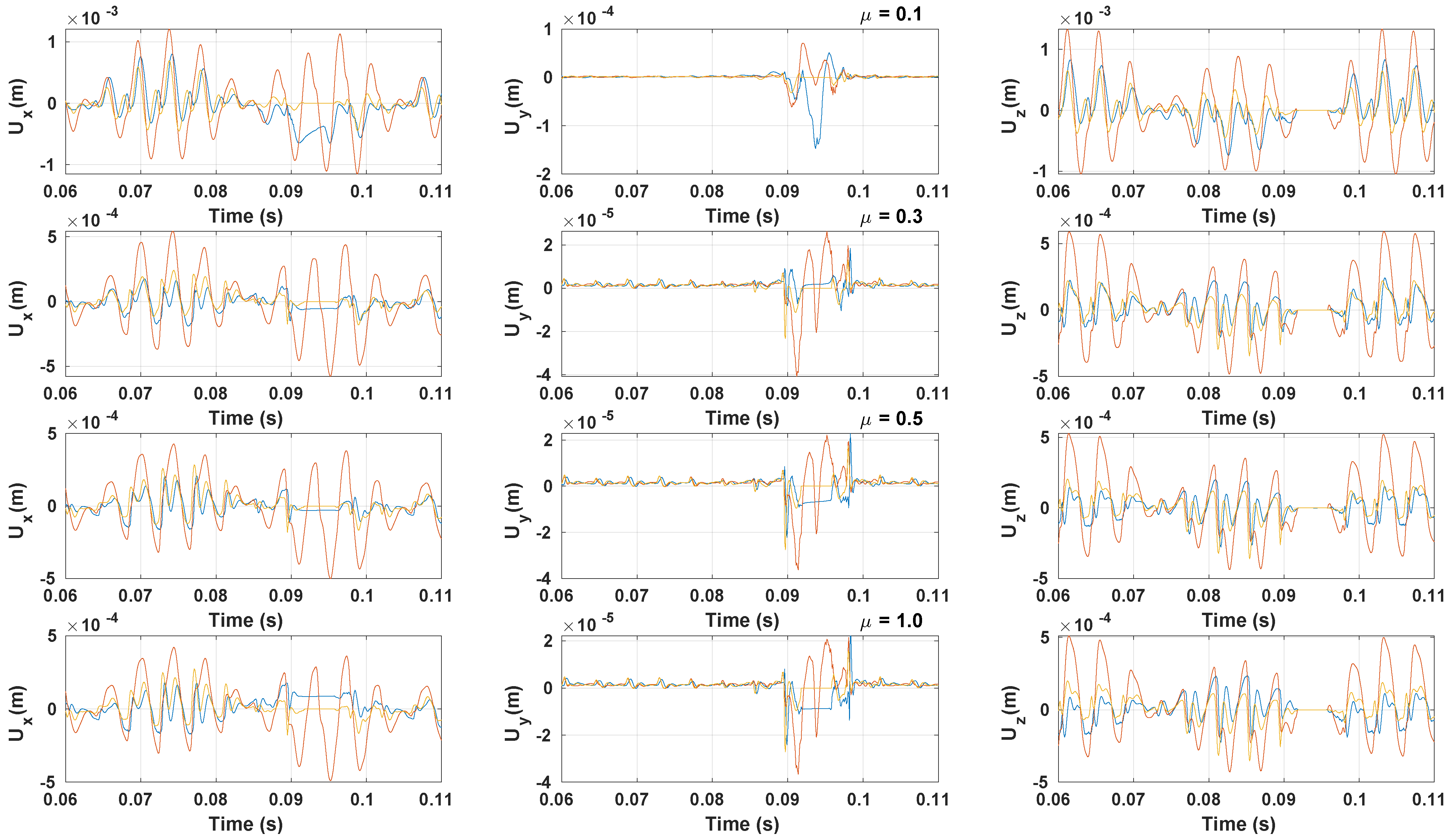
3.3. Vibration Response
3.3.1. Preample-Frictionless Contact
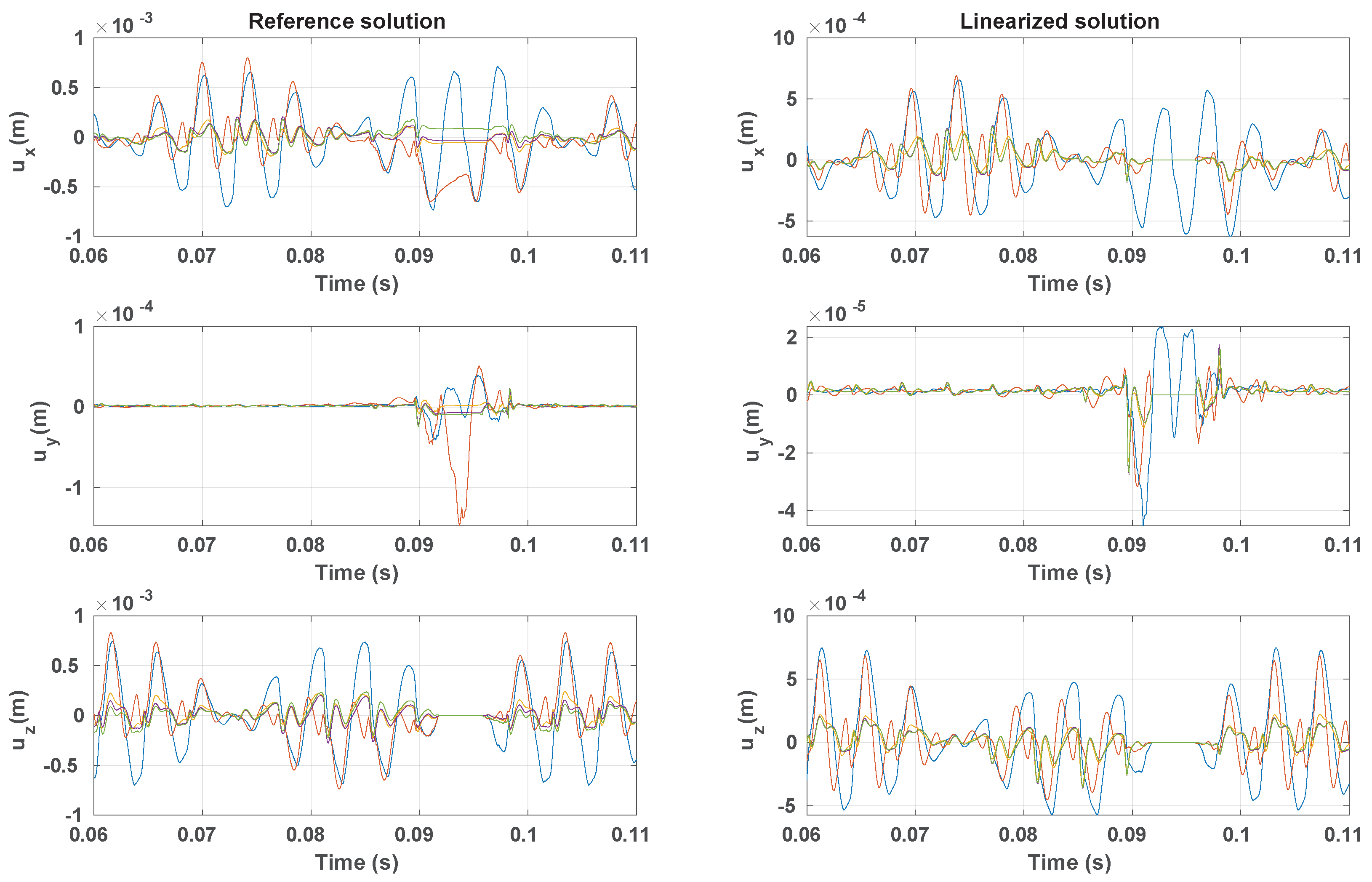
3.3.2. Coefficient of Friction Impact
4. Conclusions
- First of all, it can be noted that damping has been neglected in modeling; however, experiments show that vibration energy is not transported to the opposite of contact patch due to strong damping of tire structure for such relatively high frequencies. The vibration energy is thus concentrated close to the entrance and the exit of contact patch. Moreover the vibrations evolution in the circumferential direction shows the opposite observation; therefore, the addition of a suitable damping model seems important and is one of the priority issues to be explored. The inclusion of the Kelvin–Voigt model, often used to treat the viscosity of tire rubber, seems to be an interesting option [28].
- In a similar way, the modeling of friction at the contact interface should be completed by the consideration of the coefficient of rolling friction [29] in order to reproduce more realistic physical phenomena involved in tire/pavement interaction vibration and noise. Indeed, the consideration of only the coefficient of sliding friction force as proposed in this study may be not sufficient to accurately reproduce real phenomena of tire vibration response.
- It should be interesting to apply the proposed two-step approach on a real industrial tire structure and to compare the results with full experimental tests. One of the most important challenges may be to identify the different sources of nonlinear vibrations in order to identify and quantify the first-order physical phenomena involved. In this context, more realistic modeling including both tire rubber viscosity and Hertzian contact for polymorph materials or the model of contact 3D multi-layered structures with orthotropic material should be developed to reproduce more specifically the design of a real wheel tire.
- Extension of the prediction of non-linear vibrations in a real environment and in real conditions would also be interesting with the consideration of a more realistic pavement with imperfections. The road roughness excitation mechanism could be added to the proposed methodology by integrating the road texture in the normal gap function calculation. In this context, one of the main challenges for engineers working on the developments of optimization procedures for tire structures in a real environment should be the inclusions of modeling errors and uncertainties.
- The developments and combination of optimization procedures, genetic algorithms or artificial neural in relation with the proposed methodology should also be of prior interest to conduct parametric studies leading to increase the reliability and safety of tire structures subjected to complex and various external excitations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Reference configuration | |
| Current configuration | |
| Coordinates of reference configuration | |
| Coordinates of current configuration | |
| Dirichlet boundary condition zone in the reference configuration | |
| Dirichlet boundary condition zone in the current configuration | |
| Neumann boundary condition zone in the reference configuration | |
| Neumann boundary condition zone in the current configuration | |
| Potential contact zone | |
| Contact active zone | |
| Mapping of reference to current configuration | |
| Displacement field | |
| ∇ | Gradient with respect to |
| Identity second order tensor | |
| Deformation gradient | |
| Cauchy-Green tensor | |
| Green-Lagrange strain tensor | |
| Cauchy stress tensor | |
| First Piola-Kirchhoff stress tensor | |
| Second Piola-Kirchhoff stress tensor | |
| W | Strain energy function |
| Fourth order elasticity tensor | |
| Unit outward normal in the reference configuration | |
| Unit outward normal in the current configuration | |
| Normal contact pressure | |
| Tangential stress vector | |
| Normal displacement | |
| Tangential displacement | |
| Normal gap function | |
| Sliding direction | |
| Coulomb friction coefficient | |
| Sliding velocity | |
| V | Translation velocity |
| Rotation speed | |
| Rolling radius | |
| d | Deflection |
| Normal penalization | |
| Regularization coefficient | |
| Time step | |
| External radius of the prototype | |
| Internal radius of the prototype | |
| w | Width of the prototype |
| Lamé constants | |
| E | Young Modulus |
| Poisson coefficient | |
| Density | |
| First order Sobolev space |
References
- Sandberg, U.; Ejsmont, J.A. Tyre/Road Noise Reference Book; Informex: Kisa, Sweden, 2002. [Google Scholar]
- Li, T. Influencing parameters on tire–pavement interaction noise: Review, experiments and design considerations. Designs 2018, 2, 38. [Google Scholar] [CrossRef] [Green Version]
- Li, T.; Burdisso, R.; Sandu, C. Literature review of models on tire-pavement interaction noise. J. Sound Vib. 2018, 2, 357–445. [Google Scholar] [CrossRef]
- Ling, S.; Yu, F.; Sun, D.; Sun, G.; Xu, L. A comprehensive review of tire-pavement noise: Generation mechanism, measurement methods, and quiet asphalt pavement. J. Clean. Prod. 2021, 287, 125056. [Google Scholar] [CrossRef]
- Nackenhorst, U. The ALE-formulation of bodies in rolling contact: Theoretical foundations and finite element approach. Comput. Methods Appl. Mech. Eng. 2004, 193, 4299–4322. [Google Scholar] [CrossRef]
- Brinkmeier, M.; Nackenhorst, U.; Volk, H. A Finite Element Approach to the Transient Dynamics of Rolling Tires with Emphasis on Rolling Noise Simulation. Tire Sci. Technol. 2007, 35, 165–182. [Google Scholar] [CrossRef]
- De Gregoriis, D.; Naets, F.; Kindt, P.; Desmet, W. Development and validation of a fully predictive high-fidelity simulation approach for predicting coarse road dynamic tire/road rolling contact forces. J. Sound Vib. 2019, 452, 147–168. [Google Scholar] [CrossRef]
- Gonzalez Diaz, C.; Kindt, P.; Middelberg, J.; Vercammen, S.; Thiry, C.; Close, R.; Leyssens, J. Dynamic behaviour of a rolling tyre: Experimental and numerical analyses. J. Sound Vib. 2016, 364, 147–164. [Google Scholar] [CrossRef]
- Pinay, J.; Saito, Y.; Mignot, C.; Gauterin, F. Understanding the contribution of groove resonance to tire-road noise on different surfaces under various operating conditions. Acta Acust. 2020, 4, 1–12. [Google Scholar] [CrossRef]
- Ejsmont, J.A.; Sandberg, U.; Taryma, S. Influence of tread pattern on tire/road noise. SAE Transactions 1984, 93, 632–640. [Google Scholar] [CrossRef]
- Li, T. Influence of tread pattern on tire/road noise. Automot. Tire Noise Vib. 2020, 27–41. [Google Scholar] [CrossRef]
- Hauret, P. Méthodes Numériques Pour la Dynamique des Structures non Linéaires Incompressibles à Deux Échelles. Ph.D Thesis, Ecole Polytechnique, Palaiseau, France, 2004. [Google Scholar]
- Gonzalez, O. Exact energy and momentum conserving algorithms for general models in nonlinear elasticity. Comput. Methods Appl. Mech. Eng. 2000, 190, 1763–1783. [Google Scholar] [CrossRef] [Green Version]
- Biot, M.A. Mechanics of Incremental Deformations; John Wiley & Sons, Inc.: New York, NY, USA; London, UK; Sydney, Australia, 1965. [Google Scholar] [CrossRef] [Green Version]
- Valyaev, V. On approaches to simulation of rolling tire vibrations. Michelin Intern. Rep. 2016. [Google Scholar]
- Ogden, R.W. Non-Linear Elastic Deformations; Dover Publications Inc.: Mineola, NY, USA, 1997. [Google Scholar]
- Signorini, A. Questioni di elasticita non linearizzata e semilinearizzata. Rend. Mat. Delle Sue Appl. 1959, 18, 95–139. [Google Scholar]
- Yastrebov, V. Computational Contact Mechanics: Geometry, Detection and Numerical Techniques. Ph.D Thesis, École Nationale Supérieure des Mines de Paris, Paris, France, 2011. [Google Scholar]
- Wriggers, P. Constitutive Equations for Contact Interfaces. In Computational Contact Mechanics; Springer: Berlin/Heidelberg, Germany, 2006; pp. 69–108. [Google Scholar] [CrossRef]
- Simo, J.C.; Laursen, T.A. An augmented lagrangian treatment of contact problems involving friction. Comput. Struct. 1992, 42, 97–116. [Google Scholar] [CrossRef]
- Fortin, A.; Garon, A. Les Éléments Finis: De la Théorie à la Pratique. GIREF. 2020. Available online: https://giref.ulaval.ca/afortin/elements_finis.pdf (accessed on 14 December 2021).
- Winroth, J.; Andersson, P.; Kropp, W. Importance of tread inertia and damping on the tyre/road contact stiffness. J. Sound Vib. 2014, 333, 5378–5385. [Google Scholar] [CrossRef] [Green Version]
- Jazar, R.N. Tire Dynamics. Veh. Dyn. 2014, 95–163. [Google Scholar] [CrossRef]
- Chipato, E.T.; Shaw, A.D.; Friswell, M.I. Nonlinear rotordynamics of a MDOF rotor-stator contact system subjected to frictional and gravitational effects. Mech. Syst. Signal Process. 2021, 159, 107776. [Google Scholar] [CrossRef]
- Zhou, P.; Du, M.; Chen, S.; He, Q.; Peng, Z.; Zhang, W. Study on intra-wave frequency modulation phenomenon in detection of rub-impact fault. Mech. Syst. Signal Process. 2019, 122, 342–363. [Google Scholar] [CrossRef]
- Mlika, R.; Renard, Y.; Chouly, F. An unbiased Nitsche’s formulation of large deformation frictional contact and self-contact. Comput. Methods Appl. Mech. Eng. 2017, 325, 265–288. [Google Scholar] [CrossRef] [Green Version]
- Sundaram, N.; Farris, T. Mechanics of advancing pin-loaded contacts with friction. J. Mech. Phys. Solids 2010, 58, 1819–1833. [Google Scholar] [CrossRef]
- Scaraggi, M.; Persson, B. Friction and universal contact area law for randomly rough viscoelastic contacts. J. Phys. Condens. Matter 2015, 27, 105102. [Google Scholar] [CrossRef] [PubMed]
- Alaci, S.; Muscă, I.; Pentiuc, Ș.G. Study of the Rolling Friction Coefficient between Dissimilar Materials through the Motion of a Conical Pendulum. Materials 2020, 13, 5032. [Google Scholar] [CrossRef] [PubMed]
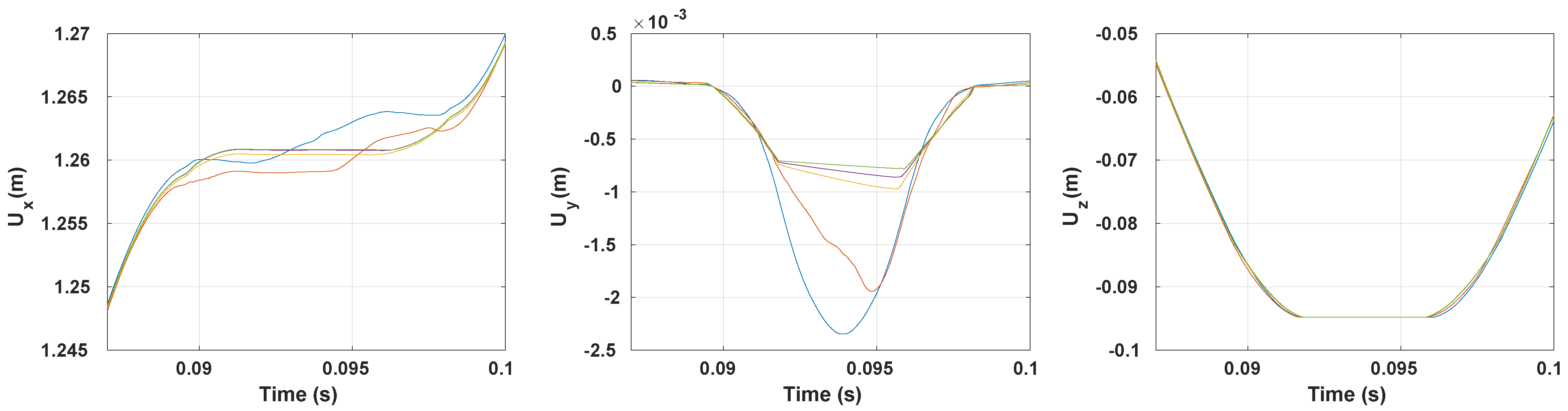
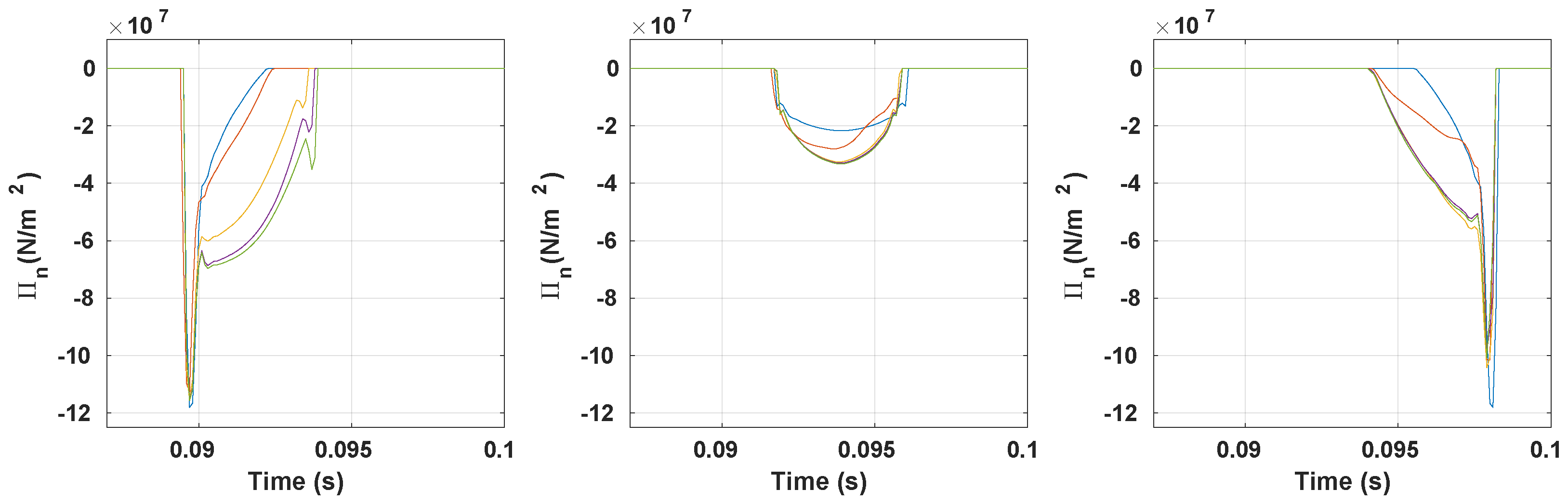
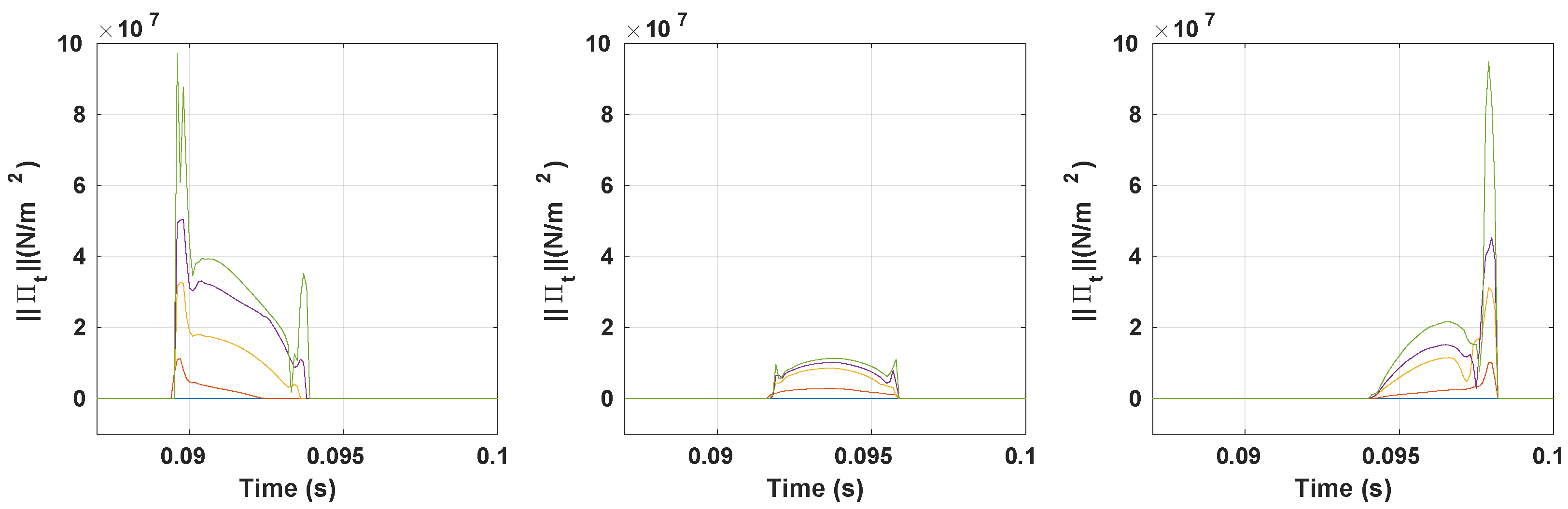
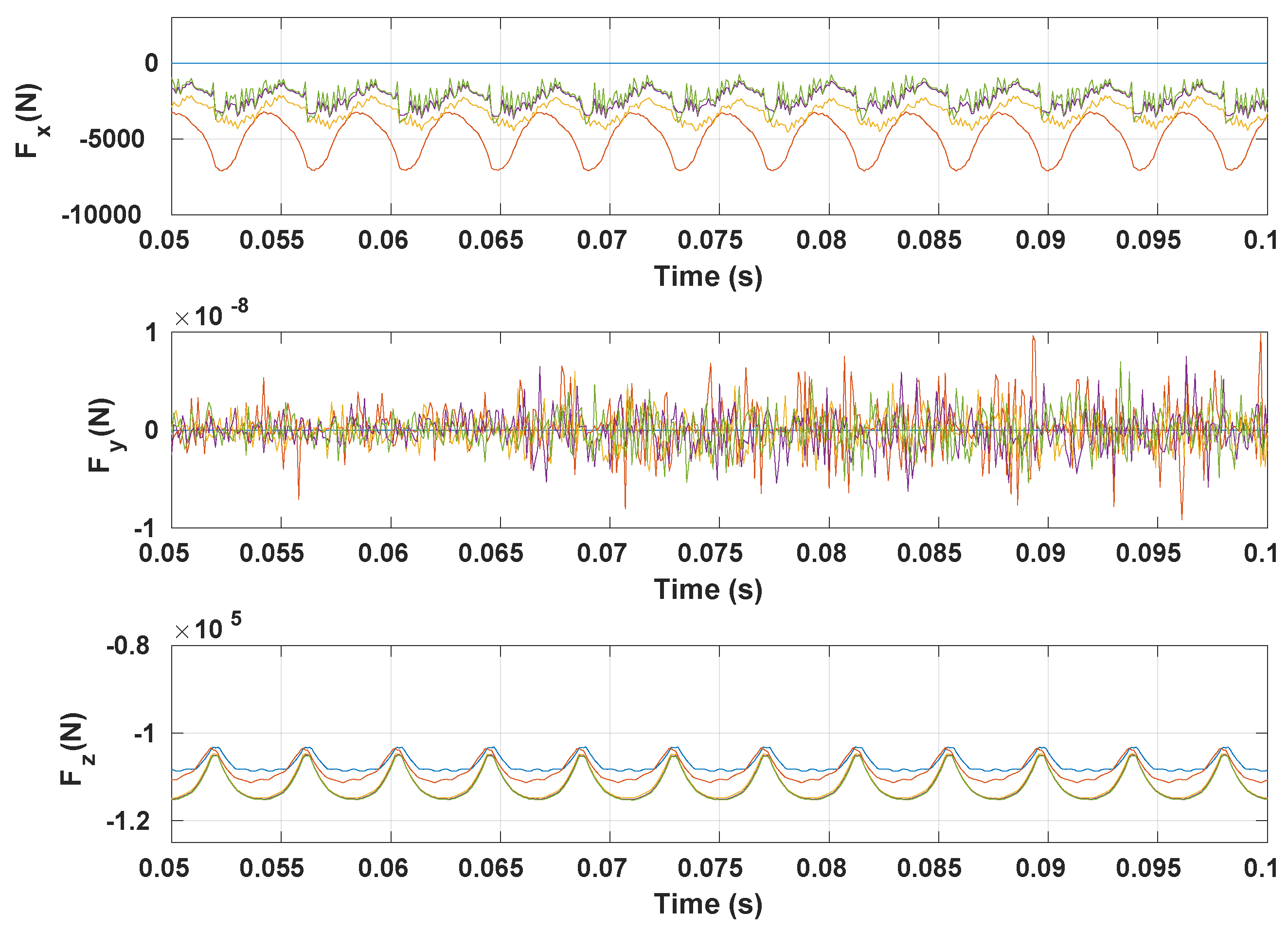


| External radius | m |
| Internal radius | m |
| Width | m |
| Young Modulus | Pa |
| Poisson coefficient | |
| Density | kg/m3 |
| Deflection | m |
| Rotation speed | rad/s |
| Normal penalization | |
| Regularization coefficient | |
| Time step | s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Knar, Z.; Sinou, J.-J.; Besset, S.; Clauzon, V. An Adapted Two-Steps Approach to Simulate Nonlinear Vibrations of Solid Undergoing Large Deformation in Contact with Rigid Plane—Application to a Grooved Cylinder. Appl. Sci. 2022, 12, 1447. https://doi.org/10.3390/app12031447
Knar Z, Sinou J-J, Besset S, Clauzon V. An Adapted Two-Steps Approach to Simulate Nonlinear Vibrations of Solid Undergoing Large Deformation in Contact with Rigid Plane—Application to a Grooved Cylinder. Applied Sciences. 2022; 12(3):1447. https://doi.org/10.3390/app12031447
Chicago/Turabian StyleKnar, Zakaria, Jean-Jacques Sinou, Sébastien Besset, and Vivien Clauzon. 2022. "An Adapted Two-Steps Approach to Simulate Nonlinear Vibrations of Solid Undergoing Large Deformation in Contact with Rigid Plane—Application to a Grooved Cylinder" Applied Sciences 12, no. 3: 1447. https://doi.org/10.3390/app12031447
APA StyleKnar, Z., Sinou, J.-J., Besset, S., & Clauzon, V. (2022). An Adapted Two-Steps Approach to Simulate Nonlinear Vibrations of Solid Undergoing Large Deformation in Contact with Rigid Plane—Application to a Grooved Cylinder. Applied Sciences, 12(3), 1447. https://doi.org/10.3390/app12031447







