A FE-Based Macro-Element for the Assessment of Masonry Structures: Linear Static, Vibration, and Non-Linear Cyclic Analyses
Abstract
Featured Application
Abstract
1. Introduction
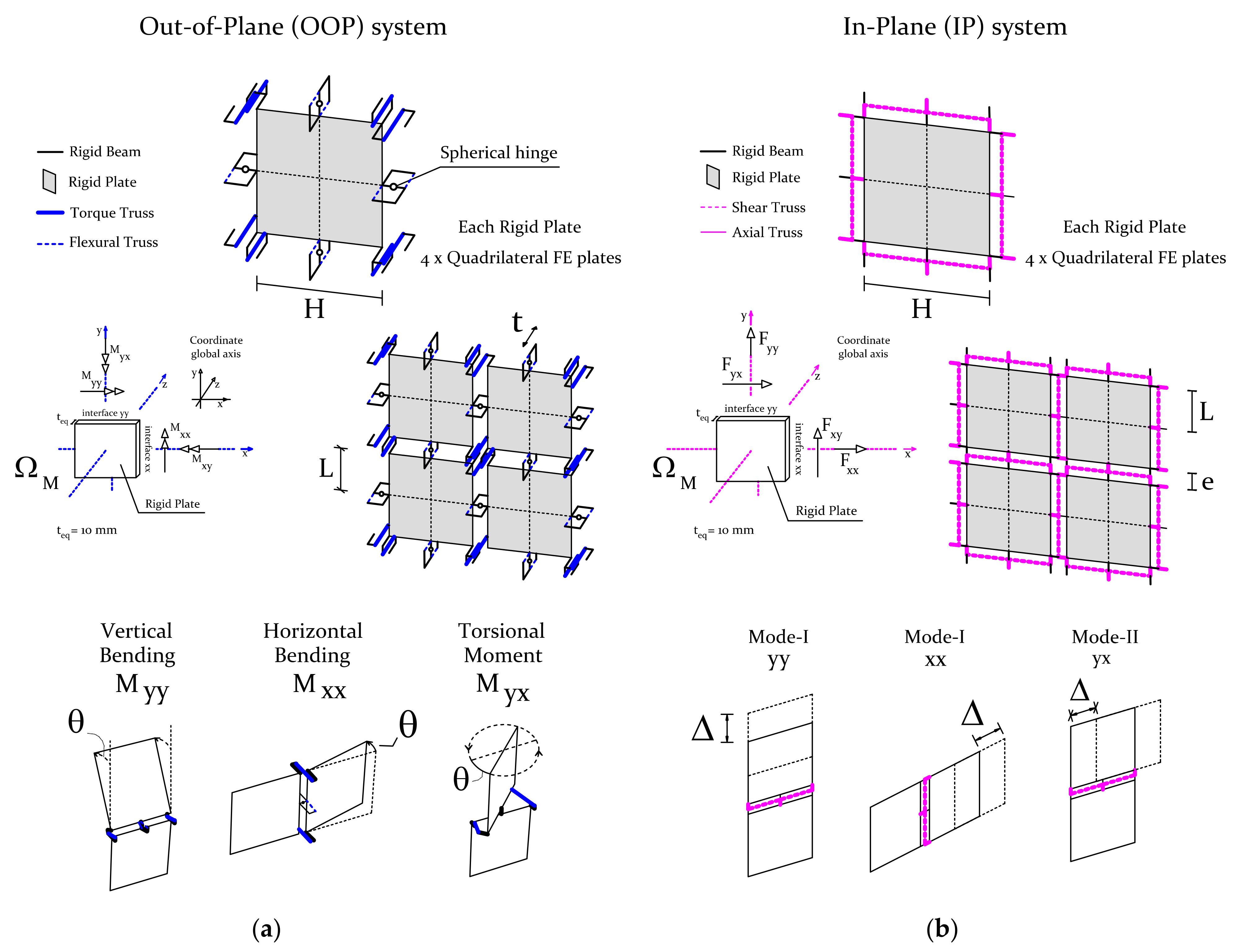
2. Macroscopic Unit–Cell
2.1. Theoretical Scope
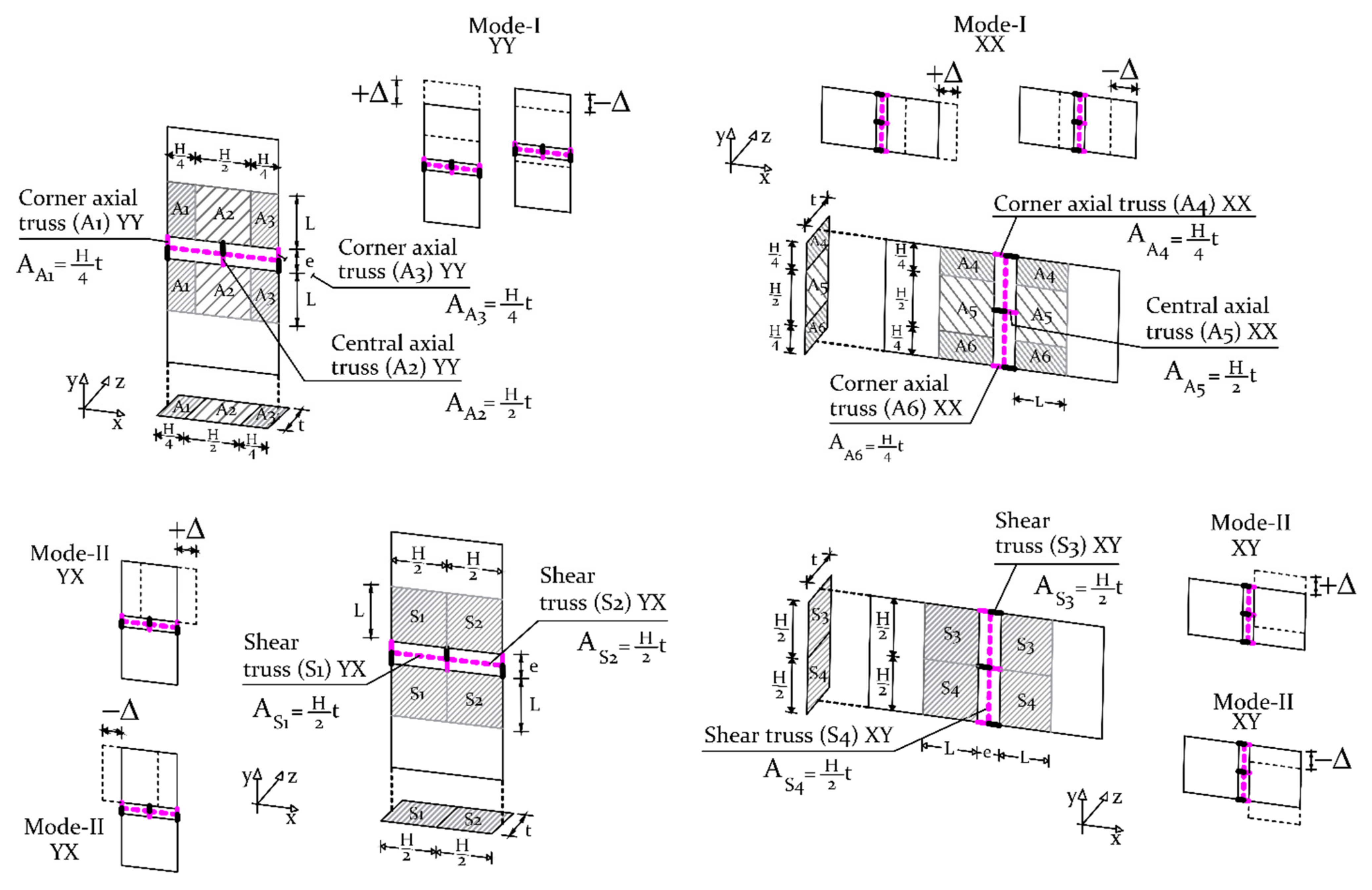
2.2. In–Plane Kinematics
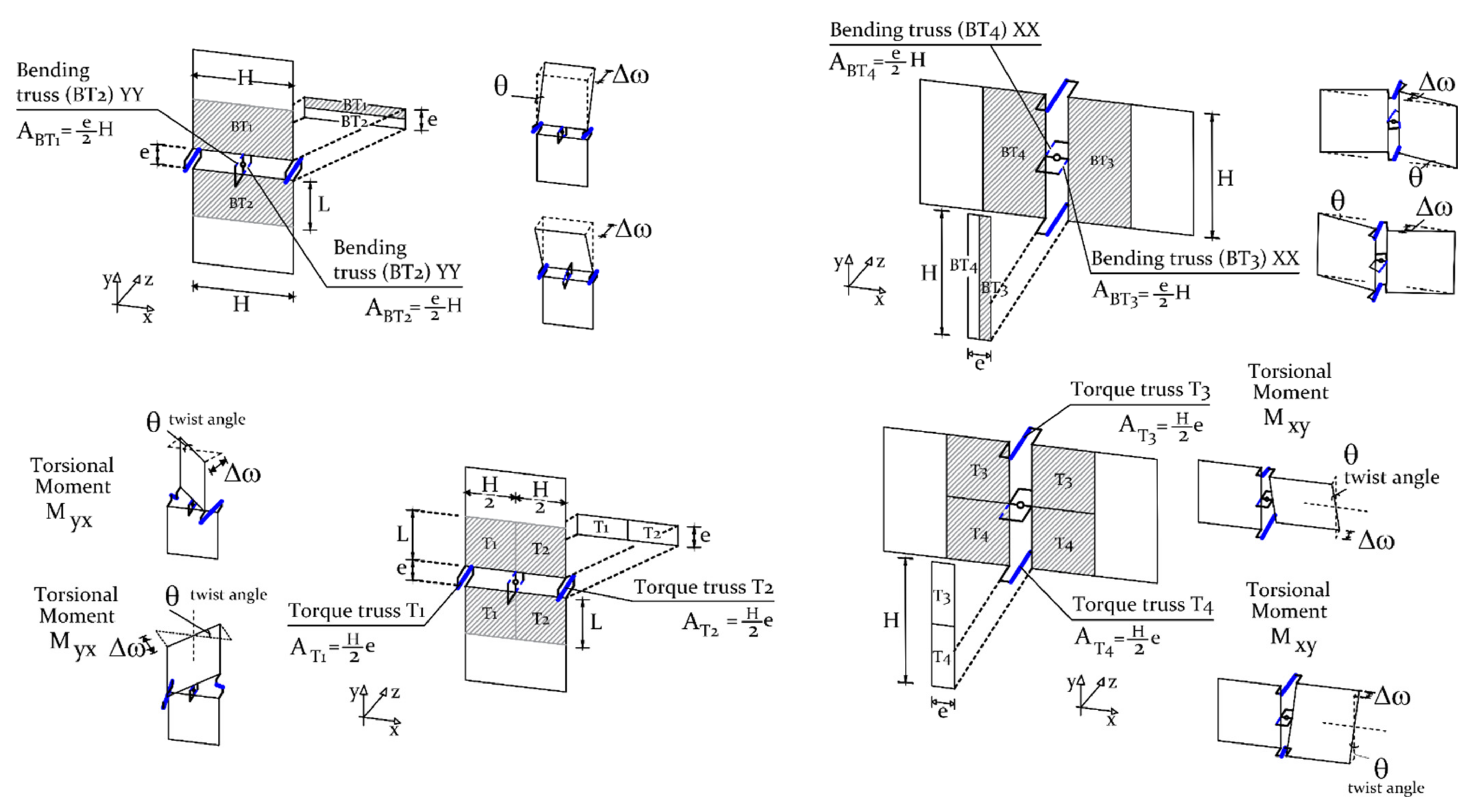
2.3. Out–of–Plane Kinematics
2.4. Material Constitutive Law and Damage Model
2.5. Material Information and Required Processing Steps
3. Macro–Element Application
3.1. Linear Range
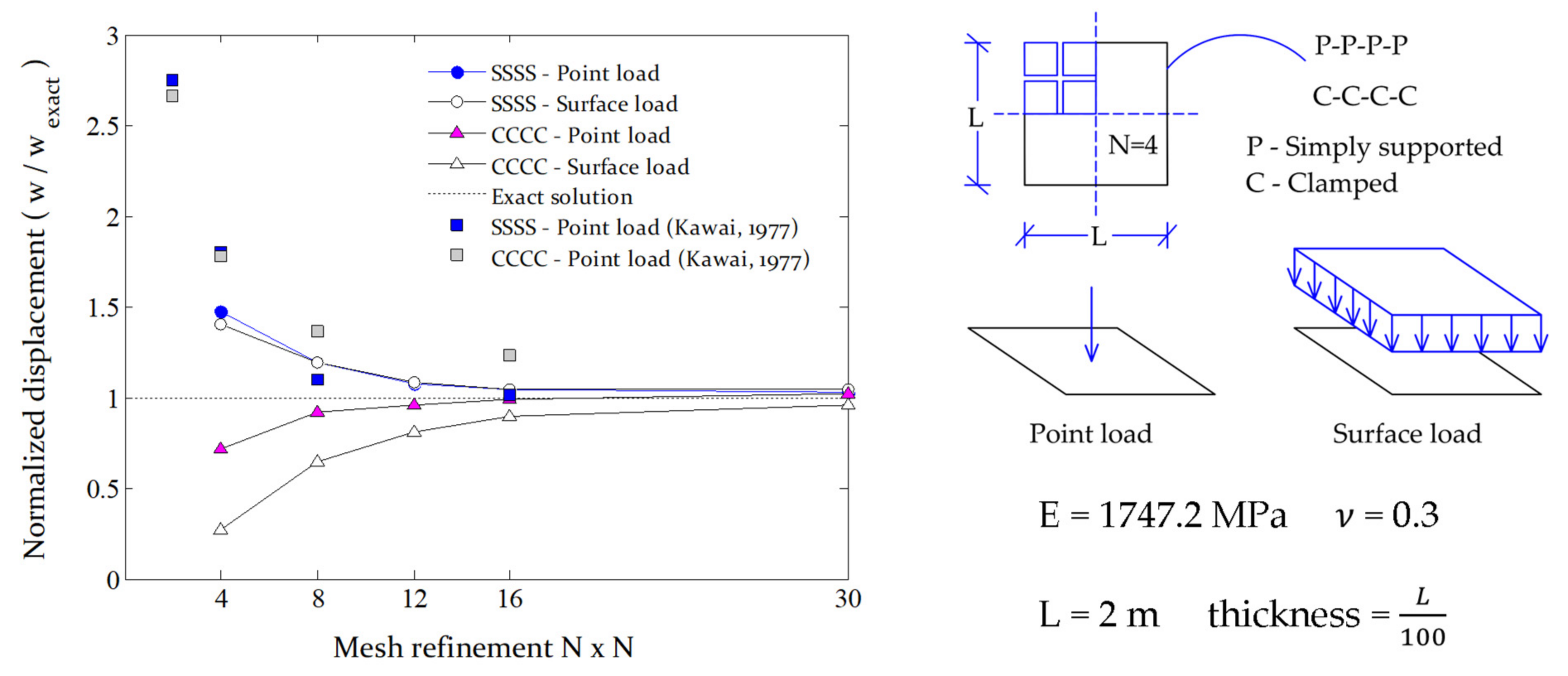
3.1.1. Elasticity Problems
3.1.2. Vibration Analysis
3.2. Non–Linear Range
3.2.1. Quasi–Static (Monotonic) Nonlinear Curve
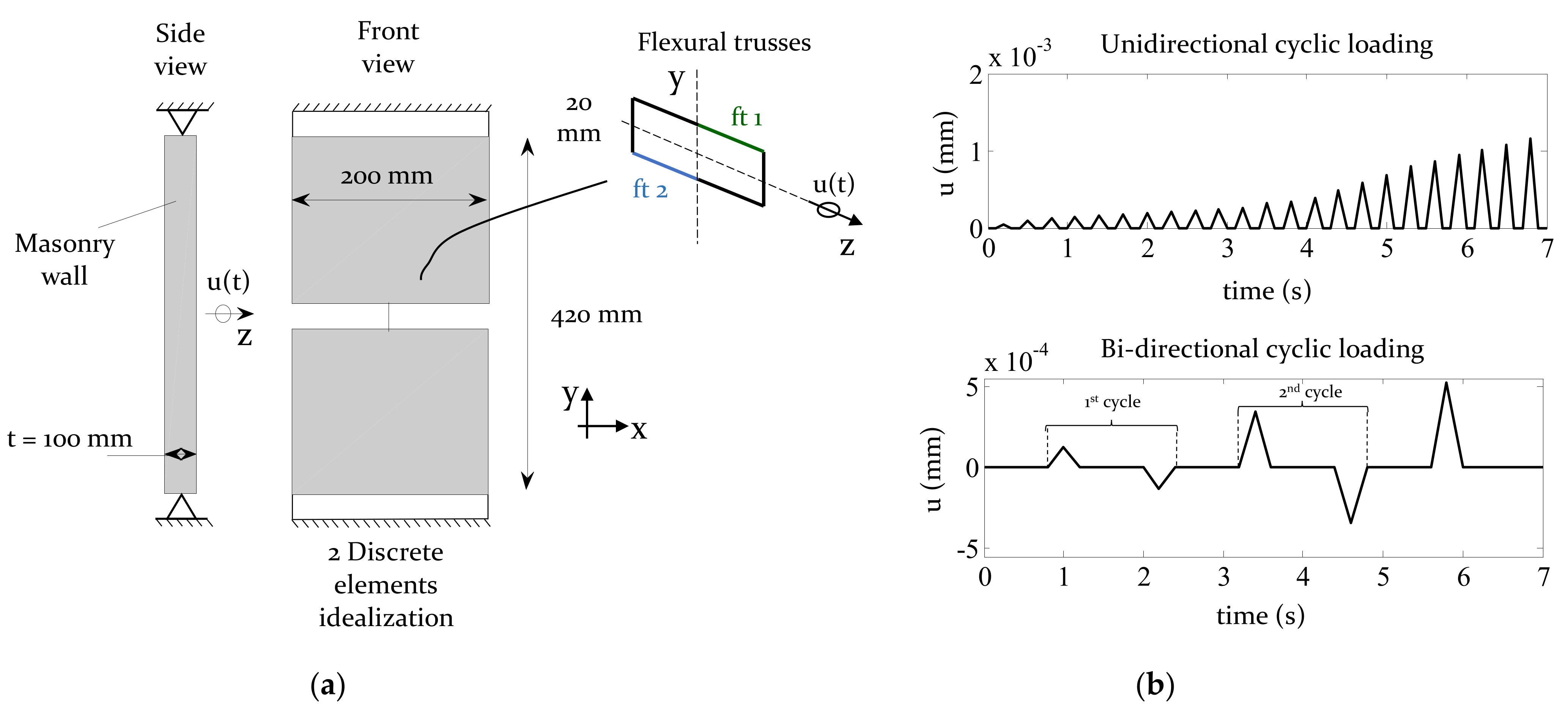
3.2.2. Uni–Directional Cyclic Loading
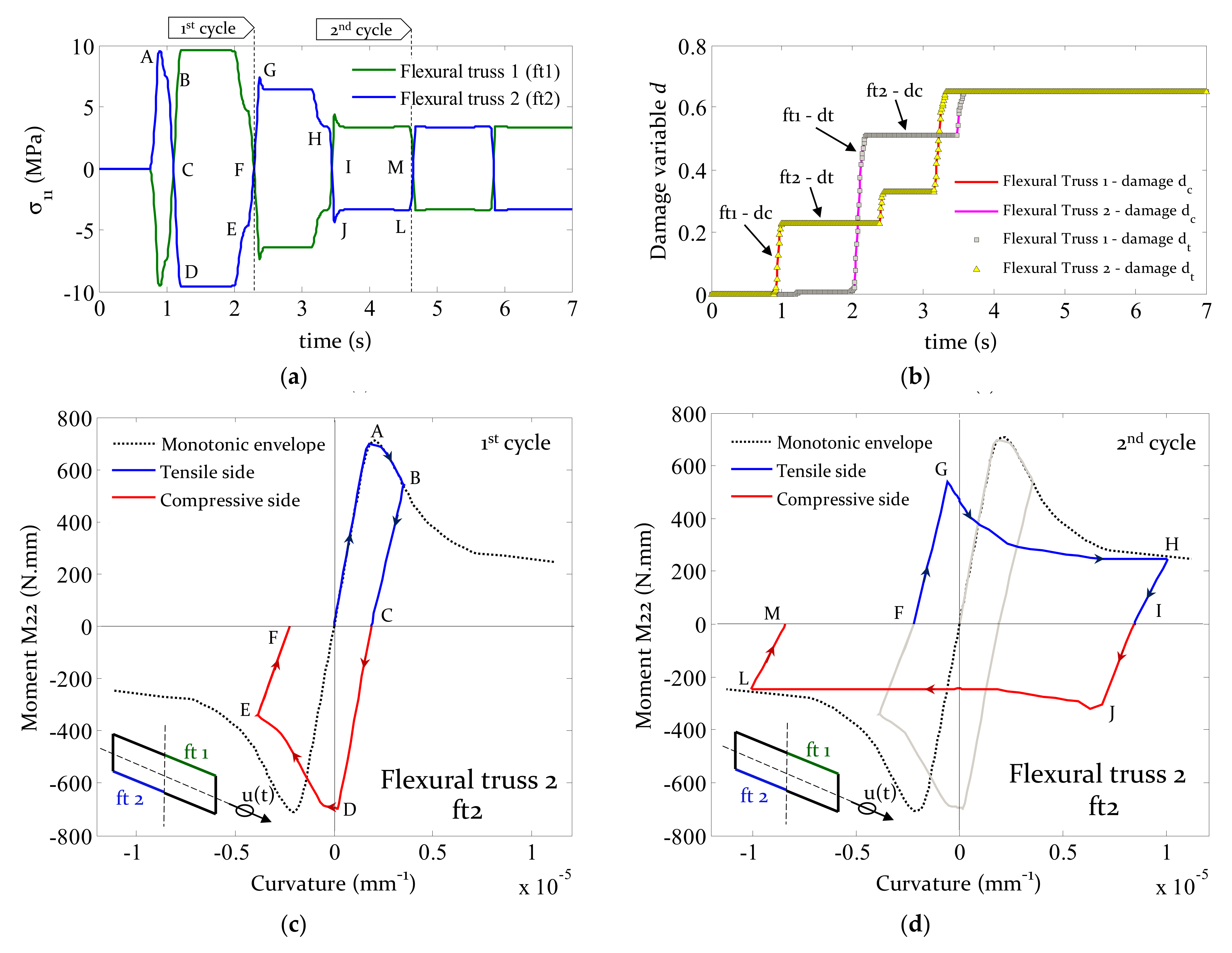
3.2.3. Bi–Directional Cyclic Loading
4. Computational Features and CPU Parallelisation
4.1. Node Renumbering Algorithm
4.2. Implicit vs. Explicit FE Analysis
4.3. Comment on the Computational Attractiveness of the (Discrete) Macro–Element
5. Final Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Giamundo, V.; Sarhosis, V.; Lignola, G.P.; Sheng, Y.; Manfredi, G. Evaluation of different computational modelling strategies for the analysis of low strength masonry structures. Eng. Struct. 2014, 73, 160–169. [Google Scholar] [CrossRef]
- de Felice, G.; De Santis, S.; Lourenço, P.B.; Mendes, N. Methods and Challenges for the Seismic Assessment of Historic Masonry Structures. Int. J. Archit. Herit. 2017, 11, 143–160. [Google Scholar] [CrossRef]
- Sacco, E.; Addessi, D.; Sab, K. New trends in mechanics of masonry. Meccanica 2018, 53, 1565–1569. [Google Scholar] [CrossRef]
- Lourenço, P.B.; Silva, L.C. Computational applications in masonry structures: From the meso–scale to the super-large/super-complex. Int. J. Multiscale Comput. Eng. 2020, 18, 1–30. [Google Scholar] [CrossRef]
- Roca, P.; Cervera, M.; Gariup, G.; Pela’, L. Structural Analysis of Masonry Historical Constructions. Classical and Advanced Approaches. Arch. Comput. Methods Eng. 2010, 17, 299–325. [Google Scholar] [CrossRef]
- Theodossopoulos, D.; Sinha, B. A review of analytical methods in the current design processes and assessment of performance of masonry structures. Constr. Build. Mater. 2013, 41, 990–1001. [Google Scholar] [CrossRef]
- Tomaževič, M. Earthquake-Resistant Design of Masonry Buildings; World Scientific: London, UK, 1999; Volume 1. [Google Scholar]
- Lagomarsino, S.; Penna, A.; Galasco, A.; Cattari, S. TREMURI program: An equivalent frame model for the nonlinear seismic analysis of masonry buildings. Eng. Struct. 2013, 56, 1787–1799. [Google Scholar] [CrossRef]
- Quagliarini, E.; Maracchini, G.; Clementi, F. Uses and limits of the Equivalent Frame Model on existing unreinforced masonry buildings for assessing their seismic risk: A review. J. Build. Eng. 2017, 10, 166–182. [Google Scholar] [CrossRef]
- Addessi, D.; Liberatore, D.; Masiani, R. Force-Based Beam Finite Element (FE) for the Pushover Analysis of Masonry Buildings. Int. J. Archit. Herit. 2015, 9, 231–243. [Google Scholar] [CrossRef]
- Brencich, A.; Lagomarsino, S. A macroelement dynamic model for masonry shear walls. Comput. Methods Struct. Mason. 1998, 4, 67–75. [Google Scholar]
- Penna, A.; Lagomarsino, S.; Galasco, A. A nonlinear macroelement model for the seismic analysis of masonry buildings. Earthq. Eng. Struct. Dyn. 2014, 43, 159–179. [Google Scholar] [CrossRef]
- Vanin, F.; Penna, A.; Beyer, K. A three-dimensional macroelement for modelling the in-plane and out-of-plane response of masonry walls. Earthq. Eng. Struct. Dyn. 2020, 49, 1365–1387. [Google Scholar] [CrossRef]
- Sangirardi, M.; Liberatore, D.; Addessi, D. Equivalent Frame Modelling of Masonry Walls Based on Plasticity and Damage. Int. J. Archit. Herit. 2019, 13, 1098–1109. [Google Scholar] [CrossRef]
- Liberatore, D.; Addessi, D. Strength domains and return algorithm for the lumped plasticity equivalent frame model of masonry structures. Eng. Struct. 2015, 91, 167–181. [Google Scholar] [CrossRef]
- D’Ayala, D.; Shi, Y. Modelling Masonry Historic Buildings by Multi-Body Dynamics. Int. J. Archit. Herit. 2011, 5, 483–512. [Google Scholar] [CrossRef]
- Konstantinidis, D.; Makris, N. The dynamics of a rocking block in three dimensions. In Proceedings of the 8th HSTAM International Congress on Mechanics Mech, Patras, Greece, 12–14 July 2007; pp. 12–14. [Google Scholar]
- D’Ayala, D.; Speranza, E. Definition of Collapse Mechanisms and Seismic Vulnerability of Historic Masonry Buildings. Earthq. Spectra 2003, 19, 479–509. [Google Scholar] [CrossRef]
- Griffith, M.; Magenes, G. Evaluation of out-of-plane stability of unreinforced masonry walls subjected to seismic excitation. J. Earthq. Eng. 2003, 7, 141–169. [Google Scholar] [CrossRef]
- Calvi, G.M.; Pinho, R.; Magenes, G.; Bommer, J.J.; Restrepo-Vélez, L.F.; Crowley, H. Development of seismic vulnerability assessment methodologies over the past 30 years. ISET J. Eathquake Technol. 2006, 43, 75–104. [Google Scholar]
- Funari, M.F.; Mehrotra, A.; Lourenço, P.B. A tool for the rapid seismic assessment of historic masonry structures based on limit analysis optimisation and rocking dynamics. Appl. Sci. 2021, 11, 942. [Google Scholar] [CrossRef]
- Funari, M.F.; Spadea, S.; Lonetti, P.; Fabbrocino, F.; Luciano, R. Visual programming for structural assessment of out-of-plane mechanisms in historic masonry structures. J. Build. Eng. 2020, 31, 101425. [Google Scholar] [CrossRef]
- Baraldi, D.; Reccia, E.; Cecchi, A. In plane loaded masonry walls: DEM and FEM/DEM models. A critical review. Meccanica 2018, 53, 1613–1628. [Google Scholar] [CrossRef]
- Cundall, P.; Hart, P. A computer model for simulating progressive large scale movements in blocky rock systems. Int. Soc. Rock Mech. (ISRM) 1971, 1, 28. [Google Scholar]
- Shi, G.H.; Goodman, R.E. Discontinuous deformation analysis—A new method forcomputing stress, strain and sliding of block systems. In Proceedings of the 29th U.S. Symposium on Rock Mechanics (USRMS), Minneapolis, MN, USA, 13–15 June 1988. [Google Scholar]
- Lemos, J.V. Discrete Element Modelling of Masonry Structures. Int. J. Archit. Herit. 2007, 1, 190–213. [Google Scholar] [CrossRef]
- Gilbert, M.; Melbourne, C. Rigid-Block Analysis of Masonry Structures; Institution of Structural Engineers: London, UK, 1994; Volume 72. [Google Scholar]
- Baggio, C.; Trovalusci, P. Stone assemblies under in-plane actions. Comparison between nonlinear discrete approaches. Comput Methods Struct. Mason. 1995, 3, 184–193. [Google Scholar]
- Sarhosis, V.; Bagi, K.; Lemos, J.V.; Milani, G. (Eds.) Computational Modelling of Masonry Structures Using the Discrete Element Method; IGI Global: Hershey, PA, USA, 2016; ISBN 1522502319. [Google Scholar]
- Schlegel, R.; Rautenstrauch, K. Failure analysis of masonry shear walls. In Numererical Modeling of Discrete Materials; Taylor and Francis Group: London, UK, 2004; pp. 15–18. [Google Scholar]
- Lemos, J.; Costa, A.; Bretas, E. Assessment of the seismic capacity of stone masonry walls with block models. In Computational Methods in Earthquake Engineering; Springer: Dordrecht, The Netherlands, 2011; pp. 221–235. [Google Scholar]
- Malomo, D.; DeJong, M.J. A Macro-Distinct Element Model (M-DEM) for out-of-plane analysis of unreinforced masonry structures. Eng. Struct. 2021, 244, 112754. [Google Scholar] [CrossRef]
- Pulatsu, B.; Erdogmus, E.; Lourenço, P.B.; Lemos, J.V.; Tuncay, K. Simulation of the in-plane structural behaviour of unreinforced masonry walls and buildings using DEM. Structures 2020, 27, 2274–2287. [Google Scholar] [CrossRef]
- Peña, F.; Prieto, F.; Lourenço, P.B.; Costa, A.C.; Lemos, J.V. On the dynamics of rocking motion of single rigid–block structures. Earthq. Eng. Struct. Dyn. 2007, 36, 2383–2399. [Google Scholar] [CrossRef]
- Itasca UDEC—Universal Distinct Element Code; Itasca Consulting Group Inc.: Minneapolis, MN, USA, 2004.
- Cascini, L.; Gagliardo, R.; Portioli, F. LiABlock_3D: A Software Tool for Collapse Mechanism Analysis of Historic Masonry Structures. Int. J. Archit. Herit. 2018, 14, 75–94. [Google Scholar] [CrossRef]
- Giambanco, G.; Rizzo, S.; Spallino, R. Numerical analysis of masonry structures via interface models. Comput. Methods Appl. Mech. Eng. 2001, 190, 6493–6511. [Google Scholar] [CrossRef]
- Macorini, L.; Izzuddin, B.A. A non-linear interface element for 3D mesoscale analysis of brick-masonry structures. Int. J. Numer. Methods Eng. 2011, 85, 1584–1608. [Google Scholar] [CrossRef]
- Lotfi, H.R.; Shing, P.B. Interface Model Applied to Fracture of Masonry Structures. J. Struct. Eng. 1994, 120, 63–80. [Google Scholar] [CrossRef]
- Macorini, L.; Izzuddin, B.A. Nonlinear analysis of masonry structures using mesoscale partitioned modelling. Adv. Eng. Softw. 2013, 60–61, 58–69. [Google Scholar] [CrossRef]
- Sejnoha, J.; Sejnoha, M.; Zeman, J.; Sykora, J.; Vorel, J. A mesoscopic study on historic masonry. Struct. Eng. Mech. 2008, 30, 99–117. [Google Scholar] [CrossRef]
- Sarhosis, V.; Tsavdaridis, K.; Giannopoulos, I. Discrete Element Modelling (DEM) for Masonry Infilled Steel Frames with Multiple Window Openings Subjected to Lateral Load Variations. Open Constr. Build. Technol. J. 2014, 8, 93–103. [Google Scholar] [CrossRef]
- Adam, J.M.; Brencich, A.; Hughes, T.G.; Jefferson, T. Micromodelling of eccentrically loaded brickwork: Study of masonry wallettes. Eng. Struct. 2010, 32, 1244–1251. [Google Scholar] [CrossRef]
- Dauda, J.A.; Silva, L.C.; Lourenço, P.B.; Iuorio, O. Out-of-plane loaded masonry walls retrofitted with oriented strand boards: Numerical analysis and influencing parameters. Eng. Struct. 2021, 243, 112683. [Google Scholar] [CrossRef]
- Silva, L.C.; Lourenço, P.B.; Milani, G. Derivation of the out-of-plane behaviour of masonry through homogenization strategies: Micro-scale level. Comput. Struct. 2018, 209, 30–43. [Google Scholar] [CrossRef]
- Aşıkoğlu, A.; Avşar, Ö.; Lourenço, P.B.; Silva, L.C. Effectiveness of seismic retrofitting of a historical masonry structure: Kütahya Kurşunlu Mosque, Turkey. Bull. Earthq. Eng. 2019, 17, 3365–3395. [Google Scholar] [CrossRef]
- Ciocci, M.P.; Sharma, S.; Lourenço, P.B. Engineering simulations of a super-complex cultural heritage building: Ica Cathedral in Peru. Meccanica 2018, 53, 1931–1958. [Google Scholar] [CrossRef]
- Reccia, E.; Leonetti, L.; Trovalusci, P.; Cecchi, A. A multiscale/multidomain model for the failure analysis of masonry walls: A validation with a combined FEM/DEM approach. Int. J. Multiscale Comput. Eng. 2018, 16, 325–343. [Google Scholar] [CrossRef]
- Tiberti, S.; Milani, G. 2D pixel homogenized limit analysis of non-periodic masonry walls. Comput. Struct. 2019, 219. [Google Scholar] [CrossRef]
- Trovalusci, P.; Ostoja-Starzewski, M.; De Bellis, M.L.; Murrali, A. Scale-dependent homogenization of random composites as micropolar continua. Eur. J. Mech.—A/Solids 2015, 49, 396–407. [Google Scholar] [CrossRef]
- Addessi, D.; Sacco, E.; Paolone, A. Cosserat model for periodic masonry deduced by nonlinear homogenization. Eur. J. Mech—A/Solids 2010, 29, 724–737. [Google Scholar] [CrossRef]
- Šejnoha, M.; Janda, T.; Vorel, J.; Kucíková, L.; Padevěd, P. Combining Homogenization, Indentation and Bayesian Inference in Estimating the Microfibril Angle of Spruce. Procedia Eng. 2017, 190, 310–317. [Google Scholar] [CrossRef]
- Otero, F.; Oller, S.; Martinez, X.; Salomón, O. Numerical homogenization for composite materials analysis. Comparison with other micro mechanical formulations. Compos. Struct. 2015, 122, 405–416. [Google Scholar] [CrossRef]
- Driesen, C.; Degée, H.; Vandoren, B. Efficient modelling of masonry failure using a multiscale domain activation approach. Comput. Struct. 2021, 251, 106543. [Google Scholar] [CrossRef]
- Funari, M.F.; Silva, L.C.; Savalle, N.; Lourenço, P.B. A concurrent micro/macro FE-model optimized with a limit analysis tool for the assessment of dry-joint masonry structures. Int. J. Multiscale Comput. Eng. 2022. [Google Scholar] [CrossRef]
- Maria D’Altri, A.; Lo Presti, N.; Grillanda, N.; Castellazzi, G.; de Miranda, S.; Milani, G. A two-step automated procedure based on adaptive limit and pushover analyses for the seismic assessment of masonry structures. Comput. Struct. 2021, 252, 106561. [Google Scholar] [CrossRef]
- Mistler, M.; Anthoine, A.; Butenweg, C. In-plane and out-of-plane homogenisation of masonry. Comput. Struct. 2007, 85, 1321–1330. [Google Scholar] [CrossRef]
- Barenblatt, G.I. The formation of equilibrium cracks during brittle fracture. General ideas and hypotheses. Axially-symmetric cracks. J. Appl. Math. Mech. 1959, 23, 622–636. [Google Scholar] [CrossRef]
- Xu, X.-P.; Needleman, A. Numerical simulations of fast crack growth in brittle solids. J. Mech. Phys. Solids 1994, 42, 1397–1434. [Google Scholar] [CrossRef]
- Belytschko, T.; Moës, N.; Usui, S.; Parimi, C. Arbitrary discontinuities in finite elements. Int. J. Numer. Methods Eng. 2001, 50, 993–1013. [Google Scholar] [CrossRef]
- De Borst, R. Computation of post-bifurcation and post-failure behaviour of strain-softening solids. Comput. Struct. 1987, 25, 211–224. [Google Scholar] [CrossRef]
- Lourenço, P.B.; De Borst, R.; Rots, J.G. A plane stress softening plasticity model for orthotropic materials. Int. J. Numer. Methods Eng. 1997, 40, 4033–4057. [Google Scholar] [CrossRef]
- de Borst, R. Fracture in quasi-brittle materials: A review of continuum damage-based approaches. Eng. Fract. Mech. 2002, 69, 95–112. [Google Scholar] [CrossRef]
- Clemente, R.; Roca, P.; Cervera, M. Damage model with crack localization—Application to historical buildings. In Proceedings of the Structural Analysis of Historical Constructions, New Delhi, India, 6–8 November 2006; Lourenço, P.B., Roca, P., Modena, C., Agrawal, S., Eds.; Macmillan Publishers India Limited: Lucknow, India, 2006; pp. 1125–1134. [Google Scholar]
- Wu, J.-Y.; Cervera, M. A thermodynamically consistent plastic-damage framework for localized failure in quasi-brittle solids: Material model and strain localization analysis. Int. J. Solids Struct. 2016, 88–89, 227–247. [Google Scholar] [CrossRef]
- Milani, G.; Lourenço, P.B.; Tralli, A. Homogenised limit analysis of masonry walls, Part II: Structural examples. Comput. Struct. 2006, 84, 181–195. [Google Scholar] [CrossRef]
- Milani, G.; Tralli, A. Simple SQP approach for out-of-plane loaded homogenized brickwork panels, accounting for softening. Comput. Struct. 2011, 89, 201–215. [Google Scholar] [CrossRef]
- Milani, G.; Venturini, G. Automatic fragility curve evaluation of masonry churches accounting for partial collapses by means of 3D FE homogenized limit analysis. Comput. Struct. 2011, 89, 1628–1648. [Google Scholar] [CrossRef]
- Casolo, S. Macroscopic modelling of structured materials: Relationship between orthotropic Cosserat continuum and rigid elements. Int. J. Solids Struct. 2006, 43, 475–496. [Google Scholar] [CrossRef]
- Casolo, S.; Milani, G. Simplified out-of-plane modelling of three-leaf masonry walls accounting for the material texture. Constr. Build. Mater. 2013, 40, 330–351. [Google Scholar] [CrossRef]
- Scacco, J.; Ghiassi, B.; Milani, G.; Lourenço, P.B. A fast modelling approach for numerical analysis of unreinforced and FRCM reinforced masonry walls under out-of-plane loading. Compos. Part B Eng. 2020, 180, 107553. [Google Scholar] [CrossRef]
- Scacco, J.; Milani, G.; Lourenço, P.B. Automatic mesh generator for the non-linear homogenized analysis of double curvature masonry structures. Adv. Eng. Softw. 2020, 150, 102919. [Google Scholar] [CrossRef]
- Casolo, S. Rigid element model for non-linear analysis of masonry façades subjected to out-of-plane loading. Commun. Numer. Methods Eng. 1999, 15, 457–468. [Google Scholar] [CrossRef]
- Silva, L.C.; Lourenço, P.B.; Milani, G. Rigid block and spring homogenized model (HRBSM) for masonry subjected to impact and blast loading. Int. J. Impact Eng. 2017, 109, 14–28. [Google Scholar] [CrossRef]
- Silva, L.C.; Lourenço, P.B.; Milani, G. Numerical homogenization-based seismic assessment of an English-bond masonry prototype: Structural level application. Earthq. Eng. Struct. Dyn. 2020, 49, 841–862. [Google Scholar] [CrossRef]
- Sharma, S.; Silva, L.C.; Graziotti, F.; Magenes, G.; Milani, G. Modelling the experimental seismic out-of-plane two-way bending response of unreinforced periodic masonry panels using a non-linear discrete homogenized strategy. Eng. Struct. 2021, 242, 112524. [Google Scholar] [CrossRef]
- Uva, G.; Tateo, V.; Casolo, S. Presentation and validation of a specific RBSM approach for the meso-scale modelling of in-plane masonry-infills in RC frames. Int. J. Mason. Res. Innov. 2020, 5, 366–395. [Google Scholar] [CrossRef]
- Silva, L.C.; Lourenço, P.B.; Milani, G. Nonlinear Discrete Homogenized Model for Out-of-Plane Loaded Masonry Walls. J. Struct. Eng. 2017, 143, 4017099. [Google Scholar] [CrossRef]
- Kawai, T. New discrete structural models and generalization of the method of limit analysis. In Proceedings of the Finite Elements in Nonlinear Mechanics, Geilo, Norway, August 1977; Tapir Publishers: Trondheim, Norway, 1977; pp. 885–906. [Google Scholar]
- Kawai, T. New discrete models and their application to seismic response analysis of structures. Nucl. Eng. Des. 1978, 48, 207–229. [Google Scholar] [CrossRef]
- Kawai, T. Discrete limit analysis of reinforced concrete structures using rigid bodies-spring models. In The Finite Element Method In the 1990’s; Springer: Berlin/Heidelberg, Germany, 1991; pp. 182–191. [Google Scholar]
- Kannan, R.; Hendry, S.; Higham, N.J.; Tisseur, F. Detecting the causes of ill-conditioning in structural finite element models. Comput. Struct. 2014, 133, 79–89. [Google Scholar] [CrossRef]
- Bertolesi, E.; Silva, L.C.; Milani, G. Validation of a two-step simplified compatible homogenisation approach extended to out-plane loaded masonries. Int. J. Mason. Res. Innov. 2019, 4, 265. [Google Scholar] [CrossRef]
- Bertolesi, E.; Milani, G.; Lourenço, P.B. Implementation and validation of a total displacement non-linear homogenization approach for in-plane loaded masonry. Comput. Struct. 2016, 176, 13–33. [Google Scholar] [CrossRef]
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. Plastic-Damage Model for Cyclic Loading of Concrete Structures. J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
- Grassl, P.; Jirásek, M. Damage-plastic model for concrete failure. Int. J. Solids Struct. 2006, 43, 7166–7196. [Google Scholar] [CrossRef]
- Duvaut, G.; Lions, J.L. Les Inéquations En Mécanique et en Physique; Dunod: Paris, France, 1972. [Google Scholar]
- Bažant, Z.P.; Oh, B.H. Crack band theory for fracture of concrete. Matériaux Constr. 1983, 16, 155–177. [Google Scholar] [CrossRef]
- Bacigalupo, A.; Gambarotta, L.; Lepidi, M. Thermodynamically consistent non-local continualization for masonry-like systems. Int. J. Mech. Sci. 2021, 205, 106538. [Google Scholar] [CrossRef]
- Addessi, D.; Sacco, E. Enriched plane state formulation for nonlinear homogenization of in-plane masonry wall. Meccanica 2016, 51, 2891–2907. [Google Scholar] [CrossRef]
- Irons, B.M. Numerical integration applied to finite element methods. In Proceedings of the Conference on Use of Digital Computers in Structural Engineering; University of Newcastle: Newcastle, UK, 1966. [Google Scholar]
- Irons, B.M.; Razzaque, A. Experience with the patch test for convergence of finite elements. In The Mathematical Foundations of the Finite Element Method with Applications to Partial Differential Equations; Academic Press: Cambridge, MA, USA, 1972; pp. 557–587. ISBN 978-0-12-068650-6. [Google Scholar]
- Irons, B.; Loikkanen, M. An engineers’ defence of the patch test. Int. J. Numer. Methods Eng. 1983, 19, 1391–1401. [Google Scholar] [CrossRef]
- Macneal, R.H.; Harder, R.L. A proposed standard set of problems to test finite element accuracy. Finite Elem. Anal. Des. 1985, 1, 3–20. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Zhu, J.Z. The superconvergent patch recovery (SPR) and adaptive finite element refinement. Comput. Methods Appl. Mech. Eng. 1992, 101, 207–224. [Google Scholar] [CrossRef]
- Hughes, T.J.R.; Cottrell, J.A.; Bazilevs, Y. Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput. Methods Appl. Mech. Eng. 2005, 194, 4135–4195. [Google Scholar] [CrossRef]
- Taylor, R.L.; Simo, J.C.; Zienkiewicz, O.C.; Chan, A.C.H. The patch test—A condition for assessing FEM convergence. Int. J. Numer. Methods Eng. 2018, 22, 39–62. [Google Scholar] [CrossRef]
- Rao, K.M.; Shrinivasa, U. A set of pathological tests to validate new finite elements. Sadhana 2001, 26, 549–590. [Google Scholar] [CrossRef][Green Version]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method: Solid Mechanics; Butterworth-Heinemann: Oxford, UK, 2000; Volume 2, ISBN 0750650559. [Google Scholar]
- Turco, E.; Caracciolo, P. Elasto-plastic analysis of Kirchhoff plates by high simplicity finite elements. Comput. Methods Appl. Mech. Eng. 2000, 190, 691–706. [Google Scholar] [CrossRef]
- Navier, C.L.M.H. Extrait des recherches sur la flexion des plans elastiques. Bull. Sci. Soc. Philomarhique 1823, 5, 95–102. [Google Scholar]
- Collins, R.J. Bandwidth reduction by automatic renumbering. Int. J. Numer. Methods Eng. 1973, 6, 345–356. [Google Scholar] [CrossRef]
- Cuthill, E.; McKee, J. Reducing the bandwidth of sparse symmetric matrices. In Proceedings of the 1969 24th National Conference, New York, NY, USA, 26–28 August 1969; ACM: New York, NY, USA, 1969; pp. 157–172. [Google Scholar]
- Bathe, K.-J.; Wilson, E.L. Solution methods for eigenvalue problems in structural mechanics. Int. J. Numer. Methods Eng. 1973, 6, 213–226. [Google Scholar] [CrossRef]
- Bathe, K.J.; Wilson, E.L. NONSAP—A nonlinear structural analysis program. Nucl. Eng. Des. 1974, 29, 266–293. [Google Scholar] [CrossRef]
- Mafteiu-Scai, L.O. The Bandwidths of a Matrix. A Survey of Algorithms. Ann. West Univ. Timisoara Math. Comput. Sci. 2014, 52, 183–223. [Google Scholar] [CrossRef]
- Pop, P.; Matei, O.; Comes, C.-A. Reducing the bandwidth of a sparse matrix with a genetic algorithm. Optimization 2014, 63, 1851–1876. [Google Scholar] [CrossRef]
- Amestoy, P.R.; Davis, T.A.; Duff, I.S. Algorithm 837: AMD, an Approximate Minimum Degree Ordering Algorithm. ACM Trans. Math. Softw. 2004, 30, 381–388. [Google Scholar] [CrossRef]
- BETA CAE Systems International. ANSA The advanced CAE pre-processing software for complete model build up. In Proceedings of the OpenFOAM User Meeting Stammtisch United, Kassel, Germany, 20–21 February 2017; BETA CAE Systems International: Root, Switzerland, 2017. [Google Scholar]
- Belytschko, T.; Lin, J.I.; Chen-Shyh, T. Explicit algorithms for the nonlinear dynamics of shells. Comput. Methods Appl. Mech. Eng. 1984, 42, 225–251. [Google Scholar] [CrossRef]
- Burnett, S.; Gilbert, M.; Molyneaux, T.; Beattie, G.; Hobbs, B. The performance of unreinforced masonry walls subjected to low-velocity impacts: Finite element analysis. Int. J. Impact Eng. 2007, 34, 1433–1450. [Google Scholar] [CrossRef]
- Hilber, H.M.; Hughes, T.J.R.; Taylor, R.L. Improved numerical dissipation for time integration algorithms in structural dynamics. Earthq. Eng. Struct. Dyn. 1977, 5, 283–292. [Google Scholar] [CrossRef]
- Rafsanjani, S.H.; Lourenço, P.B.; Peixinho, N. Implementation and validation of a strain rate dependent anisotropic continuum model for masonry. Int. J. Mech. Sci. 2015, 104, 24–43. [Google Scholar] [CrossRef]
- Riks, E. Some computational aspects of the stability analysis of nonlinear structures. Comput. Methods Appl. Mech. Eng. 1984, 47, 219–259. [Google Scholar] [CrossRef]
- Riks, E. An incremental approach to the solution of snapping and buckling problems. Int. J. Solids Struct. 1979, 15, 529–551. [Google Scholar] [CrossRef]
- Crisfield, M.A. A fast incremental/iterative solution procedure that handles “snap-through”. Comput. Struct. 1981, 13, 55–62. [Google Scholar] [CrossRef]
- Powell, G.; Simons, J. Improved iteration strategy for nonlinear structures. Int. J. Numer. Methods Eng. 1981, 17, 1455–1467. [Google Scholar] [CrossRef]
- Candeias, P.X.; Costa, A.C.; Mendes, N.; Costa, A.; Lourenço, P.B. Experimental Assessment of the Out-of-Plane Performance of Masonry Buildings Through Shaking Table Tests. Int. J. Archit. Herit. 2017, 11, 31–58. [Google Scholar] [CrossRef]
- Kruis, J.; Krejčí, T.; Šejnoha, M. Parallel computing in multi-scale analysis of coupled heat and moisture transport in masonry structures. In High Performance Computing in Science and Engineering; Kozubek, T., Blaheta, R., Šístek, J., Rozložník, M., Čermák, M., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 50–59. [Google Scholar]
- Krejčí, T.; Kruis, J.; Šejnoha, M.; Koudelka, T. Hybrid parallel approach to homogenization of transport processes in masonry. Adv. Eng. Softw. 2017, 113, 25–33. [Google Scholar] [CrossRef]
- Fortunato, G.; Funari, M.F.; Lonetti, P. Survey and seismic vulnerability assessment of the Baptistery of San Giovanni in Tumba (Italy). J. Cult. Herit. 2017, 26, 64–78. [Google Scholar] [CrossRef]
- Clementi, F.; Gazzani, V.; Poiani, M.; Lenci, S. Assessment of seismic behaviour of heritage masonry buildings using numerical modelling. J. Build. Eng. 2016, 8, 29–47. [Google Scholar] [CrossRef]
- Barontini, A.; Masciotta, M.G.; Ramos, L.F.; Amado-Mendes, P.; Lourenço, P.B. An overview on nature-inspired optimization algorithms for Structural Health Monitoring of historical buildings. Procedia Eng. 2017, 199, 3320–3325. [Google Scholar] [CrossRef]
- Funari, M.F.; Verre, S. The Effectiveness of the DIC as a Measurement System in SRG Shear Strengthened Reinforced Concrete Beams. Crystals 2021, 11, 265. [Google Scholar] [CrossRef]






| Mode | Macro–Element (Lumped Mass Approach) | Macro–Element (Consistent Mass Approach) | FEA N = 30 | Exact (rad/s) | m | n | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| N = 4 | N = 8 | N = 16 | N = 30 | N = 4 | N = 8 | N = 16 | N = 30 | |||||
| 1 | 1.276 | 1.094 | 1.026 | 1.025 | 1.244 | 1.088 | 1.064 | 1.024 | 1.000 | 19.739 | 1 | 1 |
| 2 | 1.382 | 1.085 | 1.037 | 1.037 | 1.302 | 1.069 | 1.064 | 1.034 | 1.000 | 49.348 | 1 | 2 |
| 3 | 1.382 | 1.085 | 1.037 | 1.037 | 1.294 | 1.069 | 1.064 | 1.034 | 1.000 | 49.348 | 2 | 1 |
| 4 | 1.499 | 1.129 | 1.036 | 1.028 | 1.371 | 1.103 | 1.073 | 1.031 | 1.001 | 78.957 | 2 | 2 |
| 5 | 1.405 | 1.088 | 1.047 | 1.045 | 1.276 | 1.053 | 1.063 | 1.039 | 1.000 | 98.696 | 1 | 3 |
| 6 | 1.475 | 1.084 | 1.046 | 1.045 | 1.218 | 1.049 | 1.061 | 1.039 | 1.000 | 98.696 | 3 | 1 |
| 7 | 1.569 | 1.143 | 1.045 | 1.038 | 1.356 | 1.099 | 1.075 | 1.036 | 1.001 | 128.30 | 3 | 2 |
| 8 | 1.569 | 1.143 | 1.045 | 1.038 | 1.355 | 1.099 | 1.075 | 1.036 | 1.001 | 128.30 | 2 | 3 |
| Cracking Strain (–) | Stress (MPa) | Damage Scalar D (–) |
|---|---|---|
| 0.00 | 9.61 | 0.00 |
| 5.06 × 10−5 | 9.41 | 0.20 |
| 2.11 × 10−4 | 5.57 | 0.42 |
| 3.49 × 10−4 | 4.03 | 0.58 |
| 6.09 × 10−4 | 3.36 | 0.65 |
| Node Renumbering Algorithm | None (Reference) | Geometric Algorithm | AMD Algorithm |
|---|---|---|---|
| CPU total time (s) | 14.95 | 5.523 (63.1%) | 6.006 (%) |
| Optimum physical memory RAM (Mbytes) | 137.1 | 69.93 (.0%) | 76.23 (.4%) |
| Material Properties | |||||||||
|---|---|---|---|---|---|---|---|---|---|
|
Exx (MPa) |
Eyy (MPa) | (–) | ft (MPa) | Gftension (N/mm) | fc (MPa) | Gfcompression (N/mm) | fshear (MPa) | Gfshear (N/mm) | |
| Macro–element (discrete) model | 6400 | 3600 | 0.200 | 0.105 | 0.012 | 2.480 | 3.970 | 0.20 | 0.50 |
| Smeared crack model | 5170 | 5170 | – | – | |||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
da Silva, L.C.M.; Milani, G. A FE-Based Macro-Element for the Assessment of Masonry Structures: Linear Static, Vibration, and Non-Linear Cyclic Analyses. Appl. Sci. 2022, 12, 1248. https://doi.org/10.3390/app12031248
da Silva LCM, Milani G. A FE-Based Macro-Element for the Assessment of Masonry Structures: Linear Static, Vibration, and Non-Linear Cyclic Analyses. Applied Sciences. 2022; 12(3):1248. https://doi.org/10.3390/app12031248
Chicago/Turabian Styleda Silva, Luis C. M., and Gabriele Milani. 2022. "A FE-Based Macro-Element for the Assessment of Masonry Structures: Linear Static, Vibration, and Non-Linear Cyclic Analyses" Applied Sciences 12, no. 3: 1248. https://doi.org/10.3390/app12031248
APA Styleda Silva, L. C. M., & Milani, G. (2022). A FE-Based Macro-Element for the Assessment of Masonry Structures: Linear Static, Vibration, and Non-Linear Cyclic Analyses. Applied Sciences, 12(3), 1248. https://doi.org/10.3390/app12031248







