Stochastic Analysis of a Large-Span Continuous Girder High-Speed Railway Bridge under Fully Non-Stationary Earthquake
Abstract
1. Introduction
2. Analysis Methodology
2.1. Equation of Structural Motion
2.2. Stochastic Analysis Using the PEM
2.3. Stochastic Analysis Using the MCS Method
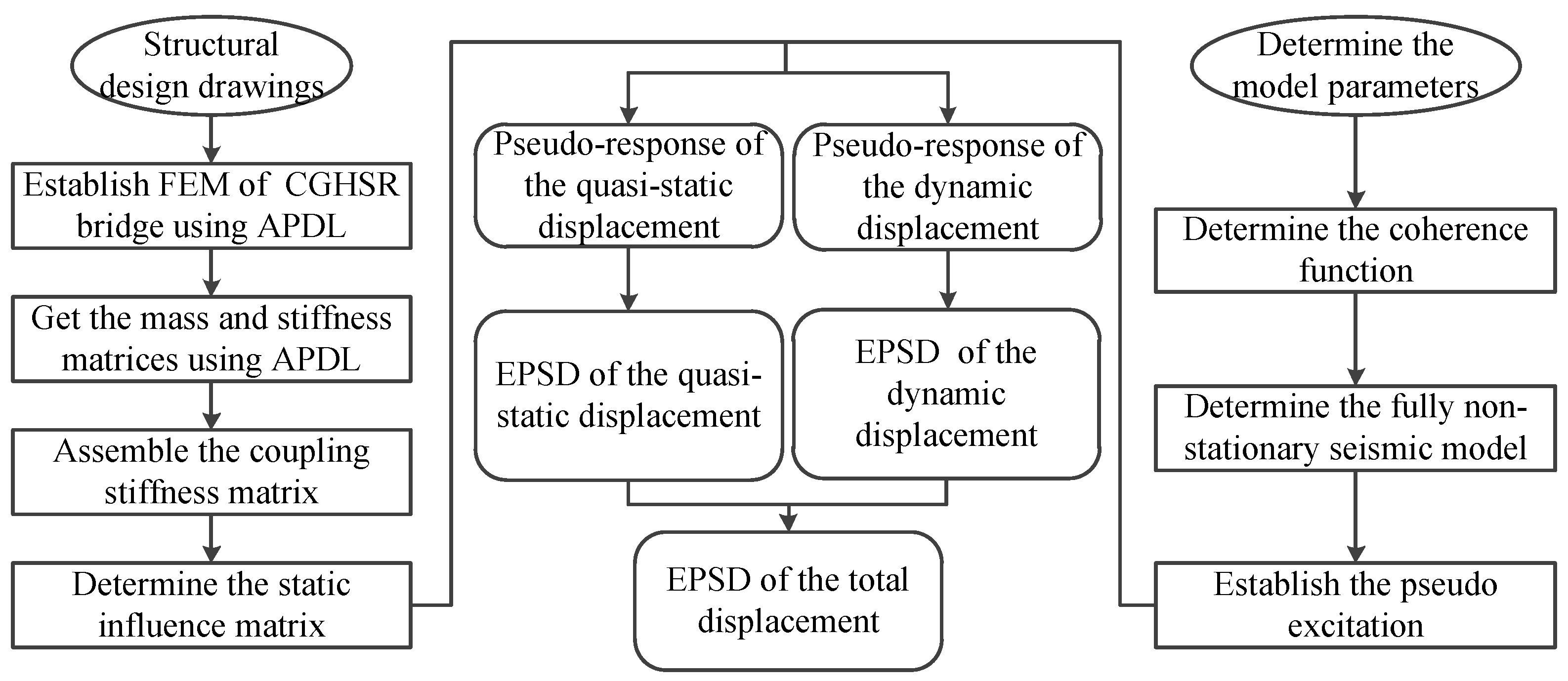
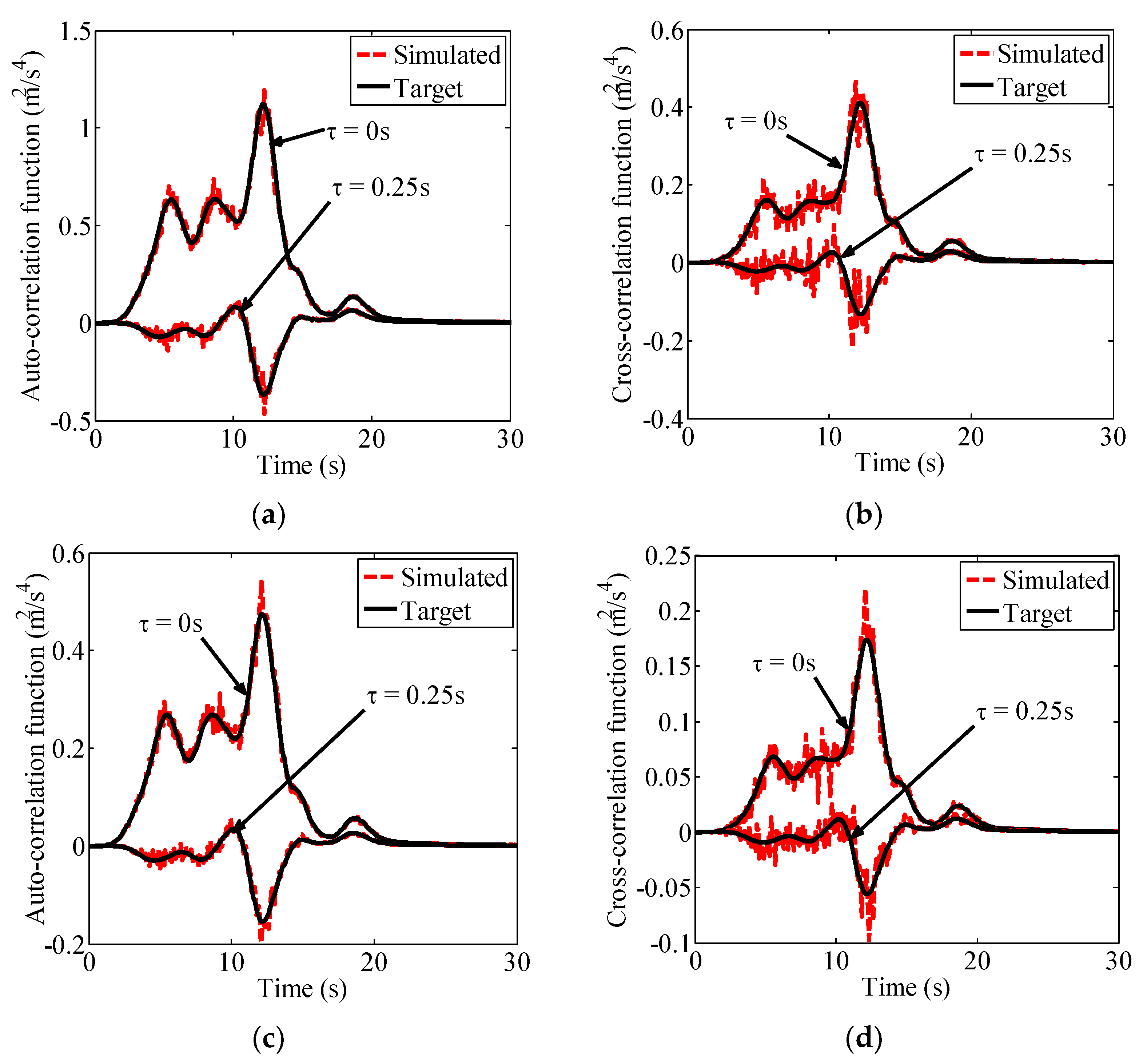
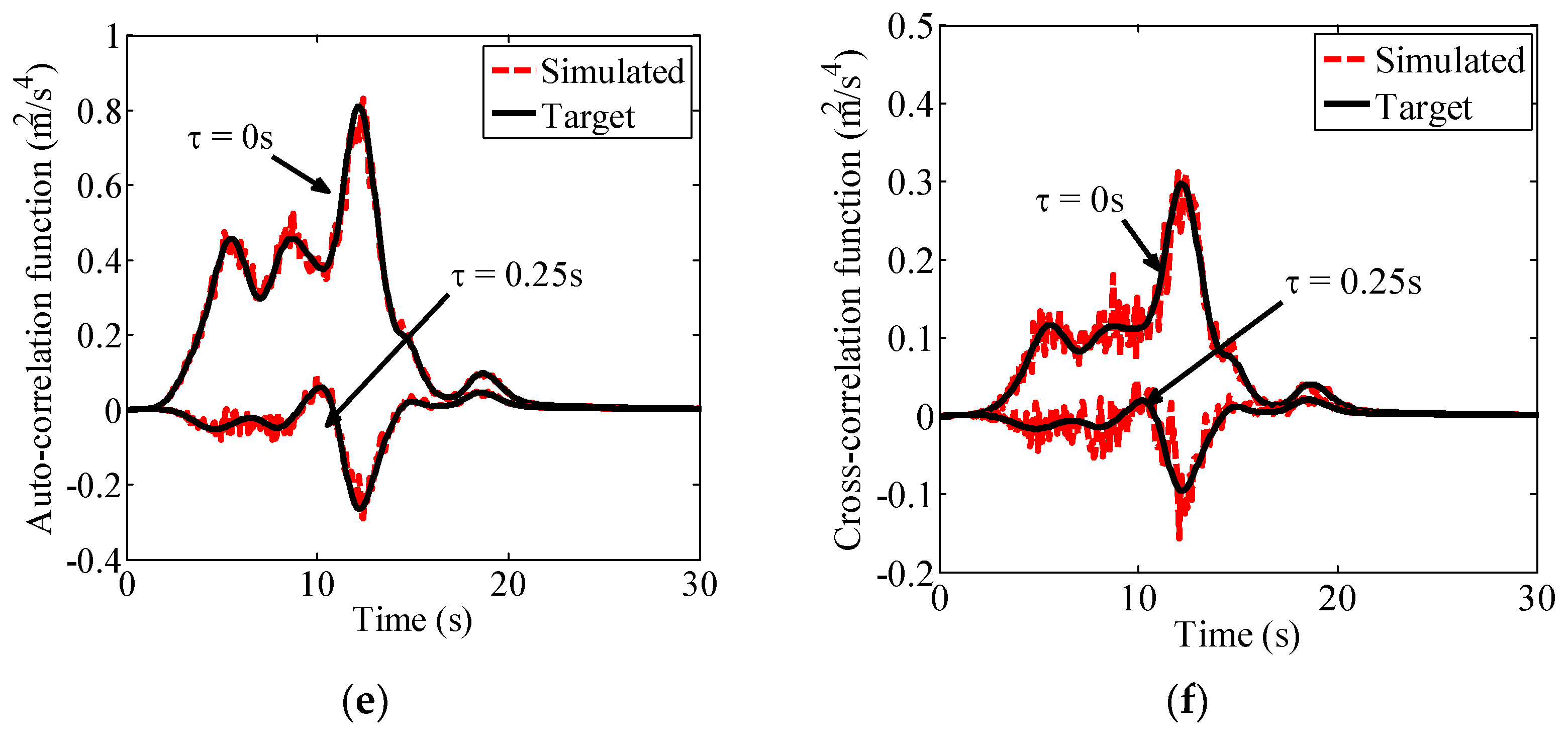
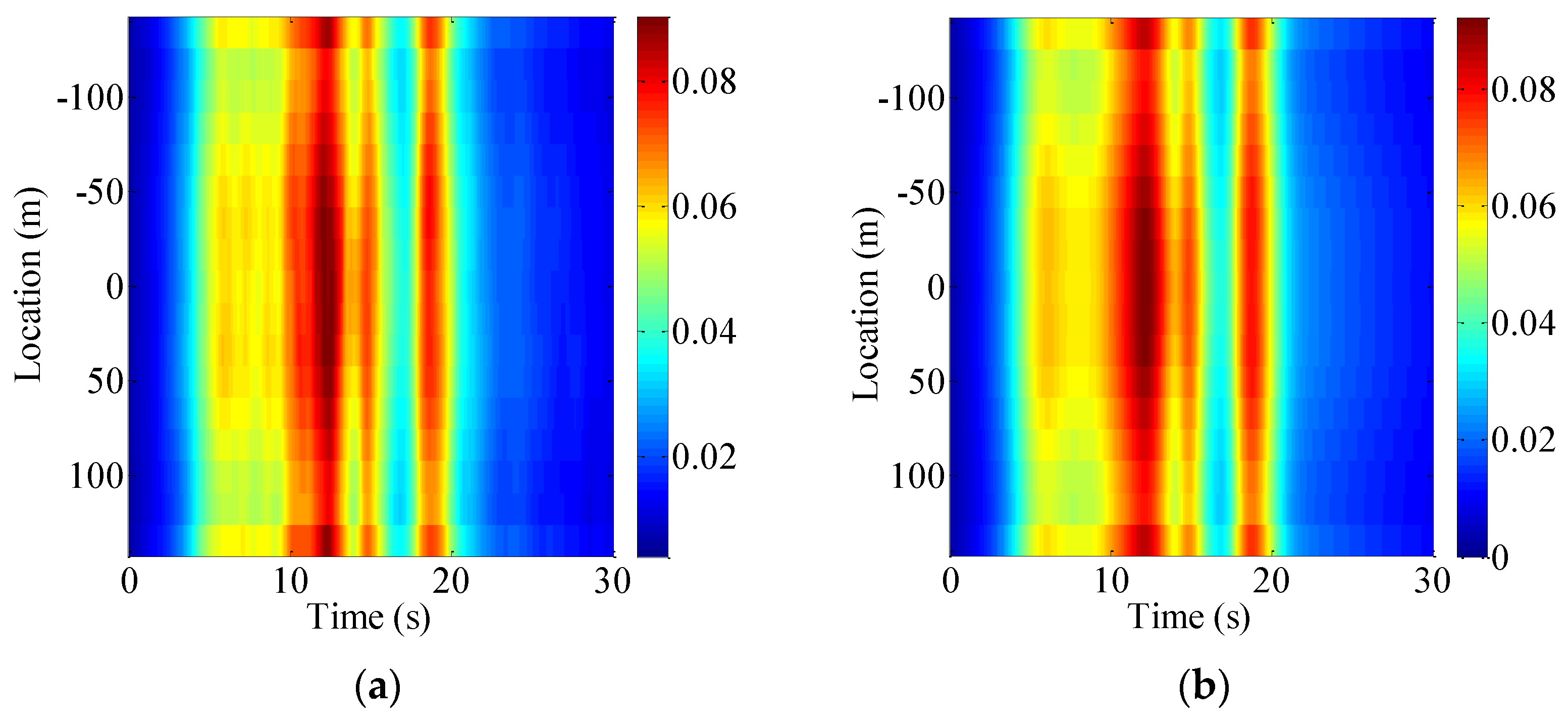
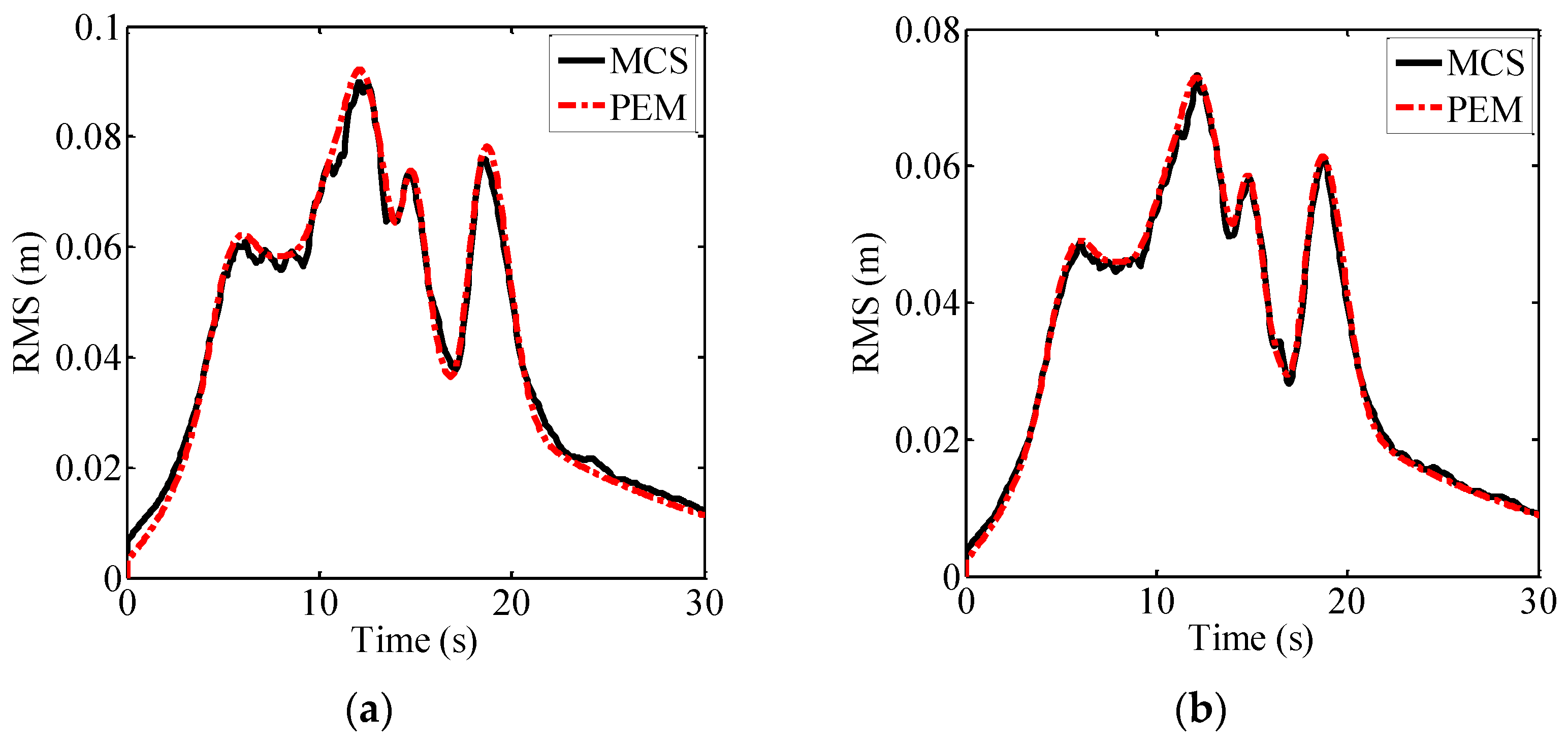
3. Stochastic Seismic Response of the Large-Span CGHSR Bridge
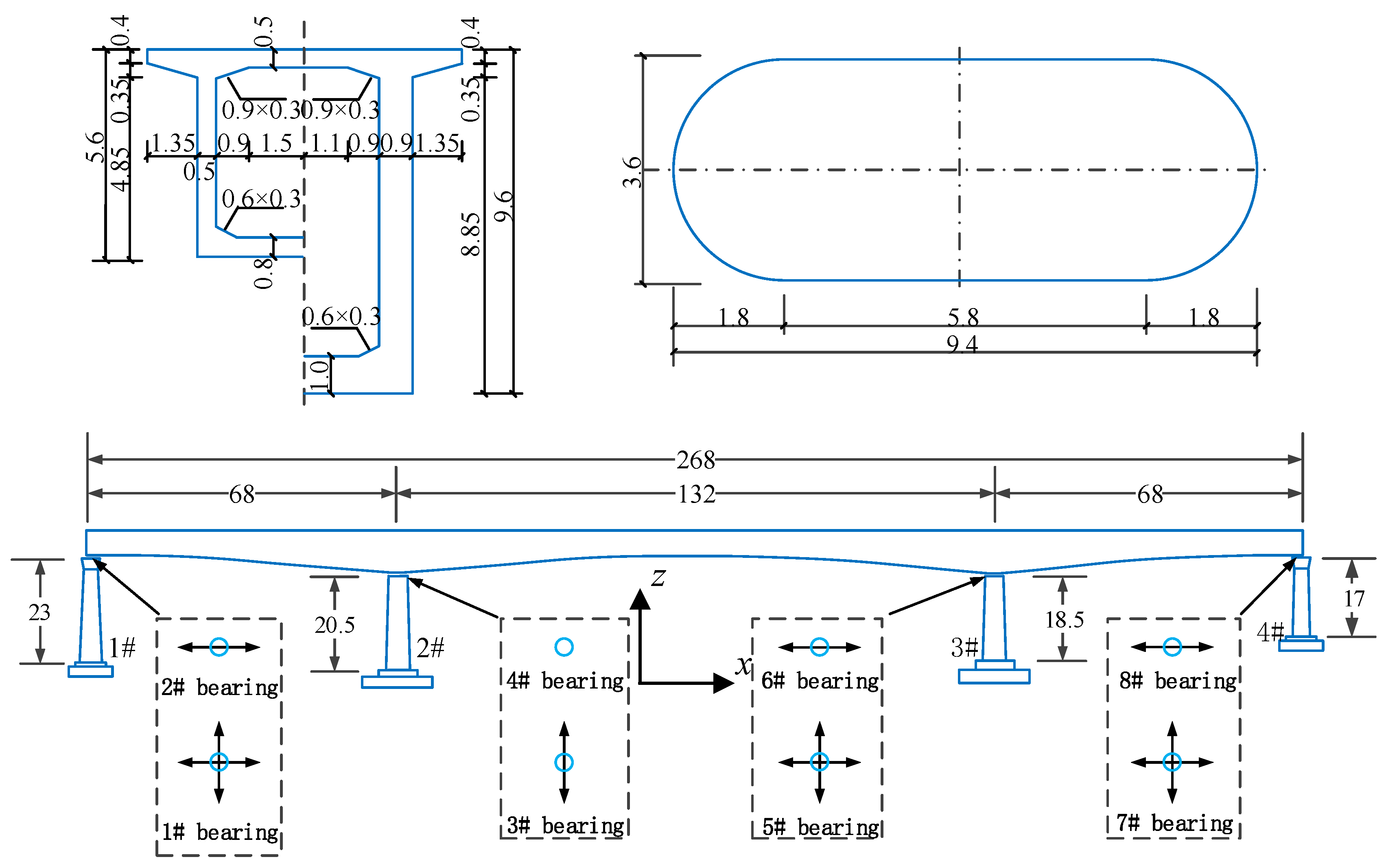
3.1. Finite Element Model (FEM) of the CGHSR Bridge
3.2. Determination of the Static Influence Matrix
3.3. Fully Non-Stationary Ground Acceleration Model
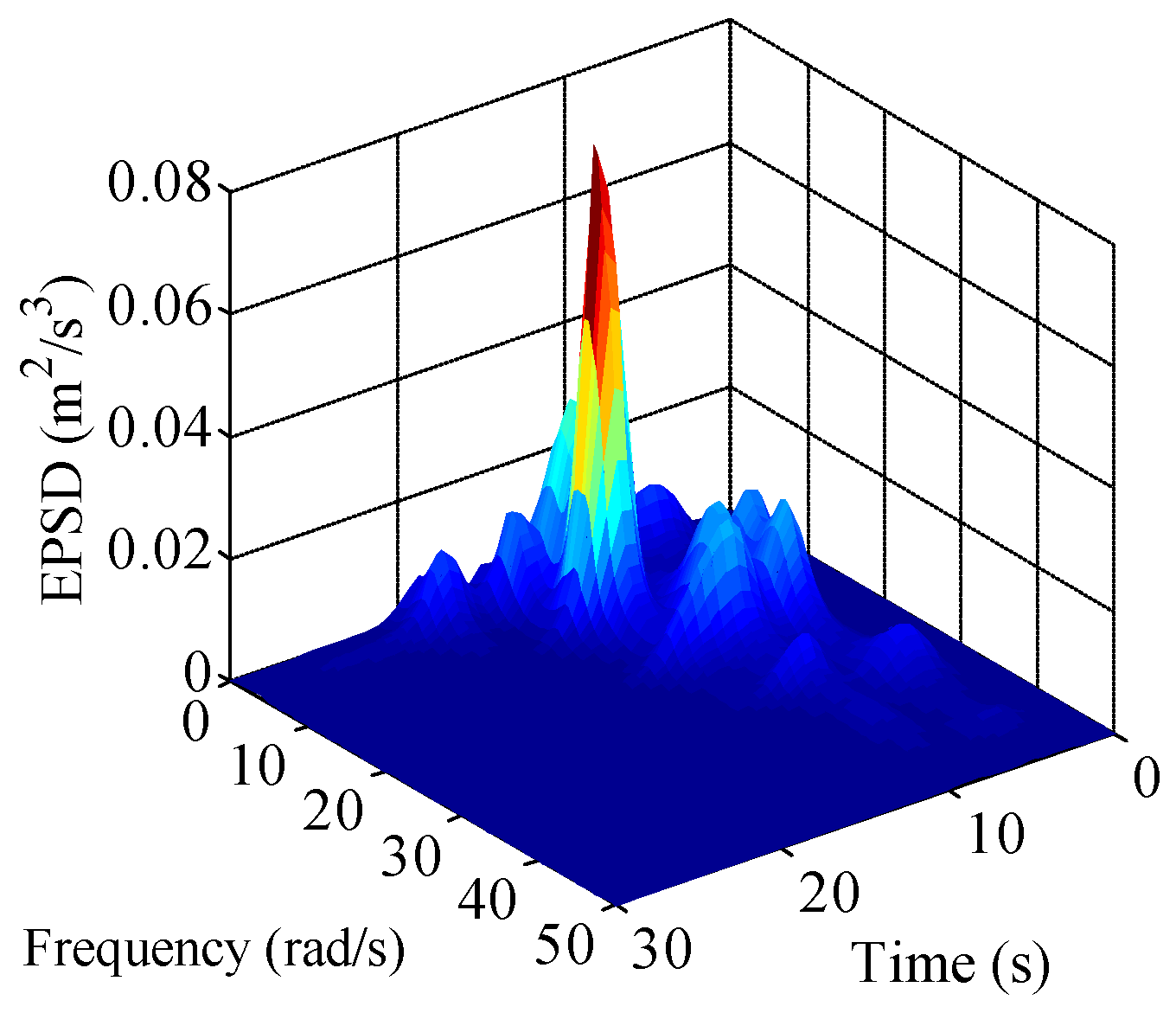
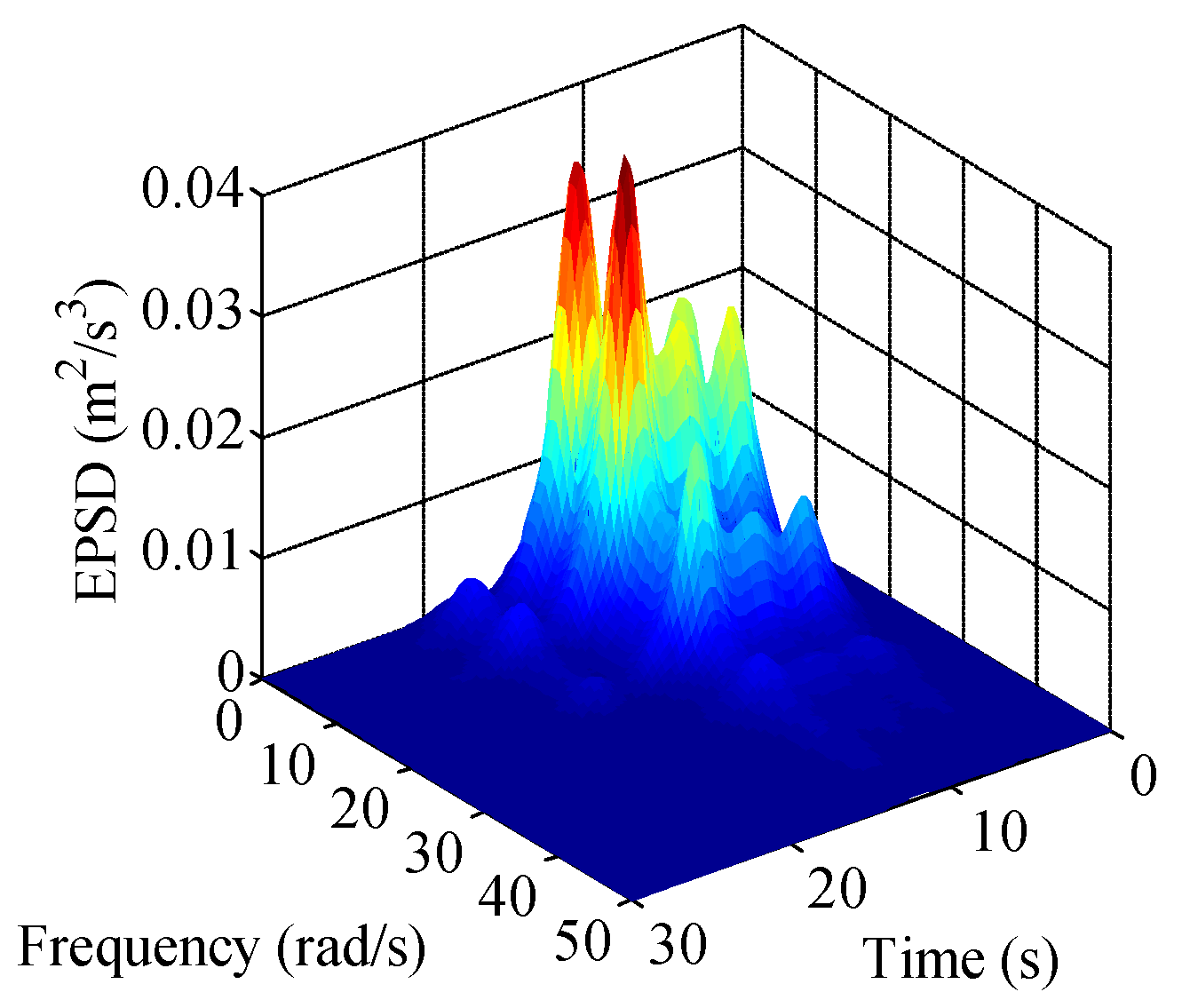
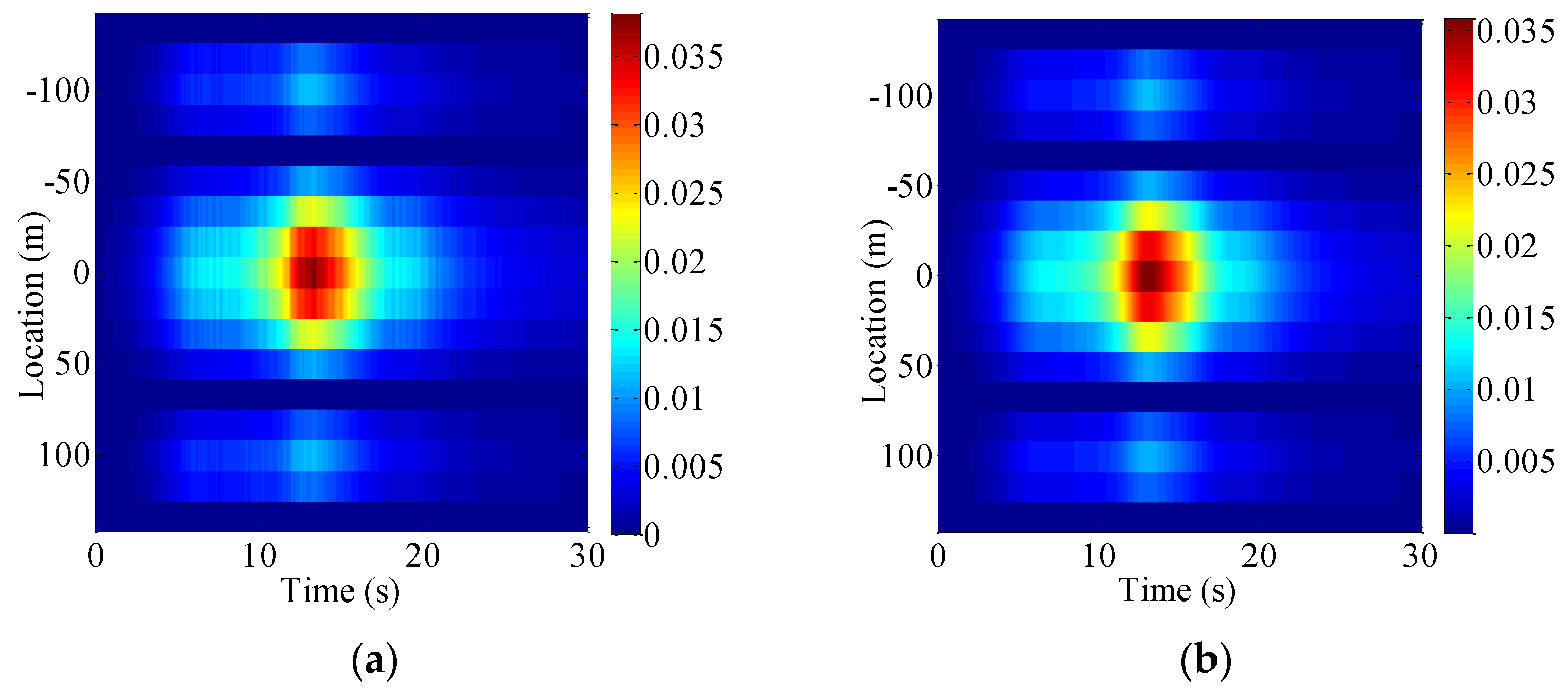
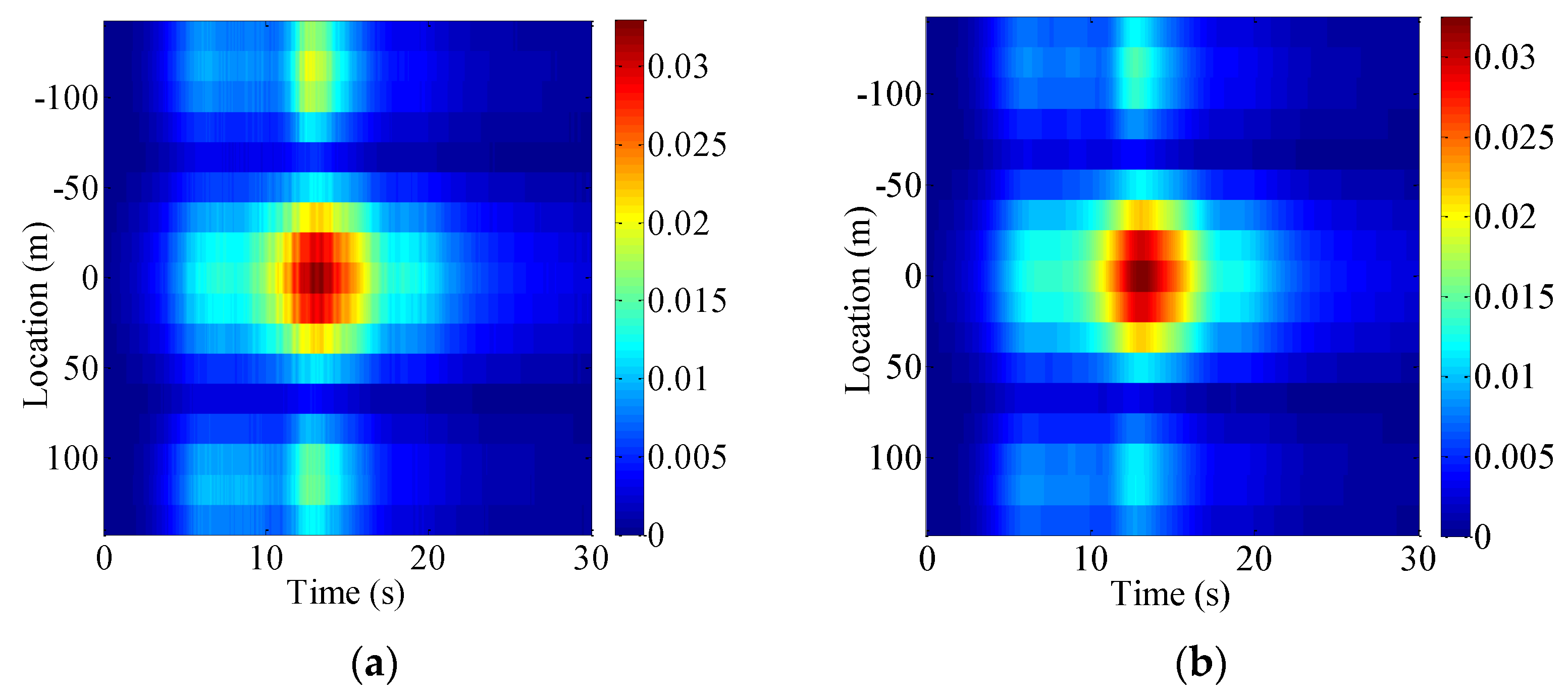
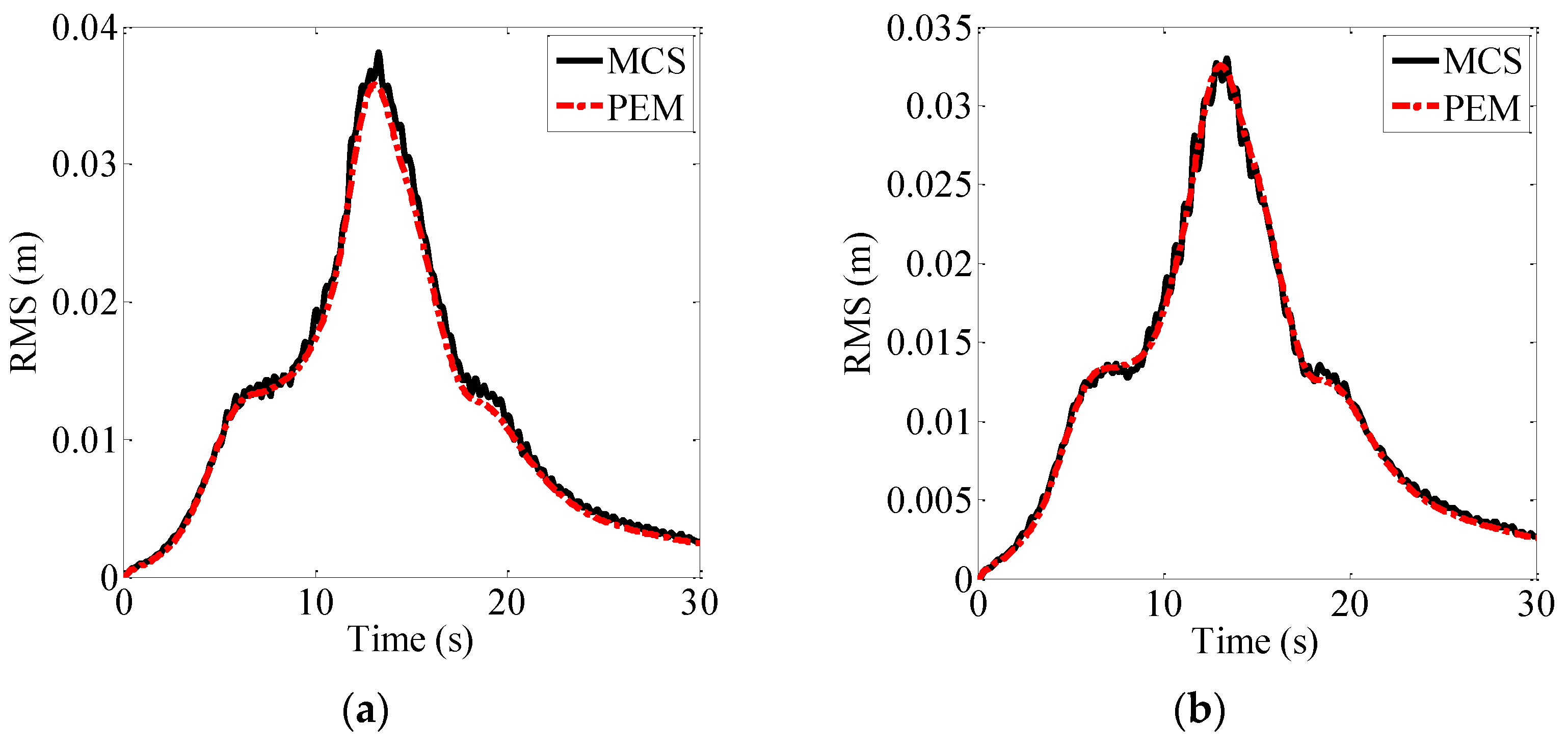
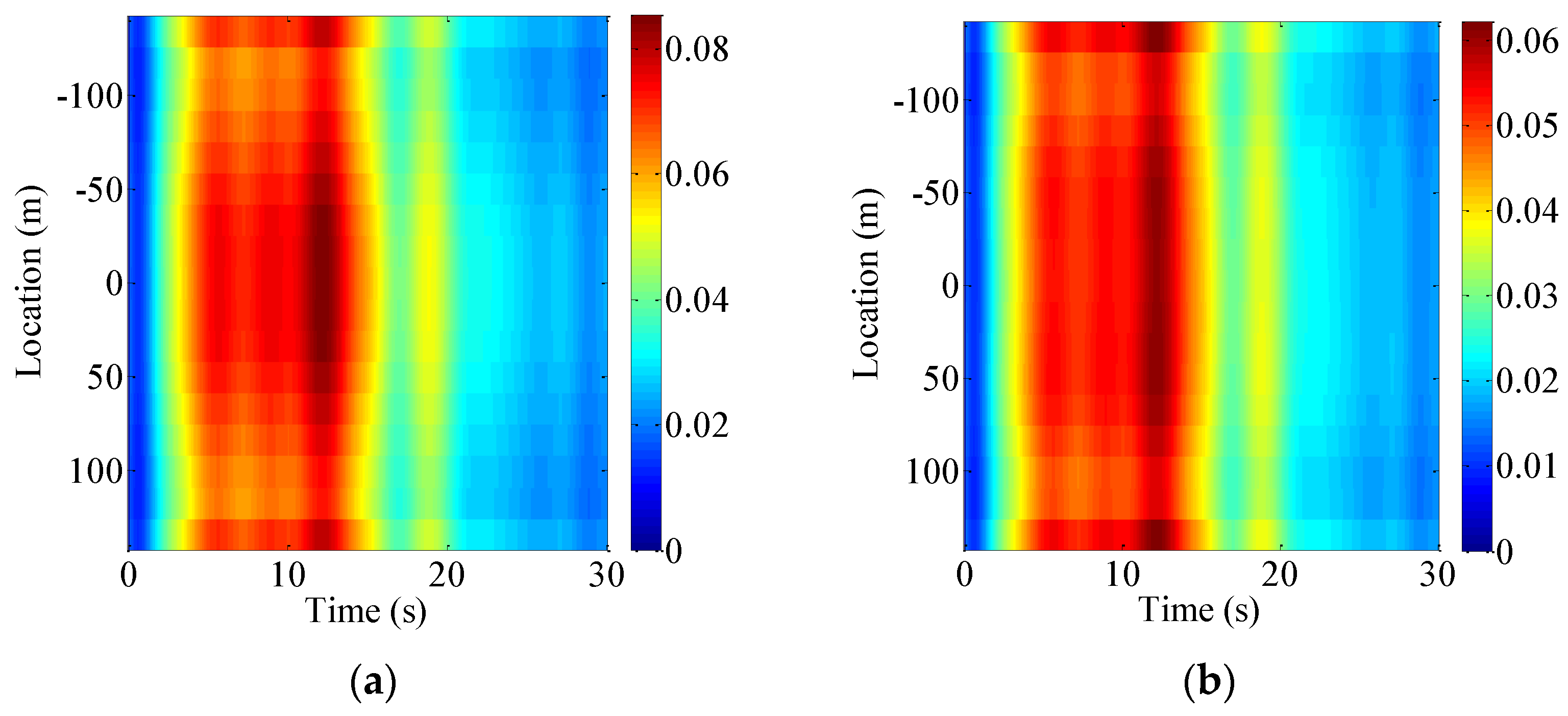
3.4. Structural Stochastic Seismic Response
4. Conclusions
- The proposed POD-based method can determine the structural pseudo static displacement for PEM under fully non-stationary seismic excitation. The structural dynamic and quasi-static displacement contributes almost the same to the absolute displacement.
- The last arrived surface waves can also cause a relatively large displacement. The frequency non-stationarity of the earthquake ground motion should be considered to avoid underestimating the structural quasi-static displacement caused by the surface waves.
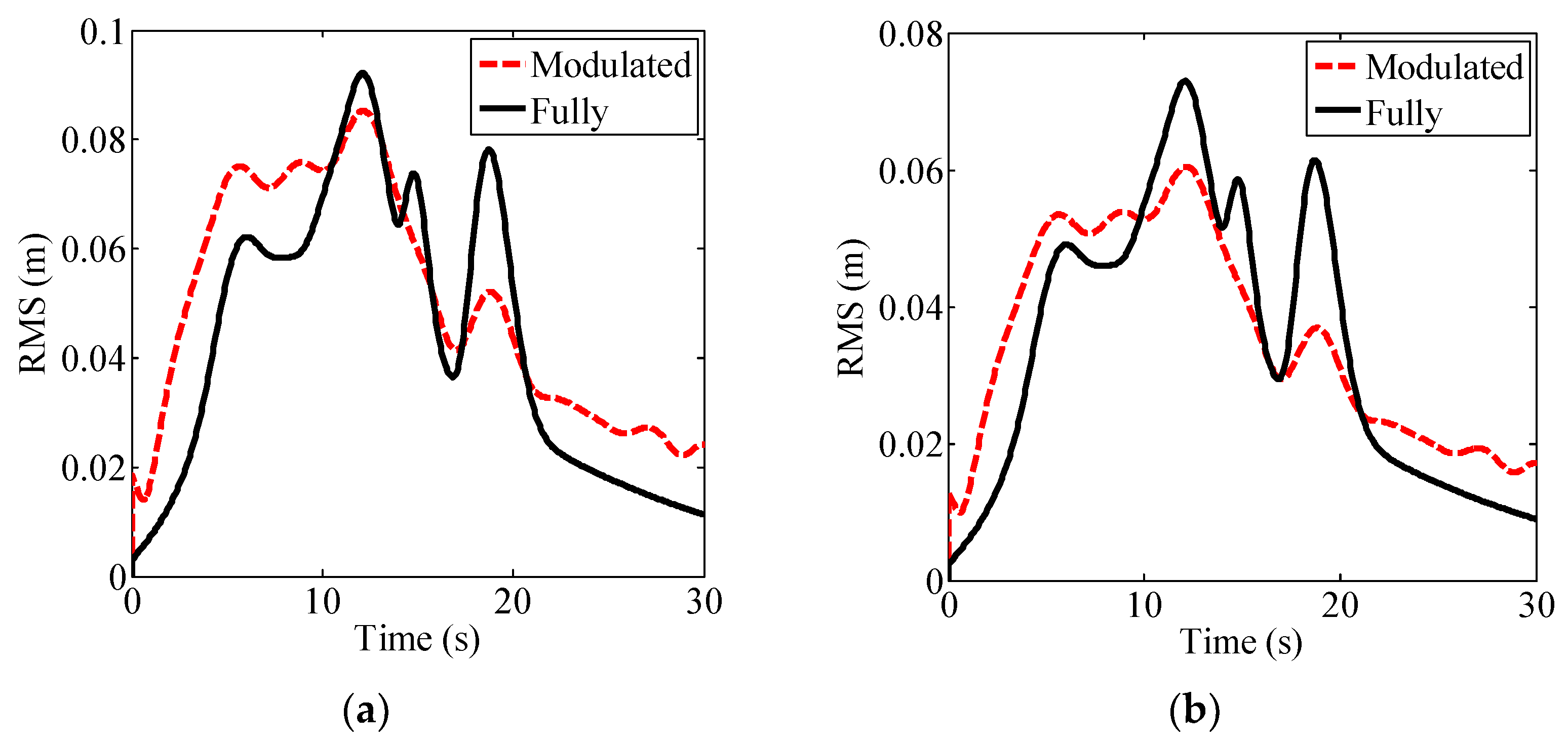
- The structural stochastic response under fully non-stationary seismic excitation is larger than that under equivalent modulated non-stationary seismic excitation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yan, B.; Dai, G.; Hu, N. Recent development of design and construction of short span high-speed railway bridges in China. Eng. Struct. 2015, 100, 707–717. [Google Scholar] [CrossRef]
- He, X.; Wu, T.; Zou, Y.; Chen, Y.; Guo, H.; Yu, Z. Recent developments of high-speed railway bridges in China. Struct. Infrastruct. Eng. 2017, 13, 1584–1595. [Google Scholar] [CrossRef]
- He, G.; Mol, A.; Zhang, L.; Lu, Y. Environmental risks of high-speed railway in China: Public participation, perception and trust. Environ. Dev. 2015, 14, 37–52. [Google Scholar] [CrossRef]
- Cai, C.; He, Q.; Zhu, S.; Zhai, W.; Wang, M. Dynamic interaction of suspension-type monorail vehicle and bridge: Numerical simulation and experiment. Mech. Syst. Signal Process. 2019, 118, 388–407. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, H.; Mao, J.; Wang, F.; Hu, S.; Zhao, X. Monitoring-Based Assessment of the Construction Influence of Benoto Pile on Adjacent High-Speed Railway Bridge: Case Study. J. Perform. Constr. Facil. 2019, 33, 04018106. [Google Scholar] [CrossRef]
- Guo, W.; Hu, Y.; Liu, H.; Bu, D. Seismic performance evaluation of typical piers of China’s high-speed railway bridge line using pushover analysis. Math. Probl. Eng. 2019, 2019, 9514769. [Google Scholar] [CrossRef]
- Zhang, Z.; Lin, J.; Zhang, Y.; Zhao, Y.; Howson, W.; Williams, F. Non-stationary random vibration analysis for train–bridge systems subjected to horizontal earthquakes. Eng. Struct. 2010, 32, 3571–3582. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, Y.; Li, L. Major natural disasters and their spatio-temporal variation in the history of China. J. Geogr. Sci. 2012, 22, 963–976. [Google Scholar] [CrossRef]
- Wei, B.; Li, C.; He, X. The applicability of different earthquake intensity measures to the seismic vulnerability of a high-speed railway continuous bridge. Int. J. Civ. Eng. 2019, 17, 981–997. [Google Scholar] [CrossRef]
- Zucca, M.; Giuseppe, C.P.; Tropeano, G.; Simoncelli, M. On the Influence of Shallow Underground Structures in the Evaluation of the Seismic Signals. Ing. Sismica 2021, 38, 23–35. [Google Scholar]
- Harichandran, R.; Hawwari, A.; Sweidan, B. Response of long-span bridges to spatially varying ground motion. J. Struct. Eng. 1996, 122, 476–484. [Google Scholar] [CrossRef]
- Zanardo, G.; Hao, H.; Modena, C. Seismic response of multi-span simply supported bridges to a spatially varying earthquake ground motion. Earthq. Eng. Struct. Dyn. 2002, 31, 1325–1345. [Google Scholar] [CrossRef]
- Lin, J.; Zhang, Y.; Yan, Z. Seismic spatial effects on long-span bridge response in nonstationary inhomogeneous random fields. Earthq. Eng. Eng. Vib. 2005, 4, 75–82. [Google Scholar]
- Jangid, R. Stochastic response of bridges seismically isolated by friction pendulum system. J. Bridge Eng. 2008, 13, 319–330. [Google Scholar] [CrossRef]
- Zhang, J.; Jia, H.; Zheng, S.; Qin, S.; Zhang, K. Analysis of a high-pier railway bridge under spatial stochastic stationary and non-stationary earthquake excitations. Teh. Vjesn. /Tech. Gaz. 2016, 23, 465–475. [Google Scholar]
- Su, C.; Liu, X.; Li, B.; Huang, Z. Inelastic response analysis of bridges subjected to non-stationary seismic excitations by efficient MCS based on explicit time-domain method. Nonlinear Dyn. 2018, 94, 2097–2114. [Google Scholar] [CrossRef]
- EN1998-2:2005; Eurocode 8: Design Provisions of Structures for Earthquake Resistance, Part 2: Bridges. European Committee for Standardization: Brussels, Belgium, 2005.
- JTG/T B02-01-2008; Guidelines for Seismic Design of Highway Bridges. China Communications Press: Beijing, China, 2008.
- Shinozuka, M. Monte Carlo solution of structural dynamics. Comput. Struct. 1972, 2, 855–874. [Google Scholar] [CrossRef]
- Xiu, D. Fast numerical methods for stochastic computations: A review. Commun. Comput. Phys. 2009, 5, 242–272. [Google Scholar]
- Hacıefendioğlu, K.; Başağa, H.; Banerjee, S. Probabilistic analysis of historic masonry bridges to random ground motion by Monte Carlo Simulation using Response Surface Method. Constr. Build. Mater. 2017, 134, 199–209. [Google Scholar] [CrossRef]
- Proppe, C.; Pradlwarter, H.; Schuëller, G. Equivalent linearization and Monte Carlo simulation in stochastic dynamics. Probabilistic Eng. Mech. 2003, 18, 1–15. [Google Scholar] [CrossRef]
- Au, S.; Beck, J. Subset simulation and its application to seismic risk based on dynamic analysis. J. Eng. Mech. 2003, 129, 901–917. [Google Scholar] [CrossRef]
- Lin, J. A fast CQC algorithm of PSD matrices for random seismic responses. Comput. Struct. 1992, 44, 683–687. [Google Scholar]
- Lin, J.; Zhang, W.; Williams, F. Pseudo-excitation algorithm for nonstationary random seismic responses. Eng. Struct. 1994, 16, 270–276. [Google Scholar] [CrossRef]
- Lin, J.; Li, J.; Zhang, W.; Williams, F. Random seismic responses of multi-support structures in evolutionary inhomogeneous random fields. Earthq. Eng. Struct. Dyn. 1997, 26, 135–145. [Google Scholar] [CrossRef]
- Lin, J.; Zhang, Y.; Li, Q.; Williams, F. Seismic spatial effects for long-span bridges, using the pseudo excitation method. Eng. Struct. 2004, 26, 1207–1216. [Google Scholar] [CrossRef]
- Jia, H.; Zhang, D.; Zheng, S.; Xie, W.; Pandey, M. Local site effects on a high-pier railway bridge under tridirectional spatial excitations: Nonstationary stochastic analysis. Soil Dyn. Earthq. Eng. 2013, 52, 55–69. [Google Scholar] [CrossRef]
- Zhang, D.; Jia, H.; Zheng, S.; Xie, W.; Pandey, M. A highly efficient and accurate stochastic seismic analysis approach for structures under tridirectional nonstationary multiple excitations. Comput. Struct. 2014, 145, 23–35. [Google Scholar] [CrossRef]
- Lin, J.; Zhang, Y.; Zhao, Y. Pseudo excitation method and some recent developments. Procedia Eng. 2011, 14, 2453–2458. [Google Scholar] [CrossRef]
- Muscolino, G.; Alderucci, T. Closed-form solutions for the evolutionary frequency response function of linear systems subjected to separable or non-separable non-stationary stochastic excitations. Probabilistic Eng. Mech. 2015, 40, 75–89. [Google Scholar] [CrossRef]
- Yu, H.; Wang, B.; Gao, Z.; Li, Y. An exact and efficient time-domain method for random vibration analysis of linear structures subjected to uniformly modulated or fully non-stationary excitations. J. Sound Vib. 2020, 488, 115648. [Google Scholar] [CrossRef]
- Colajanni, P.; Pagnotta, S.; Testa, G. Comparison of fully non-stationary artificial accelerogram generation methods in reproducing seismicity at a given site. Soil Dyn. Earthq. Eng. 2020, 133, 106135. [Google Scholar] [CrossRef]
- Zerva, A. Spatial Variation of Seismic Ground Motions: Modeling and Engineering Applications; Crc Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Muscolino, G.; Genovese, F.; Biondi, G.; Cascone, E. Generation of fully non-stationary random processes consistent with target seismic accelerograms. Soil Dyn. Earthq. Eng. 2021, 141, 106467. [Google Scholar] [CrossRef]
- Simos, T. High-order closed Newton–Cotes trigonometrically-fitted formulae for long-time integration of orbital problems. Comput. Phys. Commun. 2008, 178, 199–207. [Google Scholar] [CrossRef]
- Gao, Q.; Wu, F.; Zhang, H.; Zhong, W.; Howson, W.; Williams, F. A fast precise integration method for structural dynamics problems. Struct. Eng. Mech. 2012, 43, 1–13. [Google Scholar] [CrossRef]
- Kerschen, G.; Golinval, J.; Vakakis, A.; Bergman, L. The method of proper orthogonal decomposition for dynamical characterization and order reduction of mechanical systems: An overview. Nonlinear Dyn. 2005, 41, 147–169. [Google Scholar] [CrossRef]
- Cacciola, P.; Deodatis, G. A method for generating fully non-stationary and spectrum-compatible ground motion vector processes. Soil Dyn. Earthq. Eng. 2011, 31, 351–360. [Google Scholar] [CrossRef]
- Du, X.; Xu, Y.; Xia, H. Dynamic interaction of bridge–train system under non-uniform seismic ground motion. Earthq. Eng. Struct. Dyn. 2012, 41, 139–157. [Google Scholar] [CrossRef]
- Huang, G. Application of proper orthogonal decomposition in fast fourier transform-assisted multivariate nonstationary process simulation. J. Eng. Mech. 2015, 141, 04015015. [Google Scholar] [CrossRef]
- Li, Y.; Togbenou, K.; Xiang, H.; Chen, N. Simulation of non-stationary wind velocity field on bridges based on Taylor series. J. Wind. Eng. Ind. Aerodyn. 2017, 169, 117–127. [Google Scholar] [CrossRef]
- Zhao, N.; Huang, G. Fast simulation of multivariate nonstationary process and its application to extreme winds. J. Wind. Eng. Ind. Aerodyn. 2017, 170, 118–127. [Google Scholar] [CrossRef]
- Wang, H.; Xu, Z.; Feng, D.; Tao, T. Non-stationary turbulent wind field simulation of bridge deck using non-negative matrix factorization. J. Wind. Eng. Ind. Aerodyn. 2019, 188, 235–246. [Google Scholar] [CrossRef]
- Deodatis, G. Non-stationary stochastic vector processes: Seismic ground motion applications. Probabilistic Eng. Mech. 1996, 11, 149–167. [Google Scholar] [CrossRef]
- Dong, L.; Liao, M.; Yang, S. Filtering modification of vibration signal integration algorism. Mach. Des. Manuf. 2010, 1, 46–48. [Google Scholar]
- Gu, M.; Lv, Z. Identification of a mechanism’s vibration velocity and displacement based on the acceleration measurement. Mech. Sci. Technol. Aerosp. 2011, 30, 522–526. [Google Scholar]
- Liang, R.; Wang, H.; Li, J.; Gao, H.; Zheng, W.; Xu, Z. Multiple tuned inerter-based dampers for seismic response mitigation of continuous girder bridges. Soil Dyn. Earthq. Eng. 2021, 151, 106954. [Google Scholar] [CrossRef]
- Crespi, P.; Zucca, M.; Valente, M.; Longarini, N. Influence of corrosion effects on the seismic capacity of existing RC bridges. Eng. Fail. Anal. 2022, 140, 106546. [Google Scholar] [CrossRef]
- Berto, L.; Vitaliani, R.; Saetta, A.; Simioni, P. Seismic assessment of existing RC structures affected by degradation phenomena. Struct. Saf. 2009, 31, 284–297. [Google Scholar] [CrossRef]
- An, L.; Li, D.; Yu, P.; Yuan, P. Numerical analysis of dynamic response of vehicle-bridge coupled system on long-span continuous girder bridge. Theor. Appl. Mech. Lett. 2016, 6, 186–194. [Google Scholar] [CrossRef][Green Version]
- Hu, L.; Xu, Y.; Huang, W. Typhoon-induced non-stationary buffeting response of long-span bridges in complex terrain. Eng. Struct. 2013, 57, 406–415. [Google Scholar] [CrossRef]





| Algorithm: determine the pseudo static displacement under lth pseudo excitation |
| for |
| 1. Calculating and using POD. |
| 2. Conducting curve fitting of with Equation (28). |
| for |
| 3. Solving the analytical solution with Equation (30). |
| 4. Conducting multiplication of with remainder in Equation (29). |
| end |
| end |
| No. | Frequency (Hz) | Characteristics of Modes | Modal Mass Participation Ratios (%) | |
|---|---|---|---|---|
| FEM | Design | |||
| 1 | 1.1291 | 1.0096 | Symmetric lateral bending vibration of the girder | 13.06 (y direction) |
| 2 | 1.1774 | 1.1334 | Longitudinal bending vibration of the pier | 91.89 (x direction) |
| 3 | 1.1824 | 1.2705 | Symmetric vertical bending vibration of the girder | -- |
| 4 | 1.9482 | 1.5872 | Lateral bending vibration of one side span | 14.13 (y direction) |
| 5 | 2.1495 | 1.7277 | Lateral bending vibration of the other side span | 55.22 (y direction) |
| 6 | 2.6168 | 2.3257 | Anti-symmetric lateral bending vibration of the girder | -- |
| 7 | 2.7394 | 2.6887 | Anti-symmetric vertical bending vibration of the girder | 0.11 (y direction) |
| 8 | 3.5933 | 3.2899 | Second symmetric lateral bending vibration | -- |
| 9 | 3.8228 | 3.6131 | Second symmetric vertical bending vibration | 7.00 (y direction) |
| 10 | 4.0072 | 4.0078 | Second Anti-symmetric lateral bending vibration of the girder | 2.16 (x direction) |
| p | αp | βp | γp | ζp | νp | ηp |
|---|---|---|---|---|---|---|
| 1 | 0.2358 | 7 | 1.3375 | 0.8672 | 1.2618 | 3.1022 |
| 2 | 0.1394 | 8 | 1.4116 | 5.5717 | 1.5856 | 4.8962 |
| 3 | 130.9862 | 10 | 4.2028 | 12.4408 | 1.9182 | 4.172 |
| 4 | 3.3724 | 9 | 2.5692 | 15.1802 | 1.9097 | 2.7679 |
| 5 | 1.0659 | 2 | 0.1612 | −1.515 | 1.2 | 3.4588 |
| 6 | 71.7647 | 2 | 0.8956 | 9.8679 | 1.2 | 11.0855 |
| 7 | 0.0044 | 11 | 1.7482 | −1.0149 | 2.0905 | 14.1349 |
| 8 | 0.2012 | 11 | 2.4117 | 3.8821 | 2.9589 | 16.4059 |
| 9 | 3.7529 | 11 | 3.0778 | 8.731 | 1.358 | 9.411 |
| 10 | 0.4901 | 11 | 2.6406 | 1.4716 | 2.4796 | 19.2596 |
| 11 | 12.6339 | 3 | 0.762 | 6.1032 | 1.2927 | 20.2079 |
| 12 | 4.1843 | 5 | 1.1922 | −0.1155 | 2.7419 | 31.4748 |
| 13 | 5.8917 | 5 | 1.3786 | 5.4048 | 1.3335 | 28.7928 |
| 14 | 2.1934 | 5 | 1.2384 | −0.1895 | 1.8083 | 43.085 |
| 15 | 20.3968 | 5 | 1.7774 | 5.449 | 4.3403 | 37.5139 |
| Pier | 1# Pier | 2# Pier | 3# Pier | 4# Pier | |
|---|---|---|---|---|---|
| Model | |||||
| Fully non-stationary | 3.730 | 17.535 | 11.729 | 9.431 | |
| Modulated non-stationary | 3.122 | 14.687 | 9.828 | 7.900 | |
| Pier | 1# Pier | 2# Pier | 3# Pier | 4# Pier | |
|---|---|---|---|---|---|
| Model | |||||
| Fully non-stationary | 2.237 | 8.593 | 6.574 | 4.098 | |
| Modulated non-stationary | 1.873 | 7.196 | 5.506 | 3.432 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xing, C.; Wang, H.; Xu, Z.; Liang, R. Stochastic Analysis of a Large-Span Continuous Girder High-Speed Railway Bridge under Fully Non-Stationary Earthquake. Appl. Sci. 2022, 12, 12684. https://doi.org/10.3390/app122412684
Xing C, Wang H, Xu Z, Liang R. Stochastic Analysis of a Large-Span Continuous Girder High-Speed Railway Bridge under Fully Non-Stationary Earthquake. Applied Sciences. 2022; 12(24):12684. https://doi.org/10.3390/app122412684
Chicago/Turabian StyleXing, Chenxi, Hao Wang, Zidong Xu, and Ruijun Liang. 2022. "Stochastic Analysis of a Large-Span Continuous Girder High-Speed Railway Bridge under Fully Non-Stationary Earthquake" Applied Sciences 12, no. 24: 12684. https://doi.org/10.3390/app122412684
APA StyleXing, C., Wang, H., Xu, Z., & Liang, R. (2022). Stochastic Analysis of a Large-Span Continuous Girder High-Speed Railway Bridge under Fully Non-Stationary Earthquake. Applied Sciences, 12(24), 12684. https://doi.org/10.3390/app122412684







