Effect of Controlled Muscle Activation in a Unilateral Vocal Fold Polyp Setting on Vocal Fold Vibration
Abstract
1. Introduction
2. Materials and Methods
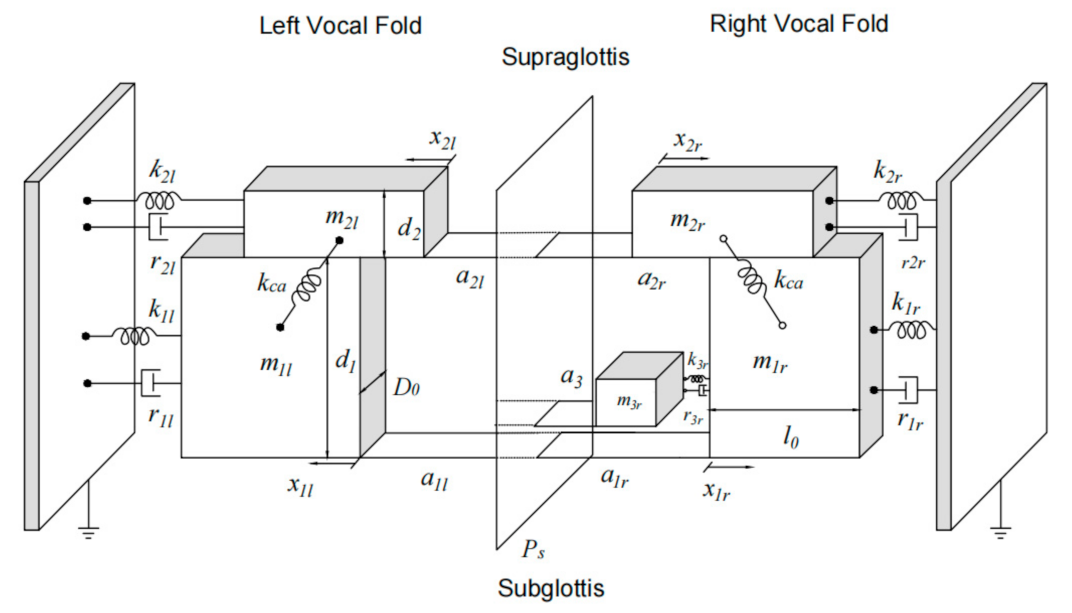
2.1. Vocal Fold Model with a Unilateral Polyp
2.2. Development of Rules for Muscle Control
2.3. Effective Parameters of Vocal Fold Vibration
2.4. Equations of Motion for the Three-Mass Model
3. Results and Discussion
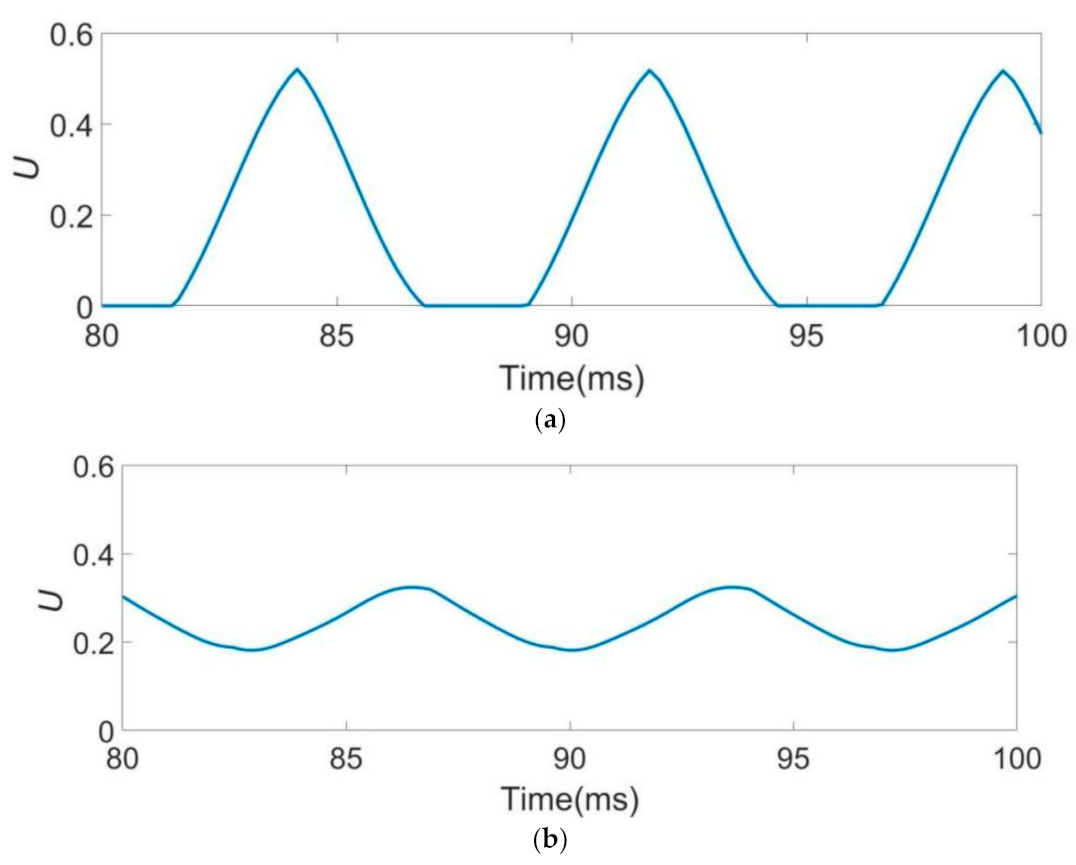
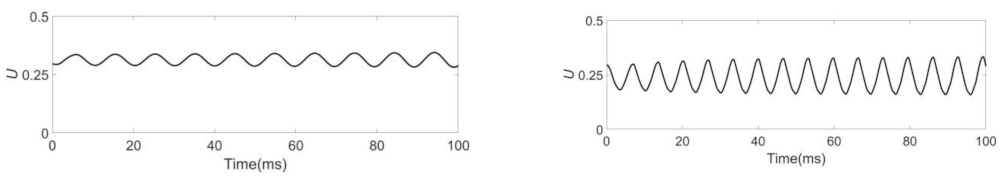
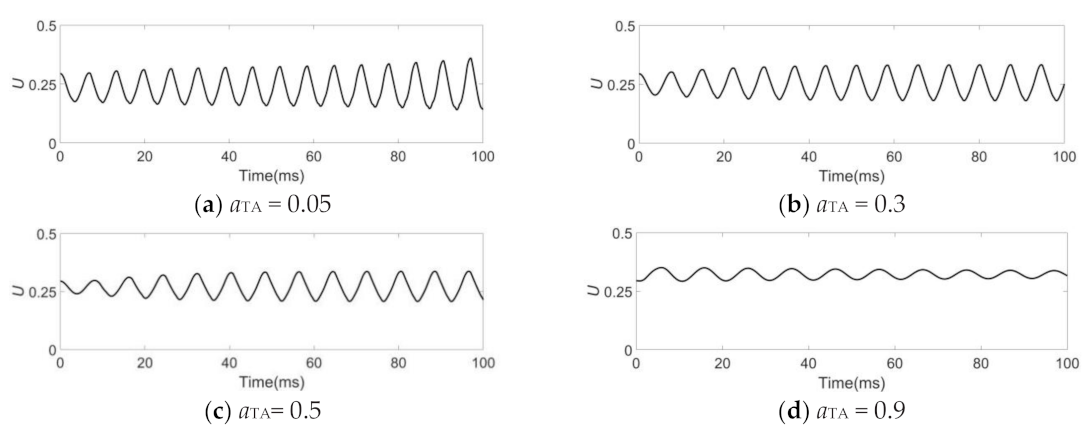
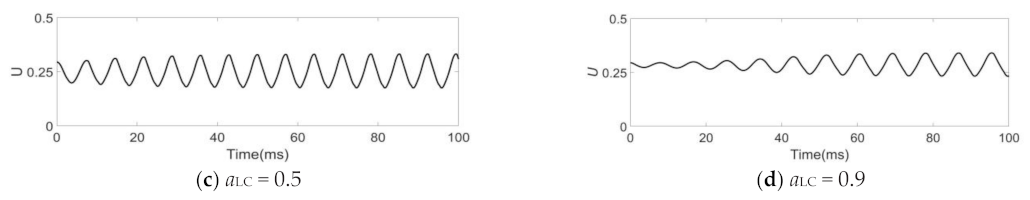
3.1. Qualitative Features of Pathological Vocal Fold
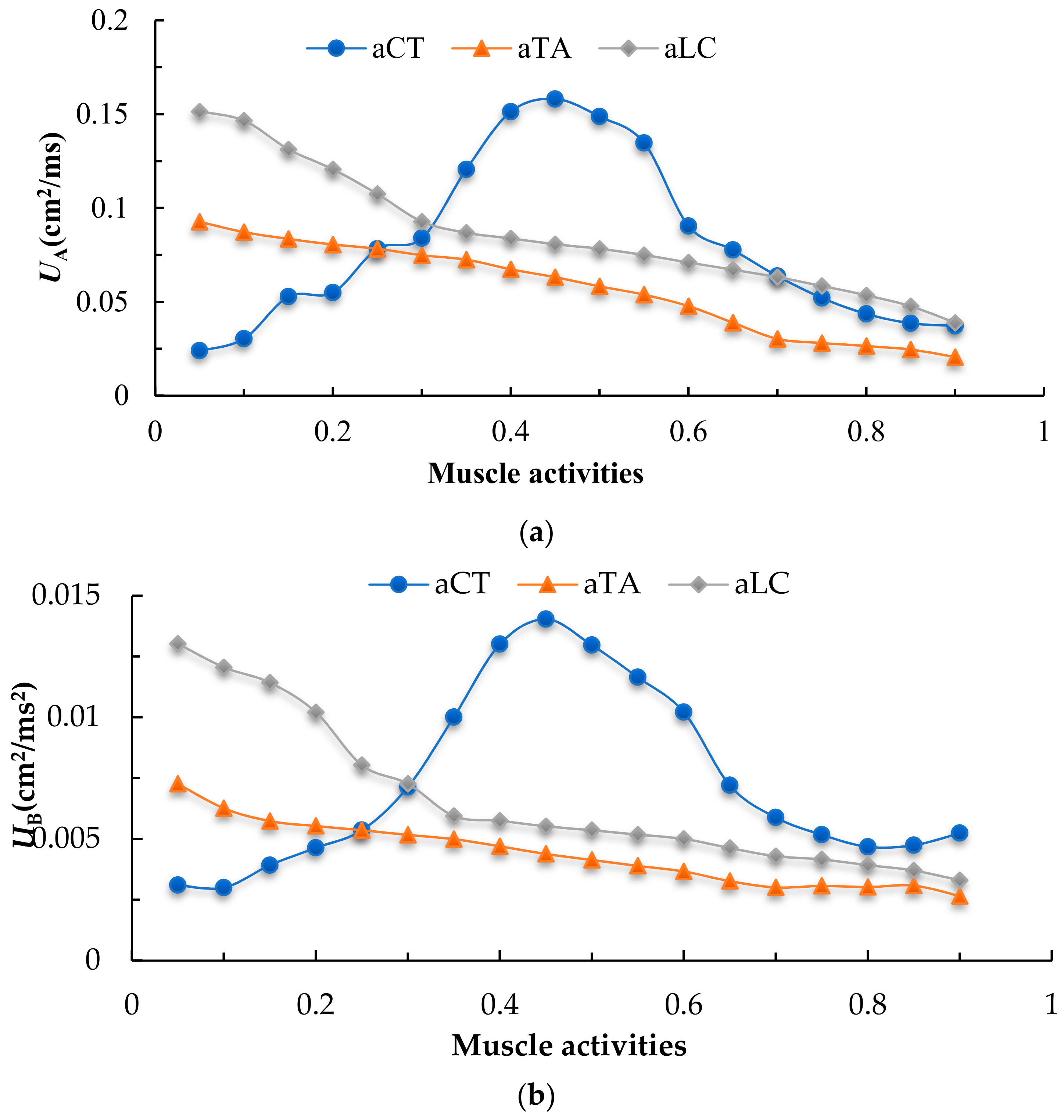
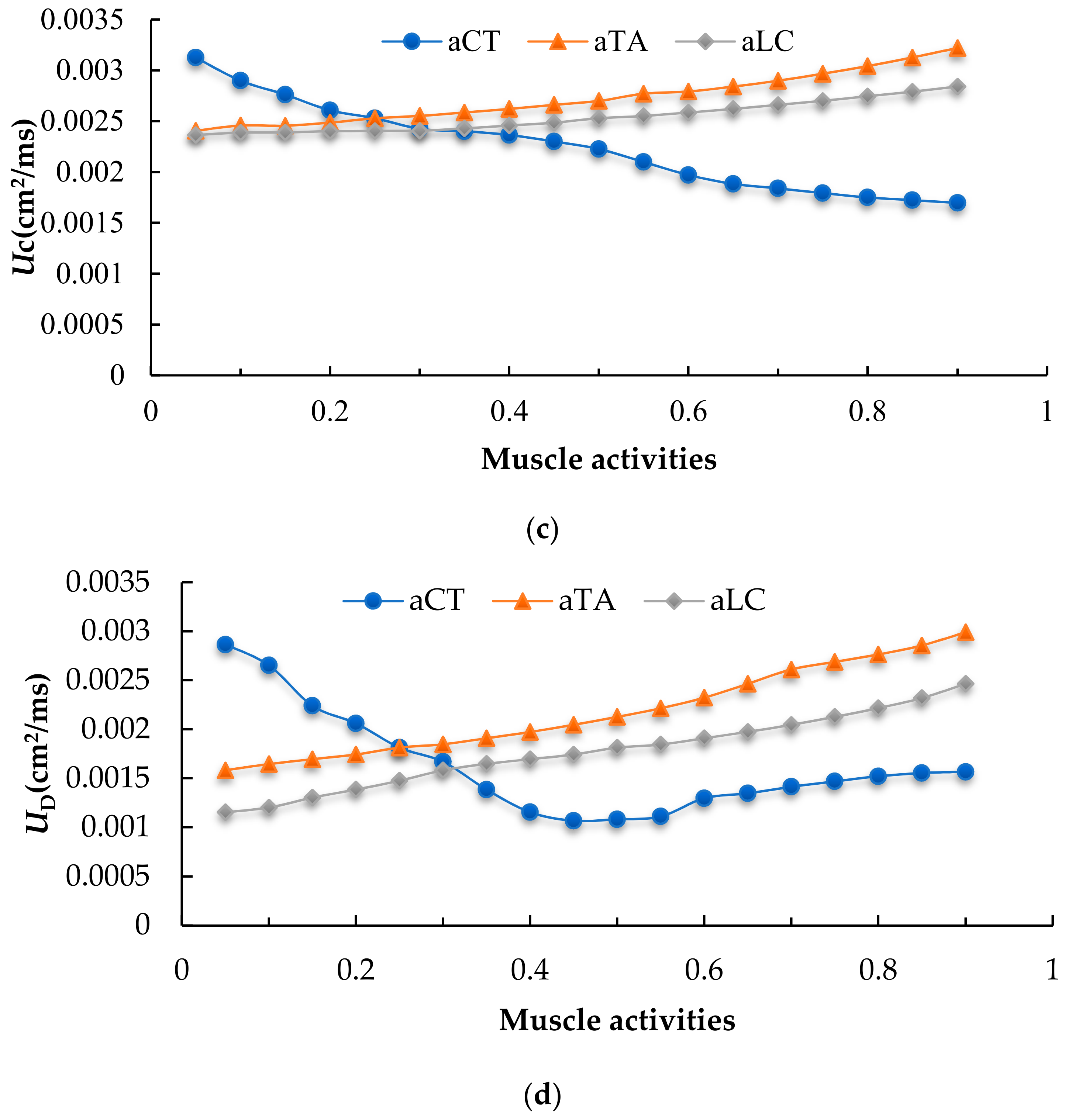
3.2. Glottal Flow and Implications for Phonation
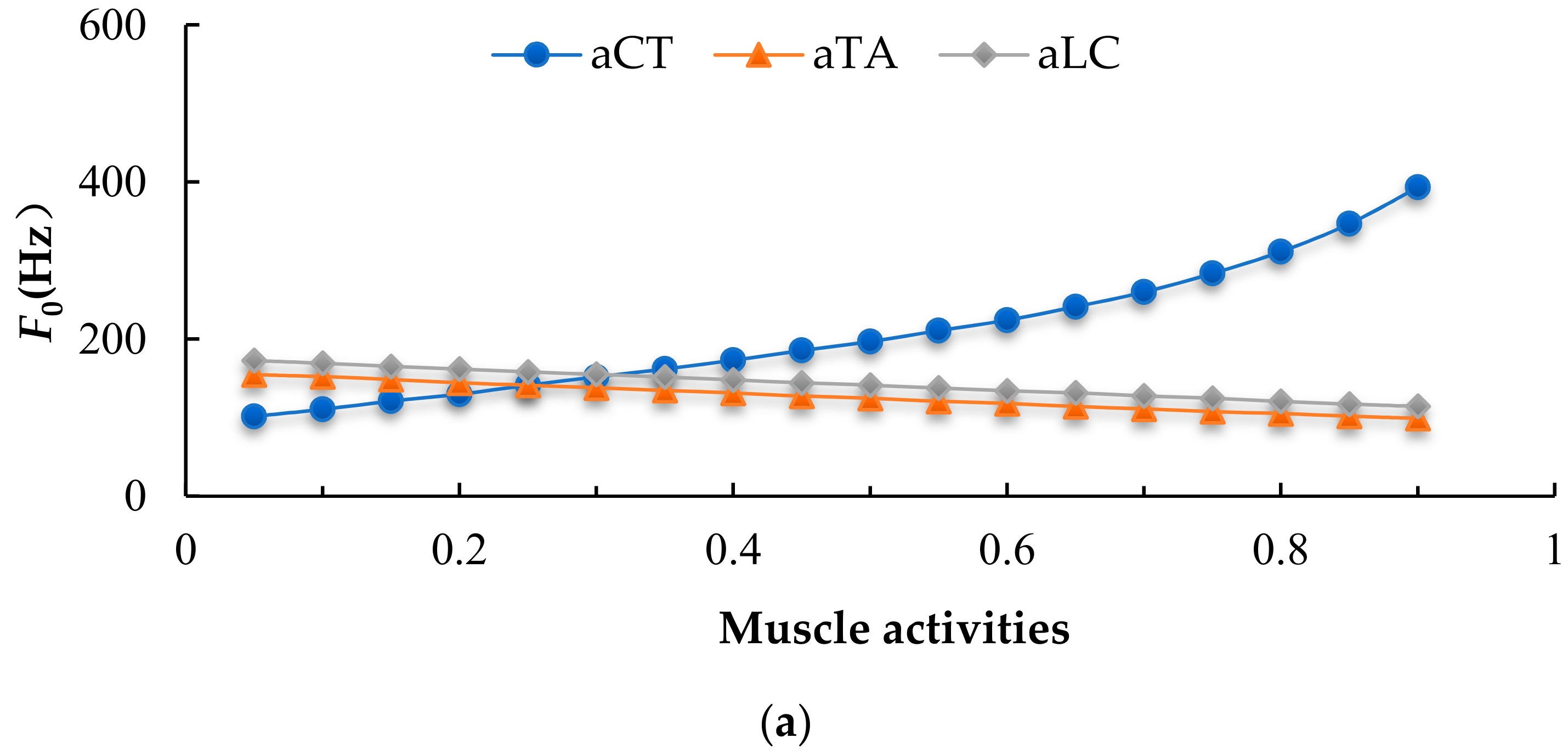
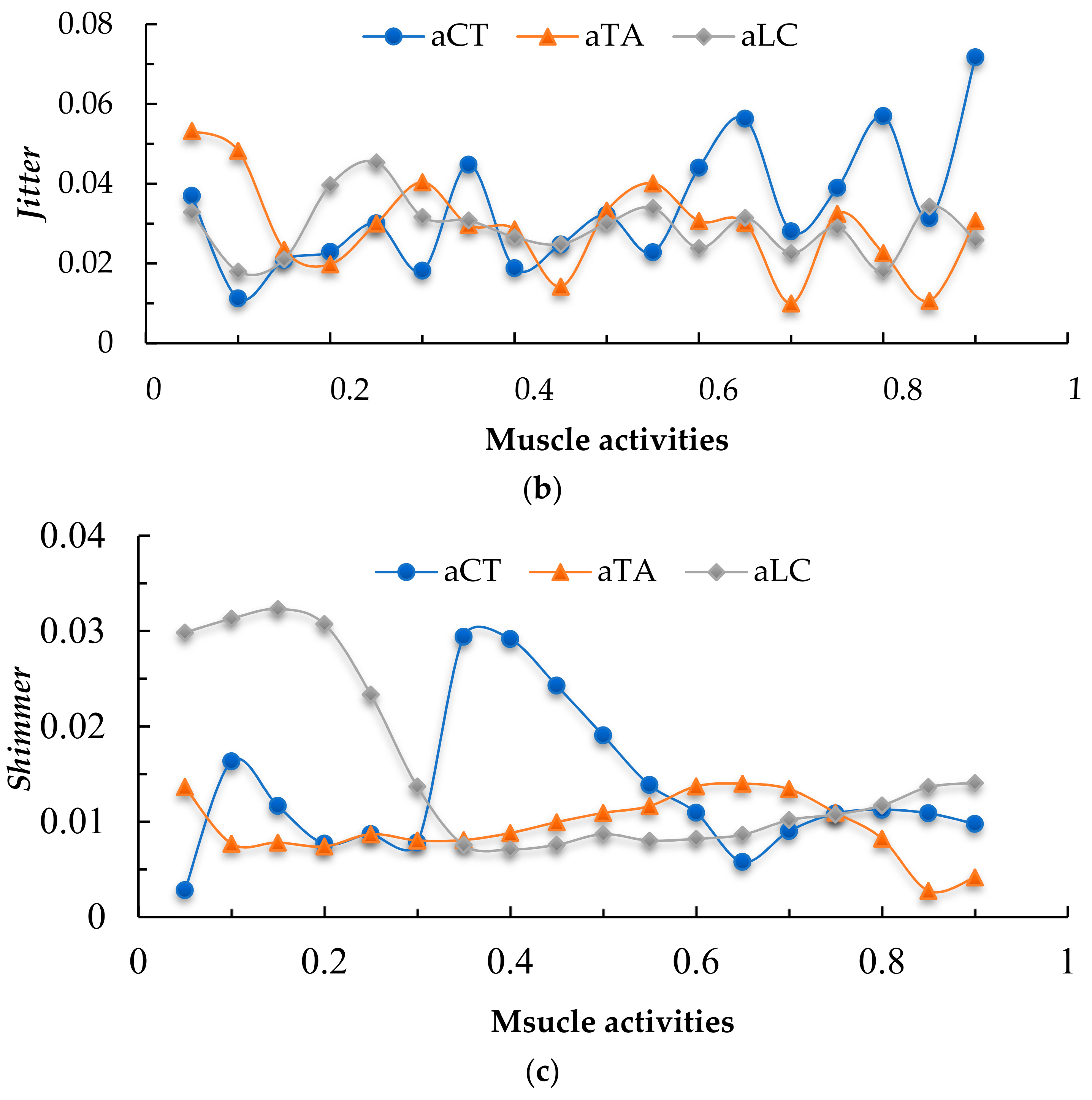
3.3. Fundamental Frequency and Related Perturbation Parameters
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vasconcelos, D.; Gomes, A.O.C.; Araújo, C.M.T. Vocal Fold Polyps: Literature Review. Int. Arch. Otorhinolaryngol. 2019, 23, 116–124. [Google Scholar] [CrossRef] [PubMed]
- Dikkers, F.G.; Nikkels, P.G.J. Benign lesions of the vocal folds: Histopathology and phonotrauma. Ann. Otol. Rhinol. Laryngol. 1995, 104, 698–703. [Google Scholar] [CrossRef] [PubMed]
- Cancer Stat Facts: Laryngeal Cancer. Available online: https://seer.cancer.gov/statfacts/html/laryn.html (accessed on 1 December 2022).
- Greiss, R.; Rocha, J.; Matida, E. Validation of a finite element for a continuum model of a vocal fold vibration under the influence of a sessile polyp. Can. Acoust. 2015, 43, 13–23. [Google Scholar]
- Mimica, X.; Hanson, M.; Patel, S.G.; McGill, M. Salvagesurgery for recurrent larynx cancer. Head Neck 2019, 41, 3906–3915. [Google Scholar] [CrossRef]
- Tsetsos, N.; Poutoglidis, A.; Vlachtsis, K. Twenty-year experience with salvage total laryngectomy: Lessons learned. J. Laryngol. Otol. 2021, 135, 729–736. [Google Scholar] [CrossRef]
- Vlot, C.; Ogawa, M.; Hosokawa, K.; Iwahashi, T. Investigation of the immediate effects of humming on vocal fold irregularity using electroglottography and high-speed laryngoscopy in patients with organic voice disorders. J. Voice 2017, 31, 48–56. [Google Scholar] [CrossRef]
- Lee, Y.S.; Lee, D.H.; Jeong, G.E.; Kim, J.W. Treatment Efficacy of Voice Therapy for Vocal Fold Polyps and Factors Predictive of Its Efficacy. J. Voice 2017, 31, 120. [Google Scholar] [CrossRef]
- Ishizaka, K.; Flanagan, J.L. Synthesis of voiced sounds from a two-mass model of the vocal cords. Bell Syst. Tech. J. 1972, 51, 1233–1268. [Google Scholar] [CrossRef]
- Story, B.H.; Titze, I.R. Voice simulation with a body-cover model of the vocal folds. J. Acoust. Soc. Am. 1995, 97, 1249–1260. [Google Scholar] [CrossRef]
- Zhang, Y.; Jiang, J.J. Chaotic vibrations of a vocal fold model with a unilateral polyp. J. Acoust. Soc. Am. 2004, 115, 1266–1269. [Google Scholar] [CrossRef]
- Xue, Q.; Mittal, R.; Zheng, X. A computational study of the effect of vocal-fold asymmetry on phonation. J. Acoust. Soc. Am. 2010, 128, 818–827. [Google Scholar] [CrossRef] [PubMed]
- Mehta, D.D.; Zanartu, M.; Quatieri, T.F. Investigating acoustic correlates of human vocal fold vibratory phase asymmetry through modeling and laryngeal high-speed videoendoscopy. J. Acoust. Soc. Am. 2010, 130, 3999–4009. [Google Scholar] [CrossRef] [PubMed]
- Chhetri, D.K.; Park, S.J. Interactions of subglottal pressure and neuromuscular activation on fundamental frequency and intensity. Laryngoscope 2016, 126, 1123–1130. [Google Scholar] [CrossRef] [PubMed]
- Dewan, K.; Vahabzadeh-Hagh, A.; Soofer, D.; Chhetri, D.K. Neuromuscular Compensation Mechanisms in Vocal Fold Paralysis and Paresis. Laryngoscope 2017, 127, 1633–1638. [Google Scholar] [CrossRef]
- Azar, S.S.; Chhetri, D.K. Phonation Threshold Pressure Revisited: Effects of Intrinsic Laryngeal Muscle Activation. Laryngoscope 2021, 132, 1427–1432. [Google Scholar] [CrossRef]
- Titze, I.R. On the mechanics of vocal-fold vibration. J. Acoust. Soc. Am. 1976, 60, 1366–1380. [Google Scholar] [CrossRef]
- Herzel, H.; Berry, D.; Titze, I.; Steinecke, I. Nonlinear dynamics of the voice: Signal analysis and biomechanical modeling. Chaos 1995, 5, 30–34. [Google Scholar] [CrossRef]
- Titze, I.R.; Story, B.H. Rules for controlling low-dimensional vocal fold models with muscle activation. J. Acoust. Soc. Am. 2002, 112, 1064–1076. [Google Scholar] [CrossRef]
- Zhang, Z.Y. Vocal instabilities in a three-dimensional body-cover phonation model. J. Acoust. Soc. Am. 2019, 143, 1216–1230. [Google Scholar] [CrossRef]
- Titze, I.R. Principles of Voice Production, 2nd ed.; Prentice Hall: Iowa City, ID, USA, 2000; pp. 211–238. [Google Scholar]
- Titze, I.R.; Jiang, J.; Druker, D. Preliminaries to the body cover theory of pitch control. J. Voice 1988, 1, 314–319. [Google Scholar] [CrossRef]
- Alipour-Haghighi, F.; Titze, I.R. Elastic models of vocal fold tissues. J. Acoust. Soc. Am. 1991, 90, 1326–1331. [Google Scholar] [CrossRef] [PubMed]
- Min, Y.; Titze, I.R.; Alipour-Haghighi, F. Stress-strain response of the human vocal ligament. Ann. Otol. Rhinol. Laryngol. 1995, 104, 563–569. [Google Scholar] [CrossRef] [PubMed]
- Jiang, J.J.; Zhang, Y.; Stern, J. Modeling of chaotic vibrations in symmetric vocal folds. J. Acoust. Soc. Am. 2001, 110, 2120–2128. [Google Scholar] [CrossRef] [PubMed]
- Teixeira, J.P.; Goncalves, A. Accuracy of jitter and shimmer measurements. Procedia Technol. 2014, 16, 1190–1199. [Google Scholar] [CrossRef]
- Wu, L.; Zhang, Z. A parametric vocal fold model based on magnetic resonance imaging. J. Acoust. Soc. Am. 2016, 140, 159–165. [Google Scholar] [CrossRef]


| Parameter | Symbol | Value |
|---|---|---|
| Lower mass | m1a | 0.125 |
| Upper mass | m2a | 0.05 |
| Lower stiffness constant | k1a | 0.08 |
| Upper stiffness constant | k2a | 0.008 |
| Coupling stiffness constant | kca | 0.025 |
| Lower mass thickness | d1 | 0.25 |
| Upper mass thickness | d2 | 0.05 |
| Thickness of the vocal fold | d0 | 0.3 |
| Length of the vocal fold | l0 | 1.4 |
| Depth of the vocal fold | D0 | 0.3 |
| Lower mass spring constant during vocal fold collisions | c1a | 0.24 |
| Upper mass spring constant during vocal fold collisions | c2a | 0.024 |
| Air density | ρ | 0.00113 |
| Subglottal pressure | Ps | 0.006 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, M.; Liu, B.; Jiang, J.; Hoffman, M.R.; Lan, J.; Fang, J. Effect of Controlled Muscle Activation in a Unilateral Vocal Fold Polyp Setting on Vocal Fold Vibration. Appl. Sci. 2022, 12, 12486. https://doi.org/10.3390/app122312486
Ji M, Liu B, Jiang J, Hoffman MR, Lan J, Fang J. Effect of Controlled Muscle Activation in a Unilateral Vocal Fold Polyp Setting on Vocal Fold Vibration. Applied Sciences. 2022; 12(23):12486. https://doi.org/10.3390/app122312486
Chicago/Turabian StyleJi, Mingjun, Boquan Liu, Jack Jiang, Matthew R. Hoffman, Jinwei Lan, and Jin Fang. 2022. "Effect of Controlled Muscle Activation in a Unilateral Vocal Fold Polyp Setting on Vocal Fold Vibration" Applied Sciences 12, no. 23: 12486. https://doi.org/10.3390/app122312486
APA StyleJi, M., Liu, B., Jiang, J., Hoffman, M. R., Lan, J., & Fang, J. (2022). Effect of Controlled Muscle Activation in a Unilateral Vocal Fold Polyp Setting on Vocal Fold Vibration. Applied Sciences, 12(23), 12486. https://doi.org/10.3390/app122312486






