Test of Broken Suspender Specimen and Equivalent Static Calculation Method for Half-Through and Through Concrete-Filled Steel Tubular Arch Bridges
Abstract
:1. Introduction
2. The Broken Suspender Specimen Test of the Through CFST Arch Bridge
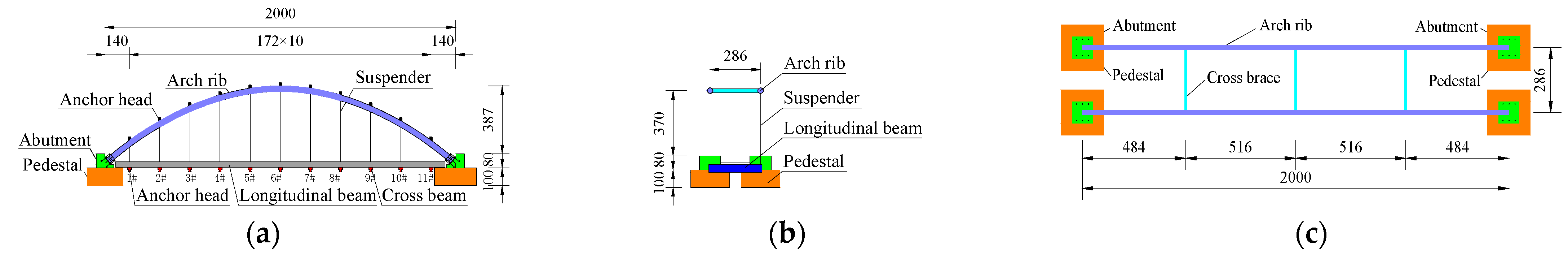
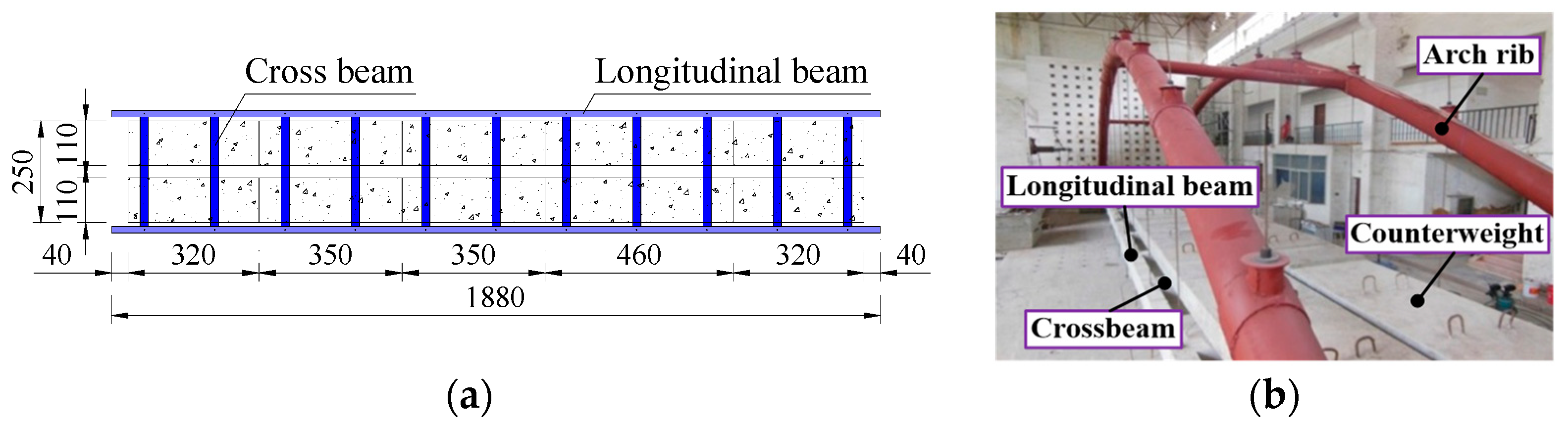
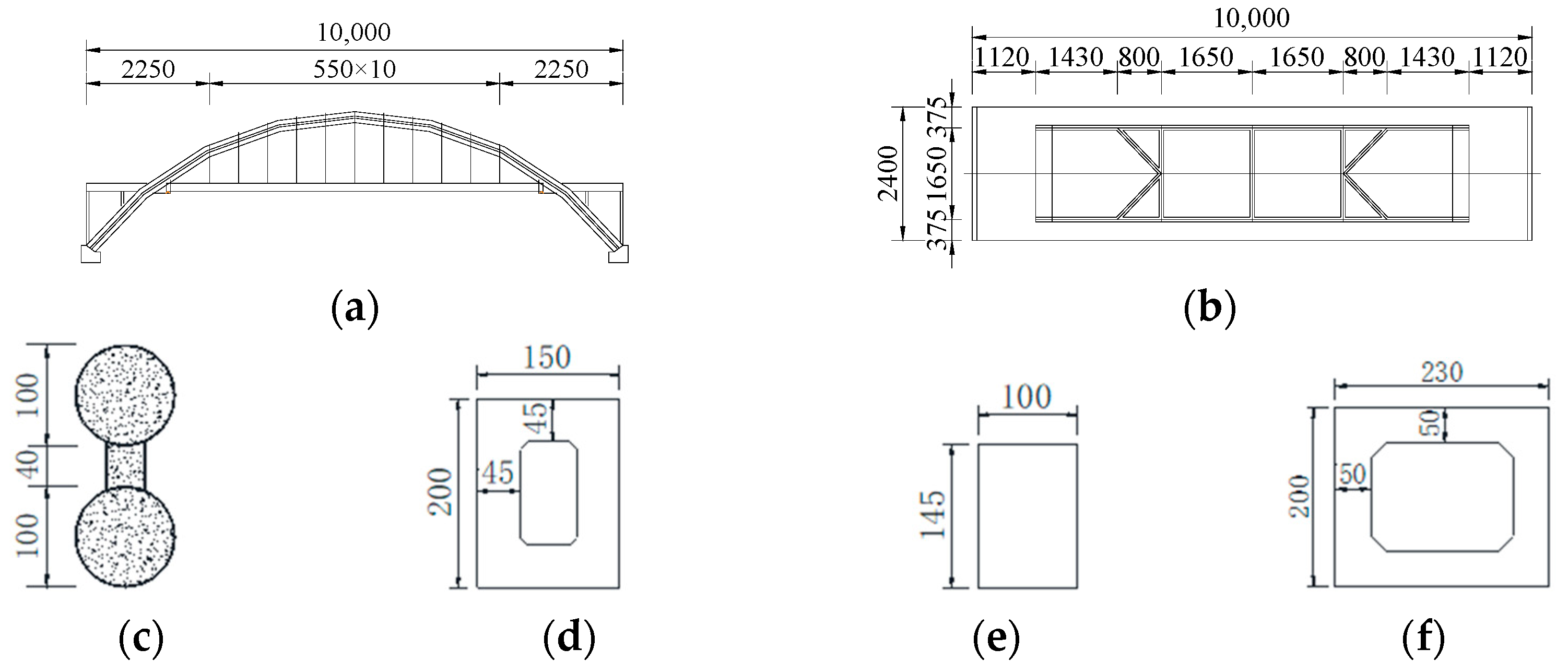
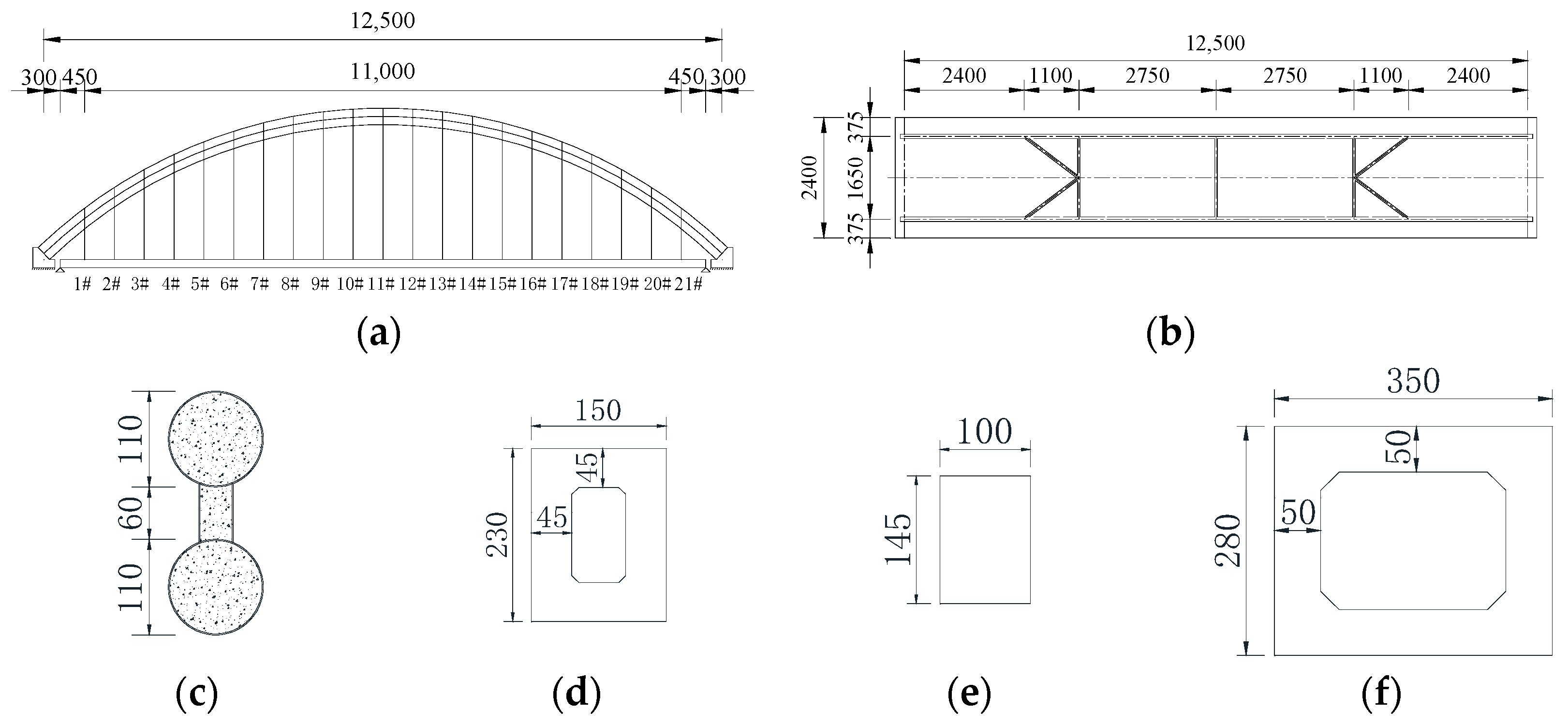
2.1. Specimen Design
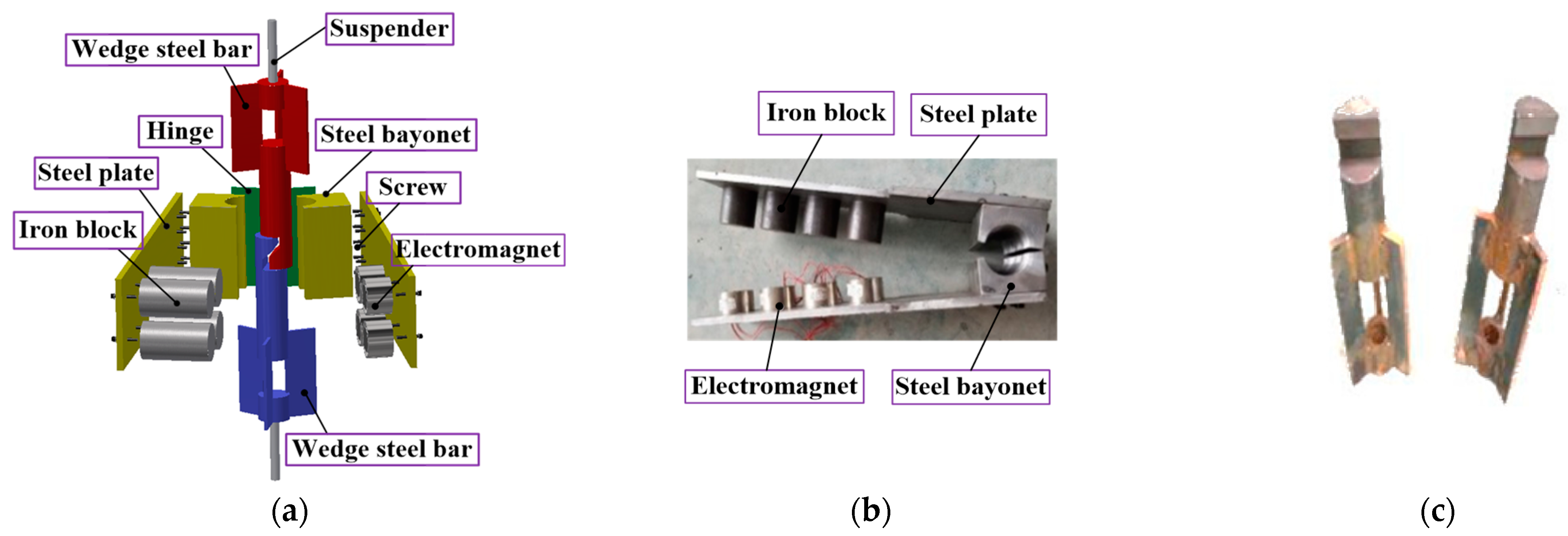
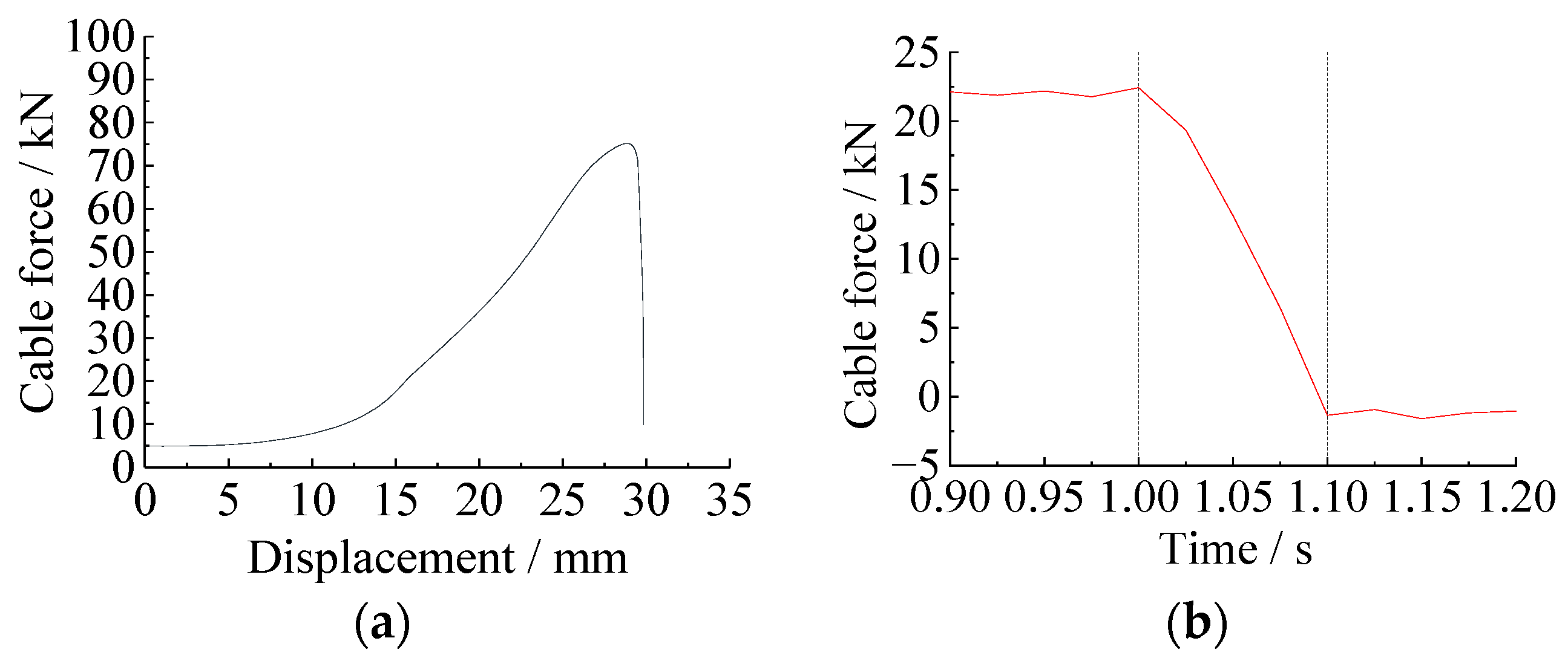
2.2. Transient Fracture Simulation of the Suspender
2.3. Suspender Fracture Condition
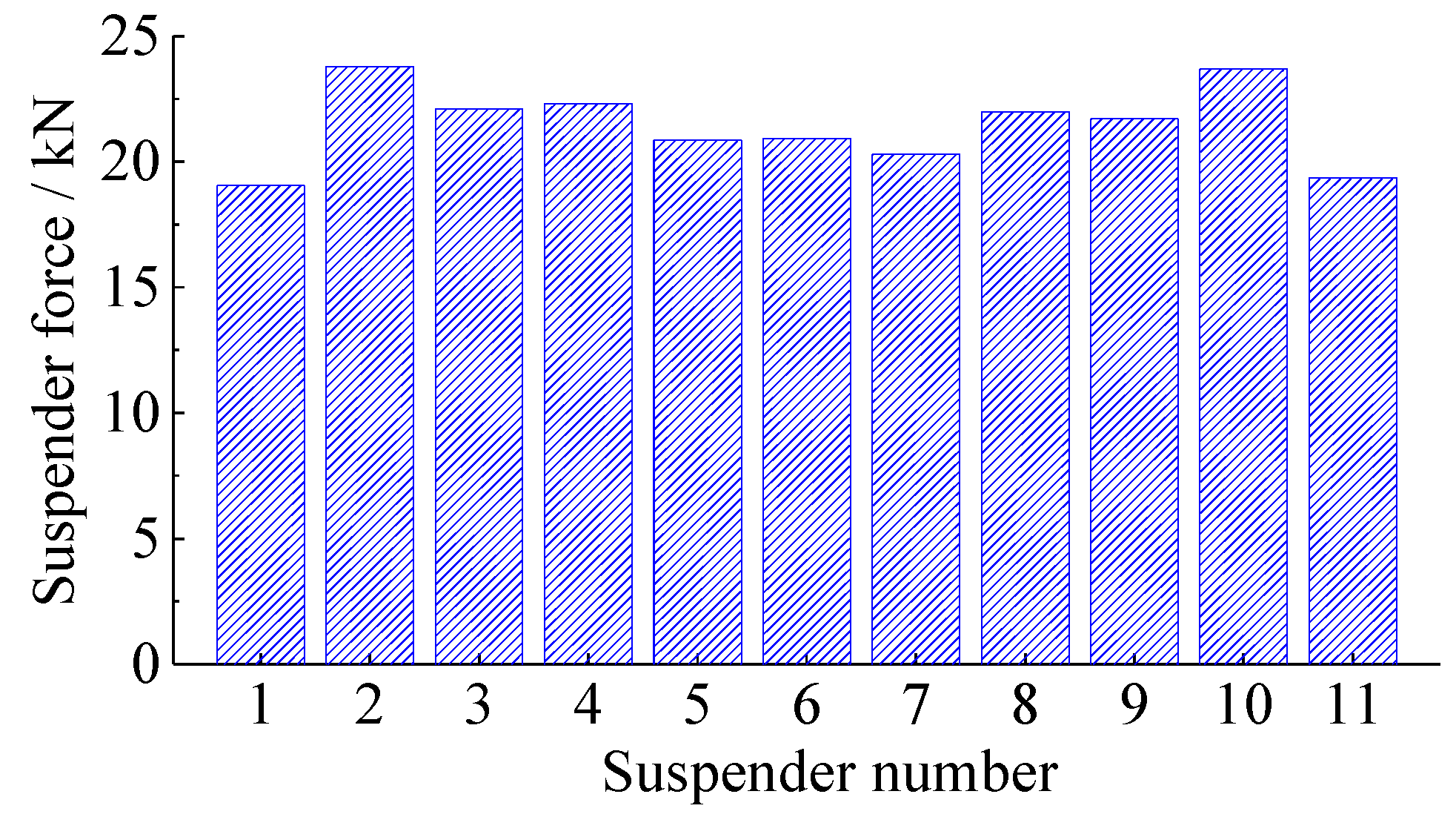
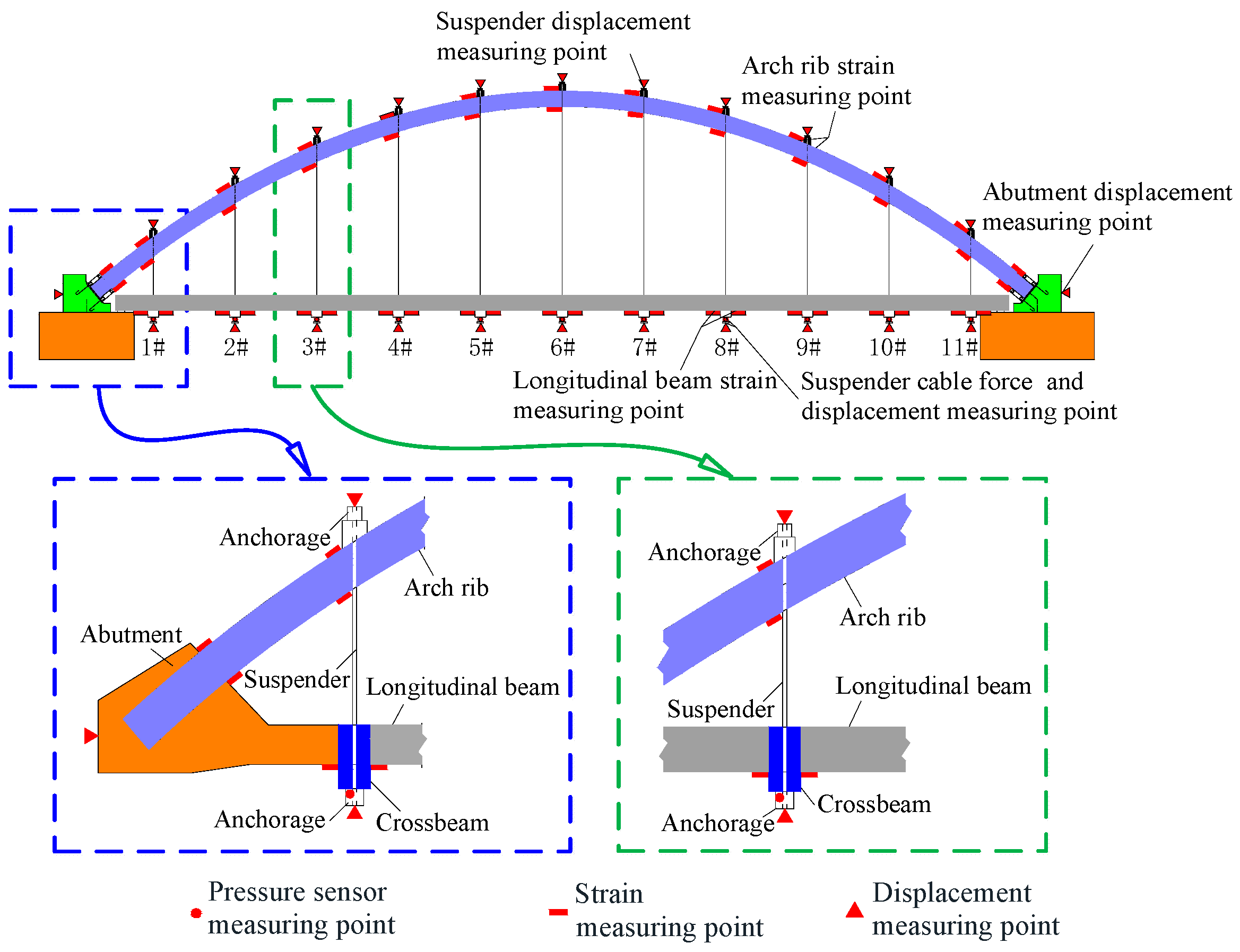
2.4. The Layouts of the Measuring Points
3. The FEM-Base Model Accounting for the Suspender Breaking Process
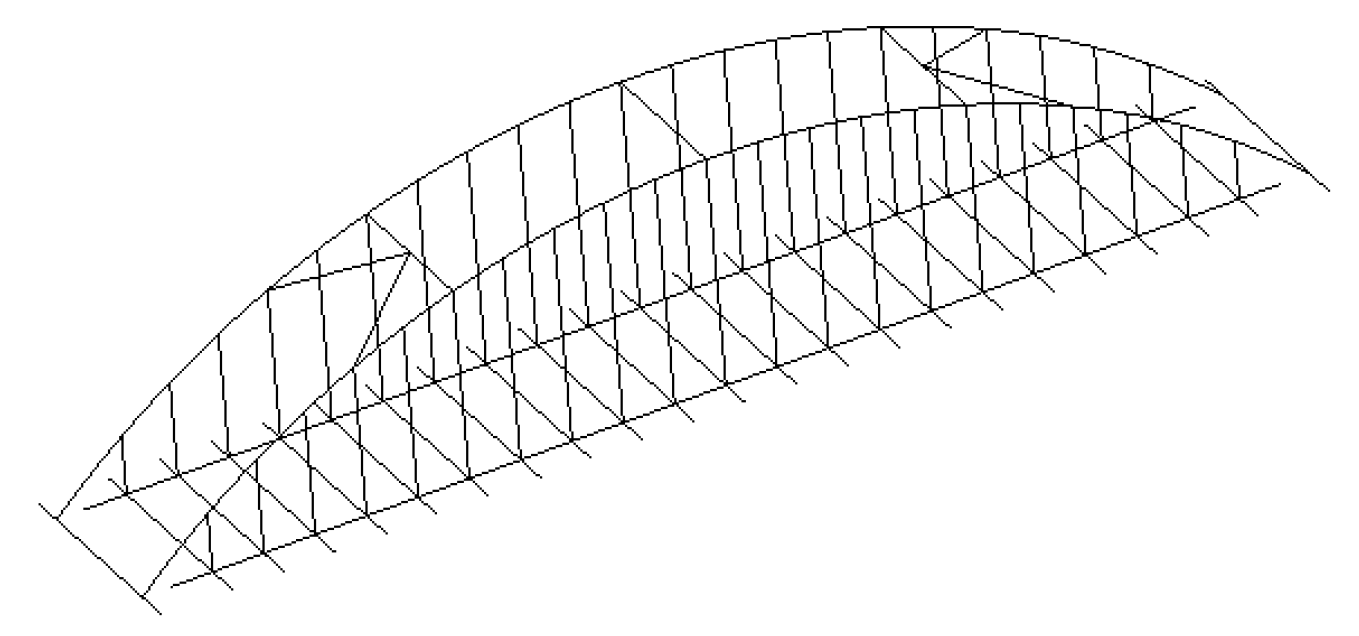
3.1. Establishment of the FE Model
3.2. Suspender Fracture Simulation
4. Test and FE Analysis
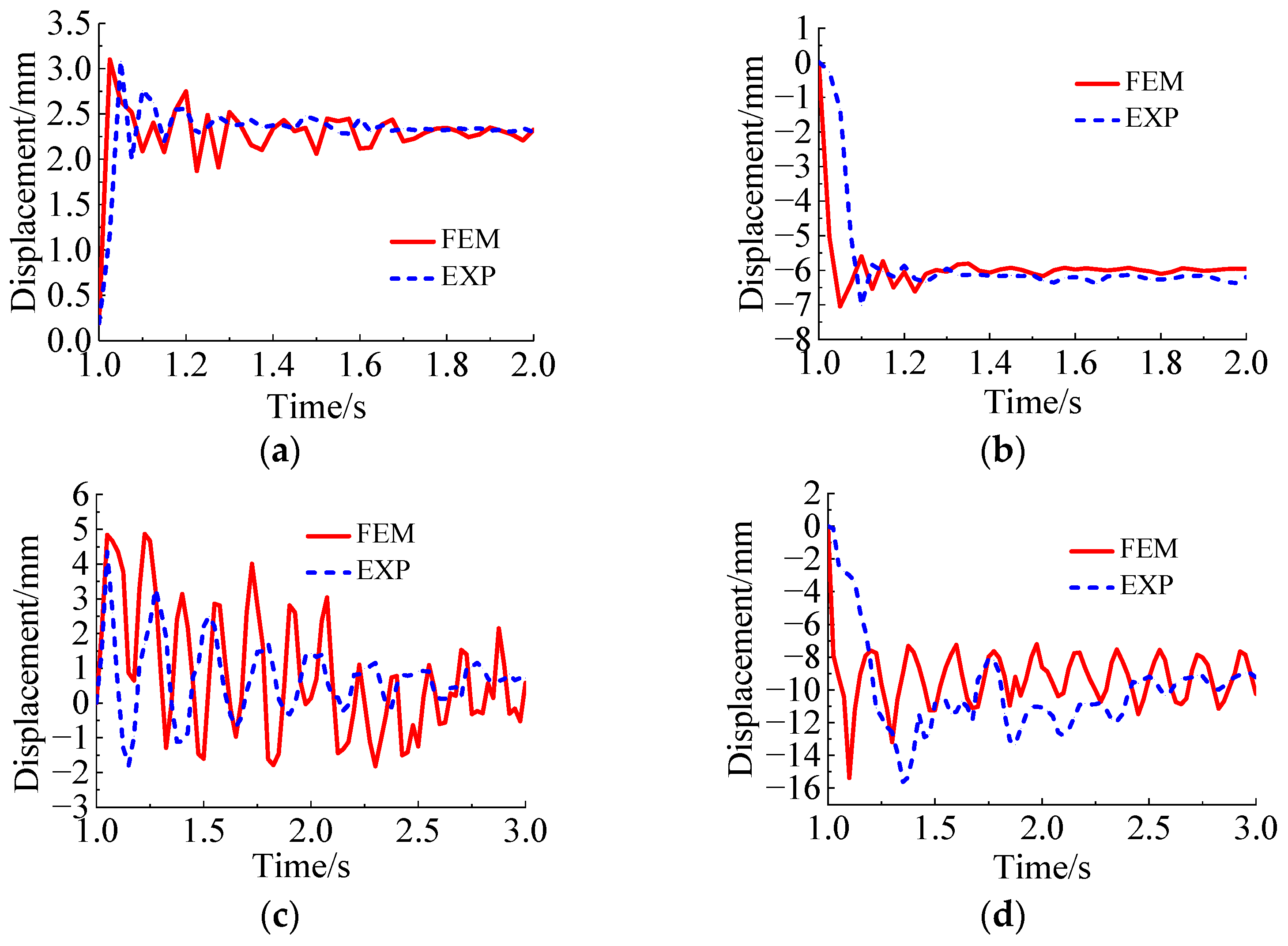
4.1. Displacement Dynamic Response Analysis
4.2. Stress Dynamic Response Analysis
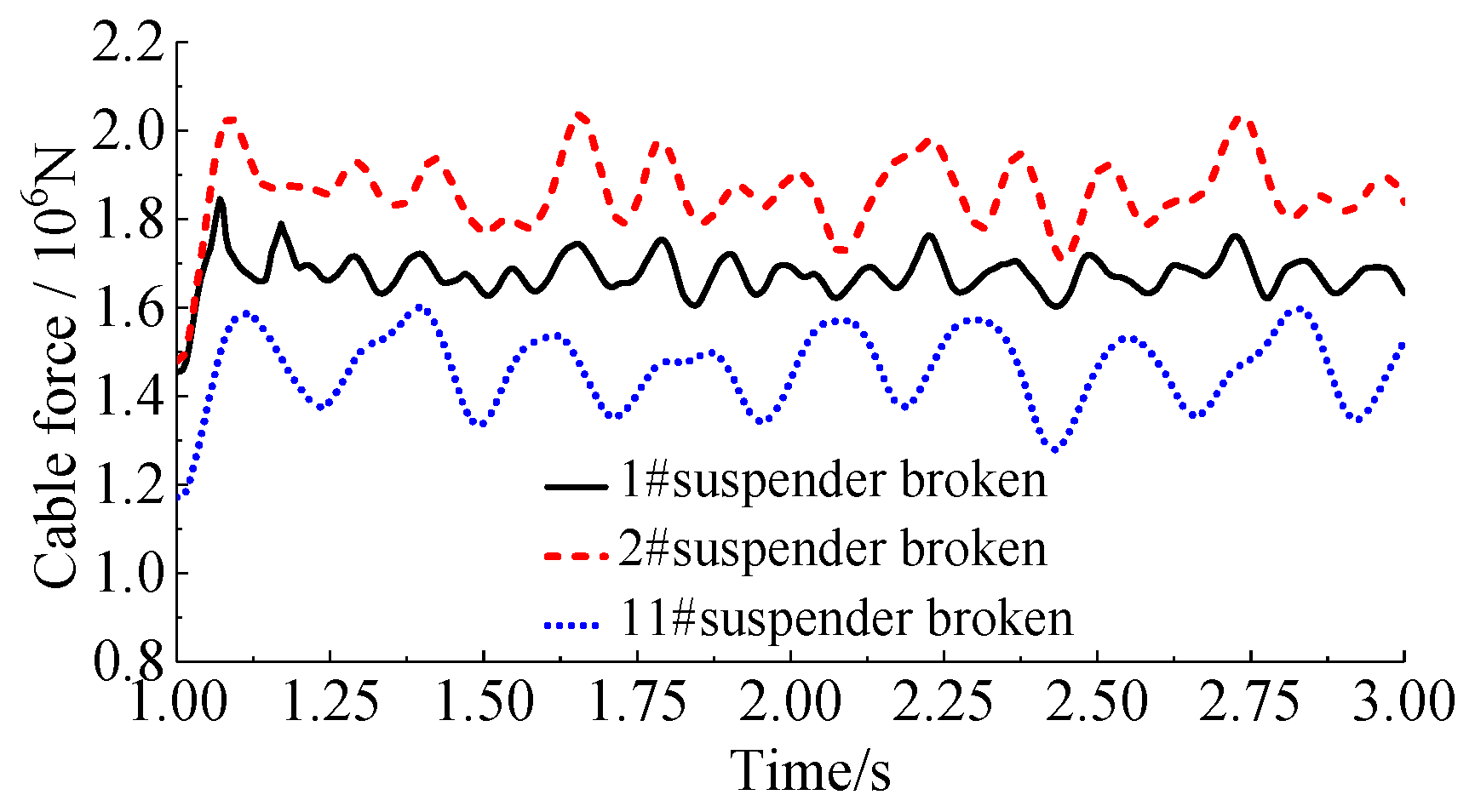
4.3. Suspender Force Dynamic Response
4.4. Accuracy Verification of Finite Element Model
5. Equivalent Static Method Considering the Suspender Fracture Process
5.1. Development of an Equivalent Static Method Accounting for the Suspender Breakage
5.2. Construction of the Half-Through and Through CFST Standard Arch Bridges
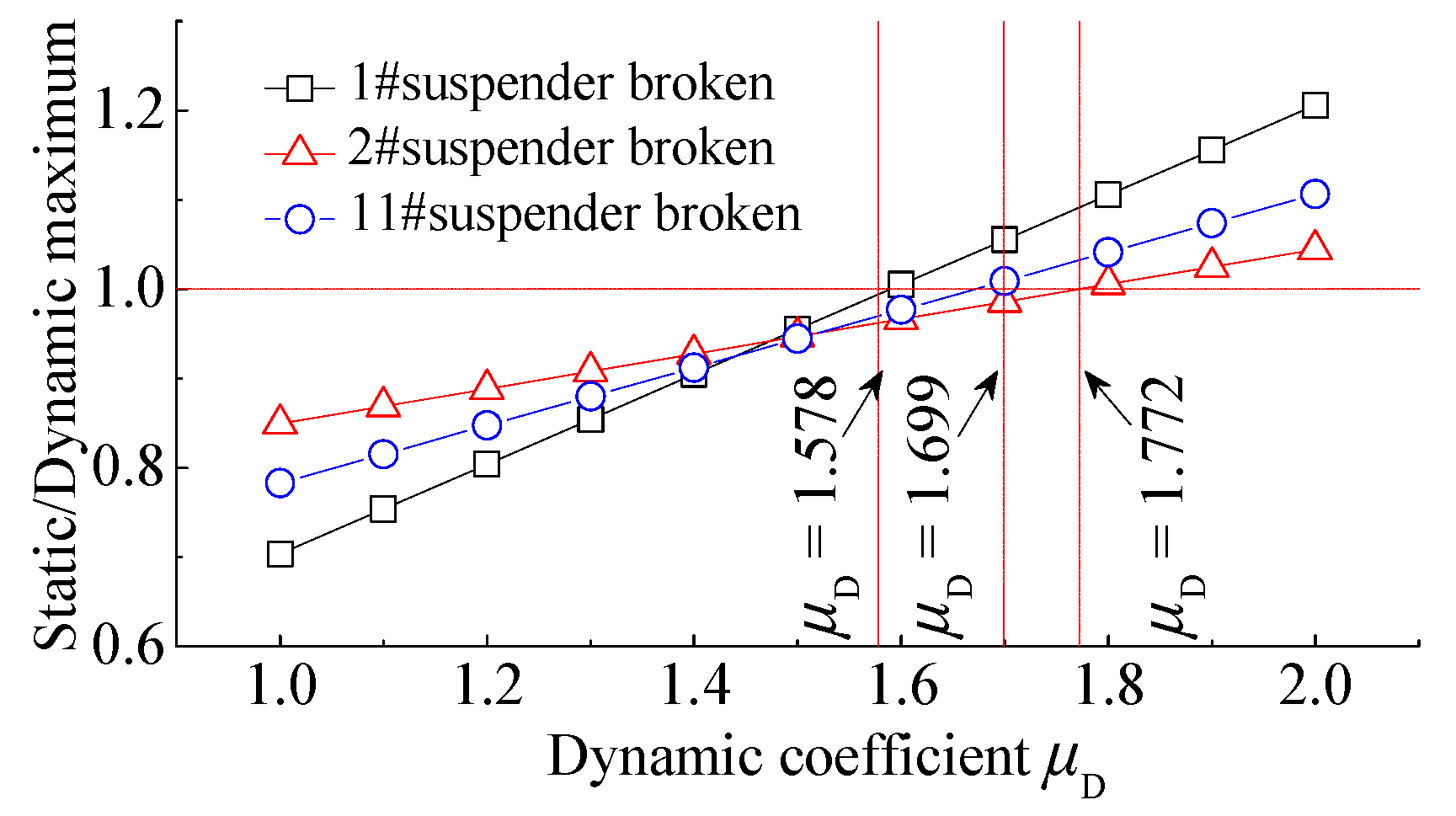
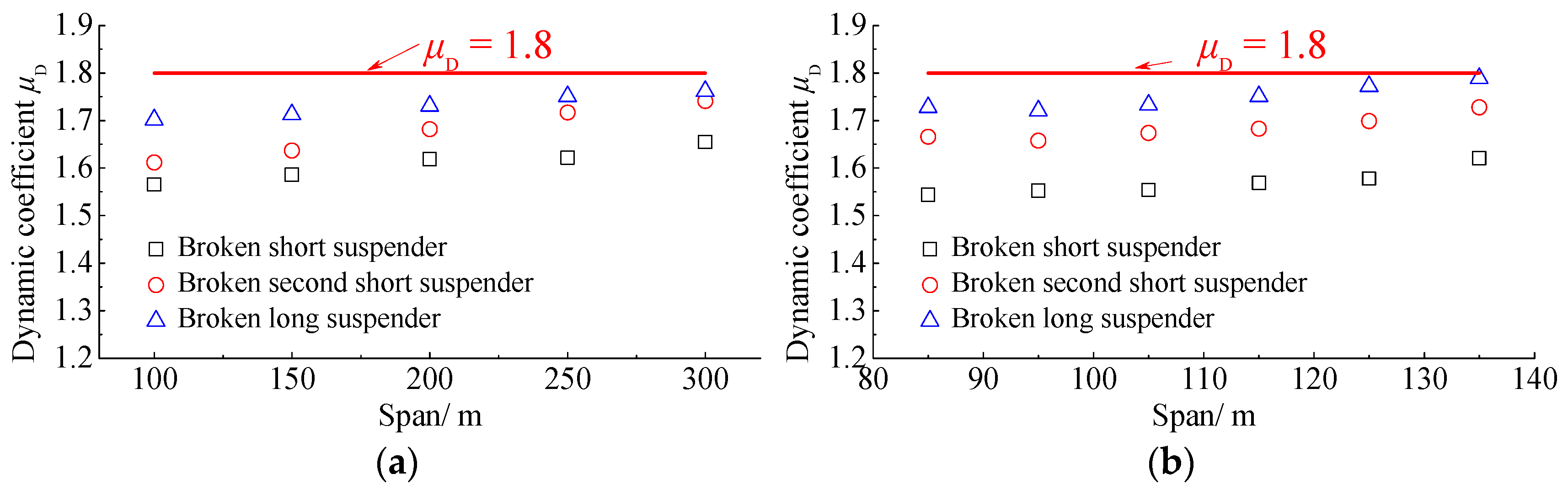
5.3. The Value Analysis of the Dynamic Coefficient
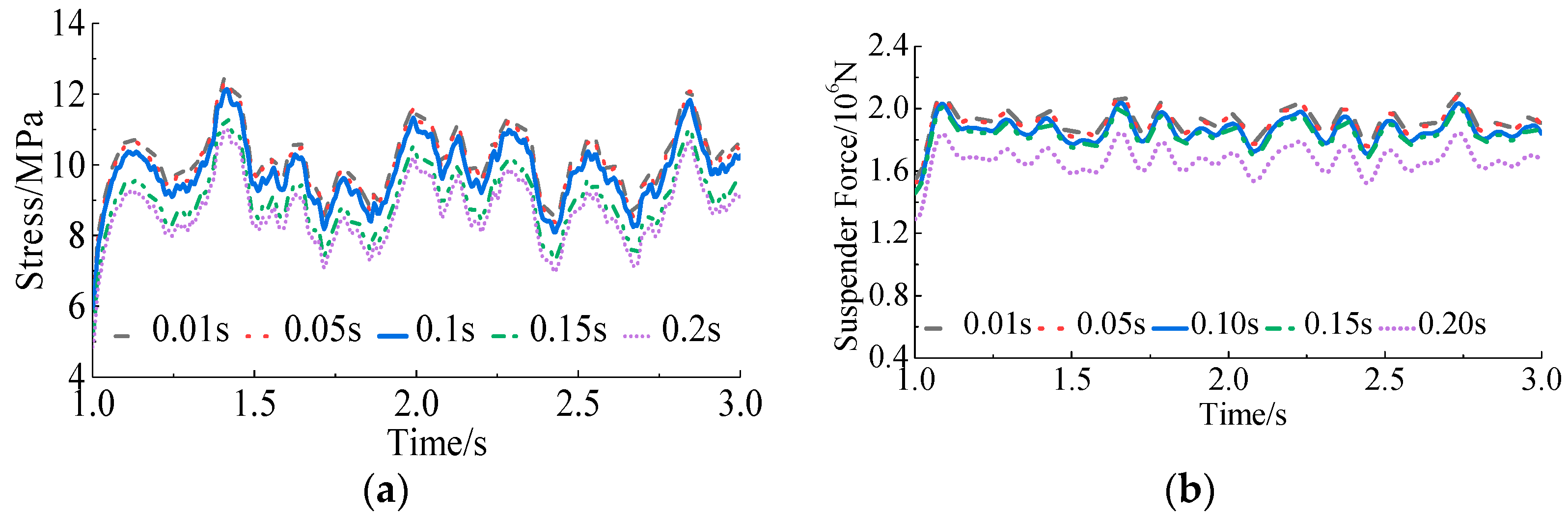
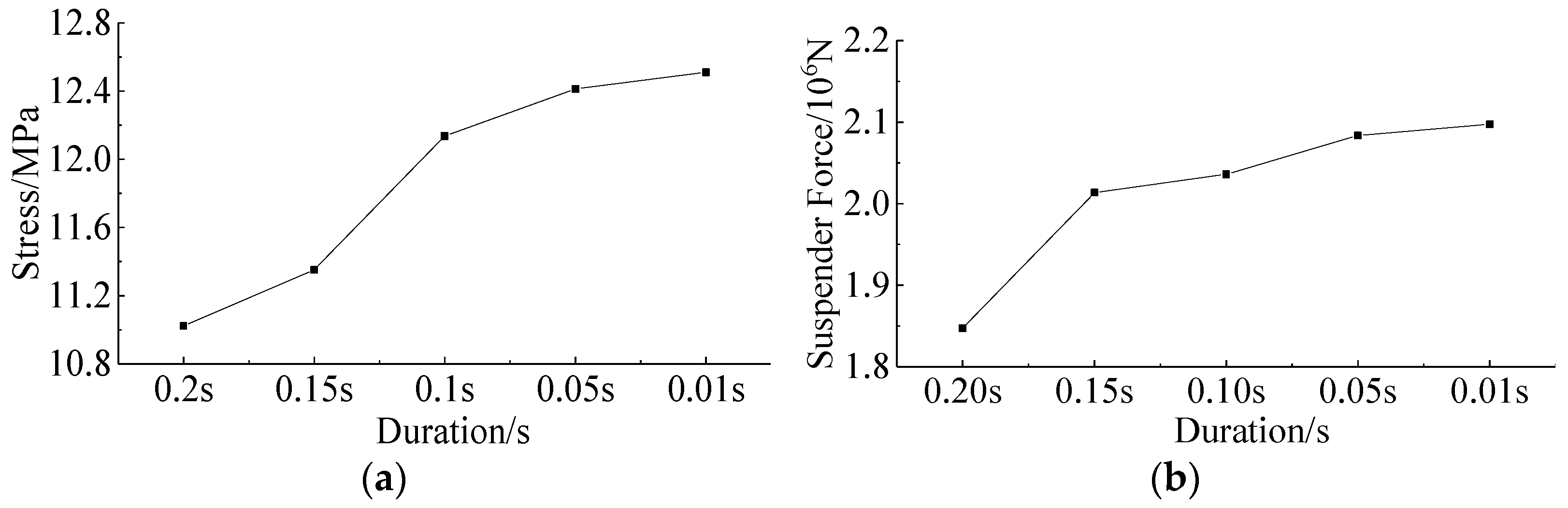
5.3.1. The Influence of Suspender Breaking Duration on Dynamic Coefficient
5.3.2. The Equivalent Dynamic Response of the Longitudinal Beam
5.3.3. The Equivalent Dynamic Response of the Suspender
5.4. The Value Determination of the Dynamic Coefficient
6. Conclusions
- (1)
- A suspender-breaking trigger device composed of a wedge-shaped steel block and electromagnet clamp is proposed. The trigger device can break the suspender within 0.1 s and accurately simulate the suspender fracture process of the real bridge. Additionally, when the suspender break duration is less than or equal to 0.15 s, the influence of the suspender break duration on the value of the dynamic coefficient can be ignored.
- (2)
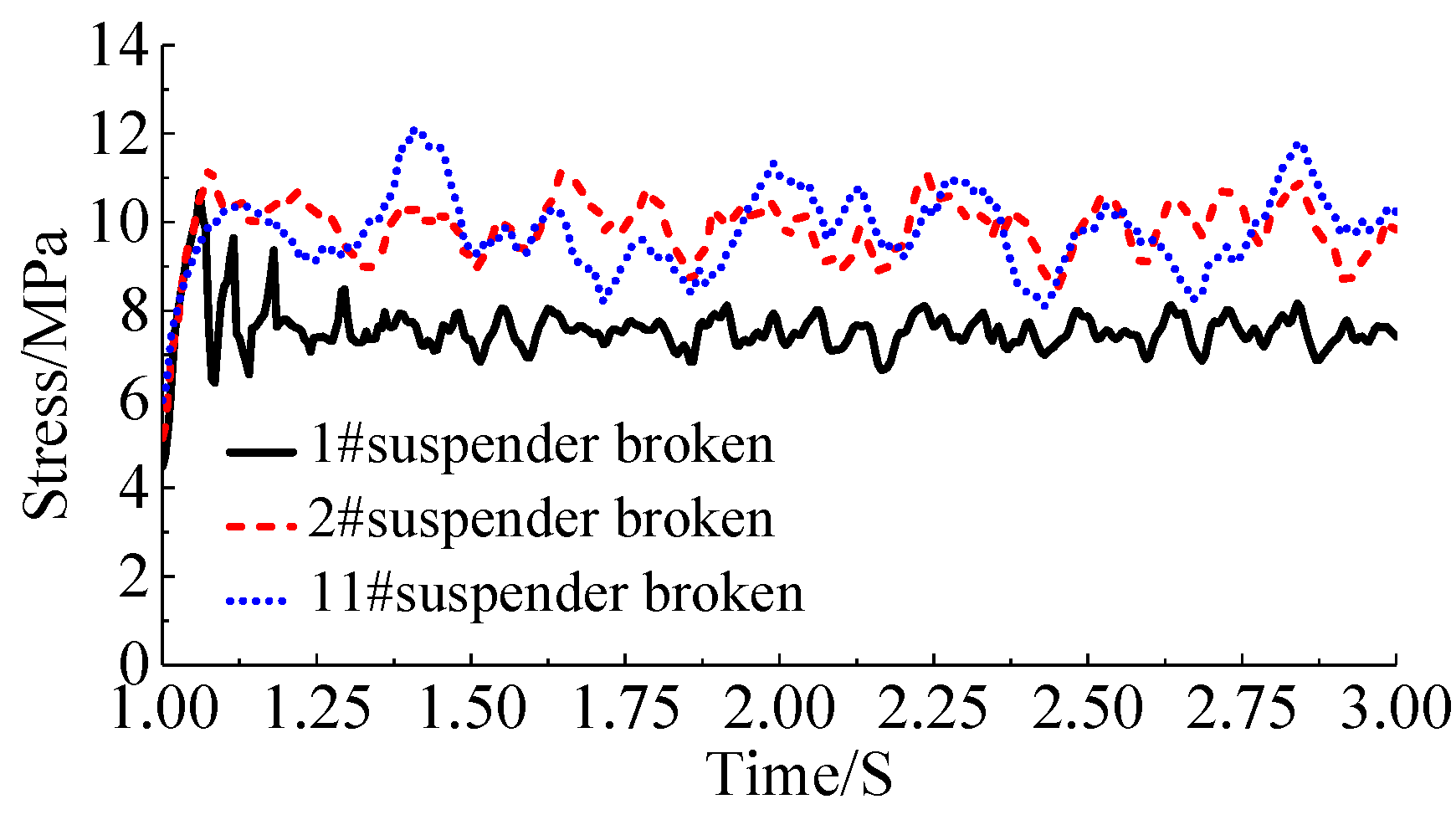
- The displacement and stress maximum variations of the deck system (arch rib) are 7.02 mm (3.05 mm) and 4.14 MPa (−35.41 MPa) when the 1# suspender is broken, respectively. The displacement and stress maximum variation of the deck system (arch rib) are 15.61 mm (4.35 mm) and 11.97 MPa (−50.16 MPa) when the 6# suspender is broken, respectively. This reveals that the influence of the suspender fracture on the displacement and stress of the longitudinal beam is much greater than that on the arch rib. The fracture of the long suspender has a great influence on the displacement and stress of the longitudinal beam and arch rib.
- (3)
- The error between the finite element analysis and the test results is within 11%. The proposed FE modeling method can accurately simulate the suspender fracture process and can be extended to the dynamic response analysis of suspension bridge deck systems of both half-through and through CFST arch bridges accounting for the suspender fracture process.
- (4)
- The dynamic coefficient is introduced to consider the dynamic effect of the suspender-breaking process. Thereafter, the equivalent static method for both half-through and through CFST-based arch bridges accounting for the dynamic effect of the suspender breaking is established. Through the comparative analysis of the static and dynamic results of eleven different span half-through and through CFST standard arch bridges with various suspenders breaking, it is observed that when the ESCM is employed, the dynamic coefficients of the longitudinal beams (suspenders) can be conservatively taken as 1.8 (1.8) and 1.8 (1.7), respectively.
- (5)
- In this paper, an equivalent static calculation method for half-through and through concrete-filled steel tubular arch bridges considering the process of suspender fracture is established, and the conservative value of the dynamic coefficient is determined. Subsequently, the influence of important parameters such as the stiffness of the longitudinal girder and arch rib on the dynamic coefficient will be studied.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Haag, J.; Reguly, A.; Strohaecker, T. Effect of tensile overloads on fatigue crack growth of high strength steel wires. Mater. Des. 2013, 52, 190–195. [Google Scholar] [CrossRef]
- Liu, J.F.; Li, Y.B.; Zhang, Q.W. Mechanical behavior of damaged strand suspender with asymmetric broken wires in arch bridges. J. Tongji Univ. (Nat. Sci.) 2019, 47, 451–457. [Google Scholar]
- Chen, B.C.; Fan, B.H.; Yu, Y.G.; Wu, Q.X.; Huang, J.Z. Robustness design of concrete-filled steel tube arch bridges. Bridge Constr. 2016, 46, 88–93. [Google Scholar]
- Huang, F.H. Vulnerability Analysis of Through Concrete-Filled Steel Tube Arch Bridge; Hefei University of Technology: Hefei, China, 2021. [Google Scholar]
- Fan, B.H.; Chen, B.H.; Wu, Q.X. Technical condition evaluation of half-through and through arch bridges considering robustness. Bridge Constr. 2018, 48, 64–68. [Google Scholar]
- GB 50923-2013; Technical Code for Concrete-Filled Steel Tube Arch Bridges. China Communications Press: Beijing, China, 2013.
- Starossek, U. Avoiding Disproportionate Collapse of Major Bridges. Struct. Eng. Int. 2009, 19, 289–297. [Google Scholar] [CrossRef] [Green Version]
- Zoli, T.P.; Steinhouse, J. Some Considerations in the Design of Long Span Bridges against Progressive Collapse; HNTB Corp.: New York, NY, USA, 2010; Volume 1, pp. 6–14. [Google Scholar]
- Zoli, T.P.; Woodward, R. Design of long span bridges for cable loss. IABSE Symp. Lisbon 2005, 90, 17–25. [Google Scholar]
- Li, Y.; He, S.H. Research on robustness performance of shallow type arch bridge based on suspender damage. J. Hefei Univ. Technol. 2014, 37, 1254–1258. [Google Scholar]
- Jiang, R.J.; Francis, T.K.A.; Wu, Q.M. Improved hanger design for robustness of through-arch bridges. Proc. Inst. Civ. Eng.-Bridge Eng. 2013, 166, 193–204. [Google Scholar] [CrossRef] [Green Version]
- Ruiz, T.A.M.; Aparicio, A.C. Response of under-deck cable-stayed bridges to the accidental breakage of stay cables. Eng. Struct. 2009, 31, 1425–1434. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.M.; Hu, X.L.; Gu, C.K.; Ruan, X. Research on structural robustness for single cable loss in combination. Struct. Eng. 2012, 28, 50–54. [Google Scholar]
- Jin, X.L.; Yang, Y.; Qian, K. Research on Force Performance of Cable Breaking on Cable-stayed Bridges Applied Mechanics and Materials. Appl. Mech. Mater. 2014, 644, 5061–5064. [Google Scholar] [CrossRef]
- Wu, Q.X.; Yu, Y.G.; Chen, B.C. Dynamic analysis for cable loss of a rigid-frame tied through concrete-filled steel tubular arch bridge. J. Vib. Shock. 2014, 33, 8–13. [Google Scholar]
- Shao, G.; Jin, H.; Jiang, R.; Xu, Y. Dynamic Response and Robustness Evaluation of Cable-Supported Arch Bridges Subjected to Cable Breaking. Shock Vib. 2021, 2021, 6689630. [Google Scholar] [CrossRef]
- Yu, Y.G. Study on Robustness of Suspended Floor System in Half-Through and Through Arch Bridges; Fuzhou University: Fuzhou, China, 2015. [Google Scholar]
- Minaei, A.; Daneshjoo, F.; Goicolea, J.M. Experimental and numerical study on cable breakage equivalent force in cable-stayed structures consisting of low-relaxation seven-wire steel strands. Structures 2020, 27, 595–606. [Google Scholar] [CrossRef]
- Qiu, W.; Jiang, M.; Zhang, Z. Responses of self-anchored suspension bridge to sudden breakage of hangers. Struct. Eng. Mech. 2014, 50, 241–255. [Google Scholar] [CrossRef]
- Loto, C.A. Stress corrosion cracking: Characteristics, mechanisms and experimental study. Int. J. Adv. Manuf. Technol. 2017, 93, 3567–3582. [Google Scholar] [CrossRef]
- Samiei, K.; Peters, B.; Bolten, M.; Frommer, A. Assessment of the potentials of implicit integration method in discrete element modelling of granular matter. Comput. Chem. Eng. 2012, 49, 183–193. [Google Scholar] [CrossRef]
- Clough, R.W.; Penzien, J. Dynamics of Structures. In Computers and Structures; McGraw-Hill College: New York, NY, USA, 2003. [Google Scholar]
- JTG/T D65-06-2015; Specifications for Design of Highway Concrete-Filled Steel Tubular Arch Bridge. China Communications Press: Beijing, China, 2015.





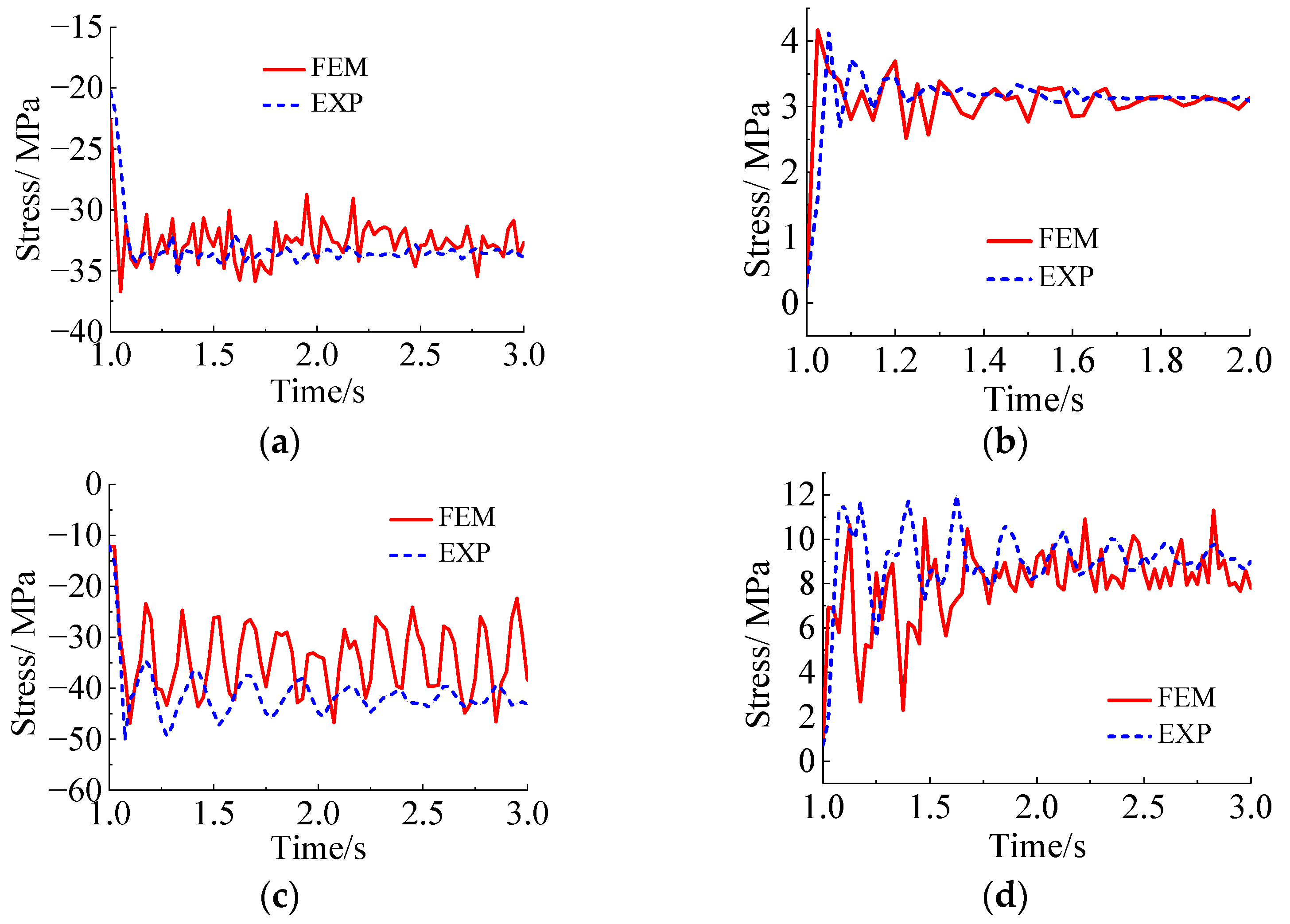
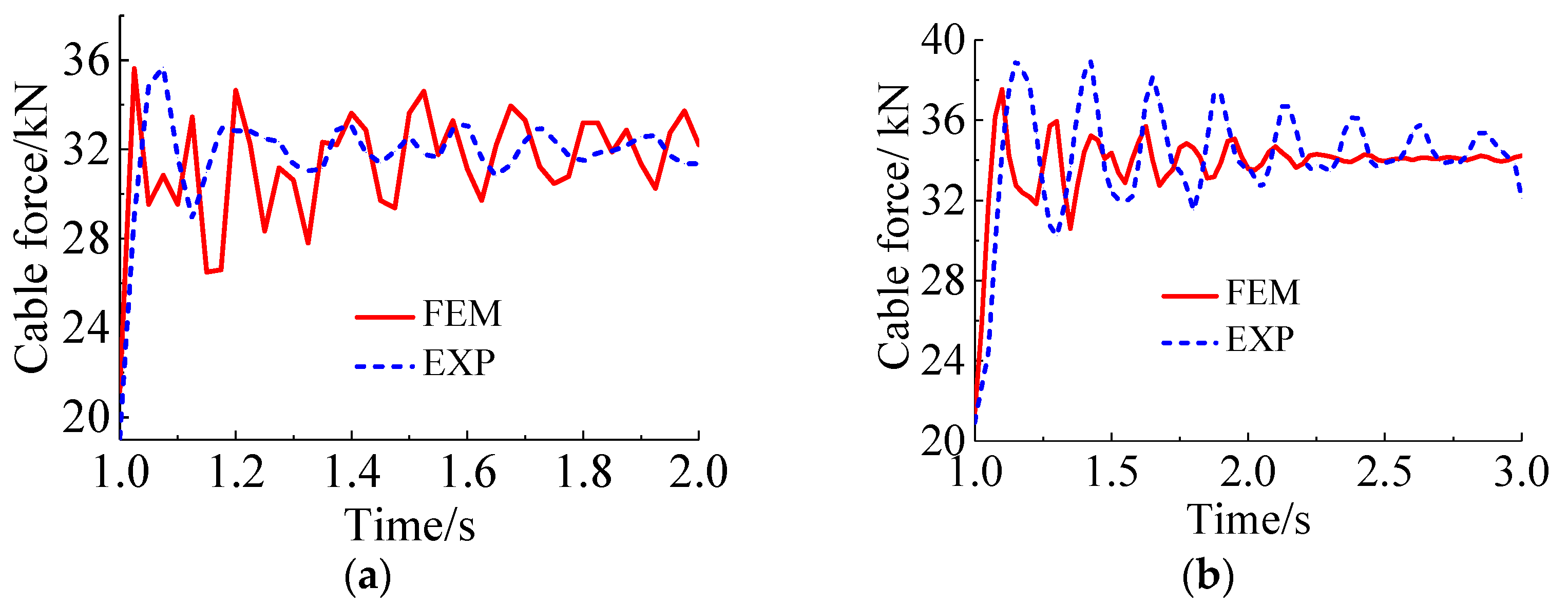



| Result | Arch Rib | Bridge Deck System | Suspender | ||
|---|---|---|---|---|---|
| Displacement/mm | Stress/MPa | Displacement/mm | Stress/MPa | Suspender Force/kN | |
| Calculated value | 3.10 | −36.73 | 6.49 | 4.17 | 35.62 |
| Measured value | 3.05 | −35.41 | 7.02 | 4.14 | 35.68 |
| Calculated/Measured | 1.02 | 1.04 | 0.92 | 1.01 | 0.99 |
| Result | Arch Rib | Bridge Deck System | Suspender | ||
|---|---|---|---|---|---|
| Displacement/mm | Displacement/mm | Stress/MPa | Displacement/mm | Displacement/mm | |
| Calculated value | 4.84 | −46.83 | 15.38 | 11.29 | 37.54 |
| Measured value | 4.35 | −50.16 | 15.61 | 11.97 | 38.97 |
| Calculated/Measured | 1.11 | 0.93 | 0.98 | 0.94 | 0.96 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, K.; Luo, J.; Wu, Q.; Wang, H. Test of Broken Suspender Specimen and Equivalent Static Calculation Method for Half-Through and Through Concrete-Filled Steel Tubular Arch Bridges. Appl. Sci. 2022, 12, 11619. https://doi.org/10.3390/app122211619
Chen K, Luo J, Wu Q, Wang H. Test of Broken Suspender Specimen and Equivalent Static Calculation Method for Half-Through and Through Concrete-Filled Steel Tubular Arch Bridges. Applied Sciences. 2022; 12(22):11619. https://doi.org/10.3390/app122211619
Chicago/Turabian StyleChen, Kangming, Jianping Luo, Qingxiong Wu, and Huanwei Wang. 2022. "Test of Broken Suspender Specimen and Equivalent Static Calculation Method for Half-Through and Through Concrete-Filled Steel Tubular Arch Bridges" Applied Sciences 12, no. 22: 11619. https://doi.org/10.3390/app122211619
APA StyleChen, K., Luo, J., Wu, Q., & Wang, H. (2022). Test of Broken Suspender Specimen and Equivalent Static Calculation Method for Half-Through and Through Concrete-Filled Steel Tubular Arch Bridges. Applied Sciences, 12(22), 11619. https://doi.org/10.3390/app122211619





