Analysis of Rewetting Characteristics and Process Parameters in Tobacco Strip Redrying Stage
Abstract
1. Introduction
2. Model Construction
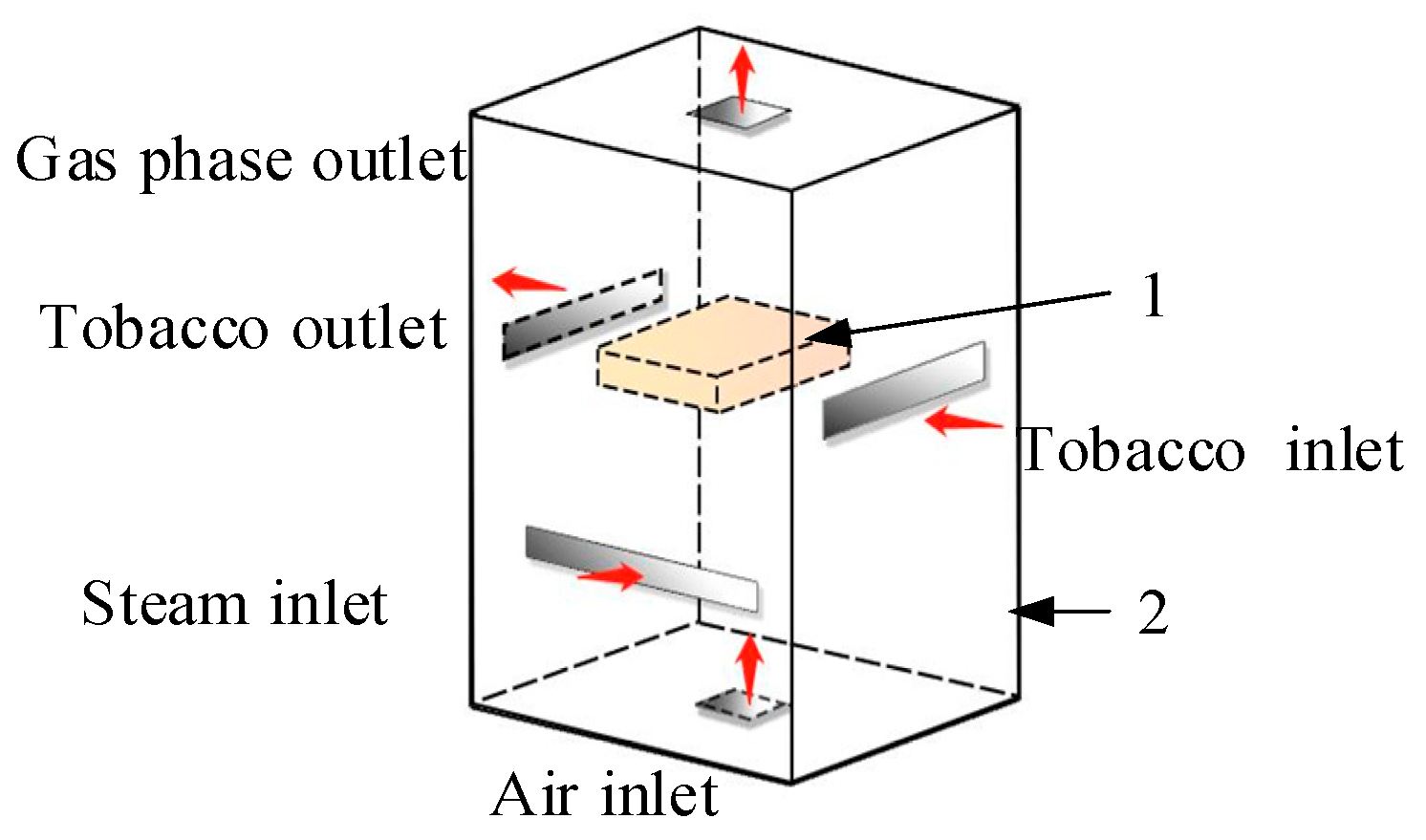
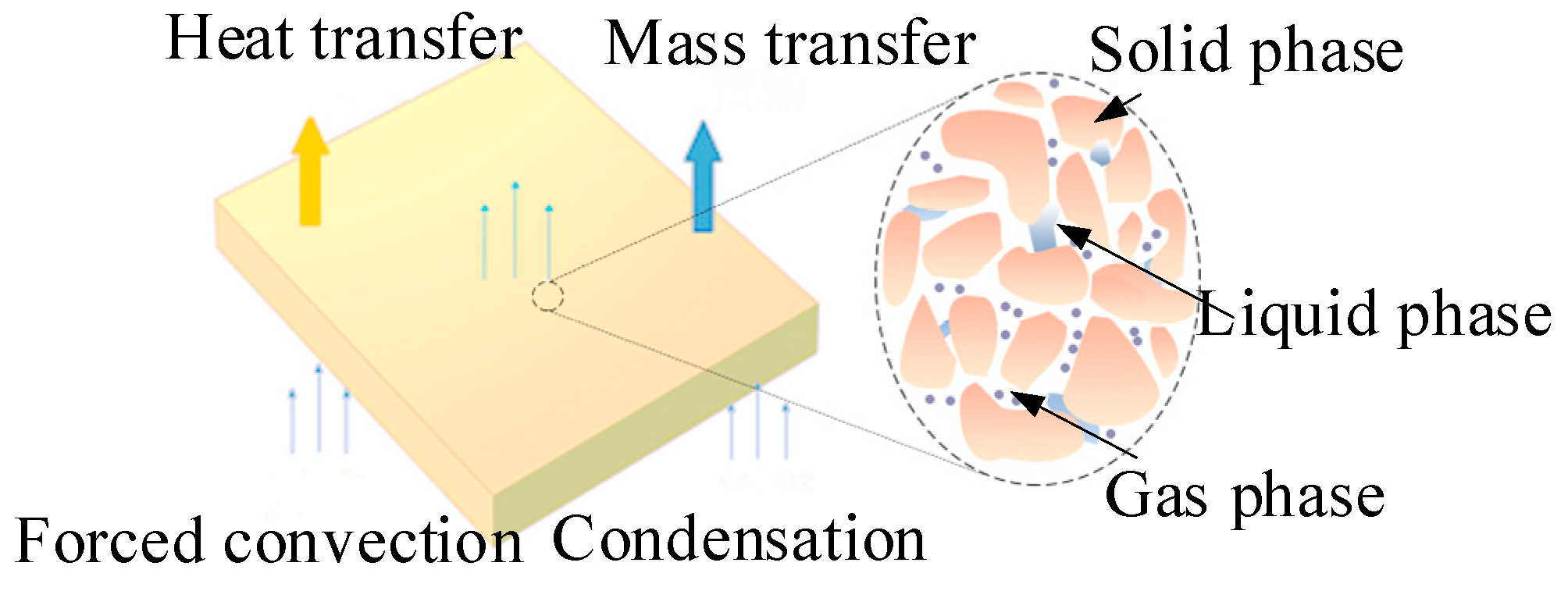
2.1. Physical Model
2.2. Mathematical Models
2.2.1. Mass Equation
2.2.2. Energy Equation
2.3. Initial and Boundary Conditions
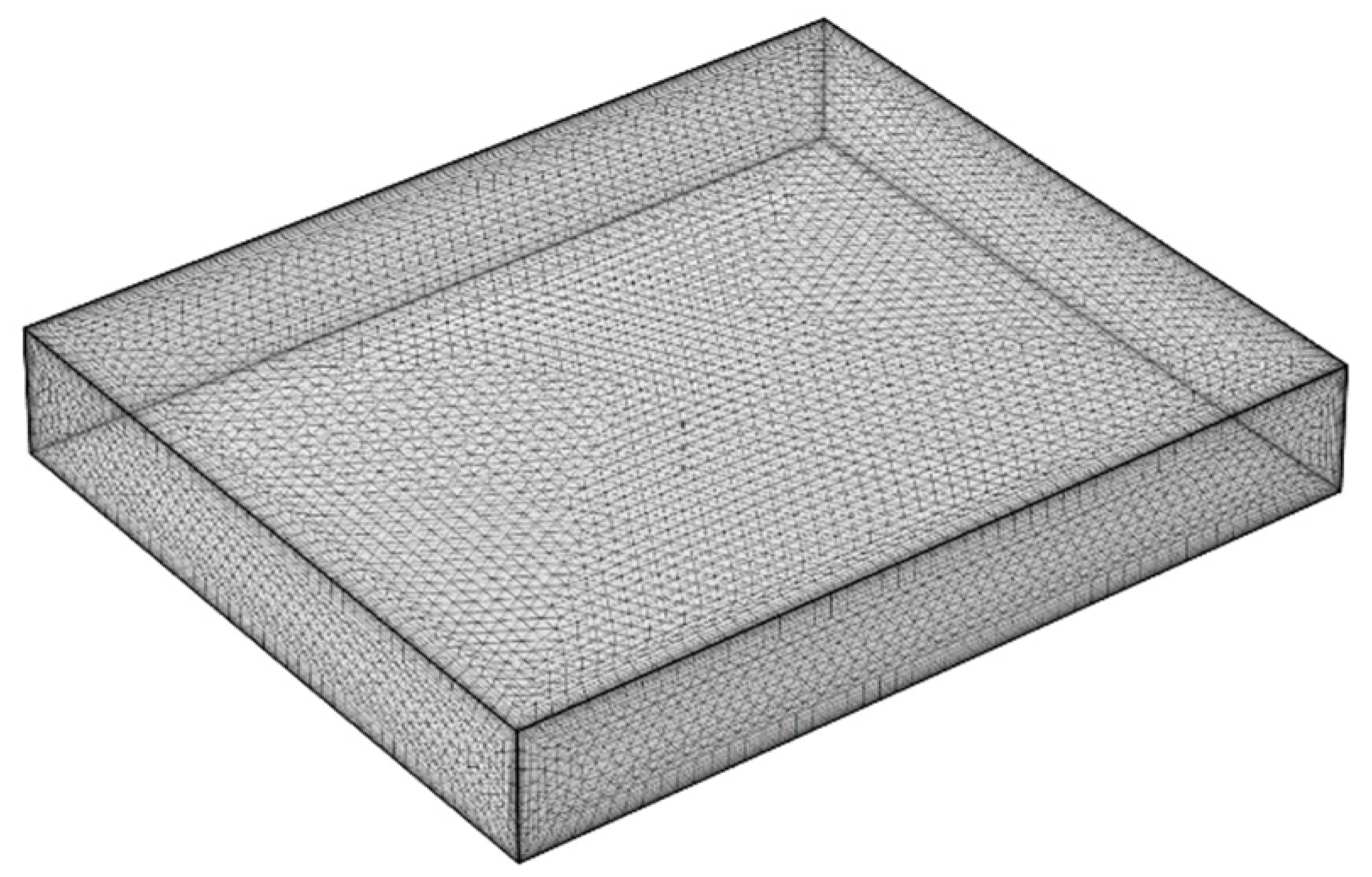
2.4. Model Solution
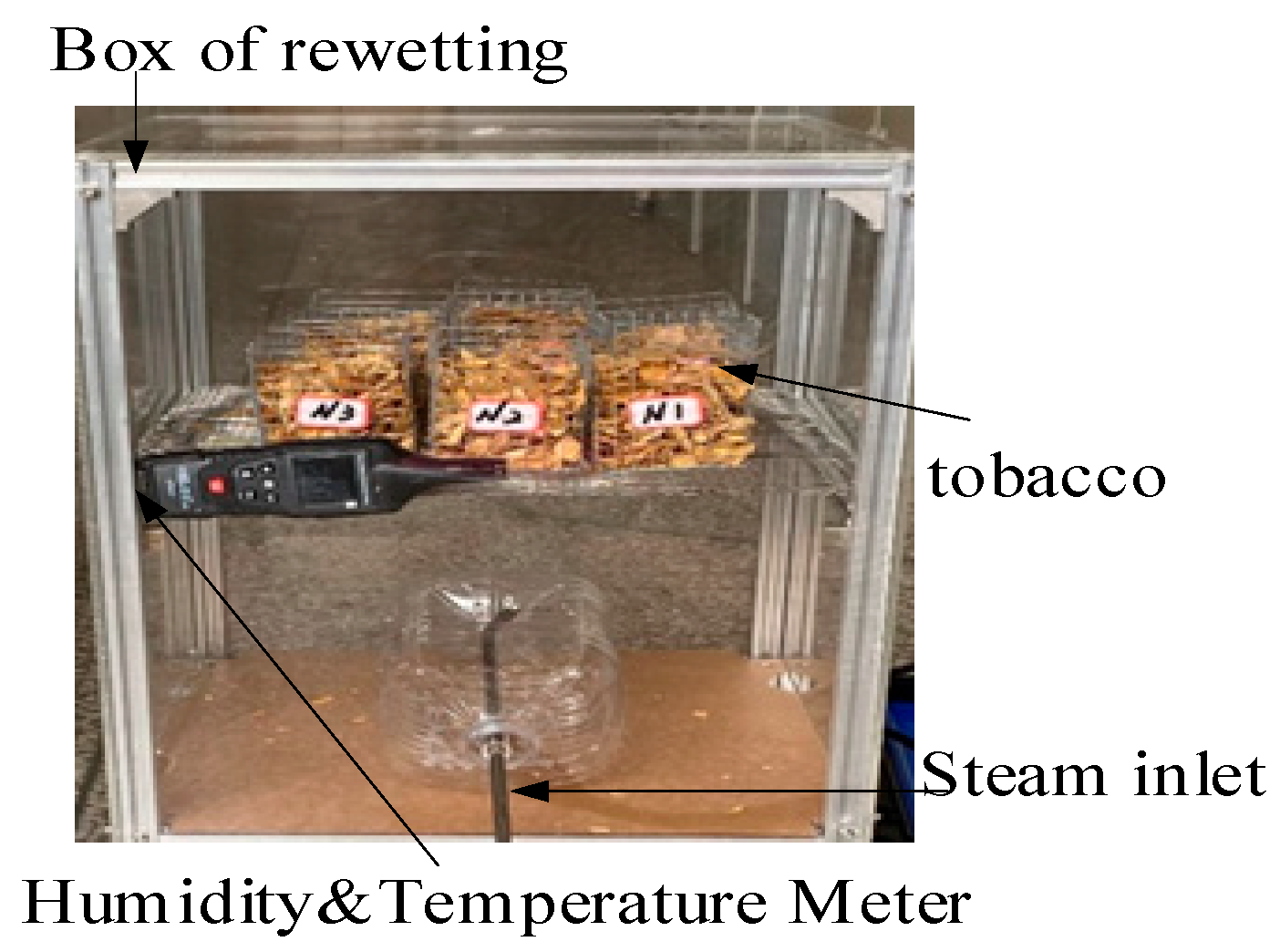
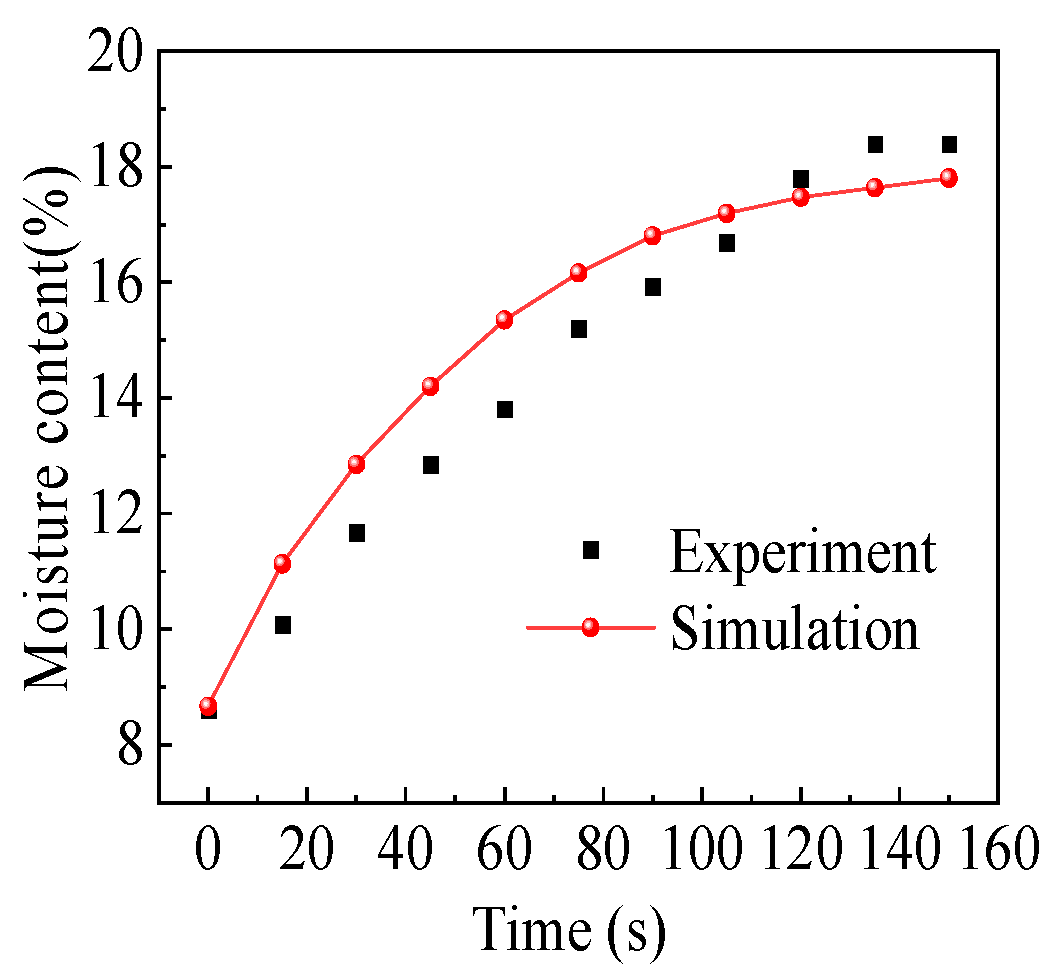
3. Experimental Verification of the Moisture Content
4. Results and Discussion
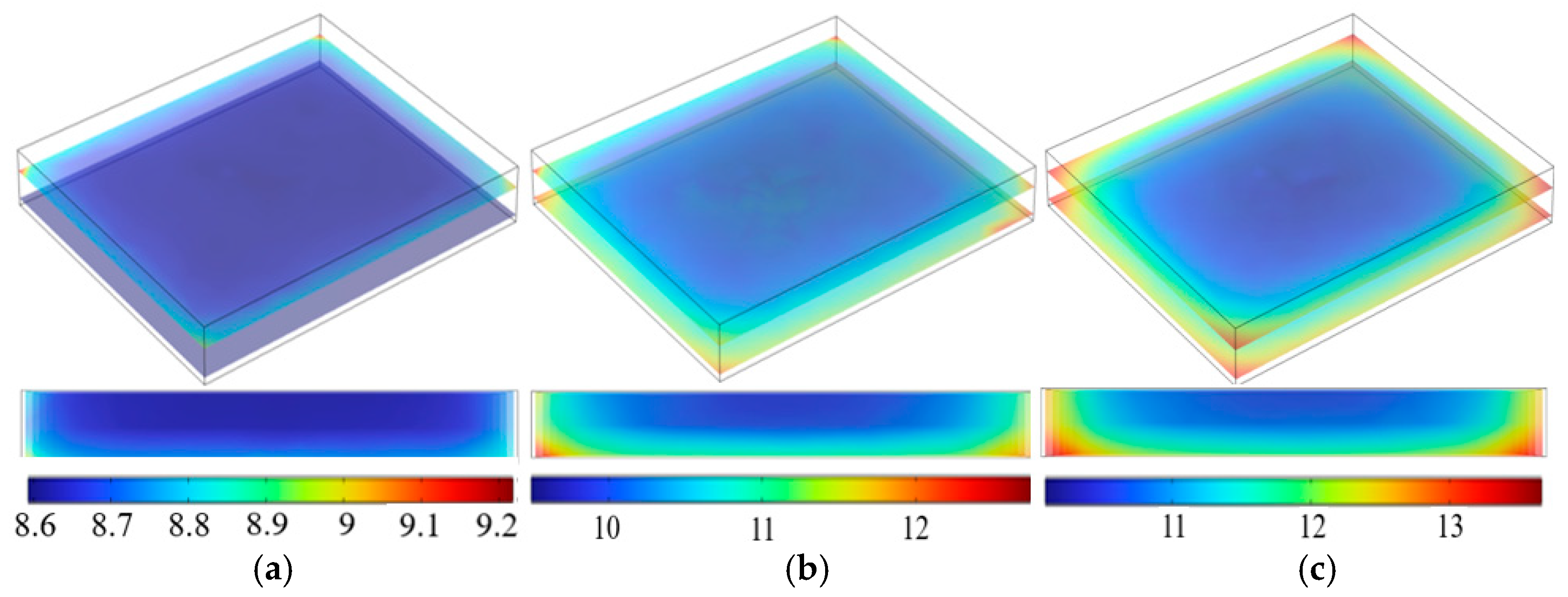
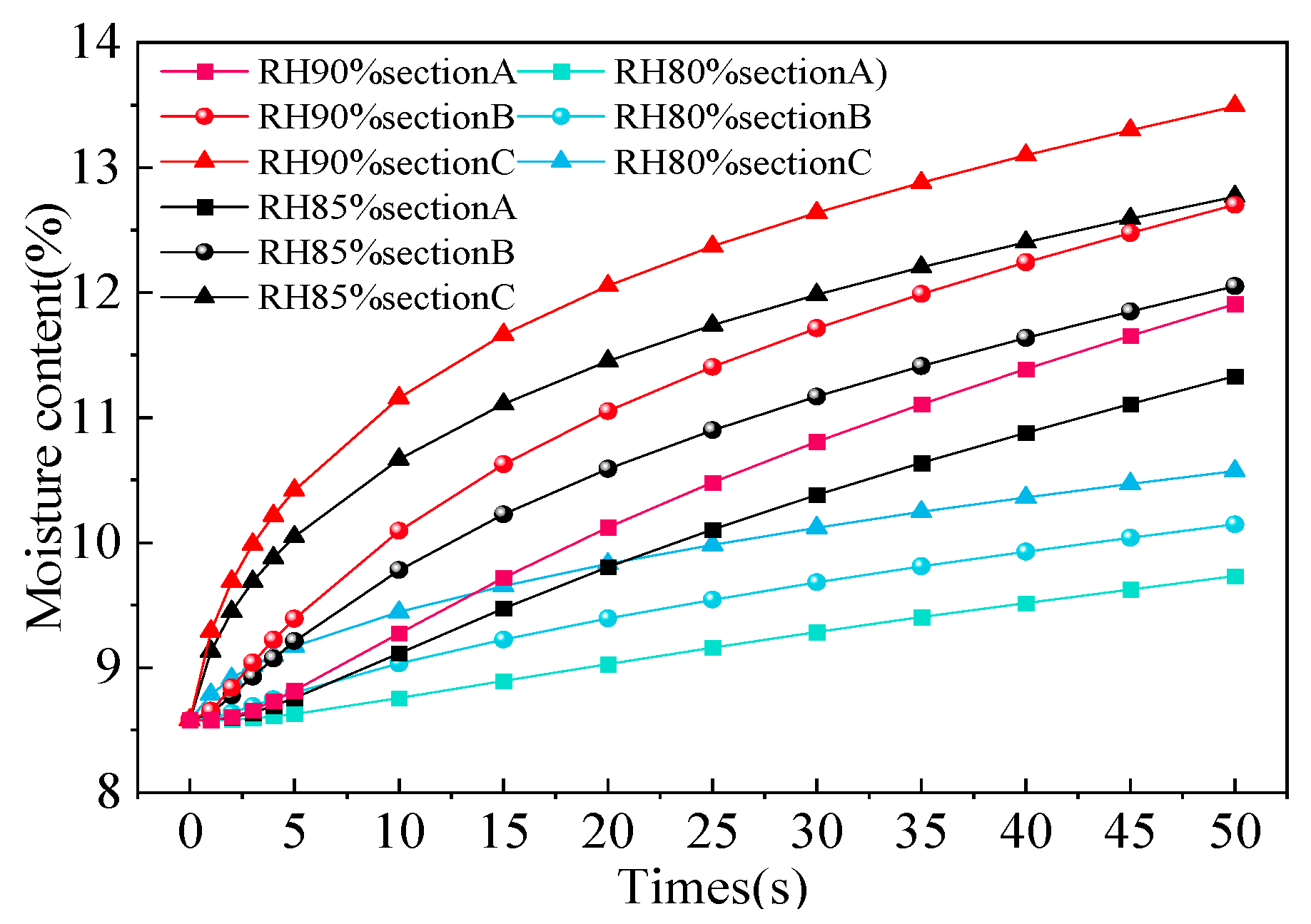
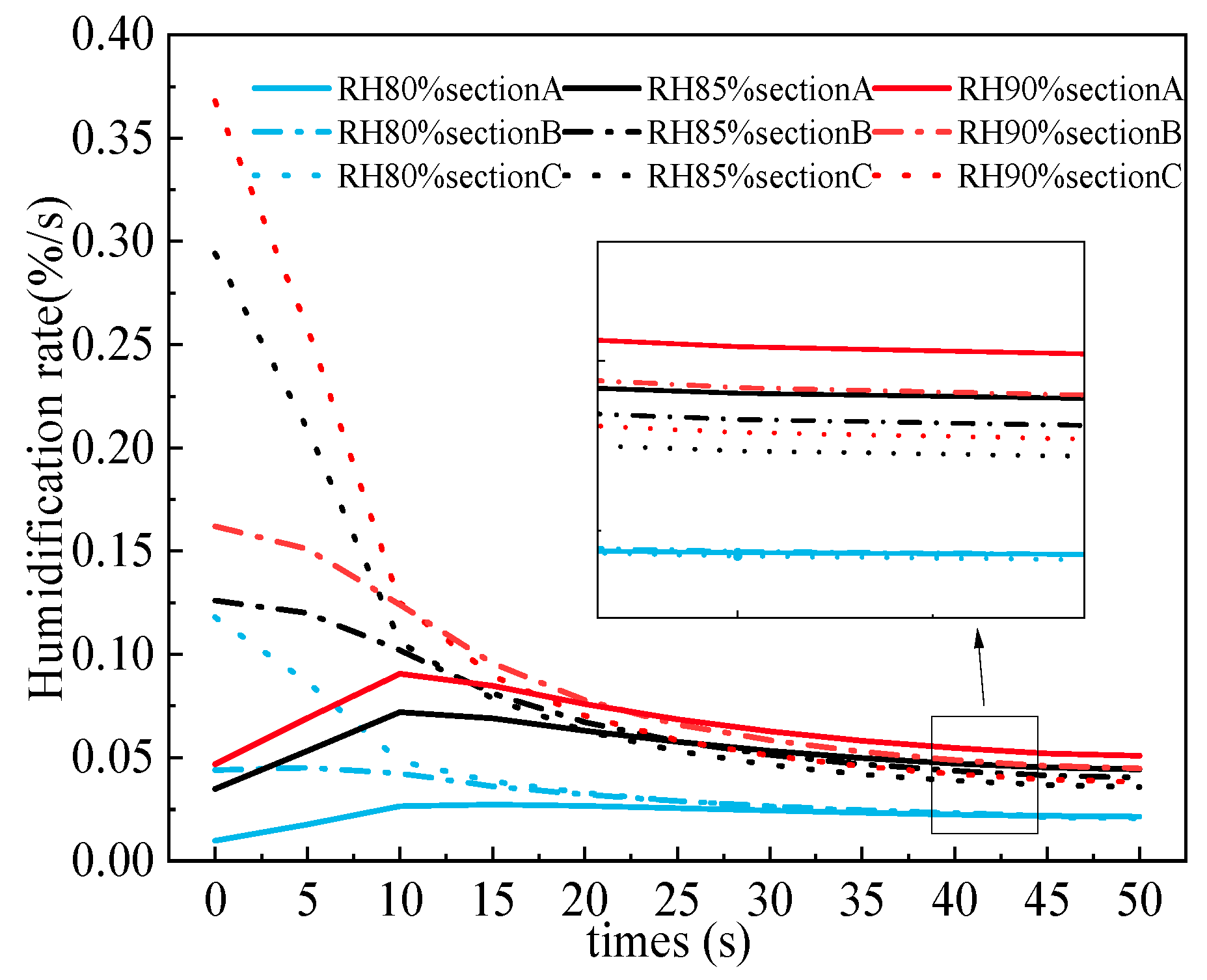
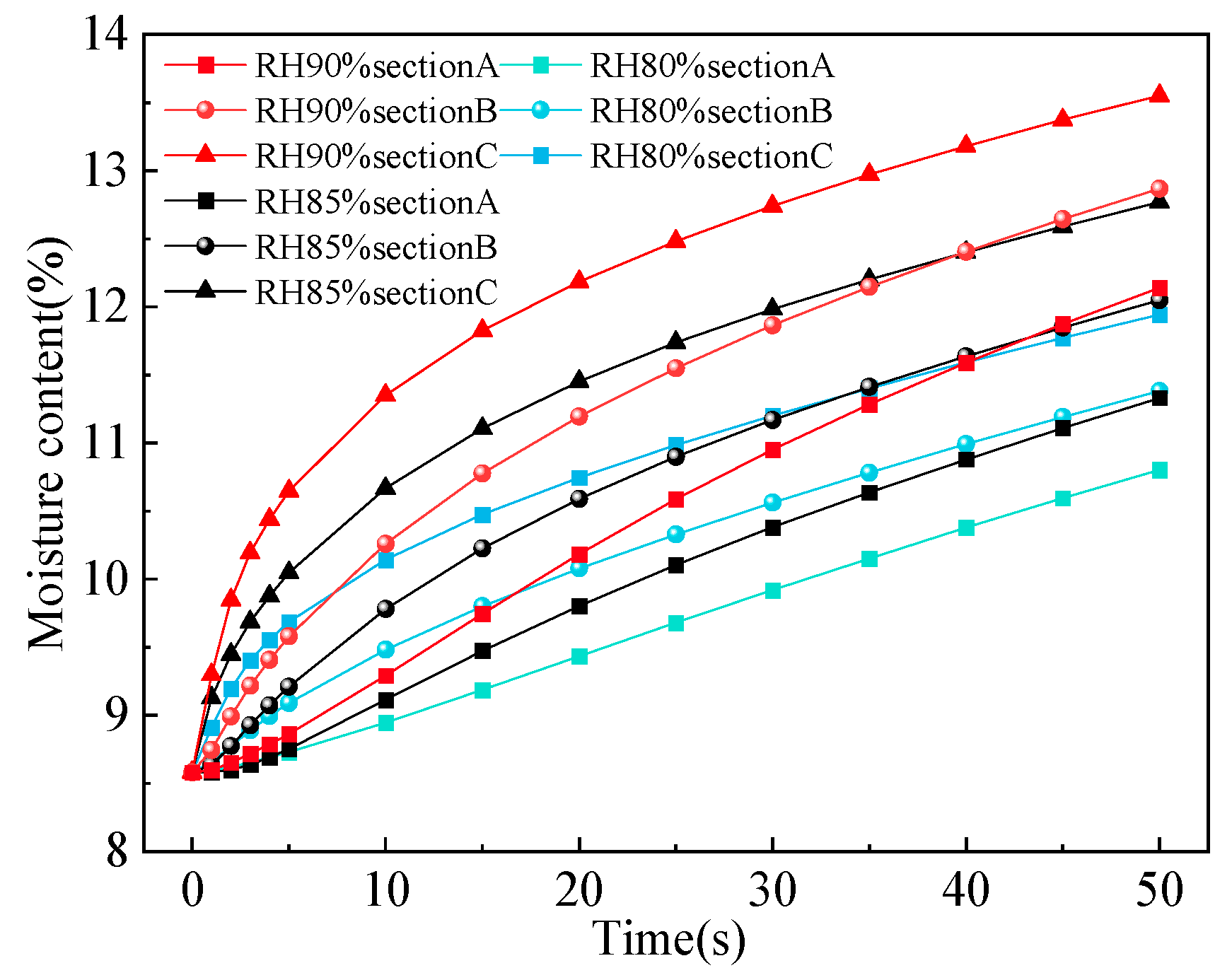
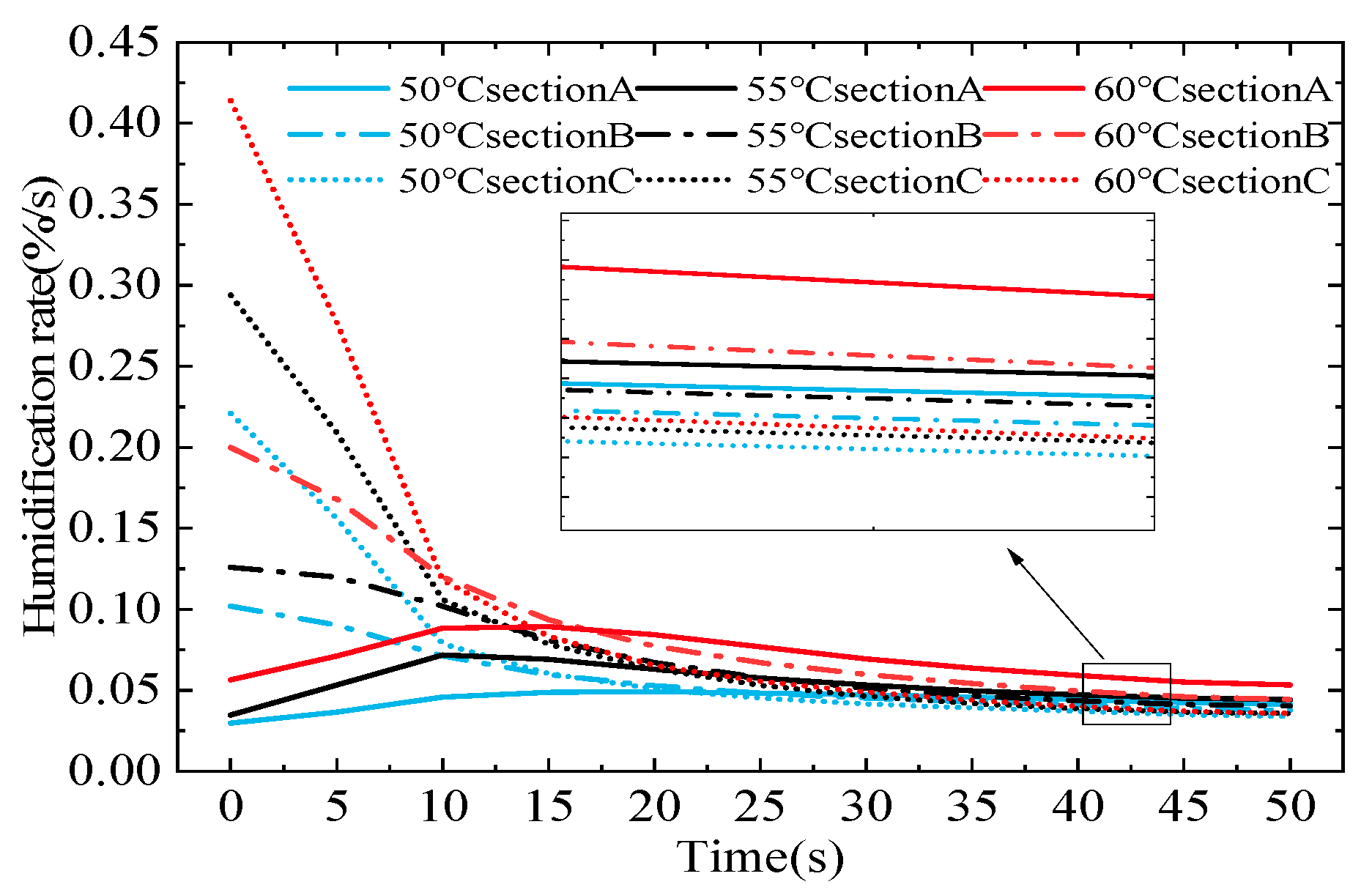
4.1. The Effect of the Relative Humidity on Moisture Content and Humidification Rate of Tobacco Strips
4.2. The Effect of Relative Humidity on Moisture Content and Humidification Rate of Tobacco Strips
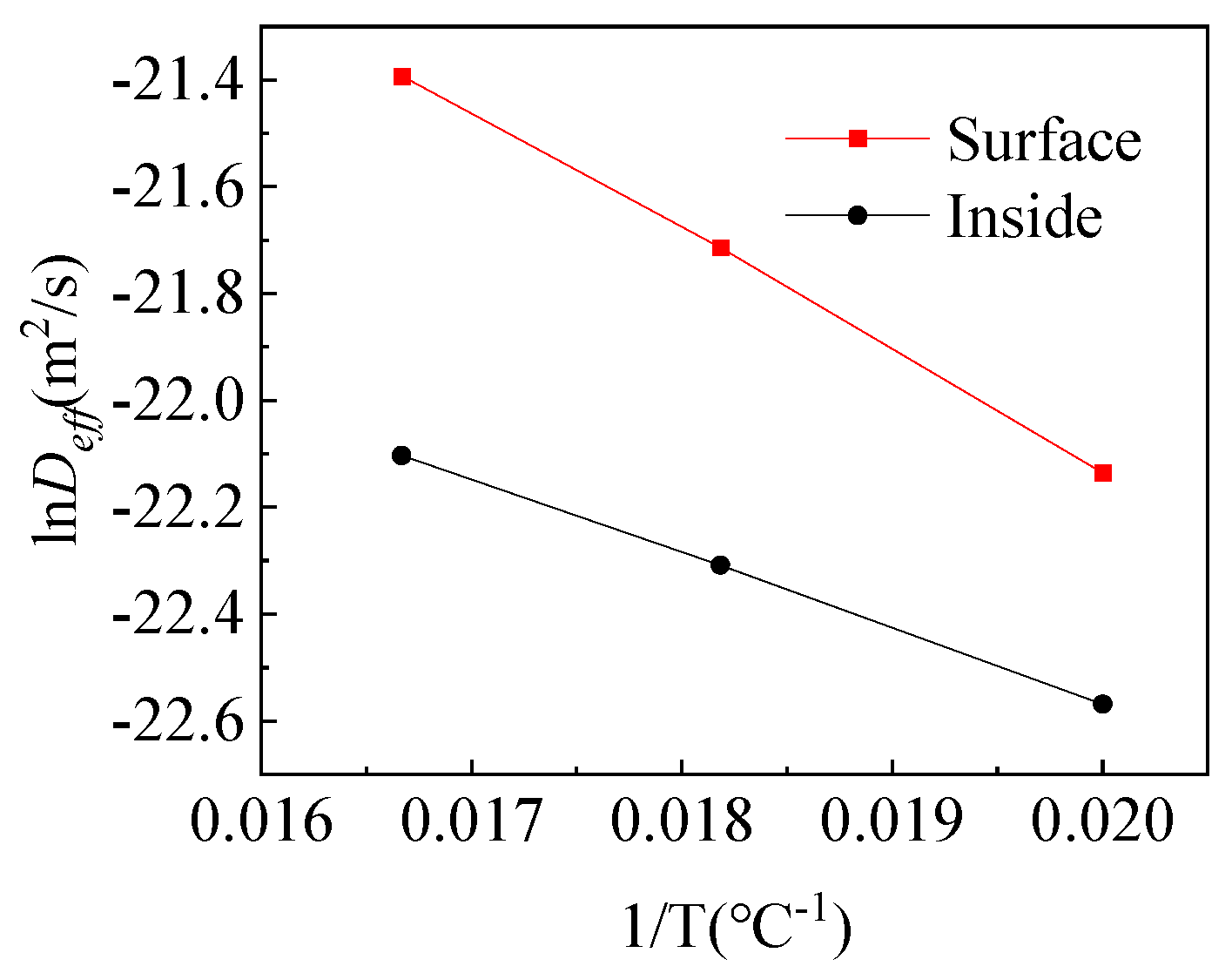
4.3. The Effective Moisture Diffusivity (Deff) and Activation Energy (Ea)
5. Conclusions
- The rewetting of heaped tobacco strips during the redrying stage includes two stages, the accelerating stage and approximate constant rate stage. The vapor mainly condensates in the accelerated stage, which is characterized by fast growth and a large increment of moisture content. The diffusivity of the liquid phase mainly occurs in the approximate constant rate stage, which is characterized by the slow growth of the moisture content and stable increments.
- The relative humidity and temperature have a great influence on the rewetting characteristics of heaped tobacco strips. The higher the relative humidity and temperature, the higher the moisture content of heaped tobacco strips, and the greater the increment and the faster the humidification rate. The best conditions for rewetting tobacco strips are a relative humidity of 85% and a temperature of 55 °C. This maintains the moisture content in the range from 11 to 13 percent.
- An effective moisture diffusivity is greatly influenced by the relative humidity and temperature, showing a changing law of rapid increase and then stable increase. The higher the relative humidity and temperature, the greater the variation, and the faster the rate of increase. At the same time, the effective moisture diffusivity of the heaped tobacco strips’ surface is more sensitive than the interior.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shi, Y. Control and Application of BP Neural Network Based on Rewetting Zone in Tobacco Redrying Oven. Master’s Thesis, Yunnan University, Kunming, China, 2015. [Google Scholar]
- Hu, K. Technique and Equipment in Tobacco Threshing and Redrying, 1st ed.; Chemical Industry Press: Beijing, China, 2002; pp. 91–94. [Google Scholar]
- Zhao, C. Research on the Influence of Threshing and Redrying Technology on the Processing Quality of Tobacco Leaves. Master’s Thesis, Kunming University of Science and Technology, Kunming, China, 2020. [Google Scholar]
- Huang, J. Tobacco Industry Handbook, 1st ed.; Guangdong Economy Publishing House: Guangzhou, China, 1999; pp. 547–551. [Google Scholar]
- Chen, C.; Zhang, F.; Cao, D. Optimization of moisture regain process parameters in tobacco leaf redryer. Manuf. Autom. 2015, 37, 111–114. [Google Scholar]
- Hu, Y.; Xu, L.; Zhang, W.; Peng, L.; Zhang, W. Study on the hydroscopic characteristics of flie-cured tobacco from Yunnan. Tob. Sci. Technol. 2000, 9, 8–12. [Google Scholar]
- Zhang, Y. Reducing breakage rate of strips during redrying. Tob. Sci. Technol. 2009, 4, 21–24. [Google Scholar]
- Jiang, W.; Wang, L.; Tang, J.; Yin, Y.; Zhang, H.; Jia, T.; Qin, J.; Wang, H.; Wei, Q. Calibration and Experimental Validation of Contact Parameters in a Discrete Element Model for Tobacco Strips. Processes 2022, 10, 998. [Google Scholar] [CrossRef]
- Dai, Y.; Shen, Y.; Xia, F.; Peng, L. Design of moistening system in reordering section in strip redryer. Tob. Sci. Technol. 2019, 52, 115–120. [Google Scholar]
- Liu, Y.; Zhang, Y.; Liu, Y.; Pang, X.; Huang, K.; Dai, X.; Pang, T. Analysis of influencing factors on threshing and redrying strips yield. J. Anhui Agric. Sci. 2021, 49, 185–188. [Google Scholar]
- Fu, L.; Ren, Z. Application of novel dual control strategy in tobacco re-drying process. Control. Eng. China 2014, 21, 100–103, 106. [Google Scholar]
- Wang, W.; Feng, X.; Fan, Z.; Ma, Y.; Chen, H.; Ren, Z. Design and application of control strategy for moisture content in redried tobacco strips packed in cases. Tob. Sci. Technol. 2020, 53, 103–107, 112. [Google Scholar]
- Ji, J.; Zhu, Y.; Wang, J.; Zhang, H.; He, Z. Experimental study on optimization design of tobacco moisture regain machine based on thermodynamics. J. Agric. Mech. Res. 2019, 41, 34–39. [Google Scholar]
- Zhao, Y.; Zhang, G.; Cao, W.; You, X.; Factory, T.C. Optimization design of exhaust hood in tunnel type tobacco conditioner. Tob. Sci. Technol. 2016, 49, 87–92. [Google Scholar]
- Tang, J.; Tang, L.; Wen, L.; He, B.; Lin, W.; Zeng, Z.; Ma, N.; Zhou, B. Construction and prediction of Bayesian network model of relationship between process parameters and discharge quality in loosening and conditioning. Food Mach. 2020, 36, 207–210. [Google Scholar]
- Xu, M.; Gong, J.; Jin, W.; Sun, K.; Zhu, J.; Wu, X.; Zhang, M.; Zheng, C.; Lin, B.; Zhu, X.; et al. Modification of conditioning cylinder for expanded tobacco based on fluent simulation. Tob. Sci. Technol. 2021, 54, 107–112. [Google Scholar]
- Qin, J.; Wang, L.; Jiang, W.; Wang, J.; Jia, T. Simulation analysis of tobacco leaf redrying and drying process based on COMSOL. Food Mach. 2021, 37, 136–141. [Google Scholar]
- Bejan, A. Convection Heat Transfer, 4th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2013; pp. 633–636. [Google Scholar]
- Datta, A.K. Porous media approaches to studying simultaneous heat and mass transfer in food processes. I: Problem formulations. J. Food Eng. 2007, 80, 80–95. [Google Scholar] [CrossRef]
- Alberghini, M.; Boriskina, S.V.; Asinari, P.; Fasano, M. Characterisation and modelling of water wicking and evaporation in capillary porous media for passive and energy-efficient applications. Appl. Therm. Eng. 2022, 208, 118159. [Google Scholar] [CrossRef]
- Nordlund, M.; Michaud, V. Dynamic saturation curve measurement for resin flow in glass fibre reinforcement. Compos. Part A Appl. Sci. Manuf. 2012, 43, 333–343. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Ni, H.; Datta, A.K.; Torrance, K. Moisture transport in intensive microwave heating of biomaterials: A multiphase porous media model. Int. J. Heat Mass Transf. 1999, 42, 1501–1512. [Google Scholar] [CrossRef]
- Cengel, Y.A.; Boles, M.A. Thermodynamics: An Engineering Approach, 8th ed.; McGraw-Hill Education: New York, NY, USA, 2009; p. 898. [Google Scholar]
- Halder, A.; Dhall, A.; Datta, A.K. Modeling transport in porous media with phase change: Applications to food processing. J. Heat Transf. 2011, 133, 031010. [Google Scholar] [CrossRef]
- Datta, A.K. Porous media approaches to studying simultaneous heat and mass transfer in food processes. II: Property data and representative results. J. Food Eng. 2007, 80, 96–110. [Google Scholar] [CrossRef]
- Gulati, T.; Datta, A.K. Enabling computer-aided food process engineering: Property estimation equations for transport phenomena-based models. J. Food Eng. 2013, 116, 483–504. [Google Scholar] [CrossRef]
- Rafiee, S.; Sharifi, M.; Keyhani, A.; Omid, M.; Jafari, A.; Mohtasebi, S.S.; Mobli, H. Modeling effective moisture diffusivity of orange slice (Thompson Cv.). Int. J. Food Prop. 2010, 13, 32–40. [Google Scholar] [CrossRef]
- Roache, P.J. Quantification of uncertainty in computational fluid dynamics. Annu. Rev. Fluid Mech. 1997, 29, 123–160. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, Z.; Xia, L.; Ma, Z. Optimization of the hot-air drying technical parameters for ginkgo fruit based on response surface methodology. Trans. Chin. Soc. Agric. Mach. 2012, 43, 140–145. [Google Scholar]
- Ghazanfari, A.; Emami, S.; Tabil, L.G.; Panigrahi, S. Thin-layer drying of flax fiber: II. Modeling drying process using semi-theoretical and empirical models. Dry. Technol. 2006, 24, 1637–1642. [Google Scholar] [CrossRef]
- Li, S.; Fu, Z.; Hu, R.; Shi, W.Q.; Cheng, X.Q.; Xiao, R.G.; Ling, P.; Fan, H.E.; Jian, B. Moisture change of tobacco leaves during curing and establishment of drying mathematical model. J. South. Agric. 2018, 49, 121–129. [Google Scholar]
- Chen, X.D. Moisture diffusivity in food and biological materials. Dry. Technol. 2007, 25, 1203–1213. [Google Scholar] [CrossRef]
- Doymaz, İ. Drying of green bean and okra under solar energy. Chem. Ind. Chem. Eng. Q./CICEQ 2011, 17, 199–205. [Google Scholar] [CrossRef]
- Shen, F.; Peng, L.; Zhang, Y.; Wu, J.; Zhang, X.; Yang, G.; Peng, H.; Qi, H.; Deng, S. Thin-layer drying kinetics and quality changes of sweet sorghum stalk for ethanol production as affected by drying temperature. Ind. Crops Prod. 2011, 34, 1588–1594. [Google Scholar] [CrossRef]

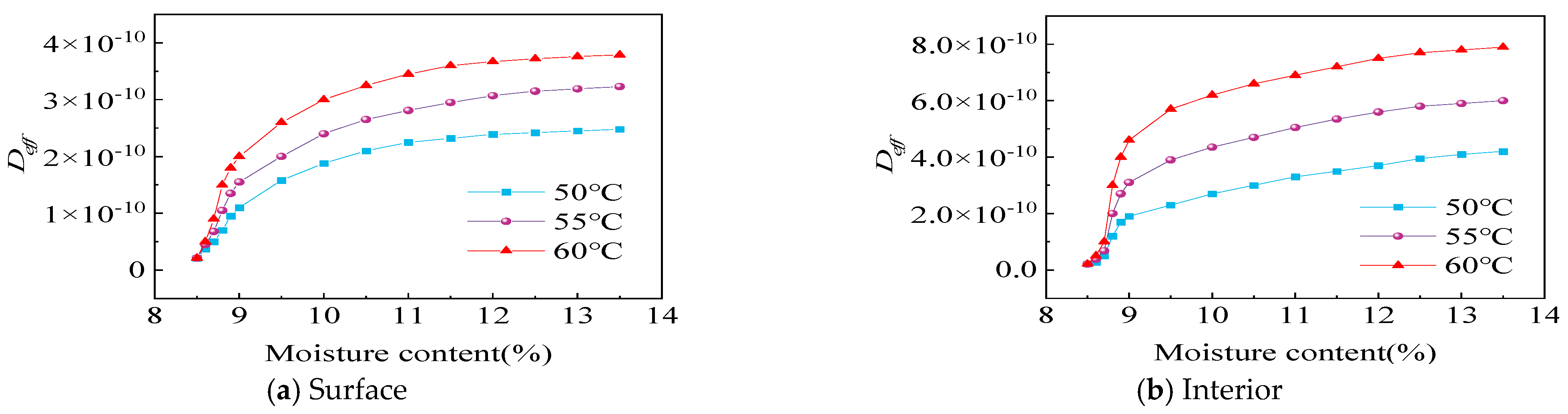
| Name | Value |
|---|---|
| porosity φ | 0.55 [17] |
| density of tobacco ρ | kg/m3 [8] |
| initial vapor mass fraction ωv | 0.85 |
| air heat capacity cp,a | 1006 J/kg/K [18] |
| vapor heat capacity cp,v | 2029 J/kg/K [18] |
| condensation constant Kcon | 1000 s−1 |
| universal gas constant R | 8.314 J/mol/K |
| initial moisture content cl(t=0) | 8.5% |
| initial pressure P | 1 atm |
| Grid | Element Number | Key Variable (Moisture Content) | Relative Error | GCI | α | |
|---|---|---|---|---|---|---|
| Grid1 | 10702 | 0.12253 | 1.0101 | |||
| Grid2 | 41090 | 0.12262 | 1→2 | 0.073% | 0.117% | |
| Grid3 | 229393 | 0.12264 | 2→3 | 0.016% | 0.026% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Wang, L.; Jiang, W.; Wei, Q.; Zhang, H. Analysis of Rewetting Characteristics and Process Parameters in Tobacco Strip Redrying Stage. Appl. Sci. 2022, 12, 11510. https://doi.org/10.3390/app122211510
Wang H, Wang L, Jiang W, Wei Q, Zhang H. Analysis of Rewetting Characteristics and Process Parameters in Tobacco Strip Redrying Stage. Applied Sciences. 2022; 12(22):11510. https://doi.org/10.3390/app122211510
Chicago/Turabian StyleWang, Huaiyu, Lihua Wang, Wei Jiang, Qike Wei, and Hao Zhang. 2022. "Analysis of Rewetting Characteristics and Process Parameters in Tobacco Strip Redrying Stage" Applied Sciences 12, no. 22: 11510. https://doi.org/10.3390/app122211510
APA StyleWang, H., Wang, L., Jiang, W., Wei, Q., & Zhang, H. (2022). Analysis of Rewetting Characteristics and Process Parameters in Tobacco Strip Redrying Stage. Applied Sciences, 12(22), 11510. https://doi.org/10.3390/app122211510






