Temperature-Dependent Density and Magnetohydrodynamic Effects on Mixed Convective Heat Transfer along Magnetized Heated Plate in Thermally Stratified Medium Using Keller Box Simulation
Abstract
1. Introduction and Literature Review
2. Flow Problem and Mathematical Description
3. The Similarity Transformation and Stream Function Formulation
4. The Solution and Computing Techniques
5. The Arrangement of Difference Equations in Vector Notation/Matrix
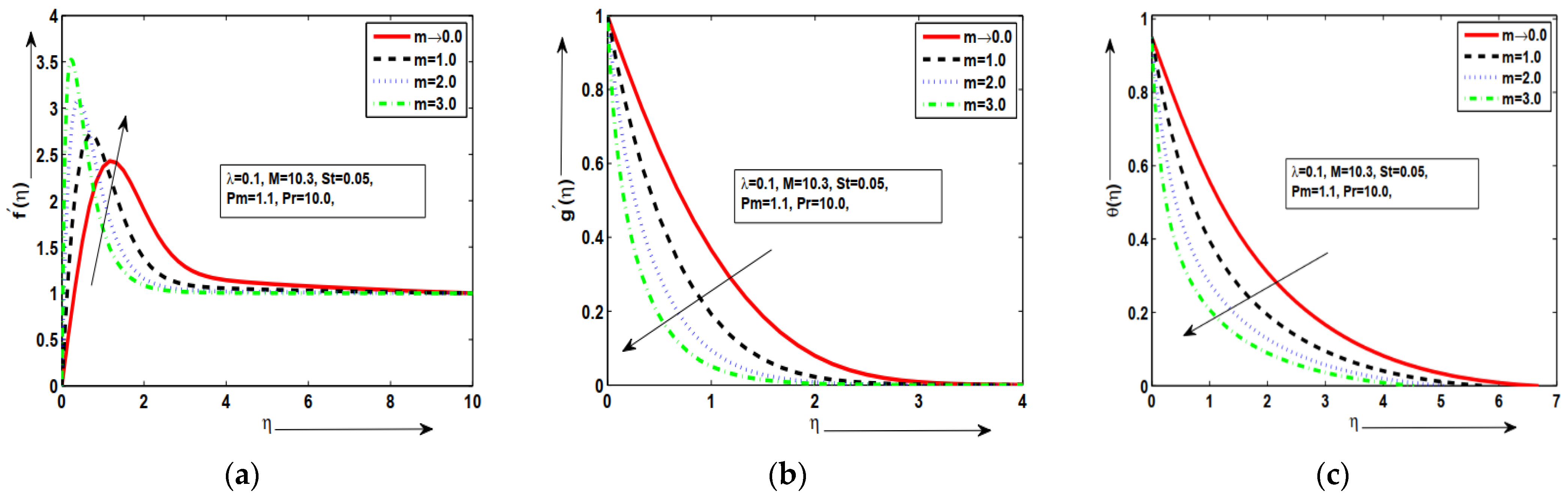
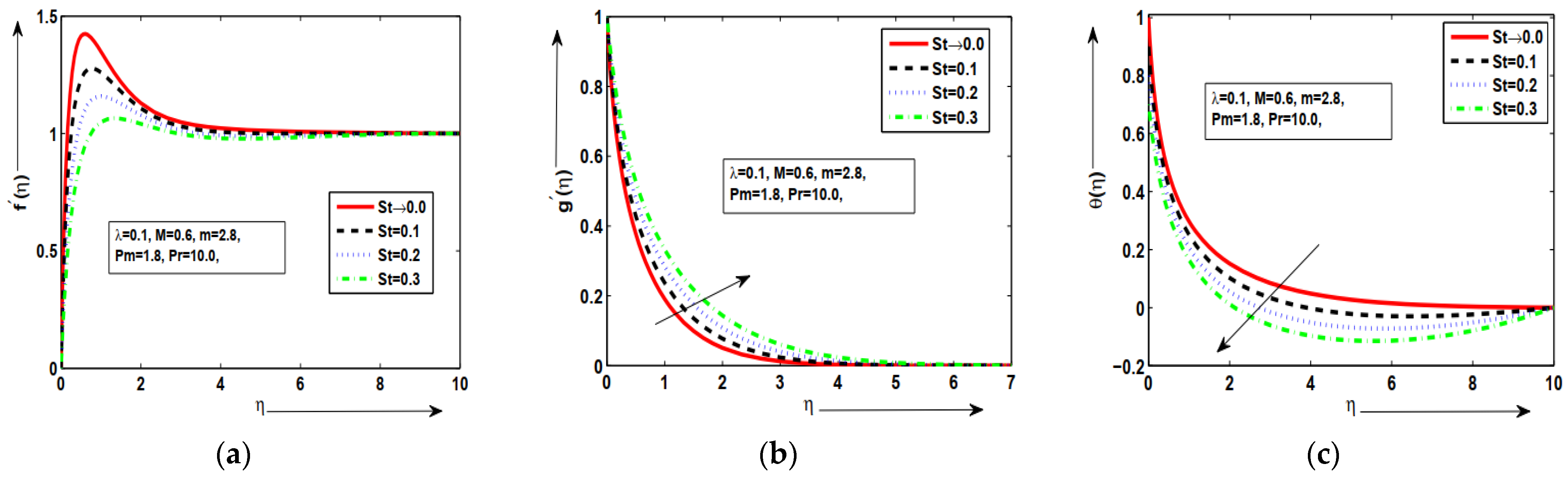
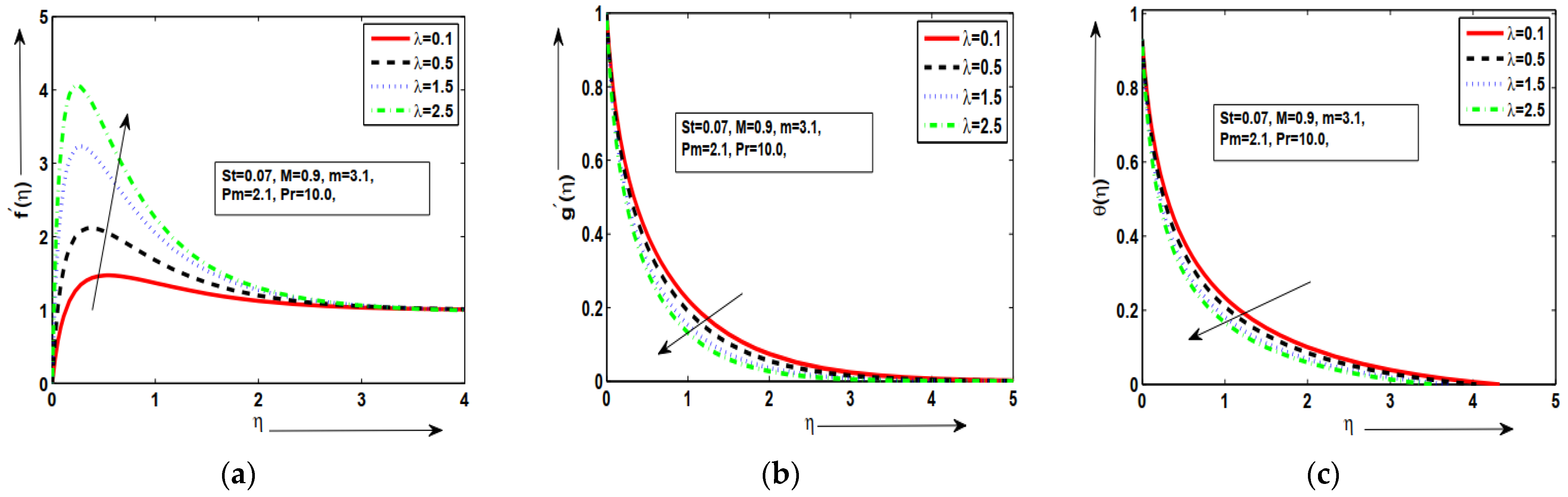
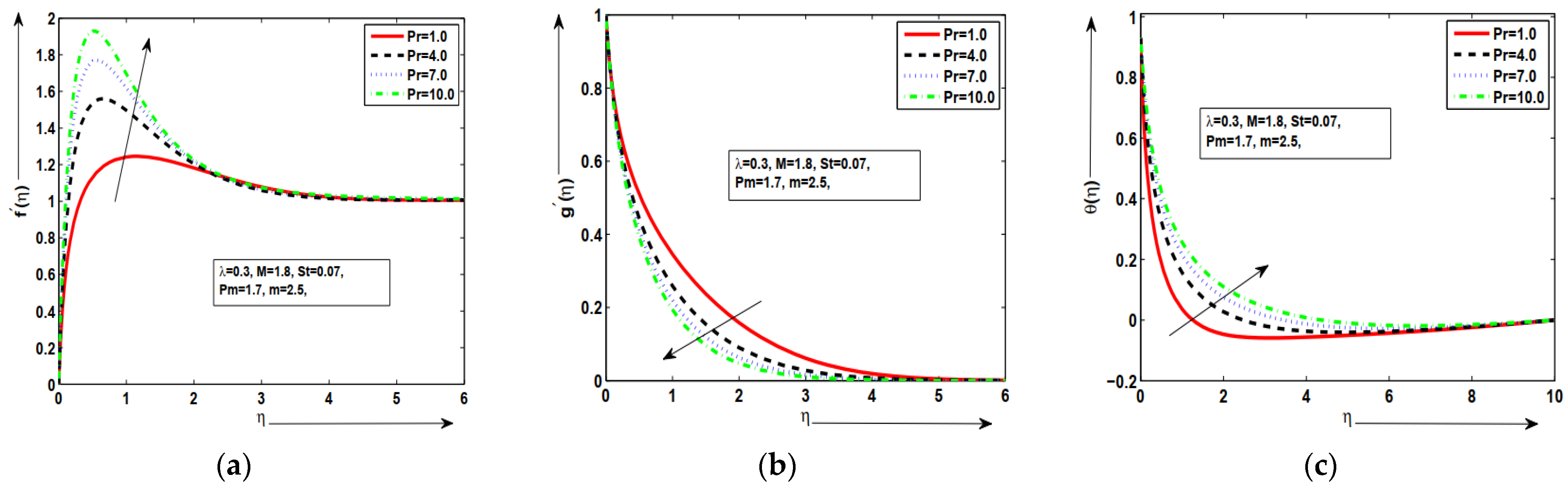
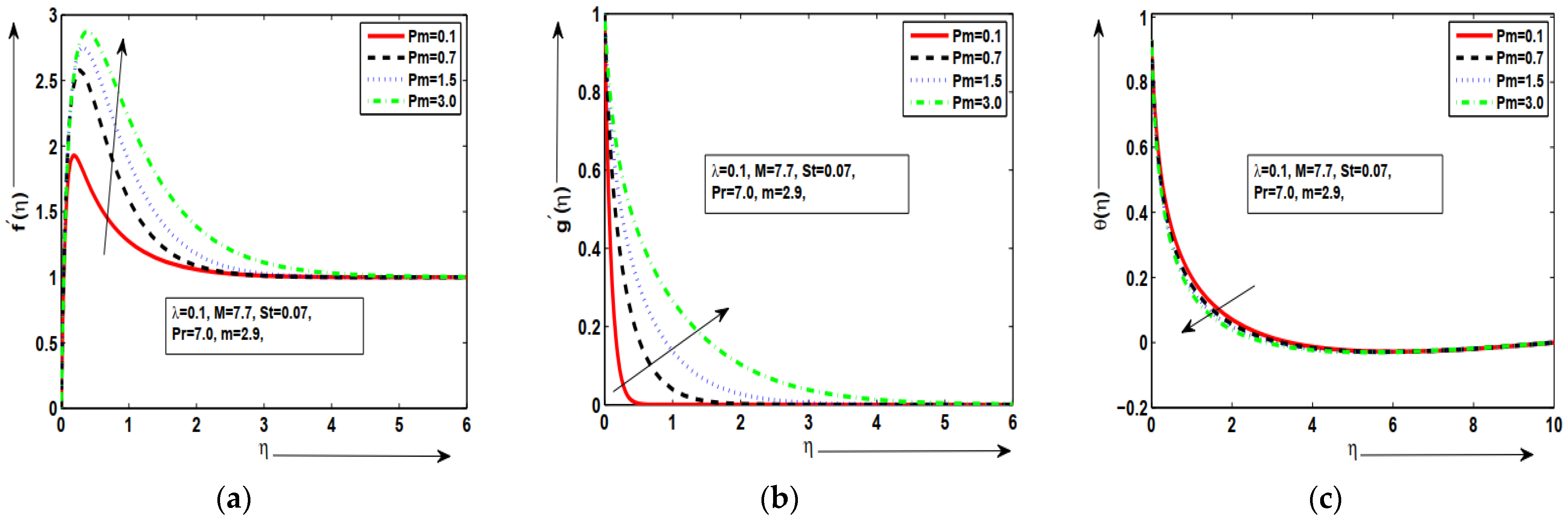
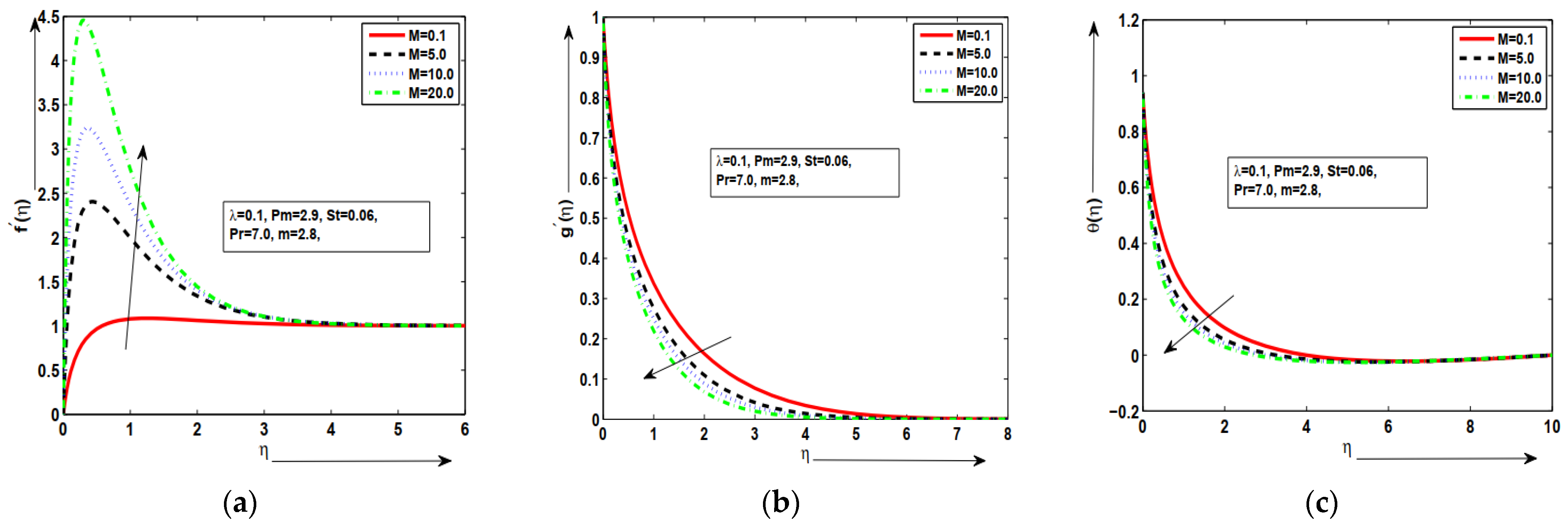
6. Analysis and Discussion of Results
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nima, N.I.; Ferdows, M. Dual Solutions in Mixed Convection Flow Along Non-Isothermal Inclined Cylinder Containing Gyrotactic Microorganism. J. Adv. Res. Fluid Mech. Therm. Sci. 2021, 87, 51–63. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Gireesha, B.J.; Gorla, R.S.R. Mixed convection squeezing three-dimensional flow in a rotating channel filled with nanofluid. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 1460–1485. [Google Scholar] [CrossRef]
- Bansod, V.J.; Ambedkar, B. An integral treatment for combined heat and mass transfer by mixed convection along vertical surface in a saturated porous medium. J. Mech. Eng. Res. 2011, 3, 138–151. [Google Scholar]
- Hazarika, S.; Ahmed, S. Numerical Investigation of Endothermic/Exothermic Reaction in MHD Natural Convective Nano-Fluid Flow over a Vertical Cone with Heat Source/Sink. Walailak J. Sci. Technol. 2021, 18, 22834. [Google Scholar] [CrossRef]
- Chaudhary, K.; Sharma, A.; Jha, A. Laminar mixed convection flow from a vertical surface with induced magnetic field and convective boundary. Int. J. Appl. Mech. Eng. 2018, 23, 307–326. [Google Scholar] [CrossRef]
- Ramreddy, C.; Kairi, R. The effect of melting on mixed convection heat and mass transfer in non-Newtonian nanofluid SATURATEED in porous medium. Front. Heat Mass Transf. 2015, 6, 6. [Google Scholar] [CrossRef]
- Hussain, A.; Haider, Q.; Rehman, A.; Abdussattar, A.; Malik, M.Y. A New Heat Dissipation Model and Convective Two-Phase Nanofluid in Brittle Medium Flow over a Cone. Math. Probl. Eng. 2021, 2021, 6688747. [Google Scholar] [CrossRef]
- Mahdy, A. Free convection flow over a vertical flat plate in nanofluid porous media containing gyrotactic microorganisms with prescribed density motile microorganisms flux. Sci. Technol. Asia 2017, 22, 71–85. [Google Scholar]
- Pullepu, B.; Sambath, P.; Rani, M.S.; Chamkha, A.J.; Viswanathan, K.K. Numerical Solutions of Free Convective Flow from a Vertical Cone with Mass Transfer under the Influence of Chemical Reaction and Heat Generation/Absorption in the Presence of UWT/UWC. J. Appl. Fluid Mech. 2016, 9, 343–356. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Surender, O. Effect of double stratification on mixed convection boundary layer flow of a nanofluid past a vertical plate in a porous medium. Appl. Nanosci. 2014, 5, 29–38. [Google Scholar] [CrossRef]
- Ullah, Z.; Ashraf, M.; Rashad, A.M. Magneto-thermo analysis of oscillatory flow around a non-conducting horizontal circular cylinder. J. Therm. Anal. 2020, 142, 1567–1578. [Google Scholar] [CrossRef]
- Ullah, Z.; Ashraf, M.; Zia, S.; Ali, I. Surface temperature and freestream velocity oscillation effects on mixed convention slip flow from surface of a horizontal circular cylinder. Therm. Sci. 2020, 24 (Suppl. 1), 13–23. [Google Scholar] [CrossRef]
- Ullah, Z.; Ashraf, M.; Zia, S.; Chu, Y.-M.; Khan, I.; Nisar, K.S. Computational analysis of the oscillatory mixed convection flow along a horizontal circular cylinder in thermally stratified medium. Comput. Mater. Contin. 2020, 65, 109–123. [Google Scholar] [CrossRef]
- Ullah, Z.; Ashraf, M.; Sarris, I.E.; Karakasidis, T.E. The Impact of Reduced Gravity on Oscillatory Mixed Convective Heat Transfer around a Non-Conducting Heated Circular Cylinder. Appl. Sci. 2022, 12, 5081. [Google Scholar] [CrossRef]
- Al-Farhany, K.; Al-Muhja, B.; Loganathan, K.; Periyasamy, U.; Ali, F.; Sarris, I.E. Analysis of Convection Phenomenon in Enclosure Utilizing Nanofluids with Baffle Effects. Energies 2022, 15, 6615. [Google Scholar] [CrossRef]
- Al-Mdallal, Q.; Prasad, V.R.; Basha, H.T.; Sarris, I.; Akkurt, N. Keller box simulation of magnetic pseudoplastic nano-polymer coating flow over a circular cylinder with entropy optimisation. Comput. Math. Appl. 2022, 118, 132–158. [Google Scholar] [CrossRef]
- Sofiadis, G.; Sarris, I. Reynolds number effect of the turbulent micropolar channel flow. Phys. Fluids 2022, 34, 075126. [Google Scholar] [CrossRef]
- Mehmood, K.; Hussain, S.; Sagheer, M. Mixed convection flow with non-uniform heat source/sink in a doubly stratified magnetonanofluid. AIP Adv. 2016, 6, 065126. [Google Scholar] [CrossRef]
- Elbashbeshy, E.M.; Emam, T.; Abdelgaber, K. Effects of thermal radiation and magnetic field on unsteady mixed convection flow and heat transfer over an exponentially stretching surface with suction in the presence of internal heat generation/absorption. J. Egypt. Math. Soc. 2012, 20, 215–222. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Thermal radiation on unsteady electrical MHD flow of nanofluid over stretching sheet with chemical reaction. J. King Saud Univ. -Sci. 2019, 31, 804–812. [Google Scholar] [CrossRef]
- Jena, S.; Dash, G.; Mishra, S. Chemical reaction effect on MHD viscoelastic fluid flow over a vertical stretching sheet with heat source/sink. Ain Shams Eng. J. 2016, 9, 1205–1213. [Google Scholar] [CrossRef]
- Yasin, M.H.M.; Arifin, N.M.; Nazar, R.; Ismail, F.; Pop, I. Mixed convection boundary layer flow embedded in a thermally stratified porous medium saturated by a nanofluid. Adv. Mech. Eng. 2013, 121943. [Google Scholar] [CrossRef]
- Rawi, N.A.; Ilias, M.R.; Jiann, L.Y.; Isa, Z.M.; Shafie, S. The effect of copper nanoparticles on mixed convection flow of jeffrey fluid induced by g-jitter. J. Nanofluids 2018, 7, 156–162. [Google Scholar] [CrossRef]
- Alzahrani, E.O.; Shah, Z.; Dawar, A.; Malebary, S.J. Hydromagnetic mixed convective third grade nanomaterial containing gyrotactic microorganisms toward a horizontal stretched surface. Alex. Eng. J. 2019, 58, 1421–1429. [Google Scholar] [CrossRef]
- Ullah, Z.; Ehsan, M.; Ahmad, H.; Ilyas, A. Combined effects of MHD and slip velocity on oscillatory mixed convective flow around a non-conducting circular cylinder embedded in a porous medium. Case Stud. Therm. Eng. 2022, 38, 102341. [Google Scholar] [CrossRef]
- Alsenafi, A.; Ferdows, M. Dual solution for double-diffusive mixed convection opposing flow through a vertical cylinder saturated in a Darcy porous media containing gyrotactic microorganisms. Sci. Rep. 2021, 11, 19918. [Google Scholar] [CrossRef] [PubMed]
- Babu, S.R.C.; Venkateswarlu, S.; Lakshmi, K.J. Effect of variable viscosity and thermal conductivity on heat and mass transfer flow of nanofluid over a vertical cone with chemical reaction. Int. J. Appl. Eng. Res. 2018, 13, 13989–14002. [Google Scholar]
- Salem, A.M.; El-Aziz, M.A.; Abo-Eldahab, E.M.; Abd-Elfatah, I. Effect of variable density on hydromagnetic mixed convection flow of a non-Newtonian fluid past a moving vertical plate. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 1485–1493. [Google Scholar] [CrossRef]
- Iqbal, M.S.; Khan, W.; Mustafa, I.; Ghaffari, A. Numerical study of natural convection flow of nanofluid past a circular cone with Cattaneo–Christov heat and mass flux models. Symmetry 2019, 11, 1363. [Google Scholar] [CrossRef]
- Abualnaja, K.M.; Elgendy, M.S.; Ibrahim, F.S. Unsteady Mixed Convection Flow along Symmetric Wedge with Variable Surface Temperature Embedded in a Porous Medium Saturated with a Nanofluid. J. Appl. Math. Phys. 2021, 09, 101–126. [Google Scholar] [CrossRef]
- Maranna, T.; Sneha, K.N.; Mahabaleshwar, U.S.; Sarris, I.E.; Karakasidis, T.E. An Effect of Radiation and MHD Newtonian Fluid over a Stretching/Shrinking Sheet with CNTs and Mass Transpiration. Appl. Sci. 2022, 12, 5466. [Google Scholar] [CrossRef]
- Mabood, F.; Fatunmbi, E.O.; Benos, L.; Sarris, I.E. Entropy Generation in the Magnetohydrodynamic Jeffrey Nanofluid Flow Over a Stretching Sheet with Wide Range of Engineering Application Parameters. Int. J. Appl. Comput. Math. 2022, 8, 98. [Google Scholar] [CrossRef]
- Kakac, S.; Yener, Y.; Pramuanjaroenkij, A. Convective Heat Transfer; CRC Press: Boca Raton, FL, USA, 1995; pp. 312–314, 399. [Google Scholar]

| 0.695631064636244 | 0.800391659576938 | 0.330556513906720 | |
| 0.728442870560860 | 0.843207966030059 | 0.352119633819730 | |
| 0.809106015652243 | 0.939444897313997 | 0.400936354502018 | |
| 0.913457974253570 | 1.051844490851958 | 0.458533654900388 |
| 0.984042850440267 | 0.470578779940417 | 0.386755927577512 | |
| 0.973834647776395 | 0.467462868621491 | 0.379378708437730 | |
| 0.963745902362756 | 0.464369050922372 | 0.372022244909067 | |
| 0.953775319662017 | 0.461297160471197 | 0.364686553985701 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ullah, Z.; Akkurt, N.; Alrihieli, H.F.; Eldin, S.M.; Alqahtani, A.M.; Hussanan, A.; Ashraf, M.; Jabeen, M. Temperature-Dependent Density and Magnetohydrodynamic Effects on Mixed Convective Heat Transfer along Magnetized Heated Plate in Thermally Stratified Medium Using Keller Box Simulation. Appl. Sci. 2022, 12, 11461. https://doi.org/10.3390/app122211461
Ullah Z, Akkurt N, Alrihieli HF, Eldin SM, Alqahtani AM, Hussanan A, Ashraf M, Jabeen M. Temperature-Dependent Density and Magnetohydrodynamic Effects on Mixed Convective Heat Transfer along Magnetized Heated Plate in Thermally Stratified Medium Using Keller Box Simulation. Applied Sciences. 2022; 12(22):11461. https://doi.org/10.3390/app122211461
Chicago/Turabian StyleUllah, Zia, Nevzat Akkurt, Haifaa F. Alrihieli, Sayed M. Eldin, Aisha M. Alqahtani, Abid Hussanan, Muhammad Ashraf, and Mah Jabeen. 2022. "Temperature-Dependent Density and Magnetohydrodynamic Effects on Mixed Convective Heat Transfer along Magnetized Heated Plate in Thermally Stratified Medium Using Keller Box Simulation" Applied Sciences 12, no. 22: 11461. https://doi.org/10.3390/app122211461
APA StyleUllah, Z., Akkurt, N., Alrihieli, H. F., Eldin, S. M., Alqahtani, A. M., Hussanan, A., Ashraf, M., & Jabeen, M. (2022). Temperature-Dependent Density and Magnetohydrodynamic Effects on Mixed Convective Heat Transfer along Magnetized Heated Plate in Thermally Stratified Medium Using Keller Box Simulation. Applied Sciences, 12(22), 11461. https://doi.org/10.3390/app122211461









