Study on Crack Identification with Responses Modulated by Nonlinear Energy Sink
Abstract
1. Introduction
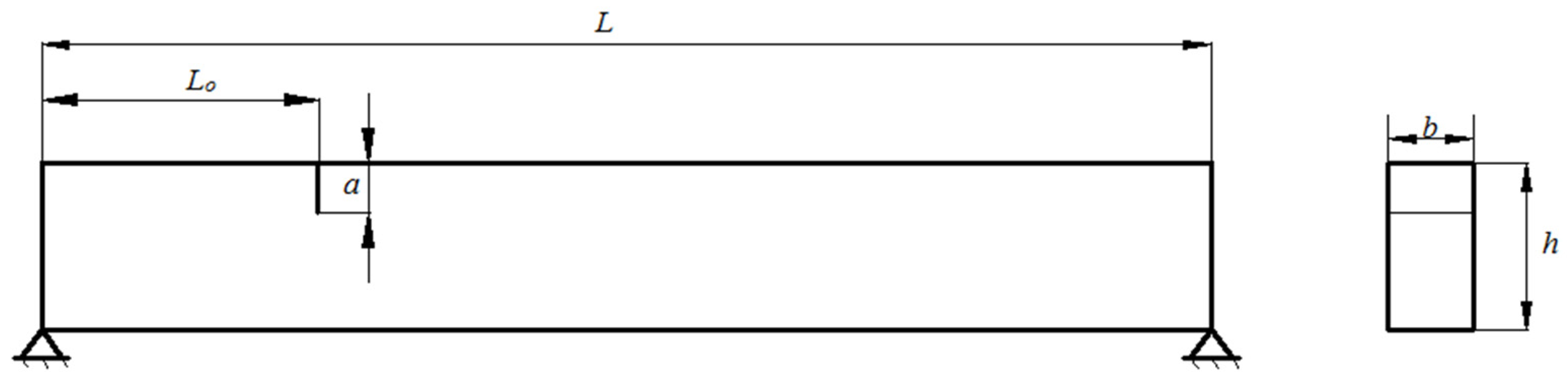
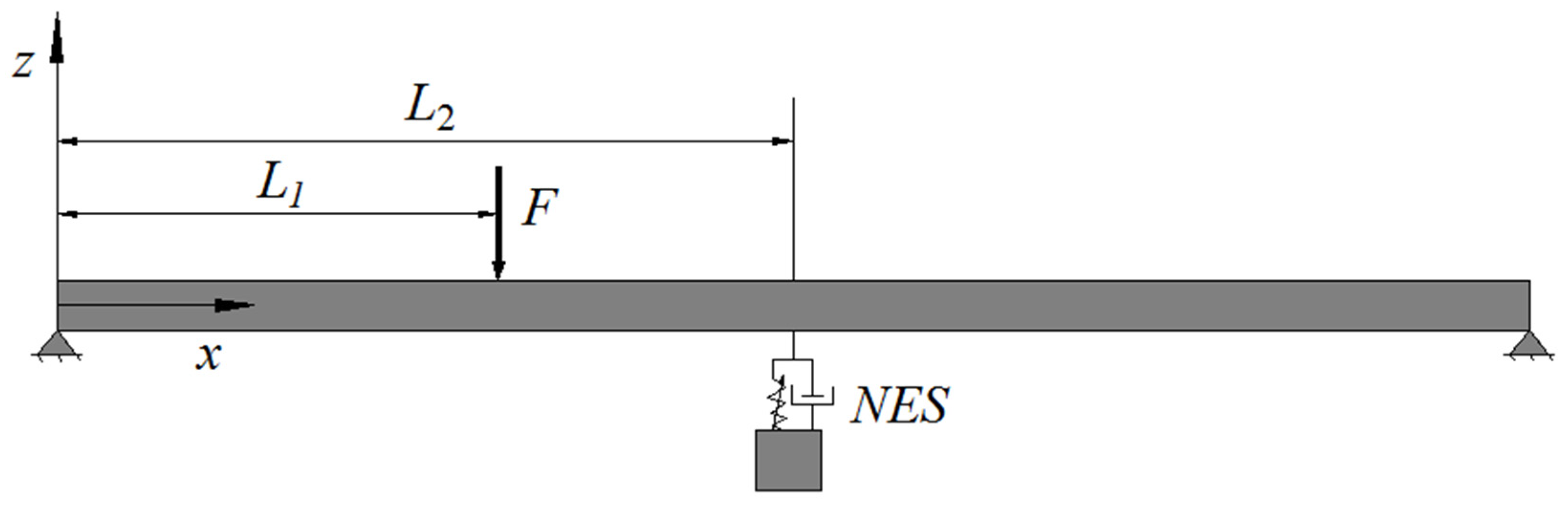
2. Dynamic Equation of a Breathing Cracked Beam-NES System
3. Crack Identification Based on Single-Period Response
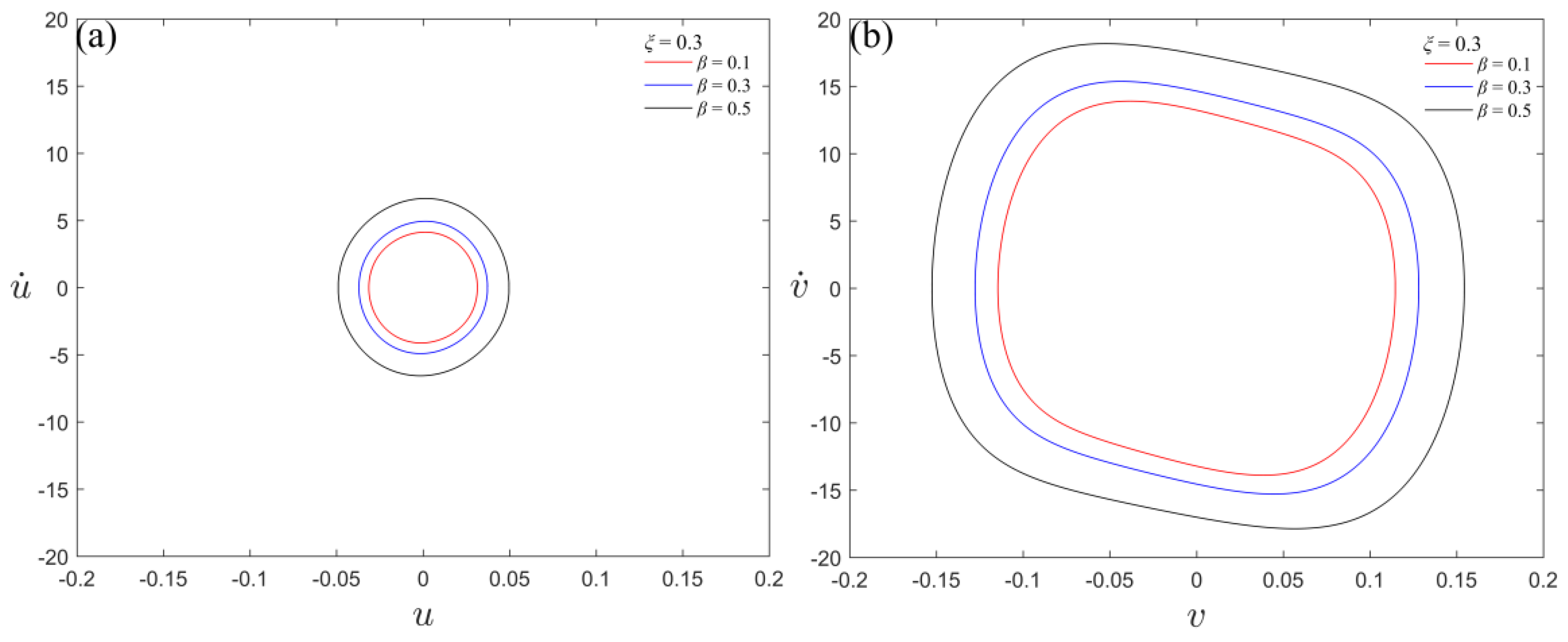
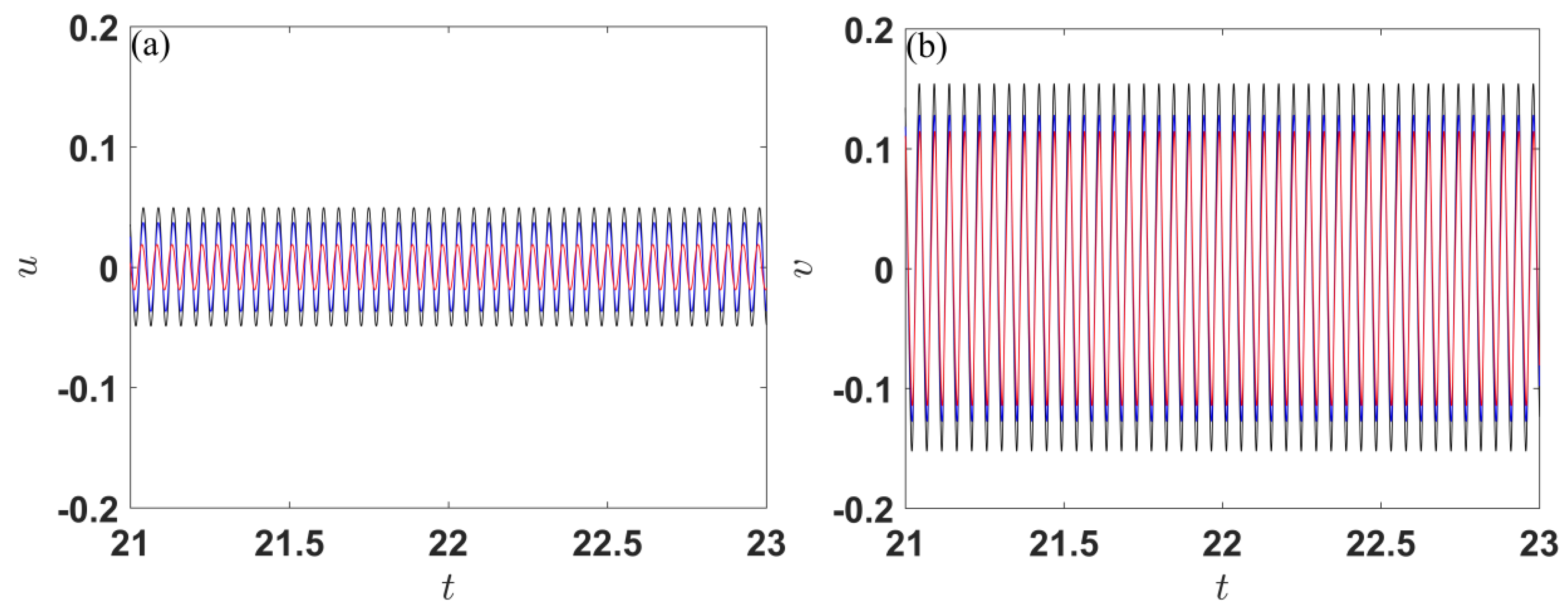
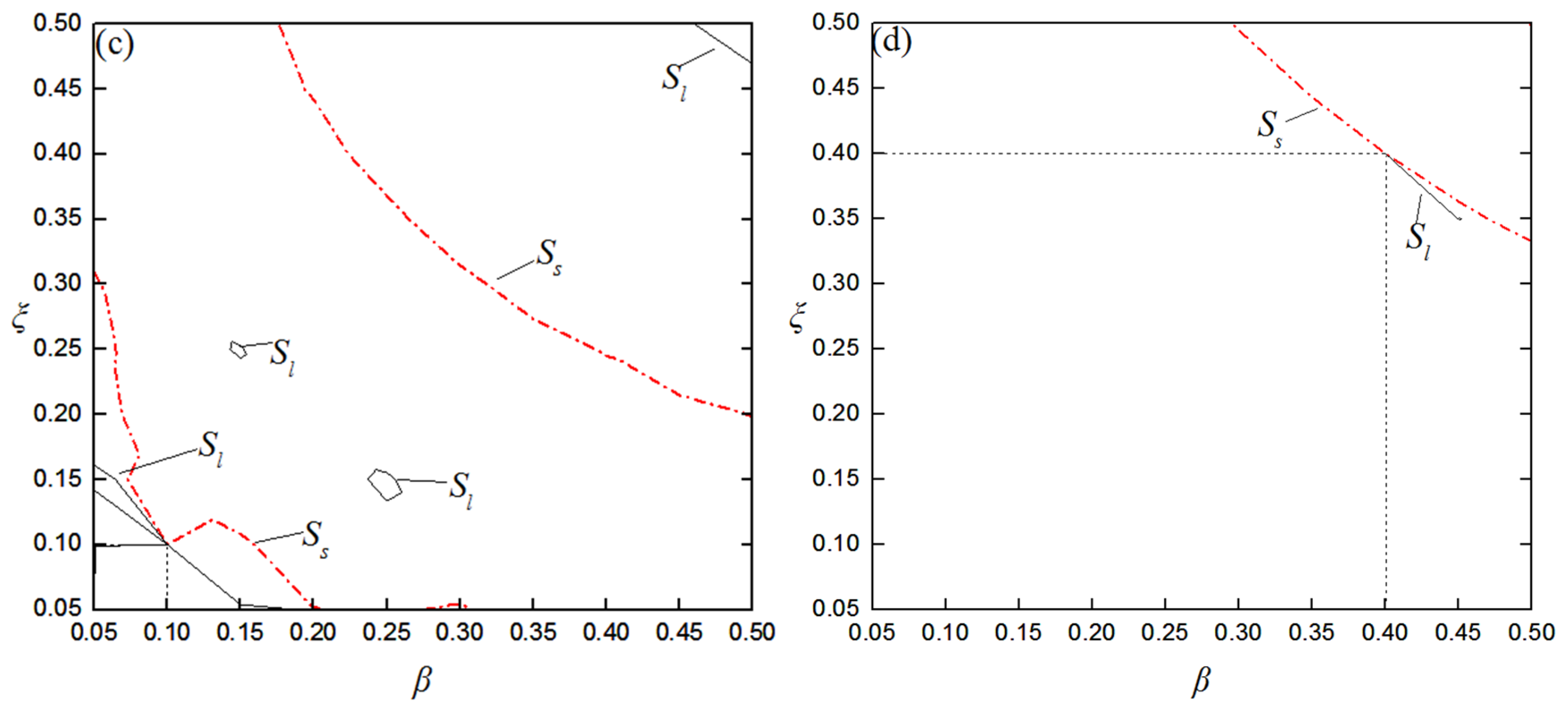
4. Crack Identification Based on Strongly Modulated Response
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vakakis, A.F. Passive nonlinear targeted energy transfer. Philos. Trans. R. Soc. A-Math. Phys. Eng. Sci. 2018, 376, 20170132. [Google Scholar] [CrossRef] [PubMed]
- Gendelman, O.V. Targeted energy transfer in systems with external and self-excitation. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 2007–2043. [Google Scholar] [CrossRef]
- Ding, H.; Chen, L.Q. Designs, analysis, and applications of nonlinear energy sinks. Nonlinear Dyn. 2020, 100, 3061–3107. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, Z.X.; Zhou, Y.; Lu, X.L. Nonlinear dissipative devices in structural vibration control: A review. J. Sound Vib. 2018, 423, 18–49. [Google Scholar] [CrossRef]
- Lee, Y.S.; Vakakis, A.F.; Bergman, L.A.; Mcfarland, D.M.; Kerschen, G. Suppression aeroelastic instability using broadband passive targeted energy transfers, part 1: Theory. AIAA J. 2007, 45, 693–711. [Google Scholar] [CrossRef]
- Nucera, F.; Lo Iacono, F.; McFarland, D.M.; Bergman, L.A.; Vakakis, A.F. Application of broadband nonlinear targeted energy transfers for seismic mitigation of a shear frame: Experimental results. J. Sound Vib. 2008, 313, 57–76. [Google Scholar] [CrossRef]
- Yang, T.Z.; Liu, T.; Tang, Y.; Hou, S.; Lv, X.F. Enhanced targeted energy transfer for adaptive vibration suppression of pipes conveying fluid. Nonlinear Dyn. 2019, 97, 1937–1944. [Google Scholar] [CrossRef]
- Bab, S.; Khadem, S.E.; Shahgholi, M.; Abbasi, A. Vibration attenuation of a continuous rotor-blisk-journal bearing system employing smooth nonlinear energy sinks. Mech. Syst. Signal Process. 2017, 84, 128–157. [Google Scholar] [CrossRef]
- Gendelman, O.V. Analytic treatment of a system with a vibro-impact nonlinear energy sink. J. Sound Vib. 2012, 331, 4599–4608. [Google Scholar] [CrossRef]
- Gourc, E.; Michon, G.; Seguy, S.; Berlioz, A. Targeted energy transfer under harmonic forcing with a vibro-impact nonlinear energy sink: Analytical and experimental developments. J. Vib. Acoust. 2015, 137, 031008. [Google Scholar] [CrossRef]
- Romeo, F.; Sigalov, G.; Bergman, L.A.; Vakakis, A.F. Dynamics of a linear oscillator coupled to a bistable light attachment: Numerical study. J. Comput. Nonlinear Dyn. 2015, 10, 011007. [Google Scholar] [CrossRef]
- Yao, H.L.; Wang, Y.W.; Cao, Y.B.; Wen, B.C. Multi-stable nonlinear energy sink for rotor system. Int. J. Non-Linear Mech. 2020, 118, 103273. [Google Scholar] [CrossRef]
- Zang, J.; Yuan, T.C.; Lu, Z.Q.; Zhang, Y.W.; Ding, H.; Chen, L.Q. A lever-type nonlinear energy sink. J. Sound Vib. 2018, 437, 119–134. [Google Scholar] [CrossRef]
- Chen, H.Y.; Mao, X.Y.; Ding, H.; Chen, L.Q. Elimination of multimode resonances of composite plate by inertial nonlinear energy sinks. Mech. Syst. Signal Process. 2020, 135, 106383. [Google Scholar] [CrossRef]
- Chen, J.E.; Sun, M.; Hu, W.H.; Zhang, J.H.; Wei, Z.C. Performance of non-smooth nonlinear energy sink with descending stiffness. Nonlinear Dyn. 2020, 100, 255–267. [Google Scholar] [CrossRef]
- Starosvetsky, Y.; Gendelman, O.V. Vibration absorption in systems with a nonlinear energy sink: Nonlinear damping. J. Sound Vib. 2009, 324, 916–939. [Google Scholar] [CrossRef]
- Sun, M.; Hu, W.H.; Liu, J.; Chen, J.E. Steady-state responses of mechanical system attached to non-smooth vibration absorber with piecewise damping and stiffness. Meccanica 2021, 56, 275–285. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Y.W.; Ding, H.; Chen, L.Q. Dynamics and evaluation of a nonlinear energy sink integrated by a piezoelectric energy harvester under a harmonic excitation. J. Vib. Control 2019, 25, 851–867. [Google Scholar] [CrossRef]
- Ahmadabadi, Z.N.; Khadem, S.E. Nonlinear vibration control and energy harvesting of a beam using a nonlinear energy sink and a piezoelectric device. J. Sound Vib. 2014, 333, 4444–4457. [Google Scholar] [CrossRef]
- Zang, J.; Cao, R.Q.; Fang, B.; Zhang, Y.W. A vibratory energy harvesting absorber using integration of a lever-enhanced nonlinear energy sink and a levitation magnetoelectric energy harvester. J. Sound Vib. 2020, 484, 115534. [Google Scholar] [CrossRef]
- Fang, Z.W.; Zhang, Y.W.; Li, X.; Ding, H.; Chen, L.Q. Integration of a nonlinear energy sink and a giant magnetostrictive energy harvester. J. Sound Vib. 2017, 391, 35–49. [Google Scholar] [CrossRef]
- Huang, D.M.; Li, R.H.; Yang, G.D. On the dynamic response regimes of a viscoelastic isolation system integrated with a nonlinear energy sink. Commun. Nonlinear Sci. Numer. Simul. 2019, 79, 104916. [Google Scholar] [CrossRef]
- Kim, J.T.; Ryu, Y.S.; Cho, H.M.; Stubbs, N. Damage identification in beam-type structures:frequency-based method vs. mode-shape based method. Eng. Struct. 2003, 25, 57–67. [Google Scholar] [CrossRef]
- Doebling, S.W.; Farrar, C.R.; Prime, M.B. A Summary review of vibration-based damage identification methods. Shock Vib. Dig. 1998, 30, 91–105. [Google Scholar] [CrossRef]
- Carden, E.P.; Fanning, P. Vibration based condition monitoring: A review. Struct. Health Monit. 2004, 3, 355–377. [Google Scholar] [CrossRef]
- Bovsunovsky, A.P.; Surace, C. Considerations regarding superharmonic vibrations of acracked beam and the variation in damping caused by the presence of the crack. J. Sound Vib. 2005, 28, 865–886. [Google Scholar] [CrossRef]
- Semperlotti, F.; Wang, K.W.; Smith, E.C. Localization of a breathing crack using superharmonic signals due to system nonlinearity. AIAA J. 2009, 47, 2076–2086. [Google Scholar] [CrossRef]
- Bovsunovsky, A.P.; Bovsunovsky, O. Crack detection in beams by means of the driving force parameters variation at non-linear resonance vibrations. Key Eng. Mater. 2007, 347, 413–420. [Google Scholar] [CrossRef]
- Xu, W.; Ding, K.Q.; Liu, J.Q.; Cao, M.S.; Radzienski, M.; Ostachowicz, W. Non-uniform crack identification in plate-like structures using wavelet 2D modal curvature under noisy conditions. Mech. Syst. Signal Process. 2019, 126, 469–489. [Google Scholar] [CrossRef]
- Rezaee, M.; Shaterian-Alghalandis, V. A new crack detection method in a beam under geometrically nonlinear vibration. Arch. Appl. Mech. 2018, 88, 1491–1506. [Google Scholar] [CrossRef]
- Cheng, C.; Nie, Z.H.; Ma, H.W. Structural damage detection of the simple beam based on responses phase space. Adv. Mater. Res. 2013, 605–607, 985–995. [Google Scholar] [CrossRef]
- Liu, W.; Barkey, M.E. Nonlinear vibrational response of a single edge cracked beam. J. Mech. Sci. Technol. 2017, 31, 5231–5243. [Google Scholar] [CrossRef]
- Andreaus, U.; Baragatti, P. Experimental damage detection of cracked beams by using nonlinear characteristics of forced response. Mech. Syst. Signal Process. 2012, 31, 382–404. [Google Scholar] [CrossRef]
- Andreaus, U.; Baragatti, P. Cracked beam identification by numerically analyzing the nonlinear behavior of the harmonically forced response. J. Sound Vib. 2011, 330, 721–742. [Google Scholar] [CrossRef]
- Huang, Y.H.; Chen, J.E.; Ge, W.M.; Bian, X.L.; Hu, W.H. Research on geometric features of phase diagram and crack identification of cantilever beam with breathing crack. Results Phys. 2019, 15, 102561. [Google Scholar] [CrossRef]
- Dimarogonas, A.D.; Paipetis, S.A.; Chondros, T.G. Analytical Methods in Rotor Dynamics; Applied Science Publishers: London, UK, 1983. [Google Scholar]
- Mousa, R.; Reza, H. Free vibration analysis of simply supported beam with breathing crack using perturbation method. Acta Mech. Solida Sin. 2010, 23, 459–470. [Google Scholar]
- Douka, E.; Hadjileontiadis, J.L. Time-frequency analysis of the free vibration response of a beam with a breathing crack. NDT&E Int. 2005, 38, 3–10. [Google Scholar]


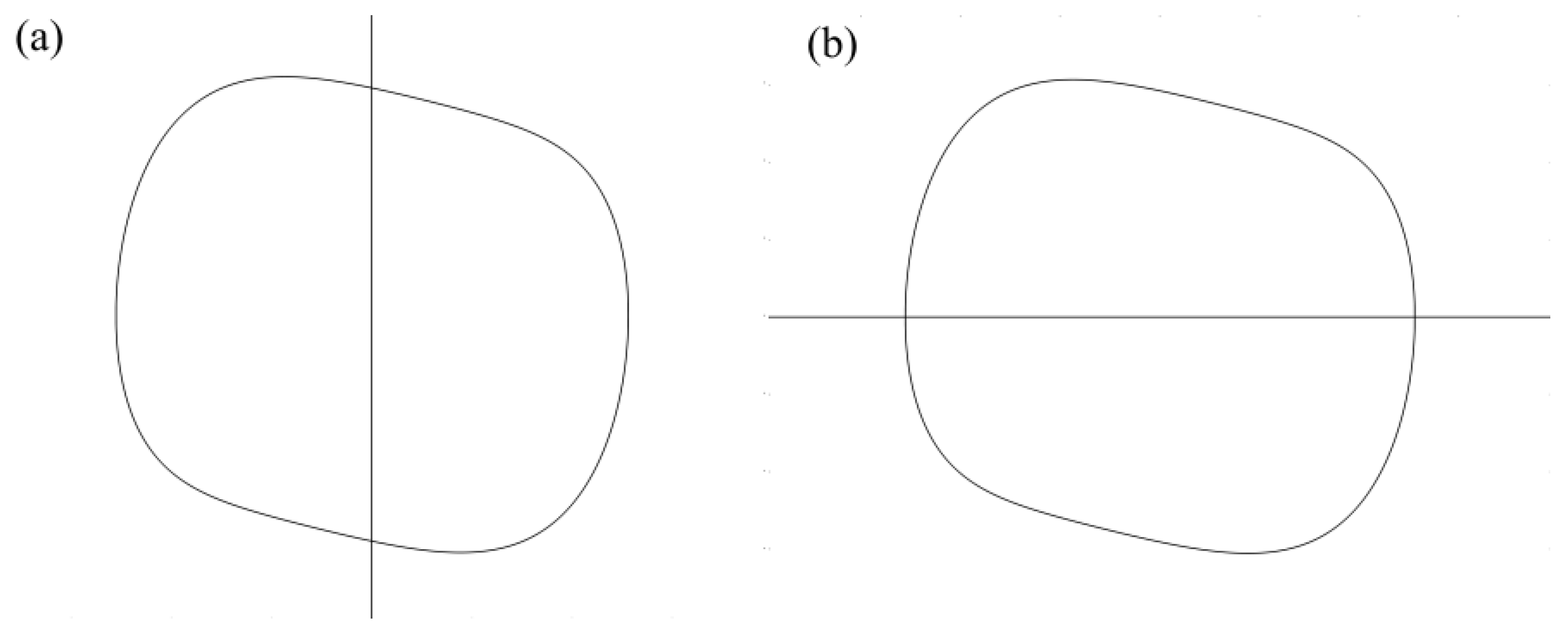
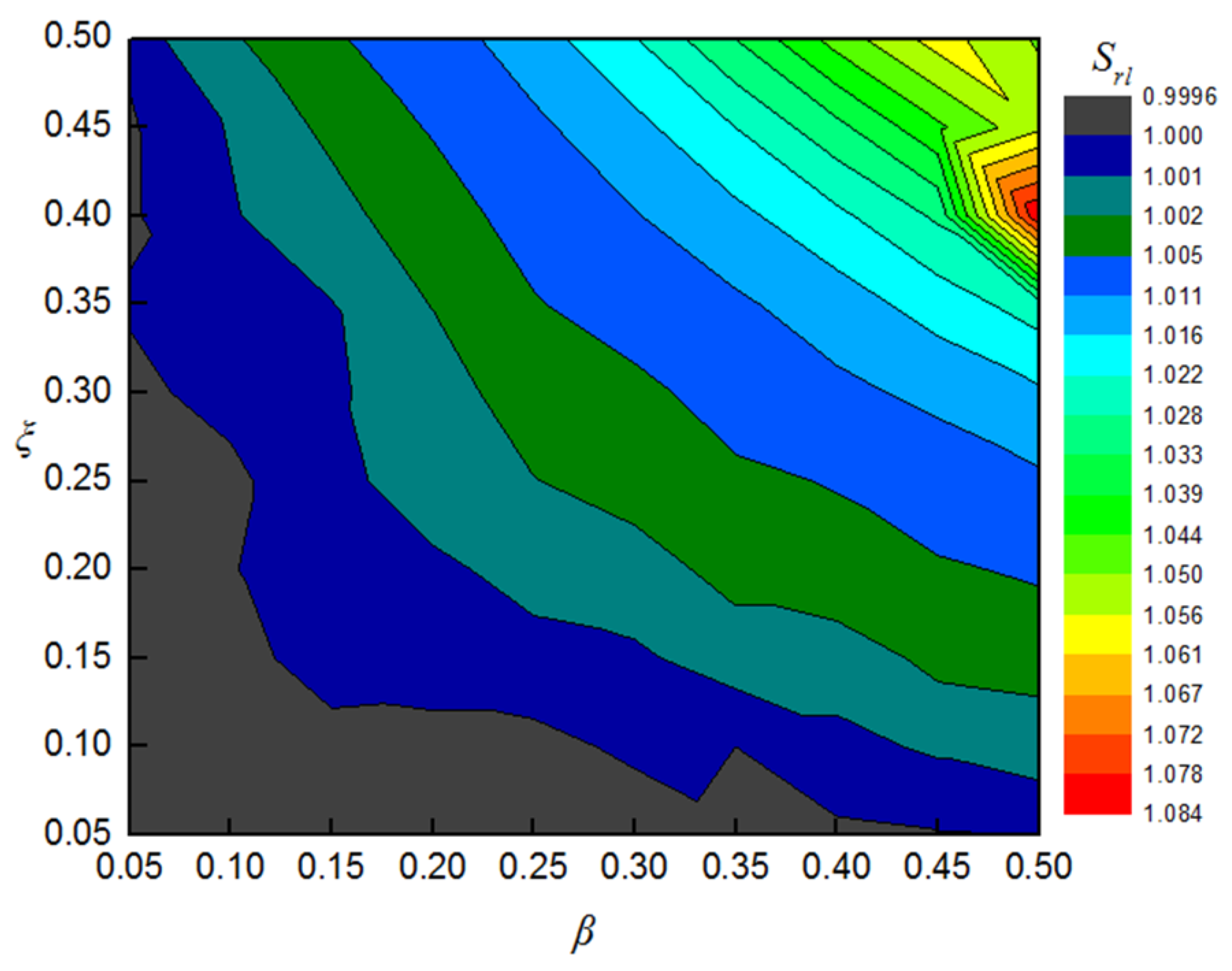
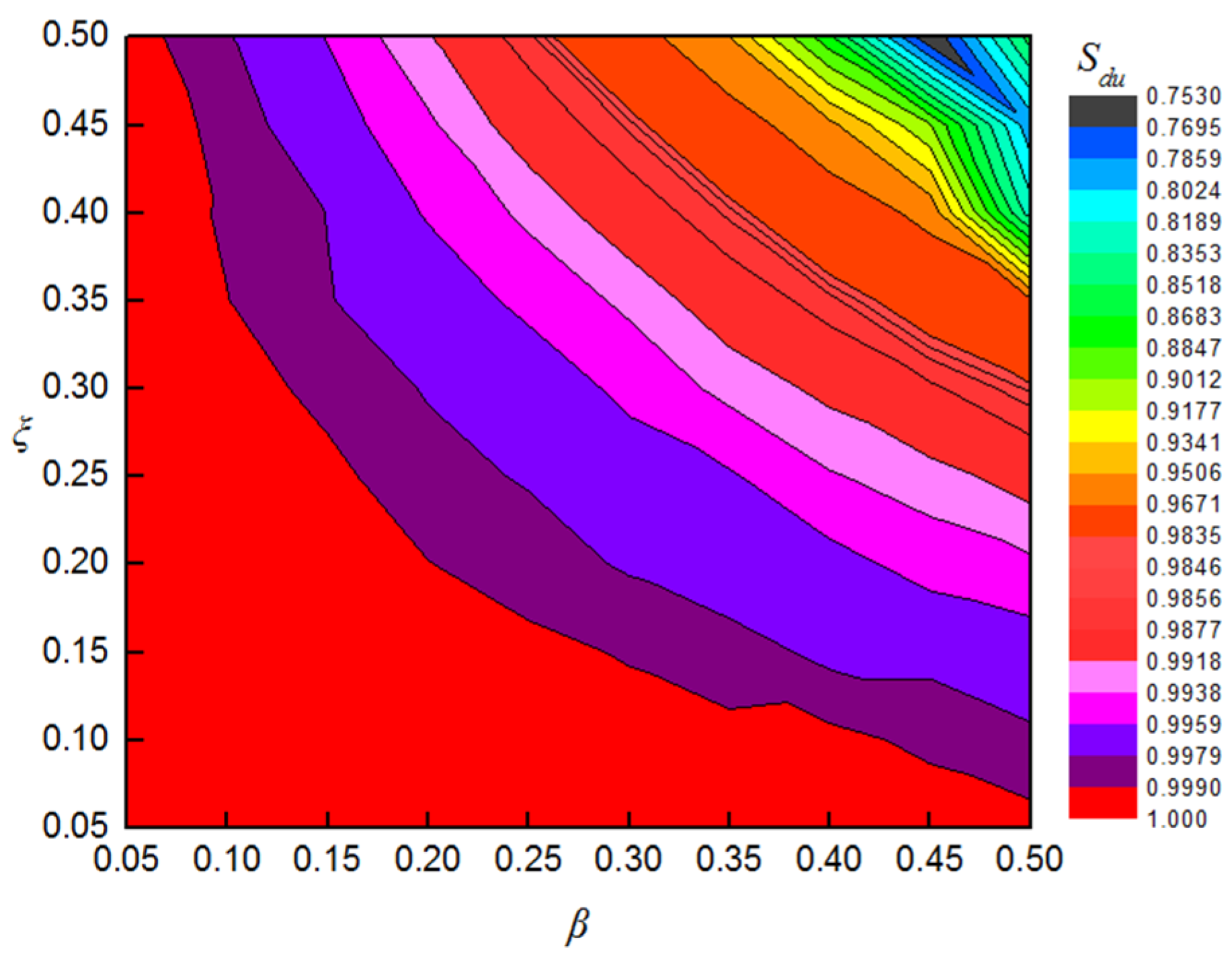
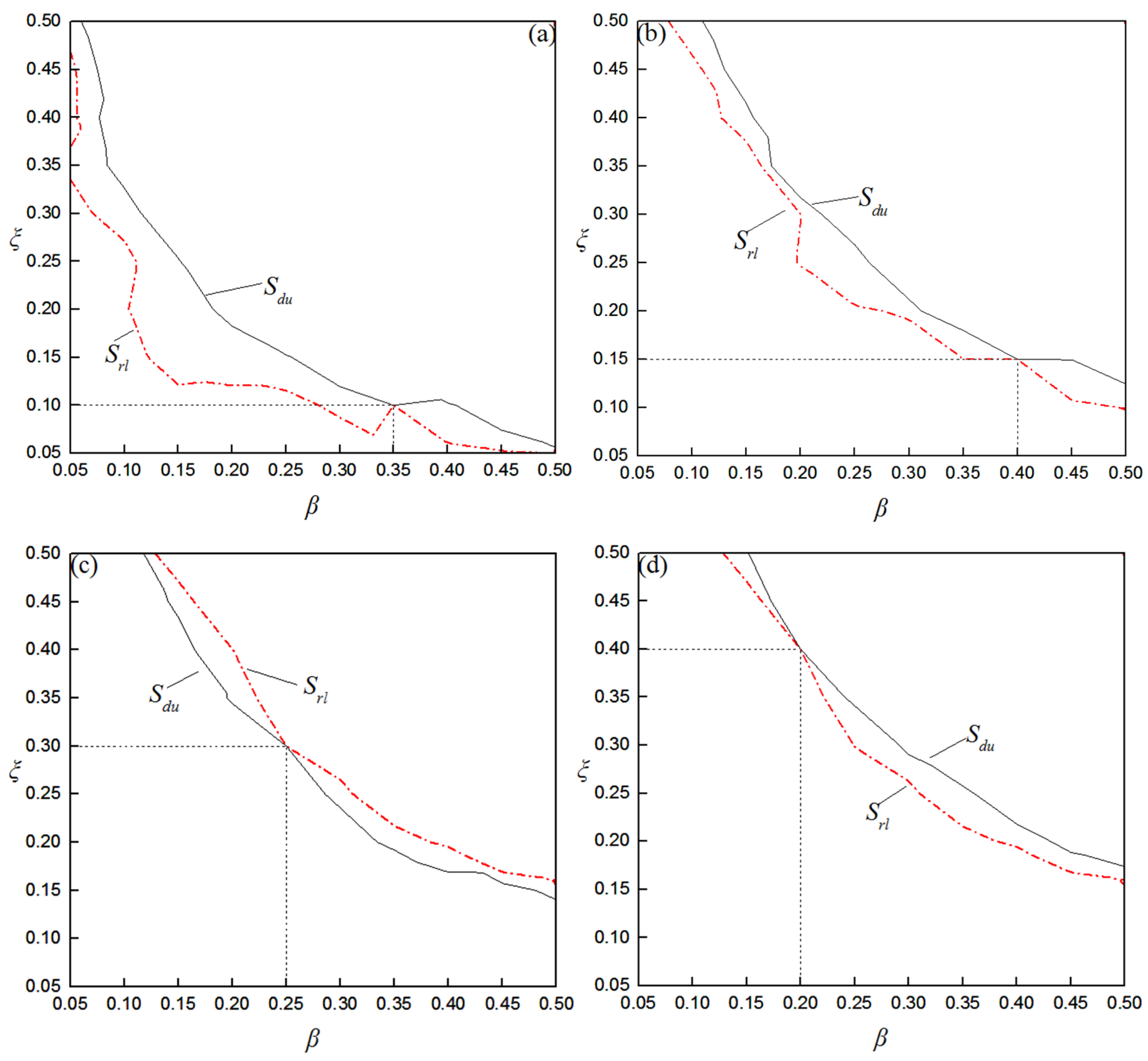

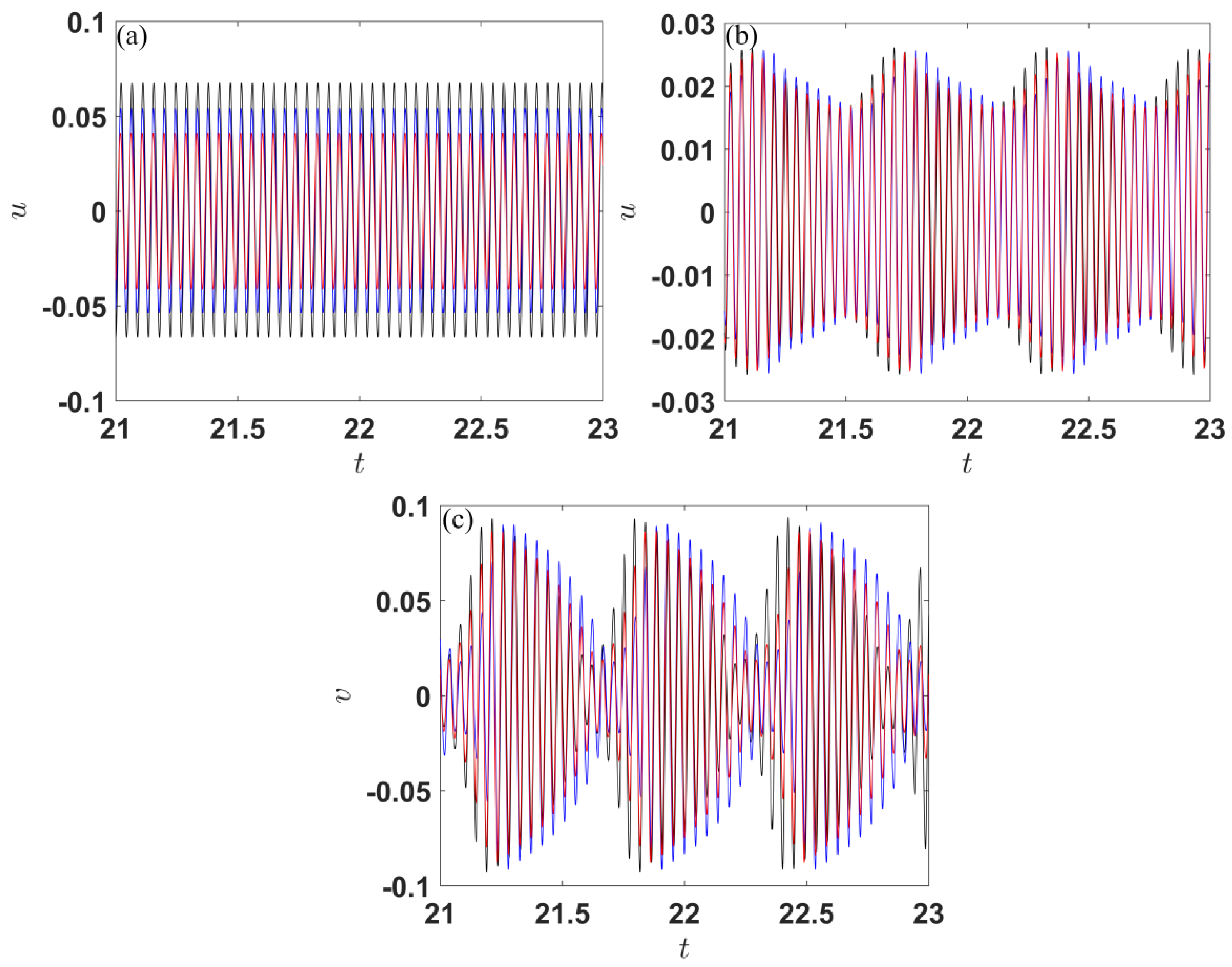
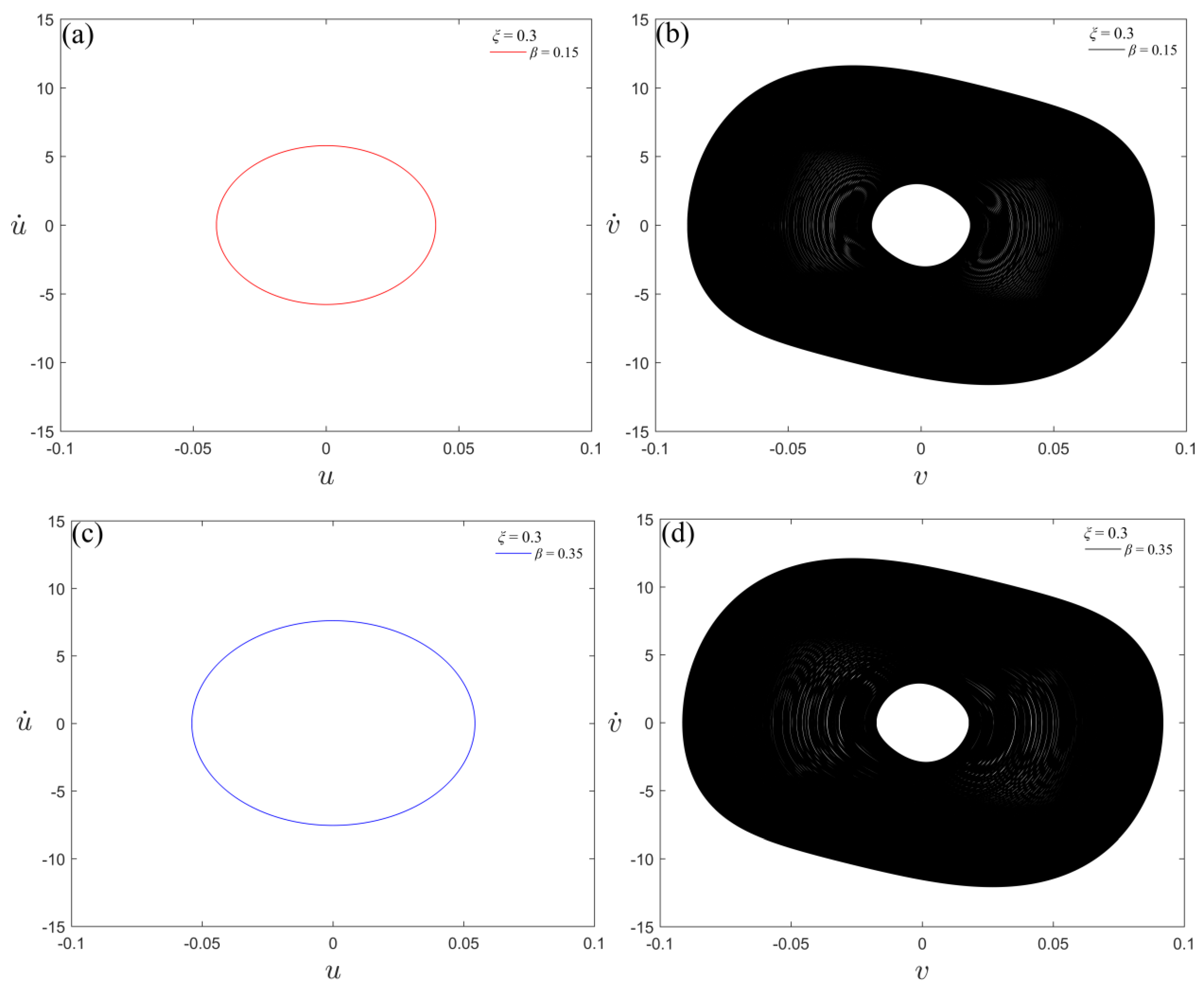
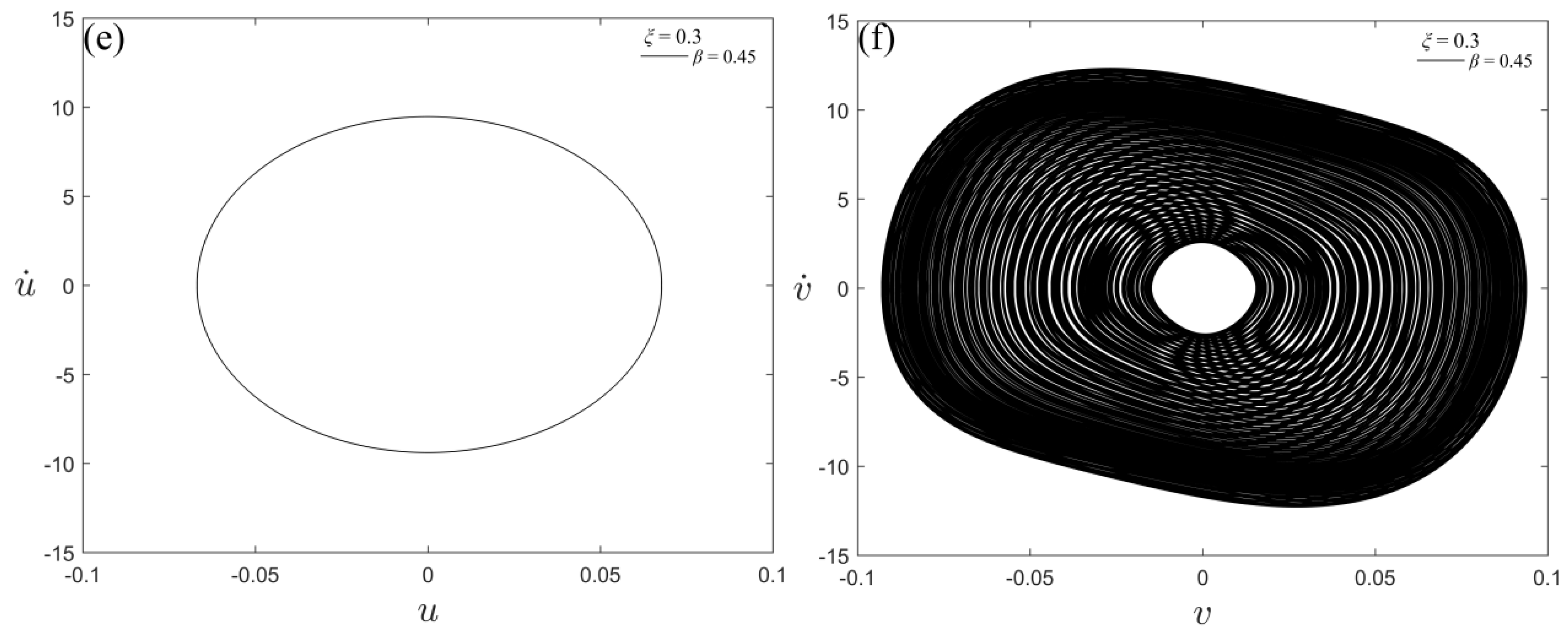


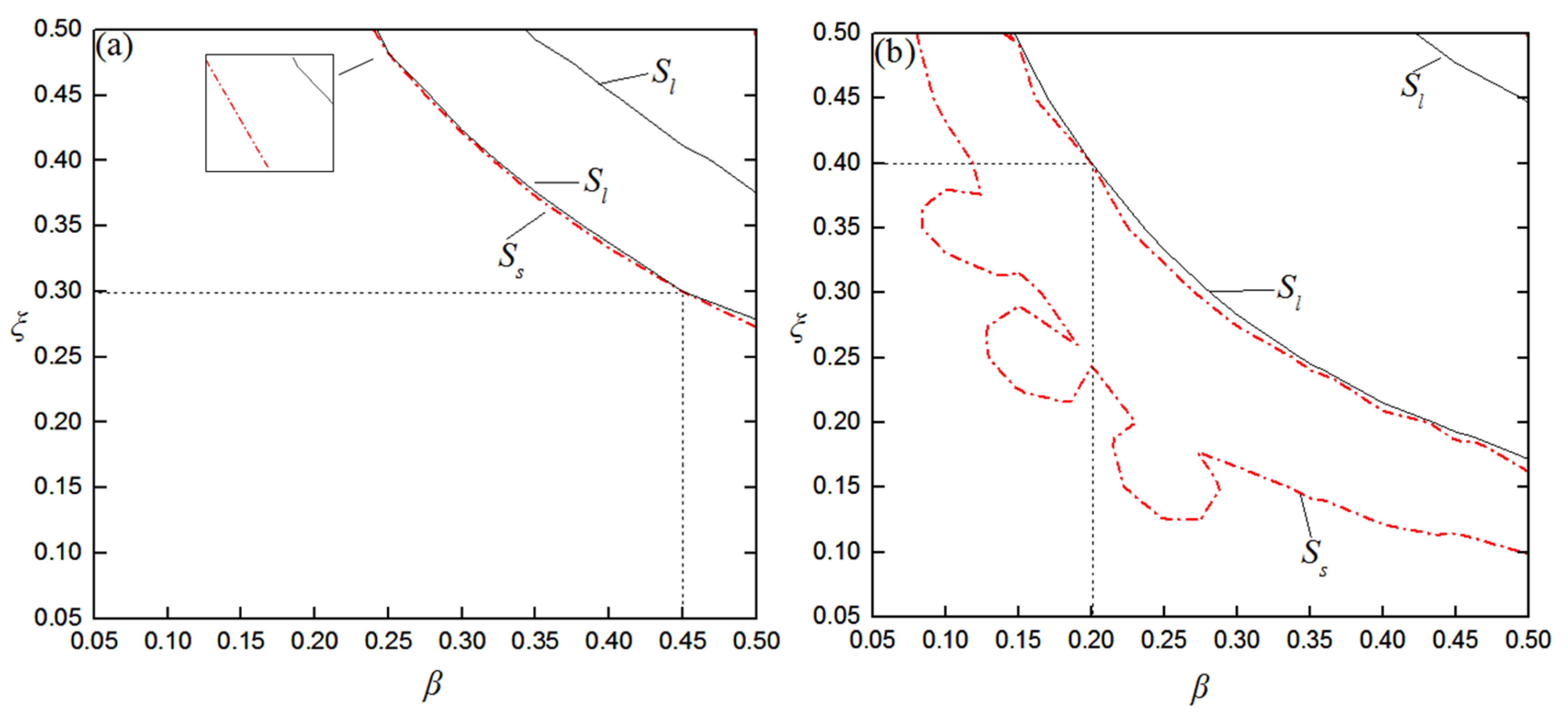
| β | ξ | Srl | Sdu |
|---|---|---|---|
| 0.35 | 0.1 | 1.003 | 0.99920 |
| 0.4 | 0.15 | 1.00138 | 0.99761 |
| 0.25 | 0.3 | 1.00358 | 0.99724 |
| 0.2 | 0.4 | 1.00354 | 0.99569 |
| β | ξ | Ss | Sl |
|---|---|---|---|
| 0.45 | 0.3 | 4383 | 138,387 |
| 0.2 | 0.4 | 6436 | 126,320 |
| 0.1 | 0.1 | 6173 | 117,276 |
| 0.4 | 0.4 | 2696 | 141,408 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, M.; Zhang, K.; Chen, J. Study on Crack Identification with Responses Modulated by Nonlinear Energy Sink. Appl. Sci. 2022, 12, 11398. https://doi.org/10.3390/app122211398
Sun M, Zhang K, Chen J. Study on Crack Identification with Responses Modulated by Nonlinear Energy Sink. Applied Sciences. 2022; 12(22):11398. https://doi.org/10.3390/app122211398
Chicago/Turabian StyleSun, Min, Kai Zhang, and Jianen Chen. 2022. "Study on Crack Identification with Responses Modulated by Nonlinear Energy Sink" Applied Sciences 12, no. 22: 11398. https://doi.org/10.3390/app122211398
APA StyleSun, M., Zhang, K., & Chen, J. (2022). Study on Crack Identification with Responses Modulated by Nonlinear Energy Sink. Applied Sciences, 12(22), 11398. https://doi.org/10.3390/app122211398





