Model Test on Effect of Ground Fissure on the Behavior of Oblique Two-Section Subway Tunnel
Abstract
:1. Introduction
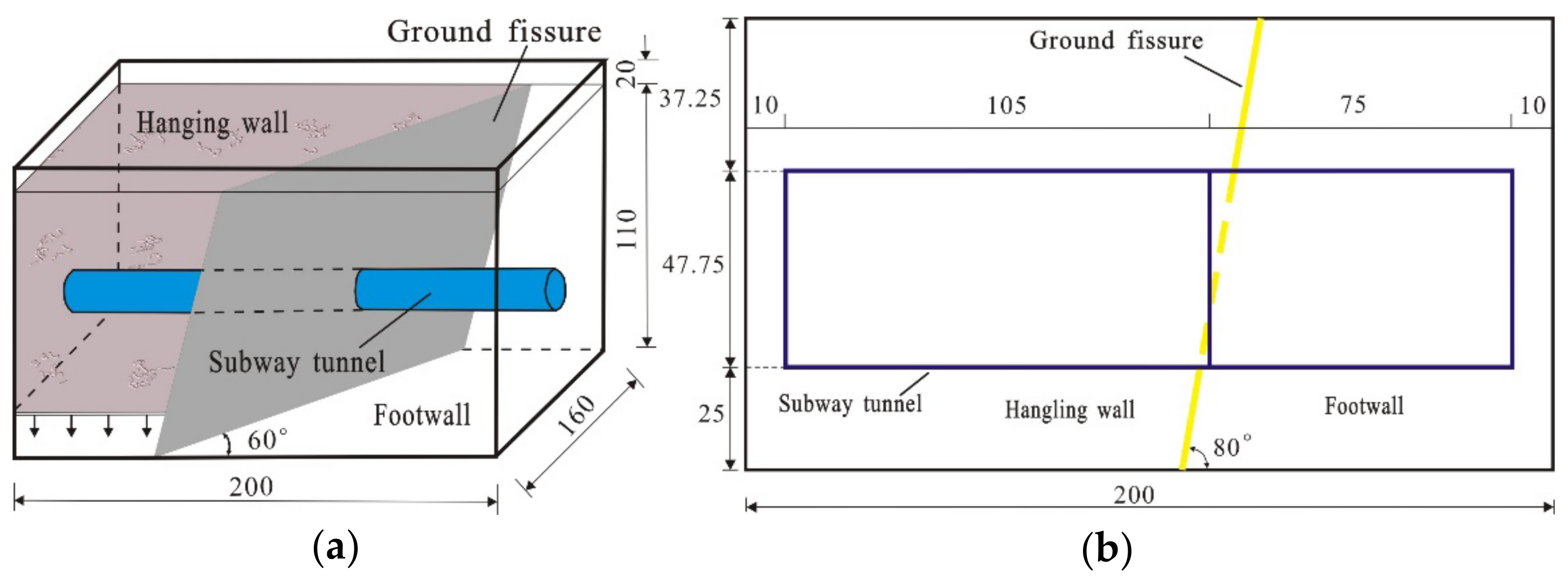
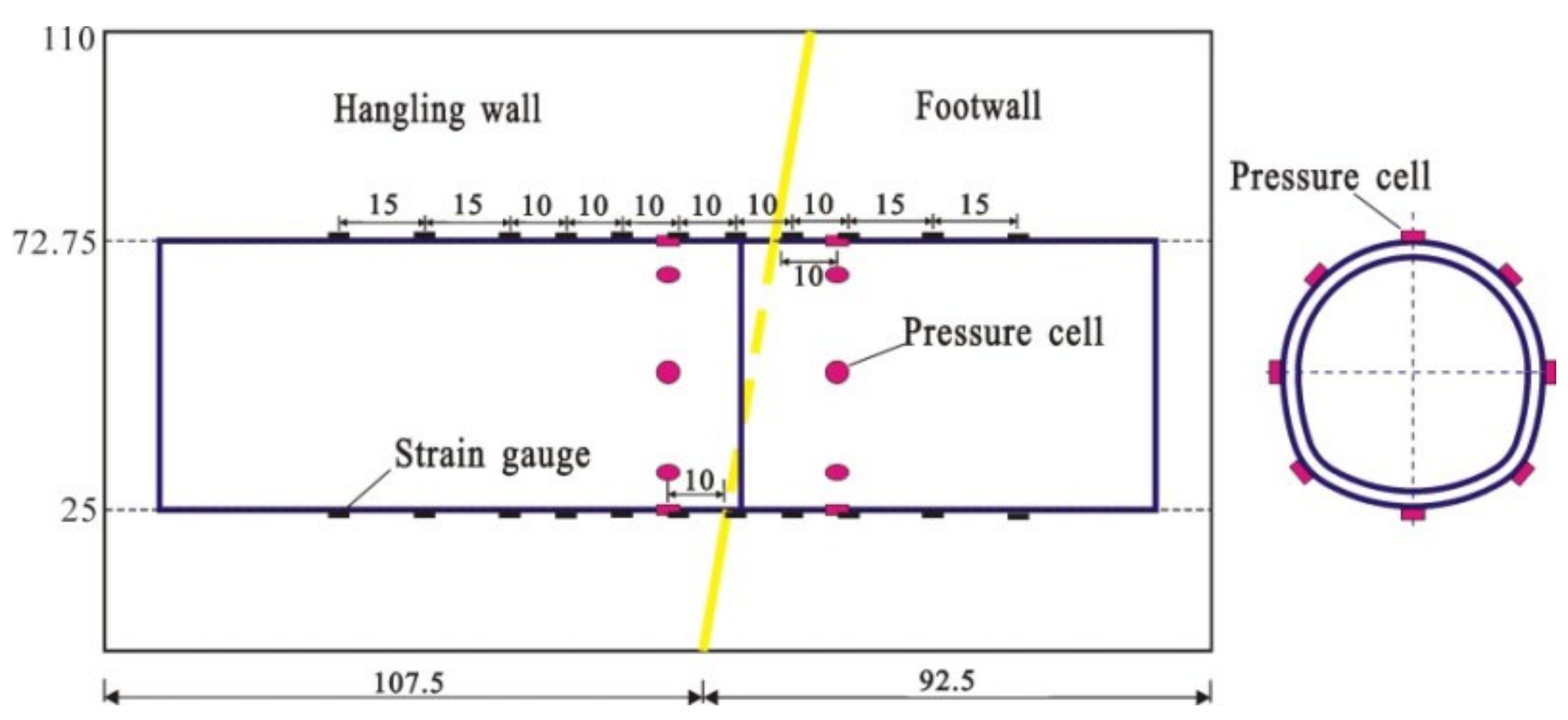
2. Experimental Design and Scheme
2.1. Test Principle
2.2. Design of Similarity Scale
2.3. Model Materials
2.3.1. Stratum
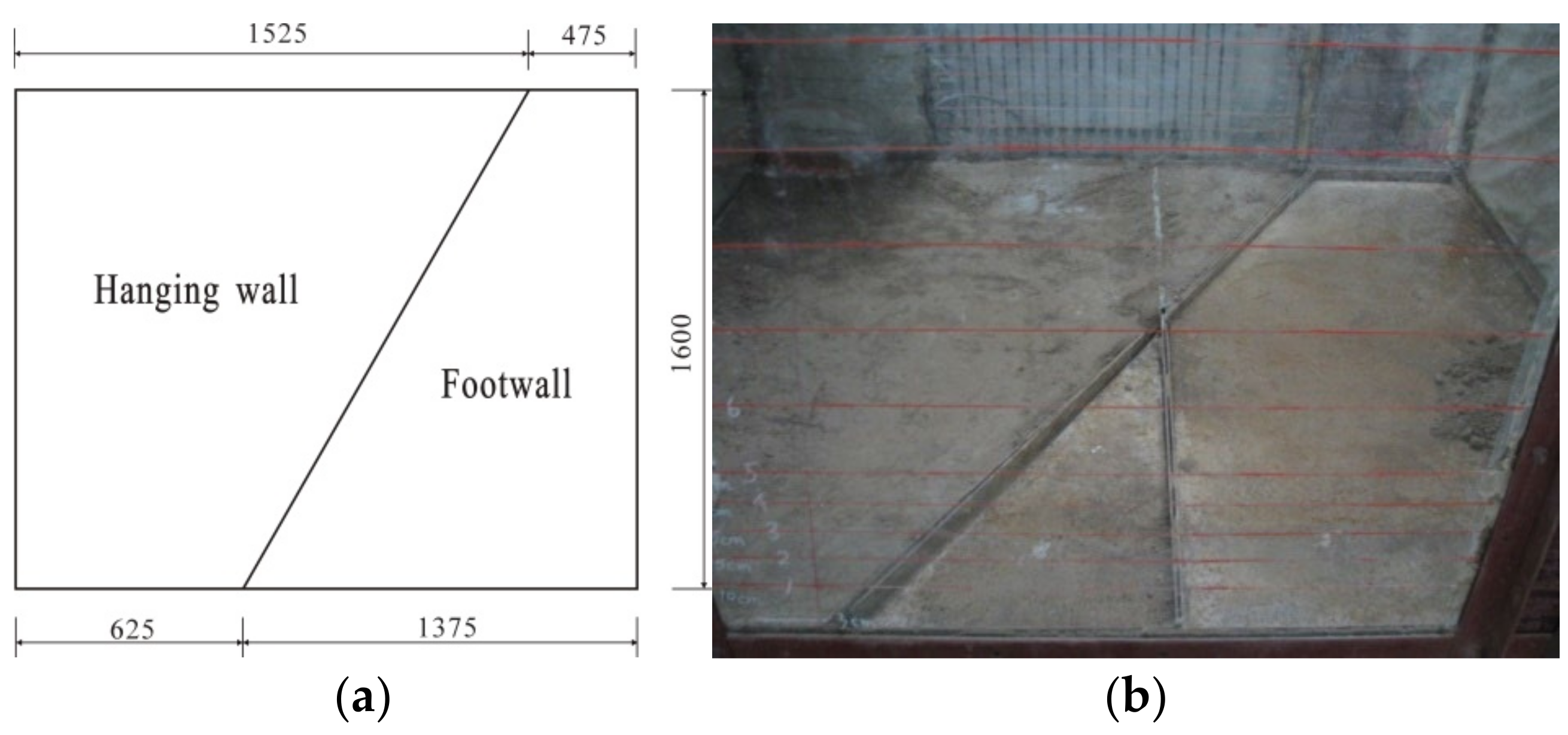
2.3.2. Ground Fissure
2.3.3. Tunnel Structure
2.4. Subway-Induced Vibration Load
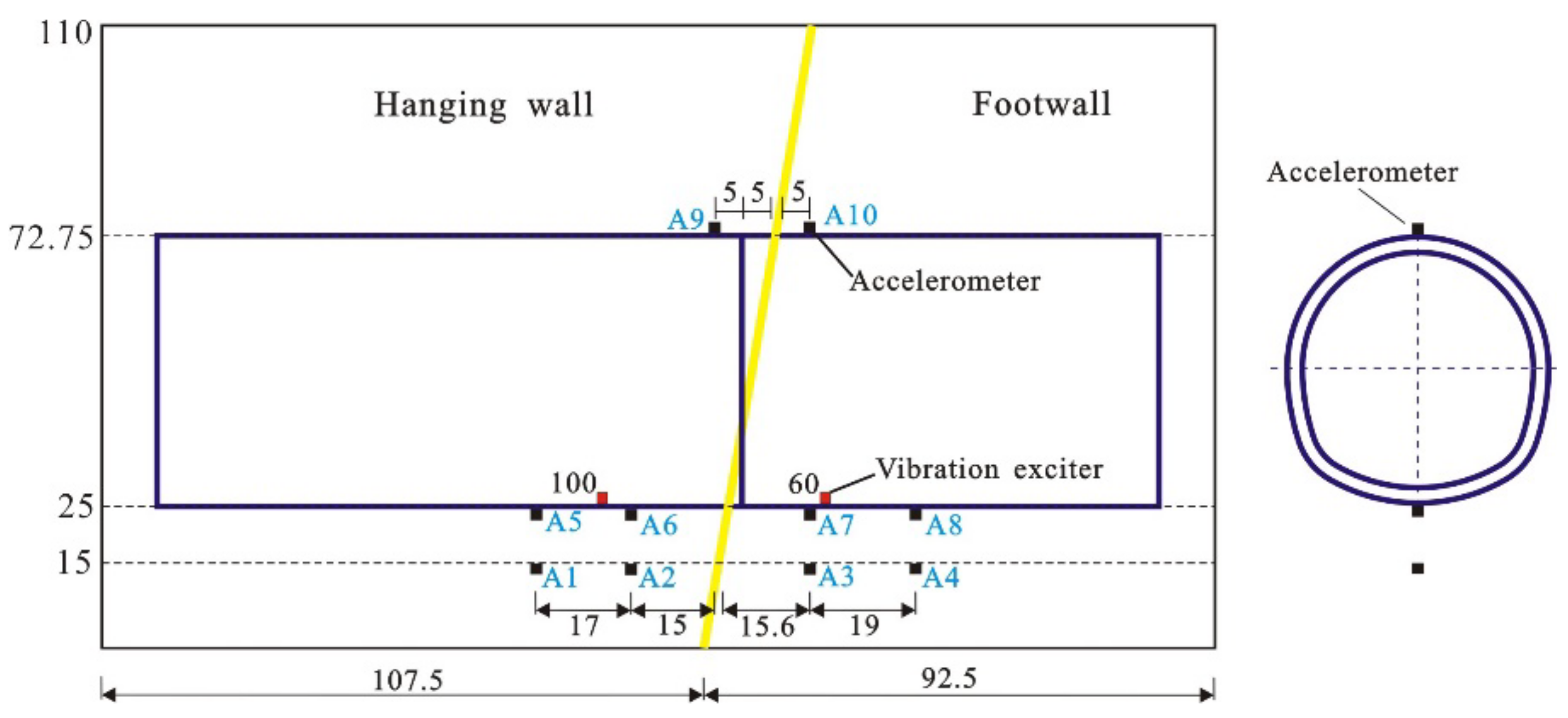
2.5. Measurement Equipment
2.5.1. Acceleration Measurements
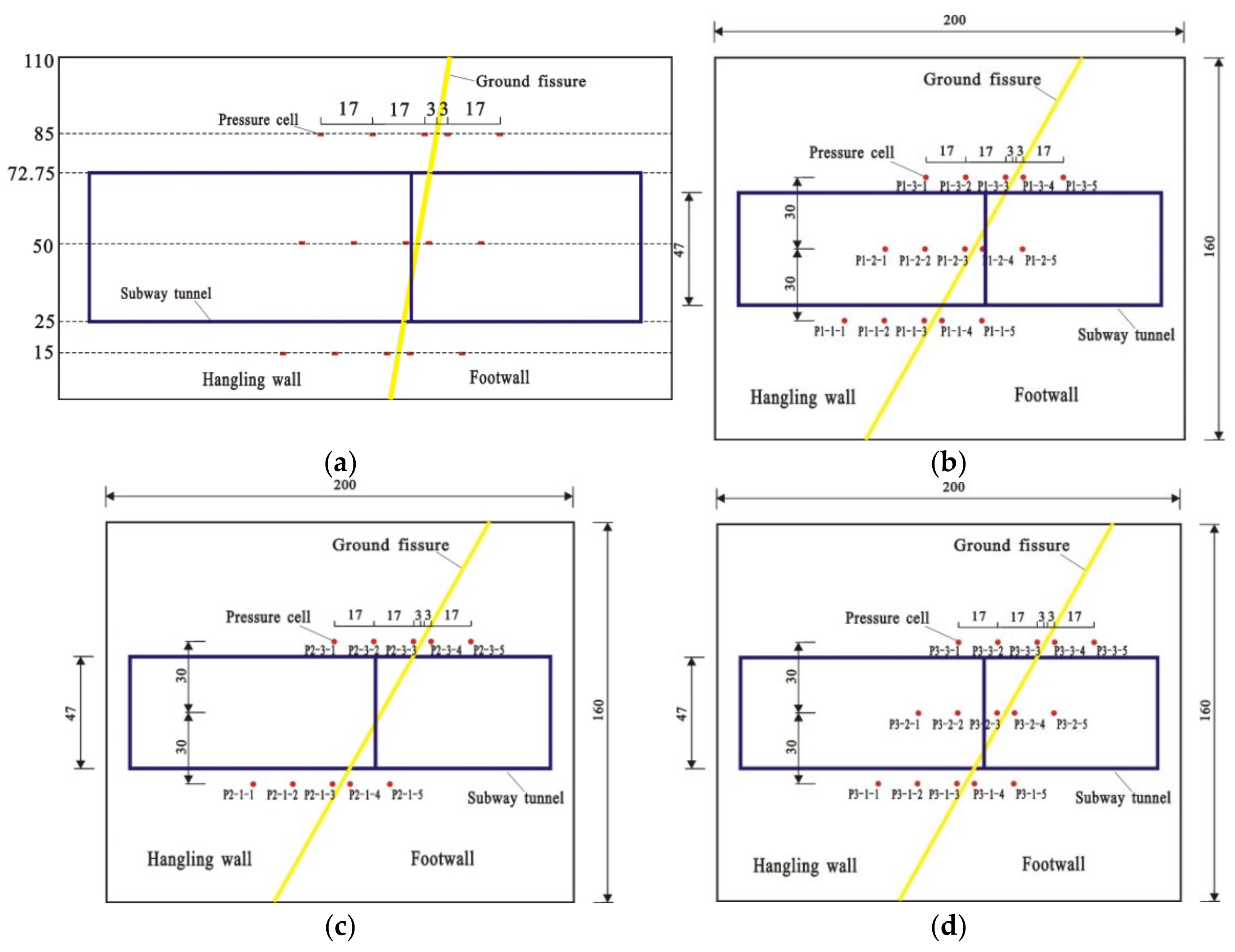
2.5.2. Earth Pressure in the Soil
2.5.3. Contact Pressure between the Tunnel and Soil Mass
2.5.4. Strain of the Tunnel
3. Results and Discussion
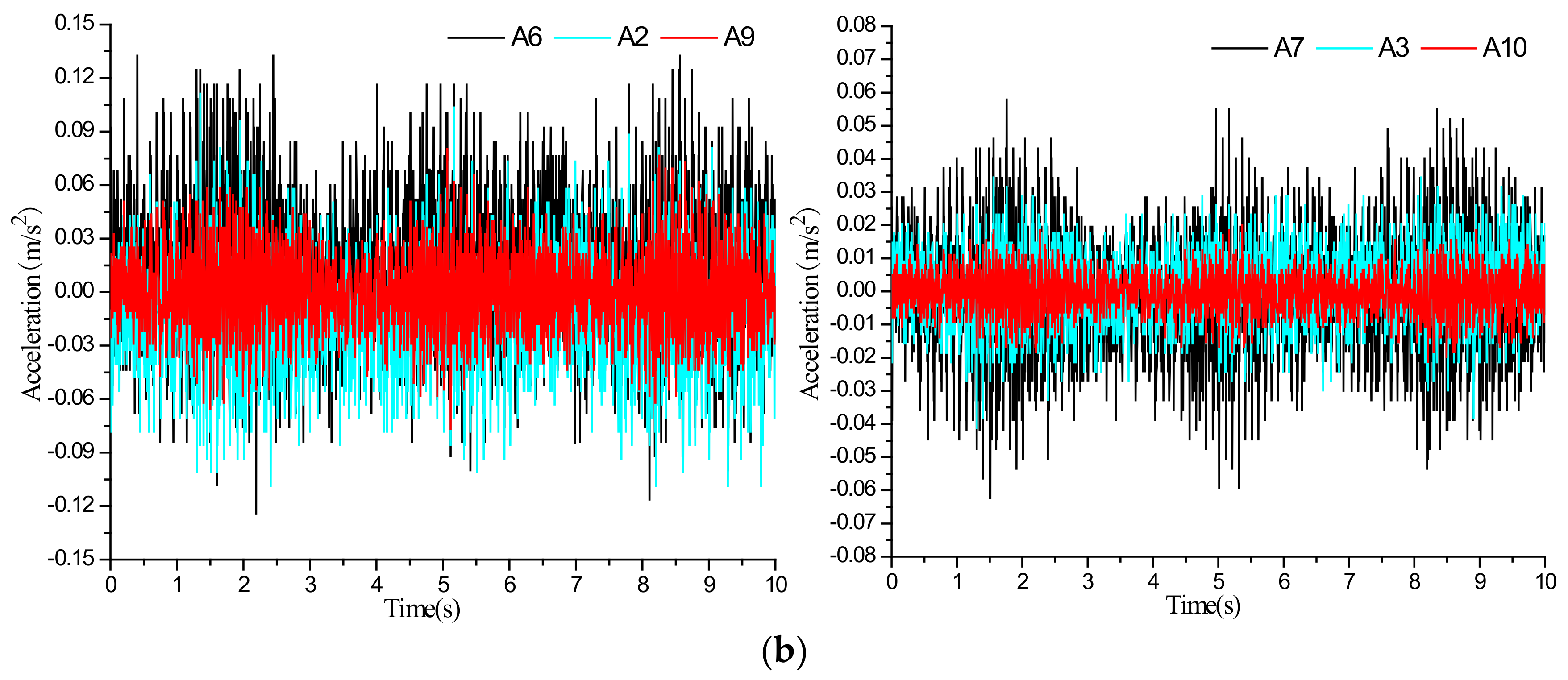
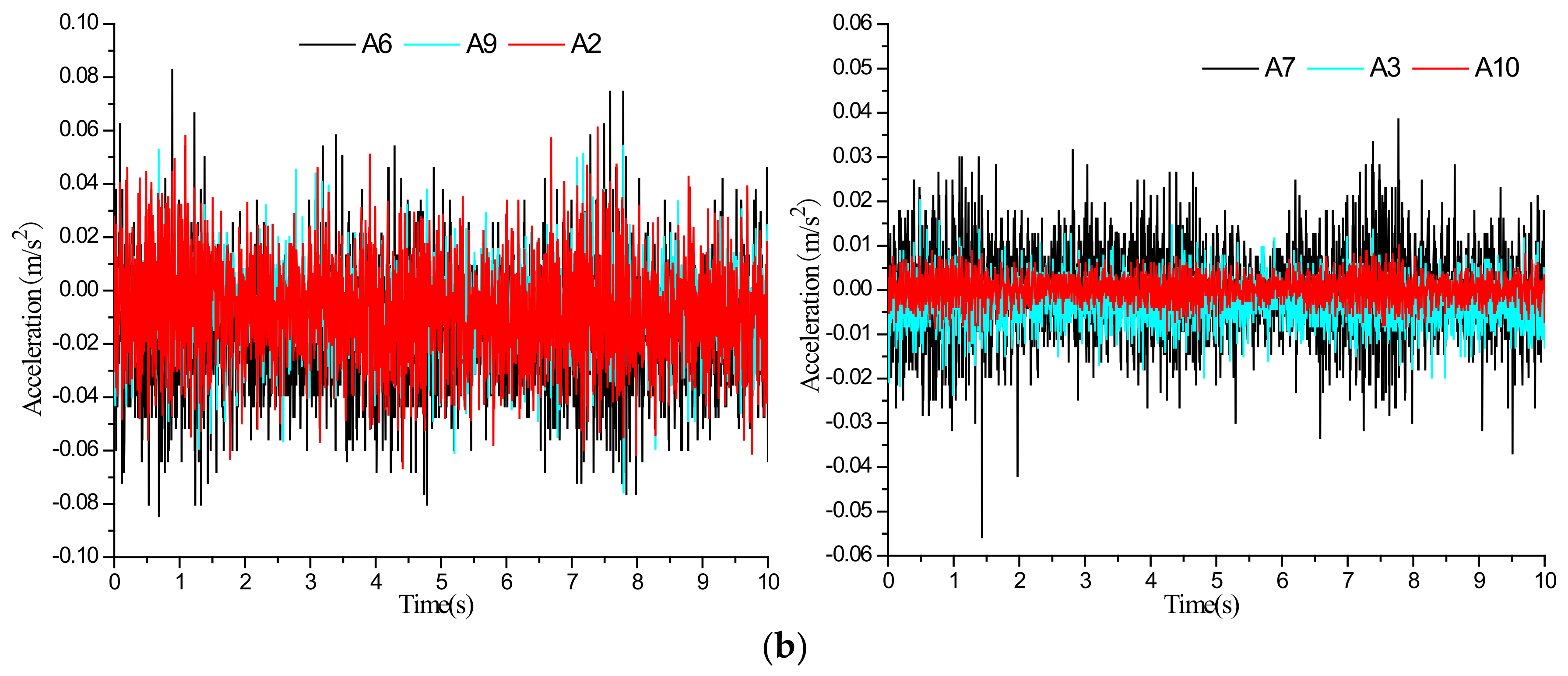
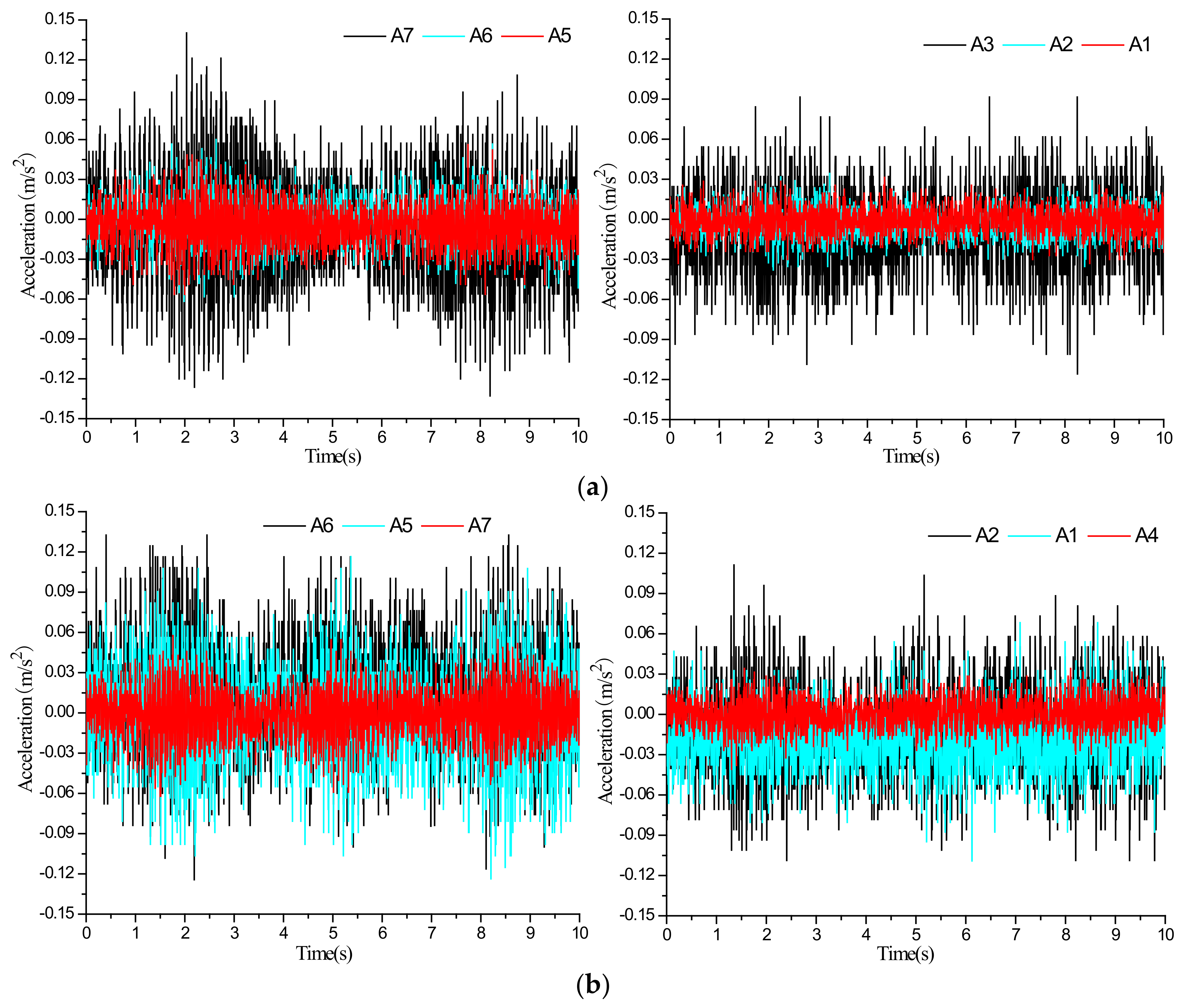
3.1. Acceleration Response of Soil
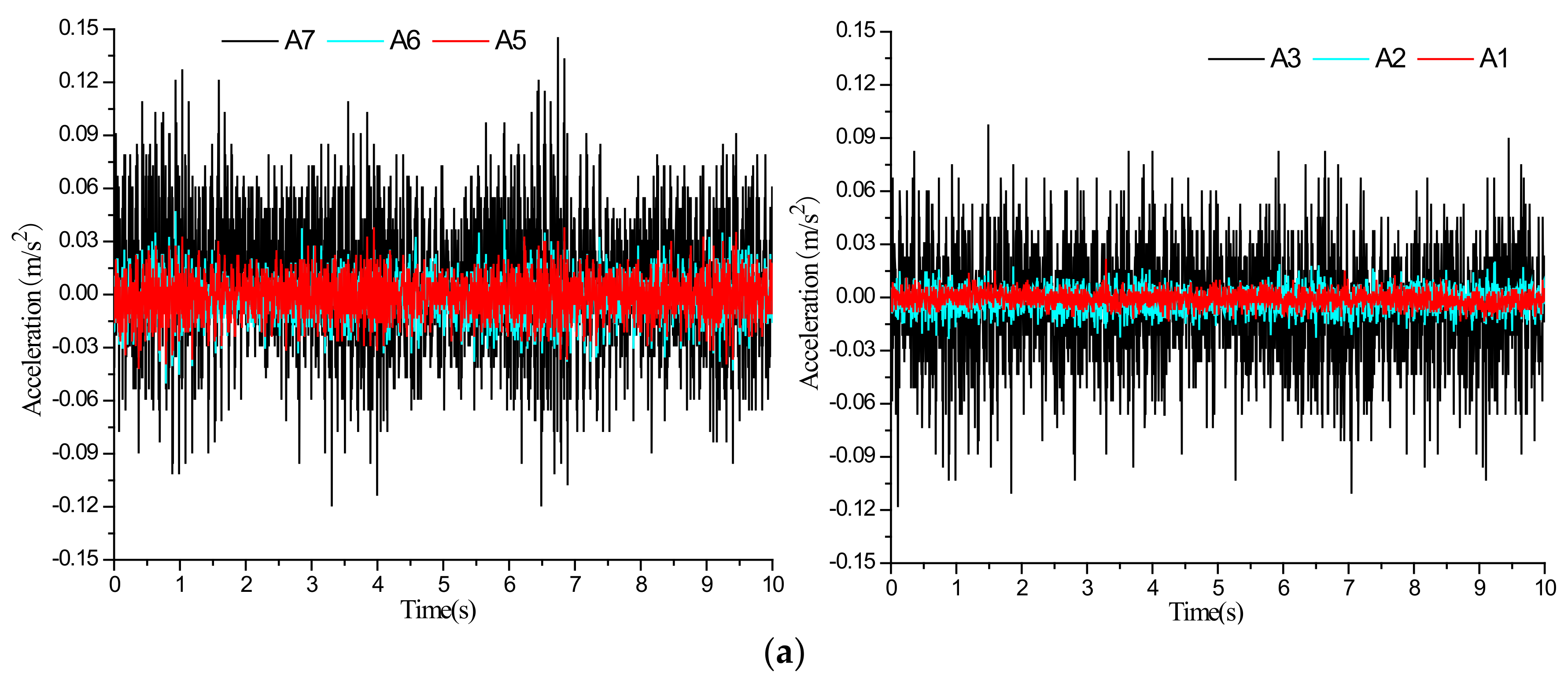
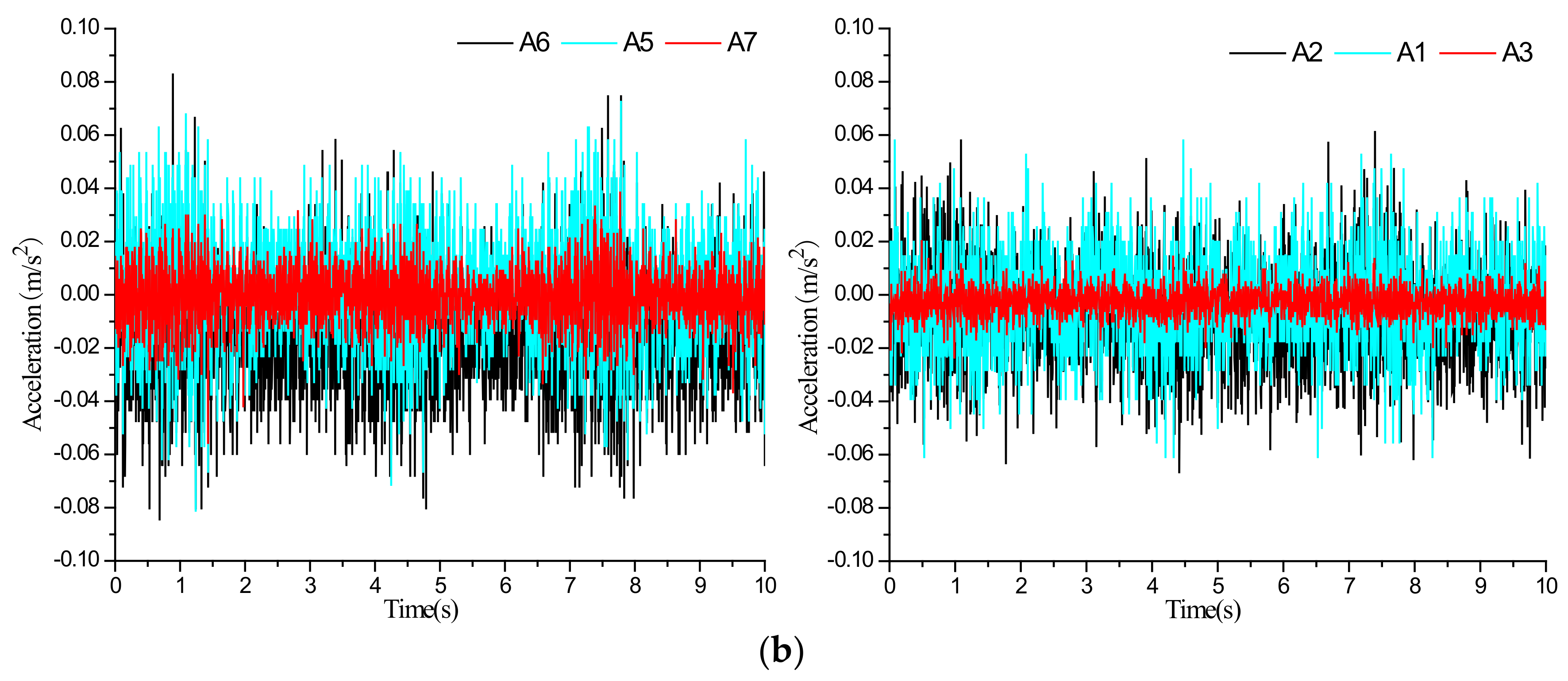
3.1.1. Dynamic Response at Different Depth
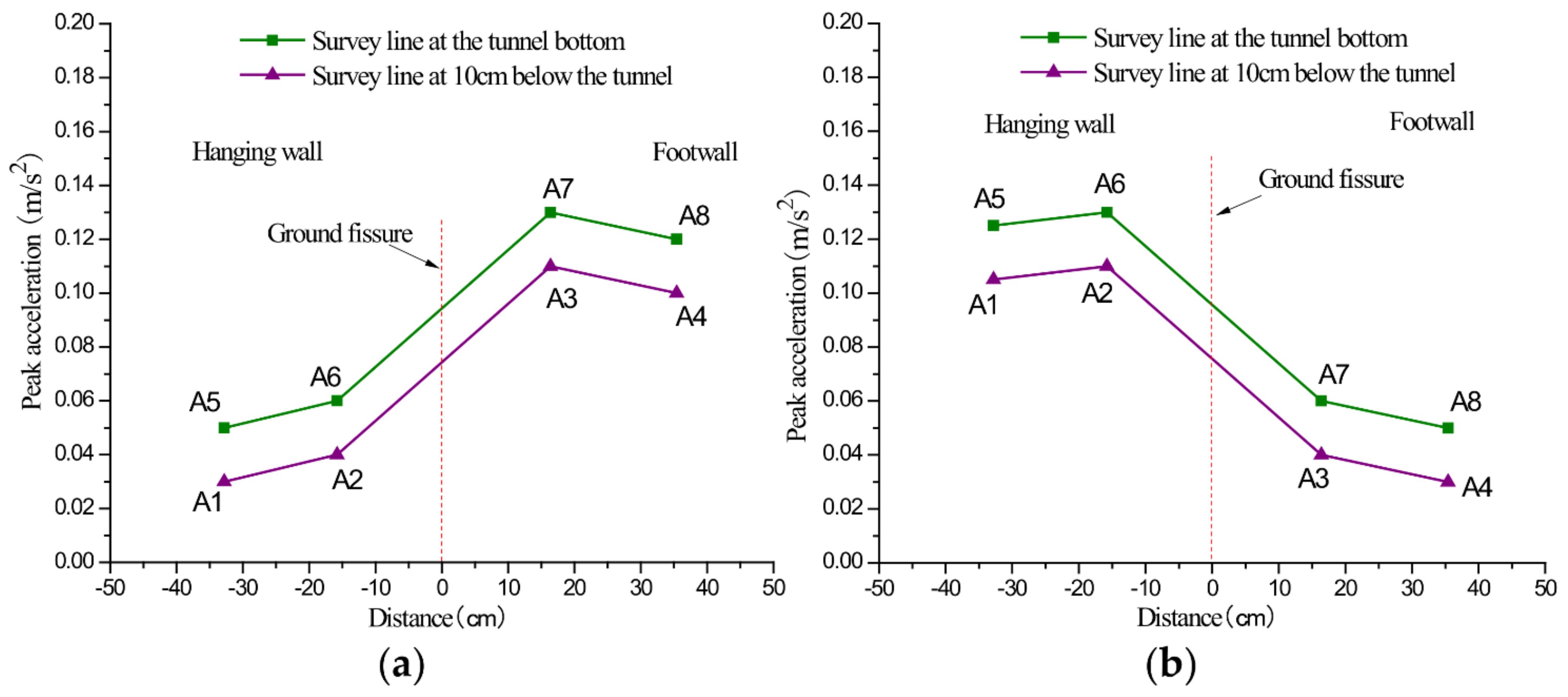
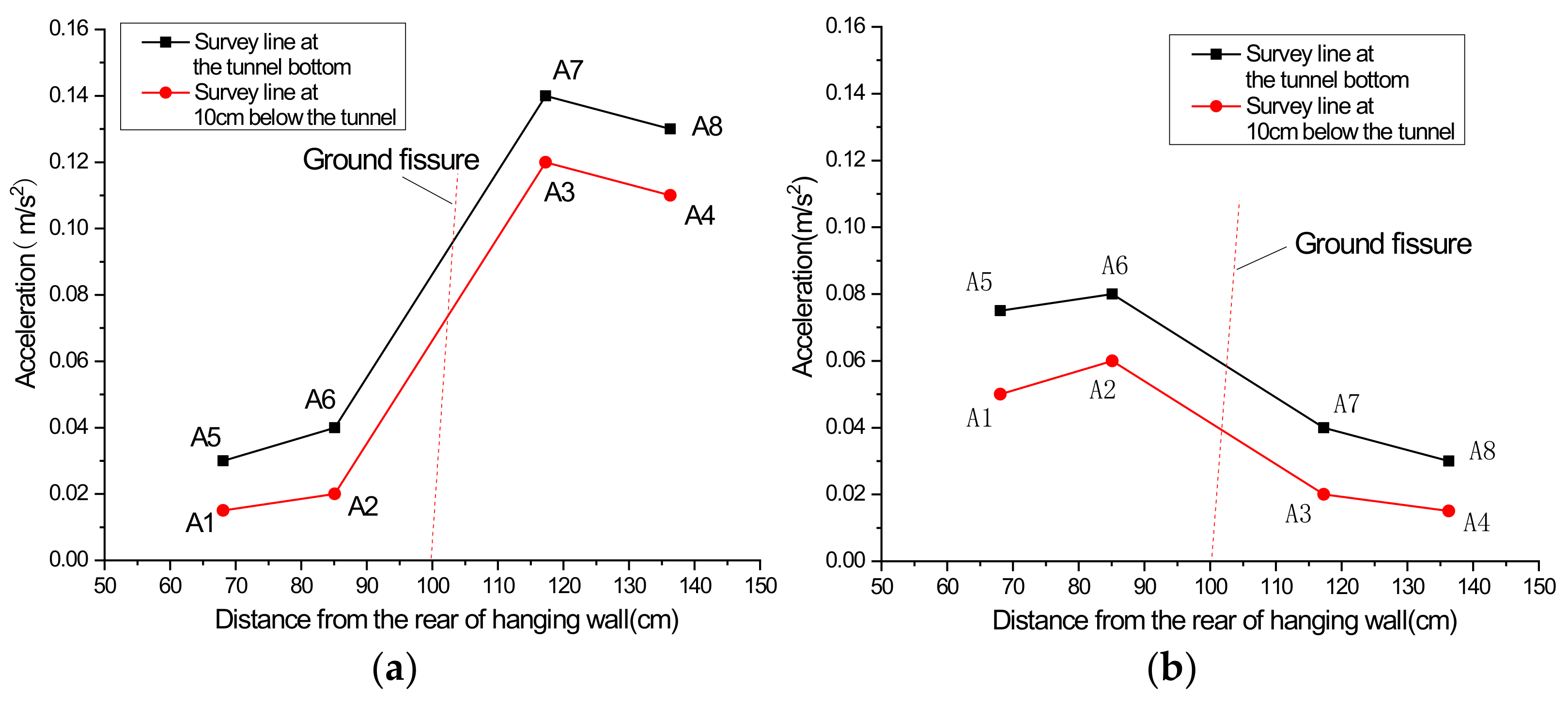
3.1.2. Dynamic Response Longitudinal along the Tunnel
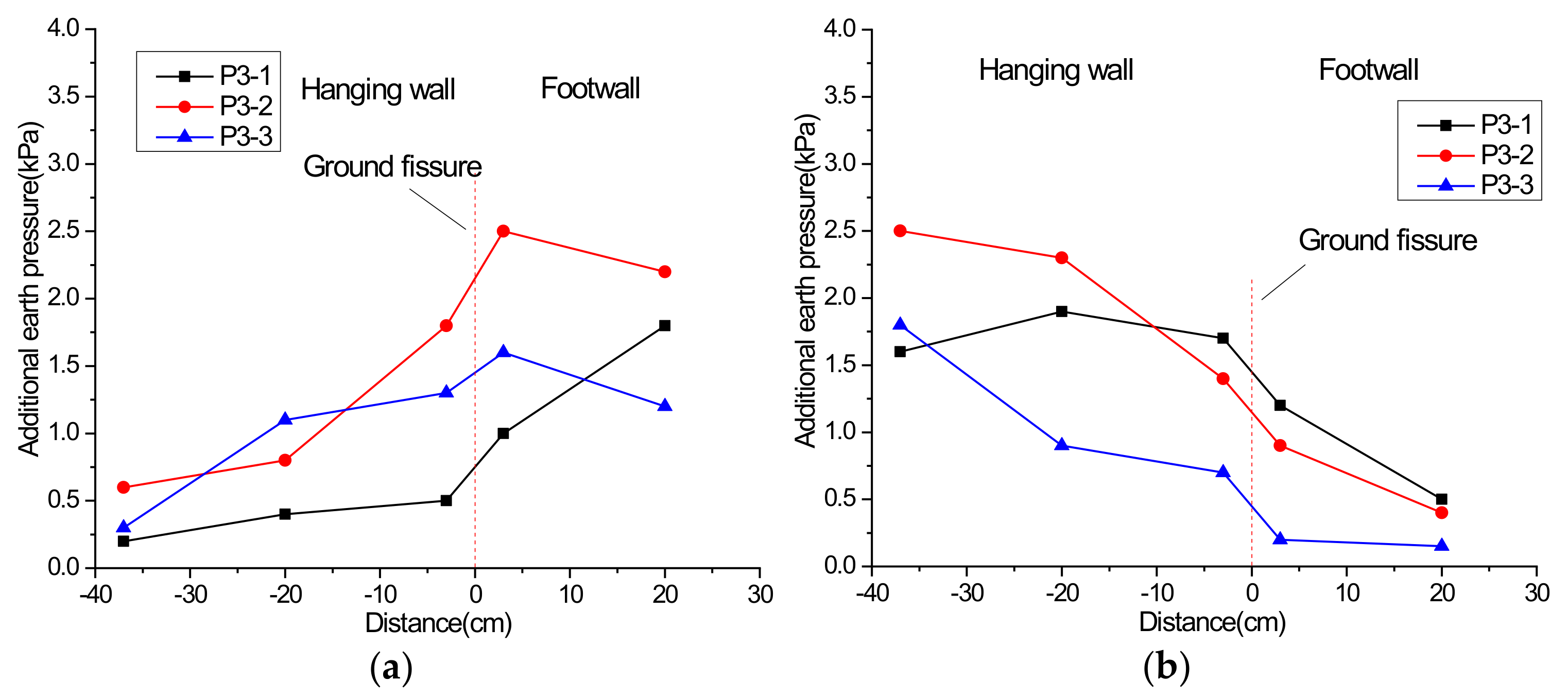
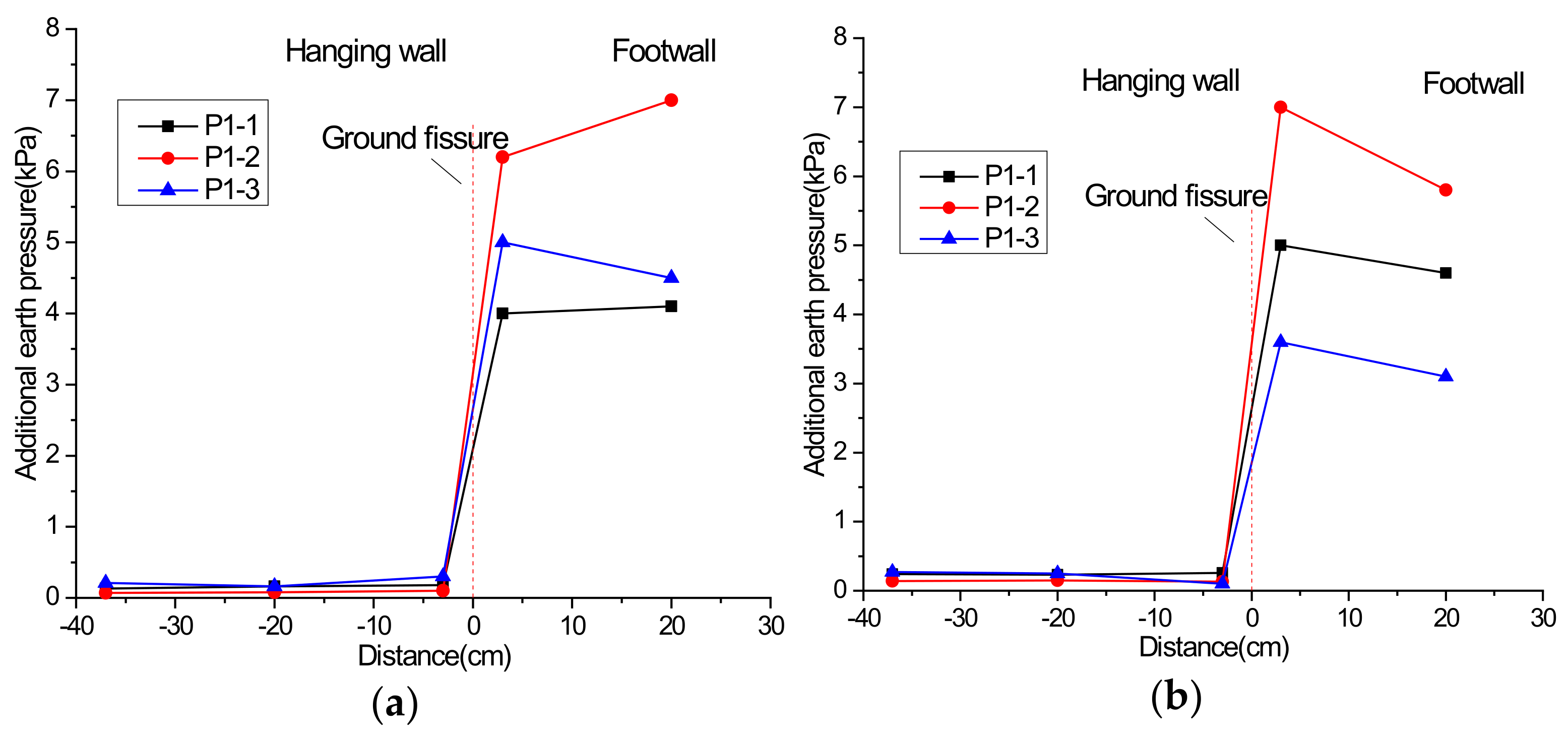
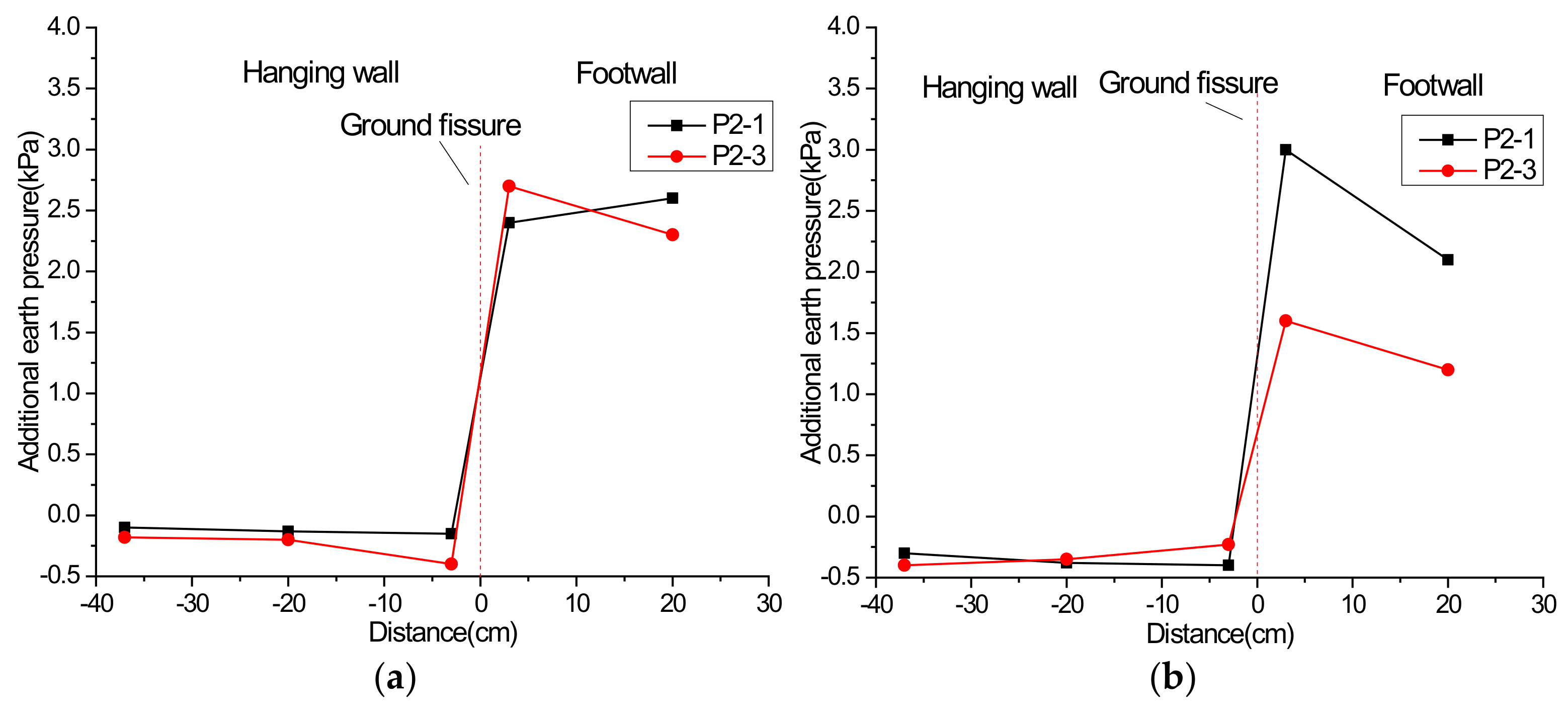
3.2. Dynamic Response of Earth Pressure
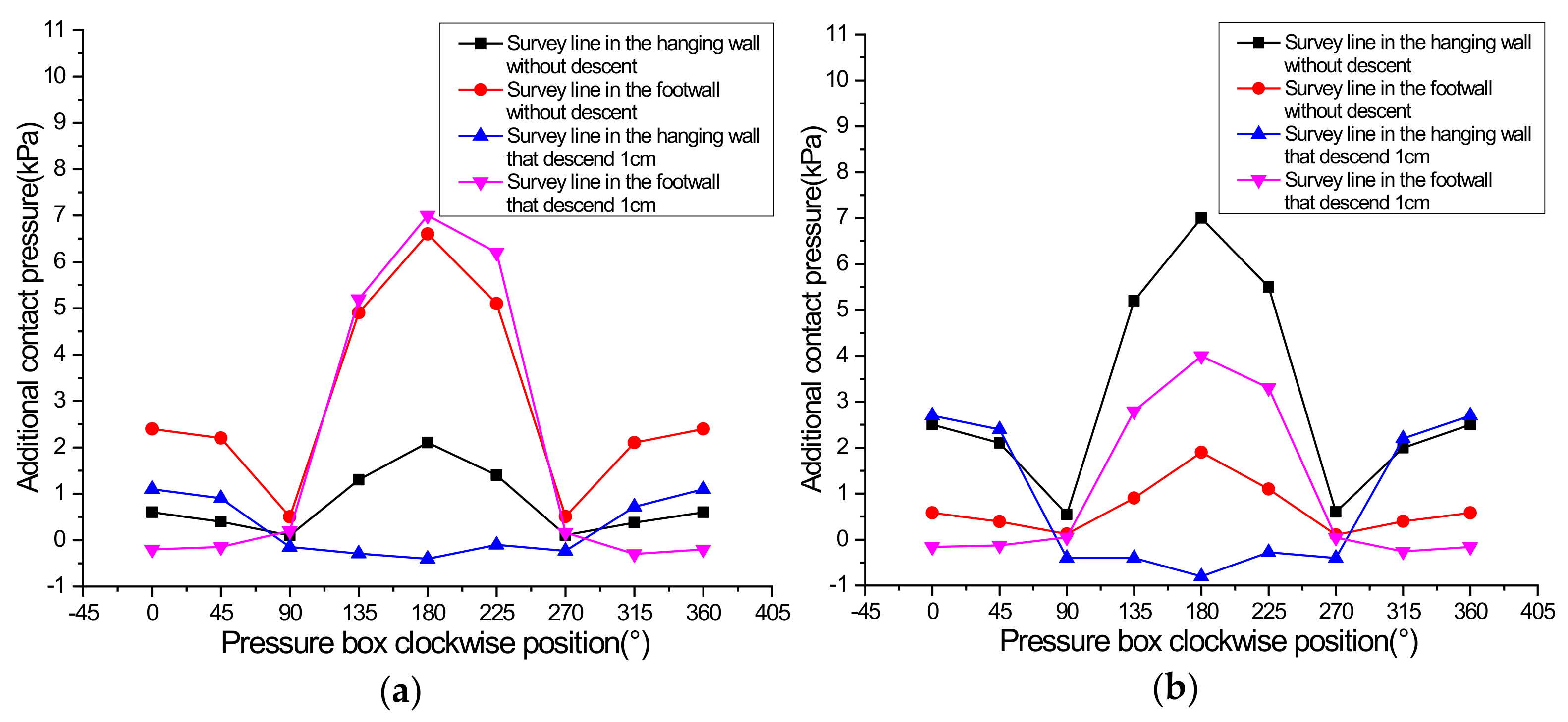
3.3. Dynamic Response of Tunnel Contact Pressure
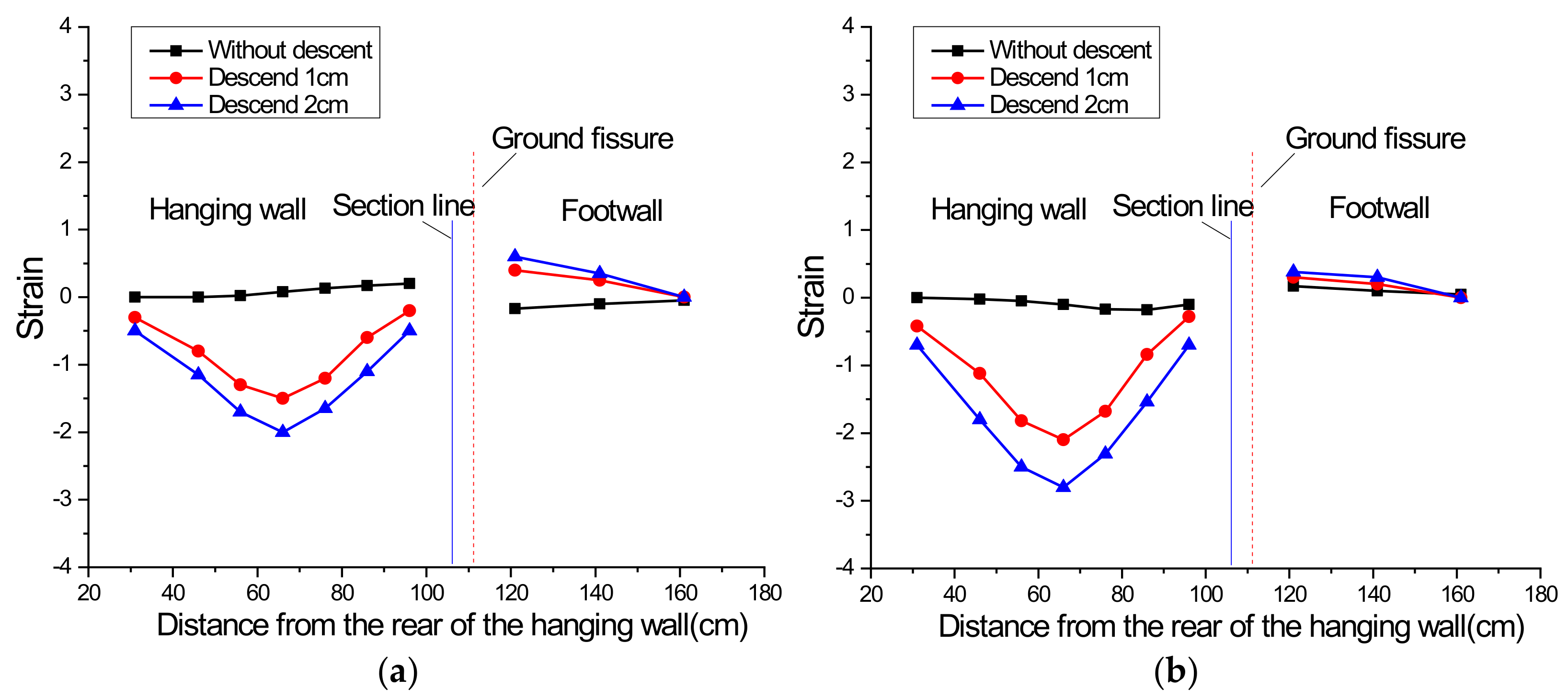
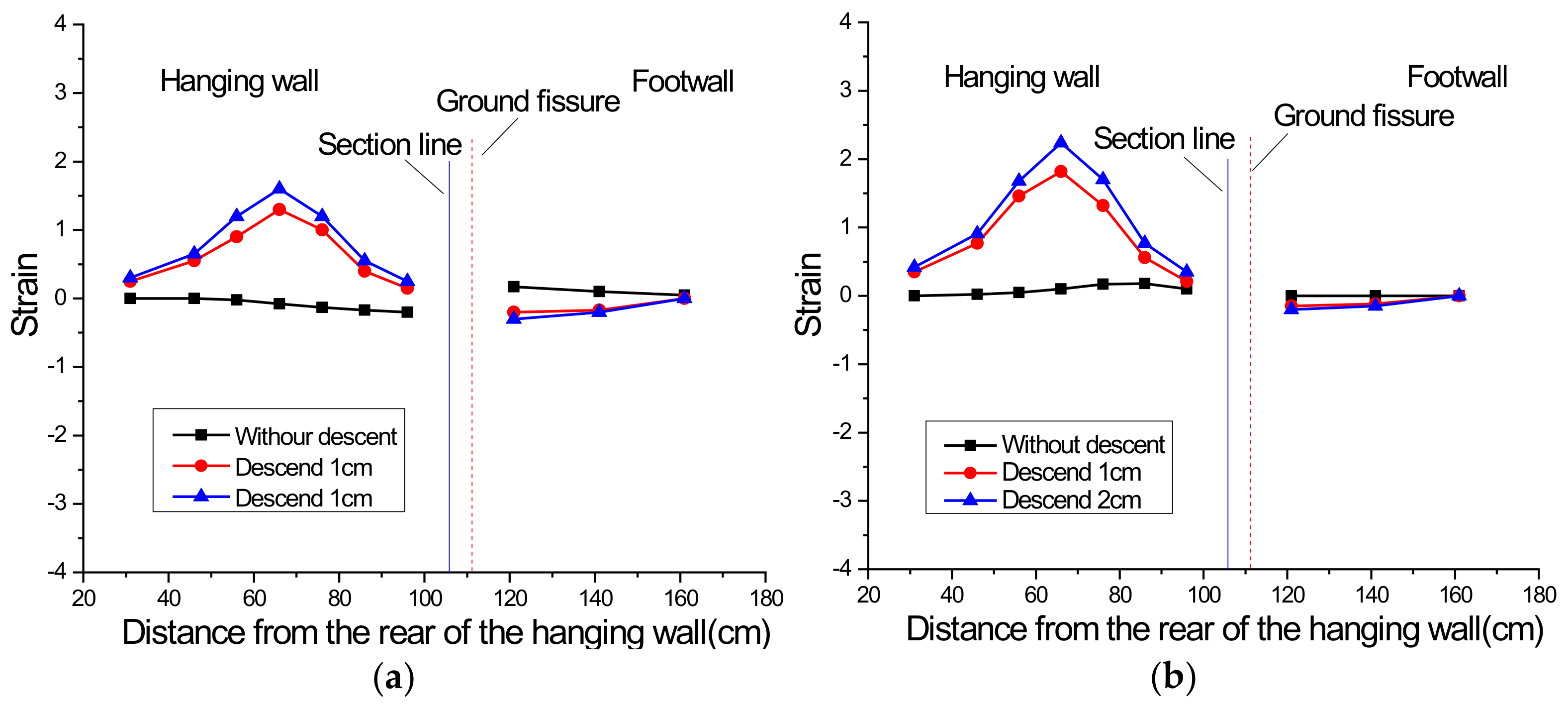
3.4. Dynamic Response of Tunnel Strain
4. Conclusions
- (1)
- The vibration-induced soil acceleration attenuates when crossing the ground fissure and the degree of attenuation has nothing to do with the propagation direction (from one side of the ground fissure to the other side). Nevertheless, for the scenario that the hanging wall of the ground fissure descends from the original place, a more significant attenuation is observed when the vibration propagates from the footwall to the hanging wall, while the enhancement phenomenon is observed with an opposite propagation direction;
- (2)
- The vibration near the ground fissure is stronger in the soil layer below the tunnel than that of the upper soil layer. The rapid attenuation of vibration intensity is identified when the vibration propagates from the lining to the lower and the upper stratum;
- (3)
- The additional contact pressure at the tunnel bottom is relatively larger than that at the tunnel crown when the hanging wall of the ground fissure does not move, and the minimum value is identified on both sides of the tunnel. A relatively larger additional contact pressure is also identified at the place on the same side as the vibration exciter. Additionally, when the hanging wall moves downward, an increase in additional contact pressure is observed at the tunnel crown in the hanging wall, while that at the tunnel bottom significantly decreases to a negative value. The additional contact pressure at the tunnel bottom in the footwall increases in such a condition, and that at the tunnel crown nearly decreases to zero. Notably, the additional contact pressure at the tunnel bottom decreases with the movement of vibration exciter from the footwall to the hanging wall, and the movement of the ground fissure has little effect on the additional contact pressure on both sides of the tunnel;
- (4)
- The excitation-induced additional strain at the crown and bottom of the tunnel are approximately zero with a condition of an inactive ground fissure. When the hanging wall of the ground fissure descends from the original place, negative and positive additional strain is identified at the crown and bottom of the tunnel in the hanging wall, respectively, implying a compression state. Meanwhile, positive and negative additional strain is observed at the crown and bottom of tunnel in the footwall, respectively, implying a tensile state.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pan, C.S.; Xie, Z.G. Measurement and analysis of vibrations caused by passing trains in subway running tunnel. China Civ. Eng. J. 1990, 23, 21–28. (In Chinese) [Google Scholar]
- Zhang, Y.E.; Bai, B.H. The method of identifying train vibration load acting on subway tunnel structure. J. Vib. Shock 2000, 19, 68–70. [Google Scholar]
- Liu, W.F.; Liu, W.N.; Degrande, G. Experimental validation of a numerical model for prediction of metro train-induced ground-surface vibration. J. Vib. Eng. 2010, 23, 373–379. (In Chinese) [Google Scholar]
- Mo, H.H.; Deng, F.H.; Wang, J.H. Analysis of dynamic responses of shield tunnel during metro operation. Chin. J. Rock Mech. Eng. 2006, 25, 3507–3512. [Google Scholar]
- Tang, Y.Q.; Wang, Y.L.; Huang, Y.; Huang, Y.; Wang, Y.; Zhou, N. Dynamic Strength and Dynamic Stress-strain Relation of Silt Soil under Traffic Loading. J. Tongji Univ. 2004, 32, 701–704. (In Chinese) [Google Scholar]
- Bian, X.; Hong, Z.S.; Ding, J.W. Evaluating the effect of soil structure on the ground response during shield tunnelling in Shanghai soft clay. Tunn. Undergr. Space Technol. 2016, 58, 120–132. [Google Scholar] [CrossRef]
- Xiong, L.X.; Li, T.B.; Liu, Y. Numerical Simulation of Seismic Response at the Entrance of the Unsymmetrical Loading Tunnel. J. Geomech. 2007, 13, 255–260. (In Chinese) [Google Scholar]
- Li, J.S.; Peng, H.; Cui, W.; Ma, X.M.; Yang, S.X.; Liao, J.S. Results of Rock Stress Measurements and Engineering Application of a Railway Tunnel in Northwestern Yunnan. J. Geomech. 2005, 11, 135–144. (In Chinese) [Google Scholar]
- Hussein, D.H. A power flow method for evaluating vibration from underground railways. J. Sound Vib. 2006, 293, 667–679. [Google Scholar] [CrossRef]
- Forrest, J.A.; Hunt, H.E.M. A three dimentional tunnel model for calculation of train induced ground vibration. J. Sound Vib. 2006, 294, 678–705. [Google Scholar] [CrossRef]
- Bian, X.; Zeng, L.L.; Li, X.Z.; Shi, X.S.; Zhou, S.M.; Li, F.Q. Fabric changes induced by super-absorbent polymer on cement and lime stabilized excavated clayey soil. J. Rock Mech. Geotech. Eng. 2021, 13, 1124–1135. [Google Scholar] [CrossRef]
- Nejati, H.R.; Ahmadi, M.; Hashemolhossein, H. Numerical analysis of ground surface vibration induced by under-ground train movement. Tunn. Undergr. Space Technol. 2012, 29, 1–9. [Google Scholar] [CrossRef]
- Bian, X.; Zeng, L.L.; Li, X.Z.; Hong, J.T. Deformation modulus of reconstituted and naturally sedimented clays. Eng. Geol. 2021, 295, 106450. [Google Scholar] [CrossRef]
- Yuan, L.Q.; Men, Y.M.; Liu, H.J.; Liu, L. A Numerical Simulation of Interactions between Ground Fissures and Xi’an Metro Tunnel. J. Catastrophology 2013, 28, 11–13. (In Chinese) [Google Scholar]
- Yuan, L.Q.; Men, Y.M.; Liu, N.N. The Dynamic Response Simulation Analysis of U-shaped Metro Tunnel Orthogonal with the Ground Fissure. J. Disaster Prev. Mitig. Eng. 2015, 35, 354–358. (In Chinese) [Google Scholar]
- Yang, M.; Men, Y.M.; Yuan, L.Q.; Yang, L.W. Numerical Analysis of Subway Vibration Responses for Different Tunnel Types in Ground Fissure Areas. J. Disaster Prev. Mitig. Eng. 2016, 36, 188–195. (In Chinese) [Google Scholar]
- Yang, M.; Men, Y.M.; Cao, R. Numerical Analysis of Stress in Soil due to Subway Moving Loads in Ground Fissure Area. Chin. J. Undergr. Space Eng. 2016, 12, 1545–1552. (In Chinese) [Google Scholar]
- Yang, L.; Yang, M.; Men, Y. Model Teston Dynamic Interaction among Ground Fissure, Tunnel, and Surrounding Rock. China Earthq. Eng. J. 2019, 41, 710–716. (In Chinese) [Google Scholar]
- Yang, J.J. Similarity Theory and Construction Model Experiment; Wuhan University of Technology Press: Wuhan, China, 2005. (In Chinese) [Google Scholar]
- Bian, X.; Zeng, L.L.; Ji, F.; Xie, M. Plasticity role in strength behaviour of cement-phosphogypsum stabilized soils. J. Rock Mech. Geotech. Eng. 2022, 14, 1343–1670. [Google Scholar] [CrossRef]
- Zhao, S.K. The Study on the Micro-structure Distortion Mechanics of Soft Clay under the Subway-Included Loading. Ph.D. Thesis, Tongji University, Shanghai, China, 2006. (In Chinese). [Google Scholar]
- Bian, X.; Zhang, W.; Li, X.Z.; Shi, X.S.; Deng, Y.F.; Peng, J. Changes in strength, hydraulic conductivity and microstructure of superabsorbent polymer stabilized soil subjected to wetting-drying cycles. Acta Geotech. 2022, 1–12. [Google Scholar] [CrossRef]
- Engineering Design and Research Institute of Chang’an University. Detailed Investigation Report on Ground Cracks along Xi’an Urban Rapid Rail Transit Line 2; Engineering Design and Research Institute of Chang’an University: Xi’an, China, 2007. (In Chinese) [Google Scholar]









| Parameter | Scaling Factor | ||
|---|---|---|---|
| Tunnel Structure | Soil Mass | ||
| Geometry | Length l | 20 | |
| Area A | 400 | ||
| Distance u | 20 | ||
| Elastic modulus E | 1.5 | ||
| Deformation modulus | 20 | ||
| Material | Strain ε | 1 | 1 |
| Stress σ | 1.5 | 20 | |
| Poisson’s ratio μ | 1 | 1 | |
| Density ρ | 1 | 1 | |
| Cohesion C | 20 | ||
| Load | Concentrated force F | 600 | |
| Surface load q | 1.5 | ||
| Mass m | 8000 | ||
| Dynamic | Time t | 16.9 | |
| Frequency ω | 0.06 | ||
| Velocity v | 1.2 | ||
| Acceleration a | 0.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Ma, T.; Yan, J.-K.; Wang, Z.-H. Model Test on Effect of Ground Fissure on the Behavior of Oblique Two-Section Subway Tunnel. Appl. Sci. 2022, 12, 10472. https://doi.org/10.3390/app122010472
Liu L, Ma T, Yan J-K, Wang Z-H. Model Test on Effect of Ground Fissure on the Behavior of Oblique Two-Section Subway Tunnel. Applied Sciences. 2022; 12(20):10472. https://doi.org/10.3390/app122010472
Chicago/Turabian StyleLiu, Lei, Tao Ma, Jin-Kai Yan, and Zhi-Hui Wang. 2022. "Model Test on Effect of Ground Fissure on the Behavior of Oblique Two-Section Subway Tunnel" Applied Sciences 12, no. 20: 10472. https://doi.org/10.3390/app122010472
APA StyleLiu, L., Ma, T., Yan, J.-K., & Wang, Z.-H. (2022). Model Test on Effect of Ground Fissure on the Behavior of Oblique Two-Section Subway Tunnel. Applied Sciences, 12(20), 10472. https://doi.org/10.3390/app122010472





