Asymmetric Constrained Control of a Cervical Orthotic Device Based on Barrier Sliding Modes
Abstract
1. Introduction
1.1. Preliminaries
1.2. Contributions
1.3. Manuscript Organization
2. Mathematical Preliminaries
3. Problem Statement
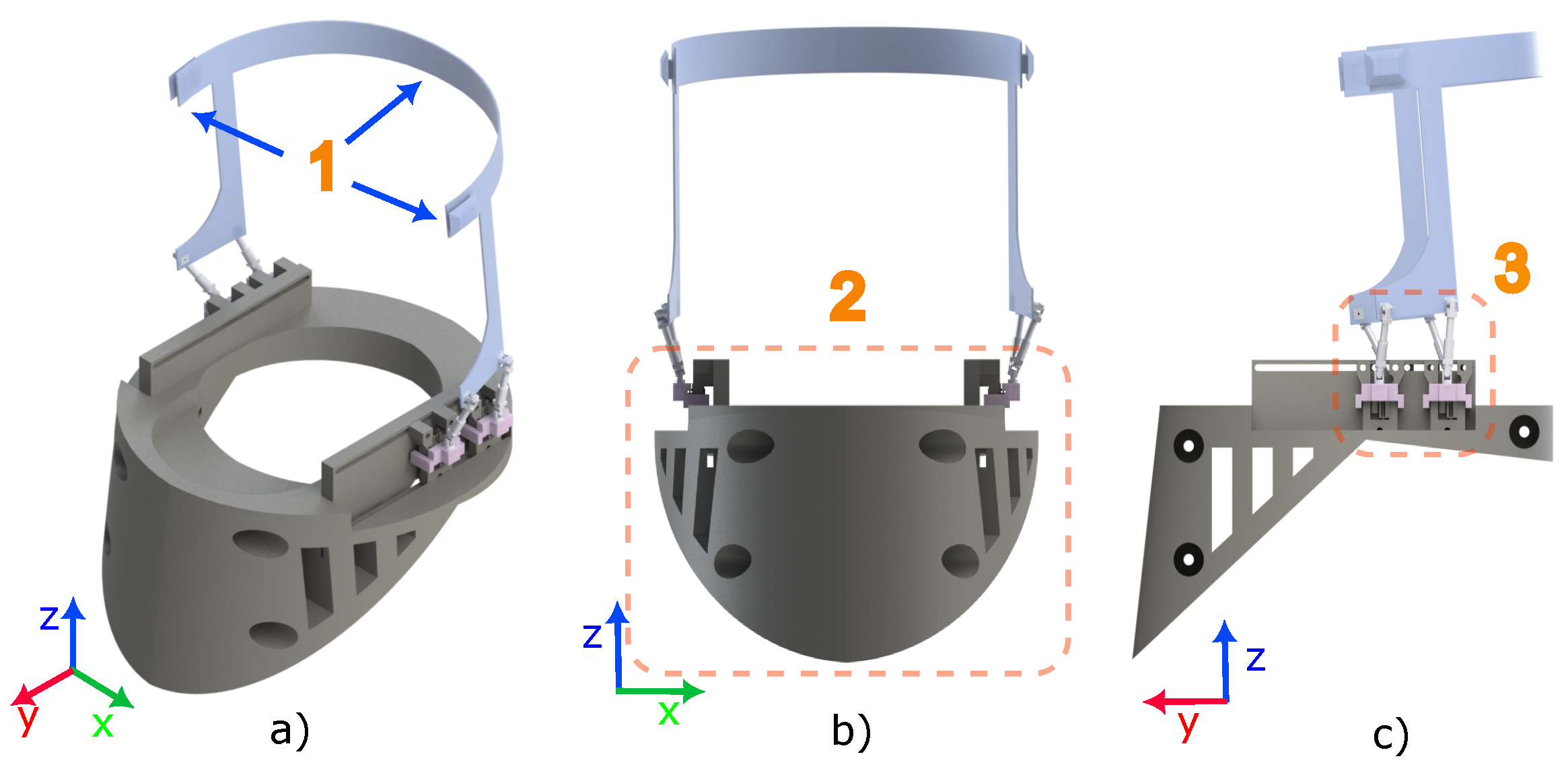
4. Mathematical Description of the Cervical Orthotic Device
5. Control Design Based on an Asymmetric Barrier Lyapunov Function
System Decomposition
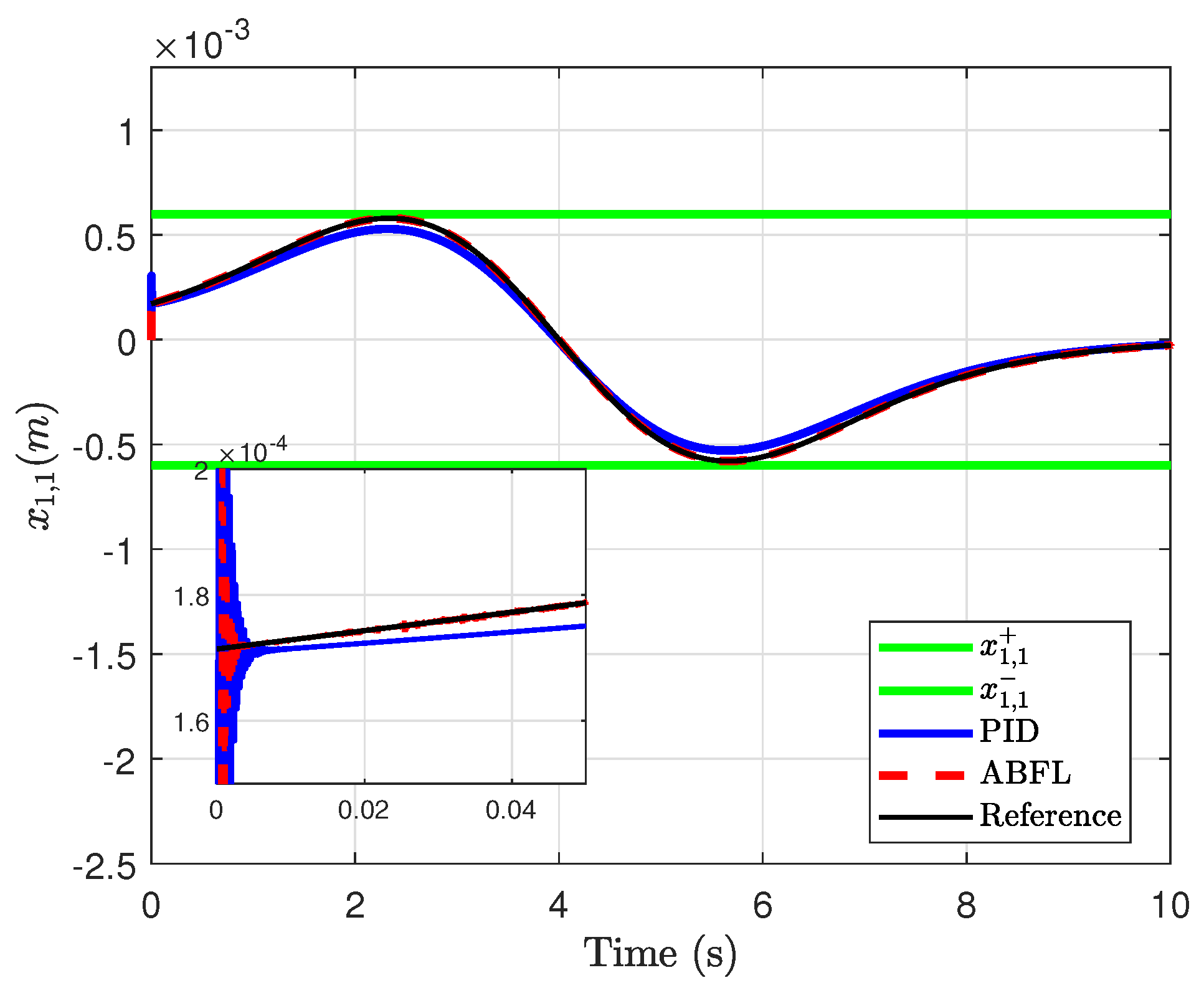
6. Numerical Simulations
Orthosis Specifications
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| BLF | Barrier Lyapunov function |
| ABLF | Asymmetric Barrier Lyapunov function |
| SM | Sliding modes |
| FOSM | First order sliding modes |
References
- Bonato, P. Advances in Wearable Technology and Applications in Physical Medicine and Rehabilitation. J. Neuroeng. Rehabil. 2005, 2, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Onose, G.; Morcov, M.V.; Sporea, C.; Mirea, A.; Ciobanu, V. Augmentation and Rehabilitation with Active Orthotic Devices. In Modern Ap-proaches to Augmentation of Brain Function; Springer: Cham, Switzerland, 2021; pp. 521–548. [Google Scholar] [CrossRef]
- Gavin, T.; Carandang, G.; Havey, R.; Flanagan, P.; Ghanayem, A.; Patwardhan, A. Biomechanical analysis of cervical orthoses in flexion and extension: A comparison of cervical collars and cervical thoracic orthoses. J. Rehabil. Res. Dev. 2003, 40, 527–537. [Google Scholar] [CrossRef] [PubMed]
- Kalita, B.; Narayan, J.; Dwivedy, S.K. Development of active lower limb robotic-based orthosis and exoskeleton devices: A systematic review. Int. J. Soc. Robot. 2021, 13, 775–793. [Google Scholar] [CrossRef]
- Ter Wengel, P.V.; de Witt Hamer, P.C.; Pauptit, J.C.; van der Gaag, N.A.; Oner, F.C.; Vandertop, W.P. Early surgical decompression improves neurological outcome after complete traumatic cervical spinal cord injury: A meta-analysis. J. Neurotrauma 2019, 36, 835–844. [Google Scholar] [CrossRef] [PubMed]
- Yozbatiran, N.; Francisco, G.E. Robot-assisted therapy for the upper limb after cervical spinal cord injury. Phys. Med. Rehabil. Clin. 2019, 30, 367–384. [Google Scholar] [CrossRef] [PubMed]
- Lendraitienė, E.; Smilgiene, L.; Petruseviciene, D.; Savickas, R. Changes and Associations between Cervical Range of Motion, Pain, Temporomandibular Joint Range of Motion and Quality of Life in Individuals with Migraine Applying Physiotherapy: A Pilot Study. Medicina 2021, 57, 630. [Google Scholar] [CrossRef] [PubMed]
- Bell, K.M.; Frazier, E.; Shively, C.; Hartman, R.A.; Ulibarri, J.C.; Lee, J.Y.; Kang, J.D.; Donaldson, W.F. Assessing range of motion to evaluate the adverse effects of ill-fitting cervical orthoses. Spine J. Off. J. N. Am. Spine Soc. 2009, 9 3, 225–231. [Google Scholar] [CrossRef]
- Frigo, C.; Pavan, E. Prosthetic and Orthotic Devices. Assist. Technol. Concepts Methodol. Tools Appl. 2013, 549–613. [Google Scholar] [CrossRef]
- Karimi, M.T.; Kamali, M.; Fatoye, F. Evaluation of the efficiency of cervical orthoses on cervical fracture: A review of literature. J. Craniovertebral Junction Spine 2016, 7, 13. [Google Scholar] [CrossRef]
- Johnson, R.M.; Hart, D.L.; Owen, J.R.; Lerner, E.; Chapin, W.; Zeleznik, R. The yale cervical orthosis: An evaluation of its effectiveness in restricting cervical motion in normal subjects and a comparison with other cervical orthoses. Phys. Ther. 1978, 58, 865–871. [Google Scholar] [CrossRef] [PubMed]
- Sarma, J.; Sahai, N.; Bhatia, D. Recent advances on ankle foot orthosis for gait rehabilitation: A review. Int. J. Biomed. Eng. Technol. 2020, 33, 159–173. [Google Scholar] [CrossRef]
- Lochner, S.; Huissoon, J.; Bedi, S. Parametric Design of Custom Foot Orthotic Model. Comput.-Aided Des. Appl. 2013, 9, 1–11. [Google Scholar] [CrossRef]
- White, A. Some Comments on Cervical Orthoses. In Clinical Prosthetics and Orthotics; 2022; Available online: http://www.oandplibrary.org/cpo/pdf/1983_03_005.pdf (accessed on 26 August 2022).
- Kumar, R.; Prakash, A. Efficacy of cervical orthoses in improving neck control of growing children with cerebral palsy: A narrative review. J. Indira Gandhi Inst. Med Sci. 2021, 7, 90. [Google Scholar] [CrossRef]
- Ricotta, V.; Campbell, R.; Ingrassia, T.; Nigrelli, V. A new design approach for customised medical devices realized by additive manufacturing. Int. J. Interact. Des. Manuf. (IJIDeM) 2020, 14, 1–8. [Google Scholar] [CrossRef]
- Iradi, I.; Zulueta, E.; Teso-Fz-Betoño, D.; Saenz-Aguirre, A.; Fernandez-Gamiz, U. Modeling of Motorized Orthosis Control. Appl. Sci. 2019, 9, 2453. [Google Scholar] [CrossRef]
- Zhang, H.; Chang, B.; Andrews, J.; Mitsumoto, H.; Agrawal, S. A robotic neck brace to characterize head-neck motion and muscle electromyography in subjects with amyotrophic lateral sclerosis. Ann. Clin. Transl. Neurol. 2019, 6, 1671–1680. [Google Scholar] [CrossRef] [PubMed]
- Font-Llagunes, J.; Pàmies-Vilà, R.; Alonso, J.; Lugrís, U. Simulation and design of an active orthosis for an incomplete spinal cord injured subject. Procedia IUTAM 2011, 2, 68–81. [Google Scholar] [CrossRef]
- Chang, B.C.; Zhang, H.; Trigili, E.; Agrawal, S.K. Bio-Inspired Gaze-Driven Robotic Neck Brace. In Proceedings of the 2020 8th IEEE RAS/EMBS International Conference for Biomedical Robotics and Biomechatronics (BioRob), New York, NY, USA, 29 November–1 December 2020; pp. 545–550. [Google Scholar] [CrossRef]
- Guzman-Victoria, I.; Salgado, I.; Mera, M.; Chairez, I.; Ahmed, H. Impedance Adaptive Controller for a Prototype of a Whiplash Syndrome Rehabilitation Device. Math. Probl. Eng. 2019, 2019, 1–21. [Google Scholar] [CrossRef]
- Zhang, H.; Santamaria, V.; Agrawal, S. Applying Force Perturbations Using a Wearable Robotic Neck Brace. In Proceedings of the 2020 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Las Vegas, NV, USA, 25–29 October 2020; pp. 4197–4202. [Google Scholar] [CrossRef]
- Cruz-Ortiz, D.; Chairez, I.; Poznyak, A. Sliding-Mode Control of Full-State Constraint Nonlinear Systems: A Barrier Lyapunov Function Approach. IEEE Trans. Syst. Man Cybern. Syst. 2022, 52, 6593–6606. [Google Scholar] [CrossRef]
- Tee, K.P.; Ge, S.S.; Tay, E.H. Barrier Lyapunov functions for the control of output-constrained nonlinear systems. Automatica 2009, 45, 918–927. [Google Scholar] [CrossRef]
- Mera, M.; Salgado, I.; Chairez, I. Robust observer-based controller design for state constrained uncertain systems: Attractive ellipsoid method. Int. J. Control 2020, 93, 1397–1407. [Google Scholar] [CrossRef]
- Li, Y.X. Barrier Lyapunov function-based adaptive asymptotic tracking of nonlinear systems with unknown virtual control coefficients. Automatica 2020, 121, 109181. [Google Scholar] [CrossRef]
- Salgado, I.; Mera, M.; Chairez, I. Suboptimal adaptive control of dynamic systems with state constraints based on barrier lyapunov functions. IET Control Theory Appl. 2018, 12, 1116–1124. [Google Scholar] [CrossRef]
- Yang, G.; Yao, J.; Dong, Z. Neuroadaptive learning algorithm for constrained nonlinear systems with disturbance rejection. Int. J. Robust Nonlinear Control 2022, 32, 6127–6147. [Google Scholar] [CrossRef]
- Cruz-Ortiz, D.; Chairez, I.; Poznyak, A. Non-singular terminal sliding-mode control for a manipulator robot using a barrier Lyapunov function. ISA Trans. 2022, 121, 268–283. [Google Scholar] [CrossRef]
- Zhang, H.; Chang, B.C.; Agrawal, S.K. Using a robotic neck brace for movement training of the head–neck. IEEE Robot. Autom. Lett. 2019, 4, 846–853. [Google Scholar] [CrossRef]
- Lozano, A.; Ballesteros, M.; Cruz-Ortiz, D.; Chairez, I. Active neck orthosis for musculoskeletal cervical disorders rehabilitation using a parallel mini-robotic device. Control Eng. Pract. 2022, 128, 105312. [Google Scholar] [CrossRef]
- Nocedal, J.; Wright, S.J. Numerical Optimization; Springer Series in Operations Research and Financial Engineering; Springer: New York, NY, USA, 2006; pp. 1–663. Available online: https://link.springer.com/book/10.1007/978-0-387-40065-5 (accessed on 26 August 2022).
- Polyakov, A.; Fridman, L. Stability notions and Lyapunov functions for sliding mode control systems. J. Frankl. Inst. 2014, 351, 1831–1865. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mireles, C.; Lozano, A.; Ballesteros, M.; Cruz-Ortiz, D.; Salgado, I. Asymmetric Constrained Control of a Cervical Orthotic Device Based on Barrier Sliding Modes. Appl. Sci. 2022, 12, 10286. https://doi.org/10.3390/app122010286
Mireles C, Lozano A, Ballesteros M, Cruz-Ortiz D, Salgado I. Asymmetric Constrained Control of a Cervical Orthotic Device Based on Barrier Sliding Modes. Applied Sciences. 2022; 12(20):10286. https://doi.org/10.3390/app122010286
Chicago/Turabian StyleMireles, Caridad, Alejandro Lozano, Mariana Ballesteros, David Cruz-Ortiz, and Ivan Salgado. 2022. "Asymmetric Constrained Control of a Cervical Orthotic Device Based on Barrier Sliding Modes" Applied Sciences 12, no. 20: 10286. https://doi.org/10.3390/app122010286
APA StyleMireles, C., Lozano, A., Ballesteros, M., Cruz-Ortiz, D., & Salgado, I. (2022). Asymmetric Constrained Control of a Cervical Orthotic Device Based on Barrier Sliding Modes. Applied Sciences, 12(20), 10286. https://doi.org/10.3390/app122010286





