Experimental and Numerical Studies on Ground Shock Generated by Large Equivalent Surface Explosions
Abstract
:1. Introduction
2. Surface Explosion Experiments
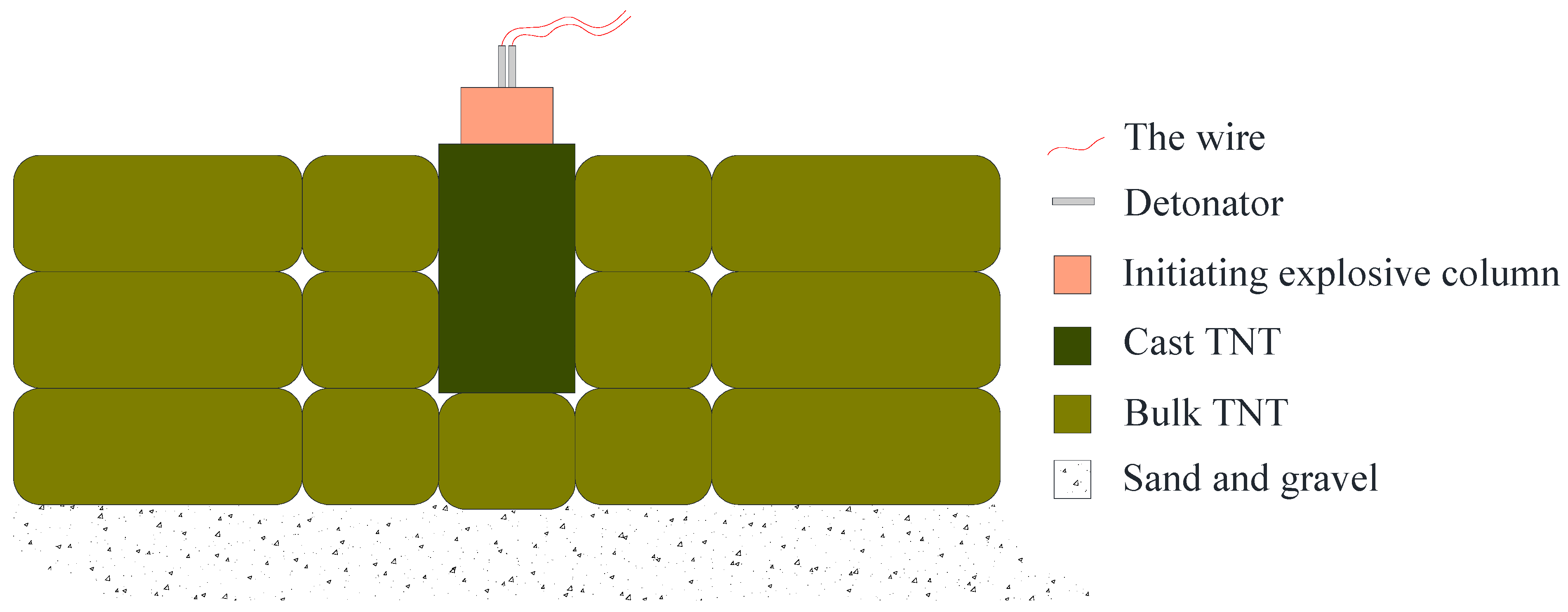
2.1. Test Charges and Method of Detonation
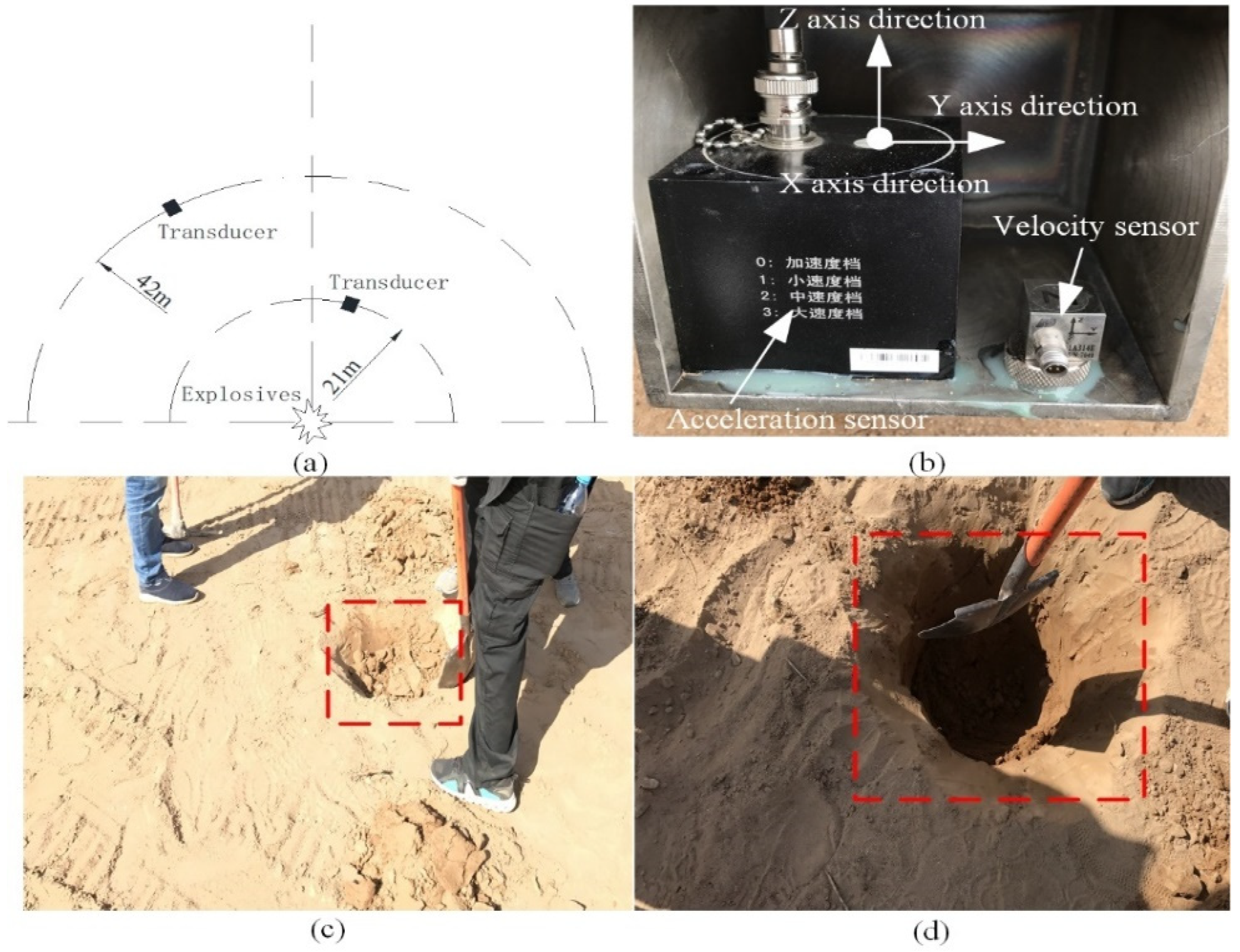
2.2. Method of Ground Shock Measurement
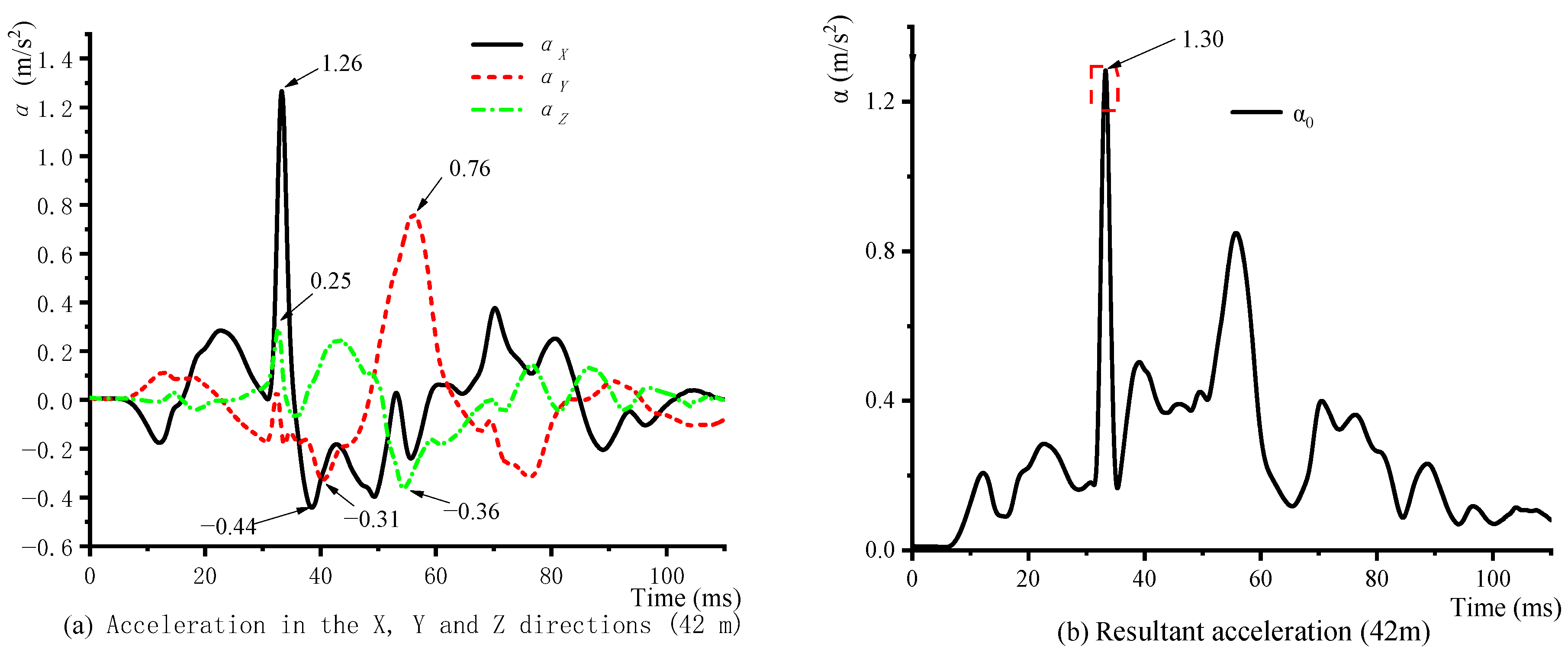
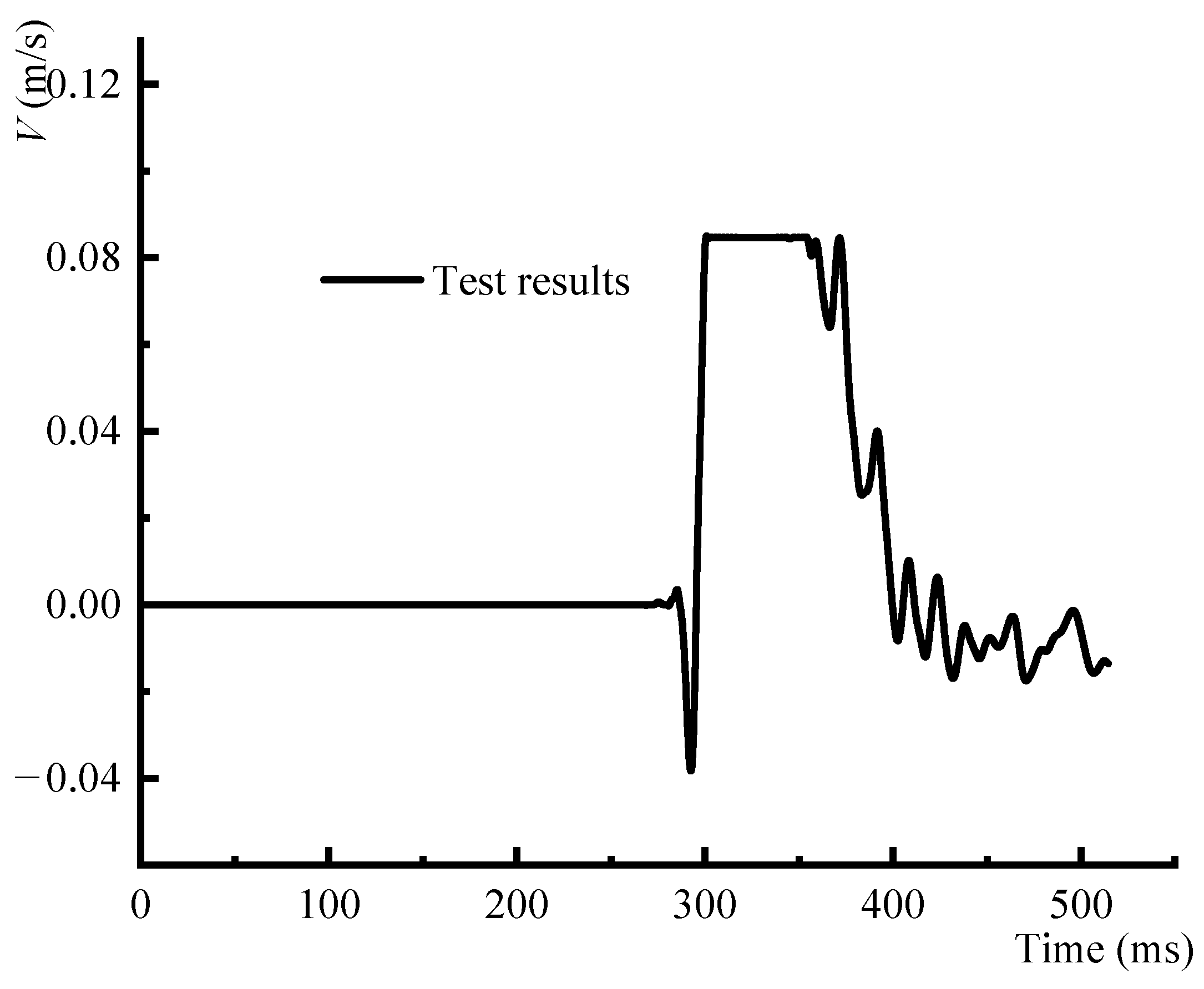
3. Experimental Results and Analysis
4. Finite Element Analysis
4.1. The Finite Element Model and Its Boundary Conditions
4.2. Material Model and Determination of Parameters
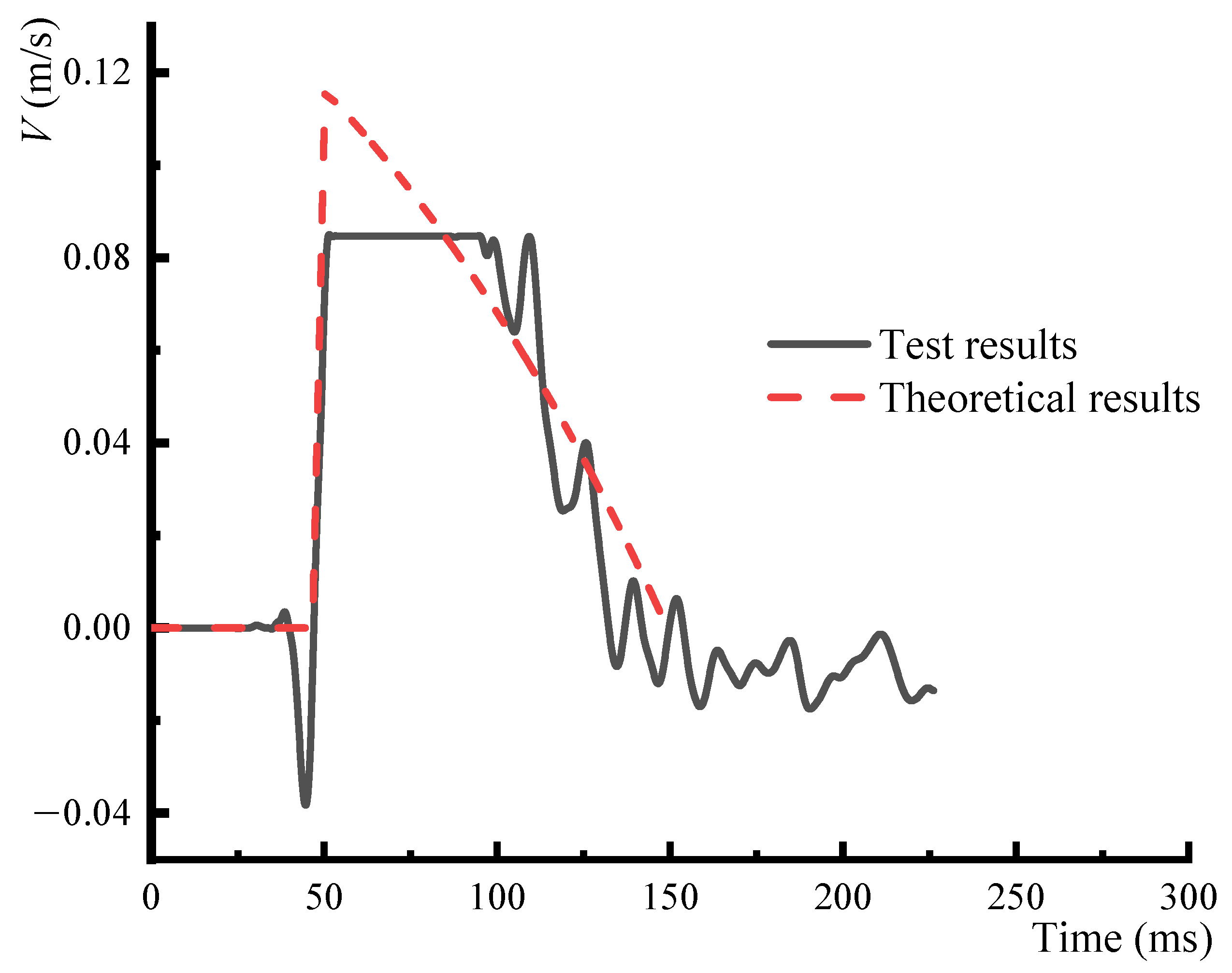
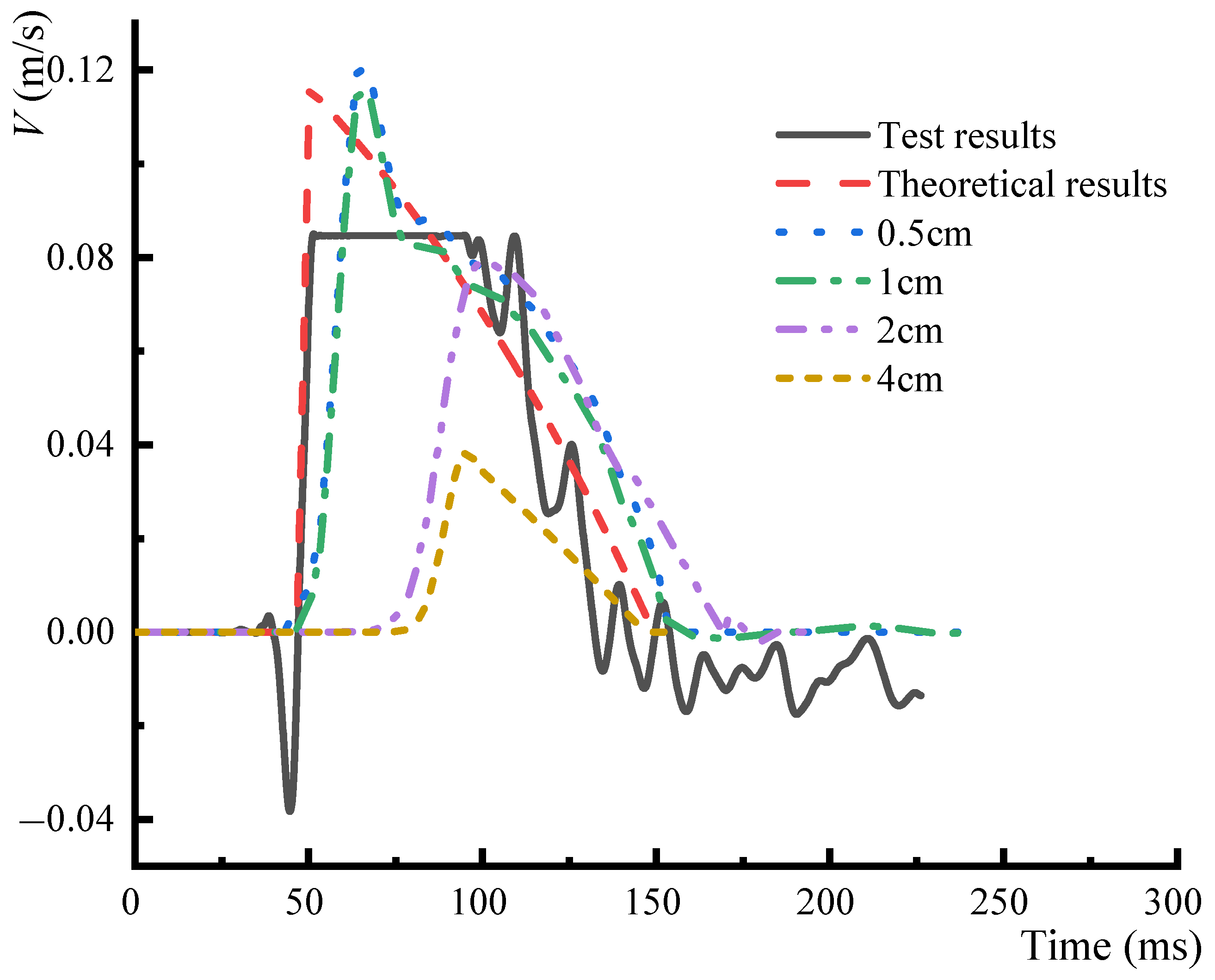
4.3. Grid Validation
5. Discussion
6. Conclusions
- (1)
- The intensity of ground shock decreased with the increase in distance from the blast site, and the accelerations in the X and Y direction were both higher than that in the Z direction.
- (2)
- The seismic wave velocity c and attenuation coefficient of the soil n at the experimental site were found to be c = 457 m/s and n = 2.55. The applicability of the empirical equations provided by the UFC 3-340-01 was validated for our experiment by the determined parameters c and n.
- (3)
- The attenuation behavior of the maximum particle vibration velocities around the epicenter of 1 t and 10 t TNT explosion were obtained, and the minimum safe distance was computed for a variety of structures.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Baker, W.E.; Cox, P.A.; Kulesz, J.J.; Strehlow, R.A.; Westine, P.S. Explosion Hazards and Evaluation; Elsevier: Amsterdam, The Netherlands, 1983. [Google Scholar]
- Henrych, J. The Dynamics of Explosion and Its Use; Elsevier: Amsterdam, The Netherlands, 1979. [Google Scholar]
- UFC 3-340-01; Design and Analysis of Hardened Structures to Conventional Weapon Effects. United Facilities Criteria, Department of Defense: Washington, DC, USA, 2002.
- Liu, H.; Wang, P.; Wei, X.; Xiao, J.; Chen, R. Longitudinal Seismic Response of Continuously Welded Track on Railway Arch Bridges. Appl. Sci. 2018, 8, 775. [Google Scholar] [CrossRef] [Green Version]
- Xu, W.; Yang, X.; Wang, F.; Chi, B. Experimental and Numerical Study on the Seismic Performance of Prefabricated Reinforced Masonry Shear Walls. Appl. Sci. 2018, 8, 1856. [Google Scholar] [CrossRef] [Green Version]
- Yao, E.; Li, W.; Miao, Y.; Ye, L.; Yang, Z. Study on the Influence of a Soft Soil Interlayer on Spatially Varying Ground Motions. Appl. Sci. 2022, 12, 1322. [Google Scholar] [CrossRef]
- Ju, B.-S.; Jeon, B.-G.; Kim, S.-W.; Son, H.-Y. Validation of Seismic Performance of Stainless Press-to-Connect Piping System under Cyclic Loadings. Appl. Sci. 2022, 12, 3896. [Google Scholar] [CrossRef]
- Martínez-Martín, F.J.; Yepes, V.; González-Vidosa, F.; Hospitaler, A.; Alcalá, J. Optimization Design of RC Elevated Water Tanks under Seismic Loads. Appl. Sci. 2022, 12, 5635. [Google Scholar] [CrossRef]
- Mohseni, I.; Lashkariani, H.A.; Kang, J.; Kang, T.H.-K. Dynamic Response Evaluation of Long-Span Reinforced Arch Bridges Subjected to Near- and Far-Field Ground Motions. Appl. Sci. 2018, 8, 1243. [Google Scholar] [CrossRef] [Green Version]
- Merkle, D.; Rochefort, M.; Tuan, C. Equipment Shock Tolerance; U.S. Air Force Civil Engineering Support Agency, Tyndall Air Force Base: Albuquerque, NM, USA, 1993. [Google Scholar]
- Krauthammer, T. Shallow-Buried RC Box-Type Structures. J. Struct. Eng. ASCE 1984, 110, 637–651. [Google Scholar] [CrossRef]
- Weidlinger, P.; Hinman, E. Analysis of underground protective structures. J. Struct. Eng. ASCE 1988, 114, 1658–1673. [Google Scholar] [CrossRef]
- Zhang, Y.D.; Fang, Q.; Liu, J.C. Experimental and numerical investigations into responses of buries RC frames subjected to impulsive loading. In Structures under Shock and Impact VII; Elservier: Amsterdam, The Netherlands, 2002; pp. 69–78. [Google Scholar]
- Nagy, N.; Mohamed, M.; Boot, J.C. A Complete Nonlinear Finite Element Analysis for the Effects of Surface Explosions on Buried Structures. In Proceedings of the Eleventh International Conference on Civil, Structural and Environmental Engineering Computing, St. Julians, Malta, 18–21 September 2007. [Google Scholar] [CrossRef]
- Wu, C.; Lu, Y.; Hao, H. Numerical prediction of blast-induced stress wave from large-scale underground explosion. Int. J. Numer. Anal. Methods Géoméch. 2003, 28, 93–109. [Google Scholar] [CrossRef]
- Wu, C.; Hao, H. Modeling of simultaneous ground shock and airblast pressure on nearby structures from surface explosions. Int. J. Impact Eng. 2005, 31, 699–717. [Google Scholar] [CrossRef]
- Lu, Y.; Wang, Z. Characterization of structural effects from above-ground explosion using coupled numerical simulation. Comput. Struct. 2006, 84, 1729–1742. [Google Scholar] [CrossRef]
- Hao, H.; Zhou, Y. Dynamic Response of Rigid Blocks to Simultaneous Horizontal and Vertical Ground Shock. Adv. Struct. Eng. 2012, 15, 1069–1082. [Google Scholar] [CrossRef]
- Longinow, A.; Mniszewski, K.R. Protecting Buildings against Vehicle Bomb Attacks. Pract. Period. Struct. Des. Constr. 1996, 1, 51–54. [Google Scholar] [CrossRef]
- Fang, Q.; Yang, S.G.; Chen, L.; Liu, J.; Zhang, Y. Analysis on the building damage, personnel casualties and blast energy of the 8 · 12 explosion in Tianjin port. China Civ. Eng. J. 2017, 50, 12–18. [Google Scholar]
- Xu, R.; Chen, L.; Fang, Q.; Zheng, Y.; Li, Z.; Cao, M. Analysis of the power and disaster consequences of "3.21" explosion accident in the chemical industry park of Xiangshui, Yancheng city. J. Disaster Prev. Mitig. Eng. 2019, 50, 12–18. [Google Scholar]
- Pasman, H.J.; Fouchier, C.; Park, S.; Quddus, N.; Laboureur, D. Beirut ammonium nitrate explosion: Are not we really learning anything? Process Saf. Prog. 2020, 39, 1–18. [Google Scholar] [CrossRef]
- Rigby, S.E.; Lodge, T.J.; Alotaibi, S.; Barr, A.D.; Clarke, S.D.; Langdon, G.S.; Tyas, A. Preliminary yield estimation of the 2020 Beirut explosion using video footage from social media. Shock Waves 2020, 30, 671–675. [Google Scholar] [CrossRef]
- Momeni, M.; Bedon, C.; Hadianfard, M.A.; Baghlani, A. An Efficient Reliability-Based Approach for Evaluating Safe Scaled Distance of Steel Columns under Dynamic Blast Loads. Buildings 2021, 11, 606. [Google Scholar] [CrossRef]
- Lee, E.; Finger, M.; Collins, W. JWL Equation of State Coefficients for High Explosives; Lawrence Livermore National Lab. (LLNL): Livermore, CA, USA, 1973. [CrossRef] [Green Version]
- Rogers, G.F.C.; Mayhew, Y.R. Thermodynamic and transport properties of fluids. Fluid Phase Equilibria 1994, 83, 85–92. [Google Scholar]
- Yang, Q.; Zheng, J.; Cheng, K.; Lv, D. Modified Finite-element Model to Analyze Soil Arching Effect in Piled Embankments. J. Civil. Eng. Manag. 2017, 34, 19–23. [Google Scholar]
- Shi, Y.; Hao, H.; Li, Z.-X. Numerical simulation of blast wave interaction with structure columns. Shock Waves 2007, 17, 113–133. [Google Scholar] [CrossRef]
- National Standard Writing Group of the People’s Republic of China. Blasting Safety Regulations GB6722-2014; China Standard Press: Beijing, China, 2015. [Google Scholar]






| Type | Model | Sensitivity (mV/m∙s−2) | Range | Frequency Range (Hz) |
|---|---|---|---|---|
| Acceleration sensors | 1B314E | ~10 | 0~500 | Y/Z 0.5–7000; X 0.5–5000 |
| Velocity sensor | 2A101E | ~4 | 1270 | 2~6000 |
| Material Description | Seismic Wave Velocity c (ft/s) | Acoustic Impedance (ρc) (lbs∙ft/in3∙s) | Attenuation Coefficient n |
|---|---|---|---|
| Loose dry sands and gravel with a low relative density | 600 | 12 | 3~3.25 |
| Sandy loam, loess, dry sands, and backfill | 1000 | 22 | 2.75 |
| Dense sand with high relative density | 1600 | 44 | 2.5 |
| Wet sandy clay with air voids (greater than 4%) | 1800 | 48 | 2.5 |
| Saturated sandy clays and sands with small amount of air voids (less than 1%) | 5000 | 130 | 2.25~2.5 |
| Heavy saturated clays and clay shales | >5000 | 150~180 | 1.5 |
| ρ (kg·m−3) | D (m·s−1) | PC–J (Pa) | A (Pa) | B (Pa) | R1 | R2 | ω | E0 (J·m−3) |
|---|---|---|---|---|---|---|---|---|
| 1630 | 6930 | 2.7 × 1010 | 3.7377 × 1011 | 3.7471 × 109 | 4.15 | 0.9 | 0.35 | 7 × 109 |
| Air Density ρ (kg·m−3) | Massic Heat Capacity at Constant Volume cE (J·kg−1·K−1) | Initial Pressure P0 (Pa) | Adiabatic Exponent γ | Initial Internal Energy E0 (J·m−3) |
|---|---|---|---|---|
| 1.225 | 717.6 | 0 | 1.4 | 2.586 × 105 |
| Parameter | Value | Parameter | Value | Parameter | Value | Parameter | Value |
|---|---|---|---|---|---|---|---|
| ρ (kg/m2) | 2050 | PREF | 0 | VEPS7 | 0.095 | P4 (MPa) | 0.156 |
| G (MPa) | 71.43 | VEPS1 | 0 | VEPS8 | 0.11 | P5 (MPa) | 0.176 |
| B (MPa) | 2.2 × 108 | VEPS2 | 0.01 | VEPS9 | 0.13 | P6 (MPa) | 0.189 |
| a0 | 1.2 × 104 | VEPS3 | 0.02 | VEPS10 | 0.157 | P7 (MPa) | 0.197 |
| a1 | 0.16 | VEPS4 | 0.039 | P1 (MPa) | 0 | P8 (MPa) | 0.202 |
| a2 | 0 | VEPS5 | 0.058 | P2 (MPa) | 0.101 | P9 (MPa) | 0.212 |
| PC | 0 | VEPS6 | 0.077 | P3 (MPa) | 0.128 | P10 (MPa) | 0.217 |
| VCR | 0 |
| Type of To-Be-Protected Structure | Safe and Permissible Particle Vibration Velocities, v (cm/s) | ||
|---|---|---|---|
| f ≤ 10 Hz | 10 Hz < f ≤ 50 Hz | f > 50 Hz | |
| Cave dwellings, adobe houses, and rubble houses | 0.15~0.45 | 0.45~0.9 | 0.9~1.5 |
| Ordinary civilian buildings | 1.5~2 | 2~2.5 | 2.5~3 |
| Industrial and commercial buildings | 2.5~3.5 | 3.5~4.5 | 4.2~5 |
| Ordinary historical buildings and sites | 0.1~0.2 | 0.2~0.3 | 0.3~0.5 |
| Central control room equipment in operational hydropower stations and power stations | 0.5~0.6 | 0.6~0.7 | 0.7~0.9 |
| Hydraulic tunnels | 7~8 | 8~10 | 10~15 |
| Traffic tunnels | 10~12 | 12~15 | 15~20 |
| Mine roadways | 15~18 | 18~25 | 25~30 |
| Permanent rocky high slopes | 5~9 | 8~12 | 10~15 |
| Age: Initial setting–3 days | 1.5~2 | 2~2.5 | 2.5~3 |
| Age: 3–7 days | 3~4 | 4~5 | 5~7 |
| Age: 7–28 days | 7~8 | 8~10 | 10~12 |
| To-Be-Protected Structure | Minimum Distance for Vibration Safety (m) |
|---|---|
| Cave dwellings, adobe houses, and rubble houses | 23.7~29.6 |
| Ordinary civilian buildings | 17.5~19.0 |
| Industrial and commercial buildings | 14.1~15.2 |
| Ordinary historical buildings and sites | 38.3~47.8 |
| Central control room equipment in operational hydropower stations and power stations | 29.6~33.1 |
| Hydraulic tunnels | 8.7~10.4 |
| Traffic tunnels | 7.4~8.7 |
| Mine roadways | 6.5~7.0 |
| Permanent rocky high slopes | 8.7~10.4 |
| Age: Initial setting–3 days | 17.5~19.0 |
| Age: 3–7 days | 12.2~14.1 |
| Age: 7–28 days | 9.6~10.4 |
| To-Be-Protected Structure | Minimum Distance for Vibration Safety (m) |
|---|---|
| Cave dwellings, adobe houses, and rubble houses | 51.1~63.8 |
| Ordinary civilian buildings | 37.8~40.9 |
| Industrial and commercial buildings | 30.3~32.7 |
| Ordinary historical buildings and sites | 82.4~102.9 |
| Central control room equipment in operational hydropower stations and power stations | 63.8~71.2 |
| Hydraulic tunnels | 18.8~22.4 |
| Traffic tunnels | 16.6~18.8 |
| Mine roadways | 13.9~15 |
| Permanent rocky high slopes | 18.8~22.4 |
| Age: Initial setting–3 days | 37.8~40.9 |
| Age: 3–7 days | 26.2~30.3 |
| Age: 7–28 days | 20.7~22.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, C.; Chen, L.; Xu, R.; Cao, M.; Chen, D.; Fang, Q. Experimental and Numerical Studies on Ground Shock Generated by Large Equivalent Surface Explosions. Appl. Sci. 2022, 12, 7987. https://doi.org/10.3390/app12167987
Xia C, Chen L, Xu R, Cao M, Chen D, Fang Q. Experimental and Numerical Studies on Ground Shock Generated by Large Equivalent Surface Explosions. Applied Sciences. 2022; 12(16):7987. https://doi.org/10.3390/app12167987
Chicago/Turabian StyleXia, Chenxi, Li Chen, Rongzheng Xu, Mingjin Cao, Dapeng Chen, and Qin Fang. 2022. "Experimental and Numerical Studies on Ground Shock Generated by Large Equivalent Surface Explosions" Applied Sciences 12, no. 16: 7987. https://doi.org/10.3390/app12167987
APA StyleXia, C., Chen, L., Xu, R., Cao, M., Chen, D., & Fang, Q. (2022). Experimental and Numerical Studies on Ground Shock Generated by Large Equivalent Surface Explosions. Applied Sciences, 12(16), 7987. https://doi.org/10.3390/app12167987





