Simulation of Nonseparable Nonstationary Spatially Varying Ground Motions with an Enhanced Interpolation Approximation Approach
Abstract
:1. Introduction
2. Theoretical Foundation
2.1. Description of Ground Motion Fields
2.2. SRM-Based Nonstationary Ground Motions Simulation
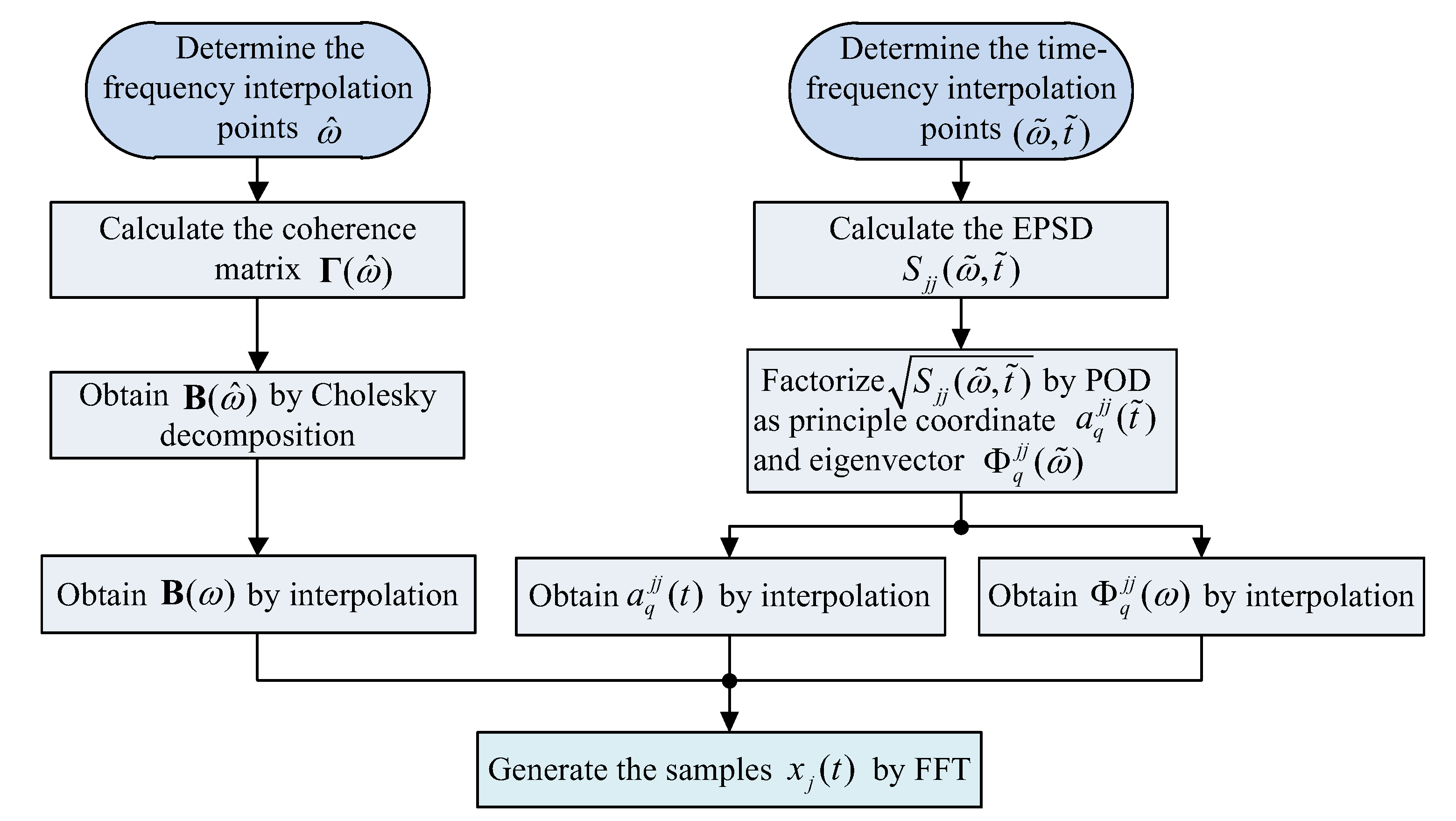
3. Enhanced Interpolation Approach
3.1. Decomposition of EPSD Matrix Based on Interpolation
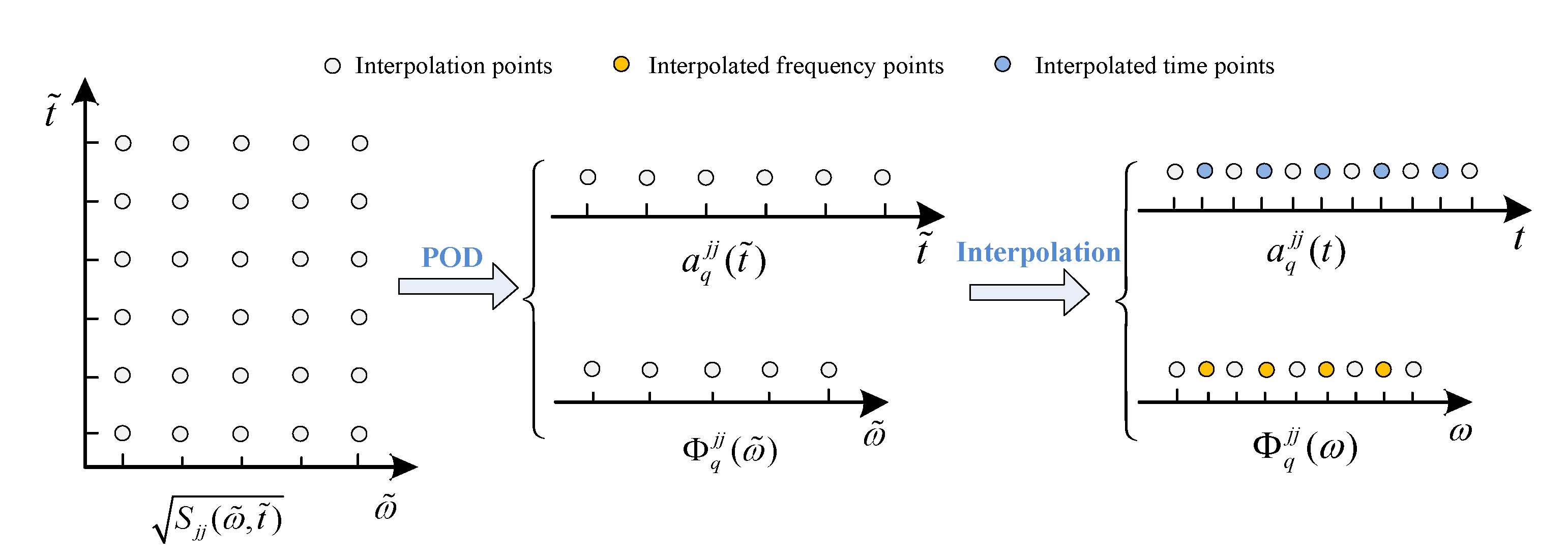
3.2. Time-Frequency Decoupling with POD Interpolation
3.3. Optimizing FFT Operations
3.4. Complete Simulation Procedure
4. Numerical Examples
4.1. Nonseparable Spectrum
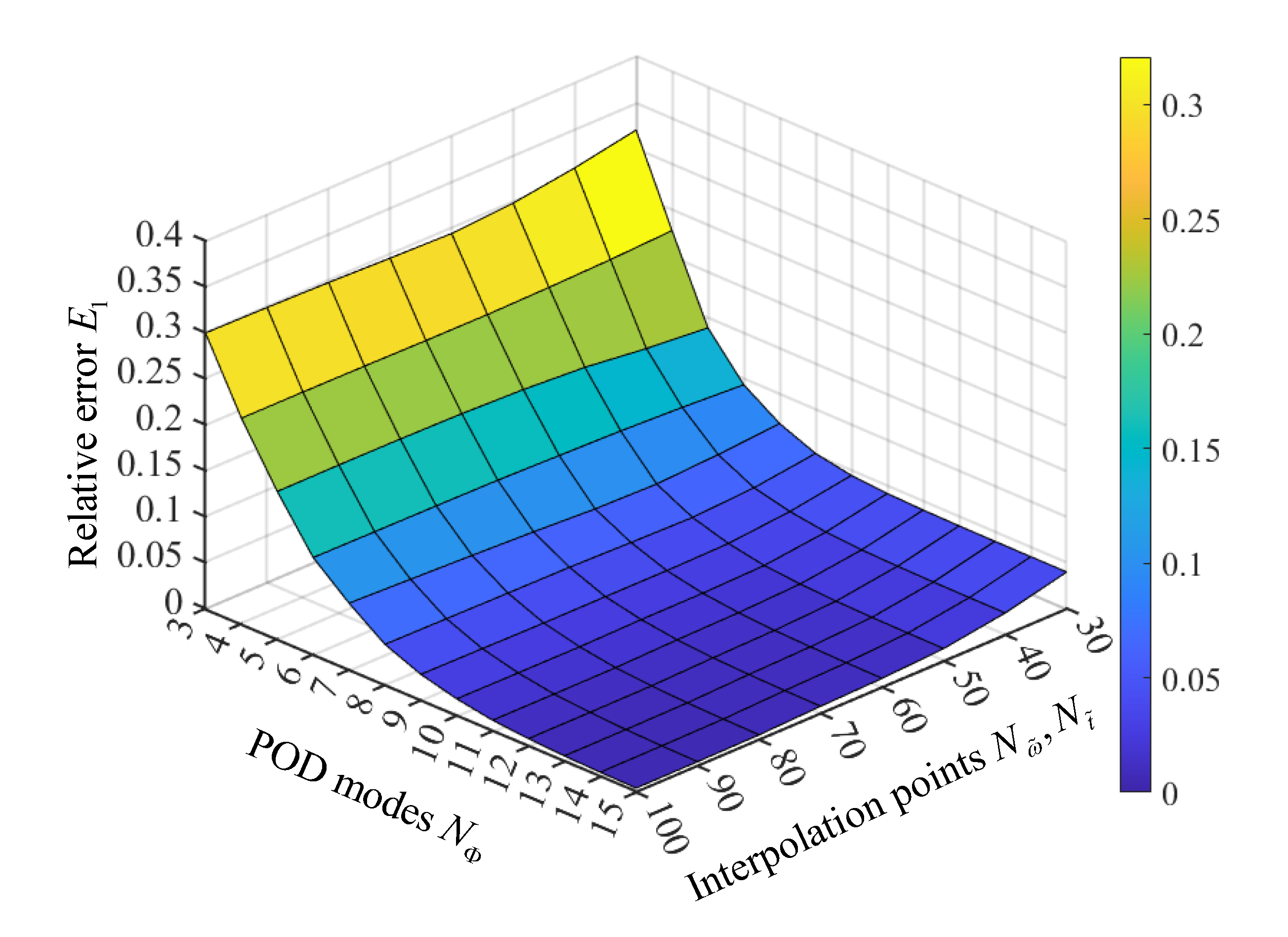
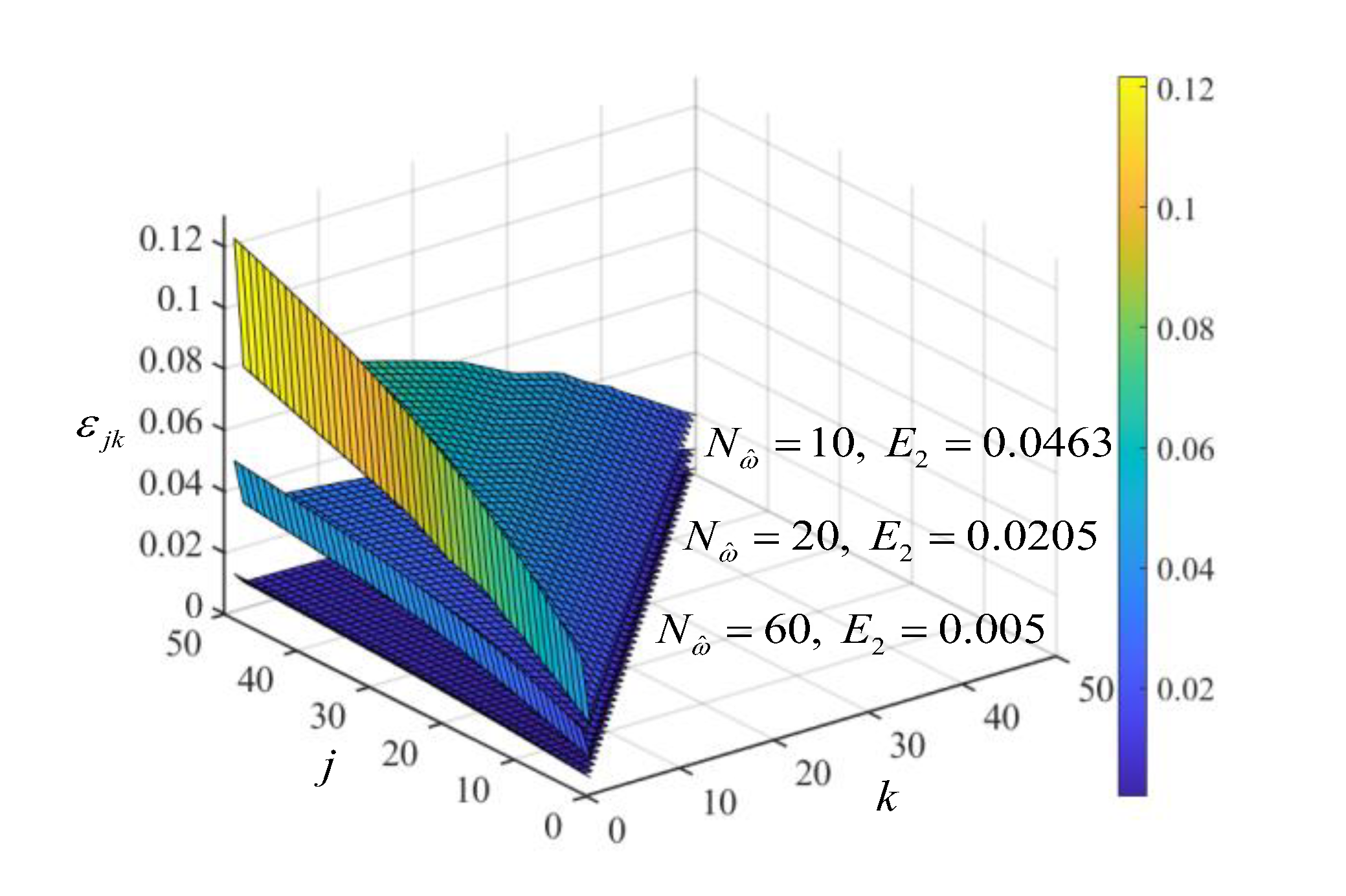
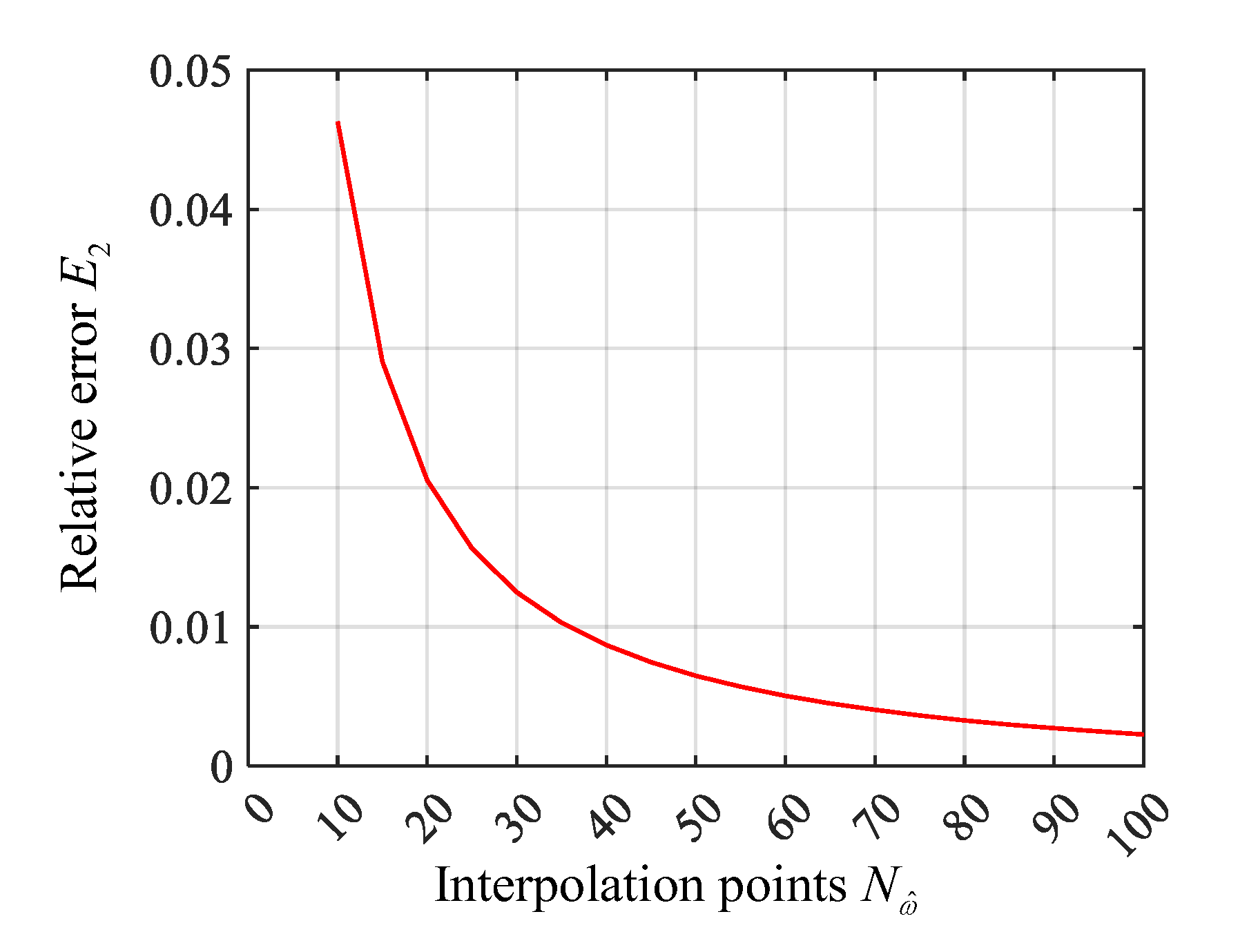
4.1.1. Parametric Analysis
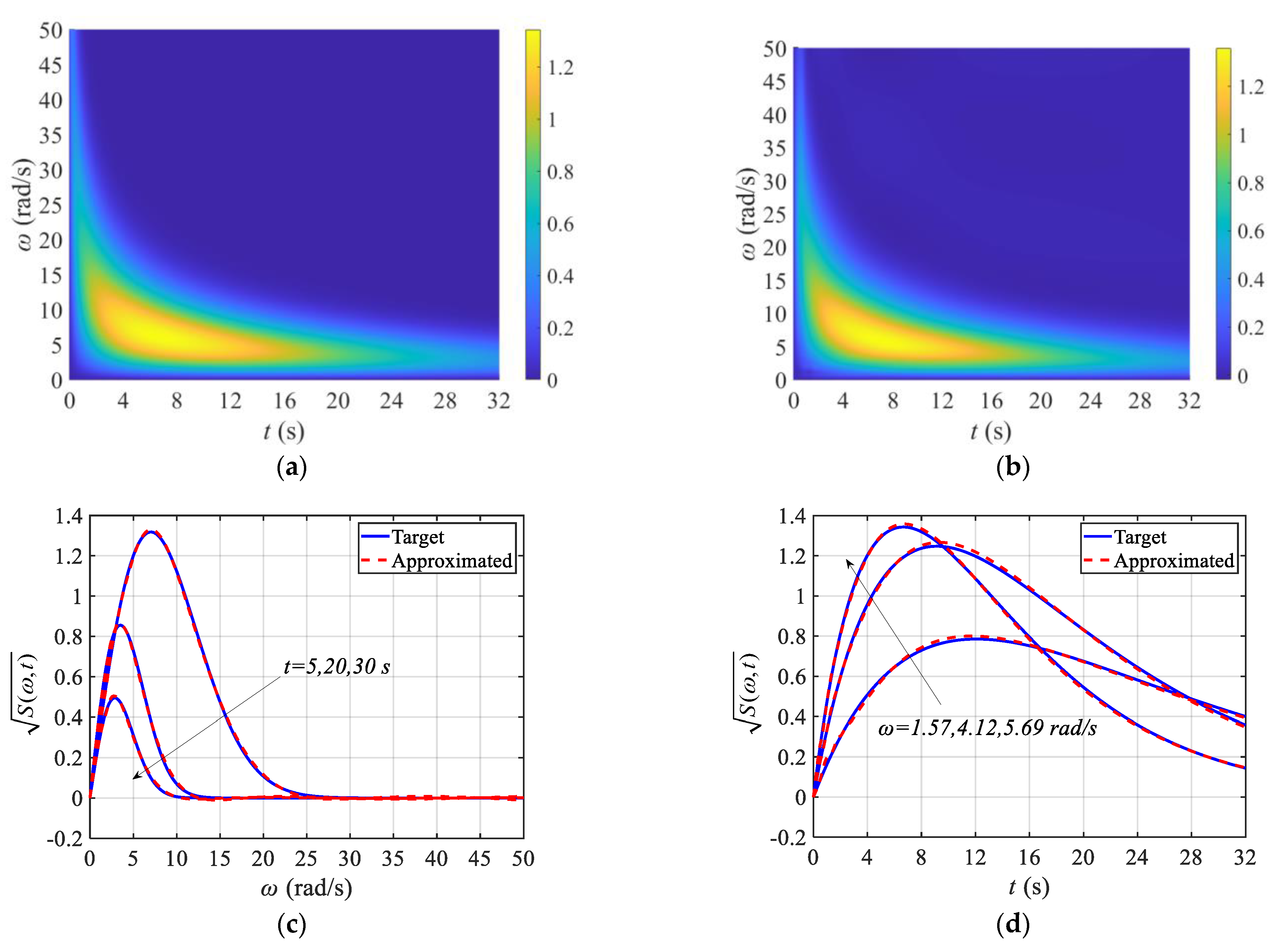
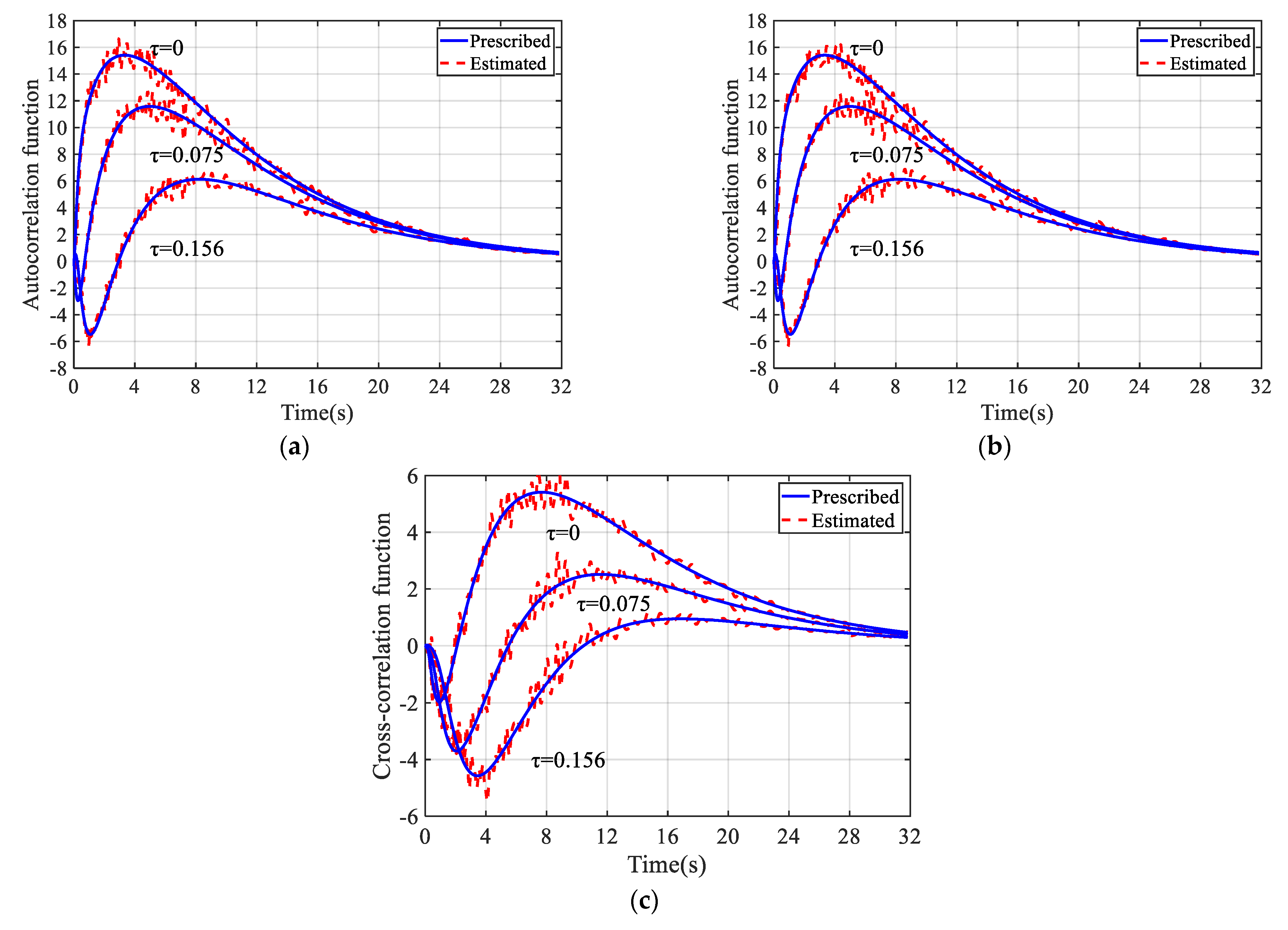
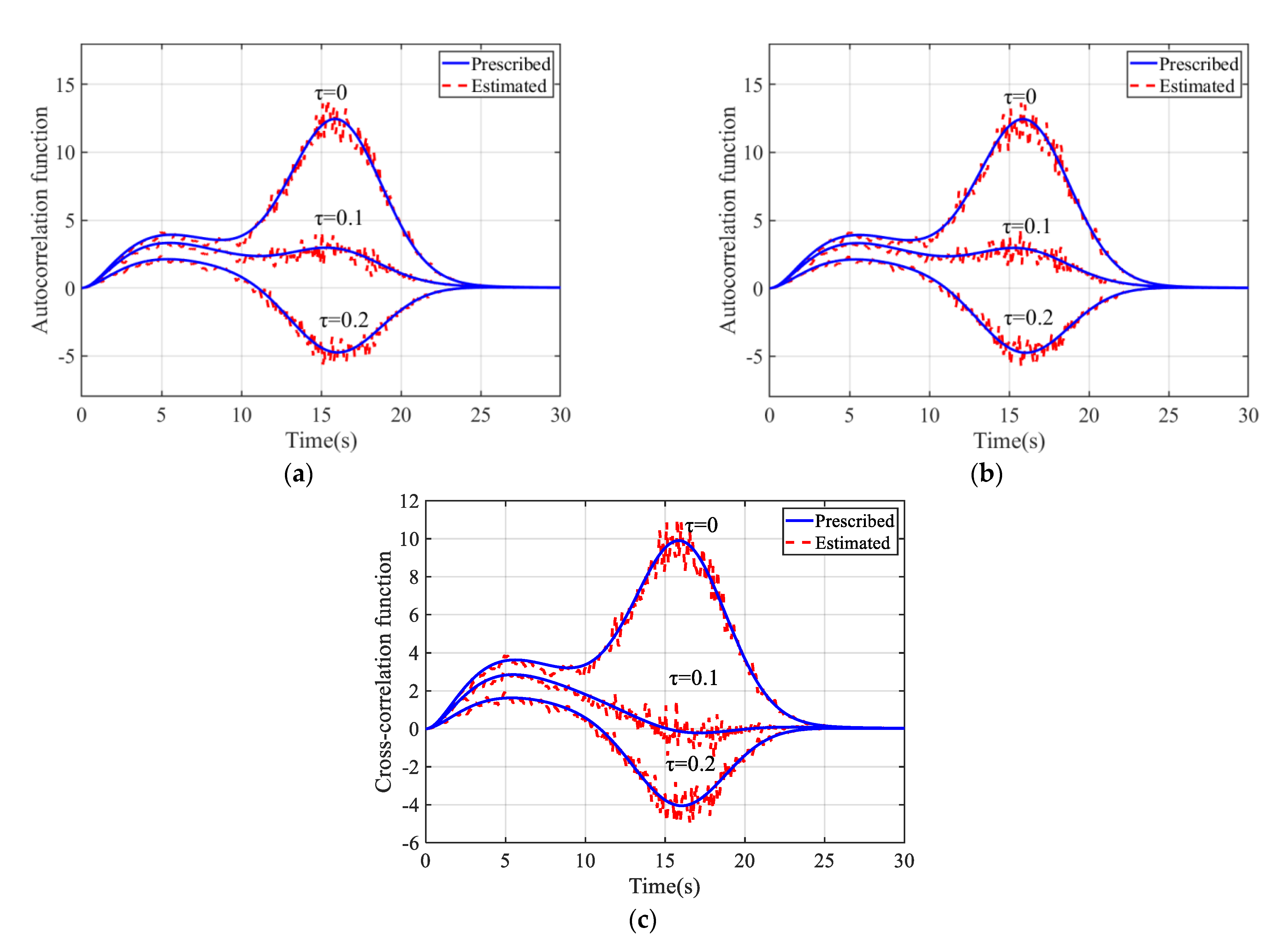
4.1.2. Simulation Accuracy
4.1.3. Simulation Efficiency
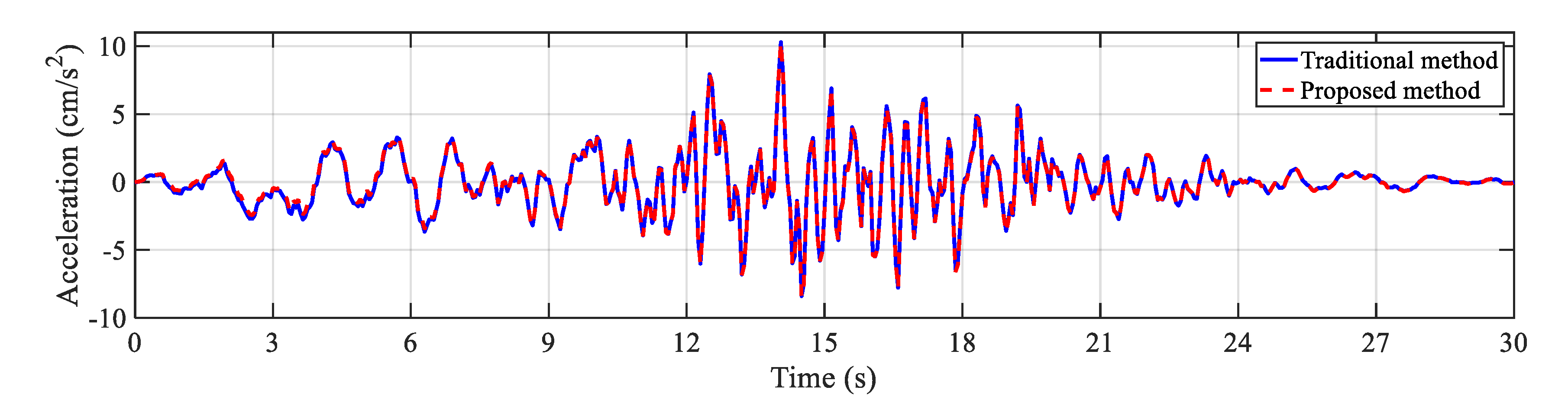
4.2. Nonuniformly Modulated Spectrum
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Application of the FFT
References
- Kiureghian, A.D. A coherency model for spatially varying ground motions. Earthq. Eng. Struct. Dyn. 1996, 25, 99–111. [Google Scholar] [CrossRef]
- Zerva, A. Spatial Variation of Seismic Ground Motions: Modeling and Engineering Application; CRC Press: New York, NY, USA, 2009. [Google Scholar]
- Hao, H.; Oliveira, C.S.; Penzien, J. Multiple-station ground motion processing and simulation based on SMART-1 array data. Nucl. Eng. Des. 1989, 111, 293–310. [Google Scholar] [CrossRef]
- Deodatis, G. Non-stationary stochastic vector processes: Seismic ground motion applications. Probabilistic Eng. Mech. 1996, 11, 149–167. [Google Scholar] [CrossRef]
- Cacciola, P.; Deodatis, G. A method for generating fully non-stationary and spectrum-compatiable ground motion vector processes. Soil Dyn. Earthq. Eng. 2011, 31, 351–360. [Google Scholar] [CrossRef] [Green Version]
- Wu, Y.; Gao, Y.; Li, D. Simulation of spatially correlated earthquake ground motions for engineering purposes. Earthq. Eng. Eng. Vib. 2011, 10, 163–173. [Google Scholar] [CrossRef]
- Bi, K.; Hao, H. Modelling and simulation of spatially correlated earthquake ground motions at sites with varying conditions. Prob. Eng. Mech. 2012, 29, 92–104. [Google Scholar] [CrossRef]
- Yang, W.W.; Chang, T.Y.P.; Chang, C.C. An efficient wind field simulation technique for bridges. J. Wind. Eng. Ind. Aerodyn. 1997, 67, 697–708. [Google Scholar] [CrossRef]
- Cao, Y.; Xiang, H.; Zhou, Y. Simulation of stochastic wind velocity field on long-span bridges. J. Eng. Mech. 2000, 126, 1–6. [Google Scholar] [CrossRef]
- Zhao, N.; Huang, G. Wind velocity field simulation based on enhanced closed-form solution of Cholesky decomposition. J. Eng. Mech. 2020, 146, 04019128. [Google Scholar] [CrossRef]
- Gao, Y.; Wu, Y.; Li, D.; Liu, H.; Zhang, N. An improved approximation for the spectral representation method in the simulation of spatially varying ground motions. Prob. Eng. Mech. 2012, 29, 7–15. [Google Scholar] [CrossRef]
- Huang, G.; Liao, H.; Li, M. New Formulation of Cholesky Decomposition and Applications in Stochastic Simulation. Prob. Eng. Mech. 2013, 34, 40–47. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhao, N.; Peng, L.; Zhao, L.; Liu, M. Simulation of stationary wind field based on adaptive interpolation-enhanced scheme. J. Wind. Eng. Ind. Aerodyn. 2019, 195, 104001. [Google Scholar] [CrossRef]
- Zhao, N.; Jiang, Y.; Peng, L.; Chen, X. Fast simulation of nonstationary wind velocity fields by proper orthogonal decomposition interpolation. J. Wind. Eng. Ind. Aerodyn. 2021, 219, 104798. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhao, N.; Peng, L.; Xin, J.; Liu, S. Fast simulation of fully non-stationary wind fields using a new matrix factorization assisted interpolation. Mech. Syst. Sig. Process. 2022, 172, 108973. [Google Scholar] [CrossRef]
- Yang, J.N. Simulation of random envelope processes. J. Sound Vib. 1972, 21, 73–85. [Google Scholar] [CrossRef]
- Wittig, L.E.; Sinha, A.K. Simulation of multicorrelated random processes using the FFT algorithm. J. Acoust. Soc. Am. 1975, 58, 630–634. [Google Scholar] [CrossRef] [Green Version]
- Spanos, P.D.; Kougioumtzoglou, I.A. Harmonic wavelets based statistical linearization for response evolutionary power spectrum determination. Prob. Eng. Mech. 2012, 27, 57–68. [Google Scholar] [CrossRef]
- Li, Y.; Kareem, A. Simulation of multivariate nonstationary random processes: Hybrid DFT and digital filtering approach. J. Eng. Mech. 1997, 123, 1302–1310. [Google Scholar] [CrossRef]
- Huang, G. An efficient simulation approach for multivariate nonstationary process: Hybrid of wavelet and spectral representation method. Prob. Eng. Mech. 2014, 37, 74–83. [Google Scholar] [CrossRef]
- Huang, G. Application of Proper Orthogonal Decomposition in Fast Fourier Transform—Assisted Multivariate Nonstationary Process Simulation. J. Eng. Mech. 2015, 141, 04015015. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, Z.; Ruan, X.; Zhang, Q. Spectral representation-based dimension reduction for simulating multivariate non-stationary ground motions. Soil Dyn. Earthq. Eng. 2018, 114, 313–325. [Google Scholar] [CrossRef]
- Ruan, X.; Liu, Z.; Liu, Z.; Fan, Y. Dimension-reduction representation of stochastic ground motion fields based on wavenumber-frequency spectrum for engineering purposes. Soil Dyn. Earthq. Eng. 2021, 143, 106604. [Google Scholar] [CrossRef]
- Priestley, M.B. Evolutionary spectra and non-stationary processes. J. R. Stat. Soc. Ser. B 1965, 27, 204–229. [Google Scholar] [CrossRef]
- Conte, J.P.; Peng, B.F. Fully nonstationary analytical earthquake ground-motion model. J. Eng. Mech. 1997, 123, 15–24. [Google Scholar] [CrossRef]
- Li, Y.; Kareem, A. Simulation of multivariate nonstationary random processes by FFT. J. Eng. Mech. 1991, 117, 1037–1058. [Google Scholar] [CrossRef]
- Konakli, K.; Kiureghian, A.D. Simulation of spatially varying ground motions including incoherence, wave-passage and differential site-response effects. Earthq. Eng. Struct. Dyn. 2012, 41, 495–513. [Google Scholar] [CrossRef]
- Zhao, N.; Huang, G. Fast simulation of multivariate nonstationary process and its application to extreme winds. J. Wind. Eng. Ind. Aerodyn. 2017, 170, 118–127. [Google Scholar] [CrossRef]
- Wang, H.; Xu, Z.; Feng, D.; Tao, T. Non-stationary turbulent wind field simulation of bridge deck using non-negative matrix factorization. J. Wind. Eng. Ind. Aerodyn. 2019, 188, 235–246. [Google Scholar] [CrossRef]
- Harichandran, R.S.; Vanmarcke, E.H. Stochastic variation of earthquake ground motion in space and time. J. Eng. Mech. 1986, 112, 154–174. [Google Scholar] [CrossRef]
- Li, B.; Peng, L.; Jiang, Y.; Wu, F.; Hui, Y.; Luo, Y. Simulation of stationary non-Gaussian stochastic vector processes using an eigenvalue-based iterative translation approximation method. Mech. Syst. Sig. Process. 2022, 175, 109128. [Google Scholar] [CrossRef]


| Parameters | Values |
|---|---|
| Considered POD mode number | |
| Frequency interpolation point number | |
| Time-frequency interpolation point number |
| Method | n = 50 | n = 100 | n = 150 | n = 200 |
|---|---|---|---|---|
| Proposed | 76.84 s | 243.64 s | 502.73 s | 832.91 s |
| Traditional | 805.35 s | 3227.53 s | 8501.64 s | 15241.56 s |
| Time ratio | 10.48 | 13.25 | 16.91 | 18.30 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, N.; Xu, Z.; Peng, L.; Li, X.; Chen, X.; Wang, X. Simulation of Nonseparable Nonstationary Spatially Varying Ground Motions with an Enhanced Interpolation Approximation Approach. Appl. Sci. 2022, 12, 6757. https://doi.org/10.3390/app12136757
Zhao N, Xu Z, Peng L, Li X, Chen X, Wang X. Simulation of Nonseparable Nonstationary Spatially Varying Ground Motions with an Enhanced Interpolation Approximation Approach. Applied Sciences. 2022; 12(13):6757. https://doi.org/10.3390/app12136757
Chicago/Turabian StyleZhao, Ning, Zhilong Xu, Liuliu Peng, Xiaolong Li, Xiaowei Chen, and Xuewei Wang. 2022. "Simulation of Nonseparable Nonstationary Spatially Varying Ground Motions with an Enhanced Interpolation Approximation Approach" Applied Sciences 12, no. 13: 6757. https://doi.org/10.3390/app12136757
APA StyleZhao, N., Xu, Z., Peng, L., Li, X., Chen, X., & Wang, X. (2022). Simulation of Nonseparable Nonstationary Spatially Varying Ground Motions with an Enhanced Interpolation Approximation Approach. Applied Sciences, 12(13), 6757. https://doi.org/10.3390/app12136757






