Featured Application
The purpose of this work was to provide a detailed derivation process of the 3D analytical solution and TMs of the RDGCs based on the previous studies on the variable ducts and propose a certain reference for designing and improving the acoustic characteristics of the duct systems used in high-speed trains.
Abstract
Rectangular ducts used in the air-conditioning system of a high-speed train should be carefully designed to achieve optimal acoustic and flow performance. However, the theoretical analysis of the rectangular ducts with gradient cross-sections (RDGC) at frequencies higher than the one-dimensional cut-off frequency is rarely published. This paper has developed the three-dimensional analytical solutions to the wave equations of the expanding and shrinking RDGCs. Firstly, a homogeneous second-order variable coefficient differential equation is derived from the wave equations. Two coefficients of the solution to the differential equation are set to zero to ensure convergence. Secondly, the transfer matrices of the duct systems composed of multiple RDGCs are derived from the three-dimensional solutions. The transmission losses of the duct systems are then calculated from the transfer matrices and validated with the measurement. Finally, the acoustic performance and flow efficiency of the RDGCs with different geometries are discussed. The results show that the REC with double baffles distributed transversely has good performance in both acoustic attenuation and flow efficiency. This study shall provide a helpful guide for designing rectangular ducts used in high-speed trains.
1. Introduction
Rectangular ducts are used in the air-conditioning systems of high-speed trains+ to guide airflow. Some rectangular ducts adopt the oblique baffles (Figure 1) to form the varying cross-sections which improve the performance of the ducts in noise attenuation [].

Figure 1.
Rectangular ducts used in a Fuxing bullet train.
The current studies on the duct acoustics of high-speed train are practiced based on simulation, such as the hybrid method of finite element and statistical energy analysis (FE-SEA) [,]. Most scholars have focused their research on ducts suitable for any vehicle based on a variety of methods. Both analytical and numerical methods have been widely used in the field of duct acoustics. Generally, the numerical methods, such as the finite element method (FEM) [,,,], boundary element method (BEM) [] and computational fluid dynamics (CFD) method [,,], are popular for analyzing duct systems with complex geometries. Assis et al. [] proposed a spectral FEM approach to compute the transfer matrix (TM) of duct systems with arbitrary geometries. Liu et al. [] proposed the time domain CFD approaches to predict the acoustic performance of the duct systems without and with mean flow.
On the other hand, the analytical method is more efficient in computation than the numerical methods. Two types of analytical methods have been used to investigate a duct system with varying cross–sections. The Wentzel–Kramers–Brillouin (WKB) method [,,,,] utilizes the high frequency approximation that allows to neglect certain terms in the nonlinear governing equations of the media in a duct. Subrahmanyam et al. [] used the WKB approximation to derive the exact solutions for one-dimensional (1D) ducts with area variations in the absence of mean flow. Rani et al. [,] derived a WKB-type solution to the generalized Helmholtz equation in 1D ducts with nonuniform cross-sectional areas and inhomogeneity in mean flow. The WKB approximation is less accurate than solving the full wave equations of the duct. The solutions to the wave equations of 1D ducts with varying cross-sections have been studied for decades [,,,,,,,]. Pillai et al. [] developed the 1D solution to a horn-like rectangular duct at frequencies lower than 250 Hz. However, the three-dimensional (3D) solutions to the wave equations in rectangular ducts with gradient cross-sections (RDGCs), which are more accurate at higher frequencies than the 1D solutions, are rarely seen.
The objective of this paper is to develop the 3D analytical solutions to the wave equations of the expanding or shrinking RDGCs at frequencies up to 1600 Hz. The derived solutions are used to obtain the TMs and transmission losses (TLs) of the duct systems consisted of RDGCs, which have been validated with the measured results. Lastly, the effects of the RDGC geometries on the acoustic performance and flow efficiency of the duct systems are discussed.
The organization of this paper is as follows: Section 2 develops the 3D analytical solutions to the wave equations of RDGCs. In Section 3, the TMs of the RDGCs are derived. In Section 4, several duct systems consisting of RDGCs are modeled to obtain the TMs. The TLs and pressure losses of the duct systems with different RDGC geometries are obtained and discussed in Section 5. Finally, the conclusions are presented in Section 6.
2. 3D Analytical Solutions to the Wave Equations of a RDGC
2.1. 3D Solutions for a Straight Rectangular Duct
Figure 2 shows a uniform rectangular duct with a width of b and a height of h. The 3D wave equation of the rectangular duct is given by []
where p, t and c are the sound pressure, advancing time and sound velocity, respectively. The Laplacian operator is given as follows:
where x, y and z are the Cartesian coordinates shown in Figure 2.
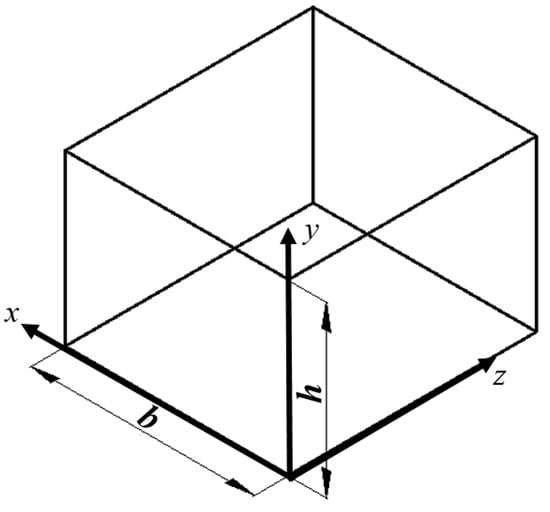
Figure 2.
A straight rectangular duct.
The general solution of Equation (1) [] is
where j is the imaginary unit. , , and are the coefficients to be determined with boundary conditions. and are the wave numbers in the x and y direction, respectively. is defined as
where and is the angular frequency. The solution of the rigid-walled duct with a width of b and a height of h is given by
where and . Here, is an eigenfunction representing the wave shape in the x-y plane at the (m, n) mode. and are the amplitudes of the waves at the (m, n) mode propagating in the positive and negative z directions.
2.2. 3D Solutions for a RDGC
A RDGC with either expanding (positive ) or shrinking (negative ) sections is shown in Figure 3. is the angle between the bevel edge and the z axis. and are the widths of the inlet and the outlet, respectively.
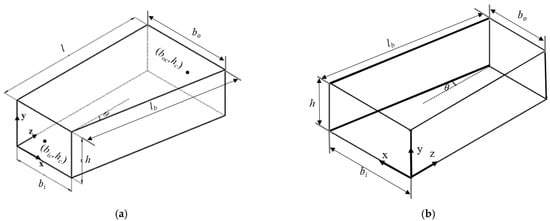
Figure 3.
(a) A rectangular duct with expanding sections, (b) a rectangular duct with shrinking sections.
Since the cross-sectional area S changes along the z direction, the 1D sound wave equation in the z direction is obtained with modifying Equation (1) as
For an expanding duct, S is given by
Substituting Equation (7) into Equation (6) yields a homogeneous second-order variable coefficient differential equation as follows:
The solution of Equation (8) is given by []
where . The quantities , , and are the corresponding amplitudes. is the zeroth order of the Bessel function of the first kind, and is the zeroth order of the modified Bessel function of the second kind []. When becomes imaginary, the values of and are possible to be infinite. To converge the solution, and are set to zero. As a result, Equation (9) is simplified as
Substituting Equation (10) into Equation (5), with b replaced by , the 3D solution of an expanding RDGC is derived as
where and are the coefficients to be determined with boundary conditions.
Solve the momentum equation []
to give the particle velocity of an expanding RDGC as follows:
where is the ambient air density and is the first order of the modified Bessel function of the second kind.
The derivation of the solutions for a shrinking RDGC is similar to that of an expanding RDGC and gives the same equations of Equations (11) and (14) with a negative .
3. Derivation of the TM for a RDGC
The transfer matrix T () is used to describe the relationship as follows:
where and are the average particle velocities at the inlet and outlet of a duct system. The quantities and are the inlet and outlet average sound pressures defined with
where is the length of the duct. The quantities and are the inlet and outlet cross-sectional areas, respectively. The four elements of the TM can be obtained as follows:
The transfer matrix T′ () of the shrinking RDGC is derived from the T with a negative .
4. The TMs and TLs of Rectangular Expansion Chambers (RECs)
4.1. The TMs of the RECs with One or Double Baffles
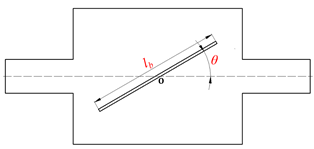
To simplify the setup of a validation experiment, the circular ducts with a diameter of 50 mm are added at the inlet and outlet of the rectangular chamber with a height (h) of 150 mm. The dimensions of the REC with one baffle are shown in Figure 4. The dimensional parameters of REC are designed according to those of the branch rectangular ducts in high-speed trains. The center o of the baffle coincides with that of the REC. All the RECs in the following sections have the same circular ducts and rectangular chamber as those in Figure 4.
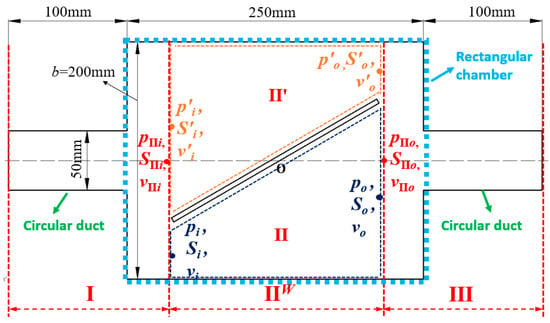
Figure 4.
The gemometry of the REC with one baffle.
The components of the REC with one baffle are specified in Table 1.

Table 1.
Description of each component of the REC with one baffle.
The transfer matrices ( and ) of the expanding RDGC and the shrinking RDGC are given by
According to the notation in Figure 4, the state variables at the two ends of each RDGC are related by
The continuity of pressure and mass velocity at the inlet and outlet of the component gives
where ′ denote the variables of the shrinking RDGC. and represent the cross-sectional areas at the inlet and outlet of the component , respectively. Solving simultaneously Equations (21)–(23) yields []
The terms of the TM are given by
The TM of the REC with one baffle is calculated with
where and are calculated with the analytical solutions in the refs. [,].
The cut-off frequencies at the mode (m, n) of a duct with a rectangular section can be calculated with
Table 2 shows the calculated cut-off frequencies with of the rectangular chamber (Figure 4). The maximum cut-off frequency at (2, 2) mode is 2858.3 Hz. As a result, the number of modes with is enough to investigate the acoustic characteristics of the RECs at frequencies below 1600 Hz.

Table 2.
Modal frequencies (Hz) of the rectangular chamber.
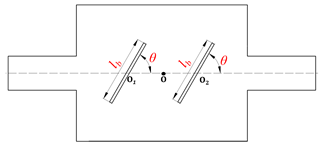
Figure 5 and Figure 6 show the geometries of the RECs with double baffles distributed either axially or transversely. The distance between the centers ( and ) of the baffles is 100 mm. The and in Figure 5 and Figure 6 are exactly the same as those in Figure 4. and in Figure 5 are calculated with the Equation (25), while the of the straight rectangular duct is obtained with the analytical solutions in the ref. [].
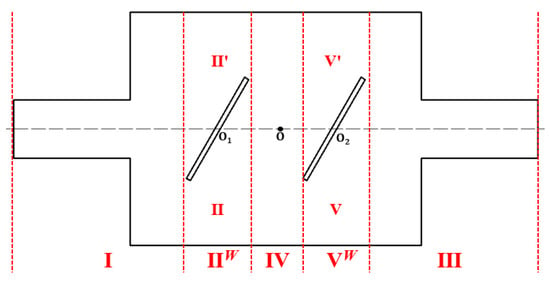
Figure 5.
The REC with double baffles distributed axially.
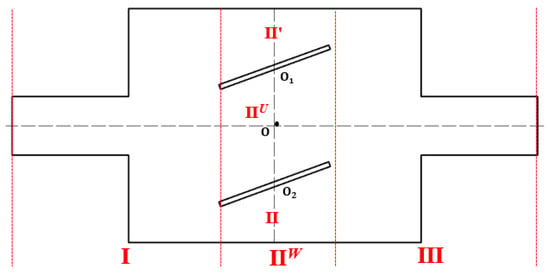
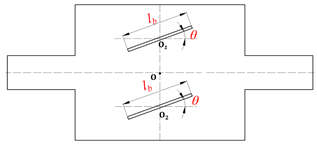
Figure 6.
The REC with double baffles distributed transversely.
The transfer matrices (, and ) of the expanding RDGC, the shrinking RDGC and the uniform duct () in Figure 6 are given by
The transfer matrix () of the component is calculated with a similar process presented in the Equations (21)–(24) and the four elements are given by
where and are the cross-sectional areas of the inlet and outlet of the component IIU. The TM () of the REC with double baffles distributed axially and the TM () with double baffles distributed transversely are given by
4.2. Geometries of the RECs
Table 3 shows the geometries of the RECs with different baffle configurations. All the baffles in the RECs have a thickness of 4 mm.

Table 3.
The geometries of the RECs with different baffle configurations.
4.3. Calculation and Measurement of the TLs for the RECs
The TL of a REC can be calculated from the derived TM as follows:
To verify the accuracy of the analytical method, the TLs of the RECs (Case 1-1, Case 2a-1 and Case 2t-1) were measured with the two-load method [] shown in Figure 7. The sound source was located at the outside of a semi-anechoic room where a REC with baffles was located. An acoustic stimulus was introduced into the REC through a metal duct, where two microphones were placed with a distance of 40 mm. The other two microphones were located with the same distance at the duct connected to the outlet of the REC. A Brüel & Kjær (B&K) 3560 C Module was adopted to acquire the data sampled with a frequency of 16,384 Hz. The experimental parameters are given in Table 4.
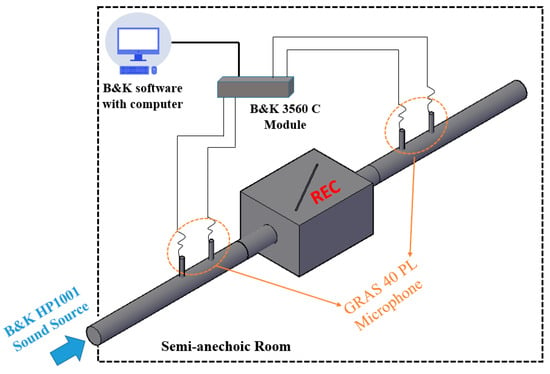
Figure 7.
Experimental setup for measuring the TL of a REC.

Table 4.
Experimental parameters.
5. Results
5.1. Experimental Validation of the Calculated Results
In order to verify the 3D analytical method, the TLs obtained by experiment, 3D analytical method and FEM are shown in Figure 8. The FEM model and boundary conditions are shown in Appendix B. Generally, the calculated results are in good agreement with the measured results and the accuracy of the analytical method is validated to a certain degree. However, the frequencies of TL peaks from FEM agree less with the experimental results than those obtained by the 3D analytical method in this paper. The inaccuracy of the measured TLs below 200 Hz shown in the Case 1-1 of Figure 8 should be attributed to the insufficient energy of the sound source in this frequency range. As a result, the TLs below 200 Hz are not presented in the other cases of Figure 8. The discrepancies between the experiment and the 3D analytical method at higher frequencies may be caused by the following reasons. First, the analytical method regards the REC as rigid, while the prototypes under test are made of plastic with certain elasticity. Second, the damping in the air is ignored with the analytical method. Third, the ignorance of the , in the Equation (9) and the in Equation (14) also causes errors.
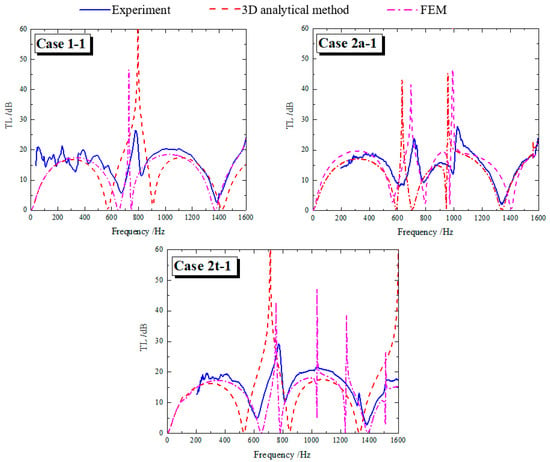
Figure 8.
The comparison of TLs obtained by experiment, 3D analytical method and FEM.
5.2. TLs of the RECs
The TLs of the RECs with one or double baffles are shown in Figure 9 and Figure 10. It can be seen that the peaks and troughs of the TL curves of all types move to a lower frequency with the decreasing θ and increasing lb. Although the Type 2a has more TL peaks, it is worse in performance than the Type 2t at frequencies from 500 Hz to 1100 Hz. Generally, the Type 2t is better in acoustic performance than the other types, especially at frequencies from 600 Hz to 1100 Hz.
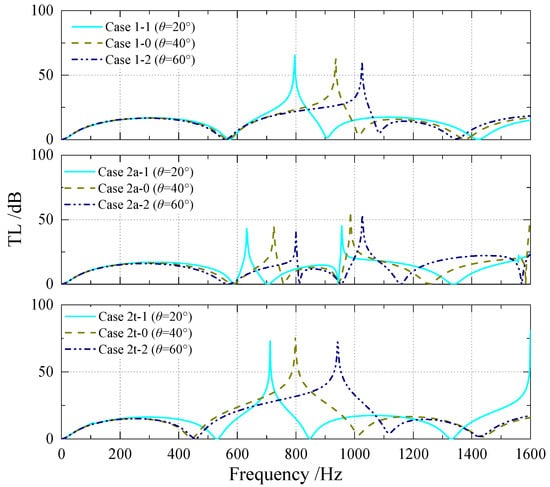
Figure 9.
TLs of the RECs with different baffle angles.
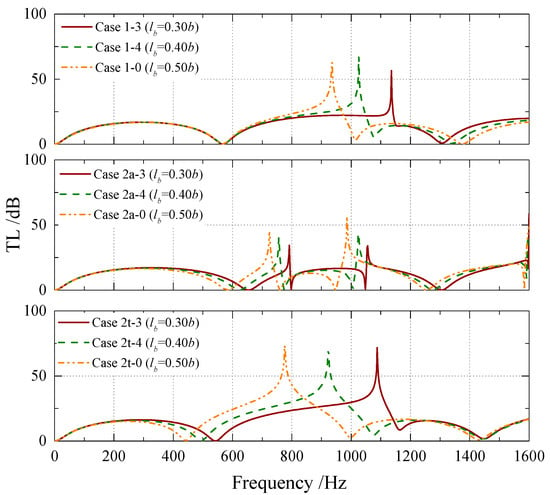
Figure 10.
TLs of the RECs with different baffle lengths.
5.3. Pressure Losses of the RECs
The improvement of the acoustic performance of a duct system cannot be at the expense of flow efficiency. A CFD model [] (Figure 11), using the standard k-ɛ turbulence model, is adopted to calculate the difference (pressure loss) between the area-weighted average pressures at the inlet and outlet of the RECs. The model is discretized by about one million unstructured tetrahedral meshes with a mesh size of 3 mm. The inlet has a velocity of 10 m/s and the outlet has a zero gauge pressure.

Figure 11.
CFD model of the REC with one baffle.
Table 5 shows the pressure losses of the RECs with one baffle or double baffles. It can be seen that the pressure losses of the Case 1-0 and Case 2a-0 are higher than the Case 2t-0. Therefore, the REC with double baffles distributed transversely (Type 2t) has good performance in both flow efficiency and TL.

Table 5.
The pressure losses of the RECs with different baffle configurations.
The influence of the baffle angles and lengths on the pressure losses of the RECs are shown in Figure 12 and Figure 13. Figure 12 shows that the pressure losses of Type 1 and Type 2t increase as θ increases, while the pressure loss of Type 2a decreases as θ increases from 40° to 60°. Figure 13 shows that Type 1 and Type 2a have much higher pressure losses than Type 2t, while the pressure loss of Type 2t increases faster than Type 1 and Type 2a as lb increases. In general, Type 2t has better performance in flow efficiency than the other types, especially at small values of θ and lb.
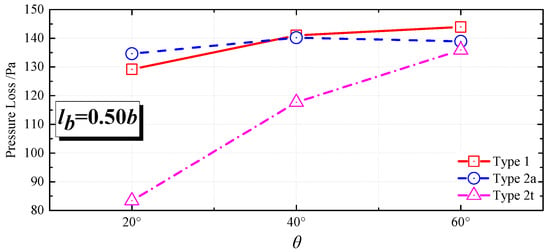
Figure 12.
The pressure losses of the RECs with different baffle angles.
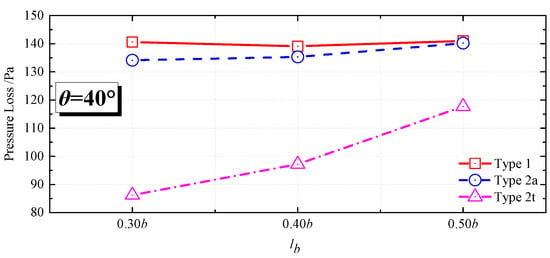
Figure 13.
The pressure losses of the RECs with different baffle lengths.
6. Conclusions
Here, the 3D analytical solutions to the wave equations of the expanding and shrinking RDGCs were derived. The TMs of the RECs consisted of multiple expanding and shrinking RDGCs, which were then calculated from the 3D solutions. The TLs calculated from the TMs were validated with the measured results.
In the derivation of the 3D analytical solution and TMs of the RDGC, the ignorance of some infinite and complex terms is risky, but it can simplify the formulas and reduce the computation. These behaviors are proved to be practicable by experiments and the TLs obtained by the theories in this paper are accurate to a certain extent.
According to the TLs of the RECs with different baffle configurations, the peaks and troughs of the TL curves of all types move to a higher frequency with the increasing angle (θ) between the bevel edge and the axial direction and move to a lower frequency with the increasing length (lb) of the baffle. The REC with double baffles distributed transversely (Type 2t) is better in acoustic performance than the other types at frequencies from 600 Hz to 1100 Hz. On the other hand, although the pressure losses of all types of RECs increase as θ or lb increases, Type 2t always has a lower pressure loss than other types. In summary, Type 2t generally has good performance in both acoustic attenuation and flow efficiency.
This achievement of research shall provide a certain reference for designing and improving the acoustic characteristics of the duct systems used in high-speed trains.
Author Contributions
This article was prepared through the collective efforts of all the authors. Conceptualization, methodology, and writing—original draft preparation, Y.S.; validation, Y.S. and L.L.; writing—review and editing, Y.Q. and X.Z. All authors have read and agreed to the published version of the manuscript.
Funding
This research was supported by the National Natural Science Foundation of China (Grant No. 51975515 and No. 51905474).
Institutional Review Board Statement
The study did not involve humans or animals.
Informed Consent Statement
The study did not involve humans.
Data Availability Statement
The data that support the findings of this study are available from the corresponding author upon reasonable request.
Conflicts of Interest
The authors declared no potential conflict of interest with respect to the research, authorship and/or publication of this article.
Appendix A. Derivation of the A, B, C and D in the TM
We set and as and 0, respectivley, where is the harmonic excitation with a constant amplitude. As a result, and are also equal to and 0. To simplify the expression, is denoted as . Substituting into Equation (14) and eliminating the yields
The term in Equation (14) is ignored here, because it is too complicated to derive a concise solution. The rationality of this mathematical operation has been verified by the following experimental results.
Substituting into Equation (14) and ignoring the term yields
The quantities and represent the at z = 0 and z = l, respectively. and represent the at z = 0 and z = l, respectively.
In order to obtain the coefficients and , operating the both sides of Equation (A1) with [] yields
According to the orthogonality property of eigenfunctions, Equation (A3) is transformed with and to the following equation:
The coefficients and can be calculated from Equations (A2) and (A4) as follows:
where
Substituting Equations (A5) and (A6) into Equation (11), the 3D solution of the pressure is obtained as follows:
where
Substituting Equation (A8) with z = 0 into Equation (16) to obtain the average pressure at the inlet as follows:
where
The quantity is obtained with and . is obtained with and . is obtained with and . Additionally, is obtained with and . are the coordinates of the center point at the inlet shown in Figure 3.
Substituting Equation (A8) with z = l into Equation (17) to obtain the average pressure at the outlet as follows:
where
The quantity is obtained with and . is obtained with and . is obtained with and . Additionally, is obtained with and . are the coordinates of the center point at the outlet in Figure 3.
The elements A and C can be calculated from Equations (18), (A10) and (A14) with the following equations
We set and as 0 and , respectively, and obtain and . Substituting into Equation (14) yields
Substituting into Equation (14) yields
Operating the both sides of Equation (A21) with and using the same transformation with and as in Equation (A3) yields
The coefficients and can be obtained from Equations (A20) and (A22) as follows:
Substituting Equations (A23) and (A24) into Equation (11), the 3D solution of the pressure is obtained as follows:
where
Substituting Equation (A25) with z = 0 into Equation (16) to obtain the average pressure at the inlet as follows:
where
The quantity is obtained with and . is obtained with and . is obtained with and . Additionally, is obtained with and .
Substituting Equation (A25) and z = l into Equation (17) to obtain the average pressure at the outlet as follows:
where
The quantity is obtained with and . is obtained with and . is obtained with and . Additionally, is obtained with and . The elements B and D can be calculated from Equations (19), (A18), (A19), (A27) and (A29) with the following equations
Appendix B. FEM Methodology
Figure A1 shows the FEM model used the automatic matched layer (AML) method [] in LMS Virtual.Lab software to calculate TL. Tetrahedral mesh (2 mm) was used to guarantee the calculation accuracy and the total number of grid cells of every REC was more than 400,000. The fluid material among the REC was defined as air, whose velocity was 340 m/s and density was 1.225 kg/m3. Then, the outlet was AML property, which could simulate the nonreflecting boundary condition. The inlet acoustic boundary condition was defined as the plane wave with 1 W sound power.
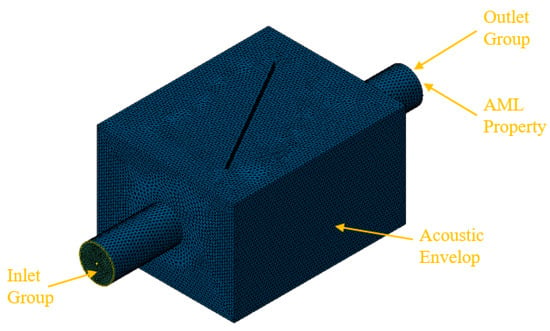
Figure A1.
The FEM model of the REC.
References
- Sun, Y.H.; Zhang, J.; Han, J.; Gao, Y.; Xiao, X.B. Acoustic transmission characteristics and optimum design of the wind ducts of high-speed train. J. Mech. Eng. Chin. Ed. 2018, 54, 129–137. (In Chinese) [Google Scholar] [CrossRef]
- Sun, Y.H.; Zhang, J.; Han, J.; Gao, Y.; Xiao, X.B. Sound transmission characteristics of silencer in wind ducts of high-speed train. J. Zhejiang Univ. Eng. Sci. 2019, 53, 1389–1397. (In Chinese) [Google Scholar]
- Gopalakrishnan, S.; Raut, M.S. Longitudinal wave propagation in one-dimensional waveguides with sinusoidally varying depth. J. Sound Vib. 2019, 463, 114945. [Google Scholar] [CrossRef]
- Assis, G.F.C.A.; Beli, D.; Miranda, E.J.P., Jr.; Camino, J.F.; Dos Santos, J.M.C.; Arruda, J.R.F. Computing the complex wave and dynamic behavior of one-dimensional phononic systems using a state-space formulation. Int. J. Mech. Sci. 2019, 163, 105088. [Google Scholar] [CrossRef]
- Liu, J.; Wang, T.; Chen, M. Analysis of sound absorption characteristics of acoustic ducts with periodic additional multi-local resonant cavities. Symmetry 2021, 13, 2233. [Google Scholar] [CrossRef]
- Terashima, F.J.H.; de Lima, K.F.; Barbieri, N.; Barbieri, R.; Filho, N.L.M.L. A two-dimensional finite element approach to evaluate the sound transmission loss in perforated silencers. Appl. Acoust. 2022, 192, 108694. [Google Scholar] [CrossRef]
- Junge, M.; Brunner, D.; Walz, N.-P.; Gaul, L. Simulative and experimental investigations on pressure-induced structural vibrations of a rear muffler. J. Acoust. Soc. Am. 2010, 128, 2782–2791. [Google Scholar] [CrossRef]
- Liu, L.L.; Zheng, X.; Hao, Z.Y.; Qiu, Y. A computational fluid dynamics approach for full characterization of muffler without and with exhaust flow. Phys. Fluids 2020, 32, 066101. [Google Scholar]
- Liu, L.L.; Hao, Z.Y.; Zheng, X.; Qiu, Y. A hybrid time-frequency domain method to predict insertion loss of intake system. J. Acoust. Soc. Am. 2020, 148, 2945. [Google Scholar] [CrossRef]
- Liu, L.L.; Zheng, X.; Hao, Z.Y.; Qiu, Y. A time-domain simulation method to predict insertion loss of a dissipative muffler with exhaust flow. Phys. Fluids 2021, 33, 067114. [Google Scholar] [CrossRef]
- Tolstoy, I. The WKB approximation, turning points, and the measurement of phase velocities. J. Acoust. Soc. Am. 1972, 52, 356–363. [Google Scholar] [CrossRef]
- Subrahmanyam, P.B.; Sujith, R.I.; Lieuwen, T.C. A family of exact transient solutions for acoustic wave propagation in inhomogeneous, non-uniform area ducts. J. Sound. Vib. 2001, 240, 705–715. [Google Scholar] [CrossRef]
- Li, J.; Morgans, A.S. The one-dimensional acoustic field in a duct with arbitrary mean axial temperature gradient and mean flow. J. Sound. Vib. 2017, 400, 248–269. [Google Scholar] [CrossRef]
- Rani, V.K.; Rani, S.L. WKB solutions to the quasi 1-D acoustic wave equation in ducts with non-uniform cross-section and inhomogeneous mean flow properties—Acoustic field and combustion instability. J. Sound. Vib. 2018, 436, 183–219. [Google Scholar] [CrossRef]
- Basu, S.; Rani, S.L. Generalized acoustic Helmholtz equation and its boundary conditions in a quasi 1-D duct with arbitrary mean properties and mean flow. J. Sound. Vib. 2021, 512, 116377. [Google Scholar] [CrossRef]
- Eisner, E. Complete solutions of the “Webster” horn equation. J. Acoust. Soc. Am. 1967, 41, 1126–1146. [Google Scholar] [CrossRef]
- Miles, J.H. Verification of a one-dimensional analysis of sound propagation in a variable area duct without flow. J. Acoust. Soc. Am. 1982, 72, 621–624. [Google Scholar] [CrossRef]
- Lee, S.K.; Mace, B.R.; Brennan, M.J. Wave propagation, reflection and transmission in non-uniform one-dimensional waveguides. J. Sound. Vib. 2007, 304, 31–49. [Google Scholar] [CrossRef]
- Martin, P.A. On Webster’s horn equation and some generalizations. J. Acoust. Soc. Am. 2014, 116, 1381–1388. [Google Scholar] [CrossRef] [Green Version]
- Donskoy, D.M. Directionality and gain of small acoustic velocity horns. J. Acoust. Soc. Am. 2017, 142, 3450–3458. [Google Scholar] [CrossRef]
- Pagneux, V.; Amir, N.; Kergomard, J. A study of wave propagation in varying cross-section waveguides by modal decomposition. Part I. Theory and validation. J. Acoust. Soc. Am. 1996, 100, 2034–2048. [Google Scholar] [CrossRef]
- Amir, N.; Pagneux, V.; Kergomard, J. A study of wave propagation in varying cross-section waveguides by modal decomposition. Part II. Results. J. Acoust. Soc. Am. 1997, 101, 2504–2517. [Google Scholar] [CrossRef] [Green Version]
- Maurel, A.; Mercier, J.F.; Pagneux, V. Improved multimodal admittance method in varying cross section waveguides. Proc. Math. Phys. Eng. Sci. 2014, 470, 20130448. [Google Scholar] [CrossRef] [PubMed]
- Pillai, M.A.; Ebenezer, D.D.; Deenadayalan, E. Transfer matrix analysis of a duct with gradually varying arbitrary cross-sectional area. J. Acoust. Soc. Am. 2019, 146, 4435–4445. [Google Scholar] [CrossRef] [PubMed]
- Munjal, M.L. Acoustics of Ducts and Mufflers, 2nd ed.; John Wiley & Sons: New York, NY, USA, 2014. [Google Scholar]
- Polyanin, A.D.; Zaitsev, V.F. Handbook of Exact Solutions for Ordinary Differential Equations; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Fucci, G.; Kirsten, K. Expansion of infinite series containing modified Bessel functions of the second kind. J. Phys. A Math. Theor. 2015, 48, 435203. [Google Scholar] [CrossRef] [Green Version]
- Torregrosa, A.J.; Broatch, A.; Payri, R. A study of the influence of mean flow on the acoustic performance of Herschel–Quincke tubes. J. Acoust. Soc. Am. 2000, 107, 1874–1879. [Google Scholar] [CrossRef]
- Ih, J.G. The reactive attenuation of rectangular plenum chambers. J. Sound. Vib. 1992, 157, 93–122. [Google Scholar] [CrossRef]
- Mechel, F.P. Formulas of Acoustics, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- ASTM E2611-09; Standard Test Method for Measurement of Normal Incidence Sound Transmission of Acoustical Materials Based on the Transfer Matrix Method. ASTM International: West Conshohocken, PA, USA, 2009.
- ANSYS Inc. Ansys Fluent 19.1 Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2018. [Google Scholar]
- Fu, J.; Chen, W.; Tang, Y.; Yuan, W.H.; Li, G.M.; Li, Y. Modification of exhaust muffler of a diesel engine based on finite element method acoustic analysis. Adv. Mech. Eng. 2015, 7, 1–2. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).