Determination and Evaluation of a Three-Wheeled Tilting Vehicle Prototype’s Dynamic Characteristics
Abstract
1. Introduction
2. Three-Wheeled Vehicle Description
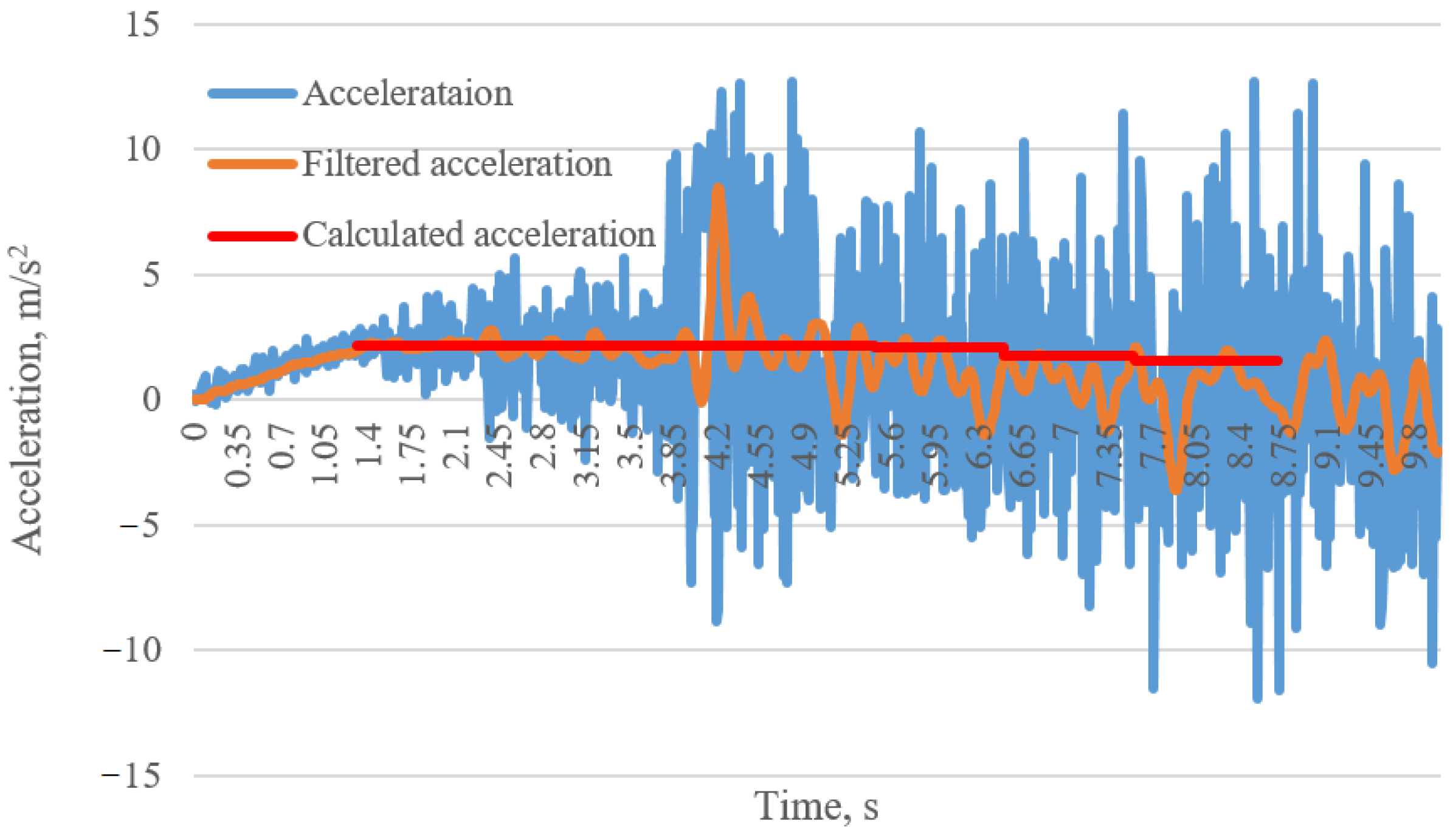
2.1. Theoretical Determination of Acceleration
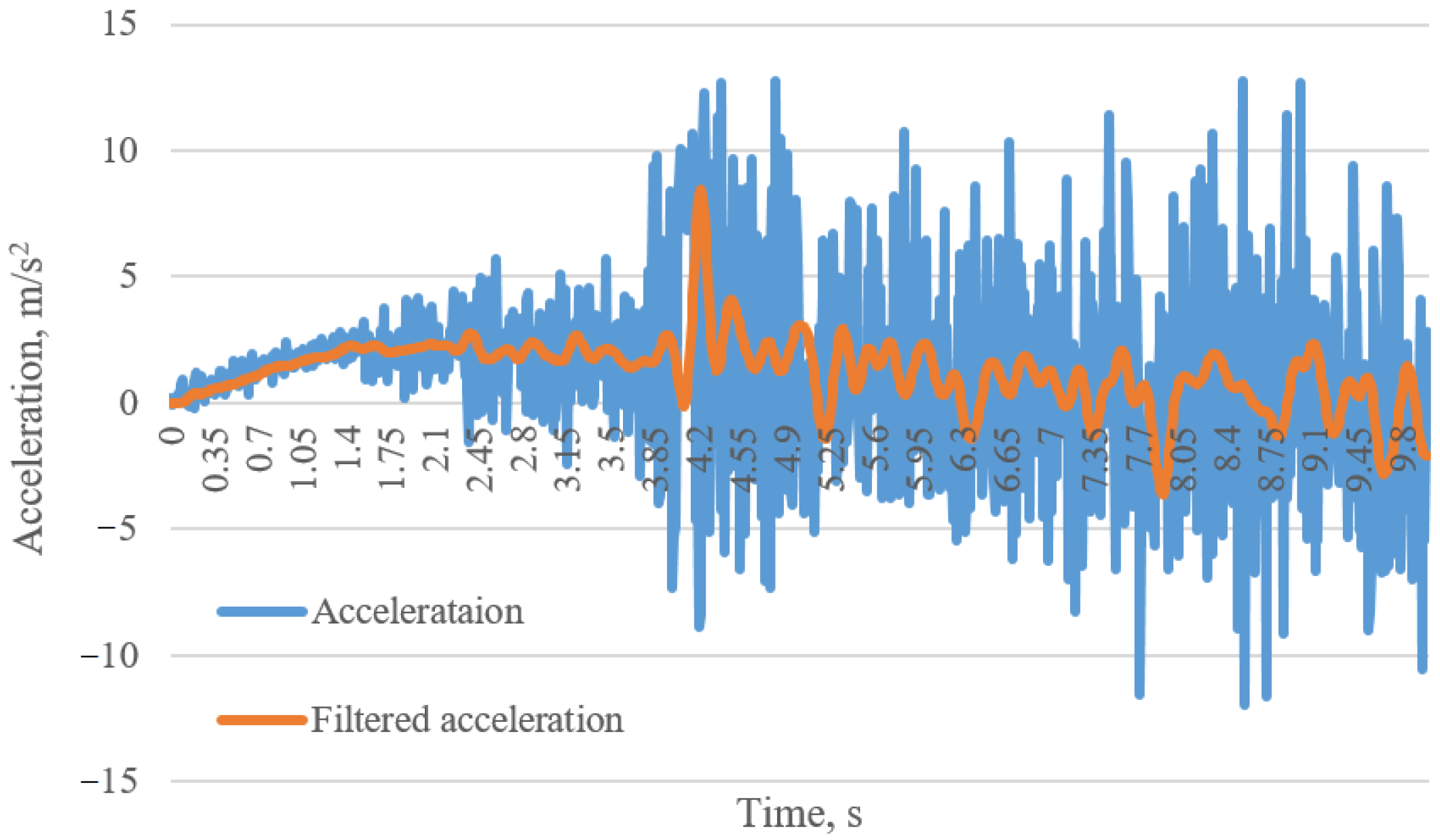
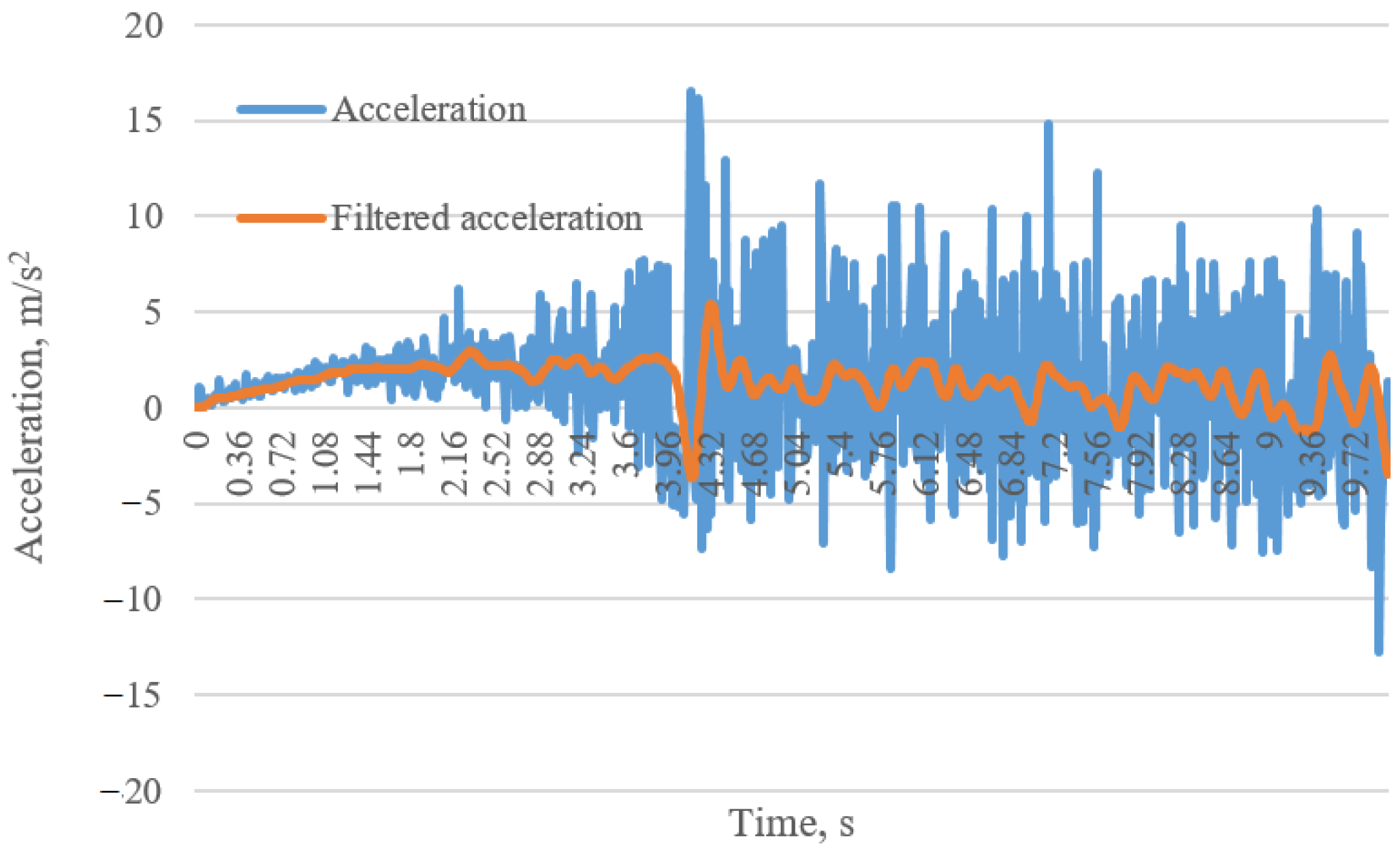
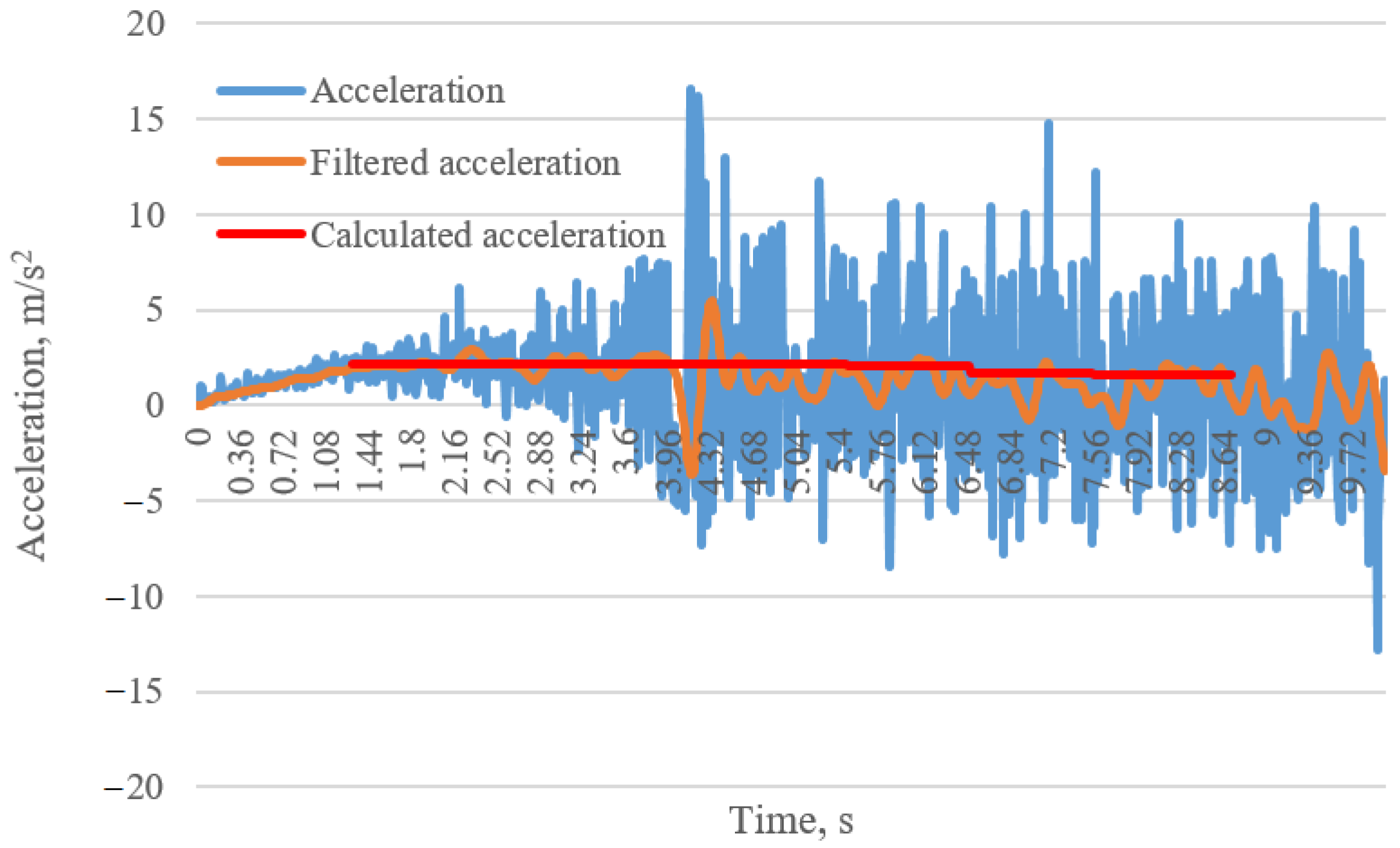
2.2. Experimental Determination of Acceleration
3. Results and Discussion
4. Conclusions and Future Research
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tilting-Three Whelers Reference Web Site. 2022. Available online: http://www.maxmatic.com/ttw_moto.htm (accessed on 24 April 2022).
- Hibbard, R.; Karnopp, D. Twenty first century transportation system solutions—A newtype of small, relatively tall and narrow active tilting commuter vehicle. Veh. Syst. Dyn. 1996, 25, 321–347. [Google Scholar] [CrossRef]
- Amati, N.; Festini, A.; Pelizaa, L.; Tonoli, A. Dynamic moedlling and experimental validations of three sheeld tilting vehicles. Veh. Syst. Dyn. 2011, 49, 889–914. [Google Scholar] [CrossRef]
- Official Mercedes-Benz Group. Media Web Site. 2022. Available online: https://group-media.mercedes-benz.com/marsMediaSite/en/instance/ko/The-curve-master--F-300-Life-Jet.xhtml?oid=9272608 (accessed on 24 April 2022).
- Stark, J.; Neumann, A.; Sammer, G. Proposals for Adapttion of Legal Framwork and Realisation Strategies Compact Low Emission Vehicle for Urban Transport (D11); Technical Report; European Commission: Brussels, Belgium, 2006; 40p. [Google Scholar]
- Carver Technology Web Site. 2022. Available online: http://www.carver-technology.nl/ (accessed on 24 April 2022).
- Piaggio&C. s.p.a., Official MP3 Web Site. 2022. Available online: https://www.piaggio.com/en_EN/models/mp3/ (accessed on 24 April 2022).
- Official Triggo Web Site. 2022. Available online: https://www.triggo.city/ (accessed on 24 April 2022).
- Electrec Official Web Site. 2022. Available online: https://electrek.co/2020/01/07/niu-rqi-gt-electric-motorcycle-unveiled-affordability/ (accessed on 24 April 2022).
- Electrec Official Web Site. 2022. Available online: https://electrek.co/2019/03/19/arcimoto-emergency-delivery-electric-car/ (accessed on 24 April 2022).
- Sato, F.E.K.; Nakata, T. Energy consumption analysis for vehicle production through a material flow approach. Energies 2020, 13, 2396. [Google Scholar] [CrossRef]
- Waseem, M.; Suhaib, M.; Sherwani, A.F. Modelling and analysis of gradient effect on the dynamic performance of three-wheeled vehicle system using Simscape. SN Appl. Sci. 2019, 1, 225. [Google Scholar] [CrossRef]
- Waseem, M.; Sherwani, A.F.; Suhaib, M. Highway Gradient Effects on Hybrid Electric Vehicle Performance. In Smart Cities-Opportunities Challenges; Springer: Singapore, 2020; pp. 583–592. [Google Scholar] [CrossRef]
- Al-jameel, H.A.; Abdulamer, A.H. Effect of three-wheeled vehicle on the capacity of traffic stream. J. Eng. Sustain. Dev. 2021, 3, 165–173. [Google Scholar] [CrossRef]
- Knowles, M.; Scott, H.; Baglee, D. The effect of driving style on electric vehicle perfmornace, economy and perception. Int. J. Electr. Hybrid Veh. 2012, 4, 228–247. [Google Scholar] [CrossRef]
- Bingham, C.; Walsh, C.; Carroll, S. Impact of driving characteristics on electric vehicle energy consuption and range. IET Intell. Transp. Syst. 2012, 6, 29–35. [Google Scholar] [CrossRef]
- Chakraborty, D.; Vaz, W.; Nandi, A.K. Optimal driving during electric vehicle acceleration using evolutionary algorithms. Appl. Soft Comput. 2017, 34, 217–235. [Google Scholar] [CrossRef]
- AASHTO. A Policy on Geometric Design of Highways and Streets, Green Book; American Association of State Highway and Transportation Officials: Washington, DC, USA, 2011. [Google Scholar]
- Bokare, P.S.; Maurya, A.K. Acceleration-deceleration behavior of various vehicle types. Transp. Res. Procedia 2017, 25, 4733–4749. [Google Scholar] [CrossRef]
- Snare, M. Dynamic Model for Predicting Maximum and Typical Acceleration Rates of Passenger Vehicles. Master’s Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2002. [Google Scholar]
- Haas, R.; Inman, V.; Dixson, A.; Warren, D. Use of intelligent transportation system data to determine driver deceleration and acceleration behavior. Transp. Res. Rec. 2004, 1899, 3–10. [Google Scholar] [CrossRef]
- Gattis, J.; Bryant, M.A.; Duncan, L.K. Truck acceleration speeds and distances at weigh stations. Transp. Res. Rec. J. Transp. Res. Board 2010, 2195, 2026. [Google Scholar] [CrossRef]
- Dey, P.P.; Biswas, P. Acceleration of queue leaders at signalized intersections. Indian Highw. 2011, 3, 49–54. [Google Scholar]
- Nimje, R.; Manivasagam, S.; Patil, A. Stability and Handling of a Three Wheeled Personal Vehicle; SAE Technical Paper; SAE International: Pittsburgh, PA, USA, 2021. [Google Scholar] [CrossRef]
- Zhang, F.; Hu, X.; Langari, R.; Cao, D. Energy management strategies of connected HEVs and PHEVs: Recent progress and outlook. Prog. Energy Combust. Sci. 2019, 73, 235–256. [Google Scholar] [CrossRef]
- Miao, C.; Liu, H.; Zhu, G.G.; Chen, H. Connectivity-based optimization of vehicle route and speed for improved fuel economy. Transp. Res. Part C 2018, 91, 353–368. [Google Scholar] [CrossRef]
- Huston, J.C.; Graves, B.J.; Johnons, D.B. Three Wheeld Vehicle Dynamics; SAE Technical Paper 8210139; SAE International: Pittsburgh, PA, USA, 1982. [Google Scholar] [CrossRef]
- Zhang, B.; Zhang, J.; Shen, T. Optimal control design for comfortable-driving of hybrid electric vehicles in acceleration mode. Appl. Energy 2022, 305, 117885. [Google Scholar] [CrossRef]
- Wang, C.; He, H.; Xiong, R.; Zhang, Y. A Novel Efficiency Modeling Method for a DC-DC Converter in the Hybrid Energy Storage System for Electric Vehicles. Energy Procedia 2016, 88, 935–939. [Google Scholar] [CrossRef][Green Version]
- He, H.; Xiong, R.; Guo, H.; Li, S. Comparison study on the battery models used for the energy management of batteries in electric vehicles. Energy Convers. Manag. 2012, 64, 113–121. [Google Scholar] [CrossRef]
- Feng, Y.; Zhang, C. Core Loss Analysis of Interior Permanent Magnet Synchronous Machines under SVPWM Excitation with Considering Saturation. Energies 2017, 10, 1716. [Google Scholar] [CrossRef]
- He, H.; Cao, J.; Cui, X. Energy optimization of electric vehicle’s acceleration process based on reinforcement learning. J. Clean. Prod. 2020, 248, 119302. [Google Scholar] [CrossRef]
- Li, L.; Liu, Q. Acceleration curve optimization for electric vehicle based on energy consumption and battery life. Energy 2019, 169, 1039–1053. [Google Scholar] [CrossRef]
- Liu, H.; Rodgers, M.O.; Guensler, R. The impact of road grade on vehicle accelerations behavior, PM2.5 emissions, and dispersion modeling. Transp. Res. Part D 2019, 75, 297–319. [Google Scholar] [CrossRef]
- Ali, G.; McLaughlin, S.; Ahmadian, M. Quantifying the effect of roadway, driver, vehicle, and location characteristics on the frequency of longitudinal and lateral accelerations. Accid. Anal. Prev. 2021, 161, 106356. [Google Scholar] [CrossRef] [PubMed]
- Shul, Y.; Lim, S.; Moon, S.; Park, N.C. Localization of rattle noise sources in the vehicle underbody using acceleration signals. Mech. Syst. Signal Process. 2022, 166, 108447. [Google Scholar] [CrossRef]
- Official AKO Web Site. 2022. Available online: https://ako.tech/ako-p150/ (accessed on 24 April 2022).
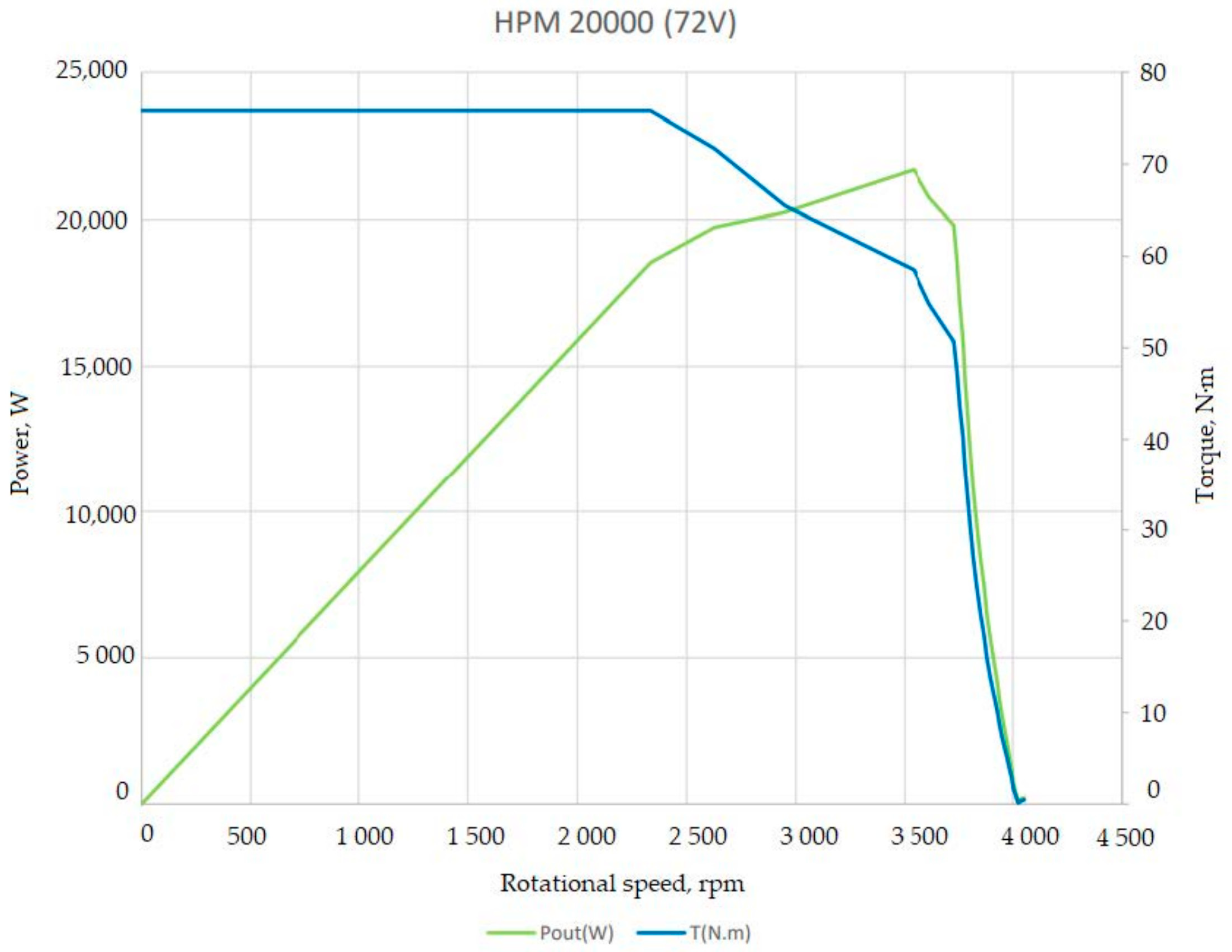
- Official Miromax Website. 2022. Available online: https://www.miromax.lt/userfiles/6/files/HPM20000_72V_Test_Results.pdf (accessed on 24 April 2022).
- Gillespie, T. Fundamentals of Vehicle Dynamics; Society of Automotive Engineers: Pittsburgh, PA, USA, 2000. [Google Scholar]



| Nr. | Parameter | Symbol | Dimension | Value |
|---|---|---|---|---|
| 1. | Gear ratio | ipp | - | 4.365 |
| 2. | Transmission efficiency coefficient | ηtr | - | 0.93 |
| 3. | Three-wheeled tilting vehicle mass with driver | Ga | kg | 486 |
| 3.1. | Mass to drive axle | G2 | kg | 212 |
| 4. | Three-wheeled vehicle mass without driver | G0 | kg | 406 |
| 4.1. | Mass to drive axle | G02 | kg | 177 |
| 5. | Front axle wheel base | Ba | m | 0.892 |
| 6. | Height | Ha | m | 1.6 |
| 7. | Wheels dimensions | B–d | - | R17 170/60 |
| Cycle | Test Number | Hz | Wind Speed, m/s | Air Temperature, °C | Clamping Force, N | Air Resistance Force (Fo), N |
|---|---|---|---|---|---|---|
| 1 | 1 | 30 | 10.6 | 15.6 | 1.2 | 20.2 |
| 2 | 35 | 11.9 | 15.6 | 6.8 | 27.1 | |
| 3 | 40 | 13.3 | 15.6 | 7.8 | 33.6 | |
| 4 | 45 | 14.7 | 15.3 | 9.5 | 38.8 | |
| 5 | 50 | 15.9 | 15.1 | 10.6 | 51.9 | |
| 6 | 55 | 16.1 | 15.6 | 12.8 | 56.9 | |
| 7 | 60 | 16.9 | 15.7 | 23.6 | 60.9 | |
| 8 | 65 | 17.6 | 15.5 | 26.4 | 62.6 | |
| 9 | 70 | 18.3 | 15.6 | 27.8 | 64.5 | |
| 2 | 1 | 30 | 9.2 | 15.4 | 5.8 | 11.9 |
| 2 | 35 | 11.3 | 14.5 | 2.2 | 17.4 | |
| 3 | 40 | 12.9 | 13.6 | 7.5 | 24.2 | |
| 4 | 45 | 13.4 | 13.3 | 15.8 | 33.4 | |
| 5 | 50 | 15.3 | 13.1 | 13.6 | 38.5 | |
| 6 | 55 | 15.9 | 12.9 | 20.7 | 47.6 | |
| 7 | 60 | 16.7 | 12.7 | 24.6 | 56.2 | |
| 8 | 65 | 17.2 | 12.6 | 26.3 | 64.4 | |
| 9 | 70 | 17.4 | 12.7 | 25.8 | 66.7 | |
| 3 | 1 | 30 | 9.3 | 13 | 4.8 | 12.1 |
| 2 | 35 | 10.9 | 12.9 | 9.1 | 19.3 | |
| 3 | 40 | 12.4 | 13 | 11.3 | 26.4 | |
| 4 | 45 | 14.1 | 13 | 9.8 | 34.8 | |
| 5 | 50 | 15 | 12.4 | 14.5 | 41.8 | |
| 6 | 55 | 15.7 | 12.3 | 22.4 | 49.5 | |
| 7 | 60 | 17 | 12.6 | 24.8 | 57.4 | |
| 8 | 65 | 17.3 | 12.5 | 26 | 62.8 | |
| 9 | 70 | 18,5 | 14.7 | 28 | 64.4 | |
| 4 | 1 | 30 | 9.7 | 14.3 | 8.5 | 12.9 |
| 2 | 35 | 11 | 14.3 | 9.5 | 17 | |
| 3 | 40 | 12.3 | 15 | 10.6 | 24.4 | |
| 4 | 45 | 13.6 | 14.6 | 14.2 | 29.6 | |
| 5 | 50 | 14.6 | 14.6 | 13.3 | 40.9 | |
| 6 | 55 | 15.9 | 15.5 | 23.5 | 43.6 | |
| 7 | 60 | 17.2 | 14.2 | 22.9 | 56.6 | |
| 8 | 65 | 17.6 | 14.4 | 26.9 | 60.6 | |
| 9 | 70 | 18.1 | 14.9 | 29.8 | 62.5 |
| Parameter | Values | ||||||
|---|---|---|---|---|---|---|---|
| va, m/s | 3.55 | 7.09 | 10.64 | 14.19 | 17.74 | 21.28 | 24.83 |
| Fr, N | 1142.66 | 1142.66 | 1142.66 | 1142.66 | 1127.625 | 962.24 | 902.1 |
| Fo, N | 1.22 | 4.88 | 10.98 | 19.50 | 30.48 | 43.90 | 59.73 |
| D | 0.2394 | 0.2386 | 0.2374 | 0.2356 | 0.2301 | 0.1926 | 0.1767 |
| j, m/s2 | 2.19 | 2.18 | 2.17 | 2.15 | 2.10 | 1.73 | 1.58 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Navikas, D.; Pitrėnas, A. Determination and Evaluation of a Three-Wheeled Tilting Vehicle Prototype’s Dynamic Characteristics. Appl. Sci. 2022, 12, 5121. https://doi.org/10.3390/app12105121
Navikas D, Pitrėnas A. Determination and Evaluation of a Three-Wheeled Tilting Vehicle Prototype’s Dynamic Characteristics. Applied Sciences. 2022; 12(10):5121. https://doi.org/10.3390/app12105121
Chicago/Turabian StyleNavikas, Deividas, and Aurelijus Pitrėnas. 2022. "Determination and Evaluation of a Three-Wheeled Tilting Vehicle Prototype’s Dynamic Characteristics" Applied Sciences 12, no. 10: 5121. https://doi.org/10.3390/app12105121
APA StyleNavikas, D., & Pitrėnas, A. (2022). Determination and Evaluation of a Three-Wheeled Tilting Vehicle Prototype’s Dynamic Characteristics. Applied Sciences, 12(10), 5121. https://doi.org/10.3390/app12105121






