Analytical Solutions of the Diffusion–Wave Equation of Groundwater Flow with Distributed-Order of Atangana–Baleanu Fractional Derivative
Abstract
1. Introduction
2. The Formulation of the Generalized Problem
- (1)
- for an unconfined aquifer, the diffusivity is
- (2)
3. Solution to the Problem
3.1. The Case
3.2. The Case (The Ordinary Case)
3.3. The Case
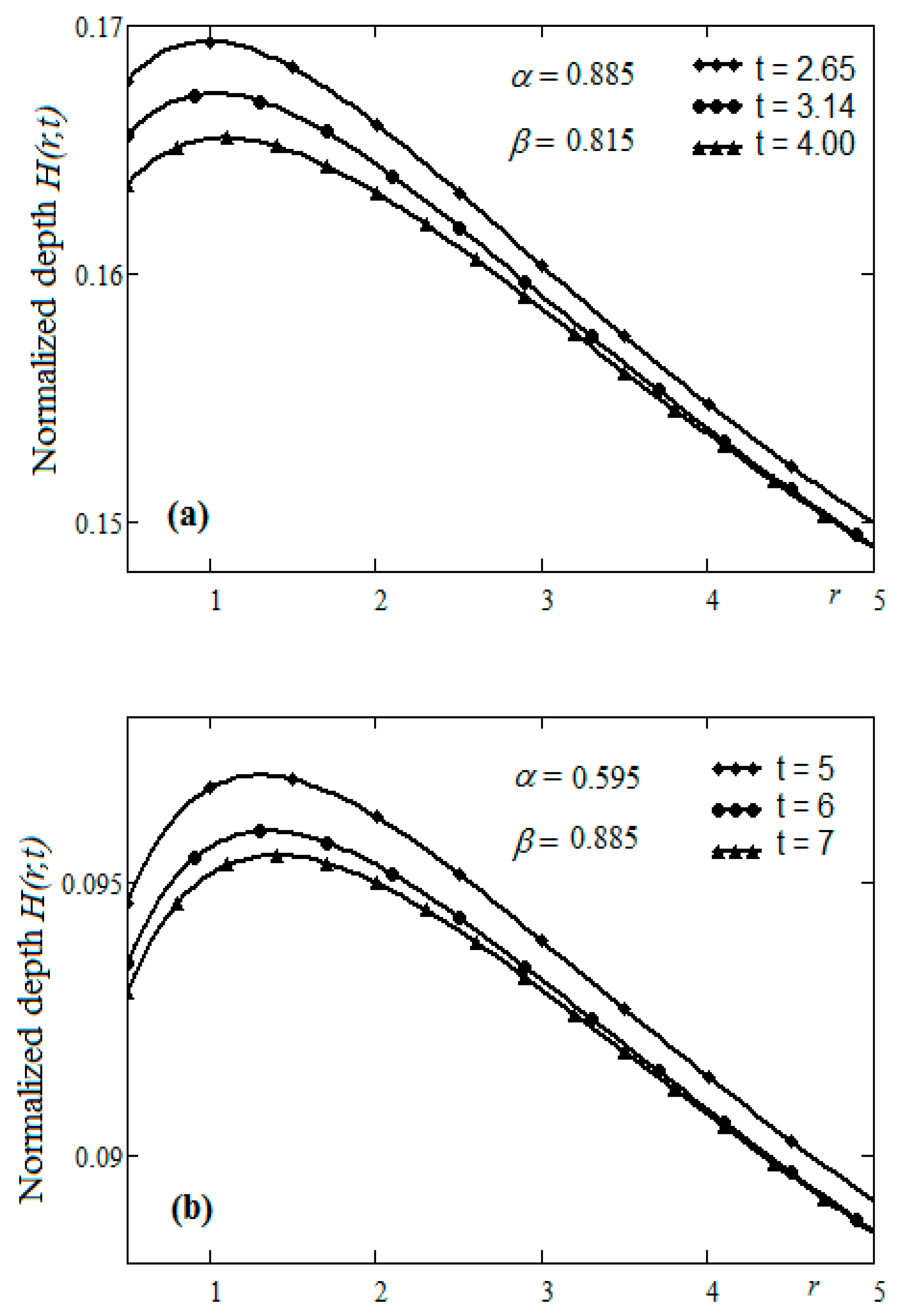
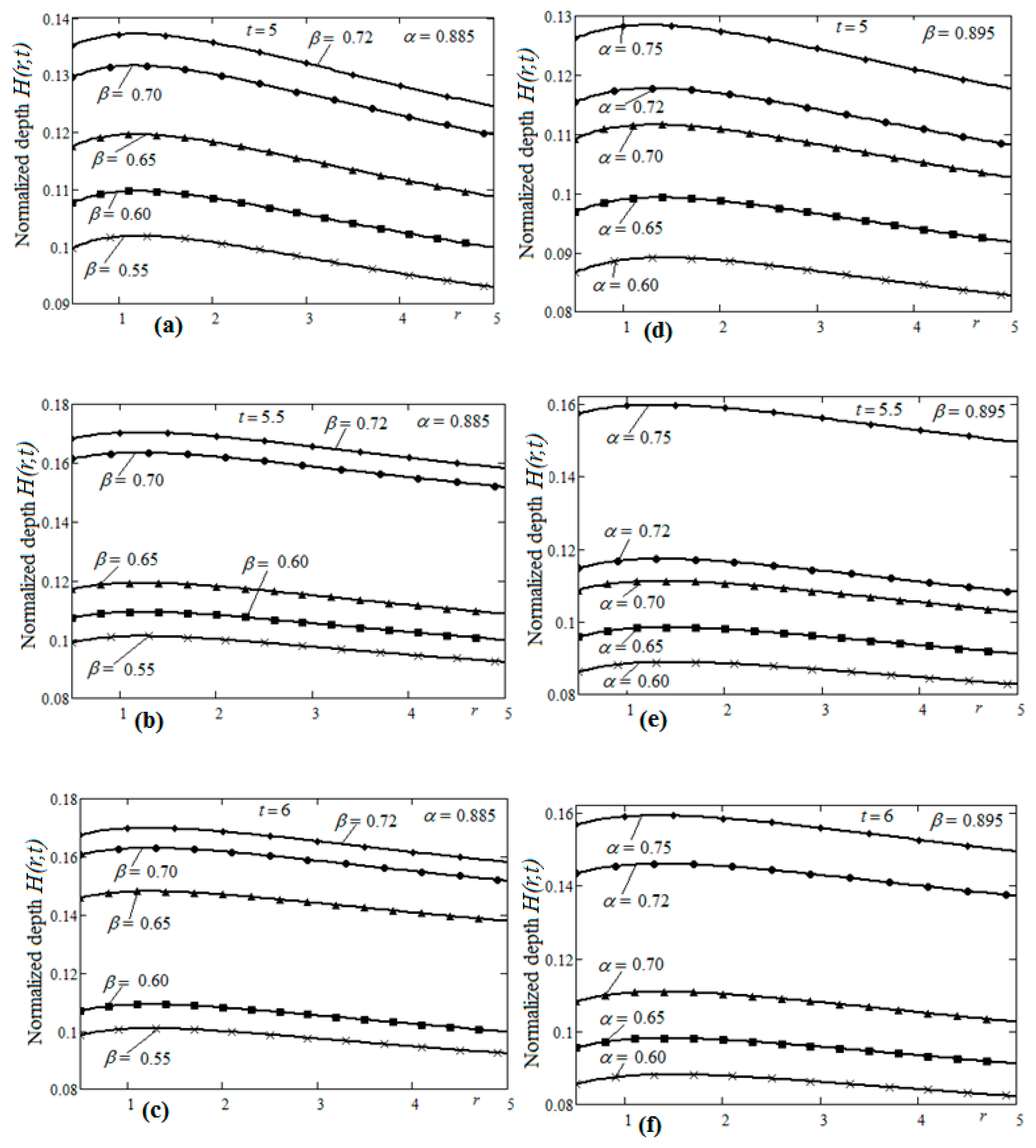
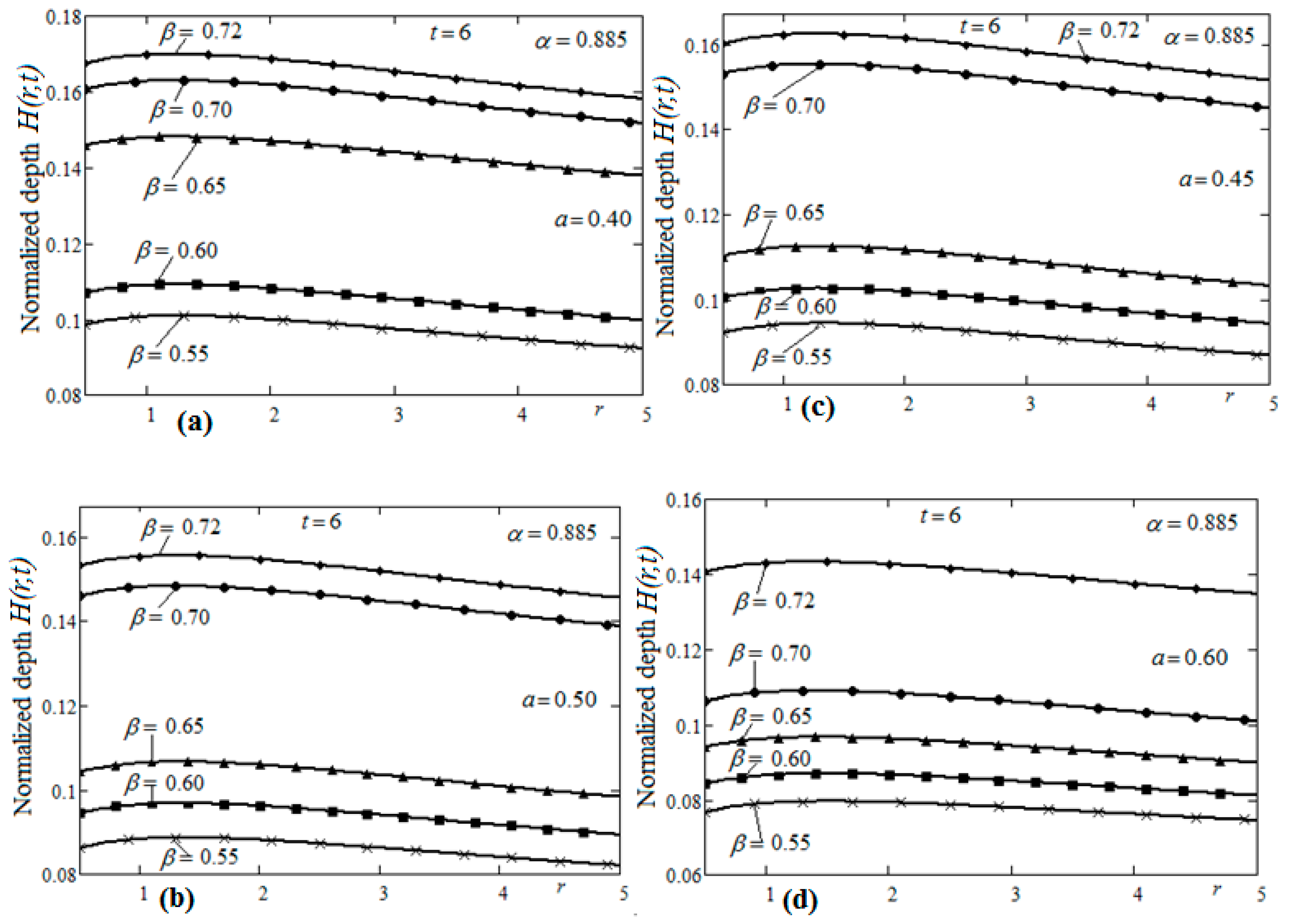
4. Conclusions and Discussions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Haitjema, H.M. Analytic Element Modeling of Groundwater Flow; Academic Press. Inc.: San Diego, CA, USA, 1995. [Google Scholar]
- Batu, V. Aquifer Hydraulics: A Comprehensive Guide to Hydrogeologic Data Analysis; John Wiley & Sons: New York, NY, USA, 1998. [Google Scholar]
- Black, B. Cross-hole investigation-the method, theory and analysis of crosshole sinusoidal pressure tests in fissured rock. Stripa Proj. IR 86-03. 1986. Available online: https://ci.nii.ac.jp/naid/10004123831/ (accessed on 3 February 2021).
- Botha, J.F.; Verwey, J.P.; Van der Voort, I.; Vivier, J.J.P.; Colliston, W.P.; Loock, J.C. Karoo Aquifers. In Their Geology, Geometry and Physical Behavior WRC Report No 487/1/98; Water Research Commission: University of the Free State: Bloemfontein, South Africa, 1998; pp. 75–92. [Google Scholar]
- Van Tonder, G.J.; Botha, J.F.; Chiang, W.H.; Kunstmann, H.; Xu, Y. Estimation of the sustainable yields of boreholes in fractured rock formations. J. Hydrol. 2001, 241, 70–90. [Google Scholar] [CrossRef]
- Barker, J.A. A generalized radial flow model for hydraulic tests in fractured rock. Water Resour. Res. 1988, 24, 1796–1804. [Google Scholar] [CrossRef]
- Cloot, A.; Botha, J.F. A generalised groundwater flow equation using the concept of non-integer order derivatives. Water SA 2006, 32, 1–7. [Google Scholar] [CrossRef][Green Version]
- Butzer, P.L.; Westphal, U. An introduction to fractional calculus. Appl. Fract. Calc. Phys. World Sci. 2000, 1–85. [Google Scholar] [CrossRef]
- Metzler, R.; Glöckle, W.G.; Nonnenmacher, T.F. Fractional model equation for anomalous diffusion. Phys. A Stat. Mech. Its Appl. 1994, 211, 13–24. [Google Scholar] [CrossRef]
- Cello, P.A.; Walker, D.D.; Valocchi, A.J.; Loftis, B. Flow dimension and anomalous diffusion of aquifer tests in fracture networks. Vadose Zone J. 2009, 8, 258–268. [Google Scholar] [CrossRef]
- Atangana, A.; Bildik, N. The use of fractional order derivative to predict the groundwater flow. Math. Probl. Eng. 2013, 2013, 9. [Google Scholar] [CrossRef]
- Atangana, A.; Vermeulen, P.D. Analytical solutions of a space-time fractional derivative of groundwater flow equation. Abstr. Appl. Anal. 2014, 2014, 11. [Google Scholar] [CrossRef]
- Atangana, A. Drawdown in prolate spheroidal–spherical coordinates obtained via Green’s function and perturbation methods. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 1259–1269. [Google Scholar] [CrossRef]
- Su, N. Equations of anomalous absorption onto swelling porous media. Mater. Lett. 2009, 63, 2483–2485. [Google Scholar] [CrossRef]
- Su, N. N-dimensional fractional Fokker–Planck equation and its solutions for anomalous radial two-phase flow in porous media. Appl. Math. Comput. 2009, 213, 506–515. [Google Scholar] [CrossRef]
- Su, N. Theory of infiltration: Infiltration into swelling soils in a material coordinate. J. Hydrol. 2010, 395, 103–108. [Google Scholar] [CrossRef]
- Su, N. Distributed-order infiltration, absorption and water exchange in mobile and immobile zones of swelling soils. J. Hydrol. 2012, 468, 1–10. [Google Scholar] [CrossRef]
- Su, N. Mass-time and space-time fractional partial differential equations of water movement in soils: Theoretical framework and application to infiltration. J. Hydrol. 2014, 519, 1792–1803. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Progr. Fract. Differ. Appl. 2015, 1, 1–13. [Google Scholar]
- Podlubny, I. Fractional Differential Equations Mathematics in Science and Engineering; Academic Press: San Diego, CA, USA, 1999; Volume 198. [Google Scholar]
- Caputo, M. Linear models of dissipation whose Q is almost frequency independent–II. Geophys. J. Int. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Xiao-Jun, X.J.; Srivastava, H.M.; Machado, J.T. A new fractional derivative without singular kernel. Therm. Sci. 2016, 20, 753–756. [Google Scholar]
- Hristov, J. Transient space-fractional diffusion with a power-law superdiffusivity: Approximate integral-balance approach. Fundam. Inf. 2017, 151, 371–388. [Google Scholar] [CrossRef]
- Ali, F.; Murtaza, S.; Khan, I.; Sheikh, N.A.; Nisar, K.S. Atangana–Baleanu fractional model for the flow of Jeffrey nanofluid with diffusion-thermo effects: Applications in engine oil. Adv. Differ. Equ. 2019, 2019, 346. [Google Scholar] [CrossRef]
- Syam, M.I.; Al-Refai, M. Fractional differential equations with Atangana–Baleanu fractional derivative: Analysis and applications. Chaos Solitons Fractals X 2019, 2, 100013. [Google Scholar] [CrossRef]
- Ahmed, N.; Shah, N.A.; Vieru, D. Two-dimensional advection–diffusion process with memory and concentrated source. Symmetry 2019, 11, 879. [Google Scholar] [CrossRef]
- Abate, J.; Valko, P.P. Multi-precision Laplace transform inversion. Int. J. Numer. Methods Eng. 2004, 60, 979–993. [Google Scholar] [CrossRef]
- Hristov, J. Linear viscoelastic responses and constitutive equations in terms of fractional operators with non-singular kernels. Eur. Phys. J. Plus 2019, 134, 283. [Google Scholar] [CrossRef]
- Trzmiel, J.; Weron, K.; Janczura, J.; Placzek-Popko, E. Properties of the relaxation time distribution underlying the Kohlraush-Williams-Watts photoionization of the DX centers in Cd1−xMnxTe mixed crystals. J. Phys. Condens. Matter 2009, 21, 345801. [Google Scholar] [CrossRef]
- Berberan-Santos, M.N.; Bodunov, E.N.; Valeur, B. Luminescence decays with underlying distributions of rate constants: General properties and selected cases. In Fluorescence of Supermolecules, Polymers, and Nanosystems; Springer: Berlin/Heidelberg, Germany, 2007; pp. 67–103. [Google Scholar]
- Giusti, A.; Colombaro, I.; Garra, R.; Garrappa, R.; Polito, F.; Popolizio, M.; Mainardi, F. A practical guide to Prabhakar fractional calculus. Fract. Calc. Appl Anal. 2020, 23, 9–54. [Google Scholar] [CrossRef]
- Long, J.; Xiao, R.; Chen, W. Fractional viscoelastic models with non-singular kernels. Mech. Mater. 2018, 127, 55–64. [Google Scholar] [CrossRef]
- Cresson, J.; Szafránska, A. Comments on various extensions of the Riemann–Liouville fractional derivatives: About the Leibniz and chain rule properties. Commun. Nonlinear Sci. Numer. Simulat. 2020, 82, 104903. [Google Scholar] [CrossRef]
- Tarasov, V.E.; Tarasova, S.S. Fractional derivatives and integrals: What are they needed for? Mathematics 2020, 8, 164. [Google Scholar] [CrossRef]
- Su, N.; Nelson, P.N.; Connor, S. The distributed-order fractional diffusion-wave equation of groundwater flow: Theory and application to pumping and slug tests. J. Hydrol. 2015, 529, 1262–1273. [Google Scholar] [CrossRef]
- Bras, R.L. Hydrology; Addison-Wesley: Reading, MA, USA, 1990. [Google Scholar]
- Bear, J.; Verruijt, A. Modelling Groundwater Flow and Pollution; D. Reidel: Dordrecht, The Netherlands, 1987. [Google Scholar]
- de Marsily, G. Quantitative Hydrogeology; Academic Press: San Diego, CA, USA, 1986. [Google Scholar]
- Bear, J. Dynamics of Fluids in Porous Media; Dover: New York, NY, USA, 1972. [Google Scholar]
- Atangana, A.; Baleanu, D. New fractional derivatives with non-local and non-singular kernel. Theory and Application to Heat Transfer Model. Therm. Sci. 2016, 20, 763–769. [Google Scholar] [CrossRef]
- Haubold, H.; Mathai, A.M.; Saxena, R.K. Mittag-Leffler functions and their applications. J. Appl. Math. 2011, 2011, 51. [Google Scholar] [CrossRef]
- Zhang, X.; Tong, D. A generalized Weber transform and its inverse formula. Appl. Math. Comput. 2007, 193, 116–126. [Google Scholar] [CrossRef]
- Lorenzo, C.F.; Hartley, T.T. Generalized Functions for the Fractional Calculus. NASA/TP-1999-209424/REV1. Available online: https://ntrs.nasa.gov/api/citations/19990110709/downloads/19990110709.pdf (accessed on 3 February 2021).
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shah, N.A.; Rauf, A.; Vieru, D.; Sitthithakerngkiet, K.; Kumam, P. Analytical Solutions of the Diffusion–Wave Equation of Groundwater Flow with Distributed-Order of Atangana–Baleanu Fractional Derivative. Appl. Sci. 2021, 11, 4142. https://doi.org/10.3390/app11094142
Shah NA, Rauf A, Vieru D, Sitthithakerngkiet K, Kumam P. Analytical Solutions of the Diffusion–Wave Equation of Groundwater Flow with Distributed-Order of Atangana–Baleanu Fractional Derivative. Applied Sciences. 2021; 11(9):4142. https://doi.org/10.3390/app11094142
Chicago/Turabian StyleShah, Nehad Ali, Abdul Rauf, Dumitru Vieru, Kanokwan Sitthithakerngkiet, and Poom Kumam. 2021. "Analytical Solutions of the Diffusion–Wave Equation of Groundwater Flow with Distributed-Order of Atangana–Baleanu Fractional Derivative" Applied Sciences 11, no. 9: 4142. https://doi.org/10.3390/app11094142
APA StyleShah, N. A., Rauf, A., Vieru, D., Sitthithakerngkiet, K., & Kumam, P. (2021). Analytical Solutions of the Diffusion–Wave Equation of Groundwater Flow with Distributed-Order of Atangana–Baleanu Fractional Derivative. Applied Sciences, 11(9), 4142. https://doi.org/10.3390/app11094142







