Prediction of Theoretical Derailments Caused by Cross-Winds with Frequency
Abstract
1. Introduction
- Aerodynamics
- Vehicle dynamics
2. Vehicle Behaviors under a Constant Cross-Wind
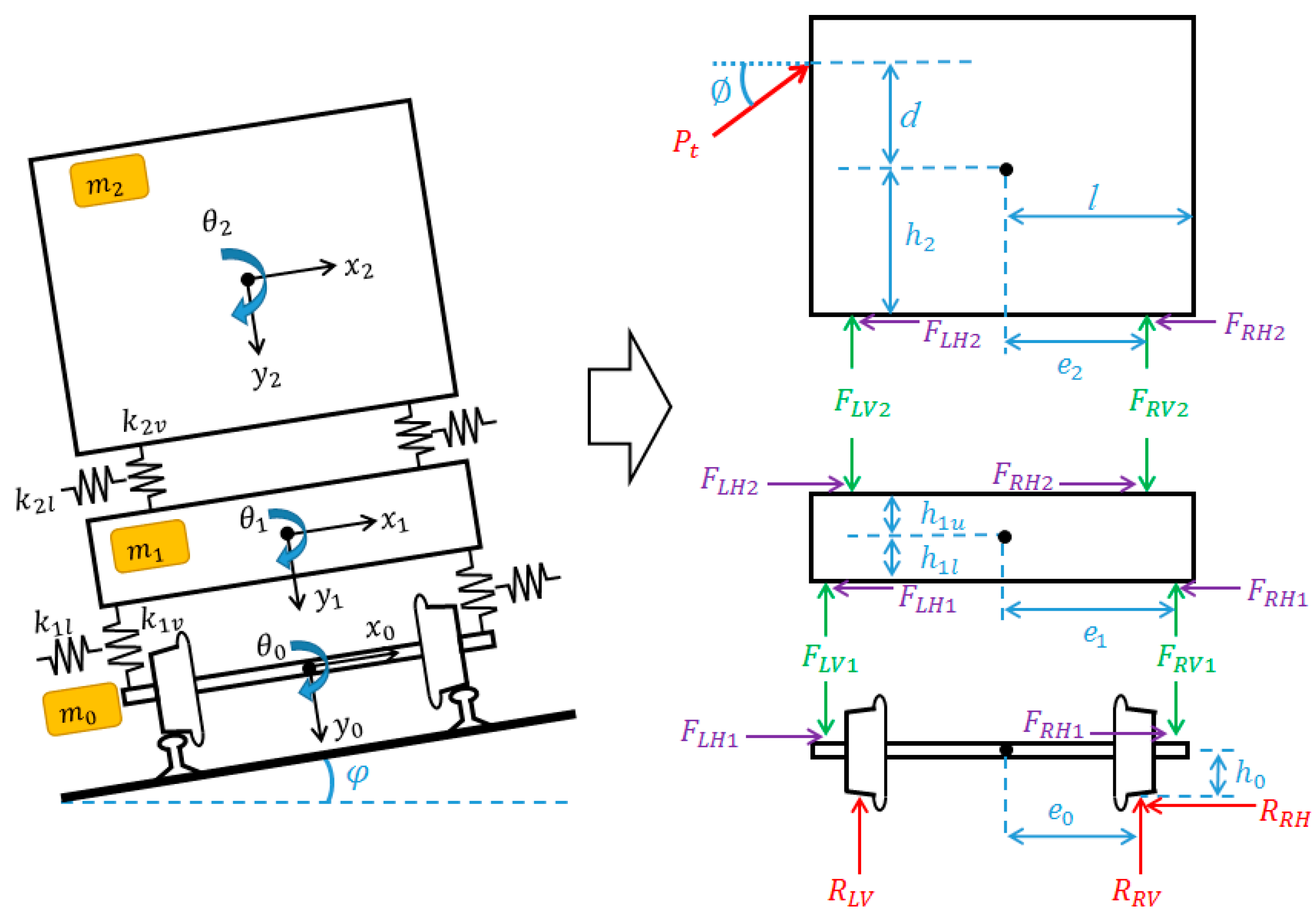
2.1. Formula for a Constant Cross-Wind Acting on a Car Body
- Static equilibrium equation for the car body:
- Static equilibrium equation for the bogie:
- Static equilibrium equation for a wheelset:
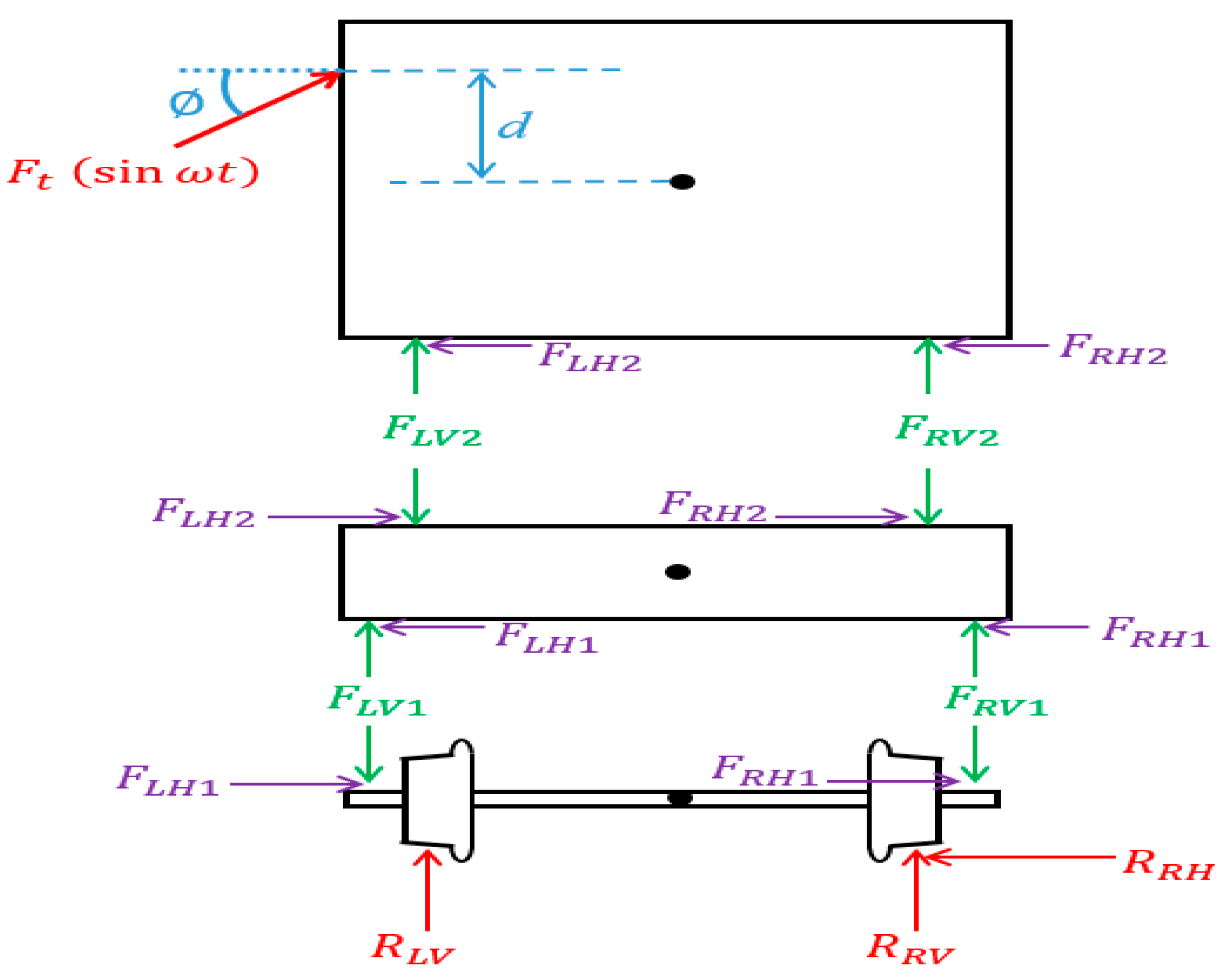
2.2. Dynamic Equations for a Cyclical Cross-Wind Acting on the Body
- The equation of motion for the bogie frame:
- The equation of motion for the wheelset:
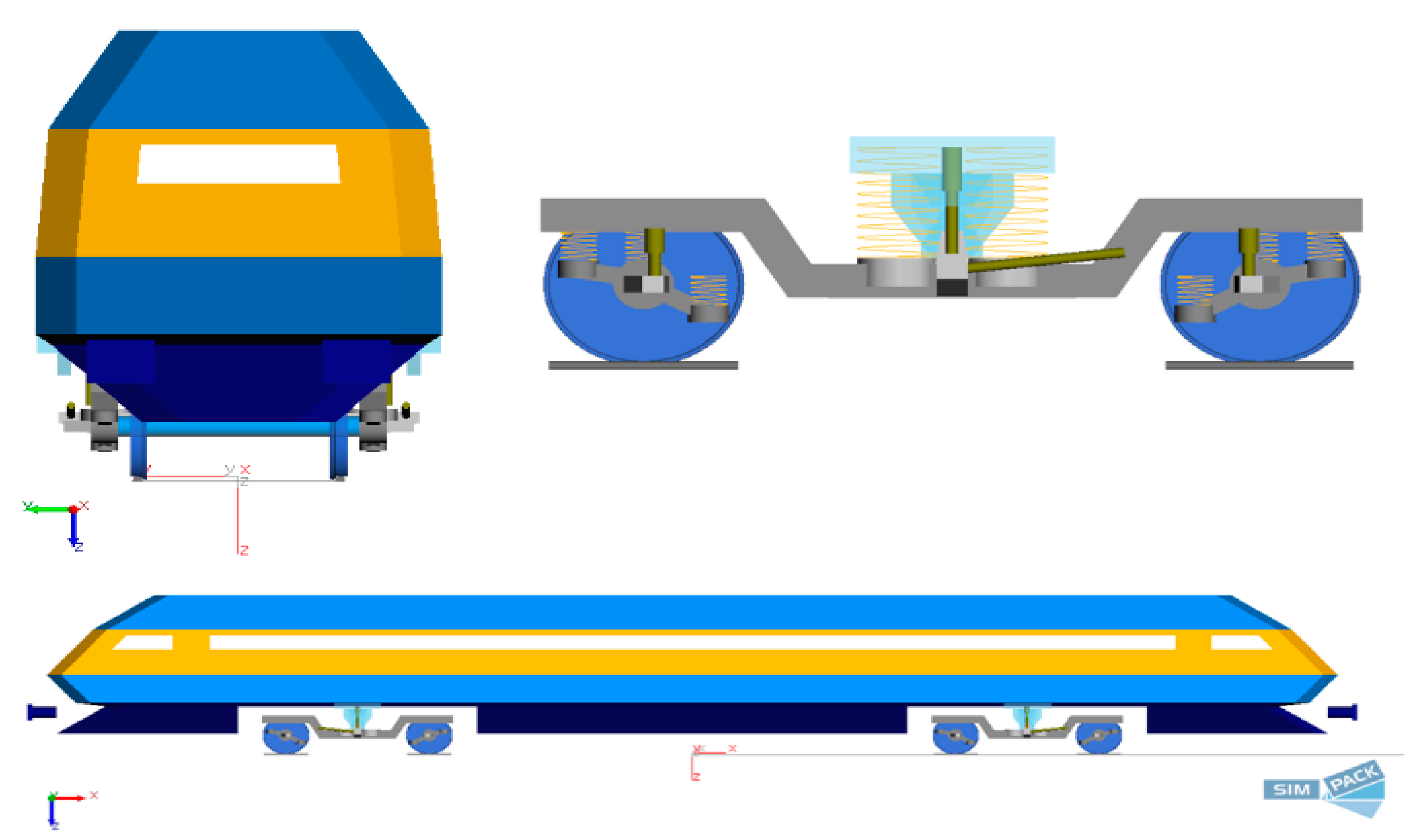
3. A Dynamic Simulation Model to Evaluate the Formulation
3.1. A Model for Multibody Dynamics Simulation
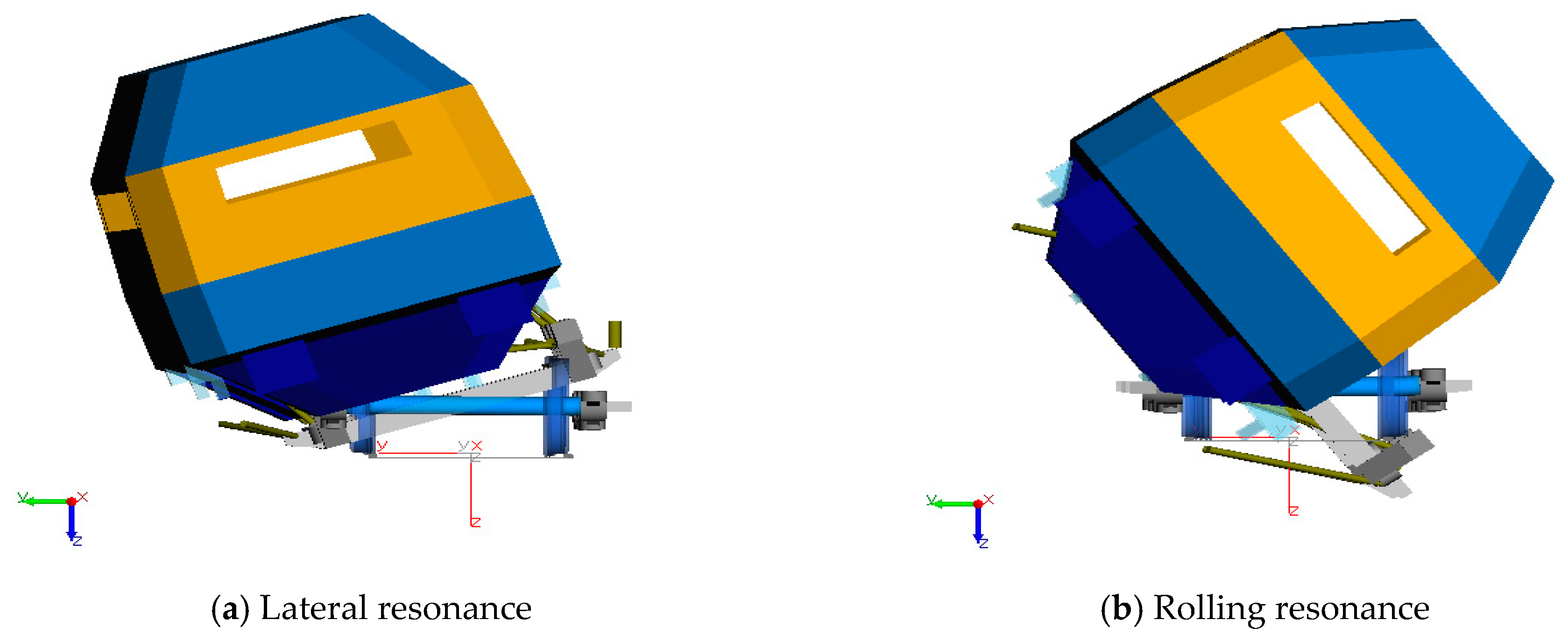
3.2. Evaluation of Natural Frequency of the Vehicle
4. Evaluation of Derailment Safety under Cross-Wind
4.1. Operating Regulations of the KTX According to Wind Speed
4.2. Criteria of Derailment
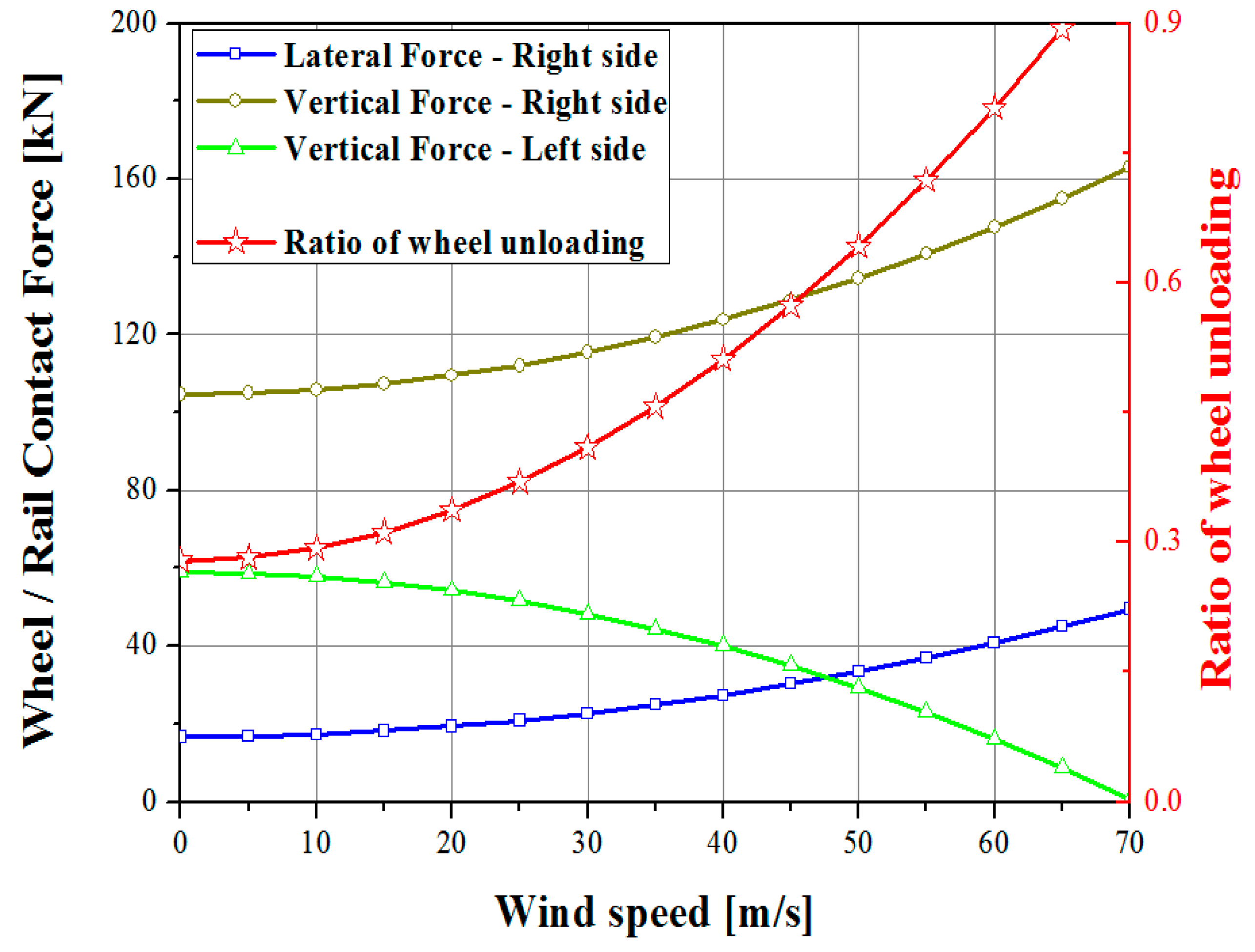
4.3. Derailment Safety According to the Cross-Wind Regulations
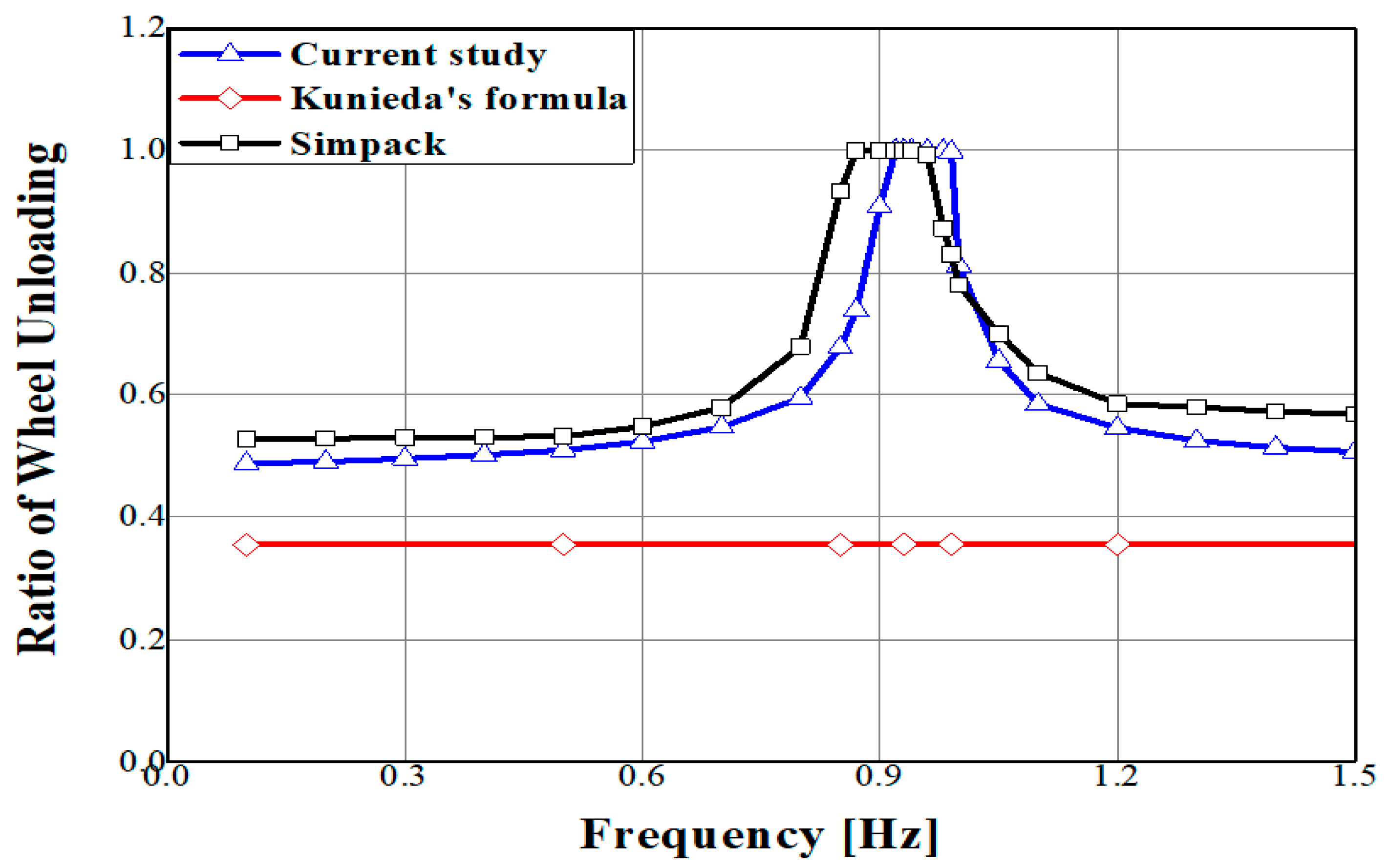
4.4. Derailment Safety Due to the Cross-Wind Frequencies
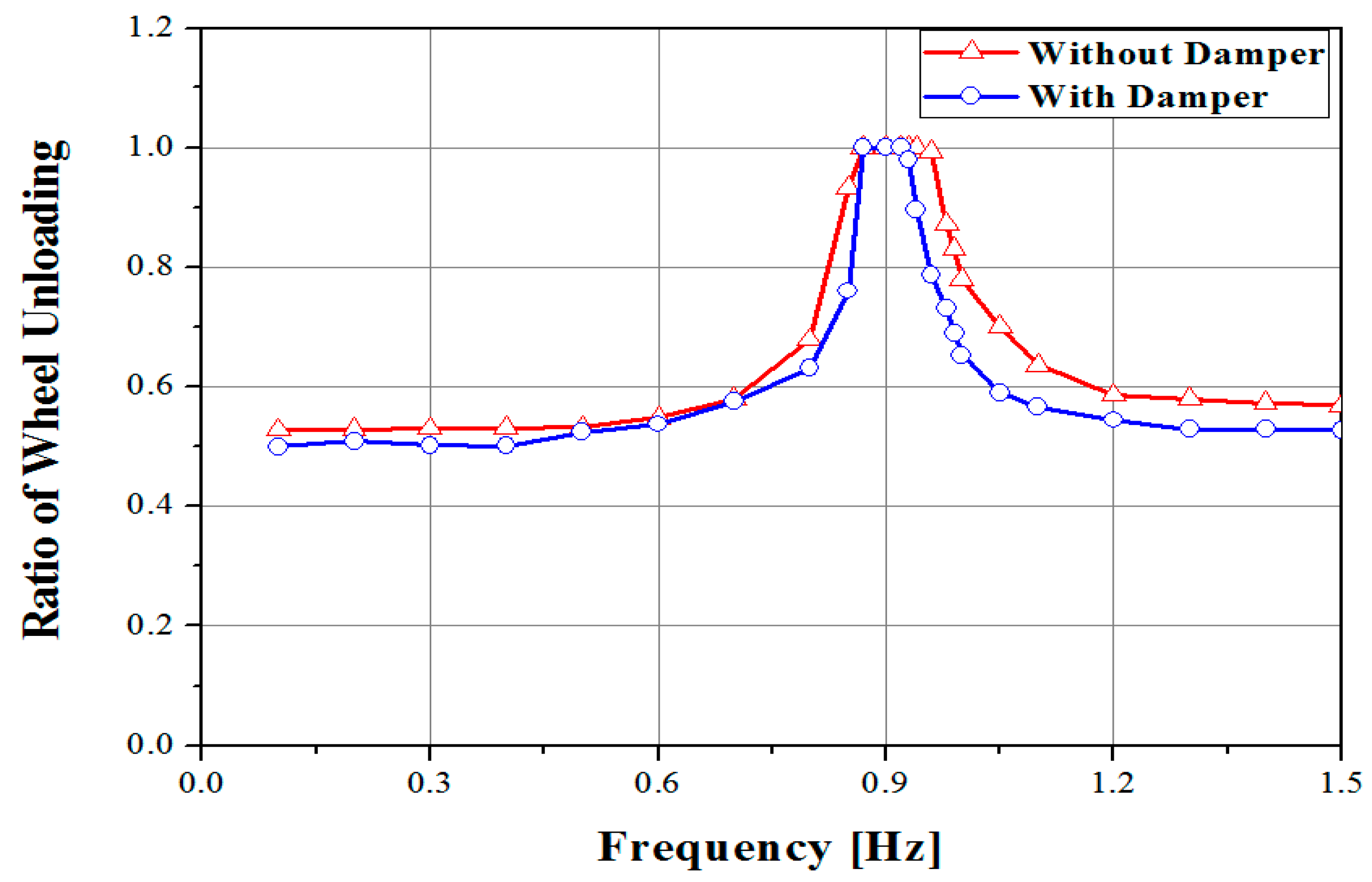
4.4.1. The 2D Dynamic Vehicle Model without Dampers
4.4.2. The 2D Dynamic Vehicle with Dampers
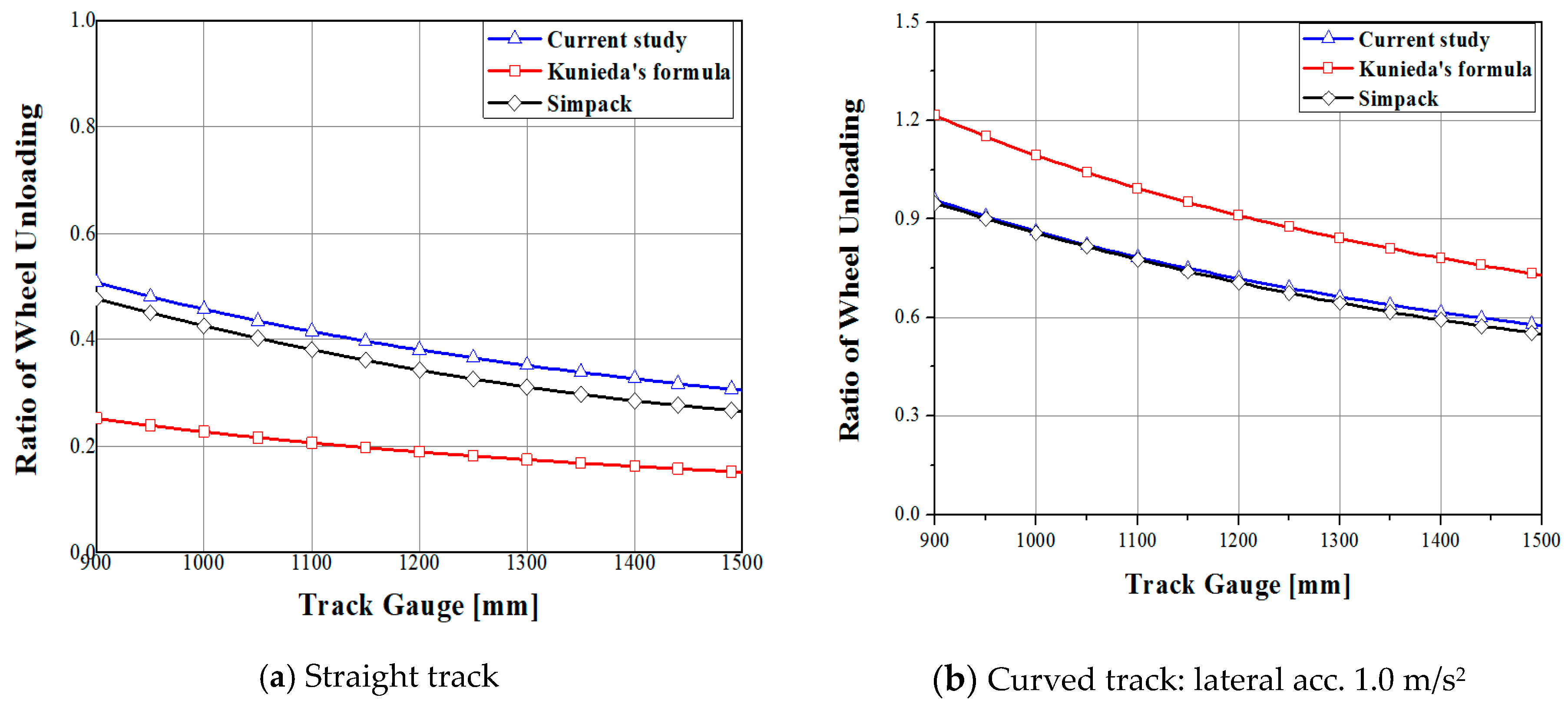
4.5. Derailment Safety According to Track Gauges
5. Conclusions
- (1)
- The static equilibrium equations for the body, the bogie, and the wheelset were derived under a constant force of winds applied to the vehicle using the 2D free body diagram. The wheel–rail and suspension forces, and the displacements and rotation angle of the vehicle were obtained by solving the equations for each static variable.
- (2)
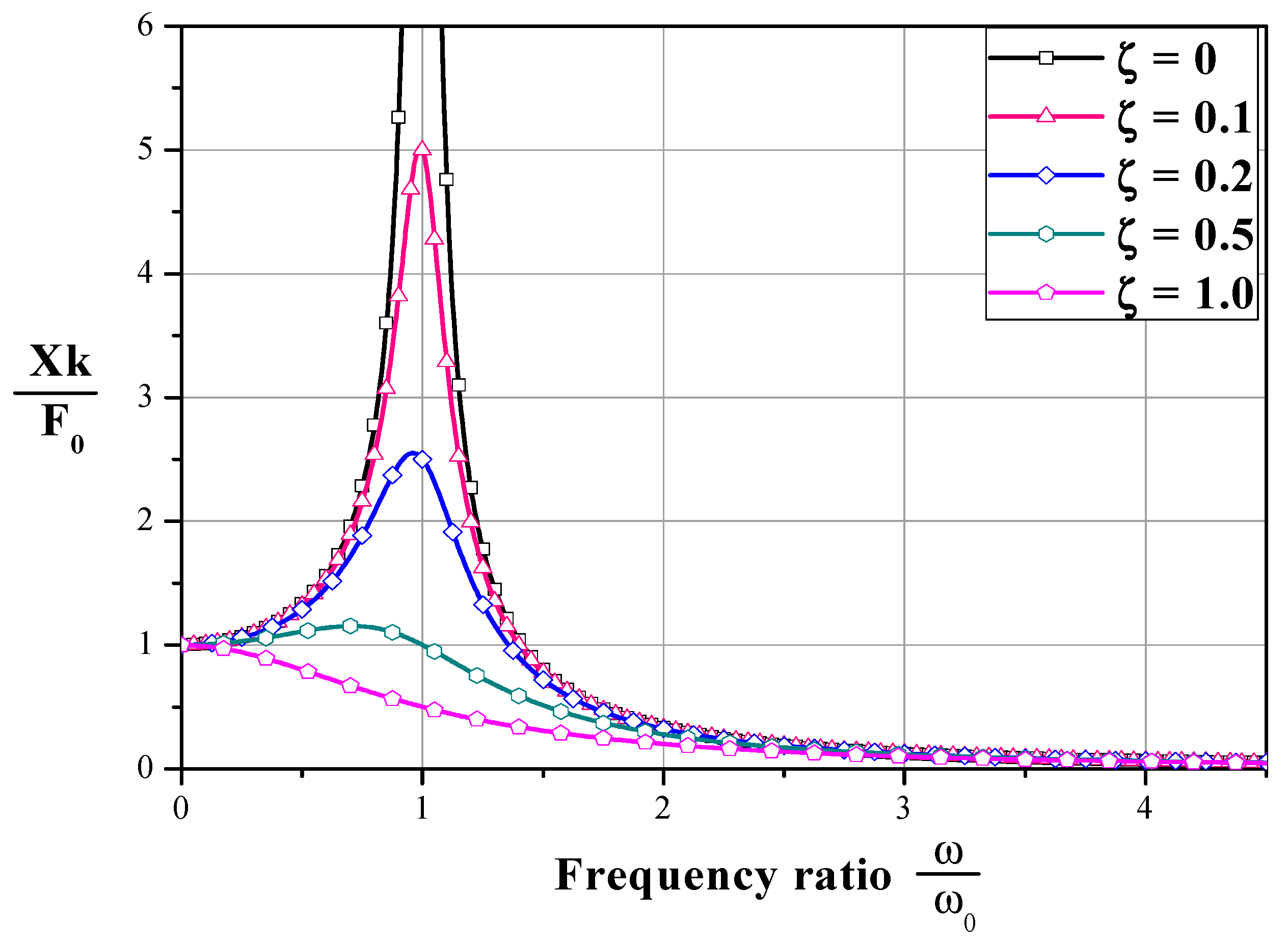
- The dynamic equations for the body, the bogie, and the wheelset were derived under a wind force with frequency components applied to the vehicle using the 2D free body diagram. The wheel–rail and suspension forces, and the displacements and rotation angle of the vehicle were obtained by solving the equations for each dynamic variable. In addition, the lateral and rolling natural frequencies of the vehicle were derived by solving the motion equations for the lateral and rolling directions.
- (3)
- The wheel–rail forces obtained from summing the dynamic and static ones were improved using a known derailment coefficient to consider wheel–rail interactions during a normal train running without winds.
- (4)
- The multibody dynamics software (Simpack) model was generated using the specifications of a Korean high-speed train (KTX) to evaluate our theoretical formula. For the operational regulations of the KTX under cross-wind conditions, the ratios of wheel unloading on the dynamic simulation and our theoretical formula were compared and well matched.
- (5)
- The ratio changes of wheel unloading were investigated under the presence or absence of suspension dampers considering the natural frequency of a vehicle and the cross-wind frequency. As a result, the ratios were found to exceed the TSI criteria regardless of the presence or absence of dampers if the natural frequency of the vehicle and the cross-wind frequency were matched.
- (6)
- The ratio changes of wheel unloading were investigated according to track gauges using the simulations, Kunieda’s formula, and our theoretical one. Although all the results were similar on the straight and curved tracks, the wheel unloading ratios using Kunieda’s formula were larger than the simulations and ours which are in a good agreement. So, our theoretical formula gives more accurate results than Kunieda’s formula, because it considers various operational conditions like a cross-wind frequency and a normal running derailment coefficient due to wheel–rail interaction.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Deng, Y.; Xiao, X. Effect of Cross-Wind on High-Speed Vehicle Dynamic Derailment. Logistics 2009, 5, 2287–2293. [Google Scholar]
- Gerd, M.; Peter, D.; Burkhard, S.-W. Effects of Strong Cross Winds on High-Speed Trains: A Methodology for Risk Assessment and Development of Countermeasures; Deutsche Bahn AG Research & Technology Center: Berlin, Germany, 2001; pp. 1–15. [Google Scholar]
- Bae, H.U.; Min, K.H.; Go, Y.S.; Cheon, H.S.; Lim, N.H. Collision Behavior Analysis between Derailed Train and Structure According to Collision Analysis Condition. J. Korean Soc. Hazard Mitig. 2017, 17, 45–54. [Google Scholar] [CrossRef]
- Tamura, Y. Wind-induced damage to buildings and disaster risk reduction. In Proceedings of the APCWE-VII, Taipei, Taiwan, 8–12 November 2009. [Google Scholar]
- Zhai, W.; Yang, J.; Li, Z.; Han, H. Dynamics of high-speed train in crosswinds based on an air-train- track interaction model. Wind Struct. 2015, 20, 143–168. [Google Scholar] [CrossRef]
- DB Netz AG. Ausgewählte Maßnahmen und Anforderungen an das Gesamtsystem Fahrweg/Fahrzeug-Aerodynamik/Seitenwind; DB Netz AG: Frankfurt, Germany, 2006. [Google Scholar]
- Baker, C.; Cheli, F.; Orellano, A. Nicolas Paradot, Carsten Proppe and Daniele Rocchi, “Cross-wind effects on road and rail vehicles”. Veh. Syst. Dyn. 2009, 47, 983–1022. [Google Scholar] [CrossRef]
- European Committee for Standardization. EN 14067-5, Railway Applications—Aerodynamics—Part 5: Requirements and Test Procedures for Aerodynamics in Tunnels; European Committee for Standardization: Brussels, Belgium, 2010. [Google Scholar]
- European Committee for Standardization. EN 14067-6, Railway Applications—Aerodynamics—Part 6: Requirements and Test Procedures of Cross Wind Assessment; European Committee for Standardization: Brussels, Belgium, 2016. [Google Scholar]
- Kunieda, M. Theoretical Study on the Mechanics of Overturn of Rail Rolling Stock. Rtri Railw. Tech. Res. Rep. 1972, 793, 173–186. [Google Scholar]
- Sun, Z.; Dai, H.; Hemida, H.; Li, T.; Huang, C. Safety of High-Speed Train Passing by Windbreak Breach with Different Sizes. Veh. Syst. Dyn. 2020, 58, 1935–1952. [Google Scholar] [CrossRef]
- Biadgo Asress, M.; Svorcan, J. Numerical investigation on the aerodynamic characteristics of high-speed train under turbulent crosswind. J. Mod. Transp. 2014, 22, 225–234. [Google Scholar] [CrossRef]
- Giappino, S.; Rocchi, D.; Schito, P.; Tomasini, G. Cross wind and rollover risk on lightweight railway vehicles. J. Wind Eng. Ind. Aerodyn. 2016, 153, 106–112. [Google Scholar] [CrossRef]
- Yu, M.; Jiang, R.; Zhang, Q.; Zhang, J. Crosswind stability evaluation of high-speed train using different wind models. Chin. J. Mech. Eng. 2019, 32, 1–13. [Google Scholar] [CrossRef]
- Hosoi, T.; Tanifuji, K. Effect of Crosswind on Derailment of Railway Vehicles Running on Curved Track at Low Speed. Int. J. Railw. 2012, 5, 93–101. [Google Scholar] [CrossRef][Green Version]
- Baker, C.; Hemida, H.; Iwnicki, S.; Xie, G.; Ongaro, D. Integration of Crosswind Forces into Train Dynamic Modelling. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2011, 225, 154–164. [Google Scholar] [CrossRef]
- Yu, H.; Shimomura, T.; Tanifuji, K. Full-scale experiment on the behavior of a railway vehicle being subjected to lateral force. J. Mech. Syst. Transp. Logist. 2010, 3, 35–43. [Google Scholar]
- Yu, H.; Misu, Y.; Kurihara, T. Atsushi Moriyama and Makoto Shimamura, Study of New Methods for Train Operation Control in Strong. Wind. Jr East Tech. Rev. 2011, 19, 31–36. [Google Scholar]
- Nam, S.W. Theoretical Analysis on Overturn Safety of Train affected by Wind Pressure. J. Korean Soc. Railw. 2012, 15, 537–542. [Google Scholar] [CrossRef]
- Xiao, X.B.; Ling, L.; Xiong, J.Y.; Zhou, L.; Jin, X.S. Study on the safety of operating high-speed railway vehicles subjected to crosswinds. J. Zhejiang Univ. Sci. A 2014, 15, 694–710. [Google Scholar] [CrossRef]
- Hong, T.D. A Study on the Improvement of KTX Bogie Instability. Master’s Thesis, University of Seoul, Seoul, Korea, 2006; pp. 1–88. [Google Scholar]
- Eom, B.G.; Han, B.Y.; Kang, B.B.; Lee, H.S. A Study on 1/5 Scaled Bogie Design of KTX-Sancheon using Similarity Laws. In Proceedings of the Korean Society for Railway Spring Conference, Korea, Mokpo, 17–19 May 2012; pp. 1772–1777. [Google Scholar]
- Kim, M.S. A Study on Derailment Prediction of Railway Vehicle Due to Cross-Wind Using the Derailment Theory of Single Wheelset. Master’s Thesis, Seoul National University of Science & Technology, Seoul, Korea, 2014; pp. 1–59. [Google Scholar]
- Choi, J.H.; Park, T.W.; Sim, K.S.; Kwak, M.H.; Lee, D.H. Stability Evaluation on Aerodynamics of High Speed Railway Train. Trans. Korean Soc. Noise Vib. Eng. 2012, 22, 244–252. [Google Scholar] [CrossRef]
- Koo, J.S.; Choi, S.Y. Theoretical development of a simplified wheelset model to evaluate collision-induced derailments of rolling stock. J. Sound Vib. 2012, 331, 3172–3198. [Google Scholar] [CrossRef]
- Oh, H.S. A Study on Mechanical Parameters Influencing Derailment of a Wheelset. Master’s Thesis, Seoul National University of Science & Technology, Seoul, Korea, 2013; pp. 1–51. [Google Scholar]
- Kim, M.S.; Kim, G.Y.; Kim, H.T.; Koo, J.S. Theoretical cross-wind speed against rail vehicle derailment considering the cross-running wind of trains and the dynamic wheel-rail effects. J. Mech. Sci. Technol. 2016, 30, 3487–3498. [Google Scholar] [CrossRef]
- Kim, G.Y.; Koo, J.S.; Rho, J.H. Theoretical Evaluation of Rollingstock Derailment Safety under Cyclical Crosswind Condition. J. Mech. Sci. Technol. 2018, 42, 95–101. [Google Scholar]
- Choi, S.Y. Development of a Wheelset Derailment Model for Prediction of Derailment Behavior after Train Collision. Master’s Thesis, Seoul National University of Science & Technology, Seoul, Korea, 2011; pp. 1–54. [Google Scholar]
- Dassault System Simulia Corp. Simpack Documentation; Dassault System Simulia Corp.: Velizy-Villacoublay, France, 2017. [Google Scholar]
- Kwon, H.B.; You, W.H.; Cho, T.H. Measurement of Aerodynamic Loads on Railway Vehicles under Crosswind. Trans. Korean Soc. Mech. Eng. A 2011, 35, 91–98. [Google Scholar] [CrossRef]
- Yi, S. The Effect Law of the Curve Parameters of a High-Speed Railway on Vehicle-Line Dynamic Performance. Dyn. Anal. High-Speed Railw. Alignment 2018, 73–108. [Google Scholar] [CrossRef]
- Wang, P. Wheel-Rail Relation Design. Des. High-Speed Railw. Turnouts 2015, 97–162. [Google Scholar] [CrossRef]
- Soper, D. The Aerodynamics of a Container Freight Train; Springer: Berlin/Heidelberg, Germany, 2016; pp. 10–32. [Google Scholar]
- Official Journal of the European Union. COMMISSION DECISION of 21 February 2008 Concerning a Technical Specification for Interoperability Relating to the ‘Rolling Stock’ Sub-System of the Trans-European High-Speed Rail System. Available online: https://eur-lex.europa.eu (accessed on 26 March 2008).
- Kim, J.C.; Ham, Y.S. Analysis on Running Safety for KTX Vehicle. Korean Soc. Railw. 2007, 10, 473–479. [Google Scholar]
- Ham, Y.S. The Evaluation of Running Safety in the Double Connection Operation for KTX-Sancheon Train. In Proceedings of the the Korean Society of Mechanical Engineers Autumn Conference, Jeju, Korea, 28–30 October 2010; pp. 155–156. [Google Scholar]
- Sun, Z.; Dai, H.; Gao, H.; Li, T.; Song, C. Dynamic performance of high-speed train passing windbreak breach under unsteady crosswind. Int. J. Veh. Mech. Mobil. 2018, 408–424. [Google Scholar] [CrossRef]
- Inman, D.J. Engineering Vibrations; PEARSON: London, UK, 2012; pp. 109–121. [Google Scholar]







| Suspension | Double Coil Spring | Guide Spring | Vertical Oil Damper | |
|---|---|---|---|---|
| specifications | X direction | 0.5813 | 20.0 | - |
| Y direction | 0.5813 | 4.5 | - | |
| Z direction | 0.75 | 0.25 | 0.01 | |
| T direction | 0.1 | 0.044 | - | |
| P direction | 0.1 | 0.03 | - | |
| W direction | 0.1 | 0.473 | - | |
| Units | MN/m MN·m/rad | MN/m MN·m/rad | MN·s/m | |
| Specification | ||||||
|---|---|---|---|---|---|---|
| Velocity [m/s] | 0.0 | 0.0026 | 0.01 | 0.02 | 0.3 | |
| Force [kN] | 0.0 | 11.0 | 11.6 | 12.0 | 19.0 | |
| Suspension | Coil Spring | Vertical Oil Damper | Lateral Oil Damper | Pivot Joint | |
|---|---|---|---|---|---|
| Specifications | X direction | 0.15 | - | - | 10.29 |
| Y direction | 0.15 | - | 0.1 | - | |
| Z direction | 0.634 | 0.02 | - | - | |
| T direction | 0.1 | - | - | - | |
| P direction | 0.1 | - | - | - | |
| W direction | 0.1 | - | - | - | |
| Units | MN/m MN·m/rad | MN/m MN·m/rad | MN·s/m | MN/m | |
| Values | |||
|---|---|---|---|
| Wheelset | Wheel weight | 1745 | [kg] |
| Moment of inertia | 740 | [kg·m2] | |
| Height of weight center | 0.46 | [m] | |
| Bogie frame | Bogie weight | 2420 | [kg] |
| Moment of inertia | 972.5 | [kg·m2] | |
| Height of weight center | 0.56 | [m] | |
| Car body | Car body weight | 54,960 | [kg] |
| Moment of inertia | 13,246.36 | [kg·m2] | |
| Height of weight center | 1.72 | [m] | |
| Height of the wind force center on the car body | 2.015 | [m] | |
| Parameters | Values | Parameters | Values | ||
|---|---|---|---|---|---|
| 1745 | [kg] | 0.46 | [m] | ||
| 740 | [kg·m2] | 0.7175 | [m] | ||
| 1210 | [kg] | 0.067 | [m] | ||
| 972.5 | [kg·m2] | 0.435 | [m] | ||
| 1 | [m] | ||||
| 13,740 | [kg] | 0.295 | [m] | ||
| 13,246 | [kg·m2] | 1.335 | [m] | ||
| 1.025 | [m] | 1.093 | [m] | ||
| 100 | [kg/mm] | 100 | [kg/mm] | ||
| 9.58 | [kg/mm] | 1.25 | [kg/mm] | ||
| 0.51 | [kg/mm] | 0.74 | [kg/mm] | ||
| Resonant Frequencies [Hz] | |||
|---|---|---|---|
| Lateral Direction | Rolling Direction | ||
| Theory | 0.94 | 2.51 | |
| Simpack | 0.90 | 2.77 | |
| Wind Speed [m/s] | Driving Conditions |
|---|---|
| 45~ | Operation stop |
| 40~45 | Under 90 [km/h] |
| 30~40 | Under 170 [km/h] |
| ~30 | Decelerated operation |
| Parameters | Values | ||
|---|---|---|---|
| Aerodynamic side force coefficient | () | 0.6 | |
| Side area | () | 70.5 | [m2] |
| Air density | () | 1.275 | [kg/m3] |
| Country/ Regulation | Method/Criteria | Note |
|---|---|---|
| EU | 2 m moving average of derailment coefficient | |
| UIC | Derailment coefficient | |
| TSI | Ratio of wheel unloading | |
| North America | Derailment coefficient | |
| Weinstock coefficient | ||
| Ratio of wheel unloading | ||
| RTRI (Japan) | Ratio of wheel unloading |
| Track Condition | Wind Speeds [m/s] | Ratios of Wheel Unloading | Differences | |
|---|---|---|---|---|
| Theory | Simulation | |||
| Straight track | 0 | 0 | 0 | 0 |
| 30 | 0.120 | 0.119 | 0.001 | |
| 40 | 0.233 | 0.220 | 0.013 | |
| 45 | 0.292 | 0.276 | 0.016 | |
| Curved track (Lateral Acc. = 0.5 m/s2) | 0 | 0.128 | 0.120 | 0.008 |
| 30 | 0.248 | 0.248 | 0 | |
| 40 | 0.343 | 0.353 | 0.010 | |
| 45 | 0.398 | 0.395 | 0.003 | |
| Curved track (Lateral Acc. = 1.0 m/s2) | 0 | 0.256 | 0.242 | 0.014 |
| 30 | 0.376 | 0.364 | 0.012 | |
| 40 | 0.471 | 0.463 | 0.008 | |
| 45 | 0.526 | 0.526 | 0 | |
| Track Condition | Wind Speeds [m/s] | Ratios of Wheel Unloading | Differences | |
|---|---|---|---|---|
| Theory | Simulation | |||
| Straight track | 0 | 0.198 | 0.173 | 0.025 |
| 30 | 0.318 | 0.304 | 0.014 | |
| 40 | 0.413 | 0.403 | 0.010 | |
| 45 | 0.468 | 0.462 | 0.006 | |
| Curved track (Lateral Acc. = 0.5 m/s2) | 0 | 0.323 | 0.305 | 0.018 |
| 30 | 0.443 | 0.429 | 0.014 | |
| 40 | 0.538 | 0.528 | 0.010 | |
| 45 | 0.593 | 0.587 | 0.006 | |
| Curved track (Lateral Acc. = 1.0 m/s2) | 0 | 0.448 | 0.454 | 0.006 |
| 30 | 0.568 | 0.579 | 0.011 | |
| 40 | 0.663 | 0.681 | 0.018 | |
| 45 | 0.718 | 0.739 | 0.021 | |
| Parameters | Values | ||
|---|---|---|---|
| Track | Straight | ||
| Rail irregularity (P/Q) | 0.315 | ||
| Vehicle velocity | 300 | [km/h] | |
| Wind | Average speed | 45 | [m/s] |
| Fluctuation speed | 4.5 | [m/s] | |
| Frequency [Hz] | Theory | Simulation (P/Q = 0.315) | |
|---|---|---|---|
| Current Study (P/Q = 0.315) | Kunieda’s Formula (P/Q = 0) | ||
| 0.1 | 0.488 | 0.355 | 0.528 |
| 0.3 | 0.496 | 0.355 | 0.530 |
| 0.5 | 0.510 | 0.355 | 0.532 |
| 0.7 | 0.548 | 0.355 | 0.579 |
| 0.9 | 0.908 | 0.355 | 1.000 |
| 1.1 | 0.585 | 0.355 | 0.636 |
| 1.3 | 0.525 | 0.355 | 0.580 |
| 1.5 | 0.507 | 0.355 | 0.569 |
| Natural frequency | |
| Critical damping | |
| Damping factor | |
| Parameters | Values | ||||
|---|---|---|---|---|---|
| Wind | Speed | 30 | [m/s] | ||
| Angle | 0 | [°] | |||
| Vehicle speed | 300 | [km/h] | |||
| Rail irregularity (P/Q) | 0.315 | ||||
| Track | Straight | Curved | |||
| Lateral acc. | - | 1 | [m/s2] | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.; Song, I.; Koo, J. Prediction of Theoretical Derailments Caused by Cross-Winds with Frequency. Appl. Sci. 2021, 11, 2947. https://doi.org/10.3390/app11072947
Kim J, Song I, Koo J. Prediction of Theoretical Derailments Caused by Cross-Winds with Frequency. Applied Sciences. 2021; 11(7):2947. https://doi.org/10.3390/app11072947
Chicago/Turabian StyleKim, JunWoo, InHo Song, and JeongSeo Koo. 2021. "Prediction of Theoretical Derailments Caused by Cross-Winds with Frequency" Applied Sciences 11, no. 7: 2947. https://doi.org/10.3390/app11072947
APA StyleKim, J., Song, I., & Koo, J. (2021). Prediction of Theoretical Derailments Caused by Cross-Winds with Frequency. Applied Sciences, 11(7), 2947. https://doi.org/10.3390/app11072947






