Stimuli-Responsive Photonic Crystals

Abstract

Recently, tunable photonic crystals (PhCs) have received great research interest, thanks to the wide range of applications in which they can be employed, such as light emission and sensing, among others. In addition, the versatility and ease of fabrication of PhCs allow for the integration of a large range of responsive elements that, in turn, can permit active tuning of PhC optical properties upon application of external stimuli, e.g., physical, chemical or even biological triggers. In this work, we summarize the most employed theoretical tools used for the design of optical properties of responsive PhCs and the most used fabrication techniques. Furthermore, we collect the most relevant results related to this field, with particular emphasis on electrochromic devices.

1. Introduction

Photonic crystals (PhCs) represent versatile building blocks in optics, although they are mostly used as passive optical elements. In these systems, the periodic arrangement of materials with different refractive indices gives rise to the so-called photonic band gap (PBG) and, thus, to structural coloration [1,2]. In this context, however, anything that can interfere with either the periodicity or the refractive index contrast can be translated into an active modulation of the PBG. This in fact enables application of PhCs as optical active elements. From conceptualization to fabrication, the simplest photonic crystal is represented by a multilayer that alternates materials with different refractive indices [3,4]. From an application point of view, multilayer photonic crystals, also known as distributed Bragg reflectors (DBRs) or Bragg stacks (BSs), have been used as resonators for distributed feedback lasers [5,6], smart dielectric layers for light-emitting transistors [7] and light-induced tunable filters [8], among others.

In this paper, we will review the latest advancements in the field of responsive 1D PhCs, with particular attention to electro-responsive systems. In particular, this paper will discuss both the theoretical methods that are commonly used to predict the optical properties of 1D PhCs, as well as the most employed fabrication techniques. In addition, we will describe the different approaches utilized to achieve active tuning of the photonic band gap.

2. Theoretical Background

First, we consider a multilayer of materials deposited on a substrate on one side (with refractive index n_s) and in contact with air (with refractive index n₀ = 1.000277 $\cong$ 1) on the other side. The transfer matrix method is exhaustively explained in the literature, such as in reference [9]. The transfer matrix for the k_th layer is given by [9]:

$$M_k=\left[\begin{array}{cc}cos\left(\frac{2\pi }{\lambda }{n}_k{d}_k\right)& -\frac{i}{p_k}sin\left(\frac{2\pi }{\lambda }{n}_k{d}_k\right)\\ -i{p}_{k}sin\left(\frac{2\pi }{\lambda }{n}_k{d}_k\right)& cos\left(\frac{2\pi }{\lambda }{n}_k{d}_k\right)\end{array}\right]$$

(1)

with ${\varphi }_k=\frac{2\pi }{\lambda }{n}_k{d}_{k}cos{\alpha }_k$ that is the light phase variation passing through the kth layer, n_k being the refractive index, d_k the thickness of the layer and $cos{\alpha }_k$ the parameter that takes into account the light beam propagating through the layer with refractive index n_k, related to the angle of incidence ϑ₀ (as displayed in Figure 1a) of the light on the structure:

$$\mathit{c}\mathit{o}\mathit{s}{\mathit{\alpha }}_{\mathit{k}}={\left[\mathbf{1}-\frac{{\mathit{n}}_{\mathbf{0}}^{\mathbf{2}}\mathit{s}\mathit{i}{\mathit{n}}^{\mathbf{2}}{\mathit{\vartheta }}_{\mathbf{0}}}{{\mathit{n}}_{\mathit{k}}^{\mathbf{2}}}\right]}^{\raisebox{1ex}{$\mathbf{1}$}\!\left/ \!\raisebox{-1ex}{$\mathbf{2}$}\right.}$$

(2)

For a transverse electric (TE) wave, the parameter $p_k$ in Equation (1) can be written as $p_{k,TE}=n_{k}cos{\alpha }_k$, while for a transverse magnetic (TM) wave $p_{k,TM}=\frac{cos{\alpha }_k}{n_k}$. The matrix product $M=M_1·M_2\dots M_k\dots M_s=\left[\begin{array}{cc}{m}_{11}& m_{12}\\ m_{21}& m_{22}\end{array}\right]$ gives the matrix of the multilayer made of s layers. We can write the transmission coefficient t as

$$\mathit{t}=\frac{\mathbf{2}{\mathit{p}}_{\mathbf{0}}}{\left({\mathit{m}}_{\mathbf{11}}+{\mathit{m}}_{\mathbf{12}}{\mathit{p}}_{\mathit{s}}\right){\mathit{p}}_{\mathbf{0}}+\left({\mathit{m}}_{\mathbf{21}}+{\mathit{m}}_{\mathbf{22}}{\mathit{p}}_{\mathit{s}}\right)}$$

(3)

For a transverse electric (TE) wave $p_{0,\mathrm{TE}}=n_{0}cos{\alpha }_0$ and $p_{s,TE}=n_s{\left[1-\frac{n_0^{2}si{n}^2{\vartheta }_0}{n_s^2}\right]}^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$, while for a transverse magnetic (TM) wave $p_{0,\mathrm{TM}}=\frac{cos{\alpha }_0}{n_0}$ and $p_{s,TM}=\frac{{\left[1-\frac{n_0^{2}si{n}^2{\vartheta }_0}{n_s^2}\right]}^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}}{n_s}$. Finally, to calculate the light transmission of the multilayer photonic crystal we can use the following equation:

$$\mathit{T}=\frac{{\mathit{n}}_{\mathbf{0}}}{{\mathit{n}}_{\mathit{s}}}{\left|\mathit{t}\right|}^{\mathbf{2}}$$

(4)

If, in the spectral range of interest, the refractive index does not show a significant wavelength dependence, its value can be essentially considered as constant. Otherwise, we should consider the refractive index as a function of the wavelength. The refractive indexes of many materials are reported in the literature [10] and can be expressed with a Sellmeier equation. For example, the Sellmeier equation for silicon dioxide, which is widely employed for the fabrication of one-dimensional photonic crystals, is [11]:

$${\mathit{n}}_{\mathit{S}\mathit{i}{\mathit{O}}_{\mathbf{2}}}^{\mathbf{2}}\left(\mathit{\lambda }\right)-\mathbf{1}=\frac{\mathbf{0.6961663}{\mathit{\lambda }}^{\mathbf{2}}}{{\mathit{\lambda }}^{\mathbf{2}}-{\mathbf{0.0684043}}^{\mathbf{2}}}+\frac{\mathbf{0.4079426}{\mathit{\lambda }}^{\mathbf{2}}}{{\mathit{\lambda }}^{\mathbf{2}}-{\mathit{0.1162414}}^{\mathbf{2}}}+\frac{\mathbf{0.8974794}{\mathit{\lambda }}^{\mathbf{2}}}{{\mathit{\lambda }}^{\mathbf{2}}-{\mathbf{9.896161}}^{\mathbf{2}}}$$

(5)

For porous materials, we can determine the refractive index by employing the Maxwell–Garnett effective medium approximation [12,13]:

$${\epsilon }_{eff}={\epsilon }_{air}\frac{2\left(1-f\right){\epsilon }_{air}+\left(1+2f\right){\epsilon }_{material}}{2\left(2+f\right){\epsilon }_{air}+\left(1-f\right){\epsilon }_{material}}$$

(6)

where ${\epsilon }_{material}$ is the dielectric constant of the material, ${\epsilon }_{air}$ is the dielectric constant of air and f is the filling factor.

In Figure 1, we show the simulation of the angular dependent transmission of a 1D photonic crystal, consisting of the alternation of five bilayers of TiO₂-SiO₂ nanoparticles (filling factor = 0.7). The wavelength-dependent refractive index of TiO₂ is given by $n_{Ti{O}_2}\left(\lambda \right)={\left(4.99+\frac{1}{96.6{\lambda }^{1.1}}+\frac{1}{4.60{\lambda }^{1.95}}\right)}^{1/2}$ [14]. From these simulations, it is also possible to determine the electric field at the k_th interface [15].

3. Fabrication Techniques

Photonic crystals can be fabricated by following two main approaches: (1) top-down methods; and (2) bottom-up methods. In particular, bottom-up techniques can be more conveniently used on the laboratory scale, while top-down approaches rely on the use of microfabrication methods permitting development of microstructures with selected size and shape from bulk materials [16,17]. Besides these advantages, both of them also show some disadvantages. Bottom-up methods usually suffer from a relatively low throughput, whereas top-down techniques require substantial initial investment in terms of money and person hours for dedicated setups. For these reasons, it is thus essential to select the most suitable approach according to the desired goal. Self-assemble techniques are surely the most used bottom-up methods, combining building blocks such as nanoscale structures (e.g., nanoparticles) or block copolymers. These techniques are particularly suitable for the fabrication of responsive photonic crystals as, in this way, one can combine different unitary structures and materials to integrate different functionalities in a one single photonic device. A list of the most used techniques is reported in

Table 1. Main manufacturing techniques of photonic crystals. The type of approach and the most significant advantages are indicated for each technique.

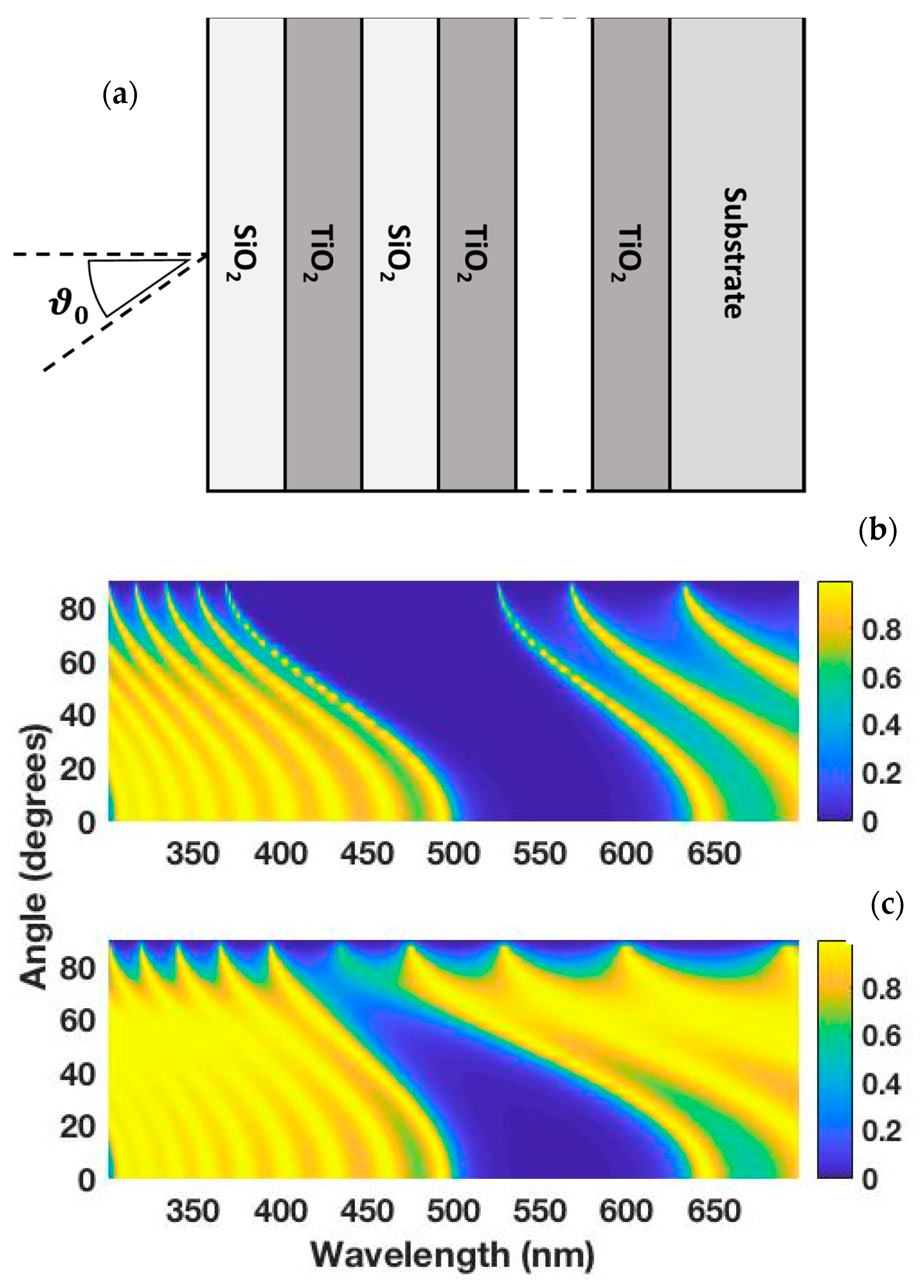
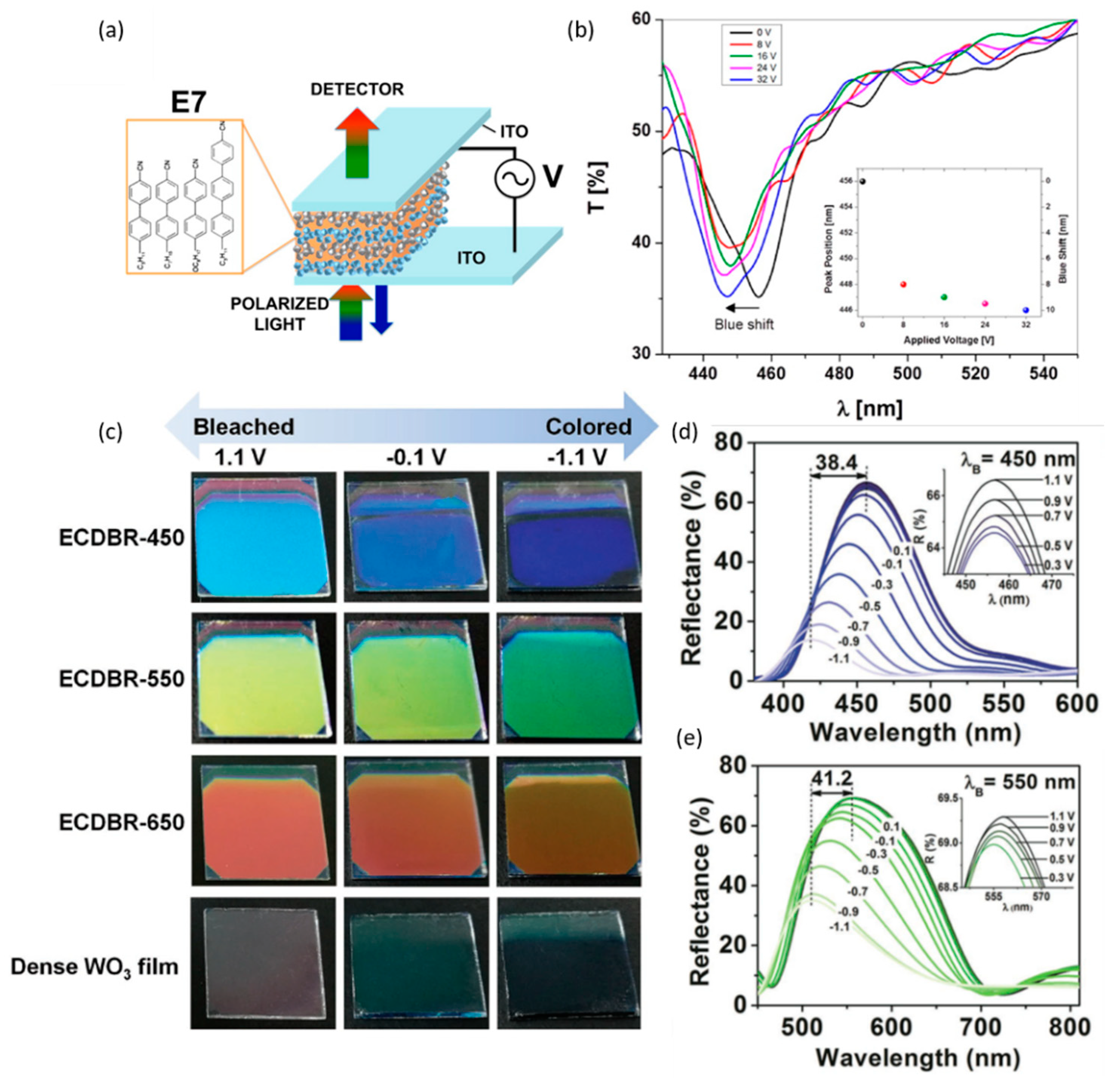
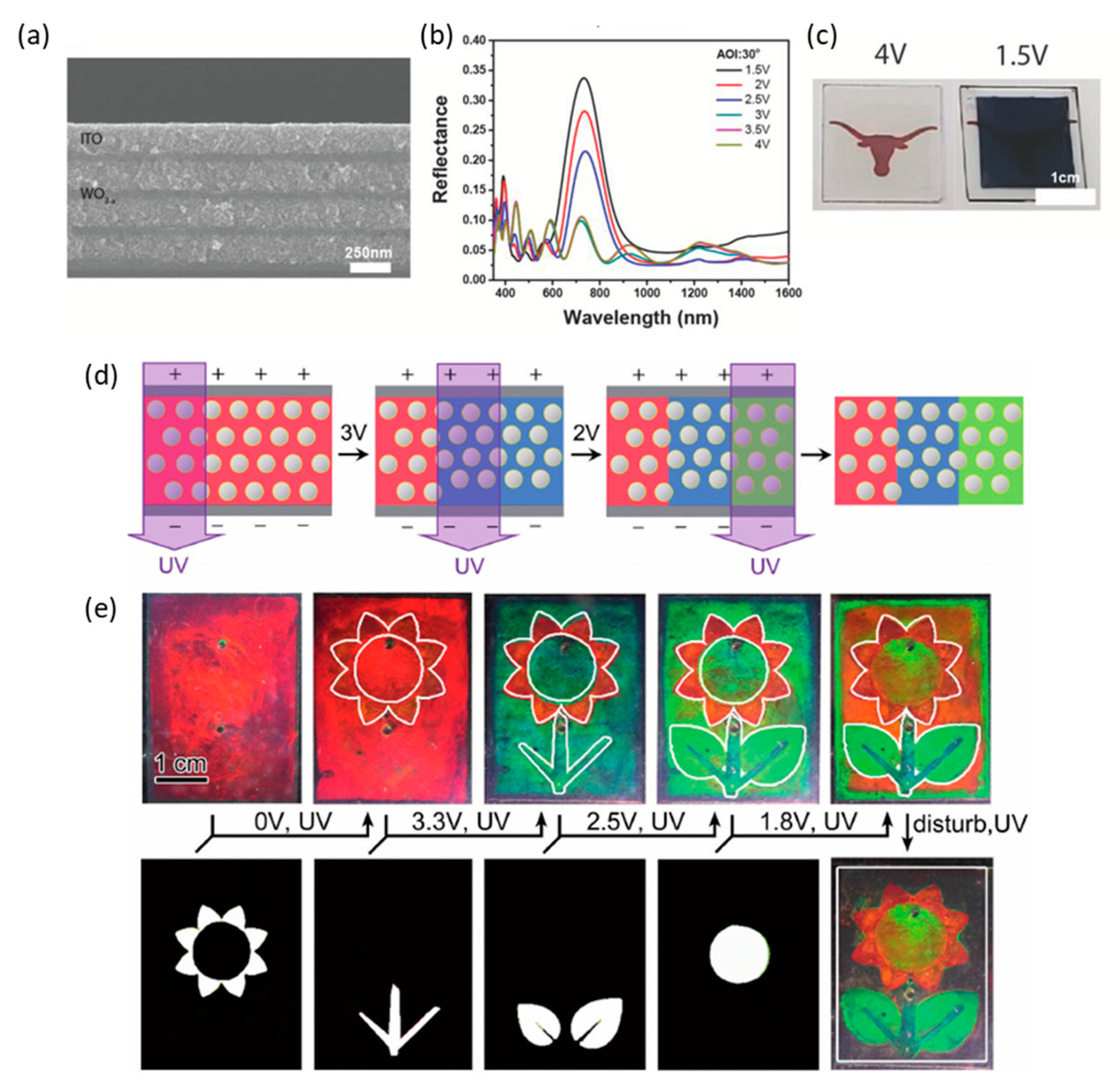
Table 18.	Approach	Advantages	References
Co-Extrusion	Bottom-up	Control of thickness Low cost Large area deposition High number of layers	[18]
Sputtering	Bottom-up	High reproducibility High yield High precision	[19,20,21]
Molecular Beam Epitaxy (MBE)	Top-down	High precision Instant thickness control High reproducibility	[22,23]
Pulsed Laser Deposition (PLD)	Bottom-up	High reproducibility High yield High precision	[24]
Glancing Angle Deposition (GLAD)	Bottom-up	High reproducibility Choose the deposition angle	[25,26]
Spin Coating	Bottom-up	High reproducibility Easy and fast execution	[27,28,29,30,31,32,33]
Nanoimprint Lithography	Top-down	Low cost High throughput High resolution	[34,35,36,37,38]
Self-Assemble	Bottom-up	Easy No need of instrumentation Reaction engineering	[39,40,41,42,43,44,45,46]
Plasma Enhanced Chemical Vapor Deposition (PECVD)	Bottom-up	High uniformity Low temperatures Fast deposition High yield	[20,47]
Inverse Opal Template	Bottom-up	Possibility to insert active materials in the structure	[48,49,50,51,52]

.

4. Active Tuning of the Photonic Band Gap

Any stimulus that can modify either the periodicity or the refractive index contrast (or both) of the PhC can lead to a shift of the PBG, according to Bragg–Snell’s law:

$${\mathsf{\lambda }}_{\max }=\frac{2}{\mathrm{m}}\mathrm{d}\sqrt{{\mathrm{n}}_{\mathrm{eff}}^2-{\sin }^2\mathsf{\theta }}$$

(7)

where λ_max is the wavelength of the maximum reflection (photonic band gap) peak, d is the lattice constant, m is the order of diffraction, n_eff is the effective refractive index and θ is the angle of incidence of the light with respect to the PhC [53]. It is noteworthy that the effective refractive index can be determined with different approaches [54]. A large number of external stimuli that are able to modulate the PBG are reported in the literature. Main examples include chemical, thermal, magnetic, biological, mechanical, light and electrical stimuli.

Table 2. Summary of main stimuli used for tunable photonic crystals.

Stimuli	Mechanism	References
Chemical	Infiltration of vapours, solvents or ions	[20,30,55,56,57,58,59,60,61]
Thermal	Application of ΔT to thermoresponsive materials	[48,62,63,64]
Magnetic	Interaction between an external magnetic field and magnetic nanoparticles	[65,66,67,68,69]
Biological	Detection of different species by functionalization with recognition groups	[27,40,42,43,70,71,72,73,74,75]
Mechanic	Application of a mechanical force to an elastomeric matrix	[17,31,39,44,47]
Light	Stimulation of photosensitive materials, dyes and LC by light	[51,75,76,77]
Electric	Reorientation of infiltrated LC	[78,79,80,81,82,83]
	Electrochemical process	[33,46,84,85,86,87,88,89,90]
	Electrophoretic forces	[91,92,93,94,95]

summarizes the main stimuli and tuning mechanisms mentioned in this article.

4.1. Chemical Stimuli

One of the most used methods to tune the PBG via chemical methods relies on the interaction between a soft structure (e.g., a hydrogel) and a given chemical species. For instance, the interaction with ions can generate a swelling and a shrinkage of the soft structure, which in turn changes the geometrical features of the PhC and leads to a shift of the photonic band gap [45,61,96,97,98]. Furthermore, this approach can be used for the detection H⁺ ions, and thus for building up pH sensors [45,60,62,99,100]. Another class of chemically tuned PhCs consist in the integration of porous materials in the PhC, in which refractive index modulation is given by infiltration of vapors [30,55,56,101] or solvents [20,29,57,58,59,102,103,104]. Wang et al. fabricated 1D photonic crystals alternating films of poly methyl methacrylate-co-hydroxyethyl methacrylate-co-ethylene glycol dimethacrylate (PMMA-co-PHEMA-co-PEGDMA) and titania nanoparticles by spin-coating, drastically changing its structural color when immersed in different solvents (Figure 2a,b) [32].

4.2. Thermal and Magnetic Stimuli

Photonic crystals fabricated using thermoresponsive materials like polymers or colloidal dispersions can be easily tuned through the application of a temperature gradient [48,62,63,105,106]. Chunfang et al. fabricated a SiO₂ PhC and infiltrated the pores with a thermo-sensitive Poly (N-isopropylacrylamide) (PNIPAM) hydrogel. The thermal variation generates a blue shift of the photonic band gap and exhibits a reversible response in the range from 24 °C to 31 °C [64].

Magnetically responsive PhCs are usually fabricated by integrating magnetic nanoparticles in the structure [16]. Herein, an external magnetic field interacts with the active material and changes its optical properties, orienting it according to the direction of the field [65,66,67,68,69,107,108,109]. Ge et al. synthesized some polyacrylate-capped superparamagnetic magnetite (Fe₃O₄) colloidal nanocrystal clusters (CNCs) with sizes from 30 to 180 nm. These clusters self-assembled into colloidal photonic crystals in solution. In Figure 2c,d, the change in the optical response is visible, as the magnetic field changes by controlling the distance between the sample and an NdFeB magnet [110].

4.3. Biologic Stimuli

Photonic crystals can be easily functionalized with appropriate recognition groups that allow for the detection of specific biomolecules. Due to the change in color, colorimetric detection is quick and easy [16,111]. These sensors change their optical properties not only when in contact with classical biomolecules like sugars, creatinine or glucose [40,112,113], but also larger ones such DNA [42,43,70,71] and proteins [72,73,74,114].

Recently, silver has been integrated inside 1D PhCs in order to exploit its antibacterial properties and detect the presence of bacteria [27,75,115,116,117]. Paternò et al. fabricated a hybrid plasmonic–photonic device applying a silver layer on top of a TiO₂/SiO₂ PhC. At the Ag/bacteria interface, there is a generation of polarization charges due to a “biodoping” mechanism. This triggers a change in the PBG of the sensor when exposed to Escherichia coli [27].

4.4. Mechanical Stimuli

Mechanically tuned photonic crystals exploit the elastic properties of the constituent materials. In general, they are usually compounded by an elastomeric matrix [17,39,44,47,51] that actively responds to mechanical stimuli. In this case, the mechanical force deforms the polymer and this changes the periodicity of the lattice, thus changing the optical response [16]. Karrock et al. fabricated a 400 nm periodical linear grating made of a nanostructured polydimethylsiloxane membrane by nanoimprinting replication. Subsequently, a high refractive index TiO2 nanoparticle layer was spin-coated, showing a guided-mode resonance. The elastomeric behavior of the membrane allows for a 20% elongation when subjected to stretching, varying the resonance peak position up to ≈80 nm. In Figure 2e, it is possible to see the change of structural colors brought about by the mechanical deformation [31].

4.5. Light Stimuli

Incorporating a photosensitive material [75,118,119,120] or a dye [77,121] inside a photonic crystal structure allows for tuning of its optical properties upon exposure to light stimuli. Light can change the refractive index or lead to a modification of the structural properties. For instance, PhCs can be used like a casing for liquid crystals and they will then be stimulated by light [50,51,76]. Paternò et al. fabricated an optically switchable SiO₂/ITO 1D photonic crystal. Through a UV-light photodoping process, it is possible to tune the indium tin oxide (ITO) plasmonic response in the near-infrared range and translate the effect to the visible light range, switching the optical properties of the device [8].

4.6. Electric Stimuli

Electrically tunable photonic crystals represent an incredible opportunity for technological applications, ranging from colorful displays to sensitive claddings and electrochromic windows. Their electrotunability can be triggered in three ways: (1) reorientation of infiltrated liquid crystals; (2) an electrochemical process; and (3) electrophoretic forces in crystalline colloidal arrays. Liquid crystals (LCs) are a class of materials combining the properties of solid crystals and fluids. According to their alignment axis orientation, LCs assume a different distribution inside the liquid, switching from a randomly distributed phase (nematic) to an oriented phase (smectic or chiral). These materials own different refractive indices in different directions, so by changing their phase it is possible tune their optical properties. Thus, it is possible to tune their dielectric constant by applying an external electric field. LCs are usually infiltrated inside a porous structure [78,79,80,81,82,122,123,124]. Criante et al. fabricated a porous silicon dioxide/zirconium dioxide 1D photonic crystal infiltrated with a nematic liquid crystal (Figure 3a). The device is tuned by applying an external electric field and so changing the LC alignment, producing a blue shift (Figure 3b) of the peak of 8 nm at 8 V [83].

Electrochemically tuned photonic crystals consist in an electrochemical cell immersed in a liquid electrolyte. By applying an electric field, it is possible to activate an electrolytic process, which promotes an oxidation–reduction effect or an acid–base exchange. The stimulus produces an electrostatic repulsion; thus, the original structure undergoes a destabilization due to a localized charge variation. The consequent reorganization of the structure generates a geometric variation of the sample, which, in accordance with Bragg–Snell’s law, causes a shift of the peak [46,84,85,86,125,126]. In the case of polymers, it is possible to incorporate an electro-responsive material inside the main chain, generating a swelling of the matrix under the application of the field [48,87,127,128,129]. Xiao et al. fabricated a WO₃-based electrochromic PhC by a facile, reproducible, one-step room temperature glancing-angle electron-beam evaporation (GLAD). By changing the deposition angle, it is possible to obtain layers with different porosities corresponding to different refractive indices. The PhC is then immersed in 1M LiClO₄ in propylene carbonate solution and subjected to an external electric field of −1.1 V (vs. Ag/AgCl). This leads to an electrochromic effect, as reported in Figure 3c. A gradual decrease of the reflectance and a shift of the reflection peak are attributed to colored Li_xWO₃, which decreases the optical thickness (reduces the refractive index) and increases the light absorption (Figure 3d,e). When an anodic potential is applied (+1.1 V), the process is completely reversed [26].

In recent years, the integration of plasmonic nanoparticles in PhCs has attracted the interest of the scientific community. In these systems, quantized carrier oscillations generate localized surface plasmon resonances (LSPRs) that span over a wide range of wavelengths, depending mostly on the charge carrier density and the surrounding refractive environment. For instance, the charge carrier density in heavily doped metal oxide nanocrystals lies in the infrared (IR) region [130,131,132] as it is significantly lower compared with bulk materials (10 ²¹ cm⁻³ and 10 ²³ cm⁻³, respectively). This allows for easy manipulation of this parameter and, hence, of the dielectric function upon application of external electrochemical bias. In particular, by applying an electric field it is possible to induce a capacitive depletion or accumulation and, consequently, a modulation of optical properties [65,132,133]. For these reasons, photonic crystals fabricated with plasmonic materials [27,33,65,88,89,134] have emerged in the in the last decade. Heo et al. exploited these materials to manufacture 1D photonic crystals composed of alternated layers of WO_3-x and indium tin oxide (ITO) nanocrystals. In this case, the selected materials show a very similar refractive index in the discharge state (2.19 for ITO and 2.1 for WO_3-x in the bulk), while charging leads to a strong modification in the WO_3-x refractive index, thus causing a change of the refractive index contrast (Figure 4a–c). Interestingly, the same procedure can be used to deposit the photonic crystals on an ITO-coated flexible polyethylene terephthalate substrate [90].

Another approach relies on the immersion of electrotunable PhCs in liquid electrolytes, with the aim to increase the migration of electrons and ions. Despite the relatively high electrotunability achieved in such devices, the electrolyte can lead to a degradation of the samples and restrict the possibilities of their applications [26,90,135]. To address this problem, all solid-state devices have been developed over the past decade, in order to minimize such a detrimental effect [8,49,136] For instance, we have recently proposed a 1D electrolyte-free photonic crystal, combining indium tin oxide nanoparticles with TiO₂ nanoparticles on top of a fluorine-doped tin oxide substrate acting as an electrode. The structure was contacted with a top fluorine-doped tin oxide (FTO) substrate and clipped with a paper binder to ensure mechanical stability. By applying a bias to this circuit, charges accumulate at the doped semiconductor/TiO₂ interface, leading to an increase of the charge carrier density and an increase of the plasma frequency, according to equation:

$${\omega }_p=\sqrt{\frac{N{e}^2}{m^{*}{\epsilon }_0} }$$

(8)

where N is the carrier density, e is the electron charge, ε₀ is the dielectric constant under vacuum and m* is the effective mass [33].

Tunability of photonic crystals can be also achieved by means of electrophoretic forces, which occurs when an external electric field is applied on a high concentration colloidal system. In this way, the particles are in dynamic equilibrium between packing force and electrostatic repulsive force, leading to a specific interparticle distance and, thus, to a specific optical signal. The applied field generates an electrophoretic force between the particles that are forced to reorganize into a more stable structure. Finally, this lattice modification translates into a shift of the photonic band gap [41,92,93,94,95,137]. For instance, Chen et al. exploited this mechanism to fabricate an electric-field-assisted multicolor printing (Figure 4d,e) based on electrically tunable and photocurable colloidal photonic crystals [91].

5. Conclusions

In this review, we have summarized some notable examples of tunable and stimuli-responsive 1D photonic crystals, with particular emphasis on electrotunable devices. These systems, which can usually be fabricated using easy and low-cost processes, permit the conversion of an external stimulus into an easily recognizable optical response. Given these properties, they have attracted increasing attention from both the scientific community and industry, as they can be employed in a wide range of applications, such as display, sensing and lighting.