Computation of Effective Elastic Properties Using a Three-Dimensional Semi-Analytical Approach for Transversely Isotropic Nanocomposites
Abstract
1. Introduction
2. Statement of the Problem for Heterogeneous Media
3. Asymptotic Homogenization Method: Homogeneous Problem, Local Problems and Effective Coefficients
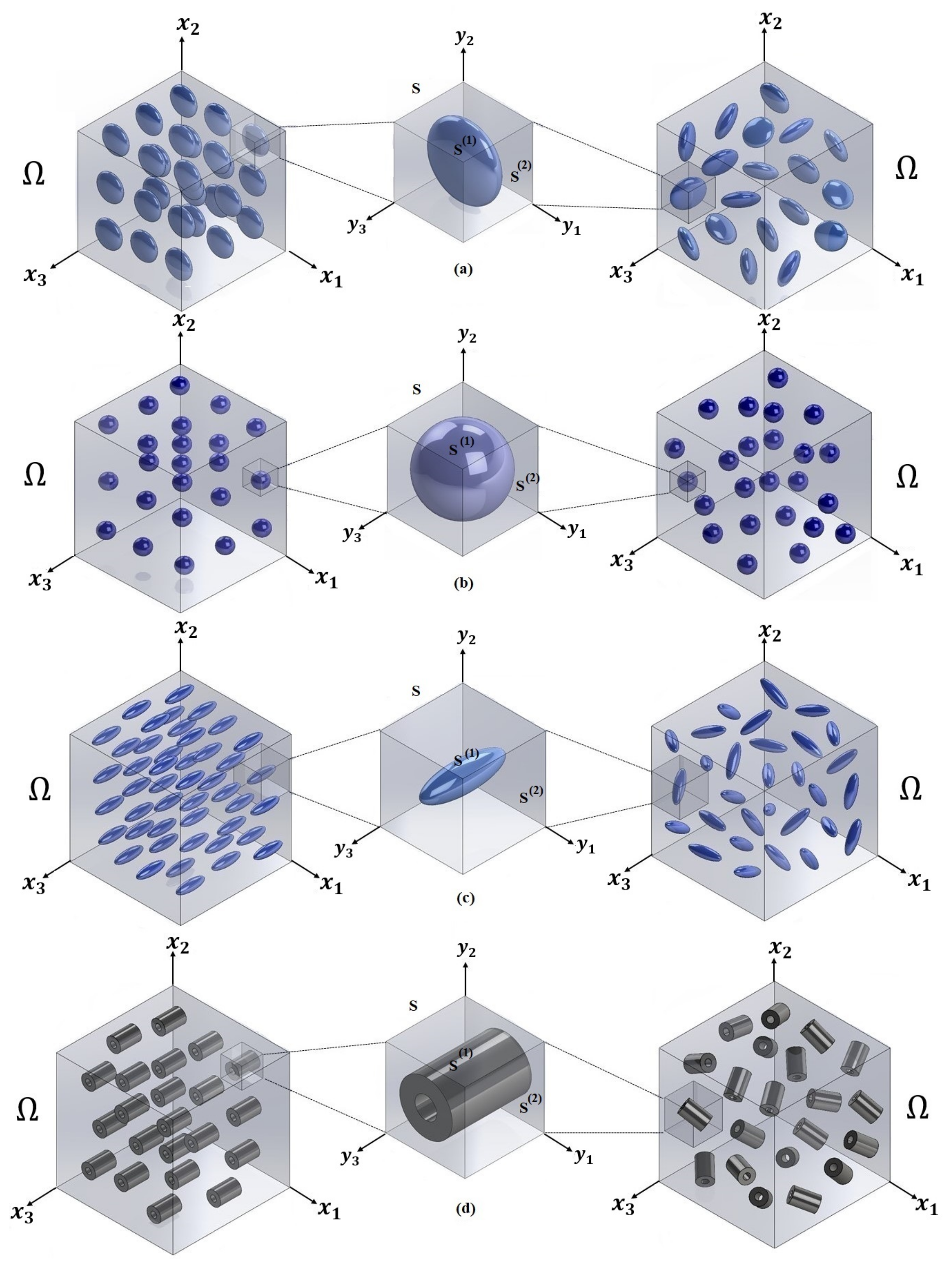
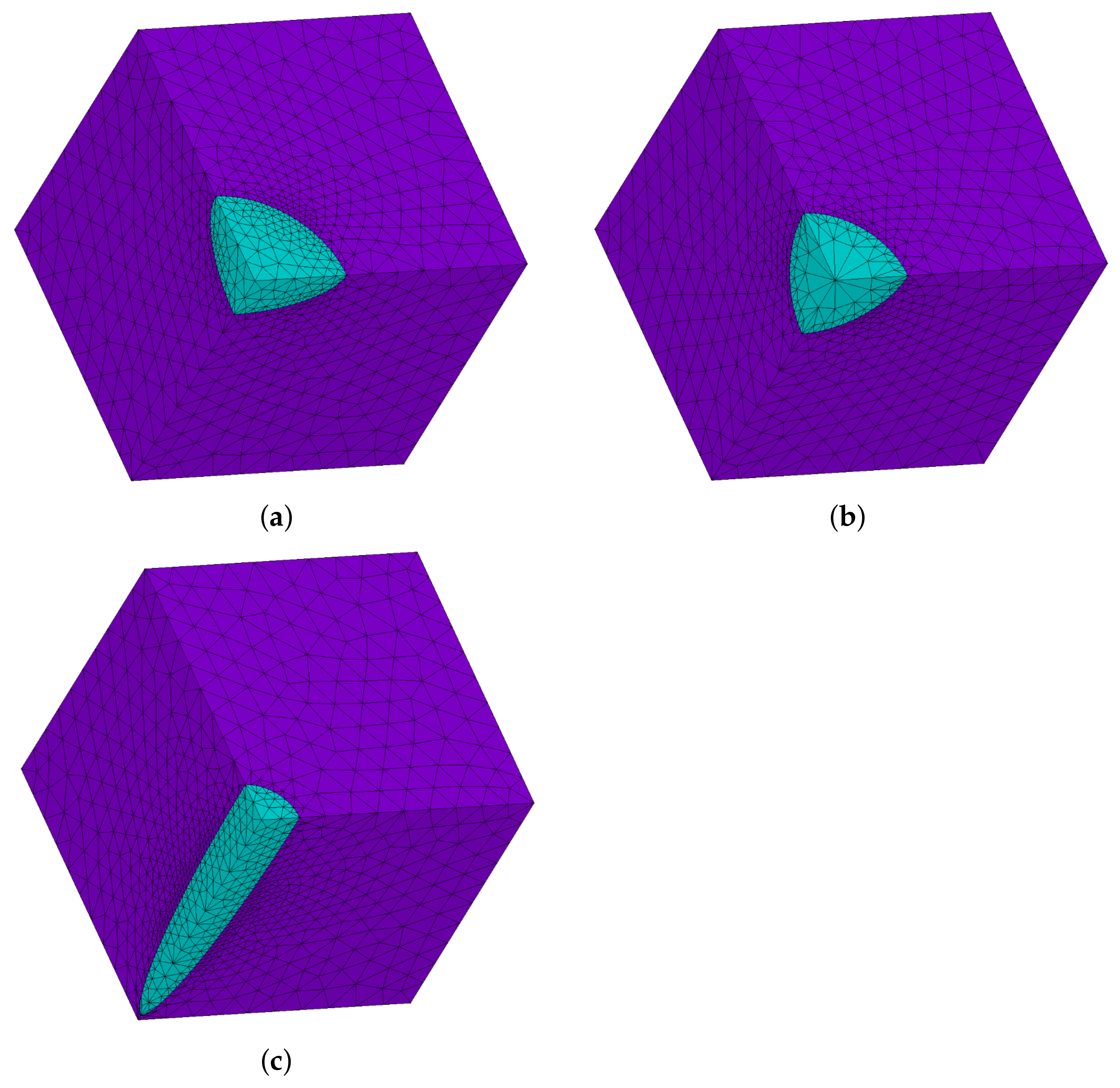
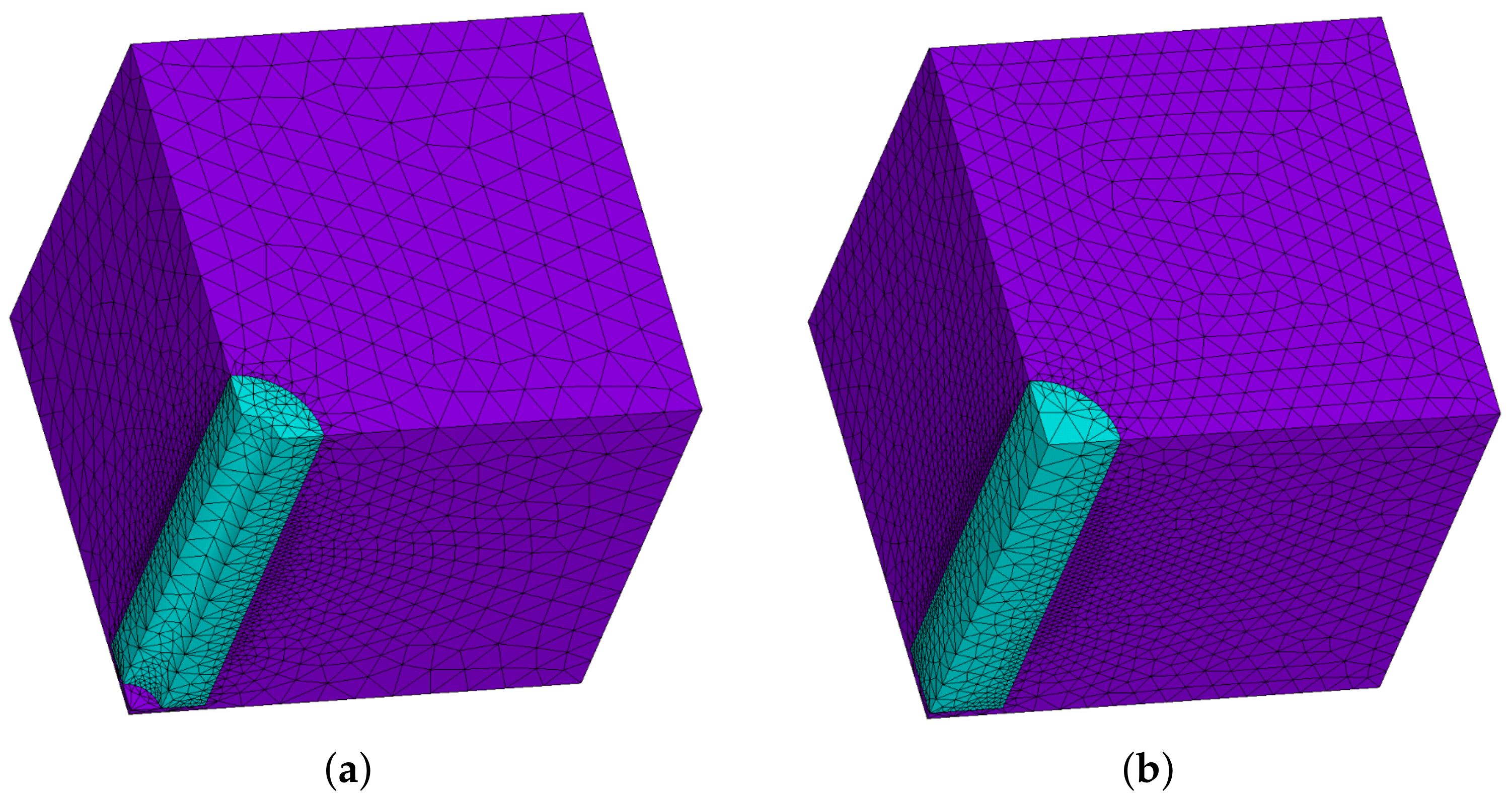
Solution of Local Problems through the Finite Element Method
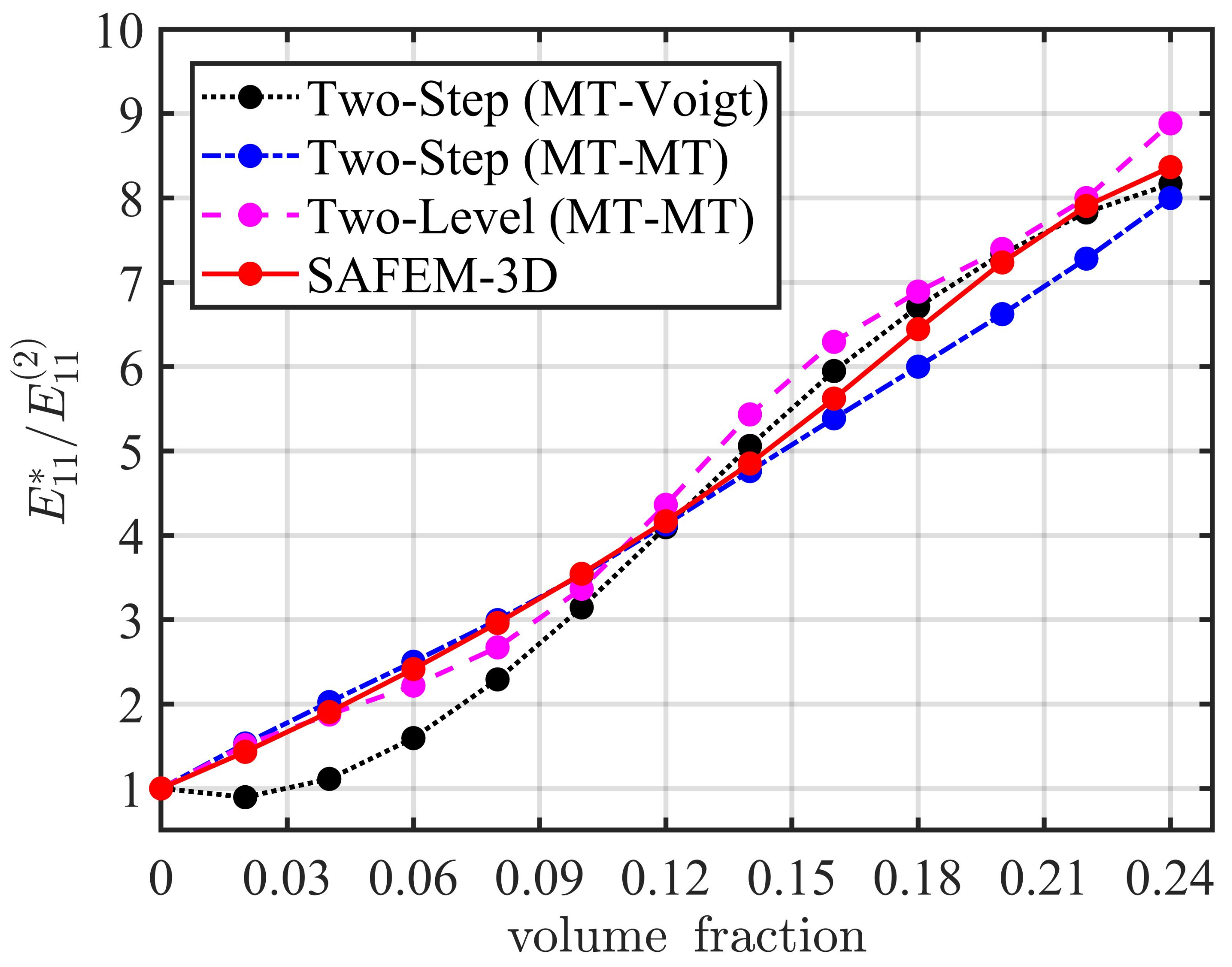
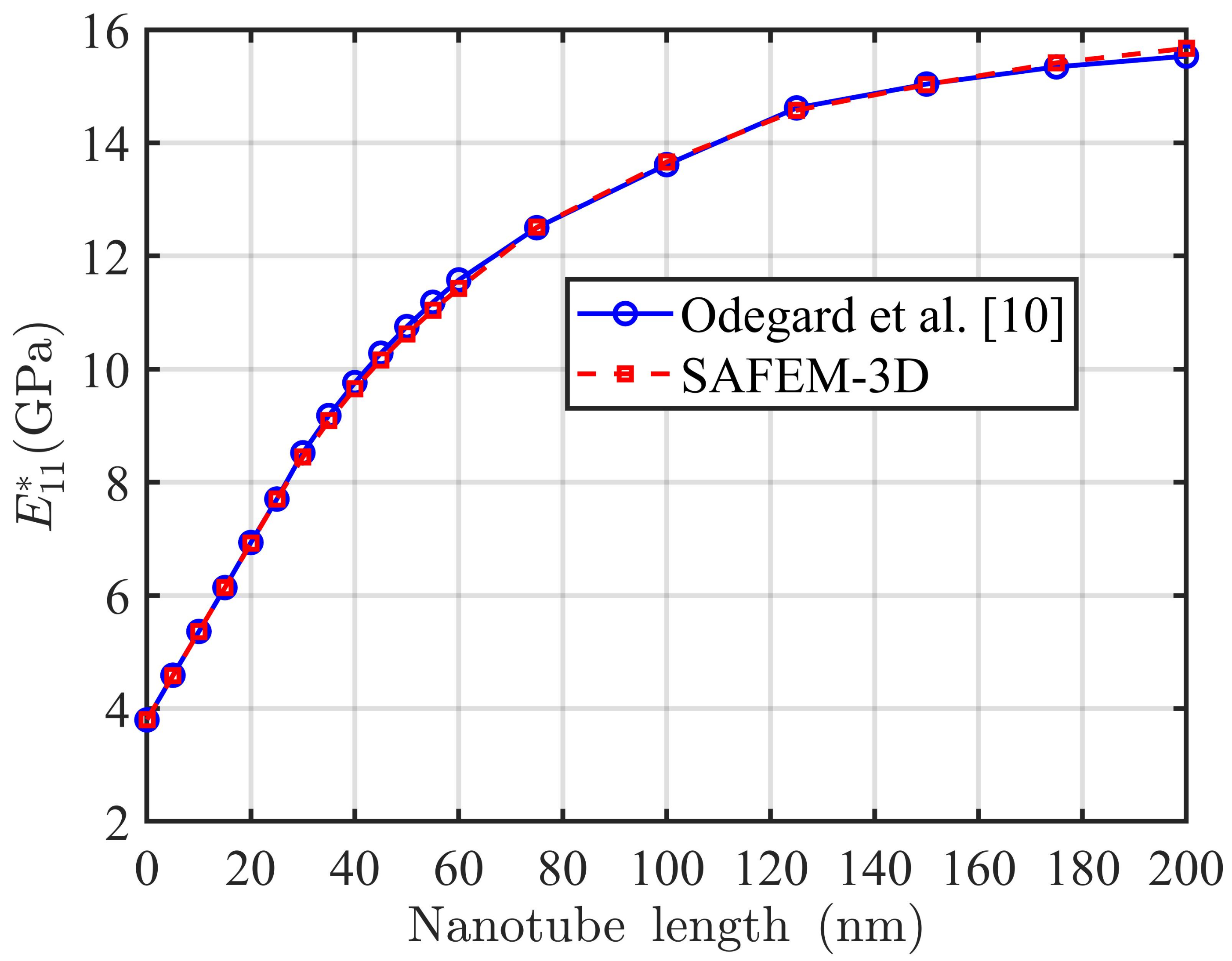
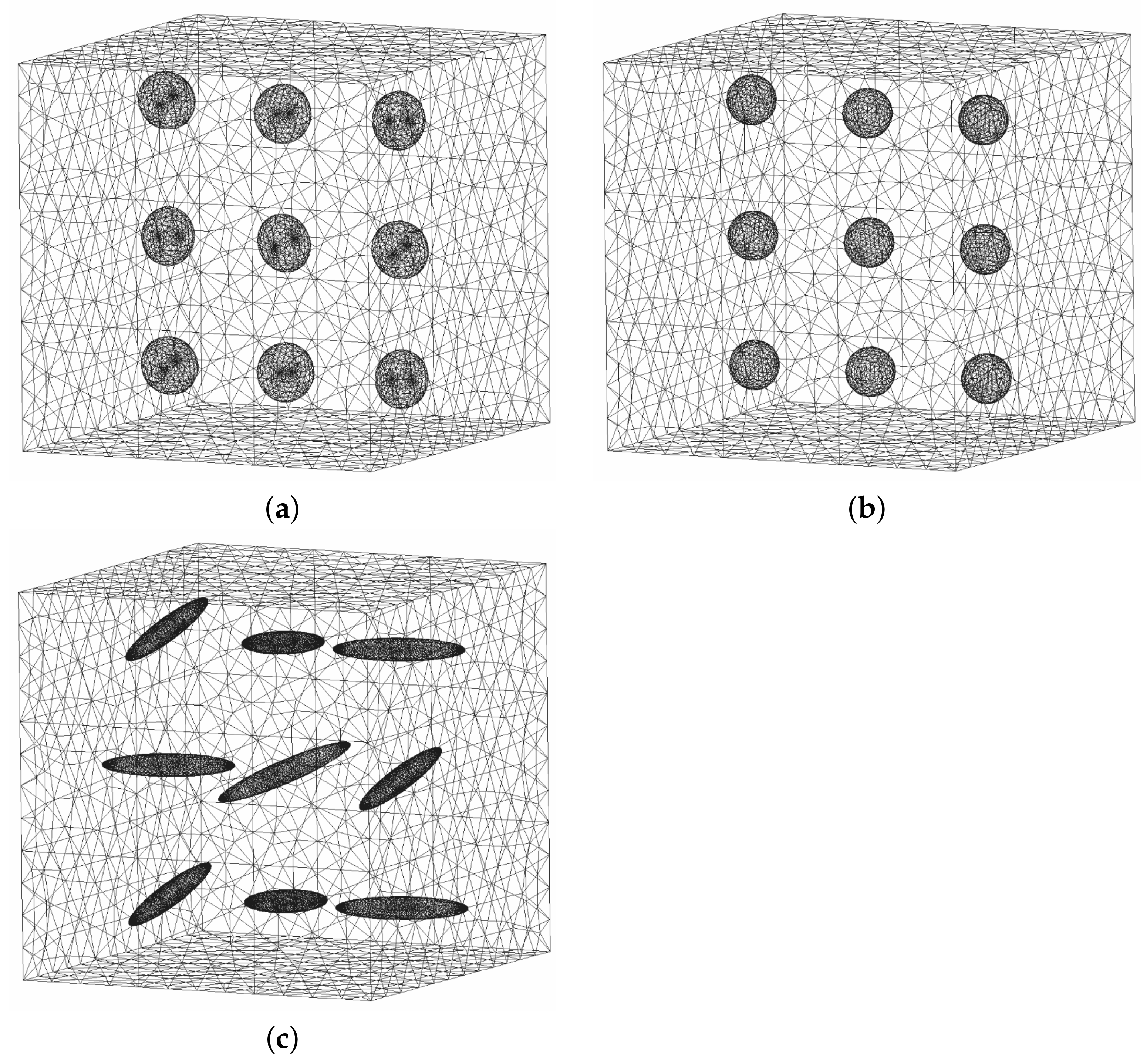
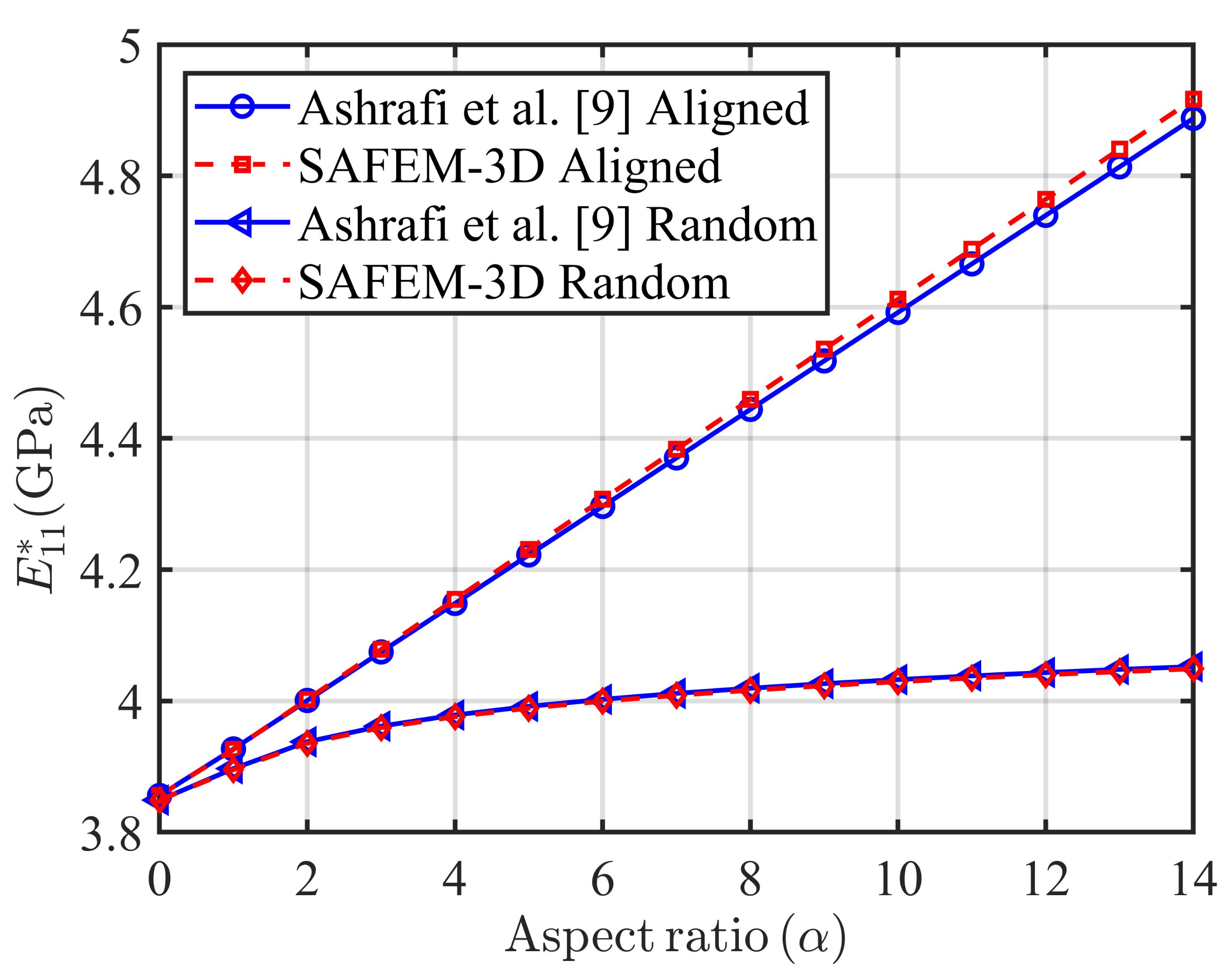
4. Results and Discussion
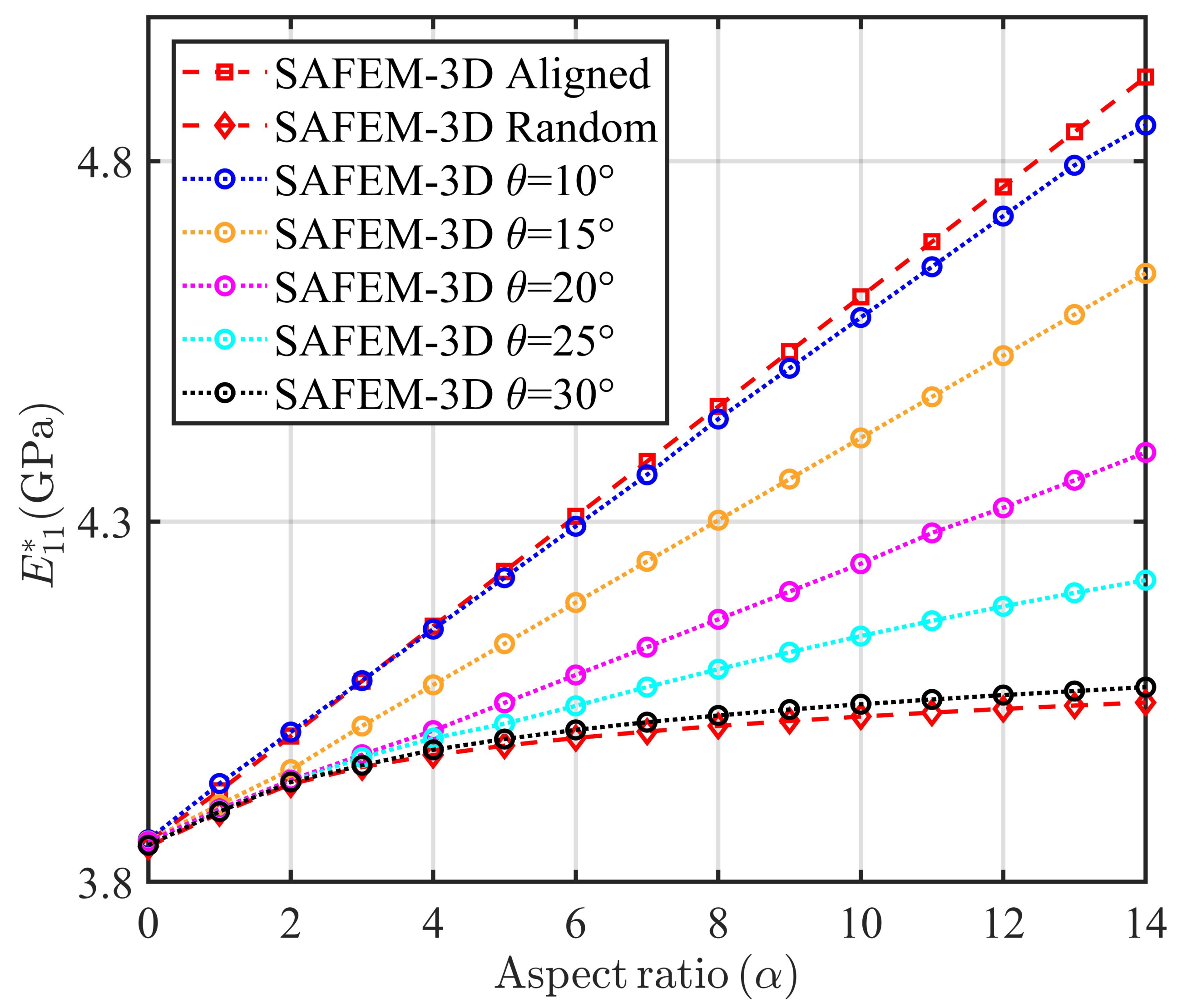
4.1. Aligned NTC-Reinforced Composites
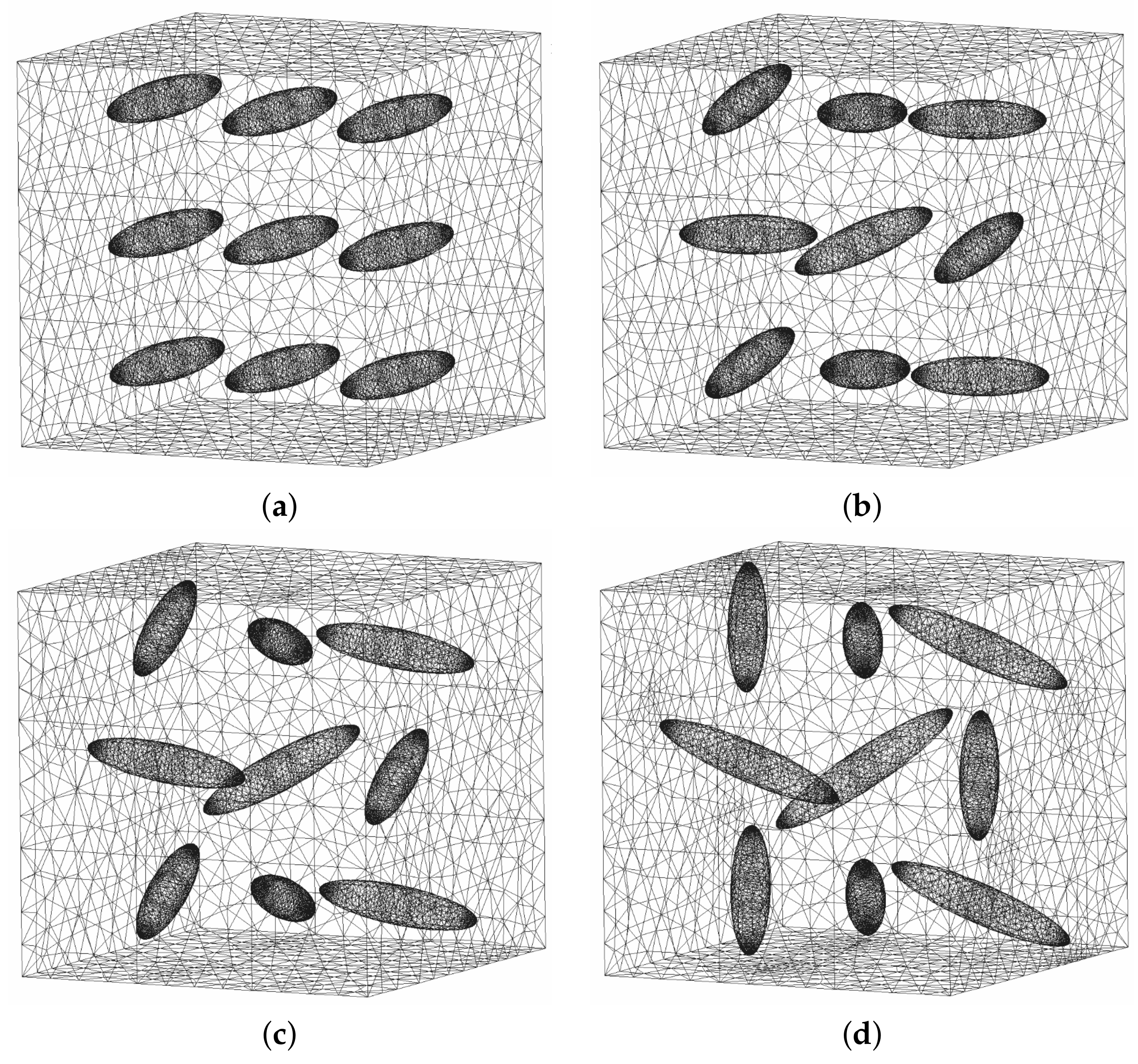
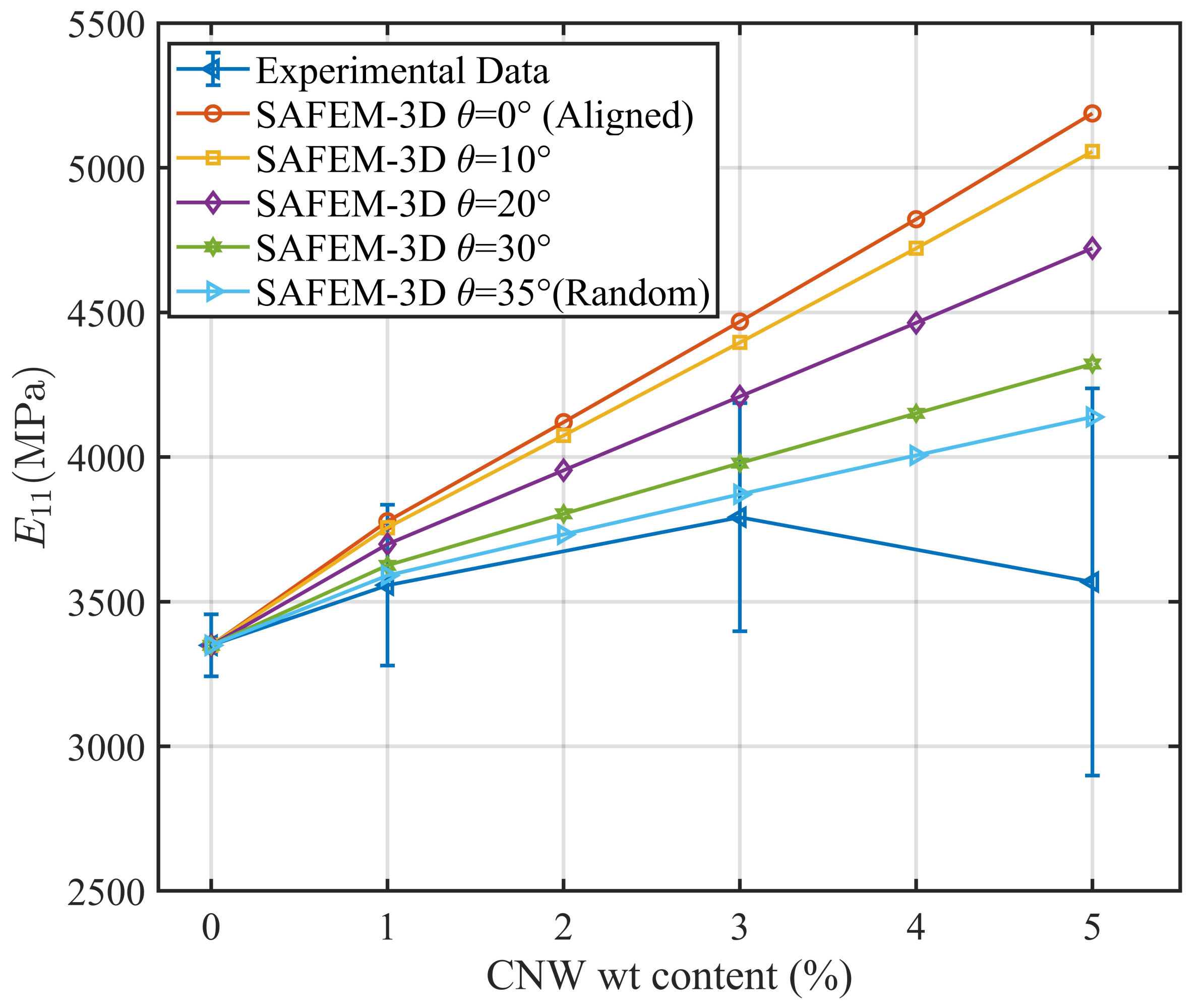
4.2. Random NTC Reinforced Composites
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Mathematical Derivation of Local Problems and Homogenized Equation
Appendix B. Boundary Conditions
- ,
- and
- .
Appendix C. Matrix H
Appendix D. Steps for the Numerical Implementation
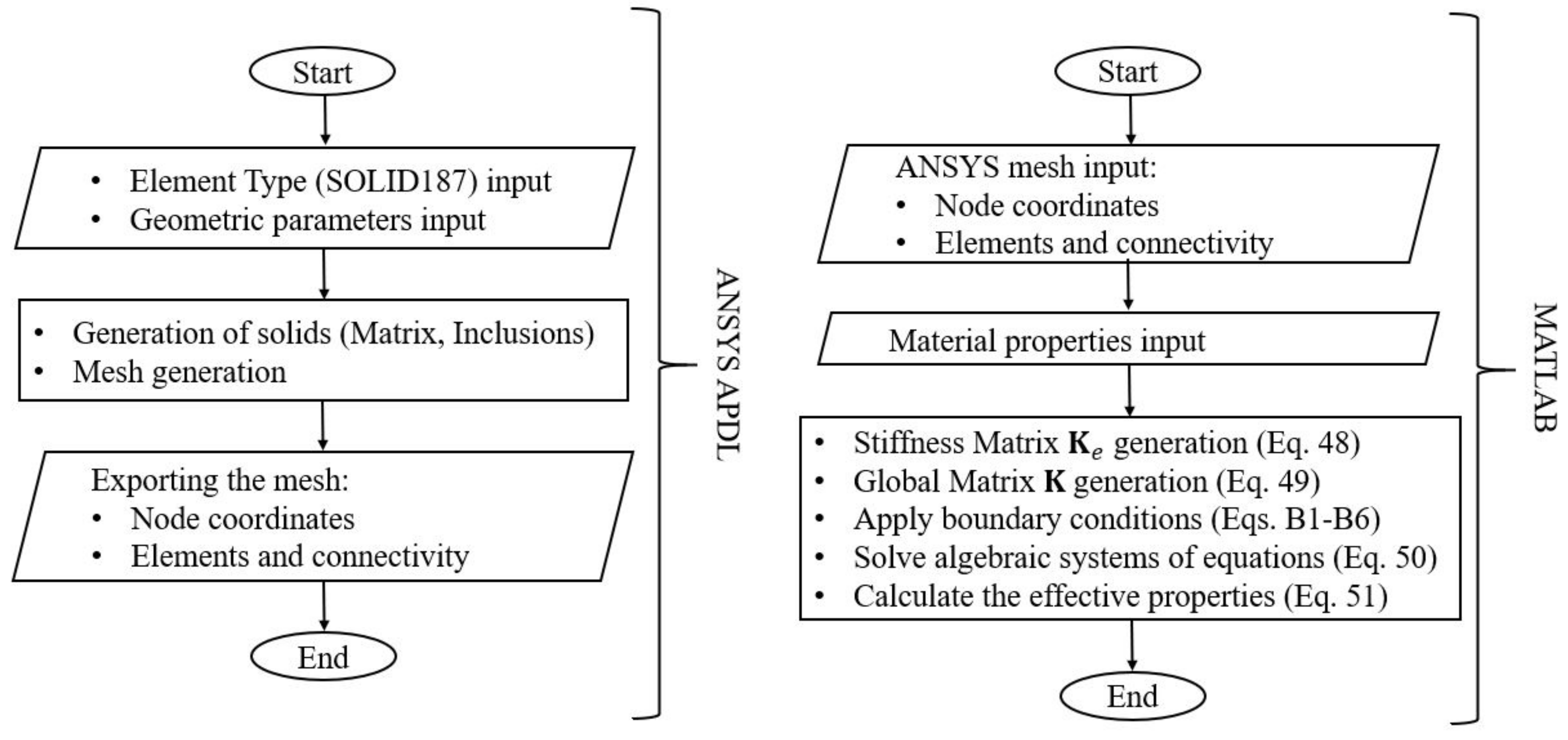
References
- Kausar, A.; Rafique, I.; Muhammad, B. Review of applications of polymer/carbon nanotubes and epoxy/CNT composites. Polym. Plast. Technol. Eng. 2016, 55, 1167–1191. [Google Scholar] [CrossRef]
- Fujisawa, K.; Kim, H.J.; Go, S.H.; Muramatsu, H.; Hayashi, T.; Endo, M.; Hirschmann, T.C.; Dresselhaus, M.S.; Kim, Y.A.; Araujo, P.T. A Review of Double-Walled and Triple-Walled Carbon Nanotube Synthesis and Applications. Appl. Sci. 2016, 6, 109. [Google Scholar] [CrossRef]
- Kausar, A.; Rafique, I.; Muhammad, B. Aerospace application of polymer nanocomposite with carbon nanotube, graphite, graphene oxide, and nanoclay. Polym. Plast. Technol. Eng. 2017, 56, 1438–1456. [Google Scholar] [CrossRef]
- Elmarakbi, A.; Karagiannidis, P.; Ciappa, A.; Innocente, F.; Galise, F.; Martorana, B.; Bertocchi, F.; Cristiano, F.; Villaro, E.; Gomez, J. 3-Phase hierarchical graphene-based epoxy nanocomposite laminates for automotive applications. J. Mater. Sci. Technol. 2019, 35, 2169–2177. [Google Scholar] [CrossRef]
- Oraon, R.; De Adhikari, A.; Tiwari, S.K.; Nayak, G.C. Enhanced specific capacitance of self-assembled three-dimensional carbon nanotube/layered silicate/polyaniline hybrid sandwiched nanocomposite for supercapacitor applications. ACS Sustain. Chem. Eng. 2016, 4, 1392–1403. [Google Scholar] [CrossRef]
- Kinloch, I.A.; Suhr, J.; Lou, J.; Young, R.J.; Ajayan, P.M. Composites with carbon nanotubes and graphene: An outlook. Science 2018, 362, 547–553. [Google Scholar] [CrossRef]
- DeGraff, J.; Liang, R.; Le, M.Q.; Capsal, J.F.; Ganet, F.; Cottinet, P.J. Printable low-cost and flexible carbon nanotube buckypaper motion sensors. Mater. Des. 2017, 133, 47–53. [Google Scholar] [CrossRef]
- Kim, J.H.; Hwang, J.Y.; Hwang, H.; Kim, H.S.; Lee, J.H.; Seo, J.W.; Shin, U.S.; Lee, S.H. Simple and cost-effective method of highly conductive and elastic carbon nanotube/polydimethylsiloxane composite for wearable electronics. Sci. Rep. 2018, 8, 1–11. [Google Scholar] [CrossRef]
- Go, M.-S.; Park, S.-M.; Kim, D.-W.; Hwang, D.-S.; Lim, J.H. Random Fiber Array Generation Considering Actual Noncircular Fibers with a Particle-Shape Library. Appl. Sci. 2020, 10, 5675. [Google Scholar] [CrossRef]
- Kostas, V.; Baikousi, M.; Barkoula, N.-M.; Giannakas, A.; Kouloumpis, A.; Avgeropoulos, A.; Gournis, D.; Karakassides, M.A. Synthesis, Characterization and Mechanical Properties of Nanocomposites Based on Novel Carbon Nanowires and Polystyrene. Appl. Sci. 2020, 10, 5737. [Google Scholar] [CrossRef]
- Weng, G.J. Some elastic properties of reinforced solids, with special reference to isotropic ones containing spherical inclusions. Int. J. Eng. Sci. 1984, 22, 845–856. [Google Scholar] [CrossRef]
- Odegard, G.M.; Gates, T.S.; Wise, K.E.; Park, C.; Siochi, E.J. Constitutive modeling of nanotube–reinforced polymer composites. Compos. Sci. Technol. 2003, 63, 1671–1687. [Google Scholar] [CrossRef]
- Ashrafi, B.; Hubert, P. Modeling the elastic properties of carbon nanotube array/polymer composites. Compos. Sci. Technol. 2006, 66, 387–396. [Google Scholar] [CrossRef]
- Selmi, A.; Friebel, C.; Doghri, I.; Hassis, H. Prediction of the elastic properties of single walled carbon nanotube reinforced polymers: A comparative study of several micromechanical models. Compos. Sci. Technol. 2007, 67, 2071–2084. [Google Scholar] [CrossRef]
- Guinovart-Díaz, R.; Rodríguez-Ramos, R.; Bravo-Castillero, J.; Sabina, F.J.; Otero- Hernández, J.A.; Maugin, G.A. A recursive asymptotic homogenization scheme for multi-phase fibrous elastic composites. Mech. Mater. 2005, 37, 1119–1131. [Google Scholar] [CrossRef]
- Hadjiloizi, D.A.; Georgiades, A.V.; Kalamkarov, A.L. Dynamic modeling and determination of effective properties of smart composite plates with rapidly varying thickness. Int. J. Eng. Sci. 2012, 56, 63–85. [Google Scholar] [CrossRef]
- Bakhvalov, N.S.; Panasenko, G.P. Homogenisation: Averaging Processes in Periodic Media; (Soviet Series); Kluwer Academic Publishers Group: Dordrecht, The Netherlands, 1989. [Google Scholar]
- Challagulla, K.S.; Georgiades, A.V.; Kalamkarov, A.L. Asymptotic homogenization modeling of smart composite generally orthotropic grid-reinforced shells: Part I–theory. Eur. J. Mech. A Solids 2010, 29, 530–540. [Google Scholar] [CrossRef]
- Berger, H.; Kari, S.; Gabbert, U.; Rodríguez-Ramos, R.; Bravo-Castillero, J.; Guinovart-Díaz, R. Calculation of effective coefficients for piezoelectric fiber composites based on a general numerical homogenization technique. Compos. Struct. 2005, 71, 397–400. [Google Scholar] [CrossRef]
- Kalamkarov, A.L.; Andrianov, I.V.; Danishevs’kyy, V.V. Asymptotic homogenization of composite materials and structures. Appl. Mech. Rev. 2009, 62, 030802. [Google Scholar] [CrossRef]
- Pal, G.; Kumar, S. Modeling of carbon nanotubes and carbon nanotube–polymer composites. Prog. Aerosp. Sci. 2016, 80, 33–58. [Google Scholar] [CrossRef]
- Bravo-Castillero, J.; Rodríguez-Ramos, R.; Mechkour, H.; Otero, J.A.; Hernández-Cabanas, J.; Sixto, L.M.; Guinovart-Díaz, R.; Sabina, F.J. Homogenization and effective properties of periodic thermomagnetoelectroelastic composites. J. Mech. Mater. Struct. 2009, 4, 819–836. [Google Scholar] [CrossRef]
- Rodríguez-Ramos, R.; Berger, H.; Guinovart-Díaz, R.; López-Realpozo, J.C.; Würkner, M.; Gabbert, U.; Bravo-Castillero, J. Two approaches for the evaluation of the effective properties of elastic composite with parallelogram periodic cells. Int. J. Eng. Sci. 2012, 58, 2–10. [Google Scholar] [CrossRef]
- Zhao, J.; Li, H.; Cheng, G.; Cai, Y. On predicting the effective elastic properties of polymer nanocomposites by novel numerical implementation of asymptotic homogenization method. Compos. Struct. 2016, 135, 297–305. [Google Scholar] [CrossRef]
- Baek, K.; Shin, H.; Yoo, T.; Cho, M. Two-step multiscale homogenization for mechanical behaviour of polymeric nanocomposites with nanoparticulate agglomerations. Compos. Sci. Technol. 2019, 179, 97–105. [Google Scholar] [CrossRef]
- Rossi, M.; Meo, M. On the estimation of mechanical properties of single-walled carbon nanotubes by using a molecular-mechanics based FE approach. Compos. Sci. Technol. 2009, 69, 1394–1398. [Google Scholar] [CrossRef]
- Cheng, G.-D.; Cai, Y.-W.; Xu, L. Novel implementation of homogenization method to predict effective properties of periodic materials. Acta Mech. Sin. 2013, 29, 550–556. [Google Scholar] [CrossRef]
- Kassa, M.K.; Arumugam, A.B. Micromechanical modeling and characterization of elastic behavior of carbon nanotube-reinforced polymer nanocomposites: A combined numerical approach and experimental verification. Polym. Compos. 2020, 41, 3322–3339. [Google Scholar] [CrossRef]
- Otero, J.A.; Rodríguez-Ramos, R.; Monsivais, G. Computation of effective properties in elastic composites under imperfect contact with different inclusion shapes. Math. Meth. Appl. Sci. 2016, 40, 3290–3310. [Google Scholar] [CrossRef]
- Espinosa-Almeyda, Y.; Camacho-Montes, H.; Otero, J.A.; Rodríguez-Ramos, R.; López-Realpozo, J.C.; Guinovart-Díaz, R.; Sabina, F.J. Interphase effect on the effective magneto-electro-elastic properties for three-phase fiber-reinforced composites by a semi-analytical approach. Int. J. Eng. Sci. 2020, 154, 103310. [Google Scholar] [CrossRef]
- Gabbert, U.; Kari, S.; Bohn, N.; Berge, H. Numerical homogenization and optimization of smart composite materials. In Mechanics and Model-Based Control of Smart Materials and Structures; Irschik, H., Ed.; Springer: Vienna, Austria, 2010; pp. 59–68. [Google Scholar]
- Nasution, M.R.E.; Watanabe, N.; Kondo, A.; Yudhanto, A. A novel asymptotic expansion homogenization analysis for 3-D composite with relieved periodicity in the thickness direction. Compos. Sci. Technol. 2014, 97, 63–73. [Google Scholar] [CrossRef]
- Pike, M.G.; Oskay, C. Three-Dimensional Modeling of Short Fiber-Reinforced Composites with Extended Finite-Element Method. J. Eng. Mech. 2016, 142, 1–12. [Google Scholar] [CrossRef]
- Fisher, F.T.; Bradshaw, R.D.; Brinson, L.C. Effects of nanotube waviness on the modulus of nanotube-reinforced polymers. Appl. Phys. Lett. 2002, 80, 4647–4649. [Google Scholar] [CrossRef]
- Fisher, F.T.; Bradshaw, R.D.; Brinson, L.C. Fiber waviness in nanotube–reinforced polymer composites—I: Modulus predictions using effective nanotube properties. Compos. Sci. Technol. 2003, 63, 1689–1703. [Google Scholar] [CrossRef]
- Thakur, A.K.; Kumar, P.; Srinivas, J. Studies on Effective Elastic Properties of CNT/Nano-Clay Reinforced Polymer Hybrid Composite. IOP Conf. Ser. Mater. Sci. Eng. 2016, 115, 12007. [Google Scholar] [CrossRef]
- David Müzel, S.; Bonhin, E.P.; Guimarães, N.M.; Guidi, E.S. Application of the Finite Element Method in the Analysis of Composite Materials: A Review. Polymers 2020, 12, 818. [Google Scholar] [CrossRef]
- Tarfaoui, M.; Lafdi, K.; El Moumen, A. Mechanical properties of carbon nanotubes based polymer composites. Compos. Part B Eng. 2016, 103, 113–121. [Google Scholar] [CrossRef]
- Choi, J.; Shin, H.; Cho, M. A multiscale mechanical model for the effective interphase of SWNT/epoxy nanocomposite. Polymer 2016, 89, 159–171. [Google Scholar] [CrossRef]
- El Moumen, A.; Tarfaoui, M.; Lafdi, K. Computational Homogenization of Mechanical Properties for Laminate Composites Reinforced with Thin Film Made of Carbon Nanotubes. Appl. Compos. Mater. 2017, 25, 569–588. [Google Scholar] [CrossRef]
- Yengejeh, S.I.; Kazemi, S.A.; Öchsner, A. Carbon nanotubes as reinforcement in composites: A review of the analytical, numerical and experimental approaches. Comput. Mater. Sci. 2017, 136, 85–101. [Google Scholar] [CrossRef]
- Saad, M.H. Elasticity: Theory, Applications, and Numerics; Elsevier: Burlington, MA, USA; Butterworth Heinemann: Oxford, UK, 2005. [Google Scholar]
- Landau, L.D.; Lifshits, E.M. Theory of Elasticity, 2nd ed.; Pergamon Press Inc.: New York, NY, USA, 1970. [Google Scholar]
- Bensoussan, A.; Lions, J.L.; Papanicolaou, G. Asymptotic Analysis for Periodic Structures; North-Holland: New York, NY, USA, 1978. [Google Scholar]
- Sanchez-Palencia, E. Non-Homogeneous Media and Vibration Theory; Lecture Notes in Physics, 127; Springer: Berlin, Germany, 1980. [Google Scholar]
- Otero, J.A.; Rodríguez-Ramos, R.; Bravo-Castillero, J.; Guinovart-Díaz, R.; Sabina, F.J.; Monsivais, G. Semi-analytical method for computing effective properties in elastic composite under imperfect contact. Int. J. Solids Struct. 2013, 50, 609–622. [Google Scholar] [CrossRef]
- Chandrupatla, T.R.; Belegundu, A.D. Introduction to Finite Elements in Engineering, 3rd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2002; pp. 13–102. [Google Scholar]
- Zienkiewicz, O.C.; Taylor, R.L.; Zhu, J.Z. The Finite Element Method: Its Basis and Fundamentals, 7th ed.; Butterworth-Heinemann: Oxford, UK, 2013. [Google Scholar]
- Dvorak, G.J. Micromechanics of Composite Materials, 1st ed.; Springer: Dordrecht, The Netherlands, 2013. [Google Scholar]
- Sabina, F.J.; Gandarrilla-Pérez, C.A.; Otero, J.A.; Rodríguez-Ramos, R.; Bravo-Castillero, J.; Guinovart-Díaz, R.; Valdiviezo-Mijangos, O. Dynamic homogenization for composites with embedded multioriented ellipsoidal inclusions. Int. J. Solids Struct. 2015, 69, 121–130. [Google Scholar] [CrossRef]
- Sohn, Y.-S.; Park, J.; Yoon, G.; Song, J.; Jee, S.-W.; Lee, J.-H.; Na, S.; Kwon, T.; Eom, K. Mechanical Properties of Silicon Nanowires. Nanoscale Res. Lett. 2010, 5, 211–216. [Google Scholar] [CrossRef]

| 0 | 0 | 0 | |||
| 0 | 0 | 0 | |||
| 0 | 0 | 0 | |||
| 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 0 | 0 | 0 |
| Epoxy [11] | Graphite [11] | LaRC-SI [12,13,14] | SWNT [14] | Matrix [46] | Inclusion [46] | |
|---|---|---|---|---|---|---|
| 8.93 | 234.23 | 8.14 | 3024 | 94.23 | 483.68 | |
| 6.47 | 12.14 | 5.42 | 1008 | 40.38 | 99.06 | |
| 6.47 | 12.14 | 5.42 | 1008 | 40.38 | 99.06 | |
| 8.93 | 28.25 | 8.14 | 3024 | 94.23 | 483.68 | |
| 2.46 | 16.12 | 2.71 | 2016 | 53.84 | 384.61 | |
| 2.46 | 20.00 | 2.71 | 2016 | 53.84 | 384.61 | |
| SiC [50] | Al 6061 [50] | Matrix [50] | SWNT [12] | SWNT [13] | ||
| 474.20 | 110.50 | 265.30 | 1074.8 | 586.1 | ||
| 98.00 | 57.10 | 83.70 | 220.1 | 17.09 | ||
| 98.00 | 57.10 | 83.70 | 220.1 | 17.09 | ||
| 474.20 | 110.50 | 265.30 | 1074.8 | 49.96 | ||
| 188.10 | 26.70 | 90.80 | 854.7 | 4.94 | ||
| 188.10 | 26.70 | 90.80 | 854.7 | 34.4 |
| AHM | SAFEM-2D | SAFEM-3D | |||
| 0.05 | 1.0556 | 1.0556 | 1.0555 | 0.0095 | 0.0095 |
| 0.2 | 1.2606 | 1.2607 | 1.2519 | 0.6949 | 0.7029 |
| 0.35 | 1.5436 | 1.5436 | 1.5266 | 1.1136 | 1.1136 |
| AHM | SAFEM-2D | SAFEM-3D | |||
| 0.05 | 1.0405 | 1.0445 | 1.0427 | 0.2110 | 0.1726 |
| 0.2 | 1.1565 | 1.1565 | 1.2119 | 4.5713 | 4.5713 |
| 0.35 | 1.2599 | 1.2599 | 1.4511 | 13.1762 | 13.1762 |
| AHM | SAFEM-2D | SAFEM-3D | |||
| 0.05 | 1.0223 | 1.0223 | 1.0296 | 0.7090 | 0.7090 |
| 0.2 | 1.1001 | 1.1001 | 1.1269 | 2.3782 | 2.3782 |
| 0.35 | 1.2001 | 1.2001 | 1.2343 | 2.7708 | 2.7708 |
| AHM | SAFEM-2D | SAFEM-3D | |||
| 0.05 | 1.2010 | 1.2010 | 1.1526 | 4.1992 | 4.1992 |
| 0.2 | 1.8052 | 1.8052 | 1.5447 | 16.8641 | 16.8641 |
| 0.35 | 2.4119 | 2.4119 | 1.9401 | 24.3183 | 24.3183 |
| AHM | SAFEM-2D | SAFEM-3D | |||
| 0.05 | 1.0784 | 1.0784 | 1.0765 | 0.1765 | 0.1765 |
| 0.2 | 1.3555 | 1.3555 | 1.3688 | 0.9717 | 0.9717 |
| 0.35 | 1.7201 | 1.7201 | 1.7633 | 2.4500 | 2.4500 |
| AHM | SAFEM-2D | SAFEM-3D | |||
| 0.05 | 1.0625 | 1.0625 | 1.0624 | 0.0094 | 0.0094 |
| 0.2 | 1.2619 | 1.2619 | 1.2788 | 1.3216 | 1.3216 |
| 0.35 | 1.5041 | 1.5041 | 1.5611 | 3.6513 | 3.6513 |
| SAM | SCM | SAFEM-3D | |||
| 2.506 | 2.499 | 2.506 | 0.0000 | 0.2793 | |
| 8.097 | 8.108 | 8.108 | 0.1357 | 0.0000 | |
| 8.002 | 8.045 | 8.002 | 0.0000 | 0.5374 | |
| 2.402 | 2.402 | 2.402 | 0.0000 | 0.0000 | |
| 7.949 | 8.032 | 7.948 | 0.0126 | 1.0569 | |
| 8.459 | 8.440 | 8.458 | 0.0118 | 0.2128 | |
| SAM | SCM | SAFEM-3D | |||
| 2.565 | 2.563 | 2.566 | 0.0390 | 0.1169 | |
| 8.220 | 8.225 | 8.220 | 0.0000 | 0.0608 | |
| 8.199 | 8.209 | 8.209 | 0.1218 | 0.0000 | |
| 2.521 | 2.517 | 2.521 | 0.0000 | 0.1587 | |
| 8.524 | 8.534 | 8.525 | 0.0117 | 0.1056 | |
| 8.703 | 8.703 | 8.707 | 0.0459 | 0.0459 | |
| SAM | SCM | SAFEM-3D | |||
| 2.569 | 2.568 | 2.570 | 0.0389 | 0.0778 | |
| 8.241 | 8.242 | 8.242 | 0.0121 | 0.0000 | |
| 8.224 | 8.243 | 8.224 | 0.0000 | 0.2310 | |
| 2.554 | 2.542 | 2.544 | 0.3931 | 0.0786 | |
| 8.621 | 8.632 | 8.626 | 0.0580 | 0.0696 | |
| 8.710 | 8.720 | 8.720 | 0.1147 | 0.0000 | |
| SAM | SCM | SAFEM-3D | |||
| 2.589 | 2.587 | 2.589 | 0.0000 | 0.0772 | |
| 8.279 | 8.276 | 8.279 | 0.0000 | 0.0362 | |
| 8.259 | 8.280 | 8.280 | 0.2536 | 0.0000 | |
| 2.586 | 2.575 | 2.577 | 0.3492 | 0.0776 | |
| 8.748 | 8.759 | 8.761 | 0.1484 | 0.0228 | |
| 8.781 | 8.795 | 8.799 | 0.2046 | 0.0455 | |
| SAM | SCM | SAFEM-3D | |||
| 4.492 | 4.519 | 4.484 | 0.1784 | 0.7806 | |
| 9.782 | 9.686 | 9.700 | 0.8454 | 0.1443 | |
| 9.654 | 9.713 | 9.650 | 0.0415 | 0.6528 | |
| 4.496 | 4.483 | 4.477 | 0.4244 | 0.1340 | |
| 1.748 | 1.764 | 1.750 | 0.1143 | 0.8000 | |
| 1.765 | 1.775 | 1.761 | 0.2271 | 0.7950 | |
| SAM | SCM | SAFEM-3D | |||
| 4.436 | 4.468 | 4.426 | 0.2259 | 0.9489 | |
| 9.780 | 9.658 | 9.678 | 1.0539 | 0.2067 | |
| 9.621 | 9.690 | 9.605 | 0.1666 | 0.8850 | |
| 4.438 | 4.424 | 4.417 | 0.4754 | 0.1585 | |
| 1.719 | 1.737 | 1.719 | 0.0000 | 1.0471 | |
| 1.741 | 1.751 | 1.734 | 0.4037 | 0.9804 | |
| SAM | SCM | SAFEM-3D | |||
| 4.360 | 4.396 | 4.346 | 0.3221 | 1.1505 | |
| 9.778 | 9.619 | 9.644 | 1.3895 | 0.2592 | |
| 9.572 | 9.658 | 9.536 | 0.3775 | 1.2794 | |
| 4.358 | 4.342 | 4.334 | 0.5538 | 0.1846 | |
| 1.678 | 1.700 | 1.675 | 0.1791 | 1.4925 | |
| 1.709 | 1.717 | 1.698 | 0.6478 | 1.1190 | |
| SAM | SCM | SAFEM-3D | |||
| 4.253 | 4.293 | 4.231 | 0.5200 | 1.4654 | |
| 9.775 | 9.561 | 9.593 | 1.8972 | 0.3336 | |
| 9.495 | 9.608 | 9.426 | 0.7320 | 1.9308 | |
| 4.243 | 4.224 | 4.213 | 0.7121 | 0.2611 | |
| 1.620 | 1.646 | 1.611 | 0.5587 | 2.1726 | |
| 1.665 | 1.668 | 1.646 | 1.1543 | 1.3366 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tapia, M.; Espinosa-Almeyda, Y.; Rodríguez-Ramos, R.; Otero, J.A. Computation of Effective Elastic Properties Using a Three-Dimensional Semi-Analytical Approach for Transversely Isotropic Nanocomposites. Appl. Sci. 2021, 11, 1867. https://doi.org/10.3390/app11041867
Tapia M, Espinosa-Almeyda Y, Rodríguez-Ramos R, Otero JA. Computation of Effective Elastic Properties Using a Three-Dimensional Semi-Analytical Approach for Transversely Isotropic Nanocomposites. Applied Sciences. 2021; 11(4):1867. https://doi.org/10.3390/app11041867
Chicago/Turabian StyleTapia, Monica, Y. Espinosa-Almeyda, R. Rodríguez-Ramos, and José A. Otero. 2021. "Computation of Effective Elastic Properties Using a Three-Dimensional Semi-Analytical Approach for Transversely Isotropic Nanocomposites" Applied Sciences 11, no. 4: 1867. https://doi.org/10.3390/app11041867
APA StyleTapia, M., Espinosa-Almeyda, Y., Rodríguez-Ramos, R., & Otero, J. A. (2021). Computation of Effective Elastic Properties Using a Three-Dimensional Semi-Analytical Approach for Transversely Isotropic Nanocomposites. Applied Sciences, 11(4), 1867. https://doi.org/10.3390/app11041867







