Fluid-Structure Interaction Analysis of Aircraft Hydraulic Pipe with Complex Constraints Based on Discrete Time Transfer Matrix Method
Abstract
:1. Introduction
2. Theoretical Modeling
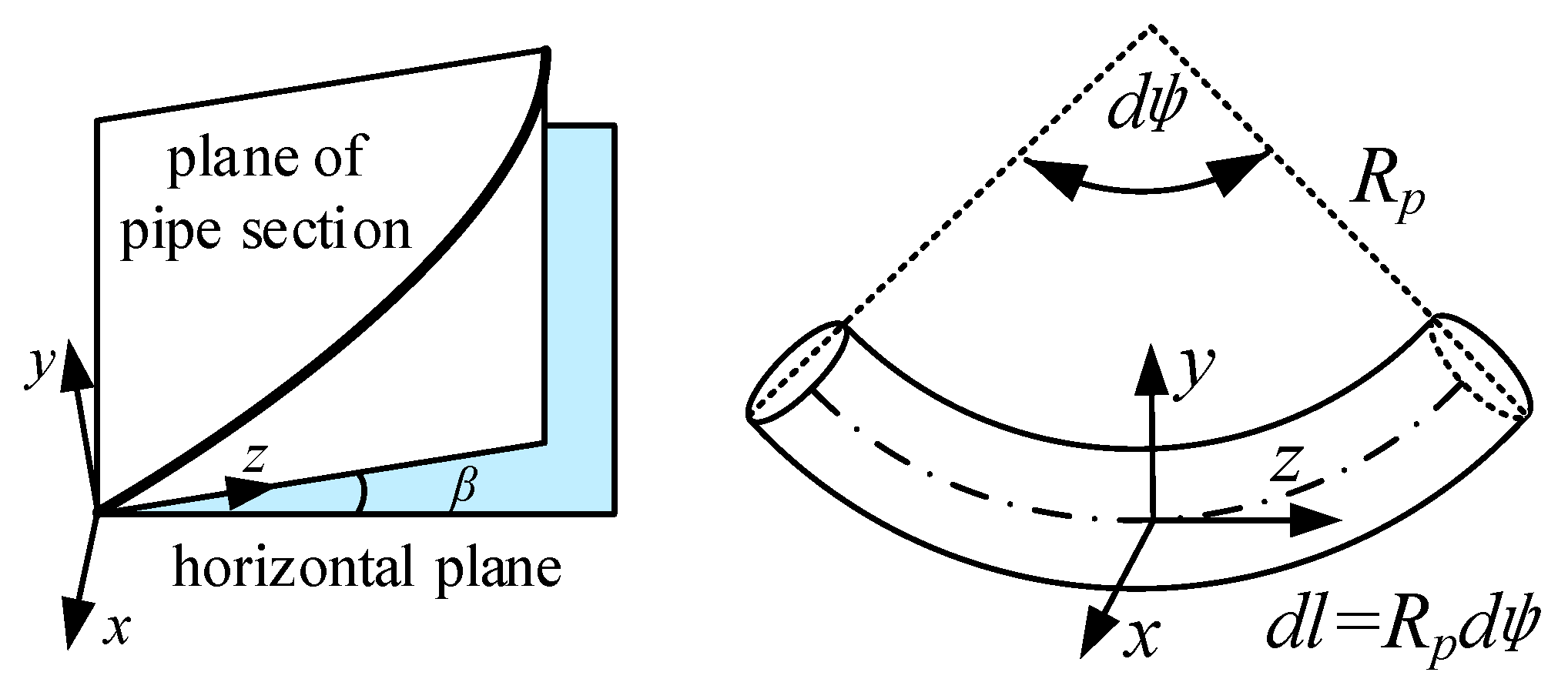
2.1. FSI Fourteen-Equation Model
2.2. Excitation Matrix Model
2.3. Boundary Matrix Model
2.4. Middle Constraint Matrix Model
3. Discrete Time Transfer Matrix Method
4. Analysis Example and Methods
4.1. Aircraft Hydraulic Pipe Model
4.2. Numerical Solution and Experimental Methods
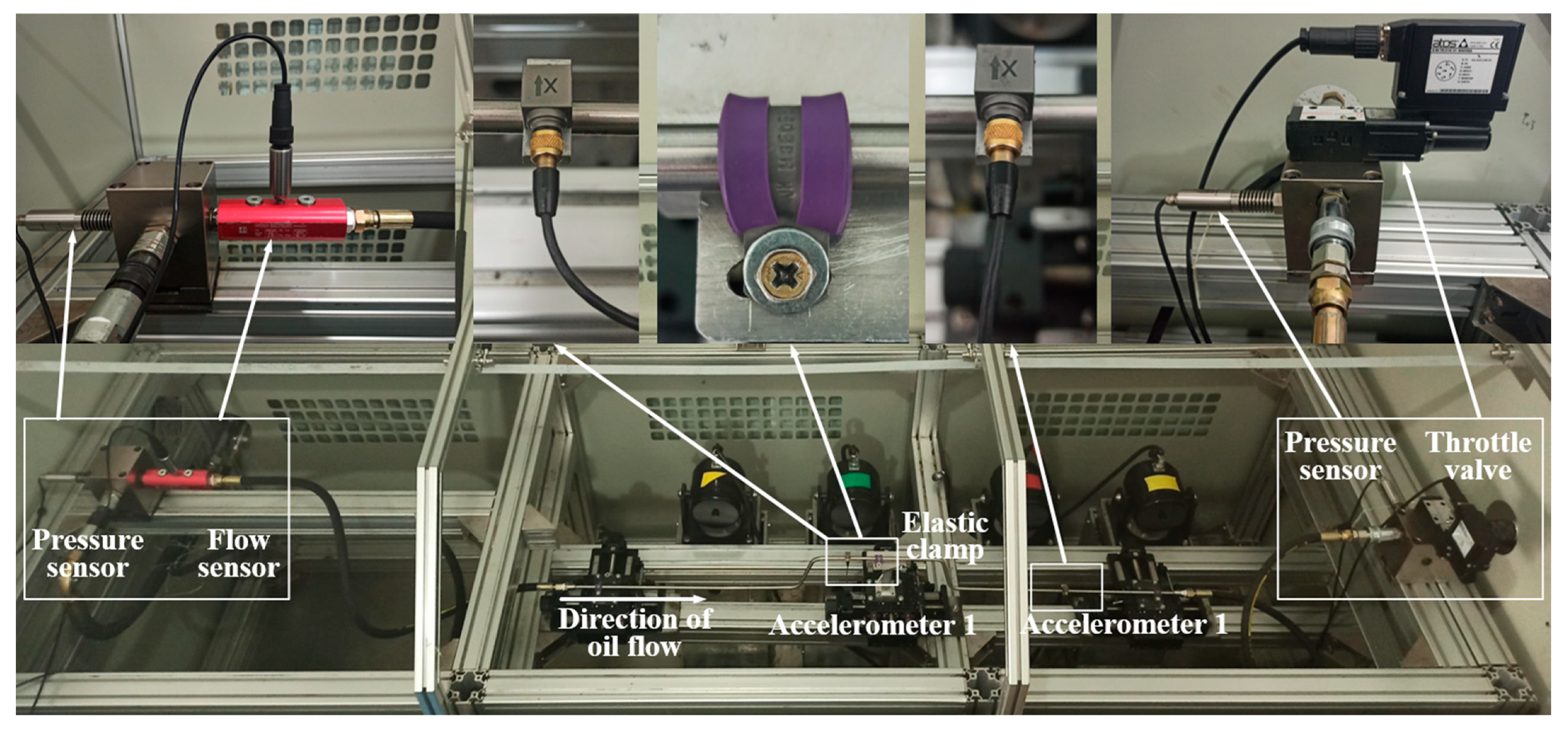
4.2.1. Experimental System
4.2.2. System Work Conditions
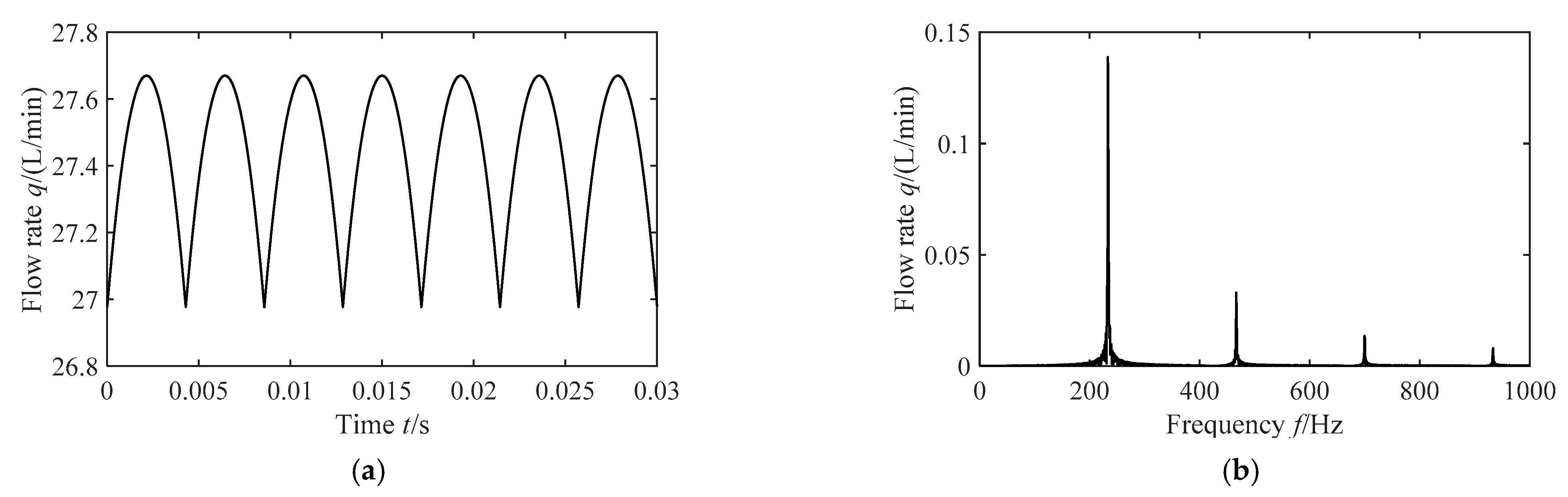
4.2.3. Flow Pulsation Excitation
4.2.4. Boundary Conditions
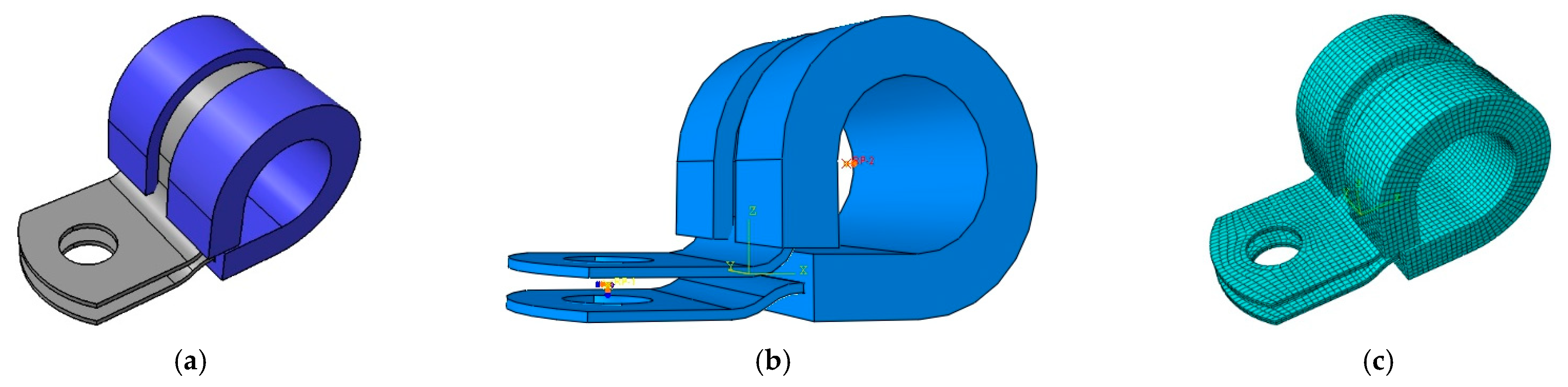
4.2.5. Middle Constraint
5. Results and Discussion
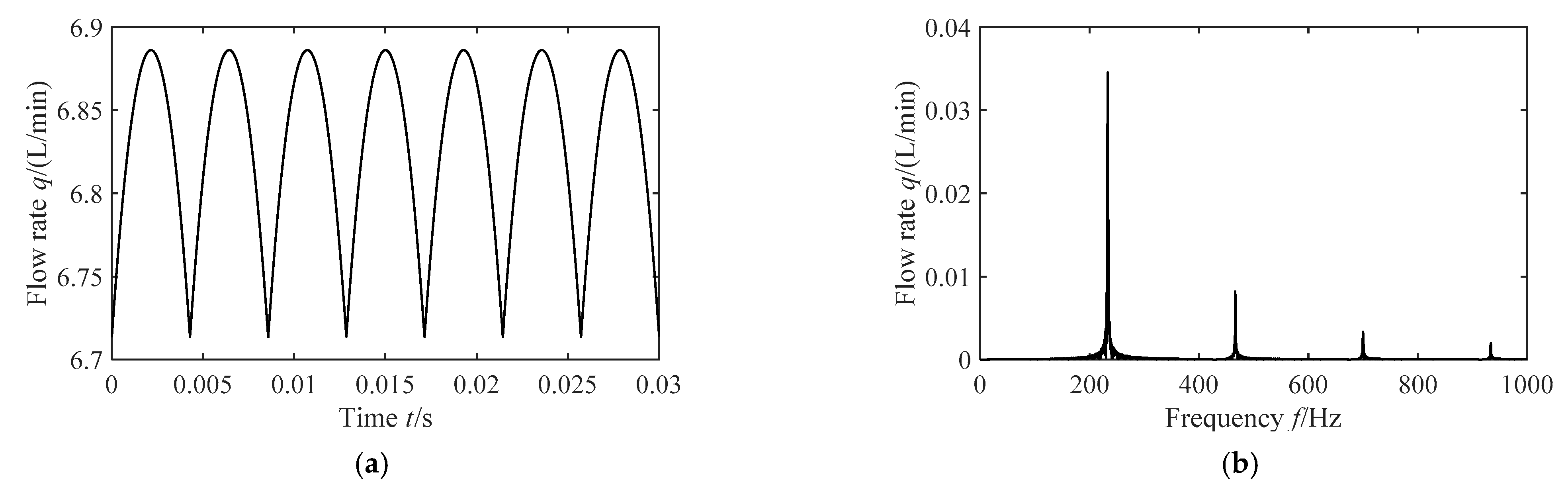
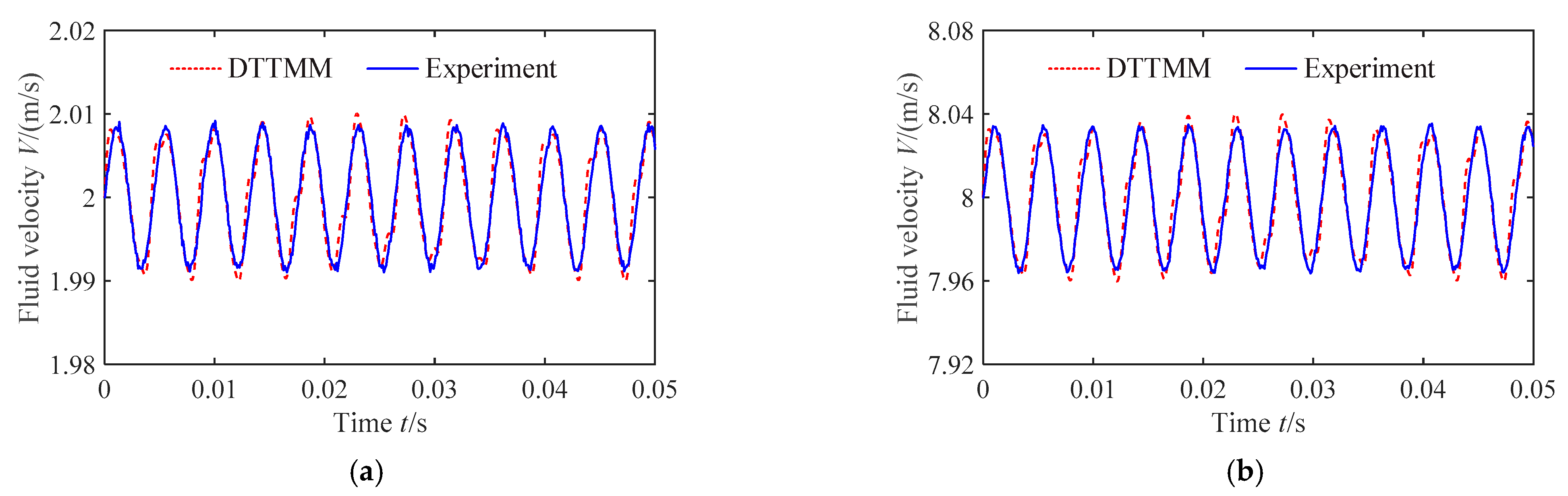
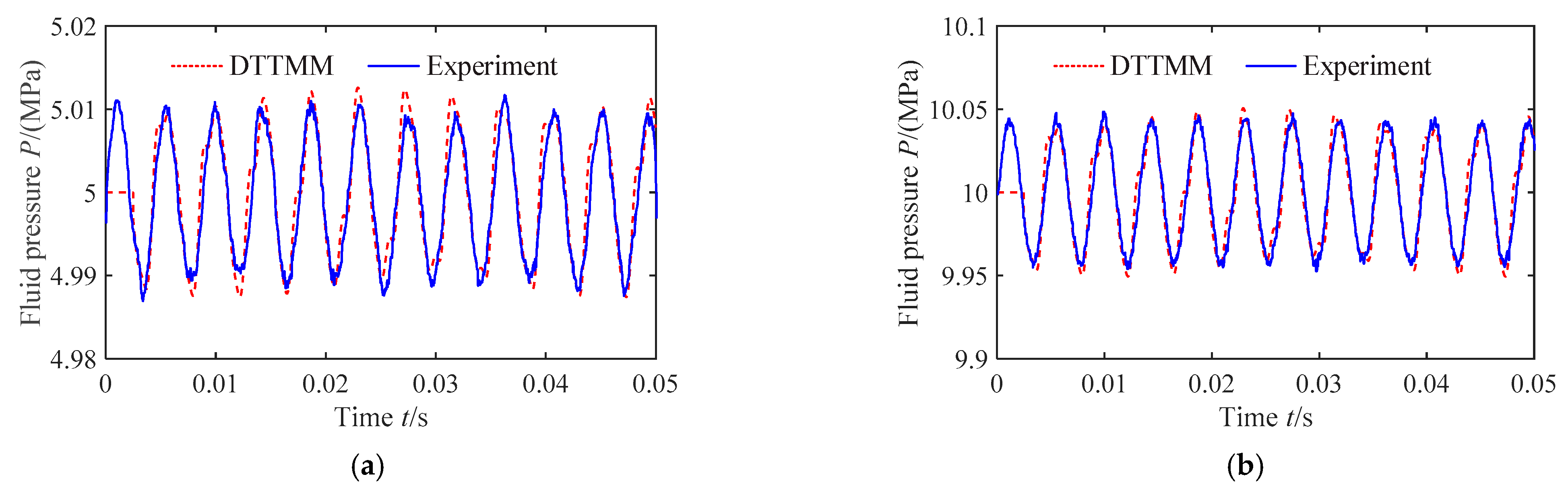
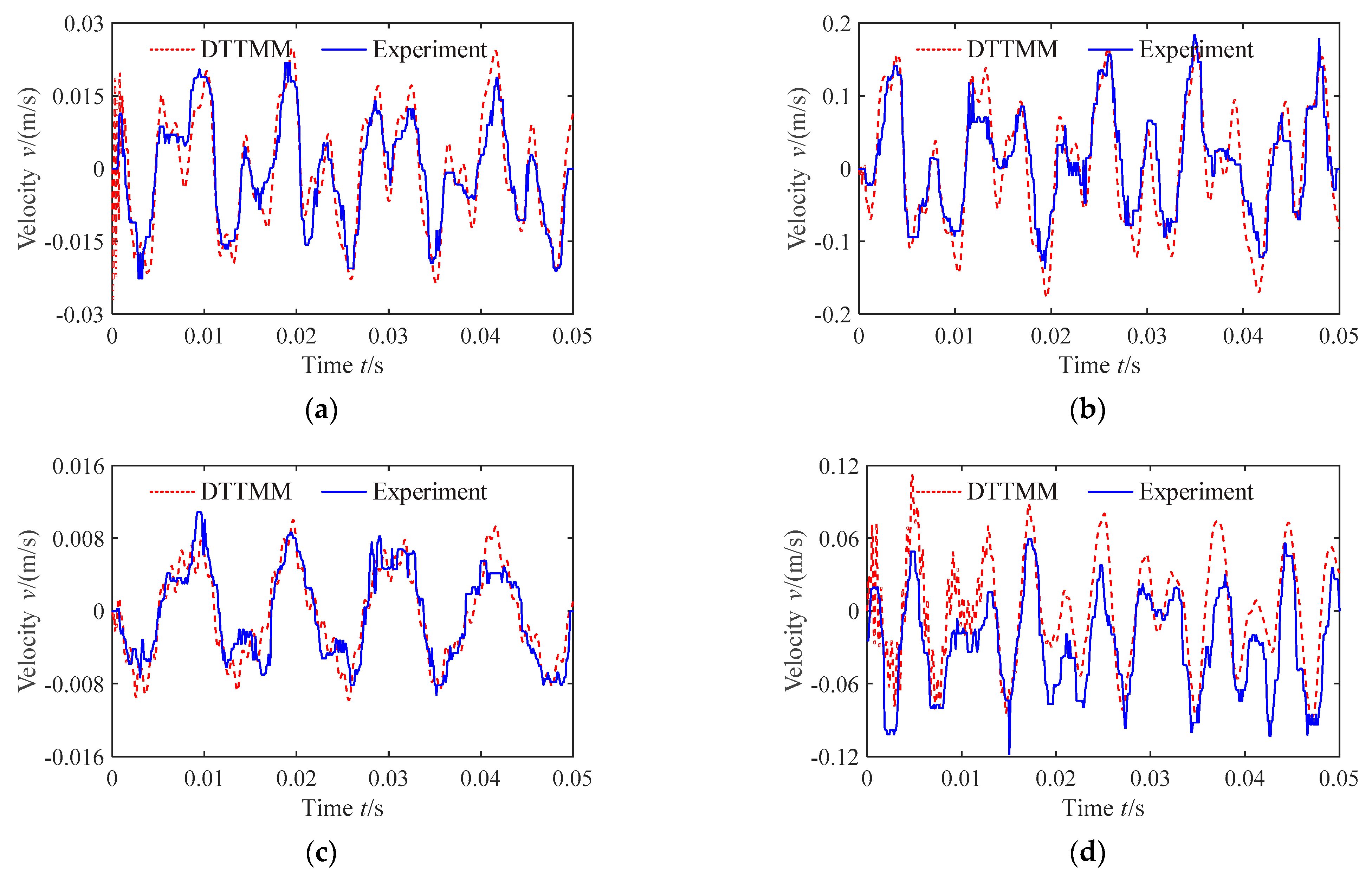
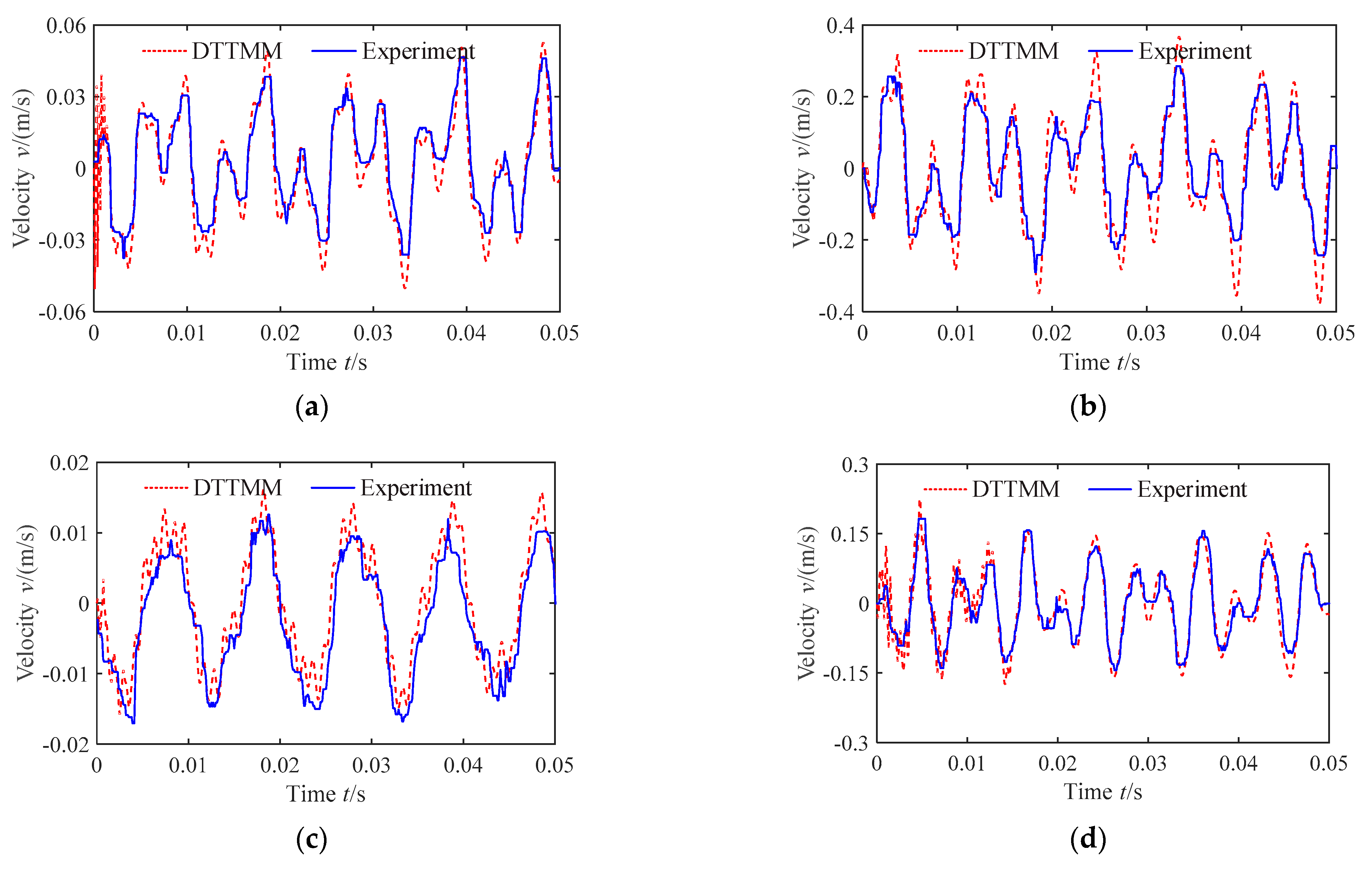
5.1. Dynamic Response Characteristics of Fluid
5.2. Dynamic Response Characteristics of Pipes
- Calculation error of theoretical results from analytical approximations and numerical error, etc.
- There are manufacturing error and installation deviation in the manufacturing and installation process of the test pipe.
- The textile-reinforced rubber hoses and elastic clamps exist in the experimental system, which will increase the damping characteristics of the system, but the damping dissipation of the above components is neglected in numerical calculation.
- There are measurement noises in the process of data acquisition, such as sensor noise, supply line noise and signal line noise, etc.
6. Conclusions
- The FSI fourteen-equation theoretical model of pipe conveying fluid is developed. The modified friction coupling model is contained in the axial motion equations, and the impacts of gravity, centrifugal force, Coriolis force and the moment of inertia caused by the fluid within the pipe, takes into account in the method that is applicable for describing the FSI of pipe conveying fluid in wide pressure and Reynolds number range.
- The external excitation model, boundary condition model and middle constraint are developed for solving the FSI fourteen-equation model. These models contain the flow pulsation excitation of axial piston pump, the velocity-inlet boundary condition, pressure-outlet boundary condition and the middle constraint (elastic clamp), which can be applied to solve the complex hydraulic pipeline system with various fluid and structural excitation, when complex constraints are contained.
- A discrete time transfer matrix method (DTTMM) for solving the FSI fourteen-equation model in time domain is presented. The excellent feature of the present method is that the whole solution procedure can be independently described by a unified matrix expression. It means that there is not any modification to the solution procedures from one analysis model to another, which makes a stylization solution method and further comprehensive investigation easier compared to most existing solution methods.
- The numerical solution and experiment of an ARJ21-700 aircraft hydraulic pipe with complex constraints are carried out under four working conditions to prove the theoretical model and solution method presented in this work. The results calculated by the DTTMM method are in good agreement with the experimental data, and the research shows that the pulsating amplitude of fluid increases with the increasing flow velocity and fluid pressure. As for the pipe, the vibration response under the flow pulsation excitation shows a forced vibration with periodic characteristics, and the flow pulsation excitation will cause large amplitude radial vibration of pipe. Moreover, the vibration amplitude increases with the increasing flow velocity, and the higher the flow velocity is, the earlier the vibration velocity reaches the response peak, but the fluid pressure has relatively less influence on the vibration response.
7. Patents
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Nomenclature of Parameters in Theoretical Modeling
| Parameter | Definition | Unit | Parameter | Definition | Unit |
|---|---|---|---|---|---|
| R | Inner radius of pipe | m | l | Length of pipe | m |
| R0 | Outer radius of pipe | m | Deflection angle of pipe | rad | |
| D | Pipe diameter | m | ρ | Density | kg/m3 |
| A | Cross-sectional area | m2 | rp | Centrifugal radius of fluid | m |
| M | Moment | Nm | Angle between pipe and Horizontal plane | rad | |
| G | Shear modulus | Pa | Angular velocity of pipe wall | rad/s | |
| V | Fluid velocity | m/s | Pipe velocity | m/s | |
| P | Fluid pressure | MPa | Bending angle of pipe | rad | |
| K | Fluid bulk modulus | MPa | f | Forces in cross-section | N |
| Corrected fluid bulk modulus | MPa | Shear stress of pipe wall | Pa | ||
| I | Flexure moment of inertia | m4 | σ | Stress | N/m2 |
| J | Polar moment of inertia | m4 | k | Shear coefficient | - |
| T | External moment of constraints | m4 | Poisson’s ratio | - | |
| e | Thickness of pipe wall | m | Strain | - | |
| m | Mass | g | x,y,z | Directional subscripts | - |
| E | Modulus of elasticity | MPa | f,p | Structural subscripts | - |
Appendix B. The Boundary Matrices of Closed Ends and Complex Constraints
Appendix C. The Specification of Experimental Apparatus and Measurement System

| System | Item | Manufacturer/Type | Performance |
|---|---|---|---|
| Experimental apparatus | Axial piston pump | Rexroth A4VSO40DR10RPPB13N00N | Displacement: 92 L/min Pressure rating: 35 MPa |
| Throttle valve | ATOS E-RI-TE-01H-41 | Pressure range: 0~40 MPa | |
| Accelerometer | B&K BK4525-B-001 | Measuring range: ±700 m/s2 Frequency range: 0–20 kHz | |
| Flow sensor | HYDAC EVS3104-A-0060-000 | Measuring range: 6–60 L/min Output signal: 4–20 mA Measuring error: ≤2% | |
| Pressure sensor | Shanghai Dingwei Electronic Materials Co., Ltd. FST800-216G335C-400B | Measuring range: 0~40 MPa Output signal: 0–10 V Measuring error ≤: 0.5% | |
| Measurement and control system | PXIe chassis | National Instruments PXIe-1078 | 9 AC hybrid slots System slot bandwidth: 250 MB/s System bandwidth: 1 GB/s |
| PXIe controller | National Instruments PXIe-8820 | Dual-core processor (2.2 GHz) System slot bandwidth: 250 MB/s System bandwidth: 1 GB/s | |
| Analog output card | National Instruments PXI-6723 | 32 analog output channels; Conversion rate: 10 kHz; Maximum sampling rate: 800 kS/s | |
| Data acquisition card | National Instruments PXI-6221 | 16 AI channels 2 AO channels Maximum sampling rate: 250 kS/s | |
| Vibration acquisition card | National Instruments PXIe-4497 | 24 channels 24 resolution Maximum sampling rate: 204.8 kS/s |
References
- Gao, P.; Yu, T.; Zhang, Y.; Wang, J.; Zhai, J. Vibration analysis and control technologies of hydraulic pipeline system in aircraft: A review. Chin. J. Aeronaut. 2021, 34, 83–114. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, X.; Rong, W.; Gao, P.; Yu, T.; Han, H.; Xu, L. Vibration and Damping Analysis of Pipeline System Based on Partially Piezoelectric Active Constrained Layer Damping Treatment. Materials 2021, 14, 1209. [Google Scholar] [CrossRef]
- Song, X.; Cao, T.; Gao, P.; Han, Q. Vibration and damping analysis of cylindrical shell treated with viscoelastic damping materials under elastic boundary conditions via a unified Rayleigh-Ritz method. Int. J. Mech. Sci. 2020, 165, 105158. [Google Scholar] [CrossRef]
- Gao, P.; Li, J.; Zhai, J.; Tao, Y.; Han, Q. A Novel Optimization Layout Method for Clamps in a Pipeline System. Appl. Sci. 2020, 10, 390. [Google Scholar] [CrossRef] [Green Version]
- Zhai, J.; Li, J.; Wei, D.; Gao, P.; Yan, Y.; Han, Q. Vibration Control of an Aero Pipeline System with Active Constraint Layer Damping Treatment. Appl. Sci. 2019, 9, 2094. [Google Scholar] [CrossRef] [Green Version]
- Yan, Y.; Zhai, J.; Gao, P.; Han, Q. A multi-scale finite element contact model for seal and assembly of twin ferrule pipeline fittings. Tribol. Int. 2018, 125, 100–109. [Google Scholar] [CrossRef]
- Wang, M.; Jiao, Z.; Xu, Y. Analytical method of thermal-fluid transients in aerohydraulic systems. In Proceedings of the 2015 International Conference on Fluid Power and Mechatronics, Haerbin, China, 14–15 September 2015; pp. 63–67. [Google Scholar]
- Li, Y.; Jiao, Z.; Xu, Y. Nonlinear Analysis of Oscillations in Aero-hydraulic Actuation System Considering Load Effect. In Proceedings of the 2015 International Conference on Fluid Power and Mechatronics 2015, Haerbin, China, 14–15 September 2015; pp. 934–938. [Google Scholar]
- Quan, L.; Che, S.; Guo, C.; Gao, H.; Guo, M. Axial Vibration Characteristics of Fluid-Structure Interaction of an Aircraft Hydraulic Pipe Based on Modified Friction Coupling Model. Appl. Sci. 2020, 10, 3548. [Google Scholar] [CrossRef]
- Zhang, Q.; Kong, X.; Huang, Z.; Yu, B.; Meng, G. Fluid-Structure-Interaction Analysis of an Aero Hydraulic Pipe Considering Friction Coupling. IEEE Access 2019, 7, 26665–26677. [Google Scholar] [CrossRef]
- Joukowsky, N. On the hydraulic hammer in water supply pipes. Proc. Am. Water Works Assoc. 1904, 24, 341–424. [Google Scholar]
- Skalak, R. An extension of the theory of water hammer. Trans. ASME 1956, 78, 105–116. [Google Scholar]
- Wiggert, D.C.; Otwell, R.S.; Hatfield, F.J. The Effect of Elbow Restraint on Pressure Transients. J. Fluid. Eng. 1985, 107, 402–406. [Google Scholar] [CrossRef]
- You, J.H.; Inaba, K. Fluid-structure interaction in water-filled thin pipes of anisotropic composite materials. J. Fluid. Struct. 2013, 36, 162–173. [Google Scholar] [CrossRef]
- Walker, J.S.; Phillips, J.W. Pulse propagation in fluid-filled tubes. J. Press. Vess. Technol. ASME 1977, 77, 31–35. [Google Scholar] [CrossRef]
- Davidson, L.C. Liquid-Structure Coupling in Curved Pipes. Shock Vib. Bull. 1969, 40, 197–207. [Google Scholar]
- Wiggert, D.C.; Tijsseling, A.S. Fluid transients and fluid-structure interaction in flexible liquid-filled piping. Appl. Mech. Rev. 2001, 54, 455–481. [Google Scholar] [CrossRef]
- Liu, G.; Li, S.; Li, Y.; Chen, H. Vibration analysis of pipelines with arbitrary branches by absorbing transfer matrix method. J. Sound Vib. 2013, 332, 6519–6536. [Google Scholar] [CrossRef]
- Xu, Y.; Johnston, D.N.; Jiao, Z.; Plummer, A.R. Frequency modelling and solution of fluid-structure interaction in complex pipelines. J. Sound Vib. 2014, 333, 2800–2822. [Google Scholar] [CrossRef] [Green Version]
- Lesmez, M.W.; Wiggert, D.C.; Hatfield, F.J. Modal Analysis of Vibrations in Liquid-Filled Piping Systems. J. Fluid. Eng. 1990, 112, 311–318. [Google Scholar] [CrossRef]
- Zhang, L.; Tijsseling, S.A.; Vardy, E.A. Fsi Analysis of Liquid-Filled Pipes. J. Sound Vib. 1999, 224, 69–99. [Google Scholar] [CrossRef] [Green Version]
- Hatfield, F.J.; Wiggert, D.C.; Otwell, R.S. Fluid Structure Interaction in Piping by Component Synthesis. J. Fluid. Eng. 1982, 104, 318–325. [Google Scholar] [CrossRef]
- Zhang, X.M. Parametric studies of coupled vibration of cylindrical pipes conveying fluid with the wave propagation approach. Comput. Struct. 2002, 80, 287–295. [Google Scholar] [CrossRef]
- Burmann, W. Water hammer in coaxial pipe system. ASCE J. Hydraul. Div. 1975, 101, 699–715. [Google Scholar] [CrossRef]
- Hu, C.K.; Phillips, J.W. Pulse Propagation in Fluid-Filled Elastic Curved Tubes. J. Press. Vess.-Technol. ASME 1981, 103, 43–49. [Google Scholar] [CrossRef]
- Ellis, J. A study of pipe-liquid interaction following pump trip and check-valve closure in a pumping station. In Proceedings of the 3rd International Conference on Pressure Surges, Canterbury, UK, 25–27 March 1980; pp. 203–220. [Google Scholar]
- Ruoff, J.; Hodapp, M.; Kück, H. Finite element modelling of Coriolis mass flowmeters with arbitrary pipe geometry and unsteady flow conditions. Flow Meas. Instrum. 2014, 37, 119–126. [Google Scholar] [CrossRef]
- Sreejith, B.; Jayaraj, K.; Ganesan, N.; Padmanabhan, C.; Chellapandi, P.; Selvaraj, P. Finite element analysis of fluid-structure interaction in pipeline systems. Nucl. Eng. Des. 2004, 227, 313–322. [Google Scholar] [CrossRef]
- Zhang, Y.L.; Gorman, D.G.; Reese, J.M. A finite element method for modelling the vibration of initially tensioned thin-walled orthotropic cylindrical tubes conveying fluid. J. Sound Vib. 2001, 245, 93–112. [Google Scholar] [CrossRef]
- Achouyab, E.H.; Bahrar, B. Numerical modeling of phenomena of waterhammer using a model of fluid-structure interaction. Comptes Rendus Mec. 2011, 339, 262–269. [Google Scholar] [CrossRef]
- Ahmadi, A.; Keramat, A. Investigation of fluid-structure interaction with various types of junction coupling. J. Fluid. Struct. 2010, 26, 1123–1141. [Google Scholar] [CrossRef]
- Wiggert, D.C.; Hatfield, F.J.; Stuckenbruck, S. Analysis of Liquid and Structural Transients in Piping by the Method of Characteristics. J. Fluid. Eng. 1987, 109, 161–165. [Google Scholar] [CrossRef]
- Tijsseling, A.S. Fluid-Structure Interaction in Case of Waterhammer with Cavitation. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1993. [Google Scholar]
- Ouyang, X.; Gao, F.; Yang, H.; Wang, H. Two-dimensional stress analysis of the aircraft hydraulic system pipeline. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2011, 226, 532–539. [Google Scholar] [CrossRef]
- Tentarelli, S.C. Propagation of Noise and Vibration in Complex Hydraulic Tubing Systems. Ph.D. Thesis, Lehigh University, Bethlehem, PA, USA, 1990. [Google Scholar]
- Gale, J.; Tiselj, I. Eight equation model for arbitrary shaped pipe conveying fluid. In Proceedings of the International Conference Nuclear Energy for New Europe, Portoroz, Slovenia, 18–21 September 2006. [Google Scholar]
- Guan, C.; Jiao, Z.; He, S. Theoretical study of flow ripple for an aviation axial-piston pump with damping holes in the valve plate. Chin. J. Aeronaut. 2014, 27, 169–181. [Google Scholar] [CrossRef] [Green Version]
- Bahr, M.K.; Svoboda, J.; Bhat, R.B. Vibration analysis of constant power regulated swash plate axial piston pumps. J. Sound Vib. 2003, 259, 1225–1236. [Google Scholar] [CrossRef]
- Tijsseling, A.S. Fluid-Structure Interaction and Cavitation in a Single-Elbow Pipe System. J. Fluid. Struct. 1996, 10, 395–420. [Google Scholar] [CrossRef]
- Budny, D.D.; Wiggert, D.C.; Hatfield, F.J. The Influence of Structural Damping on Internal Pressure during a Transient Pipe Flow. J. Fluid. Eng. 1991, 113, 424–429. [Google Scholar] [CrossRef]
- Kwong, A.; Edge, K.A. A method to reduce noise in hydraulic systems by optimizing pipe clamp locations. Proc. Inst. Mech. Eng. J. Syst. Control Eng. 1998, 212, 267–280. [Google Scholar] [CrossRef]
- Wu, J.-S.; Shih, P.Y. The dynamic analysis of a multispan fluid-conveying pipe subjected to external load. J. Sound Vib. 2001, 239, 201–215. [Google Scholar] [CrossRef]
- Ke, Y.; Li, Q.S.; Zhang, L. Longitudinal vibration analysis of multi-span liquid-filled pipelines with rigid constraints. J. Sound Vib. 2004, 273, 125–147. [Google Scholar]
- Xu, Y.; Jiao, Z. Exact solution of axial liquid-pipe vibration with time-line interpolation. J. Fluid. Struct. 2017, 70, 500–518. [Google Scholar] [CrossRef]
- Gao, P.X.; Zhai, J.Y.; Han, Q.K. Dynamic response analysis of aero hydraulic pipeline system under pump fluid pressure fluctuation. Proc. Inst. Mech. Eng. 2019, 233, 1585–1595. [Google Scholar] [CrossRef]
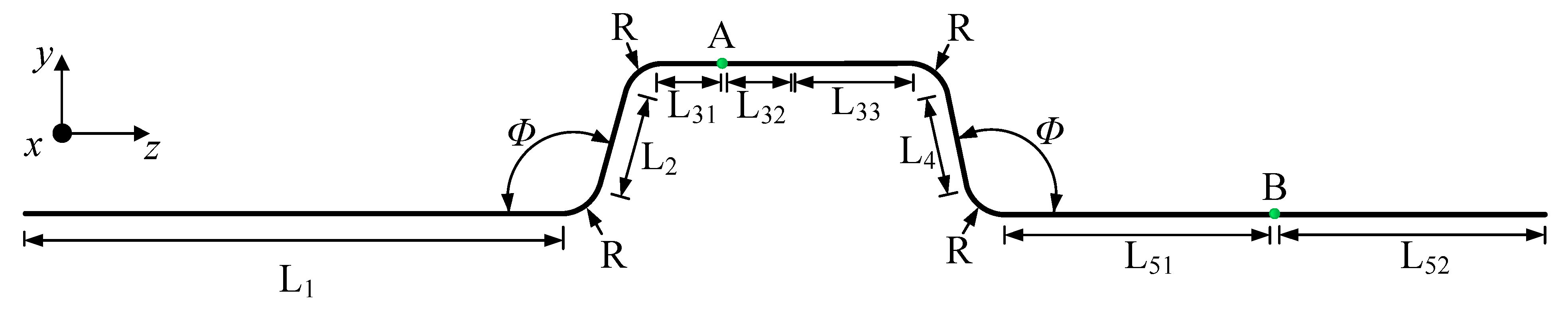




| Quantity | Symbol | Value | Unit | Quantity | Symbol | Value | Unit |
|---|---|---|---|---|---|---|---|
| pipe length | L1 | 534.261 | mm | Bending angle | 1.649 | rad | |
| L2 | 72.996 | mm | Pipe density | 7760 | kg/m3 | ||
| L31, L32 | 61.630 | mm | Young’s modulus | E | 190 | GPa | |
| L33 | 123.260 | mm | Poisson’s ratio | 0.27 | - | ||
| L4 | 72.996 | mm | Oil density | 872 | kg/m3 | ||
| L51, L52 | 267.131 | mm | Bulk modulus | Kf | 1.95 | GPa | |
| outer diameter | D | 9.525 | mm | Kinematic viscosity | v | 19.7 | mm2/s |
| pipe wall thickness | e | 0.889 | mm | Transducer mass | ms | 0.006 | kg |
| bending radius | R | 38.100 | mm | Oil brand | 10# aircraft hydraulic oil | ||
| Work Conditions | Fluid Pressure (MPa) | Flow Velocity (m/s) | Flow Rate (L/min) | Reynolds Number | Flow Form |
|---|---|---|---|---|---|
| 1 | 5 | 2 | 6.82 | 967.01 | Laminar |
| 2 | 5 | 8 | 27.3 | 3868.02 | Turbulence |
| 3 | 10 | 2 | 6.82 | 967.01 | Laminar |
| 4 | 10 | 8 | 27.3 | 3868.02 | Turbulence |
| Flow Velocity (m/s) | f1 (Hz) | q1 (L/min) | f2 (Hz) | q2 (L/min) | f3 (Hz) | q3 (L/min) | f4 (Hz) | q4 (L/min) |
|---|---|---|---|---|---|---|---|---|
| 2 | 227.8 | 0.03281 | 484.1 | 0.00594 | 711.9 | 0.00415 | 939.7 | 0.00270 |
| 8 | 227.8 | 0.13180 | 484.1 | 0.02390 | 711.9 | 0.01668 | 939.7 | 0.01083 |
| Flow Velocity (m/s) | VA1 (m/s) | VA1 (m/s) | VA1 (m/s) | VA1 (m/s) | ||||
|---|---|---|---|---|---|---|---|---|
| 2 | 1430.584 | 0.00851 | 3040.148 | 0.00154 | 4470.732 | 0.00108 | 5901.316 | 0.00070 |
| 8 | 1430.584 | 0.03418 | 3040.148 | 0.00620 | 4470.732 | 0.00433 | 5901.316 | 0.00281 |
| Work Conditions | Fluid Pressure (MPa) | Flow Velocity (m/s) | Flow Rate (L/min) | kq Pa/(m/s) |
|---|---|---|---|---|
| 1 | 5 | 2 | 6.82 | 2.5 × 106 |
| 2 | 5 | 8 | 27.3 | 6.25 × 105 |
| 3 | 10 | 2 | 6.82 | 5 × 106 |
| 4 | 10 | 8 | 27.3 | 1.25 × 106 |
| Material | Density (kg/m3) | Elastic Modulus (GPa) | Poisson’s Ratio | |
|---|---|---|---|---|
| Metal band | Aluminium alloy (2A12) | 2750 | 70 | 0.33 |
| Rubber washer | Rubber (EPDM8370) | C10 | C01 | D0 |
| 0.774 | 0.193 | 0.025 |
| Translational Stiffness (N/m) | Rocking Stiffness (N∙m/rad) | ||||
|---|---|---|---|---|---|
| kx | ky | kz | tx | ty | tz |
| 8.74 × 106 | 5.84 × 105 | 7.29 × 106 | 1830 | 239 | 1890 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, H.; Guo, C.; Quan, L. Fluid-Structure Interaction Analysis of Aircraft Hydraulic Pipe with Complex Constraints Based on Discrete Time Transfer Matrix Method. Appl. Sci. 2021, 11, 11918. https://doi.org/10.3390/app112411918
Gao H, Guo C, Quan L. Fluid-Structure Interaction Analysis of Aircraft Hydraulic Pipe with Complex Constraints Based on Discrete Time Transfer Matrix Method. Applied Sciences. 2021; 11(24):11918. https://doi.org/10.3390/app112411918
Chicago/Turabian StyleGao, Haihai, Changhong Guo, and Lingxiao Quan. 2021. "Fluid-Structure Interaction Analysis of Aircraft Hydraulic Pipe with Complex Constraints Based on Discrete Time Transfer Matrix Method" Applied Sciences 11, no. 24: 11918. https://doi.org/10.3390/app112411918
APA StyleGao, H., Guo, C., & Quan, L. (2021). Fluid-Structure Interaction Analysis of Aircraft Hydraulic Pipe with Complex Constraints Based on Discrete Time Transfer Matrix Method. Applied Sciences, 11(24), 11918. https://doi.org/10.3390/app112411918







