Biomechanical Effects on Lower Extremities in Human-Robot Collaborative Agricultural Tasks
Abstract
1. Introduction
2. Materials and Methods
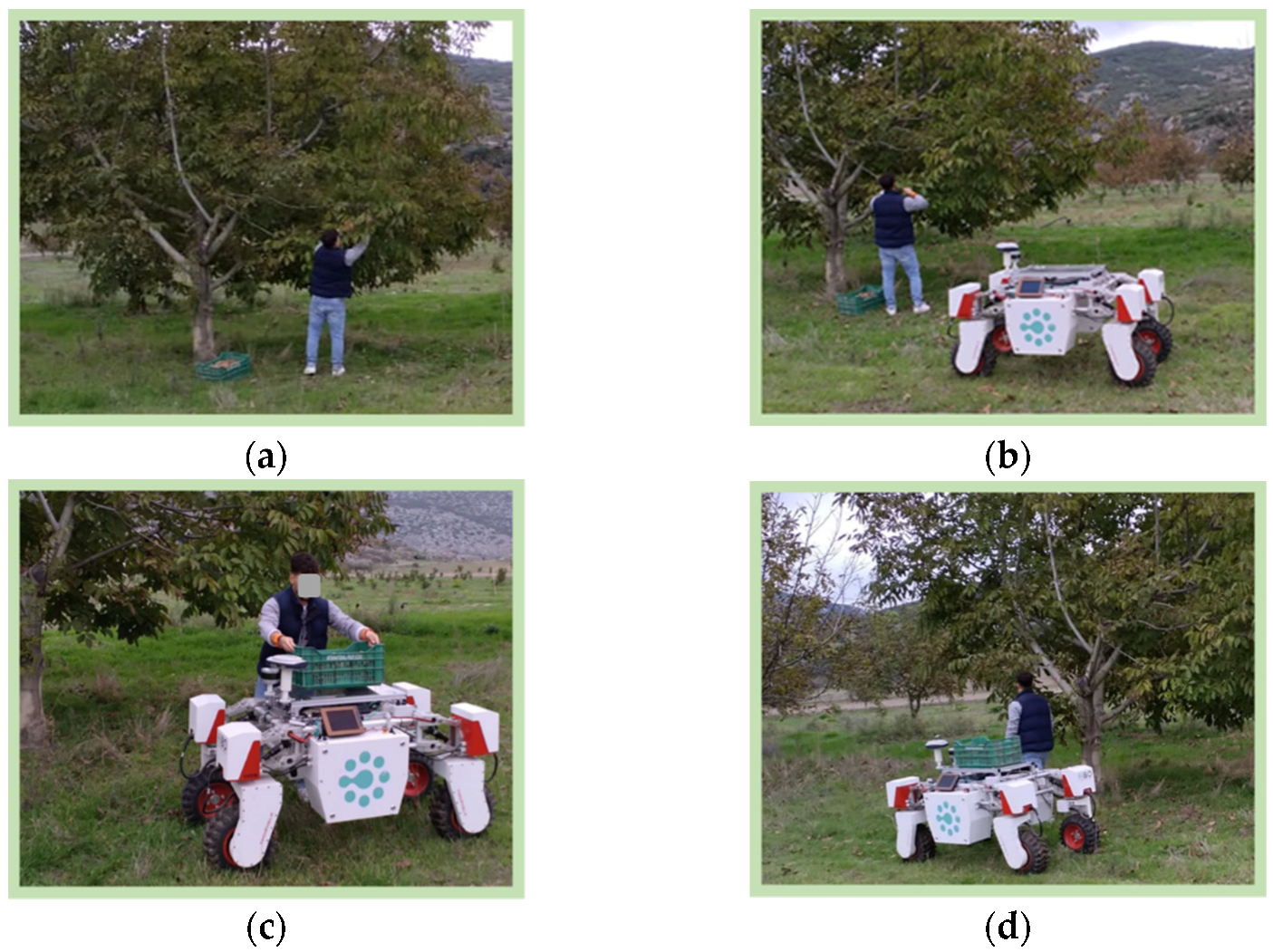
2.1. Experimentation Setup
2.1.1. Participants
- To be free from any MSD (e.g., low-back discomfort, knee or hip pain, ankle sprain) 6 months prior to participating in the study.
- To have had experience in agricultural material handling (for at least two years).
2.1.2. Data Collection
2.1.3. Experimental Protocol
2.2. Data Analyses
- The first phase was defined between the initiation of the trial and the completion of the forward bending motion to reach the agricultural crate.
- The second phase lasted from the latter time point until lifting-up the crate.
- The third phase was defined between the completion of the second phase and the landing of the crate on the custom-made shelf.
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Moysiadis, V.; Tsolakis, N.; Katikaridis, D.; Sørensen, C.G.; Pearson, S.; Bochtis, D. Mobile Robotics in Agricultural Operations: A Narrative Review on Planning Aspects. Appl. Sci. 2020, 10, 3453. [Google Scholar] [CrossRef]
- Perry, M.J. Agricultural Health and Safety. In International Encyclopedia of Public Health; Elsevier Inc.: Amsterdam, The Netherlands, 2016; pp. 38–44. ISBN 9780128037089. [Google Scholar]
- Holmberg, S.; Stiernström, E.-L.; Thelin, A.; Svärdsudd, K. Musculoskeletal symptoms among farmers and non-farmers: A population-based study. Int. J. Occup. Environ. Health 2002, 8, 339–345. [Google Scholar] [CrossRef] [PubMed]
- O’Connor, T.; Meredith, D.; McNamara, J.; O’Hora, D.; Kinsella, J. Farmer Discussion Groups Create Space for Peer Learning about Safety and Health. J. Agromed. 2020, 26, 120–131. [Google Scholar] [CrossRef]
- Kirkhorn, S.R.; Earle-Richardson, G.; Banks, R.J. Ergonomic risks and musculoskeletal disorders in production agriculture: Recommendations for effective research to practice. J. Agromed. 2010, 15, 281–299. [Google Scholar] [CrossRef] [PubMed]
- Kumaraveloo, K.S.; Lunner Kolstrup, C. Agriculture and musculoskeletal disorders in low- and middle-income countries. J. Agromed. 2018, 23, 227–248. [Google Scholar] [CrossRef]
- Bovenzi, M. Health effects of mechanical vibration. G. Ital. Med. Lav. Erg. 2005, 27, 58–64. [Google Scholar]
- Pelmear, P.L.; Leong, D. Review of occupational standards and guidelines for hand-arm (Segmental) vibration syndrome (havs). Appl. Occup. Environ. Hyg. 2000, 15, 291–302. [Google Scholar] [CrossRef]
- Benos, L.; Tsaopoulos, D.; Bochtis, D. A Review on Ergonomics in Agriculture. Part II: Mechanized Operations. Appl. Sci. 2020, 10, 3484. [Google Scholar] [CrossRef]
- Fathallah, F.A. Musculoskeletal disorders in labor-intensive agriculture. Appl. Ergon. 2010, 41, 738–743. [Google Scholar] [CrossRef]
- Benos, L.; Tsaopoulos, D.; Bochtis, D. A Review on Ergonomics in Agriculture. Part I: Manual Operations. Appl. Sci. 2020, 10, 1905. [Google Scholar] [CrossRef]
- Khan, M.I.; Bath, B.; Boden, C.; Adebayo, O.; Trask, C. The association between awkward working posture and low back disorders in farmers: A systematic review. J. Agromed. 2019, 24, 74–89. [Google Scholar] [CrossRef] [PubMed]
- Burdorf, A.; Sorock, G. Positive and negative evidence of risk factors for back disorders. Scand. J. Work. Environ. Health 1997, 23, 243–256. [Google Scholar] [CrossRef] [PubMed]
- Hoozemans, M.J.M.; Kingma, I.; de Vries, W.H.K.; van Dieën, J.H. Effect of lifting height and load mass on low back loading. Ergonomics 2008, 51, 1053–1063. [Google Scholar] [CrossRef]
- Sorensen, C.J.; Haddad, O.; Campbell, S.; Mirka, G.A. The effect of stance width on trunk kinematics and trunk kinetics during sagitally symmetric lifting. Int. J. Ind. Ergon. 2011, 41, 147–152. [Google Scholar] [CrossRef]
- Lavender, S.A.; Li, Y.C.; Andersson, G.B.; Natarajan, R.N. The effects of lifting speed on the peak external forward bending, lateral bending, and twisting spine moments. Ergonomics 1999, 42, 111–125. [Google Scholar] [CrossRef]
- Waters, T.R.; Putz-Anderson, V.; Garg, A.; Fine, L.J. Revised NIOSH equation for the design and evaluation of manual lifting tasks. Ergonomics 1993, 36, 749–776. [Google Scholar] [CrossRef]
- Middlesworth, M. A Step-by-Step Guide to Using the NIOSH Lifting Equation for Single Tasks. Available online: https://ergo-plus.com/niosh-lifting-equation-single-task/ (accessed on 20 October 2021).
- Plamondon, A.; Denis, D.; Delisle, A.; Lariviere, C.; Salazar, E. Biomechanical differences between expert and novice workers in a manual material handling task. Ergonomics 2010, 53, 1239–1253. [Google Scholar] [CrossRef]
- Fathallah, F.A.; Miller, B.J.; Miles, J.A. Low back disorders in agriculture and the role of stooped work: Scope, potential interventions, and research needs. J. Agric. Saf. Health 2008, 14, 221–245. [Google Scholar] [CrossRef]
- Del Vecchio, L. Choosing a Lifting Posture: Squat, Semi-Squat or Stoop. MOJ Yoga Phys. Ther. 2017, 2. [Google Scholar] [CrossRef]
- Harari, Y.; Bechar, A.; Riemer, R. Workers’ biomechanical loads and kinematics during multiple-task manual material handling. Appl. Ergon. 2020, 83, 102985. [Google Scholar] [CrossRef]
- Harari, Y.; Riemer, R.; Bechar, A. Shoulder moments and angles during single and combined manual material handling tasks. Ergonomics 2021, 64, 613–624. [Google Scholar] [CrossRef] [PubMed]
- Harari, Y.; Riemer, R.; Bechar, A. Differences in spinal moments, kinematics and pace during single-task and combined manual material handling jobs. Appl. Ergon. 2019, 81, 102871. [Google Scholar] [CrossRef]
- Kirkhorn, S.; Greenlee, R.T.; Reeser, J.C. The Epidemiology of Agriculture-related Osteoarthritis and its Impact on Occupational Disability. Wis. Med. J. 2003, 102, 38–44. [Google Scholar]
- Croft, P.; Coggon, D.; Cruddas, M.; Cooper, C. Osteoarthritis of the hip: An occupational disease in farmers. BMJ 1992, 304, 1269–1272. [Google Scholar] [CrossRef] [PubMed]
- Harris, E.C.; Coggon, D. HIP osteoarthritis and work. Best Pract. Res. Clin. Rheumatol. 2015, 29, 462–482. [Google Scholar] [CrossRef]
- Sahlström, A.; Lanshammar, H.; Adalberth, G. Knee joint moments in work-related situations. Ergonomics 1995, 38, 1352–1359. [Google Scholar] [CrossRef]
- Bevan, S. Economic impact of musculoskeletal disorders (MSDs) on work in Europe. Best Pract. Res. Clin. Rheumatol. 2015, 29, 356–373. [Google Scholar] [CrossRef]
- Le Pen, C.; Reygrobellet, C.; Gérentes, I. Financial cost of osteoarthritis in France: The “COART” France study. Jt. Bone Spine 2005, 72, 567–570. [Google Scholar] [CrossRef]
- Puig-Junoy, J.; Zamora, A.R. Socio-economic costs of osteoarthritis: A systematic review of cost-of-illness studies. Semin. Arthritis Rheum. 2015, 44, 531–541. [Google Scholar] [CrossRef]
- Nikolic, G.; Nedeljkovic, B.; Trajkovic, G.; Rasic, D.; Mirkovic, Z.; Pajovic, S.; Grbic, R.; Sipetic, S.; Vujcic, I. Pain, Physical Function, Radiographic Features, and Quality of Life in Knee Osteoarthritis Agricultural Workers Living in Rural Population. Pain Res. Manag. 2019, 2019, 7684762. [Google Scholar] [CrossRef]
- Bochtis, D.; Benos, L.; Lampridi, M.; Marinoudi, V.; Pearson, S.; Sørensen, C.G. Agricultural workforce crisis in light of the COVID-19 pandemic. Sustainability 2020, 12, 8212. [Google Scholar] [CrossRef]
- Kuorinka, I.; Jonsson, B.; Kilbom, A.; Vinterberg, H.; Biering-Sørensen, F.; Andersson, G.; Jørgensen, K. Standardised Nordic questionnaires for the analysis of musculoskeletal symptoms. Appl. Ergon. 1987, 18, 233–237. [Google Scholar] [CrossRef]
- Spielholz, P.; Silverstein, B.; Morgan, M.; Checkoway, H.; Kaufman, J. Comparison of self-report, video observation and direct measurement methods for upper extremity musculoskeletal disorder physical risk factors. Ergonomics 2001, 44, 588–613. [Google Scholar] [CrossRef] [PubMed]
- Perreault, N.; Brisson, C.; Dionne, C.E.; Montreuil, S.; Punnett, L. Agreement between a self-administered questionnaire on musculoskeletal disorders of the neck-shoulder region and a physical examination. BMC Musculoskelet. Disord. 2008, 9, 34. [Google Scholar] [CrossRef] [PubMed]
- Schall, M.C.; Fethke, N.B.; Chen, H.; Oyama, S.; Douphrate, D.I. Accuracy and repeatability of an inertial measurement unit system for field-based occupational studies. Ergonomics 2016, 59, 591–602. [Google Scholar] [CrossRef] [PubMed]
- Çakmak, B.; Ergül, E. Interactions of personal and occupational risk factors on hand grip strength of winter pruners. Int. J. Ind. Ergon. 2018, 67, 192–200. [Google Scholar] [CrossRef]
- Dewi, N.S.; Komatsuzaki, M. On-body personal assist suit for commercial farming: Effect on heart rate, EMG, trunk movements, and user acceptance during digging. Int. J. Ind. Ergon. 2018, 68, 290–296. [Google Scholar] [CrossRef]
- Hudson, D.S.; Copeland, J.L.; Hepburn, C.G.; Doan, J.B. Stooped Postures Are Modified by Pretask Walking in a Simulated Weed-Pulling Task. J. Agromed. 2014, 19, 27–34. [Google Scholar] [CrossRef] [PubMed]
- Ulrey, B.L.; Fathallah, F.A. Evaluation of a personal device in reducing the risk of low back disorders during stooped work. Work 2012, 41, 2381–2383. [Google Scholar] [CrossRef]
- Ulrey, B.L.; Fathallah, F.A. Effect of a personal weight transfer device on muscle activities and joint flexions in the stooped posture. J. Electromyogr. Kinesiol. 2013, 23, 195–205. [Google Scholar] [CrossRef] [PubMed]
- Anagnostis, A.; Benos, L.; Tsaopoulos, D.; Tagarakis, A.; Tsolakis, N.; Bochtis, D. Human activity recognition through recurrent neural networks for human-robot interaction in agriculture. Appl. Sci. 2021, 11, 2188. [Google Scholar] [CrossRef]
- Fathallah, F.A.; Tang, S.C.H.; Waters, T. Development and Evaluation of Ergonomic Interventions for Bucket Handling on Farms. Hum. Factors 2016, 58, 758–776. [Google Scholar] [CrossRef]
- de Looze, M.P.; Bosch, T.; Krause, F.; Stadler, K.S.; O’Sullivan, L.W. Exoskeletons for industrial application and their potential effects on physical work load. Ergonomics 2016, 59, 671–681. [Google Scholar] [CrossRef]
- Ulrey, B.L.; Fathallah, F.A. Subject-specific, whole-body models of the stooped posture with a personal weight transfer device. J. Electromyogr. Kinesiol. 2013, 23, 206–215. [Google Scholar] [CrossRef]
- Thamsuwan, O.; Milosavljevic, S.; Srinivasan, D.; Trask, C. Potential exoskeleton uses for reducing low back muscular activity during farm tasks. Am. J. Ind. Med. 2020, 63, 1017–1028. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; Zha, S.; Xia, Y.; Gu, J.; Lin, X. A Hip Active Assisted Exoskeleton That Assists the Semi-Squat Lifting. Appl. Sci. 2020, 10, 2424. [Google Scholar] [CrossRef]
- Bergerman, M.; Maeta, S.M.; Zhang, J.; Freitas, G.M.; Hamner, B.; Singh, S.; Kantor, G. Robot farmers: Autonomous orchard vehicles help tree fruit production. IEEE Robot. Autom. Mag. 2015, 22, 54–63. [Google Scholar] [CrossRef]
- Vasconez, J.P.; Kantor, G.A.; Auat Cheein, F.A. Human–robot interaction in agriculture: A survey and current challenges. Biosyst. Eng. 2019, 179, 35–48. [Google Scholar] [CrossRef]
- Benos, L.; Tagarakis, A.C.; Dolias, G.; Berruto, R.; Kateris, D.; Bochtis, D. Machine Learning in Agriculture: A Comprehensive Updated Review. Sensors 2021, 21, 3758. [Google Scholar] [CrossRef]
- Tzounis, A.; Katsoulas, N.; Bartzanas, T.; Kittas, C. Internet of Things in agriculture, recent advances and future challenges. Biosyst. Eng. 2017, 164, 31–48. [Google Scholar] [CrossRef]
- Benos, L.; Bechar, A.; Bochtis, D. Safety and ergonomics in human-robot interactive agricultural operations. Biosyst. Eng. 2020, 200, 55–72. [Google Scholar] [CrossRef]
- Marinoudi, V.; Lampridi, M.; Kateris, D.; Pearson, S.; Sørensen, C.G.; Bochtis, D. The Future of Agricultural Jobs in View of Robotization. Sustainability 2021, 13, 2109. [Google Scholar] [CrossRef]
- Grimstad, L.; From, P.J. The Thorvald II Agricultural Robotic System. Robotics 2017, 6, 24. [Google Scholar] [CrossRef]
- SYNERGIE—iBO. Available online: https://ibo.certh.gr/project/synergie/ (accessed on 19 November 2021).
- Tagarakis, A.C.; Benos, L.; Aivazidou, E.; Anagnostis, A.; Kateris, D.; Bochtis, D. Wearable Sensors for Identifying Activity Signatures in Human-Robot Collaborative Agricultural Environments. Eng. Proc. 2021, 9, 5. [Google Scholar] [CrossRef]
- Humadi, A.; Nazarahari, M.; Ahmad, R.; Rouhani, H. In-field instrumented ergonomic risk assessment: Inertial measurement units versus Kinect V2. Int. J. Ind. Ergon. 2021, 84, 103147. [Google Scholar] [CrossRef]
- Tsarouhas, A.; Iosifidis, M.; Kotzamitelos, D.; Spyropoulos, G.; Tsatalas, T.; Giakas, G. Three-Dimensional Kinematic and Kinetic Analysis of Knee Rotational Stability After Single- and Double-Bundle Anterior Cruciate Ligament Reconstruction. Arthrosc. J. Arthrosc. Relat. Surg. 2010, 26, 885–893. [Google Scholar] [CrossRef]
- Schwartz, M.H.; Rozumalski, A. A new method for estimating joint parameters from motion data. J. Biomech. 2005, 38, 107–116. [Google Scholar] [CrossRef] [PubMed]
- Ford, K.R.; Myer, G.D.; Hewett, T.E. Valgus knee motion during landing in high school female and male basketball players. Med. Sci. Sports Exerc. 2003, 35, 1745–1750. [Google Scholar] [CrossRef]
- Vicon Motion Systems Lower Body Modeling with Plug-in Gait. Available online: https://docs.vicon.com/display/Nexus25/Lower+body+modeling+with+Plug-in+Gait (accessed on 20 October 2021).
- Ehrig, R.M.; Taylor, W.R.; Duda, G.N.; Heller, M.O. A survey of formal methods for determining the centre of rotation of ball joints. J. Biomech. 2006, 39, 2798–2809. [Google Scholar] [CrossRef]
- Ehrig, R.M.; Taylor, W.R.; Duda, G.N.; Heller, M.O. A survey of formal methods for determining functional joint axes. J. Biomech. 2007, 40, 2150–2157. [Google Scholar] [CrossRef]
- Zaman, R.; Xiang, Y.; Cruz, J.; Yang, J. Three-dimensional asymmetric maximum weight lifting prediction considering dynamic joint strength. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2021, 235, 437–446. [Google Scholar] [CrossRef] [PubMed]
- Pan, F.; Firouzabadi, A.; Zander, T.; Schmidt, H. Sex-dependent differences in lumbo-pelvic coordination for different lifting tasks: A study on asymptomatic adults. J. Biomech. 2020, 102, 109505. [Google Scholar] [CrossRef]
- Mina, M.A.; Blazevich, A.J.; Tsatalas, T.; Giakas, G.; Seitz, L.B.; Kay, A.D. Variable, but not free-weight, resistance back squat exercise potentiates jump performance following a comprehensive task-specific warm-up. Scand. J. Med. Sci. Sports 2019, 29, 380–392. [Google Scholar] [CrossRef] [PubMed]
- Graveling, R.A.; Melrose, A.S.; Hanson, M.A. The Principles of Good Manual Handling: Achieving a Consensus; HSE Books: London, UK, 2003. [Google Scholar]
- Plamondon, A.; Delisle, A.; Bellefeuille, S.; Denis, D.; Gagnon, D.; Larivière, C. Lifting strategies of expert and novice workers during a repetitive palletizing task. Appl. Ergon. 2014, 45, 471–481. [Google Scholar] [CrossRef] [PubMed]
- Han, D.; Nam, S.; Song, J.; Lee, W.; Kang, T. The effect of knee flexion angles and ground conditions on the muscle activation of the lower extremity in the squat position. J. Phys. Ther. Sci. 2017, 29, 1852–1855. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Hwang, S.; Kim, Y.; Kim, Y. Lower extremity joint kinetics and lumbar curvature during squat and stoop lifting. BMC Musculoskelet. Disord. 2009, 10, 15. [Google Scholar] [CrossRef] [PubMed]
- Marras, W.S.; Granata, K.P.; Davis, K.G.; Allread, W.G.; Jorgensen, M.J. Spine loading and probability of low back disorder risk as a function of box location on a pallet. Hum. Factors Ergon. Manuf. 1997, 7, 323–336. [Google Scholar] [CrossRef]
- ISO. ISO—ISO 9241-210:2010—Ergonomics of Human-System Interaction—Part 210: Human-Centred Design for Interactive Systems. Available online: https://www.iso.org/standard/52075.html (accessed on 25 June 2020).

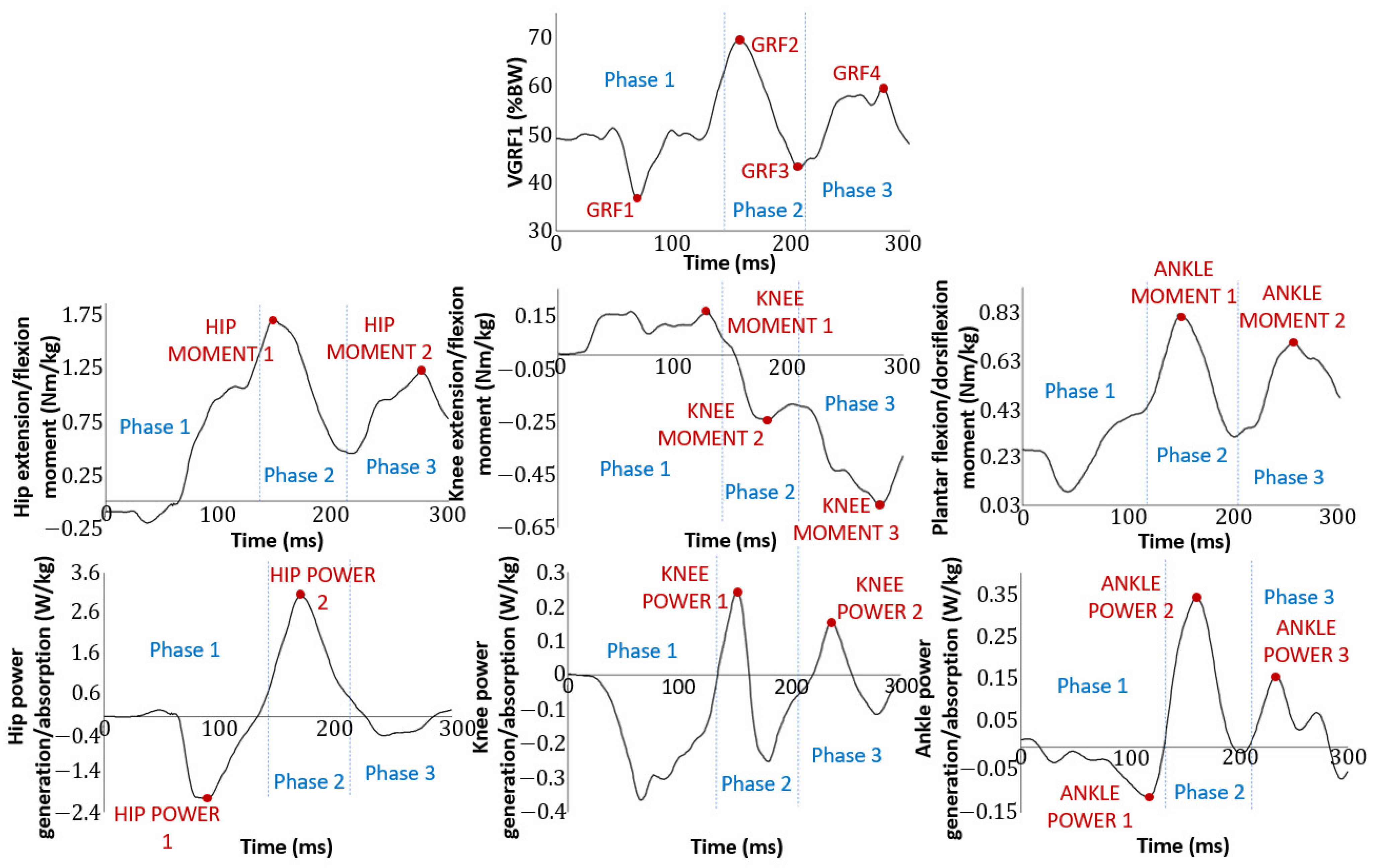
| Variables | Units | Description |
|---|---|---|
| GRF1 | % BW | Minimum VGRF during phase 1 |
| GRF2 | % BW | Maximum VGRF during phase 2 |
| GRF3 | % BW | Minimum VGRF between phases 2 and 3 |
| GRF4 | % BW | Maximum VGRF during phase 3 |
| KNEE MOMENT 1 | Nm/kg | Maximum knee extension moment during phase 1 |
| KNEE MOMENT 2 | Nm/kg | Maximum knee flexion moment during phase 2 |
| KNEE MOMENT 3 | Nm/kg | Maximum knee flexion moment during phase 3 |
| KNEE ANGLE MAX | degrees | Maximum knee flexion angle |
| HIP ANGLE MAX | degrees | Maximum hip flexion angle |
| HIP MOMENT 1 | Nm/kg | Maximum hip extension moment during phase 1 |
| HIP MOMENT 2 | Nm/kg | Maximum hip extension moment during phase 3 |
| ANKLE ANGLE MAX | degrees | Maximum dorsiflexion angle |
| ANKLE MOMENT 1 | Nm/kg | Maximum dorsiflexion moment during phase 1 |
| ANKLE MOMENT 2 | Nm/kg | Maximum dorsiflexion moment during phase 3 |
| KNEE POWER 1 | W/kg | Maximum knee absorption power |
| KNEE POWER 2 | W/kg | Maximum knee generation power |
| HIP POWER 1 | W/kg | Maximum hip absorption power |
| HIP POWER 2 | W/kg | Maximum hip generation power |
| ANKLE POWER 1 | W/kg | Maximum ankle absorption power |
| ANKLE POWER 2 | W/kg | Maximum ankle generation power during phase 2 |
| ANKLE POWER 3 | W/kg | Maximum ankle generation power during phase 3 |
| Variables | Height | p | ||
|---|---|---|---|---|
| 70 cm | 80 cm | 90 cm | ||
| GRF1 | 34.81 ± 5.00 | 35.35 ± 5.55 | 36.87 ± 4.18 | NS |
| GRF2 | 76.64 ± 7.33 | 77.69 ± 6.88 | 74.63 ± 5.73 | NS |
| GRF3 | 43.08 ± 7.77 | 45.39 ± 5.76 | 44.98 ± 4.60 | NS |
| GRF4 | 63.22 ± 6.58 | 62.39 ± 4.06 | 62.50 ± 5.70 | NS |
| KNEE MOMENT 1 | 0.65 ± 0.34 | 0.69 ± 0.27 | 0.74 ± 0.27 | NS |
| KNEE MOMENT 2 | −0.27 ± 0.16 | −0.25 ± 0.13 | −0.22 ± 0.10 | NS |
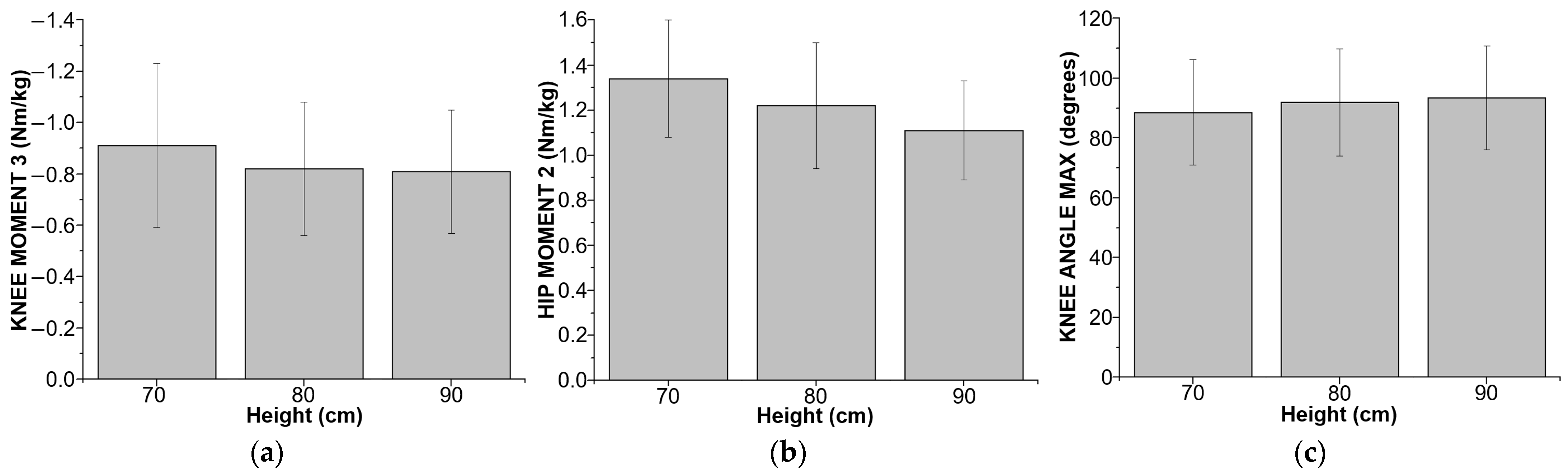
| KNEE MOMENT 3 | −0.91 ± 0.32 | −0.82 ± 0.26 | −0.81 ± 0.24 | <0.05 |
| KNEE ANGLE MAX | 88.55 ± 17.59 | 91.93 ± 17.94 | 93.44 ± 17.42 | <0.05 |
| HIP ANGLE MAX | 101.45 ± 4.40 | 101.50 ± 4.95 | 101 ± 6.20 | NS |
| HIP MOMENT 1 | 1.73 ± 0.20 | 1.78 ± 0.25 | 1.75 ± 0.24 | NS |
| HIP MOMENT 2 | 1.34 ± 0.26 | 1.22 ± 0.28 | 1.11 ± 0.22 | <0.05 |
| ANKLE ANGLE MAX | 28.26 ± 10.82 | 29.33 ± 11.11 | 29.01 ± 9.99 | NS |
| ANKLE MOMENT 1 | 0.97 ± 0.16 | 0.97 ± 0.15 | 0.92 ± 0.15 | NS |
| ANKLE MOMENT 2 | 0.92 ± 0.15 | 0.90 ± 0.14 | 0.89 ± 0.15 | NS |
| KNEE POWER 1 | −1.12 ± 0.51 | −1.03 ± 0.40 | −1.07 ± 0.63 | NS |
| KNEE POWER 2 | 0.94 ± 0.81 | 1.03± 0.77 | 1.05 ± 1.10 | NS |
| HIP POWER 1 | −1.84 ± 0.44 | −1.88 ± 0.58 | −1.76± 0.49 | NS |
| HIP POWER 2 | 3.14 ± 0.84 | 3.30 ± 0.91 | 3.11 ± 0.72 | NS |
| ANKLE POWER 1 | −0.21 ± 0.09 | −0.21 ± 0.09 | −0.18 ± 0.07 | NS |
| ANKLE POWER 2 | 0.69 ± 0.39 | 0.63 ± 0.21 | 0.64 ± 0.29 | NS |
| ANKLE POWER 3 | 0.20 ± 0.07 | 0.21 ± 0.09 | 0.20 ± 0.57 | NS |
| Variables | Height | p | ||
|---|---|---|---|---|
| 70 cm | 80 cm | 90 cm | ||
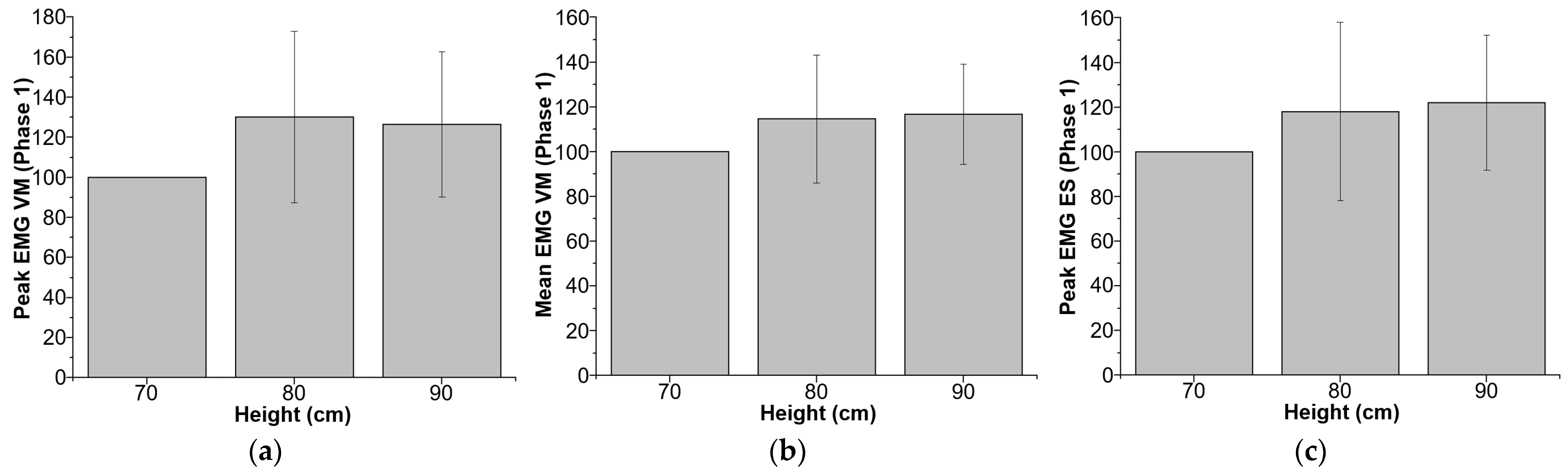
| Mean EMG VM (Phase 1) | 100 ± 0 | 114.60 ± 28.61 | 116.76 ± 22.41 | <0.05 |
| Peak EMG VM (Phase 1) | 100 ± 0 | 130.14 ± 42.82 | 126.49 ± 36.37 | <0.05 |
| Mean EMG VM (Phase 2) | 100 ± 0 | 109.11 ± 36.26 | 99.46 ± 28.44 | NS |
| Peak EMG VM (Phase 2) | 100 ± 0 | 122.61 ± 27.63 | 111.98 ± 26.99 | NS |
| Mean EMG VM (Phase 3) | 100 ± 0 | 97.01 ± 25.11 | 107.06 ± 35.99 | NS |
| Peak EMG VM (Phase 3) | 100 ± 0 | 86.85 ± 37.29 | 115.96 ± 63.73 | NS |
| Mean EMG ES (Phase 1) | 100 ± 0 | 98.43 ± 17.34 | 113.94 ± 23.35 | NS |
| Peak EMG ES (Phase 1) | 100 ± 0 | 118.04 ± 39.98 | 122.03 ± 30.31 | <0.05 |
| Mean EMG ES (Phase 2) | 100 ± 0 | 104.10 ± 22.11 | 113.31 ± 24.35 | NS |
| Peak EMG ES (Phase 2) | 100 ± 0 | 97.52 ± 20.91 | 104.75 ± 19.66 | NS |
| Mean EMG ES (Phase 3) | 100 ± 0 | 99.20 ± 22.96 | 102.66 ± 24.07 | NS |
| Peak EMG ES (Phase 3) | 100 ± 0 | 101.59 ± 33.57 | 107.84 ± 34.97 | NS |
| Mean EMG GASTRO (Phase 2) | 100 ± 0 | 134.48 ± 63.20 | 120.52 ± 79.51 | NS |
| Peak EMG GASTRO (Phase 2) | 100 ± 0 | 143.11 ± 95.06 | 149.10 ± 197.03 | NS |
| Mean EMG GASTRO (Phase 3) | 100 ± 0 | 111.37 ± 36.05 | 104.60 ± 31.10 | NS |
| Peak EMG GASTRO (Phase 3) | 100 ± 0 | 114.00 ± 39.83 | 106.74 ± 21.09 | NS |
| Mean EMG BF (Phase 2) | 100 ± 0 | 115.22 ± 40.03 | 99.67 ± 30.77 | NS |
| Peak EMG BF (Phase 2) | 100 ± 0 | 127.06 ± 65.50 | 109.12 ± 44.57 | NS |
| Mean EMG BF (Phase 3) | 100 ± 0 | 100.93 ± 25.54 | 94.50 ± 24.11 | NS |
| Peak EMG BF (Phase 3) | 100 ± 0 | 91.60 ± 36.09 | 89.70 ± 31.13 | NS |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Benos, L.; Kokkotis, C.; Tsatalas, T.; Karampina, E.; Tsaopoulos, D.; Bochtis, D. Biomechanical Effects on Lower Extremities in Human-Robot Collaborative Agricultural Tasks. Appl. Sci. 2021, 11, 11742. https://doi.org/10.3390/app112411742
Benos L, Kokkotis C, Tsatalas T, Karampina E, Tsaopoulos D, Bochtis D. Biomechanical Effects on Lower Extremities in Human-Robot Collaborative Agricultural Tasks. Applied Sciences. 2021; 11(24):11742. https://doi.org/10.3390/app112411742
Chicago/Turabian StyleBenos, Lefteris, Christos Kokkotis, Themistoklis Tsatalas, Evangeli Karampina, Dimitrios Tsaopoulos, and Dionysis Bochtis. 2021. "Biomechanical Effects on Lower Extremities in Human-Robot Collaborative Agricultural Tasks" Applied Sciences 11, no. 24: 11742. https://doi.org/10.3390/app112411742
APA StyleBenos, L., Kokkotis, C., Tsatalas, T., Karampina, E., Tsaopoulos, D., & Bochtis, D. (2021). Biomechanical Effects on Lower Extremities in Human-Robot Collaborative Agricultural Tasks. Applied Sciences, 11(24), 11742. https://doi.org/10.3390/app112411742










