The Melt Enthalpy of Pu₆Fe

Abstract

Schwartz, et al., previously reported calorimetry measurements conducted on a Pu-Pu${}_6$Fe mixture, from which they derived a melt enthalpy of 31.2 J/g (46.6 kJ/mol) for Pu${}_6$Fe. This was the first—and remains the only—such value to appear in the literature. We reanalyze those results in light of two contributions to the measured heat flow not considered in the original report: the melt enthalpy of the excess Pu and the subsequent heating of the liquid mixture. These corrections yield a revised value of 24.4 J/g (36.3 kJ/mol), which we show to be consistent with the melt enthalpy of U${}_6$Fe.

1. Introduction

The interaction of Pu with Fe is an important consideration in reactor design, a fact first discovered in the 1940s as part of the Los Alamos Molten Plutonium Reactor (LAMPRE) program. Pu heated above ∼410 C will react with the Fe of its steel confinement, then melt with formation of a liquid having the eutectic composition. This can pose an issue even with small quantities of Pu, as alloy formation can still lead to local melting and embrittlement upon migration to grain boundaries [1]. The presence of Pu${}_6$Fe in Ga-stabilized Pu alloys was first confirmed by X-ray diffraction in Reference [2].

Schwartz, Tobash, and Richmond (STR) recently reported differential scanning calorimetry (DSC) measurements on Pu${}_6$Fe [3], providing the first estimate of its melt enthalpy. Because this was—and currently remains—the only measurement of $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ available in the literature, STR compared their value to that of U${}_6$Fe. The aims of this brief report are to (1) demonstrate that the STR analysis is incorrect, yielding a $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ almost 30% higher than what was actually measured; (2) provide a corrected estimate of $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ based on reanalysis of the data; and (3) propose a consistency check superior to straightforward comparison with $\Delta H_{\mathrm{fus}}$ in related materials such as U${}_6$Fe.

The first experimental Pu${}_6$Fe phase diagram was published in 1955 [4], then reproduced with considerably more detail in 1957 [5]. The latter was republished in 1961 [6], then updated in 1968 to its current state [7]. It was recently computed using the CALculation of PHAse Diagrams (CALPHAD) method informed by first principles electronic structure calculations [8]. Pu${}_6$Fe has a body-centered tetragonal crystal structure with space group $I4/mcm$, identical to that of U${}_6$Fe.

2. Original Analysis

The DSC heating cycle used in the STR analysis is reproduced in Figure 1. The solid line is the data, and dashed lines are “baselines” added for the purpose of integrating the area under peaks associated with phase transitions. The $\alpha \to \beta$ and $\gamma \to \delta$ transitions in pure Pu are readily apparent at approximately 350 and 595 K, as is the prominent melt feature at the eutectic temperature of $T_{\mathrm{eu}}\approx 685$ K. Through comparison of their measured $\alpha \to \beta$ enthalpy change with a reference value of $\Delta H_{\alpha \to \beta }=15.7$ J/g, STR inferred that 12 wt% of their $m=29.7$ mg sample was pure Pu. This translates to a mass $m_{\mathrm{Pu}}=3.56\times {10}^{-3}$g, or $n_{\mathrm{Pu}}=1.49\times {10}^{-5}$ mol. Similarly, $m_{{\mathrm{Pu}}_6\mathrm{Fe}}=2.61\times {10}^{-2}$ g or $n_{{\mathrm{Pu}}_6\mathrm{Fe}}=1.75\times {10}^{-5}$ mol. The atom percentage of Fe was then a/o Fe $=12.7$. This is less than the ideal Pu${}_6$Fe composition of 14.3 a/o Fe, as anticipated based on the Pu transitions visible in Figure 1.

STR’s best estimate of raw heat absorption at melt was $Q\sim 27.5$ J/g, from which they derived a Pu${}_6$Fe melt enthalpy of

$$\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}=\frac{A_{{\mathrm{Pu}}_6\mathrm{Fe}}}{y_{{\mathrm{Pu}}_6\mathrm{Fe}}}Q=46.6\mathrm{kJ}/\mathrm{mol},$$

(1)

where $A_{{\mathrm{Pu}}_6\mathrm{Fe}}=1490.56584$ (g/mol) is the molar mass of Pu${}_6$Fe and $y_{{\mathrm{Pu}}_6\mathrm{Fe}}$ is the mass fraction of Pu${}_6$Fe in the mixture. This is equivalent to 6.67 kJ/(mol atoms), or 31.2 J/g. The logic of Equation (1) is simple and intuitive: $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ is the heat absorbed at $T_{\mathrm{eu}}$, after correction for the excess Pu on a per mass basis. The excess Pu itself is a thermal spectator, and the steadily accumulating liquid does not absorb additional heat.

3. Reanalysis

3.1. Composition and Heat Flow

Because it plays such an important role in identifying the composition, first we reexamine the $\alpha \to \beta$ transition highlighted in the inset of Figure 1. STR obtained a heat absorption of $Q_{\alpha \to \beta }=1.87$ J/g only by neglecting to subtract a baseline like the dashed one shown. Although this correction appears small, it represents a significant contribution to the integral and by it we obtain a revised value of 1.43 J/g. STR’s reference enthalpy of 15.7 J/g for the $\alpha \to \beta$ transition was uncited, and we have chosen the 15.5 J/g “selected value” of Reference [9] (see Table 33 of that work) as our benchmark instead. The combination of these two adjustments yields a revised a/o Fe of 13.1, a small increase over that of STR. Similar reanalysis of the area under the melt peak returned values close to that of STR and will be discussed within the context of uncertainties in Section 4.3.

3.2. Phase Diagram

For reasons that will become clear, the remainder of our reanalysis relies heavily on the Pu${}_6$Fe liquidus curve. As noted in the Introduction, four versions have appeared in the literature. We rejected Konobeevsky’s [4] due to the small size of the image and its notable lack of detail. Schonfeld’s report [6] is clear in stating that the diagram presented has no empirical basis beyond that already given by Mardon, leaving only Mardon [5] and Ellinger [7] as options.

These two are reproduced in Figure 2, where circles represent actual data, dashed curves are interpolations through regions where there were no data, and solid curves are fits through regions where there were data. The distinctions between dashed and solid are those of the original authors (not our own), and the revised STR composition is indicated by the vertical dotted line. Compositions relevant to our reanalysis are a/o Fe ∼ 10 − 14, where the Mardon liquidus curve is clearly higher than that of Ellinger. Note, however, that Mardon’s liquidus in this region is entirely an interpolation between data near the eutectic temperature and those at much much higher (>50%) Fe concentrations. The Ellinger phase diagram, on the other hand, was further constrained by data (red points) collected as part of the LAMPRE program and only reported in 1964 [10], well after Mardon’s work. For this reason, we regard the Ellinger liquidus as the most reliable and will use it exclusively in what follows.

Figure 2. Pu${}_6$Fe liquidus curves according to References [5,7,10]. Points are data, solid curves are fits to data, and dashed curves are interpolations between data sets. The solid/dashed distinctions are as they appear in the original references. Our revised STR composition is indicated by the vertical dotted line.

Figure 2. Pu${}_6$Fe liquidus curves according to References [5,7,10]. Points are data, solid curves are fits to data, and dashed curves are interpolations between data sets. The solid/dashed distinctions are as they appear in the original references. Our revised STR composition is indicated by the vertical dotted line.

An enlarged version of the relevant phase space is provided in Figure 3. In addition to the liquidus (black), we have added the eutectic and peritectic temperatures as horizontal lines. The STR composition is still indicated by the vertical line, where now its intersection with the Ellinger liquidus is emphasized with a box. It is worth noting that this temperature ($T_{\mathrm{liq}}\left(13.1\right)\equiv T^{\ast }=441$ C) is just below the highest temperature reached in the STR experiments, as shown in Figure 1. Heat flow in excess of that required for simple temperature increase appears to terminate at around this point, as would be expected near the liquidus. In other words, the STR DSC results appear to be consistent with the Ellinger liquidus curve.

With this background and the help of Figure 3, we now explain our correction of the STR melt enthalpy in more detail. As the system was heated, it moved upward along the vertical dotted line until reaching $T=T_{\mathrm{eu}}$ at (1). At this point, all of the excess Pu melted, accompanied by enough Pu${}_6$Fe to yield a liquid of composition given by the liquidus curve at $T_{\mathrm{eu}}$; this liquid composition is indicated by (2). The temperature of the liquid then rose to $T_{\mathrm{peri}}$ and its composition evolved from (2) to (3), then from $T_{\mathrm{peri}}$ to $T^{\ast }$ as its composition evolved from (3) to (4). At (4), all of the solid was melted and the temperature continued to rise along the vertical dotted line.

Figure 3. Enlarged view of the Pu${}_6$Fe phase diagram relevant to reanalysis of the DSC data shown in Figure 1. The liquidus curve is solid black, the eutectic and peritectic are dashed green, and the STR composition is dotted green.

Figure 3. Enlarged view of the Pu${}_6$Fe phase diagram relevant to reanalysis of the DSC data shown in Figure 1. The liquidus curve is solid black, the eutectic and peritectic are dashed green, and the STR composition is dotted green.

This discussion frames the two ways in which Equation (1) is incomplete, in that it fails to correct total heat flow for contributions from (a) the latent heat of melting of the excess Pu at $T_{\mathrm{eu}}$ [11], and (b) absorption of heat by the liquid once all of the excess Pu melted and the temperature again rose. A modified form of Equation (1) accounting for both of these is

$$\begin{array}{cc}\hfill \Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}& =\frac{mQ-n_{\mathrm{Pu}}\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)-{\int }_{T_{\mathrm{eu}}}^{T_{\mathrm{liq}}}{n}_{\mathrm{liq}}\left(T\right)C_P^{\mathrm{mix}}\left(T\right)dT}{n_{{\mathrm{Pu}}_6\mathrm{Fe}}}\hfill \\ \hfill & =\frac{mQ-n_{\mathrm{Pu}}\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)-\Delta H_{\mathrm{liq}}}{n_{{\mathrm{Pu}}_6\mathrm{Fe}}}\hfill \\ \hfill & =\frac{m\left(Q-\frac{Q_{\alpha \to \beta }}{A_{\mathrm{Pu}}\Delta H_{\alpha \to \beta }}\right)\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)-\Delta H_{\mathrm{liq}}}{\frac{m}{A_{{\mathrm{Pu}}_6\mathrm{Fe}}}\left(1-\frac{Q_{\alpha \to \beta }}{\Delta H_{\alpha \to \beta }}\right)},\hfill \end{array}$$

(2)

where $n_{\mathrm{liq}}$ is the total moles of liquid (Pu+Fe) as a function of temperature and $C_P^{\mathrm{mix}}$ is the specific heat of the liquid mixture. The second equality has been used to define $\Delta H_{\mathrm{liq}}$ as the heat absorbed by the accumulating liquid, and the third has been used to highlight the close connection between composition and the $\alpha \to \beta$ transition in pure Pu. We have distinguished the enthalpy of this transition as inferred from the DSC data of Figure 1 from the reference value by use of Q and $\Delta H$, respectively. Note that $\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)$ is Pu’s latent heat of fusion evaluated at the Pu-Pu${}_6$Fe eutectic—not the Pu fusion—temperature.

The remainder of this section will be devoted to deriving the two corrections included in Equation (2). Correction (a) is detailed in Section 3.4, and (b) in Section 3.5. Both are based on the following assumptions:

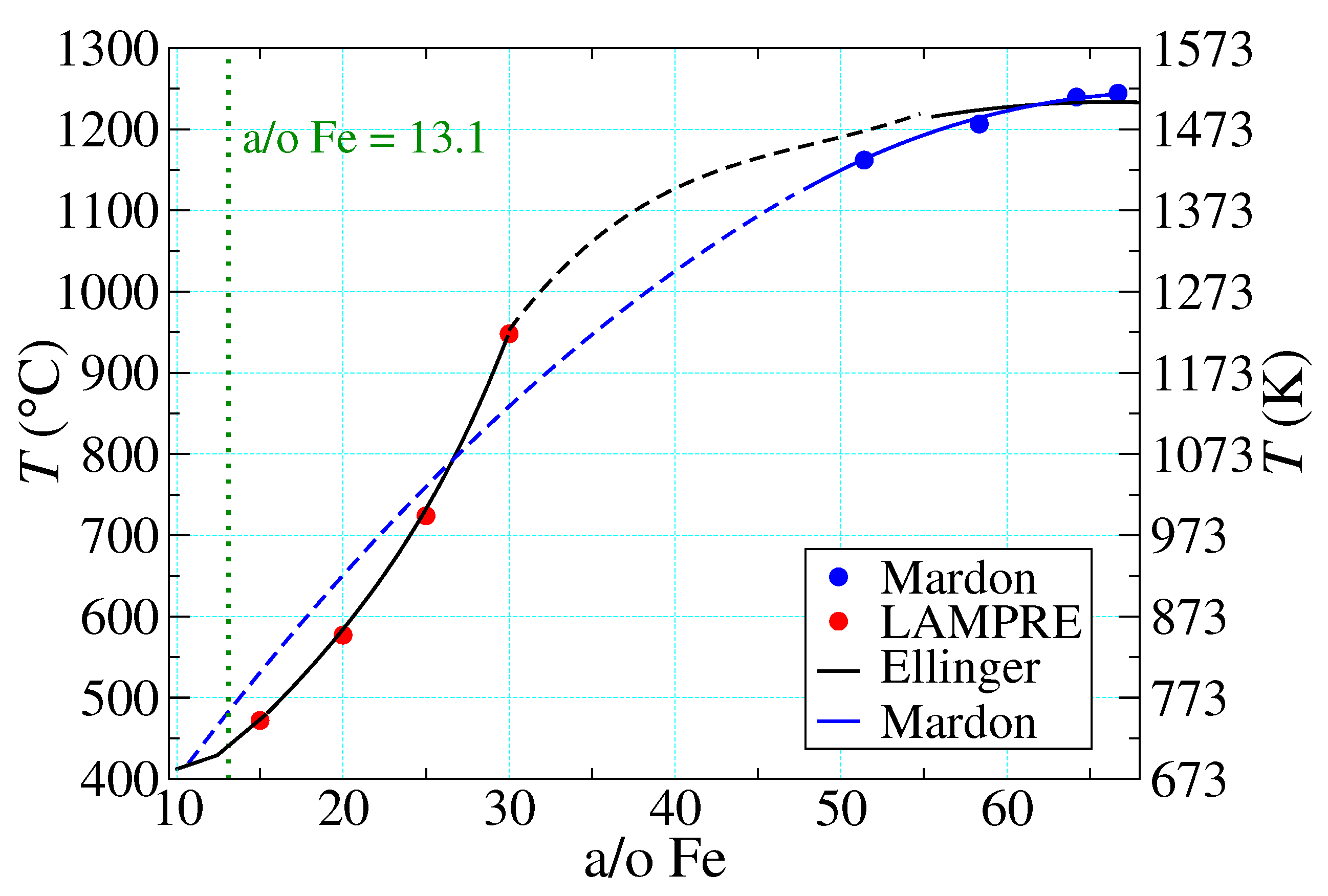
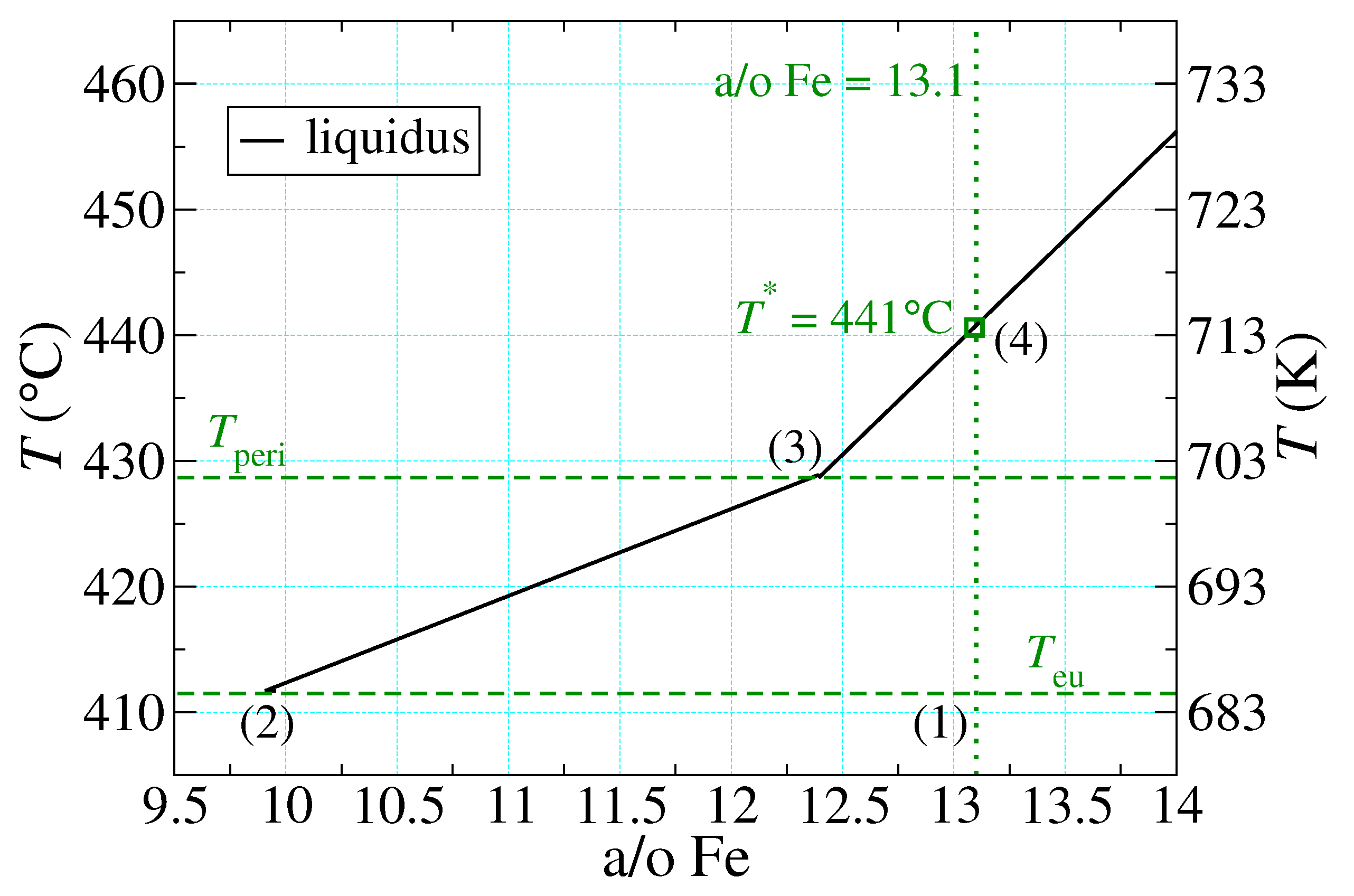
{1}

The properties of “supercooled” liquid Pu and Fe are the same as those of the normal liquids, which do not form until temperatures much higher than those of the Pu${}_6$Fe liquidus curve are reached. In particular, their thermal response is governed by the same (constant) specific heats characteristic of the normal liquids.

{2}

Liquid Pu and liquid Fe mix ideally, as described by Equation (11) below.

{3}

Once all of the excess Pu has melted at $T_{\mathrm{eu}}$, the remaining Pu${}_6$Fe melts linearly in T. This is described by Equation (10) below.

We note the existence of the peritectic reaction

$${\mathrm{Pu}}_6\mathrm{Fe}\leftrightarrow \mathrm{L}+{\mathrm{PuFe}}_2$$

(3)

at 628 C (701 K) [5] but do not add this point to the above list because the presence or absence of reaction (3) is irrelevant to the reanalysis of total heat absorption. Enthalpy is a thermodynamic potential, and therefore its value at a particular state—such as the liquidus temperature for the STR composition—is a function only of the state itself, independent of the path taken to reach it [12]. Aside from this fact, there is good reason to believe that reaction (3) is too slow to have been a factor in the STR measurements [5].

3.3. Materials, Conventions, and Data Sources

Reanalysis of the STR data involves numerous properties of Pu, Fe, and Pu${}_6$Fe, and we will compare our results to analogous quantities in U and U${}_6$Fe. We will also refer to the many phases of Pu and the transitions between them, as well as properties defined at more than one reference temperature. The combination of these factors inevitably produces cumbersome notation, which we have attempted to simplify by consistency. Material and phase designations typically will be indicated by superscripts and phase transitions by subscripts, although this convention will be reversed in designations of quantity such as mole numbers, masses, mole fractions, and mass fractions. When a thermodynamic property such as entropy or latent heat is defined at multiple temperatures, the temperature in question will be given explicitly in parenthesis.

Table 1, Table 2 and Table 3 list the inputs needed for correcting the melt enthalpy of Reference [3]. We have adopted the eutectic temperature $T_{\mathrm{eu}}=411.5$C of STR, which is simply the mean of those reported in References [4,5]. It is important not to conflate (mol of atoms)${}^{-1}$ with (mol of formula units)${}^{-1}$, and we will use the latter unless indicated explicitly. Per mol of atoms units are most appropriate for comparison of alloy results with those of the pure metals, whereas per mol of formula units are needed for conversion from per mol to per mass units. We will assume the isotopic composition of weapons grade Pu described in Table 14 of Reference [13], so $A_{\mathrm{Pu}}=239.12014$ g/mole.

Table 1. Transition temperatures used in the reanalysis of Pu${}_6$Fe melt data.

Quantity	C/K	Source
$T_{\mathrm{eu}}$	411.5/684.5	Reference [3]
$T_{\mathrm{peri}}$	428/701	Reference [5]
$T_{\alpha \to \beta }$	127/400	Reference [14]
$T_{\beta \to \gamma }$	213/486	Reference [14]
$T_{\gamma \to \delta }$	323/596	Reference [14]
$T_{\delta \to {\delta }^{\prime }}$	468/741	Reference [9,14]
$T_{{\delta }^{\prime }\to ϵ}$	486/759	Reference [9,14]
$T_{\mathrm{fus}}^{\mathrm{Pu}}$	640/913	Reference [9]
$T_{\mathrm{fus}}^{\mathrm{Fe}}$	1536/1809	Reference [15]
$T_{\mathrm{fus}}^{\mathrm{U}}$	1134/1407	Reference [9]
$T_{\mathrm{eu}}^{{\mathrm{U}}_6\mathrm{Fe}}$	827/1100	Reference [16]

Table 1. Transition temperatures used in the reanalysis of Pu${}_6$Fe melt data.

Quantity	C/K	Source
$T_{\mathrm{eu}}$	411.5/684.5	Reference [3]
$T_{\mathrm{peri}}$	428/701	Reference [5]
$T_{\alpha \to \beta }$	127/400	Reference [14]
$T_{\beta \to \gamma }$	213/486	Reference [14]
$T_{\gamma \to \delta }$	323/596	Reference [14]
$T_{\delta \to {\delta }^{\prime }}$	468/741	Reference [9,14]
$T_{{\delta }^{\prime }\to ϵ}$	486/759	Reference [9,14]
$T_{\mathrm{fus}}^{\mathrm{Pu}}$	640/913	Reference [9]
$T_{\mathrm{fus}}^{\mathrm{Fe}}$	1536/1809	Reference [15]
$T_{\mathrm{fus}}^{\mathrm{U}}$	1134/1407	Reference [9]
$T_{\mathrm{eu}}^{{\mathrm{U}}_6\mathrm{Fe}}$	827/1100	Reference [16]

Table 2. Specific heats and entropies used in the reanalysis of Pu${}_6$Fe melt data.

Quantity	J/mol/K	Source
$C_P^{\alpha }$	17.619 + 45.552T	Reference [9]
$C_P^{\beta }$	27.416 + 13.060T	Reference [9]
$C_P^{\gamma }$	22.023 + 22.959T	Reference [9]
$C_P^{\delta }$	28.4781 + 0.010807T	Reference [9]
$C_P^{{\delta }^{\prime }}$	35.56	Reference [9]
$C_P^{ϵ}$	33.72	Reference [9]
$C_P^l$	42.80	Reference [9]
$C_P^{\mathrm{Fe}}$	46.024	Reference [15]
$S^{\mathrm{Pu}}\left(298\right)$	54.46	Reference [9]
$S^{\mathrm{Fe}}\left(T_{\mathrm{fus}}\right)$	99.823	Reference [15]
$S^l(T_{\mathrm{fus}}$)	8344	this work

Table 2. Specific heats and entropies used in the reanalysis of Pu${}_6$Fe melt data.

Quantity	J/mol/K	Source
$C_P^{\alpha }$	17.619 + 45.552T	Reference [9]
$C_P^{\beta }$	27.416 + 13.060T	Reference [9]
$C_P^{\gamma }$	22.023 + 22.959T	Reference [9]
$C_P^{\delta }$	28.4781 + 0.010807T	Reference [9]
$C_P^{{\delta }^{\prime }}$	35.56	Reference [9]
$C_P^{ϵ}$	33.72	Reference [9]
$C_P^l$	42.80	Reference [9]
$C_P^{\mathrm{Fe}}$	46.024	Reference [15]
$S^{\mathrm{Pu}}\left(298\right)$	54.46	Reference [9]
$S^{\mathrm{Fe}}\left(T_{\mathrm{fus}}\right)$	99.823	Reference [15]
$S^l(T_{\mathrm{fus}}$)	8344	this work

Table 3. Transition enthalpies used in the reanalysis of Pu${}_6$Fe melt data. Quantity in parenthesis is in kJ/(mol atoms).

Quantity	kJ/mol	Source
$\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{fus}}^{\mathrm{Pu}}\right)$	2.766	Reference [9]
$\Delta H_{\mathrm{fus}}^{{\mathrm{U}}_6\mathrm{Fe}}$	111.65 (15.95)	Reference [16], this work
$\Delta H_{\mathrm{fus}}^{\mathrm{U}}$	8.470	Reference [9]
$\Delta H_{\alpha \to \beta }$	3.706	Reference [9]
$\Delta H_{\beta \to \gamma }$	0.478	Reference [9]
$\Delta H_{\gamma \to \delta }$	0.713	Reference [9]
$\Delta H_{\delta \to {\delta }^{\prime }}$	0.065	Reference [9]
$\Delta H_{{\delta }^{\prime }\to ϵ}$	1.711	Reference [9]

Table 3. Transition enthalpies used in the reanalysis of Pu${}_6$Fe melt data. Quantity in parenthesis is in kJ/(mol atoms).

Quantity	kJ/mol	Source
$\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{fus}}^{\mathrm{Pu}}\right)$	2.766	Reference [9]
$\Delta H_{\mathrm{fus}}^{{\mathrm{U}}_6\mathrm{Fe}}$	111.65 (15.95)	Reference [16], this work
$\Delta H_{\mathrm{fus}}^{\mathrm{U}}$	8.470	Reference [9]
$\Delta H_{\alpha \to \beta }$	3.706	Reference [9]
$\Delta H_{\beta \to \gamma }$	0.478	Reference [9]
$\Delta H_{\gamma \to \delta }$	0.713	Reference [9]
$\Delta H_{\delta \to {\delta }^{\prime }}$	0.065	Reference [9]
$\Delta H_{{\delta }^{\prime }\to ϵ}$	1.711	Reference [9]

3.4. Evaluating $\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)$

Our reanalysis requires the melt enthalpy of Pu at $T_{\mathrm{eu}}$, which is far below the fusion temperature of Pu ($T_{\mathrm{eu}}=412$ K, $T_{\mathrm{fus}}^{\mathrm{Pu}}=913$ K) and therefore not physically observable. To estimate $\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)$, we begin with $\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{fus}}\right)$ and subtract the (known) entropy increase in the solid due to heating from $T_{\mathrm{eu}}$ to $T_{\mathrm{fus}}$, do the same for the liquid based on the assumption that the specific heat does not change upon “supercooling,” then reevaluate the entropy difference at $T_{\mathrm{eu}}$:

$$\begin{array}{cc}\hfill \Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)& =T_{\mathrm{eu}}\Delta S\left(T_{\mathrm{eu}}\right)=T_{\mathrm{eu}}\left\{S^l\left(T_{\mathrm{eu}}\right)-S^{\delta }\left(T_{\mathrm{eu}}\right)\right\}\hfill \\ \hfill & =\phantom{iii}{T}_{\mathrm{eu}}\left[S^l\left(T_{\mathrm{fus}}\right)-{\int }_{T_{\mathrm{eu}}}^{T_{\mathrm{fus}}}\frac{C_P^l}{T}dT\right]\hfill \\ \hfill & \phantom{iii}-T_{\mathrm{eu}}\left[S^{ϵ}\left(T_{\mathrm{fus}}\right)-{\int }_{T_{{\delta }^{\prime }\to ϵ}}^{T_{\mathrm{fus}}}\frac{C_P^{ϵ}}{T}dT-\frac{\Delta H_{{\delta }^{\prime }\to ϵ}}{T_{{\delta }^{\prime }\to ϵ}}-{\int }_{T_{\delta \to {\delta }^{\prime }}}^{T_{{\delta }^{\prime }\to ϵ}}\frac{C_P^{{\delta }^{\prime }}}{T}dT-\frac{\Delta H_{\delta \to {\delta }^{\prime }}}{T_{\delta \to {\delta }^{\prime }}}-{\int }_{T_{\mathrm{eu}}}^{T_{\delta }\to T_{{\delta }^{\prime }}}\frac{C_P^{\delta }}{T}dT\right]\hfill \\ \hfill & =\phantom{iii}{T}_{\mathrm{eu}}\left[\frac{\Delta H_{\mathrm{fus}}}{T_{\mathrm{fus}}}+\frac{\Delta H_{\delta \to {\delta }^{\prime }}}{T_{\delta \to {\delta }^{\prime }}}+\frac{\Delta H_{{\delta }^{\prime }\to ϵ}}{T_{{\delta }^{\prime }\to ϵ}}\right]\hfill \\ \hfill & \phantom{iii}+T_{\mathrm{eu}}\left[{\int }_{T_{\mathrm{eu}}}^{T_{\delta }\to T_{{\delta }^{\prime }}}\frac{C_P^{\delta }}{T^{\prime }}d{T}^{\prime }+{\int }_{T_{\delta \to {\delta }^{\prime }}}^{T_{{\delta }^{\prime }\to ϵ}}\frac{C_P^{{\delta }^{\prime }}}{T}dT+{\int }_{T_{{\delta }^{\prime }\to ϵ}}^{T_{\mathrm{fus}}}\frac{C_P^{ϵ}}{T}dT-{\int }_{T_{\mathrm{eu}}}^{T_{\mathrm{fus}}}\frac{C_P^l}{T}dT\right].\hfill \end{array}$$

(4)

3.5. Liquid Heating Contribution

3.5.1. Liquid Composition

The stoichiometry of the material permits specification of liquid composition through the mol fraction of Fe only,

$$x_{\mathrm{Fe}}\left(T\right)=1-x_{\mathrm{Pu}}\left(T\right)\equiv x\left(T\right).$$

(5)

Because its mol fraction in the liquid is equivalent to its a/o on the liquidus, we obtain $x\left(T\right)$ by fitting segments of the liquidus curve from Reference [7]. These are well-reproduced by fits of the form $x=a+bT$ over the relevant temperature domain, with coefficients listed in

Table 4. Coefficients of fits to the liquidus curve of Ellinger [7]. Fits were of the form $x=a+bT$, for x defined in Equation (5).

$\mathit{a}\times 10$	$\mathit{b}\times {10}^4$ (1/K)	Domain
$-8.92$	14.5	$T_{\mathrm{eu}}\le T<T_{\mathrm{peri}}$
$-2.87$	5.85	$T_{\mathrm{peri}}\le T<T^{\ast }$

. Note that we have calculated the composition as $x\left(T\right)$ because that is what Equation (2) requires, whereas the standard phase diagram representation of Figure 2 and Figure 3 is $T\left(x\right)$. Denoting temperature derivatives by ${}^{\prime }$, this yields

$$x_{\mathrm{Fe}}^{\prime }\left(T\right)=-x_{\mathrm{Pu}}^{\prime }\left(T\right)\equiv x^{\prime }=b,$$

(6)

and all higher derivatives obviously vanish.

Equation 5 prescribes the composition of the liquid as $f\left(T\right)$ but not its total amount, as Equation (2) requires. However, it uniquely determines the initial composition at $T_{\mathrm{eu}}$ through

$$x=\frac{n_{\mathrm{Fe}}^l}{n_{\mathrm{Fe}}^l+n_{\mathrm{Pu}}^l}=\frac{n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l}{n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l+n_{\mathrm{Pu}}+6n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l}=\frac{n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l}{7n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l+n_{\mathrm{Pu}}}=a+bT.$$

(7)

This can be solved for $n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l$ to yield

$$n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l\left(T_{\mathrm{eu}}\right)=\frac{n_{\mathrm{Pu}}^l(a+b{T}_{\mathrm{eu}})}{1-7(a+b{T}_{\mathrm{eu}})},$$

(8)

the total amount of Pu${}_6$Fe that melts at the eutectic temperature. One then has to make an assumption regarding the rate at which the remaining solid melts as $f\left(T\right)$, but the simplest is that of linear increase in T up to the completion of melt at $T^{\ast }$,

$$n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l\left(T\right)=n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l\left(T_{\mathrm{eu}}\right)+\frac{n_{{\mathrm{Pu}}_6\mathrm{Fe}}-n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l\left(T_{\mathrm{eu}}\right)}{T^{\ast }-T_{\mathrm{eu}}}(T-T_{\mathrm{eu}}).$$

(9)

Total moles of liquid at a given T then follows as

$$n_{\mathrm{liq}}\left(T\right)=n_{\mathrm{Pu}}+7n_{{\mathrm{Pu}}_6\mathrm{Fe}}^l\left(T\right),$$

(10)

whereby specification of the liquid composition is complete.

3.5.2. Ideal Mixing

Because all quantities are functions only of temperature and evaluated at atmospheric pressure, we will omit explicit temperature-dependence and designation of the reference pressure. We will also ignore the trivial differences between Fe quantities evaluated at 1 bar vs. 1 atm. The mixture free energy under the assumption of ideal mixing is then

$$\begin{array}{cc}\hfill G& =x_{\mathrm{Pu}}{G}_{\mathrm{Pu}}+x_{\mathrm{Fe}}{G}_{\mathrm{Fe}}+RT(x_{\mathrm{Pu}}ln{x}_{\mathrm{Pu}}+x_{\mathrm{Fe}}ln{x}_{\mathrm{Fe}})\hfill \\ \hfill & =(1-x)G_{\mathrm{Pu}}+x{G}_{\mathrm{Fe}}+RT[(1-x)ln(1-x)+xlnx)].\hfill \end{array}$$

(11)

The entropy follows as

$$\begin{array}{cc}\hfill S& =-G^{\prime }=x^{\prime }{G}_{\mathrm{Pu}}-(1-x)G_{\mathrm{Pu}}^{\prime }-x^{\prime }{G}_{\mathrm{Fe}}-x{G}_{\mathrm{Fe}}^{\prime }\hfill \\ \hfill & \phantom{iiiiiiiiiiii}-R\left[xlnx+(1-x)ln(1-x)\right]-RT\left[-x^{\prime }ln(1-x)-x^{\prime }+x^{\prime }lnx+x^{\prime }\right]\hfill \\ \hfill & =x^{\prime }\left(G_{\mathrm{Pu}}-G_{\mathrm{Fe}}\right)+\left[x{S}_{\mathrm{Fe}}+(1-x)S_{\mathrm{Pu}}\right]-R\left[xlnx+\left(1-x\right)ln\left(1-x\right)\right]\hfill \\ \hfill & \phantom{iii}-RT{x}^{\prime }ln\left(\frac{x}{1-x}\right)\hfill \end{array}$$

(12)

and the specific heat as

$$\begin{array}{cc}\hfill C_P=H^{\prime }=T{S}^{\prime }& =2x^{\prime }T\left(S_{\mathrm{Fe}}-S_{\mathrm{Pu}}\right)+x{C}_{P,\mathrm{Fe}}+(1-x)C_{P,\mathrm{Pu}}\hfill \\ \hfill & \phantom{iii}-2x^{\prime }RTln\left(\frac{x}{1-x}\right)-R{T}^2\frac{{\left(x^{\prime }\right)}^2}{x(1-x)}.\hfill \end{array}$$

(13)

Equation (13) requires forms for $S_{\mathrm{Pu}}$ and $S_{\mathrm{Fe}}$ as $f\left(T\right)$. Again assuming that Pu and Fe exist in a supercooled liquid state for $T<T_{\mathrm{fus}}$,

$$S\left(T\right)=S\left(T_{\mathrm{fus}}\right)-{\int }_T^{T_{\mathrm{fus}}}\frac{C_P\left(T^{\prime }\right)}{T^{\prime }}d{T}^{\prime }=S\left(T_{\mathrm{fus}}\right)-C_P^{l}ln\left(\frac{T_{\mathrm{fus}}}{T}\right),$$

(14)

taking as inputs $C_P^l$, $T_{\mathrm{fus}}$, and $S\left(T_{\mathrm{fus}}\right)$ for Fe or Pu. All Fe quantities were taken directly from the NIST-JANAF table [15], and all Pu quantities have been explained previously. The entropy of liquid Pu at $T_{\mathrm{fus}}^{\mathrm{Pu}}$ is

$$\begin{array}{cc}\hfill S^l\left(T_{\mathrm{fus}}^{\mathrm{Pu}}\right)& =S^{\mathrm{Pu}}\left(298\right)+{\int }_{298}^{T_{\alpha \to \beta }}\frac{C_P^{\alpha }}{T}dT+{\int }_{T_{\alpha \to \beta }}^{T_{\beta \to \gamma }}\frac{C_P^{\beta }}{T}dT+{\int }_{T_{\beta \to \gamma }}^{T_{\gamma \to \delta }}\frac{C_P^{\gamma }}{T}dT\hfill \\ \hfill & \phantom{iii}+{\int }_{T_{\gamma \to \delta }}^{T_{\delta \to {\delta }^{\prime }}}\frac{C_P^{\delta }}{T}dT+{\int }_{T_{\delta \to {\delta }^{\prime }}}^{T_{{\delta }^{\prime }\to ϵ}}\frac{C_P^{{\delta }^{\prime }}}{T}dT+{\int }_{T_{{\delta }^{\prime }\to ϵ}}^{T_{\mathrm{fus}}}\frac{C_P^{ϵ}}{T}dT\hfill \\ \hfill & \phantom{iii}+\frac{\Delta H_{\alpha \to \beta }}{T_{\alpha \to \beta }}+\frac{\Delta H_{\beta \to \gamma }}{T_{\beta \to \gamma }}+\frac{\Delta H_{\gamma \to \delta }}{T_{\gamma \to \delta }}+\frac{\Delta H_{\delta \to {\delta }^{\prime }}}{T_{\delta \to {\delta }^{\prime }}}+\frac{\Delta H_{{\delta }^{\prime }\to ϵ}}{T_{{\delta }^{\prime }\to ϵ}}+\frac{\Delta H_{\mathrm{fus}}}{T_{\mathrm{fus}}}\hfill \\ \hfill & =8.344\mathrm{kJ}/\mathrm{mol}/\mathrm{K}.\hfill \end{array}$$

(15)

Equations (14), (5), and (6) were substituted into  (13), and then the product of Equations (10) and (13) was integrated numerically by Simpson’s Rule using the scipy.optimize.simps package [17].

4. Results and Discussion

4.1. Corrected Result

The results of our reanalysis are summarized in

Table 5. Reanalysis of the STR data [3] based on Equation (2), using the phase diagram of Reference [7]. $T^{\ast }$ is the liquidus temperature at an a/o Fe of 13.1, and R is defined in Equation (17) of the text.

${\mathit{T}}^{\ast }$ °C/K	$\mathbf{\Delta }{\mathit{H}}_{\mathbf{fus}}^{\mathbf{Pu}}\left({\mathit{T}}_{\mathbf{eu}}\right)$ kJ/mol J/g	${\mathit{n}}_{\mathbf{Pu}}\mathbf{\Delta }{\mathit{H}}_{\mathbf{fus}}^{\mathbf{Pu}}\left({\mathit{T}}_{\mathbf{eu}}\right)$ J	$\mathbf{\Delta }{\mathit{H}}_{\mathbf{liq}}$ J	$\mathbf{\Delta }{\mathit{H}}_{\mathbf{fus}}^{{\mathbf{Pu}}_6\mathbf{Fe}}$ kJ/mol J/g kJ/(mol atoms)	R
	2.05			36.3
441/714	8.58	0.0234	0.115	24.4	2.50
				5.19

. The correction due to liquid heating ($\Delta H_{\mathrm{liq}}$) is 6× greater than that of Pu melting. As a point of interest, we note that Equation (4) gives an answer differing by ∼1% with the far simpler assumption that melt entropy is independent of absolute temperature,

$$\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)=\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{fus}}^{\mathrm{Pu}}\right)\frac{T_{\mathrm{eu}}}{T_{\mathrm{fus}}^{\mathrm{Pu}}}=2.08\mathrm{kJ}/\mathrm{mol}=8.69\mathrm{J}/\mathrm{g}.$$

(16)

Our corrected $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ of 24.4 J/g is 28% lower than the 31.2 J/g value obtained using Equation (1).

4.2. Consistency

Because there is no other experimental value for $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ with which to compare, we require an alternative means of checking our result for consistency. STR compared to $\Delta H_{\mathrm{fus}}^{{\mathrm{U}}_6\mathrm{Fe}}$, a natural choice based on proximity in the periodic table and the isostructural character of the two compounds. However, the precise significance of this comparison is unclear for at least two reasons. First, $\Delta H_{\mathrm{fus}}\propto T_{\mathrm{fus}}$ (or $T_{\mathrm{eu}}$, in the case of the alloy), and one should normalize for the $1.6\times$ difference between $T_{\mathrm{eu}}^{{\mathrm{U}}_6\mathrm{Fe}}$ and $T_{\mathrm{eu}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$. Such an adjustment leads naturally to comparison of the melt entropies instead, but this also requires contextualization due to the $2\times$ difference in $\Delta S_{\mathrm{fus}}$ of the pure actinides. For these reasons, we compare the ratios of the melt entropies of the alloys to those of the pure metals.

This comparison requires values for $\Delta H_{\mathrm{fus}}^{{\mathrm{U}}_6\mathrm{Fe}}$ and $\Delta H_{\mathrm{fus}}^{\mathrm{Fe}}$. Assuming standard isotopic abundance of U, U${}_6$Fe is 96.24% U by mass. This is slightly higher than the highest entry in Table 3 of Reference [16] (95%), but linear extrapolation based on the 90%→95% shift gives 15.95 kJ/(mol atoms). Although values for $\Delta H_{\mathrm{fus}}^{\mathrm{U}}$ drawn from a simple web search vary widely, we have adopted the “selected value” from Table 29 of Reference [9]; this figure is quite close to the mean of the four others listed there. With all enthalpies expressed in kJ/(mol atoms), this gives

$$R{(\mathrm{U}}_6\mathrm{Fe})\equiv \frac{\Delta S_{\mathrm{fus}}^{{\mathrm{U}}_6\mathrm{Fe}}}{\Delta S_{\mathrm{fus}}^{\mathrm{U}}}=\frac{T_{\mathrm{fus}}^{\mathrm{U}}}{T_{\mathrm{eu}}^{{\mathrm{U}}_6\mathrm{Fe}}}\frac{\Delta H_{\mathrm{fus}}^{{\mathrm{U}}_6\mathrm{Fe}}}{\Delta H_{\mathrm{fus}}^{\mathrm{U}}}=\frac{1407}{1100}\frac{15.95}{8.47}=2.41,$$

to be compared with the R value listed in Table 5. A difference of less than 4% gives us considerable confidence that our analysis is reasonable.

4.3. Uncertainty Estimates

The random uncertainty in our corrected $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ is readily obtained by applying standard error propagation rules [18] to Equation (2). However, the dominant sources of uncertainty in the correction terms $\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)$ and $\Delta H_{\mathrm{liq}}$ are almost certainly systematic rather than random, direct consequences of assumptions {1}–{3} listed above. As such, they are difficult to estimate. As a result, we will ignore these as sources of random uncertainty and instead add their combined systematic contribution in quadrature (see Section 4.6 of Ref. [18], p. 107). Denoting the total relative uncertainty as $\delta$, its systematic and random components as ${\delta }_{\mathrm{sys}}$ and ${\delta }_{\mathrm{rand}}$, and the relative uncertainty in quantity Y as $\delta Y$,

$$\begin{array}{cc}\hfill {\delta }^2& ={\delta }_{\mathrm{sys}}^2+{\delta }_{\mathrm{rand}}^2\hfill \\ \hfill & ={\delta }_{\mathrm{sys}}^2+\frac{{\left(mQ\right)}^2\left({\delta }^{2}m+{\delta }^{2}Q\right){\left[\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)n_{\mathrm{Pu}}\delta n_{\mathrm{Pu}}\right]}^2}{{\left[mQ-n_{\mathrm{Pu}}\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)-\Delta H_{\mathrm{liq}}\right]}^2}+{\delta }^2{n}_{{\mathrm{Pu}}_6\mathrm{Fe}}.\hfill \end{array}$$

(17)

Uncertainties in composition can be related directly to those in measured heat flow at the $\alpha \to \beta$ transition,

$${\delta }^2{n}_{\mathrm{Pu}}={\delta }^{2}m+{\delta }^2{Q}_{\alpha \to \beta }+{\delta }^2\Delta H_{\alpha \to \beta }$$

(18)

and

$${\delta }^2{n}_{{\mathrm{Pu}}_6\mathrm{Fe}}={\delta }^{2}m+\frac{{\left(Q_{\alpha \to \beta }\Delta H_{\alpha \to \beta }\right)}^2\left[{\delta }^2{Q}_{\alpha \to \beta }+{\delta }^2\Delta H_{\alpha \to \beta }\right]}{{\left(1-\frac{Q_{\alpha \to \beta }}{\Delta H_{\alpha \to \beta }}\right)}^2}.$$

(19)

We estimate $\delta m$ at 0.2% based on STR’s stated precision, $\delta \Delta H_{\alpha \to \beta }$ at 0.8% based on Table 33 of Reference [9], and $\delta Q_{\alpha \to \beta }$ at 0.7% based on our own analysis of the STR DSC data. The largest source of random uncertainty is $\delta Q$, which we place at 3% based on variation of integration limits within the range of reported $T_{\mathrm{eu}}$ (at the lower limit) and by visual inspection (at the upper). This figure corresponds also to the difference between STR’s estimate of $Q\sim 27.5$ J/g and our own of $Q\approx 26.7$ J/g based on the Ellinger liquidus. As

Table 6. Total relative uncertainty, $\delta$, as a function of systematic uncertainty in the correction terms $\Delta H_{\mathrm{fus}}^{\mathrm{Pu}}\left(T_{\mathrm{eu}}\right)$ and $\Delta H_{\mathrm{liq}}$.

${\mathit{\delta }}_{\mathbf{sys}}$ (%)	$\mathit{\delta }$ (%)
0	4
5	6
10	11
25	25

makes clear, the sum of all random contributions is relatively minor unless ${\delta }_{\mathrm{sys}}\sim 10$% or less.

5. Summary and Conclusions

We have reanalyzed the DSC data of Reference [3], beginning with reestimation of the measured heat flow associated with the $\alpha \to \beta$ transition in pure Pu. This produced slight adjustment of the sample composition, which was then combined with the liquidus curve of Reference [7] to estimate the heat absorbed by the accumulating liquid once melting began. This heat was added to the melt enthalpy of pure Pu at the eutectic temperature to yield a substantially revised melt enthalpy for Pu${}_6$Fe.

As noted in the preceding subsection, the random uncertainties inherent to our analysis are small, at the level of a few percent. The systematic uncertainties, on the other hand, are unknown but potentially much larger. We believe the most significant source of uncertainty to be Assumption {2}, which assumes all activity coefficients to be unity [19]. An intriguing alternative would be to estimate these on the basis of ab initio molecular dynamics simulations instead [20]. Such simulations would have to produce estimates of the free energy, a non-standard (and non-trivial) exercise [21]. A number of techniques for doing so exist, however, and that of Reference [22] seems particularly promising.

It is worth noting that new measurements of $\Delta H_{\mathrm{fus}}^{{\mathrm{Pu}}_6\mathrm{Fe}}$ will also require reanalysis in the form of Equation (2) unless samples have exactly the eutectic composition. The solid-state synthesis method used to produce the STR sample can be viewed as relatively crude, yielding a bulk sample of imperfect quality. Efforts to prepare purer materials are ongoing, and these should afford more precise characterization and thermophysical property measurements.